1 Introduction

The present article investigates the surface roughness of the objects printed on an indigenously developed multi-jet based sub-zero temperature 3D printer. It has an inkjet printhead similar to PolyJet and MultiJet Printing (MJP) techniques. The novelty of the present research is that the authors use water and aqueous solutions as AM materials, which is unique. Very few studies are available that discuss the surface roughness of ice objects.

One of the earliest works reported for ice 3D printing is Rapid Freeze Prototyping (RFP) technique by M. C. Leu et al. RFP uses a piezoelectric nozzle that dispenses water in a layered fashion to realize the ice parts [1,2,3,4,5,6]. The surface roughness of the ice parts is modelled using ice line analysis which assumes that the nozzle is dispensing a single line of water layer-by-layer to create a wall [2, 6].

Several studies are available on the surface roughness of the polyjet parts which discuss the experimentally found surface roughness [7,8,9,10,11,12,13,14]. Krishnan and Gurunathan (2015) have elaborated on the theoretical and experimental surface roughness obtained by the PolyJet process [9]. They employ a model of individual droplets in the shape of a spherical cap. They have optimized the surface finish using three parameters: layer thickness, type of finish (glossy or matte), and local surface orientation. Experimentally observed surface roughness values for the PolyJet process are reported by Kechagias and Maropoulos (2015) [7]. There is another study of surface roughness available with indigenously developed multi-jet AM machine by Y. L. Cheng et al., but they use roller for levelling and obtain smoother surface, hence, it is not considered [15]. Ice line and spherical cap models are explained in detail subsequently.

1.1 Ice line model

The ice line model is developed, assuming the droplets are deposited in a line. Multiple such passes stack the droplets in layers to form an ice line, as shown in Fig. 1. According to this model, surface irregularities are caused by droplets freezing and forming a spherical shape.

Fig. 1
figure 1

Cross-section of ice line [2]

Full size image

The sphericity of the forming droplets causes surface imperfections, as demonstrated in Fig. 1a, b. In the case of a slant surface, an angle ϕ is considered, the remaining parameters being the same for the ice line. This model considers the waviness of the surface due to the droplet sphericity. However, this model does not consider the staircase effect, a crucial cause of surface roughness in AM parts.

1.2 Spherical cap model

The theoretical surface roughness achieved by the PolyJet technique has been explored by Kumar and Kumar [9]. They have optimized the surface finish with three parameters: layer thickness, type of finish (glossy or matte, which corresponds to the DPI; 360 and 720, respectively [9, 16]), and local surface orientation. As illustrated in Fig. 2, their model considers the spherical cap form of the droplets. Their material of interest is the resin generally used for the PolyJet technique.

Fig. 2
figure 2

Spherical cap model [9]

Full size image

The surface profile predicted by the spherical cap model is shown in Fig. 2a, b. Figure 2c gives the controlling parameters and mathematical representation of surface roughness (Ra), where \(\varphi\) is the contact angle of the droplet with the surface and \(\theta\) is the inclination angle. The proposed model agrees well with the experimental results and is more suitable for the matte finish, i.e. lower DPI values in the PolyJet process. Kechagias and Maropoulos have experimentally verified the same, and have concluded that DPI, i.e. build style in terms of PolyJet (Glossy and Matte) affects the surface roughness the most [7]. The analysis is also fortified by the experimental analysis made by Kumar and Kumar.

As seen above, the spherical cap model considers the staircase effect; however, it does not consider the sphericity of the droplets, which is the source of the roughness in horizontal and inclined surfaces.

The authors propose a model that considers both individual droplet geometry and the staircase effect caused by slant surfaces. The proposed model overcomes these limitations in ice line and spherical cap models.

2 Proposed mathematical model

The proposed model assumes that the droplets take the shape of a spherical cap as soon as they land on the build platform, as illustrated in Fig. 3. The droplet that landed and solidified on the surface is called a bead to distinguish it from the falling droplet. The surface tension and gravity of the bead determine its spread. Bond number (Bo), a dimensionless ratio of gravitational and capillary forces, indicates the spread [17].

$$Bo = \frac{\rho gd^{2}}{\sigma},$$
(1)

where \(\rho\) is the density of the liquid in kg/m3, \(g\) is the acceleration due to gravity, i.e. 9.81 m/s2, \(d\) is the characteristic dimension. Generally, the radius of the curvature of the bead in m and \(\sigma\) is the surface tension in N/m. \(Bo\) < 1 indicates that surface tension is more influential, and \(Bo\) > 1 indicates gravity is more influential. In the present case for water, \(\rho\) is 1000 kg/m3, \(d\) is 1.23 × 10−5 m, and surface tension is 0.076 N/m. \(Bo\) = 1.9 × 10−5 \(\ll\) 1. Therefore, the droplet spread is less and surface tension is more prominent, which gives the drop the shape of a spherical section, as shown in Fig. 3a. Because of the small volume of the droplets, it is assumed that they solidify as soon as they touch the built plate.

Fig. 3
figure 3

Schematic representation of droplet formation: a isometric view, b top and front view

Full size image

Layer thickness is found empirically. The object’s height was measured after printing the known number of layers of an object to measure the layer thickness. Average layer height was calculated from the ratio of part height and the number of layers. The layer height was determined to be 6 µm.

2.1 Part A: Horizontal surface

Figure 3a depicts the beads. The dimensions of the bead are as illustrated in Fig. 3b:

  • h = layer thickness (height of the bead cross-section)

  • R = radius of the bead cross-section

  • a = radius of the bead at the build platform (overlapping circles in the top view)

  • d = centre distance between two consecutive beads

  • Vb = bead volume

Equation 2 can be used to calculate the radius of the bead at the build platform, a, for a known bead volume Vb and a predetermined layer height h. Equation 3 is used to compute the centre distance d between two consecutive drops as a function of Droplets per Inch (DPI), a characteristic of the multi-jet dispensing process to determine deposition rate.

$$V_{\text{b}} = \frac{\pi h}{6}\left( {3a^2 + h^2 } \right)$$
(2)
$$d = \frac{25.4 \times 10^3 }{{{\text{DPI}} - 1}} ({\mu m})$$
(3)

The overlapped volume Vo is displaced towards the interface of the two beads in the form of curvature of radius r, as shown in Fig. 4a [18]. To determine Vo, a unit volume of dxdydz lying inside the overlap region between two beads is considered in Fig. 4a. X-axis limits are from d/2 to \(\sqrt {R^2 - z^2 - y^2 }\), as shown in Fig. 4b (Front View). Y limits range from 0 to \(\sqrt {{R^2 - z^2 - \left( \frac{d}{2} \right)^2 }}\), and Z limits range from \((R - h)\) to \(\sqrt {{R^2 - \left( \frac{d}{2} \right)^2 }}\).

Fig. 4
figure 4

Displaced volume between a the overlap region b Schematic diagram of an overlapped volume

Full size image

As per the mentioned limits, the triple integral is formulated to determine the overlapped volume between the two droplets (Eq. 4).

$$\begin{aligned} & V_{\text{o}} = 2 \int \limits_{R - h}^{\sqrt{R^{2} - (d/2)^{2}}} \int \limits_0^{\sqrt{R^{2} - z^{2} - (d/2)^{2}}} \int \limits_{d/2}^{\sqrt{R^{2} - z^{2} - y^{2}}}{\text{d}}x{\text{d}}y{\text{d}}z \\ & V_{\text{o}} = \frac{d}{4}\left[{\left( {R^{2} - \frac{d^{2} }{4}} \right)\left( {\frac{\pi }{2} - {\text{sin}}^{ - 1} \left( {\frac{R - h}{{\sqrt {{R^{2} - \frac{d^{2} }{4}}} }}} \right)} \right) - 3(R - h)\sqrt {{(2R - h)h - \frac{d^{2} }{4}}} } \right] - (R - h)(2R - h)h\,{\text{sin}}^{ - 1} \sqrt{1 - \frac{d^{2}}{4(2R - h)h}} \end{aligned}$$
(4)

As shown in Fig. 5, the displaced volume forms a meniscus with a radius r. A cross-sectional area Ac is considered to calculate r. It is symmetrical at about x = d/2. A small elemental area dxdz (marked red) is considered that lies within area Ac to determine Ac. Ac is symmetrical. ϕ is considered an angle subtended at the sphere centre at the contact point of the displaced water meniscus. It is determined within the limits of R cos ϕ and d/2 in the X direction, as given in Eq. 5.

$$\begin{aligned} & A_{c} = 2 \int \limits_{R\cos \emptyset }^{d/2} z\,{\text{d}}x \\ & = 2 \int \limits_{\frac{d}{2}\left( {\frac{R}{r + R}} \right)}^{d/2} \left( {\sqrt {{(R + r)^{2} - \frac{d^{2} }{4}}} - \sqrt {{r^{2} - \left( {\frac{d}{2} - x} \right)^{2} }} - \sqrt {R^{2} - x^{2} } } \right){\text{d}}x \\ & = d\left( {\frac{R}{r + R}} \right)\sqrt {{(R + r)^{2} - \frac{d^{2} }{4}}} - r^{2} \left[ {\sin^{ - 1} \left( {\frac{d}{2(R + r)}} \right) + \frac{d}{2(R + r)}\sqrt {{1 - \left( {\frac{{\frac{d}{2}\left( {\frac{R}{r + R}} \right)}}{R}} \right)^{2} }} } \right] \hfill \\ & - R^{2} \left[ {\sin^{ - 1} \left( \frac{d}{2R} \right) - \sin^{ - 1} \left( {\frac{d}{{2\left( {R + r} \right)}}} \right) + \frac{d}{2R}\sqrt {{1 - \frac{d^{2} }{{4R^{2} }}}} - \frac{d}{2(R + r)}\sqrt {{1 - \frac{d^{2} }{{4(R + r)^{2} }}}} } \right] \end{aligned}$$
(5)
Fig. 5
figure 5

Schematic diagram for estimation of overlap cross-sectional area

Full size image

Contact length l is the distance along the interface section of the two beads in the yz plane, as shown in Fig. 6. Mathematically, contact length is given by Eq. 6.

$$l = \frac{{V_{\text{o}} }}{{A_{\text{c}} }}$$
(6)
Fig. 6
figure 6

Schematic for estimation of swept length

Full size image

The contact length is also determined using Eq. 7.

$$l = 2\delta b = 2\left( {\cos^{ - 1} \left( {\frac{R - h}{{\sqrt {{R^2 - \frac{d^2 }{4}}} }}} \right)} \right)x\sqrt {{R^2 - \frac{d^2 }{4}}}$$
(7)

The values of \(V_{\text{o}}\), \(A_{\text{c}}\) and \(l\) are substituted in Eq. 6 to determine r. For a given set of process parameters (droplet volume, layer height and DPI), the droplet dimensions and spacing (R, a, d) can be determined. These values are then used to calculate the overlapped volume and swept length. Further, the surface roughness is determined using Eq. 8.

$$R_{\text{a}} = h + r + \sqrt {R^2 - a^2 } - \sqrt {{\left( {R + r} \right)^2 - \frac{d^2 }{4}}} .$$
(8)

For a SAM system with a droplet volume as 5 pl (pico-litres) working at 720 DPI and 6 \({\mu m}\) layer thickness, the surface roughness is 3.827 \({\mu m}\). Table 1 summarizes these results.

Table 1 Peak-to-valley surface roughness results
Full size table

2.2 Part B: Inclined surface at an angle of β°

The above analysis is valid for a horizontal surface. The angle of inclination for the horizontal surface is 0°. Hence, roughness value is termed Ra0. For a surface inclined at an angle β, the roughness is termed Raβ. The fixed point (O) is marked at the intersection of the common tangents to the inclined surface and the horizontal axis, as shown in Fig. 7.

Fig. 7
figure 7

Schematic for estimation of surface roughness on an inclined surface

Full size image

As the working scale is micro-level, it is assumed that the peak-to-valley distances for horizontal and inclined surface roughness lie on the same vertical axis. Figure 7 shows that the vertical axis is lying at the central distance between two consecutive drops of the first deposition layer. The distance between the vertical axis and the fixed point is denoted by D and is calculated using Eq. 9.

$$D = R\left[ {\frac{{1 - \left( {1 - \frac{h}{R}} \right)\cos \beta }}{\sin \beta }} \right] + \frac{d}{2}$$
(9)

The angle subtended by the horizontal surface roughness at the fixed point is given by Eq. 10.

$$\varphi = \tan^{ - 1} \left( \frac{h}{D} \right) - \tan^{ - 1} \left( {\frac{{h - R_{z0} }}{D}} \right).$$
(10)

At the fixed point, the same angle is also subtended by the inclined surface roughness (Eq. 11).

$$\varphi = \beta - \tan^{ - 1} \left( {\frac{{D\,\tan \,\beta - R_{z\beta } }}{D}} \right).$$
(11)

By equating the angles from Eqs. 14 and 15 and solving for \(R_{z\beta }\) yields the inclined surface roughness (Eq. 12).

$$R_{z\beta } = D\left[ {\tan \beta - \tan \left( {\beta + \tan^{ - 1} \left( {\frac{{h - R_{z0} }}{D}} \right) - \tan^{ - 1} \left( \frac{h}{D} \right)} \right)} \right].$$
(12)

Surface inclined at 90° is a particular case. It involves the deposition of the droplets precisely one above the other. Equation 12 does not help determine the Ra value since the tangent ratio is undefined at 90°. In order to determine the surface roughness over vertical, 90° inclination surfaces, the model proposed by Sui and Leu, 2003 is considered. According to their model, the surface roughness, Ra over a vertical surface is given by Eq. 13 [1],

$$R_{\text{a}} = \frac{I}{\Delta z} = \frac{\alpha - \sin \alpha }{{8\sin^{3/2} (\alpha /2)}}\sqrt {A} ,$$
(13)

where I is the shaded area for the surface roughness calculation, \(\Delta z\) is the layer height, \(\alpha\) is the water–ice contact angle, and A is the shaded area of the water line, as shown in Fig. 8.

Fig. 8
figure 8

Schematic for roughness estimation of vertical surface

Full size image

The shaded area of the water line, A for a spherical cap (Fig. 8), is calculated using Eq. 14.

$$A = ah + R^2 \left[ {\sin^{ - 1} \left( \frac{a}{R} \right) - \frac{a}{R}} \right].$$
(14)

Also, the water–ice contact angle, \(\alpha\), can be calculated by equating the layer height with line width, w (Eq. 15).

$$\Delta z = w\sin (\alpha /2).$$
(15)

Solving for A & \(\alpha\), mean surface roughness, \(R_a\) for a vertical ice surface (90° inclination), with process parameters as mentioned in Table 1, came out to be 0.1 \({\mu m}\). It should be noted that the inter-droplet spacing should be less than the diameter of the base circle (d < 2a) for droplet overlap. For low values of DPI, such as 360, there is no overlap of the droplet. Hence, the peak-to-valley distance is the same as the layer height, h. For such cases, the horizontal surface roughness, \(R_{\text{a}}\), is 6 \({\mu m}.\) There is no droplet overlap at low DPI settings, such as 360. As a result, the peak-to-valley distance equals the layer height, h. In such circumstances, Ra was found to be 6 µm.

3 Experimental methods

Metals, ceramics, and polymers are the three broad categories of AM materials [19, 20]. However, AM technology is evolving, and some non-conventional materials such as water, aqueous solutions, gels, colloids and slurries are emerging as AM materials. These materials are liquid at room temperature. Hence, they require sub-zero temperatures for their deposition to create objects. Therefore, the technique is named Sub-zero Additive Manufacturing (SAM). M. C. Leu et al. [21], Pieter Sijpkes et al. [22], Zhang et al. [23], Zheng et al. [24], and Pushkar Kamble et al. [5, 25] have all demonstrated AM of ice objects.

With the advent of these materials, newer and environment-friendly applications are opening up for AM objects. One such application is using ice objects as patterns for investment casting. Traditionally, wax is used, which gives out hydrocarbons when melted for dewaxing the moulds. Ice is a much greener and cheaper alternative as compared to wax. Investment casting is well known for producing near-net-shape geometries with a smoother surface finish than sand casting. Hence, it is used for producing jewellery and dental implants. Therefore, it is essential to assess the surface roughness of the frozen objects produced by SAM.

Authors have developed a prototype of SAM based on the multi-jet dispensing technology similar to Stratasys’ PolyJet (Fig. 9). It uses a multi-jet printer to distribute water and aqueous solution in the raster pattern layer by layer. The printhead has four rows of staggered nozzles, each row devoted to one liquid tank. There are 320 nozzles in each row of 28.8 mm. For model and support liquids, we deploy two rows each. The printhead driver software allows for selecting two DPIs, 360 and 720, which equate to dot sizes of 73 µm and 36 µm, respectively. The build process takes place inside a refrigerated chamber at −30 °C. The chamber size is 200 mm × 200 mm × 150 mm. The build platform and the chamber are maintained at −30 °C using a vapour compression refrigeration system augmented by liquid nitrogen for cooling.

Fig. 9
figure 9

Sub-zero additive manufacturing (SAM) machine

Full size image

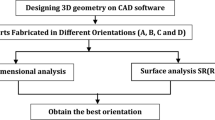
The objective of the experiments is to determine the relationship between surface roughness and surface orientation at two distinct DPIs. Surface roughness is measured at five distinct orientations for 360 DPI and 720 DPI: 0°, 30°, 45°, 60°, and 90°. Surface orientation and DPI are two variables that can influence surface finish. A full factorial design was used for the experimental plan. Five values of the surface orientation angles (0°, 30°, 45°, 60° and 90°) and two values of the DPI (360, 720) lead to 51 × 21 = 10 experiments. These experiments were repeated four times to ensure that the results were statistically significant.

3.1 Specimens

The specimen surfaces were 3D printed as shown in Fig. 10. The angles from 10° to 90° were printed as shown. A flat surface printed separately was used to measure the roughness of the horizontal surface (0°).

Fig. 10
figure 10

Specimens for the test

Full size image

3.2 UV templating

It is difficult to measure the surface roughness of ice objects by traditional methods. Ice objects cannot be transported to the measuring instrument without causing geometry loss, and if left in the air for an extended period, ambient water vapour tends to condense on the surface. Therefore, surface finish is measured using an indirect, non-contact method termed as templating technique. The ice geometry to be measured is copied to another material with a significantly lower freezing point than ice and remains solid at ambient temperature.

UV resin, a liquid monomer with a solidification point of −8 °C, is helpful for this purpose. UV resin, pre-cooled to −2 °C, is poured as a thin layer on the ice object to be measured and allowed to settle for 15 s. A UV lamp with a wavelength of 400 nm is employed to cure the resin (Fig. 11a). Even at the sub-zero temperature of the build chamber, the resin polymerizes into a solid after 120 s. After curing, the lamp is removed, and the resin-covered ice object is removed. The ice object melts, leaving behind the desired geometry on the resin (Fig. 11b).

Fig. 11
figure 11

Investigation of surface roughness at various inclinations

Full size image

3.3 Measurement method

A 3D non-contact Surface Profilometer by Alicona (InfiniteFocus®) was used to measure the templates’ surface roughness. The templates were neatly cut into the required sizes, the surface under observation was aligned horizontally by mounting properly. The mounted samples were neatly placed on the stage of the profilometer. A profile length of 1.4 mm was used. The values of Ra, Rq, Rt, Rz and Rmax were read from the interface of the IF-MeasureSuite software for Alicona. Measurements were carried out at the room temperature of 24 °C with 44% RH.

4 Results and discussion

In Figs. 12 and 13, the experimental results are displayed against the theoretical prediction. To comprehend the trend of surface roughness with surface orientation, the surface orientation is chosen for ten levels starting from 0° to 90° with an increment of 10°.

Fig. 12
figure 12

Variation of surface roughness with surface inclination (360 DPI)

Full size image
Fig. 13
figure 13

Variation of surface roughness with surface inclination (720 DPI)

Full size image

It is observed that the Ra value tends to increase with the increase in the inclination angle, a trend that is similar to the spherical cap model discussed by Krishan and Kumar. The experimental result matches the theoretical model’s inclination range of 0–45°. From 45° to 60° inclination, the model predicts higher values of the Ra than what was experimentally found.

It could be because the model assumes the droplets are stacked above the other, and they all freeze at the same rate. Also, DPI is low, resulting in droplets being deposited sparser than those at 720 DPI (Fig. 13). It theoretically reduces the overlapped region between the adjacent droplets on the inclined surface, which predicts higher roughness values than expected. However, as the part height increases, the freezing time of the droplet also increases since the ice layers below the topmost layer act as insulators. It may allow more spread of the droplet resulting in more overlap.

The proposed model agrees well with the experiments at 720 DPI. Higher DPI results in closer droplets and higher overlap between the two beads, leading to a smoother surface than the 360 DPI case (Fig. 12). The average surface roughness is 20% lesser in 720 DPI case than in 360 DPI. It is observed that the proposed model agrees well with the experimental results at higher DPI values.

In the case of 90° inclination, the droplets are stacked vertically. As a result, DPI does not affect vertical surface roughness. The average surface roughness value found by the ice line model at 360 and 720 DPI is 0.1 µm. Although the empirically discovered Ra values are 20–30 times higher than the theoretically predicted value, they are closer to the Ra values predicted by Sui and Leu’s ice line model (e.g., 5 µm roughly). Because the layers tend to expand out in reality, adding to the surface roughness, the experimental values are higher than predicted.

4.1 Comparison with the previous models

The proposed model combines the two existing models, viz., ice line and spherical cap model, that considers droplet sphericity and staircase effect. In the previous study by Krishnan and Gurunathan, the surface roughness ranges from 5 to 25 µm. Parts built with UV resin on polyjet in another study exhibit the surface roughness ranging from 0.2 to 23.82 µm depending on the print orientation [10]. It is observed by the spherical cap model that the surface roughness increases with an increase in the inclination angle, which agrees with the trend presented in this paper. ANOVA studies are also available, where the effect of the process parameters is discussed, where the type of finish has found to be the most influential parameter followed by surface orientation. Other parameters like layer thickness and the interactions between layer thickness and type of finish, layer thickness and local surface orientation were found less significant (<2%). Therefore, they are not considered for the present study. The present study is more focused on type of finish and surface orientation.

The proposed model agrees with the surface roughness values found by previous studies. The proposed model is developed and validated with a low contact angle of the ice-water interface (12 ± 1° at sub-zero temperatures); however, for the same contact angle range, the Krishnan–Gurunathan model predicts lower surface roughness values than experimentally found. It shows that the proposed model is more accurate since it considers the shape of the droplets.

4.2 Limitation of the proposed model

The proposed model is limited in predicting vertical surfaces’ roughness. The proposed model fails to predict the roughness values of the vertical surfaces. Therefore, the ice line model is used for the vertical surface since it is free from any effects of the variation in the DPI. The roughness of the vertical surface is almost similar to the horizontal surface. The experiments are carried out for the multi-jet deposition at sub-zero temperatures. However, the model can be generalized for all material jetting processes.

5 Conclusion

In the droplet-based AM processes, droplet geometry as well as staircase effect play a vital role in determining the surface roughness. The presented model considers DPI and surface orientation. DPI indicates the proximity of the droplets, whereas part orientation indicates the staircase effect. Previous models, such as the ice line model, do not account for the inaccuracy caused by the staircase effect, and the spherical cap model does not account for bead geometry. The current model combines both approaches, considering the droplet (bead) shape and the staircase effect caused by an inclined surface.

The present model is useful in assessing the surface roughness of the parts based on the printing parameters. The parameters can therefore be adjusted to obtain the desired surface roughness range. The parts can be used as patterns for precision casting; for example, jewellery, dental and other biomedical implants, the surface roughness becomes an important aspect of the process. The desired surface roughness can be obtained on the casting as the precision casting offers net shape capability.

As future scope of this research, various liquids with different contact angles and the flow properties can be tested for their surface roughness to validate this model to make it more universally applicable.