Abstract
Self-dual cyclic codes over rings and their generalizations have become of interest due to their rich algebraic structures and wide applications. Cyclic and self-dual cyclic codes over the ring  have been quite well studied, where p is a prime, k is a positive integer, and \(u^2=0\). We focus on negacyclic codes over
have been quite well studied, where p is a prime, k is a positive integer, and \(u^2=0\). We focus on negacyclic codes over  , where p is an odd prime and k is a positive integer. An alternative and explicit algebraic characterization of negacyclic codes of length \(p^s\) over
, where p is an odd prime and k is a positive integer. An alternative and explicit algebraic characterization of negacyclic codes of length \(p^s\) over  is presented. Based on this result, representation and enumeration of self-dual negacyclic codes of length \(p^s\) over
is presented. Based on this result, representation and enumeration of self-dual negacyclic codes of length \(p^s\) over  are given under both the Euclidean and Hermitian inner products.
are given under both the Euclidean and Hermitian inner products.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
1 Introduction
Self-dual cyclic and negacyclic codes over finite fields have been extensively studied for both theoretical and practical reasons. Codes over finite rings have been of interest since it was proven that some binary non-linear codes such as the Kerdock, Preparata, and Goethal codes are the Gray images of linear codes over \({\mathbb {Z}}_4\) (see [9]). Cyclic codes, negacyclic codes and their generalizations have extensively been studied over \({\mathbb {Z}}_{p^r}\), Galois rings,  with \(u^2=0\) and finite chain rings (see [1,2,3,4,5,6,7,8, 10, 11, 13,14,15,16] and references therein).
with \(u^2=0\) and finite chain rings (see [1,2,3,4,5,6,7,8, 10, 11, 13,14,15,16] and references therein).
Recently, a lot attention has been paid to families of negacyclic and self-dual codes over  , and there is a significant progress on these topics (see [3, 6,7,8, 14]). In [3], characterization and presentation of negacyclic codes of length \(p^s\) over
, and there is a significant progress on these topics (see [3, 6,7,8, 14]). In [3], characterization and presentation of negacyclic codes of length \(p^s\) over  have been established. Later, the algebraic structure of negacyclic codes of lengths \(2p^s\) and \(4p^s\) over the ring
have been established. Later, the algebraic structure of negacyclic codes of lengths \(2p^s\) and \(4p^s\) over the ring  has been studied in [6,7,8, 14]. Subsequently, properties of Euclidean duals of negacyclic codes and Euclidean self-dual negacyclic codes of lengths \(2p^s\) and \(4p^s\) over
has been studied in [6,7,8, 14]. Subsequently, properties of Euclidean duals of negacyclic codes and Euclidean self-dual negacyclic codes of lengths \(2p^s\) and \(4p^s\) over  have been studied in [8, 14] and [6, 7], respectively. To the best of our knowledge, a complete characterization and enumeration of self-dual negacyclic codes of length \(p^s\) have not been explicitly given so far.
have been studied in [8, 14] and [6, 7], respectively. To the best of our knowledge, a complete characterization and enumeration of self-dual negacyclic codes of length \(p^s\) have not been explicitly given so far.
Using a method which is a modification of the one in [13], the algebraic structure and number of cyclic codes of length \(p^s\) over  have been presented in [2]. In loc. cit., a complete characterization and enumeration of self-dual cyclic codes of length \(p^s\) over
have been presented in [2]. In loc. cit., a complete characterization and enumeration of self-dual cyclic codes of length \(p^s\) over  have been given under both the Euclidean and Hermitian inner products.
have been given under both the Euclidean and Hermitian inner products.
In this paper, we focus on negacyclic codes of length \(p^s\) over  which makes sense only in the case where p is an odd prime and k is a positive integer. Throughout, assume that p is an odd prime and k is a positive integer. It is not difficult to verify that the map
which makes sense only in the case where p is an odd prime and k is a positive integer. Throughout, assume that p is an odd prime and k is a positive integer. It is not difficult to verify that the map

defined by

is a ring isomorphism. Hence, the results on cyclic codes and self-dual cyclic codes of length \({p^s}\) over  in [2] can be carried over to negacyclic codes and self-dual negacyclic codes of length \({p^s}\) over
in [2] can be carried over to negacyclic codes and self-dual negacyclic codes of length \({p^s}\) over  . Here, an alternative and direct method to study negacyclic codes and self-dual negacyclic codes of length \({p^s}\) over
. Here, an alternative and direct method to study negacyclic codes and self-dual negacyclic codes of length \({p^s}\) over  is given. Characterization and enumeration of negacyclic codes of length \({p^s}\) over
is given. Characterization and enumeration of negacyclic codes of length \({p^s}\) over  are given by extending the techniques used in [2, 10, 12, 13]. Furthermore, characterization of self-dual negacyclic codes of length \({p^s}\) over
are given by extending the techniques used in [2, 10, 12, 13]. Furthermore, characterization of self-dual negacyclic codes of length \({p^s}\) over  is established under both the Euclidean and Hermitian inner products. Based on this characterization, enumeration of such self-dual codes is provided as well.
is established under both the Euclidean and Hermitian inner products. Based on this characterization, enumeration of such self-dual codes is provided as well.
The paper is organized as follows. In Sect. 2, some basic results on the ring  are recalled. The characterization and enumeration of negacyclic codes of length \({p^s}\) over
are recalled. The characterization and enumeration of negacyclic codes of length \({p^s}\) over  are provided in Sect. 3. In Sect. 4, the characterization and enumeration of self-dual negacyclic codes of length \({p^s}\) over
are provided in Sect. 3. In Sect. 4, the characterization and enumeration of self-dual negacyclic codes of length \({p^s}\) over  are discussed.
are discussed.
2 Preliminaries
In this section, some definitions and basic properties of negacyclic codes over  are recalled. For a prime p and positive integer k, denote by
are recalled. For a prime p and positive integer k, denote by  the finite field of order \({p^k}\). The set
the finite field of order \({p^k}\). The set  forms a commutative chain ring with identity, where addition and multiplication are defined as in the usual polynomial ring over
forms a commutative chain ring with identity, where addition and multiplication are defined as in the usual polynomial ring over  with indeterminate u together with the condition
with indeterminate u together with the condition  . In the case where k is even, the map
. In the case where k is even, the map  defined by
defined by

for all  is a ring automorphism. For more details concerning properties of
is a ring automorphism. For more details concerning properties of  , we refer the reader to [3].
, we refer the reader to [3].
A linear code of length n over  is defined to be an
is defined to be an  -submodule of the
-submodule of the  -module
-module  .
.
The Euclidean inner product on  is defined by
is defined by

for all  and
and  in
in  . In addition, if k is even, the Hermitian inner product on
. In addition, if k is even, the Hermitian inner product on  is defined to be
is defined to be

for all  and
and  in
in  . The Euclidean dual of a linear code C of length n over
. The Euclidean dual of a linear code C of length n over  is defined to be
is defined to be

Similarly, if k is even, the Hermitian dual of C is defined as

A linear code C is said to be Euclidean self-dual (resp., Hermitian self-dual) if  (resp.,
(resp.,  ).
).
In the case where p is odd, \({-1\ne 1}\) in  . Then we have the following concepts. A linear code C of length n over
. Then we have the following concepts. A linear code C of length n over  is called a negacyclic code if C is invariant under the negacyclic shift, i.e.,
is called a negacyclic code if C is invariant under the negacyclic shift, i.e.,  for all
for all  . It is well known that there exists a one-to-one correspondence between the negacyclic codes of length n over
. It is well known that there exists a one-to-one correspondence between the negacyclic codes of length n over  and the ideals in the quotient ring
and the ideals in the quotient ring  . Precisely, a negacyclic code C of length n can be represented by the ideal
. Precisely, a negacyclic code C of length n can be represented by the ideal

in  .
.
Here, we focus on negacyclic and self-dual negacyclic codes over  . For convenience, denote by
. For convenience, denote by  and
and  the number of negacyclic codes, the number of Euclidean self-dual negacyclic codes, and the number of Hermitian self-dual negacyclic codes of length n over
the number of negacyclic codes, the number of Euclidean self-dual negacyclic codes, and the number of Hermitian self-dual negacyclic codes of length n over  , respectively.
, respectively.
3 Negacyclic codes of length \({p^s}\) over 
In this section, the characterization and enumeration of negacyclic codes of length \({p^s}\) over  are given. By extending techniques used for cyclic codes of length \({p^s}\) over
are given. By extending techniques used for cyclic codes of length \({p^s}\) over  in [2], the algebraic structure and presentation of negacyclic codes of length \({p^s}\) over
in [2], the algebraic structure and presentation of negacyclic codes of length \({p^s}\) over  can be derived in terms of ideals in
can be derived in terms of ideals in  . Since the proofs are quite straightforwardly extended from [2], they will be omitted.
. Since the proofs are quite straightforwardly extended from [2], they will be omitted.
First, we note that the map  defined by
defined by
is a surjective ring homomorphism.
For each negacyclic code C in  and \({i\in \{0,1\}}\), let
and \({i\in \{0,1\}}\), let

For each \({i \in \{0,1\}}\), \({{\mathrm{Tor}}_i(C)}\) is called the ith torsion code of C. The codes \({{\mathrm{Tor}}_0({C})=\mu (C)}\) and \({{\mathrm{Tor}}_1(C)}\) are sometimes called the residue and torsion codes of C, respectively. It is not difficult to see that for each  , \({c(x) \in {\mathrm{Tor}}_i(C)}\) if and only if
, \({c(x) \in {\mathrm{Tor}}_i(C)}\) if and only if  for some
for some  . Consequently, \({{\mathrm{Tor}}_0(C)\subseteq {\mathrm{Tor}}_1(C)}\) are ideals in
. Consequently, \({{\mathrm{Tor}}_0(C)\subseteq {\mathrm{Tor}}_1(C)}\) are ideals in  , i.e., they are negacyclic codes of length \({p^s}\) over
, i.e., they are negacyclic codes of length \({p^s}\) over  . We note that every ideal in
. We note that every ideal in  is of the form
is of the form  for some \({0\leqslant i\leqslant p^s}\) and its
for some \({0\leqslant i\leqslant p^s}\) and its  -dimension is \({p^{s-i}}\).
-dimension is \({p^{s-i}}\).
Proposition 3.1
([2, Proposition 5]) Let C be an ideal in  and let \({i\in \{0,1\}}\). Then the following statements hold:
and let \({i\in \{0,1\}}\). Then the following statements hold:
-
(i)
\({{\mathrm{Tor}}_i(C)}\) is an ideal of
 and \({{\mathrm{Tor}}_i(C)=\langle (x+1)^{T_i} \rangle }\) for some \({0\leqslant T_i \leqslant p^s}\).
and \({{\mathrm{Tor}}_i(C)=\langle (x+1)^{T_i} \rangle }\) for some \({0\leqslant T_i \leqslant p^s}\). -
(ii)
If \({{\mathrm{Tor}}_i(C)=\langle (x+1)^{T_i} \rangle }\), then \({|{\mathrm{Tor}}_i (C) |= (p^k)^{p^s-T_i}}\).
-
(iii)
 .
.
With the notations given in Proposition 3.1, for each \({i\in \{0,1\}}\),  is called the ith-torsional degree of C. Since \({{\mathrm{Tor}}_0(C)\subseteq {\mathrm{Tor}}_1(C)}\), we have \(0\leqslant T_1(C)\leqslant T_0(C)\leqslant p^s\). Moreover, if \({u(x+1)^t \in C}\), then \({t\geqslant T_1(C)}\).
is called the ith-torsional degree of C. Since \({{\mathrm{Tor}}_0(C)\subseteq {\mathrm{Tor}}_1(C)}\), we have \(0\leqslant T_1(C)\leqslant T_0(C)\leqslant p^s\). Moreover, if \({u(x+1)^t \in C}\), then \({t\geqslant T_1(C)}\).
Next, we focus on negacyclic codes of length \({p^s}\) over  , or equivalently, ideals in
, or equivalently, ideals in  .
.
Theorem 3.2
([2, Theorem 8]) Let C be an ideal in  ,
,  , and
, and  . Then
. Then
where

and

with  is either zero or a unit with
is either zero or a unit with  . Moreover, \({(f_0(x),f_1(x))}\) is unique in the sense that if there exists a pair \({(g_0(x),g_1(x))}\) of polynomials satisfying the above conditions, then \({f_0(x)=g_0(x)}\) and \({f_1(x)=g_1(x)}\).
. Moreover, \({(f_0(x),f_1(x))}\) is unique in the sense that if there exists a pair \({(g_0(x),g_1(x))}\) of polynomials satisfying the above conditions, then \({f_0(x)=g_0(x)}\) and \({f_1(x)=g_1(x)}\).
For each ideal C in  , denote by
, denote by  the unique representation of the ideal C obtained in Theorem 3.2.
the unique representation of the ideal C obtained in Theorem 3.2.
In order to determine the number of ideals in  , the definition and some properties of the annihilator of an ideal in
, the definition and some properties of the annihilator of an ideal in  are revisited. For an ideal C in
are revisited. For an ideal C in  , the annihilator of C is defined to be
, the annihilator of C is defined to be  . The following properties can be derived easily (cf. results for
. The following properties can be derived easily (cf. results for  in [10, Theorems 12–13]).
in [10, Theorems 12–13]).
Theorem 3.3
Let C be an ideal of  . Then the following statements hold:
. Then the following statements hold:
-
(i)
 is an ideal of
is an ideal of  .
. -
(ii)
If \({|C|=(p^k)^d}\), then
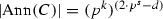 .
. -
(iii)
 .
.
Theorem 3.4
Let \({{\mathscr {I}}}\) denote the set of ideals of  , and let \({{\mathscr {A}}=\{ C\in {\mathscr {I}}: T_0(C)+T_1(C)\leqslant p^s \}}\) and \({{\mathscr {A}}'=\{ C\in {\mathscr {I}}: T_0(C)+T_1(C)\geqslant p^s \}}\). Then the map \({\phi :{\mathscr {A}}\rightarrow {\mathscr {A}}'}\) defined by
, and let \({{\mathscr {A}}=\{ C\in {\mathscr {I}}: T_0(C)+T_1(C)\leqslant p^s \}}\) and \({{\mathscr {A}}'=\{ C\in {\mathscr {I}}: T_0(C)+T_1(C)\geqslant p^s \}}\). Then the map \({\phi :{\mathscr {A}}\rightarrow {\mathscr {A}}'}\) defined by  is a bijection.
is a bijection.
In view of Theorem 3.4, it suffices to focus on the ideals in \({{\mathscr {A}}}\). For each  in \({{\mathscr {A}}}\), if \({f_0(x)=0}\), then \({T_0(C)=p^s}\) and \({T_1(C)=0}\). Hence, the only ideal in \({{\mathscr {A}}}\) with \({f_0(x)=0}\) is of the form
in \({{\mathscr {A}}}\), if \({f_0(x)=0}\), then \({T_0(C)=p^s}\) and \({T_1(C)=0}\). Hence, the only ideal in \({{\mathscr {A}}}\) with \({f_0(x)=0}\) is of the form  . Under the assumption that \({f_0(x)\ne 0}\), the following two results can be obtained (cf. [10, Theorems 14–15] for cyclic codes).
. Under the assumption that \({f_0(x)\ne 0}\), the following two results can be obtained (cf. [10, Theorems 14–15] for cyclic codes).
Theorem 3.5
Let  be the representation of an ideal in
be the representation of an ideal in  . Then it is a representation of an ideal in \({{\mathscr {A}}}\) if and only if \({i_0,i_1,t}\) are integers and
. Then it is a representation of an ideal in \({{\mathscr {A}}}\) if and only if \({i_0,i_1,t}\) are integers and  such that \({0\leqslant i_0<p^s}\),
such that \({0\leqslant i_0<p^s}\),  \({t\geqslant 0,}\)
\({t\geqslant 0,}\)  , and h(x) is either zero or a unit in
, and h(x) is either zero or a unit in  .
.
Since every polynomial \({\sum _{i=0}^{m}a_i(x+1)^i}\) in  is either 0 or \({(x+1)^t h(x)}\), where h(x) is a unit in
is either 0 or \({(x+1)^t h(x)}\), where h(x) is a unit in  and
and  , Theorem 3.5 can be rewritten as follows.
, Theorem 3.5 can be rewritten as follows.
Theorem 3.6
The expression  represents an ideal in \({{\mathscr {A}}}\) if and only if \({i_0}\) and \({i_1}\) are integers such that \({0\leqslant i_0<p^s}\),
represents an ideal in \({{\mathscr {A}}}\) if and only if \({i_0}\) and \({i_1}\) are integers such that \({0\leqslant i_0<p^s}\),  , \({i_0+i_1\leqslant p^s}\), and
, \({i_0+i_1\leqslant p^s}\), and  for all \({0\leqslant j<i_1}\).
for all \({0\leqslant j<i_1}\).
Proposition 3.7
Let \({0\leqslant d\leqslant p^s}\). Then the number of distinct ideals in  with \({T_0+T_1=d}\) is
with \({T_0+T_1=d}\) is

where  .
.
Proof
Let \({T_1 = i_1}\) and \({i_0 :=T_0=d-T_1}\) be fixed.
Case 1: \({d< p^s}\). Then \({i_0\leqslant i_0+i_1=T_0+T_1=d< p^s}\). By Theorem 3.6, it follows that  . Then the choice for
. Then the choice for  is \({(p^k)^{i_1}}\). By Theorem 3.6 again, we also have
is \({(p^k)^{i_1}}\). By Theorem 3.6 again, we also have  . Since \({T_0+T_1=d}\), we obtain that \({T_1\leqslant \lfloor {d}/{2} \rfloor \leqslant T_0}\), and hence,
. Since \({T_0+T_1=d}\), we obtain that \({T_1\leqslant \lfloor {d}/{2} \rfloor \leqslant T_0}\), and hence,  . Now, vary \({T_1}\) from 0 to K, we obtain that there are \({1+p^k+\cdots +(p^k)^K=({p^{k(K+1)}-1})/({p^k-1})}\) ideals with \({T_0+T_1=d}\).
. Now, vary \({T_1}\) from 0 to K, we obtain that there are \({1+p^k+\cdots +(p^k)^K=({p^{k(K+1)}-1})/({p^k-1})}\) ideals with \({T_0+T_1=d}\).
Case 2: \({d=p^s}\). If \({i_0=p^s}\), then the only ideal with \({T_0+T_1=p^s}\) is the ideal represented by  . If \({i_0<p^s}\), then we have \({p^k+(p^k)^2+\cdots +(p^k)^K}\) ideals by arguments similar to those in Case 1. \(\square \)
. If \({i_0<p^s}\), then we have \({p^k+(p^k)^2+\cdots +(p^k)^K}\) ideals by arguments similar to those in Case 1. \(\square \)
For a negacyclic code C in \({{\mathscr {A}}}\), we have  whenever \({T_0(C)+T_1(C)<p^s}\). In the case where \({T_0(C)+T_1(C)=p^s}\), by Theorem 3.5, the annihilator of the negecyclic code
whenever \({T_0(C)+T_1(C)<p^s}\). In the case where \({T_0(C)+T_1(C)=p^s}\), by Theorem 3.5, the annihilator of the negecyclic code  is of the form
is of the form  . Since p is odd, then
. Since p is odd, then  occurs only in the case \({h(x)=0}\). By Proposition 3.7 and the bijection given in Theorem 3.4, we have
occurs only in the case \({h(x)=0}\). By Proposition 3.7 and the bijection given in Theorem 3.4, we have
Corollary 3.8
The number of negacyclic codes of length \({p^s}\) over  is
is

Proof
From Theorem 3.4, the number of negacyclic codes of length \({p^s}\) over  is \({|{\mathscr {A}}\cup {\mathscr {A}}'|=|{\mathscr {A}}|+|{\mathscr {A}}'|-|{\mathscr {A}}\cap {\mathscr {A}}'|}\). The desired result follows immediately from the discussion above. \(\square \)
is \({|{\mathscr {A}}\cup {\mathscr {A}}'|=|{\mathscr {A}}|+|{\mathscr {A}}'|-|{\mathscr {A}}\cap {\mathscr {A}}'|}\). The desired result follows immediately from the discussion above. \(\square \)
4 Self-dual negacyclic codes of length \({p^s}\) over 
In this section, the characterization and enumeration of self-dual negacyclic codes of length \({p^s}\) over  are established under both the Euclidean and Hermitian inner products.
are established under both the Euclidean and Hermitian inner products.
4.1 Euclidean self-dual negacyclic codes of length \({p^s}\) over 
We define a conjugation  on
on  to be the map that fixes
to be the map that fixes  and sends \({x^l}\) to \({x^{-l}}\) for all \({l\in {\mathbb {Z}}_{p^s}}\). For each subset A of the ring
and sends \({x^l}\) to \({x^{-l}}\) for all \({l\in {\mathbb {Z}}_{p^s}}\). For each subset A of the ring  , we denote by \({{\overline{A}}}\) the set of polynomials \({\overline{f(x)}}\) for all f(x) in A. The following result can be derived similarly to [10, Theorem 18].
, we denote by \({{\overline{A}}}\) the set of polynomials \({\overline{f(x)}}\) for all f(x) in A. The following result can be derived similarly to [10, Theorem 18].
Theorem 4.1
Let C be an ideal in  . Then
. Then  .
.
Theorem 4.2
The Euclidean dual \({C^{\bot _{\mathrm {E}}}}\) of the ideal  given in Theorem 3.6 is of the form
given in Theorem 3.6 is of the form

Proof
From Theorem 3.6, it can be concluded that

By Theorem 4.1, it follows that  . Hence, \({C^{\bot _{\mathrm {E}}}}\) contains the elements \({u(x+1)^{p^s-i_{0}}}\) and
. Hence, \({C^{\bot _{\mathrm {E}}}}\) contains the elements \({u(x+1)^{p^s-i_{0}}}\) and

By writing \({x=(x+1)-1}\) and applying the Binomial Theorem, it can be concluded that \({C^{\bot _{\mathrm {E}}}}\) contains an element of the form

Hence,
 Comparing the cardinalities, the two sets are equal. Updating the indices, it follows that
Comparing the cardinalities, the two sets are equal. Updating the indices, it follows that

as desired. \(\square \)
We note that, if \({i_1=0}\), then \({C=\langle u \rangle }\) is the only Euclidean self-dual ideal.
Assume that C is Euclidean self-dual. Then \({C=C^{\bot _{\mathrm{E}}}}\) which implies that \({| C |=(p^k)^{p^s}}\) and \({i_0+i_1=p^s}\).
Assume that \({i_1\geqslant 1}\). Since

is Euclidean self-dual in  , it follows that \({p^s=i_0+i_1}\) and
, it follows that \({p^s=i_0+i_1}\) and

in \({{\mathbb {F}}_{\! p^k}}\) for all \({0\leqslant t\leqslant i_i-1}\) by Theorem 4.2.
Let  be an
be an  -matrix defined by
-matrix defined by

It is easily seen that the \({i_1}\) equations from (2) are equivalent to the matrix equation

where \({{\varvec{h}}=(h_0,h_1,\ldots ,h_{i_1-1})^{\mathrm{T}}}\) and \({{\varvec{0}}=(0,0,\ldots ,0)^{\mathrm{T}}}\).
Hence, the ideal C in  is Euclidean self-dual if and only if \({p^s=i_0+i_1}\) and \({h_0,h_1,\ldots ,h_{i_1-1}}\) satisfy (4). Since \({h_0=h_1=\cdots =h_{i_1-1}=0}\) is a solution to (4), the corresponding ideal
is Euclidean self-dual if and only if \({p^s=i_0+i_1}\) and \({h_0,h_1,\ldots ,h_{i_1-1}}\) satisfy (4). Since \({h_0=h_1=\cdots =h_{i_1-1}=0}\) is a solution to (4), the corresponding ideal  is a Euclidean self-dual negacyclic code in
is a Euclidean self-dual negacyclic code in  . Hence, for a fixed first torsion degree \({1\leqslant i_1\leqslant p^s}\), a Euclidean self-dual ideal in
. Hence, for a fixed first torsion degree \({1\leqslant i_1\leqslant p^s}\), a Euclidean self-dual ideal in  always exists. By solving (4), all Euclidean self-dual ideals in
always exists. By solving (4), all Euclidean self-dual ideals in  can be constructed.
can be constructed.
In order to determine the number of solutions to (4), or equivalently, the nullity of  , we recall the
, we recall the  -matrix
-matrix  over
over  defined in [13] as
defined in [13] as

The nullity of  can be determined in terms of
can be determined in terms of  as in the following theorem.
as in the following theorem.
Theorem 4.3
Let \({i_1}\) be a positive integer such that \({i_1\leqslant p^s}\). Then the nullity of  is
is  .
.
Proof
First, we show that  and
and  have the same nullity. We consider the following two cases.
have the same nullity. We consider the following two cases.
Case 1: \({i_1}\) is odd. Let  be an
be an  -matrix over
-matrix over  . It is not difficult to see that A is invertible and
. It is not difficult to see that A is invertible and  .
.
Case 2: \({i_1}\) is even. Let  be an \({i_1{\times } i_1}\) matrix over
be an \({i_1{\times } i_1}\) matrix over  . It is not difficult to see that A is invertible and
. It is not difficult to see that A is invertible and  .
.
From the two cases, the nullity of  and the nullity of
and the nullity of  are equal. From [13, Proposition 3.3], the nullity of
are equal. From [13, Proposition 3.3], the nullity of  is
is  . Hence, the nullity of
. Hence, the nullity of  is
is  as desired. \(\square \)
as desired. \(\square \)
Proposition 4.4
Let \({i_1>0}\) and let \({\kappa }\) be the nullity of  over
over  . Then the number of Euclidean self-dual negacyclic codes of length \({p^s}\) over
. Then the number of Euclidean self-dual negacyclic codes of length \({p^s}\) over  with first torsional degree \({i_1}\) is \({(p^k)^\kappa }\).
with first torsional degree \({i_1}\) is \({(p^k)^\kappa }\).
From Theorem 3.6, we have \({0\leqslant i_1\leqslant \lfloor {p^s}/{2}\rfloor }\) since \({i_0+i_1=p^s}\).
Corollary 4.5
Let k and s be positive integers and let p an odd prime. Then the number of Euclidean self-dual negacyclic codes of length \({p^s}\) over  is
is

Proof
From Theorem 4.3 and Proposition 4.4, the number of Euclidean self-dual negacyclic codes of length \({p^s}\) over  is
is  . Note that \({p^s \equiv 3 \ \mathrm{mod }\, 4}\) (resp., \({p^s \equiv 1 \ \mathrm{mod }\, 4}\)) if and only if \({({p^{s}+1})/{4}}\) (resp., \({({p^{s}-1})/{4}}\)) is an integer. Hence, the results follow from a geometric sum. \(\square \)
. Note that \({p^s \equiv 3 \ \mathrm{mod }\, 4}\) (resp., \({p^s \equiv 1 \ \mathrm{mod }\, 4}\)) if and only if \({({p^{s}+1})/{4}}\) (resp., \({({p^{s}-1})/{4}}\)) is an integer. Hence, the results follow from a geometric sum. \(\square \)
4.2 Hermitian self-dual negacyclic codes of length \({p^s}\) over 
Under the assumption that k is even, characterization and enumeration Hermitian self-dual negacyclic codes of length \({p^s}\) over  are given. For a subset A of
are given. For a subset A of  , let
, let

where  is the automorphism defined in (1).
is the automorphism defined in (1).
Based on the unique presentation of a negacyclic code given in Theorem 3.6, its Hermitian dual can be determined using Theorem 4.2 and the fact that \({C^{\bot _{\mathrm {H}}}=\widetilde{C^{\perp _{\mathrm{E}}}}}\).
Theorem 4.6
The Hermitian dual \({C^{\bot _{\mathrm {H}}}}\) of the ideal  given in Theorem 3.6 has the representation
given in Theorem 3.6 has the representation

If \({i_1=0}\), then it is not difficult to see that the ideal generated by u is the only Hermitian self-dual negacyclic code of length \({p^s}\) over  .
.
Assume that C is Hermitian self-dual. Then \({C=C^{\bot _{\mathrm{H}}}}\) implies that \({| C |=(p^k)^{p^s}}\) and \({i_0+i_1=p^s}\).
Assume that \({i_1\geqslant 1}\). Then, by Theorems 3.6 and 4.6, we have

for all \({0\leqslant t\leqslant i_1-1.}\)
From the \({i_1}\) equations above and the definition of  , we have
, we have

where \({{\varvec{x}}=(x_1,x_2,\ldots ,x_{i_1})^{\mathrm{T}}}\),  and
and  . It can be concluded that an ideal C in
. It can be concluded that an ideal C in  is Hermitian self-dual if and only if \({p^s=i_0+i_1}\) and \({h_0,h_1,\ldots ,h_{i_1-1}}\) satisfy (5). Since \({h_0=h_1=\dots =h_{i_1-1}=0}\) is a solution to (5), the corresponding ideal
is Hermitian self-dual if and only if \({p^s=i_0+i_1}\) and \({h_0,h_1,\ldots ,h_{i_1-1}}\) satisfy (5). Since \({h_0=h_1=\dots =h_{i_1-1}=0}\) is a solution to (5), the corresponding ideal  is a Hermitian self-dual negacyclic code in
is a Hermitian self-dual negacyclic code in  . Hence, for a fixed first torsion degree \({1\leqslant i_1\leqslant p^s}\), a Hermitian self-dual negacyclic code in
. Hence, for a fixed first torsion degree \({1\leqslant i_1\leqslant p^s}\), a Hermitian self-dual negacyclic code in  always exists. By solving (5), all Hermitian self-dual negacyclic codes in
always exists. By solving (5), all Hermitian self-dual negacyclic codes in  can be constructed.
can be constructed.
In order to determine the number of solutions to (5), we shall need some properties of  . For integers i and j such that \({1\leqslant i, j \leqslant i_1}\), let \({v_{ij}}\) denote the entry in the ith row and the jth column of the matrix
. For integers i and j such that \({1\leqslant i, j \leqslant i_1}\), let \({v_{ij}}\) denote the entry in the ith row and the jth column of the matrix  . For an integer l, \({1 \leqslant l \leqslant j< i\leqslant i_1}\), we have
. For an integer l, \({1 \leqslant l \leqslant j< i\leqslant i_1}\), we have
and
The following two maps in [10] are key to determine the number of solutions to (5) in  :
:
-
The map
 is defined by
is defined by  for all
for all  .
. -
The trace map
 is defined by
is defined by  for all
for all  .
.
From [10], we have that \({{\Psi }}\) and \({{\mathrm{Tr}}}\) are  -linear.
-linear.
Lemma 4.7
([10, Lemma 3.2]) Let \({{\mathrm{Tr}}}\) and \({{\Psi }}\) be defined as above. Then the following statements hold:
-
For each
 , \({{\Psi } (\alpha )=0}\) if and only if
, \({{\Psi } (\alpha )=0}\) if and only if  .
. -
 .
. -
For each
 , \({| {\Psi } ^{-1}(a)|=p^{k/2}}\).
, \({| {\Psi } ^{-1}(a)|=p^{k/2}}\). -
For each
 , \({|{\mathrm{Tr}}^{-1}(a)|=p^{k/2}}\).
, \({|{\mathrm{Tr}}^{-1}(a)|=p^{k/2}}\).
Similarly to [10, Proposition 3.3], we have
Proposition 4.8
Let k and s be positive integers such that k is even, and let p be an odd prime. Let \({i_1}\) be a positive integer such that \({i_1\leqslant \lfloor {p^{s}}/{2}\rfloor }\). Then the number of solutions to (5) in  is \({p^{ki_1/2}}\).
is \({p^{ki_1/2}}\).
Proof
From (3), the diagonal of  has two different presentations depending on the parity of \({i_1}\). Hence, the proof consists of two cases.
has two different presentations depending on the parity of \({i_1}\). Hence, the proof consists of two cases.
Case 1: \({i_1}\) is odd. Then \({i_1=2\mu +1}\) for some non-negative integer \({\mu }\). From (3), the matrix  can be written as
can be written as

where \({*}\) denotes an entry of the matrix  as defined in (3). It is easily seen that
as defined in (3). It is easily seen that
for all integers \({1\leqslant i \leqslant \mu }\). We observe that (5) has a solution if and only if the right-hand sides of (6) and (8) are in  and the right-hand side of (7) is in
and the right-hand side of (7) is in  . In this case, it can be deduced that
. In this case, it can be deduced that

for all integers \({1\leqslant i \leqslant \mu }\). Equivalently, the number of solutions to (5) is \({p^{ki_1/2}}\) by Lemma 4.7.
To reason the discussion above, by Lemma 4.7, it suffices to show that the images under \({{\Psi }}\) of the right-hand sides of (6) and (8) are 0 and the image under the trace map \({{\mathrm{Tr}}}\) of the right-hand side of (7) is 0. We note that the image under \({{\Psi }}\) of the right-hand sides of (6) is 0.
Let \({1\leqslant i \leqslant \mu }\) be an integer. Using calculation similar to the one in [10, Equation (3.11)], it follows that

Using calculation similar to the one in [10, Equation (3.12)], it follows that

Case 2: \({i_1}\) is even. Then \({i_1=2\mu }\) for some positive integer \({\mu }\). From (3), the matrix  can be written as
can be written as

where \({*}\) denotes an entry of the matrix  defined in (3). We see that
defined in (3). We see that
for all integers \({1\leqslant i \leqslant \mu }\).
We observe that (5) has a solution if and only if the right-hand side of (9) is in  and the right-hand side of (10) is in
and the right-hand side of (10) is in  . In this case, the number of solutions to (5) is \({p^{ki_1/2}}\) by Lemma 4.7. By Lemma 4.7 again, it is sufficient to show that the image under the trace map \({{\mathrm{Tr}}}\) of right-hand side of (9) and the image under the \({{\Psi }}\) of right-hand side of (10) are 0. By using calculation similar to the one in Case 1, the proof is completed. \(\square \)
. In this case, the number of solutions to (5) is \({p^{ki_1/2}}\) by Lemma 4.7. By Lemma 4.7 again, it is sufficient to show that the image under the trace map \({{\mathrm{Tr}}}\) of right-hand side of (9) and the image under the \({{\Psi }}\) of right-hand side of (10) are 0. By using calculation similar to the one in Case 1, the proof is completed. \(\square \)
Corollary 4.9
Let k and s be positive integers such that k is even, and let p be an odd prime. Then the number of Hermitian self-dual negacyclic codes of length \({p^s}\) over  is
is

References
Cao, Y.: On constacyclic codes over finite chain rings. Finite Fields Appl. 24, 124–135 (2013)
Choosuwan, P., Jitman, S., Udomkavanich, P.: Self-dual abelian codes in some nonprincipal ideal group algebras. Math. Probl. Eng. 2016, # 9020173 (2016)
Dinh, H.Q.: Constacyclic codes of length \({p^s}\) over \({{\mathbb{F}}_{p^m}+u{\mathbb{F}}_{p^m}}\). J. Algebra 324(5), 940–950 (2010)
Dinh, H.Q.: Negacyclic codes of length \({2^s}\) over Galois rings. IEEE Trans. Inform. Theory 51(12), 4252–4262 (2005)
Dinh, H.Q., López-Permouth, S.R.: Cyclic and negacyclic codes over finite chain rings. IEEE Trans. Inform. Theory 50(8), 1728–1744 (2004)
Dinh, H.Q., Nguyen, B.T., Sriboonchitta, S.: Negacyclic codes of length \({4p^s}\) over \({{\mathbb{F}}_{p^m}+u{\mathbb{F}}_{p^m}}\) and their duals. Discrete Math. 341(4), 1055–1071 (2018)
Dinh, H.Q., Sharma, A., Rani, S., Sriboonchitta, S.: Cyclic and negacyclic codes of length \({4p^s}\) over \({{\mathbb{F}}_{p^m}+u{\mathbb{F}}_{p^m}}\). J. Algebra Appl. 9, # 1850173 (2018)
Dinh, H.Q., Wang, L., Zhu, S.: Negacyclic codes of length \({2p^s}\) over \({{\mathbb{F}}_{p^m}+u{\mathbb{F}}_{p^m}}\). Finite Fields Appl. 31, 178–201 (2015)
Hammons Jr., A.R., Kumar, P.V., Calderbank, A.R., Sloane, N.J.A., Solé, P.: The \({{\mathbb{Z}}_4}\) linearity of Kerdock, Preparata, Goethals and related codes. IEEE Trans. Inform. Theory 40(2), 301–319 (1994)
Jitman, S., Ling, S., Sangwisut, E.: On self-dual cyclic codes of length \({p^a}\) over \({{\rm GR}(p^2, s)}\). Adv. Math. Commun. 10(2), 255–273 (2016)
Kai, X., Zhu, S.: Negacyclic self-dual codes over finite chain rings. Des. Codes Cryptogr. 62(2), 161–174 (2012)
Kiah, H.M., Leung, K.H., Ling, S.: Cyclic codes over \({{\rm GR}(p^2, m)}\) of length \({p^k}\). Finite Fields Appl. 14(3), 834–846 (2008)
Kiah, H.M., Leung, K.H., Ling, S.: A note on cyclic codes over \({{\rm GR}(p^2, m)}\) of length \({p^k}\). Des. Codes Cryptogr. 63(1), 105–112 (2012)
Liu, X., Xu, X.: Cyclic and negacyclic codes of length \({2p^s}\) over \({{\mathbb{F}}_{p^m}+u{\mathbb{F}}_{p^m}}\). Acta Math. Sci. Ser. B Engl. Ed. 34(3), 829–839 (2014)
Sobhani, R., Esmaeili, M.: Cyclic and negacyclic codes over the Galois ring \({{\rm GR} (p^2, m)}\). Discrete Appl. Math. 157(13), 2892–2903 (2009)
Wolfman, J.: Negacyclic and cyclic codes over \({{\mathbb{Z}}_4}\). IEEE Trans. Inform. Theory 45(7), 2527–2532 (1999)
Acknowledgements
The authors would like to thank the anonymous referees for very helpful comments.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
P. Choosuwan was partially supported by the Faculty of Science and Technology, Rajamangala University of Technology Thanyaburi (RMUTT). S. Jitman was supported by the Thailand Research Fund and Silpakorn University under Research Grant RSA6280042.
Rights and permissions
About this article
Cite this article
Choosuwan, P., Jitman, S. & Udomkavanich, P. A note on self-dual negacyclic codes of length \(p^s\) over  .
European Journal of Mathematics 6, 1424–1437 (2020). https://doi.org/10.1007/s40879-019-00378-9
.
European Journal of Mathematics 6, 1424–1437 (2020). https://doi.org/10.1007/s40879-019-00378-9
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40879-019-00378-9




 and
and  .
. is an ideal of
is an ideal of  .
.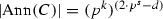 .
. .
.


 is defined by
is defined by  for all
for all  .
. is defined by
is defined by  for all
for all  .
. ,
,  .
. .
. ,
,  ,
,