1 Introduction

Structure formation in the astrophysical plasma environment is one of the most important and fascinating processes in modern astrophysics and cosmology. Structures are formed in the astrophysical plasma environment because of unstable modes produced by the thermally unstable medium. Thermally unstable modes are produced due to thermal instability in the interstellar medium. Thermal instability takes places in a medium which can turn out to be cooler owing to radiation and fluid reduction. Additionally, reduction in the temperature constructs the arrangement unbalanced and directs to configuration of novel configurations due to density strengthening. In this instability, the critical length scale is smaller than that of the other dynamical instability like the Jeans instability, i.e., a system can become thermally unbalanced even if the organization is stable beside the gravitational instability. Hence, we can say that the physical basis of smaller-scale configurations is owing to thermal instability rather than energetic instability. It is straightly correlated with the structure of different stages in the diffuse interstellar and intergalactic media, as well as in the solar atmosphere. The thermal and radiative instability arising due to various heat-loss mechanisms could definitely be the reason for the astrophysical condensation and the configuration of large-scale structures as well as of small objects. Several authors investigated the phenomenon of thermal instability arising due to heat-loss mechanism in plasma. Field [1] has discussed the importance of thermal instability in the formation of solar prominences, condensation in planetary nebula, and condensation of galaxies from the intergalactic medium. Hunter [2] has discussed the role of thermal instability in star formation. Raju [3] has emphasis on the role of thermal instability in the formation of solar prominences. Aggarwal and Talwar [4] have investigated magneto-gravitational instability in a rotating gravitating fluid taking radiative heat-loss function. Ibanez [5] has studied the sound and thermal waves in a fluid with an arbitrary heat-loss function. Hoven and Mok [6] have carried out the problem of thermal instability in a sheared magnetic field. Bodo et al. [7] have investigated magnetohydrodynamic thermal instability in cool inhomogeneous atmosphere. Bora and Talwar [8] have discussed the magnetothermal instability with generalized Ohm’s law taking the effects of electrical resistivity, electron inertia, thermal conductivity, and radiative heat-loss function. Burkert and Lin [9] have pointed out the importance of thermal instability in the formation of clumpy gas clouds, and have shown that the thermal instability can lead to the breakup of large clouds into cold dense clumps. Shadmeri and Ghanbari [10] have discussed the problem of radiative cooling flows of self-gravitating filamentary clouds. Nejad-Asghar and Ghanbari [11] have discussed the formation of small-scale condensation in the molecular clouds via thermal instability. Baruah et al. [12] have studied the thermal (radiative) instability in weakly ionized plasma with continuous ionization and recombination taking general heat-loss function. Fukue and Kamaya [13] have studied the thermal instability of partially ionized plasma taking radiative cooling function and two-fluid theory into account. Recently, Prajapati et al. [14] have investigated the thermal instability of rotating viscous Hall plasma with arbitrary radiative heat-loss function and electron inertia. More recently, Kaothekar and Chhajlani [15] have examined the effect of porosity and finite ion Larmor radius (FLR) corrections on Jeans instability of self-gravitating radiative thermally conducting viscous plasma. Thus, thermal and radiative effects are important in investigations of plasma instability.

In addition to this, the electron inertia parameter is important in the dynamics of interstellar matter, in magnetic reconnection processes, in stability investigation of accelerated plasmas, and in several other astrophysical situations. Tayler [16] has discussed a simple hydromagnetic stability problem involving finite conductivity, electron inertia, and Hall effect. Kalra and Talwar [17] have investigated magneto-thermal instability of unbounded plasma with electron inertia and Hall effect. Pegoraro et al. [18] have shown the importance of electron inertia in non-uniform collisionless plasma having small-scale magnetic structures. Chatterjee and Das [19] have pointed out the effect of electron inertia on the speed and shape of ion-acoustic solitary waves in plasma. Shukla et al. [20] have studied the effect of electron inertia on kinetic Alfven waves. Damiano et al. [21] have pointed out the effects of electron inertia and FLR on Hall magnetohydrodynamic waves. Uberoi [22] has discussed electron inertia effects on the transverse thermal instability incorporating the rotation parameters. Recently, Pensia et al. [23] have investigated the effect of black body radiation and electron inertia on the Jeans instability of rotating and magnetized gaseous plasma of the interstellar medium. More recently, Sutar and Pensia [24] have carried out the problem of electron inertia effects on the gravitational instability under the influence of FLR corrections and suspended particles. Thus, finite electron inertia is an important factor in the discussion of thermal instability and other hydromagnetic instability.

Along with this, as in the above discussed problems, the effect of finite ion Larmor radius is not considered. In many astrophysical plasma situations such as in solar corona, interstellar, and interplanetary plasmas, the assumption of zero Larmor radius is not valid. Roberts and Taylor [25] and Rosenbluth et al. [26] have shown the stabilizing influence of FLR effects on plasma instabilities. Herrnegger [27] has investigated the stabilizing effect of FLR on gravitational instability, and shown that the gravitational criterion is changed by FLR for wave propagation perpendicular to the magnetic field. Sharma [28] has investigated the stabilizing effect of FLR on gravitational instability of rotating plasma. Ariel [29] has discussed the stabilizing effect of FLR on gravitational instability of conducting plasma layer of finite thickness surrounded by a non-conducting matter. Vaghela and Chhajlani [30] have studied the stabilizing effect of FLR on magneto-gravitational stability of resistive plasma through a porous medium with thermal conduction. Bhatia and Chhonkar [31] have investigated the stabilizing effect of FLR on the instability of a rotating layer of self-gravitating plasma incorporating the effects of viscosity. Vyas and Chhajlani [32] have pointed out the stabilizing effect of FLR on the gravitational instability of magnetized rotating plasma incorporating the effects of viscosity, finite electrical conductivity, porosity, and thermal conductivity. Marcu and Ballai [33] have shown the stabilizing effect of FLR on thermosolutal stability of a two-component rotating plasma. Kaothekar and Chhajlani [34] have investigated the effect of radiative heat-loss function and finite Larmor radius corrections on Jeans instability of viscous thermally conducting self-gravitating astrophysical plasma. Recently, Kaothekar and Chhajlani [35] have carried out the problem of Jeans instability of self-gravitating rotating radiative plasma with finite Larmor radius corrections. More recently, Kaothekar et al. [36] have investigated the effect of Jeans instability of partially ionized self-gravitating viscous plasma with Hall effect FLR corrections and porosity. Thus, FLR effect is an important factor in the discussion of thermal instability and other hydromagnetic instability.

In the light of the above work, we find that in these studies (Vyas and Chhajlani [32], Bora and Talwar [8], Prajapati et al. [14], and Kaothekar et al. [36]), the joint influence of FLR corrections, electron inertia, radiative heat-loss functions, viscosity, electrical resistivity, thermal conductivity, and magnetic field on the thermal instability is not investigated. Therefore, in the present work, thermal instability of viscous magnetized plasma with electron inertia, FLR corrections, radiative heat-loss functions, thermal conductivity, and finite electrical resistivity for thermal configuration is studied.

This paper is organized as follows. Section 2 contains the basic equations for a magneto thermal system. In Sect. 3, linearized perturbed equations and the dispersion relation are derived for the first-order approximation. The instability criterion for thermal modes is derived for longitudinal and transverse propagation in Sect. 4. Numerical interpretation of the linear growth rate is done in Sect. 5. Finally, Sect. 6 contains the summary and discussion of the results.

2 Equations of the Problem

Let us consider an infinite homogeneous, thermally conducting, radiating, viscous plasma with finite electron inertia and finite electrical resistivity in the presence of magnetic field B (0, 0, B). The equations of the problem with these effects are written as

$$ \frac{d\boldsymbol{u}}{dt}=-\frac{1}{\rho}\mathbf{\nabla}p-\frac{\mathbf{\nabla}\cdot \overleftrightarrow{\boldsymbol{P}}}{\rho }+\frac{1}{4\pi \rho}\left(\mathbf{\nabla}\times \boldsymbol{B}\right)\times \boldsymbol{B}+\upsilon {\nabla}^2\boldsymbol{u}, $$
(1)
$$ \frac{d\rho }{dt}=-\kern0.3em \rho \mathbf{\nabla}.\boldsymbol{u}\kern0.3em , $$
(2)
$$ \frac{1}{\gamma -1}\frac{dp}{dt}-\frac{\gamma }{\gamma -1}\frac{p}{\rho}\frac{d\rho }{dt\kern0.3em }+\rho \kern0.2em L-\mathbf{\nabla}.\left(\lambda \mathbf{\nabla}T\right)=0, $$
(3)
$$ p=\rho RT, $$
(4)
$$ \frac{\partial \boldsymbol{B}}{\partial \mathrm{t}}=\mathbf{\nabla}\times \left(\boldsymbol{u}\times \boldsymbol{B}\right)+\eta {\nabla}^2\boldsymbol{B}+\frac{c^2}{\omega_{pe}^2}\frac{\partial }{\partial t}{\nabla}^2\boldsymbol{B}, $$
(5)
$$ \mathbf{\nabla}.\boldsymbol{B}=0. $$
(6)

where ρ, p, υ, T, u (u x , u y , u z ), η, λ, R, γ ,   c, and ω pe denote the fluid density, pressure, kinematic viscosity, temperature, velocity, electrical resistivity, thermal conductivity, gas constant, ratio of two specific heats, velocity of light, and electron plasma frequency, respectively. Here, L (ρ, T) is the heat-loss function per gram of the material per second exclusive of thermal conduction and is in general a function of the local values of density and temperature. The operator (d/dt) is the substantial derivative given as (d/dt) = (∂ t + u . ∇). \( \overleftrightarrow{\boldsymbol{P}} \) is the pressure tensor taking into account the effect of finite ion gyration radius for the magnetic field along the z-axis as given by Roberts and Taylor [25] which is

$$ \begin{array}{l}{P}_{xx}=-\rho {\upsilon}_0\left(\frac{\partial {u}_y}{\partial x}+\frac{\partial {u}_x}{\partial y}\right),\kern8.099995em {P}_{yy}=\rho {\upsilon}_0\left(\frac{\partial {u}_y}{\partial x}+\frac{\partial {u}_x}{\partial y}\right),\kern1.7em \\ {}{P}_{zz}=0,\kern13.80002em {P}_{xy}={P}_{yx}=\kern0.6em \rho {\upsilon}_0\left(\frac{\partial {u}_x}{\partial x}-\frac{\partial {u}_y}{\partial y}\right),\kern0.3em \\ {}\kern0.1em {P}_{xz}={P}_{zx}=-2\rho {\upsilon}_0\left(\frac{\partial {u}_y}{\partial z}+\frac{\partial {u}_z}{\partial y}\right),\kern1.9em {P}_{yz}={P}_{zy}=2\rho {\upsilon}_0\left(\frac{\partial {u}_z}{\partial x}+\frac{\partial {u}_x}{\partial z}\right).\kern0.5em \end{array} $$
(7)

The parameter υ 0 has the dimensions of the kinematic viscosity and is defined as \( {\upsilon}_0={\varOmega}_L{R}_L^2/4 \), where R L is the ion-Larmor radius and Ω L is the ion gyration frequency.

3 Linearized Perturbation Equations

The perturbation in fluid density, pressure, temperature, velocity, magnetic field, pressure tensor, and heat-loss function is given as δρ, δp, δT, u (u x , u y , u z ), δ B (δB x , δB y , δB z ), \( \boldsymbol{\delta} \overleftrightarrow{\boldsymbol{P}} \), and L, respectively. The perturbation state is given as

$$ \begin{array}{llll}\rho ={\rho}_0+\delta \rho, \hfill & p={p}_0+\delta p,\hfill & T={T}_0+\delta T,\hfill & \boldsymbol{u}={\boldsymbol{u}}_0+\boldsymbol{u}\left(\mathrm{with}\ {\boldsymbol{u}}_0=0\right),\hfill \\ {}\boldsymbol{B}={\boldsymbol{B}}_0+\boldsymbol{\delta} \boldsymbol{B},\hfill & \overleftrightarrow{\boldsymbol{P}}={\overleftrightarrow{\boldsymbol{P}}}_0+\boldsymbol{\delta} \overleftrightarrow{\boldsymbol{P}},\hfill & \mathrm{and}\hfill & L={L}_0+L\left(\mathrm{with}\ {L}_0=0\right).\hfill \end{array} $$
(8)

Suffix “0” represents the initial equilibrium state, which is independent of space and time.

Substituting the perturbation state into Eqs. (1), (2), (3), (4), (5), and (6) and linearizing them by neglecting higher order perturbations, suffix “0” is dropped from the equilibrium quantities.

The linearized perturbation equations of motion for such medium are

$$ {\partial}_t\boldsymbol{u}=-\frac{1}{\rho}\mathbf{\nabla}\delta p-\frac{\mathbf{\nabla}\cdot \overleftrightarrow{\boldsymbol{P}}}{\rho }+\frac{1}{4\pi \rho}\left(\mathbf{\nabla}\times \boldsymbol{\delta} \boldsymbol{B}\right)\times \boldsymbol{B}\kern0.5em +\upsilon {\nabla}^2\boldsymbol{u}\kern0.7em , $$
(9)
$$ \partial {\kern0.1em }_{\mathrm{t}}\kern0.3em \delta \rho \kern0.4em =\kern0.3em -\rho \mathbf{\nabla}.\boldsymbol{u}\kern0.3em , $$
(10)
$$ \frac{1}{\gamma -1}{\partial}_t\kern0.3em \delta p-\kern0.3em \frac{\gamma }{\gamma -1}\frac{p}{\rho }{\partial}_t\kern0.2em \delta \rho +\kern0.3em \rho \left({L}_{\rho}\delta \rho \kern0.3em +\kern0.3em {L}_T\delta T\right)-\kern0.3em \lambda {\nabla}^2\delta T\kern0.3em =\kern0.3em 0, $$
(11)
$$ \frac{\delta p}{p}\kern0.3em =\kern0.3em \frac{\delta T}{T}\kern0.3em +\kern0.3em \frac{\delta \rho }{\rho }, $$
(12)
$$ \partial {\kern0.1em }_{\mathrm{t}}\kern0.2em \boldsymbol{\delta} \boldsymbol{B}=\kern0.6em \mathbf{\nabla}\times \left(\boldsymbol{u}\times \boldsymbol{B}\right)\kern0.3em +\kern0.7em \eta {\nabla}^2\boldsymbol{\delta} \boldsymbol{B}\kern0.6em +\frac{c^2}{\omega_{pe}^2}\partial {\kern0.1em }_{\mathrm{t}}{\nabla}^2\boldsymbol{\delta} \boldsymbol{B}, $$
(13)
$$ \mathbf{\nabla}.\boldsymbol{\delta} \boldsymbol{B}\kern0.3em =\kern0.3em 0, $$
(14)

where L T and L ρ respectively denote partial derivatives (∂L/∂T) ρ and (∂L/∂ρ) T of the heat-loss function evaluated for the initial (unperturbed) state.

4 Dispersion Relation

We seek plain wave solution of the form

$$ \exp \left(i{k}_xx+i{k}_zz+i\sigma \kern0.1em t\right). $$
(15)

where σ  is the frequency of harmonic disturbance and k x and k z are the wave numbers of the perturbations along the x- and z-axes, where \( {k}^2={k}_x^2+{k}_z^2 \).

The perturbed pressure tensor components P xx are

$$ \begin{array}{lll}{P}_{xx}=-\rho {\upsilon}_0i{k}_x{u}_y,\hfill & {P}_{yy}=\rho {\upsilon}_0i{k}_x{u}_y,\hfill & {P}_{zz}=0,\hfill \\ {}{P}_{xy}={P}_{yx}=\rho {\upsilon}_0i{k}_x{u}_x,\hfill & {P}_{xz}={P}_{zx}=-2\rho {\upsilon}_0i{k}_z{u}_y,\hfill & {P}_{yz}={P}_{zy}=2i\rho {\upsilon}_0\left({k}_x{u}_z+{k}_z{u}_x\right).\hfill \end{array} $$
(16)

The components of Eq. (13) may be given as

$$ \begin{array}{l}\delta {B}_x=\left(iB/d\right){k}_z{u}_x,\hfill \\ {}\delta {B}_y=\left(iB/d\right){k}_z{u}_y,\hfill \\ {}\delta {B}_z=-\left(iB/d\right){k}_x{u}_x,\hfill \end{array} $$
(17)

Using Eqs. (11), (12), and (15), we write

$$ \delta p=\frac{\left\{\kern0.3em \left(\gamma -1\right)\left[\kern0.2em T{L}_T-\rho {L}_{\rho }+\left(\lambda \kern0.1em {k}^2T/\rho \right)\right]+\omega \kern0.1em {c}^2\kern0.3em \right\}}{\left\{\kern0.3em \left(\gamma -1\right)\left[\kern0.2em \left(T\rho /p\right){L}_T+\left(\lambda \kern0.1em {k}^2T/\rho \right)\right]+\omega \kern0.5em \right\}}\delta \rho . $$
(18)

Using Eqs. (10), (11), (12), (13), (14), (15), (16), (17), and (18) in Eq. (9), we may write the following algebraic equations for the components of Eq. (9)

$$ \left[\omega +\upsilon {k}^2+\frac{V^2{k}^2}{d}\right]\kern0.2em {u}_x+\left[{\upsilon}_0\left({k}_x^2+2{k}_z^2\right)\right]\kern0.2em {u}_y+\frac{i{k}_x}{k^2}{\varOmega}_T^2\kern0.2em \mathrm{s}=\kern0.3em 0, $$
(19)
$$ -\left[{\upsilon}_0\left({k}_x^2+2{k}_z^2\right)\right]\kern0.1em {u}_x+\left[\omega +\upsilon {k}^2\left.+\frac{V^2{k}_z^2}{d}\right]{u}_y-2{\upsilon}_0{k}_x{k}_z{u}_z=\kern0.3em 0,\right. $$
(20)
$$ 2{\upsilon}_0{k}_x{k}_z{u}_y+\left(\omega +\upsilon {k}^2\right){u}_z+\frac{i{k}_z}{k^2}\kern0.1em {\varOmega}_T^2\kern0.2em \mathrm{s}=\kern0.3em 0. $$
(21)

Taking the divergence of Eq. (9) and using Eqs. (10), (11), (12), (13), (14), (15), (16), (17), and (18), we obtain

$$ \left[i{k}_x\frac{V^2{k}^2}{d}\right]{u}_x+\left[i{\upsilon}_0{k}_x\left({k}_x^2+4{k}_z^2\right)\right]{u}_y-\kern0.4em \left[{\omega}^2+\omega \kern0.3em \upsilon {k}^2+{\varOmega}_T^2\right]\kern0.3em \mathrm{s}=0. $$
(22)

The set of Eqs. (19), (20), (21), and (22) can be written in the following form:

$$ \left[\begin{array}{l}\kern0.8000001em N\kern4.999998em P\kern5.499997em 0\kern5.199997em \frac{i{k}_x}{k^2}{\varOmega}_T^2\kern0.8000001em \\ {}\\ {}-P\kern4.999998em {N}_1\kern3.599999em -2{\upsilon}_0{k}_x{k}_z\kern3.399999em 0\kern2.2em \\ {}\\ {}\kern1.1em 0\kern4.299998em 2{\upsilon}_0{k}_x{k}_z\kern3.599999em {M}_1\kern3.999998em \frac{i{k}_z}{k^2}\kern0.1em {\varOmega}_T^2\kern1.7em \\ {}\\ {}\kern0.1em i{k}_x\kern0.1em D\kern4.599998em Q\kern5.599997em 0\kern4.899998em -{R}_1\kern0.1em \\ {}\kern1.4em \end{array}\right]\kern0.9000001em \left[\begin{array}{l}{u}_x\\ {}\\ {}\\ {}{u}_y\\ {}\\ {}\\ {}{u}_z\\ {}\\ {}\\ {}s\end{array}\right]=0. $$
(23)

We have made the following assumptions,

$$ \begin{array}{l}\kern0.2em {V}^2=\frac{B^2}{4\pi \rho },\kern1.2em {\varOmega}_T^2=\frac{\varOmega_I^2+\omega {\varOmega}_j^2}{\xi +\omega },\kern1.3em {\varOmega}_j^2={c}^2{k}^2,\kern1.4em {\varOmega}_I^2={k}^2A,\kern1.2em \mathrm{i}\sigma =\omega, \kern1.2em d=\left(\omega \alpha +\eta {k}^2\right),\hfill \\ {}A=\left(\gamma -1\right)\left(T{L}_T-\rho {L}_{\rho }+\frac{\lambda {k}^2T}{\rho}\right),\kern0.5em \xi =\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right),\kern0.4em \alpha =\left(1+\frac{c^2{k}^2}{\omega_{pe}^2}\right),\hfill \\ {}\kern0.1em Q=i{\upsilon}_0{k}_x\left({k}_x^2+4{k}_z^2\right)-i{k}_xE,\kern1em {N}_1={M}_1+\frac{{\left(\omega +\upsilon {k}^2\right)}^2{k}_z^2}{d},\kern0.8000001em {M}_1=\omega +\upsilon {k}^2,\kern0.7em F={\upsilon}_0\left({k}_x^2+2{k}_z^2\right),\hfill \\ {}{R}_1={\omega}^2+\omega \kern0.3em \upsilon {k}^2+{\varOmega}_T^2,\kern0.6em D=\frac{{\mathrm{V}}^2{k}^2}{d},\kern1.3em N={M}_1+D,\kern0.6em P=F,\hfill \\ {}c={\left(\gamma p/\rho \right)}^{{\scriptscriptstyle \raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}},s=\delta \rho /\rho, \mathrm{is}\ \mathrm{the}\ \mathrm{condensation}\ \mathrm{of}\ \mathrm{the}\ \mathrm{medium}.\hfill \end{array} $$
(24)

The general dispersion relation can be obtained from the determinant of the matrix of Eq. (23) which is

$$ \begin{array}{l}\left[{\omega}^2+\omega \kern0.3em \upsilon {k}^2+{\varOmega}_T^2\right]\left[\omega +\upsilon {k}^2+\frac{V^2{k}^2}{d}\right]\left\{\left(\omega +\upsilon {k}^2\right)\left.\left[\omega +\left.\upsilon {k}^2+\frac{V^2{k}_z^2}{d}\right]\right.+4{\upsilon}_0^2{k}_x^2{k}_z^2\right\}\right.\hfill \\ {}+\frac{2{\upsilon}_0i{k}_x{k}_z^2}{k^2}{\varOmega}_T^2\left[\omega +\upsilon {k}^2+\frac{V^2{k}^2}{d}\right]\left[i{\upsilon}_0{k}_x\left({k}_x^2+4{k}_z^2\right)\right]+\left[\omega +\upsilon {k}^2\right]\kern0.2em \left[{\omega}^2+\omega \kern0.1em \upsilon {k}^2\right.\left.+{\varOmega}_T^2\right]\hfill \\ {}\times {\left[{\upsilon}_0\left({k}_x^2+2{k}_z^2\right)\right]}^2+\frac{2{\upsilon}_0{k}_x^2{k}_z^2{V}^2}{d}\kern0.3em {\varOmega}_T^2\left[{\upsilon}_0\left({k}_x^2+2{k}_z^2\right)\right]+\frac{i{k}_x}{k^2}{\varOmega}_T^2\left[{\upsilon}_0\left({k}_x^2+2{k}_z^2\right)\right]\kern0.2em \left[\omega +\upsilon {k}^2\right]\hfill \\ {}\times \left[i{k}_x{\upsilon}_0\left({k}_x^2+4{k}_z^2\right)\right]-\frac{V^2{k}_x^2}{d}{\varOmega}_T^2\kern0.1em \left[\omega +\upsilon {k}^2\right]\left[\omega +\upsilon {k}^2\right]-4{\upsilon}_0^2{k}_x^4{k}_z^2\frac{V^2}{d}{\varOmega}_T^2=0.\hfill \end{array} $$
(25)

The dispersion relation (25) represents the combined influence of viscosity, finite electrical conductivity, magnetic field, thermal conductivity, radiative heat-loss function, finite electron inertia, and FLR corrections on thermal instability of plasma. In the absence of FLR corrections, dispersion relation (25) is identical to Prajapati et al. [14] for the non-rotational and non-gravitational cases. In the absence of FLR corrections and viscosity dispersion, relation (25) is identical to Bora and Talwar [8] for the non-gravitational case. In the absence of radiative heat-loss function, thermal conductivity, finite electrical resistivity, viscosity, and finite electron inertia, the general dispersion relation (25) is identical to Sharma [28] for the non-rotational and non-gravitational cases. In the absence of viscosity, finite electrical resistivity, FLR corrections, thermal conductivity, and radiative heat-loss function dispersion, relation (25) is reduced to that obtained by Damiano et al. [21]. Also, in the absence of FLR corrections, viscosity, finite conductivity, finite electron inertia, and thermal conductivity dispersion, relation (25) is reduced to that obtained by Field [1]. The general dispersion relation (25) is identical to Kaothekar et al. [36] for the non-gravitational and non-porous cases.

5 Discussion

5.1 Longitudinal Wave Propagation (k x  = 0,   k z  = k)

In this case, the perturbations are taken to be parallel to the direction of the magnetic field (i.e., k x  = 0 ,  k z  = k). The dispersion relation (25) reduces to

$$ \left[\omega +\upsilon {k}^2\right]\times \left.\left\{{\left[\omega +\upsilon {k}^2+\frac{{\mathrm{V}}^2{k}^2}{d}\right]}^2+\right.{\left[2{\upsilon}_0{k}^2\right]}^2\right\}\times \left[{\omega}^2+\omega \kern0.3em \upsilon {k}^2+{\varOmega}_T^2\right]=0. $$
(26)

The first component of the dispersion relation (26) gives

$$ \omega +\upsilon {k}^2=0. $$
(27)

This represents a damped mode modified by the presence of viscosity of the medium. Thus, the viscous force is capable of stabilizing the growth rate of the considered system. The above mode is unaffected by the presence of FLR correction, finite electron inertia, magnetic field strength, finite electrical resistivity, thermal conductivity, and radiative heat-loss function. This dispersion relation is identical to Prajapati et al. [14].

The second factor of Eq. (26) on simplification gives

$$ \begin{array}{l}{\alpha}^2{\omega}^4+2\alpha \left[\eta {k}^2+\alpha \upsilon {k}^2\right]{\omega}^3+\left\{{\left[\alpha \upsilon {k}^2+\eta {k}^2\right]}^2+2\alpha \left[{V}^2{k}^2+2\alpha {\upsilon}_0^2{k}^4\left.+\eta {k}^2\upsilon {k}^2\right]\right.\right\}{\omega}^2+2\left\{\left[\alpha \upsilon {k}^2+\eta {k}^2\right]\right.\hfill \\ {}\times \left.\left[{V}^2{k}^2+\eta {k}^2\upsilon {k}^2\right]+4\alpha \eta {k}^2{\upsilon}_0^2{k}^4\right\}\omega +\left\{{\left[{V}^2{k}^2+\eta {k}^2\upsilon {k}^2\right]}^2\right.\left.+4{\upsilon}_0^2{k}^4\left({\eta}^2{k}^4\right)\right\}=0.\hfill \end{array} $$
(28)

The above equation shows the dispersion relation for finitely conducting viscous plasma including the effects of finite electron inertia, FLR corrections, and magnetic field. It is independent of radiative heat-loss functions and thermal conductivity. Hence, the above dispersion relation represents the wave propagation. Equation (28) is a modified form of Vaghela and Chhajlani [30] by inclusion of finite electron inertia in our case.

In the absence of viscosity, finite resistivity, and FLR corrections (υ = η = υ 0 = 0), Eq. (28) becomes

$$ {\alpha}^2{\omega}^4+2\kern0.1em \alpha \kern0.2em {V}^2{k}^2{\omega}^2+{V}^4{k}^4=0. $$
(29)

The roots of the above equation are

$$ {\omega}_{1,2}^2=-\frac{V^2{k}^2}{\alpha }. $$
(30)

Equation (30) shows the Alfven mode modified by finite electron inertia, and in this mode, there is no instability. The above relation shows the modified form of Alfven mode by inclusion of finite electron inertia. Thus, finite electron inertia modifies the mode by changing the growth rate.

In the absence of viscosity and finite resistivity (υ = η = 0), Eq. (28) becomes

$$ {\alpha}^2{\omega}^4+\left(2\kern0.1em \alpha \kern0.2em {V}^2{k}^2+4\kern0.1em {\alpha}^2{\upsilon}_0^2{k}^4\right){\omega}^2+{V}^4{k}^4=0. $$
(31)

The roots of the above equation are

$$ {\omega}_{1,2}^2=\frac{-\left({V}^2{k}^2+2\kern0.1em \alpha \kern0.2em {\upsilon}_0^2{k}^4\right)\pm 2{\upsilon}_0{k}^2{\left(\alpha \kern0.3em {V}^2{k}^2+{\alpha}^2{\upsilon}_0^2{k}^4\right)}^{{\scriptscriptstyle \raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}}}{\alpha }. $$
(32)

The above relation shows the modified form of Alfven mode by inclusion of FLR corrections and finite electron inertia. Thus, FLR corrections and finite electron inertia modify the mode by changing the growth rate. Equation (31) is the modified form of Vaghela and Chhajlani [30] by inclusion of finite electron inertia in our case.

The third component of the dispersion relation (26) on simplifying gives

$$ \begin{array}{l}{\omega}^3+\kern0.3em \left[\upsilon {k}^2+\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right]{\omega}^2+\left[\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\kern0.3em \upsilon {k}^2\right.\left.+{c}^2{k}^2\right]\omega \hfill \\ {}+\left[{k}^2\left(\gamma -1\right)\left(T{L}_T-\rho {L}_{\rho }+\frac{\lambda {k}^2T}{\rho}\right)\right]=0.\hfill \end{array} $$
(33)

The above equation represents the combined influence of thermal conductivity, radiative heat-loss function, and viscosity on the thermal instability of plasma, but there is no effect of finite electron inertia, finite electrical conductivity, FLR corrections, and magnetic field strength on the thermal instability of the considered system. When the constant term of cubic Eq. (33) is less than zero, this allows at least one positive real root which corresponds to the instability of the system. The condition of instability obtained from the constant term of Eq. (33) is given as

$$ {k}^{2_2}\left(\gamma -1\right)\left(T{L}_T-\rho {L}_{\rho }+\frac{\lambda {k}^2T}{\rho}\right)<0. $$
(34)

The above condition of instability is independent of FLR corrections, finite electron inertia, finite electrical conductivity, magnetic field strength, and viscosity. The above inequality (34) is the reduced form of Bora and Talwar [8]. In the present case, we have considered the effects of FLR correction and viscosity, but Bora and Talwar [8] have not considered these effects. Thus, the dispersion relation in the present analysis is modified due to the presence of FLR correction and viscosity, but the condition of instability is unaffected by the presence of FLR correction and viscosity. Thus, we conclude that the FLR correction and viscosity of the medium have no effect on the condition of instability. Also, it is clear that the growth rate of dispersion relation given by Bora and Talwar [8] is getting modified due to the presence of FLR corrections and viscosity in our present case. Thus, we conclude that FLR corrections and viscosity modify the growth rate of instability in the present case. Hence, these are the new findings in our case than that of Bora and Talwar [8]. Equation (33) can be written in the form, according to Field [1]:

$$ {\omega}^3+c\left(\frac{\kern0.1em \upsilon {k}^2}{c}+{k}_T+\frac{k^2}{k_{\lambda }}\right){\omega}^2+c\left[\left.\frac{\kern0.1em \upsilon {k}^2}{c}\left({k}_T+\frac{k^2}{k_{\lambda }}\right)+{k}^2\right]\omega \right.+\frac{k^2{c}^3}{\gamma}\left({k}_T-{k}_{\rho }+\frac{k^2}{k_{\lambda }}\right)=0. $$
(35)

where we have used

$$ {k}_T=\frac{\left(\gamma -1\right){L}_T}{Rc},\kern0.5em {k}_{\lambda }=\frac{Rc\rho }{\left(\gamma -1\right)\lambda },\kern0.5em \mathrm{and}\kern0.7em {k}_{\rho }=\frac{\left(\gamma -1\right)\rho {L}_{\rho }}{RcT}. $$
(36)

To study the effects of viscosity and radiative heat-loss functions on the growth rate of thermal instability, we solve Eq. (33) numerically. Eq. (33) can be written in non-dimensional form with the help of the following dimensionless quantities

$$ {\omega}^{\ast }=\frac{\omega }{k_{\rho }c},{\upsilon}^{\ast }=\frac{\upsilon {k}_{\rho }}{c},{k}^{\ast }=\frac{k}{k_{\rho }},{k}_{\lambda}^{\ast }=\frac{k_{\rho }}{k_{\lambda }},{k}_T^{\ast }=\frac{k_T}{k_{\rho }}. $$
(37)

Using Eq. (37), we write Eq. (33) in non-dimensional form as

$$ {\omega}^{*3}+\left[{\upsilon}^{*}{k}^{*2}+{k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}\;\right]{\omega}^{*2}+\left[{\upsilon}^{*}{k}^{*2}\left({k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}\right)+{k}^{*2}\right]{\omega}^{*}+\frac{k^{*2}}{\gamma}\left({k}_T^{*}-1+{k}_{\lambda}^{*}{k}^{*2}\right)=0. $$
(38)

The parameters are taken as non-dimensional. Numerical calculations were performed to determine the roots of ω * from dispersion relation (38), as a function of wave number k * for several values of the different parameters involved, taking γ = 5/3. Out of the three modes, only one mode is unstable, for which the calculations are presented in Figs. 1, 2, and 3, where the growth rate ω * (positive real value of ω) has been plotted against the wave number k * to show the dependence of the growth rate on the different physical parameters such as heat-loss function and viscosity ν *.

Fig. 1
figure 1

The normalized growth rate (ω *) as a function of normalized wave number (k *), for different values of \( {k}_{\lambda}^{*} \), having \( {k}_T^{*} \) = 0.5 and v * = 1.

Full size image
Fig. 2
figure 2

The normalized growth rate (ω *) as a function of normalized wave number (k *), for different values of \( {k}_T^{*} \), having \( {k}_{\lambda}^{*} \) = 0.1 and v * = 1.

Full size image
Fig. 3
figure 3

The normalized growth rate (ω *) as a function of normalized wave number (k *), for different values of v *, having \( {k}_T^{*} \) = 0.5 and \( {k}_{\lambda}^{*}=0.1. \)

Full size image

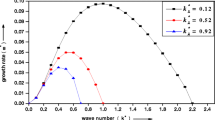
Figure 1 shows the effect of \( {k}_{\lambda}^{*} \) on the growth rate of thermal instability for fixed values of other parameters. From the figure, it is clear that as the value of \( {k}_{\lambda}^{*} \) increases both the peak value and the growth rate of thermal instability decrease. Thus, the parameter \( {k}_{\lambda}^{*} \) moves the present system toward the stabilization. In Fig. 2, we have plotted the growth rate of thermal instability against the wave number for different values of the parameter \( {k}_T^{*} \). From the figure, we conclude that as the value of \( {k}_T^{*} \) increases, the peak value of curves decreases and the area of the growth rate also decreases. Hence, the presence of \( {k}_T^{*} \) also stabilizes the system. In Fig. 3, we have shown the effect of viscosity on the growth rate of thermal instability. The figure displays that on increasing the value of viscosity the growth rate of thermal instability also decreases. Therefore, the parameters \( {k}_{\lambda}^{*} \), \( {k}_T^{*} \), and ν * viscosity stabilize the system.

If the constant term of cubic Eq. (33) is greater than zero, then all the coefficients of the equation (33) must be positive. Equation (33) is a third degree in the power of ω having its coefficients positive, which is a necessary condition for the stability of the system. To achieve the sufficient condition, the principal diagonal minors of the Hurwitz matrix must be positive. The principal diagonal minors are

$$ \begin{array}{l}{\varDelta}_1=\left[\upsilon {k}^2+\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right]>0,\hfill \\ {}{\varDelta}_2=\upsilon {k}^2\left[{\varDelta}_1\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)+{c}^2{k}^2\right]+\left(\gamma -1\right){k}^2\rho {L}_{\rho }>0,\hfill \\ {}{\varDelta}_3={\varDelta}_2\left[{k}^2\left(\gamma -1\right)\left(T{L}_T-\rho {L}_{\rho }+\frac{\lambda {k}^2T}{\rho}\right)\right]>0.\hfill \end{array} $$
(39)

Since \( {\varOmega}_j^2>0,\kern0.3em {\varOmega}_I^2>0 \) and γ > 1, it is clear that all the Δs are positive, hence the system represented by Eq. (33) is a stable system.

In the absence of thermal conductivity (  λ = 0), dispersion relation (33) gives

$$ {\omega}^3+\left[\upsilon {k}^2+\frac{\gamma {L}_T}{c_p}\right]{\omega}^2+\left[\kern0.1em \upsilon {k}^2\frac{\gamma {L}_T}{c_p}+{c}^2{k}^2\right]\omega +\frac{\gamma {L}_T}{c_p}\left[{k}^2\left({c^{\prime}}^2-\frac{p{L}_{\rho }}{T{L}_T}\right)\right]=0. $$
(40)

The condition of instability obtained from the constant term of the above equation is given as

$$ \left[{k}^2\left(c{\prime}^{{}_2}-\frac{p{L}_{\rho }}{T{L}_T}\right)\right]<0, $$
(41)

Thus, we conclude that for longitudinal wave propagation as given by Eq. (33), the system is unstable only for thermal criterion of instability; otherwise, it is stable. Also, for longitudinal wave propagation, the thermal criterion remains unaffected by FLR corrections, viscosity, magnetic field, and finite electrical resistivity, but thermal conductivity and radiative heat-loss function modify the fundamental expression and the fundamental thermal instability criterion becomes radiative instability criterion.

5.2 Transverse Wave Propagation (k x  = k,   k z  = 0)

In this case, the perturbations are taken to be perpendicular to the direction of the magnetic field (i.e.,  k x  = k ,  k z  = 0). The dispersion relation (25) reduces to

$$ {\left[\omega +\upsilon {k}^2\right]}^2\left.\left\{\left[\omega +\upsilon {k}^2\right]\times \left[{\omega}^2+\omega \kern0.3em \upsilon {k}^2+{\varOmega}_T^2+\frac{\omega {\mathrm{V}}^2{k}^2}{d}\right]\right.+\omega {\upsilon}_0^2{k}^4\right\}=0. $$
(42)

The first component of the dispersion relation (42) gives

$$ \omega +\upsilon {k}^2=0. $$
(43)

This represents a stable viscous mode, and is discussed in Eq. (26).

The second component of the dispersion relation (42) on simplifying gives

$$ \begin{array}{l}{\omega}^5\alpha +\left\{\alpha \left[2\upsilon {k}^2+\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right]+\eta {k}^2\right\}{\omega}^4+\left\{\alpha \left[2\upsilon {k}^2\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right.\right.+{\upsilon}^2{k}^4\hfill \\ {}\left.+{\upsilon}_0^2{k}^4+{c}^2{k}^2\right]+\eta {k}^2\left.\left.\left[\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right.+2\upsilon {k}^2\right]+{V}^2{k}^2\right\}{\omega}^3+\left\{\alpha \left[\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\upsilon {k}^2+\left(\gamma -1\right)\right.\right.\hfill \\ {}\times \left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right){\upsilon}_0^2{k}^4+\upsilon {k}^2\left({c}^2{k}^2\right)+{k}^2\left(\gamma -1\right)\left.\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right]+\upsilon {k}^2\left[2\eta {k}^2\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)+\eta {k}^2\upsilon {k}^2\right.\hfill \\ {}\left.+{V}^2{k}^2\right]+\eta {k}^2\left[{\upsilon}_0^2{k}^4\right.\left.\left.+{c}^2{k}^2\right]+\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)+{V}^2{k}^2\right\}{\omega}^2+\left\{\upsilon {k}^2\left[\eta {k}^2\upsilon {k}^2\right.\right.\hfill \\ {}\times \left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)+{V}^2{k}^2\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)+\eta {k}^2\left({c}^2{k}^2\right)+\alpha \left[{k}^2\left(\gamma -1\right)\left.\left.\left(T{L}_T-\rho {L}_{\rho }+\frac{\lambda {k}^2T}{\rho}\right)\right]\right]\right.\hfill \\ {}+\eta {k}^2{\upsilon}_0^2{k}^4\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\left.+\eta {k}^2\left[{k}^2\left(\gamma -1\right)\left(T{L}_T-\rho {L}_{\rho }+\frac{\lambda {k}^2T}{\rho}\right)\right]\right\}\omega +\eta {k}^2\upsilon {k}^2\hfill \\ {}\times \left[{k}^2\left(\gamma -1\right)\left(T{L}_T-\rho {L}_{\rho }+\frac{\lambda {k}^2T}{\rho}\right)\right]=0.\hfill \end{array} $$
(44)

The above equation represents the combined influence of radiative heat-loss function, FLR corrections, finite electron inertia, finite electrical conductivity, thermal conductivity, viscosity, and magnetic field on thermal instability of the plasma. If we neglect the effect of FLR corrections, Eq. (44) is identical to Prajapati et al. [14] for the non-rotational and non-gravitational cases. In the present case, we have considered the effects of FLR corrections, but Prajapati et al. [14] have not considered this effect. Thus, the dispersion relation in the present analysis is modified due to the presence of FLR corrections, but the condition of instability is unaffected by the presence of FLR corrections. Thus, we conclude that FLR corrections have no effect on the condition of radiative instability, but the growth rate of the dispersion relation given by Prajapati et al. [14] gets modified due to the presence of FLR corrections in our present case. Thus, we conclude that FLR corrections modify the growth rate of radiative instability in the present case. Hence, this is the new finding in our case, compared to that of Prajapati et al. [14].

When the constant term of Eq. (44) is less than zero, this allows at least one positive real root which corresponds to the instability of the system. The condition of instability obtained from constant term of Eq. (44) is given as

$$ \left[{k}^2\left(\gamma -1\right)\left(T{L}_T-\rho {L}_{\rho }+\frac{\lambda {k}^2T}{\rho}\right)\right]<0. $$
(45)

The above inequality (45) is the reduced form of Bora and Talwar [8]. From Eq. (45), we see that if a heat-loss function decreases with density, thermal instability does not arise, but when the heat-loss function increases with density (L ρ  > 0), thermal instability occurs if λ < (ρ 2 L ρ  − ρTL T )/(k 2 T), and for purely density-dependent heat-loss function, thermal instability occurs if λ < (ρ 2 L ρ )/(k 2 T).

Thus, to discuss the effect of each parameter (viz., heat-loss function, viscosity, and FLR corrections) on the growth rate of unstable modes, we solve Eq. (44) numerically by introducing the following dimensionless quantities

$$ {\omega}^{\ast }=\frac{\omega }{k_{\rho }c},{\upsilon}^{\ast }=\frac{\upsilon {k}_{\rho }}{c},{k}^{\ast }=\frac{k}{k_{\rho }},{k}_{\lambda}^{\ast }=\frac{k_{\rho }}{k_{\lambda }},{k}_T^{\ast }=\frac{k_T}{k_{\rho }},{\upsilon}_0^{\ast }=\frac{\upsilon_0{k}_{\rho }}{c}. $$
(46)

Using Eq. (46), we write Eq. (44) in a non-dimensional form as

$$ \begin{array}{l}{\omega}^{*5}\alpha +\left\{\alpha \left[2{\upsilon}^{*}{k}^{*2}+{k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}\right]+{\eta}^{*}{k}^{*2}\right\}{\omega}^{*4}+\left\{\alpha \left[2{\upsilon}^{*}{k}^{*2}\left({k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}\right)+{\upsilon}^{*2}{k}^{*4}+{\upsilon}_0^{*2}{k}^{*4}+{k}^{*2}\right]\right.+{\eta}^{*}{k}^{*2}\hfill \\ {}\times \left[{k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}+2{\upsilon}^{*}{k}^{*2}\right]\left.+{V}^{*2}{k}^{*2}\right\}{\omega}^{*3}+\left\{\alpha \left[\left({k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}\right){\upsilon}^{*}{k}^{*2}+\left({k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}\right){\upsilon}_0^{*2}{k}^{*4}+{\upsilon}^{*}{k}^{*4}+\left({k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}\right)\right]\right.+{\upsilon}^{*}{k}^{*2}\hfill \\ {}\times \left[2{\eta}^{*}{k}^{*2}\left({k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}\right)+{\eta}^{*}{k}^{*2}{\upsilon}^{*}{k}^{*2}+{V}^{*2}{k}^{*2}\right]+{\eta}^{*}{k}^{*2}\left[{\upsilon}_0^{*2}{k}^{*4}\right.\left.\left.+{k}^{*2}\right]+\left({k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}\right)+{V}^{*2}{k}^{*2}\right\}{\omega}^{*2}+\left\{{\upsilon}^{*}{k}^{*2}\left[{\eta}^{*}{k}^{*2}{\upsilon}^{*}{k}^{*2}\right.\right.\hfill \\ {}\times \left({k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}\right)+{V}^{*2}{k}^{*2}\left({k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}\right)+{\eta}^{*}{k}^{*4}+\alpha \left.\left[\frac{k^{*2}}{\gamma}\left({k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}-1\right)\right]\right]+{\eta}^{*}{k}^{*2}{\upsilon}_0^{*2}{k}^{*4}\left({k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}\right)+{\eta}^{*}{k}^{*2}\hfill \\ {}\left.\times \left[\frac{k^{*2}}{\gamma}\left({k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}-1\right)\right]\right\}{\omega}^{*}+{\eta}^{*}{k}^{*2}{\upsilon}^{*}{k}^{*2}\left[\frac{k^{*2}}{\gamma}\left({k}_T^{*}+{k}_{\lambda}^{*}{k}^{*2}-1\right)\right]=0.\hfill \end{array} $$
(47)

In Figs. 4, 5, 6, 7, 8, and 9, the dimensionless growth rate (ω *) has been plotted against the dimensionless wave number (k *) to see the effect of various physical parameters such as viscosity, radiative heat-loss function, resistivity, and FLR corrections. From Fig. 4, we see that as the value of \( {k}_{\lambda}^{*} \) increases, the growth rate decreases. Thus, the effect of parameter \( {k}_{\lambda}^{*} \) is stabilizing. It is clear from Fig. 5 that the growth rate decreases with increasing parameter \( {k}_T^{*} \). Thus, the presence of \( {k}_T^{*} \) stabilizes the growth rate of the system. From Fig. 6, we conclude that the growth rate decreases with increasing the value of viscosity. Thus, the effect of viscosity is stabilizing.

Fig. 4
figure 4

The normalized growth rate (ω *) as a function of normalized wave number (k *), for different values of \( {k}_{\lambda}^{*} \), having \( \alpha =1,\ {k}_T^{*} \) = 0.5 and \( {v}^{*}={v}_0^{*}={\eta}^{*}=1 \)

Full size image
Fig. 5
figure 5

The normalized growth rate (ω *) as a function of normalized wave number (k *), for different values of \( {k}_T^{*} \), having \( \alpha =1,\ {k}_{\lambda}^{*} \) = 0.5 and \( {v}^{*}={v}_0^{*}={\eta}^{*}=1 \)

Full size image
Fig. 6
figure 6

The normalized growth rate (ω *) as a function of normalized wave number (k *), for different values of v *, having \( \alpha =1,\ {k}_T^{*} \) = 0.5 and \( {k}_{\lambda}^{*}=0.1,{v}_0^{*}={\eta}^{*}=1 \)

Full size image
Fig. 7
figure 7

The normalized growth rate (ω *) as a function of normalized wave number (k *), for different values of \( {v}_0^{*} \), having \( \alpha =1,\ {k}_{\lambda}^{*} \) = 0.1, \( {k}_T^{*} \) = 0.5, and v * = η * = 1

Full size image
Fig. 8
figure 8

The normalized growth rate (ω *) as a function of normalized wave number (k *) for different values of α with \( {k}_T^{*} \) = 0.5, \( {k}_{\lambda}^{*}=0.1 \) and \( {v}^{*}={v}_0^{*}={\eta}^{*}=1 \)

Full size image
Fig. 9
figure 9

The normalized growth rate (ω *) as a function of normalized wave number (k *), for different values of η *, having \( \alpha =1,\ {k}_{\lambda}^{*} \) = 0.1, \( {k}_T^{*} \) = 0.5, and \( {v}^{*}={v}_0^{*}=1 \)

Full size image

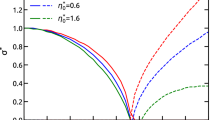
Figure 7 displays the influence of FLR corrections on the growth rate of thermal instability. From the figure, it is clear that the FLR corrections have a stabilizing effect on the growth rate of thermal instability. Figure 8 shows the effect of finite electron inertia α on the growth rate of thermal instability. From the curves, it is clear that the growth rate of thermal instability increases as the value of finite electron inertia increases. Hence, the finite electron inertia α has a destabilizing influence on the system. Figure 9 displays the influence of resistivity on the growth rate of thermal instability. From the figure, it is clear that the resistivity has a destabilizing effect on the growth rate of thermal instability. Therefore, the parameters radiative heat-loss functions, viscosity, and FLR corrections have a stabilizing influence on the system while the finite electron inertia and resistivity have a destabilizing influence on the growth rate of the system.

Now we wish to examine the effect of finite electron inertia, FLR corrections, and radiative heat-loss functions on the considered system with some simplifications, and at the same time we wish to investigate the physics involved in such simplifications in the present problem.

In the absence of thermal conductivity (λ = 0), Eq. (44) reduces to

$$ \begin{array}{l}\alpha {\omega}^5+\left\{\alpha \left[2\upsilon {k}^2+\frac{\gamma {L}_T}{c_p}\right]+\eta {k}^2\right\}{\omega}^4+\left.\left\{\alpha \left[2\upsilon {k}^2\frac{\gamma {L}_T}{c_p}+{\upsilon}^2{k}^4\right.\right.+{\upsilon}_0^2{k}^4+{c}^2{k}^2\right]+\left(\eta {k}^2\left[2\upsilon {k}^2+\frac{\gamma {L}_T}{c_p}\right]+{V}^2{k}^2\right\}{\omega}^3\hfill \\ {}+\left\{\alpha \left[\frac{\gamma {L}_T}{c_p}\left({\upsilon}^2{k}^4+{\upsilon}_0^2{k}^4\right)\right.\right.+\upsilon {k}^2\left({c}^2{k}^2\right)+\frac{\gamma {L}_T}{c_p}\left[{k}^2\left({c^{\prime}}^2-\frac{p{L}_{\rho }}{T{L}_T}\right)\right]+\upsilon {k}^2\left.\left[2\eta {k}^2\frac{\gamma {L}_T}{c_p}+\eta {k}^2\upsilon {k}^2\right.+{V}^2{k}^2\right]\hfill \\ {}+\eta {k}^2\left.\left[{\upsilon}_0^2{k}^4+{c}^2{k}^2\right]+\left(\frac{\gamma {L}_T}{c_p}\right){V}^2{k}^2\right\}{\omega}^2+\left\{\upsilon {k}^2\left[\eta {k}^2\upsilon {k}^2\frac{\gamma {L}_T}{c_p}\right.\right.+{V}^2{k}^2\frac{\gamma {L}_T}{c_p}+\eta {k}^2\left({c}^2{k}^2\right)+\alpha \frac{\gamma {L}_T}{c_p}\hfill \\ {}\left.\left.\times \left[{k}^2\left({c^{\prime}}^2-\frac{p{L}_{\rho }}{T{L}_T}\right)\right]\right]+\eta {k}^2\left(\frac{\gamma {L}_T}{c_p}\right)\kern0.2em \left[{\upsilon}_0^2{k}^4+{c^{\prime}}^2{k}^2-\frac{p{L}_{\rho }{k}^2}{T{L}_T}\right]\right\}\omega +\eta {k}^2\upsilon {k}^2\frac{\gamma {L}_T}{c_p}\left[{k}^2\left({c^{\prime}}^2-\frac{p{L}_{\rho }}{T{L}_T}\right)\right]=0.\hfill \end{array} $$
(48)

The condition of instability obtained from the constant term of Eq. (48) is given as

$$ \left[{k}^2\left({c^{\prime}}^2-\frac{p{L}_{\rho }}{T{L}_T}\right)\right]<0, $$
(49)

It is already discussed in Eq. (41). On comparing Eqs. (40) and (48), we see that no new mode comes due to inclusion of thermal conductivity, but the condition of instability and growth rate of instability both get modified by inclusion of thermal conductivity. Also, on comparing Eq. (48) with Eq. (29) of Aggarwal and Talwar [4], we conclude that the growth rate of radiative instability gets modified by the inclusion of finite electron inertia and FLR corrections in our case, but the condition of instability is independent of finite electron inertia and FLR corrections.

For infinitely conducting medium (η = 0), Eq. (44) becomes

$$ \begin{array}{l}\alpha {\omega}^4+\left\{\alpha \left[2\upsilon {k}^2+\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right]\right\}{\omega}^3+\left\{\alpha \left[2\upsilon {k}^2\right.\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right.+{\upsilon}^2{k}^4\hfill \\ {}\left.\left.+{\upsilon}_0^2{k}^4+{c}^2{k}^2\right]+{V}^2{k}^2\right\}{\omega}^2+\left\{\alpha \left[\upsilon {k}^2\right.\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right.+{\upsilon}_0^2{k}^4\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\hfill \\ {}\left.+\upsilon {k}^2\left({c}^2{k}^2\right)+\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{\rho}\right)\right]\left.+\upsilon {k}^2{V}^2{k}^2+\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)+{V}^2{k}^2\right\}\omega \hfill \\ {}+\upsilon {k}^2\left\{\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right.\left.\left.{V}^2{k}^2+\alpha \left[{k}^2\left(\gamma -1\right)\left(T{L}_T-\rho {L}_{\rho }+\frac{\lambda {k}^2T}{\rho}\right)\right.\right]\right\}=0.\hfill \end{array} $$
(50)

The condition of instability obtained from the constant term of Eq. (50) is given as

$$ \left\{\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right){V}^2{k}^2+\alpha \left[{k}^2\left(T{L}_T-\rho {L}_{\rho }+\frac{\lambda {k}^2T}{\rho}\right)\right.\right\}<0. $$
(51)

This relation is the reduced form of Bora and Talwar [8]. From Eq. (51), we see that magnetic field tries to stabilize the system. On comparing Eqs. (44) and (50), we see that one mode is increased due to inclusion of finite resistivity. Also, on comparing Eqs. (45) and (53), we conclude that inclusion of finite resistivity removes the effect of magnetic field and finite electron inertia from condition of instability and tries to destabilize the system. Also, on comparing Eq. (50) with Eq. (29) of Aggarwal and Talwar [4], we conclude that the condition of instability gets modified by inclusion of finite electron inertia, and the growth rate of radiative instability gets modified by inclusion of finite electron inertia and FLR corrections in our case. Hence, these are the new results in our case, compared to those of Aggarwal and Talwar [4]. In the case of purely temperature-dependent heat-loss function (L ρ  = 0), increasing with temperature (L T  > 0), thermal instability does not occur for transverse wave propagation; if the heat-loss function decreases with an increase in temperature (L T  < 0), thermal instability arises with λ < (|L T |ρ)/(k 2). Again, for a purely density-dependent heat-loss function (L T  = 0), thermal instability arises for λ < (L ρ )/(Tk 4)[1 + (ρV 2)/(pα)] in the case when heat-loss function increases with density (L ρ  > 0). In that case, thermal instability is modified due to the presence of magnetic field strength.

In the absence of viscosity (υ = 0), Eq. (44) becomes

$$ \begin{array}{l}\alpha {\omega}^4+\left[\eta {k}^2+\alpha \left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right]{\omega}^3+\left.\left[\alpha \left({\upsilon}_0^2{k}^4+{c}^2{k}^2\right)+\eta {k}^2\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right.+{V}^2{k}^2\right]{\omega}^2\hfill \\ {}+\left\{\alpha \left[{\upsilon}_0^2{k}^4\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)+{k}^2\left(\gamma -1\right)\left(T{L}_T-\rho {L}_{\rho }+\frac{\lambda {k}^2T}{\rho}\right)\right.\right.+\eta {k}^2\left[{\upsilon}_0^2{k}^4+{c}^2{k}^2\right]+\left(\gamma -1\right)\hfill \\ {}\left.\times \left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right){V}^2{k}^2\right\}\omega +\eta {k}^2\left[\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right.{\upsilon}_0^2{k}^4\left.+{k}^2\left(\gamma -1\right)\left(T{L}_T-\rho {L}_{\rho }+\frac{\lambda {k}^2T}{\rho}\right)\right]=0.\hfill \end{array} $$
(52)

The condition of instability obtained from the constant term of Eq. (52) is given as

$$ \left.\left[\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right){\upsilon}_0^2{k}^4\right.+{k}^2\left(T{L}_T-\rho {L}_{\rho }+\frac{\lambda {k}^2T}{\rho}\right)\right]<0. $$
(53)

From Eq. (53), we see that FLR corrections try to stabilize the radiative instability. This is the reduced form of Bora and Talwar [8]. On comparing Eqs. (44) and (52), we see that one mode is increased due to inclusion of viscosity; also, the inclusion of viscosity removes the effect of FLR corrections from the condition of instability.. On comparing Eqs. (45) and (53), we conclude that the condition of instability given by Bora and Talwar [8] gets modified by inclusion of FLR corrections, thus the present results are the improvement of Bora and Talwar [8]. In the case of purely temperature-dependent heat-loss function (L ρ  = 0), increasing with temperature (L T  > 0), thermal instability does not occur for transverse wave propagation; if the heat-loss function decreases with an increase in temperature (L T  < 0), thermal instability arises with λ < (|L T |ρ)/(k 2). Again, for a purely density-dependent heat-loss function (L T  = 0), thermal instability arises for \( \lambda <\left({L}_{\rho}\right)/\left(T{k}^6\right)\left[1+\left(\rho {\upsilon}_0^2\right)/(p)\right] \) in the case when the heat-loss function increases with density (L ρ  > 0). In that case, thermal instability is modified due to the presence of the magnetic field strength..

For an inviscid perfectly conducting medium (υ = η = 0), Eq. (44) becomes

$$ \begin{array}{l}\alpha {\omega}^3+\left[\alpha \left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right]{\omega}^2+\left[\alpha \left({\upsilon}_0^2{k}^4+{c}^2{k}^2\right)+{V}^2{k}^2\right]\omega +\left\{\alpha \left[{\upsilon}_0^2{k}^4\left(\gamma -1\right)\left(\frac{T\rho {L}_T}{p}\right.\right.\right.\left.+\frac{\lambda {k}^2T}{p}\right)\hfill \\ {}+{k}^2\left(\gamma -1\right)\left.\left(T{L}_T-\rho {L}_{\rho }+\frac{\lambda {k}^2T}{\rho}\right)\right]+{V}^2{k}^2\left(\gamma -1\right)\left.\times \left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right\}=0.\hfill \end{array} $$
(54)

The above equation is the reduced form of Bora and Talwar [8] in the absence of FLR corrections. The condition of instability obtained from the constant term of Eq. (54) is given as

$$ \left\{\alpha \left[{\upsilon}_0^2{k}^4\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)+{k}^2\left(T{L}_T-\rho {L}_{\rho }+\frac{\lambda {k}^2T}{\rho}\right)\right]\right.\left.+{V}^2{k}^2\left(\frac{T\rho {L}_T}{p}+\frac{\lambda {k}^2T}{p}\right)\right\}<0. $$
(55)

From Eq. (55), we see that FLR corrections and magnetic field try to stabilize the radiative instability. This is the reduced form of Bora and Talwar [8]. On comparing Eqs. (44) and (55), we conclude that the condition of radiative instability and the growth rate given by Bora and Talwar [8] are modified by inclusion of FLR corrections, thus the present results are the improvement of Bora and Talwar [8]. In the case of purely temperature-dependent heat-loss function (L ρ  = 0), increasing with temperature (L T  > 0), thermal instability does not occur for transverse wave propagation; if the heat-loss function decreases with an increase in temperature (L T  < 0), thermal instability arises with λ < (|L T |ρ)/(k 2). Again, for a purely density-dependent heat-loss function (L T  = 0), thermal instability arises for \( \lambda <\left(\rho p{L}_{\rho}\right)/\left({k}^2T\right)\left[\alpha {\upsilon}_0^2{k}^2+{V}^2+\left(p/\rho \right)\right] \) in the case when the heat-loss function increases with density (L ρ  > 0). In that case, thermal instability is modified due to the presence of magnetic field strength, finite electron inertia, and FLR corrections.

In the absence of viscosity, finite resistivity, thermal conductivity, and radiative heat-loss function (υ = η = λ = L T , ρ  = 0), Eq. (44) becomes

$$ \left(1+\frac{c^2{k}^2}{\omega_{pe}^2}\right){\omega}^2+\left[\left(1+\frac{c^2{k}^2}{\omega_{pe}^2}\right)\kern0.3em \left({\upsilon}_0^2{k}^4+{c}^2{k}^2\right)+{V}^2{k}^2\right]=0. $$
(56)

The above Eq. (56) is the modified form of Uberoi [22] by inclusion of FLR corrections in our problem. The condition of instability obtained from Eq. (56) is given as

$$ \left[\left(1+\frac{c^2{k}^2}{\omega_{pe}^2}\right)\kern0.3em \left({\upsilon}_0^2{k}^4+{c}^2{k}^2\right)+{V}^2{k}^2\right]<0. $$
(57)

From Eq. (57), we see that magnetic field and FLR corrections stabilize the system. On comparing Eqs. (44) and (56), we see that the dispersion relation given by Uberoi [22] is modified by inclusion of FLR corrections, radiative heat-loss function, thermal conductivity, viscosity, and finite electrical resistivity in our case. Hence, we improve the result of Uberoi [22].

Thus, we conclude that for transverse wave propagation, the thermal criterion is affected by finite electron inertia, FLR corrections, radiative heat-loss functions, viscosity, magnetic field strength, thermal conductivity, and finite electrical resistivity. From the curves, we find that FLR corrections, viscosity, and heat-loss function have a stabilizing influence on the growth rate of thermal instability, whereas finite electron inertia and finite electrical resistivity have a destabilizing influence on the thermal instability of plasma.

6 Conclusion

The thermal instability of an infinite homogeneous viscous thermally and electrically conducting, radiating fluid including FLR corrections and finite electron inertia has been investigated. For simplicity, the longitudinal and transverse wave propagation to the direction of external magnetic field has been considered. It is found that the thermal criterion remains valid and gets modified because of radiative heat-loss function and thermal conductivity. For longitudinal wave propagation, finite electron inertia, FLR correction, viscosity, magnetic field strength, and finite resistivity have no effect on thermal criterion. But thermal and radiative effects independently as well as jointly modify the thermal criterion. Also, FLR corrections and finite electron inertia modify the growth rate of the Alfven mode.

For transverse wave propagation, FLR corrections, magnetic field strength, viscosity, and finite resistivity affect the condition of radiative instability. FLR corrections stabilize the system in the case of the non-viscous medium. Also, magnetic field stabilizes the system but finite conductivity removes the effect of magnetic field, thereby destabilizing the system. Numerical calculation shows the stabilizing effect of heat-loss function, viscosity and FLR corrections, and the destabilizing effect of finite electron inertia and finite electrical resistivity on the thermal instability.