Asymptotic cross section and scaling law: positronium formation in Rydberg states in positron–hydrogen collisions

Abstract

Positronium formation in Rydberg states from the ground state of the hydrogen atom by positron impact has been studied within the framework of a distorted wave theory which includes static dipole polarization potential. The distorted wave scattering amplitude has been obtained in a closed form. A detailed investigation has been made on the differential and total cross sections in the energy range 25–300 eV of incident positron. It has been found that asymptotic cross sections for the positronium formation into different angular momentum states obey a simple law.

1 Introduction

Formation of positronium (Ps) in highly excited states during collisions is of fundamental interest because of its important applications in diverse fields of physics, such as plasma physics [1–3], astrophysics [4–9], material science [10–14], chemical physics [14, 15], high energy physics [16, 17]. Positronium atoms are of particularly suitable to verify the prediction of quantum electrodynamics (QED). The annihilation of positronium has been a bench mark of bound state QED (BSQED) for many years. Positron annihilation are utilized most exclusively in the material science and chemical physics. Positronium has been known for a long time to exist in excited states and many experimental attempts have been made to study the properties of excited states of Ps with principle quantum number $n>1$ [18–21]. Collision cross section data for various excited states are frequently required for plasma diagnostics and astrophysics. Moderately high excited states, such as $8 \le n \le 25$ are of practical importance from the point of view of laboratory production of hot plasmas [3]. Since atoms in ground state and low-lying excited states, such as $1 \le n \le 7,$ are not significantly ionized, moderately excited atoms ($8 \le n \le 25$) being easily ionized are suitable for this purpose. Annihilation radiation observed in the solar flare, in the stellar atmospheres and in the interstellar space shows the formation of positronium atoms in different excited states in astrophysical environments [8, 9]. Furthermore, Rydberg states of positronium atom are used to produce antihydrogen in antimatter experiments [16, 17].

Positronium formation in positron–hydrogen collisions has been studied extensively with various degree of sophistication during the last five decades, both experimentally and theoretically [18–46] (and further references therein). But those studies are mostly concerned with the positronium formation in the ground state. The number of studies on positronium formation in excited states is very few; in particular positronium formation in nlm state is rare [42] and that study used a first-order calculation. It seems that carrying out sophisticated quantum mechanical calculations involving highly excited states becomes very complicated because of the presence of a large number of oscillations in the final bound state wavefunction. Recently, Ghoshal and Mandal [45] have investigated Ps formation in $n^{\prime }s$ state in $e^+ + \mathrm{H(ns)}$ collisions. But, calculation involving arbitrary nlm states is rather difficult than arbitrary ns states, because of the appearance of angular part in the former.

In this paper we focus our attention to investigate the reaction process:

$$\mathrm{e^+ + H(1s)\, \mapsto\, Ps(nlm) + p},$$

(1)

for arbitrary n, l and m. Our endeavour is to make a comprehensive study on the differential cross section and the total cross section.

We have extended the second order distorted wave theory put forward by Ghoshal and Mandal [46] in the momentum space to study the dynamics of the rearrangement process (Eq. (1)). In this theory the distortion potential in a particular channel has been approximated by the average (over internal motions) of perturbation of that particular channel over the bound states [28, 47]. This approximation works well for intermediate and high energies of incident positron. In the present work, we have modified the distorted wave formalism to take into account the effect of dipole polarization of the target atom into the distorted wave theory.

2 Theory and calculations

To describe the process given in Eq. (1), we use the center-of-mass coordinate system (as shown in Fig.1) in which the electron 3, initially bound to the target proton 2 of mass $m_p,$ is finally captured by the incident positron 1. In this coordinate system the non-relativistic Hamiltonian (in a.u.) of the positron–hydrogen system, is given by

$$H = -\frac{1}{2 m_i}\nabla _{R_1}^2 - \frac{1}{2m_H}\nabla _{r_2}^2 + \frac{1}{R_{12}} - \frac{1}{r_1} - \frac{1}{r_{2}},$$

(2)

where $m_i = (m_p+1)/(m_p+2)$ and $m_H = m_p/(m_p+1).$ The Hamiltonian H can be written in terms of the channel Hamiltonians as

$$H = H_i + V_i = H_f + V_f$$

such that

$$H_i\Phi _i = E_i\Phi _i,\quad H_f\Phi _f = E_f\Phi _f ,$$

where $\Phi _i ,\Phi _f$ are unperturbed initial and final states corresponding to the Hamiltonians

$$ H_{i} = - \frac{1}{{2m_{i} }}\nabla _{{R_{1} }}^{2} - \frac{1}{{2m_{H} }}\nabla _{{r_{2} }}^{2} - \frac{1}{{r_{2} }} $$

and

$$ H_{f} = - \frac{1}{{2m_{f} }}\nabla _{{R_{2} }}^{2} - \frac{1}{{2m_{{{\text{Ps}}}} }}\nabla _{{r_{1} }}^{2} - \frac{1}{{r_{1} }} $$

in the incident and final channel, respectively, with $m_f = 2m_p/(m_p+2), m_{\mathrm{Ps}} = 1/2$ and the residual interactions $V_i = (1/R_{12} - 1/r_{1})$ and $V_f= (1/R_{12} - 1/r_{2})$ having energies $E_i$ and $E_f$,

$$E_{\alpha } = \frac{k_{\alpha }^2}{2 m_{\alpha }} + \epsilon _{\alpha }\quad (\alpha = i,f).$$

Here $(\epsilon _i,\epsilon _f),\,(k_i, k_f)$ are respectively, the eigen energies of bound states and positron momenta in the incident and final channels, whereas $(m_i, m_f)$ are the reduced masses for the center of mass motion in the scattering system.

Fig. 1

Coordinate system used to describe positron–hydrogen system

In the present investigation we use the partial wave distorted wave amplitude as obtained by Ghoshal and Mandal [46]:

$$A_{fi}^{(L)} (k_f, k_i) = g^{(L)}_B(k_f ,k_i) + D^{(L)}_{fi}(k_f, k_i)$$

(3)

where $g^{(L)}_B(k_f ,k_i)$ and $D^{(L)}_{fi}(k_f, k_i)$ are obtained as:

$$ g_{B}^{{(L)}} (k_{f} ,k_{i} ) = \sqrt {k_{i} k_{f} } /2~\int\limits_{{ - 1}}^{{ + 1}} ~ [g_{B} (\vec{k}_{f} ,\vec{k}_{i} )]~P_{L} (\cos \theta )~d(\cos \theta ), $$

and

$$ D_{{fi}}^{{(L)}} (k_{f} ,k_{i} ) = \sqrt {k_{i} k_{f} } /2~\int\limits_{{ - 1}}^{{ + 1}} ~ [D(\vec{k}_{f} ,\vec{k}_{i} )]~P_{L} (\cos \theta )~d(\cos \theta ), $$

in which the two-body amplitudes are given by

$$ \begin{aligned} g_{B} (\vec{k}_{f} ,\vec{k}_{i} ) & = \left( { - \frac{{m_{f} }}{{2\pi }}} \right)\left\langle {\Phi _{f} |V_{f} |\Phi _{i} } \right\rangle ; \\ f_{{f\gamma }} (\vec{k}_{f} ,\vec{k}_{i} ) & = \left( { - \frac{{m_{\gamma } }}{{2\pi }}} \right)\left\langle {\Phi _{f} |V_{f} |\Phi _{\gamma }^{{\prime \prime }} } \right\rangle ; \\ \bar{f}_{{\gamma i}} (\vec{k}_{f} ,\vec{k}_{i} ) & = \left( { - \frac{{m_{i} }}{{2\pi }}} \right){\text{ }}\left\langle {\Phi _{\gamma }^{{\prime \prime }} |U_{i} |\Phi _{i} } \right\rangle ; \\ \end{aligned} $$

with the double scattering matrix elements

$$D_{fi}(\vec {k}_f, \vec {k}_i) = {1\over (2 \pi )^3} \sum _{\gamma } \left( - \frac{2\pi }{m_{\gamma }} \right) \int \frac{d \vec {k^{\prime \prime }}}{E - E^{\prime \prime }_{\gamma } + i \epsilon } f_{f\gamma }(\vec {k}_f, \vec {k}^{\prime \prime })\bar{f}_{\gamma i}(\vec {k}^{\prime \prime }, \vec {k}_i). $$

(4)

Here $\gamma$ denotes the intermediate states of hydrogen atom. The distorted-wave amplitude $A_{fi}^{(L)} (k_f, k_i)$ is a function of the scattering energies for any partial wave L. In calculating $g^{(L)}_B$ and $D^{(L)}_{fi}$ we take $\phi _f$ to be zero, where $\phi _f$ is the azimuthal angle of $\vec {k}_f$ about $\vec {k}_i$ as $z-$ axis. In the present context this is no restriction. It corresponds to taking $\phi _p =0$ in (Eq. (17)), where $\phi _p$ denotes the azimuthal angle of $\vec {p}.$

As proposed earlier, we choose the potentials $U_i$ and $U_f$ as:

$$U_i = \langle\phi _i|V_i|\phi _i\rangle \quad \mathrm{and} \quad U_f = \langle\eta _f|V_f|\eta _f\rangle,$$

where $\phi _i$, $\eta _f$ are the bound state wave functions in the initial and final channel respectively and the integration is performed over the bound state coordinates. For $e^+ + \mathrm{H(1s)} \mapsto \mathrm{Ps(nlm)} + \mathrm{p}$ we have

$$ \begin{aligned} U_{i} & = \left\langle {\phi _{i} |V_{i} |\phi _{i} } \right\rangle = \left\langle {\phi _{{1s}} |V_{i} |\phi _{{1s}} } \right\rangle \\ U_{f} & = \left\langle {\eta _{f} |V_{f} |\eta _{f} } \right\rangle = \left\langle {\eta _{{nlm}} |V_{f} |\eta _{{nlm}} } \right\rangle = 0, \\ \end{aligned} $$

where $V_i=(1/R_{12} - 1/r_{1})$, $V_f=(1/R_{12} - 1/r_{2}).$ The unperturbed states in the incident and the final channel are given by

$$\Phi _i(\vec {r}_1,\vec {r}_2) = e^{i\vec {k}_i.\vec {R}_1}\phi _{i}(\vec {r}_2) \quad \mathrm{and} \quad \Phi _f(\vec {r}_1,\vec {r}_2) = e^{-i\vec {k}_f.\vec {R}_2}\eta _{f}(\vec {r}_1).$$

$\phi _i(\vec {r}_2), \eta _f(\vec {r}_{1})$ are the initial and final wave functions of H-atom and Ps-atom respectively, where i corresponds to 1s,  and f corresponds to nlm, $n=2,3, \ldots; l=0,1,2, \ldots ,n-1; m = -l ,\ldots ,+l$. It has been possible to obtain all two-body amplitudes in closed form and thus the distorted wave amplitude (Eq. (3)). Evaluation of the $1s \rightarrow nlm$ capture amplitude $g_{B}(\vec {k}_f, \vec {k}_i)$ is shown in the Appendix.

Here we have included only $\gamma = 1s$ intermediate state of hydrogen to evaluate the double scattering matrix elements (Eq. (4)). This makes a truncation of the contribution from transition to an intermediate excited state followed by super-elastic scattering from that state. Such a truncation does not affect the results too much at intermediate and high energies, because it has been observed from vast amount of collision calculations that the contribution from the ground state (1s) is most essential for the calculation of the scattering amplitude at intermediate and high energies. Thus, inclusion of only one intermediate state reduces the complexity of the problem without affecting the accuracy of the results much. However, effects of higher intermediate states can be compensated by taking into account the effect of the dipole polarization potential explicitly, which we do in the present work. It is well known that the hydrogen atom gets distorted by the slowly moving positron and the dominant long-range interaction is the polarization interaction. In the present work, we have incorporated the effect of the distortion of the target atom by means of the following polarization potential [48]:

$$V_{\mathrm{pol}}(r) = -\frac{\alpha r^2}{2(r^2 +d^2)^3}~~\mathrm{(in\;a.u.)},$$

(5)

where $\alpha =4.5$ (a.u.) is the static dipole polarizability of the hydrogen atom and d is a variable parameter. The polarization potential (Eq. (5)) shows a minima at $r = d/\sqrt{2},$ and is, therefore, most attractive there. The parameter d is, then, approximately of the order of the size of the atom [48]. Inclusion of the polarization potential leads to a modification of two-body amplitudes, $f(\vec {k}_f , \vec {k}_i),$ in the elastic channel by $f(\vec {k}_f , \vec {k}_i) + f_{\mathrm{pol}}(\vec {k}_f , \vec {k}_i),$ where $f_{\mathrm{pol}}(\vec {k}_f , \vec {k}_i)$ is the amplitude due to the polarization potential (Eq. (5)). In other words, the elastic two-body amplitude is replaced by its polarized-Born counterpart. So it is expected that the inclusion of the effect of the dipole polarization potential explicitly will extend the applicability of the distorted wave theory towards further low energies, as well as to improve the accuracy of the results over previously reported results.

3 Results and discussion

In Table 1 we present the partial wave contributions to the Ps(2s) and Ps(2p) formation cross sections for different incident positron energies. This table also includes the results obtained by using some other methods, such as the Glauber eikonal approximation [26], analytical results [29], distorted wave Born approximation [30], 33-state approximation [31], 18-state approximation [32], boundary-corrected continuum intermediate state(BCCIS) approximation [38], convergent close-coupling approach [40] and distorted wave approximation results [44] . From this table we see that our distorted wave results are fairly accurate. Partial wave contributions to the positronium formation in various states are shown in Table 2 for 100 eV of incident positron energy. It is worthy to be mentioned here that for particular values of n and l we sum the contributions of all m degenerate states.

Table 1 Partial wave contributions to the positronium formation cross sections (in units of $\pi a_0^2$) for the processes of $\mathrm{e^+ + H(1s) \rightarrow Ps(nlm) + p}$

Table 2 Partial wave contributions to the positronium formation cross sections (in units of $\pi a_0^2$) for the reaction $\mathrm{e^+ + H(1s) \rightarrow Ps(nlm) + p}$ at 100 eV positron energy

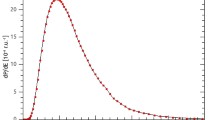
We present the differential cross sections for the positronium formation in some excited states in Fig. 2. From this figure we note that that the differential cross section for Ps(2s) formation exhibits a zero. With increasing incident energy the angular position of the zero slowly moves towards forward direction. The origin of this zero is due to the mutual cancelation of the contributions of the attractive and repulsive part of the interaction potential (i.e. due to the destructive interference between the two). This feature is common for the positronium formation in s states as can be seen from the surface plots in Fig. 3. While for the 2p state the curve falls almost monotonically as the angle increases without showing any structure, because for 2p state, as shown in Fig. 2, the sum of the contributions of all m degenerate states produces a swirl instead of a zero, so the 2p state does not exhibit such a minimum. This feature is also true for $l>1$ states. Total cross sections for the positronium formation in various excited states at various incident energies are shown in Table 3 and Fig. 4. From Table 3 we note that cross section for the Ps formation in 4s state is greater than that of the 3d state. Similarly, cross section for the 5s state is greater than that of the 4f state, and so on.

Fig. 2

Differential cross section (in a.u.) as a function of scattering angle (in degree) for positronium formation in (a) 2s state, (b) 2p state, (c) 3p state and (d) 3d state

Fig. 3

Differential cross section (in a.u.) as a function of incident energy (in eV) and scattering angle (in degree) for (a) $\mathrm{e^{+} + H(1s) \rightarrow Ps(3s) + p}$ and (b) $\mathrm{e^{+} + H(1s) \rightarrow Ps(4s) + p}$

Fig. 4

Cross section (in $\pi a_0^2$) as a function of incident positron energy (in eV) for positronium formation in (a) $n=2$ state and (b) $n=3$ state

Table 3 Ps(nlm) formation cross section $\sigma$ (in units of $\pi a_0^2$)

3.1 Scaling law

It is observed that the Ps formation cross sections for different angular momentum states are scaled according to the law:

$$\sigma _\mathrm{Ps(nl)} = \frac{1}{n^3} \prod _{i=0}^{l} \left( 1 - \frac{i^2}{n^2} \right) (n_0^3 \sigma _\mathrm{Ps(n_0l)} )_{n_0 \rightarrow \infty }.$$

(6)

The origin of such type of scaling law is due to the fact that $[f_{fi}(\vec {k}_f,\vec {k}_i)/(n^{-3} \prod _{i=0}^{l} (1-i^2/n^2))^{1/2}]$ remains more or less unchanged at moderate or high energies, the asymptotic cross section data can be used to obtain a reasonable estimate of the Ps formation cross sections for excited states with increase of the principal quantum number n. The values of the cross section obtained by using distorted-wave approximation and the scaling law (Eq. (6)) are shown in Table 4 at various incident positron energies ranging from 50 to 300 eV. From this table we notice that the results obtained by using scaling law (Eq. (6)) are in good accord with the results obtained by using distorted wave approximation. For higher values of n and E the agreement is quite good. However, at moderate energies the scaling law can still be applied to obtain cross section within limited accuracy. Even when n is not too large (say Eq. (4) or Eq. (5)) the scaling law can still be applied. Note that the scaling law (Eq. (6)) holds good for $(l/n) \ll 1$ so that $\prod _{i=0}^{l} (1-i^2/n^2) \approx 1 .$ It is to be mentioned here that the $1/n^3$ scaling law for the cross section of positronium formation is also suggested earlier [31, 32, 42, 49]. Moreover, the asymptotic cross sections for the positron impact excitation of the hydrogen atom also obey a similar type of scaling law [50]. But in that case the scaling factor is slightly different.

Table 4 Comparison of cross sections (in units of $\pi a_0^2$) calculated by using distorted wave theory and scaling law

4 Conclusions

The dynamics of the reaction: $e^+ + \mathrm{H(1s)} \mapsto \mathrm{Ps(nlm) + p}$ has been investigated by using a distorted wave theory which includes the effect of static dipole polarizability explicitly. It has been possible to obtain the scattering amplitude by some straight-forward steps. We report reasonably accurate results for differential and total cross sections for the incident positron energy in the range 25–300 eV. Moreover, a simple law for obtaining asymptotic cross sections has been presented. We present sufficient analytical and numerical evidences for the sake of further theoretical and experimental works in this field.

Appendix

Evaluation of the $1s \rightarrow nlm$ capture amplitude $g_{B}(\vec {k}_f, \vec {k}_i).$

The wave function of Ps atom in nlm state is given by

$$ \begin{aligned} \eta _{{nlm}} (\vec{r}_{1} ) & = R_{{nl}} (r_{1} )Y_{{lm}} (\theta _{1} ,\phi _{1} ) \\ & = \left\{ { - \left[ {\left( {\frac{2}{{na_{0} }}} \right)^{3} \frac{{(n - l - 1)!}}{{2n[(n + l)!]^{3} }}} \right]^{{1/2}} e^{{ - \frac{{r_{1} }}{{na_{0} }}}} \left( {\frac{2}{{na_{0} }}r_{1} } \right)^{l} L_{{n + l}}^{{2l + 1}} \left( {\frac{2}{{na_{0} }}r_{1} } \right)} \right\}Y_{{lm}} (\theta _{1} ,\phi _{1} ), \\ \end{aligned} $$

(7)

where $Y_{lm}$ denote the spherical harmonics, and $L_{n+l}^{2l+1}$ denote the associated Laguerre polynomial of degree $(n+l)$ and order $(2l+1).$

$1s \rightarrow nlm$ capture amplitude $g_{B}(\vec {k}_f, \vec {k}_i)$ is given by

$$ \begin{aligned} g_{B} (\vec{k}_{f} ,\vec{k}_{i} ) & = \left( { - \frac{{m_{f} }}{{2\pi }}} \right)\left\langle {\Phi _{f} |V_{f} |\Phi _{i} } \right\rangle \\ & = \left( { - \frac{{m_{f} }}{{2\pi }}} \right)\int {e^{{i[\vec{A}.\vec{r}_{2} - \vec{B}.\vec{r}_{1} ]}} } \eta _{{nlm}}^{*} (\vec{r}_{1} )\left[ {\frac{1}{{R_{{12}} }} - \frac{1}{{r_{2} }}} \right]\phi _{{1s}} (\vec{r}_{2} )d\vec{r}_{1} d\vec{r}_{2} \\ & = \left( { - \frac{{m_{f} }}{{2\pi }}} \right)\left[ {I_{1} - I_{2} } \right], \\ \end{aligned} $$

(8)

where

$$ \vec{A} = \frac{{m_{p} }}{{m_{p} + 1}}\vec{k}_{i} - \vec{k}_{f} ,\quad \vec{B} = \vec{k}_{i} - \frac{{\vec{k}_{f} }}{2} $$

$$ I_{1} = \int {e^{{i[\vec{A} \cdot \vec{r}_{2} - \vec{B} \cdot \vec{r}_{1} ]}} } \eta _{{nlm}}^{*} (\vec{r}_{1} )\frac{1}{{R_{{12}} }}\phi _{{1s}} (\vec{r}_{2} )d\vec{r}_{1} d\vec{r}_{2} , $$

(9)

$$ I_{2} = \int {e^{{i[\vec{A} \cdot \vec{r}_{2} - \vec{B} \cdot \vec{r}_{1} ]}} } \eta _{{nlm}}^{*} (\vec{r}_{1} )\frac{1}{{r_{2} }}\phi _{{1s}} (\vec{r}_{2} )d\vec{r}_{1} d\vec{r}_{2} $$

(10)

We first consider the integral $I_1$ which contains positron–proton interaction and hence rather difficult to evaluate. Taking Fourier transforms of the function $exp(-\lambda r)/r$ and then utilizing the $\delta -$function properties $\vec {r}_2$ integration can be carried our easily and we obtain

$$I_1 = -2\left( \frac{\gamma _{1}}{\pi } \right) ^{3/2} \frac{\partial }{\partial \gamma _{1}} \int \frac{e^{i(\vec {s}-\vec {B}).\vec {r}_1}}{(|\vec {A}-\vec {s}|^2 + \gamma _1^2)s^2}\eta _{nlm}^*(\vec {r}_1)~ d \vec {r}_1 d \vec {s}.$$

(11)

where $\gamma _1 = 1/a_0.$ Using integral representation of Feynman

$$\frac{1}{ab} = \int \limits _{0}^{1} \frac{dx}{[ax+b(1-x)]^2}$$

and setting $\lambda ^2 = A^2x(1-x) + \gamma _{1}^2 x$ we obtain

$$ I_{1} = - 2\left( {\frac{{\gamma _{1} }}{\pi }} \right)^{{3/2}} \frac{\partial }{{\partial \gamma _{1} }}\int\limits_{0}^{1} d x~e^{{i(x\vec{A} - \vec{B}) \cdot \vec{r}_{1} }} \eta _{{nlm}}^{*} (\vec{r}_{1} )~d\vec{r}_{1} \int {\frac{{e^{{i(\vec{s} - x\vec{A}) \cdot \vec{r}_{1} }} }}{{[|s - x\vec{A}|^{2} + \lambda ^{2} ]^{2} }}} ~d\vec{s} $$

or,

$$\begin{aligned} I_{1} &= - 2\pi ^{2} \left( {\frac{{\gamma _{1} }}{\pi }} \right)^{{3/2}} \frac{\partial }{{\partial \gamma _{1} }}\int\limits_{0}^{1} {\frac{{e^{{i\vec{p} \cdot \vec{r}_{1} - \lambda r_{1} }} }}{\lambda }} \eta _{{nlm}}^{*} (\vec{r}_{1} )~d\vec{r}_{1} dx,\\ \vec{p} &= x\vec{A} - \vec{B} \end{aligned}$$

or,

$$ I_{1} = - 2\pi ^{2} \left( {\frac{{\gamma _{1} }}{\pi }} \right)^{{3/2}} \frac{\partial }{{\partial \lambda }}\int\limits_{0}^{1} {\frac{F}{\lambda }} \left( {\frac{{\partial \lambda }}{{\partial \gamma _{1} }}} \right)dx $$

or,

$$ I_{1} = {\text{ }}A_{1} \left[ {\int\limits_{0}^{1} {\frac{x}{{\lambda ^{2} }}} \frac{\partial }{{\partial \lambda }}(F)~dx - \int\limits_{0}^{1} {\frac{{xF}}{{\lambda ^{3} }}} ~dx} \right], $$

(12)

where

$$ A_{1} = - 2\pi ^{2} \gamma _{1} \left( {\frac{{\gamma _{1} }}{\pi }} \right)^{{3/2}} , $$

$$ F = \int {e^{{i\vec{p} \cdot \vec{r}_{1} - \lambda r_{1} }} } \eta _{{nlm}}^{*} (\vec{r}_{1} )~d\vec{r}_{1} . $$

(13)

Using the expansion formula of plane wave in terms of spherical Bessel’s function $J_L,$ such as $exp(i\vec {p}.\vec {r}_1) = 4\pi \sum _{L,M} i^L j_L(pr_1)Y_{LM}^*(\hat{p}) Y_{LM}(\hat{r}_1),$ and then utilizing the orthogonal property of spherical harmonics $Y_{LM}$ we obtain

$$F = 4\pi i^lY_{lm}^*(\hat{p})N_{lm}\int \limits _{0}^{\infty } j_l(pr_1) e^{-ar_1} L_{n+l}^{2l+1}(2\gamma _{n}r_1)r_1^{l+2}~dr_1, \quad a = \lambda + \gamma _{n} \;\mathrm{and}\;\gamma _{n} =\frac{1}{n a_0} .$$

(14)

Now using the expansion formula of the associated Laguerre polynomial and a typical integral involving spherical Bessel function, such as

$$ L_{{n + l}}^{{2l + 1}} (x) = \sum\limits_{{i = 0}}^{{n - l - 1}} {( - 1)^{{i + 1}} } \frac{{[(n + l)!]^{2} }}{{(n - l - 1 - i)!(2l + 1 + k)!}}\frac{{x^{i} }}{{i!}} $$

and

$$ \int\limits_{0}^{\infty } {e^{{ - ax}} } j_{l} (bx)x^{{l + 1}} dx = \frac{{(2b)^{l} l!}}{{(a^{2} + b^{2} )^{{l + 1}} }},~~({\text{Re}}(a) > |{\text{Im}}(b)|), $$

(15)

we finally obtain

$$F = C Y_{lm}^*(\hat{p}) \sum _{k=0}^{n-l-1} g(k)~D(p,a,l+1,k+1),$$

(16)

where

$$\begin{aligned}& D(x,y,l,m) = \frac{{d^{m} }}{{dy^{m} }}\left[ {\frac{1}{{(x^{2} + y^{2} )^{l} }}} \right]~\;{\text{and}}\\ & g(k) = \frac{{(2\gamma _{n} )^{k} }}{{(n - l - 1 - k)!(2l + 1 + k)!k!}}. \end{aligned}$$

Substituting (Eq. (16)) into (Eq. (12)) we obtain

$$I_1 = A_1C \sum _{k=0}^{n-l-1} g(k) \int \limits _{0}^{1} \frac{xY_{lm}^*(\hat{p})}{\lambda ^2} \left[ \frac{D(p,a,l+1,k+1)}{\lambda } - D(p,a,l+1,k+2) \right] (2p)^l~dx .$$

(17)

In the similar fashion the integral $I_2$ can be evaluated. The one-dimensional integration over [0, 1] appearing in integral (Eq. (17)) has been evaluated numerically by employing Gauss–Legendre quadrature formula. Note that the integral (Eq. (17)) has a fictitious singularity , which has been removed by taking a transformation of the form $x=z^2.$