Introduction

In recent decades the influence of vibrations on mechanical processes in multicomponent fluid systems has become the perspective research direction for many areas of fundamental and applied science. The findings of investigations on vibrational fluid dynamics have guided the improvement in planning the microgravity experiments, managing the heat-mass transfer and flow structure in crystal growth processes, protecting the natural environment, as well as the development of new computational algorithms and software tools (see, e.g., Gershuni and Lyubimov 1998; Lyubimov et al. 2003; Lappa 2004; Mialdun et al. 2008; Andreev et al. 2012).

For various purposes, among them practical applications, it would be much desirable to stabilize the process as a whole. That is the necessity to understand the physical mechanisms determining the fluid dynamics stability.

The problem of convective instability to small normal disturbances of horizontal, pure liquid layer heated from below has been well studied both theoretically and experimentally (Gershuni and Zhukhovitsky 1976; Lappa 2010). In a binary-mixture layer the convective instability owes its initiation to the interaction between the externally imposed temperature gradient and the Soret driven concentration gradient in a mass-conserving system. The linear analysis enables to identify mechanisms and modes prevailing at the threshold of convective instability in a horizontal binary-mixture layer in accordance with values of control parameters like the Rayleigh number and the separation ratio (Platten and Legros 1984; Jiang et al. 1991; Platten 2006). The addition to the static gravity acceleration of its vibrational part manifests itself as the mechanism affecting the processes relevant to physics of fluids or materials science, either intensifying or slowing down heat and mass exchange. Deep understanding of this specific dependence on modified gravity conditions expands the possibilities for technological applications in space environment (Ostrach 1977, 1982; Alexander 1990; Mialdun et al. 2008).

As for studying thermal vibrational convection in binary mixtures with thermodiffusion, the published results relate to the presence of vibrations which vary in direction, frequency or amplitude. The bibliography on the problem is also quite extensive. Let us set off some of the articles concerning the effect of high frequency and small amplitude vibrations on the base state of two-component liquid system. In this limiting case the mathematical model of the process has the form of the initial-boundary problem for the set of equations for averaged fields of real physical variables. As a rule it is written in accordance with the Boussinesq assumptions, so density non-uniformity has the only influence on the flow field in the term representing the buoyancy force. The authors of works (Gershuni et al. 1997, 1999; Chacha et al. 2002; Smorodin et al. 2002; Shevtsova et al. 2006, 2007, 2015; Lyubimov et al. 2013; Gaponenko and Shevtsova 2016; Ouadhani et al. 2017) give special attention to the conceptions, physical mechanisms, mathematical correctness of computational procedures and reliability of the results obtained. In work (Gershuni et al. 1997) the convection of binary gas or fluid mixtures filling infinite horizontal layer subjected to longitudinal vibrations is theoretically modeled. The quasi-equilibrium state is considered as the base one. As a result of linear stability analysis the boundaries of stability and the critical disturbance characteristics are determined for representative parameter values, different instability mechanism and forms are discussed. As it is shown in work (Gershuni et al. 1999) the effect of transversal vibrations is always stabilizing. The series of comprehensive microgravity researches (Chacha et al. 2002; Shevtsova et al. 2006, 2007, 2015; Gaponenko and Shevtsova 2016) of the various types of convection possible in Space, demonstrates that the low-gravity fluid dynamics may exhibit principal dissimilarity from that under terrestrial conditions. The results obtained by means of direct numerical simulations are in good agreement with experimental observations performed on the International Space Station and during parabolic flights. Linear stability of incompressible plane-parallel inviscid pulsational flow is studied in Lyubimov et al. (2013) ignoring diffusion effect. The results concerning long-wave instability have been worked out analytically and instability to the perturbations with finite wavelength – numerically. In Ouadhani et al. (2017) the influence of transversal high-frequency and small-amplitude vibrations on the separation of a binary mixture, which penetrates a porous cavity, is under analytical and numerical considerations. It is shown that vibrations may conduce to the unicellular flow which loses its stability via oscillatory bifurcation. The papers (Cross and Hohenberg 1993; Moses et al. 1987; Jung et al. 2004; Smorodin et al. 2008; Smorodin and Lücke 2009) present theoretical and experimental evidences of nonlinear oscillatory convective regimes emerging in plane horizontal binary fluid layer in traveling wave pattern near the instability threshold as a result of local bifurcation analysis or direct numerical simulations.

The purpose of the present paper is to describe analytical and numerical results of high-frequency thermovibrational convection in binary liquid mixtures in the case of arbitrary inclination of the vibration axis to the boundaries of the horizontal layer.

The paper is organized as follows. “Statement of the Problem” contains the physical description and mathematical formulation of the thermovibrational convection problem for binary mixture. The critical stability characteristics are discussed in “Linear Stability Analysis”. The bifurcation diagrams and some properties of nonlinear solutions are presented in “Nonlinear Solutions”. “Conclusion” contains concluding remarks.

Statement of the Problem

Let us consider an infinite plane horizontal layer of finite depth h bounded by two parallel rigid plates and filled with a binary liquid mixture (for example, ethanol-water solution). The origin of the space Cartesian coordinate system is located at the lower boundary, the x-axis is directed along the layer and the z-axis is oriented positively upwards, as in the Fig. 1. The dependence of phase variables on y-coordinate is neglected. Both boundaries z = 0 and z = h are treated as perfect thermal conductors and impermeable to mixture components. The lower boundary is maintained at fixed temperature T(x,0,t) = Θ while the upper boundary is held at temperature zero T(x,h,t) = 0. The temperature difference between the plates causes the vertical temperature gradient in the quiescent mixture. Due to the nature of thermal diffusion a temperature gradient gives rise to the relative motion of mixture ingredients which results in the concentration gradient in an originally homogeneous mixture.

Fig. 1
figure 1

Geometry of the problem and the system of coordinates

Full size image

The equation of binary fluid state can be written in the form resulting from the mixture density expansion

$$\rho =\rho_{{\kern 1pt}0} (1-\alpha \,(\,T-\bar{{T}}\,)-\beta \,(\,C-\bar{{C}}\,)\,). $$

Here ρ 0 is the reference mixture density at certain mean values of temperature \(\bar {{T}}\) and concentration \(\bar {{C}}\), α and β are the thermal and solutal expansion coefficients, respectively, and deviations from the mean values are assumed to be small. Presupposing C the concentration of the light component, we obtain β > 0.

The layer is situated in a static gravitational field g = −g γ, where γ is the upward unit vector, and subjected to a harmonic vibration having the displacement amplitude b, angular frequency Ω, and the direction oriented at an angle δ to the layer boundaries. We consider the limit of high-frequency (but below acoustic) vibrations with the period T v = 2π/Ω, which is much smaller than all characteristic time scales (like hydrodynamic, thermal and diffusion):

$$T_{v} \ll \min \left[{{h^{2}}/\nu,{h^{2}} /\chi ,{h^{2}}/D} \right]. $$

Here D is the diffusion coefficient of the mixture, and ν and χ designate, respectively, the coefficients of its kinematic viscosity and thermal diffusivity.

The mathematical model has been developed to describe the dynamics of certain characteristics of thermovibrational binary-mixture convection, like mean parts of the velocity v, temperature T, concentration C and additional variable w, correctly representing the amplitude of pulsation velocity. All the fields v, T, C and w vary slowly with time, and the set of governing equations is obtained by means of the standard averaging procedure applied to the buoyancy convection equations within the framework of the Oberbeck–Boussinesq approximation (Zen’kovskaya and Simonenko 1966; Gershuni and Zhukhovitsky 1979; Gershuni and Lyubimov 1998). To nondimensionalize physical quantities the following appropriate scales are used: the layer thickness h for a length, χ/h for a velocity, h 2/χ for a time, Θ for a temperature, α Θ/β for a concentration, α Θ for w field and ρ 0 ν χ/h 2 for a pressure. As a result one can write the set of averaged dimensionless equations of the thermovibrational convection in binary mixture as follows (Gershuni and Lyubimov 1998):

$$\begin{array}{@{}rcl@{}} \frac{{1}}{\text{Pr}}\frac{\partial \boldsymbol{v}}{\partial t}&+&\frac{{1}}{\text{Pr}}(\boldsymbol{v}\nabla )\boldsymbol{v}=-\nabla p+{\Delta} \boldsymbol{v}+\text{Ra}\,\mathbf{(}T\mathbf{+}C\mathbf{)}\boldsymbol{n}\\&+&\text{Gs}(\boldsymbol{w}\nabla )\left[ {\mathbf{(}T\mathbf{+}C\mathbf{)}\boldsymbol{n}-\boldsymbol{w}} \right], \end{array} $$
$$ \frac{\partial T}{\partial t}+(\boldsymbol{v}\nabla )T={\Delta} T, $$
(1)
$$\frac{\partial C}{\partial t}+(\boldsymbol{v}\nabla )C=\text{Le}\,{\Delta} (C-\psi T), $$
$$\nabla \cdot \,\boldsymbol{v}= 0,\,\,\,\quad \nabla \cdot \,\boldsymbol{w}= 0,\quad \text{curl} \boldsymbol{w}=\nabla \left( {T+C} \right)\times \boldsymbol{n}. $$

Here p is the pressure deviation from the hydrostatic one at the density ρ 0,Ra = g αΘh 3/(ν χ) is the Rayleigh number, Gs = (bΩαΘh)2/(2ν χ) is the Gershuni number, Pr = ν/χ is the Prandtl number, Le = D/χ is the Lewis number, and \(\psi ={-\kappa _{T} \beta } / {(\bar {T}\alpha )}=-\bar {C}(1-\bar {C})\mathrm {S}_{\mathrm {T}}{\beta }/\alpha \) is the separation ratio, respectively, while \(\kappa _{T} =\bar {T}\bar {C}(1-\bar {C})\mathrm {S}_{\mathrm {T}}\) is the thermodiffusion coefficient and ST is the Soret coefficient. The unit vector n characterizes the direction of vibrations.

The problem specified by the system of Eq. (1) with appropriate initial and boundary conditions has the quasi-equilibrium solution describing the linear equilibrium fields of temperature, concentration and variable w:

$$\begin{array}{@{}rcl@{}} &&\!\boldsymbol{v}_{0} \!= \!0, \quad T_{0} \,=\,1\,-\,z, \quad \!{d C_{0}} /{d z}\,=\,- \psi, \quad \!\boldsymbol{w}_{0} \,=\,(\boldsymbol{w}_{0x} ,0,0),\\&&\!\boldsymbol{w}_{0x}=-(1+\psi)(z-1/2)\cos \delta. \end{array} $$
(2)

It is seen that in the case of transversal vibrations δ = π/2, the pulsating velocity vector w 0 = 0 and the system has mechanical equilibrium as its base state (Gershuni et al. 1999).

To work out the problem in the shape of y-axial rolls, the stream function and vorticity formulation is developed. We introduce two stream functions denoted by Ψ and F and the vorticity Φ, which are velocity-related as follows

$$\begin{array}{@{}rcl@{}} &&v_{x} =\frac{\partial {\Psi} }{\partial z},\quad v_{z} =-\,\frac{\partial {\Psi} }{\partial \,x}, \quad w_{x} =\frac{\partial F}{\partial z},\,\;\quad \!w_{z} =-\,\frac{\partial F}{\partial \,x}, \\ &&{\Phi} =(\nabla \times \mathbf{v})_{y} . \end{array} $$
(3)

The set of governing equations for two-dimensional thermovibrational convection of incompressible binary mixture can be written thus:

$$\begin{array}{@{}rcl@{}} \frac{1}{\Pr}\frac{\partial {\Phi} }{\partial t}&+&\frac{1}{\Pr}\left\{ {\frac{\partial {\Psi} }{\partial z}\frac{\partial {\Phi} }{\partial x}-\frac{\partial {\Psi} }{\partial x}\frac{\partial {\Phi} }{\partial z}} \right\}={\Delta} {\Phi} +\text{Ra}\frac{\partial (T+C)}{\partial x} \\ &+& \text{Gs}\left\{\cos\delta \left[ {\frac{\partial (T+C)}{\partial z}\frac{\partial^{2}F}{\partial x\partial z}-\frac{\partial^{2}F}{\partial z^{2}}\frac{\partial (T+C)}{\partial x}} \right]\right.\\ &+&\left.\sin\delta \left[ {\frac{\partial (T+C)}{\partial x}\frac{\partial^{2}F}{\partial x \partial z}-\frac{\partial^{2}F}{\partial x^{2}}\frac{\partial (T+C)}{\partial z}} \right] \right\} \\ \frac{\partial T}{\partial t}&+&\frac{\partial {\Psi} }{\partial z}\frac{\partial T}{\partial x}-\frac{\partial {\Psi} }{\partial x}\frac{\partial T}{\partial z}={\Delta} T, \\ \frac{\partial C}{\partial t}&+&\frac{\partial {\Psi} }{\partial z}\frac{\partial C}{\partial x}-\frac{\partial {\Psi} }{\partial x}\frac{\partial C}{\partial z}=\text{Le}({\Delta} C-\psi {\Delta} T), \\ {\Phi} &=&-{\Delta} {\Psi} , \\ {\Delta} F&=&\frac{\partial (T+C)}{\partial z}\cos \delta -\frac{\partial (T+C)}{\partial x}\sin \delta . \end{array} $$
(4)

We seek and find the solution of this problem satisfying certain boundary conditions, which correspond to no-slip, impermeable, and isothermal horizontal plates:

$$\begin{array}{@{}rcl@{}} z&=&0:\quad {\Psi} = 0,\quad \frac{\partial {\Psi} }{\partial z}= 0,\quad F = 0,\\ T&=&1,\quad \frac{\partial C}{\partial z}-\psi \frac{\partial T}{\partial z}= 0, \\ z&=&1:\quad {\Psi} = 0,\quad \frac{\partial {\Psi} }{\partial z}= 0,\quad F = 0,\\ T&=&0,\quad \frac{\partial C}{\partial z}-\psi \frac{\partial T}{\partial z}= 0. \end{array} $$
(5)

Linear Stability Analysis

Let us consider small normal-mode disturbances of the base state (2) taken as

$$ \{\Psi ,T,{\Xi} ,F\}=\exp (\lambda t+ikx)\{\varphi (t,z),\theta (t,z),\xi (t,z),f(t,z)\}, $$
(6)

where Ξ = C − ψ T is the new variable, k is the wave number along the x-axis, λ = λ r + i λ i is the complex decrement of disturbances with the growth rate λ r and oscillation frequency λ i = ω.

After linearization one can obtain the following spectral boundary value problem for amplitudes of the disturbances:

$$\begin{array}{@{}rcl@{}} &&\frac{\lambda}{\text{Pr}}\left( {\frac{d^{2}}{dz^{2}}-k^{2}} \right)\varphi =\left( {\frac{d^{2}}{dz^{2}}-k^{2}} \right)^{2}\varphi +ik\text{Ra}[(1+\psi )\theta +\xi ]\\ &+&\text{Gs} \left\{ {ik\cos \delta \left[ {(1+\psi )\theta +\xi -\frac{df}{dz}} \right]-k^{2}\sin \delta (1+\psi )f} \right\},\\ &&\lambda \theta-ik\varphi =\;\left( {\frac{d^{2}}{dz^{2}}-k^{2}}\right)\theta , \end{array} $$
(7)
$$\begin{array}{@{}rcl@{}} &&\lambda \xi -ik\psi \varphi =\text{Le}\left( {\frac{d^{2}}{dz^{2}}-k^{2}} \right)\xi -\psi \;\left( {\frac{d^{2}}{dz^{2}}-k^{2}} \right)\theta , \\ &&\left( {\frac{d^{2}}{dz^{2}}-k^{2}} \right)f\!=\cos \delta \left[ {(1+\psi )\frac{d\theta }{dz}+\frac{d\xi }{dz}} \right]\!-ik\sin \delta [(1+\psi )\theta +\xi ], \\ &&z = 0;1:\quad \varphi = 0,\quad \frac{\partial \varphi }{\partial z}= 0,\quad f = 0,\quad \theta = 0,\quad \frac{\partial \xi }{\partial z}= 0. \end{array} $$
(8)

The linear theory of hydrodynamic stability assumes that small normal-mode perturbations of the base conductive state may be amplified or damped. If their growth rates, i.e. real parts of eigenvalues, are nonpositive, and at least one of them equals zero, the base state of the system is marginally (or neutrally) stable. Anticipating we emphasize that in a binary fluid mixture with negative Soret coupling the onset of the base state instability is conditioned by the oscillatory-mode perturbations.

To find the stability threshold in the limiting case of long wavelength disturbances, when k tends to zero, we assume, in accordance with the perturbation technique (Nayfeh 1973), that the approximate solution of the problem (7), (8) has the form of asymptotic series in terms of the small parameter k for the decrement and all the amplitudes, that is, we let

$$\begin{array}{@{}rcl@{}} &&\lambda \,=\,{\sum}_{n = 0}^{\infty} {\lambda_{n} k^{n}} , \quad \varphi \,=\,{\sum}_{n = 0}^{\infty} {\varphi_{n} k^{n}} , \quad \theta \,=\,{\sum}_{n = 0}^{\infty} {\theta_{n} k^{n}} , \\ &&\xi ={\sum}_{n = 0}^{\infty} {\xi_{n} k^{n}} . \end{array} $$
(9)

Substituting expansions (9) into the set (7) and matching the factors of like powers of k, we derive the simplified sets of equations of different orders, which are solved in succession.

In the zeroth order of approximation to k, the following system is derived:

$$\begin{array}{@{}rcl@{}} &&\frac{\lambda_{0} }{\Pr }\frac{d^{2}\varphi_{0} }{dz^{2}}=\frac{d^{4}\varphi_{0} }{dz^{4}}, \\ &&\lambda_{0} \theta_{0} =\frac{d^{2}\theta_{0} }{dz^{2}}, \\ &&\lambda_{0} \xi_{0} =\text{Le}\frac{d^{2}}{dz^{2}}(\,\xi_{0} -\psi \theta_{0} ), \\ &&\frac{d^{2}f_{0} }{dz^{2}}=\left[ {(1+\psi )\frac{d\theta_{0} }{dz}+\frac{d\xi_{0} }{dz}} \right]\cos \delta . \end{array} $$
(10)

This implies that φ 0 = 0,𝜃 0 = 0,f 0 = 0,λ 0 = 0, and ξ 0 = c o n s t is the only nonzero function. An arbitrary value may be assigned to it, e.g., ξ 0 = 1.

In the first order of approximation to k, the solution {φ 1,𝜃 1,ξ 1,f 1,λ 1} is determined by the following set of equations:

$$\begin{array}{@{}rcl@{}} &&\frac{d^{4}\varphi_{1} }{dz^{4}}=-i\left[ {\text{Ra}+\text{Gs}(1+\psi )\cos \delta } \right], \\ &&\frac{d^{2}\theta_{1} }{dz^{2}}= 0, \\ &&\lambda_{1} =\text{Le}\frac{d^{2}}{dz^{2}}(\xi_{1} -\psi \theta_{1} ), \\ &&\frac{d^{2}f_{1} }{dz^{2}}=\left[ {(1+\psi )\frac{d\theta_{1} }{dz}+\frac{d\xi_{1} }{dz}} \right]\cos \delta -i\sin \delta. \end{array} $$
(11)

The first-order approximation is

$$\begin{array}{@{}rcl@{}} &&\lambda_{1} = 0, \quad \theta_{1} = 0, \quad \xi_{1} =const,\\ &&f_{1} =-i\frac{z(1-z)}{2}\sin \delta ,\\ &&\varphi_{1} =-i\left[ {\text{Ra}+\text{Gs}(1+\psi )\cos \delta } \right]\,z^{2}{(1-z)^{2}} / {24}. \end{array} $$
(12)

To find an improved solution to the spectral problem, the approximation of the second order to k is considered. The correction λ 2 is obtained from the equation for the concentration amplitude function:

$$ \lambda_{2} -i\psi \varphi_{1} =\text{Le}\frac{d^{2}}{dz^{2}}(\,\xi_{2} -\psi \,\theta_{2} \,-1). $$
(13)

Equation (13) is integrated with respect to z across the layer to give the relationship

$$\lambda_{2} =-\text{Le}+i\psi {{\int}_{0}^{1}} {\varphi_{1} dz} . $$

Using (12) one can achieve the following expression

$$ \lambda_{2} =-\text{Le}+\frac{\psi }{720}\left[ {\text{Ra}+\text{Gs}(1+\psi )\cos \delta } \right]. $$
(14)

Since the decrement correction proves to be real, the long wavelength perturbations are monotonic.

The stability boundary can be found by the requirement λ 2 = 0, resulting in a dependence

$$ \text{Ra}=\frac{720\text{Le}}{\psi }-\text{Gs}(1+\psi )\cos \delta . $$
(15)

It is in agreement with the results (Gershuni et al. 1997, 1999) for the limiting cases of longitudinal vibrations and transversal ones.

Under pure weightless conditions (no buoyancy, Ra = 0) the relationship (15) takes the form

$$ \text{Gs}=\frac{720\text{Le}}{\psi (1+\psi )\cos \delta }. $$
(16)

As can be seen from (16), under zero gravity the long-wave instability exists in horizontal binary-mixture layers with negative Soret coupling in the range ψ < − 1. Depending on the vibration axis orientation, the critical value of the Gershuni number is minimal when the inclination angle δ = 0, i.e. in the case of longitudinal vibrations. The threshold value grows with δ and would be infinitely high when δ = π/2, i.e. under transversal vibrations.

Nonlinear Solutions

We consider secondary nonlinear regimes branching from the base quasi-equilibrium state as a result of its instability with respect to finite-amplitude disturbances periodic along the horizontal coordinate:

$$ \left\{{\varphi ,{\Psi} ,F,T,C}\right\}(x,z,t)=\left\{{\varphi,{\Psi} ,F,T,C} \right\}(x+L, z, t). $$
(17)

The period L = 2 of spatial translation along the x-axis is the dimensionless distance that is close to the wave number k = π of the disturbance which is, by the linear stability theory, the most “dangerous” for stability of established quasi-equilibrium state.

The numerical solution of the boundary value problem (4)–(5) for thermovibrational convection is obtained by means of finite-difference technique. The computational procedure is based on the implicit factored scheme of the second order in space and used the ADI Thomas algorithm to get temperature, concentration and vortex fields. The stream function fields at each time step are calculated with the iterative successive over relaxation method. To describe the flow dynamics and convective heat and mass transfer the integral and local characteristics are studied depending on nondimensional process parameters. In the present paper, we hold fixed the set of parameters relevant for molecular liquid mixtures, e.g., the ethanol-water solution (Kolodner et al. 1988): the Lewis number Le = 0.01, the Prandtl number Pr = 10, and the negative separation ratio ψ = − 0.25.

To compare the findings with known experimental, analytical, or numerical results that had been previously exposed in literature, the reduced Rayleigh number is introduced r = Ra/Ra0, where Ra0 = 1686 is the critical Rayleigh number for the onset of convection in homogeneous liquid obtained by means of our numerical code. The increase of the reduced Rayleigh number leads to the perturbation growth and convection excitation via Hopf bifurcation at the critical value r o s c . The oscillatory bifurcation threshold r = r o s c (Gs) and the Hopf frequency ω H = ω H (Gs) calculated at several Gershuni number values for the longitudinal or transversal orientation of vibration axis have been compared with the corresponding critical data reported earlier. It has been found that the worked out set of critical parameters is close to estimated by the numerical linear stability analysis in Gershuni et al. (1997, 1999).

Near the oscillatory bifurcation point there is the range of the reduced Rayleigh number values where several solutions exist, either stable or unstable. The accurate computation of unstable finite-amplitude flows is impossible by means of discrete numerical techniques. But not far from the critical Rayleigh number, and within the numerical accuracy we have, the location of unstable branches has been established, using the branch-following algorithm of parameter continuation method. The computational procedure starts in the small vicinity of the bifurcation point where sought for solution exists. The incorrect initial guess may allow the convergence to the solution on a different branch. In successful cases the approximation has been observed to remain for long within the vicinity of the unstable branch. Using repeated computational experiments successive intermediate solutions have been constructed as starting fields for the next challenge in sequence.

Figure 2 represents the bifurcation diagram of nonlinear convective regimes depending on the intensity of longitudinal vibrations. In a qualitative sense, the curves for the considered Gershuni number values, look similar to each other. The base state (2) becomes unstable at r = r o s c relative to the finite periodic perturbations (17), and the Hopf bifurcation gives rise to the traveling wave convection pattern of propagating rolls. Two characteristics of this flow structure are plotted in Fig. 2 as functions of the reduced Rayleigh number. They are a maximum of the vertical velocity component v Z and the oscillation frequency ω. Stable solutions are distinguished by solid lines and unstable ones – by dashed lines. It is seen in the upper part of Fig. 2 that the unstable TW convective flow bifurcates backwards out of the base state (2). This regime acquires stability via a saddle-node bifurcation at the reduced Rayleigh number value \(r=r_{S}^{TW} \). The TW frequency on the upper branch of stable TWs, as shown in the lower part of Fig. 2, demonstrates a monotonic descending dependence on r and disappears at the point of TW conversion to the steady convective rolls pattern. A comprehensive analysis of TW’s bifurcation properties in the case of no vibration (Gs = 0) is contained in Barten et al. (1995). When the heating intensity is low enough, and \(r<r_{S}^{TW}\), the binary mixture convection fades away and the dynamic system turns to the conductive state (2). Referring to Fig. 2, bifurcation values of the reduced Rayleigh number r o s c and \(r_{S}^{TW}\) tend to decrease as the intensity of longitudinal vibrations is increased, while the traveling wave frequency does not depend on Gs.

Fig. 2
figure 2

Bifurcation characteristics of the TW solution: (a) – the maximum of vertical convective velocity; (b) – the oscillation frequency. Solid lines –stable states, dashed lines – unstable states. 1 – Gs = 0; 2 – Gs = 500; 3 – Gs = 1000; 4 – Gs = 1500; 5 – Gs = 2000

Full size image

Figure 3 illustrates the effect of the vibration axis inclination at an angle δ on the resulting bifurcation diagram by correlation with that in the case of longitudinal vibration represented in Fig. 2. Although the pictures look qualitatively similar to each other, it is seen that the increase of the inclination angle leads to a significant growth of critical values r o s c and \(r_{S}^{TW}\) of the reduced Rayleigh number.

Fig. 3
figure 3

Effect of varying the vibration axis direction. The presentation is as in Fig. 2. Gs = 1000. 1 – δ = 0; 2 – δ = π/4; 3 – δ = π/2

Full size image

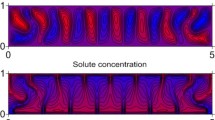
It is necessary to note that within the range of the reduced Rayleigh number values r 1 < r T W < r 2 the modulated traveling wave regime (MTW) manifests itself under the high-intensity transversal vibration. The results depicted in Fig. 4 elucidate the behavior of two associated characteristics:

$${\Psi}_{\max}(t)=\underset{i, j}{\max}{\Psi} (x_{i} ,z_{j} ,t);\quad{\Psi}_{loc}(t)\,=\,{\Psi}(x=L / 4,z\,=\,1 / 2,t) $$

The maximum-over-domain stream function value Ψmax(t) oscillates within the interval δΨ with the period T mod. The local stream function Ψ l o c (t) demonstrates both amplitude modulation and strong phase modulation. So that the oscillation period in the fixed point of convective cell changes within the interval 8.73 < T l o c < 32.2. Figure 5a represents the modulation depth δΨ as a monotonic increasing function of the Gershuni number, while the modulation period T mod changes nonmonotonically (see Fig. 5b).

Fig. 4
figure 4

(Color online) Modulated traveling-wave regime. Time dependence of the maximum stream function value over grid domain and local stream function value at the fixed point. r = 2.472;δ = π/2;Gs = 5100

Full size image
Fig. 5
figure 5

The depth of modulation and period of modulated traveling wave versus the reduced Rayleigh number for parameters of Fig. 4

Full size image

Conclusion

Thus we can say that mentioned above theoretical results focus on the noticeable features of thermovibrational convection, like the critical circumstances for the long wavelength instability excitation in an initially quiescent and heated from below horizontal layer of binary liquid mixture with negative Soret coupling, which oscillates with high frequency, small amplitude and arbitrarily oriented axis about the layer boundaries. The mathematical model obtained by applying the averaging technique to the set of governing equations and appropriate boundary conditions allowed us to study the vibrational effect on scenarios of transition to dynamic phenomena in the system.

The linear stability approach has made it feasible to find the neutral stability characteristics. They are evidently comparable, in the relevant aspects, to those published earlier. Further the nonlinear evolution of the base state with respect to the finite periodic perturbations of sufficiently large amplitude is considered. The spatiotemporal dynamics and pattern formation are studied by means of direct numerical simulations as well as bifurcation diagrams in the range of control parameters adapted to experiments. The analysis of bifurcation properties of nonlinear convective flows allowed us to answer the question, what will the perturbations grow into. It has been demonstrated that at the oscillatory instability threshold the two-dimensional convective roll pattern flow in the form of traveling wave convection bifurcates subcritically out of the base state. These unstable branches of solutions meet the stable ones at the saddle-node points in the bifurcation diagram. The vibration axis inclination over the layer boundaries may essentially shift the fixed point position on the phase diagram. Far from the quasi-equilibrium state the secondary bifurcation is observed to flow pattern in the form of modulated traveling wave. The depth and period of modulation are represented and discussed.