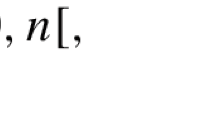Brake orbit solutions for semilinear elliptic systems with asymmetric double well potential

Abstract

We consider a class of semilinear elliptic system of the form:

$$\begin{aligned} -\Delta u(x,y)+\nabla W(u(x,y))=0,\quad (x,y)\in {\mathbb {R}}^{2}, \end{aligned}$$

(0.1)

where $W:{\mathbb {R}}^{2}\rightarrow {\mathbb {R}}$ is a double well potential with minima $\mathbf{a}_\pm \in {\mathbb {R}}^2$. We show, via variational methods, that if the set of minimal heteroclinic solutions to the one-dimensional system $-\ddot{q}(x)+\nabla W(q(x))=0,\ x\in {\mathbb {R}}$, up to translations, is finite and constituted by not degenerate functions, then Eq. (0.1) has infinitely many solutions $u\in C^{2}({\mathbb {R}}^{2})^{2}$, parametrized by an energy value, which are periodic in the variable y and satisfy $\lim _{x\rightarrow \pm \infty }u(x,y)=\mathbf{a}_{\pm }$ for any $y\in {\mathbb {R}}$.

1 Introduction

We consider semilinear elliptic system of the form:

$$\begin{aligned} -\Delta u(x,y)+\nabla W(u(x,y))=0,\quad (x,y)\in {\mathbb {R}}^{2}, \end{aligned}$$

(1.1)

where $W\in {\mathcal {C}}^{2}({\mathbb {R}}^{2})$ satisfies

• $(W_{1})$ There exist $\mathbf{a}_{\pm }\in {\mathbb {R}}^{2}$, such that $W(\mathbf{a}_{\pm })=0$, $W(\xi )> 0$ for every $\xi \in {\mathbb {R}}^{2}{\setminus }\{\mathbf{a}_{\pm }\}$ and $D^{2}W(\mathbf{a}_{\pm })$ are definite positive.

• $(W_{2})$ There exists $R>0$, such that $\inf _{|\xi |=R}W(\xi )=w_{0}>0$ and $\nabla W(\xi )\xi \ge 0$ for $|\xi |>R$;

The problem of existence of differently shaped entire solutions for equations or systems of the form (1.1) has been widely studied in the last years, both in the autonomous or non-autonomous cases (see [3, 7, 8, 10–18, 21–27] and the references therein).

Here, we look for solutions $u\in C^{2}({\mathbb {R}}^{2})^{2}$ of (1.1) satisfying the asymptotic conditions:

$$\begin{aligned} \lim _{x\rightarrow \pm \infty }u(x,y)=\mathbf{a}_{\pm }\quad \hbox {uniformly w.r.t. } \quad y\in {\mathbb {R}}. \end{aligned}$$

(1.2)

Problems (1.1)–(1.2) arise considering the reaction-diffusion system

$$\begin{aligned} \partial _tu(x,y)-\varepsilon ^2\Delta u(x,y)+\nabla W(u(x,y))=0,\quad (x,y)\in \Omega \subset {\mathbb {R}}^2 \end{aligned}$$

(1.3)

in the limit as $\varepsilon \rightarrow 0^+$. Solutions to (1.3) converge almost everywhere to global minima of W and sharp phase interfaces appear (see [19, 29, 31]) with the first term in the expansion represented by solution to (1.1)–(1.2).

The problem of existence of planar solutions to (1.1)–(1.2) was studied by Alama et al. in [1] under the additional symmetry condition on the potential:

• $(W_{3})$ $W(-\xi _{1},\xi _{2})=W(\xi _{1},\xi _{2})$    for any    $(\xi _{1},\xi _{2})\in {\mathbb {R}}^{2}$.

In [1] is first considered the set of one-dimensional minimal solutions to (1.1)–(1.2), that is, the set of solutions to

$$\begin{aligned} {\left\{ \begin{array}{ll}-\ddot{q}(x)+\nabla W(q(x))=0,&{}x\in {\mathbb {R}}\\ {\displaystyle \lim _{x\rightarrow \pm \infty }q(x)=\mathbf{a}_{\pm }}.&{}\end{array}\right. } \end{aligned}$$

(1.4)

which are, furthermore, minima of the action

$$\begin{aligned} V(q)=\int _{{\mathbb {R}}}\tfrac{1}{2}|\dot{q}|^{2}+W(q)\, \mathrm{d}x \end{aligned}$$

on the class of symmetric functions

$$\begin{aligned} \Gamma _{s}=\{q\in H^{1}({\mathbb {R}})^{2}\, |\, q(\pm \infty )=a_{\pm }\quad \hbox { and } \quad q(-x)=(-q_{1}(x),q_{2}(x))\}. \end{aligned}$$

Denoting ${\mathcal {M}}_{s}=\{ q\in \Gamma _{s}\mid V(q)=\inf _{q\in \Gamma _{s}}V(q)\}$ in [1], it is proved that if ${\mathcal {M}}_{s}$ is a finite set, i.e., if ${\mathcal {M}}_{s}=\{ q_{1},\dots , q_{k}\}$ with $k\ge 2$ and $q_{i}\not = q_{j}$ when $i\not =j$, then there exists a solution $v\in C^{2}({\mathbb {R}}^{2})^{2}$ to (1.1) and (1.2) which is asymptotic as $y\rightarrow \pm \infty$ to two different one-dimensional solutions $q_{\pm }\in {\mathcal {M}}_{s}$.

The result in [1] was strengthen in [2] where assuming ($W_{1}$), ($W_{2}$), and ($W_{3}$) and adapting to the vectorial case an energy constrained variational argument used in [4–6, 9], it is shown that (1.1)–(1.2) admit infinitely many planar solutions whenever the set of one-dimensional minimal symmetric heteroclinic solutions is not a continuum. More precisely, a first result states that if ${\mathcal {M}}_{s}$ decomposes in the union of two disjoint set:

$$\begin{aligned} (*_{s}) \qquad {\mathcal {M}}_{s}={\mathcal {M}}_{s,+}\cup {\mathcal {M}}_{s,-} \quad \mathrm{with} \quad \, {\mathrm {dist}}_{L^{2}({\mathbb {R}})^{2}}({\mathcal {M}}_{s,+},{\mathcal {M}}_{s,-})>0, \end{aligned}$$

then, there exists a solution $u_{m}\in C^{2}({\mathbb {R}}^{2})^{2}$ of (1.1)–(1.2) verifying $u_{m}(\cdot ,y)\in \Gamma _{s}$ for all $y\in {\mathbb {R}}$ and ${\mathrm {dist}}_{L^{2}({\mathbb {R}}^{2})^{2}}(u_{m}(\cdot ,y),{\mathcal {M}}_{s,\pm })\rightarrow 0\hbox { as } y\rightarrow \pm \infty$. Together with this first existence result, in [2], it is proved the existence of infinitely many energy prescribed solutions of (1.1)–(1.2). To give an idea of the result, let us observe that any solution u of (1.1)–(1.2) verifying $u(\cdot ,y)\in \Gamma _{s}$ for all $y\in {\mathbb {R}}$ can be roughly seen as a trajectory $y\in {\mathbb {R}}\mapsto u(\cdot ,y)\in \Gamma _{s}$, solution to the infinite dimensional Lagrangian system:

$$\begin{aligned} \frac{\mathrm{d}^{2}}{\mathrm{d}y^{2}} u(\cdot ,y)=V'(u(\cdot ,y)). \end{aligned}$$

Since y is cyclic in the equation, the corresponding energy is conserved along such solutions, i.e., the function $E_{u}(y)= \frac{1}{2}\Vert \partial _{y}u(\cdot ,y)\Vert ^{2}_{L^{2}({\mathbb {R}})^{2}}-V(u(\cdot ,y))$ is constant on ${\mathbb {R}}$ (see [20] for more general identities of this kind). In particular, denoting $m=\min _{\Gamma _{s}}V$, the above solution $u_{m}$ is such that $E_{u_{m}}(y)=-m$ for every $y\in {\mathbb {R}}$ and it connects as $y\rightarrow \pm \infty$ the two disjoint parts ${\mathcal M}_{s,\pm }$ of the level set $\{q\in \Gamma _{s}\,|\,V(q)\le m\}$. In [2], this kind of result is generalized to different value of the energy. Indeed, if $b\in (m,m+\lambda )$ with $\lambda >0$ small enough, by $(*_{s})$, the sublevel set $\{q\in \Gamma _{s}\,|\, V(q)\le b \}$ separates into two well disjoint parts: $\{q\in \Gamma _{s}\,|\, V(q)\le b \}={\mathcal V}^b_{-}\cup {\mathcal V}^b_{+}\hbox { with } \hbox {dist}_{L^{2}}({\mathcal V}^b_{-}, {\mathcal V}^b_{+})>0$. Theorem 1.2 in [2] establishes in particular that for any $b\in (m,m+\lambda )$, there exists a solution $u_{b}\in C^{2}({\mathbb {R}}^{2})^{2}$ of (1.1) and (1.2) with energy $E_{u_{b}}(y)=-b$ which connects (periodically or asymptotically depending on whether the value b is regular or not for V) the set ${\mathcal V}_{-}^{b}$ and ${\mathcal V}_{+}^{b}$.

Both the papers [1] and [2] use minimization arguments and the symmetry assumption ($W_{3}$) is used to obtain compactness in the problem. The existence problem for planar solutions of (1.1) and (1.2) avoiding the use of the symmetry condition ($W_{3}$) was first done by M. Schatzman in [30]. To overcame the difficulties due to lack of compactness, in [30], it is assumed that the set of (geometrically distinct) minimal one-dimensional heteroclinic connections consists of two elements which are supposed to be non-degenerate, i.e., the kernels of the corresponding linearized operators are one dimension. In [30], it is shown that this assumption is generically satisfied by potentials W satisfying ($W_{1}$) and ($W_{2}$).

Precisely, letting $z_{0}$ any smooth function, such that $z_{0}(x)=\mathbf{a}_{+}$ for $x>1$ and $z_{0}(x)=\mathbf{a}_{-}$ for $x<-1$ and defining

$$\begin{aligned} \Gamma =z_{0}+H^{1}({\mathbb {R}}),\quad m=\inf _{\Gamma }V,\quad {\mathcal {M}}=\{q\in \Gamma \mid V(q)=m\}. \end{aligned}$$

It is well known that ($W_{1}$) and ($W_{2}$) are sufficient to guarantee that ${\mathcal {M}}\not =\emptyset$. Then, in [30], it is assumed that

• $(*)$–(i) There exists $z_{-}\not =z_{+}\in \Gamma$ such that

  $$\begin{aligned} {\mathcal {M}}=\{z_{-}(\cdot -t),z_{+}(\cdot -s)\mid t,s\in {\mathbb {R}}\}. \end{aligned}$$

• $(*)$–(ii) The operators $A_{\pm }:H^{2}({\mathbb {R}})^{2}\subset L^{2}({\mathbb {R}})^{2}\rightarrow L^{2}({\mathbb {R}})^{2}$, $A_{\pm }h=-\ddot{h}+D^{2}W(z_{\pm })h$, are such that $\mathrm {Ker}(A_{\pm })=\mathrm {span}\{\dot{z}_{\pm }\}$.

By the discreteness assumption $(*)$–(i), the minimal set ${\mathcal {M}}$ decomposes in the disjoint union of the set of the translated of $z_{-}$ and $z_{+}$:

$$\begin{aligned} {\mathcal {M}}=\mathcal {C}(z_{-})\cup \mathcal {C}(z_{+}) \end{aligned}$$

where we denote $\mathcal {C}(z_{\pm })=\{z_{\pm }(\cdot -s)\mid s\in {\mathbb {R}}\}$. The non-degeneracy assumption $(*)$-(ii) implies, roughly speaking, that in the directions orthogonal to $\mathcal {C}(z_{\pm })$, V has a local quadratic behaviour (see Lemma 2.11 below) which allows to avoid sliding phenomena for the minimizing sequence of the problem along the (non-compact) sets $\mathcal {C}(z_{\pm })$. In [30], it is proved that if $(W_{1})$, $(W_{2})$, and $(*)$ are satisfied (with $W\in C^{3}({\mathbb {R}}^{2})$), then there exists $u\in C^{2}({\mathbb {R}}^{2})^{2}$ solution of (1.1) and (1.2), such that $\lim _{y\rightarrow \pm \infty }u(x,y)=z_{\pm }(x-s_{\pm })$ for certain constants $s_{\pm }\in {\mathbb {R}}$.

The aim of the present paper is to obtain as in [2] energy prescribed solutions in the non-symmetric setting studied in [30]. Indeed by $(*)$-(i), analogously to what happens in the symmetric case, we have that if $\lambda >0$ is sufficiently small and $b\in (m,m+\lambda )$, then

$$\begin{aligned} \{q\in \Gamma \,|\, V(q)\le b \}={\mathcal V}^b_{-}\cup {\mathcal V}^b_{+}\quad \hbox { with } \quad \hbox {dist}_{L^{2}}({\mathcal V}^b_{-} {\mathcal V}^b_{+})>0, \end{aligned}$$

and we prove that there is a solution $v_{b}$ of (1.1), (1.2) with energy $E_{v_{b}}=-b$ which connects in a periodic way the sets ${\mathcal V}^b_{\pm }$. More precisely

Theorem 1.1

Let $W\in {\mathcal {C}}^{2}({\mathbb {R}}^{2})$ be such that ($W_{1}$), ($W_{2}$) and ($*$) are satisfied. Then, there exists $\lambda _{0}>0$, such that for any $b\in (m,m+\lambda _{0})$, there are $v_{b}\in {\mathcal C}^{2}({\mathbb {R}}^{2})^{2}$ and $T_{b}>0$, such that $v_{b}$ solves (1.1)–(1.2) on ${\mathbb {R}}^{2}$, and moreover

• (i) $E_{v_{b}}(y)=\tfrac{1}{2}\Vert \partial _{y} v(\cdot ,y)\Vert _{L^{2}({\mathbb {R}})^{2}}^{2}-V(v(\cdot ,y))=-b$ for all $y\in {\mathbb {R}}$.

• (ii) $v_{b}(\cdot ,0)\in {\mathcal V}^{b}_{-}$, $v_{b}(\cdot ,T_{b})\in {\mathcal V}^{b}_{+}$ (and so $\partial _{y} v(\cdot ,0)=\partial _{y} v(\cdot ,T)=0).$

• (iii) $v_{b}(\cdot ,-y)=v_{b}(\cdot ,y)$ and $v_{b}(\cdot ,y+T)=v_{b}(\cdot ,T-y)$ for any $y\in {\mathbb {R}}$.

• (iv) $V(v_{b}(\cdot ,y))>b$ for $y\in (0,T)$.

Note that the solution $v_{b}$ is a periodic solution of period $2T_{b}$ being symmetric with respect to $y=0$ and $y=T_{b}$. As a trajectory, the function $y\in {\mathbb {R}}\rightarrow v_{b}(\cdot ,y)\in \Gamma$ oscillates back and forth along a simple curve inside the set $\{q\in \Gamma \mid V(q)>b\}$ connecting the two turning points at its boundary $v_{b}(\cdot ,0)\in {\mathcal V}^{b}_{-}$ and $v_{b}(\cdot ,T_{b})\in {\mathcal V}^{b}_{+}$. In the dynamical system language, we can say (see [32]) that $v_{b}$ is a brake orbit solution of (1.1) and (1.2).

To prove Theorem 1.1, we apply an energy constrained variational argument analogous to the one used in [2] (see also [3–6, 9] for different problems in the scalar situation). Given $b\in (m,m+\lambda _{0})$, we look for minima of the renormalized functional

$$\begin{aligned} \varphi (v)=\int _{{\mathbb {R}}} \tfrac{1}{ 2}\Vert \partial _{y} v (\cdot ,y)\Vert ^{2}_{L^{2}({\mathbb {R}})^2}+(V(v(\cdot ,y)) -b)\, \mathrm{d}y \end{aligned}$$

on the class of functions $u\in H^{1}_{loc}({\mathbb {R}}^{2})^{2}$, such that $u(\cdot ,y)\in \Gamma$ for almost every $y\in {\mathbb {R}}$ and which verify the constraint condition:

$$\begin{aligned} \liminf _{y\rightarrow \pm \infty }{\mathrm {dist}}_{L^{2}({\mathbb {R}})^{2}}(u(\cdot ,y),{\mathcal V}^{b}_{\pm })= 0 \quad \hbox { and }\quad \inf _{y\in {\mathbb {R}}}V(u(\cdot ,y))\ge b. \end{aligned}$$

The lack of compactness due to the lack of symmetry in the problem is overcome using ($*$). The quadratic behaviour of the functional V around the sets $\mathcal {C}(z_{\pm })$ allows us to adapt to the present context some arguments developed in [9] to control sliding phenomena constructing a suitably precompact minimizing sequence $(v_{n})$ (see Lemma 3.12). Denoting $\bar{v}$ its weak limit and defining ${\bar{\sigma }}=\sup \{y\in {\mathbb {R}}\, /\, \bar{v}(\cdot ,y)\in {\mathcal V}^{b}_{-}\}$ and ${\bar{\tau }}=\inf \{y>{\bar{\sigma }}\, /\, \bar{v}(\cdot ,y)\in {\mathcal V}^{b}_{+}\}$, we prove that ${\bar{\sigma }}<{\bar{\tau }}\in {\mathbb {R}}$, $\bar{v}(\cdot ,{\bar{\sigma }})\in {\mathcal V}^{b}_{-}$, $\bar{v}(\cdot ,{\bar{\tau }})\in {\mathcal V}^{b}_{+}$ and $V(\bar{v}(\cdot ,y))>b$ for any $y\in ({\bar{\sigma }},{\bar{\tau }})$. From the minimality properties of $\bar{v}$, we recover that $\bar{v}$ solves in a classical sense (1.1)–(1.2) on ${\mathbb {R}}\times ({\bar{\sigma }},{\bar{\tau }})$ and $E_{\bar{v}}(y)=-b$ for any $y\in ({\bar{\sigma }},{\bar{\tau }})$. Then, $\bar{v}$ satisfies the boundary conditions $\lim _{y\rightarrow {\bar{\sigma }}^{+}}\partial _{y}\bar{v}(\cdot ,y)=\lim _{y\rightarrow {\bar{\tau }}^{-}}\partial _{y}\bar{v}(\cdot ,y)=0$, and the solution $v_{b}$ is constructed from $\bar{v}$ by translations, reflections, and periodic continuation.

We conclude with a brief outline of the paper. In Sect. 2, we present a list of preliminary properties of the one-dimensional problem studying in particular some consequences of the assumption (*). In Sect. 3, we introduce our variational framework and prove Theorem 1.1.

Remark 1.1

We precise some consequences of the assumptions $(W_{1})-(W_{2})$, fixing some constants and notation. For all $x\in {\mathbb {R}}^{2}$, we set

$$\begin{aligned} \chi (x)=\min \{|x-\mathbf{a}_{-}|, |x-\mathbf{a}_{+}|\}. \end{aligned}$$

First, we note that since $W\in {\mathcal {C}}^{2}({\mathbb {R}})$ and $D^{2}W(\mathbf{a}_{\pm })$ are definite positive, then

$$\begin{aligned} \forall \, r>0 \exists \,\omega _{r}>0\quad \mathrm{such\, that\, if} \quad \chi (x)\le r \quad \mathrm{then }\quad W(x)\ge \omega _{r}\chi (x)^{2}. \end{aligned}$$

(1.5)

Then, since $W(\mathbf{a}_{\pm })=0$, $DW(\mathbf{a}_{\pm })=0$, and $D^{2}W(\mathbf{a}_{\pm })$ are definite positive, we have that there exists ${\overline{{\delta }}}\in (0,\frac{1}{8})$ two constants ${\overline{w}}>{\underline{w}}>0$, such that if $\chi (x)\le 2\overline{{\delta }}$, then

$$\begin{aligned} 4{\underline{w}}|\xi |^{2}\le D^{2}W(x)\xi \cdot \xi \le 4{\overline{w}}|\xi |^{2} \quad \hbox { for all } \quad \xi \in {\mathbb {R}}^{2}, \end{aligned}$$

(1.6)

and

$$\begin{aligned} {\underline{w}}\chi (x)^{2}\le W(x)\le {\overline{w}}\chi (x)^{2} \quad \hbox { and } \quad |\nabla W(x)|\le 2{\underline{w}}\chi (x). \end{aligned}$$

(1.7)

Finally, given $q_{1}, q_{2}\in L^{2}({\mathbb {R}})^{2}$, we denote $\Vert q_{1}\Vert \equiv \Vert q_{1}\Vert _{L^{2}({\mathbb {R}})^{2}}$, $\langle q_{1}, q_{2}\rangle \equiv \langle q_{1}, q_{2}\rangle _{L^{2}({\mathbb {R}})^{2}}$, and given $A,\,B\subset L^{2}({\mathbb {R}})^{2}$, we denote

$$\begin{aligned} {\mathrm {dist}}(A,B)=\inf \{\Vert q_{1}-q_{2}\Vert \,|\, q_{1}\in A,\, q_{2}\in B\}. \end{aligned}$$

2 The potential functional

Preliminaries. In this section, we recall and list some well-known properties of the functional

$$\begin{aligned} V(q)=\int _{{\mathbb {R}}} \frac{1}{2}|\dot{q}|^{2}+W(q)\, \mathrm{d}t \end{aligned}$$

on the space $\Gamma =z_{0}+H^{1}({\mathbb {R}})^{2}.$ Endowing $\Gamma$ with the Hilbertian structure induced by the map $Q:H^{1}({\mathbb {R}})^{2}\rightarrow \Gamma$, $Q(z)=z_{0}+z$, we have that $V\in {\mathcal {C}}^{2}(\Gamma )$ and that critical points of V are classical solutions to the one-dimensional heteroclinic problem associated to (1.1), that is

$$\begin{aligned} {\left\{ \begin{array}{ll} -\ddot{q}(t)+\nabla W(q(t))=0,&{} t\in {\mathbb {R}},\\ \displaystyle \lim _{t\rightarrow \pm \infty }q(t)=\mathbf{a}_{\pm }.&{} \end{array}\right. } \end{aligned}$$

(2.1)

In particular, we are interested in the minimal properties of V on $\Gamma$ and we set

$$\begin{aligned} m=\inf _{\Gamma }V\quad \hbox {and}\quad {\mathcal {M}}=\{q\in \Gamma \mid V(q)=m\} \end{aligned}$$

More generally, if I is an interval in ${\mathbb {R}}$, we set

$$\begin{aligned} V_{I}(q)=\int _{I}\tfrac{1}{2}|\dot{q}(t)|^{2}+W(q(t))\, \mathrm{d}t, \end{aligned}$$

noting that $V_{I}$ is well defined on $H^{1}_{loc}({\mathbb {R}})^{2}$ with values in $[0,+\infty ]$ for any $I\subset {\mathbb {R}}$.

Note that if $q\in H^{1}_{loc}({\mathbb {R}})^{2}$ is such that $W(q(t))\ge \mu >0$ for all $t\in (\sigma ,\tau )\subset {\mathbb {R}}$, then

$$\begin{aligned} V_{(\sigma ,\tau )}(q) \ge {\textstyle {\frac{1}{ 2(\tau -\sigma )}}}{|q(\tau )-q(\sigma )|}^{2}+ \mu (\tau -\sigma ) \ge \sqrt{2 \mu } \ |q(\tau )-q(\sigma )|. \end{aligned}$$

(2.2)

As consequence, by $(W_{2})$, we obtain

Lemma 2.1

For any $\lambda >0$, there exists $R_{\lambda }>0$, such that if $q\in \Gamma$ and $\Vert q \Vert _{L^{\infty }({\mathbb {R}})^{2}}\ge R_{\lambda }$, then $V(q)\ge m+\lambda$.

Remark 2.2

By Lemma 2.1, we can fix $R_{m}\ge R$, such that if $\Vert q\Vert _{L^{\infty }({\mathbb {R}})^{2}}\ge R_{m}$ and $q\in \Gamma$, then $V(q)\ge 2m$.

By Lemma 2.1, if $(q_{n})\subset \{V\le m+\lambda \}:=\{q\in \Gamma \,/\,V(q)\le m+\lambda \}$, then $\Vert q_{n}\Vert _{L^\infty ({\mathbb {R}})}\le R_{\lambda }$ and $\Vert \dot{q}_{n}\Vert _{L^{2}({\mathbb {R}})^{N}}\le 2(m+\lambda )$ for any $n\in {\mathbb {N}}$. Hence, by the semicontinuity of the $L^{2}$ norm with respect to the weak convergenze and the Fatou Lemma, we recover

Lemma 2.3

Let $(q_{n})\subset \{V\le m+\lambda \}$ for some $\lambda >0$. Then, there exists $q\in H^{1}_{loc}({\mathbb {R}})^2$ with $\Vert q\Vert _{L^\infty ({\mathbb {R}})^2}\le R_{\lambda }$, such that, along a subsequence, $q_{n}\rightarrow q$ in $L^{\infty }_{loc}({\mathbb {R}})^2$, $\dot{q}_{n}\rightarrow \dot{q}$ weakly in $L^{2}({\mathbb {R}})^2$, and moreover, $V(q)\le \liminf _{n\rightarrow \infty }V(q_{n})$

We can strength the result in Lemma 2.3 when $\lambda$ is sufficiently small proving that, in this case, the set $\{V\le m+\lambda \}$ is weakly precompact with respect to the $H^{1}_{loc}({\mathbb {R}})^{2}$ topology. To this aim, observe first that using (2.2) and (1.7), one obtains

Lemma 2.4

For all $\delta \in (0,2\overline{\delta })$ if $q\in \Gamma$, $t_{-}<t_{+}\in {\mathbb {R}}$ are such that $|q(t_{\pm })-\mathbf{a}_{\pm }|=\delta$, then

$$\begin{aligned} V_{(t_{-}, t_{+})}(q)\ge m-\delta ^{2}(1+{2{\overline{w}}}). \end{aligned}$$

Then, fixing $\delta _{0}\in (0,\overline{{\delta }})$ and setting $\mu _{0}=\inf \{ W(\xi )\mid \chi (\xi )\ge {\delta }_{0}\}>0$, we choose a constant:

$$\begin{aligned} \bar{\lambda }\in (0,\min \{ \sqrt{\mu _{0}/2}\,\overline{{\delta }}, \delta _{0}^{2}(1+{2{\overline{w}}})\}). \end{aligned}$$

(2.3)

Moreover, given $q\in \Gamma$, we define

$$\begin{aligned} {\sigma }_{q}=\sup \{t\in {\mathbb {R}}\, /\, |q(t)-\mathbf{a}_{-}|\le {\delta }_{0}\}\hbox { and } \tau _{q}=\inf \{t>{\sigma }_{q}\, /\, |q(t)-\mathbf{a}_{+}|\ge {\delta }_{0}\}. \end{aligned}$$

Since $q\in \Gamma$ and it is continuous, we have ${\sigma }_{q}<\tau _{q}\in {\mathbb {R}}$ and

$$\begin{aligned} |q({\sigma }_{q})-\mathbf{a}_{-}|=|q(\tau _{q})-\mathbf{a}_{+}|={\delta }_{0}\hbox { and }\chi (q(t))>{\delta }_{0}\hbox { for all }t\in ({\sigma }_{q},\tau _{q}). \end{aligned}$$

(2.4)

There results

Lemma 2.5

There exists $L_{0}>0$, such that for every $q\in \{V\le m+\bar{\lambda }\}$, we have

• (i) $\tau _{q}-{\sigma }_{q}\le L_{0}$.

• (ii) If $t<{\sigma }_{q}$, then $|q(t)-\mathbf{a}_{-}|\le 2{\overline{{\delta }}}$, and if $t>\tau _{q}$, then $|q(t)-\mathbf{a}_{+}|\le 2{\overline{{\delta }}}$.

Proof

By (2.2) and (2.4), we have $V_{({\sigma }_{q},\tau _{q})}(q)\ge \mu _{0}(\tau _{q}-{\sigma }_{q})$ and since $V_{({\sigma }_{q},\tau _{q})}(q)\le V(q)\le m+\bar{\lambda }$, (i) follows with $L_{0}=(m+\bar{\lambda })/\mu _{0}$.

To prove (ii), assume by contradiction that there exists ${\sigma }<{\sigma }_{q}$, such that $|q({\sigma })-\mathbf{a}_{-}|>2\overline{\delta }$ or $\tau >\tau _{q}$, such that $|q(\tau )-\mathbf{a}_{+}|>2\overline{\delta }$. In both the cases, there exists an interval $(t_{-},t_{+})\subset {\mathbb {R}}{\setminus }({\sigma }_{q},\tau _{q})$, such that $|\chi (q(t))|\ge {\overline{{\delta }}}$ for any $t\in (t_{-},t_{+})$ and $|q(t_{+})-q(t_{-})|={\overline{{\delta }}}$. Then, $W(q(t))\ge \mu _{0}$ for any $t\in (t_{-},t_{+})$, and hence, by (2.2) and (2.3), $V_{(t_{-},t_{+})}(q)\ge \sqrt{2\mu _{0}}\,\overline{{\delta }}>2\bar{\lambda }$. By Lemma 2.4, we conclude $m+\lambda _{0}\ge V(q)\ge V_{({\sigma }_{q},\tau _{q})}(q) +V_{(x_{-},x_{+})}(q)> m-\delta _{0}^{2}(1+{2{\overline{w}}})+2\bar{\lambda }>m+\bar{\lambda }$, a contradiction which proves (ii). $\square$

The concentration property of the functions $q\in \{ V\le m+\bar{\lambda }\}$ described in Lemma 2.5 allows us to obtain the following compactness result.

Lemma 2.6

Let $(q_{n})\subset \{V\le m+\bar{\lambda }\}$ be such that the sequence $(\sigma _{q_{n}})$ is bounded in ${\mathbb {R}}$. Then, there exists a subsequence $(q_{n_{k}})\subset (q_{n})$ and $q\in \Gamma$, such that $q_{n_{k}}-q\rightarrow 0$ weakly in $H^{1}({\mathbb {R}})^{2}$. Moreover, if $V(q_{n_{k}})\rightarrow V(q)$, then $q_{n_{k}}-q\rightarrow 0$ strongly in ${H^{1}({\mathbb {R}})^{2}}$.

Proof

By Lemma 2.3, there exists a subsequence $(q_{n_{k}})\subset (q_{n})$, $q\in H^{1}_{loc}({\mathbb {R}})^{2}$, such that $\dot{q}\in L^{2}({\mathbb {R}})^{2}$, $\Vert q\Vert _{L^\infty ({\mathbb {R}})}\le R_{\bar{\lambda }}$, $q_{n_{k}}\rightarrow q$ weakly in $H^{1}_{loc}({\mathbb {R}})^{2}$, $\dot{q}_{n_{k}}\rightarrow \dot{q}$ weakly in $L^{2}({\mathbb {R}})^{2}$. For the first part of the lemma, we have to show that $q\in \Gamma$ and that $q_{n_{k}}- q\rightarrow 0$ weakly in $L^{2}({\mathbb {R}})^{2}$.

To this aim note that since the sequence $(\sigma _{q_{n}})$ is bounded in ${\mathbb {R}}$ and $(q_{n})\subset \{V\le m+\bar{\lambda }\}$, by Lemma 2.5, there exists $T_{0}>0$, such that for any $n\in {\mathbb {N}}$

$$\begin{aligned} \hbox {if }t<-T_{0} \hbox { then } |q_{n}(t)-\mathbf{a}_{-}|\le 2{\overline{{\delta }}} \hbox { and if }t>T_{0} \hbox { then }| q_{n}(t)-\mathbf{a}_{+}|\le 2{\overline{{\delta }}}. \end{aligned}$$

(2.5)

By the $L^{\infty }_{loc}$ convergence, we derive

$$\begin{aligned} \hbox {if }t<-T_{0} \hbox { then } |q(t)-\mathbf{a}_{-}|\le 2{\overline{{\delta }}} \hbox { and if }t>T_{0} \hbox { then }| q(t)-\mathbf{a}_{+}|\le 2{\overline{{\delta }}}. \end{aligned}$$

(2.6)

Then, by (1.7) and (2.6), we have

$$\begin{aligned}&\int _{t<-T_{0}}|q-\mathbf{a}_{-}|^{2}\mathrm{d}t=\int _{t<-T_{0}}\chi (q)^{2}\mathrm{d}t\\&\quad \le \tfrac{2}{\underline{w}}\int _{t<-T_{0}}W(q)\mathrm{d}t\le \tfrac{2}{\underline{w}}V(q)\le \tfrac{2}{ \underline{w}}(m+\bar{\lambda }) \end{aligned}$$

and analogously $\int _{t>T_{0}}|q-\mathbf{a}_{+}|^{2}\le \tfrac{2}{\underline{w}}(m+\bar{\lambda })$. Since we already know that $\dot{q}\in L^{2}({\mathbb {R}})^{2}$, this implies that $q-z_{0}\in H^{1}({\mathbb {R}})^{2}$, i.e., $q\in {\Gamma }$.

By (1.7) and (2.5), we obtain also $\int _{|t|>T_{0}}\chi (q_{n})^{2}dt\le \tfrac{4}{ \underline{w}}(m+\bar{\lambda })$ for any $n\in {\mathbb {N}}$, and so, by Lemma 2.1, the sequence $\Vert q_{n}-q\Vert _{L^{2}({\mathbb {R}})^{2}}$ is bounded. This implies, as we claimed, that $q_{n_{k}}-q\rightarrow 0$ weakly in $L^{2}({\mathbb {R}})^{2}$.

To prove the second part of the lemma, assume $V(q_{n_{k}})\rightarrow V(q)$. Since $q_{n_{k}}\rightarrow q$ in $L^\infty _{loc}({\mathbb {R}})$ and $\dot{q}_{n_{k}}\rightarrow \dot{q}$ weakly in $L^2({\mathbb {R}})$, given any $T\ge T_{0}$, we have

$$\begin{aligned} V(q_{n_{k}})-V(q)= \frac{1}{2}\Vert \dot{q}_{n_{k}}-\dot{q}\Vert ^{2}+\int _{|t|>T}W(q_{n_{k}})- W(q)\, \mathrm{d}t+o(1), \quad \hbox { as }\quad k\rightarrow \infty \end{aligned}$$

and since $V(q_{n_{k}})\rightarrow V(q)$, we derive

$$\begin{aligned} \frac{1}{2}\Vert \dot{q}_{n_{k}}-\dot{q}\Vert ^{2}+\smallint _{|t|>T}W(q_{n_{k}})\, \mathrm{d}t=\int _{|t|>T} W(q)\, \mathrm{d}t+o(1), \quad \hbox { as } \quad k\rightarrow \infty . \end{aligned}$$

(2.7)

By (1.7) and (2.5), we have $W(q_{n_{k}}(t))\ge {{\underline{w}}}\chi (q_{n_{k}}(x))^{2}$ for $|t|\ge T_{0}$, and so, by (2.7)

$$\begin{aligned} \frac{1}{2}\Vert \dot{q}_{n_{k}}-\dot{q}\Vert ^{2}+ {{\underline{w}}}\int _{|t|>T}\chi (q_{n_{k}})^{2}\, \mathrm{d}t\le \int _{|t|\ge T}W(q)\, \mathrm{d}t+o(1),\quad \hbox { as } \quad k\rightarrow \infty . \end{aligned}$$

(2.8)

By (2.5), for $|t|\ge T_{0}$, we have $|q_{n_{k}}(t)-q(t)|^{2}\le 2(\chi (q_{n_{k}}(t))^{2}+\chi (q(t))^{2})$. Since for any $\eta >0$, we can choose $T_{\eta }\ge T_{0}$, such that $\int _{|t|>T_{\eta }}W(q)\, dt+ {{\underline{w}}}\int _{|t|>T_{\eta }}\chi ( q)^{2}\, \mathrm{d}t<\eta /2$, by (2.8), we finally obtain

$$\begin{aligned} \frac{1}{2}\Vert \dot{q}_{n_{k}}-\dot{q}\Vert ^{2}+ {{\underline{w}}}\int _{|t|>T_{\eta }}|q_{n_{k}}- q|^{2}\mathrm{d}t\le \eta +o(1) \quad \hbox { as }\quad k\rightarrow +\infty . \end{aligned}$$

Since $\eta$ is arbitrary and $q_{n_{k}}\rightarrow q$ in $L^\infty _{loc}({\mathbb {R}})^{2}$, we conclude $\Vert q_{n_{k}}- q\Vert _{H^{1}({\mathbb {R}})^{2}}\rightarrow 0$ as $k\rightarrow \infty$ and the lemma is proved. $\square$

By Lemma 2.6, we derive in particular the compactness of the minimizing sequences of V in ${\Gamma }$.

Lemma 2.7

Let $(q_{n})\subset {\Gamma }$ be such that $V(q_{n})\rightarrow m$. Then, there exists $q\in {\mathcal {M}}$, such that, along a subsequence, $\Vert q_{n}(\cdot +\sigma _{q_{n}})-q\Vert _{H^{1}({\mathbb {R}})^{2}}\rightarrow 0$ as $n\rightarrow \infty$.

Remark 2.8

Lemma 2.7 readily implies the following property: for any $r>0$, there exists $\lambda _{r}>0$, such that

$$\begin{aligned} \hbox {if } \inf _{\bar{q}\in {\mathcal {M}}}\Vert q-\bar{q}\Vert _{H^{1}({\mathbb {R}})^{2}}\ge r \quad \hbox { then } \quad V(q)\ge m+\lambda _{r}. \end{aligned}$$

(2.9)

Consequences of the assumption $(*)$. Recall the assumption:

• $(*)$–(i) There exists $z_{-}\not = z_{+}\in \Gamma$, such that

  $$\begin{aligned} {\mathcal {M}}=\{z_{-}(\cdot -t),z_{+}(\cdot -s)\mid t,s\in {\mathbb {R}}\}. \end{aligned}$$

• $(*)$–(ii) The operators $A_{\pm }:H^{2}({\mathbb {R}})^{2}\subset L^{2}({\mathbb {R}})^{2}\rightarrow L^{2}({\mathbb {R}})^{2}$, $A_{\pm }h=-\ddot{h}+D^{2}W(z_{\pm })h$ are such that $\mathrm {Ker}(A_{\pm })=\mathrm {span}\{\dot{z}_{\pm }\}$.

Here, below z will denote any one of the functions $z_{\pm }$, and A the corresponding operator. Since z is a minimum for V on $\Gamma$, we have $V''(z)hh\ge 0$ for any $h\in H^{1}({\mathbb {R}})^{2}$; since $V''(z)hk=\int _{{\mathbb {R}}}\dot{h}\cdot \dot{k}+W''(z)hk\, \mathrm{d}x=\langle Ah,k\rangle$ for any $h,k \in H^{2}({\mathbb {R}})^{2}$, we derive that A is a positive self-adjoint operator. The assumption $(*)$ implies, moreover, the following.

Lemma 2.9

There exists $\bar{\mu }>0$, such that

$$\begin{aligned} V''(z)hh\ge \bar{\mu }\Vert h\Vert _{H^{1}({\mathbb {R}})^{2}}^{2},\quad \forall \,h\in H^{1}({\mathbb {R}})^{2}\ /\ \langle h,\dot{z}\rangle =0. \end{aligned}$$

Proof

Let $w_{0}$ be the minimum of the lowest eigenvalues of $D^{2}W(\mathbf{a}_{-})$ and $D^{2}W(\mathbf{a}_{+})$, then the essential spectrum of A is $[w_{0},+\infty )$. Since $\mathrm {Ker}(A)=\mathrm {span}\{\dot{z}\}$, we have that 0 is a simple eigenvalue of A, whose eigenspace is $\mathrm {span}\{\dot{z}\}$, and since we already know that $\langle A h ,h\rangle =V''(z)hh\ge 0$ for any $h\in H^{2}({\mathbb {R}})^{2}$, 0 is the minimum eigenvalue of A. By the min–max eigenvalue characterization ([28], Theorem XIII.1), we have $\sigma (A)\cap (-\infty ,w_{0}]=\{{0}\le \mu _{1}\le \ldots \}$, where

$$\begin{aligned} \mu _{j}=\sup _{X\subset H^{2}({\mathbb {R}}), \mathrm{dim} X=j}\inf _{\psi \bot X,\ \Vert \psi \Vert =1}\langle A\psi ,\psi \rangle \end{aligned}$$

We have that $\mu _{1}$ is either equal to $w_{0}$ or strictly less than it. In any case, since 0 is a simple eigenvalue, we obtain that $\mu _{1}>0$. If $h\in H^{2}({\mathbb {R}})^{2}$ is such that $h\bot \dot{z}$, using, e.g., the resolution of the identity relative to A, we obtain $V''(z)hh=\langle Ah,h\rangle \ge \mu _{1}\Vert h\Vert ^{2}$ for any $h\in H^{2}({\mathbb {R}})^{2}$ such that $h\bot \dot{z}$. By density

$$\begin{aligned} V''(z)hh\ge \mu _{1}\Vert h\Vert ^{2}\quad \hbox { for any } \quad h\in H^{1}({\mathbb {R}})^{2}\quad \hbox { such that } \quad h\bot \dot{z}. \end{aligned}$$

(2.10)

To conclude the proof, setting $\omega =\max _{t\in {\mathbb {R}}}|D^{2}W(z(t))|$, we note that if $h\in H^{1}({\mathbb {R}})^{2}$ is such that $\langle h,\dot{z}\rangle =0$, then

$$\begin{aligned} \int _{{\mathbb {R}}} |\nabla h|^{2}+ D^{2}W(z)hh \mathrm{d}t \ge \mu _{1}\Vert h\Vert \ge -\mu _{1} \int _{{\mathbb {R}}}\tfrac{D^{2}W(z)}{\omega }hh\mathrm{d}t. \end{aligned}$$

Hence, $\int _{{\mathbb {R}}} |\nabla h|^{2}+ D^{2}W(z)hh \mathrm{d}t \ge \frac{\mu _{1}}{\omega +\mu _{1}}\Vert \nabla h\Vert ^{2}$ and the Lemma follows.

$\square$

We now set

$$\begin{aligned} \mathcal {C}(z)=\{ z(\cdot -s)\mid s\in {\mathbb {R}}\}. \end{aligned}$$

Note that the functions $z-\mathbf{a}_{\pm }$, $\dot{z}$, $\ddot{z}$, and $\dddot{z}$ are continuous on ${\mathbb {R}}$ and, by $(W_{1})$, converge exponentially to 0 as $t\rightarrow \pm \infty$. Then, $\dot{z}\in H^{2}({\mathbb {R}})^{2}$. In particular, the function $s\in {\mathbb {R}}\mapsto z(\cdot -s)\in \Gamma$ is $C^{2}$ with respect to the $H^{1}$ topology on $\Gamma$ and $\tfrac{d}{ds}z(\cdot -s)= -\dot{z}(\cdot -s)$ and $\tfrac{d^{2}}{dm^{2}}z(\cdot -s)=\ddot{z}(\cdot -s)$. We have

Lemma 2.10

There exists $\bar{r}\in (0,1)$, such that if $q\in \Gamma$ and ${\mathrm {dist}}(q,\mathcal {C}(z))\le \bar{r}$, then there is a unique $\zeta _{q}\in {\mathbb {R}}$ verifying $\Vert q-z(\cdot -\zeta _{q})\Vert ={\mathrm {dist}}( q,\mathcal {C}(z))$. Moreover

$$\begin{aligned} \langle q-z(\cdot -\zeta _{q}), \dot{z}(\cdot -\zeta _{q})\rangle =0. \end{aligned}$$

Proof

We set $c_{1}=\Vert \dot{z}\Vert$, $c_{2}=\Vert \ddot{z}\Vert$, $c_{3}=\max _{{\mathbb {R}}}|D^{2}W(z(t))|$, and let $\rho (\eta )=\inf \{\Vert z-z(\cdot -s)\Vert \mid |s|\ge \eta \}$ for $\eta \ge 0$. Clearly, $\rho (0)=0$, $0<\rho (\eta _{1})<\rho (\eta _{2})$ whenever $0<\eta _{1}<\eta _{2}$ and $\rho (\eta )\rightarrow +\infty$ as $\eta \rightarrow +\infty$. Moreover, $\Vert z(\cdot -s_{1})-z(\cdot -s_{2})\Vert \ge \rho (|s_{1}-s_{2}|)$ for any $s_{1}, s_{2}\in {\mathbb {R}}$. Let

$$\begin{aligned} \eta _{0}=\min \left\{ \tfrac{1}{2c_{1}}, \tfrac{c_{1}}{c_{3}}\right\} \quad \hbox { and } \quad 2\bar{r}=\min \left\{ 1, \tfrac{c_{1}^{2}}{c_{2}}, \rho (\eta _{0})\right\} \end{aligned}$$

and let $q\in \Gamma$ be such that ${\mathrm {dist}}(q,\mathcal {C}(z))\le \bar{r}$. Since the function $s\rightarrow \Vert q-z(\cdot -s)\Vert ^{2}$ is continuous and tends to $+\infty$ as $s\rightarrow \pm \infty$, we derive that there exists $\zeta _{q}\in {\mathbb {R}}$, such that $\Vert q-z(\cdot -\zeta _{q})\Vert ={\mathrm {dist}}( q,\mathcal {C}(z))$. We have

$$\begin{aligned} \tfrac{d}{ds}\Vert q-z(\cdot -\zeta _{q})\Vert ^{2}&=2\langle q-z(\cdot -\zeta _{q}),\dot{z}(\cdot -\zeta _{q})\rangle =0\\ \tfrac{d^{2}}{ds^{2}}\Vert q-z(\cdot -\zeta _{q})\Vert ^{2}&=2\Vert \dot{z}\Vert ^{2}-2\langle q-z(\cdot -\zeta _{q}),\ddot{z}(\cdot -\zeta _{q})\rangle \ge 2 c_{1}^{2}-2c_{2}\bar{r}\ge c_{1}^{2}. \end{aligned}$$

Moreover, if $s\in {\mathbb {R}}$, since $\ddot{z}=\nabla W(z)$ and $\langle \dot{z},\ddot{z}\rangle =0$, we have

$$\begin{aligned} |\nonumber \tfrac{\mathrm{d}^{3}}{\mathrm{d}s^{3}}\Vert q-z(\cdot -s)\Vert ^{2}|&=2|\langle q-z(\cdot -s),D^{2}W(z(\cdot -s))\dot{z}(\cdot -s)\rangle |\\&\le 2c_{1}c_{3}\Vert q-z(\cdot -s)\Vert . \end{aligned}$$

(2.11)

Let $\bar{s}\in {\mathbb {R}}$ be such that $\Vert q-z(\cdot -\bar{s})\Vert =\Vert q-z(\cdot - \zeta _{q})\Vert$. Clearly, $\Vert z(\cdot -\bar{s})-z(\cdot -\zeta _{q})\Vert \le 2 \bar{r}$, and so, since $2\bar{r}\le \rho (\eta _{0})$, we have $|\bar{s}-\zeta _{q}|\le \eta _{0}$. Then, since $z(\cdot -s)=z(\cdot -\zeta _{q})-\int _{\zeta _{q}}^{s}\dot{z}(\cdot -t)\, dt$, we derive that for s between $\bar{s}$ and $\zeta _{q}$, we have $\Vert z(\cdot -s)-z(\cdot -\zeta _{q})\Vert \le c_{1}|\bar{s}-\zeta _{q}|\le \eta _{0}c_{1}$, and so

$$\begin{aligned} \Vert q-z(\cdot -s)\Vert \le {\mathrm {dist}}(q,\mathcal {C}(z))+\eta _{0}c_{1}\le \bar{r}+\eta _{0}c_{1}. \end{aligned}$$

By (2.11), we obtain that for any s between $\bar{s}$ and $\zeta _{q}$, we have

$$\begin{aligned} |\tfrac{\mathrm{d}^{3}}{\mathrm{d}s^{3}}\Vert q-z(\cdot -s)\Vert ^{2}|\le 2(\bar{r}+\eta _{0}c_{1})c_{3}c_{1} \end{aligned}$$

and by the Taylor Formula and the choice of $\eta _0$ and $\bar{r}$, we obtain

$$\begin{aligned} \Vert q-z(\cdot -\bar{s})\Vert ^{2}&\ge {\mathrm {dist}}(q,\mathcal {C}(z))^{2}+\tfrac{c_{1}^{2}}{2} |\bar{s}-\zeta _{q}|^{2}- \tfrac{1}{3}(\bar{r}+\eta _{0}c_{1})c_{3}c_{1}\eta _{0}|\bar{s}-\zeta _{q}|^{2}\\&\ge {\mathrm {dist}}(q,\mathcal {C}(z))^{2}+\tfrac{c_{1}^{2}}{6} |\bar{s}-\zeta _{q}|^{2} \end{aligned}$$

which shows that $\bar{s}=\zeta _{q}$. $\square$

By Lemma 2.10 we can uniquely associate to any $q\in \Gamma$, such that ${\mathrm {dist}}(q,\mathcal {C}(z))\le \bar{r}$, the nearest point $z(\cdot -\zeta _{q})$ in $\mathcal {C}(z)$ which, for the sake of brevity in the notation, we will denote from now on with $z_{q}$. Using Lemma 2.9, we can further characterize the behaviour of V in a suitable $H^{1}$-neighborhood of $\mathcal {C}(z)$ in $\Gamma$.

Lemma 2.11

There exists $r_{0}\in (0,\bar{r})$, such that if $q\in \Gamma$ and ${\mathrm {dist}}_{H^{1}({\mathbb {R}})^{2}}(q,\mathcal {C}(z))\le r_{0}$, then

$$\begin{aligned} \tfrac{d^{2}}{ds^{2}}V(z_{q}+s(q-z_{q}))\ge \tfrac{\bar{\mu }}{2}\Vert q-z_{q}\Vert _{H^{1}({\mathbb {R}})^{2}}^{2}\quad \hbox { for any } \quad s\in [0,1]. \end{aligned}$$

Proof

Set $\bar{W}=\sup _{|\xi |\le \Vert z\Vert _{\infty }}|D^{3}W(\xi )|$. We claim that there exists $r_{0}\in (0,\bar{r})$, such that if ${\mathrm {dist}}_{H^{1}({\mathbb {R}})^{2}}(q,\mathcal {C}(z))\le r_{0}$, then

$$\begin{aligned} \sup _{t\in {\mathbb {R}}}|q(t)-z_{q}(t)|\le \tfrac{\bar{\mu }}{2\bar{W}}. \end{aligned}$$

(2.12)

Indeed, let us assume by contradiction that there exists $(q_{j})\subset \Gamma$ and $(s_{j})\in {\mathbb {R}}$, such that $\Vert q_{j}-z(\cdot -s_{j})\Vert _{H^{1}({\mathbb {R}})^{2}}\rightarrow 0$ as $j\rightarrow +\infty$ and $\Vert q_{j}-z_{q_{j}}\Vert _{L^{\infty }({\mathbb {R}})^{2}}> \tfrac{\bar{\mu }}{2\bar{W}}$ for any $j\in {\mathbb {N}}$. Then, $q_{j}(\cdot +s_{j})-z\rightarrow 0$ in $H^{1}({\mathbb {R}})^{2}$, and since $\Vert q_{j}-z_{q_{j}}\Vert \le \Vert q_{j}-z(\cdot -s_{j})\Vert _{H^{1}({\mathbb {R}})^{2}}\rightarrow 0$, we derive that $z_{q_{j}}(\cdot +s_{j})-z=z(\cdot -\zeta _{q_{j}}+s_{j})-z\rightarrow 0$ in $L^{2}({\mathbb {R}})^{2}$. This implies $\zeta _{q_{j}}-s_{j}\rightarrow 0$ and consequently $z_{q_{j}}(\cdot +s_{j})-z\rightarrow 0$ in $H^{1}({\mathbb {R}})^{2}$. Hence

$$\begin{aligned}&\Vert q_{j}-z_{q_{j}}\Vert _{H^{1}({\mathbb {R}})^{2}}\le \Vert q_{j}-z(\cdot -s_{j})\Vert _{H^{1}({\mathbb {R}})^{2}}+\Vert z_{q_{j}}-z(\cdot -s_{j})\Vert _{H^{1}({\mathbb {R}})^{2}}\rightarrow 0 \\&\quad \hbox { as } \quad j\rightarrow +\infty \end{aligned}$$

in contradiction with the assumption $\Vert q_{j}-z_{q_{j}}\Vert _{L^{\infty }({\mathbb {R}})^{2}}> \tfrac{\bar{\mu }}{2\bar{W}}$ for any $j\in {\mathbb {N}}$.

Now note that for any $s\in [0,1]$, we have $|D^{2}W(z_{q}+s(q-z_{q}))-D^{2}(z_{q})|\le \bar{W}|q-z_{q}|$, and so by (2.12)

$$\begin{aligned} |(V''(z_{q}+s(q-z_{q}))-V''(z_{q}))(q-z_{q})(q-z_{q})|\le \tfrac{\bar{\mu }}{2}\Vert q-z_{q}\Vert ^{2}. \end{aligned}$$

Since by Lemma 2.10, we have $(q-z_{q})\,\bot \, \dot{z}_{q}$, by Lemma 2.9, we derive that for any $s\in (0,1)$, we have

$$\begin{aligned} \tfrac{\mathrm{d}^{2}}{\mathrm{d}s^{2}}V(z_{q}\!+\!s(q-z_{q}))\!=\!V''(z_{q}+s(q-z_{q}))(q-z_{q})(q-z_{q})\!\ge \! \tfrac{\bar{\mu }}{2}\Vert q-z_{q}\Vert _{H^{1}({\mathbb {R}})^{2}}^{2}. \end{aligned}$$

$\square$

Remark 2.12

By Lemma 2.11, we recover that if $q\in \Gamma$ and ${\mathrm {dist}}_{H^{1}({\mathbb {R}})^{2}}(q,\mathcal {C}(z))\le r_{0}$, then

$$\begin{aligned} V'(q)(q-z_{q})=\int _{0}^{1}\tfrac{\mathrm{d}^{2}}{\mathrm{d}s^{2}}V(z_{q}+s(q-z_{q}))\, \mathrm{d}s\ge \tfrac{\bar{\mu }}{2}\Vert q-z_{q}\Vert _{H^{1}({\mathbb {R}})^{2}}^{2}. \end{aligned}$$

Lemma 2.11 holds true both for $z=z_{-}$ or $z=z_{+}$ and we can assume that this occurs for the same value of $r_{0}$. In particular, denoting $N_{r_{0}}(\mathcal {C}(z))=\{q\in H^{1}({\mathbb {R}})^{2}\mid {\mathrm {dist}}_{H^{1}({\mathbb {R}})^{2}}(q,\mathcal {C}(z))< r_{0}\}$, we have that $N_{r_{0}}(\mathcal {C}(z_{-}))\cap N_{r_{0}}(\mathcal {C}(z_{+}))=\emptyset$. Considering $r_{0}$ smaller, if necessary, we can, furthermore, assume that

$$\begin{aligned} {\mathrm {dist}}(N_{r_{0}}(\mathcal {C}(z_{-})), N_{r_{0}}(\mathcal {C}(z_{+})))\ge 5r_{0}. \end{aligned}$$

(2.13)

By Remark 2.8, we can fix $\lambda _{0}\le \min \{\bar{\lambda },m\}$ ($\bar{\lambda }$ given by (2.3)), such that

$$\begin{aligned} \hbox {if }V(q)\le m+\lambda _{0} \quad \hbox { then } \quad q\in N_{r_{0}}(\mathcal {C}(z_{-}))\cup N_{r_{0}}(\mathcal {C}(z_{+})). \end{aligned}$$

(2.14)

For any $b\in (m,m+\lambda _{0})$, we then have that $\{V\le b\}=\mathcal {V}_{-}^{b}\cup \mathcal {V}_{+}^{b}$, where

$$\begin{aligned} \mathcal {V}_{-}^{b}=\{V\le b\}\cap N_{r_{0}}(\mathcal {C}(z_{-})) \quad \hbox { and } \quad \mathcal {V}_{+}^{b}=\{V\le b\}\cap N_{r_{0}}(\mathcal {C}(z_{+})). \end{aligned}$$

The set $\mathcal {V}_{\pm }^{b}$ is invariant with respect to the action of the group of translations and it is not weakly closed. The following lemma states that it is ”locally” weakly closed

Lemma 2.13

If $(q_{n})\subset \mathcal {V}_{\pm }^{b}$ is such that $(\sigma _{q_{n}})$ is bounded, then there exists $q\in \mathcal {V}_{\pm }^{b}$, such that, along a subsequence, $q_{n}\rightarrow q$ weakly in $H^{1}_{loc}({\mathbb {R}})^{2}$.

Proof

Let $(q_{n})\subset \mathcal {V}_{-}^{b}$ be such that $(\sigma _{q_{n}})$ is bounded. By Lemma 2.6, there exists $q\in \Gamma$, such that, along a subsequence, $q_{n}\rightarrow q$ weakly in $H^{1}_{loc}({\mathbb {R}})^{2}$ and $V(q)\le b$. Since ${\mathrm {dist}}_{H^{1}({\mathbb {R}})^{2}}(q_{n},\mathcal {C}(z_{-}))<r_{0}$, there exists $s_{n}$, such that $\Vert q_{n}-z_{-}(\cdot -s_{n})\Vert \le r_{0}$. Since $(\sigma _{q_{n}})$ is bounded, by Lemma 2.5, we recognize that also $(s_{n})$ is bounded and so convergent to $s_{0}\in {\mathbb {R}}$ up to a subsequence. Then, $z_{-}(\cdot -s_{n})-z_{-}(\cdot -s_{0})\rightarrow 0$ in $H^{1}({\mathbb {R}})^{2}$, and by semicontinuity, we conclude $\Vert q-z_{-}(\cdot -s_{0})\Vert _{H^{1}({\mathbb {R}})^{2}}\le \liminf \Vert q_{n}-z_{-}(\cdot -s_{n})\Vert _{H^{1}({\mathbb {R}})^{2}}\le r_{0}$, which implies that $q\in \mathcal {V}_{-}^{b}$. The case $(q_{n})\subset \mathcal {V}_{+}^{b}$ is analogous. $\square$

Remark 2.14

By Lemma 2.13, we obtain in particular that if $(q_{n})\subset \mathcal {V}_{\pm }^{b}$ is bounded in $\Gamma$ with respect to the $L^{2}({\mathbb {R}})^{2}$ metric, since this implies that $(\sigma _{q_{n}})$ is bounded in ${\mathbb {R}}$, there exists $q\in \mathcal {V}^{b}_{\pm }$, such that along a subsequence, $q_{n}\rightarrow q$ weakly in $H^{1}_{loc}({\mathbb {R}})^{2}$.

Remark 2.15

Since ${\mathcal {M}}={\mathcal {C}}(z_{-})\cup {\mathcal {C}}(z_{+})$, we easily recognize that if $(q_{n})\subset \Gamma$ and ${\mathrm {dist}}_{H^{1}({\mathbb {R}})^{2}}(q_{n},{\mathcal {M}})\rightarrow 0$ then $V(q_{n})\rightarrow m$. Equivalently, we can say that for any $b>m$ there exists $r_{b}>0$, such that if $V(q)\ge b$ then ${\mathrm {dist}}_{H^{1}({\mathbb {R}})^{2}}(q,{\mathcal {M}})\ge r_{b}$. In particular, by Remark 2.12, we derive that for any $b\in (m,m+\lambda _{0})$, we have

$$\begin{aligned} \inf _{q\in \mathcal {V}_{\pm }^{m+\lambda _{0}}{\setminus } \mathcal {V}_{\pm }^{b}}V'(q)(q-z_{q})\ge \frac{\bar{\mu }\, r_{b}^{2}}{4}\equiv \nu (b)>0. \end{aligned}$$

(2.15)

3 Planar solutions

The variational setting. We denote $S(y_{1},y_{2}):={\mathbb {R}}\times (y_{1},y_{2})$ for $(y_{1},y_{2})\subset {\mathbb {R}}$ and, more simply, $S_{L}:=S(-L,L)$ for $L>0$. We consider the space

$$\begin{aligned} {\mathcal {H}}= z_{0}+\cap _{L>0}H^{1}(S_{L})^{2}. \end{aligned}$$

Note that, if $v\in {\mathcal {H}}$, then $v(\cdot ,y)\in \Gamma$ for a.e. $y\in {\mathbb {R}}$. Moreover

$$\begin{aligned} \int _{{\mathbb {R}}}|v(x,y_{2})-v(x, y_{1})|^{2}\, \mathrm{d}x \le |y_{2}-y_{1}|\int _{{\mathbb {R}}} \int _{y_{1}}^{y_{2}}|\partial _{y}v(x,y)|^{2}\,\mathrm{d}y\mathrm{d}x \end{aligned}$$

and so, any $v\in {\mathcal {H}}$ verifies the continuity property

$$\begin{aligned} \Vert v(\cdot , y_{2})-v(\cdot , y_{1})\Vert ^{2} \le \Vert \partial _{y} v\Vert ^{2}_{L^{2} (S{(y_{1},y_{2})})} |y_{2}-y_{1}|,\quad \forall \, (y_{1},y_{2})\subset {\mathbb {R}}. \end{aligned}$$

(3.1)

Considering the functional $V$ extended on $z_{0}+L^{2}({\mathbb {R}})^{2}$ as

$$\begin{aligned} V(u)={\left\{ \begin{array}{ll} V(u), &{}\hbox {if } u\in \Gamma , \\ +\infty , &{}\hbox {if } u\in z_{0}+L^{2}({\mathbb {R}})^{2}{\setminus } H^{1}({\mathbb {R}})^{2}, \end{array}\right. } \end{aligned}$$

we have

Lemma 3.1

If $v\in {\mathcal {H}}$ then the function $y\in {\mathbb {R}}\mapsto V(v(\cdot ,y))\in {\mathbb {R}}\cup \{+\infty \}$ is lower semicontinuous.

Proof

Let $y_{n}\rightarrow y_{0}\in {\mathbb {R}}$ be such that $\liminf _{y\rightarrow y_{0}}V(v(\cdot ,y))=\lim _{n\rightarrow +\infty }V(v(\cdot ,y_{n}))$. By (3.1), we have $v(\cdot ,y_{n})- v(\cdot ,y_{0})\rightarrow 0$ in $L^{2}({\mathbb {R}})^{2}$. Up to subsequences, we have either: $\hbox { (a) }\sup _{n\in {\mathbb {N}}}\Vert \partial _{x}v(\cdot ,y_{n})\Vert <+\infty$ or $\hbox {(b)}\lim _{n\rightarrow +\infty }\Vert \partial _{x}v(\cdot ,y_{n})\Vert =+\infty$. In the case (a), we have $v(\cdot ,y_{n})- v(\cdot ,y_{0})\rightarrow 0$ weakly in $H^{1}({\mathbb {R}})^{2}$ and by semicontinuity $\lim _{n\rightarrow +\infty }V(v(\cdot ,y_{n}))\ge V(v(\cdot ,y_{0}))$. If (b) occurs, then $\lim _{n\rightarrow +\infty }V(v(\cdot ,y_{n}))=+\infty$, and the Lemma follows. $\square$

Fixed any $b\in (m,m+\lambda _{0})$, we consider the subspace of ${\mathcal H}$

$$\begin{aligned} {\mathcal H}_{b}=\{ v\in {\mathcal {H}}\, /\, \liminf _{y\rightarrow \pm \infty }\hbox {dist}(v(\cdot ,y),{\mathcal V}^{b}_{\pm })= 0 \quad \hbox { and } \quad \inf _{y\in {\mathbb {R}}}V(v(\cdot ,y))\ge b\} \end{aligned}$$

on which we look for minima of the functional

$$\begin{aligned} {\varphi }(v)=\int _{{\mathbb {R}}} \tfrac{1}{2}\Vert \partial _{y}v(\cdot ,y)\Vert ^{2}+ (V(v(\cdot ,y))-b) \,\mathrm{d}y. \end{aligned}$$

Remark 3.2

Note that, if $v\in {\mathcal H}_{b}$, then $V(v(\cdot ,y))\ge b$ for every $y\in {\mathbb {R}}$, and so ${\varphi }$ is well defined and non-negative on ${\mathcal H}_{b}$. Moreover, we plainly recognize that ${\mathcal H}_{b}\not =\emptyset$ and $m_{b}=\inf _{v\in {\mathcal H}_{b}}{\varphi }(v)<+\infty .$

Remark 3.3

More generally, given an interval $I\subset {\mathbb {R}}$, we consider the functional

$$\begin{aligned} {\varphi }_{I}(v)=\int _{I}\tfrac{1}{2}\Vert \partial _{y}v(\cdot ,y)\Vert ^{2}+ V(v(\cdot ,y))-b \,\mathrm{d}y \end{aligned}$$

which is well defined for any $v\in {\mathcal {H}}$, such that $V(v(\cdot ,y))\ge b$ for a.e. $y\in I$ or for every $v\in {\mathcal {H}}$ if I is bounded.

We will make use of the following immediate semicontinuity property of ${\varphi }_{I}$.

Lemma 3.4

Let $v\in {\mathcal {H}}$ be such that $V(v(\cdot ,y))\ge b$ for a.e. $y\in I\subset {\mathbb {R}}$. If $(v_{n})\subset {\mathcal H}_{b}$ is such that $v_{n}\rightarrow v$ weakly in $H^{1}(S_{L})$ for any $L>0$, then ${\varphi }_{I}(v)\le \displaystyle {\liminf _{n\rightarrow \infty }} {\varphi }_{I}(v_{n})$.

Remark 3.5

Concerning coerciveness properties of ${\varphi }$, it is important to display the following simple estimate. Given $v\in {\mathcal {H}}$ and $(y_{1},y_{2})\subset {\mathbb {R}}$, we have

$$\begin{aligned} {\varphi }_{(y_{1},y_{2})}(v)&= \tfrac{1}{2} \int _{y_{1}}^{y_{2}} \Vert \partial _{y}v(\cdot ,y)\Vert _{2}^{2}\,\mathrm{d}y +\int _{y_{1}}^{y_{2}}V(v(\cdot ,y))-b\, \mathrm{d}y\\&\ge \tfrac{1}{ 2(y_{2}-y_{1})}\int _{{\mathbb {R}}^{2}}(\int _{y_{1}}^{y_{2}} |\partial _{y}v(x,y)|\, \mathrm{d}y)^{2}\, \mathrm{d}x+\int _{y_{1}}^{y_{2}}V(v(\cdot ,y))-b\, \mathrm{d}y\\&\ge \tfrac{1}{2(y_{2}-y_{1})} \Vert v(\cdot ,y_{1})-v(\cdot ,y_{2})\Vert ^{2}+\int _{y_{1}}^{y_{2}}V(v(\cdot ,y))-b\, \mathrm{d}y. \end{aligned}$$

In particular, if $V(v(\cdot ,y))\ge b+\nu >b$ for any $y\in (y_{1},y_{2})$, then

$$\begin{aligned} {\varphi }_{(y_{1},y_{2})}(v) \!\ge \!\tfrac{1}{2(y_{2}-y_{1})} \Vert v(\cdot ,y_{1})-v(\cdot ,y_{2})\Vert ^{2}+\nu (y_{2}-y_{1}) \!\ge \! \sqrt{2\nu }\,\Vert v(\cdot ,y_{1})-v(\cdot ,y_{2})\Vert . \end{aligned}$$

(3.2)

Remark 3.6

By (2.13), (2.14), and (3.1), if $v\in {\mathcal H}_{b}$, there exist $y_{1}<y_{2}\in {\mathbb {R}}$, such that $\Vert v(\cdot ,y_{1})-v(\cdot ,y_{2})\Vert \ge 4r_{0}$ and $V(v(\cdot ,y))>m+\lambda _{0}$ for any $y\in (y_{1},y_{2})$. Then, by (3.2), we obtain ${\varphi }_{(y_{1},y_{2})}(u)\ge 4\sqrt{m+\lambda _{0}-b}\,r_{0}>0$. In particular

$$\begin{aligned} m_{b}\ge 4r_{0}\sqrt{m+\lambda _{0}-b}. \end{aligned}$$

Estimates around ${\mathcal V}^{b}_{-}$ and ${\mathcal V}_{+}^{b}$. The study of the coerciveness properties of ${\varphi }$ needs some local results. Given $b\in (m,m+\lambda _{0})$, we define the constants

$$\begin{aligned} {\beta }=b+ \tfrac{m+\lambda _{0}-b}{4},\ \ \hbox { and }\ \Lambda _{0}=\sqrt{\tfrac{m+\lambda _{0}-b}{2}}\, \tfrac{r_{0}}{4} \end{aligned}$$

(3.3)

where $\lambda _{0}$ and $r_{0}$ are defined by (2.13) and (2.14), noting that

$$\begin{aligned} \mathrm {dist}({\mathcal V}^{b}_{-},{\mathcal V}^{b}_{+})\ge \mathrm {dist}({\mathcal V}^{{\beta }}_{-},{\mathcal V}^{{\beta }}_{+})\ge 5r_{0}. \end{aligned}$$

(3.4)

We denote $I_{-}=(-\infty ,0)$, $I_{+}=(0,+\infty )$, and, given $q_{0}\in \Gamma$,

$$\begin{aligned} {\mathcal H}^{\pm }_{b,q_{0}}&=\!\{v\!\in \!{\mathcal {H}}\,/\, v(\cdot ,0)=q_{0},\ \inf _{y\in I_{\pm }}V(v(\cdot ,y))\!\ge \!b,\ \liminf _{y\rightarrow \pm \infty }\hbox {dist}(v(\cdot ,y),{\mathcal V}^{b}_{\pm })=0\}. \end{aligned}$$

Next Lemma states that if ${\varphi }_{I_{\pm }}(v)$ is small for a $v\in {\mathcal H}^{\pm }_{b,q_{0}}$, then $v(\cdot ,y)$ remains close for $y\in I_{\pm }$ to the set ${\mathcal V}^{{\beta }}_{\pm }$ with respect to the $L^{2}({\mathbb {R}})^{2}$ metric.

Lemma 3.7

If $q_{0}\in \Gamma$, $V(q_{0})\ge b$, $v\in {\mathcal H}^{\pm }_{b,q_{0}}$, and ${\varphi }_{I_{\pm }}(v)\le \Lambda _{0}$, then

$$\begin{aligned} \mathrm {dist}(v(\cdot ,y),{\mathcal V}^{{\beta }}_{\pm })\le r_{0} \quad \hbox { for every } \quad y\in I_{\pm }. \end{aligned}$$

Proof

By (3.1), the function $y\in [0,+\infty )\mapsto v(\cdot ,y)-z_{0}\in L^{2}({\mathbb {R}})^{2}$ is continuous. If, by contradiction, $y_{0}\ge 0$ is such that $\mathrm {dist}(v(\cdot ,y_{0}),{\mathcal V}^{{\beta }}_{+})> r_{0}$, since $\liminf _{y\rightarrow +\infty }\mathrm {dist}(v(\cdot ,y),{\mathcal V}^{b}_{+})=0$, by continuity, there exists an interval $(y_{1},y_{2})\subset {\mathbb {R}}$ such that $r_{0}/2<\mathrm {dist}(v(\cdot ,y),{\mathcal V}^{{\beta }}_{+})< r_{0}$ for any $y\in (y_{1},y_{2})$ and $\Vert v(\cdot ,y_{1})-v(\cdot ,y_{2})\Vert \ge r_{0}/2$. By (3.4), $v(\cdot ,y)\notin {\mathcal V}^{{\beta }}_{+}\cup {\mathcal V}^{{\beta }}_{-}$ and so $V(v(\cdot ,y))-b\ge {\beta }-b=(m+\lambda _{0}-b)/4$ for all $y\in (y_{1},y_{2})$ . By (3.2), we conclude

$$\begin{aligned} \Lambda _{0}\ge {\varphi }_{(0,+\infty )}(v)\ge {\varphi }_{(y_{1},y_{2})}(v)\ge \sqrt{\tfrac{m+\lambda _{0}-b}{2}}\Vert v(\cdot ,y_{1})-v(\cdot ,y_{2})\Vert \ge 2\Lambda _{0}, \end{aligned}$$

a contradiction. Analogous is the case $v\in {\mathcal H}^{-}_{b,q_{0}}$. $\square$

Clearly, the infimum value of ${\varphi }_{I_{\pm }}$ on ${\mathcal H}^{\pm }_{b,q_{0}}$ is close to 0 if ${\mathrm {dist}}(q_{0},{\mathcal V}^{b}_{\pm })$ is small. Next result displays a test function $w^{\pm }_{q_{0}}\in {\mathcal H}^{\pm }_{b,q_{0}}$ which gives us refined information

Lemma 3.8

For all $b\in (m,m+\lambda _{0})$, there exists $C(b)>0$ such that for every $q_{0}\in {\mathcal V}^{{\beta }}_{\pm }{\setminus } {\mathcal V}^{b}_{\pm }$, there is $w^{\pm }_{q_{0}}\in {\mathcal H}^{\pm }_{b,q_{0}}$, such that we have

$$\begin{aligned} \sup _{y\in I_{\pm }}\Vert w^{\pm }_{q_{0}}(\cdot ,y)-q_{0}\Vert \!\le \! \tfrac{r_{0}}{\nu (b)}(V(q_{0})-b) \ \hbox { and}\quad {\varphi }_{I_{\pm }}(w^{+}_{q_{0}})\!\le \!C(b)(V(q_{0})-b)^{3/2}, \end{aligned}$$

where $\nu (b)$ is defined in (2.15).

Proof

Assume $q_{0}\in {\mathcal V}^{{\beta }}_{+}{\setminus } {\mathcal V}^{b}_{+}$ (the proof is symmetric in the case $q_{0}\in {\mathcal V}^{{\beta }}_{-}{\setminus } {\mathcal V}^{b}_{-}$). Since $q_{0}\in {\mathcal V}^{{\beta }}_{+}\subset N_{r_{0}}(\mathcal {C}(z_{-}))$, by Lemma 2.11, there exists a unique $s_{0}\in (0,1)$, such that $V(z_{q_{0}}+s(q_{0}-z_{q_{0}}))>b$ for any $s\in [s_{0},1)$ and $V(z_{q_{0}}+s_{0}(q_{0}-z_{q_{0}}))=b$. Moreover, for the constant $\nu (b)$ defined in (2.15), we have

$$\begin{aligned} 1-s_{0}\le \tfrac{1}{\nu (b)}(V(q_{0})-b). \end{aligned}$$

(3.5)

Indeed, by Lemma 2.11

$$\begin{aligned} V(q_{0})-b&=\int _{s_{0}}^{1}\int _{0}^{s}\frac{\mathrm{d}^{2}}{\mathrm{d}s^{2}}V(z_{q_{0}}\!+\!\sigma (q_{0}-z_{q_{0}}))\, \mathrm{d}\sigma \,\mathrm{d}s\!\ge \!\int _{s_{0}}^{1}s\frac{\bar{\mu }}{2}\Vert q_{0}\!-\!z_{q_{0}}\Vert ^{2}_{H^{1}({\mathbb {R}})^{2}}\,\mathrm{d}s\\&\ge (1-s_{0}^{2})\frac{\bar{\mu }}{4}\Vert q_{0}-z_{q_{0}}\Vert ^{2}_{H^{1}({\mathbb {R}})^{2}}\ge (1-s_{0})\nu (b). \end{aligned}$$

We define

$$\begin{aligned} w^{+}_{q_{0}}(x,y)={\left\{ \begin{array}{ll}q_{0}(x)&{}y\le 0,\\ z_{q_{0}}(x)+\left( 1-\tfrac{y^{2}}{2}\right) (q_{0}(x)-z_{q_{0}}(x))&{}y\in (0,{\scriptstyle \sqrt{2(1-s_{0})}}),\\ z_{q_{0}}(x)+s_{0}(q_{0}(x)-z_{q_{0}}(x))&{}y\ge {\scriptstyle \sqrt{2(1-s_{0})}}.\end{array}\right. } \end{aligned}$$

We have $w^{+}_{q_{0}}\in {\mathcal H}^{+}_{b,q_{0}}$ and by (3.5)

$$\begin{aligned} \sup _{y\ge 0}\Vert w^{+}_{q_{0}}(\cdot ,y)-q_{0}\Vert =(1-s_{0})\Vert q_{0}-z_{q_{0}}\Vert \le \tfrac{1}{\nu (b)}(V(q_{0})-b)r_{0}. \end{aligned}$$

Again, using (3.5), we obtain

$$\begin{aligned} {\varphi }_{(0,+\infty )}(w^{+}_{q_{0}})&=\int _{0}^{{\scriptstyle \sqrt{2(1-s_{0})}}}\tfrac{1}{2}\Vert \partial _{y}\left( 1-\tfrac{y^{2}}{2}\right) (q_{0}-z_{q_{0}})\Vert _{2}^{2}\, \mathrm{d}y\\&\phantom {=}+\int _{0}^{{\scriptstyle \sqrt{2(1-s_{0})}}}V(z_{0}+\left( 1-\tfrac{y^{2}}{2})(q_{0}-z_{q_{0}})\right) -b\, \mathrm{d}y\\&\le \int _{0}^{{\scriptstyle \sqrt{2(1-s_{0})}}}\tfrac{1}{2}y^{2}\Vert q_{0}-z_{q_{0}}\Vert _{2}^{2}\, \mathrm{d}y+\int _{0}^{{\scriptstyle \sqrt{2(1-s_{0})}}}V(q_{0})-b\, \mathrm{d}y\\&\le {\scriptstyle \sqrt{2(1-s_{0})}}\left( \tfrac{(1-s_{0})}{3}r_{0}^{2}+(V(q_{0})-b)\right) \\&\le {\scriptstyle \sqrt{\tfrac{2}{\nu (b)}}}\left( \tfrac{1}{3\nu (b)}r_{0}^{2}+1)(V(q_{0})-b\right) ^{3/2} \end{aligned}$$

and the Lemma follows considering $C(b)= {\scriptstyle \sqrt{\tfrac{2}{\nu (b)}}}(\tfrac{r_{0}^{2}}{3\nu (b)}+1)$. $\square$

For any $b\in (m,m+\lambda _{0})$, we fix $b^{*}\in (b,\beta ]$, such that the following inequalities hold true:

$$\begin{aligned} \tfrac{b^{*}-b}{\nu (b)}<\tfrac{1}{2},\quad \max \{1,C(b)\}(b^{*}-b)^{1/4}<\tfrac{1}{4},\quad C(b)(b^{*}-b)^{3/2}\le \Lambda _{0}, \end{aligned}$$

(3.6)

where $\Lambda _0$ is defined in (3.3). Together with Lemma 3.8, next, result will play an important role in the study of the compactness properties of our minimization problem.

Lemma 3.9

Assume that $q_{0}\in {\mathcal V}^{b^{*}}_{+}{\setminus }{\mathcal V}^{ b}_{+}$ and $v\in {\mathcal H}_{b,q_{0}}^{+}$ verify

$$\begin{aligned} \hbox { if }V(v(\cdot ,y))\le b^{*}\hbox { for a }y\in [0,1)\hbox { then }{\varphi }_{(y,+\infty )}(v)\le C(b)(V(v(\cdot , y))-b)^{3/2}. \end{aligned}$$

(3.7)

Then, there exists $\bar{y}\in (0,1)$, such that $V(v(\cdot , \bar{y}))=b$, $v(\cdot , \bar{y})\in {\mathcal V}^{b}_{+}$ and $v(\cdot ,y)=b$ for every $y\in [\bar{y},+\infty ).$

Proof

We first note that, since $q_{0}\in {\mathcal V}^{b^{*}}_{+}{\setminus }{\mathcal V}^{ b}_{+}$ and $v\in {\mathcal H}_{b,q_{0}}^{+}$, we have $V(v(\cdot ,0))=V(q_{0})\le b^{*}$, and hence, by (3.7) and (3.6), we have ${\varphi }_{(0,+\infty )}(v)\le C(b)(V(q_{0})-b)^{3/2}\le \Lambda _{0}$. By Lemma 3.7, we then deduce that $\mathrm {dist}(v(\cdot ,y),{\mathcal V}^{\beta }_{+})\le r_{0}$ for any $y>0$ and by the definition of $r_{0}$, we obtain that $v(\cdot ,y)\notin {\mathcal V}^{b^{*}}_{-}$ for any $y>0$. In particular, if $y>0$ and $V(v(\cdot ,y))\le b^{*}$, then $v(\cdot ,y)\in {\mathcal V}^{b^{*}}_{+}$.

We claim that there exists a sequence $(\xi _{n})\subset [0,\frac{1}{2})$, such that

$$\begin{aligned} \xi _{n-1}\!<\!\xi _{n}\!\le \!\xi _{n-1}+(\tfrac{b^{*}-b}{4^{2(n-1)}})^{1/4}\!<\!\tfrac{1}{2} \quad \hbox { and } \quad V(v(\cdot ,\xi _{n}))-b\le \tfrac{b^{*}-b}{4^{n}}, \quad \forall n\in {\mathbb {N}}. \end{aligned}$$

(3.8)

Indeed, defining $\xi _{0}=0$, by (3.6) and (3.7), we have that for any $\xi >\xi _{0}$

$$\begin{aligned} \int _{\xi _{0}}^{\xi }V(v(\cdot ,s))-b\, ds&\le {\varphi }_{(\xi _{0},+\infty )}(v)\le C(b)(V(v(\cdot ,\xi _{0}))-b)^{3/2}\\&\le C(b)(b^{*}-b)^{3/2}\le \tfrac{b^{*}-b}{4}(b^{*}-b)^{1/4}, \end{aligned}$$

and so

$$\begin{aligned} \exists \, \xi _{1}\in (\xi _{0},\xi _{0}+(b^{*}-b)^{1/4}) \quad \hbox { such that } \quad V(\bar{v}(\cdot ,\xi _{1}))-b\le \tfrac{b^{*}-b}{4}, \end{aligned}$$

(3.9)

Note that, by (3.6), $\xi _{0}+(b^{*}-b)^{1/4}<\xi _{0}+\tfrac{1}{4}<\tfrac{1}{2}$, and so $\xi _{1}\in (0,\frac{1}{2})$.

Now, if $\xi _{n}$ verifies (3.8), by (3.7), we obtain that for any $\xi >\xi _{n}$

$$\begin{aligned} \int _{\xi _{n}}^{\xi }V( v(\cdot ,s))-b\, ds&\le {\varphi }_{(\xi _{n},+\infty )}(v)\le C(b)(V(v(\cdot ,\xi _{n}))-b)^{3/2}\\&\le C(b)(b^{*}-b)^{1/4}(\tfrac{b^{*}-b}{4^{n}})(\tfrac{b^{*}-b}{4^{2n}})^{1/4}< \tfrac{b^{*}-b}{4^{n+1}}(\tfrac{b^{*}-b}{4^{2n}})^{1/4}, \end{aligned}$$

implying that

$$\begin{aligned} \exists \, \xi _{n+1}\in (\xi _{n},\xi _{n}+(\tfrac{b^{*}-b}{4^{2n}})^{1/4}) \quad \hbox { such that } \quad V(v(\cdot ,\xi _{n+1}))-b\le \tfrac{b^{*}-b}{4^{n+1}}, \end{aligned}$$

and, by (3.6)

$$\begin{aligned} \xi _{n+1}<\sum _{j=0}^{n}(\tfrac{b^{*}-b}{4^{2j}})^{1/4}=(b^{*}-b)^{1/4}\sum _{j=0}^{+\infty }\tfrac{1}{2^{j}}<\tfrac{1}{2}. \end{aligned}$$

Then, by induction, (3.8) holds true for any $n\in {\mathbb {N}}$.

Now, note that by (3.8), we have $\xi _{n}\rightarrow \bar{y}\in (0,\frac{1}{2}]$ as $n\rightarrow +\infty$. Moreover, since $v\in {\mathcal H}_{b,q_{0}}$ there result $V( v(\cdot ,\xi _{n}))\ge b$ for all $n\in {\mathbb {N}}$, and hence, by (3.8), $V( v(\cdot ,\xi _{n}))\rightarrow b$. Then, by Lemma 3.1, we deduce $V(v(\cdot ,\bar{y}))=b$. Moreover, by (3.1), $v(\cdot ,\xi _{n})- v(\cdot ,\bar{y})\rightarrow 0$ in $L^{2}({\mathbb {R}})^{2}$ and weakly in $H^{1}({\mathbb {R}})^{2}$. Then, by Remark 2.14, we have $v(\cdot ,\bar{y})\in {\mathcal V}^{b}_{+}$, and hence, using (3.7) that ${\varphi }_{(\bar{y},+\infty )}(v)\le C(b)(V(v(\cdot ,\bar{y}))-b)^{3/2}=0$, which implies $v(\cdot ,y)= b$ for every $y\ge \bar{y}$. $\square$

Remark 3.10

A symmetric argument shows that: if $q_{0}\in {\mathcal V}^{b^{*}}_{-}{\setminus }{\mathcal V}^{ b}_{-}$ and $v\in {\mathcal H}_{b,q_{0}}^{-}$ verify

$$\begin{aligned} \hbox { if }V(v(\cdot ,y))\le b^{*}\hbox { for a }y\in (-1,0]\hbox { then }{\varphi }_{(-\infty ,y)}(v)\le C(b)(V(v(\cdot , y))-b)^{3/2}, \end{aligned}$$

then there exists $\bar{y}\in (-1,0)$, such that $V(v(\cdot , \bar{y}))=b$, $v(\cdot , \bar{y})\in {\mathcal V}^{b}_{-}$ and $v(\cdot ,y)=b$ for every $y<\bar{y}$.

Lemma 3.9, Remark 3.10, and Lemma 3.8 have the following consequence which will be used in the construction of minimizing sequences for ${\varphi }$ with suitable compactness properties.

Lemma 3.11

Let $b\in [m,m+\lambda _{0})$, then, for every $q_{0}\in {\mathcal V}^{b^{*}}_{\pm }{\setminus }{\mathcal V}^{ b}_{\pm }$ and $v\in {\mathcal H}^{\pm }_{b,q_{0}}$, there exists $\tilde{v}\in {\mathcal H}^{\pm }_{b,q_{0}}$, such that

$$\begin{aligned} \sup _{y\in I_{\pm }}\Vert \tilde{v}(\cdot ,y)-q_{0}\Vert \le 1 \quad \hbox { and } \quad {\varphi }_{I_{\pm }}(\tilde{v})\le \min \{\Lambda _{0},{\varphi }_{I_{\pm }}(v)\}. \end{aligned}$$

Proof

We prove the lemma only in the case $q_{0}\in {\mathcal V}^{b^{*}}_{+}{\setminus } {\mathcal V}^{b}_{+}$, since the same argument can be used in a symmetric way for the case $q_{0}\in {\mathcal V}^{b^{*}}_{-}{\setminus } {\mathcal V}^{b}_{-}$.

By Lemma 3.8 and (3.6), since $q_{0}\in {\mathcal V}^{b^{*}}_{+}{\setminus } {\mathcal V}^{b}_{+}$, we have that there exists $w^{+}_{q_{0}}$, such that ${\varphi }_{I_{+}}(w^{+}_{q_{0}})\le \Lambda _{0}$ and $\Vert w^{+}_{q_{0}}(\cdot ,y)-q_{0}\Vert \le \tfrac{1}{2}$ for any $y>0$. In particular, if $v\in {\mathcal H}^{+}_{b,q_{0}}$ is such that ${\varphi }_{I_{+}}(v)>\Lambda _{0}$, then the statement of the lemma holds true with $\tilde{v}=w^{+}_{q_{0}}$.

To prove the lemma, we argue by contradiction assuming that there exist $q_{0}\in {\mathcal V}^{b^{*}}_{+}{\setminus }{\mathcal V}^{ b}_{+}$ and $v\in {\mathcal H}^{+}_{b,q_{0}}$ with ${\varphi }_{I_{+}}(v)\le \Lambda _{0}$, such that

$$\begin{aligned} {\varphi }_{I_{+}}(\tilde{v})>{\varphi }_{I_{+}}( v) \quad \hbox { for every } \quad \tilde{v}\in {\mathcal H}^{+}_{b,q_{0}}\hbox { such that }\sup _{y\in I_{+}}\Vert \tilde{v}(\cdot ,y)-q_{0}\Vert \le 1. \end{aligned}$$

(3.10)

By (3.10), we have $\sup _{y\in I_{+}}\Vert v(\cdot ,y)-q_{0}\Vert > 1$, and since $v(\cdot ,0)=q_{0}$, by (3.1), we recover that

$$\begin{aligned}&\exists \, y_{0}>0\hbox { such that }\Vert v(\cdot ,y_{0})-q_{0}\Vert =\tfrac{1}{2}\hbox { and }\Vert v(\cdot ,y)-q_{0}\Vert <\tfrac{1}{2} \\ \nonumber&\quad \text{ for } \text{ any } \quad y\in [0,y_{0}). \end{aligned}$$

(3.11)

As already noted in the proof of the previous Lemma, by Lemma 3.7, since ${\varphi }_{I_{+}}(v)\le \Lambda _{0}$, we have that if $y>0$ and $V( v(\cdot ,y))\le b^{*}$, then $v(\cdot ,y)\in {\mathcal V}^{b^{*}}_{+}$. We claim that

$$\begin{aligned}&\hbox { if }\tilde{y}\in [0,y_{0}) \quad \hbox { and } \quad V(v(\cdot ,\tilde{y}))\le b^{*} \quad \hbox { then } \nonumber \\&\quad {\varphi }_{(\tilde{y},+\infty )}( v)\le C(b)(V(v(\cdot , \tilde{y}))-b)^{3/2}. \end{aligned}$$

(3.12)

Indeed, considering the function

$$\begin{aligned} \tilde{v}(\cdot ,y)={\left\{ \begin{array}{ll} v(\cdot ,y)&{}0\le y< \tilde{y}\\ w^{+}_{ v(\cdot ,\tilde{y})}(\cdot ,y-\tilde{y})&{}y\ge \tilde{y},\end{array}\right. } \end{aligned}$$

we have $\tilde{v}\in {\mathcal H}^{+}_{b,q_{0}}$. Now note that for every $y\in [0,\tilde{y})\subset [0,y_{0})$, by definition of $y_{0}$, we have $\Vert \tilde{v}(\cdot ,y)-q_{0}\Vert =\Vert v(\cdot ,y)-q_{0}\Vert <\frac{1}{2}$, while if $y\ge \tilde{y}$ by Lemmas 3.8 and (3.6)

$$\begin{aligned} \Vert \tilde{v}(\cdot ,y)-q_{0}\Vert&=\Vert w^{+}_{ v(\cdot ,\tilde{y})}(\cdot ,y-\tilde{y})-q_{0}\Vert \\&\le \Vert w^{+}_{ v(\cdot ,\tilde{y})}(\cdot ,y-\tilde{y})- v(\cdot ,\tilde{y})\Vert +\Vert v(\cdot ,\tilde{y})-q_{0}\Vert \le \frac{b^{*}-b}{\nu (b)}+\frac{1}{2}<1. \end{aligned}$$

This shows that $\sup _{y>0}\Vert \tilde{v}(\cdot ,y)-q_{0}\Vert \le 1$, and so, by (3.10), $0<{\varphi }_{I_{+}}(\tilde{v})-{\varphi }_{I_{+}}( v)={\varphi }_{I_{+}}(w^{+}_{v(\cdot ,\tilde{y})})-{\varphi }_{(\tilde{y},+\infty )}(\tilde{v})$ which together with Lemma 3.8 imply (3.12).

Finally note that, by Remark 3.5, ${\varphi }_{(0,y_{0})}(v)\ge \tfrac{1}{2y_{0}}\Vert v(\cdot ,y_{0})-q_{0}\Vert ^{2}= \tfrac{1}{8y_{0}}$, and so, by (3.6) and (3.12), $y_{0}\ge \tfrac{1}{8C(b)(b^{*}-b)^{3/2}}>1$. Then, by (3.12) and Lemma 3.9, there exists $\bar{y}\in (0,1)$, such that $v(\cdot ,\bar{y})\in {\mathcal V}^{b}_{+}$ and $v(\cdot , y)= v(\cdot ,\bar{y})$ for any $y\ge \bar{y}$. Hence, using (3.11), we obtain $1<\sup _{y\in I_{+}}\Vert v(\cdot ,y)-q_{0}\Vert =\sup _{y\in (0,\bar{y}]}\Vert \ v(\cdot ,y)-q_{0}\Vert \le \sup _{y\in (0,y_{0}]}\Vert v(\cdot ,y)-q_{0}\Vert =\tfrac{1}{2}$, a contradiction which proves the Lemma. $\square$

Minimizing ${\varphi }$. Our first step in minimizing ${\varphi }$ on ${\mathcal H}_{b}$ is to select a minimizing sequence with suitable compactness properties.

Lemma 3.12

For every $b\in (m,m+\lambda _{0})$, there exists $L_{0}>0$, $\bar{C}_{1},\bar{C}_{2}>0$ and $(v_{n})\subset {\mathcal H}_{b}$, such that ${\varphi }(v_{n})\rightarrow m_{b}$ and

• (i) $\mathrm {dist}(v_{n}(\cdot ,y),{\mathcal V}^{\beta }_{-})\le r_{0}$ for any $y\le 0$ and $n\in {\mathbb {N}}$.

• (ii) $\mathrm {dist}(v_{n}(\cdot ,y),{\mathcal V}^{\beta }_{+})\le r_{0}$ for any $y\ge L_{0}$ and $n\in {\mathbb {N}}$.

• (iii) $\Vert v_{n}(\cdot ,y)-z_{-}\Vert \le \bar{C}_{1}$ for any $y\in {\mathbb {R}}$ and $n\in {\mathbb {N}}$.

• (iv) For every bounded interval $(y_{1},y_{2})\subset {\mathbb {R}}$, there exists $C>0$, depending on $y_{2}-y_{1}$, such that $\Vert v_{n}-z_{-}\Vert _{H^{1}(S(y_{1},y_{2}))^2}\le C$.

• (v) $\Vert v_{n}\Vert _{L^{\infty }({\mathbb {R}}^{2})^{2}}\le \bar{C}_{2}$ for any $n\in {\mathbb {N}}$.

Proof

Let $b\in (m_{0},m_{0}+\lambda _{0})$ and $(w_{n})\subset {\mathcal H}_{b}$ be such that ${\varphi }(w_{n})\le m_{b}+1$ for any $n\in {\mathbb {N}}$ and ${\varphi }(w_{n})\rightarrow m_{b}$. We prove the lemma producing various modifications of the minimizing sequence $w_{n}$. The first step is to modify $(w_{n})$ with a simple cutoff procedure to obtain a new minimizing sequence $(\psi _{n})$ bounded in $L^{\infty }({\mathbb {R}})^{2}$.

Let $R_{m}$ be given by Remark 2.2. We define

$$\begin{aligned} \psi _{n}(x,y)=\min \left\{ 1,\frac{2R_{m}}{|w_{n}(x,y)|}\right\} w_{n}(x,y) \end{aligned}$$

(3.13)

($\psi _{n}(x,y)=0$ if $w_{n}(x,y)=0$) observing that $\Vert \psi _{n}\Vert _{L^{\infty }({\mathbb {R}})^{2}}\le 2R_{m}$. We claim that

$$\begin{aligned} ( \psi _{n})\subset {\mathcal H}_{b}, \quad \hbox { and } \quad {\varphi }( \psi _{n})\rightarrow m_{b}. \end{aligned}$$

(3.14)

Indeed, let us first show that $( \psi _{n})\subset {\mathcal H}_{b}$, and so that for any $n\in {\mathbb {N}}$, we have

$$\begin{aligned} \inf _{y\in {\mathbb {R}}}V(\psi _{n}(\cdot ,y))\ge b\hbox { and }\liminf _{y\rightarrow \pm \infty }\hbox {dist}(\psi _{n}(\cdot ,y),{\mathcal V}^{b}_{\pm })= 0. \end{aligned}$$

(3.15)

To this aim, we observe that given $y\in {\mathbb {R}}$, if $\Vert w_{n}(\cdot ,y)\Vert _{L^{\infty }({\mathbb {R}})^{2}}\le R_{m}$, then, by definition, $\psi _{n}(\cdot ,y)=w_{n}(\cdot ,y)$ and $V( \psi _{n}(\cdot ,y))=V(w_{n}(\cdot ,y))\ge b$. If otherwise $\Vert w_{n}(\cdot ,y)\Vert _{L^{\infty }({\mathbb {R}})^{2}}> R_{m}$, again, by definition, we have also $\Vert \psi _{n}(\cdot ,y)\Vert _{L^{\infty }({\mathbb {R}})^{2}}> R_{m}$, and by Remark 2.2, we conclude $V( \psi _{n}(\cdot ,y))\ge 2m>m+\lambda _{0}>b$. Then, $V( \psi _{n}(\cdot ,y))\ge b$ for any $y\in {\mathbb {R}}$ and the first part of (3.15) is proved. For the second part, observe that since $w_{n}\in {\mathcal H}_{b}$, there exist a sequence $y_{j}\rightarrow -\infty$ and a sequence $(q_{j})\subset {\mathcal V}^{b}$, such that $\Vert w_{n}(\cdot ,y_{j})-q_{j}\Vert \rightarrow 0$. By Remark 2.2, we have $\Vert q_{j}\Vert _{L^{\infty }({\mathbb {R}})^{2}}\le R_{m}$. Moreover, by definition of $\psi _{n}$, if $|w_{n}(x,y_{j})| >2R_{m}$, we have $\psi _{n}(x,y_{j})=\frac{2R_{m}}{|w_{n}(x,y_{j})|}w_{n}(x,y_{j})$, so that we derive

$$\begin{aligned}&|w_{n}(x,y_{j})-q_{j}(x)|^{2}- |\psi _{n}(x,y_{j})-q_{j}(x)|^{2}\\&\quad =|w_{n}(x,y_{j})|^{2}-4R_{m}^{2}-2\frac{|w_{n}(x,y_{j})|-2R_{m}}{|w_{n}(x,y_{j})|} q_{j}(x)w_{n}(x,y_{j})\\&\quad \ge (|w_{n}(x,y_{j})|-2R_{m})(|w_{n}(x,y_{j})|+2R_{m}-2|q_{j}(x)|)>0. \end{aligned}$$

Hence, we have $|\psi _{n}(x,y_{j})-q_{j}(x)|\le |w_{n}(x,y_{j})-q_{j}(x)|$ for any $x\in {\mathbb {R}}$ and $j\in {\mathbb {N}}$, and so $\Vert \psi _{n}(\cdot ,y_{j})-q_{j}\Vert \le \Vert w_{n}(\cdot ,y_{j})-q_{j}\Vert \rightarrow 0$ as $j\rightarrow +\infty$. This shows that $\liminf _{y\rightarrow -\infty }\hbox {dist}(\psi _{n}(\cdot ,y),{\mathcal V}^{b}_{-})\rightarrow 0$ and (3.15) follows showing in a symmetric way that $\liminf _{y\rightarrow +\infty }\hbox {dist}(\psi _{n}(\cdot ,y),{\mathcal V}^{b}_{+})\rightarrow 0$. To conclude the proof of (3.14), observe now that $|\partial _{y}\psi _{n}(x,y)|\le |\partial _{y} w_{n}(x,y)|$ for almost every $(x,y)\in {\mathbb {R}}^{2}$, and since, by ($W_{2}$), $V( \psi _{n}(\cdot ,y))\le V( w_{n}(\cdot ,y))$ for any $y\in {\mathbb {R}}$, we derive $m_{b}\le {\varphi }( \psi _{n})\le {\varphi }(w_{n})\rightarrow m_{b}$.

We now further modify the sequence $(\psi _{n})$. Let

$$\begin{aligned} s_{n}=\sup \{y\in {\mathbb {R}}\, |\, {\varphi }_{(-\infty ,y)}(\psi _{n})\le \Lambda _{0}\}. \end{aligned}$$

By Remark 3.6, (3.3), and (3.15), we have $\Lambda _{0}<m_{b}\le {\varphi }(\psi _{n})$, and so $s_{n}\in {\mathbb {R}}$ and ${\varphi }_{(-\infty ,s_{n})}(\psi _{n})=\Lambda _{0}$. Since $\psi _{n}(\cdot ,\cdot +s_{n})\in {\mathcal H}^{-}_{b,\psi _{n}(\cdot ,s_{n})}$ and ${\varphi }_{I_{-}}(\psi _{n}(\cdot ,\cdot +s_{n}))=\Lambda _{0}$, by Lemma 3.7, we derive that $\mathrm {dist}(\psi _{n}(\cdot ,y+s_{n}),{\mathcal V}^{\beta }_{-})\le r_{0}$ for any $y\le 0$, and so, by (3.4) and (3.6), $\mathrm {dist}(\psi _{n}(\cdot ,y),{\mathcal V}^{b^{*}}_{+})\ge 4r_{0}$ for any $y\le s_{n}$. In particular,

$$\begin{aligned} \hbox {if }y\le s_{n}\hbox { and }V(\psi _{n}(\cdot ,y))\le b^{*}\hbox { then }\psi _{n}(\cdot ,y)\in {\mathcal V}^{b^{*}}_{-}. \end{aligned}$$

(3.16)

A symmetric argument shows that there exists $t_{n}>s_{n}$, such that

$$\begin{aligned} \hbox {if }y\ge t_{n}\hbox { and }V(\psi _{n}(\cdot ,y))\le b^{*}\hbox { then }\psi _{n}(\cdot ,y)\in {\mathcal V}^{b^{*}}_{+}. \end{aligned}$$

(3.17)

Define now

$$\begin{aligned} y^{-}_{n}=\sup \{y\in {\mathbb {R}}\,|\, \psi _{n}(\cdot ,y)\in {\mathcal V}^{b^{*}}_{-}\}. \end{aligned}$$

By (3.17), we have $y_{n}^{-}<t_{n}$, and since $\liminf _{y\rightarrow -\infty }V(\psi _{n}(\cdot ,y))=b<b^{*}$, by (3.16), we obtain that $y_{n}^{-}\in {\mathbb {R}}$. Defining, furthermore

$$\begin{aligned} y_{n}^{+}=\inf \{ y\ge y_{n}^{-}\,|\, \psi _{n}(\cdot ,y)\in {\mathcal V}^{b^{*}}_{+}\}, \end{aligned}$$

by Remark 2.14 and (3.1), we obtain that

$$\begin{aligned} y_{n}^{-}<y_{n}^{+}\in {\mathbb {R}},\ \psi _{n}(\cdot ,y^{-}_{n})\in {\mathcal V}^{b^{*}}_{-}\hbox { and }\psi _{n}(\cdot ,y^{+}_{n})\in {\mathcal V}^{b^{*}}_{+}. \end{aligned}$$

Moreover, $V(\psi _{n}(\cdot ,y))>b^{*}$ for any $y\in (y_{n}^{-},y_{n}^{+})$ and by (3.2), we derive

$$\begin{aligned}&y_{n}^{+}-y_{n}^{-}\le \tfrac{{\varphi }_{(y_{n}^{-},y_{n}^{+})}(\psi _{n})}{b^{*}-b}\le \tfrac{m_{b}+1}{b^{*}-b}:= L_{0}\hbox { and }\nonumber \\&\sup \limits _{y\in (y_{n}^{-},y_{n}^{+})}\Vert \psi _{n}(\cdot ,y)-\psi _{n}(\cdot ,y_{n}^{-})\Vert \le \tfrac{m_{b}+1}{\scriptstyle {\sqrt{2(b^{*}-b)}}}. \end{aligned}$$

(3.18)

By Lemma 3.11, there exist $\tilde{v}_{n}^{-}\in {\mathcal H}^{-}_{b,\psi _{n}(\cdot ,y_{n}^{-})}$ and $\tilde{v}_{n}^{+}\in {\mathcal H}^{+}_{b,\psi _{n}(\cdot ,y_{n}^{-})}$, such that

$$\begin{aligned}&\sup _{y\in (-\infty ,0)}\Vert \tilde{v}^{-}_{n}(\cdot ,y)-\psi _{n}(\cdot ,y_{n}^{-})\Vert \le 1,\ \nonumber \\&\quad \sup _{y\in (0,+\infty )}\Vert \tilde{v}^{+}_{n}(\cdot ,y)-\psi _{n}(\cdot ,y_{n}^{+})\Vert \le 1,\nonumber \\ \nonumber&{\varphi }_{(-\infty , 0)}(\tilde{v}_{n}^{-})\le \min \{\Lambda _{0},{\varphi }_{(-\infty , y_{n}^{-})}(\psi _{n})\},\ {\varphi }_{(0,+\infty )}(\tilde{v}_{n}^{+})\\&\le \min \{\Lambda _{0},{\varphi }_{(y_{n}^{+},+\infty )}(\psi _{n})\}. \end{aligned}$$

(3.19)

Eventually retracting the functions $\tilde{v}^{\pm }_{n}$ as in (3.13), the argument used at the beginning of the proof shows that we can assume also that

$$\begin{aligned} \sup _{y\le 0}\Vert \tilde{v}^{-}_{n}(\cdot ,y)\Vert _{L^{\infty }({\mathbb {R}})^{2}}\le 2R_{m}\hbox { and }\sup _{y\ge 0}\Vert \tilde{v}^{+}_{n}(\cdot ,y)\Vert _{L^{\infty }({\mathbb {R}})^{2}}\le 2R_{m}. \end{aligned}$$

(3.20)

We modify the function $\psi _{n}$ defining

$$\begin{aligned} \hat{\psi }_{n}(x,y)={\left\{ \begin{array}{ll}\tilde{v}_{n}^{-}(x,y-y_{n}^{-})&{}\hbox {if }y\in (-\infty , y_{n}^{-}),\\ \psi _{n}(x,y)&{}\hbox {if }y\in [y_{n}^{-}, y_{n}^{+}],\\ \tilde{v}_{n}^{+}(x,y-y_{n}^{+})&{}\hbox {if }y\in ( y_{n}^{+},+\infty ),\end{array}\right. } \end{aligned}$$

observing that $\hat{\psi }_{n}\in {\mathcal H}_{b}$ and $m_{b}\le {\varphi }(\hat{\psi }_{n})\le {\varphi }(\psi _{n})\rightarrow b$. By (3.20) and the definition of $\psi _{n}$, we also have

$$\begin{aligned} \Vert \hat{\psi }_{n}\Vert _{L^{\infty }({\mathbb {R}}^{2})^{2}}\le 2R_{m}. \end{aligned}$$

(3.21)

We can now finally verify that suitable translated of the function $\hat{\psi }_{n}$ satisfies (i)–(v). Indeed, since $\hat{\psi }_{n}(\cdot ,y_{n}^{-})\in \mathcal {V}^{b^{*}}_{-}\subset N_{r_{0}}(\mathcal {C}(z_{-}))$, there exists $\sigma _{n}$, such that

$$\begin{aligned} \Vert \hat{\psi }_{n}(\cdot ,y_{n}^{-})-z_{-}(\cdot -\sigma _{n})\Vert \le r_{0}. \end{aligned}$$

(3.22)

Then, for any $n\in {\mathbb {N}}$, we consider the functions

$$\begin{aligned} v_{n}(x,y)=\hat{\psi }_{n}(x+\sigma _{n},y+y_{n}^{-}). \end{aligned}$$

We plainly have $(v_{n})\subset {\mathcal H}_{b}$ and ${\varphi }(v_{n})={\varphi }(\hat{\psi }_{n})\rightarrow m_{b}$ as $n\rightarrow +\infty$. By (3.21) $\Vert v_{n}\Vert _{L^{\infty }({\mathbb {R}}^{2})^{2}}=\Vert \hat{\psi }_{n}\Vert _{L^{\infty }({\mathbb {R}}^{2})^{2}}\le 2R_{m}$ and (v) follows. By (3.19) and (3.18), we have ${\varphi }_{(-\infty , 0)}(v_{n})\le \Lambda _{0}$, and ${\varphi }_{(L_{0},+\infty )}(v_{n})\le \Lambda _{0}$. Then, by Lemma 3.7, we derive (i) and (ii).

To prove (iii) we observe that, by (3.22), $\Vert v_{n}(\cdot ,0)-z_{-}\Vert =\Vert \hat{\psi }_{n}(\cdot +\sigma _{n},y_{n}^{-})-z_{-}\Vert \le r_{0}$. Then, by (3.19), we derive that for any $y\le 0$, we have

$$\begin{aligned} \Vert v_{n}(\cdot ,y)-z_{-}\Vert&=\Vert \hat{\psi }_{n}(\cdot +\sigma _{n},y+y_{n}^{-})-z_{-}\Vert \le \Vert \hat{\psi }_{n}(\cdot +\sigma _{n},y+y_{n}^{-}) \\&-\hat{\psi }_{n}(\cdot +\sigma _{n},y_{n}^{-})\Vert +\Vert \hat{\psi }_{n}(\cdot +\sigma _{n},y_{n}^{-})-z_{-}\Vert \\&= \Vert \tilde{v}^{-}_{n}(\cdot ,y)-\hat{\psi }_{n}(\cdot ,y_{n}^{-})\Vert +r_{0}\le 1+r_{0} \end{aligned}$$

Moreover, by (3.18), for any $y\in (0,y_{n}^{+}-y_{n}^{-})$, we have

$$\begin{aligned} \Vert v_{n}(\cdot ,y)-z_{-}\Vert&\le \Vert \hat{\psi }_{n}(\cdot ,y+y_{n}^{-})-\psi _{n}(\cdot ,y_{n}^{-})\Vert +\Vert \hat{\psi }_{n}(\cdot +\sigma _{n},y_{n}^{-})-z_{-}\Vert \\&\le \tfrac{m_{b}+1}{\scriptstyle {\sqrt{2(b^{*}-b)}}}+r_{0}. \end{aligned}$$

Again, using (3.19), if $y\ge y_{n}^{+}-y_{n}^{-}$, we finally derive

$$\begin{aligned} \Vert v_{n}(\cdot ,y)-z_{-}\Vert&=\Vert \hat{\psi }_{n}(\cdot +\sigma _{n},y+y_{n}^{-})-z_{-}\Vert \\&\le \Vert \hat{\psi }_{n}(\cdot ,y+y_{n}^{-})-\hat{\psi }_{n}(\cdot ,y_{n}^{+})\Vert +\Vert \hat{\psi }_{n}(\cdot +\sigma _{n},y_{n}^{+})-z_{-}\Vert \\&= \Vert \tilde{v}^{+}_{n}(\cdot ,y+y_{n}^{-}-y_{n}^{+})-\hat{\psi }_{n}(\cdot ,y_{n}^{+})\Vert +\Vert \hat{\psi }_{n}(\cdot ,y_{n}^{+})\\&\quad -\psi _{n}(\cdot ,y_{n}^{-})\Vert +r_{0} \le 1+\tfrac{m_{b}+1}{\scriptstyle {\sqrt{2(b^{*}-b)}}}+2r_{0} \end{aligned}$$

and (iii) follows.

Finally, if $y_{1}<y_{2}\in {\mathbb {R}}$, we have

$$\begin{aligned} \Vert \nabla (v_{n}-z_{-})\Vert _{L^{2}(S_{(y_{1},y_{2})})^{2}}^{2}&\le 2(\Vert \nabla v_{n}\Vert _{L^{2}(S_{(y_{1},y_{2})})^{2}}^{2}+(y_{2}-y_{1})\Vert \dot{z}_{-}\Vert _{L^{2}({\mathbb {R}})^{2}}^{2})\\&\le 2(2{\varphi }(v_{n})+2(y_{2}-y_{1})(b+\Vert \dot{z}_{-}\Vert _{L^{2}({\mathbb {R}})^{2}}^{2})), \end{aligned}$$

and (iv) follows from (iii) concluding the proof of the lemma. $\square$

By Lemma 3.12, $(v_n)$ be the minimizing sequence which verifies (i)–(v), then there exists $\bar{v}\in \mathcal {X}$, such that, up to a subsequence

$$\begin{aligned} v_{n}-z_{-}\rightarrow \bar{v}-z_{-}\hbox { weakly in }H^{1}(S_{L})^{2}\hbox { for any }L>0. \end{aligned}$$

We do not know if $\bar{v}\in {\mathcal H}_{b}$, since the constraint $V(v(\cdot ,y))\ge b$ for any $y\in {\mathbb {R}}$ is not necessarily preserved by the weak convergence. In any case, using arguments similar to the ones introduced in [2, 6, 9], we can conclude the proof of Theorem 1.1 showing that the minimality properties of the function $\bar{v}$ are sufficient to recover from it an entire solution as in the statemen of our main Theorem.

The following Lemma lists some immediate properties of the function $\bar{v}$.

Lemma 3.13

For any $b\in (m, m+\lambda _{0})$, there exists $\bar{v}\in {\mathcal {H}}$ satisfies

• (i) Given any interval $I\subset {\mathbb {R}}$, such that $V(\bar{v}(\cdot ,y))\ge b$ for a.e. $y\in I$, we have ${\varphi }_{I}(\bar{v})\le m_{b}$.

• (ii) $\mathrm {dist}(\bar{v}(\cdot ,y),{\mathcal V}^{\beta }_{-})\le r_{0}$ for any $y\le 0$.

• (iii) $\mathrm {dist}(\bar{v}(\cdot ,y),{\mathcal V}^{\beta }_{+})\le r_{0}$ for any $y\ge L_{0}$.

• (iv) $\Vert \bar{v}(\cdot ,y)-z_{-}\Vert \le \bar{C}_{1}$ for any $y\in {\mathbb {R}}$.

• (v) for every $(y_{1},y_{2})\subset {\mathbb {R}}$, $\Vert \bar{v}-z_{-}\Vert _{H^{1}(S(y_{1},y_{2}))}\le C(y_{1},y_{2})$,

• (vi) $\Vert \bar{v}\Vert _{L^{\infty }({\mathbb {R}}^{2})^{2}}\le \bar{C}_{2}$,

where $L_{0}$, $\bar{C}_{1}$, $\bar{C}_{2}$, and $C(y_{1},y_{2})$ are given by Lemma 3.12.

Proof

Let us consider the function $\bar{v}$ described above. Property (i) follows by Lemma 3.4, since $\liminf _{n\rightarrow +\infty } {\varphi }_{I}(v_{n})\le \lim _{n\rightarrow +\infty }{\varphi }(v_{n})=m_{b}$. Properties iv), v), and vi) are direct consequences of Lemma 3.12 (iii), (iv), and (v). To show (ii) observe that by Lemma 3.12 (iii), we have $\Vert v_{n}(\cdot ,y)-z_{-}\Vert \le \bar{C}_{1}$ for any $y\in {\mathbb {R}}$ and $n\in {\mathbb {N}}$. In particular, for any $y\le 0$, the sequence $(v_{n}(\cdot ,y))$ is bounded in $\Gamma$ with respect to the $L^{2}({\mathbb {R}})^{2}$ metric. Since by Lemma 3.12 (i), we have $\mathrm {dist}(v_{n}(\cdot ,y),{\mathcal V}^{\beta }_{-})\le r_{0}$ for any $y\le 0$ and $n\in {\mathbb {N}}$, using Lemma 2.13 and Remark 2.14, we recover $\mathrm {dist}(v(\cdot ,y),{\mathcal V}^{\beta }_{-})\le r_{0}$ for any $y\le 0$. In a symmetric way, we derive also (iii) and the Lemma is proved. $\square$

Even if we do not know if $\bar{v}\in {\mathcal H}_{b}$, we can now select an interval $({\bar{\sigma }},{\bar{\tau }})\subset {\mathbb {R}}$ on which the trajectory $y\rightarrow \bar{v}(\cdot ,y)$ makes a transition between the sets $\mathcal {V}^{b}_{-}$ and $\mathcal {V}^{b}_{+}$ satisfying the property $V(\bar{v}(\cdot ,y))> b$ for any $y\in ({\bar{\sigma }},{\bar{\tau }})$. Precisely, we let

$$\begin{aligned} {\bar{\sigma }}&=\sup \{y\in {\mathbb {R}}\, /\, {\mathrm {dist}}(\bar{v}(\cdot ,y),{\mathcal V}^{b}_{-})\le r_{0}\hbox { and } V(\bar{v}(\cdot ,y))\le b\},\\ {\bar{\tau }}&=\inf \{y>{\bar{\sigma }}\, /\, V(\bar{v}(\cdot ,y))\le b\} \end{aligned}$$

with the agreement that ${\bar{\sigma }}=-\infty$ whenever $V(\bar{v}(\cdot ,y))>b$ for every $y\in {\mathbb {R}}$, such that ${\mathrm {dist}}(\bar{v}(\cdot ,y),{\mathcal V}^{b}_{-})\le r_{0}$ and that ${\bar{\tau }}=+\infty$ whenever $V(\bar{v}(\cdot ,y))>b$ for every $y>{\bar{\sigma }}$. The following Lemma states some natural properties of ${\bar{\sigma }},\, {\bar{\tau }}$.

Lemma 3.14

We have ${\bar{\sigma }}\in [-\infty , L_{0}]$ and ${\bar{\tau }}\in [0,+\infty ]$, and moreover

• (i) ${\bar{\sigma }}<{\bar{\tau }}$.

• (ii) If ${\bar{\sigma }}\in {\mathbb {R}}$, then $\bar{v}(\cdot ,{\bar{\sigma }})\in {\mathcal V}^{b}_{-}$ and if ${\bar{\tau }}\in {\mathbb {R}}$ then $\bar{v}(\cdot ,{\bar{\tau }})\in {\mathcal V}^{b}_{+}$.

• (iii) If $[y_{1},y_{2}]\subset ({\bar{\sigma }},{\bar{\tau }})$, then $\inf _{y\in [y_{1},y_{2}]}V(\bar{v}(\cdot ,y))>b$. Moreover, ${\varphi }_{({\bar{\sigma }},{\bar{\tau }})}(\bar{v})\le m_{b}$.

• (iv) If ${\bar{\sigma }}=-\infty$, then $\liminf _{y\rightarrow -\infty }V(\bar{v}(\cdot ,y))-b=\liminf _{y\rightarrow -\infty }{\mathrm {dist}}(\bar{v}(\cdot ,y),{\mathcal V}^{b}_{-})=0$.

• (v) If ${\bar{\tau }}=+\infty$, then $\liminf _{y\rightarrow +\infty }V(\bar{v}(\cdot ,y))-b=\liminf _{y\rightarrow +\infty }{\mathrm {dist}}(\bar{v}(\cdot ,y),{\mathcal V}^{b}_{+})=0$.

Proof

We prove only (iv) (and symmetrically (v)), since the other properties can be showed following the reasoning displayed in [9] (see Remark 3.19).

Let ${\bar{\sigma }}=-\infty$. Then, $V(\bar{v}(\cdot ,y))> b$ for any $y\in (-\infty ,{\bar{\tau }})$. By Lemma 3.13–(i), we then have ${\varphi }_{(-\infty ,{\bar{\tau }})}(\bar{v})\le m_{b}$ and we derive that there exists a sequence $y_{n}\rightarrow -\infty$, such that $V(\bar{v}(\cdot ,y_{n}))\rightarrow b$. By Lemma 3.13–(ii), we have moreover that ${\mathrm {dist}}(\bar{v}(\cdot ,y),{\mathcal V}^{\beta }_{-})\le r_{0}$ for every $y\le 0$ and so we can assume $\bar{v}(\cdot ,y_{n})\in {\mathcal V}_{-}^{\beta }$ and ${\mathrm {dist}}(\bar{v}(\cdot ,y_{n}),{\mathcal V}^{b}_{+})\ge 4r_{0}$. Arguing as in the proof of Lemma 3.8 and using (2.14) and Lemma 2.10, for any $n\in {\mathbb {N}}$, there exist $z_{\bar{v}(\cdot ,y_{n})}\in \mathcal {C}(z_{-})$ and $s_{n}\in (0,1]$, such that $\Vert \bar{v}(\cdot ,y_{n})-z_{\bar{v}(\cdot ,y_{n})}\Vert \le r_{0}$, $V( z_{\bar{v}(\cdot ,y_{n})}+s_{n }(\bar{v}(\cdot ,y_{n})-z_{\bar{v}(\cdot ,y_{n})}))=b$, $z_{\bar{v}(\cdot ,y_{n})}+s_{n }(\bar{v}(\cdot ,y_{n})-z_{\bar{v}(\cdot ,y_{n})})\in {\mathcal V}_{-}^{b}$ with $1-s_{n}\le (V(\bar{v}(\cdot ,y_{n}))-b)/\nu (b)\rightarrow 0$. Then, we derive as we claimed that ${\mathrm {dist}}(\bar{v}(\cdot ,y_{n}),{\mathcal V}^{b}_{-})\le (1-s_{n})\Vert \bar{v}(\cdot ,y_{n})-z_{\bar{v}(\cdot ,y_{n})}\Vert \rightarrow 0$. $\square$

Lemma 3.14 explains what we mean when we say that the trajectory $y\mapsto \bar{v}(\cdot ,y)$ makes a transition between the sets $\mathcal {V}^{b}_{-}$ and $\mathcal {V}^{b}_{+}$ on the interval $({\bar{\sigma }},{\bar{\tau }})\subset {\mathbb {R}}$. Moreover, by (iii), we know that ${\varphi }_{({\bar{\sigma }},{\bar{\tau }})}(\bar{v})\le m_{b}$. Thanks to the following Lemma (whose proof can be obtained by mirroring the one of Lemma 3.4 in [6]), we have in fact that ${\varphi }_{({\bar{\sigma }},{\bar{\tau }})}(\bar{v})= m_{b}$.

Lemma 3.15

Let $v\in {\mathcal {H}}$ and $-\infty \le {\sigma }<\tau \le +\infty$ be such that

• (i) $V(v(\cdot ,y))>b$ for any $y\in ({\sigma },\tau )$;

• (ii) either ${\sigma }=-\infty$ and $\liminf _{y\rightarrow -\infty }{\mathrm {dist}}(v(\cdot ,y),{\mathcal V}^{b}_{-})=0$ or ${\sigma }\in {\mathbb {R}}$ and $v(\cdot ,{\sigma })\in {\mathcal V}^{b}_{-}$;

• (iii) either $\tau =+\infty$ and $\liminf _{y\rightarrow +\infty }{\mathrm {dist}}(v(\cdot ,y),{\mathcal V}^{b}_{+})=0$ or $\tau \in {\mathbb {R}}$ and $v(\cdot ,\tau )\in {\mathcal V}^{b}_{+}$

then ${\varphi }_{({\sigma },\tau )}(v)\ge m_{b}$. Finally, ${\varphi }_{({\sigma },\tau )}(v)> m_{b}$ if $\liminf _{y\rightarrow {\sigma }^{+}}V(v(\cdot ,y))>b$ or $\liminf _{y\rightarrow \tau ^{-}}V(v(\cdot ,y))>b$.

We can now conclude the proof of Theorem 1.1. Even if from now on the arguments are closely related to the ones used in some previous works (we refer in particular to [9]), we give for completeness the details of the proofs.

Lemma 3.16

For any $b\in (m,m+\lambda _{0})$, we have

• (i) ${\varphi }_{({\bar{\sigma }},{\bar{\tau }})}(\bar{v})=m_{b}$ and $\liminf _{y\rightarrow {\bar{\tau }}^{-}}V(\bar{.} v(\cdot ,y))=\liminf _{y\rightarrow {\bar{\sigma }}^{+}}V(\bar{v}(\cdot ,y))=b$,

• (ii) ${\bar{\sigma }},{\bar{\tau }}\in {\mathbb {R}}$.

• (iii) For every $h\in C_{0}^\infty ({\mathbb {R}}\times ({\bar{\sigma }},{\bar{\tau }}))^2$, with $\mathrm {supp}\, h\subset {\mathbb {R}}\times [y_{1},y_{2}]\subset {\mathbb {R}}\times ({\bar{\sigma }},{\bar{\tau }})$, there exists $\bar{t}>0$, such that ${\varphi }_{({\bar{\sigma }},{\bar{\tau }})}(\bar{v}+th)\ge {\varphi }_{({\bar{\sigma }},{\bar{\tau }})}(\bar{v}),\quad \forall \, t\in (0,\bar{t}).$

• (iv) $E_{y}(\bar{v}(\cdot ,y))=\tfrac{1}{2}\Vert \partial _{y}\bar{v}(\cdot ,y)\Vert ^{2}-V(\bar{v}(\cdot ,y))=-b\,$ for every $y\in ({\bar{\sigma }},{\bar{\tau }})$.

• (v) $\liminf _{y\rightarrow {\bar{\tau }}^{-}}\Vert \partial _{y}\bar{v}(\cdot ,y)\Vert =\liminf _{y\rightarrow {\bar{\sigma }}^{+}}\Vert \partial _{y}\bar{v}(\cdot ,y)\Vert =0$.

Proof

• (i) As already noted, we have that ${\varphi }_{({\bar{\sigma }},{\bar{\tau }})}(\bar{v})= m_{b}$, and, by Lemma 3.15, we derive that $\liminf _{y\rightarrow {\bar{\tau }}^{-}}V(\bar{v}(\cdot ,y))=\liminf _{y\rightarrow {\bar{\sigma }}^{+}}V(\bar{v}(\cdot ,y))=b$.

• (ii) Assume by contradiction that ${\bar{\sigma }}=-\infty$. Fixed a $y_{0}<\tau$, such that $q_{0}:=\bar{v}(\cdot ,y_{0})\in {\mathcal V}^{b^{*}}_{-}{\setminus }{\mathcal V}^{ b}_{+}$, we have $\bar{v}(\cdot ,\cdot +y_{0})\in {\mathcal H}_{b,q_{0}}^{-}$. To obtain a contradiction, we show that

  $$\begin{aligned}&\hbox {if }V(\bar{v}(\cdot , y))\le b^{*} \quad \hbox { for a } \quad y\le y_{0} \quad \hbox { then } \nonumber \\&\quad \quad {\varphi }_{( -\infty ,y)}(\bar{v})\le C(b)(V(\bar{v}(\cdot , y))-b)^{3/2}. \end{aligned}$$

  (3.23)

  By (3.23), Lemma 3.9 states the existence of a $\bar{y}\in (y_{0}-1,y_{0})$, such that $V(\bar{v}(\cdot , \bar{y}))=b$, contradicting that ${\bar{\sigma }}=-\infty$. If (3.23) does not hold, by Lemma 3.8, there exists $\tilde{y}\le y_{0}$ with $\bar{v}(\cdot ,\tilde{y})\in {\mathcal V}^{b^{*}}_{-}$ and ${\varphi }_{( -\infty ,\tilde{y})}(\bar{v})> {\varphi }_{( -\infty ,0)}(w^{-}_{\bar{v}(\cdot , \tilde{y})})$. Then, defining

  $$\begin{aligned} \tilde{v}(\cdot , y)={\left\{ \begin{array}{ll}\bar{v}(\cdot ,y)&{}y\ge \tilde{y}\\ w^{-}_{\bar{v}(\cdot ,\tilde{y})}(\cdot ,\cdot -\tilde{y})&{}y< \tilde{y}, \end{array}\right. } \end{aligned}$$

  we obtain ${\varphi }_{(-\infty ,{\bar{\tau }})}(\tilde{v})<{\varphi }_{(-\infty ,{\bar{\tau }})}(\bar{v})=m_{b}$. Using Lemma 3.15, this leads to a contradiction. Indeed, by definition of $w^{-}_{\bar{v}(\cdot ,\tilde{y})}$, there exists $y_{-}<\tilde{y}$, such that $\tilde{v}(\cdot , y_{-})\in \mathcal {V}^{b}_{-}$, $V(\tilde{v}(\cdot ,y))>b$ for any $y\in (y_{-},{\bar{\tau }})$ and either $\tilde{v}(\cdot ,{\bar{\tau }})=\bar{v}(\cdot ,{\bar{\tau }})\in \mathcal {V}^{b}_{+}$ if ${\bar{\tau }}<+\infty$ or $\liminf _{y\rightarrow +\infty }{\mathrm {dist}}(\tilde{v}(\cdot ,y),{\mathcal V}^{b}_{+})=0$ if ${\bar{\tau }}=+\infty$. In other words, $\tilde{v}$ satisfies the assumption of Lemma 3.15 on the interval $(y_{-},{\bar{\tau }})$ and we get the contradiction $m_{b}\le {\varphi }_{(y_{-},{\bar{\tau }})}(\tilde{v})\le {\varphi }_{(-\infty ,{\bar{\tau }})}(\tilde{v})<m_{b}$. To prove also that ${\bar{\tau }}\in {\mathbb {R}}$, we can argue in a symmetric way.

• (iii) Let $h\in C_{0}^\infty ({\mathbb {R}}\times ({\bar{\sigma }},{\bar{\tau }}))^2$ with $\mathrm {supp}\, h\subset {\mathbb {R}}\times [y_{1},y_{2}]\subset {\mathbb {R}}\times ({\bar{\sigma }},{\bar{\tau }})$. By Lemma 3.14 that there exists $\mu >0$ such that $V(\bar{v}(\cdot ,y))\ge b+\mu$ for any $y\in [y_{1},y_{2}]$. We also recognize that

  $$\begin{aligned}&\exists \bar{t}>0 \quad \hbox { such that } \quad V(\bar{v}(\cdot ,y)+th(\cdot ,y))>b \quad \hbox { for any } \nonumber \\&\quad \quad y\in [y_{1},y_{2}] \quad \hbox { and } \quad t\in (0,\bar{t}). \end{aligned}$$

  (3.24)

  Indeed, if this is not true, there exists a sequence $t_{n}\rightarrow 0$ and a sequence $(s_{n})\subset [y_{1},y_{2}]$, such that $s_{n}\rightarrow y_{0}\in [y_{1},y_{2}]$ and $V(\bar{v}(\cdot ,s_{n})+t_{n}h(\cdot ,s_{n}))\le b$. Arguing as in the proof of Lemma 3.1, a semicontinuity argument shows that $b<V(\bar{v}(\cdot ,y_{0}))\le \liminf _{n\rightarrow +\infty }V(\bar{v}(\cdot ,s_{n})+t_{n}h(\cdot ,s_{n}))\le b$, a contradiction. By (3.24), the function $v+th$ verifies the assumption of Lemma 3.15 on the interval $({\bar{\sigma }}, {\bar{\tau }})$ for any $t\in (0,\bar{t})$ and we deduce ${\varphi }_{({\bar{\sigma }},{\bar{\tau }})}(\bar{v}+th)\ge m_{b}={\varphi }_{({\bar{\sigma }},{\bar{\tau }})}(\bar{v})$ for any $t\in (0,\bar{t})$.

• (iv) For any $\xi \in ({\bar{\sigma }},{\bar{\tau }})$ and $s>0$, the function

  $$\begin{aligned} \bar{v}_{s}(\cdot ,y)={\left\{ \begin{array}{ll}\bar{v}(\cdot ,y+\xi )&{}y\le 0,\\ \bar{v}(\cdot ,\tfrac{y}{s}+\xi )&{}0<y. \end{array}\right. } \end{aligned}$$

  verifies the assumption of Lemma 3.15 on the interval $({\bar{\sigma }}-\xi , s({\bar{\tau }}-\xi ))$. Then, ${\varphi }_{({\bar{\sigma }}-\xi , s({\bar{\tau }}-\xi ))}(\bar{v}_{s})\ge m_{b}={\varphi }_{({\bar{\sigma }}-\xi , {\bar{\tau }}-\xi )}(\bar{v}(\cdot ,\cdot +\xi ))$, and so, for any $s>0$, we have

  $$\begin{aligned} 0&\le {\varphi }_{({\bar{\sigma }}-\xi , s({\bar{\tau }}-\xi ))}(\bar{v}_{s})-{\varphi }_{({\bar{\sigma }}-\xi , {\bar{\tau }}-\xi )}(\bar{v}(\cdot ,\cdot +\xi ))\\&=(\tfrac{1}{s}-1)\int _{\xi }^{{\bar{\tau }}}\tfrac{1}{2}\Vert \partial _{y}\bar{v}(\cdot ,y)\Vert ^{2}\, \mathrm{d}y +(s-1)\int _{\xi }^{{\bar{\tau }}}V(\bar{v}(\cdot , y))-b\, \mathrm{d}y. \end{aligned}$$

  Setting $A=\int _{\xi }^{{\bar{\tau }}}\tfrac{1}{2}\Vert \partial _{y}\bar{v}(\cdot ,y)\Vert ^{2}\, \mathrm{d}y$ and $B=\int _{\xi }^{{\bar{\tau }}}V(\bar{v}(\cdot , y))-b\, \mathrm{d}y$, the real function $s\mapsto \psi (s)=A(\tfrac{1}{s}-1)+B(s-1)$ is non-negative on $(0,+\infty )$ and then that $0\le \min \psi (s)=\psi ({\scriptstyle \sqrt{\tfrac{A}{B}}})= -(\sqrt{A}-\sqrt{B})^{2},$ that implies $A=B$, that is

  $$\begin{aligned}&\int _{\xi }^{{\bar{\tau }}}V(\bar{v}(\cdot ,y))-b\, \mathrm{d}y=\int _{\xi }^{{\bar{\tau }}}\tfrac{1}{2}\Vert \partial _{y}\bar{v}(\cdot ,y)\Vert ^{2}\, \mathrm{d}y \nonumber \\&\quad \hbox { for any} \quad \xi \in ({\bar{\sigma }},{\bar{\tau }}). \end{aligned}$$

  (3.25)

  By (iii) and since $\bar{v}\in L^{\infty }({\mathbb {R}}^{2})^{2}$, we have that $\bar{v}$ is a weak solution of (1.1)–(1.2) on ${\mathbb {R}}\times ({\bar{\sigma }},{\bar{\tau }})$, and, again using the fact that $\bar{v}\in L^{\infty }({\mathbb {R}}^{2})^{2}$, regularity elliptic arguments (see [17]) give $\bar{v}\in C^{2}({\mathbb {R}}\times ({\bar{\sigma }},{\bar{\tau }}))^2$ verifies (1.1) and (1.2) and $\bar{v}-z\in H^{2}({\mathbb {R}}\times (y_{1},y_{2}))^2$ whenever $[y_{1},y_{2}]\subset ({\bar{\sigma }},{\bar{\tau }})$. This implies that the function $y\rightarrow \tfrac{1}{2}\Vert \partial _{y}\bar{v}(\cdot ,y)\Vert ^{2}-V(\bar{v}(\cdot ,y))$ is continuous and (iv) follows by (3.25).

• (v) It follows by (i) and (iv).

$\square$

The brake orbit-type solution. For every $b\in (m,m+\lambda _0)$, by Lemma 3.16, starting from the function $\bar{v}$ given by Lemma 3.13, by reflection and periodic continuation, we can construct a solution to (1.1)–(1.2) on all ${\mathbb {R}}^{2}$ which is periodic in the variable y. In fact, we define

$$\begin{aligned} v_b(x,y)={\left\{ \begin{array}{ll}\bar{v}(x,y+{\bar{\sigma }})&{}\hbox {if }x\in {\mathbb {R}}\hbox { and }y\in [0, {\bar{\tau }}-{\bar{\sigma }})\\ \bar{v}(x,{\bar{\tau }}+({\bar{\tau }}-{\bar{\sigma }}-y))&{}\hbox {if }x\in {\mathbb {R}}\hbox { and }y\in [{\bar{\tau }}-{\bar{\sigma }}, 2({\bar{\tau }}-{\bar{\sigma }})]\end{array}\right. } \end{aligned}$$

and $v_b(x,y)= v(x,y+2k({\bar{\tau }}-{\bar{\sigma }}))$ for all $(x,y)\in {\mathbb {R}}^{2}$, $k\in {\mathbb {Z}}$.

Remark 3.17

Let $T={\bar{\tau }}-{\bar{\sigma }}$

• (i) The function $y\in {\mathbb {R}}\mapsto v_b(\cdot ,y)\in L^{2}({\mathbb {R}})^{2}$ is continuous and periodic with period 2T. By Lemma 3.14 (ii) and (iv), we have $v_b(\cdot ,0)\in {\mathcal V}^{b}_{-}\hbox { and } v_b(\cdot ,T)\in {\mathcal V}^{b}_{+}$. By definition, $v_b(\cdot ,-y)=v_b(\cdot ,y)$ and $v_b(\cdot ,y+T)=v_b(\cdot ,T-y)$ for any $y\in {\mathbb {R}}$.

• (ii) $v_b\in {\mathcal {H}}$ and, by (v) of Lemma 3.14, $V(v_b(\cdot ,y))>b$ for any $y\in {\mathbb {R}}{\setminus } \{kT\,/\, k\in {\mathbb {Z}}\}$.

• (iii) By (v) of Lemma 3.16, for any $k\in {\mathbb {Z}}$, we have $\liminf _{y\rightarrow kT^{\pm }}\Vert \partial _{y}v_b(\cdot ,y)\Vert =0.$

• (iv) By (iii) of Lemma 3.16 , $v_b\in C^{2}({\mathbb {R}}\times (0, T))^2$ satisfies $-\Delta v(x,y)+v(x,y)-f(v(x,y))=0$ for $(x,y)\in {\mathbb {R}}\times (0, T)$.

Theorem 1.1 finally follows by the following result.

Lemma 3.18

For every $b\in (m,m+\lambda _0)$, $v_b\in {\mathcal C}^{2}({\mathbb {R}}^{2})^2$ is a solution of (1.1)–(1.2) on ${\mathbb {R}}^{2}$. Moreover, $E_{v}(y)=\tfrac{1}{2}\Vert \partial _{y} v(\cdot ,y)\Vert ^{2}-V(v(\cdot ,y))=-b$ for all $y\in {\mathbb {R}}$ and $\partial _{y} v(\cdot ,0)=\partial _{y} v(\cdot ,T)=0$.

Proof

Let us prove that $v_b$ is a classical solution to (1.1)–(1.2) on ${\mathbb {R}}^{2}$. To this aim, we first note that by Remark 3.17 (iii), there exist sequences $(\varepsilon ^{\pm }_{n}), (\eta ^{\pm }_{n})$, such that $\varepsilon ^{-}_{n}<0<\varepsilon ^{+}_{n}$, $\eta ^{-}_{n}<0<\eta ^{+}_{n}$ for any $n\in {\mathbb {N}}$, $\varepsilon ^{\pm }_{n},\, \eta ^{\pm }_{n}\rightarrow 0$ and

$$\begin{aligned} \lim _{n\rightarrow +\infty }\Vert \partial _{y}v_b(\cdot ,\varepsilon ^{\pm }_{n})\Vert =\lim _{n\rightarrow +\infty }\Vert \partial _{y}v_b (\cdot ,T+\eta ^{\pm }_{n})\Vert = 0. \end{aligned}$$

(3.26)

Fixed any $\psi \in C_{0}^\infty ({\mathbb {R}}^{2})$, by Remark 3.17 (i)–(iv), we obtain that for any $k\in {\mathbb {Z}}$ and n sufficiently large, we have

$$\begin{aligned} 0&= \int _{{\mathbb {R}}}\int _{2kT+\varepsilon _{n}^{+}}^{(2k+1)T+\eta _{n}^{-}}-\Delta v_b\,\psi +\nabla W(v_b)\phi \, \mathrm{d}y\, \mathrm{d}x\\&= \int _{{\mathbb {R}}}\int _{2kT+\varepsilon _{n}^{+}}^{(2k+1)T+\eta _{n}^{-}}\nabla v_b\nabla \psi +\nabla W(v_b)\phi \, \mathrm{d}y\, \mathrm{d}x \nonumber \\&\qquad + \int _{{\mathbb {R}}}\partial _{y}v(x,2kT+\varepsilon _{n}^{+})\psi (x,2kT+\varepsilon _{n}^{+})\, \mathrm{d}x\\&\quad -\int _{{\mathbb {R}}}\partial _{y}v_b(x,(2k+1)T+\eta _{n}^{-})\psi (x,(2k+1)T+\eta _{n}^{-})\, \mathrm{d}x \end{aligned}$$

and analogously

$$\begin{aligned}&0= \int _{{\mathbb {R}}}\int _{(2k-1)T+\eta _{n}^{+}}^{2kT+\varepsilon _{n}^{-}}\nabla v_b\nabla \psi +\nabla W(v_b)\phi \, \mathrm{d}y\, \mathrm{d}x\nonumber \\&\quad -\int _{{\mathbb {R}}}\partial _{y}v_b(x,2kT+\varepsilon _{n}^{-})\psi (x,2kT+\varepsilon _{n}^{-})\, \mathrm{d}x\\&\quad +\int _{{\mathbb {R}}}\partial _{y}v_b(x,(2k-1)T+\eta _{n}^{+})\psi (x,(2k-1)T+\eta _{n}^{+})\, \mathrm{d}x. \end{aligned}$$

By (3.26), in the limit for $n\rightarrow +\infty$, we obtain that for any $k\in {\mathbb {Z}}$, we have

$$\begin{aligned} 0= & {} \int _{{\mathbb {R}}}\int _{(2k-1)T}^{2kT}\nabla v_b\nabla \psi +\nabla W(v_b)\phi \, \mathrm{d}y\, \mathrm{d}x\\= & {} \int _{{\mathbb {R}}}\int _{2kT}^{(2k+1)T}\nabla v_b\nabla \psi +\nabla W(v_b)\phi \, \mathrm{d}y\, \mathrm{d}x. \end{aligned}$$

Then, $v_b$ satisfies

$$\begin{aligned} \int _{{\mathbb {R}}^{2}}\nabla v_b\nabla \psi +\nabla W(v_b)\phi \, \mathrm{d}x\, \mathrm{d}y=0,\quad \forall \psi \in C_{0}^\infty ({\mathbb {R}}^{2})^{2}, \end{aligned}$$

and so, since $v_b\in L^{\infty }({\mathbb {R}}^{2})^{2}$, elliptic regularity arguments (see [17]) give that $v_b$ is a classical solution to (1.1)–(1.2) on ${\mathbb {R}}^{2}$ which is periodic of period 2T in the variable y. Since by (v) of Lemma 3.13, we have $\Vert \bar{v}_b(\cdot ,y)\Vert _{H^{1}(S(0,T))^{2}}\le \hat{C}$ depending only on T, by definition of $v_b$ and using (1.1), we recover that $v_b\in H^{2}({\mathbb {R}}\times (y_{1},y_{2}))^{2}$ for any bounded interval $(y_{1},y_{2})\subset {\mathbb {R}}$ and $\Vert v_b\Vert _{H^{2}(S(y_{1},y_{2}))^2}\le C$ with C depending only on $y_{2}-y_{1}$. Then, the functions $y\in {\mathbb {R}}\mapsto \partial _{y}v_b(\cdot ,y)\in L^{2}({\mathbb {R}})^{2}$ and $y\in {\mathbb {R}}\mapsto v_b(\cdot ,y)\in H^{1}({\mathbb {R}})^{2}$ are uniformly continuous and so $\lim _{y\rightarrow 0^{+}}V(v_b(\cdot ,y))-b=\liminf _{y\rightarrow 0^{+}}\Vert \partial _{y}v_b(\cdot ,y)\Vert =0$ and $\lim _{y\rightarrow T^{-}}V(v_b(\cdot ,y))-b=\lim _{y\rightarrow T^{-}}\Vert \partial _{y}v_b(\cdot ,y)\Vert =0$. By continuity, we derive that $\partial _{y} v_b(\cdot ,0)=\partial _{y} v_b(\cdot ,T)=0$. $\square$