Introduction

Terahertz (THz) technology has garnered significant academic attention in recent years owing to its expanding range of applications in fields such as sensing [1,2,3], medical imaging [4, 5], and communications [6, 7]. THz radiation is generated by various processes, such as the nonlinear mixing of two lasers [8, 9], optical rectification (OR) [10, 11], laser (or electron beam)-plasma interaction [12, 13], and photoconductive antennas [14, 15].

There is presently a significant focus on the investigation of graphene’s potential applications, driven by its remarkable electronic, mechanical, and optical characteristics [16]. In particular, the development of graphene-based devices in the terahertz (THz) regime has garnered attention due to recent discoveries regarding the tunability of surface plasmons (SPs) supported by graphene sheets. This tunability can be achieved by adjusting the external gate voltage or through chemical doping [17, 18]. THz SPs generation over a rippled graphene surface studied using linear mode conversion and second harmonic by Srivastav and Panwar [19, 20]. Li et al. [21] investigated harmonic THz SPs generation by electron beam over a uniform graphene surface. Bhasin and Tripathi [22] reported excitation of THz SPs on metallic surfaces by OR. Bhasin and Tripathi [23] investigated THz radiation generation by OR in magnetized rippled density plasma using an x-mode laser. Also, Singh et al. [24] theoretically studied the excitation of THz radiation by OR in transversely magnetized rippled density plasma using a hyperbolic-secant laser pulse. Generation of THz radiation over carbon nanotubes can be achieved using various laser profiles, such as self-focusing lasers and cross-focused Gaussian lasers, both in the presence and absence of a magnetic field [25,26,27,28,29,30]. Unadkat and Kumar [31] reported the generation of sub-THz radiation by an electron beam within the drift space of a plasma-assisted slow wave structure using particle-in-cell simulation.

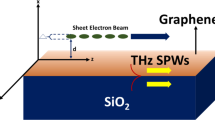
This paper presents an analytical and numerical study of the excitation of THz SPs over a rippled graphene surface using a modulated amplitude of a p-polarized laser beam by OR (see Fig. 1). The excitation of SPs may be accomplished by the utilization of an amplitude-modulated p-polarized laser, obliquely incident at an angle on the interface between graphene and free space. This objective may be accomplished through two methods: the attenuated total reflection configuration or by directly irradiating the laser onto a rippled graphene surface that possesses a certain wave number. SPs wave, which is modulated amplitude exerts a ponderomotive force on free electrons in graphene. This ponderomotive force imparts oscillatory velocity to free electrons at the modulation frequency. The current density is produced by coupling oscillatory velocity with modulated electron density and resonant excitation of THz SPs at the modulation frequency. The linear current density is represented in “Linear Current Density” section. The expression of THz SPs is represented in “Terahertz Graphene Surface Plasmons Amplitude” section. Results and discussion in “Results and Discussion” section and conclusion in “Conclusion” section.

Fig. 1
figure 1

(Color Online) Schematic diagram of THz SPs generation by linear mode conversion of amplitude modulated laser (p-polarized)

Full size image

Linear Current Density

The electric field of SPs wave is given as follows [22]:

$$\begin{aligned} \vec {E} = E e^{- i (\omega t - k_{z} z)} {\left\{ \begin{array}{ll} (\hat{z} + \gamma _{1} \hat{x}) e^{- \alpha _{1 } x}, & \text{ for } \text{ x } > 0 \\ (\hat{z} + \gamma _{2} \hat{x}) e^{\alpha _{2 } x}, & \text { for x} \le 0 \end{array}\right. } \end{aligned}$$
(1)

where \(E=E_{0}(1+\mu \cos \Omega (t-z/v_{g}))\), \(\alpha ^2_1 = \left( k_{z}^2 - ({\omega ^2}/{c^2}) \right)\), \(\alpha _2^2 = k_{z}^2 - ({\omega ^2}/({c^2} \epsilon _{eff}))\), \(\gamma _{1} = -(i k_{z}/\alpha _{1})\), \(\gamma _{2} =-\left( { i k_{z}}/{ \alpha _{2} }\right)\), \(\epsilon _{eff}^{\Omega } = \epsilon _{\text {SiO}_2} + (i \sigma _{g})/(\Omega \epsilon _{0})\), \(\sigma _{g} = (i e^{2} \text {E}_\text {F})/(\pi \hbar ^{2}(\omega +i\nu ))\) is conductivity of graphene, e is electron charge and \(\hbar\) is Plank constant, \(\text {E}_\text {F}\) is Fermi energy of graphene, \(\nu\) is collision frequency, \(k_{z}\) is propagation constant of SPs wave in direction of \(\hat{z}\), \(\mu\) is modulation index, \(\Omega\) is modulation frequency and \(v_{g}\) is group velocity of SPs wave.

The SPs electric field imparts oscillatory velocity \(\vec {v}_{\omega }\) to the electrons of the graphene surface, may be written as follows:

$$\begin{aligned} v_{\omega }^x = \frac{ - i \gamma _{2} e}{m_e^{*} (\omega + i \nu ) } E e^{\alpha x} e^{- i (\omega t - k_{z} z)} \end{aligned}$$
(2)
$$\begin{aligned} v_{\omega }^z = \frac{- i e}{m_e^{*} (\omega + i \nu )} E e^{\alpha x} e^{- i (\omega t - k_{z} z)} \end{aligned}$$
(3)

where \(m_{e}^{*}\) is the effective mass, \(m_{e}^{*} = E_{F}/v_{F}^{2}\) [32] and \(v_{F}\) is Fermi velocity.

The SPs wave exerts ponderomotive force \(\vec {F}_p =- (m_e^{*}/4) (\vec {v}_s \cdot \nabla \vec {v}_s^{*} + \vec {v}_s^{*} \cdot \nabla \vec {v}_s) - (e/4) (\vec {v}_s \times \vec {B}_s^{*} + \vec {v}_s^{*} \times \vec {B}_s)\) on free electrons and it represented as follows:

$$\begin{aligned} \vec {F}_p = \frac{1}{4} \frac{e^2}{m_e^{*}} E^2 e^{(\alpha _2 + \alpha ^{*}_2) x} (- F_{P x} \hat{x} + F_{P z} \hat{z}) \end{aligned}$$
(4)

where \(F_{P x} = (\tilde{v}_{\omega }^x \alpha ^{*}_2 \tilde{v}^{*x}_{\omega } - \tilde{v}_{\omega }^z i k_z \tilde{v}^{*x}_{\omega }) + \left( {{v^{*x}_{\omega }} } \alpha _2 \tilde{v}_{\omega }^x {{+ v^{*z}_{\omega }} } i k_z \tilde{v}_{\omega }^x \right) + (1 / i \omega ) \left( \tilde{v}_{\omega }^{*z} \left( \alpha _2 - i k_z {\beta _2} \right) + \tilde{v}_{\omega }^z (\alpha ^{*}_2 + i k_z \beta _2^{*}) \right)\) and

\(F_{P z} = (1 / i \omega ) \left( \tilde{v}_{\omega }^{*x} \left( \alpha _2 - i k_z {\beta _2} \right) + \tilde{v}_{\omega }^x (\alpha ^{*}_2 + i k_z \beta _2^{*}) \right) - \left( (\tilde{v}_{\omega }^x \alpha ^{*}_2 \tilde{v}^{*z}_{\omega } - \tilde{v}_{\omega }^z i k_z \tilde{v}^{*z}_{\omega }) + \left( {{v^{*x}_{\omega }} } \alpha _2 \tilde{v}_{\omega }^z +{{ v^{*z}_{\omega }} } i k_z \tilde{v}_{\omega }^z \right) \right)\)

Considering the ponderomotive force at modulation frequency \(\Omega\) can be written as follows:

$$\begin{aligned} F_{p \Omega }^x = - \frac{e^2}{m_e^{*}} E_0^2 \mu F_{P x} e^{(\alpha _2 + \alpha ^{*}_2) x} {e^{- i \Omega \left( t - \frac{z}{v_g} \right) }} \end{aligned}$$
(5)

Now, the oscillatory velocity components of free electrons at modulation frequency \(\Omega\) is given as follows:

$$\begin{aligned} v_{\Omega }^x = \tilde{v}_{\Omega }^x \frac{e^2}{(m^{*}_e)^{2}} E_0^2 \mu e^{(\alpha _2 + \alpha ^{*}_2) x} {e^{- i \Omega \left( t - \frac{z}{v_g} \right) }} \end{aligned}$$
(6)

and

$$\begin{aligned} v_{\Omega }^z = 0 \end{aligned}$$
(7)

where \(\tilde{v}_{\Omega }^x = i F_{P x} / (\Omega + i \nu )\).

The oscillatory velocity couples with modulated electron density to produce current density \(\vec {J}_{\Omega }\) at modulation frequency \(\Omega\) and it is given as follows:

$$\begin{aligned} \vec {J}_{\Omega } = \frac{1}{2} n_0 \tilde{v}_{\Omega }^x \frac{e^3}{(m^{*}_e)^{2}} E_0^2 e^{(\alpha _2 + \alpha ^{*}_2) x} e^{- i \Omega t} e^{i \left( \frac{\Omega }{v_g} + q \right) z} \mu h \delta (x) \hat{x} \end{aligned}$$
(8)

Terahertz Graphene Surface Plasmons Amplitude

By using Maxwell’s equation, \(\Omega\) modulation frequency SPs wave field can be expressed as follows:

$$\begin{aligned} \vec {\nabla } \times \vec {E}_{s} = i \Omega \vec {B}_{s} \end{aligned}$$
(9)

and

$$\begin{aligned} \vec {\nabla } \times \vec {B}_{s} = \vec {J}_{\Omega } - i \Omega \epsilon _0 \epsilon _{eff}^{\Omega } \vec {E}_{s} \end{aligned}$$
(10)

where \(\epsilon _{eff}^{\Omega } = \epsilon _{\text {SiO}_2} + (i \sigma _{g})/(\Omega \epsilon _{0}) \text { for x} \le 0\) and \(\varepsilon _{eff}^{\Omega } = 1 \text { for x} > 0\), and s represents the SPs.

The wave equation may be written as follows:

$$\begin{aligned} \vec {\nabla }^{2} \vec {E}_{s} - \vec {\nabla } (\vec {\nabla } \cdot \vec {E}_{s}) - \frac{\Omega ^2}{c^2} \left( \varepsilon _{eff}^{\Omega } \vec {E}_{s} \right) = -\mu _{0} i \Omega \vec {J}_{\Omega } \end{aligned}$$
(11)

Here, \(\epsilon _{eff}^{\Omega }\) is graphene effective permittivity at frequency \(\Omega\). When r.h.s term of Eq. (11) is zero that is \(\vec {J}_{\Omega }\) is absence then SPs eigen mode structure with

$$\begin{aligned} \vec {E}_{s} = \vec {E}_{s,0}, \vec {B}_{s} = \vec {B}_{s,0} \end{aligned}$$
(12)

where \(\vec {E}_{s,0}\) and \(\vec {B}_{s,0}\) is given as follows:

$$\begin{aligned} \vec {\nabla } \times \vec {E}_{s,0} = i \Omega \vec {B}_{s,0} \end{aligned}$$
(13)

and

$$\begin{aligned} \vec {\nabla } \times \vec {B}_{s,0} = - i \Omega \epsilon _0 \epsilon _{eff}^{\Omega } \vec {E}_{s,0} \end{aligned}$$
(14)

The SPs electric field can be represented as follows:

$$\begin{aligned} \vec {E}_{s,0} = e^{- i (\Omega t - k_z^{\Omega } z)} {\left\{ \begin{array}{ll} (\hat{z} + \gamma _{1}^{\Omega } \hat{x}) e^{- \alpha _{1}^{\Omega } x}, & \text{ for } \text{ x } > 0 \\ (\hat{z} + \gamma _{2}^{\Omega } \hat{x}) e^{\alpha _{2}^{\Omega } x}, & \text {for x} \le 0 \end{array}\right. } \end{aligned}$$
(15)

where \(\gamma _{1}^{\Omega } = -(i k_{z}^{\Omega }/ \alpha _{1}^{\Omega })\), \(\gamma _{2}^{\Omega } = -i k_{z}^{\Omega }/\alpha _{2}^{\Omega }\), \((\alpha _{2}k_z^{\Omega })^2 = (k_z^{\Omega })^2 -(\Omega ^2/c^2) \epsilon _{eff}^{\Omega }\), \((\alpha _{1}^{\Omega })^2 = (k_z^{\Omega })^2 -(\Omega ^2/c^2)\), \(k_{z}^{\Omega } = q + \Omega /v_{g}\) and q provides the extra wave number for phase matching condition.

Fig. 2
figure 2

(Color Online) Variation of ripple wave number of q with THz frequency \(\omega\) for three values of graphene Fermi energy \(E_{F}= 0.35\)eV (green line), \(E_{F}= 0.40\)eV (blue dashed line) and \(E_{F}= 0.45\)eV (red dotted line)

Full size image

Figure 2 represents the variation of q with THz frequency \(\omega\) for different values of graphene Fermi energy \(E_{F}= 0.35\)eV (green line), \(E_{F}= 0.40\)eV (blue dashed line) and \(E_{F}= 0.45\)eV (red dotted line) at \(\Omega =1\) THz.

If the current density \(\vec {J}_{\Omega }\) is present, then the electric and magnetic fields of the THz SPs wave can be expressed as follows:

$$\begin{aligned} \vec {E}_{s} = a(t) \vec {E}_{s,0}, \vec {B}_{s} = b(t) \vec {B}_{s,0} \end{aligned}$$
(16)

Solving Eqs. (10) and (11) with Eq. (16), and using Eq. (13), we get

$$\begin{aligned} (a - b) i \Omega \epsilon _0 \epsilon _{eff}^{\Omega } {\vec {E}_{s,0}} = \vec {J}_{\Omega } + {\vec {E}_{s,0}} \frac{\partial a }{\partial t} \epsilon _0 \epsilon _{eff} \end{aligned}$$
(17)

and

$$\begin{aligned} b - a = \frac{1}{i \Omega } \frac{\partial b (t)}{\partial t} \end{aligned}$$
(18)

Using Eq. (17) in Eq. (18) and taking \(\partial a /\partial t \approx \partial b /\partial t\), we get

$$\begin{aligned} \frac{\partial a }{\partial t} {\vec {E}_{s,0}} = - \frac{1}{2 \epsilon _0 \epsilon _{eff}^{\Omega }} \vec {J}_{\Omega } \end{aligned}$$
(19)

Multiplying Eq. (19) by \(\vec {E}_{s,0}^{*} d x\) and integrate \(- \infty\) to \(\infty\), we obtained

$$\begin{aligned} \frac{\partial a }{\partial t} = - \frac{1}{2 \epsilon _0 \epsilon _{eff}^{\Omega }} \frac{I_2}{I_1} \end{aligned}$$
(20)

where \(I_{1} = \int ^{\infty }_{- \infty } \vec {E}_{s,0} \cdot \vec {E}_{s,0}^{*} d x\) and \(I_{2} = \int ^{\infty }_{- \infty } \vec {J}_{\Omega } \cdot \vec {E}_{s,0}^{*} d x\).

Solving Eq. (20), one may obtained

$$\begin{aligned} \frac{\partial a }{\partial t} = \frac{E_0^{2} \mu h}{2 \epsilon _{eff}^{\Omega }} \frac{n_0 e^3}{\epsilon _0 (m_e^{*})^{2}} \frac{ \tilde{v}_{\Omega }^x \left( {\gamma ^{\Omega }_2} \right) ^{*} }{\left( \frac{1 + \gamma ^2_2}{\alpha ^{\Omega }_2} + \frac{1 + \gamma ^2_1}{\alpha ^{\Omega }_1} \right) } \end{aligned}$$
(21)

Let \(\partial /\partial t = - i \Omega\), we obtain

$$\begin{aligned} \mid \frac{a}{E_{0}}\mid = \mid \frac{E_0 e h \mu \omega ^2_{p}}{2 i \Omega \epsilon _{eff}^{\Omega } m_e^{*}} \frac{ \tilde{v}_{\Omega }^x \left( {\gamma ^{\Omega }_2} \right) ^{*} }{\left( \frac{1 + \gamma ^2_2}{\alpha ^{\Omega }_2} + \frac{1 + \gamma ^2_1}{\alpha ^{\Omega }_1} \right) }\mid \end{aligned}$$
(22)

Equation (22) represents the THz SPs amplitude ratio \(\mid {a/A_{0}}\mid\). It depends upon the ripple height h, modulation index \(\mu\), and Fermi energy of graphene surface \(\text {E}_{\text {F}}\).

Fig. 3
figure 3

(Color Online) THz SPs amplitude ratio \(\mid {a/A_{0}}\mid\) verses THz frequency \(\omega\) for three values of graphene Fermi energy \(E_{F}= 0.35\)eV (green line), \(E_{F}= 0.40\)eV (red dashed line) and \(E_{F}= 0.45\)eV (blue dotted line). Other parameters are \(\mu = 0.3\), \(h=50 \mu m\) and \(\epsilon _{\text {SiO}_{2}} = 3.9\)

Full size image

Results and Discussion

The ripple wave number q increases with an increase in Fermi energy of graphene surface \(E_{F}\) at particular values of THz frequency \(\omega\). Also, the Ripple wave number q increases with an increase in THz frequency \(\omega\) at particular values of Fermi energy of graphene surface \(E_{F}\). Ripple wave number q gives the extra wave number to resonantly excite the THz SPs wave. THz SPs amplitude ratio \(\mid {a/A_{0}}\mid\) verses THz frequency \(\omega\) is plotted in Fig. 3 for three values of graphene Fermi energy \(E_{F}= 0.35\)eV (green line), \(E_{F}= 0.40\)eV (red dashed line) and \(E_{F}= 0.45\)eV (blue dotted line) at \(\mu = 0.3\), \(h=50 \mu m\), \(\Omega =2\) THz and \(\epsilon _{\text {SiO}_{2}} = 3.9\). THz SPs amplitude increases with a decrease in THz frequency \(\omega\). Also, it increases with a decrease in Fermi energy of graphene surface \(\text {E}_{\text {F}}\).

Conclusion

In the present paper, theoretically and numerically examined the generation of THz SPs over a rippled graphene surface through OR of an amplitude-modulated p-polarized laser. The generation of THz SPs was examined within the frequency range of 1 to 3 THz, with the Fermi energy of graphene \(E_{F}\) vary from 0.35 to 0.45 eV. The amplitude of THz SPs was found to increase as the THz frequency and Fermi energy of graphene decreased. Additionally, the amplitude of THz SPs increased with an increase in the modulation index, ripple height, and laser amplitude. This work explores the potential use of the Fermi energy of graphene to control the excitation of THz waves at specific frequencies, thereby enabling the development of THz devices [33,34,35].