Introduction

There is a substantial interest of terahertz radiation generation due to its wide applications in outer space communications, biomedical imaging, tomography, precision radar, and other areas [1,2,3,4,5,6,7]. Due to the lack of efficient sources and detectors in THz range or so-called THz gap (0.1–10THz), however, this THz gap still remains a challenge for modern technology and poses significant limitations for further developments in this field and these limitations, arising due to the nature of light/material interaction at THz frequencies.

The laser-based THz radiation generation sources are attractive due to relative simplicity and suitability for modest THz power [8, 9]. The short pulse laser–semiconductor interactions produce the THz radiation by modulation of transient dc current in photoconductors [10, 11]. Intense short pulse laser plasma interaction also offers potential schemes [12,13,14,15] for the generation of long pulse and high power THz, required for THz communication, remote sensing, and ultrafast material characterization.

Highly confined guided electromagnetic waves are used as a probing radiation and having large number of applications [16,17,18,19,20,21,22,23,24], known as surface plasmons (SPs). SPs are guided electromagnetic modes propagate along the interface between a conductor and a dielectric or conductor and air with their field amplitude falling off exponentially away from it in either medium [25,26,27]. The structured surfaces that also support SPs at THz frequency range are called designer plasmonic structures or “spoof” SP structures [28,29,30]. Two structures, an ultrathin metal film dielectric substrate and a metal-coated optical fiber, are shown to support the low loss THz SPs with weak attenuation [31, 32].

Graphene is a 2D material, which has unique properties and potential applications for making the nano devices [33,34,35,36,37,38,39,40]. The most striking feature of graphene is that all its Dirac electrons have the same speed, as energy versus momentum relation is linear, irrespective of their energy. In graphene, the localized electron motion in a plane with very small electron effective mass giving rise to high in-plane conductivity. The two-dimensional nature of graphene also supports plasmons with wavelengths substantially smaller than free-space electromagnetic radiation of the same frequency by approximately two orders of magnitude and generating large non-local effects. Graphene plasmons (GPs) are used for making the compact radiation sources on chip in infrared–visible–ultraviolet range [41].

The difference frequency generation (DFG) at mid-infrared is enhanced due to the presence of plasmons resonance [42,43,44,45]. The graphene holds a great promise for designing the plasmonic sensors or new THz sources. An all-optical coupling scheme is used for plasmon generation in graphene. An electron beam is used for coherent and tunable THz radiation generation from multilayer graphene deposited on a substrate [46]. Despite the recent intensive research, generation of coherent and tunable THz radiation remains a significant challenge. Graphene-coated dielectric nanowire (GNW) supports the Eigen GPs [47]. These modes are depending on the nanowire radius, nanowire permittivity, and chemical potential of graphene that are studied as well; the radius of optical fiber has a strong impact on the modal behavior in graphene-coated optical fiber, such as the number of supported mode. Montasir Qasymeh proposed a THz plasmonic waveguide in frequency range 1–10 THz, which is based on metal–insulator-metal nanostructure filled with optical nonlinear material lithium niobate [48]. Montasir Qasymeh proposed a THz waveguide that is designed to confine a single THz mode having a novel nanostructure comprising graphene parallel plates filled with lithium niobate (i.e., LiNbO3) crystal [49, 50].

In this paper, we put forth the new and efficient scheme of THz GPs generation via the nonlinear mixing of two laser beams in the graphene-coated optical fiber. The fiber has a dielectric ripple (volume grating) of wave number q. The ripple imparts spatial harmonics to lasers shifted by q. The lasers and their spatial harmonics exert a difference frequency ponderomotive force on film electrons with wave number \(k_z=k_{1z}\;-k_{2z}\;+q\) , where \(k_{1z}\) and \(k_{2z}\) are the wave numbers of the lasers. Two lasers exert a ponderomotive force at difference frequency on the electrons in graphene and it induces a nonlinear current which driving the THz SPs. Our calculations and approach in this manuscript are based on single mode propagation optical fiber.

In the “THz Graphene Plasmons in Graphene-Coated Optical Fiber” section, we developed the formalism of THz GPs in graphene-coated fiber and the nonlinear mixing of lasers and THz excitation is discussed in the “THz Surface Plasmons Generation in Graphene-Coated Optical Fiber by Nonlinear Mixing of Two Laser Beams” section. We discussed the results in the “Conclusion” section.

THz Graphene Plasmons in Graphene-Coated Optical Fiber

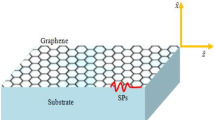
Consider an optical fiber (cf.1) of radius “a” is coated by graphene sheet of conductivity \(\sigma_g\) Consider the thin film of graphene is a layer zero thickness and infinite conductivity and the current density in graphene layer may be defined as:

$$J={\sigma }_{g}d\delta \left(r-a\right){\left.{E}_{z}\right|}_{r=a}\widehat{z}={\sigma }_{g}d{\left.{E}_{z}\right|}_{r=a}\widehat{z}.$$
(1)

The graphene plasmons (GPs) propagate along \(\widehat z\) direction of fiber, i.e., length of fiber with variation as \(exp\left[-i\left(\omega t\;-\;k_zz\right)\right]\) is shown in Fig. 1. The electric and magnetic fields of the GPs in different regions can be written as for \(r<\;a\)(glass) region 1:

Fig. 1
figure 1

THz GPs propagation in graphene-coated optical fiber

Full size image
$$E_{1z}=\;A_{01}I_{0\;}\left(\alpha_1r\right)\;exp\;\left[-i\left(\omega_1t-k_{1z}z\right)\right],\;H_{1\varphi}\;=\frac{\omega\varepsilon_0\varepsilon_1}{i\alpha_1}\;A_{01}I'_0\left(\alpha_1r\right)\;exp\;\left[-i\left(\omega_1t-k_{1z}z\right)\right],$$
(2)

where \(\alpha_1^2\;=\;k_z^{2\;}-\frac{\omega^2}{c^2}\varepsilon_1\) . For region \(r>a\)  (vacuum) region 2, the electric and magnetic fields of the GPs are:

$$\begin{aligned}&E_{2Z}\;=\;A_{02}K_0\left(\alpha_2r\right)\;exp\;\left[-i\left(\omega_2t-k_{2z}z\right)\right],\\&H_{2\varphi}=-\frac{\omega\varepsilon_0\varepsilon_2}{i\alpha_2}\;A_{02}K'_0\left(\alpha_2r\right)\;exp\;\left[-i\left(\omega_2t-k_{2z}z\right)\right],\end{aligned}$$
(3)

where \(a_2^2\;=\;k_z^2\;-\frac{\omega^2}{c^2}\varepsilon_2\) . Using jump condition on \(\overrightarrow H\) (at \(r>a\) ):  

$$\overrightarrow{H}_\phi\mid_2-\overrightarrow{H}_\phi\mid_1=\sigma_gdE_z\mid_{r-a^{}},$$
(4)

Putting the Eqs. (2) and (3) into Eq. (4), one may get:

$$\begin{aligned}\frac {\varepsilon_2}{a_2} \frac {K_0^{\prime}(\alpha_2a)}{K_0(\alpha_2a)}-\frac {\varepsilon_1}{a_1} \frac {I_0^{\prime}(\alpha_2a)}{I_0(\alpha_2a)} = -\frac{i\sigma_gd}{\omega\varepsilon_0}\end{aligned}.$$
(5)

A similar dispersion relation is derived for Eigen GP modes in graphene-coated dielectric nanowire (GNW) which are proposed by solving the Maxwell equations in cylindrical coordinate [47]. In Fig. 2, we have plotted the normalized frequency \(\omega/\;\omega_p\) versus normalized wave vector \(k_zc\;/\;\omega_p\) for two normalized radii \(a\omega_{p\;}/\;c\;=10^{-2}\) , \(a\omega_{p\;}/\;c\;=\;10^{-3}\) of optical fiber, normalized graphene sheet thickness \(d\omega_p\;/c\;=\;0.08\) . The figure shows that with increasing the radius of optical fiber, the coupling is between lasers and graphene plasmons become weak. As the frequency increases with wave number, however, the phase velocity of graphene plasmons slows down with increasing the frequency. The graphene-coated microfiber Bragg grating (GMFBG) is using gas sensing which is reported by Yu Wu et al. [51]. There is surface field enhancement and gas absorption of a GMFBG. In graphene-coated optical fiber, the confinement of modes is very high, so it has high sensitivity.

Fig. 2
figure 2

Dispersion relation of graphene plasmons

Full size image

THz Surface Plasmons Generation in Graphene-Coated Optical Fiber by Nonlinear Mixing of Two Laser Beams

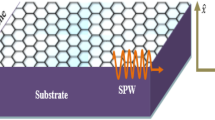
Let two laser beams are propagated in the azimuthally symmetric mode inside the graphene-coated fiber (Fig. 2). The electric fields of the laser beams inside the fiber are:

 

$$\overrightarrow{E}_1=A_1\left[\widehat z{J}_0\left(k_{1\perp}r)\right.+i\widehat r\frac{k_{1z}}{k_{1\perp}}J_0^{\prime}\left(k_{1\perp}r)\right.\right]\;exp\;\left[-i\left(\omega_1t-k_{1z}z)\right.\right].$$
(6)
$$\overrightarrow{E}_2=A_2\left[\widehat z{J}_0\left(k_{2\perp}r)\right.+i\widehat r\frac{k_{2z}}{k_{2\perp}}J_0^{\prime}\left(k_{2\perp}r)\right.\right]\;exp\;\left[-i\left(\omega_2t-k_{2z}z)\right.\right].$$
(7)

The core of fiber to have a dielectric constant ripple for phase matching condition is:

$$\varepsilon_g\;=\;\varepsilon_{g0\;}\;+\;\varepsilon_{gq\;},\;\varepsilon_{gq\;}=\;\varepsilon_{g2}e^{iqz}.$$
(8)

The displacement vector inside the fiber is \(\overrightarrow{D}_1=\varepsilon_g\overrightarrow{E}_1=\varepsilon_{g0}{\overrightarrow E}_1\;+\;\frac12\varepsilon_{gq}\overrightarrow E\) and acquires a component that goes as \(e^{-i\left[\omega_1t-\left(k_{1z}+q\right)z\right]}\). This component produces an electromagnetic field (in compliance with \(\nabla. \overrightarrow{D\;}=0\)):

$${\overrightarrow E}_{1+}\;\cong-\frac{\varepsilon_{gq}}{2\varepsilon_{g0}}{\overrightarrow E}_1\;\approx\;exp\;\left[-i\left\{\omega_1t-\left(k_{1z}+q_z\right)z\right\}\right].$$
(9)

Similarly:

$${\overrightarrow E}_{2+}\;\cong-\frac{\varepsilon_{gq}}{2\varepsilon_{g0}}{\overrightarrow E}_2\;\approx\;exp\;\left[-i\left\{\omega_2t-\left(k_{2z}+q_z\right)z\right\}\right].$$
(10)

Here, the phase matching conditions demands that:

$$\omega_{SPTHz}\;=\;\omega_1\;-\;\omega_2\;,\;k_{SPTHz}\;=\;k_{1z}\;-\;k_{2z}\;+q.$$
(11)

The lasers impart oscillatory velocities to the electrons, given as:

$$\overrightarrow{v}_{1+}=\frac{{\overrightarrow E}_{1+}}{mi{\omega_1}},\,\rightarrow\,\overrightarrow{v}_{2+}=\frac{{e\overrightarrow E}_{2+}}{mi{\omega_2}}.$$
(12)

These laser beams also impart the ponderomotive force \(F_{pz}\) on electrons at beat frequency given by:

$$F_{pz}\;\approx\;\frac{mi\left(k_{1z}\;-\;k_{2z}\;+\;q\right)}2{\overrightarrow v}_1\overrightarrow v_2^\ast\;\frac{\varepsilon_{gq}}{2\varepsilon_{g0}}\cdot$$
(13)

This ponderomotive force also imparts oscillatory velocity to electrons at frequency \(\left(\omega_1\;-\;\omega_2\right)\) and may be written as:

$${\overrightarrow v}_\omega\;-=\;\frac{\widehat zF_{pz}}{\;im\omega},$$
(14)

>and nonlinear current in graphene sheet may be written as:

$$\overrightarrow J_\omega^{NL}\;=\;\widehat Z\frac{n_0e\left(k_{1z}\;-\;k_{2z}\;+\;q\right)}{2\omega}{\overrightarrow v}_1\overrightarrow v_2^\ast\frac{\varepsilon_{gq}}{2\varepsilon_{g0}}\;\cong\;\widehat zFd\delta\;\left(r\right)\;exp\;\left[-i\;\left(\omega_{SP}t\;-\;k_{SP}z\right)\right].$$
(15)

where:

$$\overrightarrow F\;=\;\frac{n_0ek_{sp}}{4\omega}\frac{e^2\mid E_1\mid\mid E_2\mid}{m^2\;\omega_1\;\omega_2}\frac{\varepsilon_{gq}}{\varepsilon_{g0}}\;\cdot$$
(16)

This nonlinear current density is localized in graphene sheet, which is the source of THz radiation graphene plasmons. The electric and magnetic field vectors for GPs are given Fig. 3 as:

Fig. 3
figure 3

THz GPs excitation via nonlinear mixing of lasers in graphene-coated optical fiber

Full size image

For \(r<a\) (glass):

$$\begin{aligned}\overrightarrow{E}_1&=A_1\left[\widehat z{I}_0\left(\alpha_1r)\right.-i\widehat r\frac{k_z}{a_1}\;I_0^{\prime}\left(\alpha_1r)\right.\right]\;exp\;\left[-i\left(\omega_1t\;-\;k_1z)\right.\right],\\\overrightarrow{H}_\phi&=\frac{i\omega\varepsilon_0\varepsilon_1}{\alpha_1}\;A_1I_0^{\prime}\left(\alpha_1r)\right.\;exp\;\left[-i\left(\omega_1t\;-\;k_1z)\right.\right]\;\cdot\end{aligned}$$
(17)

For \(r>a\) (vacuum), the electric and magnetic field vectors for GPs are given as:

$$\begin{aligned}\overrightarrow{E}_2&=A_2\left[\widehat z{K}_0\left(\alpha_2r)\right.-i\widehat r\frac{k_z}{a_2}\;K_0^{\prime}\left(\alpha_2r)\right.\right]\;exp\;\left[-i\left(\omega_2t\;-\;k_2z)\right.\right],\\\overrightarrow{H}_\phi&=\frac{i\omega\varepsilon_0}{\alpha_2}\;A_2K_0^{\prime}\left(\alpha_2r)\right.\;exp\;\left[-i\left(\omega_2t\;-\;k_2z)\right.\right]\;\cdot\end{aligned}$$
(18)

The jump condition on the \(\overrightarrow{H_\phi}\) component of the GPs at \(r\;=a\) gives:

$$DA_0\;=\;\frac{-i\;Fd\;/\;\omega\varepsilon_0}{K_0^{\prime}\left(\alpha_2a\right)},$$
(19)

where:

$$D\;=\;\frac{\varepsilon_1\;}{\alpha_1}\frac{I_0^{\prime}\left(\alpha_1a\right)}{I_0\left(\alpha_1a\right)}-\frac1{a_2}\frac{K_0^{\prime}\left(\alpha_1a\right)}{K_0\left(\alpha_1a\right)}-\frac{\sigma_gd}{i\omega\varepsilon_0}.$$
(20)

Solving Eq. (19), it yields the amplitude of THz GPs:

$$A_0=\frac{FdL/\omega\varepsilon_0}{K_0^{\prime}\left(\alpha_2a\right)\partial D/\partial k_{sp}}.$$
(21)

At exact phase matching condition, D is vanishing. However, due to finite length of interaction \(L\;=\;2r_0\) where \(r_0\) is laser spot size, so D can be replaced by region \(D\;\cong\;-i\;\left(\partial D/\partial k_z\right)\left(\partial/\partial_z\right)\) , Eq. (19) can be written as:

$$\frac{\partial D}{\partial k_z}=-k_z\left[\frac{\varepsilon_1}{\alpha_1^3}\frac{I_0^{\prime}\left(\alpha_1a\right)}{I_0\left(\alpha_1a\right)}-\frac1{\alpha_1^3}\frac{K_0^{\prime}\left(\alpha_2a\right)}{K_0\left(a_2a\right)}\right].$$
(22)

Solving and rearranging the terms in Eq. (22), we have the normalized amplitude of THz graphene plasmons:

$$\left|\frac{A_0}{A_1}\right|\;=\frac{1/\left(\omega/\omega_P\right)\left(\omega/4\omega_1\right)\left(k_{SP}\;/\;k_z\right)\left(\left|v_2\right|/c\right)\left(\varepsilon_{gq}/\varepsilon_{g0}\right)\left(d\omega_p/c\right)\left(L\omega/c\right)}{K_0^{\prime}\left(\alpha_0a\right)\left({\displaystyle\frac{\varepsilon_1}{\left(k_z^2\;-\left(\omega^2/c\right)\varepsilon_1\right)^{3/2}}\frac{I_0^{\prime}\left(\alpha_1a\right)}{I_0\left(\alpha_1a\right)}-\frac1{\left(\omega^2/c\right)^{3/2}}\frac{K_0^{\prime}\left(\alpha_2a\right)}{K_0\left(\alpha_2a\right)}}\right)}\cdot$$
(23)

Equation (23) gives the amplitude of terahertz graphene plasmon. For following parameters: \(v_2\;=\;10^6m\;/s\); \(c\;=\;3\;\times\;10^8m\;/\;s\); \(\omega=10^{13}\;rad\;/\;s\); \(k_z^2\;c^2\;/\;\omega^2\;=\;2.6\); \(\omega_1\;=\;2\times10^{12}rad\;/\;s\); \(\omega_p\;\approx\;2.5\;\times10^{12}rad\;/\;s\), normalized thickness \(d\omega_p/c\;=\;0.08,0.10,0.12\), normalized radius of optical fiber \(a\omega_{p,}/c=10^{-2},10^{-3}\;L\omega/c=10^3,\;\varepsilon_{g0}=0.2\). For CO2 laser, \(e\left|E_2\right|/\;m\omega_2c=10^{-2}\), corresponds to an intensity \(3\times10^{12}W\;/\;cm^2\) and power ~ 8 MW. For \(1\mu m\) wavelength, it corresponds to an intensity of \(3\times10^{12}W\;/\;cm^2\). Figures 4, 5, and 6 show that as the frequency raises, the normalized amplitude of THz SPW decreases. It is due to the weakening of the nonlinear coupling of SPs at higher frequencies.

Fig. 4
figure 4

Normalized THz graphene surface plasmons amplitude variation with normalized frequency for different two different normalized radii of optical fiber and fixed thickness of graphene sheet

Full size image
Fig. 5
figure 5

Normalized THz graphene surface plasmons amplitude variation with normalized frequency for different normalized thickness of graphene sheet and fixed normalized radius of optical fiber

Full size image
Fig. 6
figure 6

Normalized THz graphene surface plasmons amplitude variation with normalized frequency for different normalized thickness of graphene sheet and fixed normalized radius of optical fiber

Full size image

Conclusion

In conclusion, the graphene-coated fiber offers the promise of producing phase-matched THz graphene plasmons using laser moderate power (MW range) and intensity. The nonlinearity arises through the ponderomotive force and phase matching is provided by the volume grating of suitable wave number inside the fiber. The efficiency is one order of magnitude smaller than the ideal efficiency given by Manley Rowe relations. The thickness of the graphene sheet and radius of an optical fiber are important parameters to influence the THz graphene surface plasmons resonant frequency. The normalized amplitude of THz GPS decrease with frequency as the nonlinear coupling gets weaker. The fiber configuration is more suitable as the grating structures in optical fibers are routinely available. The efficiency of the device is around 0.01% at a laser intensity of 3 × 1014 W/cm2.