Pricing commodity-linked bonds with stochastic convenience yield, interest rate and counterparty credit risk: application of Mellin transform methods

Abstract

This paper investigates the effects of the spot underlying commodity price, stochastic convenience yield, interest rate and counterparty credit risk on the pricing of the commodity-linked bonds. The stochastic factors or state variables in the model are the spot price of the underlying commodity follows geometrical Brownian motion process with a stochastic drift, the net convenience yield and the short-term interest rate are formulated as a mean-reverting Ornstein–Uhlenbeck stochastic process and the value of the firm issuing the bonds follows a geometrical Brownian motion process. Furthermore, we develop the two- and three-factor(I, II) pricing models for valuing the commodity-linked bonds. Closed-form pricing formulas of the commodity-linked bonds are derived based on the Mellin transform techniques, which are simply provided with standard (bivariate) normal cumulative distribution function so that the pricing and hedging of the commodity-linked bonds can be computed very accurately and rapidly. At last, numerical analysis compares the results of this four pricing models with realistic parameter values and demonstrates how the spot underlying commodity price, convenience yield, interest rate and counterparty credit risk affect the values of the commodity-linked bonds.

1 Introduction

Although relatively little research has focused on the pricing of commodity-linked bonds, efficient modeling and computation of commodity derivatives have been studied for decades. In a seminal paper, Schwartz (1982) provides a general framework for valuing the commodity-linked bonds based on the option pricing framework as pioneered by Black and Scholes (1973) and extended by Merton (1973) and Cox and Ross (1976). This very general valuation framework considers the spot commodity price risk, default risk, and interest rate risk, along with interest payments and dividends, and takes the form of a second-order partial equation in four variables which governs the value of the commodity-linked bonds at any point in time, but not to derive the closed-form solution subject to certain boundary condition. Schwartz states that the solution to general problem is difficult even by numerical methods. To derive a closed-form pricing formula, Carr (1987) assumes that a default-free unit discounted bond follows a third-order geometric Browian motion without referring to any interest rate dynamics, and by restricting the values of certain parameters in the formula, results given previously by Schwartz (1982) arise as special cases. Ignoring the default risk, Yan (2002) gives a closed-form solution for commodity-linked bond under stochastic volatility and jumps. Rajan and Mundial (1988) apply the binomial model to price a commodity bond is an effective way in the presence of commodity price risk and default risk. However, in Schwartz (1982), Carr (1987) and Rajan and Mundial (1988) pricing models, there are no costs of carrying or convenience yield for the underlying commodity.

The convenience yield of a commodity is defined as the stream of benefits received by holding an extra unit of the commodity in storage rather than buying the unit from the futures market. This stream of benefits comes from the fact that holding commodity in storage enables the holder to respond flexibly and efficiently to supply and demand shocks. Kaldor (1939) first gives this definition for the convenience yield of a commodity in the economic literature, and it is an instructive concept for understanding the theory of storage in the context of financial markets, because it serves to quantify the benefit accrued in the form of an incentive to buy and store certain commodities. Fama and French (1987), Fama and French (1988), Brennan (1991), Miltersen and Schwartz (1998) and Schoene and Spinler (2017) have proved that the convenience yield play a central rule in commodity price modeling as it derives the relationship between futures and spot prices of many commodities. Empirically, several studies have also found the convenience yield to have economic and statistical significance, such as Gibson and Schwartz (1990), Schwartz and Smith (2000), Almansour (2016), Lai and Mellios (2016), Mellios et al. (2016), Ewald et al. (2019). Ingersoll (1982) points out that the convenience yield is a portion of the return on the commodity (asset) not reflected in the price change and should be considered when pricing the commodity-linked bonds. Therefore, Miura and Yamauchi (1998) extend the Schwartz (1982) and Carr (1987) models to include stochastic continence yield and take the approach of Gibson and Schwartz (1990) and Bjerksund (1991) to express the price change of the reference commodity in relation to its convenience yield.

In the commodity-linked bonds, the bondholders are exposed to potential credit risk due to the possibility of their counterparty being unable or unwilling to make the necessary payments at exercise date, in case of default occurs, and the bondholders receives a smaller or zero payment. So expectations of possible future losses due to counterparty credit risk by bond issuer should be reflected from bonds prices. To model the effect of credit risk when pricing contingent claims, structural approach has extensively been used in previous studies, in which credit events is assumed to take place once the value of the assets of the firm is less than some boundary, for example, Black and Scholes (1973), Merton (1974), Black and Cox (1976), Lee (1981) and Chance (1990), and many others. The structural approach is intuitive because it links the default risk to the firm’s economic fundamentals. Based on the default model of corporate bonds proposed by Merton (1974) and Johnson and Stulz (1987) obtain a pricing formula under the assumption that the option holder will receive the total assets of the option writer in default at the expiration date and that the option could be treated as the only liability in the option writer’s capital structure. Schwartz (1982), Carr (1987), Johnson and Stulz (1987) and Miura and Yamauchi (1998) adopt the same approach to modeling the effect of credit risk on bonds prices. But this approach implies a variable default boundary equal to the value of the option upon the exercise date, and may not properly measure the credit risk in most business situations. Later, a more realistic assumption is made in Klein (1996), where the final payout in default depends on the terminal market value of assets as well as the amount of other equally ranking liabilities of the writer. By incorporating this more realistic default condition, the Klein model is able to measure the credit risk more consistent with those observed in corporate debt markets. Subsequently, many extensions and variants of the models used by Johnson and Stulz (1987) and Klein (1996) have been proposed, such as Klein and Inglis (1999, 2001), Hung and Liu (2005), Liao and Huang (2005), Niu and Wang (2015) and Wang et al. (2017).

Up to now, to find the analytic formula for the valuation of financial derivatives, most of the previous literature has used mainly probabilistic techniques. However, the pricing of a given financial derivatives with probabilistic approaches requires the complexity of the calculation. To solve this problem, the Mellin transform approach has been used to obtain the pricing formulas of the various financial derivatives, we can refer to Panini and Srivastav (2004), Yoon and Kim (2015), Jeon and Kim (2019), Li and Rodrigo (2017) and Ma et al. (2020). The Mellin transform is an integral transform, which is regarded as the multiplicative version of the two-sided Laplace transform. In particular, the Mellin transform approach exploits the properties of this transform to reduce the pricing PDE into an ODE that can be solved easily. The option price is then recovered by the convolution property. Therefore, this method will help us to obtain the analytic integral formula of the commodity-linked bonds with stochastic convenience yield, interest rate and counterparty credit risk more easily and effectively.

In this paper we consider the four sources of uncertain, the spot underlying commodity price risk, the instantaneous convenience yield, the spot interest rate and counterparty credit risk, related to the value of the commodity-linked bonds in terms of their implication with respect to the valuation of other financial and real assets, and present the two- and three-factor(I, II) commodity-linked bonds pricing models. The first model is a simple one-factor model in which the spot price of the underlying commodity is only uncertain factor and assumes that it follows a geometric Brownian motion process. In the two-factor model we consider another uncertain source is the convenience yield of the underlying commodity and it is assumed to follow a mean reverting process and positively correlated with the spot underlying commodity price.¹ The third model we consider is a variation of the three-factor Miura and Yamauchi (1998) model. In this three-factor model, the value of the firm issuing the bonds is also assumed to follow a geometrical Brownian motion process, and a model of expected credit loss is replaced by Klein (1996). In the fourth model, we consider interest rate risk in the three-factor model and allow for stochastic interest rate by specifying the dynamics of interest rates in Vasicek (1977). For these four models, we derive the corresponding closed-form solutions for pricing the commodity-linked bonds in each model using the Mellin transform techniques, which are simply provided with standard (bivariate) normal cumulative distribution function so that the pricing and hedging of the commodity-linked bonds can be computed very accurately and rapidly.

The remainder of this paper is structured as follows. Section 2 gives a one-factor commodity-linked bond model incorporates with the only uncertain source is the spot underlying commodity price risk. In Sect. 3, we consider the two uncertain risk factors are the spot underlying commodity price and stochastic convenience yield, derive a two-factor closed-form pricing formula for valuing the commodity-linked bonds. A three-factor(I) pricing model together with closed-form solution to the valuation of the commodity-linked bonds is derived in Sect. 4. Section 5 considers interest rate risk in the three-factor model and derives a three-factor(II) pricing model with constant convenience yield for valuing the commodity-linked bonds. Section 6 presents numerical examples to examine if the underlying commodity price, stochastic convenience yield and counterparty credit risk have significant impacts on the values of the commodity-linked bonds. Finally, Sect. 7 concludes this paper. The detailed proofs are shown in the “Appendix”.

2 One-factor commodity-linked bonds value model

This section gives a one-factor model of the commodity-linked bonds. The stochastic factor or state variable in the model is the spot price of the underlying commodity. To develop the one-factor model, we first assume that the spot price of an underlying commodity, S(t), proposed by Brennan and Schwartz (1985), satisfies the following stochastic differential equation (SDE, hereafter):

$$\begin{aligned} \begin{array}{lll} dS_{t}=\mu _{s}S_{t}dt+\sigma _{s}S_{t}dW_{t}^{s}, \end{array} \end{aligned}$$

(1)

where $\sigma _{s}$ represents the volatility of proportional price changes, $\mu _{s}$ denotes the expected rate of price changes, $dW_{t}^{s}$ is the increment to a standard Wiener process.

To obtain the risk-neutral processes for the spot price of the underlying commodity, Brennan and Schwartz (1985) substitutes $\mu _{s}$ by the risk free interest rate r deducts the net convenience yield $\delta$ as the risk adjusted drift of the commodity price process.² Therefore, the stochastic process for the spot price under the equivalent martingale measure can be expressed as:

$$\begin{aligned} \begin{array}{lll} dS_{t}=(r-\delta )S_{t}dt+\sigma _{s}S_{t}dW_{t}^{*s}, \end{array} \end{aligned}$$

(2)

where $dW_{t}^{*s}$ is the increment to a standard Wiener process, r is the risk-free interest rate and $\delta$ represents the current marginal net rate of convenience yield(assumed constant), defined as convenience yield minus physical storage costs, and the convenience yield can be interpreted as the flow of services that accrues to the holder of the physical commodity, but not to the owner of a commodity-linked bond contract for future delivery of the commodity.³

This paper makes a similar assumption of Carr (1987) that there are no payouts from the firm to bondholders before the maturity date of the bond, that is to say no dividends and coupons. Then commodity-linked bonds have promised payment of the bond is equivalent to the face value of a bond F for sure plus a call option, which gives the bearer an option to buy the reference commodity bundle at a pre-specified exercise price K. This payoff structure is an important characteristic of the commodity-linked bonds. Therefore, the payoff of a commodity-linked bond at maturity T is defined as follows:

$$\begin{aligned} \begin{array}{lll} h(S_{T})&=F+\max \{S_{T}-K,0\}, \end{array} \end{aligned}$$

(3)

where F and K are constants.

By the risk neutral pricing rule, the no-arbitrage price of a commodity-linked bond at the time to maturity $\tau$ under measure Q can be written as

$$\begin{aligned} \begin{array}{lll} B(S, \tau )&=e^{-r\tau }E^{Q}_{t}[h(S_{T})\mid S_{t}=S], \end{array} \end{aligned}$$

(4)

satisfies a PDE given by

$$\begin{aligned} \begin{array}{lll} \frac{\partial B}{\partial \tau }&=\frac{1}{2}\sigma _{s}^{2}S^{2}\frac{\partial ^{2} B}{\partial S^{2}}+(r-\delta )S\frac{\partial B}{\partial S}-rB, \end{array} \end{aligned}$$

(5)

with $\tau =T-t$ and the terminal condition $B(T,S)=h(S_{T})$.

In this model the underlying commodity is treated as an asset that pays a continuous dividend at a rate equal to the underlying commodity price S times the net convenience yield $\delta$. Thus, based on the diffusion in Eq. 2, Black and Cox (1976) solve for the commodity option price and obtains the well-known one-factor cost-of-carry formula as follows:

$$\begin{aligned} \begin{array}{lll} C(S, \tau )=e^{-\delta \tau }S\mathcal {N}(d_{1})-e^{-r\tau }K\mathcal {N}(d_{2}), \end{array} \end{aligned}$$

(6)

where $\mathcal {N}$ is the standard normal cumulative distribution as

$$\begin{aligned} \mathcal {N}(d)=\frac{1}{\sqrt{2\pi }}\int _{-\infty }^{d}e^{-\frac{1}{2}x^{2}}dx, \end{aligned}$$

where $d_{1}$ and $d_{2}$ are given by

$$\begin{aligned} d_{1}&=\frac{\ln (\frac{S}{K})+(r+\frac{1}{2}\sigma _{s}^{2}-\delta )\tau }{\sigma _{s}\sqrt{\tau }},\\ d_{2}&=\frac{\ln (\frac{S}{K})+(r-\frac{1}{2}\sigma _{s}^{2}-\delta )\tau }{\sigma _{s}\sqrt{\tau }}. \end{aligned}$$

Then, we give a closed-form formula for the one-factor commodity-linked bonds price. The price of the one-factor commodity-linked bonds, defined by Eq. 4, is expressed by

$$\begin{aligned} \begin{array}{lll} B(\tau , S)=e^{-\delta \tau }S\mathcal {N}(d_{1})-e^{-r\tau }K\mathcal {N}(d_{2})+e^{-r\tau }F, \end{array} \end{aligned}$$

(7)

where $d_{1},d_{2}$ and $\mathcal {N}$ are given by Eq. 6.

3 Two-factor commodity-linked bonds value model

In this section, we adopt a two-factor model of commodity prices analyzed in Schwartz (1997) is based on the model developed in Gibson and Schwartz (1990) and derive the corresponding formula for valuing the commodity-linked bonds. The stochastic factors or state variables in the model are the spot price of the underlying commodity follows a geometrical Brownian motion process with a stochastic drift⁴ and the instantaneous convenience yield is formulated as a mean-reverting Ornstein–Uhlenbeck stochastic process.⁵

3.1 The model formulation

To stat with, we define our stochastic variables and deduce a PDE for valuing the commodity-linked bonds. Let $S_{t}$ be the spot price of the underlying commodity, $\delta _{t}$ represents instantaneous net marginal convenience yield rate. Under the equivalent martingale measure, the joint stochastic process of the two state variables in Schwartz (1997) is given by

$$\begin{aligned}&\begin{array}{lll} dS_{t}=(\mu _{s}-\delta _{t})S_{t}dt+\sigma _{S}S_{t}dW_{t}^{*s}, \end{array} \end{aligned}$$

(8)

$$\begin{aligned}&\begin{array}{lll} d\delta _{t}=\kappa (\theta ^{*}-\delta _{t})dt+\sigma _{\delta }dW_{t}^{*\delta }, \end{array} \end{aligned}$$

(9)

where $\sigma _{S}$ and $\sigma _{\delta }$ are constant volatility,⁶$\kappa >0$ is the speed of adjustment, $\theta ^{*}=\theta -\frac{\lambda _{\delta }\sigma _{\delta }}{\kappa }$ is the long-run mean yield, $\lambda _{\delta }$ are the market prices of risk for the convenience yield, and $dW_{t}^{*s}$ and $dW_{t}^{*\delta }$ are correlated increments to standard Browian processes and $dW_{t}^{*s}dW_{t}^{*\delta }=\rho _{s\delta }dt$. $\rho _{s\delta }$ denotes the positively correlation coefficient between the two Browian motions. From the no-arbitrage condition, the risk-adjusted drift of the price process is $r-\delta$.⁷

Commodity-linked bonds have the same payment structure as the one-factor model. Therefore, by the risk neutral pricing rule, the no-arbitrage price of a commodity-linked bond at the time t under measure Q can be written as

$$\begin{aligned} \begin{array}{lll} B_{t}(\tau , S,\delta )&=e^{-r\tau }E^{Q}_{t}[h(S_{T})\mid S_{t}=S], \end{array} \end{aligned}$$

(10)

satisfies a PDE given by

$$\begin{aligned}&-\frac{\partial B}{\partial \tau }+\frac{1}{2}\sigma _{S}^{2}S^{2}\frac{\partial ^{2} B}{\partial ^{2} S}+\frac{1}{2}\sigma _{\delta }^{2}\frac{\partial ^{2} B}{\partial ^{2}\delta }+\rho _{s\delta }\sigma _{s}\sigma _{\delta }S\frac{\partial ^{2} B}{\partial S \partial \delta }\nonumber \\&\quad +(r-\delta )S\frac{\partial B}{\partial S}+\kappa (\theta ^{*}-\delta )\frac{\partial B}{\partial \delta }=rB, \end{aligned}$$

(11)

with $\tau =T-t$ and the terminal condition $B(T,S,\delta )=h(S_{T})$.

3.2 Derivation of two-factor price formula

In this subsection, we derive the closed-form formula of the two-factor commodity-linked bonds price $B_{t}(t,S,\delta )$ by solving the PDE (11) through the Mellin transform technique. Then, by using Proposition 1, reported in “Appendix A”, Eq. 11 yields

$$\begin{aligned}&-\frac{\partial \hat{B}}{\partial \tau }+[\frac{1}{2}\sigma ^{2}_{S}\omega (\omega +1)-(1+\omega )r+\delta \omega ]\hat{B}+[\kappa (\theta ^{*}-\delta )\nonumber \\&\quad -\rho _{s\delta }\sigma _{s}\sigma _{\delta }\omega ]\frac{\partial \hat{B}}{\partial \delta }+\frac{1}{2}\sigma _{\delta }^{2}\frac{\partial ^{2}\hat{B}}{\partial \delta ^{2}}=0, \end{aligned}$$

(12)

with $\hat{B}(0,\omega ,\delta )=\hat{h}(\omega )$ which is the Mellin transform of h(S).

To simplify (12), we let

$$\begin{aligned} \hat{B}(\tau , \omega )=\exp \left\{ \left[ \frac{1}{2}\sigma ^{2}_{s}\omega (\omega +1)\right] \tau \right\} \hat{f}(\tau ,\omega ,\delta ), \end{aligned}$$

(13)

then Eq. 12 is transformed into the following PDE for $\hat{f}$

$$\begin{aligned} \begin{array}{lll} -\frac{\partial \hat{f}}{\partial \tau }-[(1+\omega )r-\delta \omega ]\hat{f}+[\kappa (\theta ^{*}-\delta )-\rho _{s\delta }\sigma _{s}\sigma _{\delta }\omega ]\frac{\partial \hat{f}}{\partial \delta }+\frac{1}{2}\sigma _{\delta }^{2}\frac{\partial ^{2}\hat{f}}{\partial \delta ^{2}}=0, \end{array} \end{aligned}$$

(14)

with $\hat{f}(0,\omega , \delta )=\hat{B}(0,\omega , \delta )=\hat{h}(\omega )$.

To solve Eq. 14, we let $\hat{f}(\tau ,\omega ,\delta )=\hat{h}(\omega )L(\tau ,\omega )e^{-H(\tau )((1+\omega )r-\delta \omega )}$ with terminal condition $\hat{f}(0,\omega , \delta )=\hat{h}(\omega )$. By substituting this function form into (14), we get the following ODEs with respect to $L(\tau ,\omega )$ and $H(\tau )$

$$\begin{aligned} \left\{ \begin{aligned} -\frac{\partial L}{\partial \tau }+[\kappa \theta ^{*}\omega -\rho _{s\delta }\sigma _{S}\sigma _{\delta }\omega ^{2}-\kappa (1+\omega )r]HL+\frac{1}{2}\sigma _{\delta }^{2}\omega ^{2}H^{2}L&=0,\\ \frac{\partial H}{\partial \tau }+\kappa H-1&=0,\\ \end{aligned} \right. \end{aligned}$$

(15)

where $L(0,\omega )=1$ and $H(0)=0$. By solving (15), we have

$$\begin{aligned} \left\{ \begin{aligned} L(\tau ,\omega )&=\exp \left\{ \frac{\sigma _{\delta }^{2}}{2\kappa ^{2}}(\tau -H(\tau )-\frac{\kappa }{2}H^{2}(\tau ))\omega ^{2}+ [\theta ^{*}\omega -\gamma _{1}\omega ^{2}\right. \\&\left. \quad -(1+\omega )r](\tau -H(\tau )) \right\} ,\\ H(\tau )&=\frac{1-e^{-\kappa \tau }}{\kappa },\\ \end{aligned} \right. \end{aligned}$$

where $\gamma _{1}=\frac{\rho _{s\delta }\sigma _{S}\sigma _{\delta }}{\kappa }$. Then, by substituting $L(\tau ,\omega ),H(\tau )$ and $\hat{f}(\tau ,\omega , \delta )$ into (13), we have

$$\begin{aligned} \begin{array}{lll} \hat{B}(\tau , \omega , \delta )=\hat{h}(\omega )\exp \{\hat{Q}(\tau ,\omega ,\delta )\}, \end{array} \end{aligned}$$

(16)

where

$$\begin{aligned} \hat{Q}(\tau ,\omega ,\delta )&=M(\tau )\omega ^{2}+\frac{1}{2}\sigma ^{2}_{s}\omega (\omega +1)\tau +(\theta ^{*}\omega -\gamma _{1}\omega ^{2})(\tau -H(\tau ))\\&\quad -(1+\omega )\tau r+\delta \omega H(\tau ),\\ M(\tau )&=\frac{\sigma _{\delta }^{2}}{2\kappa ^{2}}(\tau -H(\tau )-\frac{\kappa }{2}H^{2}(\tau )). \end{aligned}$$

Therefore, the price of the two-factor commodity-linked bonds is given

$$\begin{aligned} B(\tau ,\omega , \delta )=\frac{1}{2\pi i}\int ^{c+i\infty }_{c-i\infty }\hat{h}(\omega )\exp (\hat{Q}(\tau ,\omega ,\delta ))S^{-\omega }d\omega . \end{aligned}$$

(17)

Let

$$\begin{aligned} \varphi (\tau ,S,\delta )= & {} \frac{1}{2\pi i}\int ^{c+i\infty }_{c-i\infty }\exp (\hat{Q}(\tau ,\omega ,\delta ))S^{-\omega }d\omega \nonumber \\= & {} \frac{1}{2\pi i}\int ^{c+i\infty }_{c-i\infty }e^{c^{*}}\exp \{E(\tau )(\omega +\frac{G_{2}(\tau )}{2E(\tau )})^{2}\}S^{-\omega }d\omega , \end{aligned}$$

(18)

where

$$\begin{aligned} E(\tau )&=M(\tau )+\frac{1}{2}\sigma _{s}^{2}\tau -\gamma _{1}(\tau -H(\tau )),\\ G_{2}(\tau )&=\frac{1}{2}\sigma _{s}^{2}\tau +\theta ^{*}(\tau -H(\tau ))+\delta H(\tau )-\tau r,\\ c^{*}(\tau )&=-\frac{(G_{2}(\tau ))^{2}}{4E(\tau )} -\tau r. \end{aligned}$$

By setting

$$\begin{aligned} \hat{\sigma }_{s}^{2}(\tau )=\sigma _{s}^{2}-2\rho _{s\delta }\sigma _{s}\sigma _{\delta }H(\tau )+\sigma ^{2}_{\delta }H^{2}(\tau ), \end{aligned}$$

we then have

$$\begin{aligned} \frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(t)dt&= \frac{1}{2}\int ^{\tau }_{0}\sigma _{S}^{2}-2\rho _{s\delta }\sigma _{S}\sigma _{\delta }\frac{1-e^{-\kappa t}}{\kappa }+\sigma ^{2}_{\delta }\frac{(1-e^{-\kappa t})^{2}}{\kappa ^{2}}dt\\&=M(\tau )+\frac{1}{2}\sigma _{s}^{2}\tau -\gamma _{1}(\tau -H(\tau ))\\&=E(\tau )\\&\ge \frac{1}{2}\int ^{\tau }_{0}\left( \sigma _{S}-\sigma _{\delta }\frac{(1-e^{-\kappa t})}{\kappa }\right) ^{2}dt>0 \end{aligned}$$

holds for $\tau >0$. Therefore, by using Proposition 4, reported in “Appendix A”, we have

$$\begin{aligned} \begin{array}{lll} \varphi (\tau ,S,\delta )&=\frac{1}{2\sqrt{\pi E(\tau )}}S^{\frac{G_{2}(\tau )}{2E(\tau )}}e^{c^{*}-\frac{(\ln S)^{2}}{4E(\tau )}}. \end{array} \end{aligned}$$

(19)

Because $\hat{h}(\omega )$ and $\exp \{\hat{Q}(\tau ,\omega ,\delta )\}$ are the Mellin transforms of h(S) and $\varphi (\tau ,S,\delta )$, respectively. Applying Proposition 2, reported in “Appendix A”, we have the following result:

$$\begin{aligned} B(\tau ,S,\delta )= & {} \int _{0}^{\infty }h(u)\varphi (\tau ,\frac{S}{u},\delta )u^{-1}du \nonumber \\= & {} \int _{K}^{\infty }(F+u-K)\varphi (\tau ,\frac{S}{u},\delta )u^{-1}du+\int _{0}^{K}F\varphi (\tau ,\frac{S}{u},\delta )u^{-1}du.\qquad \end{aligned}$$

(20)

Now we give a closed-form formula for the two-factor commodity-linked bonds price.

Theorem 1

The price of the commodity-linked bonds, defined by Eq. 10, is expressed by

$$\begin{aligned} \begin{array}{lll} B(\tau , S,\delta )=P(\tau , \delta )S\mathcal {N}(d_{1})-e^{-r\tau }K\mathcal {N}(d_{2})+e^{-r\tau }F, \end{array} \end{aligned}$$

(21)

where $\mathcal {N}$ is the standard normal cumulative distribution as

$$\begin{aligned} \mathcal {N}(d)=\frac{1}{\sqrt{2\pi }}\int _{-\infty }^{d}e^{-\frac{1}{2}x^{2}}dx, \end{aligned}$$

and

$$\begin{aligned} P(\tau , \delta )&=A(\tau )\exp (-\delta H(\tau )),\\ A(\tau )&=\exp \left\{ (\theta ^{*}+\gamma _{1}-\frac{\sigma _{\delta }^{2}}{2\kappa ^{2}})(H(\tau )-\tau )-\frac{\sigma _{\delta }^{2}}{4\kappa }H^{2}(\tau )\right\} ,\\ \hat{\sigma }_{s}^{2}(\tau )&=\sigma _{s}^{2}-2\rho _{s\delta }\sigma _{s}\sigma _{\delta }H(\tau )+\sigma ^{2}_{\delta }H^{2}(\tau ),\\ H(\tau )&=\frac{1-e^{-\kappa \tau }}{\kappa },\\ \gamma _{1}&=\frac{\rho _{s\delta }\sigma _{S}\sigma _{\delta }}{\kappa },\\ d_{1}&=\frac{\ln (\frac{S}{K})+r\tau +\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(t)dt+\ln (P(\tau ,\delta ))}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}},\\ d_{2}&=\frac{\ln (\frac{S}{K})+r\tau -\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(t)dt+\ln (P(\tau ,\delta ))}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}}. \end{aligned}$$

Proof

See “Appendix B”. $\square$

4 Three-factor commodity-linked bonds value model under constant interest rate

This section presents a three-factor model of the commodity-linked bonds. The stochastic factors or state variables in the model are the spot price of the underlying commodity, the value of the firm and the instantaneous convenience yield. Given that our proposed three-factor model can be seen as a reformulated version of the Miura and Yamauchi (1998) model by replacing a model of expected credit loss proposed by Klein (1996) in this paper.

4.1 The model formulation

Let $V_{t}$ designate the total value of the assets of the firm issuing the bonds and follow a geometrical Brownian motion process. Then the joint stochastic process for the factors $S_{t}$, $V_{t}$ and $\delta _{t}$ under the equivalent martingale measure can be expressed as:

$$\begin{aligned}&\begin{array}{lll} dS_{t}=(\mu _{s}-\delta _{t})S_{t}dt+\sigma _{S}S_{t}dW_{t}^{*s}, \end{array} \end{aligned}$$

(22)

$$\begin{aligned}&\begin{array}{lll} d\delta _{t}=\kappa (\theta ^{*}-\delta _{t})dt+\sigma _{\delta }dW_{t}^{*\delta }, \end{array} \end{aligned}$$

(23)

$$\begin{aligned}&\begin{array}{lll} dV_{t}=(\mu _{v}-\lambda _{v}\sigma _{V})V_{t}dt+\sigma _{V}V_{t}dW_{t}^{*v}, \end{array} \end{aligned}$$

(24)

where $\sigma _{S}$, $\sigma _{\delta }$ and $\sigma _{V}$ are constant volatility, $\lambda _{v}$ is the market prices of risk for the value of the firm, $\theta ^{*}$ is the long-run mean yield, and $W_{t}^{*s}$,$W_{t}^{*v}$ and $W_{t}^{*\delta }$ are the stand Wiener processes and their correlation are such that $dW_{t}^{*s}dW_{t}^{*v}=\rho _{sv}dt$, $dW_{t}^{*s}dW_{t}^{*\delta }=\rho _{s\delta }dt$ and $dW_{t}^{*\delta }dW_{t}^{*v}=\rho _{\delta v}dt$. Also, the correlation coefficients $\rho _{sv}$, $\rho _{s\delta }$ and $\rho _{\delta v}$ are constant parameters satisfying

$$\begin{aligned} \begin{array}{lll} 0<\rho _{sv}<1, 0<\rho _{s\delta }<1, 0<\rho _{\delta v}<1, \rho _{sv}^{2}+\rho _{s\delta }^{2}+\rho _{\delta v}^{2}-2\rho _{sv}\rho _{s\delta }\rho _{\delta v}-1<0. \end{array} \end{aligned}$$

(25)

Commodity-linked bonds have the promised payment on the bonds at the maturity such that the bondholder has right to receive, in case of no default, the face value F of the bonds plus an option to buy the reference commodity bundle at a pre-specified exercise price K. The promised payment can only be made if the value of the firm $V_{T}$ at maturity is greater than that amount $D^{*}$. Otherwise, in case where the default is considered, following Klein (1996), a credit loss occurs if the value of the firm at maturity is less than some amount $D^{*}$. This amount is not set to the value of the bond but roughly corresponds the amount claims D outstanding at maturity. $D^{*}$ may be less than D due to the possibility of a bond issuer continuing in operation even while $V_{T}$ is less than D. Once a credit loss occurs at maturity, the recovery rate is $\frac{(1-\alpha )V_{T}}{D}$, where $\alpha V_{T}$ represents the deadweight costs due to the bankruptcy or reorganization, and the remaining value of $(1-\alpha )V_{T}$ is paid to the bondholders and other liabilities. The payoff of a commodity-linked bond at maturity is defined as follows:

$$\begin{aligned} \begin{array}{lll} h(S_{T},V_{T})&{}=(F+\max \{S_{T}-K,0\})[1_{\{V_{T}\ge D^{*}\}}+1_{\{V_{T}< D^{*}\}}\frac{(1-\alpha )V_{T}}{D}]\\ &{}=\max \{F+S_{T}-K,F\}[1_{\{V_{T}\ge D^{*}\}}+1_{\{V_{T}< D^{*}\}}\frac{(1-\alpha )V_{T}}{D}], \end{array} \end{aligned}$$

(26)

where F and K are constants.

By the risk neutral pricing rule, the no-arbitrage price of a commodity-linked bond at the time to maturity $\tau$ under measure Q can be written as

$$\begin{aligned} \begin{array}{lll} B(\tau , S,V,\delta )&=e^{-r\tau }E^{Q}_{t}[h(S_{T},V_{T})\mid S_{t}=S, V_{t}=V, \delta _{t}=\delta ], \end{array} \end{aligned}$$

(27)

satisfies a PDE given by

$$\begin{aligned} \begin{array}{lll} \frac{\partial B}{\partial \tau }&{}=\frac{1}{2}\sigma _{s}^{2}S^{2}\frac{\partial ^{2} B}{\partial S^{2}}+\frac{1}{2}\sigma _{v}^{2}V^{2}\frac{\partial ^{2} B}{\partial V^{2}}+\frac{1}{2}\sigma _{\delta }^{2}\frac{\partial ^{2} B}{\partial \delta ^{2}}+\rho _{sv}\sigma _{s}\sigma _{v}SV\frac{\partial ^{2} B}{\partial S \partial V}\\ &{}\quad +\rho _{s\delta }\sigma _{s}\sigma _{\delta }s\frac{\partial ^{2} B}{\partial S \partial \delta }+\rho _{\delta v}\sigma _{\delta }\sigma _{v}V\frac{\partial ^{2} B}{\partial \delta \partial V}+(r-\delta )S\frac{\partial B}{\partial s}+(r-\lambda _{v}\sigma _{v})V\frac{\partial B}{\partial V}\\ &{}\quad +\kappa (\theta ^{*}-\delta )\frac{\partial B}{\partial \delta }-rB, \end{array} \end{aligned}$$

(28)

with $\tau =T-t$ and the terminal condition $B(T,S,V,\delta )=h(S_{T},V_{T})$.

4.2 Derivation of three-factor price formula

In this subsection, we derive the closed-form formula of the commodity-linked bonds price $B_{t}(\tau ,S,V,\delta )$ by solving the PDE (28) through the double Mellin transform technique. Then, by using Proposition 1, reported in “Appendix A”, Eq. 28 yields

$$\begin{aligned} \begin{array}{lll} &{}-\frac{\partial \hat{B}}{\partial \tau }+[\frac{1}{2}\sigma ^{2}_{S}\omega _{1}(\omega _{1}+1)+\rho _{sv}\sigma _{s}\sigma _{v}\omega _{1}\omega _{2}+\frac{1}{2}\sigma ^{2}_{v}\omega _{2}(\omega _{2}+1)-(1+\omega _{1}+\omega _{2})r \\ &{}\quad +\delta \omega _{1}+\lambda _{v}\sigma _{v}\omega _{2}]\hat{B}+[\kappa (\theta ^{*}-\delta )-\rho _{s\delta }\sigma _{s}\sigma _{\delta }\omega _{1}-\rho _{\delta v}\sigma _{\delta }\sigma _{v}\omega _{2}]\frac{\partial \hat{B}}{\partial \delta }+\frac{1}{2}\sigma _{\delta }^{2}\frac{\partial ^{2}\hat{B}}{\partial \delta ^{2}} =0, \end{array} \end{aligned}$$

(29)

with $\hat{B}(0,\omega _{1},\omega _{2},\delta )=\hat{h}(\omega _{1},\omega _{2})$ which is the double Mellin transform of h(S, V).

To simplify Eq. 29, we let

$$\begin{aligned} \begin{array}{lll} \hat{B}(\tau , \omega _{1},\omega _{2}, \delta )&{}=\exp \{[\frac{1}{2}\sigma ^{2}_{s}\omega _{1}(\omega _{1}+1)+\rho _{sv}\sigma _{s}\sigma _{v}\omega _{1}\omega _{2}\\ &{}\quad +\frac{1}{2}\sigma ^{2}_{v}\omega _{2}(\omega _{2}+1)]\tau \}\hat{f}(\tau ,\omega _{1},\omega _{2}, \delta ), \end{array} \end{aligned}$$

(30)

then Eq. 29 is transformed into the following PDE for $\hat{f}$

$$\begin{aligned} \begin{array}{lll} &{}-\frac{\partial \hat{f}}{\partial \tau }-[(1+\omega _{1}+\omega _{2})r-\delta \omega _{1}-\lambda _{v}\sigma _{v}\omega _{2}]\hat{f}+[(\kappa (\theta ^{*}-\delta )\\ &{}\quad -\rho _{s\delta }\sigma _{s}\sigma _{\delta }\omega _{1}-\rho _{\delta v}\sigma _{\delta }\sigma _{v}\omega _{2}]\frac{\partial \hat{f}}{\partial \delta }+\frac{1}{2}\sigma _{\delta }^{2}\frac{\partial ^{2}\hat{f}}{\partial \delta ^{2}}=0, \end{array} \end{aligned}$$

(31)

with $\hat{f}(0,\omega _{1},\omega _{2}, \delta )=\hat{B}(0,\omega _{1},\omega _{2}, \delta )=\hat{h}(\omega _{1},\omega _{2})$.

To solve (31), we let $\hat{f}(\tau ,\omega _{1},\omega _{2}, \delta )=\hat{h}(\omega _{1},\omega _{2})L(\tau ,\omega _{1},\omega _{2}) e^{-H(\tau )((1+\omega _{1}+\omega _{2})r-\delta \omega _{1}-\lambda _{v}\sigma _{v}\omega _{2})}$ with terminal condition $\hat{f}(0,\omega _{1},\omega _{2}, \delta )=\hat{h}(\omega _{1},\omega _{2})$. By substituting this function form into (31), we get the following ODEs with respect to $L(\tau ,\omega _{1},\omega _{2})$ and $H(\tau )$

$$\begin{aligned} \left\{ \begin{array}{l} \displaystyle -\frac{\partial L}{\partial \tau }+[\kappa \theta ^{*}\omega _{1}-\rho _{s\delta }\sigma _{S}\sigma _{\delta }\omega _{1}^{2}-\rho _{\delta v}\sigma _{\delta }\sigma _{v}\omega _{1}\omega _{2}\\ \displaystyle -\kappa (1+\omega _{1}+\omega _{2})r+\kappa \lambda _{v}\sigma _{v}\omega _{2}]HL+\frac{1}{2}\sigma _{\delta }^{2}\omega _{1}^{2}H^{2}L=0,\\ \displaystyle \frac{\partial H}{\partial \tau }+\kappa H-1=0, \end{array} \right. \end{aligned}$$

(32)

where $L(0,\omega _{1},\omega _{2})=1$ and $H(0)=0$. By solving the ODEs (32), we have

$$\begin{aligned} \left\{ \begin{aligned}&L(\tau ,\omega _{1},\omega _{2})=\exp \left\{ \frac{\sigma _{\delta }^{2}}{2\kappa ^{2}}(\tau -H(\tau )-\frac{\kappa }{2}H^{2}(\tau ))\omega _{1}^{2}+ [\theta ^{*}\omega _{1}-\gamma _{1}\omega _{1}^{2}\right. \\&\quad \left. \quad -\gamma _{2}\omega _{1}\omega _{2}-(1+\omega _{1}+\omega _{2})r+\lambda _{v}\sigma _{v}\omega _{2}](\tau -H(\tau ))\right\} ,\\&H(\tau )=\frac{1-e^{-\kappa \tau }}{\kappa },\\ \end{aligned} \right. \end{aligned}$$

where $\gamma _{1}=\frac{\rho _{s\delta }\sigma _{S}\sigma _{\delta }}{\kappa }$ and $\gamma _{2}=\frac{\rho _{\delta v}\sigma _{\delta }\sigma _{v}}{\kappa }$. Then, by substituting $L(\tau ,\omega _{1},\omega _{2}), H(\tau )$ and $\hat{f}(\tau ,\omega _{1},\omega _{2}, \delta )$ into (30), we have

$$\begin{aligned} \begin{array}{lll} \hat{B}(\tau , \omega _{1},\omega _{2}, \delta )=\hat{h}(\omega _{1},\omega _{2})\exp (\hat{Q}(\tau ,\omega _{1},\omega _{2},\delta )), \end{array} \end{aligned}$$

(33)

where

$$\begin{aligned} \hat{Q}(\tau ,\omega _{1},\omega _{2},\delta )&=M(\tau )\omega _{1}^{2}+\left[ \frac{1}{2}\sigma ^{2}_{s}\omega _{1}(\omega _{1}+1)+\rho _{sv}\sigma _{s}\sigma _{v}\omega _{1}\omega _{2}+\frac{1}{2}\sigma ^{2}_{v}\omega _{2}(\omega _{2}+1)\right] \tau \\&\quad +\left[ \theta ^{*}\omega _{1}-\gamma _{1}\omega _{1}^{2}-\gamma _{2}\omega _{1}\omega _{2}\right] (\tau -H(\tau ))-(1+\omega _{1}+\omega _{2})\tau r\\&\quad +\lambda _{v}\sigma _{v}\omega _{2}\tau +\delta \omega _{1}H(\tau ),\\ M(\tau )&=\frac{\sigma _{\delta }^{2}}{2\kappa ^{2}}\left( \tau -H(\tau )-\frac{\kappa }{2}H^{2}(\tau )\right) . \end{aligned}$$

Therefore, the price of the three-factor commodity-linked bonds is given

$$\begin{aligned} B(\tau ,S,V, \delta )=\frac{1}{(2\pi i)^{2}}\int ^{c_{1}+i\infty }_{c_{1}-i\infty }\int ^{c_{2}+i\infty }_{c_{2}-i\infty }\hat{h}(\omega _{1},\omega _{2})\exp (\hat{Q}(\tau ,\omega _{1},\omega _{2},\delta ))S^{-\omega _{1}}V^{-\omega _{2}}d\omega _{1}d\omega _{2}. \end{aligned}$$

(34)

Let

$$\begin{aligned} \varphi (\tau ,S,V,\delta )= & {} \frac{1}{(2\pi i)^{2}}\int ^{c_{1}+i\infty }_{c_{1}-i\infty }\int ^{c_{2}+i\infty }_{c_{2}-i\infty }\exp (\hat{Q}(\tau ,\omega _{1},\omega _{2},\delta ))S^{-\omega _{1}}V^{-\omega _{2}}d\omega _{1}d\omega _{2}\nonumber \\= & {} \frac{1}{(2\pi i)^{2}}\int ^{c_{2}+i\infty }_{c_{2}-i\infty }e^{c^{*}}\left[ \int ^{c_{1}+i\infty }_{c_{1}-i\infty }\exp \left\{ E(\tau )\left( \omega _{1}+\frac{G_{2}(\tau )+G(\tau )\omega _{2}}{2E(\tau )}\right) ^{2}\right\} \right. \nonumber \\&\left. \quad \cdot S^{-\omega _{1}}d\omega _{1}\right] V^{-\omega _{2}}d\omega _{2}, \end{aligned}$$

(35)

where

$$\begin{aligned} E(\tau )&=M(\tau )+\frac{1}{2}\sigma _{s}^{2}\tau -\gamma _{1}(\tau -H(\tau )),\\ G(\tau )&=\rho _{sv}\sigma _{s}\sigma _{v}\tau -\gamma _{2}(\tau -H(\tau )),\\ G_{2}(\tau )&=\frac{1}{2}\sigma _{s}^{2}\tau +\theta ^{*}(\tau -H(\tau ))+\delta H(\tau )-\tau r,\\ c^{*}(\tau )&=-\frac{(G_{2}(\tau )+G(\tau )\omega _{2})^{2}}{4E(\tau )} -\tau r+\frac{1}{2}\sigma ^{2}_{v}\tau \omega _{2}^{2}+\left( \frac{1}{2}\sigma ^{2}_{v}+\lambda _{v}\sigma _{v}-r\right) \tau \omega _{2}. \end{aligned}$$

Because $E(\tau )>0$ holds for $\tau >0$, so by using Proposition 4, reported in “Appendix A”, we have

$$\begin{aligned}&\int ^{c_{1}+i\infty }_{c_{1}-i\infty }\exp \left\{ E(\tau )\left( \omega _{1}+\frac{G_{2}(\tau )+G(\tau )\omega _{2}}{2E(\tau )}\right) ^{2}\right\} S^{-\omega _{1}}d\omega _{1}\\&\quad =\frac{1}{2\sqrt{\pi E(\tau )}} S^{\frac{G_{2}(\tau )+G(\tau )\omega _{2}}{2 E(\tau )}}e^{-\frac{(\ln S)^{2}}{4 E(\tau )}}, \end{aligned}$$

then Eq. 35 becomes

$$\begin{aligned} \varphi (\tau ,S,V,\delta )=\frac{e^{-\frac{(\ln S)^{2}}{4 E(\tau )}}}{2\sqrt{\pi E(\tau )}} S^{\frac{G_{2}(\tau )}{2 E(\tau )}}\frac{1}{2\pi i}\int ^{c_{1}+i\infty }_{c_{1}-i\infty }e^{c^{*}}S^{\frac{G(\tau )\omega _{2}}{2 E(\tau )}}V^{-\omega _{2}}d\omega _{2}. \end{aligned}$$

(36)

Let

$$\begin{aligned} \psi (\tau ,V,\delta )= & {} \frac{1}{2\pi i}\int ^{c_{2}+i\infty }_{c_{2}-i\infty }e^{c^{*}}S^{\frac{G(\tau )\omega _{2}}{2 E(\tau )}}V^{-\omega _{2}}d\omega _{2}\nonumber \\= & {} e^{C(\tau )}\frac{1}{2\pi i}\int ^{c_{2}+i\infty }_{c_{2}-i\infty }\exp \Bigg \{(G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )})\Bigg [\omega _{2}\nonumber \\&\quad +\frac{G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )(G_{2}(\tau )-\ln S)}{2E(\tau )}}{2(G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )})}\Bigg ]^{2}\Bigg \}V^{-\omega _{2}}d\omega _{2}, \end{aligned}$$

(37)

where

$$\begin{aligned} G_{1}(\tau )&=\frac{1}{2}\sigma _{v}^{2}\tau ,\\ G_{3}(\tau )&=(\lambda _{v}\sigma _{v}-r)\tau ,\\ C(\tau )&=-\tau r-\frac{G_{2}^{2}(\tau )}{4E(\tau )}-\frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )(G_{2}(\tau )-\ln S)}{2E(\tau )}\right) ^{2}}{4(G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )})}. \end{aligned}$$

In order to apply inverse Mellin transform of exponential function, the condition $(G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )})>0$ needs to be guaranteed. Therefore, we first introduce the following lemma which is useful in our analysis.

Lemma 1

If $\rho _{sv}$, $\rho _{s\delta }$ and $\rho _{\delta v}$ are constants satisfying conditions (25), then $G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )}>0$ is satisfied for $\tau >0$.

Proof

See “Appendix C”. $\square$

Therefore, using Proposition 4, reported in “Appendix A”, we have

$$\begin{aligned} \begin{array}{lll} \psi (\tau ,V,\delta )=e^{C(\tau )}\frac{\exp \left\{ -\frac{(\ln V)^{2}}{4\left( G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )}\right) }\right\} }{2\sqrt{\pi \left( G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )}\right) }}V^{\frac{G_{1}(\tau ) +G_{3}(\tau )-\frac{G(\tau )(G_{2}(\tau )-\ln S)}{2E(\tau )}}{2\left( G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )}\right) }}. \end{array} \end{aligned}$$

(38)

Combining (34), (36) and (38) together, we obtain the following explicit result of $\varphi (\tau ,S,V,\delta )$

$$\begin{aligned} \begin{array}{lll} \varphi (\tau ,S,V,\delta )&=e^{C(\tau )}S^{\frac{G_{2}(\tau )}{2 E(\tau )}}V^{\frac{G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )(G_{2}(\tau )-\ln S)}{2E(\tau )}}{2(G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )})}}\frac{e^{-\frac{(\ln S)^{2}}{4 E(\tau )}}}{2\sqrt{\pi E(\tau )}}\frac{\exp \left\{ -\frac{(\ln V)^{2}}{4\left( G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )}\right) }\right\} }{2\sqrt{\pi (G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )})}}. \end{array} \end{aligned}$$

(39)

Because $\hat{h}(\omega _{1},\omega _{2})$ and $\exp \{\hat{Q}(\tau ,\omega _{1},\omega _{2},\delta )\}$ are the double Mellin transforms of h(S, V) and $\varphi (\tau ,S,V,\delta )$, respectively. Applying Proposition 3, reported in “Appendix A”, we have the following result

$$\begin{aligned} B(\tau ,S,V,\delta )= & {} \int _{0}^{\infty }\int _{0}^{\infty }h(u,w)\varphi (\tau ,\frac{S}{u},\frac{V}{w},\delta )u^{-1}w^{-1}dudw\nonumber \\= & {} \int _{K}^{\infty }\int _{D^{*}}^{\infty }(F+u-K)\varphi (\tau ,\frac{S}{u},\frac{V}{w},\delta )u^{-1}w^{-1}dudw\nonumber \\&\quad +\int _{0}^{K}\int _{D^{*}}^{\infty }F\varphi (\tau ,\frac{S}{u},\frac{V}{w},\delta )u^{-1}w^{-1}dudw\nonumber \\&\quad +\frac{1-\alpha }{D}\int _{K}^{\infty }\int _{0}^{D^{*}}w(F+u-K)\varphi (\tau ,\frac{S}{u},\frac{V}{w},\delta )u^{-1}w^{-1}dudw\nonumber \\&\quad +\frac{1-\alpha }{D}\int _{0}^{K}\int _{0}^{D^{*}}w F\varphi (\tau ,\frac{S}{u},\frac{V}{w},\delta )u^{-1}w^{-1}dudw. \end{aligned}$$

(40)

Setting

$$\begin{aligned} \eta&=-\tau r-\frac{G_{2}^{2}(\tau )}{4E(\tau )},\\ \theta _{1}(u)&=-\frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )(G_{2}(\tau ) -\ln (\frac{S}{u}))}{2E(\tau )}\right) ^{2}}{4(G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )})},\\ \theta _{2}(u,w)&=\left( \frac{S}{u}\right) ^{\frac{G_{2}(\tau )}{2 E(\tau )}}\left( \frac{V}{w}\right) ^{\frac{G_{1} (\tau )+G_{3}(\tau )-\frac{G(\tau )\left( G_{2}(\tau )-\ln \left( \frac{S}{u}\right) \right) }{2E(\tau )}}{2(G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )})}} \frac{e^{-\frac{\left( \ln \left( \frac{S}{u}\right) \right) ^{2}}{4 E(\tau )}}}{2\sqrt{\pi E(\tau )}} \frac{\exp \left\{ -\frac{\left( \ln \left( \frac{V}{w}\right) \right) ^{2}}{4\left( G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )}\right) }\right\} }{2\sqrt{\pi \left( G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )}\right) }}. \end{aligned}$$

Then Eq. 40 yields

$$\begin{aligned} \begin{array}{lll} B(\tau , S,V,\delta )&{}=e^{\eta }\int _{K}^{\infty }\int _{D^{*}}^{\infty }e^{\theta _{1}(u)}(F+u-K)\theta _{2}(u,w)u^{-1}w^{-1}dudw\\ &{}\quad +e^{\eta }\int _{0}^{K}\int _{D^{*}}^{\infty }e^{\theta _{1}(u)}F\theta _{2}(u,w)u^{-1}w^{-1}dudw\\ &{}\quad +e^{\eta }\frac{1-\alpha }{D}\int _{K}^{\infty }\int _{0}^{D^{*}}e^{\theta _{1}(u)}w(F+u-K)\theta _{2}(u,w)u^{-1}w^{-1}dudw\\ &{}\quad +e^{\eta }\frac{1-\alpha }{D}\int _{0}^{K}\int _{0}^{D^{*}}e^{\theta _{1}(u)}w F\theta _{2}(u,w)u^{-1}w^{-1}dudw\\ &{}=I_{B}^{1}(S,V,\delta ,\tau )+I_{B}^{2}(S,V,\delta ,\tau )+I_{B}^{3}(S,V,\delta ,\tau )+I_{B}^{4}(S,V,\delta ,\tau ). \end{array} \end{aligned}$$

(41)

Now we give a closed-form formula for the three-factor commodity-linked bonds price.

Theorem 2

The price of the commodity-linked bonds, defined by Eq. 27, is expressed by

$$\begin{aligned} \begin{array}{lll} B(\tau , S,V,\delta )&{}=P(\tau ,\delta )S\mathcal {N}_{2}(a'_{1},b'_{1},\rho )+e^{-r\tau }(F-K)\mathcal {N}_{2}(a'_{2},b'_{2},\rho )\\ &{}\quad +e^{-r\tau }F\mathcal {N}_{2}(-c'_{2},d'_{2},-\rho )\\ &{}\quad +\frac{(1-\alpha )V}{D}(P'(\delta ,\tau )Se^{(r+\rho _{sv}\sigma _{s}\sigma _{v}-\lambda _{v}\sigma _{v})\tau }\mathcal {N}_{2}(e'_{1},-f'_{1},-\rho )\\ &{}\quad +e^{-\lambda _{v}\sigma _{v}\tau }(F-K)\mathcal {N}_{2}(e'_{2},-f'_{2},-\rho ))\\ &{}\quad +\frac{(1-\alpha )V}{D}e^{-\lambda _{v}\sigma _{v}\tau }F\mathcal {N}_{2}(-g'_{2},-h'_{2},\rho ), \end{array} \end{aligned}$$

(42)

where $\mathcal {N}_{2}$ is the standard bivariate normal cumulative distribution as

$$\begin{aligned} \mathcal {N}_{2}(a,b,\rho )=\frac{1}{2\pi \sqrt{1-\rho ^{2}}}\int _{-\infty }^{a}\int _{-\infty }^{b}e^{-\frac{1}{2(1-\rho ^{2})}(x^{2}-2\rho xy+y^{2})}dxdy, \end{aligned}$$

and

$$\begin{aligned} \rho&=\frac{(\rho _{sv}\sigma _{s}\sigma _{v}-\gamma _{2})\tau +\gamma _{2}H(\tau )}{\sigma _{v}\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds\tau }},\\ a'_{1}&=\frac{\ln \left( \frac{S}{K}\right) +\ln P(\delta ,\tau )+r\tau +\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}{\sqrt{\int ^{\tau }_{\tau }\hat{\sigma }^{2}_{s}(s)ds}},\\ b'_{1}&=\frac{\ln \left( \frac{V}{D^{*}}\right) +\left( r+\rho _{sv}\sigma _{s}\sigma _{v}-\gamma _{2}-\lambda _{v}\sigma _{v}-\frac{1}{2}\sigma _{v}^{2}\right) \tau +\gamma _{2}H(\tau )}{\sigma _{v}\sqrt{\tau }},\\ a'_{2}&=\frac{\ln \left( \frac{S}{K}\right) +\ln P(\delta ,\tau )+(r-\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}},\\ b'_{2}&=\frac{\ln \left( \frac{V}{D^{*}}\right) +\left( r-\lambda _{v}\sigma _{v}-\frac{1}{2}\sigma _{v}^{2}\right) \tau }{\sigma _{v}\sqrt{\tau }},\\ e'_{1}&=\frac{\ln \left( \frac{S}{K}\right) +\ln P'(\delta ,\tau )+(r+\rho _{sv}\sigma _{s}\sigma _{v})\tau +\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}},\\ f'_{1}&=\frac{\ln \left( \frac{V}{D^{*}}\right) +\left( r+\rho _{sv}\sigma _{s}\sigma _{v}-\gamma _{2}-\lambda _{v}\sigma _{v}+\frac{1}{2}\sigma _{v}^{2}\right) \tau +\gamma _{2}H(\tau )}{\sigma _{v}\sqrt{\tau }},\\ e'_{2}&=\frac{\ln \left( \frac{S}{K}\right) +\ln P'(\delta ,\tau )+ (r+\rho _{sv}\sigma _{s}\sigma _{v})\tau -\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}},\\ f'_{2}&=\frac{\ln \left( \frac{V}{D^{*}}\right) +(r-\lambda _{v}\sigma _{v}+\frac{1}{2}\sigma _{v}^{2})\tau }{\sigma _{v}\sqrt{\tau }},\\ c'_{2}&=a'_{2}, d'_{2}=b'_{2},g'_{2}=e'_{2},h'_{2}=f'_{2}. \end{aligned}$$

Proof

See “Appendix D”. $\square$

5 Three-factor commodity-linked bonds value model under stochastic interest rate

This section investigates the effect of stochastic interest rate on commodity-linked bonds prices. The model given in the three-factor model is extended to a world with stochastic interest rate, but it assumes a constant convenience yield. Therefore, the stochastic factors or state variables in the model are the spot price of the underlying commodity, the value of the firm and the interest rate. To allow for random interest rate in the model, the short-term riskless interest rate r is assumed to evolve according to a Vasicek type of mean-reverting process under the equivalent probability measure:

$$\begin{aligned} \begin{array}{lll} dr=q(m^{*}-r)dt+\sigma _{r}dW^{*r}_{t}, \end{array} \end{aligned}$$

(43)

where $q, m^{*}$ and $\sigma _{r}$ are positive constant parameters, $W^{*r}_{t}$ is a standard Wiener process.

In the Vasicek (1977) framework, the price of a default-free unit discount bond is expressed by

$$\begin{aligned} B^{*}(r,\tau )&=\zeta (\tau )e^{-\varsigma (\tau )r},\\ \zeta (\tau )&=\exp \left\{ \left( m^{*}-\frac{\sigma _{r}^{2}}{2q^{2}}\right) (\varsigma (\tau )-\tau )-\frac{\sigma _{r}^{2}}{4q}\varsigma ^{2}(\tau )\right\} ,\\ \varsigma (\tau )&=\frac{1-e^{-q \tau }}{q},\\ m^{*}&=m-\frac{\sigma _{r}}{q}\lambda _{r}, \end{aligned}$$

where $\lambda _{r}$ represents the market prices of risk for the interest rate and is assumed to be constant. The relationship between $dW^{*s}_{t}$, $dW^{*v}_{t}$ and $dW^{*r}_{t}$ are as follows: $dW_{t}^{*s}dW_{t}^{*v}=\rho _{sv}dt$, $dW_{t}^{*s}dW_{t}^{*r}=\rho _{sr}dt$ and $dW_{t}^{*r}dW_{t}^{*v}=\rho _{r v}dt$. Also, the correlation coefficients $\rho _{sv}$, $\rho _{sr}$ and $\rho _{r v}$ are constant parameters satisfying

$$\begin{aligned} \begin{array}{lll} 0<\rho _{sv}<1, -1<\rho _{sr}<1, -1<\rho _{r v}<1, \rho _{sv}^{2}+\rho _{sr}^{2}+\rho _{r v}^{2}-2\rho _{sv}\rho _{sr}\rho _{r v}-1<0. \end{array} \end{aligned}$$

(44)

By the risk neutral pricing rule, the no-arbitrage price $B(\tau ,S,V,r)$ of a commodity-linked bond at the time to maturity $\tau$ under measure Q can be written as

$$\begin{aligned} \begin{array}{lll} B(S,V,r,\tau )&=e^{-r\tau }E^{Q}_{t}[h(S_{T},V_{T})\mid S_{t}=S, V_{t}=V, r_{t}=r], \end{array} \end{aligned}$$

(45)

satisfies a PDE given by

$$\begin{aligned} \begin{array}{lll} \frac{\partial B}{\partial \tau }&{} =\frac{1}{2}\sigma _{s}^{2}S^{2}\frac{\partial ^{2} B}{\partial S^{2}} +\frac{1}{2}\sigma _{v}^{2}V^{2}\frac{\partial ^{2} B}{\partial V^{2}}+\frac{1}{2}\sigma _{r}^{2}\frac{\partial ^{2}B}{\partial r^{2}}+\rho _{sv}\sigma _{s}\sigma _{v}SV\frac{\partial ^{2}B}{\partial S \partial V}+\rho _{sr}\sigma _{s}\sigma _{r}S\frac{\partial ^{2}B}{\partial S\partial r}\\ {} &{}\quad +\rho _{r v}\sigma _{r}\sigma _{v}V\frac{\partial ^{2}B}{\partial r \partial V}+(r-\delta )S\frac{\partial B}{\partial s}+(r-\lambda _{v}\sigma _{v})V\frac{\partial B}{\partial V}+q(m^{*}-r)\frac{\partial B}{\partial r}-rB, \end{array} \end{aligned}$$

(46)

with the boundary conditions $B(\tau , 0,V,r)=0$ and $B(0,S,V,r)=h(S_{T},V_{T})=\max \{F+S_{T}-K,F\}[1_{\{V_{T}\ge D^{*}\}}+1_{\{V_{T}< D^{*}\}}\frac{(1-\alpha )V_{T}}{D}]$, where $h(S_{T},V_{T})$ is defined in Eq. 26.

Now we follow the same procedure as described above, by applying the doubly Mellin transform techniques, we get a closed-form formula for the three-factor commodity-linked bonds price under stochastic interest rate.

Theorem 3

The price of the commodity-linked bonds, defined by Eq. 45, is expressed by

$$\begin{aligned} \begin{array}{lll} B(\tau , S,V,r)&{}=e^{-\delta \tau }S\mathcal {N}_{2}(a'_{1},b'_{1},\rho )+B^{*}(r,\tau )(F-K)\mathcal {N}_{2}(a'_{2},b'_{2},\rho )\\ &{}\quad +B^{*}(r,\tau )F\mathcal {N}_{2}(-c'_{2},d'_{2},-\rho )\\ &{}\quad +\frac{(1-\alpha )V}{D}(\frac{S}{B^{*}(r,\tau )}e^{\int _{0}^{\tau }\rho _{sv}\sigma _{s}\sigma _{v}-\lambda _{v}\sigma _{v}-\delta +(\rho _{sr}\sigma _{s}\sigma _{r}+\rho _{rv}\sigma _{r}\sigma _{v})\varsigma (s)ds}\mathcal {N}_{2}(e'_{1},-f'_{1},-\rho )\\ &{}\quad +e^{-\lambda _{v}\sigma _{v}\tau }(F-K)\mathcal {N}_{2}(e'_{2},-f'_{2},-\rho ))\\ &{}\quad +\frac{(1-\alpha )V}{D}e^{-\lambda _{v}\sigma _{v}\tau }F\mathcal {N}_{2}(-g'_{2},-h'_{2},\rho ), \end{array} \end{aligned}$$

(47)

where $\mathcal {N}_{2}$ is the standard bivariate normal cumulative distribution as

$$\begin{aligned} \mathcal {N}_{2}(a,b,\rho )=\frac{1}{2\pi \sqrt{1-\rho ^{2}}}\int _{-\infty }^{a}\int _{-\infty }^{b}e^{-\frac{1}{2(1-\rho ^{2})}(x^{2}-2\rho xy+y^{2})}dxdy, \end{aligned}$$

and

$$\begin{aligned}&\hat{\sigma }_{s}^{2}(\tau )=\sigma _{s}^{2}-2\rho _{sr}\sigma _{s}\sigma _{r}\varsigma (\tau )+\sigma ^{2}_{r}\varsigma ^{2}(\tau ),\\&\hat{\sigma }_{v}^{2}(\tau )=\sigma _{v}^{2}-2\rho _{rv}\sigma _{r}\sigma _{v}\varsigma (\tau )+\sigma ^{2}_{r}\varsigma ^{2}(\tau ),\\&\rho =\frac{(\int _{0}^{\tau }\rho _{sv}\sigma _{s}\sigma _{v}+(\rho _{sr}\sigma _{s}\sigma _{r}+\rho _{rv}\sigma _{r}\sigma _{v})\varsigma (s)+\sigma _{r}^{2}\varsigma ^{2}(s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{v}(s)ds}},\\&a'_{1}=\frac{\ln \left( \frac{S}{K}\right) -\ln B^{*}(r,\tau )-\delta \tau +\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}},\\&b'_{1} =\frac{\ln \left( \frac{V}{D^{*}}\right) -\ln B^{*}(r,\tau )-\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{v}(s)ds+\int _{0}^{\tau }\rho _{sv}\sigma _{s}\sigma _{v}-\lambda _{v}\sigma _{v}+(\rho _{sr}\sigma _{s}\sigma _{r}+\rho _{rv}\sigma _{r}\sigma _{v})\varsigma (s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{v}(s)ds}},\\&a'_{2}=\frac{\ln \left( \frac{S}{K}\right) -\ln B^{*}(r,\tau )-\delta \tau -\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(S)dS}},\\&b'_{2}=\frac{\ln \left( \frac{V}{D^{*}}\right) -\ln B^{*}(r,\tau )-\lambda _{v}\sigma _{v}\tau -\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{v}(s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{v}(s)ds}},\\&e'_{1}=\frac{\ln \left( \frac{S}{K}\right) -\ln B^{*}(r,\tau )+\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds+\int _{0}^{\tau }\rho _{sv}\sigma _{s}\sigma _{v}-\delta +(\rho _{sr}\sigma _{s}\sigma _{r}+\rho _{rv}\sigma _{r}\sigma _{v})\varsigma (s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}},\\&f'_{1} =\frac{\ln \left( \frac{V}{D^{*}}\right) -\ln B^{*}(r,\tau )+\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{v}(s)ds+\int _{0}^{\tau }\rho _{sv}\sigma _{s}\sigma _{v}-\lambda _{v}\sigma _{v}+(\rho _{sr}\sigma _{s}\sigma _{r}+\rho _{rv}\sigma _{r}\sigma _{v})\varsigma (s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{v}(s)ds}},\\&e'_{2}=\frac{\ln \left( \frac{S}{K}\right) -\ln B^{*}(r,\tau )-\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds+\int _{0}^{\tau }\rho _{sv}\sigma _{s}\sigma _{v}-\delta +(\rho _{sr}\sigma _{s}\sigma _{r}+\rho _{rv}\sigma _{r}\sigma _{v})\varsigma (s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}},\\&f'_{2}=\frac{\ln \left( \frac{V}{D^{*}}\right) -\ln B^{*}(r,\tau )-\lambda _{v}\sigma _{v}\tau +\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{v}(s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{v}(s)ds}},\\&c'_{2}=a'_{2}, d'_{2}=b'_{2},g'_{2}=e'_{2},h'_{2}=f'_{2}. \end{aligned}$$

6 Numerical analysis

In this section, numerical examples are presented to examine if the underlying commodity price, convenience yield and counterparty credit risk have significant impacts on the prices of the commodity-linked bonds. As a reference point of numerical results, based on the values reported by Klein (1996), Miura and Yamauchi (1998), Chen (2010) and Lai and Mellios (2016), the parameter values for the base case are listed in Table 1 whenever they are required to be specified throughout the numerical analysis. The issuer of the commodity-linked bonds has the business of producing and selling the commodity which is the underlying asset of the commodity-linked bonds. The correlation coefficients $\rho _{sv}$, $\rho _{s\delta }$, $\rho _{\delta v}$, $\rho _{sr}$ and $\rho _{r v}$ need to satisfy condition (25), (44) and $|\rho |< 1$. Without loss of generality, we set bond with face value $F=100$ and the time to maturity $\tau$ of 5 years. The initial interest rate is assumed to be equal to $4\%$ per year. The spot prices of the underlying commodity $S_{t}$ is 20. The strike price K is set equal to the price of the underlying commodity $S_{t}$ at the time of issuance. Ingersoll (1982) points out when handling the convenience yield is to use the futures price of the commodity rather than the spot price(if one with a sufficiently long maturity is available). Therefore, to set the initial values of the parameters of the process of the commodity prices and the net marginal convenience yield, we use the parameter estimates for the WTI light sweet crude oil data presented in Lai and Mellios (2016). In their paper, based on all weekly observations of futures contracts traded prices on ICE from 2001/01/05 to 2010/12/31 for West Texas Intermediate (WTI) light sweet crude oil, they estimated the parameters of the process $S_{t}$ and $\delta _{t}$ for WTI crude oil. The estimated parameters were such that:$\kappa =0.729,\theta =0.080,\sigma _{s}=0.399, \sigma _{\delta }=0.227,\lambda _{\delta }=0.251$ and $\rho _{s\delta }=0.728$. We set the current level of the convenience yield rate $\delta _{t}$ is 0.02. Now we turn to focus on the parameters for the commodity-linked bonds issuer’s assets. The instantaneous volatility is set to be $\sigma _{v}=0.3$ and default barrier $D^{*}=D$(outstanding claims) is assumed to be 75 percent of the initial value of the issuer’s assets $V_{t}$ is 200 which consists of this commodity-linked bonds and the equity. Chen (2010) finds that bonds have recovery rate of around 0.60 across nine different aggregate states. We set the deadweight costs $\alpha =0.40$. Because we suppose this issuing company sells this commodity to the market, the value of this issuer is positively correlated to the changes of this commodity prices and the convenience yield. So the underlying commodity prices and the value of the issuer is set to behave with correlation coefficient $\rho _{sv}$ is 0.3. Also, we set the correlation parameter between the value of the issuer and the convenience yield $\rho _{\delta v}$ is 0.66. In the following tables and figures, we change one or more of the parameter values to investigate the impacts of the spot underlying commodity price, stochastic convenience yield and counterparty credit risk on the commodity-linked bonds prices with other variables taking on the values listed in Table 1.

Table 1 Preference parameters in the base case

Table 2 compares the one-, two- and three-factor(I, II) commodity-linked bonds prices for different parameter choices of the underlying commodity price, convenience yield, interest rate and counterparty credit risk. The one-factor theoretical commodity-linked bonds prices are calculated by uncertain commodity prices price model in Eq. 7, where the net convenience yield rate is fixed through the life of the commodity-linked bonds contract. The two-factor commodity-linked bonds prices are computed by uncertain commodity price and stochastic convenience yield by Eq. 21. The three-factor commodity-linked bonds pricing formula in Eq. 42 which involves stochastic commodity price, stochastic convenience yield and counterparty credit risk. The valuation of the three-factor commodity-linked bonds with a constant convenience yield in Eq. 47 which considers stochastic interest rate in the three-factor commodity-linked bonds model.

Table 2 Values of commodity-linked bonds

As can be observed in Table 2, increasing the standard deviation on the return on the underlying commodity increases the value of the commodity-linked bonds in Panel 2. This because a higher standard deviation on the return on the underlying commodity causing a higher premium portion of the commodity-linked bonds. In addition, we can understand the effect of $S_{0}$ in the same way. What’s more, the values of the three-factor(I) commodity-linked bonds with increase with $\rho _{sv}$ and decrease with $\rho _{s\delta }$ and $\rho _{\delta v}$ as shown in Table 2. A higher value of correlation coefficient $\rho _{sv}$ ensures that the values of the underlying commodity $S_{t}$ and the issuer $V_{t}$ tend to move in line with $\rho _{sv}$. We can also find that the values of the three-factor(I, II) bonds are smaller than those of the one- and two-factor bonds, which results from the default risk of the counterparty, there is the largest reduction in the value of commodity-linked bonds, as shown in Panel 17($-45.1027$ and $-41.8915$), implying that the probability the bond writer will default increases when the standard deviation of the return of the issuer’s asset increases. Conversely, a higher value of the issuer’s asset, there is the smallest reduction in Panel 12$(-18.5792,-16.5654)$. In addition, we find that the one-factor commodity-linked bonds prices are higher than those of the two-factor bonds prices due to the lower convenience yield in the one-factor model. Based on the results of Table 2, these findings show that the parameter variables of the underlying commodity price, stochastic convenience yield, stochastic interest rate and the vale of the issuer play important roles in valuing the commodity-linked bonds. These results are expected and consistent with previous studies, such as Hilliard and Reis (1998). And what’s more, such a conclusion seems more clear in Figs. 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13 and 14.

Fig. 1

Values of commodity-linked bonds against spot convenience yield. Baseline parameter values are given in Table 1, unless otherwise noted

Fig. 2

Values of commodity-linked bonds against time to maturity. Baseline parameter values are given in Table 1, unless otherwise noted

Fig. 3

Values of commodity-linked bonds against underlying-to-strike ratio. Baseline parameter values are given in Table 1, unless otherwise noted

Fig. 4

Values of commodity-linked bonds against the debt ratio. Baseline parameter values are given in Table 1, unless otherwise noted

Fig. 5

Values of commodity-linked bonds against speed of adjustment. Baseline parameter values are given in Table 1, unless otherwise noted

Fig. 6

Values of commodity-linked bonds against long-run mean yield. Baseline parameter values are given in Table 1, unless otherwise noted

Fig. 7

Values of commodity-linked bonds against deadweight cost. Baseline parameter values are given in Table 1, unless otherwise noted

Fig. 8

Values of commodity-linked bonds against correlation coefficient between the return of the underlying commodity and the return convenience yield. Baseline parameter values are given in Table 1, unless otherwise noted

Fig. 9

Values of commodity-linked bonds against correlation coefficient between the return convenience yield and the value of the issuer’s asset. Baseline parameter values are given in Table 1, unless otherwise noted

Fig. 10

Values of commodity-linked bonds against correlation coefficient between the return of the underlying commodity and the return on the issuer’s asset. Baseline parameter values are given in Table 1, unless otherwise noted

Fig. 11

Values of commodity-linked bonds against standard deviation of the return of the underlying commodity. Baseline parameter values are given in Table 1, unless otherwise noted

Fig. 12

Values of commodity-linked bonds against standard deviation of the return of the convenience yield. Baseline parameter values are given in Table 1, unless otherwise noted

Fig. 13

Values of commodity-linked bonds against standard deviation of the return of the issuer’s asset. Baseline parameter values are given in Table 1, unless otherwise noted

Fig. 14

Values of commodity-linked bonds against standard deviation of the interest rate. Baseline parameter values are given in Table 1, unless otherwise noted

Figure 1 graphs how the values of commodity-linked bonds changes with the spot convenience yield. Not surprising, the bonds price is decreasing in the convenience yield. As can be seen in Fig. 1, when the initial level of convenience yield approaches the long-run value $\theta$(0.08), the one- and two-factor commodity-linked bonds prices are not significantly different. With $\delta _{0}$ deviation from the long-run value $\theta$, the difference in price between the one-factor and the two-factor bonds models increases, and the difference between the three-factor(I) and the three-factor(II) bonds price also increases.

Figure 2 indicates the sensitivity of the commodity-linked bonds price with respect to the time to maturity. On the whole, the commodity-linked bonds price decreases as the time to maturity increases, no matter which model is applied. It can be seen that the gap in prices between the one-factor and the two-, three-factor models increases as the time to maturity rises. A longer time to maturity has three different effects on bonds values: first, it is well known that the value of a bond decreases with the time to maturity due to the time value of money; but second, convenience yield decreases with $\tau$ and tends to higher bonds value, this is because the longer the time to maturity, the lower is the average of the convenience yield and the higher the commodity-linked bonds price; on the other hand, the possibility of default by the issuer also increases with $\tau$ reduces option portion of bonds price.

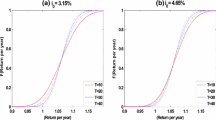
Figures 3 and 4 suggest how the ratio of the underlying commodity value to strike price, S/K, and debt ratio, D/V, affect the values of the commodity-linked bonds. In Fig. 3, increasing the ratio of the underlying commodity value to strike price S/K increases the value of the commodity-linked bonds. This can also be seen from Eqs. 7, 21, 42 and 47, where it is clear that the commodity-linked bonds value increases with S/K. Figure 4 shows that the impact of default risk on the value of the commodity-linked bonds in the three-factor model is more apparent if the debt ratio approaches to one. This means that if default becomes more and more possible, the decrease in the prices of the commodity-linked bonds becomes more rapidly. The value of the commodity-linked bonds decreases as the issuer is leveraged over a $15\%$ debt ratio. The greater the default risk, the greater the reduction in the value of the commodity-linked bonds.

Figure 5 displays that a smaller speed of adjustment $\kappa$ makes the bonds prices higher than that of a larger speed of adjustment $\kappa$. This means that the premium portion of bonds prices decrease as the speed of adjustment $\kappa$ becomes large. As expected, it can be observed that the difference in prices between the one-factor and the two-, three-factor(I) models increases first and sequently decreases as the speed of adjustment $\kappa$ increases.⁸ This is because with a high speed of adjustment, the convenience yield returns to its higher long-run level $\theta$ faster. As we know, commodity option portion is decreasing function of the convenience yield rate, keeping the convenience yield at a higher level leads to a lower option price, and the standard (bivariate) normal cumulative distribution simultaneously increases with the convenience yield increases, causing a higher bond price. However, in the one-factor model, the convenience yield is fixed and maintained at a lower level through the entire life of the commodity-linked bonds contract. In summary, as in the result of Fig. 5 indicates that a fixed convenience yield in the one-factor and three-factor(II) model can lead to significantly different bonds prices relative to those obtained by a stochastic convenience yield in the two- and three-factor(I) model. In Fig. 6, increasing the level of long-run yield decreases the commodity-linked bonds value in the two- and three-factor models. The effect of the deadweight cost, $\alpha$, is shown in Fig. 7. Clearly, it follows from (42) and (47) that the deadweight cost $\alpha$ affects only the recovery when default event occurs. Therefore, the commodity-linked bonds prices in the three-factor model is a linear decrease function with respect to $\alpha$.

Figures 8, 9 and 10 illustrate how the commodity-linked bonds price under the one-, two- and three-factor models changes with the correlation between the return of the underlying commodity and the return of the convenience yield $\rho _{s \delta }$, between the return of the convenience yield and the return of the issuer’s asset $\rho _{\delta v}$, between the return of the underlying commodity and the return of the issuer’s asset $\rho _{sv}$. Not surprisingly, the commodity-linked bonds price is increasing with respect to $\rho _{sv}$ in the three-factor model and decreasing with respect to $\rho _{s\delta }$ in the two- and three-factor models, and decreasing with respect to $\rho _{\delta v}$ in the three-factor model. Figure 8 plots the effect of varying degrees of correlation $\rho _{s \delta }$. It is evident that the spot commodity price and the convenience yield have opposing effect on bonds values. This is because the premium portion of bonds prices increase as the spot commodity prices increase, but the spot commodity prices decrease as convenience yields increase. In Fig. 9, the effect of $\rho _{\delta v}$ can be explained in a similar way. Figure 10 shows the effect of the correlation $\rho _{sv}$. Since there are no counterparty credit risk in the one- and two-factor models do not vary with $\rho _{sv}$. In the case of a positive correlation between the return on the underlying commodity and the return on the issuer’s asset in the three-factor model, if the issuer’s asset increases, there is a tendency for the underlying commodity to increase and for the value of the commodity-linked bonds to increase. Therefore a stronger positive correlation between the return on the underlying commodity and the return on the issuer’s asset in the three-factor model corresponds to a smaller effect of credit risk on the commodity-linked bonds. However, from Fig. 10 we also can see that the three-factor model prices are less than the one- and two-factor commodity-linked bonds prices. As Schwartz (1997) points out that most of default risk comes not from the firm being unable to pay the face value of the commodity-linked bonds, as is the case for regular bonds, but from the firm being unable to pay the value of the option for high commodity prices, even under substantial increases in the value of the firm.

Table 3 Sensitivity analysis for the commodity-linked bonds prices

Figures 11, 12, 13 and 14 reveal how the price of the commodity-linked bonds are affected by the standard deviation of the return of the underlying commodity, the convenience yield, the issuer’s asset and the interest rate. It can be seen that $\sigma _{s}$ impose a positive effect on the values of the commodity-linked bonds in the one-, two-, and three-factor models, and $\sigma _{\delta }$ impose a positive effect on the values of the commodity-linked bonds in the two- and three-factor models. On the contrary, $\sigma _{v}$ impose a negative effect on the values of the commodity-linked bonds in the three-factor model. Figure 11 depicts the effect of the standard deviation of the return of the underlying commodity on the value of the commodity-linked bonds. A higher standard deviation of the return of the underlying commodity has two contrary effects on the values of the commodity-linked bonds: first, it is obviously that the option portion of bonds prices increases with the standard deviation of the underlying commodity; and second the possibility of default also increases with $\sigma _{s}$, this tends to a lower bonds prices. The former effect is shown to dominate the latter effect during the life of the commodity-linked bonds contract. The U-shaped curves for $\sigma _{\delta }$ in the two- and three-factor(I) models are illustrated in Fig. 12. Figure 13 implies that when the standard deviation of the return of the assets of the issuer is increased, the possibility of default also increases. Consequently, the value of the commodity-linked bonds decreases as $\sigma _{v}$ increases. The effect of $\sigma _{r}$ can be explained in a similar way depicted in Fig. 14.

In conclusion, the effects of different parameters on the value of the commodity-linked bonds vary significantly, depending on the parameter considered. The effects of the parameters considered in the one-, two- and three-factor(I, II) models are summarized in Table 3.

7 Conclusion

This paper presents the two- and three-factor(I, II) commodity-linked bonds pricing models where the spot underlying commodity price dynamics follow a geometrical Brownian motion process with a stochastic drift, the dynamics of the net convenience yield are formulated as a mean-reverting Ornstein–Uhlenbeck stochastic process and the dynamics of the value of the issuer’s assets are given by a geometrical Brownian motion process. Based on the Mellin transform techniques, we obtain the closed-form pricing formulas of the commodity-linked bonds to study the impact of the underlying commodity value, convenience yield, interest rate and counterparty credit risk on bonds values. These formulas are simply provided with standard (bivariate) normal cumulative distribution function so that the pricing and hedging of the commodity-linked bonds can be computed very accurately and rapidly. We conduct the numerical evaluations of sensitivity analysis for the commodity-linked bonds, and we use tables and graphs to illustrate the significant movements of the prices with respect to parameters of the commodity-linked bonds. From the results, we could find out how convenience yield, interest rate and counterparty credit risk have a significant effect on the commodity-linked bonds prices.

Appendices

Review of the Mellin transform

To obtain help in solving the PDE (11), (28) and (46) with given terminal condition, we first summarize the definition and some basic properties of without proof for readers who are unfamiliar with the double Mellin transforms. The interested reader can refer to Erdelyi et al. (1954) and Sneddon (1972) as well.

Definition 1

(Definition of the Mellin transform and inverse transform) The Mellin transform $\hat{g}(\omega )$ of a complex-valued function g(x) defined over positive reals is

$$\begin{aligned} \mathcal {M}_{x}[g(x);\omega ]:=\hat{g}(\omega )=\int _{0}^{\infty }g(x)x^{\omega -1}dx, \end{aligned}$$

with $\omega$ is complex number. Then the function g(x) can be recovered from its Mellin transform by the inverse Mellin transformation formula

$$\begin{aligned} g(x):=\mathcal {M}_{x}^{-1}[\hat{g}(\omega )]=\frac{1}{2\pi i}\int _{c-i\infty }^{c+i\infty }\hat{g}(\omega )x^{-\omega }d\omega , \end{aligned}$$

with $a<Re(\omega )$ and $a<c_{1}<b$ exist.

Definition 2

(Definition of the double Mellin transform and inverse transform) The double Mellin transform $\hat{g}(\omega _{1},\omega _{2})$ of a complex-valued function g(x, y) defined over positive reals is

$$\begin{aligned} \mathcal {M}_{x,y}[g(x,y);\omega _{1},\omega _{2}]:=\hat{g}(\omega _{1},\omega _{2})=\int _{0}^{\infty }\int _{0}^{\infty }g(x,y)x^{\omega _{1}-1}y^{\omega _{2}-1}dxdy, \end{aligned}$$

with $\omega _{1}$ and $\omega _{2}$ are complex numbers. Then the function g(x, y) can be recovered from its Mellin transform by the inverse Mellin transformation formula

$$\begin{aligned} g(x,y):=\mathcal {M}_{x,y}^{-1}[\hat{g}(\omega _{1},\omega _{2})]=\frac{1}{(2\pi i)^{2}}\int _{c_{1}-i\infty }^{c_{1}+i\infty }\int _{c_{2}-i\infty }^{c_{2}+i\infty }\hat{g}(\omega _{1},\omega _{2})x^{-\omega _{1}}y^{-\omega _{2}}d\omega _{1}d\omega _{2}, \end{aligned}$$

with $a<Re(\omega _{1}), Re(\omega _{2})<b$ and $a<c_{1},c_{2}<b$ exist.

Proposition 1

(Basic properties of the Mellin transform) Suppose that there exists a double Mellin transform of f(x, y). Then the following relations hold:

$$\begin{aligned} \mathcal {M}_{x,y}\left( x\frac{\partial ^{2}}{\partial x }f(x,y);\omega _{1},\omega _{2}\right)&=-\omega _{1}\hat{f}(\omega _{1},\omega _{2}),\\ \mathcal {M}_{x,y}\left( y\frac{\partial ^{2}}{\partial y }f(x,y);\omega _{1},\omega _{2}\right)&=-\omega _{2}\hat{f}(\omega _{1},\omega _{2}),\\ \mathcal {M}_{x,y}\left( xy\frac{\partial ^{2}}{\partial x \partial y}f(x,y);\omega _{1},\omega _{2}\right)&=\omega _{1}\omega _{2}\hat{f}(\omega _{1},\omega _{2}),\\ \mathcal {M}_{x,y}\left( x^{2}\frac{\partial ^{2}}{\partial x^{2}}f(x,y);\omega _{1},\omega _{2}\right)&=\omega _{1}(\omega _{1}+1) \hat{f}(\omega _{1},\omega _{2}),\\ \mathcal {M}_{x,y}\left( y^{2}\frac{\partial ^{2}}{\partial y^{2}}f(x,y);\omega _{1},\omega _{2}\right)&=\omega _{2}(\omega _{2}+1) \hat{f}(\omega _{1},\omega _{2}). \end{aligned}$$

Proposition 2

(Convolution of the Mellin transform) Let f(x) and g(x) be locally integrable functions on positive reals. $\hat{f}(\omega )$ and $\hat{g}(\omega )$ are two Mellin transforms of the functions f(x) and g(x), respectively. Then, the Mellin convolution is given by the inverse Mellin transform of $\hat{f}(\omega _{1})\hat{g}(\omega _{1})$ as follows:

$$\begin{aligned} f(x)\vee g(x):&=\frac{1}{2\pi i}\int _{c-i\infty }^{c+i\infty }\hat{f}(\omega )\hat{g}(\omega )x^{-\omega }d\omega \\&=\int _{0}^{\infty }f\left( \frac{x}{\omega }\right) g(x)\frac{d\omega }{\omega }. \end{aligned}$$

Proposition 3

(Convolution of the double Mellin transform) Let f(x, y) and g(x, y) be locally integrable functions on positive reals. $\hat{f}(\omega _{1},\omega _{2})$ and $\hat{g}(\omega _{1},\omega _{2})$ are two double Mellin transforms of the functions f(x, y) and g(x, y), respectively. Then, the double Mellin convolution is given by the inverse Mellin transform of $\hat{f}(\omega _{1},\omega _{2})\hat{g}(\omega _{1},\omega _{2})$ as follows:

$$\begin{aligned} f(x,y)\vee g(x,y):&=\frac{1}{(2\pi i)^{2}}\int _{c_{1}-i\infty }^{c_{1}+i\infty }\int _{c_{2}-i\infty }^{c_{2}+i\infty }\hat{f}(\omega _{1},\omega _{2})\hat{g}(\omega _{1},\omega _{2})x^{-\omega _{1}}y^{-\omega _{2}}d\omega _{1}d\omega _{2}\\&=\int _{0}^{\infty }\int _{0}^{\infty }f(\frac{x}{\omega _{1}},\frac{y}{\omega _{2}})g(x,y)\frac{d\omega _{1}}{\omega _{1}}\frac{d\omega _{2}}{\omega _{2}}. \end{aligned}$$

Proposition 4

(Inverse Mellin transform of exponential function) Given complex numbers $\alpha$ and $\beta$ with $Re(\alpha )\ge 0$, let $f(x)=\frac{1}{2\pi i}\int _{c-i\infty }^{c+i\infty }\hat{f}(s)x^{-s}ds$, where $\hat{f}(s)=e^{\alpha (s+\beta )^{2}}$. Then

$$\begin{aligned} f(x)=\frac{1}{2\sqrt{\pi \alpha }}x^{\beta }e^{-\frac{(\ln x)^{2}}{4\alpha }} \end{aligned}$$

holds.

Proof of Theorem 3.1

Proof

First of all, let $x=\frac{\ln \left( \frac{S}{u}\right) }{\sqrt{2E(\tau )}}$. By applying the change of variable from u to x , Eq. 20 becomes

$$\begin{aligned} B(\tau ,S,\delta )&=-\frac{1}{\sqrt{2\pi }}\int _{\frac{\ln \left( \frac{S}{K}\right) }{\sqrt{2E(\tau )}}}^{-\infty }(Se^{-\sqrt{2E(\tau )}x}+F-K)e^{c^{*}-\frac{1}{2}x^{2}+\frac{G_{2}(\tau )}{\sqrt{2E(\tau )}}x}dx\nonumber \\&\quad -\frac{1}{\sqrt{2\pi }}\int _{\infty }^{\frac{\ln \left( \frac{S}{K}\right) }{\sqrt{2E(\tau )}}}Fe^{c^{*}-\frac{1}{2}x^{2}+\frac{G_{2}(\tau )}{\sqrt{2E(\tau )}}x}dx\nonumber \\&=\frac{S}{\sqrt{2\pi }}\int ^{\frac{\ln \left( \frac{S}{K}\right) }{\sqrt{2E(\tau )}}}_{-\infty }e^{-\frac{1}{2}\left( x+\frac{2E(\tau )-G_{2}(\tau )}{\sqrt{2E(\tau )}}\right) ^{2}+E(\tau )-G_{2}(\tau )-\tau r}dx+\frac{F-K}{\sqrt{2\pi }}\int ^{\frac{\ln \left( \frac{S}{K}\right) }{\sqrt{2E(\tau )}}}_{-\infty }\nonumber \\&\qquad e^{-\frac{1}{2}\left( x-\frac{G_{2}(\tau )}{\sqrt{2E(\tau )}}\right) ^{2}-\tau r}dx+\frac{F}{\sqrt{2\pi }}\int _{\frac{\ln (\frac{S}{K})}{\sqrt{2E(\tau )}}}^{\infty }e^{-\frac{1}{2}\left( x-\frac{G_{2}(\tau )}{\sqrt{2E(\tau )}}\right) ^{2}-\tau r}dx\nonumber \\&=P(\tau ,\delta )S\frac{1}{\sqrt{2\pi }}\int ^{\frac{\ln \left( \frac{S}{K}\right) }{\sqrt{2E(\tau )}}}_{-\infty }e^{-\frac{1}{2}(x+\frac{2E(\tau )-G_{2}(\tau )}{\sqrt{2E(\tau )}})^{2}}dx-e^{-\tau r}K\frac{1}{\sqrt{2\pi }}\int ^{\frac{\ln \left( \frac{S}{K}\right) }{\sqrt{2E(\tau )}}}_{-\infty }\nonumber \\&\qquad e^{-\frac{1}{2}\left( x-\frac{G_{2}(\tau )}{\sqrt{2E(\tau )}}\right) ^{2}}dx+e^{-\tau r}F. \end{aligned}$$

(48)

Here

$$\begin{aligned} P(\tau ,\delta )&=e^{E(\tau )-G_{2}(\tau )-\tau r}\\&=e^{\left( \theta ^{*}+\gamma _{1}-\frac{\sigma _{\delta }^{2}}{2\kappa ^{2}}\right) (H(\tau )-\tau )-\frac{\sigma _{\delta }^{2}}{4\kappa }H^{2}(\tau )-\delta H(\tau )}\\&=A(\tau )\exp (-\delta H(\tau )), \end{aligned}$$

where

$$\begin{aligned} A(\tau )&=\exp \left\{ \left( \theta ^{*}+\gamma _{1}-\frac{\sigma _{\delta }^{2}}{2\kappa ^{2}}\right) (H(\tau )-\tau )-\frac{\sigma _{\delta }^{2}}{4\kappa }H^{2}(\tau )\right\} \\ H(\tau )&=\frac{1-e^{-\kappa \tau }}{\kappa }. \end{aligned}$$

Then, $B(S,\tau )$ is given by the formula

$$\begin{aligned} B(S,\tau )&=P(\tau ,\delta )S\frac{1}{\sqrt{2\pi }}\int ^{\frac{\ln \left( \frac{S}{K}\right) }{\sqrt{2E(\tau )}}}_{-\infty }e^{-\frac{1}{2}(x'_{1})^{2}}dx-e^{- r\tau }K\frac{1}{\sqrt{2\pi }}\int ^{\frac{\ln \left( \frac{S}{K}\right) }{\sqrt{2E(\tau )}}}_{-\infty }\nonumber \\&\qquad e^{-\frac{1}{2}(x'_{2})^{2}}dx+e^{-r\tau }F\nonumber \\&=P(\tau ,\delta )S\mathcal {N}(d_{1})-e^{-r\tau }K\mathcal {N}(d_{2})+e^{-r\tau }F, \end{aligned}$$

(49)

where $d_{1}$ and $d_{2}$ are given by

$$\begin{aligned} d_{1}&=\frac{\ln \left( \frac{S}{K}\right) +r\tau +\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(t)dt+\ln (P(\delta ,\tau ))}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}},\\ d_{2}&=\frac{\ln \left( \frac{S}{K}\right) +r\tau -\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(t)dt+\ln (P(\delta ,\tau ))}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}}. \end{aligned}$$

$\square$

Proof of Lemma 4.1

Proof

For $\tau >0$, because $E(\tau )>0$, so the inequality $G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )}>0\Leftrightarrow 4E(\tau )G_{1}(\tau )>G^{2}(\tau )$. And $E(\tau )=\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(t)dt$ and $G_{1}(\tau )=\frac{1}{2}\sigma _{v}^{2}\tau =\frac{1}{2}\int ^{\tau }_{0}\sigma _{v}^{2}dt$. From the Cauchy–Schwartz inequality, we have

$$\begin{aligned} 4E(\tau )G_{1}(\tau )&=\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(t)dt\frac{1}{2}\sigma _{v}^{2}\tau =\frac{1}{2}\int ^{\tau }_{0}\sigma _{v}^{2}dt\\&\ge \left( \int ^{\tau }_{0}\hat{\sigma }_{s}(t)\sigma _{v}dt\right) ^{2}\\&=\left( \int ^{\tau }_{0}\sqrt{\sigma _{v}^{2}(\sigma _{s}^{2}-2\rho _{s\delta }\sigma _{s}\sigma _{\delta }H(t)+\sigma ^{2}_{\delta }H^{2}(t))}dt\right) ^{2}, \end{aligned}$$

and we also know $G(\tau )=\rho _{sv}\sigma _{s}\sigma _{v}\tau -\gamma _{2}(\tau -H(\tau ))=\int ^{\tau }_{0}\rho _{sv}\sigma _{s}\sigma _{v}-\rho _{\delta v}\sigma _{\delta }\sigma _{v}H(t)dt$. Therefore $4E(\tau )G_{1}(\tau )>G^{2}(\tau )$ is satisfied if and only if

$$\begin{aligned} \nabla&=\sigma _{v}^{2}\left( \sigma _{s}^{2}-2\rho _{s\delta }\sigma _{s}\sigma _{\delta }H(t)+\sigma ^{2}_{\delta }H^{2}(t))-(\rho _{sv}\sigma _{s}\sigma _{v}-\rho _{\delta v}\sigma _{\delta }\sigma _{v}H(t)\right) ^{2}\\&=\sigma _{v}^{2}\left[ (1-\rho _{\delta v}^{2})\sigma _{\delta }^{2}H^{2}(t)+2(\rho _{sv}\rho _{\delta v}-\rho _{s\delta })\sigma _{s}\sigma _{\delta }H(t)+(1-\rho _{sv}^{2})\sigma _{s}^{2}\right] >0. \end{aligned}$$

If we consider $\nabla$ as a quadratic equation of $\sigma _{\delta }H(t)$, then $\nabla >0$ is satisfied if and only if $\sigma _{v}^{2}>0$, $1-\rho _{\delta v}^{2}>0$ and

$$\begin{aligned} \Delta&=(2(\rho _{sv}\rho _{\delta v}-\rho _{s\delta })\sigma _{s})^{2}-4(1-\rho _{\delta v}^{2})(1-\rho _{sv}^{2})\sigma _{s}^{2}\\&=4\sigma _{s}^{2}\left[ \rho _{sv}^{2}+\rho _{\delta v}^{2}+\rho _{s\delta }^{2}-2\rho _{sv}\rho _{\delta v}\rho _{s\delta }-1\right] <0 \end{aligned}$$

are satisfied. Because $\sigma _{v}^{2}>0$ for $\tau >0$ is satisfied, and according to conditions (25), the two later conditions are obviously true. Consequently, $G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )}>0$ has been verified. $\square$

Proof of Theorem 4.1

Proof

First of all, let $y=\frac{\ln \left( \frac{V}{w}\right) }{\sqrt{2G_{1}(\tau )}}$ and $\rho =\frac{G(\tau )}{2\sqrt{E(\tau )G_{1}(\tau )}}$. By applying the change of variables from u and w to x and y, $I_{B}^{1}(t,S,V,\delta )$ of Eq. 41 becomes

$$\begin{aligned}&I_{B}^{1}(t,S,V,\delta )=\frac{1}{2\pi }\sqrt{\frac{G_{1}(\tau )}{{G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )}}}}\int ^{-\infty }_{\frac{\ln (\frac{S}{K})}{\sqrt{2E(\tau )}}}\int ^{-\infty }_{\frac{\ln \left( \frac{V}{D^{*}}\right) }{\sqrt{2G_{1}(\tau )}}} e^{\eta }\exp \left\{ -\frac{(G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )(G_{2}(\tau )-\sqrt{2E(\tau )}x)}{2E(\tau )})^{2}}{4(G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )})}\right\} \nonumber \\&\qquad (Se^{-\sqrt{2E(\tau )}x}+F-K)\exp \Bigg \{\frac{y\sqrt{2G_{1}(\tau )}(G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )(G_{2}(\tau )-\sqrt{2E(\tau )}x)}{2E(\tau )})}{2(G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )})}\nonumber \\&\quad +\frac{G_{2}(\tau )}{\sqrt{2E(\tau )}}x\Bigg \}e^{-\frac{1}{2}x^{2}}e^{-\frac{G_{1}(\tau )y^{2}}{2\left( G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )}\right) }}dxdy\nonumber \\&=\frac{S}{2\pi \sqrt{1-\rho ^{2}}} \int ^{-\infty }_{\frac{\ln \left( \frac{S}{K}\right) }{\sqrt{2E(\tau )}}}\int ^{-\infty }_{\frac{\ln \left( \frac{V}{D^{*}}\right) }{\sqrt{2G_{1}(\tau )}}}\exp \Bigg \{\eta -\frac{1}{2(1-\rho ^{2})}(x^{2}+y^{2}) -\Bigg [\sqrt{2E(\tau )}-\frac{G_{2}(\tau )}{\sqrt{2E(\tau )}}\nonumber \\&\quad +\frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )}\right) \frac{G(\tau )}{\sqrt{2E(\tau )}}}{2G_{1}(\tau )(1-\rho ^{2})} \Bigg ]x +\frac{y\sqrt{2G_{1}(\tau )}\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )}\right) }{2G_{1}(\tau )(1-\rho ^{2})}\nonumber \\&\quad +\frac{\rho xy}{(1-\rho ^{2})}-\frac{(G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )})^{2}}{4G_{1}(\tau )(1-\rho ^{2})}\Bigg \}dxdy\nonumber \\&\quad +\frac{F-K}{2\pi \sqrt{1-\rho ^{2}}} \int ^{-\infty }_{\frac{\ln \left( \frac{S}{K}\right) }{\sqrt{2E(\tau )}}}\int ^{-\infty }_{\frac{\ln \left( \frac{V}{D^{*}}\right) }{\sqrt{2G_{1}(\tau )}}}\exp \Bigg \{\eta -\frac{1}{2(1-\rho ^{2})}(x^{2}+y^{2}) -\Bigg [\frac{(G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )})}{2G_{1}(\tau )(1-\rho ^{2})}\nonumber \\&\quad \cdot \frac{G(\tau )}{\sqrt{2E(\tau )}}-\frac{G_{2}(\tau )}{\sqrt{2E(\tau )}}\Bigg ]x+\frac{y\sqrt{2G_{1}(\tau )}(G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )})}{2G_{1}(\tau )(1-\rho ^{2})}+\frac{\rho xy}{(1-\rho ^{2})}\nonumber \\&\quad -\frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )}\right) ^{2}}{4G_{1}(\tau )(1-\rho ^{2})}\Bigg \}dxdy. \end{aligned}$$

(50)

To evaluate the first term in Eq. 50, we introduce an auxiliary function

$$\begin{aligned}&I_{B}^{10}(\tau ,S,V,\delta )=\frac{S}{2\pi \sqrt{1-\rho ^{2}}}\int ^{-\infty }_{\frac{\ln (\frac{S}{K})}{\sqrt{2E(\tau )}}}\int ^{-\infty }_{\frac{\ln (\frac{V}{D^{*}})}{\sqrt{2G_{1}(\tau )}}} \exp \left\{ \eta -\frac{1}{2(1-\rho ^{2})}(x^{2}+y^{2}) -\left[ \sqrt{2E(\tau )}-\frac{G_{2}(\tau )}{\sqrt{2E(\tau )}}\right. \right. \nonumber \\&\left. \quad +\frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )}\right) \frac{G(\tau )}{\sqrt{2E(\tau )}}}{2G_{1}(\tau )(1-\rho ^{2})} \right] x+\frac{y\sqrt{2G_{1}(\tau )}(G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )})}{2G_{1}(\tau )(1-\rho ^{2})}\nonumber \\&\quad \left. +\frac{\rho xy}{(1-\rho ^{2})}-\frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )}\right) ^{2}}{4G_{1}(\tau )(1-\rho ^{2})}\right\} dxdy. \end{aligned}$$

(51)

Then the exponent of the integrand of Eq. 51 can be expressed as

$$\begin{aligned} M_{1}-\frac{1}{2(1-\rho ^{2})}[(x+a_{1})^{2}+(y+b_{1})^{2}]+\frac{\rho }{1-\rho ^{2}}(x+a_{1})(y+b_{1}), \end{aligned}$$

where $a_{1}$, $b_{1}$ and $M_{1}$ are given by

$$\begin{aligned} a_{1}&=\frac{2E(\tau )-G_{2}(\tau )}{\sqrt{2E(\tau )}},\\ b_{1}&=\frac{G(\tau )-G_{1}(\tau )-G_{3}(\tau )}{\sqrt{2G_{1}(\tau )}},\\ M_{1}&=\eta -\frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )}\right) ^{2}}{4G_{1}(\tau )(1-\rho ^{2})}+\frac{a_{1}^{2}+b^{2}_{1}}{2(1-\rho ^{2})}-\frac{\rho a_{1}b_{1}}{1-\rho ^{2}}\\&=E(\tau )-G_{2}(\tau )-\tau r\\&=\ln (P(\delta , \tau )). \end{aligned}$$

Similarly, to evaluate the second term in Eq. 50, we introduce an auxiliary function

$$\begin{aligned} I_{B}^{11}(\tau ,S,V,\delta )&=\frac{F-K}{2\pi \sqrt{1-\rho ^{2}}}\int ^{-\infty }_{\frac{\ln \left( \frac{S}{K}\right) }{\sqrt{2E(\tau )}}}\int ^{-\infty }_{\frac{\ln \left( \frac{V}{D^{*}}\right) }{\sqrt{2B_{1}(\tau )}}} \exp \left\{ \eta -\frac{1}{2(1-\rho ^{2})}(x^{2}+y^{2}) -\left[ \frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )}\right) }{2G_{1}(\tau )(1-\rho ^{2})}\right. \right. \nonumber \\&\left. \quad \cdot \frac{G(\tau )}{\sqrt{2E(\tau )}}-\frac{G_{2}(\tau )}{\sqrt{2E(\tau )}}\right] x+\frac{y\sqrt{2G_{1}(\tau )}(G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )})}{2G_{1}(\tau )(1-\rho ^{2})}+\frac{\rho xy}{2E(\tau )G_{1}(\tau )(1-\rho ^{2})}\nonumber \\&\quad \left. -\frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )}\right) ^{2}}{4G_{1}(\tau )(1-\rho ^{2})}\right\} dxdy. \end{aligned}$$

(52)

Then the exponent of the integrand of Eq. 52 can be expressed as

$$\begin{aligned} M_{2}-\frac{1}{2(1-\rho ^{2})}[(x+a_{2})^{2}+(y+b_{2})^{2}]+\frac{\rho }{1-\rho ^{2}}(x+a_{2})(y+b_{2}), \end{aligned}$$

where $a_{2}$, $b_{2}$ and $M_{2}$ are given by

$$\begin{aligned} a_{2}&=-\frac{G_{2}(\tau )}{\sqrt{2E(\tau )}},\\ b_{2}&=-\frac{G_{1}(\tau )+G_{3}(\tau )}{\sqrt{2G_{1}(\tau )}},\\ M_{2}&=\eta -\frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )}\right) ^{2}}{4G_{1}(\tau )(1-\rho ^{2})}+\frac{a_{2}^{2}+b^{2}_{2}}{2(1-\rho ^{2})}-\frac{\rho a_{2}b_{2}}{1-\rho ^{2}}\\&=-\tau r. \end{aligned}$$

Then, $I_{B}^{1}(\tau ,S,V,\delta )$ is given by the formula

$$\begin{aligned} \begin{array}{lll} I_{B}^{1}(\tau ,S,V,\delta )&{}= P(\delta ,\tau )S\frac{1}{2\pi \sqrt{1-\rho ^{2}}}\int _{-\infty }^{a'_{1}}\int _{-\infty }^{a'_{2}}e^{-\frac{1}{2(1-\rho ^{2})}(x_{1}^{'2}-2\rho x'_{1}y'_{1}+y_{1}^{'2})}dx'_{1}dy'_{1}\\ &{}\qquad +e^{-r\tau }(F-K)\frac{1}{2\pi \sqrt{1-\rho ^{2}}}\int _{-\infty }^{b'_{1}}\int _{-\infty }^{b'_{2}}e^{-\frac{1}{2(1-\rho ^{2})}(x_{2}^{'2}-2\rho x'_{2}y'_{2}+y_{2}^{'2})}dx'_{2}dy'_{2}\\ &{}=P(\delta ,\tau )S\mathcal {N}_{2}(a'_{1},b'_{1},\rho )+e^{-r\tau }(F-K)\mathcal {N}_{2}(a'_{2},b'_{2},\rho ), \end{array} \end{aligned}$$

(53)

where

$$\begin{aligned} a'_{1}&=\frac{\ln \left( \frac{S}{K}\right) +\ln P(\delta ,\tau )+\tau r+\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}},\\ b'_{1}&=\frac{\ln \left( \frac{V}{D^{*}}\right) +\left( r-\lambda _{v}\sigma _{v}-\frac{1}{2}\sigma _{v}^{2}\right) \tau +\rho _{sv}\sigma _{s}\sigma _{v}-\gamma _{2}(\tau -H(\tau ))}{\sigma _{v}\sqrt{\tau }},\\ a'_{2}&=\frac{\ln \left( \frac{S}{K}\right) +\ln P(\delta ,\tau )+\tau r-\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}},\\ b'_{2}&=\frac{\ln \left( \frac{V}{D^{*}}\right) +\left( r-\lambda _{v}\sigma _{v}-\frac{1}{2}\sigma _{v}^{2}\right) \tau }{\sigma _{v}\sqrt{\tau }}. \end{aligned}$$

As with the same procedure for $I_{B}^{1}(\tau ,S,V,\delta )$, then $I_{B}^{2}(\tau ,S,V,\delta )$, $I_{B}^{3}(\tau ,S,V,\delta )$ and $I_{B}^{4}(\tau ,S,V,\delta )$ of Eq. 41 are given respectively,

$$\begin{aligned} \begin{array}{lll} I_{B}^{2}(\tau ,S,V,\delta )&{}=\frac{F}{2\pi \sqrt{1-\rho ^{2}}}\int ^{\infty }_{\frac{\ln \left( \frac{S}{K}\right) }{\sqrt{2E(\tau )}}}\int ^{-\infty }_{\frac{\ln \left( \frac{V}{D^{*}}\right) }{\sqrt{2B_{1}(\tau )}}} \exp \left\{ \eta -\frac{1}{2(1-\rho ^{2})}(x^{2}+y^{2}) -\left[ \frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )}\right) }{2G_{1}(\tau )(1-\rho ^{2})}\right. \right. \\ &{}\left. \quad \cdot \frac{G(\tau )}{\sqrt{2E(\tau )}}-\frac{G_{2}(\tau )}{\sqrt{2E(\tau )}}\right] x+\frac{y\sqrt{2G_{1}(\tau )}(G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )})}{2G_{1}(\tau )(1-\rho ^{2})}+\frac{\rho xy}{2E(\tau )G_{1}(\tau )(1-\rho ^{2})}\\ &{}\left. \quad -\frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )}\right) ^{2}}{4G_{1}(\tau )(1-\rho ^{2})}\right\} dxdy\\ &{}=e^{-r\tau }F\mathcal {N}_{2}(-c'_{2},d'_{2},-\rho ), \end{array} \end{aligned}$$

(54)

where

$$\begin{aligned} c'_{2}&=a'_{2}, d'_{2}=b'_{2}. I_{B}^{3}(\tau ,S,V,\delta )\nonumber \\&\quad =\frac{(1-\alpha )V}{D}\frac{1}{2\pi }\sqrt{\frac{G_{1}(\tau )}{{G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )}}}}\int ^{-\infty }_{\frac{\ln (\frac{S}{K})}{\sqrt{2E(\tau )}}}\int ^{\frac{\ln \left( \frac{V}{D^{*}}\right) }{\sqrt{2G_{1}(\tau )}}}_{\infty } \exp \left\{ -\frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )(G_{2}(\tau )-\sqrt{2E(\tau )}x)}{2E(\tau )}\right) ^{2}}{4\left( G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )}\right) }\right\} \nonumber \\&\qquad e^{\eta -\sqrt{2G_{1}(\tau )}y}\left( Se^{-\sqrt{2E(\tau )}x}+F-K\right) \exp \Bigg \{\frac{y\sqrt{2G_{1}(\tau )}(G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )(G_{2}(\tau )-\sqrt{2E(\tau )}x)}{2E(\tau )})}{2\left( G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )}\right) }\nonumber \\&\qquad +\frac{G_{2}(\tau )}{\sqrt{2E(\tau )}}x\Bigg \}e^{-\frac{1}{2}x^{2}}e^{-\frac{G_{1}(\tau )y^{2}}{2(G_{1}(\tau )-\frac{G^{2}(\tau )}{4E(\tau )})}}dxdy\nonumber \\&\quad =\frac{(1-\alpha )V}{D}S\frac{1}{2\pi \sqrt{1-\rho ^{2}}}\int ^{-\infty }_{\frac{\ln \left( \frac{S}{K}\right) }{\sqrt{2E(\tau )}}}\int ^{\frac{\ln (\frac{V}{D^{*}})}{\sqrt{2G_{1}(\tau )}}}_{\infty } \exp \Bigg \{\eta -\frac{1}{2(1-\rho ^{2})}(x^{2}+y^{2}) -\Bigg [\sqrt{2E(\tau )}\nonumber \\&\qquad -\frac{G_{2}(\tau )}{\sqrt{2E(\tau )}}+ \frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )}\right) \frac{G(\tau )}{\sqrt{2E(\tau )}}}{2G_{1}(\tau )(1-\rho ^{2})} \Bigg ]x+[\frac{\sqrt{2G_{1}(\tau )}(G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )})}{2G_{1}(\tau )(1-\rho ^{2})}\nonumber \\&\qquad -\sqrt{2G_{1}(\tau )}]y+\frac{\rho xy}{2E(\tau )G_{1}(\tau )(1-\rho ^{2})}-\frac{(G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )})^{2}}{4G_{1}(\tau )(1-\rho ^{2})}\Bigg \}dxdy\nonumber \\&\qquad +\frac{(1-\alpha )V}{D}\frac{F-K}{2\pi \sqrt{1-\rho ^{2}}}\int ^{-\infty }_{\frac{\ln (\frac{S}{K})}{\sqrt{2E(\tau )}}}\int ^{\frac{\ln \left( \frac{V}{D^{*}}\right) }{\sqrt{2G_{1}(\tau )}}}_{\infty } \exp \left\{ \eta -\frac{1}{2(1-\rho ^{2})}(x^{2}+y^{2}) +\left[ \frac{G_{2}(\tau )}{\sqrt{2E(\tau )}}\right. \right. \nonumber \\&\qquad \left. -\frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )}\right) }{2G_{1}(\tau )(1-\rho ^{2})}\frac{G(\tau )}{\sqrt{2E(\tau )}}\right] x+\left[ \frac{\sqrt{2G_{1}(\tau )}(G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )})}{2G_{1}(\tau )(1-\rho ^{2})}\right. \nonumber \\&\qquad \left. \left. -\sqrt{2G_{1}(\tau )}\right] y+\frac{\rho xy}{2E(\tau )G_{1}(\tau )(1-\rho ^{2})}-\frac{(G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )})^{2}}{4G_{1}(\tau )(1-\rho ^{2})}\right\} dxdy\nonumber \\&\quad =\frac{(1-\alpha )V}{D}(P'(\delta ,\tau )Se^{(r+\rho _{sv}\sigma _{s}\sigma _{v}-\lambda _{v}\sigma _{v})\tau }\mathcal {N}_{2}(e'_{1},-f'_{1},-\rho )\nonumber \\&\qquad +e^{-\lambda _{v}\sigma _{v}\tau }(F-K)\mathcal {N}_{2}(e'_{2},-f'_{2},-\rho )), \end{aligned}$$

(55)

where

$$\begin{aligned} P'(\delta ,\tau )&=A'(\tau )\exp (-\delta H(\tau )), \nonumber \\ A'(\tau )&=\exp \left\{ \left( \theta ^{*}+\gamma _{1}+\gamma _{2}-\frac{\sigma _{\delta }^{2}}{2\kappa ^{2}}\right) (H(\tau )-\tau )-\frac{\sigma _{\delta }^{2}}{4\kappa }H^{2}(\tau )\right\} ,\nonumber \\ e'_{1}&=\frac{\ln \left( \frac{S}{K}\right) +\ln P'(\delta ,\tau )+\tau r+\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds+\rho _{sv}\sigma _{s}\sigma _{v}\tau }{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}},\nonumber \\ f'_{1}&=\frac{\ln \left( \frac{V}{D^{*}}\right) +\left( r-\lambda _{v}\sigma _{v}+\frac{1}{2}\sigma _{v}^{2}\right) \tau +\rho _{sv}\sigma _{s}\sigma _{v}-\gamma _{2}(\tau -H(\tau ))}{\sigma _{v}\sqrt{\tau }},\nonumber \\ e'_{2}&=\frac{\ln \left( \frac{S}{K}\right) +\ln P'(\delta ,\tau )+\tau r-\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds+\rho _{sv}\sigma _{s}\sigma _{v}\tau }{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}},\nonumber \\ f'_{2}&=\frac{\ln \left( \frac{V}{D^{*}}\right) +\left( r-\lambda _{v}\sigma _{v}+\frac{1}{2}\sigma _{v}^{2}\right) \tau }{\sigma _{v}\sqrt{\tau }}. \nonumber \\ I_{B}^{4}(\tau ,S,V,\delta )&=\frac{(1-\alpha )V}{D}\frac{F}{2\pi \sqrt{1-\rho ^{2}}}\int ^{\frac{\ln \left( \frac{S}{K}\right) }{\sqrt{2E(\tau )}}}_{\infty }\int ^{\frac{\ln \left( \frac{V}{D^{*}}\right) }{\sqrt{2G_{1}(\tau )}}}_{\infty } \exp \Bigg \{\eta -\frac{1}{2(1-\rho ^{2})}(x^{2}+y^{2}) +\Bigg [\frac{G_{2}(\tau )}{\sqrt{2E(\tau )}}\nonumber \\&\quad -\frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )}\right) }{2G_{1}(\tau )(1-\rho ^{2})}\frac{G(\tau )}{\sqrt{2E(\tau )}}\Bigg ]x+[\frac{\sqrt{2G_{1}(\tau )}(G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )})}{2G_{1}(\tau )(1-\rho ^{2})}\nonumber \\&\quad -\sqrt{2G_{1}(\tau )}]y+\frac{\rho xy}{2E(\tau )G_{1}(\tau )(1-\rho ^{2})} -\frac{\left( G_{1}(\tau )+G_{3}(\tau )-\frac{G(\tau )G_{2}(\tau )}{2E(\tau )}\right) ^{2}}{4G_{1}(\tau )(1-\rho ^{2})}\Bigg \}dxdy\nonumber \\&=\frac{(1-\alpha )V}{D}e^{-\lambda _{v}\sigma _{v}\tau }F\mathcal {N}_{2}(-g'_{2},-h'_{2},\rho ), \end{aligned}$$

(56)

where

$$\begin{aligned} g'_{2}=e'_{2},h'_{2}=f'_{2}. \end{aligned}$$

Finally, we can recombine $I_{B}^{1}(\tau ,S,V,\delta )$, $I_{B}^{2}(\tau ,S,V,\delta )$, $I_{B}^{3}(\tau ,S,V,\delta )$ and $I_{B}^{4}(\tau ,S,V,\delta )$ in the following formula:

$$\begin{aligned} B(S,V,\delta ,\tau )&=P(\delta ,\tau )S\mathcal {N}_{2}(a'_{1},b'_{1},\rho )+e^{-r\tau }(F-K)\mathcal {N}_{2}(a'_{2},b'_{2},\rho )\nonumber \\&\quad +\,e^{-r\tau }F\mathcal {N}_{2}(-c'_{2},d'_{2},-\rho )\nonumber \\&\quad +\,\frac{(1-\alpha )V}{D}(P'(\delta ,\tau )Se^{(r-\lambda _{1}\sigma _{v}+\rho _{sv}\sigma _{s}\sigma _{v})\tau }\mathcal {N}_{2}(e'_{1},-f'_{1},-\rho )\nonumber \\&\quad +\,e^{-\lambda _{v}\sigma _{v}\tau }(F-K)\mathcal {N}_{2}(e'_{2},-f'_{2},-\rho ))\nonumber \\&\quad +\,\frac{(1-\alpha )V}{D}e^{-\lambda _{v}\sigma _{v}\tau }F\mathcal {N}_{2}(-g'_{2},-h'_{2},\rho ), \end{aligned}$$

(57)

where $\mathcal {N}_{2}$ is the standard bivariate normal cumulative distribution as

$$\begin{aligned} \mathcal {N}_{2}(a,b,\rho )=\frac{1}{2\pi \sqrt{1-\rho ^{2}}}\int _{-\infty }^{a}\int _{-\infty }^{b}e^{-\frac{1}{2(1-\rho ^{2})}(x^{2}-2\rho xy+y^{2})}dxdy, \end{aligned}$$

and

$$\begin{aligned} \rho&=\frac{(\rho _{sv}\sigma _{s}\sigma _{v}-\gamma _{2})\tau +\gamma _{2}H(\tau )}{\sigma _{v}\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds\tau }},\\ a'_{1}&=\frac{\ln \left( \frac{S}{K}\right) +\ln P(\delta ,\tau )+r\tau +\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}},\\ b'_{1}&=\frac{\ln \left( \frac{V}{D^{*}}\right) +\left( r-\lambda _{v}\sigma _{v}+\rho _{sv}\sigma _{s}\sigma _{v}-\gamma _{2}-\frac{1}{2}\sigma _{v}^{2}\right) \tau +\gamma _{2}H(\tau )}{\sigma _{v}\sqrt{\tau }},\\ a'_{2}&=\frac{\ln \left( \frac{S}{K}\right) +\ln P(\delta ,\tau )+r\tau -\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(S)dS}},\\ b'_{2}&=\frac{\ln \left( \frac{V}{D^{*}}\right) +\left( r-\lambda _{v}\sigma _{v}-\frac{1}{2}\sigma _{v}^{2}\right) \tau }{\sigma _{v}\sqrt{\tau }},\\ e'_{1}&=\frac{\ln \left( \frac{S}{K}\right) +\ln P'(\delta ,\tau )+(r+\rho _{sv}\sigma _{s}\sigma _{v})\tau +\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}},\\ f'_{1}&=\frac{\ln \left( \frac{V}{D^{*}}\right) +\left( r-\lambda _{v}\sigma _{v}+\rho _{sv}\sigma _{s}\sigma _{v}-\gamma _{2}+\frac{1}{2}\sigma _{v}^{2}\right) \tau +\gamma _{2}H(\tau )}{\sigma _{v}\sqrt{\tau }},\\ e'_{2}&=\frac{\ln \left( \frac{S}{K}\right) +\ln P'(\delta ,\tau )+ (r+\rho _{sv}\sigma _{s}\sigma _{v})\tau -\frac{1}{2}\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}{\sqrt{\int ^{\tau }_{0}\hat{\sigma }^{2}_{s}(s)ds}},\\ f'_{2}&=\frac{\ln \left( \frac{V}{D^{*}}\right) +\left( r-\lambda _{v}\sigma _{v}+\frac{1}{2}\sigma _{v}^{2}\right) \tau }{\sigma _{v}\sqrt{\tau }},\\ c'_{2}&=a'_{2}, d'_{2}=b'_{2},g'_{2}=e'_{2},h'_{2}=f'_{2}. \end{aligned}$$

$\square$