1 Introduction

A great deal of attention has been attracted for studying of quantum correlations beyond entanglement [1,2,3,4]. The various quantum correlations are resources for quantum information processing. For measuring entanglement in bipartite quantum systems, some measures have been introduced such as entanglement of formation, concurrence and negativity [5,6,7,8,9,10]. In fact, entanglement presents quantum correlations while there are other non-classical correlations measures which are more general than entanglement [1,2,3]. Ollivier and Zurek and simultaneously Henderson and Vedral introduced a quantifier for quantum correlations in bipartite systems [1, 2]. It was called quantum discord which has been based on the von Neumann entropy. It is difference of quantum mutual information and classical correlations [1, 2]. There are some quantum states with zero entanglement, while they have nonzero quantum discord. The computation of quantum discord is hard task because the minimization process is complicated [1, 11]. Analytical quantum discord has been obtained for some special families of two-qubit states [3, 12, 13]. So, local quantum uncertainty has been introduced for investigating the discord type of quantum correlations [14,15,16,17]. As we know, two non-commutable quantum observables cannot be measured simultaneously with arbitrary precision [17]. The uncertainty for an observable H, in a quantum state \(\rho \) is defined by the variance as \(V(\rho ,H)=Tr(\rho H^2)- (Tr(\rho H))^2\). Wigner and Yanase introduced skew information as [14],

$$\begin{aligned} \text {I}(\rho ,H)=-\frac{1}{2}Tr[\sqrt{\rho },H]^2. \end{aligned}$$
(1)

The local quantum uncertainty is a computable quantifier of quantum correlation in bipartite quantum systems [15]. This measure is zero for classically correlated states and it is invariant under local unitary operations. Girolami et al defined the minimum skew information as local quantum uncertainty [14]. It quantifies the minimum quantum uncertainty in a state due to a measurement of local observable [15,16,17]. Local quantum uncertainty measures quantum correlations by applying local measurements on one part of quantum state. The local quantum uncertainty for a bipartite \( 2\times d \) quantum system \(\rho \) is defined as follows [14, 17]:

$$\begin{aligned} \text {I}(\rho ,H)=\min _{H_A}\text {I}({\rho },H_A\otimes \mathbbm {1} _B), \end{aligned}$$
(2)

where \(H_A\) is a Hermitian operator, which acts on the subsystem A and \( \mathbbm {1}_B \) is the identity operator which acts on the subsystem B. W is a \( 3\times 3 \) matrix whose matrix elements are defined by,

$$\begin{aligned} w_{ij}=Tr\{\sqrt{\rho }(\sigma _i\otimes \mathbbm {1}_B) \sqrt{\rho }(\sigma _j\otimes \mathbbm {1}_B)\}, \end{aligned}$$
(3)

with \(i,j \in \{1,2,3\} \) and the \(\sigma _{i(j)}\) represents one of Pauli matrices. Final form of the local quantum uncertainty for a \( 2\times d \) quantum state is given by [17],

$$\begin{aligned} LQ(\rho )=1-\max \{\eta _1,\eta _2,\eta _3\}, \end{aligned}$$
(4)

where \(\eta _1\), \(\eta _2\) and \(\eta _3\) are the eigenvalues of the W matrix. The advantage of this measure is that it is computable analytically for \(2\otimes d\) (qubit–qudit) systems [14,15,16,17]. Peres-Horodecki’s criterion is a separability criterion for bipartite quantum systems which has been based on the positive partial transpose (PPT) of a bipartite system [5]. The PPT criterion is sufficient and necessary condition for the separability in qubit-qubit and qubit-qudit systems, while for systems with higher dimensions, this condition is necessary but not sufficient [6]. The negativity is defined as follows:

$$\begin{aligned} N(\rho _{AB})=\frac{1}{2}\sum _i(|\zeta _i|-\zeta _i), \end{aligned}$$
(5)

where \(\rho _{AB}^{TA}\) is the partial transpose of \(\rho _{AB}\) with respect to the subsystem A and \( \zeta _i \)s are the eigenvalues of \(\rho _{AB}^{TA}\) [6]. When negativity is greater than zero, we can find that there is an entanglement between the subsystems. For a tripartite system (\(\rho _{ABC}\equiv \rho \)), the tripartite negativity as a measure of entanglement between three parts which is defined as follows [9, 18]:

$$\begin{aligned} N^3(\rho )=( N(\rho ^{TA}) N(\rho ^{TB}) N(\rho ^{TC}))^{\frac{1}{3}}, \end{aligned}$$
(6)

where \( N(\rho ^{TA}) \) is negativity between subsystems A and the rest of the system, \( N(\rho ^{TB}) \) is negativity between subsystems B and the rest of the system, and \( N(\rho ^{TC}) \) is negativity between subsystems C and the rest of the system. For a tripartite system \(\rho _{ABC}\), genuine tripartite correlations are those which cannot be described as a bipartite state \(\rho _{ij}\otimes \rho _k\) where \(\{i,j,k\}\in \{A,B,C\}\) and \( i\ne j\ne k \); it is defined as follows [19,20,21]:

$$\begin{aligned} \tau (\rho _{ABC})=\min \{I(\rho _{AB,C}), I(\rho _{AC,B}), I(\rho _{BC,A}) \}. \end{aligned}$$
(7)

Bipartite mutual information is as follows:

$$\begin{aligned} I(\rho _{ij,k})=S(\rho _{ij})+S(\rho _k)-S(\rho _{ijk}), \end{aligned}$$
(8)

where \( S(\rho )=-Tr(\rho \log _2\rho )\) is von Neumann entropy of quantum state \( \rho \). This measure takes both classical and quantum correlations [19].

In this paper, introduction of Hamiltonian is in Sect. 2. Sections 3 and 4 are dedicated to investigate the effects of magnetic field and spin–orbit interaction on correlations, respectively. We study effect of coupling constant in the x and z directions, respectively, in Sects. 5 and 6. Effect of temperature on correlations is considered in Sect. 7. Conclusions are provided in Sect. 8.

2 The model Hamiltonian

A spin chain model for describing systems like optical lattices and quantum dots is Heisenberg model [22,23,24]. Heisenberg model for generation of qubit states is ideal model so this model has attracted a lot of attention recently [25,26,27,28]. The Hamiltonian of N qubits in the spin chain according to the XYZ Heisenberg model with the spin–orbit interaction (Dzyaloshinskii–Moriya interaction) and external magnetic field along the z-direction which acts on N qubits is as follows [15]:

$$\begin{aligned} H=\frac{1}{2}\sum _{i=1}^{N}{\bigg [J_x\sigma _x^i\sigma _x^{i+1}+J_y\sigma _y^i\sigma _y^{i+1} +J_z\sigma _z^i\sigma _z^{i+1}+B_z\sigma _z^i+2\vec {D}.(\vec {\sigma ^i}\times \vec {\sigma ^{i+1}})\bigg ]}, \end{aligned}$$
(9)

where \( \sigma _{j} \), \( J_j \) and \(D_j\) with \(j=x,y,z\) are one of Pauli matrices, coupling constant and component of the spin–orbit interaction in j direction, respectively. The periodic boundary condition is \(N+1\equiv 1\). When system is in thermal equilibrium (T), the density matrix of system can be described as [15],

$$\begin{aligned} \rho (T)=\frac{1}{Z}\sum _{i=1}^{2^N}{\bigg [e^{-\beta E_i}|\psi _i\rangle \langle \psi _i|\bigg ]}, \end{aligned}$$
(10)

eigenvalues and eigenstates of the Hamiltonian are \(E_j\) and \(|\psi _j\rangle \), respectively. Partition function (Z) is defined as \(Z=\sum _{j=1}^{2^N}{e^{-\beta E_j}}\) when \(\beta =\frac{1}{k_BT}\) with assumption \(k_B=1\).

2.1 Spin–orbit interaction in x the direction

The Hamiltonian of system Eq. (9) with spin–orbit interaction in the x direction for a three-qubit system is as follows:

$$\begin{aligned} H_x= & {} \frac{J_x}{2}(\sigma ^1_x\sigma ^2_x+\sigma ^2_x\sigma ^3_x+\sigma ^3_x\sigma ^1_x) +\frac{J_y}{2}(\sigma ^1_y\sigma ^2_y+\sigma ^2_y\sigma ^3_y+\sigma ^3_y\sigma ^1_y)\nonumber \\{} & {} +\frac{J_z}{2}(\sigma ^{1}_z\sigma ^{2}_z+\sigma ^{2}_z\sigma ^{3}_z+\sigma ^{3}_z\sigma ^{1}_z) +\frac{B_z}{2}(\sigma ^{1}_z+\sigma ^{2}_z+\sigma ^{3}_z)\nonumber \\{} & {} +D_x(\sigma _y^1\sigma _z^2-\sigma _y^2\sigma _z^1+\sigma _y^2\sigma _z^3-\sigma _y^3\sigma _z^2 +\sigma _y^3\sigma _z^1-\sigma _y^1\sigma _z^3), \end{aligned}$$
(11)

with,

$$\begin{aligned} \omega= & {} \sqrt{{B_{{z}}}^{2}-2\,B_{{z}}J_{{z}}+J_{{x}}B_{{z}}+J_{{y}}B_{{z}}+{ J_{{z}}}^{2}-J_{{x}}J_{{z}}-J_{{y}}J_{{z}}+{J_{{x}}}^{2}-J_{{x}}J_{{y} }+{J_{{y}}}^{2}},\nonumber \\ \Omega= & {} \sqrt{{B_{{z}}}^{2}+2\,B_{{z}}J_{{z}}-J_{{x}}B_{{z}}-J_{{y}}B_{{z}}+{ J_{{z}}}^{2}-J_{{x}}J_{{z}}-J_{{y}}J_{{z}}+{J_{{x}}}^{2}-J_{{x}}J_{{y} }+{J_{{y}}}^{2}},\nonumber \\ \eta= & {} J_{{x}}+J_{{y}}+J_{{z}}. \end{aligned}$$
(12)

The eigenvalues of Hamiltonian are as follows:

$$\begin{aligned} E_{{1x}}= & {} \frac{1}{2}\,\eta -\frac{1}{2}\,B_{{z}}+\omega ,\nonumber \\ E_{{2x}}= & {} \frac{1}{2}\,\eta -\frac{1}{2}\,B_{{z}}-\omega ,\nonumber \\ E_{{3x}}= & {} \frac{1}{2}\,\eta +\frac{1}{2}\,B_{{z}}+\Omega ,\nonumber \\ E_{{4x}}= & {} \frac{1}{2}\,\eta +\frac{1}{2}\,B_{{z}}-\Omega ,\nonumber \\ E_{{5x}}= & {} E_{{6x}}=-\frac{1}{2}\,\eta +\frac{1}{2}\sqrt{48\,{D_{{x}}}^{2}+{B_{{z}}}^{2}},\nonumber \\ E_{{7x}}= & {} E_{{8x}}=-\frac{1}{2}\,\eta -\frac{1}{2}\sqrt{48\,{D_{{x}}}^{2}+{B_{{z}}}^{2}}, \end{aligned}$$
(13)

and also corresponding eigenvectors of Hamiltonian are as follows:

$$\begin{aligned} |\psi _{1x}\rangle= & {} \frac{1}{N_{1x}} \left[ \begin{array}{cccccccc} 0&\frac{1}{3}\,{\frac{3\,B_{{z}}-3\,J_{{z}}+ 2\,E_{{1x}}}{J_{{x}}-J_{{y}}}}&\frac{1}{3}\,{\frac{3\,B_{{z}}-3\,J_{{z}}+2\,E_ {{1x}}}{J_{{x}}-J_{{y}}}}&0&\frac{1}{3}\,{\frac{3\,B_{{z}}-3\,J_{{z}}+2\,E_{{1x }}}{J_{{x}}-J_{{y}}}}&0&0&1\end{array} \right] ^T,\nonumber \\ |\psi _{2x}\rangle= & {} \frac{1}{N_{2x}} \left[ \begin{array}{cccccccc} 0&\frac{1}{3}\,{\frac{2\,{ E_{2x}}+3\,B_{{z}} -3\,J_{{z}}}{J_{{x}}-J_{{y}}}}&\frac{1}{3}\,{\frac{2\,{ E_{2x}}+3\,B_{{z}}-3\, J_{{z}}}{J_{{x}}-J_{{y}}}}&0&\frac{1}{3}\,{\frac{2\,{ E_{2x}}+3\,B_{{z}}-3\,J_ {{z}}}{J_{{x}}-J_{{y}}}}&0&0&1\end{array} \right] ^T, \nonumber \\ |\psi _{3x}\rangle= & {} \frac{1}{N_{3x}}\left[ \begin{array}{cccccccc} {\frac{2\,{ E_{3x}}-2\,J_{{x}}-2\,J_{ {y}}+J_{{z}}+B_{{z}}}{J_{{x}}-J_{{y}}}}&0&0&1&0&1&1&0\end{array} \right] ^T,\nonumber \\ |\psi _{4x}\rangle= & {} \frac{1}{N_{4x}}\left[ \begin{array}{cccccccc} {\frac{2\,E_{{4x}}-2\,J_{{x}}-2\,J_{{ y}}+J_{{z}}+B_{{z}}}{J_{{x}}-J_{{y}}}}&0&0&1&0&1&1&0\end{array} \right] ^T,\nonumber \\ |\psi _{5x}\rangle= & {} \frac{1}{N_{5x}} \left[ \begin{array}{cccccccc} 0&-2&1&-\frac{1}{4}\,{\frac{-i\eta +iB_{{z}}- 2\,iE_{{5x}}}{D_{{x}}}}&1&\frac{1}{4}\,{\frac{-i\eta +iB_{{z}}-2\,iE_{{5x}}}{D_{{x}}}}&0&0\end{array} \right] ^T,\nonumber \\ |\psi _{6x}\rangle= & {} \frac{1}{N_{6x}}\left[ \begin{array}{cccccccc} 0&-\frac{1}{4}\,{\frac{i\eta +iB_{{z}}+2\,iE_ {{5x}}}{D_{{x}}}}&\frac{1}{4}\,{\frac{i\eta +iB_{{z}}+2\,iE_{{5x}}}{D_{{x}}} }&-2&0&1&1&0\end{array} \right] ^T,\nonumber \\ |\psi _{7x}\rangle= & {} \frac{1}{N_{7x}}\left[ \begin{array}{cccccccc} 0&-2&1&-\frac{1}{4}\,{\frac{i\eta -iB_{{z}}+2 \,iE_{{7x}}}{D_{{x}}}}&1&\frac{1}{4}\,{\frac{i\eta -iB_{{z}}+2\,iE_{{7x}}}{D _{{x}}}}&0&0\end{array} \right] ^T,\nonumber \\ |\psi _{8x}\rangle= & {} \frac{1}{N_{8x}} \left[ \begin{array}{cccccccc} 0&-2&1&-\frac{1}{4}\,{\frac{-i\eta +iB_{{z}}- 2\,iE_{{7x}}}{D_{{x}}}}&1&\frac{1}{4}\,{\frac{-i\eta +iB_{{z}}-2\,iE_{{7x}}}{D_{{x}}}}&0&0\end{array} \right] ^T. \end{aligned}$$
(14)

Where the symbol T is transpose operation here and the normalization constants are as follows:

$$\begin{aligned} N_{{1 x}}= & {} \frac{1}{3}\,\sqrt{9+3\,{\frac{ \left( 3\,B_{{z}}-3\,J_{{z}}+2\,E_{{1x}} \right) ^{2}}{ \left( J_{{x}}-J_{{y}} \right) ^{2}}}},\nonumber \\ N_{{2x}}= & {} \frac{1}{3}\,\sqrt{9+3\,{\frac{ \left( 2\,{ E_{2x}}+3\,B_{{z}}-3\,J_{{z}} \right) ^{2}}{ \left( J_{{x}}-J_{{y}} \right) ^{2}}}},\nonumber \\ N_{{3x}}= & {} \sqrt{{\frac{ \left( 2\,{ E_{3x}}-2\,J_{{x}}-2\,J_{{y}}+J_{{z}}+B_{{z }} \right) ^{2}}{ \left( J_{{x}}-J_{{y}} \right) ^{2}}}+3},\nonumber \\ N_{{4x}}= & {} \sqrt{{\frac{ \left( 2\,{ E_{4x}}-2\,J_{{x}}-2\,J_{{y}}+J_{{z}}+B_{{z }} \right) ^{2}}{ \left( J_{{x}}-J_{{y}} \right) ^{2}}}+3},\nonumber \\ N_{{5x}}= & {} \frac{1}{4}\,\sqrt{96+2\,{\frac{ \left( i \eta -iB_{{z}}+2\,iE_{{5x}} \right) \left( -i \eta +iB_{{z}}-2\,iE_{{5x}} \right) }{{D_{{x}}}^{2}}}},\nonumber \\ N_{{6x}}= & {} \frac{1}{4}\,\sqrt{96+2\,{\frac{ \left( -i\eta -iB_{{z}}-2\,iE_{{5x}} \right) \left( i\eta +iB_{{z}}+2\,iE_{{5x}} \right) }{{D_{{x}}}^{2}} }},\nonumber \\ N_{{7x}}= & {} \frac{1}{4}\,\sqrt{96+2\,{\frac{ \left( i\eta -iB_{{z}}+2\,iE_{{7x}} \right) \left( -i\eta +iB_{{z}}-2\,iE_{{7x}} \right) }{{D_{{x}}}^{2} }}},\nonumber \\ N_{{8x}}= & {} \frac{1}{4}\,\sqrt{96+2\,{\frac{ \left( -i\eta +iB_{{z}}-2\,iE_{{7x}} \right) \left( i\eta -iB_{{z}}+2\,iE_{{7x}} \right) }{{D_{{x}}}^{2}} }}. \end{aligned}$$
(15)

The model Hamiltonian Eq. (9) is isotropic (cyclic in x, y, and z) when \(B_z=0\). It is symmetric about the z-axis when \(B_z\ne 0\), so it leads to same eigenvalues and eigenvectors for spin–orbit interaction in y direction (\(D_y\ne 0,D_x=D_z=0\)) and spin–orbit interaction in x direction (\(D_y=D_z=0, D_x\ne 0\)). So, study of spin–orbit interaction in y direction leads to same result with spin–orbit interaction in x direction.

2.2 Spin–orbit interaction in the z direction

The Hamiltonian of system Eq. (9) with spin–orbit interaction in the z direction for a three-qubit system is as follows:

$$\begin{aligned} H_z= & {} \frac{J_x}{2}(\sigma ^1_x\sigma ^2_x+\sigma ^2_x\sigma ^3_x+\sigma ^3_x\sigma ^1_x) +\frac{J_y}{2}(\sigma ^1_y\sigma ^2_y+\sigma ^2_y\sigma ^3_y+\sigma ^3_y\sigma ^1_y)\nonumber \\{} & {} +\frac{J_z}{2}(\sigma ^{1}_z\sigma ^{2}_z+\sigma ^{2}_z\sigma ^{3}_z+\sigma ^{3}_z\sigma ^{1}_z) +\frac{B_z}{2}(\sigma ^{1}_z+\sigma ^{2}_z+\sigma ^{3}_z)\nonumber \\{} & {} +D_z(\sigma _x^1\sigma _y^2-\sigma _x^2\sigma _y^1+\sigma _x^2\sigma _y^3 -\sigma _x^3\sigma _y^2+\sigma _x^3\sigma _y^1-\sigma _x^1\sigma _y^3). \end{aligned}$$
(16)

The eigenvalues of system are as follows:

$$\begin{aligned} E_{{1z}}= & {} -\frac{1}{2}\,\eta +\frac{1}{2}\,B_{{z}}+2\,\sqrt{3}D_{{z}},\nonumber \\ E_{{2z}}= & {} -\frac{1}{2}\,\eta +\frac{1}{2}\,B_{{z}}-2\,\sqrt{3}D_{{z}},\nonumber \\ E_{3z}= & {} -\frac{1}{2}\,\eta -\frac{1}{2}\,B_{{z}}+2\,\sqrt{3}D_{{z}},\nonumber \\ E_{4z}= & {} -\frac{1}{2}\,\eta -\frac{1}{2}\,B_{{z}}-2\,\sqrt{3}D_{{z}},\nonumber \\ E_{5z}= & {} -\frac{1}{2}\,B_{{z}}+\frac{1}{2}\,\eta +\omega ,\nonumber \\ E_{6z}= & {} -\frac{1}{2}\,B_{{z}}+\frac{1}{2}\,\eta -\omega ,,\nonumber \\ E_{7z}= & {} \frac{1}{2}\,B_{{z}}+\frac{1}{2}\,\eta +\Omega ,\nonumber \\ E_{8z}= & {} \frac{1}{2}\,B_{{z}}+\frac{1}{2}\,\eta -\Omega . \end{aligned}$$
(17)

And also corresponding eigenvectors of Hamiltonian are as follows:

$$\begin{aligned} |\psi _{1z}\rangle= & {} \frac{1}{N_{1z}} \left[ \begin{array}{cccccccc} 0&-\frac{1}{2}\,i\sqrt{3} -\frac{1}{2}&\frac{1}{8}\,{\frac{- i\eta -4\,D_{{z}}+4\,i\sqrt{3}D_{{z}}}{D_{{z}}}}&0&1&0&0&0\end{array} \right] ^T,\nonumber \\ |\psi _{2z}\rangle= & {} \frac{1}{N_{2z}} \left[ \begin{array}{cccccccc} 0&\frac{1}{2}\,i\sqrt{3} -\frac{1}{2}&-\frac{1}{8}\,{\frac{i \eta +4\,D_{{z}}+4\,i\sqrt{3}D_{{z}}}{D_{{z}}}}&0&1&0&0&0\end{array} \right] ^T, \nonumber \\ |\psi _{3z}\rangle= & {} \frac{1}{\sqrt{3}} \left[ \begin{array}{cccccccc} 0&0&0&-\frac{1}{2}\,i\sqrt{3}-\frac{1}{2}&0&-\frac{1}{2}+\frac{1}{2} \,i\sqrt{3}&1&0\end{array} \right] ^T, \nonumber \\ |\psi _{4z}\rangle= & {} \frac{1}{\sqrt{3}} \left[ \begin{array}{cccccccc} 0&0&0&-\frac{1}{2}+\frac{1}{2}\,i\sqrt{3}&0&-\frac{1}{2}\,i \sqrt{3}-\frac{1}{2}&1&0\end{array} \right] ^T \nonumber \\ |\psi _{5z}\rangle= & {} \frac{1}{N_{5z}} \left[ \begin{array}{cccccccc} 0&1&1&0&1&0&0&-{\frac{2\,B_{{z}}+ \eta -3\,J_{{z}}-2\,\omega }{J_{{x}}-J_{{y}}}}\end{array} \right] ^T, \nonumber \\ |\psi _{6z}\rangle= & {} \frac{1}{N_{6z}}\left[ \begin{array}{cccccccc} 0&1&1&0&1&0&0&-{\frac{2\,B_{{z}}+\eta -3\,J_{{z}}+2\,\omega }{J_{{x}}-J_{{y}}}}\end{array} \right] ^T,\nonumber \\ |\psi _{7z}\rangle= & {} \frac{1}{N_{7z}} \left[ \begin{array}{cccccccc} {\frac{2\,B_{{z}}-\eta +3\,J_{{z}}+2 \,\Omega }{J_{{x}}-J_{{y}}}}&0&0&1&0&1&1&0\end{array} \right] ^T,\nonumber \\ |\psi _{8z}\rangle= & {} \frac{1}{N_{8z}}\left[ \begin{array}{cccccccc} {\frac{2\,B_{{z}}-\eta +3\,J_{{z}}-2 \,\Omega }{J_{{x}}-J_{{y}}}}&0&0&1&0&1&1&0\end{array} \right] ^T, \end{aligned}$$
(18)

where the symbol T is transpose operation and the corresponding normalization constants are as follows:

$$\begin{aligned} N_{1z}= & {} \frac{1}{8}\,\sqrt{-{\frac{-192\,{D_{{z}}}^{2}-{\eta }^{2}+8\,\eta \,\sqrt{3} D_{{z}}}{{D_{{z}}}^{2}}}},\nonumber \\ N_{2z}= & {} \frac{1}{8}\,\sqrt{{\frac{192\,{D_{{z}}}^{2}+{\eta }^{2}+8\,\eta \,\sqrt{3}D_ {{z}}}{{D_{{z}}}^{2}}}},\nonumber \\ N_{5z}= & {} \sqrt{3+{\frac{ \left( 2\,B_{{z}}+\eta -3\,J_{{z}}-2\,\omega \right) ^{2}}{ \left( J_{{x}}-J_{{y}} \right) ^{2}}}},\nonumber \\ N_{6z}= & {} \sqrt{3+{\frac{ \left( 2\,B_{{z}}+\eta -3\,J_{{z}}+2\,\omega \right) ^{2}}{ \left( J_{{x}}-J_{{y}} \right) ^{2}}}},\nonumber \\ N_{7z}= & {} \sqrt{{\frac{ \left( 2\,B_{{z}}-\eta +3\,J_{{z}}+2\,\Omega \right) ^{ 2}}{ \left( J_{{x}}-J_{{y}} \right) ^{2}}}+3},\nonumber \\ N_{8z}= & {} \sqrt{{\frac{ \left( 2\,B_{{z}}-\eta +3\,J_{{z}}-2\,\Omega \right) ^{ 2}}{ \left( J_{{x}}-J_{{y}} \right) ^{2}}}+3}. \end{aligned}$$
(19)

In this system calculations show that \(N_{AB}=N_{AC}=N_{BC}\), so we assume that \(N_{AB}=N_{AC}=N_{BC}\equiv N\). It is cumbersome to obtain density operator, LQ, N, \(N^3\) and \(\tau \) analytically, due to the large number of parameters, so we study measures numerically.

3 Effect of magnetic field

In this section, we consider the effect of magnetic field on quantum correlations of three-qubit Heisenberg XYZ system. Figure 1a shows quantum correlations when there is no spin–orbit interaction (\(\vec {D}=0\)) as a function of magnetic field in the z direction. The \(\tau \), \(N^3\), LQ and N measures are maximum when \(B_z=0\). After \(B_z=0\) point, as \(|B_z|\) increases, the \(\tau \) measure has sudden and sharp change; then, like other measures tend to zero slowly. \(N^3\) and N measures show more resistance against the increase in \(|B_z|\), while LQ shows little quantum correlations in the absence of magnetic field, which becomes zero with the increase in \(|B_z|\). Figure 1b demonstrates that in the presence of spin–orbit interaction \( D_x=1 \), in an interval of \(|B_z|\le 1.8\), all measures have constant values. In this interval, one can see that the values of \(\tau \) and then LQ are the highest compared to other measures, for \(|B_z|\ge 1.8\) measures decrease suddenly and tends to zero, it can be seen in this figure that LQ then \(\tau \) go zero. Both N and LQ become zero at \(|B_z|\approx 1.8\); after this point, N increases suddenly then slowly tends to zero. The figures verify that presence of spin–orbit interaction has increased the quantum correlations. In the presence of \(D_z=1\) as Fig. 1c shows at the intervals of \(B_z\in (-2.5,-0.2)\) and \(B_z\in (0.2,2.5)\), all measures have constant values as \(\tau>N^3>LQ>N\). In \(|B_z|\approx 0\), all measures have a minimum value; in fact at this point, a sudden change in all measures occurs which the range of changes is the largest for \(\tau \) and it is the smallest for N. On the other hand, values of measures in Fig. 1c are greater than values of Fig. 1a, b, so one can find that same direction of spin–orbit interaction and magnetic field enhances the correlations. An another interesting result of figures is that N and \(N^3\) are more robust than \(\tau \) and LQ to increasing the magnetic field.

Fig. 1
figure 1

LQ (black plus line), \(\tau \) (red dashed line), \(N_{AB}=N_{AC}=N_{BC}\) (green solid line) and \(N^3\) (blue space dashed line) as a function of \(B_z\) for \(T=0.02,J_x=1,J_y=1.5\) and \(J_z=-1\), a \(D_y=D_x= D_z=0\), b \(D_x=1,D_y= D_z=0\), c \(D_z=1,D_y=D_x=0\) (Color figure online)

Full size image
Fig. 2
figure 2

LQ (black plus line), \(\tau \) (red dashed line), \(N_{AB}=N_{AC}=N_{BC}\) (green solid line) and \(N^3\) (blue space dashed line) for \(T=0.02,J_x=1,J_y=1.5\) and \(J_z=-1\), a, as a function of \(D_x\) when \(D_y=D_z=0,B_z=0\), b as a function of \(D_x\) when \(D_y= D_z=0,B_z=3\), c as a function of \(D_z\) when \(D_x=0,D_y=0, B_z=3\) (Color figure online)

Full size image

4 Effect of spin–orbit interaction

In this section, we study effect of spin–orbit interaction on correlations. Figure 2a, b show effect of spin–orbit interaction in the x direction in the absence and presence of magnetic field, respectively. We can find that in the absence of magnetic field, in the intervals of \(|D_x|\ge 0.3\), all measures have constant values which \(\tau>LQ>N^3>N\). It is noticeable that they are greater than values of measures in the interval of \(|D_x|\le 0.3\), in this interval LQ ia approximately zero. In the presence of magnetic field as Fig. 2b shows the measures have fixed values such that \(\tau>LQ>N^3>N\) in the interval of \(D_x\in (-4,-1.5)\) and these values are equal to values of measures in absence of magnetic field in interval \(|D_x|\ge 0.3\), then they suddenly decrease and have constant value approximately at \(D_x\in (-1.5,1.5)\) such that \(N^3>N>\tau >LQ=0\); these values are very small in comparison to values of measures in interval of \(|D_x|\le 0.3\) in \(B=0\) case (Fig. 2a); after that, they suddenly increase and have constant value approximately at \(D_x\in (1.5,4)\), such that \(\tau>LQ>N^3>N\). Effect of spin–orbit interaction on quantum correlations in the y direction in absence and presence of magnetic field are similar to spin–orbit interaction in the x direction case (\(D_x\)). In absence of magnetic field, behavior of quantum correlations as a function of \(D_z\) are similar to behavior of quantum correlations of \(D_x\) and \(D_y\) cases. With effect of magnetic field, one can find that in interval of \(D_z\in (-4,-1.2)\) quantum correlations are constant and they have more values in comparison with other figures. As Fig. 2c demonstrates in interval of \(D_z\in (-1.2,1.2)\), all measures have the same values of Fig. 2b, which is interesting. Then, measures suddenly increase and they have constant value at interval of \(D_z\in (1.2,4)\). It is clear that same direction of magnetic field and spin–orbit interaction causes more values of correlation and increase of interval of \(D_z\) with more values of correlation. Presence of magnetic field has made bigger the interval of \(D_{x(z)}\) which measures are minimum and at this interval \(N^3\) and N are more robust than \(\tau \) and LQ against spin–orbit interaction.

5 Effect of coupling constant in the x direction

In this section, we study effect of coupling constant in the x direction. Figure 3a shows the measures in absence of spin–orbit interaction, for \(J_x<0\) they decrease as \( J_x \) increases for interval of \(-4<J_x<-1.3\) measures are \(\tau>N^3>LQ>N\). At the \(J_x=-1.3\) point, N will be more than LQ and also in \(J_x=0.3\) point, \(N^3\) will become more than \(\tau \). All measures are zero at \(J_x\approx 2\), after this point for interval of \(2<J_x<3.2\) values of all measures increase such that \(N^3>N>\tau >LQ\). One can find that at interval of \(J_x\ge 3.2\), \(\tau >N\). In the presence of spin–orbit interaction in x direction (\(D_x=1\)) as Fig. 3b shows, for starting from \(J_x<0\), firstly measures decrease as \(J_x\) increases till they reach a minimum value at \(J_x\approx 1.9\), they behave as Fig. 3a. After this minimum, all measures increase and reach a constant value at \(J_x\in (2.1,4)\) which is smaller than values of measures at \(J_x=-4\), in this interval \(\tau>LQ>N^3>N\). Measures for \(D_y=1\) case, which behave similar to \( D_x=1 \) case. For \(D_z=1\) case, Fig. 3c demonstrates that for \(J_x<0\), with increasing of \(J_x\) measurements decrease till they reach minimum values around \(J_x\approx 1\). Before this minimum, all measures behave as Fig. 3a, b. After this minimum, the measures reach to constant values which are greater than values of measures at \(J_x=-4\) (starting point) such that \(\tau>N^3>LQ>N\). By comparison of figures one can find that when magnetic field and spin–orbit interaction are in the same direction, minimum value happens in the smaller \(J_x\) and after this point we can find more quantum correlations than other cases (\(\vec {D}=0\), \(D_x=1\) and \(D_y=1\)). Presence of spin–orbit has been caused that minimum point shifts for smaller \(J_x\)s, and then reach to fixed and larger values in comparison to absence of this interaction. Figures show that \(N^3\) is more robust against change of \(J_x\) in comparison with other measures.

Fig. 3
figure 3

LQ (black plus line), \(\tau \) (red dashed line), \(N_{AB}=N_{AC}=N_{BC}\) (green solid line) and \(N^3\) (blue space dashed line) as a function of \(J_x\) for \(T=0.02, B_z=1,J_y=2\) and \(J_z=-2\) a \(D_y=D_x= D_z=0\) b \(D_x=1,D_y= D_z=0\), c \(D_z=1,D_y=D_x=0\) (Color figure online)

Full size image

6 Effect of coupling constant in the z direction

In this section, we investigate the effect of coupling constant in the z direction (\( J_z \)). Figure 4a shows, in the absence of spin–orbit interaction, in the interval of \(J_z<-0.4\) as \(J_z\) increases measures increase very little such that \(N^3>N>\tau >LQ=0\). It can be seen that there is a sudden increase for all measures at \(J_z\approx -0.4\); after this sudden change point, all measures increase suddenly; then, they reach constant values in which their values are greater than initial values at \(J_z=-4\) such that \(\tau>N^3>LQ>N\). With consideration of spin–orbit interaction in the x direction (\(D_x=1\)) as Fig. 4b represents, in interval of \(-4<J_z<-1.9\) measures are \(N^3>N>\tau >LQ\), they have a sudden change at \(J_z\approx -1.9\). After this sudden change point, all measures have constant values which are smaller than no spin–orbit interaction case (in same intervals of \(J_z\)). For \(D_y=1\) case, one can find that all measures are similar to measures of \(D_x=1\) case. Spin–orbit interaction in z direction is considered in Fig. 4c, there is a sudden change point at \(J_z\approx -2.2\), after this point all measures have been increased and they reach constant values which are greater than no spin–orbit interaction, \(D_x=1\) and \(D_y=1\), cases. The tripartite negativity is greater than local quantum uncertainty in absence of spin–orbit interaction and \(D_z=1\) cases, while for \(D_x=1\) and \(D_y=1\), after sudden change point tripartite negativity is smaller than local quantum uncertainty. Figures demonstrate that for \(J_z<0\), before sudden change point, \(N^3\) and N are more robust against \(J_z\) in comparison with \(\tau \) and LQ.

Fig. 4
figure 4

LQ (black plus line), \(\tau \) (red dashed line), \(N_{AB}=N_{AC}=N_{BC}\) (green solid line) and \(N^3\) (blue space dashed line) for \(T=0.02, B_z=1,J_x=1\) and \(J_y=2\), a \(D_y=D_x= D_z=0\), b \(D_x=1,D_y= D_z=0\), c \(D_z=1,D_y=D_x=0\) (Color figure online)

Full size image
Fig. 5
figure 5

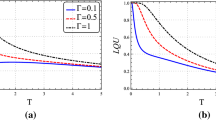
LQ (black plus line), \(\tau \) (red dashed line), \(N_{AB}=N_{AC}=N_{BC}\) (green solid line) and \(N^3\) (blue space dashed line) as a function of T for \(J_x=1,J_y=0.5\) and \(J_z=-2\) a \(D_y=D_x= D_z=0, B_z=0\) b \(D_x=0,D_y= D_z=0, B_z=3\) c \(D_x=1,D_y= D_z=0,B_z=3\) d \(D_y=1,D_x= D_z=0,B_z=3\) e \(D_z=1,D_y=D_x=0,B_z=3\) (Color figure online)

Full size image

7 Effect of temperature

In this section, we study the effect of temperature and spin–orbit interaction on correlations. Figure 5a shows measures in absence of spin–orbit interaction and magnetic field. Firstly, N at \(T=0.31\) then \(N^3\) at \(T=0.51\) go to zero. As figure shows \(\tau \) is decreasing for a small interval of temperature \(T\in (0,0.11)\), then decreases very much and at the final goes zero asymptotically. LQ is constant at \(T\in (0,0.02)\); then, it increases till \(T=0.61\); after that, it decreases and tend to zero. Figure 5a verifies that LQ and \(\tau \) are more robust than N and \(N^3\) against increasing temperature. In the presence of magnetic field in z direction in Fig. 5b, all measures have smaller values in comparison with absence of magnetic field (Fig. 5a). In the interval of \(T\in (0,0.31)\), N is constant; then, it decreases and goes zero at \(T=1.11\). One can find that from this figure, \(N^3\) is constant at the interval of \(T\in (0,0.41)\); then at \(T=0.41\), it reduces till becomes zero at \(T=1.51\). For LQ when temperature is in interval of \(T\in (0,0.5)\), quantum correlations are fixed; then, they increase till \(T=2.11\); after this point, they decrease. As Fig. 5b shows, \(\tau \) is constant at \(T\in (0,0.5)\); then, it decreases till \(T=0.71\) point; then, it increases until it reaches the point \(T=2.31\) and then it reduces. So, it is clear that in the presence of magnetic field measures become zero at higher temperature in comparison with \(B=0\) case. With considering spin–orbit interaction in x direction \(D_x=1\) and magnetic field \(B_z=3\) in Fig. 5c, one can find that quantum correlations have increased in comparison with Fig. 5b. Firstly, N is fixed till \(T=0.21\); then, it decreases in interval of \(T\in (0.21,0.5)\) and become zero at \(T=0.5\); then, it is nonzero at interval of \(T\in (0.5,2.3)\), and finally at \(T=2.3\) point, it becomes zero. For \(N^3\), in the interval of \(T\in (0,0.11)\), measure is constant; then, it decreases till at \(T=0.61\); after this point, \(N^3\) firstly increases, then decreases and become zero at \(T=3.5\). The \(\tau \) and LQ are constant at \(T\in (0,0.21)\); then, they increase at \(T=1.51\) and then they decrease. Figure 5d shows effects of spin–orbit interaction in the z direction \((D_z=1)\). It has been caused that N and \(N^3\) start from nonzero value at \(T=0\); then, they decease in intervals of \(T\in (0,0.11)\) and \(T\in (0,0.21)\), respectively; after these intervals, they have nonzero values at \(T\in (0.11,2.11)\) and \(T\in (0.21,2.21)\), respectively. The measures of N and \(N^3\) in Fig. 5d become zero at \(T=0.11\) and \(T=0.21\), respectively. These measures become zero faster in comparison with Fig. 5c, which is very noticeable. The measures of LQ and \(\tau \) increase in intervals of \(T\in (0,0.36)\) and \(T\in (0,1.11)\), respectively; after these intervals, they decrease. In comparison of Fig. 5c with Fig. 5d, the maximum values of N and \(N^3\) in \(D_z=1\) case have decreased while LQ has increased. Figures show that LQ and \(\tau \) are more robust against increasing temperature.

8 Conclusions

In this paper, we investigated effects of magnetic field, spin–orbit interaction in different directions, coupling constants and temperature on quantum correlations of a three-qubit Heisenberg XYZ system. For measuring quantum correlations, we apply LQ, \(\tau \), N and \(N^3\) measures. Increasing the magnetic field decreases the quantum correlations. Results show that quantum correlations increase more when the magnetic field and spin–orbit interaction are in the same direction (here z direction). The \(N^{3}\) and N are better measures than LQ and \(\tau \) against magnetic field and spin–orbit interaction because they are nonzero in some parameter intervals where LQ and \(\tau \) are zero. With considering coupling constant in z direction, the quantum correlations are less for the negative couplings, and as the coupling constant increases, a sudden increase is observed; after that, we will see that the quantum correlations will remain constant. With the effect of spin–orbit interaction, this sudden change point shifts toward negative couplings. When coupling constant and magnetic field are not in the same direction, the measures have high values at negative couplings; as coupling constant increases, quantum correlations decrease to reach a minimum value; then, they increase and have constant values. \(N^3\) and N are better measures in comparison with \(\tau \) and LQ, because in the some interval of coupling constant, the \(\tau =LQ=0\), while \(N^3\) and N are nonzero. As the temperature increases, the quantum correlations decrease and it can be seen the sudden death of N and \(N^3\), while the LQ and \(\tau \) increase in some points with the increase in temperature. Presence of spin–orbit interaction causes that N and \(N^3\) be nonzero in a larger range of temperature. The LQ and \(\tau \) measures are more robust against increasing temperature.