1 Introduction

Quantum entanglement, which is viewed as physical resource, plays an important role in quantum computation and quantum communication [1, 2]. Due to the nonlocal correlation between the subsystems of an entangled system, we can choose it to accomplish some tasks better than those done in classical world, for example, quantum teleportation, which enables teleporting an unknown quantum state by classical communication and local quantum operations [3].

In the past few years, the bulk of studies have concentrated on a simple solid-state system, Heisenberg spin chain [4], which has been exploited in simulating optical lattice [5], quantum dots [6, 7], electronic spins [8], nuclear spins [9], and quantum sate transfer [10]. The fundamental operations of a quantum computer have been demonstrated experimentally with high accuracy (or “high fidelity” in quantum computing language) in trapped ion systems. Promising schemes in development to scale the system to arbitrarily large numbers of qubits include shuttling ions in an array of ion traps, building large entangled states via photonically connected networks of remotely entangled ion chains, and combinations of these two ideas. This makes the trapped ion quantum computer system one of the most promising architectures for a scalable, universal quantum computer. As of May 2011, the largest number of particles to be controllably entangled is 14 trapped ions. Moreover, there is a growing interest in quantum teleportation in virtue of thermal entangled state of Heisenberg spin chain, which is approximately near to real circumstances. In references [11–14], quantum teleportation in thermal entangled state of two-qubit XX, XXX, XXZ Heisenberg models has been studied. However, three-qubit entangled states have more advantages over the two-qubit ones [3, 15–17]. So in quantum teleportation, three-qubit Heisenberg models have been demonstrated in references [18–20]. But those studies only consider the nearest neighbor spin–spin interactions. The next-to-nearest neighbor interactions and multiple spin-exchange models [21–26] should gain great attention because they are closer to real situation in quasi-one-dimensional magnets than the nearest neighbor interactions. For example, optical lattices, constructed of equilateral triangles [27], always exist three-site interactions [28–30], with which the entanglement and quantum critical behavior in Heisenberg models are investigated carefully [31–33]. Motivated by these, in this paper we focus on the thermal entanglement of a three-qubit XXZ Heisenberg model with three-site interactions in an external magnetic field, and teleport an unknown state via the chain in thermal equilibrium state. In addition, we discuss the relation between the thermal entanglement and quantum teleportation.

2 The model and its thermal equilibrium state

The Hamiltonian for a three-qubit Heisenberg XXZ spin chain with three-site interactions [24, 27] in external magnetic field is described by

$$\begin{aligned} H_{XXZ}= & {} \sum _{l=1}^3 {\left[ J\left( \sigma _l^x \sigma _{l+1}^x +\sigma _l^y \sigma _{l+1}^y \right) +J_Z \sigma _l^z \sigma _{l+1}^z +B\sigma _l^z \right] } \nonumber \\&+\sum _{l=1}^3 {J^{+}\left[ \sigma _l^x \sigma _{l+1}^z \sigma _{l+2}^x +\sigma _l^y \sigma _{l+1}^z \sigma _{l+2}^y \right] } \nonumber \\&+\sum _{l=1}^3 {J^{-}\left[ \sigma _l^x \sigma _{l+1}^z \sigma _{l+2}^y -\sigma _l^y \sigma _{l+1}^z \sigma _{l+2}^x \right] } , \end{aligned}$$
(1)

where \(J\) is the coupling strength between the nearest neighbor sites in the \(x\) and \(y\) directions, and \(J_Z \) is the coupling constant in the \(z\) directions. Here, all of the other parameters are scaled by \(J\), so the parameters \(J^{+}, J^{-}, J_Z , T\), and \(B\) are dimensionless. \(J^{+}\) and \(J^{-}\) present the \(\hbox {XZX}+\hbox {YZY}\) [34] type of three-site interaction and the \(\text{ XZY }- \hbox {YZX}\) [35] one, respectively. The model becomes the XXX model when \(J_Z =J\) and reduces to XX model when \(J_Z =0\).

By solving the Schrödiner equation \(H_{XXZ} \left| \psi \right\rangle =E\left| \psi \right\rangle \), the eigenstates and the corresponding eigenvalues are given as following,

$$\begin{aligned} \begin{array}{ll} \left| {\psi _0 } \right\rangle =\left| {000} \right\rangle , &{}\quad E_0 =3(J_Z +B), \\ \left| {\psi _1 } \right\rangle =(x\left| {001} \right\rangle +x^{2}\left| {010} \right\rangle +\left| {100} \right\rangle )/\sqrt{3}, &{}\quad E_1 =B-J_Z -2J-2J^{+}-2\sqrt{3}J^{-}, \\ \left| {\psi _2 } \right\rangle =(x^{2}\left| {001} \right\rangle +x\left| {010} \right\rangle +\left| {100} \right\rangle )/\sqrt{3}, &{}\quad E_2 =B-J_Z -2J-2J^{+}+2\sqrt{3}J^{-}, \\ \left| {\psi _3 } \right\rangle =(\left| {001} \right\rangle +\left| {010} \right\rangle +\left| {100} \right\rangle )/\sqrt{3}, &{}\quad E_3 =B-J_Z +4J+4J^{+}, \\ \left| {\psi _4 } \right\rangle =(x\left| {110} \right\rangle +x^{2}\left| {101} \right\rangle +\left| {011} \right\rangle )/\sqrt{3}, &{}\quad E_4 =-B-J_Z -2J+2J^{+}-2\sqrt{3}J^{-}, \\ \left| {\psi _5 } \right\rangle =(x^{2}\left| {110} \right\rangle +x\left| {101} \right\rangle +\left| {011} \right\rangle )/\sqrt{3}, &{}\quad E_5 =-B-J_Z -2J+2J^{+}+2\sqrt{3}J^{-}, \\ \left| {\psi _6 } \right\rangle =(\left| {110} \right\rangle +\left| {101} \right\rangle +\left| {011} \right\rangle )/\sqrt{3}, &{}\quad E_6 =-B-J_Z +4J-4J^{+}, \\ \left| {\psi _7 } \right\rangle =\left| {111} \right\rangle , &{}\quad E_7 =3(J_Z -B), \end{array}\nonumber \\ \end{aligned}$$
(2)

where \(x=\exp (2i\pi /3), \left| 0 \right\rangle \) and \(\left| 1 \right\rangle \) denote spin-up and spin-down states, respectively. The density matrix of the composite system in equilibrium at temperature \(T\) is written as

$$\begin{aligned} \rho _{ABC} =\frac{1}{Z}\exp (-\beta H)=\frac{1}{Z}\sum _{i=0}^7 {\exp (-\beta E_i )} \left| {\psi _i } \right\rangle \left\langle {\psi _i } \right| , \end{aligned}$$
(3)

where the partition function \(Z=Tr[\exp (-\beta H)]=Tr\sum \nolimits _{i=0}^7 {\exp (-\beta E_i )} \left| {\psi _i } \right\rangle \left\langle {\psi _i } \right| \) with \(\beta =1/{\kappa T}\). From here on, we let Boltzmann’s constant \(\kappa =1\).

3 Thermal entanglement of the model

The three reduced density matrices \(\rho _{AB} =Tr_C \rho _{ABC},\quad \rho _{BC} =Tr_A \rho _{ABC}, \quad \rho _{AC} =Tr_B \rho _{ABC}\) are equal because of symmetry under cyclic shifts. Concurrence [36], which is used to quantify the amount of entanglement corresponding to \(\rho _{AB} \), is calculated as,

$$\begin{aligned} C=\max \left\{ {\left. {\lambda _1 -\lambda _2 -\lambda _3 -\lambda _4 ,0} \right\} } \right. , \end{aligned}$$
(4)

where \(\lambda _1 \ge \lambda _2 \ge \lambda _3 \ge \lambda _4 \) are the square roots of the eigenvalues of the matrix \(R=\rho _{AB} (\sigma ^{y}\otimes \sigma ^{y})\rho _{AB}^{*} (\sigma ^{y}\otimes \sigma ^{y})\), and \(\rho _{AB}^{*} \) is the complex conjugation of \(\rho _{AB} \). After some straightforward algebra, we obtain

$$\begin{aligned} C=\max \left\{ {\left. {\frac{2}{Z}\left( {\left| {\left. {\eta _1 } \right| } \right. -\sqrt{\eta _2 \eta _3 }} \right) ,0} \right\} } \right. , \end{aligned}$$
(5)

here,

$$\begin{aligned} \eta _1= & {} \frac{1}{3}\left[ {-e^{-\beta E_1 }+e^{-\beta E_3 }-e^{-\beta E_4 }+e^{-\beta E_6 }} \right] , \nonumber \\ \eta _2= & {} \frac{1}{3}\left[ {3e^{-\beta E_0 }+2e^{-\beta E_1 }+e^{-\beta E_3 }} \right] , \nonumber \\ \eta _3= & {} \frac{1}{3}\left[ {3e^{-\beta E_7 }+2e^{-\beta E_4 }+e^{-\beta E_6 }} \right] . \end{aligned}$$
(6)

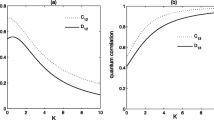
The model is said to be ferromagnetic for \(J\prec 0\) and antiferromagnetic for \(J\succ 0\) [37, 38]. Thermal entanglement of antiferromagnetic case about this model is discussed in Ref. [31], so here we only need to discuss the ferromagnetic case. If \(J^{+}=0\) and \(J^{-}=1\), it only includes \(\hbox {XZY}-\text{ YZX }\) type interaction, while \(J^{+}=1\) and \(J^{-}=0\), the model only includes \(\hbox {XZX}+\hbox {YZY}\) type interaction. When \(B\leftrightarrow -B\), we find \(E_0 \leftrightarrow E_7, E_1 \leftrightarrow E_4, E_2 \leftrightarrow E_5 \) and \(E_3 \leftrightarrow E_6 \) on the case \(J^{+}=0\). When \(J^{+}=1\), the relation vanishes. We give the plot of the concurrence \(C\) as a function of the external field \(B\) with \(T=1\) for different interactions in Fig. 1a, where the dotted line and the dot-dashed line, which stand for the system without three-site interaction and with \(\text{ XZY }- \hbox {YZX}\) type interaction, respectively, are invariant under \(B\rightarrow -B\), and there is some entanglement when \(B=0\). But for antiferromagnetic system, there is no entanglement under \(B=0\) [31], which means ferromagnetic system could induce entanglement under the case \(B=0\). When \(J^{+}=1\), entanglements emerge under \(B=0\) and quickly reach their maximum values, which are larger than the counterparts when \(J^{+}=0\). In other words, \(\hbox {XZX}+\hbox {YZY}\) interaction could increase entanglement. When \(J^{+}=1\) and \(J^{-}=0\), the range of \(B\), under which the maximal concurrence maintains, is wider than the case \(J^{+}=J^{-}=1\). In other words, when \(\hbox {XZX}+\hbox {YZY}\) interaction exists alone, there is a widest range of \(B\).

Fig. 1
figure 1

a Concurrence \(C\) as a function of magnetic field \(B\) for different interactions with \(J=-1, J_Z =1\) and \(T=1\). b Concurrence \(C\) as a function of temperature \(T\) for different interactions with \(J=-1, J_Z =1\) and \(B=0\)

Full size image

In Fig. 1b, we give the plot of the concurrence \(C\) as a function of temperature \(T\) with \(B=0\) for different interactions. It is clearly that for the four cases the entanglement monotonically descends with increasing \(T\) until it reaches the critical value \(T_C \), above which the entanglement vanishes. It is thus an interesting problem to determine the critical temperatures \(T_C \). From Eq. (5), \(T_C \) clearly depends on the external magnetic field \(B=0\) and other parameters \(J, J_Z , J^{+}\), and \(J^{-}\). \(T_C \) could be obtained by numerically solving \(\frac{2}{Z}\left( {\left| {\left. {\eta _1 } \right| } \right. -\sqrt{\eta _2 \eta _3 }} \right) =0\). As shown in the picture, whether \(\text{ XZY }- \hbox {YZX}\) interaction exists, concurrences reach their maximum values under zero-temperature limit and maintain under a range of temperature. And the maximum values of the case \(J^{+}=1\) are lager than the case \(J^{+}=0\). It means that \(\hbox {XZX}+\hbox {YZY}\) interaction enhances entanglement maximum value. When \(J^{+}=J^{-}=0\), the model reduces to XXZ Heisenberg chain without any three-site interaction, and its entanglement cannot exceed 1/3 under \(B=0\). The conclusion consists with reference [19]. When \(J^{+}=1\) and \(J^{-}=0\), the critical temperature \(T_C \) is higher than the case \(J^{+}=J^{-}=1\). In other words, when \(\hbox {XZX}+\hbox {YZY}\) interaction exists alone, the critical temperature \(T_C \) is the largest. In this case, we could get \(T_C =T_1 \approx 1.16146K\) by numerically solving \(\frac{2}{Z}\left( {\left| {\left. {\eta _1 } \right| } \right. -\sqrt{\eta _2 \eta _3 }} \right) =0\) when \(B=0\). We also note that \(T_C \) similarly increases with increasing \(B\). For large enough \(B, \frac{2}{Z}\left( {\left| {\left. {\eta _1 } \right| } \right. -\sqrt{\eta _2 \eta _3 }} \right) =0\) reduces to \(\left| {\psi _2 } \right\rangle \), with \(z\equiv e^{-\beta J}\), which can be numerically solved, giving \(T_C =T_1 ^{{\prime }}\approx 1.22459K\). So, \(T_1 \) increases with increasing \(B\) up to \(T_1^{\prime } \), as long as \(B\) is not infinitely large. Physically, one could understand this phenomenon by looking at the thermal density operator, Eq. (3), and the concurrence, Eq. (5). We can find that while an equally weighted mixture of \(\left| {\psi _2 } \right\rangle , \left| {\psi _3 } \right\rangle , \left| {\psi _5 } \right\rangle \), and \(\left| {\psi _6 } \right\rangle \) has zero concurrence, an equally weighted mixture of \(\left| {\psi _5 } \right\rangle \) and \(\left| {\psi _6 } \right\rangle \), and \(\left| {\psi _4 } \right\rangle \) has nonzero concurrence. At nonzero temperatures, increasing \(B\) creates a diminishing proportion of \(\left| {\psi _2 } \right\rangle \) and \(\left| {\psi _3 } \right\rangle \), but an increasing proportion of \(\left| {\psi _4 } \right\rangle , \left| {\psi _5 } \right\rangle , \left| {\psi _6 } \right\rangle \) (entangled states) and of course \(\left| {111} \right\rangle \) (unentangled state). The small but nonzero proportion of entangled states contributes to the nonzero thermal concurrence.

In view of the importance of the \(\text{ XZX }+\text{ YXY }\) interaction discussed above, we will next turn our attention toward the model with \(\hbox {XZX}+\hbox {YXY}\) interaction alone. In Fig. 2, we plot the concurrence \(C\) as a function of temperature \(T\) with \(B=1\) for different anisotropy parameters \(J_Z\). It indicates that with \(J_Z \) increased the critical temperature \(T_C \) is enhanced, and the concurrence is also enhanced under a fixed temperature, but cannot exceed the maximum value 2/3, which is lager than antiferromagnetic system [31].

Fig. 2
figure 2

Concurrence \(C\) as a function of temperature \(T\) for different anisotropy parameters \(J_Z\) with \(J=-1\) and \(B=1\)

Full size image

4 Quantum teleportation via the model

Now we consider the standard quantum teleportation protocol [3] using the above three-qubit thermal equilibrium state \(\rho _{ABC} \) as a quantum channel. The teleportation involves a sender Alice and two receivers Bob and Cindy. Alice has two quantum systems, one-qubit X to be teleported and the spin A of the model. Bob and Cindy are in possession of the remaining spins B and C, respectively. The total system could be written as,

$$\begin{aligned} \rho _{XABC} =\rho _X \otimes \rho _{ABC} , \end{aligned}$$
(7)

where

$$\begin{aligned} \rho _X =\left| \psi \right\rangle _X \left\langle \psi \right| , \end{aligned}$$
(8)

with

$$\begin{aligned} \left| \psi \right\rangle _X =\cos \frac{\theta }{2}\left| 0 \right\rangle _X +e^{i\phi }\sin \frac{\theta }{2}\left| 1 \right\rangle _X ,\,(0\le \theta \le \pi ,\,0\le \phi \le 2\pi ). \end{aligned}$$
(9)

To teleport the input state \(\rho _X\) to Bob and Cindy, Alice need to do a joint Bell basis measurement on her two qubits X and A, which is described by the operators \(\Pi _{XA}^j \otimes I_{BC}\) (\(j=1,2,3,4\)), where

$$\begin{aligned}&\Pi _{XA}^1 =\left| {\Phi ^{+}} \right\rangle _{XA} \left\langle {\Phi ^{+}} \right| , \quad \Pi _{XA}^2 =\left| {\Phi ^{-}} \right\rangle _{XA} \left\langle {\Phi ^{-}} \right| , \nonumber \\&\Pi _{XA}^3 =\left| {\Psi ^{+}} \right\rangle _{XA} \left\langle {\Psi ^{+}} \right| , \quad \Pi _{XA}^4 =\left| {\Psi ^{-}} \right\rangle _{XA} \left\langle {\Psi ^{-}} \right| , \end{aligned}$$
(10)

here

$$\begin{aligned} \left| {\Phi ^{\pm }} \right\rangle _{XA}= & {} \frac{1}{\sqrt{2}}\left( {\left| {00} \right\rangle _{XA} \pm \left| {11} \right\rangle _{XA} } \right) , \nonumber \\ \left| {\Psi ^{\pm }} \right\rangle _{XA}= & {} \frac{1}{\sqrt{2}}\left( {\left| {01} \right\rangle _{XA} \pm \left| {10} \right\rangle _{XA} } \right) \end{aligned}$$
(11)

are the Bell states, and \(I_{BC} \) is the identity operator on the composite system BC. When Alice gets the measurement outcome \(j\), she broadcasts the result to Bob and Cindy by a classical channel, and then, we obtain the composite state of B and C,

$$\begin{aligned} \rho _{BC}^j =\frac{1}{P_j }Tr_{XA} \left[ {\left( {\Pi _{XA}^j \otimes I_{BC} } \right) \rho _{XABC} } \right] , \end{aligned}$$
(12)

where

$$\begin{aligned} P_j =Tr_{XABC} \left[ {\left( {\Pi _{XA}^j \otimes I_{BC} } \right) \rho _{XABC} } \right] \end{aligned}$$
(13)

stands for the probability for Alice to get the measurement outcome \(j\). From Eqs. (3), (7), and (10), we obtain

$$\begin{aligned} P_1= & {} P_2 =\frac{1}{12Z}\left( {g_1 -g_2 \cos \theta } \right) , \nonumber \\ P_3= & {} P_4 =\frac{1}{12Z}\left( {g_1 +g_2 \cos \theta } \right) , \end{aligned}$$
(14)

with

$$\begin{aligned} g_1= & {} 6e^{-3\beta J_Z }\cosh 3\beta B+6e^{\beta (J_Z -4J)}\cosh \beta (B+4J^{+}) \nonumber \\&+12e^{\beta (J_Z +2J)}\cosh 2\sqrt{3}\beta J^{-}\cosh \beta (B-2J^{+}), \nonumber \\ g_2= & {} 6e^{-3\beta J_Z }\sinh 3\beta B+2e^{\beta (J_Z -4J)}\sinh \beta (B+4J^{+}) \nonumber \\&+\,4e^{\beta (J_Z +2J)}\cosh 2\sqrt{3}\beta J^{-}\sinh \beta (B-2J^{+}). \end{aligned}$$
(15)

Next, Bob and Cindy perform corresponding unitary operations \(U^{i}\) on their own qubits according to the measurement result from Alice, such that

$$\begin{aligned} \rho _{Bout}^j =\rho _{Cout}^j =\rho _{out}^j =U^{j}\rho ^{j}U^{j+}, \end{aligned}$$
(16)

where \(U^{i}\) is written as, \(U^{1}=\sigma _x , U^{2}=\sigma _y , U^{3}=I, U^{4}=\sigma _z \), and \(\rho ^{j}=Tr_C \rho _{BC}^j =Tr_B \rho _{BC}^j \).

Usually, we use fidelity [39] \(F^{j}\) to measure the quality of the quantum teleportation between the input state \(\rho _X \) and the output state \(\rho _{out}^j \). The average fidelity, averaged over all possible Alice’s measurement results \(j\) and over all the input states \(\rho _X \) on the Bloch sphere, is given by

$$\begin{aligned} \bar{{F}}=\frac{1}{4\pi }\int _0^{2\pi } {\int _0^\pi {\sin \theta } } d\theta d\phi \sum _{j=1}^4 {P_j } F^{j}, \end{aligned}$$
(17)

where \(F^{j}=Tr(\rho _{out}^j \rho _X )\).

It follows from Eqs. (8) and (16) that

$$\begin{aligned} F_1= & {} F_2 =\frac{f_1 +f_2 \cos 2\theta }{2(g_1 -g_2 \cos \theta )}, \nonumber \\ F_3= & {} F_4 =\frac{f_1 +f_2 \cos 2\theta }{2(g_1 +g_2 \cos \theta )}, \end{aligned}$$
(18)

where

$$\begin{aligned} f_1= & {} 3e^{-3\beta J_Z }\cosh 3\beta B+9e^{\beta (J_Z -4J)}\cosh \beta (B+4J^{+}) \nonumber \\&+12e^{\beta (J_Z +2J)}\cosh 2\sqrt{3}\beta J^{-}\cosh \beta (B-2J^{+}), \nonumber \\ f_2= & {} -3e^{-3\beta J_Z }\cosh 3\beta B-e^{\beta (J_Z -4J)}\cosh \beta (B+4J^{+}) \nonumber \\&+\,4e^{\beta (J_Z +2J)}\cosh 2\sqrt{3}\beta J^{-}\cosh \beta (B-2J^{+}). \end{aligned}$$
(19)

From Eqs. (14), (17), and (18), we can obtain

$$\begin{aligned} \bar{{F}}=\frac{1}{3}+\frac{4}{9Z}\left[ {\begin{array}{l} 2e^{-4\beta J}\cosh \beta (B+4J^{+}) \\ +\,e^{2\beta J}\cosh 2\sqrt{3}\beta J^{-}\cosh \beta (B-2J^{+}) \\ \end{array}} \right] e^{\beta J_Z }. \end{aligned}$$
(20)

In order to make the quantum teleportation successful, we must make sure \(\bar{{F}}\) is larger than \(2/3\), which is the best fidelity in the classical world, so we get the inequality

$$\begin{aligned}&e^{\beta (J_Z -4J)}\cosh \beta (B+4J^{+})-4e^{\beta (J_Z +2J)}\cosh 2\sqrt{3}\beta J^{-}\cosh \beta (B-2J^{+}) \nonumber \\&\quad -\,3e^{-\beta J_Z }\cosh 3\beta B\succ 0. \end{aligned}$$
(21)

It is easy to testify that only \(J\prec 0\) is suitable for the equivalent, which means only ferromagnetic chain satisfies quantum teleportation. For nonzero temperatures, it is again an interesting problem to determine the critical temperature \(T_C \) beyond which \(\overline{F} \le \frac{2}{3}\). From Eq. (21), \(T_C \) is clearly dependent on the magnetic field \(B\). They can be obtained by numerically

$$\begin{aligned}&e^{\beta (J_Z -4J)}\cosh \beta (B+4J^{+})-4e^{\beta (J_Z +2J)}\cosh 2\sqrt{3}\beta J^{-}\cosh \beta (B-2J^{+}) \nonumber \\&\quad -\,3e^{-\beta J_Z }\cosh 3\beta B=0 \end{aligned}$$
(22)

When \(B=0\), we could get \(T_C =T_1 \approx 1.25136K\). This means that all nonzero thermal entanglement is “suitable” as a resource for teleportation. The mixing of states here clearly does not have a devastating effect on the quality of the thermal entanglement. We also find that with increasing \(B\) we have an interesting range of nonzero thermal entanglement which is, however, not able to yield \(\overline{F} \succ 2/3\). Physically, one could attribute the course of the poor quality of thermal entanglement to the fact that there is now a comparable or greater proportion of unentangled \(\left| {111} \right\rangle \) than the “teleportation grade” \(\left| {\psi _1 } \right\rangle \) and \(\left| {\psi _4 } \right\rangle \).

In Fig. 3a, we give the plot of the average fidelity as a function of temperature \(T\) for different interactions with \(B=1\). We can easily find that the average fidelity of the four cases monotonically descends with increasing \(T\) until it reaches the critical value \(T_C^{\prime } \), above which average fidelity is less than the classical limit 2/3. And as \(\hbox {XZX}+\hbox {YZY}\) interaction emerges, the critical temperatures are higher than without any interaction, while the critical temperature is decreased obviously when \(\text{ XZY }- \hbox {YZX}\) interaction exists alone. So even if the two interactions exist, the critical temperature is lower than \(\hbox {XZX}+\hbox {YZY}\) interaction exists alone. In the zero-temperature limit, we can obtain the maximal average fidelity 7/9, which consists with the conclusion mentioned in [19].

Fig. 3
figure 3

a Average fidelity \(\overline{F}\) as a function of temperature \(T\) for different interactions with \(J=-1, J_Z \!=\!1, B=1\). b Average fidelity \(\overline{F} \) as a function of magnetic field \(B\) for different interactions with \(J=-1, J_Z =1\), and \(T=1\)

Full size image

In Fig. 3b, we give the plot of the average fidelity as a function of temperature \(B\) for different interactions with \(T=1\). When \(B\rightarrow -B\), eigenvalues \(E_0 \rightarrow E_7, E_1 \rightarrow E_4, E_2 \rightarrow E_5 \), and \(E_3 \rightarrow E_6 \) on the case \(J^{+}=0\). So the average fidelity is invariant under \(B\rightarrow -B\) on the case. When \(J^{+}=1\), the relation vanishes. So the average fidelity will change if \(B\rightarrow -B\). For weak magnetic field, we can get the maximal average fidelity and can also see that the maximal average fidelity of the case \(\text{ XZY }- \hbox {YZX}\) interaction is the lowest. When \(J^{+}=1\) and \(J^{-}=0\), the range of \(B\), under which the maximal concurrence maintains, is wider than the case \(J^{+}=J^{-}=1\). In other words, when \(\hbox {XZX}+\hbox {YZY}\) interaction exists alone, there is a widest range of \(B\).

Because \(\hbox {XZX}+\hbox {YZY}\) interaction is in favor of entanglement, average fidelity and critical temperatures, while \(\text{ XZY }- \hbox {YZX}\) interaction against all of them, we choose it as the resource of quantum teleportation. In Fig. 4, we show its average fidelity in terms of temperature \(T\) for some anisotropy parameters \(J_Z \) under \(B=1\). It is seen that with \(J_Z \) increased the critical temperature \(T_C^{\prime } \) is enhanced, and the average fidelity is also enhanced under a fixed temperature.

Fig. 4
figure 4

Average fidelity \(\overline{F} \) as a function of temperature \(T\) for different anisotropy parameters \(J_Z \) with \(J=-1\) and \(B=1\)

Full size image

In the end, we discuss the relation between entanglement and average fidelity about the model with only \(\hbox {XZX}+\hbox {YZY}\) type interaction. In Fig. 5a, b, we show average fidelity versus concurrence when the magnetic field and temperature are allowed to changed, respectively. From Fig. 5a where the temperatures are allowed to change for several magnetic fields, we can see that average fidelity monotonically increases with increasing \(C\) when magnetic field is lower than 6, which is larger than the model without any interaction [19]. However, when magnetic field is above 6, there exit two different average fidelities corresponding to one concurrence. Figure 5b is another case where the magnetic fields are allowed to change for several temperatures. One can see that when the average fidelity is small, the average temperature is a monotone increasing function of concurrence, but with the concurrence increased, one concurrence gives two average fidelities. From Fig. 5a, b, we find entanglement cannot completely reflect the average fidelity, which means larger amount of entanglement does not mean higher fidelity.

Fig. 5
figure 5

Average fidelity \(\overline{F}\) as a function of concurrence \(C\) with \(J=-1\) and \(J_Z =1\). a is plotted for varied temperatures under several magnetic fields. b is plotted for varied magnetic fields under several temperatures

Full size image

5 Summary

In this paper, we have investigated the thermal entanglement of a three-qubit XXZ Heisenberg model with three-site interactions in an external magnetic field, and the quantum teleportation via this model in thermal equilibrium state. It is found that when the chains are in ground states, both the entanglement and the average fidelity reach their maximal values. The entanglement and the average fidelity become decreased under high temperature, strong magnetic field, and small anisotropy parameters \(J_Z \). The ferromagnetic system is regarded as the requirement in quantum teleportation. Only \(\hbox {XZX}+\hbox {YZY}\) interaction is in favor of entanglement, average fidelity, and critical temperatures, while \(\text{ XZY }- \hbox {YZX}\) interaction against all of them. In addition, we have found entanglement does not fully reflect average fidelity, and the same entanglement corresponds to different average fidelities.