1 Introduction

In recent decades, the propagation of laser beams through turbulent environments has garnered increasing attention owing to its wide range of applications in laser communications, remote sensing, laser radar, and optical weapons, among others. A multitude of research on various properties of laser beams in turbulent media, including beam wander, beam spreading, scintillation index, intensity distribution, and field correlations (Saad and Belafhal 2017; Yuan et al. 2013; Wu et al 2022; Chang et al. 2020; Korotkova et al. 2012; Hricha et al. 2021; Nossir et al. 2021) have been investigated. Furthermore, advancements in ultra-short pulse technology have interesting advantages leading to a growing body of literature exploring spectral effects in laser beams of which propagating through different media and optical systems (Zou and Hu 2016; Duan et al. 2020; Benzehoua et al. 2021a, b; Benzehoua and Belafhal 2023a). The spectral effects on laser beams, such as spectral shifts and spectral switches having a significant potential in optical communication, information encoding and transmission, and lattice spectroscopy (Han 2009; Liu and Lü, 2004; Kandpal and Vaishya 2002).

Several investigations have been studied to understand the spectral changes of laser beams as they propagate through different media and optical systems. Since then, Zou and Hu, (2016) focused on the spectral anomalies of a diffracted chirped pulsed super-Gaussian beam, while Liu et al. (2017) explored the spectral and coherence properties of a Gaussian Schell-model pulsed beam in a turbulent atmosphere. Jo et al. (2021) examined the spectral properties of a chirped Gaussian pulsed beam propagating through a slant turbulent atmosphere path , and Duan et al. (2020) investigated the impact of biological tissue and spatial correlation on the spectral changes of a Gaussian-Schell model vortex beam .

Recently, researchers have shown an increasing interest in investigating the properties of random beam propagation in oceanic turbulence due to its practical significance in applications like optical underwater communication, imaging, and sensing. In their study, Yan et al. (2022) focus on optimizing parameters for the perfect optical vortex in optical communication within a challenging absorptive and turbulent seawater environment. Furthermore, the authors have conducted an analysis of both the bit-error rate and the average capacity of an underwater wireless communication link, characterized by absorption and turbulence, utilizing a perfect Laguerre–Gauss beam (Yang et al. 2022). In the context of oceanic turbulence, Ding et al. (2018) studied the impact of turbulence kinetic energy dissipation rate and other factors on the spectral changes of partially coherent Gaussian Schell-model pulsed beams in two dimensions. Moreover, Zhu et al. (2021) contributed to the field by studying the spectral properties of partially coherent chirped Airy pulsed beam in oceanic turbulence. Recently, Jo et al. (2022) present the study of oceanic turbulence's impact on the spectral changes of a diffracted chirped Gaussian pulsed beam, aiming to understand the distortion effects of oceanic turbulence on the intensity and shape of the beam. More recently, Benzehoua and Belafhal presented two notable studies about the field of spectral beam propagation. The first study investigated the spectral properties of pulsed Laguerre higher-order cosh-Gaussian beams as they propagated through turbulent atmospheres (Benzehoua and Belafhal 2023b). In the second study, they focused on analyzing the behavior of pulsed vortex beams in oceanic turbulence (Benzehoua and Belafhal 2023c). Additionally, the research also explored the effects of atmospheric turbulence on the spectral changes of diffracted pulsed hollow higher-order cosh-Gaussian beams (Benzehoua and Belafhal 2023d).

The aim of our current manuscript is focused on study the spectral properties of pulsed chirped GHCG beam passing through oceanic turbulence and can organize as follows: in Sect. 2, we establish the electric field distribution of the pulsed chirped GHCG beam in the Cartesian coordinate system at the source plane. Additionally, we present the theoretical model that describes the spectral behaviors of the pulsed beam as it propagates through turbulent oceanic environments. Section 3 is devoted to presenting the graphical analysis of the impact of the beam and turbulent oceanic parameters on the evolution behavior of the pulsed chirped GHCG beam. Finally, Sect. 4 provides the concluding remarks be summarized.

2 Propagation of pulsed chirped GHCG beam through the oceanic turbulence

To investigate the behavior of the pulsed chirped GHCG beam in turbulent oceanic environments, our analysis begins with a thorough examination of its Fourier monochromatic component propagation. Subsequently, we carry out the inverse Fourier transform to derive the propagation equation in the space–time domain.

In the Cartesian coordinates system, the initial electrical field of the pulsed chirped GHCG beam in space–time domain in the source plane (z = 0), can be expressed as (Saad and Belafhal 2022)

$$\begin{gathered} E\left( {s_{x1} ,s_{y1} ,t} \right) = A_{0} \left( {\frac{{s_{x1} }}{{w_{0} }}} \right)^{l} H_{u} (\beta s_{x1} )\cosh^{n} \left( {\Omega s_{x1} } \right)e^{{\left( { - s_{x1}^{2} /w_{0}^{2} } \right)}} \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \left( {\frac{{s_{y1} }}{{w_{0} }}} \right)^{l} H_{v} (\beta s_{y1} )\cosh^{n} \left( {s_{y1} \Omega } \right)e^{{\left( { - s_{y1}^{2} /w_{0}^{2} } \right)}} \,A\left( t \right), \hfill \\ \end{gathered}$$
(1)

with \(A_{0}\) is being amplification factor, \(\left( {s_{x1}^{{}} ,s_{y1}^{{}} } \right)\) represents the coordinates on the source plane, l is beam order, \(w_{0}\) is the waist radius of the Gaussian part, \(n\) are the beam order, \(\Omega\) is the displacement parameter associated with the cosh part, \(H_{u} (.)\) and \(H_{v} (.)\) denote the Hermite polynomials in the x- and y- directions, and \(A\left( t \right)\) is the temporal envelop of the initial pulsed beam.

The source field expression in Eq. (1), can be rearranged using the Euler expansion and series transformation (Gradshteyn and Ryzhik 1994) to yield the following form:

$$\begin{gathered} E\left( {s_{x1} ,s_{y1} ,z = 0,t} \right) = \frac{{A_{0} }}{{2^{2n} }}\left( {\frac{{s_{x1} }}{{w_{0} }}} \right)^{l} \left( {\frac{{s_{y1} }}{{w_{0} }}} \right)^{l} H_{u} (\beta s_{x1} )H_{v} (\beta s_{y1} )\sum\limits_{m = 0}^{n} {\sum\limits_{p = 0}^{n} {\left( {\begin{array}{*{20}c} n \\ m \\ \end{array} } \right)} \left( {\begin{array}{*{20}c} n \\ p \\ \end{array} } \right)\exp \left( {a_{m}^{2} \delta + a_{p}^{2} \delta } \right)} \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \exp \left\{ { - \left( {\frac{{s_{x1}^{2} }}{{w_{0}^{{}} }} - a_{m} \sqrt \delta } \right)^{2} } \right\}\exp \left\{ { - \left( {\frac{{s_{y1}^{2} }}{{w_{0}^{{}} }} - a_{p} \sqrt \delta } \right)^{2} } \right\}A\left( t \right), \hfill \\ \end{gathered}$$
(2)

where

$$a_{m} = \left( {m - \frac{n}{2}} \right),\;a_{p} = \left( {p - \frac{n}{2}} \right),\;{\text{and}}\;\delta = w_{0}^{2} \Omega^{2} ,$$
(3)

In Eq. (1) the incident pulsed beam is assumed as a chirped Gaussian one given by Ji et al. ( 2007)

$$A\left( t \right) = exp\left[ { - \frac{{\left( {1 + iC} \right)t^{2} }}{{2T^{2} }}} \right]\exp ( - i\omega_{0} t),$$
(4)

where \(\omega_{0}\), T and C denote central frequency, pulse duration and chirp parameter, respectively.

In the space–frequency domain, the initial field of pulsed chirped GHCG beam with the angular frequency ω can be obtained by Fourier transform

$$E\left( {s_{x1} ,s_{y1} ,\omega } \right) = \frac{1}{{\sqrt {2\pi } }}\int\limits_{ - \infty }^{ + \infty } {E\left( {s_{x1} ,s_{y1} ,t} \right)e^{ - i\omega t} } dt\, = E(s_{x1} ,s_{y1} ,z = 0)A(\omega ),$$
(5)

where \(A(\omega )\) is the original Fourier spectrum of the pulsed beam and is given by

$$A(\omega ) = \frac{1}{{\sqrt {2\pi } }}\int\limits_{ - \infty }^{ + \infty } {f(t){\text{e}}^{ - i\omega t} } dt.$$
(6)

Substituting Eqs. (2) and (4) into Eq. (5), we can obtain the initial field of space-frequency domain as

$$\begin{gathered} E\left( {s_{x} ,s_{y} ,z = 0,\omega } \right) = \frac{{A_{0} }}{{2^{2n} }}\left( {\frac{{s_{x} }}{{w_{0} }}} \right)^{l} \left( {\frac{{s_{y} }}{{w_{0} }}} \right)^{l} H_{u} (\beta s_{x} )H_{v} (\beta s_{y} )\sum\limits_{m = 0}^{n} {\sum\limits_{p = 0}^{n} {\left( {\begin{array}{*{20}c} n \\ m \\ \end{array} } \right)} \left( {\begin{array}{*{20}c} n \\ p \\ \end{array} } \right)\exp \left( {a_{m}^{2} \delta + a_{p}^{2} \delta } \right)} \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \exp \left\{ { - \left( {\frac{{s_{x}^{2} }}{{w_{0}^{{}} }} - a_{m} \sqrt \delta } \right)^{2} } \right\}\exp \left\{ { - \left( {\frac{{s_{y}^{2} }}{{w_{0}^{{}} }} - a_{p} \sqrt \delta } \right)^{2} } \right\}A(\omega ), \hfill \\ \end{gathered}$$
(7)

and the power spectrum of the resulting beam as

$$S^{\left( 0 \right)} \left( \omega \right) = \left| {A\left( \omega \right)} \right|^{2} ,$$
(8)

with the Fourier spectrum of

$$A(\omega ) = \frac{1}{{\sqrt {2\pi } }}\sqrt {\frac{T}{1 + iC}} \exp \left[ {\frac{{T(\omega - \omega_{0} )}}{2(1 + iC)}} \right].$$
(9)

The propagation of a laser beam passing through in turbulent oceanic at the output plane z can be described based on the extended Huygens–Fresnel diffraction integral as follows (Andrews and Phillips 2005)

$$\begin{gathered} E\left( {s_{x} ,s_{y} ,z = 0,\omega } \right) = - \frac{ik}{{2\pi z}}\exp \left( {ikz} \right)\int\limits_{ - \infty }^{\infty } {\,\int\limits_{ - \infty }^{\infty } \, } E\left( {s_{{x_{0} }} ,s_{{y_{0} }} ,0} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \exp \left\{ { - \frac{ik}{{2z}}\left[ {\left( {s_{{x_{0}^{{}} }}^{2} + s_{{y_{0}^{{}} }}^{2} } \right) - 2\left( {s_{x} s_{{x_{0} }} + s_{y} s_{{y_{0} }} } \right) + \psi \left( {s_{{x_{0} }} ,s_{{y_{0} }} ,s_{x} ,s_{y} } \right)} \right]} \right\}ds_{{x_{0} }} ds_{{y_{0} }} , \hfill \\ \end{gathered}$$
(10)

Here, \(k = \frac{\omega }{c}\) indicate wavenumber with c is the speed of the source radiation in vacuum, \(\left( {s_{x} ,s_{y} } \right)\) refer to receiver plane coordinates, and \(\psi \left( {s_{{x_{0} }} ,s_{{y_{0} }} ,s_{x} ,s_{y} } \right)\) represents the random part of the complex phase that defines the solution to the Rytov method. The cross-spectral density function of the pulsed chirped GHCG beam passing through the turbulent oceanic at the receiver plane is given by

$$\begin{gathered} W\left( {s_{x} ,s_{x} ,s_{y} ,s_{y} ,z,\omega } \right) = \frac{{k^{2} }}{{4\pi^{2} z^{2} }}\int\limits_{ - \infty }^{\infty } {\,\int\limits_{ - \infty }^{\infty } {\,\int\limits_{ - \infty }^{\infty } {\,\int\limits_{ - \infty }^{\infty } {E\left( {s_{{x_{01} }} ,s_{{y_{01} }} ,z = 0,\omega } \right)\,E^{ * } \left( {s_{{x_{02} }} ,s_{{y_{02} }} ,z = 0,\omega } \right)} } } } \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \exp \left[ { - \frac{ik}{{2z}}\left( {s_{{x_{01}^{{}} }}^{2} - s_{{x_{02}^{{}} }}^{2} + s_{{y_{01}^{{}} }}^{2} - s_{{y_{02}^{{}} }}^{2} } \right) - 2s_{x} \left( {s_{{x_{01} }} - s_{{x_{02} }} } \right) - 2s_{y} \left( {s_{{y_{01} }} - s_{{y_{02} }} } \right)} \right] \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \left\langle {\exp \left[ {\psi \left( {s_{{x_{01} }} ,s_{{y_{01} }} ,s_{x} ,s_{y} } \right) + \psi^{*} \left( {s_{{x_{02} }} ,s_{{y_{02} }} ,s_{x} ,s_{y} } \right)} \right]\,} \right\rangle \,\,ds_{{x_{01} }} ds_{{x_{02} }} ds_{{y_{01} }} ds_{{y_{02} }} , \hfill \\ \end{gathered}$$
(11)

where 〈 〉 represents the ensemble average over the medium statistics and * refer to complex conjugate. The ensemble average term is expressed as (Andrews and Phillips 2005)

$$\left\langle {\exp \left[ {\psi \left( {s_{{x_{01} }} ,s_{{y_{01} }} ,s_{x} ,s_{y} } \right) + \psi^{*} \left( {s_{{x_{02} }} ,s_{{y_{02} }} ,s_{x} ,s_{y} } \right)} \right]\,} \right\rangle \,\, = \exp \left\{ { - \frac{1}{{\rho_{0}^{2} }}\left[ {\left( {s_{{x_{01} }} - s_{{x_{02} }} } \right)^{2} + \left( {s_{{y_{01} }} - s_{{y_{02} }} } \right)^{2} } \right]} \right\},$$
(12)

where \(\rho_{0}\) is the spatial coherence radius of a spherical wave in oceanic turbulence, which takes the form (Nikshov and Nikishov 2000)

$$\rho_{0} = \left[ {1.28 \times 10^{ - 8} k^{2} z\eta^{ - 1/3} \chi_{t} \varepsilon^{ - 1/3} \times \left( {6.78 + 47.57\zeta^{ - 2} - 17.67\zeta^{ - 1} } \right)} \right]^{ - 1/2} .$$
(13)

Here \(\eta\) denotes the Kolmogorov micro scale (inner scale), \(\varepsilon\) is the rate of dissipation of kinetic energy per unit mass of fluid ranging from \(10^{ - 10} \,m^{2} /s^{3}\) to \(10^{ - 1} \,m^{2} /s^{3}\), \(\chi_{t}\) is the dissipation rate of temperature variance and has the range \(10^{ - 10} \,K^{2} /s\) to \(10^{ - 2} \,K^{2} /s\), ς is relative intensity of temperature and salinity fluctuations and its range is between –5 to 0 (corresponding to temperature-induced and salinity-induced turbulence, respectively).

By substituting Eqs. (12) and (13) into Eq. (11), we obtain

$$\begin{gathered} W\left( {s_{x} ,s_{x} ,s_{y} ,s_{y} ,z,\omega } \right) = = S^{\left( 0 \right)} \left( \omega \right)\frac{{A_{0}^{2} k^{2} }}{{4\pi^{2} z^{2} 2^{4N} w_{0}^{2l} }}\sum\limits_{m = 0}^{n} {\sum\limits_{n = 0}^{n} {\left( {\begin{array}{*{20}c} n \\ m \\ \end{array} } \right)} \left( {\begin{array}{*{20}c} n \\ p \\ \end{array} } \right)\sum\limits_{{m^{\prime} = 0}}^{n} {\sum\limits_{{p^{\prime} = 0}}^{n} {\left( {\begin{array}{*{20}c} n \\ {m^{\prime}} \\ \end{array} } \right)} \left( {\begin{array}{*{20}c} n \\ {p^{\prime}} \\ \end{array} } \right)} } \int\limits_{ - \infty }^{ + \infty } {\,\int\limits_{ - \infty }^{ + \infty } {\int\limits_{ - \infty }^{ + \infty } {\,\int\limits_{ - \infty }^{ + \infty } {ds_{{x_{01} }} ds_{{x_{02} }} ds_{{y_{01} }} ds_{{y_{02} }} s_{{x_{01} }}^{l} s_{{x_{02} }}^{l} } } } } s_{{y_{01} }}^{l} s_{{y_{02} }}^{l} \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times H_{u} (\beta s_{{x_{02} }} )H_{v} (\beta s_{y2} )exp\left( { - \alpha_{x} s_{{x_{01} }}^{2} + 2\left( {\gamma_{1.x} + \frac{{s_{{x_{02} }} }}{{\rho_{0}^{2} }}} \right)s_{{x_{01} }} } \right)\exp \left[ { - \alpha_{x} s_{{x_{02} }}^{2} + 2\left( {\frac{{a_{{m^{\prime}}} \sqrt \delta }}{{w_{0} }} - \frac{{iks_{x} }}{2z}} \right)s_{{x_{02} }} } \right] \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \,H_{u} (\beta s_{{x_{01} }} )H_{v} (\beta s_{{y_{01} }} )exp\left( { - \alpha_{y} s_{{y_{01} }}^{2} + 2\left( {\gamma_{1.y} + \frac{{s_{{x_{0y} }} }}{{\rho_{0}^{2} }}} \right)s_{{y_{01} }} } \right)\exp \left[ { - \alpha_{y} s_{{y_{02} }}^{2} + 2\left( {\frac{{a_{{p^{\prime}}} \sqrt \delta }}{{w_{0} }} - \frac{{iks_{y} }}{2z}} \right)s_{{y_{02} }} } \right]\,, \hfill \\ \end{gathered}$$
(14)

where

$$\alpha_{x} = \alpha_{y} = \frac{ik}{{2z}} + \frac{1}{{w_{0}^{2} }} + \frac{1}{{\rho_{0}^{2} }},$$
(15)
$$\gamma_{1,x} = \frac{{a_{m} \sqrt \delta }}{{w_{0} }} + \frac{{iks_{x} }}{2z},$$
(16)

and

$$\gamma_{1,y} = \frac{{a_{p} \sqrt \delta }}{{w_{0} }} + \frac{{iks_{y} }}{2z}.$$
(17)

Applying the following formulae (Abramowitz and Stegun 1970; Erdelyi et al. 1954; Belafhal et al. 2020)

$$H_{n} \left( {x + y} \right) = \frac{1}{{2^{{{n \mathord{\left/ {\vphantom {n 2}} \right. \kern-0pt} 2}}} }}\sum\limits_{k = 0}^{n} {\left( {\begin{array}{*{20}c} n \\ k \\ \end{array} } \right)H_{k} \left( {\sqrt 2 x} \right)} H_{n - k} \left( {\sqrt 2 y} \right),$$
(18)
$$H_{n} \left( x \right) = \sum\limits_{k = 0}^{{\left[ {{n \mathord{\left/ {\vphantom {n 2}} \right. \kern-0pt} 2}} \right]}} {\left( { - 1} \right)^{k} \frac{n!}{{k!\left( {n - 2k} \right)!}}\left( {2x} \right)^{n - 2k} } ,$$
(19)
$$\int\limits_{ - \infty }^{ + \infty } {x^{l} \exp \left( { - px^{2} + 2qx} \right)} dx = \sqrt {\frac{\pi }{p}} \exp \left( {\frac{{q^{2} }}{p}} \right)\left( {\frac{1}{2i\sqrt p }} \right)^{l} H_{l} \left( \frac{iq}{p} \right),$$
(20)

and

$$\int\limits_{ - \infty }^{ + \infty } {x^{l} \exp \left( { - px^{2} + 2qx} \right)H_{m} \left( {\alpha x} \right)\,dx} = \sqrt {\frac{\pi }{p}} \frac{{\exp \left( {{{q^{2} } \mathord{\left/ {\vphantom {{q^{2} } p}} \right. \kern-0pt} p}} \right)}}{{2^{l} }}\sum\limits_{k = 0}^{{\left[ {{m \mathord{\left/ {\vphantom {m 2}} \right. \kern-0pt} 2}} \right]}} {\frac{{\left( { - 1} \right)^{k} m!}}{{k!\left( {m - 2k} \right)!}}} H_{m + l - 2k} \left( {\frac{iq}{{\sqrt p }}} \right)\left( {\frac{\alpha }{i\sqrt p }} \right)^{m + l - 2k} ,$$
(21)

and after skipping a lot of tedious calculations, we can deduce the analytical expression of the intensity spectral of pulsed chirped GHCG beam in the turbulent oceanic as

$$S\left( {s_{x} ,s_{y} ,z,\omega } \right) = W\left( {s_{x} ,s_{x} ,s_{y} ,s_{y} ,z,\omega } \right) = S^{\left( 0 \right)} \left( \omega \right)M\left( {s_{x} ,s_{y} ,z,\omega } \right),$$
(22)

where

$$\begin{gathered} M\left( {s_{x} ,s_{y} ,z,\omega } \right) = \frac{{A_{0}^{2} k^{2} }}{{4z^{2} 2^{4n} w_{0}^{4l} }}\frac{1}{{\alpha_{1} \alpha_{2} }}\sum\limits_{m = 0}^{n} {\sum\limits_{p = 0}^{n} {\left( {\begin{array}{*{20}c} n \\ m \\ \end{array} } \right)} \left( {\begin{array}{*{20}c} n \\ p \\ \end{array} } \right)\sum\limits_{{m^{\prime} = 0}}^{n} {\sum\limits_{{p^{\prime} = 0}}^{n} {\left( {\begin{array}{*{20}c} n \\ {m^{\prime}} \\ \end{array} } \right)} \left( {\begin{array}{*{20}c} n \\ {p^{\prime}} \\ \end{array} } \right)} } \exp \left( {\frac{{\gamma_{{_{1.x} }}^{2} }}{{\alpha_{1} }} + \frac{{\gamma_{{_{2.x} }}^{2} }}{{\alpha_{2} }}} \right)\exp \left( {\frac{{\gamma_{{_{1.y} }}^{2} }}{{\alpha_{1} }} + \frac{{\gamma_{{_{2.y} }}^{2} }}{{\alpha_{2} }}} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \sum\limits_{{\mu_{1} = 0}}^{{\left[ {u/2} \right]}} {\sum\limits_{{\mu_{2} = 0}}^{{\left[ {u/2} \right]}} {\frac{{\left( { - 1} \right)^{{\mu_{1} + \mu_{2} }} (u!)^{2} }}{{\mu_{1} !\mu_{2} !\left( {u - 2\mu_{1} } \right)!u\left( {j - 2\mu_{2} } \right)!}}} } \left( {\frac{\beta }{{i\sqrt {\alpha_{1} } }}} \right)^{{l + u - 2\mu_{1} }} (2\beta )^{{j - 2\mu_{2} }} \left( {\frac{1}{{2^{{\frac{{3l + 3u - 2\mu_{1} - 4\mu_{2} }}{2}}} }}} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \sum\limits_{\nu = 0}^{{l + u - 2\mu_{1} }} {\left( {\begin{array}{*{20}c} {l + u - 2\mu_{1} } \\ \nu \\ \end{array} } \right)H_{\nu } } \left( {\frac{{i\sqrt 2 \gamma_{{_{1.y} }}^{{}} }}{{\sqrt {\alpha_{1} } }}} \right)\sum\limits_{\sigma = 0}^{{\left[ {l + u - 2\mu_{1} - \nu /2} \right]}} {\frac{{\left( { - 1} \right)^{\sigma } (l + u - 2\mu_{1} )!}}{{\sigma !\left( {l + u - 2\mu_{1} - \nu - 2\sigma } \right)!}}} \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \left( {\frac{\sqrt 2 }{{\rho_{0}^{2} \sqrt {\alpha_{1} \alpha_{2} } }}} \right)^{{l + 2u - 2\mu_{1} - 2\mu_{2} - \nu - 2\sigma }} H_{{ + 2u - 2\mu_{1} - 2\mu_{2} - \nu - 2\sigma }} \left( {\frac{{i\gamma_{{_{2.x} }}^{{}} }}{{\sqrt {\alpha_{2} } }}} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \sum\limits_{{s_{1} = 0}}^{{\left[ {v/2} \right]}} {\sum\limits_{{s_{2} = 0}}^{{\left[ {v/2} \right]}} {\frac{{\left( { - 1} \right)^{{s_{1} + s_{2} }} (v!)^{2} }}{{s_{1} !s_{2} !\left( {v - 2s_{1} } \right)!\left( {v - 2s_{2} } \right)!}}} } \left( {\frac{\beta }{{i\sqrt {\alpha_{1} } }}} \right)^{{l + v - 2s_{1} }} (2\beta )^{{v - 2s_{2} }} \left( {\frac{1}{{2^{{\frac{{3l + 3v - 2s_{1} - 4s_{2} }}{2}}} }}} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \sum\limits_{{\eta_{1} = 0}}^{{l + v - 2s_{1} }} {\left( {\begin{array}{*{20}c} {l + v - 2s_{1} } \\ {\eta_{1} } \\ \end{array} } \right)H_{{\eta_{1} }} } \left( {\frac{{i\sqrt 2 \gamma_{{_{1.y} }}^{{}} }}{{\sqrt {\alpha_{1} } }}} \right)\sum\limits_{{\eta_{2} = 0}}^{{\left[ {l + v - 2s_{1} - \eta_{1} /2} \right]}} {\frac{{\left( { - 1} \right)^{{\eta_{2} }} (l + v - 2\eta_{2} )!}}{{\eta_{2} !\left( {l + v - 2s_{1} - \eta_{1} - 2\eta_{2} } \right)!}}} \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \left( {\frac{\sqrt 2 }{{\rho_{0}^{2} \sqrt {\alpha_{1} \alpha_{2} } }}} \right)^{{2l + 2v - 2s_{1} - 2s_{2} - \eta_{1} - 2\eta_{2} }} H_{{2l + 2v - 2s_{1} - 2s_{2} - \eta_{1} - 2\eta_{2} }} \left( {\frac{{i\gamma_{{_{2.x} }}^{{}} }}{{\sqrt {\alpha_{2} } }}} \right) \hfill \\ \end{gathered}$$
(23)

with

$$\alpha_{2} = \frac{ik}{{2z}} + \frac{1}{{w_{0}^{2} }} + \frac{1}{{\rho_{0}^{2} }} - \frac{1}{{\rho_{0}^{4} \alpha_{1} }},$$
(24)
$$\gamma_{2,x} = \frac{{a_{{m^{\prime}}} \sqrt \delta }}{{w_{0} }} - \frac{{iks_{x} }}{2z} + \frac{{\gamma_{1,x} }}{{\rho_{0}^{2} \alpha_{1} }},$$
(25)

and

$$\gamma_{2,y} = \frac{{a_{{p^{\prime}}} \sqrt \delta }}{{w_{0} }} - \frac{{iks_{y} }}{2z} + \frac{{\gamma_{1,y} }}{{\rho_{0}^{2} \alpha_{1} }}.$$
(26)

From Eq. (22), \(M\left( {s_{x} ,s_{y} ,z,\omega } \right)\) is called as spectral modifier. One can see that the spectral intensity of pulsed chirped GHCG beam propagating through oceanic turbulence is a product of the original spectrum \(S^{\left( 0 \right)} \left( \omega \right)\) and spectral modifier \(M\left( {s_{x} ,s_{y} ,z,\omega } \right)\). The first part \(S^{\left( 0 \right)} \left( \omega \right)\) depends on the pulse duration T, the central frequency \(\omega_{0}\) and the chirp parameters C, while the second part depends on the observation point, propagation distance z, initial beam parameters and oceanic parameters (ε, \(\varsigma\), and \(\chi_{{_{T} }}\)).

3 Numerical simulations and discussions

In this section, we will investigate the influence of oceanic turbulence on the spectral intensity of a pulsed chirped GHCG beam through numerical calculations using Eq. (22). The normalized on-axis and off-axis spectral intensities of the beam after propagation through oceanic turbulence are analyzed using the relative spectral shift, given by \({{\delta \omega } \mathord{\left/ {\vphantom {{\delta \omega } {\omega_{0} }}} \right. \kern-0pt} {\omega_{0} }} = {{\left( {\omega_{\max } - \omega_{0} } \right)} \mathord{\left/ {\vphantom {{\left( {\omega_{\max } - \omega_{0} } \right)} {\omega_{0} }}} \right. \kern-0pt} {\omega_{0} }}\). Here, \(\omega_{\max }\) refers the frequency related to the maximum spectral intensity. Notably, for \({{\delta \omega } \mathord{\left/ {\vphantom {{\delta \omega } {\omega_{0} }}} \right. \kern-0pt} {\omega_{0} }} > 0\), the spectral intensity exhibits a blue shift, while for \({{\delta \omega } \mathord{\left/ {\vphantom {{\delta \omega } {\omega_{0} }}} \right. \kern-0pt} {\omega_{0} }} < 0\), it shows a red shift.

To simplify the analysis, the normalized original spectrum is taken as \(S^{00} (\omega ) = S^{\left( 0 \right)} \left( \omega \right)/S^{\left( 0 \right)} \left( {\omega_{0} } \right)\), the normalized spectral intensity is \(S(\omega ) = S\left( {s_{x} ,s_{y} ,z,\omega } \right)/S(s_{x} ,s_{y} ,z,\omega_{\max } )\) and the normalized spectral modifier is given by \(M(\omega_{0} ) = M\left( {s_{x} ,s_{y} ,z,\omega_{0} } \right)/M_{\max }^{{}} \left( {s_{x} ,s_{y} ,z,\omega_{0} } \right)\) where \(M_{\max }^{{}} (s_{x} ,s_{y} ,z,\omega_{0} )\) is the maximum value of \(M\left( {s_{x} ,s_{y} ,z,\omega_{0} } \right)\) at the observation point \((s_{x} ,s_{y} ,z)\). The calculation parameters are specified as follows: \(\varepsilon = 10^{ - 3} m^{2} /s^{3}\), \(\chi_{t} = 10^{ - 7} K^{2} /s,\) \(\varsigma = - 0.5\), \(\Omega = 10\,m^{ - 1}\), \(\lambda = 532\,nm\), \(w_{0} = 2mm\), \(\omega_{0} = 2\pi c/\lambda\), z = 210 m, l = 1, \(s_{y} = 0\), u = 1, v = 1, n = 2 T = 3 fs and C = 2.

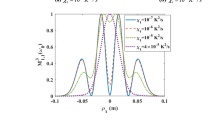
Figure 1 gives the normalized on-axis and off-axis spectral intensity of the pulsed chirped GHCGB for different values of the rate of dissipation of kinetic energy per unit mass of fluid ε. The original spectral intensity is represented by circles. In Fig. 1a, it is evident that the on-axis spectral intensity of the pulsed chirped GHCG beam undergoes a blue shift.

Fig. 1
figure 1

Normalized spectral intensity of pulsed chirped GHCG beam for different values of the rate of dissipation of kinetic energy per unit mass of fluid. a on-axis (\(s_{x}\) = 0) and b off-axis (\(s_{x}\) = 0.15 m)

Full size image

Furthermore, the magnitude of this blue shift increases with decreasing the rate of dissipation of kinetic energy per unit mass of fluid. However, as depicted in Fig. 1b, the off-axis spectral intensity displays a red shift, and with an increase in the rate of dissipation of kinetic energy per unit mass of fluid, this red shift transitions into a blue shift.

The normalized on-axis and off-axis spectral intensities of the pulsed chirped GHCG beam in turbulent oceanic environment are illustrated in Fig. 2, where the circles representing the normalized original spectrum. Figure 2a clearly demonstrates that the normalized on-axis spectrum exhibits a blue shift, and this blue the magnitude of this blue shift increases as the dissipation rate of temperature variance \(\chi_{t}\) decreases.

Fig. 2
figure 2

Normalized spectral intensity of pulsed chirped GHCG beam for different values of the dissipation rate of temperature variance. a on-axis (\(s_{x}\) = 0) and b off-axis (\(s_{x}\) = 0.15 m)

Full size image

In contrast, Fig. 2b reveals that the off-axis spectral intensity undergoes a blue shift, which transforms into a red shift with a decrease in the dissipation rate of temperature variance. For instance, for \(\chi_{T}\) values of \(\chi_{t} = 10^{ - 3} K^{2} /s\), \(\chi_{t} = 10^{ - 7} K^{2} /s\), and \(\chi_{t} = 10^{ - 8} K^{2} /s\), the corresponding spectral shifts are 0.0836, − 0.6455, − 0.7057, respectively.

Figure 3 displays the normalized on-axis and off-axis spectral intensities of the pulsed chirped GHCG beam in the turbulent oceanic environment for different values of the relative intensity of temperature and salinity fluctuations. The circles indicate the normalized original spectrum. In Fig. 3a, it is evident that the normalized on-axis spectrum experiences a blue shift, and the magnitude of this blue shift increases as the relative intensity of temperature and salinity fluctuations increases. On the other hand, Fig. 3b shows that the off-axis spectral intensity undergoes a red shift for all values of \(\varsigma\), which becomes more pronounced as the relative intensity of temperature and salinity fluctuations decreases. For instance, for relative intensity values of \(\varsigma = - 0.5\), \(\varsigma = - 1.5\) and \(\varsigma = - 3.5,\) the corresponding spectral shifts are − 0.1973, − 0.5184, and − 0.5385, respectively.

Fig. 3
figure 3

Normalized spectral intensity of pulsed chirped GHCG beam for different values of the relative intensity of temperature and salinity fluctuations. a on-axis (\(s_{x}\) = 0) and b off-axis (\(s_{x}\) = 0.1 m)

Full size image

Figure 4 shows the normalized on-axis and off-axis spectral intensities of the pulsed chirped GHCG beam for different values of the pulse duration T. The original normalized spectrum is represented by circles in the figures. In Fig. 4a, it is evident that the spectral intensity along the axis of the pulsed chirped GHCG beam exhibits a blue shift, and the magnitude of this blue shift decreases as the pulse duration T increases. Conversely, Fig. 4b shows that the off-axis spectral intensity undergoes a red shift for all values of the pulse duration T, and the red shift becomes more pronounced as the pulse duration T decreases. For example, for \(T = 3\,fs\), \(T = 5\,fs\) and \(T = 12\,fs\), we have \(\delta \omega /\omega_{0}\) = − 0.6455, − 0.2709 and − 0.0301, respectively.

Fig. 4
figure 4

Normalized spectral intensity of pulsed chirped GHCG beam for different values of pulse duration T. a on-axis (\(s_{x}\) = 0) b off-axis (\(s_{x}\) = 0.15 m)

Full size image

These observations suggest that both the on-axis and off-axis spectra tend to approach the original spectrum when the pulse duration T approaches a specific value.

Figure 5 presents the normalized on-axis and off-axis spectral intensities of the pulsed chirped GHCG beam for various values of the chirp parameter C. The original normalized spectrum is indicated by circles in the figures. In Fig. 5a, it is obvious that the spectral intensity along the beam's axis experiences a blue shift, and this blue shift diminishes as the chirp parameter C increases. Conversely, Fig. 5b demonstrates that the off-axis spectral intensity undergoes a red shift for all chirp parameter C values, and the magnitude of this red shift intensifies as the chirp parameter C decreases. These findings suggest that both the on-axis and off-axis spectra converge towards the original spectrum when the chirp parameter C approaches a specific value.

Fig. 5
figure 5

Normalized spectral intensity of pulsed chirped GHCG beam for different values of chirp parameter C a on-axis (\(s_{x}\) = 0) b off-axis (\(s_{x}\) = 0.15 m)

Full size image

4 Conclusion

In conclusion, this study delves into the spectral intensity changes of a pulsed chirped GHCG beam as it travels through a turbulent oceanic medium. Based on the Huygens-Fresnel principle and Fourier Transform method, a formula is derived and numerical simulations are performed to validate the results. The investigation sheds light on the influence of oceanic turbulence parameters and pulse duration on the spectral characteristics of the beam. The results show that the on-axis spectrum is blue-shifted and red-shifted as the transverse distance increases. The influence of medium properties and beam parameters on these spectral shifts at various observation positions is elucidated. The implications of these findings extend to information coding and transmission, holding potential significance for advancements in optical communication systems.