Coupled purely nonlinear oscillators: normal modes and exact solutions for free and forced responses

Abstract

This article is concerned first with free vibrations in a chain of two-mass oscillators with purely nonlinear springs whose power of nonlinearity can be any real number higher than unity. Similar normal modes are obtained by uncoupling the equations of motion. The corresponding time responses are determined in exact analytical forms in terms of Ateb and Jacobi elliptic functions. The external excitation is designed so as to uncouple the equations of motion again and to determine the exact solution for forced vibrations. Then, the case of a three-mass chain is investigated, and similar normal modes are found for the nonlinearity of interest. In addition, these novel results are extended to a continuous system—a rod exhibiting longitudinal vibrations when the material of the rod is characterized by a purely nonlinear stress–strain relationship.

1 Introduction

Coupled oscillators model a variety of systems, ranging from mathematical biology to physics and engineering (see, e.g., [1], and the references cited therein). As a result of this fact, they have attracted considerable interest of researchers during the past several decades. However, emerging micro- and nano-electromechanical systems renewed this interest [2–5]. In order to model these systems in accordance with experimental results, strong nonlinearity with real-valued powers should be taken into consideration [6–9], which makes the existing approaches less adequate or inadequate. This is related especially to the possibility to determine exact solutions for their free and forced response.

Fig. 1

a Coupled two-mass system in Case I; b coupled two-mass system in Case II

It was Rosenberg who defined the concept of the “exact steady state” of a strongly nonlinear, undamped, discrete system [10, 11]: the ratio of the response and the amplitude in the steady-state forced response of a single degree of freedom is “cosine-like” [11] and of the same period as the periodic forcing function. Hsu [12] considered Duffing-type oscillators and used the fact that these undamped oscillators have exact closed-form solutions in terms of Jacobi elliptic functions. Hsu designed the external excitation to be proportional to the forced displacement and, thus, expressed it also in terms of Jacobi elliptic functions. This idea has recently been extended to some other undamped nonlinear oscillators with cubic or quadratic nonlinearities that have exact closed-form solutions [13]. Caughey and Vakakis [14] examined two-degrees-of-freedom strongly nonlinear systems, where the power of nonlinearity is an odd integer. To find the exact forced response, they expressed the forcing as a certain power function of the forced displacement. It should be pointed out that this study was concerned with homogenous systems (nonlinearizable, essentially nonlinear), with stiffness nonlinearities proportional to the same power of displacement. Rosenberg [10] found that a system with a homogeneous potential function possesses similar normal mode oscillations, i.e., normal modes with straight-line trajectories in the configuration space. The existence of at least n similar normal modes in n -degree-of-freedom homogeneous systems was proven by van Groesen [15]. The number of similar normal modes, associated bifurcations, and stability were addressed in [16–19] (other references can also be found in [19]).

Similar normal modes of two-mass chains of purely nonlinear oscillators are investigated in this study, but the power of nonlinearity is assumed to be any real number higher than unity, including non-integer and integer numbers. Exact solutions for the corresponding responses are obtained for free and then also for forced vibrations with a specially designed external excitation, which represent new results. Then, a three-mass chain with purely nonlinear springs is considered, and the corresponding similar normal modes are found and illustrated. These results are subsequently generalized to continuous systems, i.e., an elastic rod exhibiting longitudinal vibrations, which is also a novel result.

2 Two-mass chain

2.1 Free vibrations

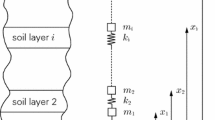
The system of coupled oscillators with two masses ($N=2$) shown in Fig. 1a, where all the springs are purely nonlinear, is governed by

$$\begin{aligned} \ddot{x}+K_{1}~x\left| x\right| ^{\alpha -1}+K_{2}~\left( x-y\right) \left| x-y\right| ^{\alpha -1}= & {} 0, \end{aligned}$$

(1)

$$\begin{aligned} \ddot{y}+K_{3}~y\left| y\right| ^{\alpha -1}+K_{2}~\left( y-x\right) \left| y-x\right| ^{\alpha -1}= & {} 0, \end{aligned}$$

(2)

where $K_{1},$ $K_{2}$, and $K_{3}$ are constants, while $\alpha$ is assumed to be a real positive number higher than unity (note that this includes both integer and non-integer numbers). The absolute value function is used to make the restoring forces be an odd function for all the values of $\alpha$ defined. The two masses are assumed to be equal to the unit mass, but in case this does not hold, an appropriate normalization process can be carried out.

Similar normal modes [10] for the system of equations (1), (2) can exist if x(t) and y(t) are related to each other as follows:

$$\begin{aligned} y=Cx, \end{aligned}$$

(3)

where C is a constant parameter to be determined. This yields:

$$\begin{aligned}&\ddot{x}+\left[ K_{1}+K_{2}\left( 1-C\right) \left| 1-C\right| ^{\alpha -1}\right] ~x\left| x\right| ^{\alpha -1} =0, \end{aligned}$$

(4)

$$\begin{aligned}&\ddot{x}+\left[ K_{3}\left| C\right| ^{\alpha -1}+K_{2}\frac{C-1}{C}\left| C-1\right| ^{\alpha -1}\right] ~x\left| x\right| ^{\alpha -1} =0.\nonumber \\ \end{aligned}$$

(5)

The problem can be simplified to a one-degree-of-freedom system

$$\begin{aligned} \ddot{x}+k_{\alpha }~x\left| x\right| ^{\alpha -1}=0,\ \end{aligned}$$

(6)

with $k_{\alpha }$ being:

$$\begin{aligned} k_{\alpha }=K_{1}+K_{2}\left( 1-C\right) \left| 1-C\right| ^{\alpha -1}, \end{aligned}$$

(7)

provided that the coefficients in front of the nonlinear terms in Eqs. (4) and (5) are equal, which leads to:

$$\begin{aligned} K_{1}+K_{2}\left| 1-C\right| ^{\alpha -1}\left( 1-C\right) \frac{1+C}{C}-K_{3}\left| C\right| ^{\alpha -1}=0. \end{aligned}$$

(8)

The form given by Eq. (6) is convenient as it has an exact, closed-form solution. Lyapunov [20] seems to have been the first one to construct a general solution of Eq. (6), but he considered odd integer powers only, introducing new Cs and Sn functions as the generalizations of the cosine and sine functions. Rosenberg [21], however, considered Eq. (6) with $\alpha$ being any positive real number and constructed Ateb functions as inversions of the half of the incomplete Beta function, naming them the cam and sam functions. His approach was further developed by Senik [22, 23], who expressed these solutions as the three-argument ca and sa functions. This notation is used herein. Thus, the solution of Eq. (6) can be expressed in the form

$$\begin{aligned} ~x=A\text { ca }\left( \alpha ,1,\left| A\right| ^{\left( \alpha -1\right) /2}\sqrt{\frac{k_{\alpha }\left( \alpha +1\right) }{2}}t\right) , \end{aligned}$$

(9)

where A is the amplitude of vibrations. The first argument in the ca solution (9) is the power of nonlinearity $\alpha$, the second argument is always unity [23], while the coefficient in front of t in the third argument represents the frequency of the ca function: $\omega _{\text {ca}}=\left| A\right| ^{\left( \alpha -1\right) /2}\sqrt{k_{\alpha }\left( \alpha +1\right) /2}$.

Fig. 2

Balancing diagrams $C-K$ for a fixed value of the power $\alpha$ and different values of the ratio $K=K_{2}/K_{1}$ in Case I for: a $\alpha =1$; b $\alpha =5/3$; c $\alpha =3$; d $\alpha =5$; e $\alpha =19/2$; f $\alpha =9$

In addition, by considering the first integral of equation Eq. (6), one can derive the following expression for the period of vibrations

$$\begin{aligned} T_{\text {ex }}&= \frac{4B\left( \frac{1}{\alpha +1},\frac{1}{2}\right) }{\sqrt{2k_{\alpha }\left( \alpha +1\right) }}\left| A\right| ^{\left( 1-\alpha \right) /2} \nonumber \\&= \sqrt{\frac{8\pi }{k_{\alpha }\left( \alpha +1\right) }}\frac{\Gamma \left( \frac{1}{\alpha +1}\right) }{\Gamma \left( \frac{\alpha +3}{2\left( \alpha +1\right) }\right) }\left| A\right| ^{\left( 1-\alpha \right) /2}, \end{aligned}$$

(10)

where B is the complete Beta function and $\Gamma$ is the Euler Gamma function.

Note that the expression for the frequency $\omega _{\text {ca}}$ is the consequence of the fact that the Ateb function ca$\left( \alpha ,1,z\right)$ has the exact period $T_{\text {ca }\left( \alpha ,1,z\right) }=2B\left( \frac{1}{\alpha +1},\frac{1}{2}\right)$ [21], so that one can use $\omega _{\text {ca}}=T_{\text {ca }\left( \alpha ,1,z\right) }/T_{\text {ex}}$ and obtain $\omega _{\text {ca}}$ as given above.

Further, for the pure cubic nonlinearity $\alpha =3$, the Ateb ca function turns into the Jacobi cn function, and the solution has the form [24]

$$\begin{aligned} ~x= & {} A\text { cn }\left( \sqrt{k_{3}}At\left| \frac{1}{2}\right. \right) , \end{aligned}$$

(11)

$$\begin{aligned} k_{3}= & {} K_{1}+K_{2}\left( 1-C\right) ^{3}. \end{aligned}$$

(12)

The frequency of the cn function is the coefficient in front of t in the first argument $\omega _{\text {cn}}=\sqrt{k_{3}}A$, while the second argument is the elliptic parameter $m=1/2$. The frequency $\omega _{\text {cn}}$ was obtained from the fact that the cn function cn $\left( z\left| \frac{1}{2}\right. \right)$ has the exact period $T_{\text {cn }\left( z\left| \frac{1}{2}\right. \right) }=4K\left( \frac{1}{2}\right)$, where K stands for the complete elliptic integral of the first kind. Consequently, one can derive (after some transformations): $\omega _{\text {cn}}=T_{\text {cn }\left( z\left| \frac{1}{2}\right. \right) }/T_{\text {ex}}$ =$\sqrt{k_{3}}A$.

When $\alpha =1$, one has $x=A\cos$ $\left( \sqrt{k_{1}}t\right)$, $k_{1}=K_{1}+K_{2}\left( 1-C\right)$, and $T_{\text {ex}}=2\pi /\sqrt{k_{1}}$ (all the solutions mentioned hold for the initial conditions $x\left( 0\right) =A$, $\dot{x}\left( 0\right) =0$ as they are of importance for the subsequent investigations).

In order to determine the solutions for all $\alpha$ and detect how they differ from the one corresponding to $\alpha =1$, one should calculate the values of C, and this is done herein for two cases: Case I refers to the equal stiffness coefficients of the anchoring springs 1 and 3 (Fig. 1a), while Case II is related to the system without the spring connecting the second mass with the base/wall on the right-hand side (Fig. 1b).

2.1.1 Case I: Equal stiffness coefficients of anchoring springs

If $K_{3}=K_{1}$, the condition given by Eq. (8) leads to

$$\begin{aligned} 1+\frac{K_{2}}{K_{1}}\left| 1-C\right| ^{\alpha -1}\left( 1-C\right) \frac{1+C}{C}-\left| C\right| ^{\alpha -1}=0. \end{aligned}$$

(13)

This expression is used to plot the diagrams shown in Fig. 2, which depict how the value of C changes with the ratio $K=K_{2}/K_{1}$ for different values of the power $\alpha$. As each of these diagrams represents the values of C that balance purely nonlinear term in Eqs. (4) and (5), they will be denoted as “balancing diagrams” (this terminology is taken from [14, 25]).

In case of the linear springs $\alpha =1$, two values of C exist: $C=1$ and $C=-1$. The former corresponds to the case when the masses oscillate with the same amplitude and in the same direction as they were connected with a rigid link. The latter corresponds to the case when they oscillate with the same amplitude but in the opposite directions. It is easy to see from Eq. (13) that the values $C=\pm 1$ exist also for all $\alpha$. However, for $\alpha \in (1,5]$, a subcritical pitchfork bifurcation occurs at the value $K^{*}$(Fig. 2b–d), as a result of which two additional modes bifurcate from $C=-1$, and both of them correspond to a negative C (note that the one corresponding to $C=-1$ is unstable here). For $K>$ $K^{*}$, the modes are the same as in the linear system and the mode corresponding to $C=-1$ is stable now (stable solutions are depicted by solid lines and unstable solutions by dashed lines; the stability analyses are carried out by using the methodology and results from [25], but all the details are omitted here). Other modes existing for higher values of the power of nonlinearity $\alpha$ are presented in Fig. 2e, f, where the stable and unstable solutions are also indicated.

Fig. 3

a Bifurcation point $K^{*}$ as a function of the power $\alpha$ in Case I; b Curves corresponding to $K^{*}$ and $K^{*}{}^{*}$ meeting at a cusp

In order to investigate the characteristics of these bifurcations, the value of $K^{*}$ is examined first. To that end, one can use Eq. (13) to express K and calculate

$$\begin{aligned} K^{*}=\underset{C->-1}{\lim }\frac{\left| C\right| ^{\alpha -1}-1}{\vert 1-C\vert ^{\alpha -1}( 1-C) ( 1+C) }C=\frac{\alpha -1}{2^{\alpha }}. \end{aligned}$$

(14)

This expression is plotted in Fig. 3a. It is interesting to note that the value of $K^{*}=1/4$ corresponding to $\alpha =2$ and $\alpha =3$ is the same. In addition, the parameter values corresponding to the maximum are

$$\begin{aligned}&\bar{\alpha }=\frac{1+\text {log}2}{\text {log}2}=2.4427,\nonumber \\&\bar{K}^{*}=\frac{1}{e\text { log}4}=0.265369. \end{aligned}$$

(15)

When $\alpha <\bar{\alpha }$, the value of $K^{*}$ increases with $\alpha$; if $\alpha >\bar{\alpha }$, the higher the value of $\alpha$, the lower value of $K^{*}$.

Fig. 4

Time history diagrams for x (solid line) and y (dotted line) corresponding to $K=K_{2}=0.2$, $K_{1}=1, A=1$ in Case I for: a $\alpha =5/3$, $C=-0.6308$; b $\alpha =5/3$, $C=-1.5851$; c $\alpha =3$, $C=-0.3819$; d $\alpha =7/2$, $C=-2.6180$

Fig. 5

Balancing diagrams $C-K$ for a fixed value of the power $\alpha$ and different values of the ratio $K=K_{2}/K_{1}$ in Case II: a $\alpha =1$; b $\alpha =5$; c $\alpha =7$; d $\alpha =17/2$

Fig. 6

a Enlarged balancing diagrams $C-K$ diagram for $\alpha =9$; b bifurcation values of K as a function of $\alpha$; c bifurcation values of C as a function of $\alpha$

For the type of bifurcation diagrams shown in Fig. 2e,f, a cusp where two curves corresponding to $K^{*}$ and $K^{*}{}^{*}\hbox {meet}$ is presented in Fig. 3b.

The exact solutions for motion can now be obtained by using the expression given in Sect. 2.1. For example, taking $\alpha =5/3$, $K=K_{2}=0.2$, $K_{1}=1$, two bifurcating values of C (see Fig. 3b) are calculated from Eq. (13) : $C=-0.6308$ and $C=-1.5851$. Equation (9) now gives

$$\begin{aligned} ~x=A\text { ca }\left( \frac{5}{3},1,\vert A\vert ^{1/3}\sqrt{\frac{4}{3}( 1+0.2( 1-C) \vert 1-C\vert ^{2/3}) }t\right) . \end{aligned}$$

(16)

The solution for y is defined by Eq. (3). The resulting responses are presented in Fig. 4a, b for both modes and for $A=1$. To provide a further insight into the physical meaning of this solution, one can use the Fourier series expansion from the “Appendix” to find out, for example, that the solution (16) corresponding to Fig. 4a consists of the following harmonics:

$$\begin{aligned} ~x&\approx 0.982522\text {cos }\left( 1.13364t\right) \nonumber \\&\quad +0.0182686\text {cos }\left( 3.40092t\right) \nonumber \\&\quad -\,0.000939578\text {cos }\left( 5.66819t\right) \nonumber \\&\quad +\,0.000190823\text {cos }\left( 7.93547t\right) \nonumber \\&\quad -\,0.0000583094\text {cos }\left( 10.2027t\right) . \end{aligned}$$

(17)

Fig. 7

Time history diagrams for x (solid line) and y (dotted line) corresponding to $\alpha =9$, $K=K_{2}$=0.04, $K_{1}$=1, $A=1$ in Case I for: a $C=-0.0668$; b $C=-0.9395$

In the second example, it is assumed that $\alpha =3$, $K=K_{2}=0.2$, $K_{1}=1$. Equation (13) gives $C=-0.3819$ and $C=-2.6180$. Equation (11) now becomes

$$\begin{aligned} ~x=A\text { cn }\left( \sqrt{1+0.2\left( 1-C\right) ^{3}}At\left| \frac{1}{2}\right. \right) . \end{aligned}$$

(18)

The corresponding time history diagrams for x and y are shown in Fig. 4c,d for both modes and for $A=1$. The Fourier series expansion from the “Appendix” can be used to present the solution (18) corresponding to Fig. 4c as follows

$$\begin{aligned} ~x&\approx 0.955006\text {cos }\left( 1.04721t\right) \nonumber \\&\quad +0.0430495\text {cos }\left( 3.14164t\right) \nonumber \\&\quad +\,0.0018605\text {cos }\left( 5.23606t\right) \nonumber \\&\quad +\,0.0000804069\text {cos }\left( 7.33049t\right) \nonumber \\&\quad +\,3.4794\times 10^{-6}\text {cos }\left( 9.42492t\right) . \end{aligned}$$

(19)

2.1.2 Case II: No right anchoring spring

If the right-hand side spring is removed (Fig. 1b), the equations of motion have the form

$$\begin{aligned} \ddot{x}+K_{1}~x\left| x\right| ^{\alpha -1}+K_{2}~\left( x-y\right) \left| x-y\right| ^{\alpha -1}= & {} 0, \end{aligned}$$

(20)

$$\begin{aligned} \ddot{y}+K_{2}~\left( y-x\right) \left| y-x\right| ^{\alpha -1}= & {} 0, \end{aligned}$$

(21)

which together with Eq. (3) yield the following condition:

$$\begin{aligned} 1+\frac{K_{2}}{K_{1}}\left| 1-C\right| ^{\alpha -1}\left( 1-C\right) \frac{1+C}{C}=0. \end{aligned}$$

(22)

This expression is used to plot the $K-C$ balancing diagrams in Fig. 5a–d, where again $K=K_{2}/K_{1}$. There are several distinctive and different features with respect to Case I. Namely, in Case II there is no fixed value of C, but it changes both with K and $\alpha$. The type of bifurcation occurring is also different. As shown in Fig. 5c,d, a saddle-node bifurcation appears for higher values of $\alpha$ in a certain narrow range of K.

The bifurcation points are defined by

$$\begin{aligned} \hat{K}= & {} -\frac{2^{\alpha }\left( \alpha -1+\Lambda \right) }{\left( -1-\alpha +\Lambda \right) }\left( \frac{\alpha }{-1+3\alpha +\Lambda }\right) ^{\alpha }, \end{aligned}$$

(23)

$$\begin{aligned} \check{K}= & {} \frac{2^{\alpha }\left( \alpha -1-\Lambda \right) }{\left( 1+\alpha +\Lambda \right) }\left( -\frac{\alpha }{1-3\alpha +\Lambda }\right) ^{\alpha }, \end{aligned}$$

(24)

where

$$\begin{aligned} \Lambda =\sqrt{\alpha ^{2}-6\alpha +1}. \end{aligned}$$

(25)

They are depicted in Fig. 6a, together with the following associated values

$$\begin{aligned} \hat{C}= & {} \frac{1-\alpha -\Lambda }{2\alpha }, \end{aligned}$$

(26)

$$\begin{aligned} \check{C}= & {} \frac{1-\alpha +\Lambda }{2\alpha }. \end{aligned}$$

(27)

They exist for $\Lambda >0$, i.e., $\alpha >3+2\sqrt{2}$ $\left( \alpha >5.82843\right)$, which corresponds to $\bar{K}=2^{-2-\sqrt{2}}=$ 0.0938036. These two values are labeled in Fig. 6b, which shows two curves meeting at the cusp in terms of the power $\alpha$. The way how $\hat{C}$ and $\check{C}$ change with $\alpha$ is presented in Fig. 6c, where it is also noted that $\bar{C}=1-\sqrt{2}=-$0.414214.

To illustrate how the exact solution for motion can be obtained, it is assumed that $\alpha$=9, K=$K_{2}$=0.04, $K_{1}$=1. Two bifurcating values of C (see Fig. 6a) are calculated from Eq. (22) : $C=-0.0668$ and $C=-0.9395$. Equation (9) now gives

$$\begin{aligned} ~A\text { ca }\left( 9,1,A^{4}\sqrt{5\left( 1+0.04\left( 1-C\right) ^{9}\right) }t\right) . \end{aligned}$$

(28)

Figure 7 contains this response for $A=1$ as well as the one corresponding to y(t). One can use the Fourier series expansion from the “Appendix” to find out that the solution (28) corresponding to Fig. 7a can be represented as the sum of the following harmonics

$$\begin{aligned} ~x&\approx 0.893115\cos \left( 0.642219t\right) \nonumber \\&\quad +\,0.0800142\cos \left( 1.92666t\right) \nonumber \\&\quad +\,0.0190361\cos \left( 3.21109t\right) \nonumber \\&\quad +\, 0.00544285\cos \left( 4.49553t\right) \nonumber \\&\quad +\,0.00164111\cos \left( 5.77997t\right) . \end{aligned}$$

(29)

2.2 Forced vibrations

This section is concerned with the system of coupled oscillators from Fig. 1a, where the first mass is excited by the forcing f, so that the equations of motion have the form:

$$\begin{aligned} \ddot{x}+K_{1}~x\left| x\right| ^{\alpha -1}+K_{2}~\left( x-y\right) \left| x-y\right| ^{\alpha -1}= & {} f, \end{aligned}$$

(30)

$$\begin{aligned} \ddot{y}+K_{3}~y\left| y\right| ^{\alpha -1}+K_{2}~\left( y-x\right) \left| y-x\right| ^{\alpha -1}= & {} 0. \end{aligned}$$

(31)

In order to find the forced response in an exact form, the expression for the external excitation is designed in a special way: it is assumed in the form related to the form of the purely nonlinear restoring force as follows

$$\begin{aligned} f=\frac{F_{0}}{A}x\left| \frac{F_{0}}{A}x\right| ^{\alpha -1}, \end{aligned}$$

(32)

where $F_{0}$ is constant. Now, assuming also that Eq. (3) holds, the excitation (32) becomes the part of the stiffness coefficient in front of the nonlinear term and the equations of motion become uncoupled:

$$\begin{aligned}&\ddot{x}+\left[ K_{1}+K_{2}\left( 1-C\right) \left| 1-C\right| ^{\alpha -1}\right. \nonumber \\&\left. -\frac{F_{0}}{A}\left| \frac{F_{0}}{A}\right| ^{\alpha -1}\right] ~x\left| x\right| ^{\alpha -1} =0, \end{aligned}$$

(33)

$$\begin{aligned}&\ddot{x}+\left[ K_{3}\vert C\vert ^{\alpha -1}+K_{2}\frac{C-1}{C}\vert C-1\vert ^{\alpha -1}\right] ~x\left| x\right| ^{\alpha -1} =0.\nonumber \\ \end{aligned}$$

(34)

Proceeding as previously, i.e., equating the coefficients in front of the nonlinear terms, one derives

$$\begin{aligned}&K_{1}+K_{2}\vert 1-C\vert ^{\alpha -1}( 1-C) \frac{1+C}{C}-K_{3}\vert C\vert ^{\alpha -1}\nonumber \\&\quad =\frac{F_{0}}{A}\left| \frac{F_{0}}{A}\right| ^{\alpha -1}. \end{aligned}$$

(35)

One can use this expression to investigate how the ratios $F_{0}/A$ and stiffness coefficients influence the value of C for a fixed value of $\alpha$. This is done separately for both cases considered in the previous section.

Fig. 8

Balancing diagrams $C-K$ diagrams for forced vibrations in Case I for $A=1$ and: a $\alpha =1$; b $\alpha =5/3$; c $\alpha =3$; d $\alpha =5$ (thin line: $F_{0}$=0, dashed line: $F_{0}=0.4$, dotted line $F_{0}=-0.4$)

Fig. 9

Diagrams for $x\left( t\right)$ (blue solid line), $y\left( t\right)$ (dotted line), $f\left( t\right)$ (green solid line) corresponding to Fig. 8b, $\alpha =5/3$, $K=K_{2}=0.2, K_{1} =1, A=1$ in Case I for: a $C=-2.84836$; b $C=0.273026$. (Color figure online)

Fig. 10

Balancing diagrams $C-K$ diagrams for forced vibrations in Case II for $A=1$ and: a $\alpha =1$; b $\alpha =5$; c $\alpha =7$; d $\alpha =17/2$ (thin line $F_{0}=0$, dashed line $F_{0}=0.4$, dotted line $F_{0}=-0.4$)

Note also that now the solution for x is defined by

$$\begin{aligned}&\ddot{x}+k_{\alpha ,F}~x\left| x\right| ^{\alpha -1} =0,\ \end{aligned}$$

(36)

$$\begin{aligned}&k_{\alpha ,F} =K_{1}+K_{2}( 1-C) \vert 1-C\vert ^{\alpha -1}-\frac{F_{0}}{A}\left| \frac{F_{0}}{A}\right| ^{\alpha -1},\nonumber \\ \end{aligned}$$

(37)

but it can easily be related to the form given by Eqs. (6) and (9). The expression for the force follows then from Eq. (32).

2.2.1 Case I: Equal stiffness coefficients of anchoring springs

Equation (35) is used to plot Fig. 8 for the forced response as an extension of the one shown in Fig. 4a–d for the same parameter values, two different values of the excitation force and $A=1$. As it is seen, the branches in some regions tend to those corresponding to the free response, which are plotted as thin solid line and represent their backbone curves.

To illustrate how x, y, and the force f change in time, Fig. 9 is created for $\alpha =5/3$, $K=0.2,A=1$ and two negative values of C. The force is in phase with x in Fig. 9a, and in phase with y in Fig. 9b.

Note that frequency–response curves are not shown here, but can be obtained by using Eq. (35) in association with the relationship for the frequency $\omega =2\pi /T_{\text {ex}}$, where $T_{\text {ex}}$ is defined by Eq. (10). Given the variety of their forms and characteristics, this will be done in a separate publication.

2.2.2 Case II: No right anchoring spring

Equation (35) is now used to create Fig. 10 for the forced response in Case II as an extension of the one shown in Fig. 4e,f for the same parameter values, two values of the excitation force and $A=1$. Unlike the linear case, for nonlinear cases the curves of a forced system are very close to those corresponding to the free system.

3 Generalizations

3.1 Three-mass chain

If the system consists of three masses ($N=3$) attached mutually as well as with the base with purely nonlinear springs of equal stiffness coefficients, the equations of motion are

$$\begin{aligned}&\ddot{x}+K~x\left| x\right| ^{\alpha -1}+K~\left( x-y\right) \left| x-y\right| ^{\alpha -1} =0, \end{aligned}$$

(38)

$$\begin{aligned}&\ddot{y}+K~\left( y-x\right) \left| y-x\right| ^{\alpha -1}\nonumber \\&\quad +K~\left( y-z\right) \left| y-z\right| ^{\alpha -1} =0, \end{aligned}$$

(39)

$$\begin{aligned}&\ddot{z}+K~z\left| z\right| ^{\alpha -1}+K~\left( z-y\right) \left| z-y\right| ^{\alpha -1} =0, \end{aligned}$$

(40)

where K is constant and $\alpha \ge 1$. Of interest is again to find similar normal modes, i.e., the solutions satisfying Eq. (3) and

$$\begin{aligned} z=D\text { }x, \end{aligned}$$

(41)

where C and D are now constant parameters to be calculated. This yields:

$$\begin{aligned}&\ddot{x}+K\left[ 1+\left( 1-C\right) \left| 1-C\right| ^{\alpha -1}\right] ~x\left| x\right| ^{\alpha -1} =0, \end{aligned}$$

(42)

$$\begin{aligned}&\ddot{x}+K\left[ \frac{C-1}{C}\left| C-1\right| ^{\alpha -1}\right. \nonumber \\&\left. \quad +\frac{C-D}{C}\left| C-D\right| ^{\alpha -1}\right] ~x\left| x\right| ^{\alpha -1} =0, \end{aligned}$$

(43)

$$\begin{aligned}&\ddot{x}+K\left[ \left| D\right| ^{\alpha -1}+\frac{D-C}{D}\left| D-C\right| ^{\alpha -1}\right] ~x\left| x\right| ^{\alpha -1} =0.\nonumber \\ \end{aligned}$$

(44)

To simplify this to a one-degree-of-freedom system, the following system of equation needs to be satisfied:

$$\begin{aligned} 1+\left( 1-C\right) \left| 1-C\right| ^{\alpha -1}= & {} \frac{C-1}{C}\left| C-1\right| ^{\alpha -1}\nonumber \\&+\frac{C-D}{C}\left| C-D\right| ^{\alpha -1}, \end{aligned}$$

(45)

$$\begin{aligned} 1+\left( 1-C\right) \left| 1-C\right| ^{\alpha -1}= & {} \left| D\right| ^{\alpha -1}\nonumber \\&+\frac{D-C}{D}\left| D-C\right| ^{\alpha -1}.\nonumber \\ \end{aligned}$$

(46)

Solving it for a fixed $\alpha$, one can obtain the values of C and D, and then calculate the corresponding frequency $\omega =2\pi /T_{\text {ex}}$, where $T_{\text {ex}}$ is defined by Eq. (10), and $k_{\alpha }$ is obtained from Eq. (7) with $K_{1}=K_{2}=K$. These results are presented in Table 1 for $K=1$. They are listed with respect to the increasing $\omega$. Every pair (C, D) and the corresponding $\omega$ have the mode number/modal index n associated with them.

Fig. 11

Modal frequencies $\omega$ versus mode number n for different values of the power of nonlinearity (blue line with circles $\alpha =1$; magenta line with squares $\alpha =5/3$; yellow line with diamonds $\alpha =3$; green line with triangles $\alpha =5$). (Color figure online)

Fig. 12

Modal responses of a three-mass chain of oscillators for $K=1$, $A=1$ and: a $\alpha =5/3$, b $\alpha =3$

Table 1 Values of C and D obtained from Eqs. (45) and (46), the corresponding modal frequency $\omega$ obtained from Eqs. (10) and (7) with $K_{1}=K_{2}=K=1$; n is the mode number/modal index associated with each of them

These results are also plotted in Fig. 11 for $A=1$ and for all the values of $\alpha$ from Table 1 as well as for $\alpha =5$ (due to a large value, one solution for $\alpha =5$, which is $\omega =$3168.06, is not shown). The mode number/modal index n is shown on the horizontal axis and the associated frequency $\omega$ on the vertical axis.

3.2 Case: $N\rightarrow \infty$

In order to examine the case of a continuous system—a rod exhibiting longitudinal vibrations—one can use the previously derived results letting the number of masses tend to infinity $\left( N\rightarrow \infty \right)$. For that purpose, we focus on Eq. (39), which can be used for an arbitrary mass in the chain, but change the notation. First, we will introduce the mass M and use $y\equiv u=u\left( x_{i}\right)$ (u is the displacement of the $i\hbox {th}$ mass and $x_{i}$ is the equilibrium position of the $i\hbox {th}$ mass), $z\equiv u\left( x_{i}+\Delta x_{i}\right)$ with $\Delta x_{i}$ being the equal spacing between the equilibrium positions of all the masses, $x\equiv u\left( x_{i}-\Delta x_{i}\right)$. This gives:

$$\begin{aligned} M\frac{\partial ^{2}u\left( x_{i}\right) }{\partial t^{2}}= & {} K~\left( u\left( x_{i}+\Delta x_{i}\right) \right. \nonumber \\&\left. -\,u\left( x_{i}\right) \right) \left| u\left( x_{i}+\Delta x_{i}\right) -u\left( x_{i}\right) \right| ^{\alpha -1} \nonumber \\&-\,K~\left( u\left( x_{i}\right) -u\left( x_{i}-\Delta x_{i}\right) \right) \left| u\left( x_{i}\right) \right. \nonumber \\&\left. -\,u\left( x_{i}-\Delta x_{i}\right) \right| ^{\alpha -1}. \end{aligned}$$

(47)

Now, by dividing the equation with $\Delta x_{i}$ and dropping the subscript i, one has

$$\begin{aligned}&\frac{M}{\Delta x}\frac{\partial ^{2}u\left( x\right) }{\partial t^{2}}\nonumber \\&\quad =K~\left\{ \frac{( u( x+\Delta x) {-}u( x) ) \vert u( x+\Delta x) {-}u( x) \vert ^{\alpha -1}}{\Delta x}\right. \nonumber \\&\quad \left. -~\frac{( u( x) -u( x-\Delta x) ) \vert u( x) -u( x-\Delta x) \vert ^{\alpha -1}}{\Delta x}\right\} .\nonumber \\ \end{aligned}$$

(48)

Considering the case $\alpha =1$, it is easy to recognize that letting $\Delta x\rightarrow$ 0 (which is the case when $N\rightarrow \infty$), the ratios on the right-hand side represent the first derivative with respect to x:

$$\begin{aligned} \frac{M}{\Delta x}\frac{\partial ^{2}u}{\partial t^{2}}=K~\Delta x\frac{u^{\prime }\left( x\right) -~u^{\prime }\left( x-\Delta x\right) }{\Delta x}, \end{aligned}$$

(49)

where the primes denote spatial derivatives. For $M/\Delta x=\rho$ and $K~\Delta x=E$, this further simplifies to

$$\begin{aligned} \rho \frac{\partial ^{2}u}{\partial t^{2}}=E\frac{\partial ^{2}u}{\partial x^{2}}, \end{aligned}$$

(50)

which corresponds to the governing equation for longitudinal vibrations as well as for a wave equation with $E/\rho =c^{2}.$

Following the analogous procedure, the right-hand side (RHS) of Eq. (48) in a general case can be written down as

$$\begin{aligned}&\text {RHS }(\text {Eq. }(48))\nonumber \\&\quad =K\Delta x\left| \Delta x\right| ^{\alpha -1}~\left\{ \frac{\frac{\left( u\left( x+\Delta x\right) -u\left( x\right) \right) }{\Delta x}\left| \frac{u\left( x+\Delta x\right) -u\left( x\right) }{\Delta x}\right| ^{\alpha -1}}{\Delta x}-~\frac{\frac{\left( u\left( x\right) -u\left( x-\Delta x\right) \right) }{\Delta x}\left| \frac{u\left( x\right) -u\left( x-\Delta x\right) }{\Delta x}\right| ^{\alpha -1}}{\Delta x}\right\} \nonumber \\&\quad =K\Delta x\left| \Delta x\right| ^{\alpha -1}\frac{\partial }{\partial x}\left[ \left( \frac{\partial u}{\partial x}\right) \left| \frac{\partial u}{\partial x}\right| ^{\alpha -1}\right] \nonumber \\&\quad =\alpha K\Delta x\left| \Delta x\right| ^{\alpha -1}\frac{\partial ^{2}u}{\partial x^{2}}\left| \frac{\partial u}{\partial x}\right| ^{\alpha -1}. \end{aligned}$$

(51)

Equation (48) now becomes:

$$\begin{aligned} \rho \frac{\partial ^{2}u}{\partial t^{2}}=\alpha E\frac{\partial ^{2}u}{\partial x^{2}}\left| \frac{\partial u}{\partial x}\right| ^{\alpha -1}, \end{aligned}$$

(52)

with $E=K\Delta x\left| \Delta x\right| ^{\alpha -1}$. This generalizes Eq. (50) to a nonlinear case when the rod is made of the material characterized by a nonlinear stress–strain relationship (some examples of the systems/materials for which this relationship is nonlinear can be found, for instance, in [26]).

In order to obtain an approximate representation of the corresponding modes, one can use the results obtained previously for the three-mass chain, presented in Table 1. The maximal displacements are calculated for $A=1$ and rotated for $90^{\text {o}}$ clockwise. The results obtained are shown for $\alpha =5/3$ in Fig. 12a and for $\alpha =3$ in Fig. 12b (these displacements are plotted by using the same scale to make them be comparable). While the results shown in Fig. 12a resemble the well-known modes corresponding to the linear system, those in Fig. 12b illustrate the appearance of two additional asymmetric modes, the existence of which is also seen in Fig. 11. Future research will be directed toward obtaining the modes corresponding to Eq. (52) exactly.

4 Conclusions

This study has been concerned with a certain class of chains of oscillators. The main characteristic is the type of springs that link them mutually and with the base: these springs are purely nonlinear, and the corresponding power of nonlinearity is assumed to be any real number higher than unity. First, similar normal modes in the free response have been considered in two configurations: when the chain is linked with the base at the left-hand and right-hand side (Case I), and when the chain is linked with the base at the left-hand side only (Case II). It has been shown how these normal modes and the corresponding balancing diagrams depend on the power of nonlinearity and the ratio between the stiffness coefficients. The associated bifurcations have been investigated in detail. It has been demonstrated how to determine the exact solutions, and the corresponding time history diagrams. The associated Fourier series have been given for illustration. The procedure that involves uncoupling equations of motion has also been extended to forced vibrations. It has been shown how to design the external excitation to determine the exact forced response. Then, chains consisting of three masses have been examined, and similar normal modes have been found as well. Finally, these novel results have been extended to a continuous system—a rod exhibiting longitudinal vibrations when the material of the rod is characterized by a purely nonlinear stress–strain relationship. Its modal responses have been linked with the modal responses of the three-mass chain of oscillators with purely nonlinear springs.

Appendix: On some Fourier series expansions

The function $g_{1}\left( \alpha ,t\right) =\hbox {ca}\left( \alpha ,1,t\right)$, where $\alpha \geqslant 1$, has the following Fourier series

$$\begin{aligned} \text {ca }\left( \alpha ,1,t\right) =\overset{\infty }{\underset{n=1}{\sum }}a_{2n-1}\cos \left[ \left( 2n-1\right) \frac{2\pi }{T}\right] , \end{aligned}$$

(53)

where the Fourier coefficients are defined by

$$\begin{aligned} a_{2n-1}\left( \alpha \right) =\frac{8}{\pi }\int _{0}^{T_{\text {ex}}/4}g_{1}\left( \alpha ,t\right) \cos \left[ \left( 2n-1\right) \frac{2\pi }{T}\right] , \end{aligned}$$

(54)

where T is defined by

$$\begin{aligned} T=\sqrt{\frac{8\pi }{\alpha +1}}\frac{\Gamma \left( \frac{1}{\alpha +1}\right) }{\Gamma \left( \frac{\alpha +3}{2\left( \alpha +1\right) }\right) }\left| A\right| ^{\left( 1-\alpha \right) /2}, \end{aligned}$$

(55)

and where A is the amplitude of oscillations and $\Gamma$ is the Euler Gamma function.

It has been derived recently [27] that the values of the Fourier coefficients $a_{2N-1}$ can be calculated by carrying out numerical integration from the expression

$$\begin{aligned} a_{2N-1}= & {} \frac{2\Gamma \left( \frac{\alpha +3}{2\left( \alpha +1\right) }\right) }{\sqrt{\pi }\Gamma \left( \frac{1}{\alpha +1}\right) }\int _{0}^{1}\frac{(1-z)^{\left( 1-\alpha \right) /\left( 1+\alpha \right) }}{\sqrt{z}}\nonumber \\&\quad \times \cos \left[ \frac{\left( 2N-1\right) \pi }{2}I\left( z,\frac{1}{2},\frac{1}{\alpha +1}\right) \right] \text {d}z,\nonumber \\ \end{aligned}$$

(56)

where I stands for the regularized incomplete Beta function.

The function $g_{2}=\hbox {cn}\left( t\left| \frac{1}{2}\right. \right)$ has the following Fourier series

$$\begin{aligned} \text {cn }\left( t\left| \frac{1}{2}\right. \right)= & {} \overset{\infty }{\underset{N=1}{\sum }}b_{N}\cos \left( \left( 2N-1\right) \frac{\pi }{2K\left( m\right) }t\right) \nonumber \\= & {} \overset{\infty }{\underset{N=1}{\sum }}b_{N}\cos \left( \left( 2N-1\right) \frac{\pi }{2K\left( 1/2\right) }t\right) ,\nonumber \\ \end{aligned}$$

(57)

where the Fourier coefficients are defined by

$$\begin{aligned} b_{N}=\frac{2\pi }{K\left( m\right) \sqrt{m}}\frac{q^{N-1/2}}{1+q^{2N-1}}=\frac{2\sqrt{2}\pi }{K\left( 1/2\right) }\frac{q^{N-1/2}}{1+q^{2N-1}}, \end{aligned}$$

(58)

with q being a special function, the so-called nome, and is defined by:

$$\begin{aligned} q\left( m=\frac{1}{2}\right) =\exp \left( -\pi \frac{K\left( 1-m\right) }{K\left( m\right) }\right) =\exp \left( -\pi \right) , \end{aligned}$$

(59)

where K is the complete elliptic integral of the first kind, which depends on the elliptic parameter m. In the case of $g_{2}$, this parameter is 1/2, so that $K\left( 1/2\right)$=1.85407. This implies that the Fourier series expansion for $g_{2}$ has the form

$$\begin{aligned} \text {cn }\left( t\left| \frac{1}{2}\right. \right)\approx & {} 0.955006\text {cos }\left( 0.847213t\right) \nonumber \\&+\,0.0430495\text {cos }\left( 2.54164t\right) \nonumber \\&+\,0.00186049\text {cos }\left( 4.23607t\right) \nonumber \\&+\,0.0000803976\text {cos }\left( 5.93049t\right) +\ldots \nonumber \\ \end{aligned}$$

(60)