1 Introduction

Modeling and simulation of thermoelastic damping is a recurrent interest in the community of nano-engineering and nano-mechanics, mainly motivated by the recent advancement of nanoelectromechanical system (NEMS) technologies. In this size regime, it is possible to attain extremely high fundamental frequencies while simultaneously preserving very high mechanical responsivity (small force constants). Such high-frequency mechanical devices have many important applications among which are ultrasensitive mass detection, mechanical signal processing, scanning probe microscopes, etc. The most important parameter of a nano-resonator is its thermoelastic damping factor, and it is closely related to the accuracy of measurement in many applications. The higher the quality factor, the less energy dissipated during vibrations and the higher the resonator’s sensitivity.

The generalized theories of thermoelasticity, which admit the finite speed of thermal signal, have been the center of interest of active research during last three decades. Biot (1956) introduced the classical theory of thermoelasticity suffer from the so-called “paradox of heat conduction,” i.e., the heat equations for both theories of a mixed parabolic–hyperbolic type, predicting infinite speeds of propagation for heat waves, contrary to physical observations. The theory of couple thermoelasticity was extended by Lord and Shulman (1967) and Green and Lindsay (1972) by including the thermal relaxation time in constitutive relations. The counterparts of our problem in the contexts of the thermoelasticity theories have been considered by using numerical and analytical methods (Ezzat and Fayik 2012; Abbas 2014; Ezzat et al. 2001; Abbas and Zenkour 2013; Sherief 1994; Abbas and Othman 2012a; Ezzat et al. 2002; Abbas and Youssef 2012; Sherief and Megahed 1999; Abbas and Abd-Alla 2008; Abbas 2012; Abbas and Othman 2012b)

Thermoelasticity with two temperatures is one of the non-classical theories of thermoelasticity of elastic solids. The thermal dependence is the main difference of this theory with respect to the classical one. Chen and Williams (1968), Chen and Gurtin (1968), Chen et al. (1969) have formulated a theory of heat conduction in deformable bodies, which depend on two distinct temperatures, the conductive temperature and thermodynamic temperature. For time independent situations, the difference between these two temperatures is proportional to the heat supply. For time dependent problems and for wave propagation problem in particular, the two temperatures are in general different, regardless of the presence of heat supply. El-Karamany and Ezzat (2011) introduced the two-temperature Green–Naghdi thermoelasticity theories. Youssef (2006) introduced the generalized Fourier law to the field equations of the two-temperature theory of thermoelasticity. Abbas and Zenkour (2014), Abbas and Youssef (2009, 2013), Kumar and Abbas (2013) studied different problems under two-temperature generalized thermoelastic theory by finite element method.

Nano-mechanical resonators have attracted considerable attention recently due to their many important technological applications. Accurate analysis of various effects on the characteristics of resonators, such as resonant frequencies and quality factors, is crucial for designing high-performance components. Many authors have studied the vibration and heat transfer process of beams. Houston et al. (2004) predicted that the internal friction in 50-nm scale silicon-based MEMS structures is strong due to thermoelastic damping. Yasumura et al. (1999) observed thermoelastic damping in single-crystal silicon and silicon nitride micro-resonators at room temperature. Nayfeh and Younis (2004a), Nayfeh et al. (2005), Nayfeh and Younis (2004b) derived analytical expressions for the quality factor of micro-plates of general shapes due to thermoelastic damping. Sun et al. (2006) investigated the thermoelastic damping in micro-beam resonators. Rezazadeh et al. (2012) investigated the thermoelastic damping in a micro-beam resonator using modified couple stress theory. Sun et al. (2013) studied the thermoelastic damping of the axisymmetric vibration of laminated trilayered circular plate resonators. Sharma and Grover (2011, 2012) studied the thermoelastic vibration analysis of Mems/Nems with voids with one relaxation time. Sharma (2011) investigated the thermoelastic damping and frequency shift in micro/nanoscale anisotropic beams. Experimentally, NEMS are expected to open up investigations of phonon mediated mechanical processes and of the quantum behavior of mesoscopic mechanical systems (Ekinci and Roukes 2005). Recently, Abbas (2015a) studied the exact solution of thermoelastic damping and frequency shifts in a nano-beam resonator under Green and Naghdi theory. Abbas (2015b) investigated a GN model for thermoelastic interaction in a microscale beam subjected to a moving heat source. Rezazadeh et al. (2015) studied the variation of deflection, temperature change in a nonlocal nano-beam resonator as NEMS based on the type III of Green–Naghdi theory (with energy dissipation). Youssef et al. (2014) studied the vibration of gold nano beam in context of two-temperature generalized thermoelasticity subjected to laser pulse. Khanchehgardan et al. (2013) investigated the thermo-elastic damping in nano-beam resonators based on nonlocal theory.

This paper investigate the effect of two-temperature parameter and relaxation time in thermoelastic damping and frequency shift in a nano-scale resonator into the context of the two-temperature generalized thermoelasticity theory with one relaxation time.

2 Basic equation

Let us consider a thermally conducting, isotropic, homogeneous, thermoelastic solid initially undeformed and at a uniform temperature \(T_{0}\). The basic governing equations of motion and heat conduction in the context of by Lord and Shulman theory (1967) with two temperatures (Youssef 2006) in the absence of heat sources and body forces, can be formulated mathematically as:

The equations of motion

$$ \sigma_{ji,j} = \rho \frac{\partial^{2}u_{i}}{\partial t^{2}}, $$
(1)

where \(\sigma_{ij}\) are the components of stress tensor, \(\rho \) is the density of the medium, \(u_{i}\) are the components of displacement vector, and \(t\) is the time. The equation of heat conduction can be written as

$$ K\varphi_{,ii} = \biggl( \frac{\partial }{\partial t} + \tau_{o} \frac{\partial^{2}}{\partial t^{2}} \biggr) ( \rho c_{e}T + \gamma T _{0}e_{kk} ) , $$
(2)

where \(\varphi = \varphi^{ *} - T_{0}\) is the conductive deviation from the reference temperature \(T_{0}\), \(T = T^{ *} - T_{0}\) is the thermodynamic temperature deviation from the reference temperature \(T_{0}\), \(e_{ij}\) are the components of strain tensor, \(K\) is the thermal conductivity, \(\tau_{o}\) is the relaxation time, \(\gamma = ( 2 \lambda + 3\mu ) \alpha_{t}\), \(\alpha_{t}\) is the coefficient of linear thermal expansion, and \(c_{e}\) is the specific heat at constant strain. The constitutive equations are

$$\begin{aligned} & \sigma_{ij} = 2\mu e_{ij} + [ \lambda e_{kk} - \gamma T ] \delta_{ij}, \end{aligned}$$
(3)
$$\begin{aligned} & \varphi - T = b \varphi_{,ii}. \end{aligned}$$
(4)

where \(\lambda \), \(\mu \) are the Lame’s constants, \(b > 0\) is the two-temperature parameter.

3 Modeling of beam structures

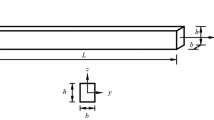
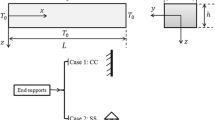
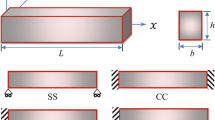
Let us consider small flexural deflection of homogeneous isotropic thermally conducting, thermoelastic beam in Cartesian coordinate systems \(\mathit{oxyz}\) for the displacement vector \(\mathbf{u} (x,y,z,t) = (u,v,w)\) and temperature change \(T(x,y,z,t)\), which have dimension length \(L\) (\(0 \le x \le L \)), width \(a\) (\(- \frac{a}{2} \le y \le \frac{a}{2} \)), and thickness \(h\) (\(- \frac{h}{2} \le z \le \frac{h}{2} \)). That is, any plane cross-section initially perpendicular to axis of beam remains, plane and perpendicular to the neutral surface during bending. Thus, the displacements are given by

$$ u = - z\frac{\partial w}{\partial x}, \quad v = 0, \quad w(x,y,z,t) = w(x,t). $$
(5)

Then, the equation of transverse motion without the pressures on the upper and lower surface of the beam is

$$ \frac{\partial^{2}M}{\partial x^{2}} + \rho A\frac{\partial^{2}w}{ \partial t^{2}} = 0, $$
(6)

where \(M\) is the flexural moment of cross-section of beam and \(A = a h\) is the area of cross-section.

The equation of heat conduction is given by

$$ K \biggl( \frac{\partial^{2}\varphi }{\partial x^{2}} + \frac{\partial ^{2}\varphi }{\partial z^{2}} \biggr) = \biggl( \frac{\partial }{ \partial t} + \tau_{o}\frac{\partial^{2}}{\partial t^{2}} \biggr) \biggl( \rho c_{e}T - \gamma T_{0}z \frac{\partial^{2}w}{\partial x^{2}} \biggr) . $$
(7)

The constitutive relation can be written as

$$\begin{aligned} & \sigma = - ( \lambda + 2\mu ) z\frac{\partial^{2}w}{\partial x^{2}} - \gamma T, \end{aligned}$$
(8)
$$\begin{aligned} & T = \varphi - b \biggl( \frac{\partial^{2}\varphi }{\partial x^{2}} + \frac{ \partial^{2}\varphi }{\partial z^{2}} \biggr) . \end{aligned}$$
(9)

The flexural moment of the cross-section of the beam is given by

$$ M(x,t) = - \int_{ - h/2}^{h/2} a \sigma z dz = ( \lambda + 2 \mu ) I \frac{\partial^{2}w}{\partial x^{2}} + \gamma M_{T}, $$
(10)

where \(I = \frac{ah^{3}}{12}\) is moment of inertia of the cross-section and \(M_{T}\) is the thermal moment of beam which given by

$$ M_{T} = \int_{ - h/2}^{h/2} a \biggl[ \varphi - b \biggl( \frac{\partial ^{2}\varphi }{\partial x^{2}} + \frac{\partial^{2}\varphi }{\partial z^{2}} \biggr) \biggr] z dz. $$
(11)

Using Eq. (10) in Eq. (6) yields

$$ ( \lambda + 2\mu ) I\frac{\partial^{4}w}{\partial x^{4}} + \gamma \frac{\partial^{2}M_{T}}{\partial x^{2}} + \rho A \frac{\partial ^{2}w}{\partial t^{2}} = 0. $$
(12)

The preceding equations can be changed into the dimensionless form using the parameters defined as

$$ \begin{aligned}[c] & \bigl(x',y',z', w'\bigr) = \frac{(x,y,z, w)}{c\chi }, \quad \bigl( t',\tau '_{o} \bigr) = \frac{ ( t,\tau_{o} ) }{\chi }, \quad M'_{T} = \frac{M_{T}}{T _{0}c^{3}\chi^{3}}, \\ & \bigl( T',\varphi ' \bigr) = \frac{ ( T, \varphi ) }{T_{0}},\quad \omega' = \omega \chi , \quad b = \frac{b}{c^{2} \chi^{2}}, \end{aligned} $$
(13)

where \(c^{2} = \frac{\lambda + 2\mu }{\rho }\), \(\chi = \frac{K}{\rho c _{e}c^{2}}\).

By substituting (13) into Eqs. (7) and (12) one may obtain (after dropping the superscript ′ for convenience)

$$\begin{aligned} & I\frac{\partial^{4}w}{\partial x^{4}} + \frac{\gamma T_{0}}{\lambda + 2\mu } \frac{\partial^{2}M_{T}}{\partial x^{2}} + A\frac{\partial^{2}w}{ \partial t^{2}} = 0, \end{aligned}$$
(14)
$$\begin{aligned} & \frac{\partial^{2}\varphi }{\partial x^{2}} + \frac{\partial^{2} \varphi }{\partial z^{2}} = \biggl( \frac{\partial }{\partial t} + \tau _{o}\frac{\partial^{2}}{\partial t^{2}} \biggr) \biggl( \varphi - b \biggl( \frac{\partial^{2}\varphi }{\partial x^{2}} + \frac{\partial ^{2}\varphi }{\partial z^{2}} \biggr) - \frac{\gamma }{\rho c_{e}}z \frac{ \partial^{2}w}{\partial x^{2}} \biggr) . \end{aligned}$$
(15)

4 Initial and boundary conditions

In order to solve the problem, both initial and boundary conditions should be considered. The initial conditions of the problem are taken as

$$ w ( x,0 ) = \frac{\partial w ( x,0 ) }{\partial t} = 0, \qquad \varphi ( x,z,0 ) = \frac{\partial \varphi ( x,z,0 ) }{\partial t} = 0. $$
(16)

These conditions are supplemented by considering the both ends of the nano-beam are clamped, so that we have the following boundary conditions:

$$ w ( 0,t ) = w ( L,t ) = 0, \qquad \frac{\partial w (0,t ) }{\partial x} = \frac{\partial w ( L,t ) }{\partial x} = 0. $$
(17)

For the case, the heat flux between the lower and upper surfaces of the beam is sufficiently small. So, zero heat flux conditions can be applied:

$$ \frac{\partial \varphi ( x, - h/2,0 ) }{\partial z} = \frac{ \partial \varphi ( x,h/2,0 ) }{\partial z} = 0. $$
(18)

5 Solution along thickness direction

In order to solve the system of Eqs. (12) and (13), the harmonic solutions can be assumed as

$$ \bigl[ w ( x,t ) ,\varphi ( x,z,t ) \bigr] = \bigl[ w ( x ) ,\varphi ( x,z ) \bigr] e^{i\omega t}. $$
(19)

From Eq. (19) in (14) and (15), we get

$$\begin{aligned} & I\frac{\partial^{4}w}{\partial x^{4}} + \frac{\gamma T_{0}}{\lambda + 2\mu } \frac{\partial^{2}M_{T}}{\partial x^{2}} - \omega^{2}Aw = 0, \end{aligned}$$
(20)
$$\begin{aligned} & \frac{\partial^{2}\varphi }{\partial x^{2}} + \frac{\partial^{2} \varphi }{\partial z^{2}} = \frac{ ( i\omega - \tau_{o}\omega^{2} ) }{1 + b ( i\omega - \tau_{o}\omega^{2} ) } \biggl( \varphi - \frac{\gamma }{\rho c_{e}}z\frac{\partial^{2}w}{\partial x ^{2}} \biggr) . \end{aligned}$$
(21)

We follow the same procedures as in Lifshitz and Roukes (2000). Noting that temperature gradients in the plane of the cross-section along the \(x\) direction are much smaller than those along the \(z\) direction and that no gradients exist in the \(y\) direction, we can replace Eq. (21) by

$$ \frac{\partial^{2}\varphi }{\partial z^{2}} = \frac{ ( i\omega - \tau_{o}\omega^{2} ) }{1 + b ( i\omega - \tau_{o}\omega^{2} ) } \biggl( \varphi - \frac{\gamma }{\rho c_{e}}z \frac{\partial^{2}w}{ \partial x^{2}} \biggr) $$
(22)

because the heat flux between the lower and upper surfaces of the beam is sufficiently small. So, zero heat flux conditions can be applied. When \(\frac{ \partial \varphi }{\partial z} = 0\), \(z = \pm h/2\), the solution of the Eq. (22) is

$$ \varphi ( x,z ) = \frac{\gamma }{\rho c_{e}} \biggl( z - \frac{ \sin ( pz ) }{p\cos ( ph/2 ) } \biggr) \frac{ \partial^{2}w}{\partial x^{2}}, $$
(23)

where \(p = \sqrt{\frac{\tau_{o}\omega^{2} - i\omega }{1 + b ( i \omega - \tau_{o}\omega^{2} ) }}\). Substituting Eq. (21) and Eq. (9) into Eq. (18), we get

$$ D_{\omega } \frac{d^{4}w}{dx^{4}} - \omega^{2}w = 0, $$
(24)

where

$$\begin{aligned} & D_{\omega } = \frac{I}{A} \bigl( 1 + \varepsilon_{T} \bigl[ \bigl( 1 + \bigl( 1 + bp^{2} \bigr) f(\omega ) \bigr) \bigr] \bigr) , \qquad \varepsilon_{T} = \frac{\gamma^{2}T_{0}}{\rho c_{e} ( \lambda + 2 \mu ) }\quad \mbox{and} \\ & f (\omega ) = \frac{24}{p^{3}h^{3}} \biggl( \frac{ph}{2} - \tan \frac{ph}{2} \biggr) . \end{aligned}$$

As \(w = w ( x ) \), Eq. (24) provides us

$$ \biggl( \frac{d^{4}}{dx^{4}} - \eta^{4} \biggr) w = 0,\quad \eta^{4} = \frac{\omega^{2}}{D_{\omega }}. $$
(25)

The solution of Eq. (25) can be written as

$$ w ( x ) = B_{1}\sin ( \eta x ) + B_{2}\cos ( \eta x ) + B_{3}\sinh ( \eta x ) + B_{4}\cosh ( \eta x ) . $$
(26)

Substituting the solution (26) into (23), we get

$$ \begin{aligned}[b] \varphi ( x,z ) & = \frac{\eta^{2}\gamma }{\rho c_{e}} \biggl( z - \frac{\sin ( pz ) }{p\cos ( ph/2 ) } \biggr) \\ & \quad {}\times \bigl( - B_{1}\sin ( \eta x ) - B_{2}\cos ( \eta x ) + B_{3}\sinh ( \eta x ) + B_{4}\cosh ( \eta x ) \bigr) . \end{aligned} $$
(27)

Thus, the solutions of all variables in physical space–time domain are given by

$$\begin{aligned} & w ( x,t ) = \bigl[ B_{1}\sin ( \eta x ) + B_{2} \cos ( \eta x ) + B_{3}\sinh ( \eta x ) + B_{4} \cosh ( \eta x ) \bigr] e^{i\omega t}, \end{aligned}$$
(28)
$$\begin{aligned} & \varphi ( x,z,t ) = \frac{\eta^{2}\gamma }{\rho c_{e}} \biggl( z - \frac{\sin ( pz ) }{p\cos ( ph/2 ) } \biggr) \left ( \textstyle\begin{array}{l} - B_{1}\sin ( \eta x ) - B_{2}\cos ( \eta x ) + \\ + B_{3}\sinh ( \eta x ) + B_{4}\cosh ( \eta x ) \end{array}\displaystyle \right ) e^{i\omega t}, \end{aligned}$$
(29)
$$\begin{aligned} & T ( x,z,t ) = \frac{\eta^{2}\gamma }{\rho c_{e}} \biggl( z - \frac{ ( 1 + bp^{2} ) \sin ( pz ) }{p\cos ( ph/2 ) } \biggr) \left ( \textstyle\begin{array}{l} - B_{1}\sin ( \eta x ) - B_{2}\cos ( \eta x ) + \\ + B_{3}\sinh ( \eta x ) + B_{4}\cosh ( \eta x ) \end{array}\displaystyle \right ) e^{i\omega t} \end{aligned}$$
(30)

where \(B_{1}\), \(B_{2}\), \(B_{3}\) and \(B_{4}\) are constants to be determined from the boundary condition of the problem.

6 Frequency shift and thermoelastic damping

Using the boundary conditions (17) in the solutions, we obtain the following characteristic equation which governs the vibrations of the beam:

$$ \cos ( \eta L ) \cosh ( \eta L ) - 1 = 0. $$
(31)

Thus, the corresponding characteristic roots of Eq. (31) are given by

$$ \eta = \frac{2m\pi }{L},\quad \mbox{where }m\ \mbox{is an integer}. $$
(32)

Now the vibration frequency of the thermoelastic beam in the presence of thermoelastic coupling \(\varepsilon_{T}\), two-temperature parameter \(b\) and thermal relaxation time \(\tau_{o}\) is given by

$$ \omega_{m} = \frac{4m^{2}\pi^{2}}{L^{2}}\sqrt{D_{\omega }} = \omega _{o}\sqrt{1 + \varepsilon_{T} \bigl[ 1 + \bigl( 1 + bp^{2} \bigr) f( \omega ) \bigr] }, $$
(33)

where \(\omega_{o} = \frac{4hm^{2}\pi^{2}}{L^{2}\sqrt{12}}\). For most of the material \(\varepsilon_{T} \ll 1\), so we can replace \(\omega \) by \(\omega_{o}\) and \(f ( \omega ) \) by \(f ( \omega_{o} ) \) to get

$$ \omega^{m} = \omega_{o}\sqrt{1 + \varepsilon_{T} \bigl[ 1 + \bigl( 1 + bp^{2} \bigr) f(\omega_{o}) \bigr] }, $$
(34)

Owing to the presence of dissipation term in the heat conduction equation and thermal stresses, the frequency equation in the general complex transcendental equation provides us a complex value of frequency \(\omega^{m} = \omega_{R}^{m} + i\omega_{I}^{m}\), where \(m\) is the mode number, which corresponds to the roots of the transcendental equation (31), and \(\omega_{R}^{m}\) and \(\omega_{I}^{m}\) are the real part and imaginary parts of frequency \(\omega^{m}\), respectively.

The frequency shift due to thermal variations is defined as

$$\omega_{s} = \biggl\vert \frac{\omega_{R}^{m} - \omega_{o}}{\omega_{o}} \biggr\vert . $$

The thermoelastic damping is expressed in terms of the inverse of quality factor as

$$Q^{ - 1} = 2\biggl\vert \frac{\omega_{I}^{m}}{\omega_{R}^{m}} \biggr\vert . $$

7 Numerical results and discussion

This section is devoted to numerically presenting the theoretical results derived in the previous sections. The two-temperature model with one relaxation time is analyzed by considering a nanoscale resonator. We assume that the nanoscale resonator is made isotropic and the silicon material has been chosen. The physical constants are listed below (Grover 2013):

$$\begin{aligned} & \lambda = 2.17 \times 10^{11}\mbox{ N}\,\mbox{m}^{ - 2}, \quad \mu = 1.08 \times 10^{11}\mbox{ N}\,\mbox{m}^{- 2},\quad T_{0} = 296\mbox{ K}, \quad \rho = 3200 \mbox{ kg}\,\mbox{m}^{ - 3}, \\ & K = 43.5\mbox{ W}\,\mbox{m}^{ - 1}\,\mbox{K}^{ - 1}, \quad c_{e} =630\mbox{ J}\,\mbox{kg}^{ - 1}\,\mbox{K}^{ - 1}, \quad \gamma = 2.71 \times 10^{6}\mbox{ N}\,\mbox{m}^{ - 2}\,\mbox{K}^{ - 1}, \quad \tau_{0} = 0.2. \end{aligned}$$

From the dimensionless parameters, it is clear that the scale of thickness and length for a nano-beam is equal to \(1.85 \times 10^{ - 9}\mbox{ m}\) and the relaxation time is equal to \(1.6 \times 10^{ -13}\mbox{ s}\). Figures 1–3 show the variation of thermoelastic damping in a clamped beam of the fixed of length for different modes with different values of two-temperature parameter and relaxation time. Figure 1 exhibits the thermoelastic damping \(Q^{ - 1}\) versus thickness \(h\) for the first four modes when the two-temperature parameter \(( b = 0.2 ) \) and the thermal relaxation time \(( \tau_{o} = 0.25 ) \) remain constant. Figure 2 shows the variation of thermoelastic damping \(Q^{ - 1}\) versus thickness \(h\) for different values of the two-temperature parameter \(( b = 0.0,0.2,0.4,0.6 ) \) for the first and second modes when the thermal relaxation time \(( \tau _{o} = 0.25 ) \) remains constant. Figure 3 illustrates the variation of thermoelastic damping \(Q^{ - 1}\) versus thickness \(h\) for different values of thermal relaxation time \(( \tau_{o} = 0.0,0.25,0.5 ) \) for the first and second modes when the two-temperature parameter \(( b = 0.2 ) \) remains constant. From Figs. 1–3, it is observed that the thermoelastic damping \(Q^{ - 1}\) increases initially to attain its maximum peak value before it decreases in order to become ultimately asymptotic with increasing \(h\). The maximum peak value of thermoelastic damping increases with increasing modes of vibration. The two-temperature parameter and relaxation times play a significant role on the thermoelastic damping \(Q^{ - 1}\).

Fig. 1
figure 1

Thermoelastic damping \(Q^{ - 1}\) versus thickness \(h\) for 2TLS theory and the first four modes having fixed length

Full size image
Fig. 2
figure 2

Thermoelastic damping \(Q^{ - 1}\) versus the thickness \(h\) for different value of \(b\) in cases the first and second modes with the fixed of length

Full size image
Fig. 3
figure 3

Thermoelastic damping \(Q^{ - 1}\) versus thickness \(h\) for different values of \(\tau_{o}\) when the first and second modes are of fixed length

Full size image

Figures 4, 5 and 6 show the variation of frequency shift \(\omega_{s}\) versus \(h\) for the first four modes of vibrations. Figure 4 shows the behavior of the frequency shift \(\omega_{s}\) versus thickness \(h\) for the first four modes when the two-temperature parameter and the thermal relaxation time remain constants. Figures 5 and 6 show the effects of the two-temperature parameter and relaxation time on the first and second modes. It can be inferred that the frequency shift \(\omega_{s}\) increases rapidly with increasing thickness to attain its maximum peak value, and it becomes stable for large values of the thickness. Also, it has been observed that the two-temperature parameter and thermal relaxation time play a significant role on the frequency shift \(\omega_{s}\).

Fig. 4
figure 4

Frequency shift \(\omega_{s}\) versus the thickness \(h\) for 2TLS theory and first four modes with the fixed of length

Full size image
Fig. 5
figure 5

Frequency shift \(\omega_{s}\) versus thickness \(h\) for different values of \(b\) when the first and second modes have fixed length

Full size image
Fig. 6
figure 6

Frequency shift \(\omega_{s}\) versus thickness \(h\) for different values of \(\tau_{o}\) when the first and second modes have fixed length

Full size image

8 Conclusions

The thermoelastic damping of a nano-beam resonator is analyzed by the two-temperature Lord and Shulman theory (2TLS). Analytical expressions for deflection, temperature change, thermoelastic damping and frequency shifts in the beam have been derived. The closed form solution obtained here opens the scope of further studies in mathematics, science, and engineering disciplines.