1 Introduction

Scholars have been aware of the importance of mechanical environment in the development of living organisms for at least four centuries. Galileo Galilei suggested in 1638 that mechanics naturally limits the size of animals due to a cubic scaling of gravitational forces [1, 2]. Nevertheless, the relation between mechanics and biology was conceptualized for a long time as static or predetermined; an active change of soft tissue mass in response to a change in its mechanical environment was considered “a physiological impossibility” [3]. This view was criticized by Henry Gassett Davis in 1867 who postulated that biological soft tissues are capable of functional adaptations to a changing mechanical environment [4]. He suggested that “Nature never wastes her … material” [5] but rather responds to an increased load by lengthening and the addition of material and to a decreased load by the opposite. Soon thereafter, Julius Wolff postulated a similar relation for bone, the famous Wolff’s law [6]. Together, these two nineteenth century concepts suggested that, despite their different structures and functions, both soft and hard tissues may be designed to promote mechanical optimality following a similar rationale.

Nowadays the importance of the intimate relation between mechanical stimuli and biological growth and remodeling (G&R) is widely acknowledged in biology, biomedical engineering, and medicine, and forms an important part of the emerging field of mechanobiology [7]. Examples range from substrate stiffness determining the lineage fate of stem cells [8] to physical disuse of tendons [9] and bone [10, 11] leading to atrophy (that is, loss of tissue mass). Mechano-regulated G&R thus plays important roles in both morphogenesis and pathogenesis, including disease progression wherein normal tissue is simply altered (e.g., aortic aneurysms [12]) or abnormal tissue develops and accumulates (e.g., malignant tumors in cancer [13]). Likewise, G&R can occur under the action of physiological loads (e.g., enlargement of an aneurysm under normal blood pressures) or under the action of non-physiological loads (e.g., post-surgical G&R due to surgical implantation of a medical device [14]). Notwithstanding the importance of understanding and modeling all aspects of G&R, from molecular to whole organism scales and related to all medical specialties from pathology to surgery, for illustrative purposes we focus on mature load-bearing soft tissues wherein collagen turnover dominates adaptive responses and multiple cases of disease progression. Moreover, we focus primarily on G&R of two types of tissues, blood vessels and tendons/ligaments. By growth, we mean a change of tissue mass, either an increase or a decrease (atrophy); by remodeling, we mean a change in tissue microstructure and thus mechanical properties. Although G&R need not occur simultaneously, they often go hand-in-hand. Hence, basic concepts and theoretical frameworks should seek a unified approach that can recover, as special cases, these potentially separate processes.

Soft tissue G&R has attracted considerable interest since the mid-1990s [15–17] and now represents one of the primary areas of study in biomechanics. Novel experimental methods continue to provide an increasingly detailed understanding of molecular and cellular mechanisms as well as tissue-to-organism level manifestations. At the same time, theoretical frameworks and computational approaches continue to advance significantly. Nevertheless, as is often the case, there is much to learn from the early observations and theoretical ideas. Thus, we first briefly summarize in Sect. 2 some of the seminal findings that continue to guide and motivate current studies. In Sect. 3 we review the state of mathematical modeling; in Sect. 4 we discuss important theoretical principles that have been identified; in Sect. 5 we list a few illustrative examples found in key references.

2 Experimental and clinical observations

2.1 Tensional homeostasis

Comparative biology is a particularly powerful approach for revealing general principles that govern biological structure–function relationships. As an example, consider the aorta, the main blood vessel in the body. The mature aortic wall consists primarily of an elastic fiber/smooth muscle rich parenchymal layer that has a lamellar structure that is surrounded by a collagen rich layer that serves as a protective sheath. Wolinsky and Glagov [18] compared values of tension and tension per lamellar unit in the normal, mature aortic wall from different species ranging from mice (28 g body mass) to pigs (200 kg body mass). They found that wall tension varied by a factor of 26 across these species, yet the tension per lamellar unit was remarkably similar (2–4 N/m) and varied by only a small factor (~2.8). This finding suggested the existence of a cell-mediated mechanism aimed to establish the local mechanical environment near a preferred value and/or to ensure a nearly constant level of Cauchy stress that is structurally favorable. This concept was supported further by observations in a single species that the aorta tends to thicken in response to sustained increases in blood pressure (i.e., hypertension) and thereby to restore Cauchy stress toward a nearly constant normal level of around 150–300 kPa [19, 20]. Related to this, Shadwick [21] subsequently reported that the aortic stiffness in vivo, evaluated at individual values of mean arterial pressure, differs in species ranging from toad, squid, and shark to rat and whale only by a factor of 1.4 (with an average value of ~0.4 MPa). Because many soft tissues, including arteries, exhibit a nearly exponential stress–strain relationship [22], stiffness and stress relate nearly linearly, thus rendering it difficult to discern whether the cells seek to establish, maintain, or restore a preferred state of stress or stiffness. Nevertheless, these early findings in arterial biomechanics support the general hypothesis that there exists a preferred mechanical state for a load-bearing soft tissue, a so-called homeostatic state. Although not recognized previously, this hypothesis is consistent with Davis’ law [4], which suggests that perturbations from a preferred homeostatic state in soft collagenous tissues are answered by biological G&R processes aimed to restore normalcy.

In vivo studies provide considerable insight and motivation, but in vitro studies often provide complementary understanding. Early cell culture studies revealed that aortic smooth muscle cells change their production of important extracellular matrix proteins and glycosaminoglycans directly in response to changes in mechanical loading [23]. Subsequent studies confirmed this mechanobiological response and showed, in the case of cyclically stretched smooth muscle cells, that altered mechanical loading changes both the production of different biomolecules by the cell and the number or sensitivity of associated membrane-bound receptors [24]. That is, these studies proved that smooth muscle cells change their production and secretion of biomolecules (i.e., mass) directly in response to changes in mechanical loading, thus confirming mechano-mediated growth processes. To similarly simplify studies of cell–matrix interactions under mechanical loading, many investigators turned to so-called tissue equivalents. Typically consisting of reconstituted collagen or fibrin gels, having various geometries and boundary conditions, these gels are seeded with smooth muscle cells or fibroblasts and monitored over short periods (hours) to study responses to mechanical stimuli [25]. In a particularly important early study, uniaxial collagen gels fixed at both ends were observed to develop within a few hours an internal stress to a certain (apparently homeostatic) plateau level when seeded with fibroblasts. If this homeostatic state was perturbed by suddenly stretching or relaxing the gel, an immediate elastic change of stress in the gel was followed by a slow, exponential return back toward the homeostatic level [26, 27]. It is important to distinguish this behavior from passive viscoelastic stress relaxation common in many biological soft tissues [28]. Passive stress relaxation always releases strain energy. In contrast, active G&R restores a certain stress level even if this requires an increase in stress and strain energy as illustrated in Fig. 1. Such studies confirmed that cells actively change the microstructure and stress of the matrix in which they reside directly in response to changes in mechanical loading, thus confirming mechano-mediated remodeling processes. Subsequent reports showed further that this active remodeling, and even initial production, of extracellular matrix requires actomyosin activity [29], hence revealing that cells not only synthesize and secrete diverse extracellular constituents, they also actively fashion these constituents to control structure, stiffness, and strength [30]. Collectively, these many findings support the notion of “tensional homeostasis”, that is, cells attempt to establish, maintain, or restore a homeostatic mechanical state.

Fig. 1
figure 1

If a uniaxial collagen gel seeded with fibroblasts is shortened from length L to \(L - \Delta L\) at time t 0 (left), a step-decrease of the elastic stress from the initial homeostatic level \(\sigma_{h}\) is followed by a slow return back toward the homeostatic stress (right)

Full size image

2.2 Turnover

An important difference between living tissues and traditional engineering materials is mass turnover. Cells in living soft tissues not only produce (synthesize and secrete new) and remodel (rearrange extant) structural constituents within the extracellular matrix, they also actively remove (degrade) these constituents. The combined deposition and degradation of constituents is referred to as turnover. It can occur either within a cell (over short time scales, often minutes) or within the extracellular space (over longer time scales, possibly hours but often days to months); it can also be either balanced (homeostatic) or unbalanced (adaptive or pathologic). Two extreme examples of unbalanced matrix turnover are fibrosis (excessive deposition of matrix, as in scars) and atrophy (unbalanced degradation, as in disuse). Importantly, homeostasis is often thought of as a state, yet this word actually describes a process—one by which cells attempt to maintain a preferred state, a biological equilibrium. Given that all biological cells have finite life-spans and all biological molecules have finite half-lives, homeostasis necessarily requires continuous turnover even when there is no macroscopic change in geometry or mechanical properties. Such a case is called tissue maintenance.

In arteries, for example, the half-life of collagen fibers has been studied using radioactive L-[2,3-3H]proline. Given that the normal half-life of arterial collagen appears to be on the order of 60–70 days, cells must continually produce new collagen fibers to maintain tissue normal. This half-life can decrease significantly in cases of disease, for example, down to 17 days in hypertension [31]. Hence, synthetic arterial cells must not only increase their production of collagen to increase wall thickness to restore wall stress toward normal in hypertension, they must further increase production to offset the increased loss of collagen. Altered rates of collagen turnover (production and removal) similarly appear to be critical in other cases of arterial disease, including aneurysms [32].

Whereas collagen fibers experience different rates of turnover within the arterial wall in normalcy and disease, elastic fibers do not turnover. Functional arterial elastic fibers are unique; they alone are exclusively produced prior to adulthood and yet persist for many years because of a remarkably long half-life, on the order of 25–70 years [33, 34]. For this reason, although arteries are subject to 107 load cycles per year in humans due to the pulsatile blood pressure, mechanical fatigue is important only in relation to the structural integrity of the elastic fibers. That is, living soft tissues appear to avoid most issues of mechanical fatigue via the process of mass turnover; extant tissue is normally replaced prior to its mechanical degradation by fatigue. In other words, one could hypothesize that one purpose of mass turnover is to serve as a continuous repair mechanism to replace extant, possibly damaged, tissue by new intact tissue. Regardless, there is abundant experimental evidence that mass turnover is controlled by both the biomechanical and biochemical environment. Increased mechanical stress correlates with an increased synthesis and secretion of structural proteins (mass production) as well as proteolytic enzymes [35], the latter of which can hasten mass removal. Moreover, changes in stress or strain can alter the rate at which matrix is degraded by proteases [36, 37]. Mechanically dependent degradation rates likely contribute to the overall process by which the arterial wall thickens in hypertension until a homeostatic wall stress is restored. Finally, whereas rates of matrix production and removal are governed, in part, by mechano-stimulated changes in gene expression, it is becoming increasingly clear that final gene products are also influenced by microRNAs (e.g., [38, 39]). There is a need to explore the effects of mechanical and other factors on microRNA activity as well.

It is important to recall that soft tissue properties also depend on the degree of cross-linking of particular constituents as well as other matrix-to-matrix interactions. In general, fibers within the extracellular matrix can interact in different ways, as, for example, by mechanical entanglements (kinematic constraints) or chemical bonds. Among other enzymes, lysyl oxidases and transglutaminases play important roles in covalently cross-linking molecules such as collagen. The former may be important in cross-linking newly synthesized molecules (i.e., in growth) whereas the latter may be important in cross-linking extant molecules (i.e., in remodeling). Recalling the desire to construct general theoretical frameworks for G&R, note that one can use the concept of “mass turnover” to describe effects of altered cross-linking. For example, one can conceptualize the cross-linking of extant fibers simply as the removal of non-cross-linked fibers and the simultaneous production of (i.e., replacement with) cross-linked fibers. Cross-linking collagen is not only important for increasing structural properties such as stiffness and strength, it is also important in tensional homeostasis. For example, inhibition of tissue transglutaminase in initially stress-free collagen gels seeded with fibroblasts reduced the ability of the cells to compact the gels, which by inference reduced their ability to achieve a preferred mechanical environment [40]. This observation is consistent with the thought that macroscopic remodeling of matrix by cells must occur in increments, with incremental changes preserved by cross-linking. These observations suggest that cell-mediated microstructural reorganization of both the orientations of and the cross-links between fibers plays an important role in establishing, maintaining, or restoring matrix homeostasis. Although the precise underlying mechanisms remain unknown, the breaking and reforming of cross-links appears to be an efficient complement to frank mass turnover and should be explored further both experimentally and computationally.

2.3 Prestress (or deposition stretch)

A vital aspect of cellular regulation of matrix is the mechanical state of the matrix that is either deposited de novo or remodeled within extant matrix. In particular, early G&R theories [41] conjectured and computational [42] and theoretical [43] studies confirmed that replacement of stressed fibers with initially unstressed fibers could not achieve homeostasis—initially stress-free or under-stressed fibers would likely extend until reaching the in vivo level of stress, which would necessarily result in tissue elongation or expansion, not maintenance. In other words, it is natural to imagine that newly produced or remodeled matrix must be prestressed and, under optimal conditions, the value of prestress should equal the homeostatic target [30]. Indirect evidence that cells prestress the matrix that they produce or remodel continues to accumulate. For example, assembly of vascular collagen requires both integrins and actomyosin activity [29], presumably so the cells can hold onto and work on the collagen. This concept is supported by experiments on collagen fiber alignment by tendon fibroblasts; these cells can produce collagen in the absence of intracellular actin, but they cannot organize it without actin [44]. Indeed, it has been suggested that elaborate control of gene expression and intracellular signaling related to actomysin activity is fundamental to tensional homeostasis [45], which is important in both normalcy and disease [46].

Contractile forces exerted by cells seem essential to endow matrix with prestress in the first place. This prestress is, however, only partially maintained by continued cellular contraction. A large and probably often dominant part of the prestress is maintained by permanent cross-links that are established between the prestressed and surrounding matrix [47]. Prestress is then maintained primarily by the matrix itself, not by the cells (which would have to consume ATP to this end). How cells actually build-in stresses within new or extant matrix remains unclear and further experiments are required. One hypothesis, however, is a lock-step or ratchet mechanism [48]. Regardless of the biological complexity, linear momentum balance must always be respected [49]. Prestress permanently incorporated in the matrix can balance external loads and thus significantly reduce the stress experienced by the cells. Indeed, the associated “stress shielding” [50] would seem to be mechanically favorable, allowing mechano-sensitive cells merely to probe the matrix to assess whether there is a need for further G&R due to increased stress rather than carrying excessive stress themselves. Finally, note that the existence of prestress in matrix (and similarly in cells, cf. [51]) influences both its stiffness (since stiffness is proportional to stress in a nonlinear material) and its role as a reservoir for a host of growth factors, cytokines, and proteases. It has been suggested, for example, that a prestressed matrix can prime latent cytokines such as transforming growth factor-beta, hence facilitating their activation via additional stresses generated through actomyosin activity [52].

2.4 Pathologic growth and remodeling

In healthy tissues, G&R enables a favorable adaptation to a changing mechanical environment, for example, by restoring nearly normal levels of wall stress in arteries despite sustained increases in blood pressure. There are, however, many cases where neither growth nor remodeling can maintain or appropriately adapt the geometry or mechanical properties of a tissue or organ. Two prominent examples in the arterial tree are aneurysms and tortuosity. Aneurysms are focal dilatations of the arterial wall; some aneurysms can enlarge to a new stable state whereas others continue to enlarge over years and eventually rupture. That is, aneurysmal G&R may or may not result in a new mechanobiologically stable state [43, 53]. Tortuosity, on the other hand, is defined as an abnormal lengthening of a blood vessel that results in a distorted geometry. It appears that tortuosity is irreversible [54], though it is yet unclear whether it can achieve a stable state. It remains an important challenge to understand the mechanisms governing these and many other forms of pathological G&R, which serves as additional motivation for the development of computational models, as noted below.

3 Mathematical modeling

G&R of load-bearing soft tissues typically involves finite deformations and thus must be treated within the context of nonlinear continuum mechanics [55]. Toward this end, a recent review summarized roles of general balance relations in G&R within a continuum mechanical framework [16]. To complement that work, we focus herein on how important biological and micromechanical aspects (such as tensional homeostasis, mass turnover, and prestress) can be appropriately addressed within a general constitutive framework, again based on nonlinear continuum mechanics.

Let us begin by considering a reference configuration \(\kappa \left( 0 \right)\) that is deformed (elastically and inelastically) into a current configuration \(\kappa \left( s \right)\) at G&R time \(s > 0\) (note: since G&R typically occurs over time scales greater than normal in vivo time scales, such as the cardiac cycle or gait frequency, we use G&R time \(s\) and reserve time \(t\) for other temporal processes). Of course, valid reference configurations need not be experienced by the physical tissue nor do they need to be stress-free. Without loss of generality, we assume the reference configuration to be the normal (homeostatic) in vivo loaded configuration at time \(s = 0\). A differential volume element in the reference configuration \(dV\) is mapped to a volume element in the current configuration \(dv = \det \left( \varvec{F} \right)dV\), with \(\varvec{F}\) the standard deformation gradient that maps the tangent space of the reference configuration to the one of the current configuration. In general, G&R in soft tissues typically happens on the time scale of days to months whereas elastic deformations occur on the time scale of seconds or less. Thus, G&R is often modeled as a quasi-static process, subject to the usual balance of linear momentum

$${\text{div}}\left( \varvec{P} \right) + \varrho_{0} \varvec{b}_{0} = 0,$$
(1)

with \(\varvec{P}\) the 1st Piola–Kirchhoff stress tensor, \(\varvec{b}_{0}\) the body force per unit mass, and \(\varrho_{0}\) the mass density per unit reference volume. In the quasi-static setting with hyperelastic materials typically assumed in soft tissue growth and remodeling, we have \(\varvec{P} = \partial \Psi /\partial \varvec{F}\). Hence, to compute deformations and stresses resulting from G&R, one typically has to define a strain energy function \(\Psi\) and a mass balance equation that allows computation of \(\varrho_{0}\) at every in time.

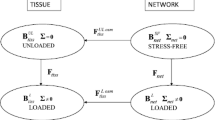
Within this standard setting of nonlinear continuum mechanics two major approaches have been proposed to address G&R (see Fig. 2), namely, kinematic growth and constrained mixture models, which can essentially be understood as two fundamentally different ways to compute the strain energy \(\Psi\) and mass density \(\varrho_{0}\).

Fig. 2
figure 2

From a reference configuration \(\kappa (0)\), a mechanical body is deformed by external loading as well as growth and remodeling into current configurations \(\kappa (\tau )\) and \(\kappa (s)\) at time \(\tau\) and \(s\). Kinematic growth theory assumes that the total deformation gradient \(\varvec{F}\) can be decomposed into an inelastic part \(\varvec{F}_{g}\), capturing growth, and an elastic part \(\varvec{F}_{e}\) that ensures mechanical equilibrium and geometric compatibility during deformation. In contrast, constrained mixture models conceptualize the body as composed of \(n\) constituents, with each constituent composed of a multitude of mass increments deposited at different times. The individual mass increments are deposited with an elastic pre-stretch \(\varvec{F}_{pre}^{i\left( \tau \right)}\) at time \(\tau\) compared to their respective stress-free natural configurations \(\kappa_{n}^{i} (\tau )\). In each volume element, mass increments from different constituents deposited at different times form a constrained mixture, that is, they undergo the same elastic deformation over time

Full size image

3.1 Kinematic growth models

The fundamental basis for current theories of kinematic growth of soft tissues [56] arose from seminal concepts by Richard Skalak that were published in the early-to-late 1980s. It appears that many of these ideas were motivated, in large part, by the desire to understand residual stresses in soft tissues (see Fig. 4 in [56]). Growth was thus conceptualized primarily as changes in the size and shape of a growing unloaded body, which could be described via two deformations: an inelastic “growth” deformation (gradient) \(\varvec{F}_{g}\) capturing stress-free changes by mass added or lost in (infinitesimal) volume elements and a subsequent elastic “assembly” deformation (gradient) \(\varvec{F}_{a}\) that ensures geometric compatibility by assembling the grown volume elements into a contiguous unloaded body. That is, growth of individual volume elements is thought, in general, to be geometrically incompatible and the assembly of these volume elements to result in residual stresses in the contiguous but yet unloaded body. Effects of external loads then require, while enforcing equilibrium, an additional elastic deformation (gradient) \(\varvec{F}_{E}\) so that the total elastic deformation (gradient) is \(\varvec{F}_{e} = \varvec{F}_{E} \varvec{F}_{a}\) and the total deformation (gradient)

$$\varvec{F} = \varvec{F}_{e} \varvec{F}_{g} .$$
(2)

The stored energy \(\Psi\) at any time \(s\) depends on the deformation, thus

$$\Psi \left( s \right) = \Psi \left( {\varvec{F}_{e} \left( s \right)} \right).$$
(3)

Given \(\varvec{F}_{g} \left( s \right)\) in (2), the elastic constitutive Eq. (3) allows one to solve the mechanical equilibrium problem (1) in the traditional way. Kinematic growth theory thus requires, in addition to the elastic constitutive Eq. (3), a second constitutive (evolution) equation for the inelastic deformation (i.e., growth) \(\varvec{F}_{g} \left( s \right)\), typically assumed to not include rigid body rotations.

Overall, this general Kinematic Theory of Growth, which is illustrated in Fig. 2, continues to be used widely (cf., [16, 17]) due to its simplicity and low computational cost. It is conceptually similar to well-known models for viscoelastic fluids (cf. Eq. (10) in [57]) and plasticity (cf. chapter 9 in [58]). Nevertheless, a major challenge in the kinematic growth theory remains the specification of a constitutive relation for \(\varvec{F}_{g}\). Recalling that soft tissue seeks to maintain some mechanical quantity, say \(\varvec{G}\), near its homeostatic value, two approaches to model mechano-regulated G&R are common. First, one can postulate an evolution equation of the form

$$\dot{\varvec{F}}_{g} = {\mathbb{S}}:\;\text{ }\Delta \varvec{G},$$
(4)

where \(\Delta \varvec{G} = \varvec{G} - \varvec{G}_{h}\) is the assumed growth stimulus with \(\varvec{G}_{h}\) the homeostatic value of the mechanical quantity \(\varvec{G}\); the colon denotes a double contraction product and \({\mathbb{S}}\) is some fourth order sensitivity-type tensor. The basis assumption of (4) is that \(\varvec{F}_{g}\) can be written as a smooth function of \(\varvec{G}\) with \(\dot{\varvec{F}}_{g} \left( {\Delta \varvec{G} = \mathbf{0}} \right) = \mathbf{0}\) so that Taylor expansion renders the first order approximation (4). Second, one can define \(\varvec{F}_{g}\) starting from a decomposition

$$\varvec{F}_{g} = \mathop \sum \limits_{j = 1}^{m} \beta^{j} \left( s \right)\varvec{B}^{j}$$
(5)

into second-order basis tensors \(\varvec{B}^{j}\) with scalar \(\beta^{j} (s)\). The \(\varvec{B}^{j}\) define growth directions, and from experimental and clinical observations one can try to define evolution equations for each \(\beta^{j}\) that define the growth in each direction. Again, one may, for example, assume some stimulus \(\Delta \varvec{G}\) as in (4) and approximate \(\beta^{j}\) by a Taylor expansion around \(\Delta \varvec{G} = 0\) with \(\dot{\beta }^{j} \left( {\Delta \varvec{G} = 0} \right) = 0\). Models based on (5) often assume, for example, isotropic growth with \(\varvec{F}_{g} = \beta \left( s \right)\varvec{I}\), with identity tensor \(\varvec{I}\), or else fiber growth with \(\varvec{F}_{g} = \beta \left( s \right)\varvec{A} \otimes \varvec{A} + \left( {\varvec{I} - \varvec{A} \otimes \varvec{A}} \right)\) for fiber direction \(\varvec{A}\) [16]. There continues to be debate about the mechanical quantity \(\varvec{G}\) governing G&R. In [56] \(\varvec{G}\) was identified with the (corotated) Cauchy stress, which is motivated by experimental observations as summarized in Sect. 2.1. Alternatively, strain [59] or, motivated from plasticity theory, the Eshelby stress [60] or Mandel stress [61] have been suggested. Another major problem is the proper definition of \({\mathbb{S}}\) in (4) or the \(\beta^{j}\) and \(\varvec{B}^{j}\) in (5). Typically, these quantities are not chosen on the basis of micromechanical or biological arguments, but rather so as to mimic phenomenologically a certain observed growth behavior (i.e., macroscopic changes in size and shape) [15, 17]. The resulting significant arbitrariness in the choice of \({\mathbb{S}}\), \(\varvec{G}\), \(\beta^{j}\), and \(\varvec{B}^{j}\) remains one of the greatest limitations of kinematic growth models.

The spatial density \(\varrho\) of a soft tissue is often assumed to remain constant not only during elastic deformation (“incompressibility”) but also during G&R. That is, \(\varrho = \varrho_{0} \left( s \right)/{ \det }\left( {\varvec{F}\left( s \right)} \right) = const\) with the density per unit reference volume \(\varrho_{0}\). From (2) we know \({ \det }\left( \varvec{F} \right) = { \det }\left( {\varvec{F}_{e} } \right){ \det }\left( {\varvec{F}_{g} } \right) = { \det }\left( {\varvec{F}_{g} } \right)\) with the incompressibility condition \({ \det }\left( {\varvec{F}_{e} } \right) = 1\). Thus, one has the net mass production per unit reference volume

$$\dot{\varrho }_{0} \left( s \right) = \varrho \frac{{d\left[ {{ \det }\left( {\varvec{F}_{g} } \right)} \right]}}{ds}.$$
(6)

This mass balance becomes, in the case of (4) always and in case of (5) at least approximately in the neighborhood of the homeostatic state,

$$\dot{\varrho }_{0} \left( s \right) = \varrho_{0} \varvec{k}_{\sigma } :\Delta \varvec{G}$$
(7)

where the colon again denotes a double contraction product and \(\varvec{k}_{\sigma }\) is a gain-type parameter (a second order tensor that depends in general on \(\varvec{F}_{g}\)). Equation (5) can not only be used for mechano-regulated growth but also in various other cases where mass deposition in a body or tissue has to be modeled. Finally, it is worth mentioning that in kinematic growth models one often defines not only evolution equations for the inelastic deformation gradient \(\varvec{F}_{g}\) but also for material properties such as structural tensors characterizing anisotropic constitutive properties. This way, for example, mechano-regulated reorientation of collagen fibers in vitro and in vivo was studied [62–64].

In summary, most implementations of the kinematic growth theory are computationally convenient, yet mechanobiologically limited. Reasons for the latter are to be expected, in part, because the theory was designed to model the growth of initially stress-free configurations of whole tissues. Growth and remodeling in vivo necessarily occurs in stressed configurations via the production, removal, or remodeling of different types of constituents at different rates and having different prestresses. These processes are not considered in kinematic growth theory, and \(\varvec{F}_{g}\) is typically defined heuristically. There is also generally no attempt to model the mechanics of separate constituents, such as elastic fibers (with a normal half-life of several decades) versus collagen fibers (with a normal half-life of 70 days) in arteries. Given that G&R of arteries in aging, aneurysms, atherosclerosis, and so forth depend strongly on the evolving relative roles of these constituents, a single phenomenological descriptor would not be expected to capture key aspects of the disease progression. Indeed, it appears that even normal residual stresses in arteries arise due to spatial heterogeneities in the different constituents that have different prestresses, hence a traditional kinematic growth model could only be expected to capture the overall change in size and shape when residual stress is relieved, not to predict how it arose or why it changes in disease.

3.2 Constrained mixture models

Whereas Skalak advocated the use of finite strain kinematics to describe growth, Fung suggested that G&R should be described in terms of mass-stress relations. Motivated by the latter as well as the desire to better incorporate mechanobiological data as they become available, so-called constrained mixture models were proposed for G&R of soft tissues [41]. These models represent a fundamentally different approach. It is assumed that, in each volume element, there exists a mixture of \(n\) structurally significant constituents. Given the aforementioned continuous turnover of most constituents, mass increments of each constituent \(i = 1,\,2, \ldots ,\,n\) are allowed to be deposited within the body at each time \(\tau \in [0,s]\). These increments possess different natural (stress-free) configurations and yet deform together with the overall tissue (i.e., in a constrained manner). Let mass increments of constituent \(i\) be incorporated within the extant matrix under the elastic pre-stretch \(\varvec{F}_{pre}^{i\left( \tau \right)}\) (relative to the stress-free configuration of the mass increment) at time \(\tau\). If the body subsequently deforms between time \(\tau\) and \(s\) such that the total mixture deformation gradient changes from \(\varvec{F}\left( \tau \right)\) to \(\varvec{F}\left( s \right)\), then the prestressed mass increment will experience at time \(s\) the elastic deformation gradient (cf. Fig. 2)

$$\varvec{F}_{e}^{i\left( \tau \right)} \left( s \right) = \varvec{F}\left( s \right)\varvec{F}^{ - 1} \left( \tau \right)\varvec{F}_{pre}^{i\left( \tau \right)} .$$
(8)

Obviously, this constituent-specific elastic stretch depends on the deposition time \(\tau\) and the pre-stretch of that constituent, and will in general be different for all mass increments within an infinitesimal volume element. Note that (8) allows a decomposition of the deformation of each mass increment into an elastic part \(\varvec{F}_{e}^{i\left( \tau \right)} \left( s \right)\) and an inelastic part \(\left( {\varvec{F}_{e}^{i\left( \tau \right)} \left( s \right)} \right)^{ - 1} \varvec{F}\left( s \right)\), similar to (2). One difference between kinematic growth theory and classical constrained mixture theory is, however, that in the latter in each volume element there is a mixture of different mass increments deposited at different times and experiencing an, in general, different elastic and inelastic deformation.

Let \(\varrho_{0}^{i} \left( s \right)\) be the constituent-specific (apparent) mass density per unit reference volume and \(\dot{\varrho }_{0 + }^{i} \left( s \right) > 0\) the associated true mass production rate per unit reference volume for the i-th constituent at any G&R time \(s \ge 0\). Once deposited, all biomolecules have a finite half-life and thus degrade over time. Let the fraction of the mass initially existing at time \(0\) and still surviving at time \(s\) be \(Q^{i} \left( s \right) \in \left[ {0,\,1} \right]\), with \(Q^{i} \left( 0 \right) = 1\), and similarly the fraction of mass deposited at time \(\tau\) and still surviving at time \(s\) be \(q^{i} \left( {s - \tau } \right) \in [0,\,1]\). Thus, directly from the mass balance relation for a mixture, the mass density of the i-th constituent in the constrained mixture, at G&R time \(s\), is

$$\varrho_{0}^{i} \left( s \right) = \varrho_{0}^{i} \left( 0 \right)Q^{i} \left( s \right) + \mathop \smallint \limits_{0}^{s} \dot{\varrho }_{0 + }^{i} \left( \tau \right)q^{i} \left( {s - \tau } \right)d\tau$$
(9)

which, when divided by the mass density of the whole tissue (i.e., constrained mixture) \(\varrho_{0} = \mathop \sum \limits_{i = 1}^{n} \varrho_{0}^{i}\) yields the evolving mass fraction for each constituent. The strain energy density of the constrained mixture of mass increments in each infinitesimal volume element is assumed, according to a simple rule of mixtures,

$$\Psi \left( s \right) = \mathop \sum \limits_{i = 1}^{n} \Psi^{i} \left( s \right)$$
(10)

where the strain energy density of the i-th constituent is typically assumed to be

$$\Psi^{i} \left( s \right) = \frac{{\varrho_{0}^{i} \left( 0 \right)}}{{ \varrho_{0} \left( s \right)}}Q^{i} \left( s \right)\hat{\Psi }^{i} \left( {\varvec{F}_{e}^{i\left( 0 \right)} \left( s \right)} \right) + \mathop \int \limits_{0}^{s} \frac{{\dot{\varrho }_{0 + }^{i} \left( \tau \right)}}{{ \varrho_{0} \left( s \right)}}q^{i} \left( {s - \tau } \right)\hat{\Psi }^{i} \left( {\varvec{F}_{e}^{i\left( \tau \right)} \left( s \right)} \right)d\tau$$
(11)

with some standard strain energy function \(\hat{\Psi }^{i}\) such as a Fung exponential function. This formulation reveals the need for three classes of constitutive relations: constituent-specific stored energy functions \(\hat{\Psi }^{i}\), rates of mass production, and survival functions, all depending on the specific time at which the constituent is incorporated within extant matrix.

Unlike in kinematic growth theory, in the constrained mixture theory net production rate \(\dot{\varrho }_{0}^{i}\) is understood as a difference between true production \(\dot{\varrho }_{0 + }^{i} > 0\) and degradation \(\dot{\varrho }_{0 - }^{i} > 0\) rates, with

$$\dot{\varrho }_{0}^{i} = \dot{\varrho }_{0 + }^{i} - \dot{\varrho }_{0 - }^{i} .$$
(12)

One often assumes a stress-dependent true mass production rate

$$\dot{\varrho }_{0 + }^{i} (\tau ) = \varrho_{0}^{i} \left[ {\frac{1}{{T^{i} }} + \varvec{k}_{\sigma }^{i} :\left( {\varvec{\sigma}\left( \tau \right) -\varvec{\sigma}_{h} } \right)} \right]$$
(13)

where \(\varvec{\sigma}_{h}\) is the (corotated) homeostatic Cauchy stress, \(T^{i}\) a time constant, and \(\varvec{k}_{\varvec{\sigma}}^{\varvec{i}}\) some second order tensor (containing gain-type parameters). The first term in the brackets on the right-hand side is called the basal mass production rate (i.e., the one in a homeostatic state) and the second term is the stress-dependent mass production rate. With (12) and (13) in a homeostatic state

$$\dot{\varrho }_{0 - }^{i} = \dot{\varrho }_{0 + }^{i} = \frac{{\varrho_{0}^{i} }}{{T^{i} }} ,$$
(14)

so that \(T^{i}\) can be interpreted as an average survival time of mass in a homeostatic state. With (12), (13), (14) and the often made assumption of a constant mass removal rate, we arrive at a net mass production rate

$$\dot{\varrho }_{0}^{i} \left( \tau \right) = \varrho_{0}^{i} \varvec{k}_{\sigma }^{i} :\left( {\varvec{\sigma}\left( \tau \right) -\varvec{\sigma}_{h} } \right),$$
(15)

which is similar to (7) found in kinematic growth theory with \(\Delta \varvec{G} =\varvec{\sigma}\left( \tau \right) -\varvec{\sigma}_{h}\).

In constrained mixture models, constituents such as collagen or smooth muscle are often represented by quasi-one-dimensional fiber families. In this case, Cauchy stress in (13) and (15) can simply be expressed by the scalar stress in the fiber direction and \(k_{\sigma }^{i}\) by a scalar gain factor. Moreover, in practice, exponential survival functions are often assumed, that is,

$$Q^{i} \left( s \right) = { \exp }\left( { - s/T^{i} } \right),\quad q^{i} \left( {s - \tau } \right) = { \exp }\left( { - (s - \tau )/T^{i} } \right).$$
(16)

For given gain factors \(\varvec{k}_{\varvec{\sigma}}^{\varvec{i}}\) and survival functions \(q^{i}\) and \(Q^{i}\), mass turnover is completely defined by (13) and (9). With a given mass turnover and deposition pre-stretch (often chosen to be constant), (10) and (11) define the mechanical behavior of soft tissue subject to growth and remodeling completely.

Unlike kinematic growth models, constrained mixture models naturally account for the simultaneous presence of multiple constituents in soft tissues and moreover for the differential turnover of these constituents in living organisms. They are based on a micromechanical model of G&R, which has helped to clarify several fundamental mechanisms of G&R (cf. Sect. 4). Nevertheless, these models require one to track a large number of different reference configurations. As can be seen from (8) to (11), the strain energy is calculated separately for each of the \(n\) constituents on the basis of an integral which, in practice, is evaluated in a discrete manner at \(n_{t}\) time points in the past. Thus, the configuration of the body has to be stored for each of these past times and a nonlinear strain energy function has to be evaluated for each of them in each time step. In general \(n_{t}\) is determined by the survival functions and time step size; in practice \(n_{t}\) will often range between 20 and 60. Both computational cost and implementation effort are therefore much higher for constrained mixture models than for kinematic growth models, which is the main disadvantage of constrained mixture approaches. For this reason, hybrid models have been developed that are as easy to implement as kinematic growth models but yet capture in some ways the effects of mass turnover as included in constrained mixture models.

3.3 Hybrid models

3.3.1 Evolving recruitment stretch models

In a series of papers published over the past decade [65–70], a hybrid approach to G&R was developed to study the enlargement of aneurysms. The tissue is modeled as a constrained mixture of \(n\) constituents with, in general, individual reference configurations. Unlike in the classical constrained mixture model [41], however, the mass of each constituent is not modeled as a constrained mixture of mass increments deposited at different times with different reference configurations. Rather only the change in the average stress-free configuration of each constituent due to increments of deposition and degradation of mass is tracked by the evolution of a “recruitment” stretch \(\lambda_{r}^{i} .\) This approach has been implemented for G&R of tissues containing quasi-one-dimensional fiber families so that a scalar recruitment stretch (in fiber direction) is sufficient. The general idea is that wavy fibers in a soft tissue start to carry load only once their initial undulations disappear at the recruitment stretch. A change of this recruitment stretch is thus equivalent to an inelastic remodeling deformation of the material, that is, to an inelastic deformation gradient

$$\varvec{F}_{r}^{i} = \lambda_{r}^{i} \varvec{A}^{\varvec{i}} \otimes \varvec{A}^{\varvec{i}} + \frac{1}{{\sqrt {\lambda_{r}^{i} } }}\left( {\varvec{I} - \varvec{A}^{\varvec{i}} \otimes \varvec{A}^{\varvec{i}} } \right)$$
(17)

with fiber direction \(\varvec{A}^{\varvec{i}}\). Evolution of the recruitment stretch is often modeled by a simple rate equation (e.g., Eq. (27) in [65])

$$\dot{\lambda }_{r}^{i} = \alpha \frac{{\left( {\lambda_{e}^{i} } \right)^{2} - \left( {\lambda_{pre}^{i} } \right)^{2} }}{{\left( {\lambda_{pre}^{i} } \right)^{2} - 1}} ,$$
(18)

where \(\lambda_{e}^{i}\) is the current elastic stretch of the i-th fiber family and \(\lambda_{pre}^{i}\) the prestretch (“attachment stretch”) with which new fibers are incorporated within the matrix during mass turnover (comparable to \(\varvec{F}_{pre}^{i\left( \tau \right)}\) in the classical constrained mixture model in Sect. 3.2). The idea is that degradation of extant fibers with elastic stretch \(\lambda_{e}^{i}\) and deposition of new fibers having an elastic pre-stretch \(\lambda_{pre}^{i}\) should make the average elastic stretch of a constituent approach the pre-stretch. This is realized by a \(\lambda_{r}^{i}\) evolving until \(\lambda_{e}^{i} = \left( {\lambda_{pre}^{i} } \right)\). For simplicity, this process is assumed to follow a first order rate equation determined by parameter \(\alpha\). At the same time, net mass production (that is, mass production minus mass degradation) is defined as (cf. Eq. (29) in [65])

$${\dot{\varrho }}_{0}^{i} = {\varrho }_{0}^{i} \frac{\beta }{{\left( {\lambda_{pre}^{i} } \right)^{2} - 1}}\left[ {\left( {\lambda_{e}^{i} } \right)^{2} - \left( {\lambda_{pre}^{i} } \right)^{2} } \right]$$
(19)

with some parameter \(\beta\). This relation is similar to the one usually assumed in constrained mixture models [cf. (15)], but based on stretch rather than stress. Models with evolving recruitment stretch were originally used in two-dimensional membrane models of blood vessels, but recently have been extended to volumetric growth [65]. There they are combined with a volumetric-deviatoric split to enforce incompressibility of the soft tissue during transient loading, which is equivalent to an assumed isotropic inelastic growth deformation accommodating an appropriate volume for the changing mass during growth. The inelastic growth deformation (gradient) is thus

$$\varvec{F}_{g}^{i} = \sqrt[3]{{\frac{{\mathop \sum \nolimits_{i = 1}^{n} {\varrho }_{0}^{i} \left( s \right)}}{{\mathop \sum \nolimits_{i = 1}^{n} {\varrho }_{0}^{i} \left( 0 \right)}}}}\varvec{I}.$$
(20)

In general, the inelastic deformation of a constituent can be written as

$$\varvec{F}_{gr}^{i} = \varvec{F}_{r}^{i} \varvec{F}_{g}^{i} ,$$
(21)

where the evolution of \(\varvec{F}_{r}^{i}\) is governed by (17) and (18). Similar to (2), one can thus decompose the total deformation gradient \(\varvec{F}\) into an elastic part \(\varvec{F}_{e}^{i}\) and an inelastic part \(\varvec{F}_{gr}^{i}\) with

$$\varvec{F} = \varvec{F}_{e}^{i} \varvec{F}_{gr}^{i}$$
(22)

with the strain energy determined at any G&R time by

$$W\left( s \right) = \mathop \sum \limits_{i = 1}^{n} W^{i} \left( {\varvec{F}_{e}^{i} \left( s \right)} \right).$$
(23)

This approach can be considered a hybrid. Similar to classical constrained mixture models, it uses the concept of a constrained mixture to account for the simultaneous presence of different constituents with different stress-free configurations. It also incorporates the idea of mass turnover and prestress (attachment stretch) for deposited mass. Yet, mass turnover is not accounted for by tracking mass increments deposited at each time. Rather, effects of mass turnover and growth are captured by an effective inelastic deformation for each constituent, whose evolution is governed by mass production and a rate equation that describes how the current stretch approaches the attachment stretch if, during turnover, extant mass is replaced by new mass deposited with the attachment stretch. Comparing (2) and (7) with (19)–(22) further reveals a conceptual similarity with kinematic growth theory. The advantage of this hybrid approach is its conceptual simplicity compared with classical constrained mixture models for G&R, as described in Sect. 3.2. Its disadvantage is its rather phenomenological basis. The rate Eq. (18) describes an evolution towards a homeostatic state that can be expected to resemble qualitatively the one produced by detailed micromechanical models, as in classical constrained mixture models. The choice of (18) is yet heuristic and it has not yet been shown how this equation corresponds to specific micromechanical assumptions about G&R processes. Moreover, this hybrid approach has been implemented so far only for quasi-one-dimensional fiber families or—in the context of volumetric growth–isotropic growth. Generalizations of these two aspects are pending.

3.3.2 Homogenized constrained mixture models

Recently, a temporally homogenized constrained mixture model has been proposed as another hybrid approach to model G&R of soft tissue [71]. It is motivated by the constrained mixture models discussed in Sect. 3.2. In constrained mixture models the total deformation gradient of each mass increment deposited at time \(\tau\) can be decomposed into an elastic part \(\varvec{F}_{e}^{i\left( \tau \right)}\) [cf. (8)] and an inelastic part \(\varvec{F}_{gr}^{i\left( \tau \right)}\) by

$$\varvec{F} = \varvec{F}_{e}^{i\left( \tau \right)} \varvec{F}_{gr}^{i\left( \tau \right)} ,$$
(24)

both of which depend explicitly on the time of constituent deposition and incorporation \(\tau \in [0,s]\) within the extant material. The main difficulty in the practical application of constrained mixture models is the implementation and evaluation of the time integral in (11). The origin of this difficulty is that all mass increments are deposited in general in different configurations with different \(\varvec{F}\left( \tau \right)\). The general idea of homogenized constrained mixture models is to perform a temporal homogenization across all mass increments within one constituent. This operation is performed on the basis of three assumptions. First, it is assumed that G&R changes the mechanical properties of a soft tissue, but the underlying strain energy function remains in the same so-called “similar set” in the sense of Definition 3 in [72], that is, differences in the average stress–strain response of each constituent caused by G&R can be captured via a single average inelastic deformation \(\varvec{F}_{gr}^{i}\) so that for each constituent the total deformation gradient can be decomposed into

$$\varvec{F} = \varvec{F}_{e}^{i} \varvec{F}_{gr}^{i} .$$
(25)

with elastic deformation gradient \(\varvec{F}_{e}^{i}\). Second, exponential survival functions as in (16) are assumed. Third, it is assumed that the inelastic deformation can be decomposed into a growth-related part \(\varvec{F}_{g}^{i}\), accommodating an appropriate volume for growth-based changes of mass, and a turnover-related part \(\varvec{F}_{r}^{i}\) with

$$\varvec{F}_{gr}^{i} = \varvec{F}_{r}^{i} \varvec{F}_{g}^{i} .$$
(26)

The turnover-based inelastic deformation is assumed to evolve such that the rate of change of the average Cauchy stress in a given configuration equals the one that would occur in a classical constrained mixture model following [41]. Under these simple assumptions, the turnover-based inelastic deformation was shown [71] to be governed by

$$\left[ {\frac{{\dot{\varrho }_{0}^{i} \left( t \right)}}{{\varrho_{0}^{i} \left( t \right)}} + \frac{1}{{T^{i} }}} \right]\left[ {\varvec{S}^{i} - \varvec{S}_{pre}^{i} } \right] = \left[ {\frac{{\partial \varvec{S}^{i} }}{{\partial \varvec{C}_{e}^{i} }}:\left[ {\varvec{C}_{e}^{i} \varvec{L}_{r}^{i} + \left( {\varvec{L}_{r}^{i} } \right)^{T} \varvec{C}_{e}^{i} } \right]} \right]_{{\varvec{F},\varvec{F}_{g}^{i} = const}}$$
(27)

with \(\varrho_{0}^{i}\) and \(T^{i}\) defined as in Sect. 3.2 for the classical constrained mixture models. Here, the second Piola–Kirchhoff stress and deposition prestress are denoted \(\varvec{S}^{i}\) and \(\varvec{S}_{pre}^{i}\), the inelastic velocity gradient \(\varvec{L}_{r}^{i} = \dot{\varvec{F}}_{r}^{i} \left( {\varvec{F}_{r}^{i} } \right)^{ - 1}\), and the elastic right Cauchy–Green deformation tensor \(\varvec{C}_{e}^{i} = \left( {\varvec{F}_{e}^{i} } \right)^{T} \varvec{F}_{e}^{i}\).

This temporally homogenized constrained mixture model was shown to converge in the neighborhood of a homeostatic state to the same solution as classical constrained mixture models and also to render far from these states at least very similar results [71]. Temporally homogenized constrained mixture models thus share the micromechanical basis of classical constrained mixture models while reducing computational cost and implementation effort to a level comparable to that of kinematic growth theory. In this way, these models combine advantages of the two thus far proposed major approaches to modeling G&R in load-bearing soft tissues.

4 Theoretical insights

Mathematical modeling of G&R in soft tissues has led to several non-trivial insights into the underlying mechanisms, which are briefly summarized in this section.

5 Further examples

The above discussed mathematical models have been used to study G&R in diverse soft tissues. In this section, we present a brief summary of illustrative examples.

5.1 Cardiovascular system

The vasculature adapts to altered mechanical loading via cell-mediated processes [12]. Smooth muscle cells and fibroblasts sense and respond to changes in their mechanical environment due to altered blood pressure and axial loads; endothelial cells similarly respond to changes in altered blood flow. Via complex signaling cascades, this sensing is translated into mechano-regulated G&R, which has been studied by kinematic growth models [60, 86, 87] and constrained mixture models [88–90]. In particular, the enlargement of aneurysms, focal pathological dilatations whose natural history may be linked to a form of unstable, ill-controlled G&R ("mechanobiological instability") [53], has attracted increasing interest over the last decade. Constrained mixture models [42, 74, 91–93] as well as hybrid models [65–67, 70, 71] have been used to examine the mechanisms driving aneurysmal enlargement (Fig. 5).

Fig. 5
figure 5

von Mises stress [kPa] in an aneurysm (right) that has grown in an initially healthy aorta (left) within 2700 days (reprinted from [94], copyright 2012, with permission from Elsevier)

Full size image

Not only blood vessels, but also the heart itself is subject to mechano-regulated G&R. For example, in [95] concentric and eccentric cardiac growth through sarcomerogenesis was studied, using anisotropic kinematic growth models.

Fig. 6
figure 6

Finite element simulation of temporal evolution of sarcomere stretch in skeletal muscle after stepwise permanent lengthening: elastic stretch is nearly completely released by sarcomerogenesis between day 0 and 14 (reprinted from [96] under Creative Commons License [97])

Full size image

5.2 Skeletal muscle

Skeletal muscle is well-known to grow by increasing the number of sarcomeres in cases of chronic lengthening. This process was studied by finite element simulations using a kinematic growth model in [96] (Fig. 6).

5.3 Skin

Mechanical loading can make skin expand by G&R. This mechanism can be exploited in plastic and reconstructive surgery to grow in a controlled process in one region of the body additional skin that can be used to compensate for skin losses in other regions. Anisotropic kinematic growth models have been developed [98] to study this process computationally (Fig. 7).

Fig. 7
figure 7

Finite element simulation of skin growth in pediatric scalp reconstruction using a kinematic growth model (reprinted from [98], copyright 2013, with permission from Elsevier)

Full size image

5.4 Eye

Soft tissue G&R in the eye may play important roles in diseases like glaucoma, and has thus been studied by kinematic growth models [99, 100]. In particular, mechano-regulated thickening of the lamina cribrosa in early glaucoma was modeled and reorientation of collagen fibers in the corneo-scleral shell (Fig. 8).

Fig. 8
figure 8

Shown is a G&R simulation of early thickening in glaucoma of the lamina cribrosa, a porous collagen structure through which axons pass. Such models can help explain experimental findings, as noted in the original paper (reprinted from [100], copyright 2012, with permission from Elsevier)

Full size image

5.5 Tissue cultures and tissue engineering

Collagen gels seeded with fibroblasts are important model systems to study fundamental mechanisms in soft tissue G&R [25, 40, 101]. To understand better the experimental observations made in such gels kept in tissue cultures, computational models of G&R have been increasingly used over the last decade [62, 102]. Similarly, such models [103–107] have been successfully applied to help tissue engineer artificial blood vessels and heart valves (Fig. 9).

Fig. 9
figure 9

Finite element simulation of radial strain in tissue engineered heart valves after load-driven remodeling (reprinted from [107], DOI 10.1016/j.jmbbm.2015.10.001, under Creative Commons license [97])

Full size image

6 Conclusions

Load-bearing collagenous soft tissues exhibit complex mechanical behaviors, but also a remarkable ability to adapt to perturbations in mechanical loading. The past two decades have seen significantly increased attention in the biomechanics community to mathematically modeling the associated growth (changes in mass) and remodeling (changes in structure). Because of the finite deformations involved, the kinematics of G&R necessarily includes multiplicative decompositions into elastic and inelastic deformations, independent of approach. Kinematic growth theory provides a computationally inexpensive means to capture consequences of G&R, though without detail on the mechanobiological processes of turnover of individual constituents having individual properties. Another approach, that of a constrained mixture, can capture many aspects of chemomechanically stimulated remodeling and turnover of individual matrix constituents, but requires a computationally expensive tracking of a multitude of reference configurations for each constituent. Hybrid approaches attempt to exploit advantages of kinematic theories while retaining some features of constrained mixture theories, though with the loss of some generality in describing actual history-dependent mechanobiological processes. As is the case with most constitutive formulations, the method chosen should be based upon the question at hand with an appreciation of the associated limitations. New concepts such as mechanobiological stability and adaptivity promise to yield increasing insight into principles of G&R, but much remains to be accomplished. Continued studies should be motivated directly by the unique mechanisms that cells use to establish, maintain, remodel, repair, or remove functional tissue and organs, including cell-mediated incorporation of new matrix under stress.