Introduction

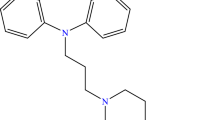
Ambuphylline is a diuretic and a muscle relaxant used mostly as a bronchodilator in association with other drugs for asthma treatment [1]. It is synthesized by the combination of theophylline and 2-amino-2-methyl-1-propanol (aminoisobutanol); its structure is shown in Fig. 1.

Fig. 1
figure 1

Molecular structure of ambuphylline

Full size image

Thermal analysis is routinely used for characterization of drugs and substances of pharmaceutical interest since the properties such as stability, compatibility, polymorphism and phase transitions can be evaluated. Kinetic studies of thermal degradation of pharmaceuticals can be used in the quality control for developing safe chemical manufacturing processes and assessing the stability of chemicals under various conditions: chemical process, storage and transportation. Several studies on thermal decomposition kinetics of organic compounds are reported [2–5]; however, information on ambuphylline thermal stability is missing.

The aim of this paper was to study the kinetics of thermal degradation of ambuphylline by thermogravimetry (TG) and differential scanning calorimetry (DSC). The kinetic data are analyzed by employing a recently developed incremental isoconversional method for kinetic analysis based on the orthogonal distance regression [6] applied for the Arrhenius and Berthelot–Hood temperature functions.

Theory

Condensed state processes are extensively studied by thermoanalytical methods. Mechanisms of these processes are very often unknown or too complicated to be characterized by a simple kinetic model. To describe their kinetics, methods based on the general rate equation are often used [7, 8]. The general rate equation expresses the rate of the processes in condensed state as

$$ \frac{{{\text{d}}\alpha }}{{{\text{d}}t}} = k\left( T \right)f\left( \alpha \right), $$
(1)

where α is the degree of conversion, t is time, T is temperature, k(T) is a temperature function depending solely on temperature T and f(α) is a conversion function depending solely on the degree of conversion of the process.

The temperature function is mostly expressed by the Arrhenius equation

$$ k(T) = A_{\text{A}}^{\prime } \exp \left( { - {\raise0.7ex\hbox{$B$} \!\mathord{\left/ {\vphantom {B T}}\right.\kern-0pt} \!\lower0.7ex\hbox{$T$}}} \right), $$
(2)

where \( A_{\text{A}}^{\prime } \) is the apparent pre-exponential factor, T is the absolute temperature and B = E/R is a ratio of the apparent activation energy and the gas constant.

In Refs. [7–9], it has been justified that, since k(T) is not the rate constant, there is no reason to be confined to the Arrhenius relationship and the use of two non-Arrhenian temperature functions was suggested, among them the Berthelot–Hood equation:

$$ k(T) = A_{\text{BH}}^{\prime } \exp (DT), $$
(3)

where \( A_{\text{BH}}^{\prime } \) and D stand for adjustable parameters.

In the incremental isoconversional methods, the conversion axis is divided into n increments by n + 1 points, and the first point is α 0 = 0 at t 0 = 0 (or at T 0 = 0). The increments may not be necessarily of the same length; however, they should be short enough so the kinetic parameters could be considered constant.

Separation of variables in Eq. (1) and integration over the ith conversion increment leads to the result

$$ \int\limits_{{\alpha_{{\text{i}} - 1} }}^{{\alpha_{{\text{i}}} }} {\frac{{{\text{d}}\alpha }}{f(\alpha )} = } \int\limits_{{t_{{\text{i}} - 1} }}^{{t_{{\text{i}}} }} {k(T){\text{d}}t} $$
(4)

If one denotes the primitive function of the inverted conversion function, 1/f(α), as F(α), it is then obtained

$$ 1 = \frac{1}{{F\left( {\alpha_{{\text{i}}} } \right) - F\left( {\alpha_{{\text{i}} - 1} } \right)}}\int\limits_{{t_{{\text{i}} - 1} }}^{{t_{{\text{i}}} }} {k(T){\text{d}}t} $$
(5)

Equation (4) is general and can be applied for any time/temperature regime. For a constant temperature, the temperature function is constant and one can get:

$$ t_{{\text{i}}} - t_{{\text{i}} - 1} = \Delta t_{{\text{i}}} = \frac{{F\left( {\alpha_{{\text{i}}} } \right) - F\left( {\alpha_{{\text{i}} - 1} } \right)}}{k(T)} $$
(6)

The time difference Δt i represents the time elapsed to change the conversion from α i−1 to α i at the constant temperature. Combination of Eq. (5) with Eqs. (2) or (3) provides:

$$ \Delta t_{{\text{i}}} = A_{\text{A}} \exp ({B \mathord{\left/ {\vphantom {B T}} \right. \kern-0pt} T}) $$
(7)
$$ \Delta t_{{\text{i}}} = A_{\text{BH}} \exp ( - DT) $$
(8)

The pre-exponential factors for the Arrhenius and Berthelot–Hood equation are defined as

$$ A_{{\text{k}}} = \frac{{F(\alpha_{{\text{i}}} ) - F(\alpha_{{\text{i}} - 1} )}}{{A_{{\text{k}}}^{\prime } }} $$
(9)

It is a matter of course that the kinetic parameters for individual increments may differ. The time to reach the final conversion, t α , is calculated as

$$ t_{\upalpha } = \sum\limits_{{{i}} = 1}^{n} {\Delta t_{{\text{i}}} } $$
(10)

The kinetic parameters A A, B or A BH, D, respectively, can be obtained from the measurements with linear heating from the treatment of experimental data by applying Eqs. (11) and (12):

$$ \beta = \int\limits_{{T_{{\text{i}} - 1} }}^{{T_{{\text{i}}} }} {\frac{{{\text{d}}T}}{{A_{\text{A}} \exp (B/T)}}} $$
(11)
$$ T_{{\text{i}}} - \frac{1}{D}\ln \left( {A_{\text{BH}} D\beta + e^{{\text{{DT}}_{{\text{i}} - 1} }} } \right) $$
(12)

where β is the heating rate. As seen from Eq. (12), a great advantage of the application of the Berthelot–Hood temperature function is that the temperature integral can be expressed in a closed form. A detailed derivation of Eqs. (4)–(12) is given in Ref. [10].

Experimental

TG/DTG curves of ambuphylline were obtained using a thermobalance TGA-51 (Shimadzu, Tokyo, Japan) in the temperature range 25–500 °C using platinum crucibles with samples of approximately 16 mg. The measurements were taken under dynamic air atmosphere with flow rate of 50 mL min−1. The heating rates (β) applied were 2.5, 5, 7.5, 10, 15 and 20 °C min−1. The temperature calibration was verified using the magnetic patterns of alumel reference material (T Curie = 154.2 °C) and nickel reference material (T Curie = 355.3 °C), and the mass axis was checked using a standard CaC2O4·H2O which exhibits three distinct mass losses.

Differential scanning calorimetry curve was obtained using DSC-50 (Shimadzu, Tokyo, Japan) using aluminum crucibles with approximately 2 mg of the sample. The purge gas used was dry nitrogen with flow rate of 100 mL min−1. The heating rate was 10 °C min−1 in the temperature range of 25–500 °C. The DSC cell was calibrated using indium.

Results and discussion

TG/DTG and DSC curves of the ambuphylline decomposition are shown in Fig. 2. As seen, ambuphylline decomposes in two steps that are clearly separated. The first step corresponds to the liberation of aminoisobutanol; the second one represents sublimation and evaporation of theophylline. The observed mass loss in the first decomposition step (32.8 %) is in a good agreement with theoretical value (33.1 %). The assignment of ambuphylline decomposition steps was also verified on the basis of a DSC run shown in Fig. 2. The first endothermic peak reaches maximum at 132 °C, and the signal abruptly returns to the baseline which is a frequently observed phenomenon in the case of evaporation and solvate decomposition. The onset of the second endothermic peak (271.3 °C) agrees well with literature data for the melting point of theophylline (271–273 °C). The comparison of the DSC and TG records suggests that theophylline exhibits a considerable vapor pressure near its melting point, as already reported by Schnitzler [11].

Fig. 2
figure 2

DSC and TG record of ambuphylline obtained at the heating rate 10 °C min−1

Full size image

Figure 3 shows TG curves of ambuphylline obtained at different heating rates. The increase in heating rate did not change the profile of the TG curves; they just were shifted to higher temperatures. From the practical point of view of the drug stability, the first step is decisive so that this step was subjected to the kinetic analysis. The isoconversional temperatures obtained from curves in Fig. 3 for the first decomposition stage are listed in Table 1. For the evaluation of the kinetic parameters, an incremental isoconversional method has been employed. The reasons for employing the incremental method were as follows: (1) For the integral methods, which are most popular, it has been proved in our recent paper [12] that they are mathematically incorrect in the case of variable activation energy. As a matter of fact, the variable activation energy is reported in most thermoanalytical kinetic studies so that it is advisable to avoid the integral methods. (2) Considering the differential methods, they are known to be very sensitive to noise and tend to be numerically unstable [9, 13]. So, the best compromise for the treatment of kinetic data is to employ the incremental methods.

Fig. 3
figure 3

TG curves of the ambuphylline decomposition. Heating rates from left to right: 2.5, 5, 7.5, 10, 15, and 20 °C min−1

Full size image
Table 1 Isoconversional temperatures at different heating rates
Full size table

For calculating the kinetic parameters of the Arrhenius and Berthelot–Hood temperature functions, the newly developed incremental isoconversional method for kinetic analysis based on the orthogonal distance regression has been employed. The mathematical fundamentals are presented in our papers [6, 10]; the program code is published as a supplementary material to paper [6]. The kinetic parameters resulting from the kinetic analysis by the incremental methods based on the Arrhenius and Berthelot–Hood temperature functions are listed in Tables 2 and 3. From the values of the kinetic parameters, it is seen that the kinetic data exhibit a strong linear correlation between ln A A and B or ln A BH and D, respectively; the correlation coefficient is close to ±1.

Table 2 Arrhenius kinetic parameters and their standard errors (incremental method, orthogonal regression)
Full size table
Table 3 Berthelot–Hood kinetic parameters and their standard errors (incremental method, orthogonal regression)
Full size table

The kinetic parameters obtained from non-isothermal data enable to calculate the conversion versus time curves for chosen constant temperatures by applying Eqs. (7) or (8). First, the α(t) curves were calculated at 110, 120, 130 and 140 °C (see Fig. 4), which represent the temperatures from the region of measurement. Here, the results based on the Arrhenius and Berthelot–Hood temperature functions provided practically identical curves since the both temperature functions are equivalent in the region of measurement [10]. The conversion curves extrapolated for the temperatures 20–60 °C are shown in Fig. 5 calculated for the kinetic parameters of the Berthelot–Hood temperature function (Table 3). In the temperature range of measurement, the uncertainties of isoconversional times were negligible; however, for the extrapolated data outside the temperature range of measurement, this was not the case; hence, the standard errors of the isothermal isoconversional times were evaluated using an error-propagation law from the standard errors of the kinetic parameters:

$$ {\text{SE}}\left( {\Delta t_{{\text{i}}} } \right) = \Delta t_{{\text{i}}} \left| {{\text{SE}}\left( {\ln A_{{{\text{BH,}}{\text{i}}}} } \right) - {\text{SE}}\left( {D_{{\text{i}}} } \right) \cdot \left. T \right|} \right.. $$
(13)

The standard errors of the kinetic parameters were obtained from the covariance matrix of the regression model [6]. To estimate a total error of the isoconversional time, SE(t α ), the standard errors of individual increments, SE(Δt i), were added in quadrature.

Fig. 4
figure 4

Calculated dependences of the conversion on time (Berthelot–Hood model) within the temperature range of the measurement

Full size image

We chose the Berthelot–Hood temperature function for calculating the conversion versus time curves since it has been documented that this temperature function gives extrapolations corresponding to reality; the Arrhenius temperature function tends to overestimate the isoconversional times [14]. The isoconversional times calculated from the Arrhenius parameters are about twice of those calculated from the Berthelot–Hood kinetic parameters. From Fig. 5, it can be seen that, for temperatures close to the ambient one, the ambuphylline degradation exhibits an induction period, i.e., the period where no process is detected so that seemingly no reaction takes place. As a matter of fact, it is a preparatory stage for the main decomposition process. From Fig. 5, it is seen that after finishing the induction period, the decomposition proceeds rapidly. From the curves, conversion versus time, the model function can be assessed without any construction of master plots.

Fig. 5
figure 5

Extrapolated low-temperature dependences of the conversion on time (Berthelot–Hood model). Error bars correspond to ±standard error of the isoconversional time

Full size image

It should be taken into account that the conversion versus time curves are extrapolated for the conditions under which the experiment was carried out, i.e., thin layer of the sample and continuous removal of the reaction product. The estimated isoconversional times in the solid state and in the equilibrium atmosphere of the product (in a blister or in a vial) will be much longer. In such a case, Eq. (1) has to involve also the pressure term, h(p) [13]:

$$ \frac{{{\text{d}}\alpha }}{{{\text{d}}t}} = k\left( T \right)f\left( \alpha \right)h\left( p \right) $$
(14)

The pressure term can be expressed as

$$ h\left( p \right) = 1 - \frac{p}{{p_{\text{eq}} }} $$
(15)

where p and p eq are the actual and equilibrium pressures of the product, respectively. Hence, Eqs. (14) and (15) follow that, in a closed system, the rate of ambuphylline decomposition approaches zero when the pressure of aminoisobutanol reaches its equilibrium value.

Conclusions

Thermal decomposition of ambuphylline proceeds in two well-separated stages. The first stage, liberation of aminoisobutanol, was subjected to isoconversional kinetic analysis. It was found that the decomposition exhibits an induction period; after its finishing, the decomposition proceeds rapidly. From the curves, conversion versus time, the model function can be assessed without any construction of master plots.