Magnetic, magnetocaloric properties, and critical behavior in a layered perovskite La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇

Abstract

We report the results of magnetic, magnetocaloric properties, and critical behavior investigation of the double-layered perovskite manganite La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇. The compounds exhibits a paramagnetic (PM) to ferromagnetic (FM) transition at the Curie temperature T _C = 248 K, a Neel transition at T _N = 180 K, and a spin glass behavior below 150 K. To probe the magnetic interactions responsible for the magnetic transitions, we performed a critical exponent analysis in the vicinity of the FM–PM transition range. Magnetic entropy change (−∆S _M) was estimated from isothermal magnetization data. The critical exponents β and γ, determined by analyzing the Arrott plots, are found to be T _C = 248 K, β = 0.594, γ = 1.048, and δ = 2.764. These values for the critical exponents are close to the mean-field values. In order to estimate the spontaneous magnetization M _S(T) at a given temperature, we use a process based on the analysis, in the mean-field theory, of the magnetic entropy change (−∆S _M) versus the magnetization data. An excellent agreement is found between the spontaneous magnetization determined from the entropy change [(−∆S _M) vs. M ²] and the classical extrapolation from the Arrott curves (µ₀H/M vs. M ²), thus confirming that the magnetic entropy is a valid approach to estimate the spontaneous magnetization in this system and in other compounds as well.

Introduction

In recent years, the magnetocaloric effect (MCE) and electrocaloric effect (ECE) have become a promising technology because of their potential advantages over the conventional gas compression refrigeration, particularly for low energy consumption, higher efficiency, low capital cost, and not using hazardous chemicals or global-warming gases [1–8]. The MCE is known to be one of the most interesting properties and has been studied for the magnetic material with the aim of suppressing the emission of pollution components. It is an intrinsic to all magnetic materials and is due to the coupling of the atomic magnetic moments with the magnetic applied field which can produce a large entropy variation [9, 10]. So the purpose of using the MCE is to seek the proper material whose transition temperature is near room temperature of the magnetocaloric materials [11, 12]. The ECE, which is associated with the temperature dependence of the macroscopic polarization under an electric field, has been spasmodically studied in ferroelectric materials in order to find an alternative to classical refrigerators using Freon [13, 14].

In the past few years, many studies have revealed that double-layered perovskite manganite RE_2−2x M_1+2x Mn₂O₇ (RE is a trivalent rare-earth cation like Pr, Nd or La, and M is a divalent alkaline earth cation like Ba, Sr, or Ca) are the ones the of most fascinating materials in the condensed matter research and exhibit intriguing features, like the notable colossal magnetoresistance (CMR) and magnetocaloric effect (MCE), due to their fascinating properties related to the ferromagnetic (FM)-paramagnetic (PM) transition [15–19]. The structure of the double-layered manganite is a stack of ferromagnetic metal sheets composed of MnO₂ bilayers separated by a rock salt nonmagnetic insulating layer (RE, M)₂O₂. Thus the RE and M ions are located in both the MnO₂ bilayers and in the rock salt layer, and their distribution is dependent on the dopant ion size.

Many previous reports were dedicated that layered manganite has a rich phase diagram which had a strong dependence on magneto-transport properties. Basically, we still believe that the origin of the ferromagnetic (FM) phase and metallicity is related to the double-exchange (DE) interactions which implies a ferromagnetic pairing between Mn³⁺($t_{2g}^{3}$↑$e_{g}^{1}$↑, S = 2) and Mn⁴⁺($t_{2g}^{3}$↑$e_{g}^{0}$, S = 3/2) ions and antiferromagnetic (AFM) super-exchange (SE) interactions of Mn³⁺–O²⁻–Mn³⁺ and Mn⁴⁺–O²⁻–Mn⁴⁺ pairs [20, 21]. In the DE interaction, the electron hops from Mn³⁺ to O²⁻, accompanied by a simultaneous hop from the latter to Mn⁴⁺. The probability of this DE electron transfer of an e.g., electron depends on the orientation of the neighboring intra-atomic Hund coupled t _2g spins. The probability of hopping is also strongly dependent on the structural parameters such as Mn–O–Mn bond angle and Mn³⁺, Mn⁴⁺ concentrations. However, metallicity and ferromagnetism are well explained by the DE interaction, which could not adequately explain the paramagnetic insulating phase occurring at high temperature. The presence of a paramagnetic phase is explained by the concept that the mobile electron carries with it the Jahn–Teller distorsion of the MnO₆ octahedron [22]. The greater distortion results in more localized charge carriers. This deformation disappears in the metallic state below the ferromagnetic transition.

To get a clear idea about the performance of materials used in magnetic refrigeration devices, it is necessary to understand how their MCE evolves in desired temperature and magnetic field ranges. A detailed analysis of ΔS _M (T, ΔH) data provides the important information about magnetocaloric properties of materials. Recently, the MCE in La_2−2x Sr_1+2x Mn₂O₇ compounds has been studied upon the magnetic entropy change (ΔS _M) [23, 24]. Also, these materials exhibit large (ΔS _M) under a moderate magnetic field [25–28]. High (ΔS _M) at low magnetic field changes and tunable magnetic phase transition properties make these materials good candidates as working elements in magnetic refrigeration applications.

In this work, we present a detailed of the magnetic, magnetocaloric effect, and critical behavior for La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇ polycrystalline sample. We have also used the phenomenological model to predict the values of the magnetocaloric properties (such as relative cooling power (RCP), heat capacity change, and entropy change) from calculation of magnetization as a function of temperature under different external magnetic fields.

Experiment

Polycrystalline La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇ was synthesized by the sol–gel method using metal nitrates as starting materials [29]. Identification of the crystalline phase and structural analysis were carried out using “PANalytical X’Pert Pro” diffractometer with filtered (Ni filter) Cu radiation (λ _{Cu Kα1} = 1.54056 Å). The data were analyzed by the Rietveld method.

The magnetization and susceptibility measurements versus temperature (T) and versus magnetic field (µ ₀ H) were made by BS2 magnetometers developed in Louis Neel Laboratory at Grenoble. The DC magnetic properties were carried out in both zero-field-cooled (ZFC) and field-cooled (FC) modes over a temperature range of 5–300 K with an applied magnetic field of 0.05 T. The isothermal M(µ ₀ H) data were measured under a magnetic field varying from 0 to 5 T at several temperatures (T).

Results and discussion

Structural characterization

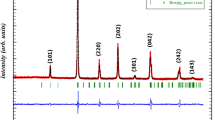
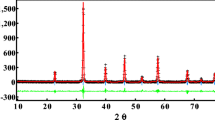
Identification of the phase and structural analysis by X-ray diffraction were reported elsewhere [29]. The Rietveld refinement at room temperature revealed the presence of single phase with a tetragonal structure (I4/mmm). The lattice parameters of the perovskite phase are a = b = 3.8714 Å, c = 19.3267 Å, and a cell volume of 289.66 Å³.

Figure 1 shows the unit cell structure of tetragonal double perovskites.

Figure 1

The unit cell structure of tetragonal double perovskites

Magnetic properties

Temperature dependence of the magnetization M(T) in zero-field-cooling (ZFC) and field-cooling (FC) modes recorded in the temperature range of 5–300 K under a magnetic field of 0.05 T are presented in Fig. 2.

Figure 2

Temperature dependence of FC and ZFC magnetizations for La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇ sample measured under low magnetic field of 0.05 T. Inset shows the magnetization derivate as a function of temperature curve for determining T _C

The M(T) curves clearly indicate that La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇ passes through three magnetic transitions: the first one is a sharp transition at higher temperatures resembling a paramagnetic (PM) to ferromagnetic (FM) transition where the Curie temperature T _C, is obtained from the inflexion point of dM/dT versus T curve, is found to be 248 K (inset of Fig. 2). This value is in accordance with those reported in the literature [28, 30].

The other one at T _N = 180 K which has been attributed to an antiferromagnetic Neel transition, and a distinct divergence of field-cooled (FC) and zero-field-cooled (ZFC) magnetization was observed below 150 K. This is also ascribed to the existence of the spin glass (SG) behavior present in this double-layered manganite phase. Similar behavior was reported in some other double-layered manganite [31]. This irreversibility arises possibly due to the canted nature of the spins or due to the random freezing of spins as observed in systems with FM cluster embedded in AFM matrix [32, 33]. In detail, in the absence of the applied field, the bilayers are stacked antiferromagnetically while within them the manganese moments are arranged ferromagnetically. However, when the magnetic field is applied, these moments remain ferromagnetically ordered (in spite of the rotation through 90° from the zero'-field direction), whereas the bilayers are stacked ferromagnetically and therefore the FM order is restored [34]. The spin glass state can be attributed to the coexistence of random competing ferromagnetic (FM) double-exchange involving the itinerant e.g., electrons and antiferromagnetic (AFM) super-exchange involving localized t ₂ g electrons together with the anisotropy originating from the layered structure [35]. In fact, the substitution of divalent ions (Sr²⁺ and Ca²⁺) for trivalent rare-earth ions (La³⁺) introduces excess electrons in the lattice. The excess electrons in the compounds enable the coexistence of Mn³⁺ and Mn⁴⁺ ions; as a result, the (FM) double-exchange interaction overcomes the inherent (AFM) super-exchange interaction. Furthermore, the electron in the itinerant e.g., state in Mn⁴⁺ increases the Mn–O orbital overlap, which increases the size of the super-exchange interaction and favors an antiferromagnetic state. Consequently, this competition between the super-exchange and double-exchange present in La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇ gives rise to the spin glass behavior [33, 36].

Figure 3 shows the temperature dependence of the inverse magnetic susceptibility χ ⁻¹ deduced from M(T) curve. Above T _C, a sharp transition with a linear relation was observed and below T _C a clear deviation can be attributed to the antiferromagnetic transition (inset of Fig. 3).

Figure 3

Variation of the inverse of the magnetic susceptibility as a function of temperature for. The red solid line is the best fit using Curie–Weiss law in the paramagnetic range (T > T _C). Inset shows a zoom of the antiferromagnetic transition

In the paramagnetic region (above T _C), the susceptibility obeys the Curie–Weiss law:

$$ \chi = \frac{C}{{T - \theta_{\text{cw}} }} $$

where

• θ _cw is the Curie–Weiss temperature.

• C is the Curie constant defined as: $C = \left( {\frac{{N_{\text{a}} }}{{3k_{\text{B}} M_{\text{m}} }}} \right)\mu_{{{\text{ef}}f}}^{2\exp }$.

With N _a is Avogadro number, M _m is the molecular mass per unit formula, k _B is Boltzmann constant, and μ ^exp_eff is the experimental effective moment expressed in Bohr magneton.

From the linear fit of the paramagnetic part (T > T _C), we determined the values of θ _cw and C which are, respectively, the intercept of the line with the temperature axis (θ _cw = 252 K) and the inverse of the slope of the straight line (C = 63.13 emu. K/g T). The positive value of θ _cw indicates the ferromagnetic interaction between spins.

The theoretical paramagnetic effective moment should be

$$ \mu_{\text{eff}}^{\text{th}} = \sqrt {x\mu_{\text{eff}}^{2} \left( {{\text{Mn}}^{3 + } } \right) + y\mu_{\text{eff}}^{2} \left( {{\text{Mn}}^{4 + } } \right)}. $$

(1)

here $\mu_{\text{eff}}^{\text{th}}$(Mn³⁺) = 4.9 μ _B , $\mu_{\text{eff}}^{\text{th}}$(Mn⁴⁺) = 3.87 μ _B and x = 0.7, y = 0.3 were determined using the same reasoning as Zhou et al. [37] (The ratio of Mn³⁺ to Mn⁴⁺ is the same for La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇ and La_0.7(SrCa)_0.3MnO₃).

It can be deduced that the experimental effective moment ($\mu_{\text{eff}}^{ \exp }$ = 6.24 μ _B) is slightly larger than the expected theoretical value ($\mu_{\text{eff}}^{\text{th}}$ = 4.61 μ _B) and the value obtained for θ _cw is higher than that of T _C. This difference confirms the presence of a magnetic inhomogeneity resulting in short range ordered slightly above T _C.

For an accurate description of the low temperature behavior of magnetic properties and in order to calculate the exchange constant J _exc, the spin wave theory can be used. In the case of long wavelength, the temperature dependence of the magnetization is given by [38].

$$ M\left( {T,\mu_{0} H} \right) = M\left( {0,\mu_{0} H} \right) * \left( {1 - BT^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-0pt} 2}}} - CT^{{{5 \mathord{\left/ {\vphantom {5 2}} \right. \kern-0pt} 2}}} } \right), $$

(2)

where M(0, µ ₀ H) and M(T, µ ₀ H) are the saturation magnetization values at 0 K and T K, respectively; B and C are the characteristic constants of the long wavelength spin wave at low temperature. However, for a bulk ferromagnetic system, the temperature thermal evolution of magnetization M below the Curie temperature follows Bloch’s T ^3/2 law of the form [39].

$$ M\left( {T,\mu_{0} H} \right) = M\left( {0,\mu_{0} H} \right) * \left( {1 - BT^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-0pt} 2}}} } \right), $$

(3)

where the pre-factor B is the Bloch constant of the spin waves that depends on the structure of the material and was obtained by fitting Eq. (3) to the experimental magnetization curves (Fig. 4) at low temperature range. This coefficient is given by the following relation [40].

$$ B = 2.612\left( {\frac{{g\mu_{\text{B}} }}{M\left( 0 \right)}} \right) * \left( {\frac{{k_{\text{B}} }}{4\pi D}} \right)^{{{\raise0.7ex\hbox{$3$} \!\mathord{\left/ {\vphantom {3 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}}}. $$

(4)

Figure 4

Plot of M(T, µ ₀ H)/M(0, µ ₀ H) versus T ^3/2 for La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇. The inset represents the variation of Ln [1−M(T, µ ₀ H)/M(0, µ ₀ H)] versus Ln(T) in order to determine the slope

In this expression, k _B is the Boltzmann constant, g is the Lande factor (g = 2), µ _B is the Bohr magnetron, and D is the spin wave stiffness constant.

This stiffness D is defined by spin wave dispersion relation [41]:

$$ E\left( q \right) = \Delta + Dq^{2}. $$

(5)

The above relation is valid for q → 0. Here, q is the momentum wave vector, Δ is the gap arising from anisotropy or applied magnetic field µ ₀ H, and E is the spin wave energy. In our analysis we assume Δ = 0.

Figure 4 shows the reduced magnetization M(T, µ ₀ H)/M(0, µ ₀ H) as a function of T ^3/2 for µ ₀ H = 0.05 T.

Where M(0, µ ₀ H) was obtained by extrapolating M(T, µ ₀ H) curves to T = 0 using a second-order polynomial. From this figure, we determine the spin-stiffness constant D according to the value of B which is the slope of the linear fit of this curve. The B and D values are listed in Table 1 and are in an excellent agreement with those reported for other manganite [42].

Table 1 Some magnetic parameter for La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇ compound

The inset of Fig. 4 shows the curve Ln(1 − (M(T, μ ₀ H)/M(0, μ ₀ H))) versus Ln(T) which is used to verify that our compound obey Bloch’s T ^3/2. In this theory, the slope of the linear fit of this curve must be close to 3/2. In our sample, we found that the slope is 1.38; therefore, we must note that Bloch’s T ^3/2 law is valid for T < 100 K.

The factor D is closely related to the degree of ferromagnetic exchange interaction (J _exc) as given in Eq. (6).

$$ J_{\text{exc}} = \frac{M\left( 0 \right)D}{{2g\mu_{\text{B}} }}. $$

(6)

The obtained parameter J _exc is listed in Table 1.

On the other hand, D and the Curie temperature T _C are linked by the following equation according to the Heisenberg model.

$$ D = \frac{{k_{\text{B}} r_{ij}^{2} T_{\text{C}} }}{{2\left( {S_{\text{Mn}} + 1} \right)}}, $$

(7)

where r _ij is the distance between nearest magnetic atoms (Mn) and S _Mn is the average spin moment between Mn³⁺ and Mn⁴⁺ atoms:

$$ S_{\text{Mn}} = x_{{{\text{Mn}}^{3 + } }} S({\text{Mn}}^{3 + } ) + y_{{{\text{Mn}}^{4 + } }} S({\text{Mn}}^{4 + } ) \,\,{\rm Here}\,\, S\left( {{\text{Mn}}^{3 + } } \right) = 2; S\left( {{\text{Mn}}^{4 + } } \right) = \frac{3}{2} $$

Katsuki and Wolhfarth [43] have discussed the correlation between D and the T _C on the basis of itinerant electron model. Using the effective mass approximation, they have obtained the following relationship:

$$ D = \frac{{\pi k_{\text{B}} T_{\text{C}} }}{{6\sqrt 2 k_{\text{F}}^{2} }}, $$

(8)

where k _F is the Fermi wave vector obtained in Table 1.

Magnetization as a function of applied magnetic field M(µ ₀ H) of La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇ has been performed at 5 K in magnetic fields strengths of the range of ±10 T and is displayed in the inset of Fig. 5. The Fig. 5 represents a zoom of the hysteresis loop at a low field and moderate values of the remenant magnetization and the coercive field. Clear hysteresis loops was observed in these curves and it can be seen that the magnetization increases sharply with the applied field for µ ₀ H < 0.4 T indicating the presence of a ferromagnetic state. After that, this magnetization increases almost linearly with increasing field value due to the presence of spin glass behavior. This phenomenon is based on the competition of AFM domains with FM ones. The magnetic hysteresis loop is not saturated at 5 T due to antiferromagnetic matrix.

Figure 5

The magnetic hysteresis loops at 5 K of the La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇ compound. The inset shows a zoom of the hysteresis loops at low field

Figure 6 represents the magnetization isotherms M(µ ₀ H) registered in magnetic fields up to 5 T in the temperature range from 152 to 287 K at a temperature increment of ΔT = 3 K. These curves show that the magnetization increases sharply at low temperature in weak fields and then rises gradually with increasing field. However, when the temperature increases, the magnetization decreases and at Curie temperature, the sample transits from ferromagnetic to paramagnetic. This is because as temperature increases, originally magnetic ordered tends to clutter and disorder, magnetic momentum deflection occurs and hence the decrease in the total magnetic moment of the entire system, the magnetization of the sample decreases; when the temperature reaches near the Curie temperature, the thermal motion of the molecular of the material overcomes the ordered spin at zero-field, paramagnetism appears instead of ferromagnetism. From this figure, no sign of saturation can be shown even at 5 T.

Figure 6

Magnetization M versus magnetic applied field (µ ₀ H) at different temperatures. The inset is Arrott curves (M ² versus µ ₀ H/M) isotherms

We draw in inset of Fig. 6 the Arrott plots M ² versus µ ₀ H/M isotherms to define the order of the magnetic phase transition. This order can be determined from the slope from this figure according to the criterion given by Banerjee [44]. A positive or negative sign of the slope corresponds to a second- or first-order magnetic phase transition. For our sample, we can see clearly that the curve indicates positive slope in the high-field regions, indicating that the FM–PM transition is of a second order.

Magnetocaloric study

The isothermal magnetization curve was used to calculate the magnetic entropy change (−∆S _M), which is associated with the magnetocaloric effect, under the influence of a magnetic field.

From the Maxwell relations [45] based on the thermodynamical theory, the magnetic entropy change, induced by changing the magnetic field from 0 to µ ₀ H, can be evaluated from the temperature using a numerical approximation as follows:

$$ \Delta S_{\text{M}} (\mu_{0} H,T) = S_{\text{M}} (\mu_{0} H,T) - S_{\text{M}} (0,T) = \int\limits_{0}^{{\mu_{0} H_{\hbox{max} } }} {\left( {\frac{{\partial M(\mu_{0} H,T)}}{\partial T}} \right)_{{\mu_{0} H}} {\text{d}}(\mu_{0} H} ), $$

(9)

where µ ₀ H is the magnetic field, T is the temperature, and µ ₀ H _max is the maximum of the magnetic field. In the case of magnetization measurements at small discrete magnetic field and temperature intervals, ∆S _M can be approximated as

$$ \Delta S_{\text{M}} \left( {\frac{{T_{1} + T_{2} }}{2}} \right) = \frac{1}{{T_{1} - T_{2} }}\left[ {\int\limits_{0}^{{\mu_{0} H_{\hbox{max} } }} {M(T_{2} ,\mu_{0} H)\mu_{0} {\text{d}}H - \int\limits_{0}^{{\mu_{0} H_{\hbox{max} } }} {M(T_{1} ,\mu_{0} H)} \mu_{0} {\text{d}}H} } \right]. $$

(10)

According to Eq. (10), the magnetic entropy change for the sample as a function of temperature at various external magnetic fields is given in Fig. 7.

Figure 7

Temperature dependence of the magnetic entropy change in various magnetic fields up to 5 T for samples

We can also observe two distinct maxima at 180 and 248 K: The lowest one is around Neel transition (T _N = 180 K). In general, in simple perovskite around this transition (−∆S _M) is negative [46], while in the double layered, we find that this (−∆S _M) can be positive [47]. It is comparable with our results. The second is around the Curie temperature (T _C = 248 K). In this temperature, (−∆S _M) increases from 0.56 to 1.71 J kg⁻¹ K⁻¹ with increasing magnetic field (0.5–5 T), and the peaks are symmetrical enlargement and remain nearly unaffected. This phenomenon is in general characteristic of a second-order magnetic transition [48].

An important parameter used to estimate the magnetic cooling efficiency of our magnetocaloric material is the relative cooling power (RCP). This factor corresponds to the amount of heat per kilogram that can be transferred between the cold and hot tanks during an ideal refrigeration cycle and is defined as [1]

$$ {\text{RCP}} = - \Delta S_{\hbox{max} } \times \delta T_{\text{FWHM}}, $$

(11)

where ∆S _max is the maximum magnetic entropy change, and (δT _FWHM = T _hot − T _cold) is the full width at half maximum of the magnetic entropy change curve.

The relative cooling power has been calculated for both cases near T _C and around T _N and are noted, respectively, RCP₁ and RCP₂.

Figure 8 shows the magnetic field dependence of RCP around T _C and T _N. From this figure, we can see that for both temperatures, the values of RCP increase with µ ₀ H. However, RCP around T _C is significantly more important than T _N. So a material in the same refrigeration cycle with higher RCP is preferred because it would confirm the transport of a greater amount of heat in an ideal refrigeration cycle [49].

Figure 8

Magnetic field (µ ₀ H) dependence of the RCP₁ and RCP₂ in both transitions

The values of ΔS _max and RCP₁ for the sample are summarized in Table 2, together with reported values of other materials for comparison.

Table 2 Method of preparation, Curie temperature (T _C), maximum magnetic entropy change, and relative cooling power for La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇ compared with other manganites

The value RCP₁ is considerable and comparable with those reported in the literature, and the magnetic entropy peak is broadening; these factors make this sample a promising candidate for magnetic refrigeration applications.

The change of specific heat (∆C _P) associated with a magnetic field variation from 0 to µ ₀ H is given by [51–53].

$$ \Delta C_{\text{P}} (T,\mu_{0} H) = C_{\text{P}} (T,\mu_{0} H) - C_{\text{P}} (T,0) = T\frac{{\partial \left( {\Delta S_{\text{M}} (T,\mu_{0} H)} \right)}}{\partial T}. $$

(12)

Figure 9 shows the ∆C _p versus temperature under different variations of the applied magnetic field of La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇ sample calculated from the ∆S _M data (Fig. 7) using Eq. (12). One can see that the ∆C _p changes suddenly around T _C from positive to negative value with a positive value above T _C and a negative value below T _C. Besides, the maximum/minimum values of ∆C _P exhibit an increasing trend with the applied magnetic field and are observed at the temperatures 261/237 K in 5 T.

Figure 9

Temperature dependence of (∆C _P ) under different variations of the applied magnetic field (µ ₀ H) for the sample

Based on Landau’s theory [54] of second-order phase transition and mean-field approximation, Amaral et al. [55, 56] have investigated a successful model applicable to the MCE with the magnetoelastic contribution and electron interaction. However, this theory may not be able to explain the features near T _N because of critical fluctuations near the phase transition [57]. For this reason, we have applied this theory around T _C and calculated the Gibb’s free energy G(M, T) which can be expressed as

$$ G(M,T) = \frac{A(T)}{2}M^{2} + \frac{B(T)}{4}M^{4} - \mu_{0} HM. $$

(13)

According to the equilibrium condition, (∂G/∂M) = 0, we obtain

$$ \mu_{0} H = A(T)M + B(T)M^{3}, $$

(14)

$$ \frac{{\mu_{0} H}}{M} = A(T) + B(T)M^{2}, $$

(15)

here A(T) and B(T) are the Landau coefficients which can be determined from the plots of µ ₀ H versus M at a different temperature as reported in the inset of Fig. 10. The sign of the B(T) determines the type of magnetic transition phase. If B(T) > 0, the magnetic transition is of second order, otherwise it is of first order [3, 58].

Figure 10

Theoretical and experimental magnetic entropy changes for the sample at a magnetic field of 5 T. The inset represents the temperature dependence of Landau coefficients A and B

From the inset of Fig. 10, it is clear that the parameter A varies with temperature and has a minimum zero at near T _C and the value of B is positive confirming the second-order magnetic transition.

The corresponding magnetic entropy change is obtained by differentiation of the magnetic part of the free energy with respect to temperature:

$$ \begin{aligned} S_{\text{M}} (T,\mu_{0} H) & = - \left( {\frac{\partial G}{\partial T}} \right)_{\text{H}} \\ & = - \frac{1}{2}\frac{\partial A}{\partial T}M^{2} - \frac{1}{4}\frac{\partial B}{\partial T}M^{4} \\ \end{aligned}. $$

(16)

Using the A(T) and B(T) parameters, their derivative in Eq. (16), and the value of M(T, µ ₀ H), the magnetic entropy change (−∆S _M) was calculated theoretically under a magnetic field change of 5T.

Figure 10 shows the experimental and calculated results obtained by the Landau theory. There is a discrepancy between the experimental magnetic entropy change and those calculated theoretically. The analysis demonstrates that magnetoelastic coupling and electron interaction do not contribute directly to the magnetic entropy in this sample. In this case, an additional effect together with the magnetoelastic and electron interaction contributions is necessary for observed magnetic entropy data [59].

Universal curve scaling analysis

Franco et al. [60] proposed the phenomenological universal curve which can be used as a further criterion to study the order of phase magnetic transition and was constructed by normalizing all the ∆S _M(T) curves against their respective maximum ∆S ^max_M , namely, ∆S’ = ∆S _M/∆S ^max_M rescaling the temperature axis below and above T _C as defined in Eq. (17) with an imposed constraint that the position of two additional reference points in the curve correspond to θ ± 1.

$$ \theta = \left\{ \begin{array}{*{20}c} { - \left( {T - T_{\text{C}} } \right)/\left( {T_{r1} - T_{\text{C}} } \right), T \le T_{\text{C}} } \\ { \left( {T - T_{\text{C}} } \right)/\left( {T_{r2} - T_{\text{C}} } \right), T > T_{\text{C}} } \\ \end{array} \right\}, $$

(17)

where T _r1 and T _r2 are the temperatures of the two reference points that have been selected as those corresponding to ∆S _M(T _r1,2) = 1/2 ∆S ^max_M .

In general, for alloys with second-order phase transition, the ∆S _M curves measured at different applied magnetic fields should collapse onto the same universal curve, while, when the scaled curves do not collapse as a single curve, the nature of the magnetic transition phase is of the first order [61].

Figure 11 shows the θ dependence of the normalized entropy change ∆S’ for the sample. It can be clearly seen that all the experimental points in a different applied magnetic field (µ ₀ H = 1–5 T) distribute on one universal curve, which confirms the occurrence of a second-order phase transition and the validity of the technique used. Materials undergoing second-order transition are good candidates for refrigerants since they have negligible hysteretic losses, while the materials undergoing first-order phase always have larger magnetic entropy change, such as gadolinium binary or ternary alloys [62]. Phan and Yu have detailed the explanation that the manganites undergoing a second-order phase transition are more active for their negligible hysteretic losses and large relative cooling capacity [19].

Figure 11

Normalized entropy change dependence of the rescaled temperatures at different applied magnetic field. Inset represents the temperature dependence of the exponent n for La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇ sample

Recently, the magnetic field dependence of the magnetic entropy change of material with a second-order phase transition at a temperature T can be defined as [62–65]

$$ \Delta S_{\text{M}} = a(\mu_{0} H)^{n}, $$

(18)

where a is a constant and n depends on the magnetic state of the sample. It can be calculated as follows:

$$ n = \frac{{{\text{d}}\ln \Delta S_{\text{M}} }}{{{\text{d}}\ln (\mu_{0} H)}}. $$

(19)

By fitting the data ∆S _M versus µ ₀ H at each temperature to Eq. (19), we determine the exponent n as a function of temperature as shown in the inset of Fig. 11.

It is eminent that in perovskite compounds the exponent is almost field-independent and is close to values of 1 and 2, around the transition temperature, respectively [63].

It can be clearly seen from this figure that the n exponent evolves with the temperature, it reaches 1 far below the Curie temperature and above the Curie temperature it increases with increasing temperature and the value approach 2 due to the Curie–Weiss law reflects an increasing sensitivity of the magnetic entropy to apply magnetic field in this temperature range. The minimum n value around T _C equal to 0.68 is close to the 2/3 value predicted by Oesterreicher and Parker [66]. It was reported that the n exponent has a significant dependence on the size of manganites and exhibits an increasing trend with reducing their size [63].

Critical behavior

In order to understand the exact nature of the second-order magnetic phase transition occurring around the Curie point, we used Arrott plots to determine the Curie temperature and the critical exponents in the vicinity of the phase transition temperature. The exponent’s β (associated with the spontaneous magnetization M _S(T) = lim _H→0 M just below T _C), δ (associated with the critical magnetization isotherm at T _C), and γ (relevant to the initial magnetic susceptibility χ₀, χ ⁻¹₀ (T) = lim  _H→0 (μ ₀ H/M) just above T _C) are given in the following relation [67–69]:

$$ M_{\text{S}} (T,0) = M_{0} ( - \varepsilon )^{\beta } ;\quad T < T_{\text{C}} ,\quad \varepsilon < 0. $$

(20)

The exponent γ describes the temperature dependence of the zero-field susceptibility and is defined as

$$ \chi_{0}^{ - 1} (T) = \left( {\frac{{h_{0} }}{{M_{0} }}} \right)\varepsilon^{\gamma } ;\quad T > T_{\text{C}} ,\quad \varepsilon = 0. $$

(21)

The exponent δ describes the field dependence of the magnetization at the Curie temperature, T _C:

$$ M = DH^{{{1 \mathord{\left/ {\vphantom {1 \delta }} \right. \kern-0pt} \delta }}} ;\quad T = T_{\text{C}}, $$

(22)

here ɛ = (T − T _C)/T _C is the reduced temperature. The critical exponents β, γ, and δ, as well as the critical amplitudes M ₀, h ₀, and D exhibit universal behavior near the phase transition point. There are several universality classes with sets of critical indices that depend on the spin and the special dimensionality. As we know, for a first-order ferromagnetic phase transition, the critical exponents are impossible to define because the applied magnetic field depends on the temperature T _C (µ ₀ H) [70].

From the Arrott plot M ² versus µ ₀ H/M in Fig. 6, the reliable values of the inverse initial susceptibility χ ⁻¹₀ (T) and the spontaneous magnetization M _S(T) have been determined by the linear extrapolation of the high-field region toward the intercepts with both axes (µ ₀ H/M) and M ² below and above T _C, respectively.

These results are shown in Fig. 12a as functions of temperature. By fitting these plots using Eqs. (20) and (21), we get to the values of β = 0.594, γ = 1.048, and T _C = 248 K.

Figure 12

a Temperature dependence of the spontaneous magnetization M _S(T, 0) and the inverse initial susceptibility χ ⁻¹₀ along with the fitting curves (solid lines) based on the power laws. b Kouvel-Fisher plots for M _S(T)(dM _s/dT)⁻¹ and χ ⁻¹₀ (T)(dχ ⁻¹₀ /dT)⁻¹

Moreover, in order to determine the parameters T _C, β and γ more precisely, we also analyzed M _S(T, 0) and χ ⁻¹₀ (T) values using the kouvel-Fisher (KF) method [71, 72]:

$$ {{M_{\text{S}} (T)} \mathord{\left/ {\vphantom {{M_{\text{S}} (T)} {\left( {{{{\text{d}}M_{\text{S}} (T)} \mathord{\left/ {\vphantom {{{\text{d}}M_{\text{S}} (T)} {{\text{d}}T}}} \right. \kern-0pt} {{\text{d}}T}}} \right) = {{(T - T_{\text{C}} )} \mathord{\left/ {\vphantom {{(T - T_{\text{C}} )} \beta }} \right. \kern-0pt} \beta }}}} \right. \kern-0pt} {\left( {{{{\text{d}}M_{\text{S}} (T)} \mathord{\left/ {\vphantom {{{\text{d}}M_{\text{S}} (T)} {{\text{d}}T}}} \right. \kern-0pt} {{\text{d}}T}}} \right) = {{(T - T_{\text{C}} )} \mathord{\left/ {\vphantom {{(T - T_{\text{C}} )} \beta }} \right. \kern-0pt} \beta }}}, $$

(23)

$$ {{\chi_{0}^{ - 1} (T)} \mathord{\left/ {\vphantom {{\chi_{0}^{ - 1} (T)} {\left( {{{{\text{d}}\chi_{0}^{ - 1} (T)} \mathord{\left/ {\vphantom {{{\text{d}}\chi_{0}^{ - 1} (T)} {{\text{d}}T}}} \right. \kern-0pt} {{\text{d}}T}}} \right)}}} \right. \kern-0pt} {\left( {{{{\text{d}}\chi_{0}^{ - 1} (T)} \mathord{\left/ {\vphantom {{{\text{d}}\chi_{0}^{ - 1} (T)} {{\text{d}}T}}} \right. \kern-0pt} {{\text{d}}T}}} \right)}} = {{(T - T_{\text{C}} )} \mathord{\left/ {\vphantom {{(T - T_{\text{C}} )} \gamma }} \right. \kern-0pt} \gamma }. $$

(24)

According to these equations, the parameters M _S(T)[dM _S(T)/dT]⁻¹ and χ ⁻¹₀ (T)[dχ ⁻¹₀ (T)/dT]⁻¹ are plotted versus T in Fig. 12b and should yield straight lines with slopes of 1/β and 1/γ, respectively, and the value of T _C is obtained from intercepts on the temperature axis.

The linear fitting to the plots following the KF method gives β = 0.572, γ = 1.029, and T _C = 248 K.

Obviously, the values of β, γ, and T _C obtained by the KF method are in good agreement with that using the Arrott plot M ² versus µ ₀ H/M.

Further, the critical exponent δ has also been determined directly by plotting the critical isotherm at T _C. In Fig. 13, the M versus µ ₀ H curve are plotted at 248 K, which is close to the T _C. The inset presents the M versus µ ₀ H plot logarithmic scale. According to Eq. (22), in the high-field region of the data, the solid straight line with the slope (1/δ) is the fitting result. This gives the third critical exponent δ = 2.549.

Figure 13

Critical isotherms of M versus µ ₀ H at T _C. The inset shows the same plot in log–log scale and the straight line is the linear fit following Eq. (22)

According to statistical theory, these three critical exponents are related according to the Widom scaling relationship [72–74] given by

$$ \delta = 1 + \frac{\gamma }{\beta }. $$

(25)

Using this scaling relation and the critical exponents β and γ, obtained above from the Arrott plot M ² versus µ ₀ H/M and KF method, we obtain δ = 2.764 and 2.798, respectively. These values are in good agreement and close to those obtained from the critical isotherms at T _C. Thus, the critical exponents, found in this work, obey the Widom scaling relation remarkably well. Moreover, we can note that these values for the critical exponents are close to the mean-field values (β = 0.5, γ = 1 and δ = 3) [75].

In the critical region, to further investigate the reliability of the calculated exponents values β, γ, and δ and the validity of the Widom scaling relation, the magnetic equation of state can be written as [76]

$$ M(\mu_{0} H,\varepsilon ) = \varepsilon^{\beta } f_{ \pm } ({{\mu_{0} H} \mathord{\left/ {\vphantom {{\mu_{0} H} {\varepsilon^{\beta + \gamma } )}}} \right. \kern-0pt} {\varepsilon^{\beta + \gamma } )}}, $$

(26)

where f ₊ for the paramagnetic state for T > T _C and f ₋ for the ferromagnetic state for T < T _C are regular analytic functions and the values of β and γ were obtained from KF method. Equation (26) implies that the scaled M/|ɛ ^β| plotted as a function of μ ₀ H/|ɛ|^β+γ in Fig. 14 yields in the vicinity of T _C two distinct curves, one for temperatures T < T _C and the other for temperatures T > T _C. The scaled data proved that the critical exponents and T _C obtained are in good accordance with the scaling hypothesis.

Figure 14

Scaling plots for compound below and above T _C, using the β and γ exponents determined from the KF method, indicating two universal curves. The insets show the same plots on a log–log scale

The inset of Fig. 14 shows the same plots in log–log scale. It is clearly observed, at higher fields, that these two curves at T < T _C and T > T _C coincided. However, the scaling becomes poor toward lower fields. This behavior is due to the Arrott plot which deviates from linearity at low field, due to the mutually misaligned magnetic domains.

Based on the renormalization group analysis of systems, performed by Fisher et al. [72], the universality class of the magnetic phase transition depends on the range of exchange interaction J(r) = 1/r ^d+σ, where r is the distance between the interacting spins, d is the dimension of the system, and σ > 0 is the range of the interaction.

For three-dimensional materials (d = 3), the relation becomes J(r) = r ^−3−σ. It has been argued that, if J(r) decreases faster than r ⁻⁵ (σ greater than 2), the Heisenberg exponents (β = 0.365, γ = 1.336 and δ = 4.8) is valid for a 3D-isotropic ferromagnet. However, if J(r) decreases slower than r ^−4.5 (σ is less than 3/2), the mean-field model (β = 0.5, γ = 1.0 and δ = 3.0) is satisfactory. In the intermediate range with 3/2 ≤ σ ≤ 2, the exponents belong to different universality class which depends upon σ (such as the tricritical mean-field theory and 3D Ising model). In general way, the evolution of the critical exponents tends toward the values of the mean-field theory.

In the following section, we will use the obtained magnetic entropy change to study the spontaneous magnetization in this sample. According to the mean-field theory, the magnetic entropy can be expressed as a function of magnetization [77, 78]:

$$ S\left( \sigma \right) = - Nk_{\text{B}} \left[ {\ln \left( {2J + 1} \right) - \ln \frac{{\sinh \left( {\frac{2J + 1}{2J}B_{J}^{ - 1} \left( \sigma \right)} \right)}}{{\sinh \left( {\frac{1}{2J}B_{J}^{ - 1} \left( \sigma \right)} \right)}} +\,B_{J}^{ - 1} \left( \sigma \right)\sigma } \right], $$

(27)

where N is the number of spins, k _B is Boltzmann constant, J is the spin value, B _J is the Brillouin function for a given J value, and σ the reduced magnetization.

(σ = (M/gJN)μ _B).

For small M values, Eq. (27) can be performed using a power expansion, and the magnetic entropy ∆S _M is proportional to M ²:

$$ - S\left( \sigma \right) = \frac{3}{2}\frac{J}{J + 1}Nk_{\text{B}} \sigma^{2} + o\left( {\sigma^{4} } \right). $$

(28)

Furthermore, in the ferromagnetique state (T < T _C), the material produces a spontaneous magnetization M _S and the σ = 0 state is never attained. If we only consider the first term of the expansion of Eq. (28), this corresponds to

$$ - S\left( \sigma \right) = \frac{3}{2}\frac{J}{J + 1}Nk_{\text{B}} \left( {\sigma^{2} + \sigma_{\text{S}}^{2} } \right). $$

(29)

Equation (29) indicates that the −∆S _M versus M ² curve shows a linear variation. We plotted this curve based on the obtained magnetic entropy change and magnetization. As shown in Fig. 15, all curves at different temperatures (224–245 K) obey the same regularity and a clear linear dependence with an approximately constant slope (0.0034), indicating that it is appropriate for us to analyze the current experimental results with the mean-field theory.

Figure 15

Isothermal (−∆S _M) versus M ² curves. The solid lines are linear fits to data

Therefore, the spontaneous magnetization M _S of material can be estimated from the linear fits of −∆S _M versus M ² curve at different temperatures, which is shown in Fig. 16.

Figure 16

Spontaneous magnetization of La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇ deduced from the extrapolation of the isothermal −∆S _M versus M ² curves and from the mean-field results

We can see that when the temperature decreases, the spontaneous magnetization becomes larger and larger, suggesting that the compound is approaching a spin ordering state, at lower temperature.

For comparison, the spontaneous magnetization can be also determined from the experimental Arrott plot (namely, M ² versus H/M), as shown in Fig. 12a.

Obviously, the excellent agreement between the two methods is obtained, confirming that the present magnetic system should be close to mean-field theory.

Conclusion

In summary, we have prepared La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇ polycrystalline sample by sol–gel route. Magnetic properties, magnetocaloric effect, and the critical properties have been studied in detail.

The variation of the magnetization versus temperature, under an applied magnetic field of 0.05 T, revealed that the compound shows ferromagnetic behavior with a Curie temperature of 248 K and a Neel transition at T _N = 170 K.

Besides the universal curves confirmed that the magnetic phase transition is of the second order in nature. Then, the maximum entropy change of 1.71 J kg⁻¹ K⁻¹ and RCP = 153.77 J/kg were observed at 5 T magnetic field. The estimated critical exponents confirm that the experimental data agree well with the mean-field model. The methodology based on the analysis of the magnetic entropy change (−∆S _M) versus M ², compared with the classical extrapolation of the Arrott curves (µ ₀ H/M versus M ²), confirm that the magnetic entropy change is a valid method to determine the spontaneous magnetization of La_1.4(Sr_0.95Ca_0.05)_1.6Mn₂O₇ system and eventually of other compounds.