1 Introduction

In recent years, two-level atoms in the external field which are important in quantum optics have been investigated in many published papers. The study of the squeezing of a two-level atom and the light field in quantum systems has also attracted considerable attention. Based on information entropy the measuring of squeezing plays an essential role in quantum information processing and it has strong applications in the optical communication systems [1, 2, 3] and weak signal detections [4, 5]. Some of its applications are in high-resolution spectroscopy, high precision atomic clocks, high polarity spin polarization measurement, quantum teleportation, cryptography and so on [6, 7, 8, 9, 10, 11, 12]. In general, the Heisenberg uncertainty relation (HUR), which is among the most important principles of quantum mechanics, is mainly a standard relation for the representation of the atomic squeezing and so one can consider this quantity for measuring quantum fluctuations. However, Fang et al. in Ref. [13] have pointed out that this relationship cannot show sufficient information about atomic squeezing for some cases, in particular, when the atomic inversion is zero. Several researchers have presented a beautiful method of correcting HUR using the quantum entropy theory and obtained an entropic uncertainty relation (EUR), which could be free from the triviality of the HUR. In Ref. [13], using the EUR relation, the squeezing based on the information entropy of a two-level atom in the Jaynes-Cummings model (JCM) in resonance mode has been studied.

Quantum entanglement (QE) is one of the most eminent features of quantum mechanics and in the three last decades, much attention has been focused on it from different aspects [14]. The quantum information processing is one of the aspects QE that can be prepared and controlled by the entangled states in experimental conditions. Consequently, the understanding of entangled states, the relationship between the atomic squeezing and the entanglement, and also the generation of entanglement and squeezing in circuit quantum electrodynamics are very important where have been studied by many researchers. In this regard, the von Neumann entropy (VNE) [15], the linear entropy (LE) [16] and the Shannon information entropy (SE) [17, 18] are used as a measure to study the entanglement of quantum systems in different aspects. In Ref. [19], the authors have been used the LE and have been studied entanglement in quantum systems of two-fermion particles. The Wehrl entropy (WE) [20, 21, 22, 23, 24, 25, 26] and recently the Fisher information (FI) [27] are as another measures which for treating entanglement have also attracted many attentions. In Ref. [28], the authors have been used the concept of the atomic FI as a quantity of the entanglement and compare it with the linear and atomic Wehrl entropies of the two-level atom in the JC model. They have also demonstrated the connections between the entanglement measures. The Fisher information relation in terms of the atomic density operator and the correlation between the FI and QE during the time evolution for a trapped ion in laser field have been studied in Refs. [7, 16]. The authors have been examined the effect of the initial state setting on the classical FI and quantum FI and shown that the FI is efficacious tool to study single qubit dynamics as an indicator of entanglement under certain conditions. Study on a quantum system of an atom–atom interaction in the presence of two external magnetic and classical fields has been done on Ref. [29] and the atomic WE, atomic FI and quantum entropy have been investigated. The authors have shown that suitable choice of kind of time dependent coupling among the two atoms and external fields, can be affected in creation and manipulation of entanglement by external fields [28, 30].

The Jaynes-Cummings model is an elementary model in quantum optics that describes the interaction between a two-level atom with a quantized electromagnetic field [31]. This is the simplest completely soluble quantum–mechanical model and is nonlinear model of interaction between field-atom. In the rotating wave approximation, this model has been extensively studied and its exact solution has been found. Tavis and Cummings studied and exactly solved the problem of N identical two-level atoms interacting through a dipole coupling with a single-mode quantized radiation field at resonance. The Tavis–Cummings Model (TCM) is a distribution of the JC model and, for historical reasons, the TCM is often called the Dicke model [32, 33]. Hence, TCM is a theoretical model that contains a large number of coupled atoms (N > 1) in a quantum field in a small cavity, which give arises many non-classical properties.

The effects of the dipole–dipole interaction and phase-interrupting collisions between atoms, the effect of the presence of a nonlinear medium and amplitude–phase metastability in the TCM have been studied in many references [6, 7, 8, 9, 34, 35, 36, 37, 38]. On the other hand, Wang has also studied sustained optimal entropy squeezing of a two-level atom via non-Hermitian operation and provided a scheme for generating the sustained optimal entropy squeezing of the atom via non-Hermitian operation [39]. Xiao and et al. have investigated the entropy squeezing of a two-level system by detuning in non-Markovian environments and shown that the atomic entropic squeezing can be preserved for a long time when both the non-Markovian effect and detuning are present together [40]. The entropy squeezing of a two-level atom in JCM has been widely reported. Zou has studied the quantum entropic uncertainty relation and entanglement witness in the two-atom system coupling with the non-Markovian environments [41, 42].

According to Ref. [43] which attracted our attention to this topic, we investigate the effect of the atomic motion and the mode field on the single-atom entropy squeezing in a two- atom TCM with atomic motion in a coherent state field with together atomic coherent states. This new class of states forms by a simple of coherent of the superposition principle and is fundamentally different from the conventional two-photon coherent squeezed states. We show that choosing the coherent atomic state changes the entropy squeezing and the results may be important for the experimental realization of the preparation of squeezing atom. We also use the linear entropy and the Fisher information as the entanglement measures and study the effect of the atomic motion and the mode field on entanglement between the two-atom and the field.

The paper is prepared as follow: In Section 2, we introduce the Tavis–Cummings Model for two identical two-level atoms and write its Hamiltonian in the dipole approximation and rotating-wave approximation (RWA). In Section 3, we introduce the atomic entropy squeezing defined by EUR, the atomic coherent Dike state for studying of the squeezing, the linear entropy as the QE measure and the Fisher information obtained by Husimi distribution as another QE measure. Section 4 is devoted to result and discussion. In the last section, we briefly report the conclusion of work.

2 Tavis-Cummings Model and the Reduced Density Operator

We consider the TCM for two identical two-level atoms which are in a single-mode cavity and move at the same speed in z-direction [43]. For simplicity, we neglect the dipole–dipole interaction of the atoms, and so in the dipole approximation and under the rotating-wave approximation (RWA), the Hamiltonian can be written in terms of two terms:

$$H={H}_{0}+{H}_{1}$$
(1)

where \({H}_{0}\) as the Hamiltonian of two atoms and the external cavity field is as (\(\mathrm{\hslash }=1\))

$$\begin{array}{cc}H_0=\omega\left(a^\dagger a+S_z\right),&S_z=\frac12\sum\limits_{j=1}^2\sigma_z^{\left(j\right)}\end{array}$$
(2)

and \({H}_{1}\) as the interaction Hamiltonian between the atoms and the cavity field is as

$$\begin{array}{cc}H_1=gf\left(z\right)\left(a^\dagger S_-+{aS}_+\right),&S_\pm=\sum\limits_{j=1}^2\sigma_\pm^{\left(j\right)}.\end{array}$$
(3)

The operators \(a\) and \(a^\dagger\) are the photon annihilation and the photon creation operators of the cavity field and \({S}_{z,\pm }\) are the pseudo-spin operators of the atoms. It is easy to see that the atom operators satisfy the su(2) algebra and the field operators satisfy the bosonic algebra as

$$\left[S_+,S_-\right]=2S_z,\left[S_z,S_\pm\right]=\pm S_\pm,\left[a,a^\dagger\right]=I.$$
(4)

We have also considered the resonance mode, in which frequencies of the atomic transition and the cavity field are both ω. The function f(z) denotes a shape function of cavity field mode and the parameter g is the coupling constant between the atoms and the cavity field. By considering the motion of atoms along in the z direction then the f(z) function can be written in terms of the atomic motion speed as \(f\left(vt\right)\). According to Ref. [43], we consider the transverse electric and magnetic mode (\(TEM\)) as the role of the electromagnetic pump by

$$f\left(z\right)=\sin\left(p\pi vt/L\right),$$
(5)

where p represents the number of half-wavelengths of the field mode inside a cavity with length L. We also assume that the two atoms at time t = 0 enter the cavity and leave the cavity after passing through p half-wavelengths of electric field.

The Schrödinger equation in the interaction picture is written by the Hamiltonian \({H}_{1}\) as:

$$i\frac{\partial }{\partial t}\left|\psi \left(t\right)\right.\rangle ={H}_{1}\left|\psi \left(t\right)\right.\rangle$$
(6)

and the time evolution operator, when the Hamiltonian \({H}_{1}\) at different times commute with together, can be written as:

$$U\left(t\right)=\exp\left(-i\int_0^tH_1d\tau\right)=\exp\left(-igF\left(t\right)T\right),$$
(7)

with

$$T=\left(a^\dagger S_-+{aS}_+\right),$$
(8)

and

$$F\left(t\right)=\int_0^tf\left(z\right)d\tau=\int_0^t\sin\left(\frac{p\pi v\tau}L\right)d\tau=\frac L{p\pi v}\left[1-\cos\frac{p\pi vt}L\right].$$
(9)

By choosing the particular value of the atomic motion velocity in terms of the coupling constant as \(v=\frac{gL}{\pi }\), we have

$$F\left(t\right)=\frac{1-\cos\left(pgt\right)}{pg}.$$
(10)

We now consider the cavity field to be in the coherent state

$$\begin{array}{cc}\left|\alpha\right.\rangle=\sum\limits_{n=0}^\infty F_n\left|n\right.\rangle,&F_n=\exp\left(-\frac{\left|\alpha\right|^2}2\right)\frac{\alpha^n}{\sqrt{n!}},\end{array}$$
(11)

where \(\alpha =\left|\alpha \right|{e}^{i\beta }=\sqrt{\overline{n}}{e }^{i\beta }\) and \(\overline{n }\) is the average photon number of the initial coherent field (the intensity of the cavity field) and \(\beta\) is the phase angle of the coherent field where we suppose \(\beta =0\). Assuming atom and field are decoupled, then the initial vector state of the system is written as

$$\left|\psi \left(0\right)\right.\rangle =\left|{\psi }_{A}\left(0\right)\right.\rangle \otimes \left|\alpha \right.\rangle ,$$
(12)

where \(\left|{\psi }_{A}\left(0\right)\right.\rangle\) is the initial state of the atom. The vector state of the system at any time \(t>0\), leads to

$$\left|\psi \left(t\right)\right.\rangle =U\left(t\right)\left|\psi \left(0\right)\right.\rangle =\sum_{n=0}^{\infty }\left[A\left|{u}_{1},n\right.\rangle +B\left|{u}_{2},n\right.\rangle +C\left|{u}_{3},n\right.\rangle \right],$$
(13)

where the coefficients A, B and C are obtained from the choice of initial atomic state and \(\left|{u}_{1}\right.\rangle \equiv \left|{e}_{1},{e}_{2}\right.\rangle\), \(\left|{u}_{2}\right.\rangle \equiv \frac{1}{\sqrt{2}}\left(\left|{e}_{1},{g}_{2}\right.\rangle +\left|{g}_{1},{e}_{2}\right.\rangle \right)\) and \(\left|{u}_{3}\right.\rangle \equiv \left|{g}_{1},{g}_{2}\right.\rangle\) are three swap-symmetry wave functions of the two identical atoms, which are used as the base vectors.

The atomic reduced density operator for this system in general form is obtained as

$$\rho_A(t)=tr_F\vert\psi(t)\rangle\langle\psi(t)\vert=\begin{bmatrix}\begin{array}{cccc}\rho_{11}&\rho_{12}&\rho_{13}&\rho_{14}\\\rho_{21}&\rho_{22}&\rho_{23}&\rho_{24}\\\rho_{31}&\rho_{32}&\rho_{33}&\rho_{34}\\\rho_{41}&\rho_{42}&\rho_{43}&\rho_{44}\end{array}\\\end{bmatrix},$$
(14 )

where

$$\begin{array}{l}\rho_{11}={\sum\limits_{n=0}^\infty\vert A\vert}^2,\rho_{22}=\rho_{23}=\rho_{32}=\rho_{33}=\frac12{\sum\limits_{n=0}^\infty\vert B\vert}^2,\rho_{44}={\sum\limits_{n=0}^\infty\vert C\vert}^2,\\\rho_{12}=\rho_{13}=\rho_{21}^\ast=\rho_{31}^\ast=\frac1{\sqrt2}\sum\limits_{n=0}^\infty AB^\ast,\\\rho_{42}=\rho_{43}=\rho_{24}^\ast=\rho_{34}^\ast=\frac1{\sqrt2}\sum\limits_{n=0}^\infty CB^\ast,\\\rho_{41}=\rho_{14}^\ast=\frac1{\sqrt2}\sum\limits_{n=0}^\infty AC^\ast.\end{array}$$
(15)

In the next section, we choice the coherent state given by Dick state as the initial atomic state and obtain the partial trace of density operator. We then calculate the atomic entropy squeezing, linear entropy and the quantum fisher information and some aspects of them are discussed.

3 Atomic Entropy Squeezing, Linear Entropy and the Quantum Fisher Information

In this section, we first express the entropy squeezing of a two-level atom by using the quantum information entropy theory [43, 44]. For a two-level atomic system, the entropy information operator of the Pauli operator \({S}_{\alpha }\) is obtained as

$$H\left(S_\alpha\right)=-\sum\limits_{i=1}^2P_i\left(S_\alpha\right)\ln P_i\left(S_\alpha\right),\alpha=x,y,z$$
(16)

where \({P}_{i}\left({S}_{\alpha }\right)=\left.\langle {\psi }_{\alpha i}\right|\rho \left|{\psi }_{\alpha i}\right.\rangle\) is the probability distribution for N possible outcomes of measurements of the operator \({S}_{\alpha }\) and \(\left|{\psi }_{\alpha i}\right.\rangle\) is an eigenvector of the operator \({S}_{\alpha }\). According to Ref. [40], for N -dimensional Hilbert space, we have

$$\sum\limits_{k=1}^{N+1}H\left(S_k\right)\geq\frac N2\ln\left(\frac N2\right)+\left(\frac N2+1\right)\ln\left(\frac N2+1\right),$$
(17)

where for N = 2

$$H\left(S_x\right)+H\left(S_y\right)\geq2\ln\left(2\right)-H\left(S_z\right).$$
(18)

For N = 2, one can also show \(0\leq H\left(S_\alpha\right)\leq\ln2\). By defining \(\delta H\left(S_\alpha\right)=\exp\left(H\left(S_\alpha\right)\right)\), it can simply satisfy the following equation

$$\delta H\left({S}_{x}\right)\delta H\left({S}_{y}\right)\ge \frac{4}{\delta H\left({S}_{z}\right)},$$
(19)

where means the entropy uncertainty relation (EUR) [13]. From this relation, there is impossibility of simultaneously having complete information about the observable \({S}_{x}\) and \({S}_{y}\). The squeezing of the atom is defined by the above EUR relation and it is named the entropy squeezing. In other words, the fluctuation in component \({S}_{\alpha }\) of the atomic dipole is said to be squeezed in entropy if the information entropy \(H\left({S}_{\alpha }\right)\) satisfies the following condition [43, 45, 46, 47, 48, 49]

$$E\left({S}_{\alpha }\right)=\delta H\left({S}_{\alpha }\right)-\frac{2}{\sqrt{\left|\delta H\left({S}_{z}\right)\right|}}<0,\alpha \equiv x,y.$$
(20)

The above relation has the similar physical significance with the relation (19), that is, the fluctuations in the atomic polarization components cannot simultaneously be arbitrarily small.

Now for the TCM, mentioned in the previous section, we shall calculate the temporal evolutions of the single atom entropy squeezing. For instance, for the first atom, we show that due to the choice of the initial state, the squeezing may be occurred in \(E\left({S}_{x}\right)\) or \(E\left({S}_{y}\right)\). We also determine the period of the entropy squeezing and the squeezing time of information entropy by the field mode structure.

For this aim, we consider A1 and A2 as the first and the second atoms respectively and so the partial trace of the density operator for the first atom can be obtained by (13) as

$$\begin{array}{l}\rho_{A_1}(t)=tr_{A_2F}\vert\psi(t)\rangle\langle\psi(t)\vert=\begin{bmatrix}q_{11}&q_{12}\\q_{21}&q_{22}\end{bmatrix}\\=q_{11}\vert e_1\rangle\langle e_1\vert+q_{12}\vert e_1\rangle\langle g_1\vert+q_{21}\vert g_1\rangle\langle e_1\vert+q_{22}\vert g_1\rangle\langle g_1\vert,\end{array}$$
(21)

where

$$q_{11}=\sum\limits_{n=0}^\infty\left({\vert A\vert}^2+\frac12{\vert B\vert}^2\right),q_{12}=q_{21}^\ast=\sum\limits_{n=0}^\infty\frac1{\sqrt2}\left(AB^\ast+BC^\ast\right),q_{22}=1-q_{11},$$
(22)

and the information entropies of the atomic operators \({S}_{x},{S}_{y},{S}_{z}\) are as:

$$\begin{array}{l}H\left(S_x\right)=-\left[\left(\frac12+Re\left(q_{12}\right)\right)\ln\left(\frac12+Re\left(q_{12}\right)\right)+\left(\frac12-Re\left(q_{12}\right)\right)\ln\left(\frac12-Re\left(q_{12}\right)\right)\right],\\H\left(S_y\right)=-\left[\left(\frac12+Im\left(q_{12}\right)\right)\ln\left(\frac12+Im\left(q_{12}\right)\right)+\left(\frac12-Im\left(q_{12}\right)\right)\ln\left(\frac12-Im\left(q_{12}\right)\right)\right],\\H\left(S_z\right)=-\left[q_{22}\ln\left(q_{22}\right)+q_{11}\ln\left(q_{11}\right)\right].\end{array}$$
(23)

Now in continue, we consider the atomic coherent state given by Dike as the initial state of atom and apply the time evolution operator on this initial state. According to Ref. [37], the general Dick state for \(A\) atoms is defined as

$$|{\psi }_{A}(0)\rangle =\sum_{k=0}^{A}\sqrt{\frac{A!}{k!(A-k)}}{\mathrm{cos}}^{k}\frac{\theta }{2}{\mathrm{sin}}^{(A-k)}\frac{\theta }{2}{e}^{i(A-2k)\frac{\phi }{2}}|k,A\rangle ,$$
(24)

where \(0\le \theta \le \pi\) denotes the atomic distribution and \(0\le \phi \le 2\pi\) is the atomic dipole phase. For \(=2\), we have

$$\left|\psi\left(0\right)\right.\rangle=\sin^2\left(\frac\theta2\right)e^{i\phi}\left|u_3,n\right.\rangle+\frac{\sqrt2}2\sin\left(\theta\right)\left|u_2,n\right.\rangle+\cos^2\left(\frac\theta2\right)e^{-i\phi}\left|u_1,n\right.\rangle.$$
(25)

In our calculations, we consider \(\overline{n }=36,\phi =\pi\), and for simplicity, we first apply the time evolution operator for each of the three swap-symmetries and then obtain the coefficients A, B and C of the Eq. (13). In other word, for atoms which are in the ground state, we have

$$\left|\psi \left(t\right)\right.\rangle =U\left(t\right)\left|{u}_{3},n\right.\rangle ,$$
(26)

where after some calculations

$$\left|\psi \left(t\right)\right.\rangle =\sum_{n=0}^{\infty }\left[\left({F}_{n}{C}_{1,n-2}\left(t\right)\right)\left|{u}_{1},n-2\right.\rangle +\left({F}_{n}{C}_{2,n}-1\left(t\right)\right)\left|{u}_{2},n-1\right.\rangle +\left({F}_{n}{C}_{3,n}\left(t\right)\right)\left|{u}_{3},n\right.\rangle \right],$$
(27)

with

$$\begin{array}{l}C_{1,n-2}=\frac{\sqrt{n(n-1)}}{2n-1}\left(\cos\left(\sqrt{4n-2}gF(t)\right)-1\right)\,\text{,}\\C_{2,n-1}=-\frac{i\sqrt n}{\sqrt{2n-1}}\sin\left(\sqrt{4n-2}gF(t)\right)\,\text{,}\\C_{3,n}=\frac1{2n-1}\left(n\cos\left(\sqrt{4n-2}gF(t)\right)+n-1\right)\text{.}\end{array}$$
(28)

In the second case, when one of the atoms is in the ground state, we have

$$\left|\psi \left(t\right)\right.\rangle =U\left(t\right)\left|{u}_{2},n\right.\rangle ,$$
(29)

where after some calculations

$$\left|\psi \left(t\right)\right.\rangle =\sum_{n=0}^{\infty }\left[\left({F}_{n}{D}_{1,n-2}t)\right)\left|{u}_{1},n-2\right.\rangle +\left({F}_{n}{D}_{2,n}\left(t\right)\right)\left|{u}_{2},n\right.\rangle +\left({F}_{n}{D}_{3,n+1}\left(t\right)\right)\left|{u}_{3},n+1\right.\rangle \right],$$
(30)

with

$$\begin{array}{l}D_{1,n-1}=-\frac{i\sqrt n}{\sqrt{2n+1}}\sin\left(\sqrt{4n+2}gF(t)\right)\,\text{,}\\D_{2,n}=cos\left(\sqrt{4n+2}gF(t)\right)\,\text{,}\\D_{3,n+1}=-\frac{i\sqrt{n+1}}{\sqrt{2n+1}}\sin(\theta)\sin\left(\sqrt{4n+2}gF(t)\right)\,\text{.}\end{array}$$
(31)

Finally, we consider the finally case,

$$|\psi (t)\rangle =U(t)|{u}_{1},n\rangle ,$$
(32)

that is, the atoms are in the excited state, and so we obtain

$$|\psi (t)\rangle =\sum_{n=0}^{\infty }[({F}_{n}{E}_{1,n}(t))|{u}_{1},n\rangle +({F}_{n}{E}_{2,n+1}(t))|{u}_{2},n+1\rangle +({F}_{n}{E}_{3,n-2}(t))|{u}_{3},n+2\rangle ],$$
(33)

where

$$\begin{array}{l}E_{1,n}=-\frac1{2n+3}\left((n+1)\cos\left(\sqrt{4n+6}gF(t)\right)+n+2\right)\,\text{,}\\E_{2,n+1}=-\frac{i\sqrt{n+1}}{\sqrt{2n+3}}\sin\left(\sqrt{4n+6}gF(t)\right),\\E_{3,n+2}=\frac{\sqrt{(n+1)(n+2)}}{2n+3}\left(\cos\left(\sqrt{4n+6}gF(t)\right)-1\right)\,\text{.}\end{array}$$
(34)

Using the above results, the coefficients A, B and C of the Eq. (13) are calculated as:

$$\begin{array}{l}A=e^{i\phi}\sin^2\frac\theta2F_{n+2}C_{1,n}(t)+\frac{\sqrt2}2\sin\;\theta F_{n+1}D_{1,n}(t)+e^{-i\phi}\cos^2\frac\theta2F_nE_{1,n}(t),\\B=e^{i\phi}\sin^2\frac\theta2F_{n+1}C_{2,n}(t)+\frac{\sqrt2}2\sin\;\theta F_nD_{2,n}(t)+e^{-i\phi}\cos^2\frac\theta2F_{n-1}E_{2,n}(t),\\C=e^{i\phi}\sin^2\frac\theta2F_nC_{3,n}(t)+\frac{\sqrt2}2\sin\;\theta F_{n-1}D_{3,n}(t)+e^{-i\phi}\cos^2\frac\theta2F_{n-2}E_{3,n}(t).\end{array}$$
(35)

Now, using the above coefficients, we calculate the density operator from the Eq. (21) and by employing it, we are able to compute the entropy squeezing from the Eq. (20) for different values of \(\theta\). Figures 1 and 2 show our numerical results where will be discussed in the next section.

Fig. 1
figure 1

Time evolution of information entropy squeezing \(E\left({S}_{y}\right)\) at θ = π, ϕ = π, and \(\overline{n }\)= 36, where a is for the atomic motion neglected; b–d are for the atoms moving at speed v = gL/π, and panel b is for p = 1; panel c is for p = 2; panel d is for p = 5

Full size image
Fig. 2
figure 2

Time evolution of information entropy squeezing factor \(E\left({S}_{y}\right)\) and \(E\left({S}_{x}\right)\) with the two atoms moving at speed v = gL/π and \(\overline{n }=36,\phi =\pi ,p=1\), for different \(\theta\)

Full size image

We also study the entanglement between the field and the atomic system by the linear entropy which defined as [50]

$$\Lambda \left(t\right)=\frac{1}{2}\left(1-{\xi }^{2}\left(t\right)\right),$$
(36)

where \(\xi \left(t\right)\) is the radius of the Bloch sphere and is given by the following equation

$$\xi \left(t\right)=\sqrt{{\langle {\sigma }_{x}\left(t\right)\rangle }^{2}+{\langle {\sigma }_{y}\left(t\right)\rangle }^{2}+{\langle {\sigma }_{z}\left(t\right)\rangle }^{2}}.$$
(37)

\(\langle {\sigma }_{i}\rangle ,i=x,y,z\) is the expectation values of the atomic variable described by the spin- 1/ 2 matrices \({\sigma }_{i}\). The linear entropy relation given in Eq. (36) is referred to as the mixedness in the bipartite and it is worth noting that, for the pure state \(\xi \left(t\right)=1\) then \(\Lambda \left(t\right)=0\), hence the system is not entangled while for \(\xi \left(t\right)=0\) or \(\Lambda \left(t\right)=\frac{1}{2}\), we have the maximum entanglement.

We have computed the linear entropy of system for some given values of parameters and shown them in Fig. 3. The results will be also discussed in Section 4.

Fig. 3
figure 3

Linear entropy against with the two atoms moving at speed v = gL/π and \(\overline{n }=36,\phi =\pi ,\), for different values of \(\theta\) and p. In a \(\theta =0,p=1\) b \(\theta =\pi ,p=1\) c \(\theta =0,p=1\) d \(\theta =\pi ,p=2\) e \(\theta =\frac{\pi }{2},p=1\)

Full size image

In continue and for another attempt, we try to obtain the Fisher information measure for the system. According to Refs. [28, 30], the atomic quasi probability distribution function \({Q}_{A}\left(\Theta ,\Phi ,t\right)\)(the Q function) is defined as

$${Q}_{{A}_{1}}\left(\Theta ,\Phi ,t\right)=\frac{1}{2\pi }\left.\langle \Theta ,\Phi \right|{\rho }_{{A}_{2}F}\left|\Theta ,\Phi \right.\rangle ,$$
(38)

which depends on the atomic phase parameters \(\Theta\) and \(\Phi\). For calculating this function, we use the atomic coherent state given by Eq. (33), and the reduced density operator \({\rho }_{{A}_{2}F}\) given by Eq. (21). On the other hands, for any distribution function \(f\left(x\right)\), the Fisher information measure is defined as [25]

$$I=\int f\left(x\right){\left\{\frac{\partial ln\left(f\left(x\right)\right)}{\partial x}\right\}}^{2}dx,$$
(39)

which has encountered many physical applications [36, 51]. For the atomic Q-function obtained from the density function, or for the Husimi distribution, the above equation becomes

$$I_{A_2F}=\sum\limits_{j=1}^2\int_0^{2\pi}\int_0^\pi Q_{A_1}\left(\mathrm\Theta,\mathrm\Phi,\mathrm t\right)\left\{\sigma_{\vartheta_j}\frac{\partial\ln Q_{A_1}\left(\Theta,\Phi,t\right)}{\partial\vartheta_j}\right\}^2\sin\left(\Theta\right)\mathrm d\Theta\mathrm d\Phi\text{,}$$
(40)

where \(\left(\vartheta_1,\vartheta_2\right)=\left(\Theta,\Phi\right)\) and \({{\sigma }_{j}}^{2}=\langle {{X}_{j}}^{2}\rangle -{\langle {X}_{j}\rangle }^{2}\) is the variances for the variables \(\Theta\) and \(\Phi\) [28].

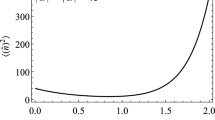
We have calculated the atomic FI via atomic coherent state by choosing the initial state of atoms and some given values of parameters of system and have shown the numerical results in Fig. 4. We discuss about the result in next section.

Fig. 4
figure 4

Time evolution of the AFI factors at ϕ = π and \(\overline{n }=36\), where a is for the atomic motion neglected; b–d are for the atoms moving at speed v = gL/π and p = 1; and panel b is for \(\theta =0\) and; panel c is for \(\theta =\pi\); panel d is for \(\theta =\frac{\pi }{2}\) panel; e–f are \(\theta =\pi\); e for p = 2 and f for p = 5

Full size image

4 Result and discussion

Figure 1 shows the time evolution of the information entropy squeezing in the direction \(E\left({S}_{y}\right)\) at \(\theta =\pi ,\phi =\pi ,\overline{n }=36.\). In Fig. 1a, we have neglected the atomic motion and the field mode structure parameter is equal to zero, and in Fig. 1b–d, the atoms are moving at a speed \(v=\frac{gL}{\pi }\) and the field mode structure parameter p is equal to 1, 2 and 5, respectively. From these figures, it can be seen that in Fig. 1a, except at initial stage of time, we cannot observe any squeezing and from Fig. 1b–d, we see that the squeezing is repeated with regular fluctuations and by increasing of p, the periodicity of \(E\left({S}_{y}\right)\) and also the squeezing time of information entropy increase. As a result, we can deduce that the atomic motion in the cavity leads to the periodic time evolution of the atomic squeezing \(E\left({S}_{y}\right)\), in other words the squeezing time of information entropy and the period of entropy squeezing can be determined by the field mode structure. These results are agreement with the results given in Ref [43]. On the other hand, in Ref. [43], it is shown that \(E\left({S}_{x}\right)\) cannot exhibit squeezing in the entropy, while in this work and by choosing the atomic coherent state as the initial state, the \(E\left({S}_{x}\right)\) can be occurred.

In Fig. 2, we have shown the time evolution of information entropy squeezing \(E\left({S}_{y}\right)\) and \(E\left({S}_{x}\right)\) with the two atoms moving at speed \(v=\frac{gL}{\pi }\) and \(\overline{n }=36,\phi =\pi ,p=1\) for different values of \(\theta\). We have plotted the figures that exhibit some degree of entropy squeezing. From the figures, it is seen that when the entropy squeezing occurs in \(E\left({S}_{y}\right)\) then the squeezing in \(E\left({S}_{x}\right)\) cannot be observed and vice versa. It is also seen that for \(\theta=0\;\text{and}\;\pi\), \(E\left({S}_{y}\right)\) exhibits maximal entropy squeezing and by increasing \(\theta\) to \(\theta =\pi /8\) and \(\theta =\pi /6\), the entropy squeezing of \(E\left({S}_{y}\right)\) decreases. In Fig. 2d, we observe that for \(\theta =\pi /4\), the entropy squeezing in \(E\left({S}_{x}\right)\) occurs and when two atoms are initially in a maximal entangled state for \(\theta =\pi /2\), the entropy squeezing \(E\left({S}_{x}\right)\) is maximal. It is noticeable that by choosing the initial state given in Ref [39, 43]. one cannot see any entropy squeezing in \(E\left({S}_{x}\right)\), while here we can see it. Therefore, the squeezing time and degree of entropy squeezing dependent on the initial atomic state of the atoms while the period of the time evolution of squeezing keeps invariant. In other words, we can deduce that the initial atomic state and the field mode structure can determine the squeezing time and the squeezing degree. The period of the atomic entropy squeezing can also be determined by choosing atomic motion and the field mode structure.

Figure 3 shows the influence of the physical parameters on the entanglement of system by the linear entropy. From this figure, we observe that the linear entropy has a periodic behavior and it is repeated with regular fluctuations and in each period the entanglement reaches to a maximum peak and in one moment, there is no entanglement. From Fig. 3a and c, which plotted for \(\theta=0\;\text{and}\;\pi\) respectively and for p = 1, we see that the entanglement becomes the maximum amount (\(\Lambda =0.5\)). Figure 3b and d show that by increasing the atomic motion, the number of periodicity is increased, and Fig. 3e shows that the amount of entanglement is reduced. Hence, we can deduce that the atomic entropy squeezing not only is an accurate measure of squeezing but also has some properties corresponding to the entanglement between the two atoms and the field.

Figure 4 shows the time evolution of the AFI for some given physical parameters of the system and it is observed that by considering the atomic motion, the AFI fluctuates regularly and periodically with period. In other word, the change of the parameter p causes the periodic behavior and AFI has the same behavior shown in Figs. 1, 2 and 3. In Fig. 4a, we have ignored the motion factor and so the AFI is not periodic while from Fig. 4b– d, it is seen that for p = 1 and for different values of θ the atomic Fisher information is periodic. Also, by increasing p, the number of periodicities is increased which shown in Fig. 4e and f. According to Figs. 3a and 4b, it can be also seen that for \(\theta =0,p=1\), the increasing of the entanglement corresponds to the decreasing of the AFI and vice versa. Similar comparison can be deduced from the other numerical results, for example, when we have the maximal entanglement then the obtained AFI is minimal. Hence, we can deduce that the statistical quantity AFI has a strongly dependent with the QE and the motion factor of the atoms and so one can find a reasonable comparison between the AFI and some other information entropies such as the Shannon entropy, squeezing and the linear entropy.

5 Conclusion

In this paper, we have investigated the entropy squeezing for a single atom interacting with an external field. We have shown that the choice of the atomic coherent state as the initial state is effective in entropy squeezing and the squeezing in x direction occurs while it was shown in Ref. [43] that squeezing occurs only in y direction. We have also shown that the period of the atomic entropy squeezing is affected by choosing atomic motion and the field mode structure and the time periodic mode of squeezing increases with increasing the motion atomic parameter p. We have also observed that when the entropy squeezing occurs in \(E\left({S}_{y}\right)\) then the squeezing in \(E\left({S}_{x}\right)\) cannot be observed and vice versa. In the following, we have investigated the influence of the physical parameters on the atomic fisher information and the linear entropy and have concluded that the entropy squeezing, AFI and entanglement can be controlled by changing of some factors such as the external field, the initial state of the atom or the choice of atomic motion. According to the results, we have deduced that the atomic entropy squeezing not only is an accurate measure of squeezing but also has some properties corresponding to the entanglement between the two atoms. On the other hands, when we consider the moving atomic coherence system, the entanglement and the AFI have the periodic behavior and repeat with regular fluctuations. We have also shown that when maximum entanglement occurs, the squeezing does not occur and it corresponds to the minimum value of AFI and increasing the entanglement corresponds to decreasing the AFI and vice versa. Therefore, these results and the squeezed states or the entangled states can offer possibilities of improving performance of optical devices and they are applicable for the optical communication networks as well as for many optical devices.