Surely, one would like to be able to deduce the quantitative laws of quantum mechanics directly from their anschaulich foundations, that is, essentially, relation \({\delta }p\,{\delta }q \thicksim h\). (Werner Heisenberg [8], p. 196)
Abstract
For a simple set of observables we can express, in terms of transition probabilities alone, the Heisenberg uncertainty relations, so that they are proven to be not only necessary, but sufficient too, in order for the given observables to admit a quantum model. Furthermore distinguished characterizations of strictly complex and real quantum models, with some ancillary results, are presented and discussed.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
1 Foreword
The problem of understanding the empirical basis of the quantum mechanical formalism has been approached, starting from eighties, by means of the method of statistical invariants [1, 2]. The main idea of this approach, borrowed from the Klein’s program of Erlangen [3], is to classify the probabilistic models according to statistical invariants, expressed in terms of the transition probabilities of the physical observables. That is to say, one considers the transition probabilities as the basic empirical data from which the mathematical model should be deduced. The statistical invariants for some simple systems were explicitly computed and it was shown how they allowed to distinguish among Kolmogorovian, real Hilbert space and complex Hilbert space models. Actually necessary and sufficient conditions for the existence of each model were found [1, 4, 5]. When applied to the quantum-mechanical transition probabilities, they proved not only the necessity of a non classical probabilistic model, but also the necessity of using complex rather than real Hilbert spaces [1, 5], so offering the solution to the open problem of “...singling out in full generality the empirical basis for the choice of complex numbers in quantum theory....” [6]. Furthermore the Kolmogorovian statistical invariant was recognized as a form of the celebrated Bell inequality [1], expressed by the transition probabilities instead of the correlation functions. In the present paper we pursue the study of a triple of two-dimensional observables undertaken in [1] and, by means of the notion of quantum models, we show as their statistical invariants represent the Heisenberg uncertainty relations expressed in terms of transition probabilities alone. This allow us to affirm that uncertainty relations are not only necessary, but sufficient too, in order for the given observables to admit a quantum model.
2 Preliminary Definitions and Results
In the oft quoted paper [1] a triple A, B, C of two-valued observables subject to take values \((a_{\alpha }),(b_{\beta }),(c_{\gamma })\) were studied, given their transition probabilities
under the symmetry assumptionsFootnote 1
By observable it is meant any quantity arising from an experiment, regardless of its nature. The transition probability \(P(A=a_{\alpha }|B=b_{\beta })\) is the conditional probability that A takes the value \(a_{\alpha }\) conditioned by the fact that B is known to assume the value \(b_{\beta }.\) We will denote the transition probabilities (1) as
assuming, unless otherwise specified, that
for which the angles can be chosen such that
The transition probabilities (1) were said to admit a complex (resp. a real) Hilbert space model if there exist three orthonormal bases \(\{\phi _{\alpha }\},\{\psi _{\beta }\},\{\chi _{\gamma }\}\) of a two-dimensional complex (resp. real) Hilbert space \(\mathscr {H}\) such that
In particular a complex Hilbert space model was said a spin model if the three o. n. bases can be taken as the normalized eigenvectors \(\psi _{\alpha }(u_A),\psi _{\beta }(u_B),\psi _{\gamma }(u_C)\) \((\alpha ,\beta ,\gamma =1,2)\) of the spin operators \(u_A\cdot \sigma ,u_B\cdot \sigma ,u_C\cdot \sigma \) for some \(u_A,u_B,u_C\in S^{(2)},\) where \(S^{(2)}\) denotes the real unit sphere in \(\mathbb {R}^3\) and \(u\cdot \sigma \) is defined in terms of the Pauli matrices
as
In the latter case we can write (8), in terms of the angles \(\widehat{u_Au_B},\widehat{u_Bu_C},\widehat{u_Cu_A},\) as
In the present paper we prefer to focus our attention on observables rather than on transition probabilities alone. So we can recover the usual formalism of quantum mechanics [7] according to which observables are postulated in correspondence with self-adjoint operators on a suitable complex Hilbert space. In this frame the uncertainty relations we are concerned with find their most general formulation, so that we are led to give the following
Definition 2.1
The observables A, B, C are said to admit a quantum model if and only if there exist a complex Hilbert space \(\mathscr {H}\) and self-adjoint operators \({\hat{A}},{\hat{B}},{\hat{C}}\) actingFootnote 2 on it such that the values of each observable coincide with the eigenvalues of the corresponding operator and the transition probabilities (1) admit the complex Hilbert space model defined by the o. n. bases of \(\mathscr {H}\) made up of the normalized eigenvectorsFootnote 3 of \({\hat{A}},{\hat{B}},{\hat{C}}.\) The model will be called a real quantum model if \(\mathscr {H}\) can be taken real, or a strictly complex quantum model otherwise.
Next theorem shows a first expected link between quantum models of observables and Hilbert space models of the relative transition probabilities.
Theorem 2.1
The following assertions are equivalent:
-
(i)
the observables A, B, C admit a quantum model;
-
(ii)
their transition probabilities admit a spin model;
-
(iii)
their transition probabilities admit a complex Hilbert space model.
Proof
To prove that (i) is equivalent to (ii) it is suffices to observe that, for every observable X (with values \(x_{1,}x_2\text{) }\) of the triple A, B, C, the operator
where \({\hat{1}}\) is the identity operator, has the eigenvalues  , so it is a spin operator and, due to \([{\hat{S}}_X,{\hat{X}}]=0,\) it has the same eigenvectors of \({\hat{X}}.\) Further, the equivalence between (ii) and (iii) was proven in [1], Theorem 7.Footnote 4 \(\square \)
, so it is a spin operator and, due to \([{\hat{S}}_X,{\hat{X}}]=0,\) it has the same eigenvectors of \({\hat{X}}.\) Further, the equivalence between (ii) and (iii) was proven in [1], Theorem 7.Footnote 4 \(\square \)
Remark 2.1
The particular case of real quantum models will be discussed later. Notice that the previous result deals with a (linear) rescaling of the observables preserving probabilities. Further observe that the spin operators \({\hat{S}}_A,\;{\hat{S}}_B,\;{\hat{S}}_C\) can be written as \(u_A\cdot \sigma ,\;u_B\cdot \sigma ,\;u_C\cdot \sigma \) respectively, for suitable unit vectorsFootnote 5 \(u_A,\;u_B,\;u_C\in S^{(2)},\) which so remain associated to the self-adjoint operators \({\hat{A}},\;{\hat{B}},\;{\hat{C}}\) respectively.
In this framework we are able to reformulate some results of [1] as follows:
Theorem 2.2
The following assertions are equivalent:
-
(i)
the observables A, B, C admit a quantum model;
-
(ii)
there existFootnote 6 \(u_A,u_B,u_C\in S^{(2)}\) such that \(u_A\cdot u_B=\cos \alpha ,\;u_B\cdot u_C=\cos \beta ,\;u_C\cdot u_A=\cos \gamma ;\)
-
(iii)
\(1-\cos ^2\alpha -\cos ^2\beta -\cos ^2\gamma +2\;\cos \alpha \;\cos \beta \;\cos \gamma \ge 0\).
Moreover inequality (iii) is saturated if and only if A, B, C admit a real quantum model; in such a case, and only then, the unit vectors in (ii) are coplanar.
Proof
By Theorem 2.1, (i) is equivalent to the existence of a spin model for the transition probabilities; this in turn, by Eqs. (8), (11) and (12), as well as due to \(\cos \widehat{u_Au_B}=u_A\cdot u_B,\cos \widehat{u_Bu_C}=u_B\cdot u_C,\widehat{u_Au_C}=u_A\cdot u_C,\) is easily recognized equivalent to (ii); the equivalence between (ii) and (iii) was established in [1] (see footnote 4), Proposition 3, just as, from Theorems 9 and 10 therein, it follows straight the last statement to be proven. \(\square \)
Remark 2.2
The inequality in (iii) of the previous theorem is said a statistical invariant [2] for a quantum model. It is said in particular a statistical invariant for a real quantum model if the inequality is saturated, or a statistical invariant for a strictly complex quantum model, otherwise. Many equivalent forms of these invariants were discovered in [1] (see footnote 4) and some others, involving uncertainty relations, will appear below.
3 Uncertainty Relations
Uncertainty relations were introduced in quantum mechanics by Heisenberg [8] and successively extended and strengthen by many authors [10, 11].Footnote 7 In this paper we will refer to the following stronger form:
Theorem 3.1
(Schrödinger [11]) For every couple of self-adjoint operators \({\hat{X}},{\hat{Y}}\) acting on a complex Hilbert space \(\mathscr {H}\) and for every stateFootnote 8 \(\psi \) the following inequality holds:Footnote 9
where \(\langle {\hat{Z}}\rangle :=\langle \psi |{\hat{Z}}|\psi \rangle \) and \( Var ({\hat{Z}}):=\langle \widehat{Z}^2\rangle -\langle {\widehat{Z}}\rangle ^2\) are resp. the average of \({\hat{Z}}\) and the variance of \({\hat{Z}}\) in the state \(\psi ,\) with \([{\hat{X}},{\hat{Y}}]:={\hat{X}}{\hat{Y}}\;-\;{\hat{Y}}{\hat{X}}\) and \(\{{\hat{X}},{\hat{Y}}\}:={\hat{X}}{\hat{Y}}\;+\;{\hat{Y}}{\hat{X}}\) being the commutator, resp. the anticommutator, of \({\hat{X}}\) and \({\hat{Y}}.\)
Proof
Cfr. for example reference [12] and, of course [11]. \(\square \)
Remark 3.1
In the following Lemma 4.4 we show that, for the operators we consider in this paper, the inequality expressing the Heisenberg–Schrödinger uncertainty relation is in fact saturated, so that it assumes the form of an identity. From now on \(\mathscr {H}\) will denote a two-dimensional complex Hilbert space.
4 Some Useful Lemmas
Lemma 4.1
Whichever \({\hat{X}},{\hat{Y}},{\hat{Z}}\) are taken among the operators \({\hat{A}},{\hat{B}},{\hat{C}}\) associated to the given observables A, B, C one has, for every state,
and
where \(z_{1,}z_2\) are the values of the observable Z, as well as
and
Consequently the Heisenberg–Schrödinger uncertainty relation (14) holds for a couple of operators \({\hat{X}},{\hat{Y}}\) acting on \(\mathscr {H}\) if and only if it holds for the associated spin operators \(u_X\cdot \sigma ,\;\;u_Y\cdot \sigma .\) Furthermore the former relation is saturated if and only if the latter is.
Proof
Cfr. Appendix 2. \(\square \)
Lemma 4.2
For every \(u,v,w\in S^{(2)}\) the following identities hold in each eigenstate \(\psi _k(w)\) of \(w\cdot \sigma \) \((k=1,2)\):
and just for every state:
Proof
Cfr. Appendix 3 \(\square \)
Lemma 4.3
For every state \(\psi \) of the Hilbert space \(\mathscr {H}\) there is a \(w\in S^{(2)}\) such that \(\psi =\psi _1(w),\) where \(\psi _1(w)\) is the eigenstateFootnote 10 of the spin operator \(w\cdot \sigma ,\) corresponding to the eigenvalue 1.
Proof
We can put, up to an irrelevant phase factor, \(\psi =\left[ \begin{matrix}\left| \psi _1\right|&\mathfrak {R}(\psi _2)+i\mathfrak {I}(\psi _2)\end{matrix}\right] ^T\) while as knownFootnote 11 \(\psi _1(w)=\left[ \begin{array}{ll}\sqrt{\frac{1+w_3}{2}}&{}\frac{w_1+i\;w_2}{\sqrt{2(1+w_3)}}\\ \end{array}\right] ^T,\) so that we can solve the vector equation \(\psi _1(w)=\psi \) for w, getting \(w_1=2\left| \psi _1\right| \;\mathfrak {R}(\psi _2),\) \(w_2=2\left| \psi _1\right| \;\mathfrak {I}(\psi _2),\) \(w_3=2\left| \psi _1\right| ^2-1.\) \(\square \)
Lemma 4.4
For every couple of self-adjoint operators \({\hat{X}},{\hat{Y}}\) on a two-dimensional complex Hilbert space \(\mathscr {H}\) and for every state \(\psi \) the following identity holds:
Proof
Due to Lemma 4.1 we can limit ourselves to the case in which \({\hat{X}},{\hat{Y}}\) are spin operators, that is \({\hat{X}}=u\cdot \sigma \) and \({\hat{Y}}=v\cdot \sigma \) for suitable \(u,v\in S^{(2)}.\) However the generic state \(\psi ,\) by Lemma 4.3, can be written as \(\psi =\psi _1(w),\) for a suitable \(w\in S^{(2)}.\) So (23) by means of Lemma 4.2 can be written as
expanding and simplifying it becomes
which is surely identically satisfied, since each side equals the square of the volume of the parallelepiped of sides u, v, w. \(\square \)
5 The Main Result
Theorem 5.1
Assuming that the two-valued observables A, B, C admit a quantum model, the following assertionsFootnote 12 hold and are equivalent:
(i) \({\hat{A}},{\hat{B}}\) satisfy the saturated Heisenberg–Schrödinger uncertainty relation for every state:
(ii) the following inequalityFootnote 13 holds in each eigenstate \(\psi _k(C)\) of C \((k=1,2):\)
(iii) the following inequality holds:
Furthermore (iii) implies the hypothesis and the previous inequalities are saturated if and only if A, B, C admit a real quantum model.
Proof
(i) follows from the hypothesis by Lemma 4.4, since the operators associated to A, B, C are self-adjoint, acting on a two-dimensional complex Hilbert space, by definition of quantum model. If (i) holds for every state then, in particular, it shall hold in each of the two eigenstates of C; so, omitting the last addend and taking the square roots, one gets (ii). By Lemma 4.1, we can replace the operators \({\hat{A}},{\hat{B}},{\hat{C}}\) with the corresponding spin operators \(u_A\cdot \sigma ,\;\;u_B\cdot \sigma ,\;\;u_C\cdot \sigma ,\) achieving in each of the two eigenstates \(\psi _k(u_C)\)
so the difference between the squares of l.h.s. and the r.h.s. must satisfy
The latter, taking account of (24) and (25) in the proof of Lemma 4.4, can be written
namely, since \(\cos \widehat{u_Au_B}=u_A\cdot u_B,\;\cos \widehat{u_Bu_C}=u_B\cdot u_C,\;\widehat{u_Cu_A}=u_C\cdot u_A,\)
and this, by virtue of (iii) of Theorem 2.2, implies the hypothesis. Furthermore (32) is also equivalent to (iii) because, with the notations of Sect. 3, its l.h.s. equalsFootnote 14 \(4(4pqr-(p+q+r-1)^2)\). Finally the saturation statement follows from the last part of Theorem 2.2. \(\square \)
Remark 5.1
It appears quite astonishing that the, apparently, much weaker relation in (ii) above turns out rather to be equivalent to the Heisenberg–Schrödinger uncertainty relation in full generality.
6 Ancillary Results
Corollary 6.1
Under the hypothesis of the preceding theorem, the observables A, B, C admit a strictly complex quantum model, resp. a real quantum model, if and only ifFootnote 15 \(\langle [{\hat{A}},{\hat{B}}]\rangle _{\psi _k(C)}\ne 0,\) resp. \(\langle [{\hat{A}},{\hat{B}}]\rangle _{\psi _k(C)}=0,\) in each eigenstate \(\psi _k(C)\) of C \((k=1,2)\).
Proof
It has been shown in the proof of the preceding theorem that the assertion ii) is equivalent to the inequality (31), whose l.h.s., taking account of (25), equals \(((u_A\times u_B)\cdot u_C)^2\) that, in turn, due to Eq. (21) of Lemma 4.2, equals \((\frac{1}{2i}\langle [u_A\cdot \sigma ,u_B\cdot \sigma ]\rangle _{\psi _1(u_C)})^2.\) Therefore the assertion ii) of the theorem, thanks to Lemma 4, turns out to be equivalent to \((\langle [{\hat{A}},{\hat{B}}]\rangle _{\psi _k(C)})^2\ge 0.\) Moreover it will be saturated as soon as (27) will be and this completes the proof. \(\square \)
Corollary 6.2
If the observables A, B, C admit a strictly complex quantum model then every couple of the associated operators do not commute. Further, for every state, at least two couples have non vanishing commutators averages.
Proof
Let X, Y, Z be whichever permutation of A, B, C. The assumption \([{\hat{X}},{\hat{Y}}]=0\) would imply \(\langle [{\hat{X}},{\hat{Y}}]\rangle _{\psi _1(Z)}=0,\) against what asserted in the preceding Corollary 6.1, and this proves the first statement. Further, by Lemma 4.4, for every state \(\psi \) there is a unit vector \(w\in S^{(2)}\) such that, due to Eq. (21) of Lemma 4.2, we can write
If this is zero then w belongs to the plane spanned by \(u_X,u_Y\) and may be neither in the plane \(u_Y,u_Z\) nor in the plane \(u_Z,u_X,\) because \(u_A,u_B,u_C\) are not coplanarFootnote 16 by Theorem 2.2. So we get \(\frac{1}{2i}\langle [u_Y\cdot \sigma ,u_Z\cdot \sigma ]\rangle _{\psi }\ne 0.\) and \(\frac{1}{2i}\langle [u_Z\cdot \sigma ,u_X\cdot \sigma ]\rangle _{\psi }\ne 0.\) and, by Eq. (18) of Lemma 4.1, the last statement is proven as well. \(\square \)
7 Conclusions
We have proven that the statistical invariant \(4pqr-(p+q+r-1)^2\ge 0\) is the expression of the Heisenberg–Schrödinger uncertainty relations for every couple of observables of the considered system. It depends neither on the values of the observables nor on their scales and units of measure but only on the transition probabilities and provides a condition not only necessary, but sufficient too, in order for a quantum model to exist. In particular the inequality is strict if and only if there exists a strictly complex quantum model. In this case some ancillary results involving commutators have been found. Furthermore real quantum models have been characterized by the saturation of the uncertainty relation (ii) in Theorem 5.1 or, alternatively, by the vanishing of the commutator average appearing in Corollary 6.1 or, definitively, in terms of transition probabilities alone, by the equation \(4pqr-(p+q+r-1)^2=0.\) The latter confirms however the exceptional character of real quantum models, requiring an unlikely functional dependence of the given transition probabilities. In closing, one should highlight that, as the transition probabilities can be estimated starting from relative frequencies experimentally observed, we are able in principle, for the considered simple system, to deduce the mathematical quantum formalism from the Heisenberg uncertainty relations alone.
Notes
In all the equations, ellipsis stands for all similar relations involving the observables left over.
\({\hat{A}},{\hat{B}},{\hat{C}}\) are defined up to a common unitary transformation, cfr. [1, Corollary 8]. A self-adjoint operator having eigenvalues
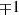 is just a spin operator.
is just a spin operator.Said eigenstates as well.
Cfr. Appendix 1.
Any common unitary transformation of \({\hat{A}},{\hat{B}},{\hat{C}},\) referred to in note 2, induces a common rotation of \(u_A,u_B,u_C,\) cfr. [1, Corollary 8] proof.
Observe that \(u_A,u_B,u_C\) are necessarily distinct and pairwise non collinear, due to the assumption (5).
For a recent review cfr. [9] and the bibliography therein.
As known a state is defined as a norm 1 element of \(\mathscr {H}\) up to a phase factor.
The first addend in the r.h.s. is said the covariance term and the second the commutator term.
One has also that \(\psi =\psi _2(-w)\), but it is not required here; w is known as a representation of the state \(\psi \) on the Bloch’s sphere \(S^{(2)}\).
Similar assertions hold taking any permutation of the operators \({\hat{A}},{\hat{B}},{\hat{C}}.\)
where \(\Delta {\hat{Z}}:=\sqrt{ Var ({\hat{Z}})}\) denotes the standard deviation of \({\hat{Z}}.\) The r.h.s. is said the correlation term.
Cfr. Appendix 4.
Cfr. Note 12. The symbol \(\langle \;\; \rangle _{\psi }\) denotes the average computed in the state \(\psi .\)
Furthermore cfr. note 6.
\(\varepsilon _{ jkl }\) denotes the Levi-Civita symbol; on repeated indices summation is understood.
References
Accardi, L., Fedullo, A.: On the statistical meaning of complex numbers in quantum mechanics. Lett. Nuovo Cim. 34(7), 161–172 (1982)
Accardi, L.: Some trends and problems in quantum probability. In: Accardi, L., Frigerio, A., Gorini, V. (eds.) Quantum probability and applications to the quantum theory of irreversible processes, pp. 1–19. Lecture Notes in Mathematics, Vol. 1055, Springer, Berlin (1984)
Klein, F.: Vergleichende Betrachtungen über neuere geometrische Forschungen. Math. Ann. 43, 63–100 (1893). Gesammelte Abh., Springer, 1, 460–497 (1921). English translation: a comparative review of recent researches in geometry, by Mellen Haskell, Bull. N. Y. Math. Soc., 2 (10), 215–249 (1893)
Gudder, S., Zanghì, N.: Probability models. Il Nuovo Cim. 79 B(2), 291–301 (1982)
Fedullo, A.: On the existence of a Hilbert space model for finite valued observables. Il Nuovo Cim. 107 B(12), 1413–1426 (1992)
Jauch, J.M.: Foundations of Quantum Mechanics. Addison-Wesley Publishing Company, Boston (1968)
von Neumann, J.: Mathematische Grundlagen der Quantenmechanik, Die Grundlehren der Mathematischen Wissenschaften, Band 38. Springer, Berlin (1932). English translation: Mathematical Foundations of Quantum Mechanics, Princeton University Press (1971)
Heisenberg, W.: Uber den anschaulichen Inhalt der quantentheoretischen Kinematik und Mechanik. Z. Phys. 43(3–4), 172–198 (1927). English translation in [13, 62–84]
Sen, D.: The uncertainty relations in quantum mechanics. Curr. Sci. 107(2), 203–218 (2014)
Robertson, H.P.: The uncertainty principle. Phys. Rev. 34, 573–574 (1929). Reprinted in [13, 127–128]
Schrödinger, E.: The uncertainty relations in quantum mechanics. Zum Heisenbergschen Unschärfeprinzip, Sitzungsberichte der Preussischen Akademie der Wissenschaften, Physikalisch-mathematische Klasse 14, 296–303 (1930)
Griffiths, D.: Quantum Mechanics. Pearson, Upper Saddle River (2005)
Wheeler, J.A., Zurek, W.H. (eds.): Quantum Theory and Measurement. Princeton University Press, Princeton (1983)
Author information
Authors and Affiliations
Corresponding author
Appendix
Appendix
1. Results quoted from [1].
Theorem 7
The following assertions are equivalent:
-
(i)
the transition matrices P, Q, R admit a complex Hilbert space model;
-
(ii)
the transition matrices P, Q, R admit a spin model;
-
(iii)
\(\cos ^2\alpha +\cos ^2\beta +\cos ^2\gamma -1\le 2\;\cos \alpha \;\cos \beta \;\cos \gamma ;\)
-
(iv)
\(-1\le \frac{\cos ^2\frac{\alpha }{2}+\cos ^2\frac{\beta }{2}+\cos ^2\frac{\gamma }{2}\;-\;1}{2\cos \frac{\alpha }{2}\cos \frac{\beta }{2}\cos \frac{\gamma }{2}}\le 1;\)
-
(v)
\(-1\;\le \;\frac{p\;+\;q\;+\;r\;-\;1}{2\sqrt{p\;q\;r}}\;\le \;1;\)
-
(vi)
\([\sqrt{pq}-\sqrt{(1-p)(1-q)}]^2\;\le \;r\;\le \;[\sqrt{pq}+\sqrt{(1-p)(1-q)}]^2\)
Proposition 3
Three vectors \(a,b,c\in S^{(2)}\) satisfying
exist if and only if
Theorem 9
The transition matrices P, Q, R admit a real Hilbert space model if and only if \(\frac{p\;+\;q\;+\;r\;-\;1}{2\sqrt{p\;q\;r}}\;=\;+1\) or \(\frac{p\;+\;q\;+\;r\;-\;1}{2\sqrt{p\;q\;r}}\;=\;-1\) or equivalently \(\sqrt{r}\;=\;\sqrt{pq}+\sqrt{(1-p)(1-q)}\) or \(\sqrt{r}\;=\;\left| \sqrt{pq}-\sqrt{(1-p)(1-q)}\right| \).
Theorem 10
The transition matrices P, Q, R admit a real Hilbert space model if and only if they admit a spin model defined by a coplanar triple of vectors in \(S^{(2)}\).
2. Proof of Lemma 4.1
Putting for simplicity \(z_{+\ }:=\frac{z_1+z_2}{2}\) and \(z_{-\ }:=\frac{z_1-z_2}{2},\) by equation (10) we get
and, since \(\langle (u_Z\cdot \sigma )^2\rangle =\langle {\hat{1}}\rangle =1,\)
from which we obtain
Further, with easily understood notations, we have
so that
last, quite directly, we get
\(\square \)
3. Proof of Lemma 4.2
By definition \(u\cdot \sigma =\left[ \begin{matrix}u_3&{}u_1-iu_2\\ u_1+iu_2&{}-u_3\end{matrix}\right] ,\) so that for every state \(\psi \) one has
SinceFootnote 17 as known \(\psi _1(w)=\left[ \begin{array}{ll}\sqrt{\frac{1+w_3}{2}}&{}\frac{w_1+i\;w_2}{\sqrt{2(1+w_3)}}\\ \end{array}\right] ^T\) and \(\psi _2(w)=\left[ \begin{array}{ll}\sqrt{\frac{1-w_3}{2}}&{}-\frac{w_1+i\;w_2}{\sqrt{2(1-w_3)}}\\ \end{array}\right] ^T,\) putting them in the former formula, with easy calculations we get \(\langle u\cdot \sigma \rangle _{\psi _1(w)}=u\cdot w\) and \(\langle u\cdot \sigma \rangle _{\psi _2(w)}=-u\cdot w\) as asserted in Eq. (19). Further it is soon seen that \((u\cdot \sigma )^2={\hat{1}},\) so that \(\langle (u\cdot \sigma )^2\rangle =1,\) thus, in the said states, \( Var (u\cdot \sigma )=\langle u\cdot \sigma \rangle ^2-(\langle u\cdot \sigma \rangle )^2=1-(u\cdot w)^2\) as stated in Eq. (20), that therefore is proven. Further, due toFootnote 18 \([\sigma _j,\sigma _k]=2i\varepsilon _{ jkl }\sigma _l\) for every j, k, one has \(\frac{1}{2i}[u\cdot \sigma ,v\cdot \sigma ]= Det \left[ \begin{matrix}\sigma _1&{}\sigma _2&{}\sigma _3\\ u_1&{}u_2&{}u_3\\ v_1&{}v_2&{}v_3\end{matrix}\right] =(u\times v)\cdot \sigma ,\) so that, taking the averages in the states \(\psi _k(w)\) and considering that \(\langle \sigma _k\rangle =w_k\) for \(k=1,2,3\), (21) is proven. Lastly, due to \(\{\sigma _h,\sigma _k\}=2\delta _{ hk }{\hat{1}}\) for every h, k, we have \(\{\sigma \cdot u,\sigma \cdot v\}=2u\cdot v{\hat{1}}\) so that, taking the averages in whichever state, we get Eq. (22) and the proof of the lemma is complete. \(\square \)
4. With the notations of Sect. 3, thanks to the trigonometric identity \(\cos \theta =2\cos ^2\frac{\theta }{2}-1,\) we can write \(\cos \alpha =2p-1,\;\cos \beta =2q-1,\;\cos \gamma =2r-1,\) so that
that suitably simplified becomes \(4(4pqr-(p+q+r-1)^2)\) as asserted. \(\square \)
Rights and permissions
About this article
Cite this article
Fedullo, A. Heisenberg Uncertainty Relations as Statistical Invariants. Found Phys 48, 1546–1556 (2018). https://doi.org/10.1007/s10701-018-0213-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10701-018-0213-9



 is just a spin operator.
is just a spin operator.