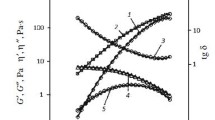
A new theory of dynamic deformation of polymer systems in a viscoelastic state provides two points (sites) for measuring rheological characteristics, which significantly increases the amount of information obtained in steady and unsteady state regimes of deformation. Theoretical dependences were obtained that determine the viscoelastic state of a polymer system in the steady state regime of deformation, when the complex viscosity remains constant, but its viscous and elastic components change within its limits.
Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.Avoid common mistakes on your manuscript.
In order to intensify the technological processes of obtaining chemical fibers and increase their physicomechanical properties, the study of the deformation properties of polymer systems (melts and polymer solutions) with elevated shear rate gradients is of particular interest. It is well known that at low velocity gradients, polymer systems behave like Newtonian fluids. With an increase in the velocity gradient, polymer systems begin to exhibit anomalous properties that manifest in decreasing viscosity and increasing flow instability. It has been established [1,2,3,4] that with a further increase in the velocity gradient, the polymer system again begins to show Newtonian properties, and the flow regime becomes steady again. At high velocity gradients, the second region of anomalous viscosity manifestation [2, 3] can occur, and even the third region of Newtonian flow and the third region of anomalous manifestation of viscosity are noted in [5]. A similar behavior of polymer systems was observed in [6] when a newly formed spinning dope was stretched when placed on a rotating roller. In addition to the region of steady stretching of the jet (at a constant flow rate), a second region of steady stretching of the jet was observed at low reception speeds, and between these, there is a non-steady state stretching zone. All this points to a complex nature of the deformation of polymer systems.
The disadvantage of studies of polymer systems in the static shear deformation mode at high velocity gradients is the difficulty of obtaining information about the change in their elastic properties. In this respect, a dynamic method for studying polymer systems has certain advantages, which, along with viscous properties, makes it possible to determine the elastic properties of polymer systems in a fairly wide range of changes in the angular frequency.
The existing theory of dynamic deformation has been developed for a long time and now has become classical. According to this theory [7,8,9,10,11,12], the behavior of viscoelastic polymer systems under dynamic deformation is estimated from the change in the complex modulus G*, the elastic modulus G′, and the loss modulus G″, correlated by G* = (G′2 + G″2)½ and their ratio in the form of the tangent of the phase angle tg = G″/G′, and the angular frequency itself is specified in the form of a sinusoidal dependence. At the same time, the viscoelastic properties of polymer systems are evaluated by the complex viscosity η*, dynamic viscosity η′ and the parameter η″, correlated by η* = (η′2 + η″2)½. The component η″ to this day has not been assigned a generally accepted name. Often it is not considered at all.
In this form, the theory of dynamic deformation of polymer systems in a viscoelastic state differs significantly from the theory of static deformation [13]. The author attempted to develop a unified theory of deformation of polymer systems for static and dynamic methods of deformation, and the first results of this work are presented in [14]. The subsequent dynamic tests in the steady and non-steady state regimes of deformation of polymer systems both in flowable and in solid state showed that this can be done.
To analyze the dynamic deformation of polymer systems in a viscoelastic state, the approach considered earlier in [13] for static deformation is applicable. It was shown that the pressure of the viscoelastic polymer system in front of the capillary is determined only by viscous tangential stresses. A similar approach is used in the study of dynamic deformation. It was assumed that the torque is also determined only by viscous tangential stresses. Although in this case elastic shear stresses are present in the deformable fluid, they are not included in the measured parameters.
To simplify the analysis, let us consider a rheometer in the form of coaxial cylinders with the investigated liquid enclosed in the space between them, in which the rotational speed (shear rate) of the inner cylinder is set and adjusted and the moment of inertia of the outer cylinder is determined. Viscoelastic properties of polymer systems during dynamic deformation will be determined separately from two points (sites) of measurements. The first point of measurement is correlated with the deformation state of the polymer system on the surface of the inner cylinder, which is driven by an electric motor. The second point of measurement is associated with the deformation state of the polymer system on the surface of the outer slowed cylinder.
It can be assumed that at each point of measurement a stress balance is performed, which is made up of the sum of the viscous and elastic components of the complex shear stress, and that the resulting stresses maintain a sinusoidal pattern of change. Inertial forces due to their small magnitude will be ignored.
Consider the first point of measurement. Usually [7, 10], the elastic properties during the deformation of polymer systems in the viscoelastic state are associated with normal stresses. In this analysis, we associate the elastic properties of polymer systems with elastic tangential stresses, implying that they correlate with normal stresses according to the theory of resistance of materials [15]. The advantage of using tangential stresses for shear analysis is that it can be assumed that in the steady-state flow regime they, with increasing flow velocity (flow velocity gradient), do not change their direction of action, whereas normal stresses (principal stresses) do (the incline angle of the main plane changes relative to the direction of action of tangential stresses).
With this in mind, we consider the process of dynamic deformation of polymer systems in a viscoelastic state. First, we define the structural model of the polymer system. Dynamic deformation of polymer systems will be considered in the state of a grid of intermolecular compounds consisting of viscous and elastic sites [13]. Viscous sites are characterized by the movement of macromolecules relative to each other without breaking contact with each other. Elastic sites arise when macromolecules cannot move freely in the sites. In this case, the sites themselves are forced to move relative to each other within the limits of the length and elasticity of the individual sites of the macromolecules connecting these sites. Upon reaching the limiting tangential stresses and shear deformation, destruction of the sites occurs, their number decreases. The polymer system begins to transition into a new structural state, characterized by the deformation of the macromolecules themselves. This transition is rather difficult to describe. Therefore, to simplify the analysis, the dynamic deformation of polymer systems will be considered in the grid state.
Under static deformation, the polymer system can be considered in two states – as a viscoelastic fluid and as a solid body, each of which is determined by its own equations of state [13]. A similar approach is applicable for dynamic deformation.
Consider the polymer system as a viscoelastic fluid. The angular frequency of impact on the polymer system in the working gap on the surface of the inner cylinder is determined by the ratio ω1 = ω1o sin(ωt), where ω1o is the amplitude value of the angular frequency of the inner cylinder, ω is the angular frequency, t is time.
As follows from the expression of the angular frequency, two methods can be used to determine the rheological characteristics. In the first case, a constant amplitude angular frequency is set, and viscoelastic characteristics are measured at different values of the current angular frequency within one rotation period (oscillation).
The second method currently used to obtain experimental data involves the use of an angular frequency ω1 = ω1o with sin(ωt) = 1. In this case, the angular frequency can be considered as the angular velocity, which is usually done with stationary circular deformation. Next, it is considered in greater detail.
In shear mode, the balance of tangential stresses (forces) for the first measurement point in the general form will be τ1sc = τ1sv + τ1su, where τ1sc is the complex (total) shear stress, τ1sv is its viscous component, and τ1su is its elastic component. For sin(ωt) = 1, we have τ1sc = τ1c, τ1sv = τ1v, τ1su = τ1u, and ω1 = ω1o. The balance of the complex tangential stress and its viscous and elastic components for the first point will take the form
Dividing this equation by ω1o, we obtain the balance equation for the complex viscosity and its viscous and elastic components
In this equation, η1c = τ1c/ω1o is the complex viscosity, η1v = τ1c/ω1o is viscosity (viscous component) and η1u = τ1u/ω1o is elasticity (elastic component). In this correlation, viscosity and elasticity will be considered as equal characteristics of polymer systems in a viscoelastic state under shear stress.
To simplify the theoretical analysis, we consider the dynamic deformation mode of a polymer system in the viscoelastic state at a constant complex viscosity of η1c = η1oc (without destroying the initial mesh). For this, equation (1) is transformed. The balance of tangential stresses and its components for this deformation regime takes the form
In this case, the mechanism for measuring the rheological properties of the polymer system at the first measurement point becomes similar to the measurement mechanism considered for static deformation [13], differing only in the method of defining shear deformation. This assumption allows for the initial Newtonian segment of the viscous component curve 1ov to determine the complex shear stress τ1oc and, from their difference, to find the elastic component τ1ou = τ1oc + τ1ov. This method of determining the elastic stress was used earlier when considering static deformation mode.
To express the components of the equation of the stress state of the polymer system during dynamic deformation (3), we use the equations of static longitudinal and circular deformation [13]
Here η1oc is the complex viscosity with its viscous η1ov and elastic η1ou components; θR is the equilibrium relaxation time; m is the exponent.
Dividing equations (4) – (6) by ω1o, we obtain the equations of the viscoelastic state of the polymer system
In these equations, viscosity and elasticity coefficients are introduced.
The viscosity and elasticity coefficients are corelated by α1ov + α1ou = 1.
As follows from dependencies (4) – (11), the complex viscosity η1oc, equilibrium relaxation time θR and the exponent m are used to describe the main rheological characteristics of a viscoelastic polymer system in a fluid state.
Next, the polymer system is considered as a solid. The Maxwell relation is used η1oc = GRθR, where GR is the equilibrium shear modulus. In this case, the equations of the stress state of the polymer system for the first measurement point (4) – (6) take the form
Here, s1o = θRω1o is the general equilibrium shear deformation, and s1ov = α1oθRω1o = α1ovs1o = s1o(1 + s1om) -1 and s1ou = α1ouθRω1o = α1ous1o = s1o1+m(1 + s1o) 1 are its viscous (irreversible) and elastic (reversible) components, correlated by s1o = s1oν + s1ou.
Note the features of the expression of shear deformation. The proposed theory of deformation of polymer systems in the solid state considers the expression s1o = θRω1o = ω1o/ω1oR as an equilibrium shear deformation. This expression will be further used for the dynamic deformation of polymer systems as a viscoelastic fluid. In this case, the expression s1o = θRω1o = ω1o/ω1oR will be considered as a reduced equilibrium flow velocity gradient (reduced angular frequency).
Now, consider the features of the dynamic deformation of polymer systems at the second point of measurement. By analogy with the first point of measurement, the balance of tangential stresses (forces) at the second point is generally represented as
where τ2v and τ2u are the viscous and elastic components.
The equations of complex stress and its viscous and elastic components on the surface of the outer cylinder are represented as
Here, η2c is the true viscosity, and η2v is its viscous component and η2u is the elastic component at the second point of measurement; ω2o is the angular frequency of the outer cylinder in the mode of free (unimpeded) rotation.
It can be assumed that the transfer of rotation from the inner cylinder to the outer cylinder is carried out only by the viscous component of the tangential stress τ2v and that this viscous component of the tangential stress at the first point of measurement can also be considered as a complex value of the tangential stress τ2c acting on the surface of the outer cylinder at the second point of measurement.
Considering equation (4), we get
Substituting equation (1) into (16), we get
From comparing equations (13), (15) and (17), it follows that
It can be seen that equations (16), (18) and (19) establish a correlation between the tangential stresses at the first and second points of measurement.
A correlation is also established between the angular frequencies of the inner and outer cylinders for the case of unimpeded rotation (oscillation) of the latter. For this, equation (16) is examined. Using equations (4) and (14), a replacement is made: η2c ω2o = α1v η1oc ω1o. It can be assumed that in the free rotation mode of the outer cylinder, the complex viscosity is constant η2c = η1oc, and angular frequency of the outer cylinder is variable. In this case, ω2o = α1v ω1o = ω1o[1 + (θR ω1o)]–1. As follows from this relationship, the angular frequency of the outer cylinder, in addition to the angular frequency of the inner cylinder ω1o, is also determined by a viscosity coefficient α1o, reflecting the viscoelastic properties of the polymer system.
Having two different angular frequencies, correspondingly, two different methods can be used to determine the complex viscosity values. The complex viscosity and its components, obtained at the first point of measurement using the angular frequency of the inner cylinder ω1•, will be considered as true. The complex viscosity and its viscous and elastic components at the second point of measurement, obtained using the angular frequency of the outer cylinder ω2o, can also be considered true. Since usually the outer cylinder is in a hindered state, the angular frequency of the outer cylinder ω2o will only be estimated as it would be in its free state. Therefore, the complex viscosity and its viscous and elastic components at the second point of measurement, obtained using the angular frequency of the first point of measurement (on the internal cylinder) ω1o, will be considered as apparent.
Dividing the components of equation (12) by the angular frequency at the first point of measurement ω1o, we obtain the apparent complex viscosity and its viscous and elastic components
We present these equations in the relative form (relative to η1c). We obtain the apparent coefficient of complex viscosity αkc = ηkc/η1c = α1v and apparent coefficients of viscosity and elasticity components αkv = ηkv/η1c = α21v and αku = = ηku/η1c = α1u α1u.
For the steady state regime of deformation, when η1c = η1oc = const, αkc = αkoc = α1v, αkov = α21v and αkou = ηku/η1c = = α1ov α1ou, equations (20) – (22) take the form
Here, αkoc = ηkoc/η1oc = α1ov = [1 + (θRω1o)m]–1 is the apparent complex viscosity coefficient; αkov = ηkov/η1oc = α21ov= = [1 + (θRω1o)m]–2 is the apparent viscosity coefficient; αkou = ηkou/η1oc = α1ov α1ou = (θR ω1o)m [1 + (θRω1o)m]–2 is the apparent elasticity coefficient.
These equations establish a relationship between the apparent values of the complex viscosity and its viscous and elastic components for the second point of measurement with the true values of the complex viscosity and its components for the first point of measurement.
The equations of state of a polymer system with an apparent complex viscosity and its viscous and elastic components in the general form take the form τ2c = ηkcω1o, τ2v = ηkvω1o, and τ2u = ηkuω1o.
Similarly to the first point of measurement, we consider the equations describing the stress of the polymer system in the solid state for the second point of measurement:
In classical polymer rheology, the method of expressing the effective viscosity with respect to the highest Newtonian viscosity, which is called the reduced viscosity, is widely used. Later [14], such a reduction was applied also for elasticity (elastic component).
In this report, we consider other ways of expressing the rheological characteristics in the above form, which, in the author’s opinion, more faithfully reflect the features of deformation of polymer systems in a viscoelastic state. To do this, we use the balance equation of tangential stresses (1). Similar results are obtained when using the balance equation for viscosity (2).
Let us consider possible ways of expressing the deformation characteristics of polymer systems in the form of reduced coefficients, when the complex shear stress and its viscous and elastic components of the stress balance are determined with respect to one of its components. As follows from the balance of tangential stresses (1), there are three ways of reducing the deformation characteristics.
The first method is the expression of the balance equation for tangential stresses and its viscous and elastic components with respect to the complex tangential stress at the first measurement point. Dividing equation (1) by τ1c, we obtain the balance equation for tangential stresses and viscosities in the reduced form α1v + α1u = α1c. Here, α1c = 1 is the complex viscosity coefficient; α1v = τ1v/τ1c = η1v/η1c is the viscosity component); α1u = τ1u/τ1c = η1u/η1c is the elasticity component. To express them, the equations of state obtained above for the steady state regime of deformation are used. We get:
In this case, it can be assumed that the exponent m can be variable.
The second method of reducing in the general form considers the components of the stress balance relative to the viscous component of the tangential stress at the first measurement point. Dividing the components of the stress balance equation (1) by τ1v or the viscosity balance by η1v, we get:
In these equations, α1pv is the reduced coefficient of viscosity, α1pu is the reduced coefficient of elasticity, and α1pc is the reduced coefficient of complex viscosity, correlated by α1pv + α1pu = α1pc.
Let us emphasize the reduced coefficient of elasticity α1pu = τ1u/τ1v. Earlier [13], this ratio of elastic and viscous components of the total shear stress was defined as “highly elastic” shear deformation. In its meaning, this expression is analogous to the expressions describing “highly elastic” shear deformation in the form of the Lodge γe = σ/τ and Weissenberg – Muni – Rivlin equations γe = 2σ/τ, in which the “highly elastic” shear deformation is considered as the ratio of normal stress to tangential. If we assume that τ1u = σ in the first case and τ1u = σ/2 in the second case, we have the correlation α1-u + τ1u = τ1v.
In the third method of reducing in the general form, the components of the stress balance are considered relative to the elastic component of the tangential stress at the first measurement point. This case will not be considered here.
Now that all the basic equilibrium rheological characteristics are known, we determine how they correlate with the rheological characteristics used by the classical theory of dynamic deformation.
Consider the rheological parameter widely used in the classical theory of deformation of polymer systems: tangent of the angle of mechanical losses tg δ = G″/G′ and its opposite, the cotangent of the angle of mechanical losses ctg δ = G′/G″. By replacing τ1v = G″ for the viscous component at the first measurement point and τ2u = G″ for the elastic component at the second measurement point, we get tg δ = τ1v/τ2u = α1u–1 and ctg δ = G′/G″ = τ2u/τ1v = α1u.
From these relations it follows that the cotangent of the angle of mechanical losses directly represents the coefficient of elasticity for the first measurement point and therefore is a more attractive rheological characteristic than the tangent of the angle of mechanical losses. Since the tangent as a characteristic is not considered in this paper, only the ratio α1u = G′/G″ will be used in the calculations. The correlation between the coefficients of viscosity α1v and elasticity α1u is α1v = 1 + α1u = 1 – G/G″.
Substituting the coefficients of viscosity α1v and elasticity α1u into the equations presented above, we obtain comparative relations for all rheological characteristics for static and dynamic deformation according to the proposed theory and classics. The obtained ratios are given in Table 1.
References
H. P. Schreiber, E. B. Bagley, and A. M. Birks, J. Appl. Polymer. Sci., 4, 362 (1960).
S. M. Aharoni, J. Appl. Polymer Sci., 17, 1507 (1973).
A. V. Ramamutry, Trans. Soc. Rheol., 18, 431 (1974).
G. V. Vinogradov and A. Ya. Malkin, Polymer Rheology [in Russian], Khimiya, Moscow (1977). 440 p.
H. Takahashi, T. Matsuoka, and T. Kurauchi, J. Appl. Polymer Sci., 30, 4669-4684 (1985).
M. S. Mezhirov, R. K. Idiatulov, et al., Khim. Volokna, 4, 14-16 (1982).
J. Ferry, Viscoelastic Properties of Polymers [in Russian], Izdatinlit, Moscow (1963). 535 p. [J. Ferry, Viscoelastic Properties of Polymers, New York-London (1961)].
G. Schramm, Fundamentals of Practical Rheology and Rheometry [in Russian], Kolos, Moscow (2003) 81 p.
C. D. Han, Rheology in Polymer Processing [transl. from English to Russian], Ed. G. V. Vinogradov and M. L. Friedman, Khimiya, Moscow (1979) 368 p. [C. D. Han, Rheology in Polymer Processing, Academic Press, New York – San Francisco – London (1976)].
I. M. Belkin, G. V. Vinogradov, and A. I. Leonov, Rotational Instruments. Measurement of Viscosity and Physico-Mechanical Characteristics of Materials [in Russian], Mashinostroenie, Moscow (1967) 272 p.
A. Y. Malkin, A. A. Askadsky, and V. V. Kovriga, Methods for Measuring the Mechanical Properties of Polymers [in Russian], Khimiya, Moscow (1978) 336 p.
G. V. Vinogradov, Yu. G. Yanovsky, and A. I. Isaev, In Coll.: Successes of Polymer Rheology [in Russian], Ed. G. V. Vinogradov, Khimiya, Moscow (1970) pp. 79-97.
Yu. A. Vinogradov, Khim. Volokna., No. 1, p. 3-6, No. 2, p. 36-39 (2006).
Yu. A. Vinogradov and N. I. Kuzmin, “A new look at the theory of dynamic deformation of polymer systems in the viscoelastic state” [in Russian], Proc. 27 th Rheology Symposium, September 8-13 (2014) Tver, pp. 54-56.
N. M. Belyaev, Strength of Materials [in Russian], Nauka, Moscow (1976) 608 p.
I. P. Briedis and L. A. Faitelson, Mechanics of Polymers [in Russian], 3, p. 523 (1975).
Author information
Authors and Affiliations
Corresponding author
Additional information
Translated from Khimicheskie Volokna, No. 1, pp. 3 – 9, January – February 2019.
Rights and permissions
About this article
Cite this article
Vinogradov, Y.A. Theory of Dynamic Deformation of Polymeric Systems in a Viscoelastic State. Fibre Chem 51, 1–8 (2019). https://doi.org/10.1007/s10692-019-10036-1
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10692-019-10036-1