A One-Stage Model of Abatement Innovation in Cournot Duopoly: Emissions vs Performance Standards

Abstract

This paper evaluates the comparative performance of emission and performance standards in a one-stage game of abatement R&D and Cournot duopoly, in terms of R&D propensity, output and social welfare. For each standard, firms simultaneously select R&D and output levels, given the standard’s exogenous constraint. A performance standard generates higher R&D investments and output, but lower profit, than the pollution-equivalent emissions standard. The same conclusion extends to social welfare only under high demand. We also conduct a similar comparison for each of the two instruments across the one-stage and the two-stage models. The two-stage model leads to higher levels of R&D and industry output for both standards. The same conclusion applies to the social welfare comparison for the emissions standard. However, for the performance standard, the same conclusion requires a damage parameter below a given threshhold. When the standards are chosen to maximize welfare, the performance comparison becomes highly parameter-dependent, except that social welfare is higher for the performance standard. Some policy implications are discussed.

1 Introduction

In order to successfully comply with the ever-tightening standards of environmental regulation, firms are increasingly expected to invest in the development and improvement of abatement technologies. It has been widely recognized that firms’ incentives to engage in environmental research and development (R&D), or to adopt less polluting production technologies, may differ significantly across policy instruments. The large body of literature that has addressed various facets of this fundamental issue keeps growing.¹

Up to recently, a perceived general conclusion of this literature is that market-based instruments are superior to commonly applied command-and-control instruments in terms of the implied incentives for firms to adopt or develop green technologies. Observing that this conclusion is largely based on studies dealing with perfectly competitive or monopoly settings, [1] compares R&D incentives provided by two command-and-control instruments — emission and performance standards — and two market-based instruments in a general two-stage model with Cournot firms deciding upon R&D levels for improved abatement technology before competing in Cournot outputs. Assuming all four instruments to be exogenously set, Montero finds that environmental emission and performance standards may offer greater incentives for technology adoption than market-based instruments.² He concludes that the conventional wisdom on the comparison of the two classes of instruments need not extend to imperfectly competitive markets.³

As Montero’s general setting did not lend itself to clear-cut results, [2] specialize Montero’s setting to a two-stage duopoly with linear demand and abatement costs and focus on an extensive comparison of the two command-and-control instruments — the emission and performance standards –.⁴ Amir et al. [2] derive a closed-form solution for the subgame-perfect equilibrium of the two-stage game under each policy instrument, which allows for a full characterization of the parameter regions for which each instrument is superior to the other in terms of resulting R&D and/or output levels, under the normalization that the final level of emissions is the same for the instruments. While complete, this detailed comparison may go either way, in a highly parameter-dependent (though fully specified) manner. The comparison of social welfare for this two-stage game is shown via examples to follow a similar conclusion.⁵ A second aim pursued in [2] is to endogenize the regulatory standards through the inclusion of a social planner maximizing welfare. This scenario involves a three-stage game wherein the planner selects the pollution constraint level that maximizes social welfare prior to firms’ R&D and then output decisions. In contrast to the two-stage game, the three-stage game leads to an unambiguous conclusion: the performance standard is welfare superior to the emission standard. Ultimately, this result emphasizes the importance of endogenizing the regulatory standard for the comparison of incentives that various policy instruments offer for the adoption of new environmental technologies ([3], p.1194; [4], p. 178).

The purpose of the present study is to examine the same comparison of emission and performance standards analyzed by [2] but in the context of a one-stage version of their two-stage game. Thus, in the present perspective, firms simultaneously choose both abatement R&D and output levels. In other words, relative to the two-stage game model, the aim of the present paper is thus to assess how the performance comparison between the two instruments would change without firms’ strategic commitment in R&D.⁶

In order to discuss the appropriateness of a one-stage formulation, it is useful to first recall that the usual meaning of simultaneous moves in game theory need not refer to decisions taken at the same instant of time, but rather to the fact that one player’s decisions are taken without any knowledge of the rival’s actions, even in settings where the decisions are actually taken at possibly very different instants of time. Thus the key determinant of whether a one-stage game or a two-stage game formulation is more appropriate is mutual observability of actions. In the present context, the key question is whether the players make their output decisions with the observation/knowledge of the rival’s R&D decision or not. The answer depends on many industry characteristics, such as the geographical distance between the firms, the extent of labor mobility and other sources of knowledge spillovers, the institutional prevalence of R&D and other strategic announcements in the industry, and their contextual empirical reliability.⁷

We now preview the main results of the present paper. For a level playing field comparison of equilibrium abatement R&D, industry output and social welfare in the one-stage game, we continue to assume that the regulator chooses the pollution constraints so as to generate the same level of final emissions under both policy instruments (as in [1] and [2]). Unlike the firms, the planner takes into account the pollution externality, which is captured by the inclusion in social welfare of a damage function that is quadratic in emission levels.⁸ The first result is that a performance standard generates higher R&D investments and industry output, and less profit, than the pollution-equivalent emission standard. The second conclusion is a complete characterization, via a single condition on market parameters, that determines when a performance standard is welfare superior or inferior to an emissions standard. The economic interpretation is that high demand (relative to cost) favors the performance standard, while the opposite holds for low demand.

In the second part of the paper, we compare the equilibrium levels of abatement R&D, industry output and social welfare across the one-stage and the two-stage models. The two-stage model leads to higher levels of R&D and industry output for both the emissions and the performance standards. We also show that, with the same emissions limit across the two game forms as a normalization, social welfare is always higher in the two-stage game than in the one-stage game for the emissions standard. In order to understand our conclusions in what follows, it will be useful to observe that this same-emissions normalization makes the welfare comparison similar to the analogous exercise in industrial organization (where emission damages are not taken into account). Thus these results are closely related to the findings of [5] in their comparison of the two different game forms for a general formulation of a model of process R&D and Cournot competition. The economic intuition for our R&D and output comparisons is similar to theirs. In the two-stage game, as firms foresee that lower abatement costs will generate a larger market share in the product market competition, they tend to engage in excessive levels of R&D, beyond what is needed to minimize the total cost for the equilibrium output produced. Since the intuition is similar to [5], we refer the reader to that study for further discussion. These authors refer to the induced differences in firms’ behavior in the two-stage game as the impact of firms’ strategic commitment in R&D (in the two-stage game).

However, for the performance standard, with the same standard across the two games as a normalization, we provide a full characterization, wherein the two-stage game is welfare superior to the one-stage game if and only if the damage parameter is low enough (with a closed-form threshold value). This conclusion becomes intuitive once one realizes that a higher output for the two-stage game will directly imply more pollution under the performance standard. Since pollution is now penalized in the social welfare function, an upper bound on the damage parameter is then needed for the welfare superiority of a performance standard.⁹ These intuitive considerations will be elaborated upon below.

In the third part of the paper, we endogenize the regulatory standards for each of the two instruments by a social planner maximizing welfare. This scenario involves a two-stage game wherein the planner selects the pollution constraint level that maximizes social welfare prior to firms’ simultaneous R&D and output decisions. While the comparison outcome now highly parameter-dependent, this part still leads to an unambiguous conclusion: the performance standard is welfare superior.

In conclusion, in industry settings for which a one-stage model is more appropriate, the performance standard emerges as the superior command-and-control instrument, with some qualifications. This is in line with conventional wisdom, as reflected in theoretical research as well as in the widespread use of this tool in real-life environmental policy-making (e.g., [6]). The standard intuition for the superiority of performance standards is that they are less intrusive to the firms’ operations, and allow for more flexibility in the firms’ responses to environmental regulation. On the whole, our results provide some novel support for the view that a performance standard tends to be superior to an emission standard. However, under low demand in a small parameter region, the welfare and the abatement R&D results yield conflicting recommendations.

As to the possible policy implications of the comparison between the two game forms, one may attribute another potential role for a social planner, that of inducing a two-stage or a one-stage interaction for a particular industry, whenever this might be possible. For instance, to give rise to a two-stage game, the planner may conceivably mandate that the firms make credible announcements of their abatement or pollution levels, or provide incentives for them to do so. One form of such incentives are matching grants to subsidize abatement R&D. One implication of Proposition 4 of interest is that a two-stage game would be welcome whenever the pollutant for the industry at hand is relatively less polluting. A noteworthy reversal is the fact that the one-stage game is welfare superior whenever the damage function is steep enough, or the underlying pollutant is highly harmful to the environment.

The rest of the paper is as follows. Section 2 sets out the basic model, Sect. 3 the comparison of equilibrium R&D, output and welfare for the two instruments. Section 4 compares the incentives for adoption in the one- and two-stage games. Section 5 endogenizes the regulatory standards and Sect. 6 concludes. All the proofs are in Appendix A, and Appendix B presents a numerical analysis on the endogenous instruments that complements Sect. 5.

2 The Model Preliminaries

Consider a duopoly market with two ex-ante symmetric and profit maximizing firms indexed i and j, with $i,j\in \{1,2\}$, and $i\ne j$. These firms produce a homogeneous good and engage in Cournot competition in the output market, where their production generates pollution in the environment. Specifically, in the absence of any environmental regulation, each unit of output produced by firm i, $q_{i}$, yields exactly one unit of polluting emissions. Thus, the aggregate quantity produced in the market equals $Q=q_{i}+q_{j}$, which also reflects the aggregate level of emissions prior to any environmental regulation. As is commonly done for such models, we assume w.l.o.g. that production is costless to focus solely on the effects of abatement costs on firms’ incentives to innovate. The inverse demand function in this market is taken to be linear, $P(Q)=a-bQ$ for $Q\le a/b$ where $a>0$ is the demand intercept.

The aim of the regulator in this market is to reduce the level of pollution in the environment. This can be achieved by means of either of two command-and-control policy instruments: an emission standard or a performance standard. The emission standard constrains the level of pollution generated by firm i to be under an upper limit $e_{i}$, so that when environmental regulation is put in place, each firm must abate the excess emissions, $q_{i}-e_{i}$, and incur the corresponding abatement cost. The latter is given by $(c-x_{i})(q_{i}-e_{i})$ for firm i, where $c>0$ is the initial unit cost of abatement (prior to any innovation), and $x_{i}<c$ is the R&D investment, or the reduction in the unit cost of abatement.

Unlike the emission standard, the performance standard imposes a restriction on firm i’s maximal ratio of emissions per output, $h_{i}=e_{i}/q_{i}$. Hence, under the performance standard, the level of emissions abated is given by $q_{i}(1-h_{i})$, where $h_{i}\in (0,1)$. The special cases of $h_{i}=0$ and $h_{i}=1$ correspond respectively to zero emissions by the firm and the original level of emissions (or no abatement). The corresponding abatement cost is then given by $(c-x_{i})q_{i}(1-h_{i})$.

The R&D technology used by firms is deterministic and aims to reduce the cost of abating excess pollution beyond what the regulatory scheme would allow through end-of-pipe abatement. Firms choose this method of compliance with environmental regulation to reduce their costs of pollution abatement. However, alternative ways of modeling R&D in abatement technology may be more appropriate for firms whose objective is to reduce the ratio of emissions per output (see, e.g., [7]).¹⁰

We assume that firm i’s cost of reducing the constant marginal abatement cost by $x_{i}\ge 0$ is given by a quadratic function $g(x_{i})=\gamma x_{i}^{2}/2$, where $\gamma >0$ is a parameter inversely related to the efficiency of R&D. This specification reflects the standard property of diminishing returns to R&D expenditures.¹¹

With either of the two command-and-control instruments, the regulator can meet the objective of restricting the total emissions generated in the market, $E=e_{i}+e_{j}$, and thus reduce the resulting environmental damage. The latter is postulated to be

$$\begin{aligned} D(E)=sE^{2}/2,\text { with }s>0. \end{aligned}$$

which implies that damage increases at an increasing rate with the aggregate emission level.

In the specification of damage functions (expressed in monetary terms) for environmental regulation, both linear and strictly convex relationships are commonly adopted in environmental economics, in particular when dealing with specific pollutants. We follow the more commonly adopted strictly convex strand, which includes theoretical studies as well as empirical/policy studies. Yet, both of these curvature assumptions ought to be seen only as educated postulates in that substantial uncertainty and disagreement persist about key aspects of the relationship between pollution and social damage (for a detailed survey, see, e.g., [8]). This is obviously a key issue for environmental and climate policies.

The one-stage game and assumptions are outlined next.

2.1 The One-Stage Game

For each policy instrument, the one-stage game under consideration postulates that both firms choose their environmental R&D levels and outputs simultaneously to maximize individual profit. Hence, the game is static (or one-shot) but has a two-dimensional action space (R&D effort and output) for each firm.

In the strategic R&D literature, both one-stage and two-stage games have been considered, with the latter being more prevalent. Both types of games are appropriate under the right industry conditions. As noted in the Introduction, the one-stage version is more appropriate when firms cannot observe each other’s R&D investment or when firms cannot commit to their R&D choices. These features may be determined by industry characteristics such as a long distance between the firms, their inability to hire each other’s employees, a tendency to operate under trade secrets, etc.¹²

For each of the two command-and-control policy instruments, we assume that the regulator sets the same appropriate exogenous pollution constraint for each firm. We then characterize the performance of each the two instruments by solving the corresponding one-stage Nash equilibrium levels of R&D and output in closed-form and analyzing the outcomes.

In line with common practice in the literature on oligopolistic R&D, we impose the following parameter restrictions to ensure that the solutions to the one-stage games for both policy instruments are interior and thus in particular economically meaningful.

Assumption (A1) (i) $a>2c,$ (ii) $3b\gamma >\frac{a}{c}$, (iii) $0<e<\frac{a-c}{3b}.$

A discussion of the specific role of each of these assumptions is now given. (A1)(i) ensures that the market is large enough compared to the abatement unit cost, similar to the classical Cournot model with linear demand and production costs. A significant side benefit of (A1)(i) is that it ensures both firms will remain in the market even if one firm chooses maximal R&D and the other firm no R&D at all, thereby dispensing with the need to worry about a cumbersome possible R&D-induced move to monopoly.

(A1)(ii) imposes a lower bound on the cost of abatement R&D in terms of market size, in line with similar second-order conditions in standard R&D models (see, e.g., [9]). This condition leads to an interior R&D level, which is convenient for comparative analysis but not really essential in the sense that, without such an assumption, equilibrium would call for maximal abatement R&D, i.e., $x^{*}=c$, rendering many of our desired comparisons of limited interest [9] and their statements more cumbersome.

Finally, (A1)(iii) limits the emissions limit e to be bounded above by the pre-regulation Cournot equilibrium per-firm output given by $\frac{a-c}{3b},$ so as to avoid uninteresting situations where the emissions limit is not binding, and thus with no effect on the firms’ behavior.

Besides ensuring that the firms’ equilibrium variables correspond to interior solutions as well as standard second-order conditions, these parameter restrictions also guarantee that, at equilibrium, abatement costs (after investment) are positive and output is strictly larger than the level of pollution.

Given Assumption (A1), we now outline the precise structures of the one-stage games that obtain when the regulator imposes a binding exogenous emission standard or performance standard on firms’ production.

2.2 The Emission Standard

Under the emissions standard, the aggregate level of pollution in the duopoly market is capped from above by $E=e_{i}+e_{j}$. As firms are symmetric, the regulator imposes an equal emissions cap e for each firm, so that $E=2e$. To avoid uninteresting situations, as noted above, we assume that the emission standard is binding, i.e., that the emissions cap e is lower than the firm’s equilibrium output in the absence of regulation.¹³ This is the role of Assumption (A1)(iii).

Then firm i ($i=1,2$) simultaneously determines its environmental R&D investment $x_{i}$, its level of output $q_{i}$, and the final level of emissions after abatement $e_{i}$ that maximize its profit given the pollution constraint e and its rival’s output, $q_{j}$. Hence, the payoff of firm i is given by its Cournot profit function net of its R&D cost, i.e.,

$$\begin{aligned} \underset{q_{i},x_{i},e_{i}}{\max }\left\{ [a-b(q_{i}+q_{j})]q_{i}-(c-x_{i})(q_{i}-e_{i})-\frac{1}{2}\gamma x_{i}^{2}\right\} \end{aligned}$$

$$\begin{aligned} \text {subject to }e_{i}\le e. \end{aligned}$$

This objective function is increasing in $e_{i}$. Hence, in light of Assumption (A1), firm i always sets $e_{i}^{*}=e$.

The payoff of firm i is thus: choosing the two variables $q_{i}$ and $x_{i}$ so as to

$$\begin{aligned} \underset{q_{i},x_{i}}{\max \text { }}\Pi (q_{1},q_{2},x_{1},x_{2})=\text { }[a-b(q_{i}+q_{j})]q_{i}-(c-x_{i})(q_{i}-e)-\frac{1}{2}\gamma x_{i}^{2}\text {.} \end{aligned}$$

(1)

The first-order conditions w.r.t. $q_{i}$ and $x_{i}$ are, at a symmetric Nash equilibrium

$$\begin{aligned} \left\{ \begin{array}{l} a-3bq-c+x=0 \\ q-e=\gamma x. \end{array} \right. \end{aligned}$$

The second-order condition is easily seen to be verified under Assumption (A1).¹⁴

Solving the system of equations yields the unique and symmetric Nash equilibrium levels of environmental R&D $x_{e}$, and output $q_{e}$, under the emission standard

$$\begin{aligned} x_{e}=\frac{(a-c)-3be}{3b\gamma -1}\text { and }q_{e}=\frac{\gamma (a-c)-e}{3b\gamma -1}\text {.} \end{aligned}$$

(2)

By Assumption (A1), it is easy to check that (2) is an interior and valid Nash equilibrium of the game, i.e., one that satisfies $0<x_{e}<c$, and $0<e\le q_{e}$.

A natural comparative statics property of some interest is seen by inspection of (2): as long as our parameter restrictions continue to hold, we have

$$\begin{aligned} \frac{\partial x_{e}}{\partial e}<0\text { and }\frac{\partial q_{e}}{\partial e}<0. \end{aligned}$$

(3)

In other words, as the emissions regulation gets less tight (i.e., e increases), the firm conducts less abatement R&D and produces less output.

We now provide the solution to the one-stage game under the performance standard.

2.3 The Performance Standard

Unlike the emissions standard, the performance standard does not impose restrictions on the absolute level of output generated by firms, but rather on the ratio of pollution to output, $h=e/q$. Thus for instance, if the regulator chooses $h=0.6$, the firm’s emissions can at most amount to $60\%$ of its output. If this firm’s output is given by $q=100$, then this firm must abate $q(1-h)=40$ units of pollution.

Given a choice of h by the regulator, the payoff or profit function of firm i is

$$\begin{aligned} \underset{q_{i},x_{i}}{\max }\text { }[a-b(q_{i}+q_{j})]q_{i}-(c-x_{i})q_{i}(1-h)-\frac{1}{2}\gamma x_{i}^{2}. \end{aligned}$$

(4)

Here firm i chooses its abatement R&D level $x_{i}$ and output $q_{i}$ simultaneously, and $q_{j}$ is the output produced by the rival firm. The first-order conditions for firm 1 (say) w.r.t. $q_{i}$ and $x_{i}$ are, at a symmetric and interior Nash equilibrium,

$$\begin{aligned} \left\{ \begin{array}{l} a-3bq-(c-x)(1-h)=0 \\ q(1-h)=\gamma x \ \ \ \end{array}\right. \end{aligned}$$

As in the previous case, the second-order condition is easily seen to hold under Assumption (A1) and the fact that $0<h<1$, i.e., the Hessian matrix of (4) is negative definite.

Solving the system of equations yields the unique and symmetric Nash equilibrium levels of environmental R&D $x_{h}$ and output $q_{h}$, under the performance standard

$$\begin{aligned} x_{h}=\frac{(1-h)[a-c(1-h)]}{3b\gamma -(1-h)^{2}}\text { and }q_{h}=\frac{\gamma [a-c(1-h)]}{3b\gamma -(1-h)^{2}}. \end{aligned}$$

(5)

It is easy to verify that $0<x_{h}<c$ and $q_{h}>0$ as a direct consequence of Assumption (A1) and the fact that $0<h<1$.

A comparative statics property of interest is that, under Assumption (A1),

$$\begin{aligned} \frac{\partial x_{h}}{\partial h}<0\text { and }\frac{\partial q_{h}}{\partial h}=\gamma \frac{3b\gamma c-2a(1-h)+c(1-h)^{2}}{\left[ 3b\gamma -(1-h)^{2}\right] ^{2}}. \end{aligned}$$

(6)

It can be verified that $\partial q_{h}/\partial h$ in (6) cannot be uniformly signed over the entire parameter region. Therefore, as the emission regulation gets less tight (i.e, h increases), the firm conducts less abatement R&D but may produce more or less output.

Remark 1

Recalling that output is always reduced in the case of a higher emissions limit e (see (3)), the possibility that equilibrium output may react in either direction as h-regulation tightens (up if a is low and down if a is high) reflects the well-known intuitive fact that a performance standard allows the firm more flexibility in its response to environmental regulation that an emissions standard.¹⁵ This simple but important observation will be invoked below when providing intuitive accounts for some results.

The next section compares the equilibrium solutions under the two policy instruments.

3 Comparison of the Standards in the One-Stage Game

In this section, we compare the two policy instruments in terms of the equilibrium propensities to invest in abatement R&D, to produce more output and to generate profits. We also provide the corresponding comparison for the resulting social welfare levels. Finally, we contrast our results with related findings from the literature.

To ensure that the results of the comparison of the R&D incentives under the two regulatory regimes are meaningful, we assume that the regulator chooses the pollution constraints e and h so as to obtain the same level of final emissions under both instruments. That is, we set $e=q_{h}h$, where $q_{h}$ is defined in Eq. (5). As a consequence, the equilibrium values of R&D and output under both regulatory instruments can be expressed in terms of the same h, which makes the comparison more transparent. Thus, we have $e=q_{h}h=\frac{\gamma h[a-c(1-h)]}{3b\gamma -(1-h)^{2}}$. To have a binding emissions standard e, we thus need (see Assumption A1)

$$\begin{aligned} \frac{\gamma h[a-c(1-h)]}{3b\gamma -(1-h)^{2}}<\frac{a-c}{3b}. \end{aligned}$$

(7)

As pollution regulation without (7) would not have any effect on firms’ behavior, making the problem uninteresting, the parameter restriction in (7) is henceforth assumed.

3.1 R&D and Output Incentives

This subsection treats the key question: which of the two policy instruments yields higher levels of environmental R&D and industry output at the respective one-stage Nash equilibria, under the normalization that the regulator picks the levels of the two instruments so as to ensure that the final pollution levels generated are identical in the two cases.

The results of this comparison are summarized in Proposition 1, the proof of which is given in Appendix A (along with all proofs for this paper).

Proposition 1

Under Assumption (A1) and the equal-pollution normalization $e=q_{h}h$, relative to the emissions standard, the performance standard leads to

• (i) more abatement R&D (i.e., $x_{h}>x_{e})$,

• (ii) more industry output (i.e., $q_{h}>q_{e}$), and thus a higher consumer surplus and a higher level of abatement,

• (iii) a lower profit per firm (i.e., $\pi _{h}<\pi _{e}).$

We now argue that the results of Proposition 1 are quite in line with common economic intuition about the two standards. As it pertains to a ratio of two variables instead of just to a single variable, the performance standard offers the firms more flexibility than the emissions standard in complying with a given level of pollution regulation. In addition, the R&D and output decisions are complements in each firm’s payoff function, which is clearly an intuitive (and well-known) property. In light of these two observations, the comparisons in Proposition 1(i)-(ii) can be intuitively expected. Given the normalization of equal pollution levels, a higher output under the performance standard implies directly that the level of abatement is larger under this instrument. Finally, the reversal of the profit comparison indicates that the extra flexibility inherent in the performance standard allows firms to compete more vigorously, thereby lowering equilibrium profit.

Thus, a very clear-cut comparison emerges between the two standards. In terms of the resulting R&D and outputs, and thus consumer surplus, the superiority of the performance standard over the emissions standard is fully unambiguous. However, in terms of producer surplus, the ranking fully reverses. A natural question thus arises, as to which of the two standards might dominate in terms of social welfare.

We now compare the resulting welfare levels for the emissions and performance standards.

3.2 Welfare

This subsection provides a comparison of the equilibrium levels of social welfare for the two standards under study. For this comparison to be on a level playing field, we maintain the equal-pollution normalization that $e=q_{h}h$.

Social welfare is defined in the usual manner in an environmental setting, as the sum of consumer surplus and producer surplus, net of a measure of the environmental damage caused by the leftover emissions. For the present model, under symmetric choices for the two firms, this takes the form

$$\begin{aligned} W(q,x)=\int _{0}^{2q}(a-bt)dt-2(c-x)(q-e)-\gamma x^{2}-2se^{2}, \end{aligned}$$

(8)

where q, x, and e denote any feasible per-firm output, investment in R&D, and emissions, respectively. The equilibrium output and R&D are given by (2) and (5) for the emission and the performance standard respectively. To find the expression for equilibrium welfare under an emission standard, substitute (2) in (8) and use $e=hq_{h}=\frac{\gamma h[a-c(1-h)]}{3b\gamma -(1-h)^{2}}$ to get

$$\begin{aligned} W_{e}=\frac{(4b\gamma -1)(\gamma (a-c)^{2}-2ae)+eb(9b\gamma -2)(e+2c\gamma )-2e^{2}(3b\gamma -1)^{2}s}{(3b\gamma -1)^{2}}. \end{aligned}$$

(9)

Similarly, for equilibrium welfare under a performance standard, by (5) and (8) (with $e\equiv q_hh$),

$$\begin{aligned} W_{h}=\frac{\gamma [a-c(1-h)]^{2}[2\gamma (2b-sh^{2})-(1-h)^{2}]}{[3b\gamma -(1-h)^{2}]^{2}}. \end{aligned}$$

(10)

The main result here deals with the comparison between the performance and the emissions standards in terms of resulting welfare.

Proposition 2

Let (A1) hold and $e=q_{h}h$. The performance standard generates more (less) social welfare than the emissions standard, $W_{h}>(<)W_{e}$ if

$$\begin{aligned} \frac{a}{c}>(<)b\gamma \frac{A}{B}, \end{aligned}$$

(11)

where $A\equiv h(6b\gamma -1)+(6b\gamma -2)>0,$ and $B\equiv (4b\gamma -1)h(1-h)+2b\gamma (3b\gamma -1)>0.$

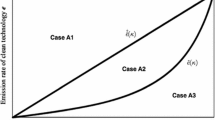
The main clause of Condition (11) may be interpreted as saying that the size of the market is sufficiently high. For such cases, the performance standard dominates in terms of social welfare, while the reverse holds for small market sizes. This is in line with the previous results that the performance standard yields higher abatement R&D and industry output, as R&D is more valuable in a larger market. Conversely, in a small market, the performance standard emerges as detrimental to welfare by giving rise to a relative excess of R&D.

It is worth stressing that Proposition 2 provides a complete characterization of the welfare comparison between the two policy instruments. In addition, the comparison is actually quite simple and lends itself to a clear-cut and intuitive economic interpretation. By contrast, in the two-stage game version of this model, the full characterization for the same comparison provided by [2] led to a much more complex outcome in terms of its dependence on the parameters of the model, offering limited scope for an intuitive understanding. In [1], the generality of the setting did not allow for a characterization, but only for a qualitative determination that the result could go either way.

A careful numerical investigation of Condition (11) reveals that the main clause (i.e., ${>}$) is much more likely to hold (given our assumptions) than the opposite clause (i.e., ${<}$ ), so that the emissions standard may be welfare-dominant only in a restricted parameter region. The following example illustrates this point and other aspects of Proposition 2.

Example 1

Consider our one-stage game formulation with $a=1.2$, $\gamma =1.8$, and $b=c=s=1$. In addition, assume that $e=q_{h}h$, so that both instruments generate the same level of final emissions. We compare the resulting equilibrium values of R&D investment, output and welfare under the emissions and performance standards.

The results of the comparison are given in Table 1, where ES stands for emissions standard, and PS for performance standard. The values of $h=0.10$, and $h=0.30$ in Table 1 are selected to show how the comparison varies with the inequality (11) in Proposition .

Table 1 The comparison of R&D, output and welfare under both policy instruments for $a=1.2$, $\gamma =1.8$, $b=c=s=1$ and two different values of emissions allowed

Table 1 shows that the equilibrium levels of R&D and output are higher under the performance standard for both $h=0.10$ and $h=0.30$ (the second and third rows in Table 1, respectively). This result conforms to Proposition 1.

To illustrate that the welfare comparison can indeed be in favor of the emissions standard, consider the case $h=0.30$ (see the fourth row in Table 1). Indeed, the LHS of (11) is then easily seen to be lower than the RHS, and the result is in line with Proposition .

In the next section, we compare our results to their analogous conclusions in the two-stage game versions of the model, where before competing à la Cournot in the second stage, the firms commit to their levels of R&D in the first stage.

4 Comparison of the One-Stage and Two-Stage Games

The incentives to invest in environmental R&D under the two command-and-control instruments have been previously compared in a Cournot competition setting by [1] and [2].¹⁶ Unlike the present study, both studies adopt a two-stage setting, where firms make their R&D and output decisions sequentially, with R&D investments chosen in the first stage and Cournot outputs in the second stage (upon observing the R&D choices). The purpose of this section is to compare the Nash equilibrium levels of R&D, output and social welfare of the one-stage game and the corresponding two-stage game.

The study that pioneered the comparison between a one-stage game and the corresponding two-stage game of oligopolistic process R&D is [5]. Using a model with very general demand and cost functions, their main result is that the equilibrium levels of R&D and output of the two-stage game exceed those of the one-stage game. To provide an intuitive account of this result, [5] decompose the incentives firms have to conduct R&D into two separate components for the two-stage game. The first or direct effect refers to a purely cost-saving motive that leads the firm to minimize the total combined R&D and production costs for a given level of output. The second or strategic effect relates to the anticipation of Cournot competition in the second stage and captures the impact that one firm’s R&D investment has on its own and rival’s outputs and thus market shares and profits. This commitment effect causes firms to undertake more R&D than is needed to minimize their total R&D and production costs. In this perspective, being absent in the one-stage game, where R&D and output choices are simultaneous, the latter effect is at the heart of the difference between the two models.

In a two-stage game of abatement R&D/Cournot competition with general demand and cost functions, [1] compares the incentives to invest in abatement R&D for the two instruments at hand (and others), and finds that neither of the two uniformly dominates the other. In his intuitive account of this conclusion, [1] invokes the direct and strategic effects of R&D as adapted from [5].

By specializing Montero’s model to the common specification of a linear oligopoly, [2] derive simple closed form solutions for the equilibrium of the two-stage game under each policy instrument. This makes possible a complete characterization of the parameter regions for which each instrument dominates in terms of equilibrium levels of R&D and/or industry output. Specifically, they establish that a performance standard generates more R&D incentives when the cost of R&D is sufficiently high (for a given market size). In contrast, when the market size is sufficiently large (for a given cost of R&D), they find that an emission standard generates more R&D incentives. For modest levels of R&D cost and market size, the stringency of environmental regulation may also affect the results of the comparison. Similar results apply in case of the equilibrium output comparison under both regulatory tools. Via this full characterization of the comparative properties of the equilibria, [2] demonstrates that neither of the two command-and-control policy instruments is uniformly superior to the other in terms of the final levels of environmental R&D and industry output.

We now contrast the outcomes of the two-stage games in [2] with the corresponding solutions to the one-stage game discussed in Sect. 3.1. The two-stage game requires the following new assumptions, taken from [2].

(A2) (i) $a>2c$, (ii) $9b\gamma >4a/c$.

Similarly to the corresponding parts of Assumption (A1), these conditions guarantee an economically meaningful solution in the two-stage game (including the second-order condition and interiority of solutions). Observe that Assumptions (A1)(i) and (A2)(ii) are the same, but (A2)(ii) is more restrictive than (A1)(i). Specifically, it reduces the upper bound of the market size, now $2c/b<a/b<9c\gamma /4$ (under (A1) we have $2c/b<a/b<3c\gamma$).

When comparing the one-stage and the two-stage games under the performance standard, the same (exogenous) value of h is used for both models, and this is the relevant level-playing field for this comparison. A similar statement applies to the value of e for the other comparison. In other words, the restriction that $e=q_{h}h$ is thus immaterial here.

4.1 R&D and output comparison

We begin by comparing the equilibrium levels of abatement R&D and output of the one-stage game and of the two-stage game. The former are given in (2) and (5) above. For the two-stage game, the equilibrium levels of per-firm abatement R&D and output are taken from [2]. They are respectively given by, for the emissions and performance standards (with a ‘tilde’ referring to the variables in the two-stage game)

$$\begin{aligned} \tilde{x}_{e}=\frac{4(a-c)-9be}{9b\gamma -4}\text { and }\tilde{q}_{e}=\frac{3\gamma (a-c)-3e}{9b\gamma -4} \end{aligned}$$

and

$$\begin{aligned} \tilde{x}_{h}=\frac{4(1-h)[a-c(1-h)]}{9b\gamma -4(1-h)^{2}}\text { and }\tilde{q}_{h}=\frac{3\gamma [a-c(1-h)]}{9b\gamma -4(1-h)^{2}}. \end{aligned}$$

Proposition 3

Under (A2), comparing the subgame-perfect equilibrium of the two-stage game with the Nash equilibrium of the one-stage game, we have

• (i) the R&D levels are higher under the two-stage game for both standards (i.e., $\tilde{x}_{e}>x_{e}$ and $\tilde{x}_{h}>x_{h}).$

• (ii) the output levels are higher under the two-stage game for both standards (i.e., $\tilde{q}_{e}>q_{e}$ and $\tilde{q}_{h}>q_{h}).$

Proposition 3 confirms that the equilibrium levels of R&D and output generated under each of the two instruments are greater in the two-stage game than their counterparts in the one-stage game. The intuition is the same as for the process R&D comparison in [5]. In the one-stage game, due to the simultaneity of the two choices, firms are solely focused on total cost minimization, and do not take into account the fact that more R&D in the first stage will lead to a bigger market share in the second stage. In contrast, in the two-stage game, firms do take into account both effects of R&D, and the strategic pursuit of the market share objective leads them to overspend in R&D (relative to one-shot game case). These results are thus fully in line with the findings of [5] as described above, and are thus one more contextual instance of the commitment effect in game theory.

As [5] noted in their comparison of the two types of games, the R&D levels in each of the two two-stage games may be viewed as excessive in the following sense. Under the emission standard, given the present description of the R&D possibilities, if a firm in isolation wished to produce the output level $q_{e}$, it would also choose to conduct the corresponding level of environmental R&D $x_{e}$. However, the analogous statement is not true for the output level $\tilde{q}_{e}$ and the equilibrium level of environmental R&D $\tilde{x}_{e}$ from the two-stage game. In other words, to produce output level $\tilde{q}_{e}$, a firm in isolation would find it cheaper to perform less R&D then the level $\tilde{x}_{e}$. A similar remark applies for the two separate games under the performance standard.

In conclusion, the sense in which the R&D levels in the two-stage games may be viewed as excessive relate to the simple criterion of total cost minimization (including both R&D and production costs). Indeed, relative to a first-best criterion say, the R&D levels of both types of games under consideration here will turn out to be insufficient, as is well known (see, e.g., [10] or [11]).

4.2 Welfare Comparison

This subsection provides a comparison between social welfare in the one-stage and the two-stage games, under each of the two regulatory instruments. The equilibrium welfare for the one-stage game under the emissions standard is given by (9). For the two-stage game, the equilibrium welfare, taken from [2], is

$$\begin{aligned} \tilde{W}_{e}=\frac{e^{2}(9b+8s-18b\gamma s)+e(18bc\gamma -8a)+4\gamma (a-c)^{2}}{9b\gamma -4}. \end{aligned}$$

The equilibrium welfare for the one- and the two-stage games under the performance standard can be reduced respectively to (10) and the following (from [2]),

$$\begin{aligned} \tilde{W}_{h}=\frac{2\gamma [a-c(1-h)]^{2}\text { }[9\gamma (2b-h^{2}s)-8(1-h)^{2}]}{[9b\gamma -4(1-h)^{2}]^{2}}. \end{aligned}$$

While each of the two results in the Proposition below offers a complete characterization of the welfare comparison, the result is parameter-dependent for the performance standard, but uniformly valid for the emissions standard.

Proposition 4

Under (A2), at the respective equilibria of the one- and two-stage games:

• (i) under an emission standard, social welfare is higher in the two-stage game than in the one-stage game.

• (ii) under a performance standard, social welfare is higher in the two-stage game than in the one-stage game if

  $$\begin{aligned} s<\frac{b}{h^{2}}\frac{9b\gamma -4(1-h)^{2}}{36b\gamma -14(1-h)^{2}}. \end{aligned}$$

  (12)

  If (12) is reversed, then social welfare is higher in the one-stage game.

In order to arrive at an intuitive understanding of Proposition 4, it is useful to separate the two parts. Proposition 4(i) is easy to grasp intuitively. Under an emission standard, the comparison is clear-cut and coincides with the general and well-known result in industrial organization described earlier [5]. In particular, the presence of a damage term is immaterial to this comparison, which follows directly from the fact that the same emissions limit is imposed across the two games (for a meaningful comparison). The equality of the two damage terms leads to the usual notion of social welfare in industrial organization (as the sum of consumer and producer surpluses) being the relevant one. In light of the well-known stylized fact about innovation, that an insufficient amount of R&D is typically undertaken, one would naturally expect the high R&D-high output scenario to lead in terms of social welfare (see, e.g., [12] and [11]).¹⁷

In contrast, under a performance standard, Proposition 4(ii) might at first appear counter-intuitive, since better performance in R&D and output does not always translate into better welfare performance here. However, the result becomes quite intuitive once the economic forces at work are clearly identified. With only the same emission per output ratio h imposed across the two games, the level of emissions must be higher under the two-stage game, since output is higher (by Proposition 3). Therefore, no matter how much higher the net-of-damage welfare is in the two-stage game (for the afore-mentioned reasons), for a sufficiently high damage parameter s, the ranking will switch in favor of the one-stage game. This is exactly the content of Proposition 4(ii) where the relevant threshold value for the parameter s is identified by Condition (12).

Interestingly, there is a tension here between the classical measures of welfare in industrial organization and in environmental economics. In the latter case, a higher output will amount to a negative contribution to overall welfare when damage due to pollution is sufficiently high. However, without environmental damage, the (Marshallian) social welfare used in industrial organization would always be higher under the two-stage game instead, due to a higher output [5]. In this respect, Proposition 4 is of particular interest in that it sheds light on conditions under which a two-stage game would be socially preferable (whenever the pollutant at hand yields mild pollution). A noteworthy reversal is the fact that the one-stage game is welfare superior whenever the damage function is steep enough, or whenever the underlying pollutant is sufficiently harmful to the environment.

The key condition (12) may also be instructively interpreted, for fixed value of s, in terms of the value of $(1-h)$, seen as a measure of the tightness of environmental regulation. As h decreases, regulation gets stricter and (12) is more likely to hold, as the RHS of Condition (12) is decreasing in h, as is easily verified by direct computation. Therefore, for each fixed value of $s>0$, there is a unique threshold value of h, say $\overline{h}$, such that condition (12) holds with equality. It follows that tighter regulation (i.e., h below the threshold $\overline{h}$) would always favor the two-stage game in terms of social welfare (with damage included), since it leads to a lower output and thus to less pollution damage (at a given s).¹⁸ The opposite holds for less tight regulation (i.e., h above the threshold $\overline{h}$).

The following example illustrates some aspects of Proposition 4(ii).

Example 2

Consider an industry with $a=2.5$, $\gamma =1.8$, and $b=c=s=1$. Table 2 shows the equilibrium of the one- and two-stage games under the performance standard. Observe that when $h=0.62$, the game is equivalent to that of the last column of Table 4 in [2]. Here, 1SG stands for one-stage game, and 2SG for the two-stage model.

A value of h of particular interest here is $h\approx 0.4979$, for which social welfare under the performance standard coincides for both games. In line with Proposition 4(ii), if h is lower (so the RHS of Condition (12) is higher), then the two-stage game provides a higher social welfare; if h is higher, the one-shot game does.

Table 2 Equilibrium R&D, output and social welfare for the one- and two-stage games under the performance standard; $a=2.5$, $\gamma =1.8$, and $b=c=s=1$

5 The Second-Best Problem with Endogenous Standards

In this section we return to the one-stage setting and endogenize the choice of the emission and performance standards by the social planner or regulator. Specifically, for each instrument, we consider a two-stage game wherein the regulator precommits to a pollution constraint level in the first stage and then each firm simultaneously decides its R&D level and output in the second period. The regulator selects the level of the constraint by maximizing social welfare while anticipating the firms’ equilibrium reactions under each policy instrument. As such, the aim is to characterize the optimal (second-best) solution to the regulator’s problem arising under both policy instruments.

A key observation to understand the upcoming results is that, since the two standards are set by the social planner to maximize welfare, there is no reason a priori to expect the resulting levels of emissions to be equal. In other words, the normalization rule used so far is not meaningful here and thus does not apply, as will be confirmed below.

5.1 The Welfare-Maximizing Emission Standard

As in the one-stage game, we make some assumptions on parameters to ensure that our results under the emission standard are economically meaningful. In this section, in addition to maintaining assumption (A1), we require the following:

(A3) (i) $s>\frac{b(c(9b\gamma -2)-a)}{2(a-c)(3b\gamma -1)},$ (ii) $\frac{b\gamma (9b\gamma -2)}{4b\gamma -1}>\frac{a}{c}$.

Condition (A3)(ii) is similar in interpretation, but more stringent than, Condition (A1)(ii). Recall that (A1) implies that $3b\gamma>a/c>2$ and hence, $3b\gamma >\frac{b\gamma (9b\gamma -2)}{4b\gamma -1}$. Thus, we are further restricting the possible values of a/c to conduct the analysis in this section. Assumption (A3)(i) plays the role of a second-order condition for welfare maimization and is exclusive to this section as we did not have endogenous instruments before; it states that social damage should be large enough for the environmental authority to establish a binding regulation. The proof of Proposition 5 provides technical details on (A3). With these assumptions in place, we can analyze the social welfare-maximization problem of the regulator under the emission and performance standards.

The regulator is concerned with maximizing social welfare by choosing the optimal level of the emission standard. Hence, by accounting for the one-stage equilibrium values of each firm’s R&D investment $x_{e}$ and output $q_{e}$ (recall Eq. (2)), the regulator solves the following social welfare-maximization problem

$$\begin{aligned} \underset{e}{\max }\int _{0}^{2q_e}(a-bt)dt-2(c-x_e)(q_e-e)-\gamma x_e^{2}-2se^{2}, \end{aligned}$$

(13)

where the industry emissions are equal to $E=2e$.

Plugging in the equilibrium values of R&D investment $x_{e}$ and output $q_{e}$ from Eq. (2), the regulator’s problem reduces to (upon simplification)

$$\begin{aligned} \underset{e}{\max }W_{e}(e)=\frac{(4b\gamma -1)(\gamma (a-c)^{2}-2ae)+eb(9b\gamma -2)(e+2c\gamma )-2e^{2}(3b\gamma -1)^{2}s}{(3b\gamma -1)^{2}}. \end{aligned}$$

(14)

The solution to this problem is given by Proposition 5, in which the variables of interest carry a subscript $(\cdot )^{*}$, to distinguish this solution from that of the one-stage game.

Proposition 5

Under Assumptions (A1) and (A3), there is a unique and symmetric second-best equilibrium under the emission standard, which entails the social welfare maximizing level of the emission standard, R&D levels and outputs respectively given by

$$\begin{array}{c} e^{*}=\frac{cb\gamma (9b\gamma -2)-a(4b\gamma -1)}{2s(3b\gamma -1)^{2}-b(9b\gamma -2)},\text { }x_{e}^{*}=\frac{2(a-c)(3b\gamma -1)s-b(c(9b\gamma -2)-a)}{2s(3b\gamma -1)^{2}-b(9b\gamma -2)},\text { and } \\ q_{e}^{*}=\frac{(3b\gamma -1)(2(a-c)\gamma s-a)}{2\,s(3b\gamma -1)^{2}-b(9b\gamma -2)}.\end{array}$$

Moreover, $0<x_{e}^{*}<c$ and $0<e^{*}<q_{e}^{*}$.

We now analyze the regulator’s problem for the performance standard.

5.2 The Welfare-Maximizing Performance Standard

Under the performance standard, the regulator chooses $h\in (0,1)$ with the objective of maximizing social welfare given the equilibrium values of the R&D investment, $x_{h}$, and output, $q_{h}$, given in (5). In analogy with (13), the regulator solves

$$\begin{aligned} \underset{h}{\max }\int _{0}^{2q_{h}}(a-bt)dt-2(c-x_{h})q_{h}(1-h)-\gamma x_{h}^{2}-2s(hq_{h})^{2}. \end{aligned}$$

(15)

This is the usual sum of consumer and producer surplus, with the latter including R&D costs, which here also includes the quadratic environmental damage costs.

Given the equilibrium values of R&D investment $x_{h}$ and output $q_{h}$ in Eq. (5), the regulator’s problem reduces to

$$\begin{aligned} \underset{h}{\max }\text { }W_{h}(h)=\frac{\gamma [a-c(1-h)]^{2}[2\gamma (2b-sh^{2})-(1-h)^{2}]}{[3b\gamma -(1-h)^{2}]^{2}}. \end{aligned}$$

Unfortunately, the solution to the social welfare maximization problem of the regulator in Eq. (15) is analytically non-tractable, but based on many numerical simulations, we establish some important observations in the next section.

5.3 Comparison of the Second-Best Solutions for the Two Standards

Before comparing the second-best solutions under the two standards, we provide a comparative statics analysis of the socially optimal standards.

Proposition 6

Assuming interior solutions, the comparative statics of $e^{*}$ and $h^{*}$ with respect to changes in the key parameters are the same:

• (i) $e^{*}$ and $h^{*}$ are decreasing in a.

• (ii) $e^{*}$ and $h^{*}$ are increasing in $\gamma .$

• (iii) $e^{*}$ and $h^{*}$ are decreasing in s.

These results are fairly intuitive. Under both policy instruments, the regulator would choose lower effective pollution limits when the willingness to pay or market size a increases (at constant b), in anticipation of a higher output and thus pollution level, as well as when pollution causes more environmental damage; but higher pollution limits when R&D is more costly so as not to overburden firms.

For the rest of the analysis, we provide numerical insights into the equilibria, since a closed-form solution under the performance standard is not possible. To do so, we assume values for parameters $b=c=1$ holding throughout and we obtain the solution to the game under the performance standard computationally. Observe that $b=1$ implies that a accounts exactly for market size, and the demand slope is 1. When $c=1$, the initial abatement cost corresponds to the level of emissions abated. Using the same restrictions on the parameters we compute the equilibrium variables under the emission standard from Proposition 5.

Thus, we compute the solution for representative sets of parameters, where we choose to vary one variable at a time while keeping the other parameters constant. Specifically, we investigate how changes in the market size a (given $b=1$), the unit cost of R&D $\gamma ,$ and the extent of damage s affect the comparison of the equilibrium values of R&D, output, emissions, abatement and welfare generated under the emission and performance standards in the two games. Some examples of the calculations made are presented in Appendix B.

From several numerical simulations, we retain that (i) the results concerning the comparison of R&D, output, and abatement levels are highly parameter-dependent (the comparisons can go either-way) and (ii) the resulting (socially optimal) welfare is consistently higher under the performance standard.

The strong dependence of the comparison on parameter values (part i above) stands in sharp contrast to the clear-cut results derived in Proposition . This is due to the endogeneity of the two standards in this section, i.e., to the absence of the normalization of equal final emissions under the two instruments, a key assumption of Proposition 1. These discrepancies in the results of the one-stage game and the second-best problem are clearly to be attributed to the actions of the regulator, who takes an active role in the second-best game and selects the pollution constraints under each instrument so as to maximize social welfare. In other words, for the second-best problem, the final pollution level generated by each firm is different across the two policy instruments (for instance, see Table 3 in Appendix B). Consequently, the results obtained in the presence of the passive regulator no longer hold, and neither of the two instruments uniformly leads in terms of equilibrium R&D, output and level of abatement.

Finally, in contrast to our earlier conclusions, the uniform welfare comparison result supports earlier findings by [2], who also conclude that the performance standard should be the preferred environmental instrument of the regulators (with a social welfare objective). The latter is clearly the most comprehensive criterion for the comparison of the two command-and-control policy instruments.

6 Conclusion

This study has examined the comparative performance of emission and performance standards in a one-stage game-theoretic model of abatement R&D and Cournot product market competition, in terms of R&D, industry output and social welfare. Firms simultaneously select R&D and output levels, given exogenous pollution constraints leading to the same emission levels for a meaningful comparison. While less prevalent than the two-stage version, the one-stage game was adopted in several well-known studies on innovation.

The first result is that a performance standard generates higher R&D investments and industry output, but lower profit, than the pollution-equivalent emissions standard. A similar conclusion extends to social welfare only under a mild condition of high demand (relative to cost). These results are far more clear-cut than their counterparts in the two-stage version of this model analyzed by [1] in a general setting and by [2] under the present specification, which were highly parameter dependent.

We also conduct a similar comparison for each of two instruments across the one-stage and the two-stage models. We find that the two-stage model leads to higher levels of R&D and industry output than the one-stage game for both the emissions and performance standards. The same conclusion applies to the social welfare comparison for the emissions standard. However, for the performance standard, the same conclusion on welfare extends if and only if the environmental damage parameter is below an identified threshold. We argue that these conclusions are in line with economic intuition when one takes into account the role of the damage term in the welfare function in environmental economics.

When the instruments are endogenized via welfare maximization by a social planner, the results concerning the comparison of R&D, output, and abatement levels turn out to be highly parameter-dependent, but nevertheless a key conclusion obtains: The performance standard always leads to a higher final social level than the emission standard.

On the whole, our results provide some novel support for the view that a performance standard tends to be superior to an emission standard. However, under low demand in a small parameter region, the welfare and the abatement R&D results yield conflicting recommendations. As to the possible policy implications of the comparison between the two game forms, one may attribute another role for a social planner, that of inducing a two-stage or a one-stage interaction for a particular industry. For instance, to give rise to a two-stage game, the planner may conceivably mandate that the firms make credible announcements of their abatement or pollution levels, or alternatively provide incentives (such as R&D matching subsidies) for them to do so in an irrevocable manner. One implication of Proposition 4 of interest is that a two-stage game would be welcome whenever the pollutant for the industry at hand is relatively mild, with the opposite holding for strong pollutants.

As possible future work on this topic, one may consider a new game intermediate between the two polar cases of one-stage and two-stage game, wherein observability of R&D choices takes place with exogenous probability p (in which case the game is two-stage) and non-observability with probability $1-p$ (one-stage game).

Appendices

Appendix A. Proofs

This section provides the proofs for all the results of the paper. Since the proofs are based on simple but sometimes involved computations, only the main lines are presented to allow the interested reader to follow the steps; details are thus mostly left out.

Proof of Proposition 1

• (i) Provided that $e=q_{h}h=\frac{\gamma h[a-c(1-h)]}{3b\gamma -(1-h)^{2}}$, we have

  $$\begin{aligned} x_{e}=\frac{(a-c)}{3b\gamma -1}-\frac{3b}{3b\gamma -1}\frac{\gamma h[a-c(1-h)]}{3b\gamma -(1-h)^{2}} \end{aligned}$$

  and

  $$\begin{aligned} x_{h}-x_{e}=\frac{h[3bc\gamma -a(1-h)]}{[3b\gamma -1][3b\gamma -(1-h)^{2}]}, \end{aligned}$$

  which is clearly (strictly) positive by (A1) and the fact that $0<h<1$.

• (ii) Similarly,

  $$\begin{aligned} q_{h}-q_{e}=\frac{\gamma h[3bc\gamma -a(1-h)]}{[3b\gamma -1][3b\gamma -(1-h)^{2}]}>0. \end{aligned}$$

• (iii) The profit result follows from the following inequalities:

  $$\begin{aligned} \pi _{e} &= {} q_{e}(a-2bq_{e})-(c-x_{e})(q_{e}-e)-\gamma x_{e}^{2}/2 \\ &\ge {} q_{h}(a-b(q_{e}+q_{h}))-(c-x_{h})(q_{h}-e)-\gamma x_{h}^{2}/2 \\ &> {} q_{h}(a-2bq_{h})-(c-x_{h})q_{h}(1-h)-\gamma x_{h}^{2}/2=\pi _{h}. \end{aligned}$$

  The first inequality follows by the Nash property, the second one, by $q_{e}<q_{h}$ (part ii) and $e=q_{h}h$. The equalities are given by definition of the two equilibrium profit levels.

This completes the proof of Proposition 1. $\square$

Proof of Proposition 2

Since we fixed $e=q_{h}h=\frac{\gamma h[a-c(1-h)]}{3b\gamma -(1-h)^{2}}$, we can write $W_{e}$ in terms of h, and then compute the difference

$$\begin{aligned} W_{h}-W_{e}=-C(bc\gamma A-aB)/D, \end{aligned}$$

where

$A=h(6b\gamma -1)+(6b\gamma -2)>0,$ $B=(4b\gamma -1)h(1-h)+2b\gamma (3b\gamma -1)>0,$

$C=\gamma (3bc\gamma -a(1-h))h>0,$ and $D=[3b\gamma -1]^{2}[3b\gamma -(1-h)^{2}]^{2}>0$.

Here the first three inequalities follow by (A1) and the last one is obvious. Then, $W_{h}>W_{e}$ if and only if $\frac{a}{c}>b\gamma \frac{A}{B}$, which leads to the desired result. $\square$

Proof of Proposition 3

Assumption (A2) is required for a well-defined, interior and symmetric subgame-perfect equilibrium in the two-stage games (see details in [2]). To prove the four desired inequalities here, we proceed by direct calculations. As the steps are very simple, we leave the details to the reader.$\square$

Proof of Proposition 4

First, observe that Assumption (A2) is imposed here for the same reasons as in the proof of Proposition 3.

• (i) Using the expressions for $W_{e}$ and $\tilde{W}_{e}$ given in the text, we have after simplification that $\tilde{W}_{e}-W_{e}=\frac{b(e-(a-c)\gamma )^{2}}{(3b\gamma -1)^{2}(9b\gamma -4)}$, which is strictly positive since $9b\gamma >8$.

• (ii) Using the expressions for $W_{h}$ and $\tilde{W}_{h}$ given in the text, we have after simplification

  $$\begin{aligned} \tilde{W}_{h}-W_{h}=\frac{\gamma ^{2}(1-h)^{2}[a-c(1-h)]^{2}[b(9b\gamma -4(1-h)^{2})-h^{2}s(36b\gamma -14(1-h)^{2})]}{[9b\gamma -4(1-h)^{2}]^{2}[3b\gamma -(1-h)^{2}]^{2}}>(<)0 \end{aligned}$$

  if $\frac{b}{h^{2}s}>(<)\frac{36b\gamma -14(1-h)^{2}}{9b\gamma -4(1-h)^{2}}$

Proof of Proposition 5

The reader can easily verify that the FOC for the social welfare maximizing problem (14) is:

$$\begin{aligned} \frac{-2 a ( 4 b \gamma -1) + 2b ( 9 b \gamma -2)(e + c \gamma ) - 4 e (3 b \gamma -1)^2\,s}{(3 b \gamma -1)^2}=0, \end{aligned}$$

which leads to $e^*=\frac{cb\gamma (9b\gamma -2)-a(4b\gamma -1)}{2\,s(3b\gamma -1)^{2}-b(9b\gamma -2)}$. Substituting this result into equations (2) leads to $x^{*}_e=\frac{2(a-c)(3b\gamma -1)s-b(c(9b\gamma -2)-a)}{2\,s(3b\gamma -1)^{2}-b(9b\gamma -2)}$ and $q^{*}_e=\frac{(3b\gamma -1)(2(a-c)\gamma s -a)}{2\,s(3b\gamma -1)^{2}-b(9b\gamma -2)}$.

The SOC of this problem is $\frac{2b(9b\gamma -2)-4\,s(3b\gamma -1)^{2}}{(3b\gamma -1)^{2}}<0,$ equivalent to $2\,s(3b\gamma -1)^{2}-b(9b\gamma -2)>0$ or $s>\frac{b(9b\gamma -2)}{2(3b\gamma -1)^2},$ that is, the denominator of $e^*$, $x^*_e$ and $q^*_e$ must be strictly positive. (A3)(i) and (A3)(ii) imply the last inequality, since $\frac{b(c(9b\gamma -2)-a)}{2(a-c)(3b\gamma -1)}>\frac{b(9b\gamma -2)}{2(3b\gamma -1)^2}$. Notice that (A1) implies that $a-c>0$, $3b\gamma -1>0$ and $9b\gamma -2>0$. If (A3)(ii) also holds, we have that $c(9b\gamma -2)-a>0$, otherwise, it must be that $3b\gamma <1,$ which contradicts (A1).

Clearly, (A3)(i) and (A3)(ii) imply that $e^*>0$; these two assumptions also imply that $x^*_e<c$. To see this, observe that $c-x^*_e=\frac{-a b + 2 ( 3 b \gamma -1) ( 3 b c \gamma -a) s}{ 2\,s(3b\gamma -1)^{2}-b(9b\gamma -2)}>0$ iff $s>\frac{ab}{2(3b\gamma -1)(3bc\gamma -a)}$; by (A1), $3b\gamma -1>0$ and $3bc\gamma -a>0$. (A3)-(ii) implies that $\frac{b(c(9b\gamma -2)-a)}{2(a-c)(3b\gamma -1)}>\frac{ab}{2(3b\gamma -1)(3bc\gamma -a)}$, and thus, (A3)(i) and (A3)(ii) guarantee that $x^*_e<c$.

Finally, observe that (A3)(i) implies that both the numerator and the denominator of $x^*_e$ are strictly positive, hence, $x^*_e>0$. In addition, $q^*_e-e^*=\frac{\gamma [2 (a - c) ( 3 b \gamma -1) s-b(c ( 9 b \gamma -2)-a) ]}{ 2\,s(3b\gamma -1)^{2}-b(9b\gamma -2)}>0$ iff $s>\frac{b(c(9b\gamma -2)-a)}{2(a-c)(3b\gamma -1)}$, corresponding to (A3)(i). $\square$

Proof of Proposition 6

The comparative statics results for $e^{*}$ are straightforward from the (closed-form) expression for $e^{*}$. Since a closed-form expression for $h^{*}$ is not tractable, we shall use [13] theorem for monotone comparative statics [13] or Vives, [14].

(i) The idea is to take $\ln W_{h}(h)$ to turn it into additively separable parts, and then take the cross-partial w.r.t. h and the relevant exogenous variable. In the case of a, we get $\frac{\partial ^{2}\ln W_{h}(h)}{\partial h\partial a}=\frac{-2c}{[a-c(1-h)]^{2}}<0$. Hence, by Topkis’s theorem, $h^{*}$ is decreasing in a.

(ii)-(iii) Similarly, it is easy to check that $\frac{\partial ^{2}\ln W_{h}(h)}{\partial h\partial \gamma }>0$ and $\frac{\partial ^{2}\ln W_{h}(h)}{\partial h\partial s}<0$, which imply that $h^{*}$ is increasing in $\gamma$ and decreasing in s. $\square$

Appendix B

This Appendix provides a comparison of the equilibrium variables of interest in the game with endogenous standards, for a given set of parameters. To distinguish the second-best solution from that of the one-stage game, we will add the superscript $^{*}$ to the variables. Thus, $e^{*}$($h^{*}$) denotes the equilibrium level of emission (performance) standard, while $W_{e}^{*}$ ($W_{h}^{*}$) stands for the corresponding welfare. Throughout this section we assume $b=c=1$, meaning that a accounts exactly for market size, the demand slope is 1, and the initial unit cost of abatement is one.

1.1 Varying Parameter a

We first consider the performance of both policy instruments assuming a variation in the size of the market a. Table 3 provides this comparison as the market size a varies.

Table 3 Second-best solution for $b=c=1$, $s=\gamma =2.5$ and chosen values of a

As seen in Table 3, as higher output leads to more abatement, firms have a higher incentive to invest in R&D (the second row in Table 3). Since higher output raises the pollution level, the regulator tightens the standards (decrease in the level of standard in the first row in Table 3) in order to reduce the damage to the environment. Overall, social welfare increases.

Further details concerning the impact that the change in parameter a has on the comparison between the equilibrium variables under the emission and performance standards are given next. Here and in the next two subsections, the given parameter values are chosen as representative and in respect of Assumption (A3).

Remark 2

Let $b=c=1$, $s=\gamma =2.5$ and $1.59\le a\le 5.69$.¹⁹ Then

• (i) for $1.59\le a<1.79,$ $x_{e}^{*}<x_{h}^{*}$, $q_e^*<q_h^*$, $e^{*}>q_{h}^{*}h^{*}$, and $q_e^*-e^*<q_h^*(1-h^*)$;

• (ii) for $1.79< a<3.09,$ $x_{e}^{*}<x_{h}^{*}$, $q_e^*<q_h^*$, $e^{*}<q_{h}^{*}h^{*}$, and $q_e^*-e^*<q_h^*(1-h^*)$;

• (iii) for $3.09< a<5.19,$ $x_{e}^{*}>x_{h}^{*}$, $q_e^*<q_h^*$, $e^{*}<q_{h}^{*}h^{*}$, and $q_e^*-e^*>q_h^*(1-h^*)$;

• (iv) for $5.19< a \le 5.69,$ $x_{e}^{*}>x_{h}^{*}$, $q_e^*>q_h^*$, $e^{*}<q_{h}^{*}h^{*}$, and $q_e^*-e^*>q_h^*(1-h^*)$;

• (v) $W_{h}^{*}>W_{e}^{*}$.

Remark 2 shows how R&D investment, output, emissions, abatement and welfare change with market size under the two policy regimes. For small market sizes (parts i-ii), the performance standard generates higher R&D, industry output and abatement, and lower pollution levels. If $1.79<a<3.09$ (part ii), all the variables are higher under the performance standard. In part (iii), the performance standard leads in terms of output, but lags in R&D and in abatement level. For a large market size (part iv), the emission standard dominates in terms of R&D, output and abatement. One consistent outcome is that the comparison of R&D investment mirrors that of the abatement level.

Overall, considering all relevant values of a, the comparison of the equilibrium levels of R&D and output under the two policy instruments is highly parameter-dependent, but the performance standard is uniformly superior for social welfare (part v).

1.2 Varying Parameter $\gamma$

We turn to the impacts that an increase in the cost of R&D, $\gamma$, has under both policy instruments. Table 4 demonstrates the results of this comparison for different values of $\gamma$.

Table 4 Second-best solution for $b=c=1$, $s=2.5$, $a=3$ and selected values of $\gamma$

As R&D becomes more costly, firms reduce their output (the third row in Table 4) and their R&D efforts (the second row in Table ), in order to lower abatement cost. In an attempt to limit the resulting consumer surplus loss, the regulator weakens the stringency of the pollution constraint under each policy instrument (the amount of pollution allowed increases as shown in the first row in Table 4). It is intuitive that welfare goes down.

Remark 3 summarizes these findings and gives details concerning the comparison between the equilibrium variables under both policy instruments; $s=2.5$ and $a=3$ were chosen for illustrative purposes.

Remark 3

Let $a=3$, $b=c=1$, $s=2.5$ and $\gamma \ge 1.30$.²⁰ Then (i) for $1.3 \le \gamma < 1.39,$ $x_{e}^{*}>x_{h}^{*},$ $q_{e}^{*}>q_{h}^{*}$ and $q^*_e-e^*>q_{h}^{*}(1-h^{*});$ (ii) for $1.39< \gamma < 2.33,$ $x_{e}^{*}>x_{h}^{*},$ $q_{e}^{*}<q_{h}^{*}$ and $q^*_e-e^*>q_{h}^{*}(1-h^{*});$ (iii) for $2.33 < \gamma ,$ $x_{e}^{*}<x_{h}^{*},$ $q_{e}^{*}<q_{h}^{*}$ and $q^*_e-e^*<q_{h}^{*}(1-h^{*});$ (iv) $e^*<q_{h}^{*}h^{*}$; (v) $W_{h}^{*}>W_{e}^{*}$.

Here, the emission standard delivers more R&D and output at low R&D cost (part i), but less of both at high R&D cost (part iii). This occurs because under the performance standard the fall in output not only has the desired effect of a reduction in abatement cost, but it also leads to a reduction in environmental damage (the term $2s(hq_{h})^{2}$ in Eq. (15)). This effect is absent under the fixed emission standard, where the reduction in output does not directly affect the level of environmental damage (i.e., the term $2se^{2}$ in Eq. (13) does not contain $q_{e}$). Then, smaller reductions in output are required under the performance standard to mitigate the increased R&D cost. Hence, for a high level of R&D cost, output is higher for the performance standard.

Once more, the performance standard dominates in terms of welfare for all our parameter values, but pollution is lower under the emission standard.

1.3 Varying Parameter s

This part evaluates the comparative performance of the two standards as the damage parameter s varies. The comparison is presented in Table 5, in which, in addition to varying values of parameter s, we also assume different values of $\gamma$. In this way, we are able to capture the range of possible results (qualitatively).

Table 5 Second-best solution for $a=3$, $b=c=1$ and A: $\gamma =2.5$, $s=10$; B: $\gamma =s=1.3$

Segments A and B of Table 5 compare the equilibrium values of R&D, output, emissions, abatement and welfare under both policy instruments for different values of parameters. In Segment A, $\gamma =2.5$ and $s=10$ have been chosen for illustrative purposes. Table 5 shows that for these parameter values the performance standard outperforms the emission standard in terms of the incentives to generate higher output and welfare, but not R&D. Remark 4 complements these results by showing how the relationship between R&D, output, emissions, abatement and welfare under the two policy instruments changes with different values of s, while $\gamma$ is kept constant.

Remark 4

Let $a=3,$ $b=c=1$, $\gamma =2.5$ and $s\ge 0.68$.²¹ Then

• (i) for $s<(>)5.86$, $x_{h}^{*}>(<)x_{e}^{*}$ and $q_{h}^{*}(1-h^{*})>(<)q_{e}^{*}-e^{*}$;

• (ii) $q_{h}^{*}>q_{e}^{*}$ and $q_{h}^{*}h^{*}>e^{*}$;

• (iii) $W_{h}^{*}>W_{e}^{*}.$

Remark 4 confirms that the comparison of R&D levels under the two policy instruments depends on the value of s. When environmental damage is not significant (part i), the performance standard generates more R&D incentives; however, high values of s lead to a reversion. This is not the case for output and welfare, which are larger under the performance standard under the present set of parameters.²²

To see that the performance standard does not generally provide more incentives to produce output, we now consider the effects of varying s under a different value of $\gamma$. An example is illustrated in part B of Table 5.

Remark 5

Let $a=3$, $b=c=1$, $\gamma =1.3$ and $s\ge 0.84$.²³ Then

• (i) $x_{h}^{*}<x_{e}^{*}$ and $q_{h}^{*}(1-h^{*})<q_{e}^{*}-e^{*}$;

• (ii) $q_{h}^{*}<q_{e}^{*}$ and $q_{h}^{*}h^{*}>e^{*}$;

• (iii) $W_{h}^{*}>W_{e}^{*}$.

Here, output under the emission standard is larger than under the performance standard, and so is environmental R&D, with the latter reversing the conclusion of Proposition 1. Hence, once more, this confirms that the results of the comparison of R&D and output incentives depends in key ways on whether the two regulatory regimes are exogenously given (on the basis of equal emissions) or endogenous (i.e., set to maximize welfare).