Introduction

Hydrogen bonding, London dispersion, and other electrostatic multipole interactions all play important roles in the spatial organization of molecules in crystalline polysaccharides, such as cellulose, chitin, and chitosan, which are composed of long linear ribbon-like sugar chains where hydroxyl groups are all in equatorial direction. Hydrogen bonds between hydroxyl groups are essentially electrostatic interaction between electronegative oxygen lone-pair electrons and slightly electropositive hydrogen whose electron were pulled by neighbor oxygen and can be considered as interaction between dipoles (Ramos-Cordoba et al. 2011). London dispersion interaction originates from synchronized polarization of atomic nuclei and is always attractive. Atoms in a molecule have different electronegativity, and thus the sum of all pair-wise electrostatic interactions can be considered as multipole interactions. This is usually considered in modern molecular modeling as interaction between partial charges, which are primarily calculated using DFT method.

Understanding these interactions is of interest in the perspective of the fundamental properties of cellulose materials. One example is the process of dispersing native cellulose fiber aggregates into isolated nanofibrils or into individual polymer chains, that is, dissolution. It has often been stated that it is the “many” and “strong” inter- and intra-molecular hydrogen bonds that hinder defibrillation and dissolution of cellulose (Wang et al. 2016). A parallel can be found in the stability of double stranded DNA, which has often been explained based on the regular hydrogen bonds between opposing nucleobases in a pair. The justification to that is that a typical interaction energy of a base pair is about one order of magnitude higher than dispersion energy from an atom pair (Gooch 2007). However, recent experiments and simulations give more weight to the contribution of dispersion interaction on the stability of double helical structure, to the point that it may even exceed the hydrogen bond interaction (Černý et al. 2008; Kolář et al. 2011; Jahiruddin and Datta 2015; Perumal and Subramanian 2017; Kumar and Patwari 2019; Feng et al. 2019).

For cellulose, the industrially most important crystalline polysaccharide, the interest in how dispersion interaction influences structural stability has been revived, especially in aqueous systems where hydrophobic effects would dominate and hydrogen bonds become insignificant (Bergenstråhle et al. 2010; Medronho et al. 2012; Glasser et al. 2012). Moreover, it has been shown that the dispersion energy contribution to molecular cohesion can be twice the hydrogen bond contribution in the native cellulose crystal (Nishiyama 2018). Therefore, the influence of the different interactions on the structure and properties of cellulose needs to be re-examined.

Another example of where the relative contributions of different molecular interaction has been considered is the intrinsic mechanical properties of cellulose, which has also sometimes been attributed specifically to hydrogen bonds (Eichhorn and Davies 2006). In this case too, a parallel can be made with other biomolecules. Recently, by combining DFT calculations and nanoindentation experiments, both the types and direction of hydrogen bond were found to be correlated to the anisotropic modulus of amino acids (Azuri et al. 2015). It was concluded that the planar hydrogen bonding network co-contributed to the molecular stiffness, resulting in unusually large Young’s moduli of amino acid molecular crystals along certain crystal facets. A similar trend for crystalline amino acid hydrogen maleates was reported, where the moduli can differ up to fivefold between certain facets. (Matveychuk et al. 2018) Furthermore, by comparing DFT calculations with and without dispersion correction, the dispersion interaction was found to induce similar enhancement as hydrogen bonds to the rigidity of diphenylalanine based peptide. (Azuri et al. 2014).

The effect of hydrogen bonds on the elastic moduli of cellulose Iβ has been studied using molecular mechanics and/or molecular dynamics by switching the explicit hydrogen bond term on and off (Tashiro and Kobayashi 1985; Eichhorn and Davies 2006), in force fields that define such terms. The effect of dispersion interactions has not been studied in isolation, but the contribution from the Lennard–Jones potential, where the dispersion term is one part, was shown to be at least twice that of the contribution from electrostatics (Wohlert et al. 2012; Djahedi et al. 2015), depending on which force field that was used. The elastic tensor has been studied using first principles approach using density functional theory (Dri et al. 2013) and with thermal vibration corrections (Dri et al. 2014), but the contribution of dispersion interaction on mechanical properties has not been calculated. In quantum mechanical calculations, one cannot switch the hydrogen bonding off, but here the dispersion interaction can be simply neglected in the calculation to see its contribution. Hence, we calculated the elastic tensor of all four cellulose allomorphs using DFT to investigate the effect of dispersion interactions on their intrinsic elastic mechanical properties.

Methods

The initial atomic coordinates of crystals are imported from X-ray and neutron studies as well as molecular dynamics simulation and DFT optimization (Langan et al. 1999; Nishiyama et al. 2002, 2003; Wada et al. 2004; Chen et al. 2015). The hydrogen bonding pattern A was used for Iα and Iβ, and the pattern B was used for II and IIII. Geometry optimization was performed using the generalized gradient approximation (GGA) functional PBE (Perdew et al. 1996), augmented with pairwise DFT-D2 correction for long-range dispersion (Grimme 2006). Different k-grids are selected according to different unit cells dimensions: 2 × 3 × 3 for Iα, 2 × 2 × 2 for Iβ and II, and 4 × 2 × 2 for IIII. The kinetic energy cutoff was 160 Ry. The convergence threshold of total energy and forces for ionic minimization are 1.0e-6 Ry and 1.0e-5 Ry/bohr respectively. Periodic calculations were carried out using Quantum Espresso (version 6.6) (Giannozzi et al. 2009, 2017) in combination with ElaStic (Yanchitsky and Timoshevskii 2001; Golesorkhtabar et al. 2013), a universal tool for calculating elastic constants from first principles. All allomorphs except cellulose Iα have P21 symmetry, thus the tensor was determined using the energy-strain method with 13/21 combinations of strain direction to deduce the 13/21 tensor elements (21 elements for triclinic and 13 for monoclinic). For each combination of strain, 11 points were calculated with strain amplitude up to 0.01 and the energy fitted with a parabolic function. To visualize the feature of elastic tensors, the elastic tensors were decomposed into 6 eigenvalues \({\lambda }_{i}\) and 6 pairs of eigentensors of stress and strain equivalents where

$$\sigma_{i} = \lambda_{i} \varepsilon_{i}$$

The stress/strain tensors have 6 independent components and can be visualized in three dimension using the PAScal software (Cliffe and Goodwin 2012). The 3D contour surface plots were generated using the online Anisotropic calculator from Zuluaga (2013) and further processed using Paraview (Ahrens et al. 2005).

Results and discussion

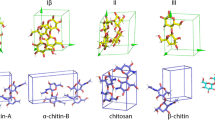
The influence of dispersion energy on the predicted unit cell parameters, volume, and d-spacing of crystalline cellulose is presented in Table 1. With the dispersion correction applied, DFT generally underestimated the experimental values by up to about 5% but can still be regarded to reproduce the experimental data within a reasonable range. This is acceptable because DFT optimized quantities represent the geometry at 0 K, whereas the experimental measurement refers to that at 300 K. Indeed, crystal structure measurements of Iβ by neutron diffraction at 15 K showed decreased unit cell dimensions compared to room temperature (Nishiyama et al. 2008), which further lowers the difference between experiment and simulation to less than 1%. In contrast, without dispersion correction, the predicted dimensions were overestimated by up to 10%. In this case, relatively large deviations can be found in the direction normal to the pyranose ring ([1 1 0] of Iα, [2 0 0] of Iβ, [0 2 0] of II, [1 1 0] and [1 -1 0] of IIII, as shown in Fig. 1b). If also thermal expansion was included, the predicted values would deviate even more from experiment. Thus, dispersion interactions contribute significantly to the tight assembly of cellulose chains in the crystalline state. This is in line with a previous report where a similar strategy was used (Bučko et al. 2011).

Table 1 The unit cell parameters (in Å and degrees), volume (Å3) and d-spacing (Å) of crystalline cellulose from experiment (exp) and DFT calculations with dispersion correction (Disp.) or without dispersion correction (No disp.) applied
Full size table
Fig. 1
figure 1

a 3D representation of the elastic moduli surface of crystalline cellulose, either with (the grid contour) or without dispersion correction (the solid contour) from two different perspectives. b: The lateral isosurface in B is multiplied by a factor of two for Iα, Iβ, and IIII, and by a factor of three for II for better visualization.The snapshots of the four crystalline cellulose allomorphs are labeled with unit cell and deformation vectors. The projections of cross-sections are consistent with the isosurface orientations above. Unit cell parameter a is parallel to x (except for Iβ, b//x), c parallel to z, and y within the ab plane

Full size image

3D contour plots of the orientation dependent Young's modulus are shown in Fig. 1. The full elastic tensor in Voigt representation is given in Table 2 and the corresponding compliance tensor is presented in Table S1. Calculated values for cellulose Iβ, especially the diagonal values of stiffness tensor, is consistent with previous DFT results (Dri et al. 2013). The off-diagonal values (Cij, i ≠ j, Table 2) are also of the same magnitude as in previous work. Cancellation of dispersion interaction resulted in a systematical reduction of the calculated values for all allomorph, varying between − 4.0 and − 72% for shear moduli (C44, C55, C66 in Table 1), and from − 10 to − 70% for tensile moduli (C11, C22, C33 in Table 1), respectively. The relative contribution of dispersion interaction on the modulus is anisotropic. It is relatively large (40% to 70%) in the ring packing direction (010 in Iβ, 1–10 in Iα, 020 in II, and 1–10 in IIII, respectively) and small in the longitudinal chain and hydrogen bonding directions. This is expected as the covalent bonds are dominating in this direction. Still, dispersion contributes between to 5 to 17% of the Young’s modulus along the chain, which is similar to the energy decomposition analysis reported (17%) using empirical force field based molecular dynamics simulation (Wohlert et al. 2012) of cellulose Iβ.

Table 2 The stiffness tensor of crystalline cellulose from DFT calculation
Full size table

In the transverse directions (C22 and C33 of Iα, C11 and C22 of Iβ, II and IIII) the reduction in stiffness tensor components without dispersion correction was mostly positive, which are 46% and 53% for Iα, 31% and 70% for Iβ, − 10% and 46% for II, 40% and 32% for IIII, respectively.

With dispersion correction applied, the C11 is 99.2 GPa. ‘1’ in cellulose Iβ is the direction to which both hydrogen bonding interactions and the pyranose rings are almost parallel. This is much higher than the other transverse modulus, which can be ascribed to the assistance of both directional hydrogen bonds and the rigidity of the glucose ring. When no dispersion correction was applied, this elastic modulus drops by 30%, indicating that the dispersion, in this direction, contributes to nearly one third of the total value.

In the sugar stacking directions, which is the directions perpendicular to the [2 0 0] direction in Iβ, [1 1 0] in Iα, [0 2 0] in II, and [1  − 1 0] in IIII (Fig. 1), the response to switching off the dispersion correction was similar, which was found to contribute 40 to 70% of the rigidity.

The dispersion correction also has significant influence on the off-diagonal elements. The values of elastic tensor elements depend on the reference frame, and the standard convention is to take the direction 1 along the a-axis, and direction 2 in the ab-plane (as shown in Fig. 2). However, the unit cell itself is not necessarily representing the principal axes of physical properties. To compare the different elastic tensors in a more universal way, we decomposed the elastic tensor into eigentensors that are stress/strain tensor pairs that are directly related by a scalar value, the corresponding eigenvalue, which is the measure of stiffness.

Fig. 2
figure 2

The 3D display of eigenvalue tensor of four cellulose allomorph with or without dispersion correction for Iα, Iβ, II, and IIII, respectively

Full size image

The stress/strain tensors have 6 independent elements corresponding to axial (normal) and shear stress/strain. They can be further reduced to three eigenvectors that translates to a pure axial stress/strain. If the corresponding eigenvalues of all eigenvectors have the same sign, they can be represented by ellipsoids, similar to thermal factor in crystallographic representation. However, the stress/strain tensor can imply axial stress/strain of opposite sign. Thus, we represent the tensor using color coding for positive and negative values, with a surface located at a distance proportional to the axial stress/strain in each direction. In general, when the mode is dominated by axial stress/strain, the color is uniform, whereas shear mode shows opposite colors in orthogonal directions.

The estimated Poisson’s ratio is shown in Table S2. In comparison to experimental measurements (Nakamura et al. 2004), Iβ showed slightly larger calculated v31 (0.49 vs. an experimental value of 0.38), and II showed a smaller v32 (0.16 vs. 0.30).

To compare the elastic tensors of different allomorphs and in different conditions (with and without dispersion correction), eigentensors were sorted in descending order of the eigenvalue (stiffest first) of the stiffness tensor calculated with dispersion correction (first row of each allomorph in Fig. 2). To find the corresponding eigentensor from the calculations without dispersion correction (shown on the second row), the closest eigentensor was chosen, based on a distance measure. This distance was calculated as an inner product, which is 1 when they match perfectly, and 0 when orthogonal. The corresponding structure is drawn in the same reference frame.

Figure 2 shows that in all cases the highest eigenvalue is dominated by axial deformation along the chain direction, which was already seen from the diagonal elements of the stiffness tensor.

For cellulose Iβ the eigentensors following the first one have a straightforward interpretation. The second eigentensor is axial deformation almost parallel to the y-axis. This is the direction of inter-molecular hydrogen bonding, parallel to the pyranose plane. The third one is orthogonal to the two, followed by three shear modes: yz, xy, and xz.

The fact that, considering lateral directions only, the structure is stiffest in the direction of inter-molecular hydrogen bonding is often discussed as manifestation of the effect of hydrogen bonding. However, it should be remembered that the density of covalent bonds is higher in this direction (along unit cell b of Iβ in Fig. 1b), while the non-covalent interactions appears only every 8 Å (d0 1 0 = 0.82 nm), compared to every 4 Å (d2 0 0 = 0.39 nm) in the perpendicular direction. Since a typical stable non-bonded separation is of the order of 3 Å, properties perpendicular to the pyranose ring plane are dominated by non-bonded interactions. In the hydrogen bonded plane ([2 0 0] of Iβ), atoms are mostly linked through covalent bonds, contributing to the high rigidity.

For cellulose II and IIII, still only considering lateral directions, the tensile deformation in the pyranose plane is highly coupled to its perpendicular direction and, in the case of cellulose II the second eigentensor almost appears as elongated doughnuts when dispersion correction is on. This tendency can be also verified in the off-diagonal element value that are similar to the diagonal element in x and y directions. The axial deformation in the direction perpendicular to the pyranose plane is coupled to xy shear (3rd tensor). At first sight, one would tend to interpret this feature as due to a zig-zag hydrogen bond pattern leading to a honeycomb-like response, but the fact that the dispersion correction enhances this tendency suggests that the mechanism is more complex and requires further clarification.

The eigentensors of cellulose Iα is strikingly different from Iβ, except for the stiffest mode. The deformation of a material is dominated by soft components, and thus cellulose Iα and Iβ would behave quite differently in complex stress environment in a dense material.

Conclusion

We have systematically calculated unit cell parameters and elastic moduli of four crystalline cellulose allomorphs using DFT. The influence of the dispersion energy on both crystal structures and elastic constants was investigated by switching the dispersion correction on and off. Our calculations reveal that the dispersion interactions dominate the stacking of cellulose chains, in all crystal forms, especially in the direction perpendicular to the pyranose rings ([2 0 0] in Iβ, [1 1 0] in Iα, [0 2 0] in II, and [1 -1 0] in IIII, respectively). In this direction, dispersion energy contributes more than 50% to the elastic mechanical properties. It further contributes about one third in the hydrogen bonding direction ([2 0 0] in Iβ, [1 1 0] in Iα, [0 2 0, 1 1 0] in II, and [1  − 1 0, 1 1 0] in IIII, respectively), and 5 to 17% in the chain direction. These findings emphasize that dispersion energy contributes significantly to the mechanical properties of cellulose, and they also indicate that the contribution from dispersion energy exceeds that of hydrogen bonding during the initial step of defibrillation or dissolution of cellulose.