1 Introduction

Phenomenological models have played a key role in the history of astronomy. Keplers’ laws were a way of organizing the observations of planetary orbits into an understandable pattern and they guided the discovery of Newton’s theory of gravity. In the XIXth century a similar situation was posed by the report in 1859 by Urbain Le Verrier of an anomalous advance of Mercury’s perihelion (Roseveare 1982). This spurred a lot of research into the problem and the suggestion of many unconventional models of gravity, based upon electromagnetism, to explain away the phenomenon as well as the claim of the existence of a new planet closer to the Sun than Mercury as responsible for the extra perturbation. It is a landmark in the history of physics and astronomy that the search for an explanation of this phenomenon finally lead to the formulation of the General Theory of Relativity in 1915 that it is our current accepted paradigm for understanding gravity. For some recent overviews on the present status of General Relativity and future perspectives see Iorio (2015b), Debono and Smoot (2016), Vishwakarma (2016). In relation to the accuracy of the tests of General Relativity we should mention that there have been a recent polemic concerning some possible astrometric anomalies (Anderson and Nieto 2010; Iorio 2015a). In this paper we will develop a model aimed at a possible unified explanation of some of these anomalies that we will briefly discuss below.

Since the sixties of the past century there are new techniques for measuring the distances among the planets that were not available at the times of Le Verrier or Einstein. After the invention of radar in World War II there appeared applications to astronomy with the early radar detection of the Moon in 1946 (Bay 1946; Mofensen 1946). This technique was refined throughout the years and it was commonly used in spacecraft missions and in planetary radar systems (Downs and Reichley 1975; Murphy 2008). This way a total dataset of 7500 ranging distances measurements was collected from the period from 1961 to 2003 only by the Jet Propulsion Laboratory (JPL). This dataset was carefully analyzed by Krasinsky and Brumberg (2004) in 2004 and they found the surprising result that the Astronomical Unit (AU), that was thought to be a constant, was increasing as an average rate of \(15\pm 4\) meters per century. Far from being a consequence of the selection of the data, this result was supported by individual analyses of the data for landers, orbiters and ranging or combinations of them but with different (positive) values for the secular trend. In connection with these early analyses, we should mention that after 2012 the AU has been fixed as a defining constant, as per the resolution of the International Astronomical Union (2012). For this reason, the discussion has been oriented towards possible secular trends in the semi-major axes of the planets (Iorio 2019).

In their work, Krasinsky and Brumberg considered and dismissed the effect of the expansion of the Universe on the local dynamics of the Solar system as a possible explanation, as Arakida made later on with similar conclusions (Arakida 2011, 2012). Another possible non-standard model implies the decrease of the gravitational constant with the rate \(\dot{G}/G\simeq -2 \times 10^{-12}\) per year (Krasinsky and Brumberg 2004; Bel 2014). However, the most recent constraint on the cosmic-time variation of \(G\) obtained from helioseismology clearly rules out that negative rate at 4-\(\sigma \) level (Bonanno and Fröhlich 2017). Models of modified gravity have been studied by Iorio (2005) and Li and Chang (2011) in connection with the problem of the secular increase of the AU. Another conventional explanation is provided by Miura et al. (2009) who suggest that tidal recession is the main cause of the phenomenon but without an adequate model of the tidal effects of the Sun’s photosphere we cannot assess the exact contribution of this effect. As said before, in 2012 the International Astronomical Union resolved that the AU is a constant of value \(149597870.7\text{ km}\) (International Astronomical Union 2012) but, of course, this do not solve the problem but it simply displaces it to another parameter, such as Gauss’ constant. Later analysis by Standish gave the value of \(7\pm 2\) meters for century for the secular increase of the AU (Standish 2005). Upper bounds on the errors of the anomalous variability of the Solar System planetary elements have been deduced by Iorio (2019) on the basis of EPM2017 ephemeris.

The first laser echoes from the Moon were retrieved in 1962 by a team at the Massachusetts Institute of Technology (Smullin and Fiocco 1962). Subsequently, the Apollo and Lunokhod missions installed a set of retroreflectors on the Moon’s surface that improved the accuracy of the Lunar Laser Ranging (LLR) experiment and allowed for a monitoring of the distance among the Earth and the Moon for a period of more than forty years (Dickey et al. 1994; Murphy et al. 2011). This experiment has allowed to set limits on the secular variation of the gravitational constant and it has also establish the rate of increase of the semi-major axis of the system in \(3.8\text{ cm}\) per year as the consequence of tidal friction (Williams et al. 2001, 2014). The models of the Lunar and Earth interiors and tidal dissipative processes are currently sufficiently developed to explain this phenomena but there is still a small secular trend in the eccentricity of the Earth-Moon system that cannot be deduced from the present status of these models. By analyzing 38 years of LLR data, Williams et al. (2001) found that the residual anomalous increase of the eccentricity of the orbit of the Moon is estimated in \((9 \pm 3)\times 10^{-12}\) and, consequently, it is significant at 2-\(\sigma \) level. Improved modeling and further data analysis has reduced this value to \((5 \pm 2)\times 10^{-12}\) but it still remains significant (Williams and Boggs 2016).

Iorio considered a possible explanation of this secular drift in the Moon’s orbital eccentrity by studying a scenario in which a trans-Plutonian massive object could perturb the orbit (Iorio 2011b). Nevertheless, the mass and distance relation for such hypothetical planet would have been unrealistic and this idea is now dismissed. Iorio (2011a) also proposed an acceleration of the form:

$$ \mathbf{A}_{\mathrm{pert}}= k H_{0} \dot{r} \hat{\mathbf{r}}, $$
(1)

where \(k\) and \(H_{0}\) are constants and \(\dot{r}\) is the radial velocity of the Moon with respect to the Earth or a given planet with respect to the Sun. With \(H_{0}\) of the order of the Hubble’s parameter and \(k\) in the range \(2.5 \lesssim k \lesssim 5\) both the anomalous increase of the Astronomical Unit and the secular drift in the Moon orbit’s eccentricity are obtained. The numerical coincidence of the prefactor with the Hubble’s constant may suggest a cosmological origin of these effects but it is well-known that any cosmological effects are smaller by ten orders of magnitude (Krasinsky and Brumberg 2004; Arakida 2011). Acedo suggested that this numerical coincidence may be attributed to the characteristics of the Solar system masses and distance scales (Acedo 2013).

A third anomaly that it is currently being discussed was found by Anderson and collaborators in their study of the spacecraft flybys of the Earth in their course to their destinations in the Solar system (Anderson et al. 2008). This phenomenon is characterized by an anomalous energy variation when these spacecraft flybys the Earth very closely with apogees at altitudes ranging 300 to \(3000\text{ km}\). Although the velocity variations are in the range of a few mm per second, they are still significant and they are unexplained in the context of standard physics (Lämmerzahl et al. 2008; Iorio 2009, 2014; Rievers and Lämmerzahl 2011; Atchison and Peck 2010; Hackmann and Laemmerzahl 2010).

Some models beyond standard physics have also been proposed (Adler 2010, 2011; Nyambuya 2008; Lewis 2009; Hafele 2009; Acedo 2014b; Varieschi 2014; Pinheiro 2014; Acedo 2015; Wilhelm and Dwivedi 2015; Pinheiro 2016; Bertolami et al. 2016) but none of them is still accepted as an explanation. A intriguing possibility not contemplated in any of these papers is that the flyby anomaly could be connected with both the anomalous increase of the astronomical unit and the residual increase in the Moon orbit’s eccentricity. In this work we propose an extra force of the form:

$$ \mathbf{F} = \kappa g_{0} e^{- \frac{r}{\beta R}} S(\theta ) \frac{\dot{r}}{c} \hat{\mathbf{r}}, $$
(2)

where \(g_{0}\) is the surface gravity of the source body, \(R\) is the corresponding radius, \(c\) is the speed of light in vacuum and \(\dot{r}\) is the radial velocity of the test body. The parameters \(\kappa \) and \(\beta \) are assumed to be constants of the order of unity and \(S(\theta )\) is a function of the colatitude that verifies the condition \(S(\theta =\pi /2)=1\). In the spirit of some proposals for a fifth force of nature, the expression in Eq. (2) corresponds to an exponential decay with the radial distance to the center of the source. Anyway, we will not assume that this force couples to the baryon number or isospin as the original proponents did (Franklin and Fischback 2016). It could also be an aspect of modified gravity sourced only by mass-energy.

The reason for this particular proposal is also substantiated in some recent research concerning the fitting of flyby orbits to orbital models. The study of possible anomalous accelerations arising from the discrepancy between orbital models and the best fits of Doppler data suggests the existence of an anomalous radial acceleration proportional to the radial velocity, \(\dot{r}\) (Acedo 2017). Together with the expected exponential decay for a fifth force, and a latitude dependence, we obtain the proposal in Eq. (2). The parameter \(\beta \) is, then, related to the length scale of the fifth force in terms of the Earth’s radius. On the other hand, \(\kappa g_{0}\) is the magnitude of the anomalous acceleration at the celestial equator (when multiplied by the radial velocity measured in terms of the speed of light in vacuum).

Notice also that, depending on the location of the perigee, the magnitude of the anomalous acceleration predicted by Eq. (2) may be different before and after the perigee. This is the case for any orbit not located at the equator and it leads, naturally, to an asymmetry between the prograde and retrograde orbital motions. This effect has been suggested in connection with the analysis of Doppler data related to the flyby anomaly.

The objective of this paper is to show that, with an adequate choice of parameters, the extra force in Eq. (2) could explain the anomalous increase of the AU, the anomalous increase of the eccentricity of the Moon orbit and the flyby anomaly (at least, in sign and order of magnitude). Moreover, we will also show that the contribution of the extra force to the precession of the perihelion of Mercury is negligible and it does not ruin the excellent agreement with standard General Relativity. The perturbations induced by this force in the geodynamics satellites, such as LAGEOS, are also studied and we conclude that they could be compatible with observations.

The paper is organized as follows: in Sect. 2 we calculate the perturbations induced by our fifth force proposal in the semi-major axes of planets and the eccentricity of the Moon’s orbit in order to estimate the parameters \(\kappa \) and \(\beta \). Section 3 is devoted to the calculations of the anomalous variations of spacecraft velocities in several flybys of the Earth. We analyze the advance of the perihelion of Mercury, the orbital decay of the LAGEOS spacecraft in Sect. 4. The paper ends with some conclusions and prospects for future work in Sect. 5.

2 Perturbations of the planetary and Moon orbits

We firstly consider a planet in orbit around the Sun. For an exclusively radial perturbation force, we have that the semi-major axis, \(a\), evolves according to (Burns 1976):

$$ \displaystyle \frac{d a}{d t}=2 \displaystyle \frac{a^{2}}{\mu } \dot{r} \mathcal{R} , $$
(3)

where \(\mu = G M_{\odot }\) is the mass constant for the Sun, \(\dot{r}\) is the radial velocity and ℛ is the radial component of the force. If we denote by \(\nu \) the true anomaly, i.e., the angle among the radius vector and the vector locating the position of the periapsis, we also have:

$$ r= \displaystyle \frac{a (1-\epsilon ^{2})}{1+ \epsilon \cos \nu }, $$
(4)

where \(\epsilon \) is the orbital eccentricity. The orbital velocity can also be written as follows (Burns 1976):

$$ \dot{r}= \sqrt{ \displaystyle \frac{\mu }{a}} \displaystyle \frac{\epsilon }{(1-\epsilon ^{2})^{1/2}} \sin \nu . $$
(5)

Another useful relation gives the differential of time in terms of the differential of the true anomaly:

$$ d t= \displaystyle \frac{P}{2 \pi } \displaystyle \frac{(1-\epsilon ^{2})^{3/2}}{(1+\epsilon \cos \nu )^{2}} d \nu , $$
(6)

\(P\) being the orbital period, which can be expressed in terms of the semi-major axis and the mass constant of the central body by means of Kepler’s third law: \(P= 2 \pi a^{3/2} \mu ^{-1/2}\). By taking into account Eqs. (2)–(6) and performing some simplifications we finally arrive at:

$$\begin{aligned} \displaystyle \frac{d a}{a} =& \displaystyle \frac{2 \kappa g_{0} P}{c} \epsilon ^{2} \bigl(1-\epsilon ^{2}\bigr)^{1/2} e ^{-\displaystyle \frac{a (1-\epsilon ^{2})}{\beta R (1+\epsilon \cos \nu )}} \\ &{}\times \displaystyle \frac{\sin ^{2} \nu }{(1+\epsilon \cos \nu )^{2}} d \nu . \end{aligned}$$
(7)

Here we have ignored the inclination of the axis of the Sun with respect to the orbital plane of the planet so we take \(\theta =\pi /2\) in Eq. (2). In order to obtain secular trends it is customary in perturbation theory to perform an orbital average. To do so, the following integral it is useful in this case:

$$ \begin{aligned}[b] I(u,\epsilon )&= \displaystyle \frac{1}{2 \pi } \displaystyle \int _{0}^{2 \pi } e^{-\displaystyle \frac{u}{1+\epsilon \cos \nu }} \displaystyle \frac{\sin ^{2} \nu }{ ( 1 + \epsilon \cos \nu )^{2}} \, d \nu \\ &= \displaystyle \frac{ e^{-u}}{u \epsilon } \displaystyle \int _{0}^{2 \pi } d \nu \cos \nu e^{u \epsilon \cos \nu } + \mathcal{O}\bigl(\epsilon ^{2}\bigr) \\ &= \displaystyle \frac{e^{-u}}{u \epsilon } I_{1}(u\epsilon )+ \mathcal{O}\bigl(\epsilon ^{2}\bigr), \end{aligned} $$
(8)

where we have used integration by parts and \(I_{1}(x)\) is the modified Bessel function of the first kind (Abramowitz and Stegun 1968). The integral representation of \(I_{1}(x)\) appears as a consequence of the approximation to first order in the eccentricity, \(\epsilon \). This is justified for most elliptical orbits in the Solar system.

From Eqs. (7) and (8) we obtain the variation in an orbital period as follows:

$$ \Delta a= \displaystyle \frac{2 \kappa g_{0} P}{c} \beta R e^{-\displaystyle \frac{a}{ \beta R}} I_{1} \biggl( \displaystyle \frac{a \epsilon }{\beta R} \biggr)+\mathcal{O}\bigl(\epsilon ^{2}\bigr), $$
(9)

and the variation in one terrestrial year can be simply be obtained by replacing \(P\) by the corresponding time span of one year.

The equation for the disturbance of the eccentricity of the elliptical orbit by the effect of a radial perturbation force is (Pollard 1966):

$$ \displaystyle \frac{d \epsilon }{d t}= \biggl( \displaystyle \frac{a (1-\epsilon ^{2})}{\mu } \biggr)^{1/2} \mathcal{R} \sin \nu . $$
(10)

By using again the Eqs. (4)–(6) and the expression of the extra force in Eq. (2), and performing an orbital average, we arrive at an expression involving the same definite integral found in the previous case:

$$ d \epsilon = \displaystyle \frac{\kappa g_{0} P}{ 2 \pi c} \epsilon \bigl(1-\epsilon ^{2}\bigr)^{3/2} I \biggl( \displaystyle \frac{a(1-\epsilon ^{2})}{\beta R},\epsilon \biggr) . $$
(11)

Performing the integral according to Eq. (8) yields:

$$ \Delta \epsilon = \displaystyle \frac{\kappa g_{0} P}{c} \displaystyle \frac{\beta R}{a} e^{-\displaystyle \frac{a}{\beta R}} I_{1} \biggl( \displaystyle \frac{a \epsilon }{\beta R} \biggr)+\mathcal{O}\bigl(\epsilon ^{2}\bigr) . $$
(12)

Now we will estimate the non-dimensional parameters, \(\kappa \) and \(\beta \), from the measured anomalies. According to Eq. (9) the effect on the secular variation of the semi-major axis would be larger for Mercury than for any other planet in the Solar system because it is closer to the Sun and its orbit has the largest eccentricity among the inner planets. The constants needed for the calculation are the surface gravity of the Sun: \(g_{0}=274\text{ m}/\text{s}^{2}\), the Sun’s radius \(R=695700\text{ km}\), the semi-major axis of Mercury’s orbit, \(a=57.91\times 10^{6}\text{ km}\), its orbital eccentricity, \(\epsilon =0.2056\), and the speed of light in vacuum, \(c=299792.458\text{ km}/\text{s}\). Following Iorio (Iorio 2019) we should use the most recent upper bounds on the secular rate of the semi-major axes of the planetary orbits in the Solar system. These upper bounds were obtained from the EPM2017 ephemerides. From Eq. (9) and parameters of order of unity we obtain results for Mercury in agreement with the upper bounds given by Iorio (2019). For example, by taking \(\kappa =1\) and \(\beta =1.98\) we obtain a secular rate for the semi-major axis of Mercury of 0.0028 meters per century, in comparison with the upper bound of 0.003 m/cty given by Iorio (2019). On the other hand, for Venus we get \(d a/dt \simeq 1.14 \times 10^{-24}\mbox{ m}/\mbox{cty}\) to be compared with the bound of 0.092 m/cty obtained from EPM2017 ephemerides. For the rest of the planets, even smaller rates are predicted, as a consequence of the exponential decay of the extra force in Eq. (2). Similarly, we can also predict the extra rate for the secular change of the orbital eccentricity. Once again, in the case of Mercury we get the larger value of \(d \epsilon /dt \simeq 1.14 \times 10^{-13}\) that it is, indeed, negligible in comparison with the upper bound of 0.0006 per century (Iorio 2019). For other planets the predictions are even smaller indicating that the contribution of the extra force to the rate of change of the orbital eccentricity would require laser ranging precision and a very accurate model of orbital perturbations to be disentangled from classical effects.

We proceed in the same way for the anomalous increase of the eccentricity of the orbit of the Moon by applying Eq. (12) with \(g_{0}=9.8\mbox{ m}/\mbox{s}^{2}\), \(\epsilon =0.0549\), \(a=384400\mbox{ km}/\mbox{s}\) and \(R=6371\text{ km}\) as the mean radius of the Earth. For a an anomalous variation in the range \(3 \times 10^{-12} < \Delta \epsilon < 7\times 10^{-12}\) per year, as reported by Williams et al., we get \(2.60 < \beta < 2.70\) for \(\kappa =1\) and \(2.36 < \beta < 2.44\) for \(\kappa =10\). It is difficult to optimize this parameter estimation as the observations have still a relatively large uncertainty but we can say that the anomalous force in Eq. (2) is compatible with both anomalies for \(\kappa \) of the order of unity and \(\beta \simeq 2\). In the section we will see to what extent this could be in agreement with the flyby anomalies.

3 Application to the flyby anomalies

In this section we consider the effect of the perturbation in Eq. (2) for a close flyby of the Earth. In a flyby a spacecraft describes an, approximately hyperbolic, orbit around the Earth in which both the Moon and the Sun act as perturbing bodies. The Jacobi’s integral remains constant during the flyby but the total energy in the Solar system’s barycenter frame may increase or decrease depending on the incoming direction of the spacecraft (Anderson et al. 2007). The objective of these manoeuvres is, usually, to gain energy in order to reach the outer Solar system. Doppler tracking using the Deep Space Network allows for a careful monitoring of the trajectories and the subsequent analysis by means of the most up-to-date ephemeris models. This thorough study allowed for the detection of a small discrepancy among theory and observations in the first Galileo flyby of the Earth that took place on December, 8th, 1990. In that event the post-encounter Doppler residuals exhibit an anomalous difference with respect to the pre-encounter ones to be interpreted as an increase of velocity around \(3.92\text{ mm}\) per second (Anderson et al. 2008; Anderson and Nieto 2010). Although this may seem a small quantity, it is significant within the accuracy of the ephemeris models. The largest discrepancy was found in the NEAR flyby of January, 23th, 1998 corresponding to \(13.36\text{ mm}\) per second. In other cases the difference has been negative, such as the second Galileo flyby of the Earth in 1992.

The objective of this section is to evaluate the effect of the extra force in Eq. (2) on a flyby. To do so, we begin with the equations of motion for the perturbation on the position, \(\delta \mathbf{r}\), and velocity, \(\delta \mathbf{v}\), of the spacecraft written as follows (Acedo 2017):

$$\begin{aligned} \displaystyle \frac{d \delta \mathbf{r}}{d \tau } =& \delta \mathbf{v} , \end{aligned}$$
(13)
$$\begin{aligned} \displaystyle \frac{d \delta \mathbf{v}}{d \tau } =& - \displaystyle \frac{ \delta \mathbf{r}}{D^{3}}+ 3 \mathbf{D} \cdot \delta \mathbf{r} \displaystyle \frac{\mathbf{D}}{D^{5}} + \mathbf{\mathcal{F}}_{\mathrm{extra}}, \end{aligned}$$
(14)

where \(\delta \mathbf{r}\) is measured in units of the semi-major axis, \(\vert a \vert \), \(\tau =t/P\), \(P\) being a characteristic time obtained from Kepler’s third law, \(P=2 \pi a^{3/2} \mu ^{-1/2}\) and \(\delta \mathbf{v}\) is scaled with the velocity \(\vert a \vert /P\). The second term on the right-hand side of Eq. (14) arises from the tidal forces exerted as a consequence of the deviation of the trajectory from the ideal hyperbolic orbit. The scaled radius vector for the ideal Keplerian orbit is given by:

$$ \mathbf{D}= (\epsilon -\cosh u) \hat{\mathbf{s}}+\sqrt{ \epsilon ^{2}-1} \sinh u \hat{\mathbf{n}} , $$
(15)

where \(\epsilon > 1\) is the orbital eccentricity and \(u\) is the eccentric anomaly whose relation with time is as follows:

$$ \tau = \epsilon \sinh u - u . $$
(16)

In Eq. (15) the unit vector \(\hat{\mathbf{s}}\) is directed from the center of the Earth towards the spacecraft perigee, and \(\hat{\mathbf{n}}\) is a unit vector in the osculating orbital plane at perigee, perpendicular to \(\hat{\mathbf{s}}\) and directed opposite to the incoming direction of the spacecraft. The scaled extra force is given as:

$$ \mathbf{\mathcal{F}}_{\mathrm{extra}}=\kappa \displaystyle \frac{g_{0} P}{c} e^{-\vert a \vert (\epsilon \cosh u -1)/(\beta R)} S(\theta ) \displaystyle \frac{\epsilon \sinh u}{\epsilon \cosh u -1} \hat{\mathbf{r}}, $$
(17)

where the scaled radial velocity has been calculated by noticing that \(\vert \mathbf{D} \vert =\epsilon \cosh u -1\) and Eq. (16) so:

$$ \displaystyle \frac{d r}{d \tau }= \displaystyle \frac{\epsilon \sinh u}{\epsilon \cosh u -1} , $$
(18)

and the unit radial vector is \(\hat{\mathbf{r}}=\mathbf{D}/(\epsilon \cosh u -1)\). The perturbing force in Eq. (17) is evaluated at the ideal Keplerian orbit, so we ignore second-order contributions to the position and velocity perturbations. We still have to define the function \(S(\theta )\) of the colatitude, \(\theta \). We will see that a reasonable agreement with the flyby anomalies is obtained is \(S(\theta )\) is defined as a linear combination of \(\sin \theta \) and \(\cos \theta \) as follows:

$$ S(\theta )=\sin \theta + \chi \cos \theta , $$
(19)

where \(\chi \) is a constant parameter to be estimated.

Finally we will need an expression for \(\sin \theta \) and \(\cos \theta \) in terms of the eccentric anomaly. Approximate values can be obtained from the ideal Keplerian radius vector in Eq. (15) in the following form:

$$ \cos \theta = \displaystyle \frac{ ( (\cosh u - \epsilon ) \hat{\mathbf{s}} \cdot \hat{\mathbf{k}}-\sqrt{\epsilon ^{2}-1} \sinh u \hat{\mathbf{n}}\cdot \hat{\mathbf{k}} )}{\epsilon \cosh u-1} , $$
(20)

with \(\sin \theta \) obtained from this equation, taking into account that it should be positive because \(0 \le \theta \le \pi \).

Now we are prepared to perform the integration of the equations of motion in Eq. (13)–(14) for particular conditions. The data for the osculating orbit at perigee in the NEAR flyby was given by Anderson et al. and other references: semi-major axis, \(a=-8494.87\), eccentricity, \(\epsilon =1.8135\), colatitude of the incoming direction in sexagesimal degrees, \(\theta _{\mathrm{in}}=69.24^{ \circ }\), colatitude of the perigee, \(\theta _{\mathrm{p}}=57.0^{\circ }\), colatitude of the outgoing direction, \(\theta _{\mathrm{out}}=161.96^{ \circ }\), orbital inclination, \(\iota =108.0^{\circ }\), right ascension of the incoming direction, \(\alpha _{\mathrm{in}}=81.17^{\circ }\) and right ascension of the perigee, \(\alpha _{\mathrm{p}}=280.43^{\circ }\). From these parameters we can also calculate the unit vectors of the orbital reference frame in terms of the unit vectors along the axes of the equatorial frame:

$$\begin{aligned} \hat{\mathbf{s}} =&0.151772 \hat{\imath }-0.824823 \hat{\jmath }+0.544639 \hat{k} , \end{aligned}$$
(21)
$$\begin{aligned} \hat{\mathbf{n}} =&-0.27079 \hat{\imath }-0.56464 \hat{\jmath }-0.779665 \hat{k}, \end{aligned}$$
(22)
$$\begin{aligned} \hat{\mathbf{w}} =&0.95061 \hat{\imath }-0.0291293 \hat{\jmath }-0.309017 \hat{k} , \end{aligned}$$
(23)

where \(\hat{\imath }\) points towards the first point of Aries and \(\hat{k}\) is directed along the Earth’s rotation axis. Notice also that here \(\hat{\mathbf{w}}\) denotes the inclination vector.

It is convenient to define the deviation of the velocity modulus from the keplerian value:

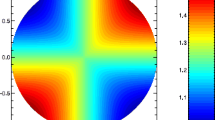
$$ \delta V(t)= \sqrt{ ( \mathbf{v}+\delta \mathbf{v} )^{2}- \mathbf{v}^{2}}, $$
(24)

where \(\mathbf{v}\) denotes the ideal keplerian value of the velocity vector. In Fig. 1 we have plotted the results of the simulation for \(\kappa =6.0\), \(\beta =0.235\) and \(\chi =5.0\). These values were chosen to obtain a good fit for the NEAR and Galileo II flybys.

Fig. 1
figure 1

Variation of the spacecraft velocity as a consequence of the perturbing effect of the extra force in Eq. (2). The vertical axis gives the velocity change in mm per second. The horizontal axis is the time in hours from the perigee. The solid line corresponds to the NEAR flyby and the dashed line to the second flyby of the Galileo spacecraft

Full size image

With these parameters values the net variation from the pre-encounter trajectory to the post-encounter one (around 15 hours before perigee and after perigee) is \(10.63\mbox{ mm}/\mbox{s}\) for NEAR and \(-4.78\mbox{ mm}/\mbox{s}\) for Galileo II (to be compared with the \(13.46\mbox{ mm}/\mbox{s}\) and \(-4.6\mbox{ mm}/\mbox{s}\) reported by Anderson et al.). By using the orbital parameters for the Galileo I and Cassini flybys we obtain a total anomalous variation of \(6.36\mbox{ mm}/\mbox{s}\) and \(-1.15\mbox{ mm}/\mbox{s}\) in comparison with the values of \(3.92\mbox{ mm}/\mbox{s}\) and \(-2.0\mbox{ mm}/\mbox{s}\) of Anderson et al. (2008). We conclude that with the force model in Eq. (2), and adequate parameters, we obtain similar values to the observed anomalies both in sign and order of magnitude. On the other hand, to obtain a reasonable agreement we must chose a characteristic length scale, \(\xi =\beta R\), smaller than 25 per cent the Earth radius. Larger values tend to overshoot the predictions of the anomalous velocity change. This means that a model as simple as the one in Eq. (2) cannot predict, simultaneously, the anomalies of the Moon and the AU and the flyby anomalies if we consider \(\beta \) as a constant. Alternatively, we could define an effective parameter \(\beta (r)\) interpolating between the values of \(\beta (r) \simeq 0.23\) for small \(r\) and \(\beta (r) \simeq 2\) for \(r \gg R\). We will discuss further this possibility on the last section.

4 The perihelion of Mercury and the LAGEOS spacecraft

A radial perturbing force, ℛ, induces a precession of the perihelion of the orbit of a planet at a instantaneous rate given by (Danby 1988; Pollard 1966):

$$ \dot{\omega }=-\sqrt{ \displaystyle \frac{a(1-\epsilon ^{2})}{\mu }} \displaystyle \frac{1}{\epsilon } \cos \nu \mathcal{R} , $$
(25)

where \(\nu \) is the true anomaly, \(a\) is the semi-major axis, \(\epsilon \) is the eccentricity and \(\mu \) is the mass constant of the Sun. The radial velocity of the planet satisfies the relation:

$$ \dot{r}= \sqrt{ \displaystyle \frac{\mu }{a(1-\epsilon ^{2})}} \epsilon \sin \nu . $$
(26)

So, it is clear that for an orbit in the equatorial plane of the Sun, \(\theta =\pi /2\), we have from Eqs. (2), (25) and (26) that the orbital average of the precession induced by the extra force is zero (because \(\langle \sin \nu \cos \nu \rangle =0\)).

However, the equatorial plane of the Sun does not coincide with the orbital planes of the planets. In particular, the orientation of the axis of the Sun with respect to the ecliptic plane is given by the Carrington’s elements (Giles 1999):

$$\begin{aligned} \iota _{\mathrm{C}} =& 7.25^{\circ } \end{aligned}$$
(27)
$$\begin{aligned} \varOmega _{\mathrm{C}} =& 73.67^{\circ }+0.013958^{ \circ } (t-1850) , \end{aligned}$$
(28)

where \(\iota _{\mathrm{C}}\) is the inclination of the Sun’s equatorial plane (in sexagesimal degrees) and \(\varOmega _{\mathrm{C}}\) is the right ascension of the intersection line with the ecliptic plane (with \(t\) being the year of observation). Similarly, the orbital plane of the planet is defined in terms of a pair of angles \((\iota , \varOmega )\) form which we can obtain the unit vectors of a convenient orthonormal reference frame:

$$\begin{aligned} \hat{\mathbf{n}}_{1} =&\cos \varOmega \hat{ \imath }+\sin \varOmega \hat{\jmath } , \end{aligned}$$
(29)
$$\begin{aligned} \hat{\mathbf{n}}_{2} =&-\cos \iota \sin \varOmega \hat{\imath }+ \cos \iota \cos \varOmega \hat{\jmath }+\sin \iota \hat{k}, \end{aligned}$$
(30)
$$\begin{aligned} \hat{\mathbf{n}}_{3} =&\sin \iota \sin \varOmega \hat{\imath }-\sin \iota \cos \varOmega \hat{\jmath }+ \cos \iota \hat{k} . \end{aligned}$$
(31)

Now we denote by \(\hat{\mathbf{m}}_{i}\), \(i=1\), 2, 3 the vectors obtained with Eqs. (29)–(31) and the Carrington’s elements in Eq. (27). The change of basis matrix from the equatorial plane of the Sun to the orbital plane of a planet is then given by \(\alpha _{ij}=\hat{\mathbf{n}}_{i}\cdot \hat{\mathbf{m}}_{j}\), \(i,j=1\), 2, 3. Using this change of basis, we can prove that the colatitude of the planet with respect to the Sun verifies the identity (Acedo 2014a):

$$ \cos \theta =\alpha _{13} \cos (\nu +\omega )+ \alpha _{23} \sin (\nu +\omega ), $$
(32)

where \(\omega \) is the argument of the perihelion and \(\nu \) is the true anomaly (measured from the perihelion). As the orbits of the planets lie close to the equatorial plane of the Sun we have that the coefficients \(\alpha _{13}\), \(\alpha _{23}\) are small and we can also obtain the approximation:

$$ \begin{aligned}[b] \sin \theta &\simeq 1-\alpha _{13} \alpha _{23} \cos (2 \omega ) \sin \nu \cos \nu \\ &\quad{} + \displaystyle \frac{1}{2} \bigl( \alpha _{13}^{2}-\alpha _{23}^{2} \bigr) \sin (2 \omega ) \sin \nu \cos \nu . \end{aligned} $$
(33)

From Eqs. (2), (19), (25), (32) and (33) we get, after some simplifications, the average precession per orbit induced by the extra force:

$$ \begin{aligned}[b] \Delta \omega &= -\kappa \displaystyle \frac{g_{0} P}{8 c} e^{-a/(\beta R)} \\ &\quad \biggl\{ -\alpha _{13}\alpha _{23} \cos (2 \omega ) + \displaystyle \frac{1}{2} \bigl(\alpha _{13}^{2}- \alpha _{23}^{2} \bigr) \sin (2 \omega ) \biggr\} \\ &\quad {}+\mathcal{O}(\epsilon ), \end{aligned} $$
(34)

where \(P\) is the orbital period of the planet, \(g_{0}\) is the surface gravity of the Sun and \(R\) its radius. This precession would be larger for Mercury than for any other planet because of the exponential term. The relevant parameters for Mercury’s orbit (NASA 2019) are: \(a=57909100\text{ km}\), \(\iota =7.005^{\circ }\), \(\varOmega =48.331^{\circ }\) and \(\omega =29.124^{\circ }\). By using the parameters estimations of Sect. 2 we find a contribution to the advance of the perihelion \(\Delta \omega =-1.013\times 10^{-10}\) mas per century. This variation is completely negligible in comparison with the well-known value of the relativistic precession and, consequently, such an effect of the extra force in Eq. (2) would be out of the reach of any measurement technique in the foreseeable future. In particular, from EPM2017, Iorio finds the upper bound of \(0.008\mbox{ mas}/\mbox{cty}\) for Mercury, \(0.315\mbox{ mas}/\mbox{cty}\) for Venus and \(0.033\mbox{ mas}/\mbox{cty}\) for the Earth. The predictions of Eq. (34) with \(\kappa =1\) and \(\beta =1.98\) are \(6.26 \times 10^{-27}\mbox{ mas}/\mbox{cty}\) for Venus and \(3.28 \times 10^{-39}\mbox{ mas}/\mbox{cty}\) for the Earth.

4.1 Effects on the orbit of the LAGEOS spacecraft

LAGEOS is a geodynamic satellite launched on May, 1976 and it constitutes one of the most successful spacecraft missions in terms of the amount of data retrieved and duration. This satellite takes the form of a sphere covered with 422 retroreflectors specially designed for laser ranging. The LAGEOS I and LAGEOS II spacecraft, as well as LARES, are currently used, among other things, for the analysis of some problems in fundamental physics such as the detection of the Lense-Thirring effect (Iorio 2017; Renzetti 2013; Iorio et al. 2011).

A few years after the beginning of the LAGEOS mission it was clear that this satellite was experiencing an orbit shrinking phenomenon at a rate of \(1.1\text{ mm}\) per day. Moreover, this shrinking rate is not constant but it fluctuates with time with a scale of several years. It is important to take into account the actual experimental uncertainties for this decay rate that it is around \(0.03\mbox{--}0.1\) meters per year (Pardini et al. 2017; Sośnica et al. 2014; Rubincam 1982; Sosnica 2014; Iorio 2018). Many studies have been devoted to this orbital decay (Rubincam 1980, 1988; Mignard et al. 1990; Rubincam 1990, 1993; Rubincam and Mallama 1995; Martin and Rubincam 1996; Rubincam et al. 1997) but there still remains the unexplained fluctuating behavior of the along-track acceleration (Rubincam 1990; Mignard et al. 1990). The general agreement is that the average orbital decay originates through the combination of three mechanisms: (i) the neutral particle drag which constitutes a 14% of the total drag, (ii) the charged particle drag by protons in the plasmasphere (around 16% of the total drag if we assume a potential of \(-1\mbox{ V}\) for the spacecraft), (iii) the Yarkovsky thermal drag caused by the differential heating of the hemispheres of the LAGEOS. As the spacecraft rotates, and because of thermal inertia, we would have a larger radiation pressure on one of the sides of the sphere and this would cause an along-track acceleration with an estimated value of a 70% of the total (Rubincam 1988, 1990).

LAGEOS’s orbit has an inclination of \(\iota =109.84^{\circ }\) and a semi-major axis, \(a=12272.57\text{ km}\). The orbit is almost circular with an eccentricity \(\epsilon =0.0045\). To obtain the colatitude of the spacecraft with respect to the equatorial plane of the Earth we can use the same arguments as those of the previous section and from Eq. (32) we have:

$$ \cos \theta =\sin \iota \sin (\nu +\omega ) . $$
(35)

From Eqs. (3) and (2), and using also Eq. (35), we can derive an approximate expression for the secular variation of the semi-major axis of the spacecraft as a consequence of the extra force that we have proposed:

$$ \begin{aligned}[b] \biggl\langle \displaystyle \frac{d a}{d t} \biggr\rangle &= 2 \kappa a \epsilon ^{2} \displaystyle \frac{g_{0}}{c} e^{-a/(\beta R)} \\ &\quad \bigl\langle \sin ^{2}\nu \sqrt{1-\sin ^{2} \iota \sin ^{2} (\nu +\omega )} \bigr\rangle + \mathcal{O}\bigl(\epsilon ^{3}\bigr), \end{aligned} $$
(36)

where \(\langle \dots \rangle \) denotes the orbital average. We must also take into account that the argument of the perigee is not a constant because it is perturbed by the Earth’s oblateness, \(J_{2}\), with a rate (Capderou 2005):

$$ \dot{\omega }= \displaystyle \frac{3\pi }{2 (1-\epsilon ^{2} )} \displaystyle \frac{J_{2}}{P} \biggl( \displaystyle \frac{R}{a} \biggr)^{2} \bigl(5 \cos ^{2} \iota -1 \bigr), $$
(37)

where \(J_{2} \simeq 1.0826 \times 10^{-3}\), \(R=6378.1363\text{ km}\) is the Earth’s reference radius and \(P=225\) minutes is the orbital period of LAGEOS. This precession can be also deduced from NORAD two-line elements sets (Kelso 2018), so we can write:

$$ \omega (t)=256.139^{\circ }-0.21427^{\circ } t, $$
(38)

where \(t\) is measured in days since May, 1st, 1976 and \(\omega \) is given in sexagesimal degrees. By using Eqs. (36) and (38) we can calculate a positive contribution to the rate of variation of the semi-major axis of the LAGEOS spacecraft as a consequence of the extra force. If we assume that the average orbital decay is around \(-1.77\text{ mm}\) per day instead of the usually assumed \(-1.1\pm 0.274\text{ mm}\) per day and we consider the orbital expansion predicted by Eq. (36) the result in Fig. 2 is obtained. In this figure we compare the prediction of the standard model for LAGEOS’s orbital decay (Rubincam 1990) including the extra effect of the anomalous radial force in Eq. (2) with the observed decay deduced from the along-track delay. We see that some amount of variability could be explained with this extra force. The value of \(-1.77\mbox{ mm}/\mbox{day}\), for the average orbital decay, can be obtained if the potential of the spacecraft is −3.75 Volts instead of −1 Volts as usually assumed (Rubincam 1990). This is not a disproportionate value for the spacecraft’s potential as far more larger values can be attained by spacecraft’s charging in the plasmasphere (Davis and Duncan 1992). The observed decay, which, on the other hand, is within \(3\sigma \) of our prediction, might be the result of a balance between the orbital expansion predicted by Eq. (9) and charged drag. The lesson is that we cannot exclude the extra force in Eq. (2) on the basis of the monitoring of the orbit of the LAGEOS spacecraft throughout the years and our current understanding of radiation models and the interaction of the plasmasphere with the spacecraft. The situation is more problematic for the LAGEOS II spacecraft that imposes a stringent test on any model of extra forces generated by the Earth. For this spacecraft we have an orbital inclination of \(\iota =52.67^{\circ }\), a semi-major axis of \(a=12158\text{ km}\) and an eccentricity \(\epsilon =0.0137\) (more than three times larger that the orbital eccentricity of LAGEOS) (Lucchesi and Peron 2014). The larger eccentricity implies also a greater expansion rate as predicted from Eq. (9). For the same values of the parameters used in Fig. 2 we have \(d a/d t=9.36\text{ mm}\) per day. If we consider a slightly smaller value for \(\beta \), such as \(\beta =0.17\), the more reasonable prediction of \(d a/d t=0.419\text{ mm}\) per day is obtained. This can be compatible with the observation of the average orbital decay of \(-1.1\pm 0.237\text{ mm}\) per day if we assume that the total decay (and taking into account the uncertainty in the spacecraft charge) could be of \(-1.5\text{ mm}\) per day. The conclusion of this analysis is that a geodynamic spacecraft with a larger eccentricity could be fundamental in disclosing the existence of a putative fifth force of the form proposed in this paper (or other similar proposals) or in, definitively, excluding it, despite of the evidence for the, still unexplained, flyby anomalies.

Fig. 2
figure 2

Decay rate for the LAGEOS spacecraft since its launch vs time in months. Solid line corresponds to a constant decay and the semi-major axis increase predicted by the perturbing force in Eq. (2). Dashed lines are the observed decays inferred from the along-track accelerations. These results were obtained for \(\kappa =6\) and \(\beta =0.235\)

Full size image

5 Conclusions

The advance of physics and astronomy is based upon the interplay of theory and observations. For this reason, it is of paramount importance to test our current theoretical models with the highest accuracy allowed by the present techniques. In this spirit, orbital models have been put to very stringent test since the beginning of the space era with the development of radar and laser ranging techniques as well as the spacecraft missions to other planets in the Solar system (Krasinsky and Brumberg 2004; Williams et al. 2014; Williams and Boggs 2016). This way, further evidence on the validity of the predictions of General Relativity, our basic framework to understand gravity, have been obtained (Will 2014). But in the process some anomalies have also been found, i.e., some observations that do not fit within the current models.

Although there is a possibility that these anomalies may be caused by data reduction problems, or by incomplete models that do not take into account some phenomena related to a well-known theory, they deserve full consideration as they might also be the consequence of some unknown physics. Even in the case that they finally are explained conventionally, we must strive to find an agreement between theory and experiment as this is the fundamental objective of any experimental science. In this paper, we have considered three, apparently, independent observations in the Solar system: (i) the anomalous increase of the length scales in the Solar system (Krasinsky and Brumberg 2004; Standish 2005) (ii) the lingering problem of the anomalous increase of the eccentricity of the orbit of the Moon (Williams and Boggs 2016; Iorio 2011b) (iii) the flyby anomaly (Anderson et al. 2008). Our objective was to develop a model that may suggest a connection among all these anomalous observations.

In this spirit we have revisited the fifth force models but with a different perspective. The original fifth force proposals were developed in the eighties of the past century in the hope of explaining some possible anomalies in the measurement of the acceleration of gravity on municipal scales. However, these short-ranged versions of a fifth force were finally abandoned by the evidence to the contrary (Franklin and Fischback 2016). Our proposal in Eq. (2) is different for several reasons: firstly, it is proportional to the radial velocity of the object with respect to the main body, it also depends on the celestial latitude with respect to the equatorial plane of that main body and the scale is of the same order of the radius of that body.

We have shown that this model can explain both the anomalous increase of the length scale and the eccentricity of the orbit of the Moon for a typical range of the extra force, \(\xi \simeq 2 R\), where \(R\) is the radius of the main body (the Sun in the first case and the Earth in the second one). The increase of the semi-major axis of the orbit of Mercury would be larger than that of any other planet and, consequently, it would dominate the contribution to the first effect. By using the same force model we have also simulated the spacecraft flybys of the NEAR, Cassini and Galileo spacecrafts and we have found that the velocity variations coincide in sign and order of magnitude to those reported by Anderson et al. (2008) if a length scale \(\xi \simeq 0.23 R\) is considered. This means that a short-range fifth force with a single length scale parameter cannot accommodate both the planetary and Moon’s phenomena and the flyby anomaly. An effective length scale for this force depending on the \(r\) coordinate will be necessary.

One of the main criticisms to the proposal of additional forces in the Solar system is that they may ruin the excellent agreement with orbital models including relativistic corrections. For this reason, we have analyzed the contribution of our force proposal to the advance of the perihelion of Mercury and the orbital decay of the LAGEOS’s spacecraft (Rubincam 1990). The result is a negligible contribution to the perihelion advance in the case of Mercury and a positive contribution of a fraction of a millimeter per day orbital expansion. This result cannot be excluded because the LAGEOS’s spacecraft suffers several effects such as charged and uncharged friction or the Yarkovsky effect (some of them not understood in detail) and the orbital decay fluctuates with a typical deviation as large as the average value. Indeed, we have proposed that part of these fluctuations could be attributed to the effect of the putative fifth force.

We have shown that despite the short-ranged fifth force is no longer a viable paradigm in physics and astronomy (Franklin and Fischback 2016) an extra force of the form given in this paper could predict new phenomena in the Solar system without conflicting with the evidence supporting current models and General Relativity, in particular. This extra force could not be assimilated to the standard concept of a fifth force because we have not assumed any particular coupling. On the other hand, if could simply represent the effect of extra degrees of freedom not taken into account in the lagrangian of the standard theory of gravity (Goenner 2004). Further analysis of the radar and laser ranging observations, as well as the flyby anomalies, would be necessary to finally establish the existence of these phenomena and their relations. If such objective in Solar system astronomy is achieved, we will have additional reasons and guidance to look for a modified model of gravity incorporating predictions of these new effects.