A new class of relativistic charged anisotropic super dense star models

Abstract

In the present article we have obtained a class of analytical solutions for an anisotropic charged fluid distribution. The neutral anisotropic fluid sphere has already been obtained by Maurya and Gupta (Phys. Scr. 86:025009, 2012). The solutions depend upon both the anisotropic and the charge parameter. The anisotropy parameter and the electric intensity is zero at the centre and monotonically increasing towards the pressure free interface. All the physical entities such as energy density, radial pressure, tangential pressure, and velocity of sound are monotonically decreasing towards the surface.

1 Introduction

The assumption of local isotropy is a common one in astrophysical studies of massive celestial objects. However, the theoretical investigation of Ruderman (1972) and Canuto (1973) of more realistic stellar models indicates that stellar matter may be anisotropic at least in certain density ranges (ρ<10¹⁵ g cm⁻³). According to them the radial pressure may not be equal to the tangential pressure in such anisotropic massive bodies.

Certainly no astronomical object has a perfect fluid distribution. Therefore it seems worthwhile to study the behaviour of the anisotropic fluid sphere in general relativity. Anisotropy in the pressure could be introduced by the existence of a solid core, by the presence of type-3A superfluid (Kippenhahn and Weigert 1990), different kinds of phase transitions (Sokolov 1980) or by other physical phenomena. On the scale of galaxies, Binney and Tremaine (1987) have considered anisotropies in spherical galaxies, from a purely Newtonian point of view. The mixture of two gases (e.g. monatomic hydrogen or ionised hydrogen and electrons) can also formally be described as an anisotropic fluid (Letelier 1980 and Bayin 1982). Bowers and Liang (1974) have investigated the possible importance of locally anisotropic equations of state for relativistic fluid spheres by generalising the equations of hydrostatic equilibrium to include the effects of local anisotropy.

Relativistic stellar models have been studied ever since the first solution of Einstein’s field equation for the interior of a compact object in hydrostatic equilibrium was obtained by Schwarzschild in 1916. The search for the exact solutions describing a static isotropic and anisotropic stellar type configuration has continuously attracted the interest of physicists. The study of general relativistic compact objects is of fundamental importance for astrophysics. After the discovery of pulsars and the explanation of their properties by assuming them to be rotating neutron stars, the theoretical investigation of super-dense stars has been done using both numerical and analytical methods, and the parameters of neutron stars have been worked out by a general relativistic treatment.

In the present paper we consider a class of exact solutions of the gravitational field equations for an anisotropic charged fluid sphere, corresponding to a specific choice of the anisotropy parameter and electric intensity. The importance of charge is due to the following reasons:

• (i) The presence of some charge may avert the gravitational collapse by counter-balancing the gravitational attraction by the electric repulsion in addition to the pressure gradient.

• (ii) The charge dust models and electromagnetic mass models provide information as regards the structure of the electron (Bijalwan 2011) and the lepton model (Kiess 2012).

It is desirable to study the Einstein–Maxwell field equations with reference to the general relativistic prediction of gravitational collapse with anisotropic matter.

All the physical parameters like the energy density, pressure and metric tensor components are regular inside the charged anisotropic star, with the speed of sound less than the speed of light. Therefore this solution can give a satisfactory description of realistic astrophysical compact objects like neutron stars. Some explicit numerical models of relativistic anisotropic stars, with possible astrophysical relevance, are also presented.

2 Field equations

Let us consider the static, spherically symmetric line element in curvature coordinates

$$ \mathrm{d}s^{2} = - \mathrm{e}^{\lambda} \,\mathrm{d}r^{2} - r^{2}\,\mathrm{d} \theta^{2} - r^{2}\sin^{2}\theta \,\mathrm{d} \varphi^{2} + \mathrm{e}^{\nu} \,\mathrm{d}t^{2}, $$

(1)

where λ=λ(r) and ν=ν(r). We have

$$ \begin{aligned}[b] T_{ij} &= R_{ij} - \frac{1}{2}R g_{ij} \\ &= - 8 \pi \bigl[ (\rho + p_{\mathrm{r}})v_{i}v_{j} - p_{\mathrm{t}} g_{ij} + (p_{\mathrm{r}} - p_{\mathrm{t}}) x_{i}x_{j} \bigr], \end{aligned} $$

(2)

where vⁱ is the fluid four-velocity vector for both the energy-momentum tensor, and xⁱ is the unit space-like vector orthogonal to vⁱ.

In the co-moving system we choose

$$ v^{i} = \bigl(v^{1}, 0, 0, 0 \bigr), \qquad x^{i} = \bigl(0, x^{2}, 0, 0 \bigr). $$

(2a)

From vⁱ v _i =xⁱ x _i =1, we obtain

$$ v^{1} = \mathrm{e}^{ - \nu /2}, \qquad x^{2} = \mathrm{e}^{ - \lambda /2}. $$

(2b)

The non-vanishing components of $T_{j}^{i}$are

$$ T_{1}^{1} = \rho, \qquad T_{2}^{2} = - p_{\mathrm{r}}, \qquad T_{3}^{3} = T_{4}^{4} = - P_{\mathrm{t}}. $$

(2c)

The field equation (2) gives the following set of equations under the metric (1):

$$ \kappa T_{4}^{4} = \frac{\lambda '}{r}\mathrm{e}^{ - \lambda} + \frac{(1 - \mathrm{e}^{ - \lambda} )}{r^{2}} = \kappa c^{2}\rho + \frac{q}{r^{4}}^{2}, $$

(3)

$$ - \kappa T_{1}^{1} = \frac{v'}{r}\mathrm{e}^{ - \lambda} - \frac{(1 - \mathrm{e}^{ - \lambda} )}{r^{2}} = \kappa p_{\mathrm{r}} - \frac{q}{r^{4}}^{2}, $$

(4)

$$ \begin{aligned}[b] - \kappa T_{2}^{2} &= - \kappa T_{3}^{3} = \biggl[ \frac{v''}{2} - \frac{\lambda 'v'}{4} + \frac{v'^{2}}{4} + \frac{v' - \lambda '}{2r} \biggr] \mathrm{e}^{ - \lambda} \\ & = \kappa p_{\mathrm{t}} + \frac{q}{r^{4}}^{2}, \end{aligned} $$

(5)

where the prime denotes differentiating with respect to r, while p_r(r), p_t(r) and ρ(r) are radial pressure (in the direction of x _i ), tangential pressure (orthogonal to x _i ) and energy density, respectively, for the anisotropic fluid. On the other hand E (${=}\frac{q}{r^{2}}$) denotes the electric intensity of anisotropic perfect fluid distribution. Also the q is given by

$$q(r) = 4\pi \int_{0}^{r} \sigma r^{2}\mathrm{e}^{\lambda /2}\,\mathrm{d}r = r^{2}\sqrt{ - F_{14} F^{14}} = r^{2}F^{41}\mathrm{e}^{(\lambda + \nu )/2}, $$

representing the total charge contained within the sphere of radius r. Also F₁₄ is the only non-vanishing component of the skew-symmetric electromagnetic tensor F _ij .

We assume p_r≠p_t. The case in which p_r=p_t corresponds to the isotropic fluid sphere. Δ=p_t−p_r is a measure of the anisotropy and is called the anisotropy factor (Herrera and Ponce de Leon 1985). The term 2(p_t−p_r)/r appears in the conservation equations $T_{k; i}^{i} = 0$ (where a semicolon denotes the covariant derivative with respect to the metric), representing a force that is due to the anisotropic nature of the fluid. This force is directed outward when p_t>p_r and inward when p_r>p_t. The existence of a repulsive force (in the case in which p_t>p_r) allows for the construction of more compact objects when using an anisotropic fluid rather than when using an isotropic fluid (Gokhroo and Mehra 1994).

Subtracting Eq. (4) from Eq. (5) we immediately get

$$ \begin{aligned}[b] &\kappa \bigl(T_{1}^{1} - T_{2}^{2} \bigr) \\ &\quad = \mathrm{e}^{ - \lambda} \biggl[ \frac{v''}{2} + \frac{v'^{2}}{4} - \frac{v'}{2r} - \frac{1}{r^{2}} \biggr] - \mathrm{e}^{ - \lambda} \lambda ' \biggl[ \frac{\nu '}{4} + \frac{1}{2r} \biggr] \\ &\quad = \kappa (p_{\mathrm{t}} - p_{\mathrm{r}}) + 2 \frac{q^{2}}{r^{4}}. \end{aligned} $$

(6)

Equation (6) reveals the equivalence of the anisotropy parameter Δ=κ(p_t−p_r).

Also the solution of Eq. (6) with the given expression of Δ may provide the anisotropic fluid distribution.

Using the transformations ψ=c₀r² (c₀ is a positive constant), e^−λ=V and e^ν=Y², Eqs. (3)–(6) assume the forms

$$ \frac{(1 - V)}{\psi} - 2\frac{\mathrm{d}V}{\mathrm{d}\psi} = \frac{\kappa c^{2}\rho}{c_{0}} + \frac{c_{0}q^{2}}{\psi^{2}}, $$

(7)

$$ 4V \biggl( \frac{\mathrm{d}Y}{\mathrm{d}\psi} \biggr)\frac{1}{Y} - \frac{(1 - V)}{\psi} = \frac{\kappa p_{\mathrm{r}}}{c_{0}} - \frac{c_{0}q^{2}}{\psi^{2}}, $$

(8)

$$ \begin{aligned}[b] &4 \psi \frac{V}{Y}\frac{\mathrm{d}^{2}Y}{\mathrm{d}\psi^{2}} + 2 \frac{\psi}{Y} \frac{\mathrm{d} Y}{\mathrm{d} \psi}\frac{\mathrm{d}V}{\mathrm{d}\psi} + 4 \frac{V}{Y}\frac{\mathrm{d} Y}{\mathrm{d}\psi} + \frac{\mathrm{d}V}{\mathrm{d}\psi} \\ &\quad = \frac{\kappa p_{\mathrm{t}}}{c_{0}} + \frac{c_{0}q^{2}}{\psi^{2}} \end{aligned} $$

(9)

and

$$ \begin{aligned}[b] &4 \psi^{2}V\frac{\mathrm{d}^{2}Y}{\mathrm{d}\psi^{2}} + 2 \psi^{2} \frac{\mathrm{d} Y}{\mathrm{d} \psi}\frac{\mathrm{d}V}{\mathrm{d}\psi} \\ &\quad {}+ \biggl[ \psi \frac{\mathrm{d}V}{\mathrm{d}\psi} - V - \frac{\varDelta \psi}{c_{0}} - \frac{2c_{0}q^{2}}{\psi} + 1 \biggr] Y = 0. \end{aligned} $$

(10)

Now let us consider the anisotropic model obtained by Maurya and Gupta (2012), which has

$$ \begin{aligned} &\mathrm{e}^{\nu} = Y^{2} = \tilde{B} (1 + \psi )^{n} \quad \mbox{and} \\ &\varDelta = \frac{n^{2} c_{0} \varDelta_{0} \psi}{ [ 1 + (n + 1) \psi ]^{(1 - n )/(1 + n )}}, \\ &\frac{c_{0}q^{2}}{\psi} = \frac{n^{2} K \psi^{2}}{2 [ 1 + (n + 1) \psi ]^{(1 - n )/(1 + n )}}. \end{aligned} $$

(11)

On inserting Y and Δ and $\frac{2c_{0}q^{2}}{\psi^{2}}$from (11) into Eq. (10) we get

$$ \begin{aligned}[b] &\frac{\mathrm{d}V}{\mathrm{d}\psi} - \frac{[ 1 + 2\psi + (1 + 2n - n^{2}) \psi^{2}]}{\psi (1 + \psi ) [1 + (n + 1) \psi ]} V \\ &\quad = \frac{ \{ \frac{n^{2} (\varDelta_{0} + K) \psi^{2}}{ [ 1 + (n + 1) \psi ]^{(1 - n )/(1 + n )}} - 1 \} (1 + \psi )}{\psi [1 + (n + 1) \psi ]}. \end{aligned} $$

(12)

This admits the solution

$$ \begin{aligned}[b] V &= \biggl[ \frac{n (\varDelta_{0} + K) \psi (1 + \psi )^{2}}{[ 1 + (n + 1) \psi ]^{2/(n + 1)}} \\ &\quad {} - \frac{B_{0} \psi}{2 (1 + \psi )^{n - 2}} + \frac{[ 1 + (n + 1) \psi ]}{( 1 + \psi )^{n - 2}} \\ &\quad {} + \frac{\tilde{A} \psi}{(1 + \psi )^{n - 2}[ 1 + (n + 1) \psi ]^{2/(n + 1)}} \\ &\quad {}-\frac{\psi [ 1 + (n + 1) \psi ] f(\psi )}{(n + 1) (1 + \psi )^{n - 1}} \biggr],\end{aligned} $$

(13)

where

$$\begin{aligned}[b] &B_{0} = \frac{(n - 1)(n - 2)}{2} - \frac{a_{n - 4}}{(n + 1)} + 2n + 2, \\ &f(\psi ) = \frac{A_{n - 4}}{(n + 3)} + \sum_{i = 0}^{n - 5} \frac{A_{i} x^{n - 4 - i}}{n^{2} - (i + 2)n - (i + 1)}, \\ &A_{i + 1} = a_{i + 1} - \frac{(n - 4 - i)A_{i}}{n^{2} - (i + 2)n - (i + 1)}, \\ &a_{i + 1} = \frac{(n - 1)!}{(i + 1)! (n - i - 2)!} - \frac{a_{i}}{(n + 1)}, \\ &A_{0} = a_{0} = 1,\qquad A_{n - j} = a_{n - j} = 0\quad \end{aligned} $$

for all n<j. We have

$$ \begin{aligned}[b] \frac{\kappa c^{2}\rho}{C} &= \biggl[ \frac{f(\psi ) \tilde{B}_{1}(\psi )}{(n + 1)(1 + \psi )^{n - 1}} + \frac{\tilde{F}(\psi )}{(1 + \psi )^{n - 1}} \\ &\quad {}+ \frac{B_{0} \tilde{B}_{2}(\psi )}{2(1 + \psi )^{n - 1}} - \frac{\tilde{A} \tilde{B}_{3}(\psi )}{(1 + \psi )^{n - 1} B_{5}(\psi )} \\ &\quad {}+ 2 \tilde{f}_{2}(\psi ) - \frac{n (\varDelta_{0} + K) \tilde{B}_{4}(\psi )}{\tilde{B}_{5}(\psi )} \\ &\quad {}- \frac{n^{2} K\psi^{2}}{2 [1 + (n + 1) \psi ]^{\frac{1 - n}{1 + n}}} \biggr], \end{aligned} $$

(14a)

$$ \begin{aligned}[b] \frac{\kappa p_{\mathrm{r}}}{c_{0}} &= \Biggl[ \frac{[ 1 + (2n + 1) \psi ]}{(1 + \psi )^{n - 1}} \biggl[ \frac{\tilde{A}}{[ 1 + (n + 1) \psi ]^{2/(n + 1)}} \\ &\quad {}- \frac{[1 + (n + 1) \psi ]f(\psi )}{(n + 1)} - \frac{B_{0}}{2} \biggr] + \frac{\tilde{B}_{6}(\psi )}{(1 + \psi )^{n - 1}} \\ &\quad {}+ \frac{n( \varDelta_{0} + K)(1 + \psi ) [ 1 + (2n + 1) \psi ]}{ [ 1 + (n + 1) \psi ]^{2/(n + 1)}} \\ &\quad {}+ \frac{n^{2} K\psi^{2}}{2 [1 + (n + 1) \psi ]^{\frac{1 - n}{1 + n}}} \Biggr] \end{aligned} $$

(14b)

and

$$ \frac{\kappa p_{\mathrm{t}}}{c_{0}} = \frac{n^{2} \varDelta_{0} \psi}{ [ 1 + (n + 1) \psi ]^{(1 - n )/(1 + n )}} + \frac{\kappa p_{\mathrm{r}}}{c_{0}}; $$

(14c)

here

$$\begin{aligned}[b] \tilde{F}(\psi ) &= \bigl[ \bigl\{ \tilde{f}_{1}(\psi ) - (n + 1) \bigr\} (1 + \psi ) \\ &\quad {}- 2 \bigl\{ 3 + \bigl(3 + 2n - n^{2} \bigr) \psi \bigr\} \bigr], \end{aligned} $$

$$\tilde{B}_{1}(\psi ) = \bigl[ 3 + (3n + 12)\psi + \bigl(9 + 7n - 2n^{2} \bigr)\psi^{2} \bigr], $$

$$\tilde{B}_{2}( \psi ) = \bigl[3 + \psi + 2(3 - n) \psi \bigr], $$

$$\tilde{B}_{3}(\psi ) = \bigl[ 3 + (6 + n) \psi + \bigl(3 + 5n - 2n^{2} \bigr) \psi^{2} \bigr], $$

$$\tilde{B}_{4}( \psi ) = (1 + \psi ) \bigl[ 3 + (3n + 6) \psi + (7n + 3) \psi^{2} \bigr], $$

$$\tilde{B}_{5}(\psi ) = \bigl[ 1 + (n + 1)\psi \bigr]^{(n + 3)/(n + 1)}, $$

$$\tilde{B}_{6}(\psi ) = \bigl[(3n + 2) - \tilde{f}_{3}( \psi ) + \bigl(2n^{2} + 3n + 1 \bigr)\psi \bigr], $$

$$\tilde{f}_{1}(\psi ) = \sum_{i = 1}^{n - 2} \frac{(n - 2)! \psi^{i - 1}}{i !(n - 2 - i)!}, $$

$$\tilde{f}_{2}(\psi ) = \frac{[1 + (n + 1) \psi ]}{(n + 1)(1 + \psi )^{n - 2}} \sum_{i = 0}^{n - 5} \frac{(n - 4 - i)A_{i}\psi^{n - 4 - i}}{n^{2} - (i + 2)n - (i + 1)}, $$

$$\tilde{f}_{3}(\psi ) = \sum_{i = 1}^{n - 1} \frac{(n - 1)! \psi^{i - 1}}{i !(n - 1 - i)!}. $$

Consequently the expressions for the density and pressure gradient read

$$ \begin{aligned}[b] \frac{\kappa c^{2}}{c_{0}}\frac{\mathrm{d}\rho}{\mathrm{d}\psi} &= \biggl[ \frac{\mathrm{d}\rho_{1}}{\mathrm{d}\psi} + \frac{\mathrm{d}\rho_{2}}{\mathrm{d}\psi} - \tilde{A}\frac{\mathrm{d}\rho_{3}}{\mathrm{d}\psi} + \frac{\mathrm{d}\rho_{4}}{\mathrm{d}\psi} \\ &\quad {}+ 2\frac{\mathrm{d}\rho_{5}}{\mathrm{d}\psi} - n^{2}(\varDelta_{0} + K)\frac{\mathrm{d}\rho_{6}}{\mathrm{d}\psi} \\ &\quad {}- \frac{n^{2}K (1 + 2n\psi )}{2 [1 + (n + 1)\psi ]^{\frac{2}{n + 1}}} \biggr], \end{aligned} $$

(15)

where

$$\begin{aligned}[b] \frac{\mathrm{d}\rho_{1}}{\mathrm{d}\psi} &= \frac{(1 + \psi )^{2}\tilde{g}_{1}(\psi ) + (2 - n)(1 + \psi )[\tilde{f}_{1}(\psi ) - (n + 1)]}{(1 + \psi )^{n}} \\ &\quad {}+\frac{- 2(3 + 2n - n^{2})[1 + (2 - n)\psi ] + 6(n - 1)}{(1 + \psi )^{n}}, \end{aligned} $$

$$\frac{\mathrm{d}\rho_{2}}{\mathrm{d}\psi} = \frac{\tilde{B}}{2}\frac{[ (2n^{2} - 11n + 14)\psi + (10 - 5n)]}{(1 + \psi )^{n}}, $$

$$\begin{aligned}[b] \frac{\mathrm{d}\rho_{3}}{\mathrm{d}\psi} &= \frac{n(1 - 8n) \psi + (9n - 10n^{2} - n^{3})\psi^{2}}{(1 + \psi )^{n}[1 + (n + 1) \psi ]^{(2n + 4)/(n + 1)}} \\ &\quad {}+\frac{ - 5n + (2n^{4} - 7n^{3} + 2n^{2} + 3n)\psi^{3}}{(1 + \psi )^{n}[1 + (n + 1) \psi ]^{(2n + 4)/(n + 1)}}, \end{aligned} $$

$$\begin{aligned}[b] \frac{\mathrm{d}\rho_{4}}{\mathrm{d}\psi} &= \frac{(1 + \psi )[ f_{4}(\psi)\tilde{B}_{1}(\psi ) + f(\psi )\tilde{B}_{7}(\psi )]}{(n + 1)(1 + \psi )^{n}} \\ &\quad {}- \frac{(n - 1)f(\psi)\tilde{B}_{1}(\psi )}{(n + 1)(1 + \psi )^{n}}, \end{aligned} $$

$$\begin{aligned}[b] \frac{\mathrm{d}\rho_{5}}{\mathrm{d}\psi} &= \frac{[(n - 4) + (n^{2} - 2n - 5)\psi - (n + 1)\psi^{2}]}{(n + 1)(1 + \psi )^{n - 1}}\tilde{f}_{4}(\psi ) \\ &\quad {}- \frac{[1 + (n + 1) \psi ]}{(n + 1)(1 + \psi )^{n - 2}}\tilde{f}_{5}(\psi ), \end{aligned} $$

$$\frac{\mathrm{d}\rho_{6}}{\mathrm{d}\psi} = \frac{\psi [ 14 + (10 n + 20) \psi + (14 n + 6) \psi^{2} ]}{ [ 1 + (n + 1) \psi ]^{(4 + 2n )/(n + 1 )}}, $$

$$ \begin{aligned}[b] \frac{\kappa}{c_{0}}\frac{\mathrm{d}p_{\mathrm{r}}}{\mathrm{d}\psi} &= \biggl[ A \frac{\mathrm{d}p_{1}}{\mathrm{d}\psi} - \frac{\mathrm{d}p_{2}}{\mathrm{d}\psi} - \frac{\mathrm{d}p_{3}}{\mathrm{d}\psi} + \frac{\mathrm{d}p_{4}}{\mathrm{d}\psi} \\ &\quad {}+ 2 n^{2}(\varDelta_{0} + K)\frac{\mathrm{d}p_{5}}{\mathrm{d}\psi} \\ &\quad {}+ \frac{n^{2}K (1 + 2n\psi )}{2 [1 + (n + 1)\psi ]^{\frac{2}{n + 1}}} \biggr], \end{aligned} $$

(16a)

where

$$\frac{\mathrm{d}p_{1}}{\mathrm{d}\psi} = \frac{n [1 + (2 - n) \psi + (1 + n - 2n^{2})\psi^{2}]}{(1 + \psi )^{n}[1 + (n + 1) \psi ]^{(n + 3)/(n + 1)}}, $$

$$\begin{aligned}[b] \frac{\mathrm{d}p_{2}}{\mathrm{d}\psi} &= \frac{\tilde{f}(\psi )\tilde{B}_{8}(\psi )}{(n + 1)(1 + \psi )^{n}} \\ &\quad {}+\frac{(1 + \psi )[1 + (2n + 1)\psi ][1 + (n + 1)\psi ]\tilde{f}_{4}(\psi )}{(n + 1)(1 + \psi )^{n}}, \end{aligned} $$

$$\frac{\mathrm{d}p_{3}}{\mathrm{d}\psi} = \frac{\tilde{B}}{2}\frac{[(n + 2) + (2 + 3n - 2n^{2}) \psi ]}{(1 + \psi )^{n}}, $$

$$\begin{aligned}[b] \frac{\mathrm{d}p_{4}}{\mathrm{d}\psi} &= \frac{(n - 1)\tilde{f}_{3}(\psi ) - (1 + \psi)f_{6}(\psi )}{(1 + \psi )^{n}} \\ &\quad {}+\frac{ (3 + 4n - n^{2}) + (2 + 5n + n^{2} - 2n^{3}) \psi}{(1 + \psi )^{n}}, \end{aligned} $$

$$\frac{\mathrm{d}p_{5}}{\mathrm{d}\psi} = \frac{ [ 1 + (n + 2) \psi + (2 n + 1) \psi^{2} ]}{ [ 1 + (n + 1) \psi ]^{(n + 3)/(n + 1)}} $$

and

$$ \frac{\kappa}{c_{0}}\frac{\mathrm{d}p_{\mathrm{t}}}{\mathrm{d}\psi} = \frac{n^{2}\varDelta_{0} (1 + 2n \psi )}{ [ 1 + (n + 1) \psi ]^{2/(n + 1)}} + \frac{\kappa}{ c_{0}} \frac{\mathrm{d}p_{\mathrm{r}}}{\mathrm{d}\psi}, $$

(16b)

with

$$\tilde{f}_{4}(\psi ) = \sum_{i = 0}^{n - 5} \frac{(n - 4 - i)A_{i}\psi^{n - 5 - i}}{n^{2} - (i + 2)n - (i + 1)}, $$

$$\tilde{f}_{5}(\psi ) = \sum _{i = 1}^{n - 5} \frac{i(n - 4 - i)A_{i}\psi^{n - 5 - i}}{n^{2} - (i + 2)n - (i + 1)}, $$

$$\tilde{g}_{1}(\psi ) = \sum_{i = 1}^{n - 3} \frac{(n - 2)! i \psi^{i - 1}}{(i + 1)! (n - i - 3)!}, $$

$$\tilde{f}_{6}(\psi ) = \sum _{i = 2}^{n - 1} (i - 1)\frac{(n - 1)! \psi^{i - 2}}{i !(n - 1 - i)!}, $$

$$\tilde{B}_{7}(\psi ) = (3n + 12) + 2 \bigl(9 + 7n - 2n^{2} \bigr) \psi, $$

$$\begin{aligned}[b] \tilde{B}_{8}(\psi ) &= (2n + 3) + \bigl(6 + 10n + n^{2} \bigr) \psi \\ &\quad {}+ \bigl(3 + 8n + 3n^{2} - 2n^{3} \bigr) \psi^{2}. \end{aligned} $$

In order to be physically meaningful, the interior solution for static fluid spheres of Einstein’s gravitational-field equations must satisfy some more general physical requirements. The following conditions have been generally recognised to be crucial for anisotropic fluid spheres; see Herrera and Santos (1997).

• (i) The solution should be free from physical and geometric singularities and non-zero positive values of e^λ and e^ν i.e. (e^λ)_r=0=1 and e^ν>0.

• (ii) The radial pressure p_r must be vanishing but the tangential pressure p_t may not vanish at the boundary r=a of the sphere. However, the radial pressure is equal to the tangential pressure at the centre of the fluid sphere.

• (iii) The density ρ and pressure p_r, p_t should be positive inside the star.

• (iv) We should have (dp_r/dr)_r=0=0 and (d² p_r/dr²)_r=0<0 so that the pressure gradient dp_r/dr is negative for 0<r≤a.

• (v) We should have (dp_t/dr)_r=0=0 and (d² p_t/dr²)_r=0<0 so that the pressure gradient dp_t/dr is negative for 0<r≤a.

• (vi) Furthermore we should have (dρ/dr)_r=0=0 and (d² ρ/dr²)_r=0<0 so that the density gradient dρ/dr is negative for 0<r≤a.

The condition (iv), (v) and (vi) imply that the pressure and density should be maximum at the centre and monotonically decreasing towards the surface.

• (vii) Inside the static configuration the speed of sound should be less than the speed of light, i.e.

  $$0 \le \sqrt{\frac{\mathrm{d}p_{\mathrm{r}}}{c^{2}\,\mathrm{d}\rho}} < 1 \quad \mbox{and} \quad 0 \le \sqrt{ \frac{\mathrm{d}p_{\mathrm{t}}}{c^{2}\,\mathrm{d}\rho}} < 1. $$

In addition to the above the velocity of sound should be decreasing towards the surface. i.e. $\frac{\mathrm{d}}{\mathrm{d}r} ( \frac{\mathrm{d}p_{\mathrm{r}}}{\mathrm{d}\rho} ) < 0$ or $( \frac{\mathrm{d}^{2}p_{\mathrm{r}}}{\mathrm{d}\rho^{2}} ) > 0$ and $\frac{\mathrm{d}}{\mathrm{d}r} ( \frac{\mathrm{d}p_{\mathrm{t}}}{\mathrm{d}\rho} ) < 0$ or $( \frac{\mathrm{d}^{2}p_{\mathrm{t}}}{\mathrm{d}\rho^{2}} ) > 0$ for 0≤r≤a i.e. the velocity of sound is increasing with the increase of density.

• (viii) A physically reasonable energy-momentum tensor has to obey the conditions ρ≥p_r+2p_t and ρ+p_r+2p_t≥0.

• (ix) The central red shift Z₀ and surface red shift Z _a should be positive and finite i.e. Z₀=[(e^−ν/2−1)_r=0]>0 and Z _a =[e^λ(a)/2−1]>0 and both should be bounded.

3 Properties of new class of solutions

We have

$$ \begin{aligned}[b] \biggl[ \frac{\kappa p_{\mathrm{r}}}{c_{0}} \biggr]_{r = 0} &= \biggl[ \frac{\kappa p_{\mathrm{t}}}{c_{0}} \biggr]_{r = 0} = - \frac{A_{n - 4}}{(n + 1)(n + 3)} - \frac{a_{n - 3}}{2} \\ &\quad {} + (n + 2) + \tilde{A} + n (\varDelta_{0} + K), \end{aligned} $$

(17a)

$$ \begin{aligned}[b] \biggl[ \frac{\kappa c^{2}\rho}{c_{0}} \biggr]_{r = 0} &= \frac{3 A_{n - 4}}{(n + 1)(n + 3)} + \frac{3 a_{n - 3}}{2} + (3n - 6) \\ &\quad {}- 3\tilde{A} - 3n (\varDelta_{0} + K), \end{aligned} $$

(17b)

$$ \biggl[ \frac{\kappa}{c_{0}}\frac{ \,\mathrm{d}p_{\mathrm{r}}}{ \,\mathrm{d}r} \biggr]_{r = 0} = 2 c_{0} r \biggl[ \frac{\kappa}{c_{0}}\frac{ \,\mathrm{d}p_{\mathrm{t}}}{\mathrm{d}\psi} \biggr]_{\psi = 0} = 0, $$

(18a)

$$\biggl[ \frac{\kappa}{c_{0}}\frac{ \,\mathrm{d}p_{\mathrm{t}}}{ \,\mathrm{d}r} \biggr]_{r = 0} = 2 c_{0} r \biggl[ \frac{\kappa}{c_{0}}\frac{ \,\mathrm{d}p_{\mathrm{t}}}{\mathrm{d}\psi} \biggr]_{\psi = 0} = 0, $$

$$ \biggl[ \frac{\kappa c^{2}}{c_{0}}\frac{\mathrm{d}\rho}{ \,\mathrm{d}r} \biggr]_{r = 0} = 2 c_{0} r \biggl[ \frac{\kappa}{c_{0}}\frac{\mathrm{d}\rho}{ \,\mathrm{d}x} \biggr]_{\psi = 0} = 0, $$

(18b)

$$ \begin{aligned}[b] \biggl[ \frac{\kappa}{c_{0}}\frac{\mathrm{d}^{2}p_{\mathrm{r}}}{\mathrm{d}r^{2}} \biggr]_{r = 0} & = 2 c_{0} \biggl[ \frac{n}{2}\frac{a_{n - 4}}{(n + 4)} + \frac{n}{2}\frac{A_{n - 5}}{(n + 1)(n + 2)(n + 3)} \\ &\quad {}- \frac{n^{3} + 5n^{2} - 6n}{4} + \tilde{A}n \\ &\quad {}+ \frac{n^{2}}{2} (4 \varDelta_{0} + 5K) \biggr], \end{aligned} $$

(19a)

$$ \begin{aligned}[b] \biggl[ \frac{\kappa}{c_{0}}\frac{\mathrm{d}^{2}p_{\mathrm{t}}}{\mathrm{d}r^{2}} \biggr]_{r = 0} & = 2 c_{0} \biggl[ \frac{n}{2}\frac{a_{n - 4}}{(n + 4)} + \frac{n}{2}\frac{A_{n - 5}}{(n + 1)(n + 2)(n + 3)} \\ &\quad {}- \frac{n^{3} + 5n^{2} - 6n}{4} + \tilde{A}n \\ &\quad {}+ \frac{n^{2}}{2} (6\varDelta_{0} + 5K) \biggr], \end{aligned} $$

(19b)

$$ \begin{aligned}[b] \biggl[ \frac{\kappa c^{2}}{c_{0}}\frac{\mathrm{d}^{2}\rho}{\mathrm{d}r^{2}} \biggr]_{r = 0} &= 2 c_{0} \biggl[ \frac{5n}{2} \biggl\{ - \frac{n^{2} + 3n - 2}{2} + \frac{a_{n - 4}}{(n + 3)} \\ &\quad {}+ \frac{A_{n - 5}}{(n + 1)(n + 2)(n + 3)} \biggr\} \\ &\quad {}+ 5n A - \frac{n^{2}}{2} K \biggr]. \end{aligned} $$

(19c)

For

$$\begin{aligned}[b] & ( p_{\mathrm{r}} )_{r = 0} \ge 0, \qquad ( \rho )_{r = 0} \ge 0, \\ &\bigl(\mathrm{d}^{2}p_{\mathrm{r}}/\mathrm{d}r^{2}\bigr)_{r = 0} < 0, \qquad \bigl(\mathrm{d}^{2}p_{\mathrm{t}}/\mathrm{d}r^{2}\bigr)_{r = 0} < 0. \end{aligned} $$

Consequently we have

$$ \begin{aligned}[b] &\frac{A_{n - 4}}{(n + 1)(n + 4)} + \frac{a_{n - 3}}{2} - (n + 2) - n (\varDelta_{0} + K) \\ &\quad < A < - \frac{a_{n - 4}}{2(n + 4)} - \frac{A_{n - 5}}{2(n^{3} + 6n^{2} + 11n + 6)} \\ &\hphantom{\quad < A <} {}+ \frac{n^{2} + 5n - 6}{4}. \end{aligned} $$

(20)

Hence the velocity of sound at the centre is given by

$$ \biggl[ \frac{ \,\mathrm{d}p_{\mathrm{r}}}{ c^{2}\mathrm{d}\rho} \biggr]_{r = 0} = \Biggl[ \frac{\frac{n}{2}\frac{a_{n - 4}}{(n + 4)} + \frac{n}{2}\frac{A_{n - 5}}{(n + 1)(n + 2)(n + 3)} - \frac{n^{3} + 5n^{2} - 6n}{4} + \tilde{A}n + 2 n^{2}(\varDelta_{0} + K)}{\frac{5n}{2} \{ - \frac{n^{2} + 3n - 2}{2} + \frac{a_{n - 4}}{(n + 3)} + \frac{A_{n - 5}}{(n + 1)(n + 2)(n + 3)} \} + 5n \tilde{A} - \frac{n^{2}}{2} K} \Biggr] $$

(21)

and

$$ \biggl[ \frac{ \,\mathrm{d}p_{\mathrm{t}}}{ c^{2}\mathrm{d}\rho} \biggr]_{r = 0} = \Biggl[ \frac{\frac{n}{2}\frac{a_{n - 4}}{(n + 4)} + \frac{n}{2}\frac{A_{n - 5}}{(n + 1)(n + 2)(n + 3)} - \frac{n^{3} + 5n^{2} - 6n}{4} + \tilde{A}n + 3 n^{2}\varDelta_{0}}{\frac{5n}{2} \{ - \frac{n^{2} + 3n - 2}{2} + \frac{a_{n - 4}}{(n + 3)} + \frac{A_{n - 5}}{(n + 1)(n + 2)(n + 3)} \} + 5n \tilde{A} - \frac{n^{2}}{2} K} \Biggr], $$

(22)

which should be

$$\begin{aligned}[b] &\biggl[ \frac{ \,\mathrm{d}p_{\mathrm{r}}}{ c^{2}\mathrm{d}\rho} \biggr]_{r = 0} < 1 \quad \mbox{and} \quad \biggl[ \frac{ \,\mathrm{d}p_{\mathrm{t}}}{ c^{2}\mathrm{d}\rho} \biggr]_{r = 0} < 1 \\ &\quad \mbox{for all values of }\varDelta_{0} \ge 0 \mbox{ and } \tilde{A}. \end{aligned} $$

The expression for the gravitational red shift Z is given by

$$ Z = \frac{(1 + \psi )^{ - n/2}}{\sqrt{\tilde{B}}} - 1. $$

(23a)

For the central value of the gravitational red shift to be non-zero positive finite, we must have

$$1 > \tilde{B} > 0. $$

Differentiating Eq. (23a) w.r.t. x, we get

$$ \biggl[ \frac{\mathrm{d}^{2}Z}{\mathrm{d}r^{2}} \biggr]_{r = 0} = - \frac{n c_{0}}{\sqrt{\tilde{B}}} < 0. $$

(23b)

The expression of the right hand side of (23b) is negative, and then the gravitational red shift is maximum at the centre and monotonically decreasing towards the surface.

4 Boundary conditions

Besides the above, the fluid ball is expected to join smoothly with the Schwarzschild exterior solution at the pressure free boundary r=a

$$ \begin{aligned}[b] \mathrm{d}s^{2}& = - \biggl( 1 - \frac{2M}{r} + \frac{q^{2}}{r^{2}} \biggr)^{ - 1}\,\mathrm{d}r^{2} - r^{2} \bigl(\mathrm{d}\theta^{2} + \sin^{2}\theta \,\mathrm{d}\varphi^{2} \bigr) \\ &\quad {}+ \biggl( 1 - \frac{2M}{r} + \frac{q^{2}}{r^{2}} \biggr) \,\mathrm{d}t^{2}, \end{aligned} $$

(24)

which requires the continuity of e^λ and e^ν across the boundary

$$ V_{\mathrm{anis}}(a) = 1 - \frac{2M}{a} + \frac{q^{2}}{r^{2}}, $$

(25)

$$ Y^{2}(a) = 1 - \frac{2M}{a} + \frac{q^{2}}{r^{2}} $$

(26)

and the radial pressure vanishes at the boundary i.e.

$$ p_{\mathrm{r}} (a) = 0. $$

(27)

The condition (27) can be utilised to compute the value of the arbitrary constants $\tilde{A}$ as follows.

On setting ψ_r=a=ψ _a =c₀a² (a being the radius of the fluid balls), we see the following.

The radial pressure at p_r(a)=0 gives

$$ \begin{aligned}[b] \tilde{A} &= \biggl[ \bigl[ 1 + (n + 1) \psi_{a} \bigr]^{2/(n + 1)} \\ &\quad {}\times \biggl[ \frac{\tilde{B}_{0}}{2} + \frac{[f_{3}(\psi_{a}) - (3n + 2) - (2n^{2} + 3n + 1)\psi_{a}]}{[ 1 + (2n + 1) \psi_{a}]} \biggr] \\ &\quad {}- \frac{n (\varDelta_{0} + K)( 1 + \psi_{a})^{n} [ 1 + (2n + 1) \psi_{a}]}{[ 1 + (2n + 1) \psi_{a} ]} \\ &\quad {}+ \frac{\tilde{B}_{5}(\psi_{a})f(\psi_{a} )}{(n + 1)} - \frac{n^{2}K(1 + \psi )^{n - 1}}{2 [ 1 + (2n + 1) \psi ]} \biggr], \end{aligned} $$

(28)

where

$$\tilde{B}_{5}(\psi_{a}) = \bigl[ 1 + (n + 1) \psi_{a} \bigr]^{(n + 3)/(n + 1)}, $$

$$f(\psi_{a}) = \frac{A_{n - 4}}{(n + 3)} + \sum_{i = 0}^{n - 5} \frac{A_{i} \psi_{a}^{n - 4 - i}}{n^{2} - (i + 2)n - (i + 1)}, $$

$$f_{3}(\psi_{a}) = \sum_{i = 1}^{n - 1} \frac{(n - 1)! \psi_{a}^{^{i - 1}}}{i !(n - 1 - i)!}. $$

The expression for the mass can be written as

$$ \begin{aligned}[b] \frac{M}{a} &= \frac{1}{2} \Biggl[ 1 - \frac{n (\varDelta_{0} + K) \psi_{a} (1 + \psi_{a})^{2}}{[ 1 + (n + 1) \psi_{a}]^{2/(n + 1)}} - \frac{[ 1 + (n + 1) \psi_{a}]}{( 1 + \psi_{a})^{n - 2}} \\ &\quad {}- \frac{\tilde{A} \psi_{a}}{(1 + \psi_{a})^{n - 2}[ 1 + (n + 1) \psi_{a}]^{2/(n + 1)}} \\ &\quad {}+ \frac{B_{0} \psi_{a}}{2 (1 + \psi_{a})^{n - 2}} +\frac{\psi_{a} [ 1 + (n + 1) \psi_{a}] f(\psi_{a})}{(n + 1) (1 + \psi_{a})^{n - 1}} \\ &\quad {}+ \frac{n^{2} K\psi_{a}^{2}}{2 [1 + (n + 1) \psi_{a}]^{\frac{1 - n}{1 + n}}} \Biggr], \end{aligned} $$

(29)

such that

$$V_{\mathrm{anis}}(a) = 1 - \frac{2M}{a} + \frac{q^{2}}{r^{2}}, $$

where M=m(a) and

$$Y^{2}(a) = 1 - \frac{2M}{a} + \frac{q^{2}}{r^{2}} $$

gives

$$ \begin{aligned}[b] B &= \frac{1}{( 1 + \psi_{a} )^{n}} \biggl[ \frac{n (\varDelta_{0} + K) \psi_{a} (1 + \psi_{a})^{2}}{[ 1 + (n + 1) \psi_{a}]^{2/(n + 1)}} + \frac{B_{0} \psi_{a}}{2 (1 + \psi_{a})^{n - 2}} \\ &\quad {}- \frac{\tilde{A} \psi_{a}}{(1 + \psi_{a})^{n - 2}[ 1 + (n + 1) \psi_{a}]^{2/(n + 1)}} \\ &\quad {}- \frac{[ 1 + (n + 1) \psi_{a}]}{( 1 + \psi_{a})^{n - 2}} \\ &\quad {}+ \frac{\psi_{a} [ 1 + (n + 1) \psi_{a}] f(\psi_{a})}{(n + 1) (1 + \psi_{a})^{n - 1}} \biggr]. \end{aligned} $$

(30)

Also, if the surface density ρ _a is prescribed as 2×10¹⁴ g cm⁻³ (super-dense star case) then the value of the constant c₀ can be calculated for a given ψ _a (=c₀a²), using the following expression:

$$ \begin{aligned}[b] \kappa c^{2}\rho &= c_{0} \biggl[ \frac{f(\psi_{a}) \tilde{B}_{1}(\psi_{a})}{(n + 1)(1 + \psi_{a})^{n - 1}} + \frac{\tilde{F}(\psi_{a})}{(1 + \psi_{a})^{n - 1}} \\ &\quad {}+ \frac{B_{0} \tilde{B}_{2}(\psi_{a})}{2(1 + \psi_{a})^{n - 1}} - \frac{\tilde{A} \tilde{B}_{3}(\psi_{a})}{(1 + \psi_{a})^{n - 1} B_{5}(\psi_{a})} \\ &\quad {}+ 2 \tilde{f}_{2}(\psi_{a}) - \frac{n (\varDelta_{0} + K) \tilde{B}_{4}(\psi_{a})}{2 \tilde{B}_{5}(\psi_{a})} \biggr], \end{aligned} $$

(31)

with

$$\begin{aligned}[b] \tilde{F}(\psi_{a}) &= \bigl[ \bigl\{ \tilde{f}_{1}( \psi_{a}) - (n + 1) \bigr\} (1 + \psi_{a}) \\ &\quad {}- 2 \bigl\{ 3 + \bigl(3 + 2n - n^{2} \bigr)\psi_{a} \bigr\} \bigr], \end{aligned} $$

$$\tilde{B}_{1}(\psi_{a}) = \bigl[ 3 + (3n + 12) \psi_{a} + \bigl(9 + 7n - 2n^{2} \bigr)\psi_{a}^{2} \bigr], $$

$$\tilde{B}_{2}(\psi_{a}) = \bigl[3 + \psi_{a} + 2(3 - n) \psi_{a} \bigr], $$

$$\tilde{B}_{3}(\psi_{a}) = \bigl[ 3 + (6 + n) \psi_{a} + \bigl(3 + 5n - 2n^{2} \bigr) \psi_{a}^{2} \bigr], $$

$$\tilde{B}_{4}( \psi_{a}) = (1 + \psi_{a}) \bigl[ 3 + (3n + 6) \psi_{a} + (7n + 3) \psi_{a}^{2} \bigr], $$

$$\tilde{B}_{5}( \psi_{a}) = \bigl[ 1 + (n + 1) \psi_{a} \bigr]^{(n + 3)/(n + 1)}, $$

$$\tilde{f}_{1}(\psi_{a}) = \sum _{i = 1}^{n - 2} \frac{(n - 2)! \psi_{a}^{i - 1}}{i !(n - 2 - i)!}, $$

$$\tilde{f}_{2}(\psi_{a}) = \frac{[1 + (n + 1) \psi_{a}]}{(n + 1)(1 + \psi_{a})^{n - 2}}\sum _{i = 0}^{n - 5} \frac{(n - 4 - i)A_{i}\psi_{a}^{n - 4 - i}}{n^{2} - (i + 2)n - (i + 1)}, $$

$$\tilde{f}_{3}(\psi_{a}) = \sum_{i = 1}^{n - 1} \frac{(n - 1)! \psi_{a}^{i - 1}}{i !(n - 1 - i)!}. $$

5 Physical analysis and conclusion

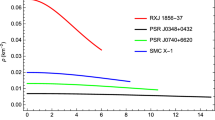
In the present article we have obtained the charge anisotropic super-dense star subject to the relevant physical requirements. The maximum mass is seen to be increasing with the increasing values of 1≤n≤4. Thereafter for n>4, the maximum mass is monotonically decreasing; however, the minimum value of the maximum mass does no go below 2.7298M _Θ . Therefore the overall maximum mass, found to be 3.9823M _Θ at n=4 with the corresponding radius 18.2273 km for the whole range of the Chandrasekhar limit, regarding the mass-radius ratio holds well. Moreover, the condition ρ−p_r−2p_t≥0 is true for all the charged anisotropic star models. The radial velocity of sound ($\sqrt{\mathrm{d}p_{\mathrm{r}}/\mathrm{d}\rho}$) and the tangential velocity of sound ($\sqrt{\mathrm{d}p_{\mathrm{t}}/\mathrm{d}\rho}$) are found to be monotonically decreasing towards the pressure free interface for n≥1. The red shift for the whole family of super-dense stars is computed. For all the models the anisotropy parameter Δ=p_t−p_r is positive throughout the range and hence helps the outward pressure to avert the gravitational collapse of the super-dense star models thus obtained. It is worth pointing out here that the space-time, describing the current models as well as the seed anisotropic perfect fluid models, gives the relation nc₀=constant=α. The above discussion helps us to write g₄₄=B(1+c₀r²)ⁿ as $g_{44} = B(1 + \frac{\alpha}{n}r^{2})^{n}$, which tends to $g_{44} = B \mathrm{e}^{\alpha r^{2}}$ as n→∞, which resembles the corresponding metric potential of the Kuchowichz space-time. The maximum mass of the charged anisotropic fluid models is shown in Tables 1(a), 1(b). For a better insight, the relevant physical quantities are presented by means of Tables 2, 4, 5, 6 and Figs. 1–6.

6 Tables for numerical values of physical quantities

Table 1(a) Maximum mass (M/M _Θ ) and radius (a) for different n, Δ₀ and c₀a²

Table 1(b) Maximum mass (M/M _Θ ) and radius (a) for different n, K, Δ₀ and c₀a²

Table 2 Maximum mass and radius for n=6, Δ₀=0.116, c₀a²=0.165 and K=0.11

Table 3 Maximum mass and radius for n=20, Δ₀=0.149, c₀a²=0.0398 and K=0.0260

Table 4 Maximum mass and radius for n=100, Δ₀=0.148, c₀a²=0.0069 and K=0.0499

Table 5 Maximum mass and radius for n=500, Δ₀=0.140, c₀a²=0.00144 and K=0.0690

Table 6 Maximum mass and radius for n=3000, Δ₀=0.119, c₀a²=0.00026 and K=0.0908

Fig. 1

Behaviour of density versus radius

Fig. 2

Behaviour of radial pressure versus radius

Fig. 3

Behaviour of tangential pressure versus radius

Fig. 4

Behaviour of radial velocity versus radius

Fig. 5

Behaviour of tangential velocity versus radius

Fig. 6

Behaviour of red shift versus radius