1 Introduction

The pipes conveying fluid can be used in many engineering fields such as submarine pipelines carrying oil and gas, hydropower engineering, and biological engineering. Since fluid-conveying pipes are constantly subjected to the initial, external and unsteady flow excitations, the poor operating performances and unexpected oscillations may induce failure or destroy the piping systems [1,2,3]. Therefore, the stability and dynamic characteristics of pipes conveying fluid have received considerable attention.

As a pump or a compressor is applied as the power source of the fluid in the system, the flow rate tends to be unstable. The pulsatile flow in the pipes constantly leads to parametric vibration, such as subharmonic and summation resonances. As a result, the parametric resonance and steady-state response have been widely studied in recent years. For example, Luo et al. [4] utilized the differential quadrature method to analyze the principal parametric resonance of a curved pipe conveying fluid. Tan et al. [5] took advantage of the finite difference method to explore the parametric resonance of Timoshenko pipes with the pulsation of supercritical high-speed fluids. Li et al. [6] employed the Runge–Kutta integration algorithm combined with the Galerkin method to analyze the three-dimensional parametric resonance of fluid-conveying pipes. Yang et al. [7] applied the method of multiple scales to examine the dynamic stability of a viscoelastic pipe conveying pulsatile fluid.

Recently, the fractional-order materials such as synthetic rubber or polymers have been widely used in industrial fields [8]. The mechanical characteristics of the fractional-order material contain the viscous and elastic properties [9,10,11]. In order to describe the particular behavior, the fractional differentiation law has been utilized to describe the dynamic model of the structures. For example, Askarian et al. [12] examined the nonlinear vibration of pipes resting on a fractional Kelvin–Voigt viscoelastic foundation and analyzed the effects of physical parameters such as the stiffness and fractional order on the stability boundary of the pipe. Yang et al. [13, 14] used the asymptotic approach to provide the analytical solutions of the steady-state responses in the axially moving fractional damped string and beam.

From the above-mentioned studies, it is conformed that the fractional-order viscoelastic property can induce more complicated dynamic behaviors, which is different from the former studies. However, to the best of the authors’ knowledge, the studies on the factional-order viscoelastic pipes conveying fluid undergoing the parametric resonance are rather limited, especially for the mechanical mechanism of pipes, which is unknown. Therefore, we performed as the first attempt to fill in the gap and conducted the study on the stability and bifurcation behaviors of the factional-order viscoelastic pipes conveying pulsatile fluid with the action of the Pasternak foundation.

2 Equations of Motion

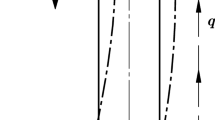
Consider a simply supported uniform fluid-conveying pipe made of fractional-order viscoelastic material with length \(L\), pipeline’s density \(\rho_{{\text{g}}}\), fluid density \(\rho_{{\text{e}}}\), time \(\hat {t}\), neutral axis coordinate x, flow’s cross-sectional area \(A_{{\text{e}}}\) and pipeline’s cross-sectional area \(A_{{\text{g}}}\), as shown in Fig. 1. The longitudinal and transverse displacements are \(\hat{u}(\hat{x},\hat{t}) \, \) and \( \, \hat{v}(\hat{x},\hat{t})\), respectively, which represent the coupled planar motion of the pipe.

Fig. 1
figure 1

The physical model of the viscoelastic pipe

Full size image

2.1 Mathematical Model of Coupled Planar Motion

The total kinetic energy of the pipes consists of two parts: the kinetic energy of fluid \(T_{{\text{e}}}\) and the kinetic energy of pipeline \(T_{{\text{g}}}\) [15,16,17],

$$ T = T_{{\text{e}}} + T_{{\text{g}}} = \frac{1}{2}\rho_{{\text{e}}} A_{{\text{e}}} \int\limits_{0}^{L} {\left[ {\left( {V + \frac{{\partial \hat{u}}}{{\partial \hat{t}}} + V\frac{{\partial \hat{u}}}{{\partial \hat{x}}}} \right)^{2} + \left( {\frac{{\partial \hat{v}}}{{\partial \hat{t}}} + V\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)^{2} } \right]} {\text{d}}\hat{x} + \frac{1}{2}\rho_{{\text{g}}} A_{{\text{g}}} \int\limits_{0}^{L} {\left[ {\left( {\frac{{\partial \hat{u}}}{{\partial \hat{t}}}} \right)^{2} + \left( {\frac{{\partial \hat{v}}}{{\partial \hat{t}}}} \right)^{2} } \right]} {\text{d}}\hat{x}$$
(1)

where \(V\) represents the flow velocity.

The axial strain is regarded as the function of planar displacements based on the Euler–Bernoulli beam theory, so we have [16, 17]

$$ \varepsilon_{{{L}}} = - z\frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}^{2} }} + \sqrt {\left( {1 + \frac{{\partial \hat{u}}}{{\partial \hat{x}}}} \right)^{2} + \left( {\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)^{2} } - 1$$
(2)

in which z is the coordinate measured from the plane of neutral fibers. The strain energy is divided into two parts. The first part \(\hat{U}_{1}\) can be written as

$$ \hat{U}_{1} = \frac{1}{2}\int\limits_{0}^{L} {\int\limits_{{A_{\rm g} }} {\hat{\sigma }_{1} \varepsilon_{L} {\text{d}}A{\text{d}}\hat{x}}} $$
(3)

which denotes the variation induced by the stress of the pipe with respective to its initial configuration, and \(\hat{\sigma }_{1}\) represents the normal stress due to the elasticity of the pipe. The second part \(\hat{U}_{2}\) is the strain energy corresponding to the subsequent induced stretching as well as the Pasternak foundation, which is expressed as

$$ \begin{aligned} \hat{U}_{2} = & \int\limits_{0}^{L} {P\left[ {\sqrt {\left( {1 + \frac{{\partial \hat{u}}}{{\partial \hat{x}}}} \right)^{2} + \left( {\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)^{2} } - 1} \right]} {\text{d}}\hat{x} \\ & \quad + \frac{1}{2}\int\limits_{0}^{L} {\left[ {k_{1} \hat{v}^{2} - k_{2} \left( {\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)^{2} } \right]} {\text{d}}\hat{x} \\ \end{aligned} $$
(4)

where \(P\) represents the initial axial tension, and \(k_{1}\) and \(k_{2}\), respectively, denote the spring and shear stiffnesses. Furthermore, the virtual work can be expressed as

$$ \delta W = - \int\limits_{0}^{L} {\int\limits_{{A_{{\text{g}}} }} {\hat{\sigma }_{2} } } \delta \varepsilon_{L} {\text{d}}A{\text{d}}\hat{x}$$
(5)

where \(\hat{\sigma }_{2}\) is the normal stress caused by the viscous behavior of the pipeline.

According to the pipeline constituted by the Kelvin–Voigt viscoelastic materials, its viscoelastic property is described by [2]

$$ \hat{\sigma } = \hat{\sigma }_{1} + \hat{\sigma }_{2} ,\left( {\hat{\sigma }_{1} = E_{0} \varepsilon_{L} ,\hat{\sigma }_{2} = E_{1} \frac{{\partial^{\alpha } }}{{\partial \hat{t}^{\alpha}_{\textrm{RL+}} }} \varepsilon_{L} } \right) $$
(6)

where \(E_{0}\) and \(E_{1}\), respectively, represent the elastic modulus and viscosity coefficient of the pipeline, \(\alpha\) is the fractional order, and \(\partial^{\alpha } /\partial \hat{t}^{\alpha } \left( {} \right)\) denotes the operator of the fractional-order derivative. To a function \(f\left( {\hat{t}} \right)\), the fractional-order differential operator can be defined according to the Riemann–Liouville form as follows [18]

$$ \frac{{d^{\alpha } }}{{{\text{d}}\hat{t}_{{{\text{RL}} + }}^{\alpha } }}f(\hat{t}) = \frac{1}{\varGamma (1 - \alpha )}\frac{d}{{{\text{d}}\hat{t}}}\int\limits_{0}^{{\hat{t}}} {(\hat{t} - \hat{s})^{ - \alpha } } f(\hat{s}){\text{d}}\hat{s}$$
(7)

where \(\varGamma\) is the Gamma function. The property of fractional order \(\alpha\) can be written as [18]

$$ \frac{{\text{d}^{\alpha } }}{{{\text{d}}\hat{t}^{\alpha}_{\textrm{RL}+} }} \text{e}^{{\text{i}w\hat{t}}} = (\text{i}w)^{\alpha } \text{e}^{{\text{i}w\hat{t}}} + \frac{\sin (\pi \alpha )}{\pi }\int\limits_{0}^{\infty } {\frac{{\hat{\varsigma }^{\alpha } }}{{\hat{\varsigma } + \text{i}w}}} \text{e}^{{ - \varsigma \hat{t}}} {\text{d}}\hat{\varsigma }$$
(8)

in which \(\text{i}\) represents the complex number \(\sqrt { - 1}\).

Introducing the generalized Hamilton’s principle as follows [2]

$$ \int\limits_{{\hat{t}_{1} }}^{{\hat{t}_{2} }} {\left( {\delta T - \delta \hat{U}_{1} - \delta \hat{U}_{2} + \delta W} \right)} {\text{d}}\hat{t} = 0 $$
(9)

and substituting Eqs. (1), (4) and (5) into Eq. (9), we have the governing equations of coupled planar motion

$$ \begin{aligned} & \rho_{{\text{e}}} A_{{\text{e}}} \left( {\frac{{\partial^{2} \hat{u}}}{{\partial \hat{t}^{2} }} + 2V\frac{{\partial^{2} \hat{u}}}{{\partial \hat{x}\partial \hat{t}}} + \frac{{{\text{d}}V}}{{{\text{d}}\hat{t}}}\frac{{\partial \hat{u}}}{{\partial \hat{x}}} + V^{2} \frac{{\partial^{2} \hat{u}}}{{\partial \hat{x}^{2} }}} \right) \\ & \quad + \rho_{{\text{g}}} A_{{\text{g}}} \frac{{\partial^{2} \hat{u}}}{{\partial \hat{t}^{2} }} - \frac{\partial }{{\partial \hat{x}}}\left[ {\frac{{\left( {P + A_{{\text{g}}} \sigma_{{{\text{in}}}} } \right)\left( {1 + \frac{{\partial \hat{u}}}{{\partial \hat{x}}}} \right)}}{{\sqrt {\left( {1 + \frac{{\partial \hat{u}}}{{\partial \hat{x}}}} \right)^{2} + \left( {\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)^{2} } }}} \right] = 0 \\ \end{aligned} $$
(10)
$$ \begin{aligned} & \rho_{{\text{e}}} A_{{\text{e}}} \left( {\frac{{\partial^{2} \hat{v}}}{{\partial \hat{t}^{2} }} + 2V\frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}\partial \hat{t}}} + \frac{{{\text{d}}V}}{{{\text{d}}\hat{t}}}\frac{{\partial \hat{v}}}{{\partial \hat{x}}} + V^{2} \frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}^{2} }}} \right) \\ & \quad + \rho_{{\text{g}}} A_{{\text{g}}} \frac{{\partial^{2} \hat{v}}}{{\partial \hat{t}^{2} }} - \frac{\partial }{{\partial \hat{x}}}\left[ {\frac{{\left( {P + A_{{\text{g}}} \sigma_{{{\text{in}}}} } \right)\left( {1 + \frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)}}{{\sqrt {\left( {1 + \frac{{\partial \hat{u}}}{{\partial \hat{x}}}} \right)^{2} + \left( {\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)^{2} } }}} \right] \\ & \quad + E_{0} I\frac{{\partial^{4} \hat{v}}}{{\partial \hat{x}^{4} }} + E_{1} I\frac{{\partial^{\alpha } }}{{\partial \hat{t}^{\alpha}_{\textrm{RL+}}}} \left( {\frac{{\partial^{4} \hat{v}}}{{\partial \hat{x}^{4} }}} \right) + \left( {k_{1} \hat{v} - k_{2} \frac{{\partial^{2} \hat{v} }}{{\partial \hat{x}^{2} }}} \right) = 0 \\ \end{aligned} $$
(11)

where the intermediate variable \(\sigma_{\rm in}\) is

$$ \sigma_{{{\text{in}}}} = \left( {E_{0} + E_{1} \frac{{\partial^{\alpha } }}{{\partial t^{\alpha}_{\textrm{RL+}}}} } \right)\left[ {\sqrt {\left( {1 + \frac{{\partial \hat{u}}}{\partial x}} \right)^{2} { + }\left( {\frac{{\partial \hat{v}}}{\partial x}} \right)^{2} } - 1} \right] $$
(12)

and \(I\) denotes the area moment of inertia of the pipeline. According to the integration by parts, the boundary conditions are satisfied by

$$ \left. {\int\limits_{{\hat{t}_{1} }}^{{\hat{t}_{2} }} {\left[ {E_{0} I\frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}^{2} }} + E_{1} I\frac{{\partial^{\alpha } }}{\partial \hat{t}^{\alpha}_{\textrm{RL+}}} \left( {\frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}^{2} }}} \right)} \right]} \delta \left( {\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)} \right|_{0}^{L} {\text{d}}\hat{t} = 0$$
(13)
$$\int\limits^{\hat{t}_2}_{\hat{t}_1}{\left.{\left[\rho_{\textrm{e}}A_{\textrm{e}}\left(V^2+V\frac{\partial\hat{u}}{\partial\hat{t}}+V^2\frac{\partial\hat{u}}{\partial\hat{x}}\right) -\frac{\left(P+A_{\textrm{g}}\sigma_{\textrm{in}}\right)\left(1+\frac{\partial\hat{u}}{\partial\hat{x}}\right)}{\sqrt{\left(1+\frac{\partial\hat{u}}{\partial\hat{x}}\right)^2 +\left(\frac{\partial\hat{v}}{\partial\hat{x}}\right)^2}}\right]\delta(\hat{u})} \right|^L_0\textrm{d}\hat{t}}=0 $$
(14)
$$ \begin{aligned} & \int\limits_{{\hat{t_{1}} }}^{{\hat{t_{2}} }} {\Bigg[ {\rho _{{\text{e}}} A_{{\text{e}}} \left( {V\frac{{\partial \hat{v}}}{{\partial \hat{t}}} + V^{2} \frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right) + E_{0} I\frac{{\partial ^{3} \hat{v}}}{{\partial \hat{x}^{3} }} + E_{1} I\frac{{\partial ^{\alpha } }}{{\partial {\hat t}^{\alpha}_{\text{RL+}}}} \left( {\frac{{\partial ^{3} \hat{v}}}{{\partial \hat{x}^{3} }}} \right)}} \\ & \quad \left. {\left. { - \frac{{\left( {P + A_{{\text{g}}} \sigma _{{{\text{in}}}} } \right)\left( {1 + \frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)}}{{\sqrt {\left( {1 + \frac{{\partial \hat{u}}}{{\partial \hat{x}}}} \right)^{2} + \left( {\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)^{2} } }}} \right]\delta (\hat{v})} \right|_{0}^{L} {\text{d}}\hat{t} = 0 \\ \end{aligned} $$
(15)

2.2 Fractional-Order Dynamic Equation of Transverse Vibration

Considering that the nonlinear oscillation is a small but limited stretching problem, we neglect the coupled state between the axial and transverse motions and retain the lowest-order terms, and thus having \(\hat{u}\) = O(\(\hat{v}\) 2). Based on the author’s previous work [2], the terms \(\left[ {\left( {1 + {{\partial \hat{u}} \mathord{\left/ {\vphantom {{\partial \hat{u}} {\partial \hat{x}}}} \right. \kern-\nulldelimiterspace} {\partial \hat{x}}}} \right)^{2} + \left( {{{\partial \hat{v}} \mathord{\left/ {\vphantom {{\partial \hat{v}} {\partial \hat{x}}}} \right. \kern-\nulldelimiterspace} {\partial \hat{x}}}} \right)^{2} } \right]^{ - 1/2}\) and \(\sqrt {\left( {1 + {{\partial \hat{u}} \mathord{\left/ {\vphantom {{\partial \hat{u}} {\partial \hat{x}}}} \right. \kern-\nulldelimiterspace} {\partial \hat{x}}}} \right)^{2} + \left( {{{\partial \hat{v}} \mathord{\left/ {\vphantom {{\partial \hat{v}} {\partial \hat{x}}}} \right. \kern-\nulldelimiterspace} {\partial \hat{x}}}} \right)^{2} }\) are expanded into the Taylor series as

$$ \begin{aligned} & \left[ {\left( {1 + {{\partial \hat{u}} \mathord{\left/ {\vphantom {{\partial \hat{u}} {\partial \hat{x}}}} \right. \kern-\nulldelimiterspace} {\partial \hat{x}}}} \right)^{2} + \left( {{{\partial \hat{v}} \mathord{\left/ {\vphantom {{\partial \hat{v}} {\partial \hat{x}}}} \right. \kern-\nulldelimiterspace} {\partial \hat{x}}}} \right)^{2} } \right]^{ - 1/2} \\ & \quad = 1 - {{\partial \hat{u}} \mathord{\left/ {\vphantom {{\partial \hat{u}} {\partial \hat{x}}}} \right. \kern-\nulldelimiterspace} {\partial \hat{x}}} - \frac{1}{2}\left( {{{\partial \hat{v}} \mathord{\left/ {\vphantom {{\partial \hat{v}} {\partial \hat{x}}}} \right. \kern-\nulldelimiterspace} {\partial \hat{x}}}} \right)^{2} + O(3) \\ \end{aligned} $$
(16)
$$ \begin{aligned} & \sqrt {\left( {1 + {{\partial \hat{u}} \mathord{\left/ {\vphantom {{\partial \hat{u}} {\partial \hat{x}}}} \right. \kern-\nulldelimiterspace} {\partial \hat{x}}}} \right)^{2} + \left( {{{\partial \hat{v}} \mathord{\left/ {\vphantom {{\partial \hat{v}} {\partial \hat{x}}}} \right. \kern-\nulldelimiterspace} {\partial \hat{x}}}} \right)^{2} } \\ & \quad = 1 + {{\partial \hat{u}} \mathord{\left/ {\vphantom {{\partial \hat{u}} {\partial \hat{x}}}} \right. \kern-\nulldelimiterspace} {\partial \hat{x}}} + \frac{1}{2}\left( {{{\partial \hat{v}} \mathord{\left/ {\vphantom {{\partial \hat{v}} {\partial \hat{x}}}} \right. \kern-\nulldelimiterspace} {\partial \hat{x}}}} \right)^{2} + O(3) \\ \end{aligned} $$
(17)

where \(O(3)\) represents small quantities of the third or higher order. Substituting Eq. (17) into Eq. (12), one has

$$ \begin{aligned} \sigma_{{{\text{in}}}} = & E_{0} \left[ {\frac{{\partial \hat{u}}}{{\partial \hat{x}}} + \frac{1}{2}\left( {\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)^{2} } \right] \\ & \quad + E_{1} \frac{{\partial^{\alpha } }}{{\partial t^{\alpha}_{\textrm{RL+}}}} \left[ {\frac{{\partial \hat{u}}}{{\partial \hat{x}}} + \frac{1}{2}\left( {\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)^{2} } \right] \\ \end{aligned} $$
(18)

In Eq. (18), the viscosity coefficient is assumed as a higher-order small quantity in comparison with \(\hat{v}\), so that \(E_{1} = O(v^{2} )\). Considering that the initial axial tension \(P\) is a constant, substituting Eqs. (16) and (18) into Eqs. (10) and (11), and then neglecting \(O\left( {\hat{v}^{2} } \right)\) as well as other higher-order terms, one has

$$ \frac{\partial }{{\partial \hat{x}}}\left[ {\frac{1}{2}\left( {\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)^{2} + \frac{{\partial \hat{u}}}{{\partial \hat{x}}}} \right] = 0 $$
(19)
$$ \begin{aligned} & \rho_{{\text{e}}} A_{{\text{e}}} \left( {\frac{{\partial^{2} \hat{v}}}{{\partial \hat{t}^{2} }} + 2V\frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}\partial \hat{t}}} + \frac{{{\text{d}}V}}{{{\text{d}}\hat{t}}}\frac{{\partial \hat{v}}}{{\partial \hat{x}}} + V^{2} \frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}^{2} }}} \right) \\ & \quad + \rho_{{\text{g}}} A_{{\text{g}}} \frac{{\partial^{2} \hat{v}}}{{\partial \hat{t}^{2} }} - P\frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}^{2} }} + \left( {k_{1} \hat{v} - k_{2} \frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}^{2} }}} \right) \\ & \quad + E_{0} I\frac{{\partial^{4} \hat{v}}}{{\partial \hat{x}^{4} }} + E_{1} I\frac{{\partial^{\alpha } }}{{\partial \hat{t}^{\alpha}_{\textrm{RL+}}}} \left( {\frac{{\partial^{4} \hat{v}}}{{\partial \hat{x}^{4} }}} \right) \\ & \quad - E_{0} A_{{\text{g}}} \frac{\partial }{{\partial \hat{x}}}\left[ {\left( {\frac{1}{2}\left( {\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)^{2} + \frac{{\partial \hat{u}}}{{\partial \hat{x}}}} \right)\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right] = 0 \\ \end{aligned} $$
(20)

Integrating Eq. (19) twice with respect to \(\hat{x}\) and considering the boundary conditions (\(\hat{u} = 0\) at \(\hat{x} = 0\) and \(\hat{x} = L\)), one obtains

$$ \hat{u} = \frac{{\hat{x}}}{2L}\int\limits_{0}^{L} {\left( {\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)^{2} } {\text{d}}\hat{x} - \frac{1}{2}\int\limits_{0}^{{\hat{x}}} {\left( {\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)^{2} } {\text{d}}\hat{x} $$
(21)

Substituting Eq. (21) into Eq. (20), one gets

$$ \begin{aligned} & \rho_{{\text{e}}} A_{{\text{e}}} \left( {\frac{{\partial^{2} \hat{v}}}{{\partial \hat{t}^{2} }}} \right.\left. { + 2V\frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}\partial \hat{t}}} + \frac{{{\text{d}}V}}{{{\text{d}}\hat{t}}}\frac{{\partial \hat{v}}}{{\partial \hat{x}}} + V^{2} \frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}^{2} }}} \right) \\ & \quad + \rho_{{\text{g}}} A_{{\text{g}}} \frac{{\partial^{2} \hat{v}}}{{\partial \hat{t}^{2} }} - P\frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}^{2} }} + \left( {k_{1} \hat{v} - k_{2} \frac{{\partial^{2} \hat{v}}}{{\partial x^{2} }}} \right) \\ & \quad + E_{0} I\frac{{\partial^{4} \hat{v}}}{{\partial \hat{x}^{4} }} + E_{1} I\frac{{\partial^{\alpha } }}{{\partial \hat{t}^{\alpha}_{\textrm{RL+}}}} \left( {\frac{{\partial^{4} \hat{v}}}{{\partial \hat{x}^{4} }}} \right)\\ & - \frac{{E_{0} A_{g} }}{2L}\frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}^{2} }}\int\limits_{0}^{L} {\left( {\frac{{\partial \hat{v}}}{{\partial \hat{x}}}} \right)^{2} } {\text{d}}\hat{x} = 0 \end{aligned} $$
(22)

Equation (22) is a nonlinear, fractional-order dynamic equation governing the transverse motion of the viscoelastic pipes conveying pulsatile fluid resting on an elastic foundation. Considering a small but limited stretching motion, the boundary conditions can be degenerated to

$$ \begin{aligned} \hat{v}(0,\hat{t}) = & 0,\left. {\left[ {E_{0} I\frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}^{2} }} + E_{1} I\frac{{\partial^{\alpha } }}{{\partial \hat{t}^{\alpha}_{\textrm{RL+}}}} \left( {\frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}^{2} }}} \right)} \right]} \right|_{{\hat{x} = 0}} = 0 \\ \hat{v}(L,\hat{t}) = & 0,\left. {\left[ {E_{0} I\frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}^{2} }} + E_{1} I\frac{{\partial^{a} }}{{\partial \hat{t}^{\alpha}_{\textrm{RL+}}}} \left( {\frac{{\partial^{2} \hat{v}}}{{\partial \hat{x}^{2} }}} \right)} \right]} \right|_{{\hat{x} = L}} = 0 \\ \end{aligned} $$
(23)

Introducing the following dimensionless variables

$$ \begin{aligned} \xi = & \frac{{\hat{x}}}{L},w = \frac{{\hat{v}}}{L},\tau = \frac{{\hat{t}}}{{L^{2} }}\sqrt {\frac{{E_{0} I}}{{\rho_{\mathrm g} A_{\mathrm g} + \rho_{{\text{e}}} A_{{\text{e}}} }}} \\ \gamma = & VL\sqrt {\frac{{\rho_{{\text{e}}} A_{{\text{e}}} }}{{E_{0} I}}} ,\beta = \frac{{\rho_{{\text{e}}} A_{{\text{e}}} }}{{\rho_{{\text{g}}} A_{{\text{g}}} + \rho_{{\text{e}}} A_{{\text{e}}} }} \\ \kappa = & \frac{{PL^{2} }}{{E_{0} I}},\varepsilon \eta = \frac{{E_{1} }}{{E_{0} L^{2\alpha } }}\sqrt {\left( {\frac{{E_{0} I}}{{\rho_{\rm g} A_{\rm g} + \rho_{\rm e} A_{\rm e} }}} \right)^{\alpha } } \\ \varepsilon \chi = & \frac{{L^{2} A_{{\text{g}}} }}{I},\overline{k}_{1} = \frac{{k_{1} L^{4} }}{{E_{0} I}},\overline{k}_{2} = \frac{{k_{2} L^{2} }}{{E_{0} I}} \\ \end{aligned} $$
(24)

where \(\varepsilon\) is a small parameter, and substituting Eq. (24) into Eqs. (22) and (23), we have

$$ \begin{aligned} \frac{{\partial^{2} w}}{{\partial \tau^{2} }} + & 2\beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma \frac{{\partial^{2} w}}{\partial \xi \partial \tau } + \beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \frac{{{\text{d}}\gamma }}{{{\text{d}}\tau }}\frac{\partial w}{{\partial \xi }} \\ + & \left( {\gamma^{2} - \kappa } \right)\frac{{\partial^{2} w}}{{\partial \xi^{2} }} + \frac{{\partial^{4} w}}{{\partial \xi^{4} }} + \varepsilon \eta \frac{{\partial^{\alpha } }}{{\partial \tau^{\alpha}_{\textrm{RL+}}}} \left( {\frac{{\partial^{4} w}}{{\partial \xi^{4} }}} \right) \\ + & \overline{k}_{1} w - \overline{k}_{2} \frac{{\partial^{2} w}}{{\partial \xi^{2} }} = \frac{1}{2}\varepsilon \chi \frac{{\partial^{2} w}}{{\partial \xi^{2} }}\int_{0}^{1} {\left( {\frac{\partial w}{{\partial \xi }}} \right)^{2} } {\text{d}}\xi \\ \end{aligned} $$
(25)
$$ \begin{aligned} w(0,\tau ) = & 0,\left. {\left[ {\frac{{\partial^{2} w}}{{\partial \xi^{2} }} + \varepsilon \eta \frac{{\partial^{\alpha } }}{{\partial \tau^{\alpha}_{\textrm{RL+}}}} \left( {\frac{{\partial^{2} w}}{{\partial \xi^{2} }}} \right)} \right]} \right|_{\xi = 0} = 0 \\ w(1,\tau ) = & 0,\left. {\left[ {\frac{{\partial^{2} w}}{{\partial \xi^{2} }} + \varepsilon \eta \frac{{\partial^{\alpha } }}{{\partial \tau^{\alpha}_{\textrm{RL+}}}} \left( {\frac{{\partial^{2} w}}{{\partial \xi^{2} }}} \right)} \right]} \right|_{\xi = 1} = 0 \\ \end{aligned} $$
(26)

3 Stability in Parametric Resonances

3.1 Approximate Analysis

First, we discuss the stability in the parametric resonances. Neglecting the nonlinear term in Eq. (25), we have

$$ \begin{aligned} \frac{{\partial^{2} w}}{{\partial \tau^{2} }} + & 2\beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma \frac{{\partial^{2} w}}{\partial \xi \partial \tau } + \beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \frac{{{\text{d}}\gamma }}{{{\text{d}}\tau }}\frac{\partial w}{{\partial \xi }} \\ + & \left( {\gamma^{2} - \kappa } \right)\frac{{\partial^{2} w}}{{\partial \xi^{2} }} + \frac{{\partial^{4} w}}{{\partial \xi^{4} }} + \varepsilon \eta \frac{{\partial^{\alpha } }}{{\partial \tau^{\alpha}_{\textrm{RL+}}}} \left( {\frac{{\partial^{4} w}}{{\partial \xi^{4} }}} \right) \\ + & \overline{k}_{1} w - \overline{k}_{2} \frac{{\partial^{2} w}}{{\partial \xi^{2} }} = 0 \\ \end{aligned} $$
(27)

Assuming that the velocity is pulsatile and fluctuates harmonically near a constant mean speed \(\gamma_{0}\), one obtains

$$ \gamma = \gamma_{0} + \varepsilon \gamma_{1} \sin \varPhi \tau $$
(28)

where \(\gamma_{1}\) and \(\varPhi\), respectively, represent the amplitude and frequency of fluctuation. Substituting Eq. (28) into Eq. (27), we get

$$ \begin{aligned} \frac{{\partial^{2} w}}{{\partial \tau^{2} }} + & 2\beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{0} \frac{{\partial^{2} w}}{\partial \xi \partial \tau } + \left( {\gamma_{0}^{2} - \kappa } \right)\frac{{\partial^{2} w}}{{\partial \xi^{2} }} \\ + & \frac{{\partial^{4} w}}{{\partial \xi^{4} }} + \varepsilon \eta \frac{{\partial^{\alpha } }}{{\partial \tau^{\alpha}_{\textrm{RL+}}}} \left( {\frac{{\partial^{4} w}}{{\partial \xi^{4} }}} \right) \\ + & \overline{k}_{1} w - \overline{k}_{2} \frac{{\partial^{2} w}}{{\partial \xi^{2} }} = - 2\varepsilon \beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{1} \sin (\varPhi \tau )\frac{{\partial^{2} w}}{\partial \xi \partial \tau } \\ - & 2\varepsilon \gamma_{0} \gamma_{1} \sin (\varPhi \tau )\frac{{\partial^{2} w}}{{\partial \xi^{2} }} - \varepsilon \beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{1} \varPhi \cos \varPhi \tau \frac{\partial w}{{\partial \xi }} \\ \end{aligned} $$
(29)

Using the direct multi-scale method, we set the solution \(w(\xi ,\tau )\) as

$$ w(\xi ,\tau ) = w_{0} \left( {\xi ,T_{0} ,T_{1} } \right) + \varepsilon w_{1} \left( {\xi ,T_{0} ,T_{1} } \right) + \cdots $$
(30)

where \(w_{0}\) and \(w_{1}\) are the displacement functions at orders \(\varepsilon^{0}\) and \(\varepsilon\); \(T_{0} = \tau\) and \(T_{1} = \varepsilon \tau\) are the fast and slow time scales, respectively. Substituting Eq. (30) into Eq. (29), then equalizing the coefficients of \(\varepsilon^{0}\) and \(\varepsilon^{1}\), and neglecting the high-order terms, one obtains

$$ \begin{aligned} \varepsilon^{0} :\frac{{\partial^{2} w_{0} }}{{\partial T_{0}^{2} }} + & 2\beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{0} \frac{{\partial^{2} w_{0} }}{\partial \xi \partial \tau } + \left( {\gamma_{0}^{2} - \kappa } \right)\frac{{\partial^{2} w_{0} }}{{\partial \xi^{2} }} \\ + & \frac{{\partial^{4} w_{0} }}{{\partial \xi^{4} }} + \overline{k}_{1} w_{0} - \overline{k}_{2} \frac{{\partial^{2} w_{0} }}{{\partial \xi^{2} }} = 0 \\ \end{aligned} $$
(31)
$$ \begin{aligned} \varepsilon^{1} :\frac{{\partial^{2} w_{1} }}{{\partial T_{0}^{2} }} + & 2\beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{0} \frac{{\partial^{2} w_{1} }}{\partial \xi \partial \tau } + \left( {\gamma_{0}^{2} - \kappa } \right)\frac{{\partial^{2} w_{1} }}{{\partial \xi^{2} }} \\ + & \frac{{\partial^{4} w_{1} }}{{\partial \xi^{4} }} + \overline{k}_{1} w_{1} - \overline{k}_{2} \frac{{\partial^{2} w_{1} }}{{\partial \xi^{2} }} = - 2\frac{{\partial^{2} w_{0} }}{{\partial T_{0} \partial T_{1} }} \\ - & 2\beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{0} \frac{{\partial^{2} w_{0} }}{{\partial T_{1} \partial \xi }} - \eta \left( {\frac{\partial }{{\partial T_{0} }}} \right)_{\rm RL + }^{\alpha } \left( {\frac{{\partial^{4} w_{0} }}{{\partial \xi^{4} }}} \right) \\ - & 2\beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{1} \sin \left( {\varPhi T_{0} } \right)\frac{{\partial^{2} w_{0} }}{{\partial \xi \partial T_{0} }} - \beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{1} \frac{{\partial w_{0} }}{\partial \xi }\varPhi \cos \varPhi T_{0} \\ - & 2\gamma_{0} \gamma_{1} \sin \left( {\varPhi T_{0} } \right)\frac{{\partial^{2} w_{0} }}{{\partial \xi^{2} }} + {\text{CC}} + {\text{NST}} \\ \end{aligned} $$
(32)

where \({\text{CC}}\) and \({\text{NST}}\), respectively, denote the complex conjugate and non-secular terms. The solution to Eq. (31) can be expressed as

$$ \begin{aligned} w_{0} = & A_{n} \left( {T_{1} } \right){\mathcal{G}}_{n} (\xi ){\text{e}}^{{{\rm{i}}\omega_{n} {{T}}_{0} }} \\ & \quad + A_{m} \left( {T_{1} } \right){\mathcal{G}}_{m} (\xi ){\text{e}}^{{{\rm{i}}\omega_{m} {{T}}_{0} }} + {\text{CC}} \\ \end{aligned} $$
(33)

where \(\omega_{n}\) and \(\omega_{m}\) are, respectively, the nth and mth natural frequencies of the corresponding linear system; \({\mathcal{G}}_{n}\) and \({\mathcal{G}}_{m}\) are, respectively, the nth and mth complex model functions, which are determined by referring to [2].

The pulsation frequency of fluid is assumed as

$$ \varPhi = \omega_{n} + \omega_{m} + \varepsilon \delta $$
(34)

where \(\delta\) is a detuning parameter. When \(m \ne n\), the summation parametric resonance occurs. When \(m = n\), the principal parametric resonance occurs. Substituting Eqs. (33) and (34) into Eq. (32), and eliminating the secular terms, we have

$$ \begin{aligned} \frac{{{\text{d}}A_{n} }}{{{\text{d}}T_{1} }} + & \eta \varUpsilon_{nn} A_{n} + \gamma_{1} {\mathcal{L}}_{nm} \overline{A}_{m} {\mathrm e}^{{\mathrm i} \delta {{T}}_1} = 0 \\ \frac{{{\text{d}}A_{m} }}{{{\text{d}}T_{1} }} + & \eta \varUpsilon_{mm} A_{m} + \gamma_{1} {\mathcal{L}}_{mn} \overline{A}_{n} {\mathrm e}^{{\mathrm i} \delta {{T}}_1} = 0 \\ \end{aligned} $$
(35)

where

$$ \begin{aligned} \varUpsilon_{jj} = & \frac{{\left( {{\text{i}}\omega_{j} } \right)^{\alpha } \int_{0}^{1} {{\mathcal{G}}_{j}^{(4)} } \cdot \overline{{\mathcal{G}}}_{j} {\text{d}}\xi }}{{2\left( {{\text{i}}\omega_{j} \int_{0}^{1} {{\mathcal{G}}_{j} } \cdot \overline{{\mathcal{G}}}_{j} \text{d}\xi + \beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{0} \int_{0}^{1} {{\mathcal{G}}_{j}^{\prime } } \cdot \overline{{\mathcal{G}}}_{j} {\text{d}}\xi } \right)}} \\ & \quad (j = n,m) \\ \end{aligned} $$
(36)
$$ \begin{aligned} {\mathcal{L}}_{kl} = & - \frac{{\left( {\omega_{l} - \omega_{k} } \right)\beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \int_{0}^{1} {{\mathcal{G}}_{l}^{\prime } } \overline{{\mathcal{G}}}_{k} {\text{d}}\xi + 2{\text{i}}\gamma_{0} \int_{0}^{1} {\overline{{\mathcal{G}}}_{l}^{\prime \prime } } \overline{{\mathcal{G}}}_{k} {\text{d}}\xi }}{{4\left( {{\text{i}}\omega_{k} \int_{0}^{1} {{\mathcal{G}}_{k} } \cdot \overline{{\mathcal{G}}}_{k} {\text{d}}\xi + \beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{0} \int_{0}^{1} {{\mathcal{G}}_{k}^{\prime } } \overline{{\mathcal{G}}}_{k} {\text{d}}\xi } \right)}} \\ & \quad (k = n,m;\quad l = m,n) \\ \end{aligned} $$
(37)

Introducing the following transformations

$$ \begin{aligned} A_{n} \left( {T_{1} } \right) = & C_{n} \left( {T_{1} } \right) {\mathrm e}^{{\mathrm i} \delta {{T}}_{1}/2} \\ A_{m} \left( {T_{1} } \right) = & C_{m} \left( {T_{1} } \right){\mathrm e}^{{\mathrm i} \delta {{{T}}_1}{/2}} \\ \end{aligned} $$
(38)

and substituting Eq. (38) into Eq. (35), one has

$$ \begin{aligned} \frac{{{\text{d}}C_{n} }}{{{\text{d}}T_{1} }} + & {\text{i}}\frac{\delta }{2}C_{n} + \eta \varUpsilon_{nn} C_{n} + \gamma_{1} {\mathcal{L}}_{nm} \overline{C}_{m} = 0 \\ \frac{{{\text{d}}C_{m} }}{{{\text{d}}T_{1} }} + & {\text{i}}\frac{\delta }{2}C_{m} + \eta \varUpsilon_{mm} C_{m} + \gamma_{1} {\mathcal{L}}_{mn} \overline{C}_{n} = 0 \\ \end{aligned} $$
(39)

Let

$$ C_{n} = c_{n} {\text{e}}^{{\varsigma {{T}}_{1} }} ,\quad C_{m} = c_{m} {\text{e}}^{{\varsigma {{T}}_{1} }} $$
(40)

substituting Eq. (40) into Eq. (39), and setting the second resulting equation as the complex conjugate, we obtain

$$ \begin{aligned} c_{n}^{\prime } = & \left( { - \varsigma - \text{i}\frac{\delta }{2} - \eta \varUpsilon_{nn} } \right)c_{n} - \gamma_{1} {\mathcal{L}}_{nm} c_{m} \\ c_{m}^{\prime } = & - \gamma_{1} \overline{{\mathcal{L}}}_{mn} c_{n} + \left( { - \varsigma + \text{i}\frac{\delta }{2} - \eta \overline{\varUpsilon}_{mn} } \right)c_{m} \\ \end{aligned} $$
(41)

For a nontrivial solution (\(c_{n} \ne 0\), \(c_{m} \ne 0\)), the coefficient matrix must be equal to zero,

$$ \begin{aligned} \varsigma^{2} + & \eta \left( {\varUpsilon_{nn} + \overline{\varUpsilon}_{mm} } \right)\varsigma + \left( {\text{i}\frac{\delta }{2} + \eta \varUpsilon_{nn} } \right)\left( { - \text{i}\frac{\delta }{2} + \eta \overline{\varUpsilon}_{mm} } \right) \\ - & \gamma_{1}^{2} {\mathcal{L}}_{nm} \overline{{\mathcal{L}}}_{mn} = 0 \\ \end{aligned} $$
(42)

Introducing

$$ \begin{aligned} \varsigma = & \varsigma^{R} + \text{i}\varsigma^{I} ,\Upsilon_{jj} = \Upsilon_{jj}^{R} + \text{i}\Upsilon_{jj}^{I} \\ & \quad (j = n,m) \\ \end{aligned} $$
(43)

and substituting Eq. (43) into Eq. (42), then dividing the resulting equation into real and image parts, and then substituting \(\varsigma^{R} = 0\) into the resulting solutions and eliminating \(\varsigma^{I}\), one obtains

$$ \begin{aligned} & \left[ \frac{\delta }{2}\left( {\varUpsilon_{nn}^{R} - \varUpsilon_{mm}^{R} } \right) + \eta \left( {\varUpsilon_{nn}^{R} \varUpsilon_{mm}^{I} - \varUpsilon_{mm}^{R} \varUpsilon_{nn}^{I} } \right)\right.\\ &\left. + \frac{{\gamma_{1}^{2} }}{\eta }{\text{Im}} \left( {{\mathcal{L}}_{nm} \overline{{\mathcal{L}}}_{mn} } \right)\right]^{2} \\ & \quad - \left( {\varUpsilon_{nn}^{R} + \varUpsilon_{mm}^{R} } \right)\left( {\varUpsilon_{mm}^{I} - \varUpsilon_{nn}^{I} } \right)\left[ \frac{\delta }{2}\left( {\varUpsilon_{nn}^{R} - \varUpsilon_{mm}^{R} } \right)\right.\\ &\left. + \eta \left( {\varUpsilon_{nn}^{R} \varUpsilon_{mm}^{I} - \varUpsilon_{mm}^{R} \varUpsilon_{nn}^{I} } \right) + \frac{{\gamma_{1}^{2} }}{\eta }\left( {{\mathcal{L}}_{nm} \overline{{\mathcal{L}}}_{mn} } \right) \right] \\ & \quad + \left( {\varUpsilon_{nn}^{R} + \varUpsilon_{mm}^{R} } \right)^{2} \left[ \gamma_{1}^{2} {\text{Re}} \left( {{\mathcal{L}}_{nm} \overline{{\mathcal{L}}}_{mn} } \right)\right.\\ &\left. - \frac{{\delta^{2} }}{4} - \frac{\eta \delta }{2}\left( {\varUpsilon_{mm}^{I} + \varUpsilon_{nn}^{I} } \right) - \eta^{2} \left( {\varUpsilon_{nn}^{R} \varUpsilon_{mm}^{R} + \varUpsilon_{mm}^{I} \varUpsilon_{nn}^{I} } \right) \right] = 0 \\ \end{aligned} $$
(44)

For principal parametric resonance, the stability boundary is satisfied by

$$ 4\varUpsilon_{nn}^{{R^{2} }} \left[ {\gamma_{1}^{2} \left| {{\mathcal{L}}_{nm} } \right|^{2} - \frac{{\delta^{2} }}{4} - \eta \delta \varUpsilon_{nn}^{I} - \eta^{2} \left| {\varUpsilon_{nn} } \right|^{2} } \right] = 0 $$
(45)

3.2 Numerical Example

In this section, the stability boundaries in the summation and principal resonances of the first two modes for the fractional-order viscoelastic pipes conveying fluid will be numerically presented. In order to validate the correctness of the presented investigation, we compare the stability boundary in the first-order principal resonance of fractional damping, axially moving beams obtained by the presented method with that determined by the solution of Yang et al. [14], as shown in Fig. 2, where good agreement exhibits, indicating the effectiveness of the presented study. In the subsequent analysis, the fixed parameters are taken as: \(L = 1{\text{ m}},P = 1{\text{ N}}\), \(E_{0} = 2000 \, \text{MPa}\), and \(I = {5} \times {10}^{ - 10} {\text{ m}}^{4}\).

Fig. 2
figure 2

Comparison of the stability boundary in the first-order principal resonance of fractional damping, axially moving beams obtained by the presented method with that determined by Yang et al. [14] (\(\alpha = 0.5\))

Full size image

The influence of fractional order on stability boundaries for the first- and second-order principal resonances as well as the summation resonance is shown in Fig. 3. It illustrates that the growth of fractional order will broaden the width of instability threshold of \(\gamma_{1}\) for all types of parametric resonances, which means that the higher fractional order can conspicuously enhance the stability of the system. Furthermore, the variations of stability boundaries in the second-order principle and summation resonances are more remarkable with the change of fractional order. It is found from Figs. 4, 5 and 6 that as the viscoelastic coefficient, the spring stiffness and shear stiffness increase, the instability range of \(\left| \delta \right|\) for given \(\gamma_{1}\) diminishes for all cases of parametric resonances. In addition, the sensitivities of the summation resonance and the second-order principal resonance to these parameters are higher than that of the first-order harmonic resonance. Besides, the stability boundaries in the second-order principal resonance are less susceptible to the spring stiffness.

Fig. 3
figure 3

The stability boundaries vs. fractional order for various parametric resonances (\(\beta = 0.5,\quad \gamma_{0} = 0.8,\quad \kappa = 1,\quad \eta = 0.001,\quad \overline{k}_{1} = \overline{k}_{2} = 0\))

Full size image
Fig. 4
figure 4

The stability boundaries vs. viscoelastic coefficient for various parametric resonances (\(\beta = 0.5,\quad \gamma_{0} = 0.8,\quad \kappa = 1,\quad \alpha = 0.5,\quad \overline{k}_{1} = \overline{k}_{2} = 0\))

Full size image
Fig. 5
figure 5

The stability boundaries vs. spring stiffness for different parametric resonances (\(\alpha = 0.5,\quad \beta = 0.5,\quad \kappa = 1,\quad \eta = 0.001, \overline{k}_{2} = 0, \gamma_{0} = 0.8\))

Full size image
Fig. 6
figure 6

The stability boundaries vs. shear stiffness for different parametric resonances (\(\alpha = 0.5,\quad \beta = 0.5,\quad \kappa = 1,\quad \eta = 0.001, \overline{k}_{1} = 0, \gamma_{0} = 1.6\))

Full size image

4 The Analysis of Stability and Bifurcation

4.1 Application of Multiple-Scale Method

Considering the nonlinear governing equation and substituting Eqs. (28) and (30) into Eq. (25), then equalizing coefficient \(\varepsilon^{1}\) (while equalizing coefficient \(\varepsilon^{0}\) is related to Eq. (31)), one obtains

$$ \begin{aligned} \varepsilon^{1} :\frac{{\partial^{2} w_{1} }}{{\partial T_{0}^{2} }} + & 2\beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{0} \frac{{\partial^{2} w_{1} }}{\partial \xi \partial \tau } + \left( {\gamma_{0}^{2} - \kappa } \right)\frac{{\partial^{2} w_{1} }}{{\partial \xi^{2} }} + \frac{{\partial^{4} w_{1} }}{{\partial \xi^{4} }} \\ + & \overline{k}_{1} w_{1} - \overline{k}_{2} \frac{{\partial^{2} w_{1} }}{{\partial \xi^{2} }} = - 2\frac{{\partial^{2} w_{0} }}{{\partial T_{0} \partial T_{1} }} - 2\beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{0} \frac{{\partial^{2} w_{0} }}{{\partial T_{1} \partial \xi }} \\ - & \eta \left( {\frac{\partial }{{\partial T_{0} }}} \right)_{\rm RL + }^{\alpha } \left( {\frac{{\partial^{4} w_{0} }}{{\partial \xi^{4} }}} \right) - 2\beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{1} \sin \left( {\varPhi T_{0} } \right)\frac{{\partial^{2} w_{0} }}{{\partial \xi \partial T_{0} }} \\ - & \beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{1} \varPhi \cos \varPhi T_{0} \frac{{\partial w_{0} }}{\partial \xi } - 2\gamma_{0} \gamma_{1} \sin \left( {\varPhi T_{0} } \right)\frac{{\partial^{2} w_{0} }}{{\partial \xi^{2} }} \\ + & \frac{1}{2}\chi \frac{{\partial^{2} w_{0} }}{{\partial \xi^{2} }}\int_{0}^{1} {\left( {\frac{{\partial w_{0} }}{\partial \xi }} \right)^{2} } {\text{d}}\xi + {\text{CC}} + {\text{NST}} \\ \end{aligned} $$
(46)

The solution to Eq. (46) can be written as

$$ \begin{aligned} w_{0} \left( {\xi ,T_{0} ,T_{1} } \right) = & A_{n} \left( {T_{1} } \right){\mathcal{G}}_{n} (\xi ){\text{e}}^{{{\rm {i}}\omega_{n} {{T}}_{0} }} \\ & \quad + \overline{A}_{n} \left( {T_{1} } \right)\overline{{\mathcal{G}}}_{n} (\xi ){\text{e}}^{{ - {\rm{i}}\omega_{n} {{T}}_{0} }} \\ \end{aligned} $$
(47)

Considering the principal parametric resonance, the pulsation frequency \(\varPhi\) is described by

$$ \varPhi = 2\omega_{n} + \varepsilon \delta $$
(48)

Substituting Eqs. (47) and (48) into Eq. (46), the solvability condition is satisfied by

$$ \frac{{{\text{d}}A_{n} }}{{{\text{d}}T_{1} }} + g_{0} {\mathrm e}^{{\mathrm i} \delta {{T}}_1} \overline{A}_{n} - g_{4} A_{n}^{2} \overline{A}_{n} + g_{3} A_{n} = 0 $$
(49)

where

$$ g_{0} = - \frac{{\left( {\gamma_{1} \omega_{n} - \frac{1}{2}\gamma_{1} \varPhi } \right)\beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \int_{0}^{1} {{\overline{\mathcal{G}}}_{n}^{\prime } } \overline{{\mathcal{G}}}_{n} {\text{d}}\xi + \text{i}\gamma_{0} \gamma_{1} \int_{0}^{1} {\overline{{\mathcal{G}}}_{n}^{\prime \prime } } \overline{{\mathcal{G}}}_{n} {\text{d}}\xi }}{{2\left( {\text{i}\omega_{n} \int_{0}^{1} {{\mathcal{G}}_{n} } \overline{{\mathcal{G}}}_{n} {\text{d}}\xi + \beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{0} \int_{0}^{1} {{\mathcal{G}}_{n}^{\prime } } \overline{{\mathcal{G}}}_{n} {\text{d}}\xi } \right)}} $$
(50)
$$ g_{3} = \frac{{\eta \left( {\text{i}\omega_{n} } \right)^{\alpha } \int_{0}^{1} {{\mathcal{G}}_{n}^{(4)} } \overline{{\mathcal{G}}}_{n} {\text{d}}\xi }}{{2\left( {\text{i}\omega_{n} \int_{0}^{1} {{\mathcal{G}}_{n} } \overline{{\mathcal{G}}}_{n} {\text{d}}\xi + \beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{0} \int_{0}^{1} {{\mathcal{G}}_{n}^{\prime } } \overline{{\mathcal{G}}}_{n} {\text{d}}\xi } \right)}} $$
(51)
$$ g_{4} = \chi \frac{{2\int_{0}^{1} {{\mathcal{G}}_{n}^{\prime \prime } } \overline{{\mathcal{G}}}_{n} {\text{d}}\xi \int_{0}^{1} {{\mathcal{G}}_{n}^{\prime } } \overline{{\mathcal{G}}}_{n}^{\prime } {\text{d}}\xi + \int_{0}^{1} {\overline{{\mathcal{G}}}_{n}^{\prime \prime } } \overline{{\mathcal{G}}}_{n} {\text{d}}\xi \int_{0}^{1} {\left( {{\mathcal{G}}_{n}^{\prime } } \right)^{2} } {\text{d}}\xi }}{{4\left( {\text{i}\omega_{n} \int_{0}^{1} {{\mathcal{G}}_{n} } \overline{{\mathcal{G}}}_{n} {\text{d}}\xi + \beta^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \gamma_{0} \int_{0}^{1} {{\mathcal{G}}_{n}^{\prime } } \overline{{\mathcal{G}}}_{n} {\text{d}}\xi } \right)}} $$
(52)

4.2 Analytical Treatment

Taking the solution to Eq. (49) in the following polar form

$$ A_{n} \left( {T_{1} } \right) = \frac{1}{2}a_{n} \left( {T_{1} } \right){\text{e}}^{{{\rm{i}}\beta_{n} \left( {{T}_{1} } \right)}} $$
(53)

where \(a_{n} \left( {T_{1} } \right)\) and \(\beta_{n} \left( {T_{1} } \right)\), respectively, denote the vibration amplitude and phase position. According to the numerical results, \(g_{3}\) and \(g_{4}\) are the complex number and pure imaginary number, respectively, so

$$ g_{3} = g_{3R} + \text{i}g_{3I} ,g_{4} = \text{i}g_{4I} $$
(54)

where \(g_{iR}\) and \(g_{iI}\) are the real and imaginary parts, respectively \(\left( {i = 3,4} \right)\). Substituting Eqs. (53) and (54) into Eq. (49), then dividing the results into real and imaginary parts, we have

$$ a_{n}^{\prime } = a_{n} \left[ {\left( {g_{0R} \cos \gamma_{n} - g_{0I} \sin \gamma_{n} } \right) - g_{3R} } \right] $$
(55)
$$ \begin{aligned} a_{n} \gamma_{n}^{\prime } = & a_{n} \delta - 2a_{n} \left( {g_{0R} \sin \gamma_{n} + g_{0I} \cos \gamma_{n} } \right) \\ & \quad + 2a_{n} g_{3I} - \frac{1}{2}g_{4I} a_{n}^{3} \\ \end{aligned} $$
(56)

where

$$ g_{0} = g_{0R} + \text{i}g_{0I} ,\gamma_{n} = \delta T_{1} - 2\beta_{n} $$
(57)

For the steady-state response, \(a_{n}^{\prime }\) and \(\gamma_{n}^{\prime }\) are supposed to be zero. Then, eliminating \(\gamma_{n}^{{}}\) from Eqs. (55) and (56), we have

$$ \delta = \frac{1}{2}g_{4l} a_{n}^{2} - 2g_{3I} \pm 2\sqrt {g_{0R}^{2} + g_{0I}^{2} - g_{3R}^{2} } $$
(58)

In order to determine the stability of the trivial solution, the following transformation is introduced as

$$ a_{n} = \frac{1}{2}\left( {p_{n} + \text{i}q_{n} } \right)\text{e}^{\text{i}(\delta T/2)} $$
(59)

in which \(p_{n}\) and \(q_{n}\) represent unknown functions. Substituting Eqs. (58) and (59) into Eqs. (55) and (56), and dividing the resulting equations into real and imaginary parts, one obtains

$$ \begin{aligned} p_{n}^{\prime } = & - \left( {g_{0R} + g_{3R} } \right)p_{n} + \left( {\frac{\delta }{2} - g_{0I} + g_{3I} } \right)q_{n} \\ & \quad - \frac{1}{4}g_{4I} q_{n} \left( {p_{n}^{2} + q_{n}^{2} } \right) = F_{1} \left( {p_{n} ,q_{n} } \right) \\ \end{aligned} $$
(60)
$$ \begin{aligned} q_{n}^{\prime } = & \left( { - \frac{\delta }{2} - g_{0I} - g_{3I} } \right)p_{n} + \left( {g_{0R} - g_{3R} } \right)q_{n} \\ & \quad + \frac{1}{4}g_{4I} p_{n} \left( {p_{n}^{2} + q_{n}^{2} } \right) = F_{2} \left( {p_{n} ,q_{n} } \right) \\ \end{aligned} $$
(61)

Based on Eqs. (60) and (61), we construct the Jacobian matrix as follows

$$ \begin{aligned} & \left[ {\begin{array}{*{20}l} {\partial F_{1} /\partial p_{n} } \hfill & {\partial F_{1} /\partial q_{n} } \hfill \\ {\partial F_{2} /\partial p_{n} } \hfill & {\partial F_{2} /\partial p_{n} } \hfill \\ \end{array} } \right]_{{p_{n} ,q_{n} = 0}} \\ & \quad = \left[ {\begin{array}{*{20}c} { - \left( {g_{0R} + g_{3R} } \right)} & {\frac{\delta }{2} - g_{0I} + g_{3I} } \\ { - \frac{\delta }{2} - g_{0I} - g_{3I} } & {g_{0R} - g_{3R} } \\ \end{array} } \right] \\ \end{aligned} $$
(62)

Therefore, the eigenvalue of the matrix in Eq. (62) is derived by

$$ \begin{aligned} \lambda^{2} + & 2g_{3R} \lambda + \left( {g_{3R} + g_{0R} } \right)\left( {g_{3R} - g_{0R} } \right) \\ - & \left( {\frac{\delta }{2} - g_{0I} + g_{3I} } \right)\left( { - \frac{\delta }{2} - g_{0I} - g_{3I} } \right) = 0 \\ \end{aligned} $$
(63)

Making use of the Routh–Hurwitz criterion, it is confirmed that the system is stable when \(\left( {g_{3R} + g_{0R} } \right)\left( {g_{3R} - g_{0R} } \right) - \left( {\frac{\delta }{2} - g_{0I} + g_{3I} } \right)\left( { - \frac{\delta }{2} - g_{0I} - g_{3I} } \right) > 0\). Therefore, the stability conditions are satisfied by

$$ \begin{aligned} \delta < \delta_{1} = & - 2g_{3I} + 2\sqrt {\left( {2g_{0I}^{2} - g_{3R}^{2} } \right)} \\ \delta > \delta_{2} = & - 2g_{3I} - 2\sqrt {\left( {2g_{0I}^{2} - g_{3R}^{2} } \right)} \\ \end{aligned} $$
(64)

To confirm the stability of nontrivial solutions, the following equations are supposed to be determined according to Eqs. (55) and (56) under the condition that \(a_{n} \ne 0\)

$$ \begin{aligned} a_{n}^{\prime } = & - a_{n} \left[ {\left( {g_{0R} \cos \gamma_{n} - g_{0I} \sin \gamma_{n} } \right) + g_{3R} } \right] \\ = & G_{1} \left( {a_{n} ,\gamma_{n} } \right) \\ \end{aligned} $$
(65)
$$ \begin{aligned} \gamma_{n}^{\prime } = & \delta + 2\left( {g_{0R} \sin \gamma_{n} + g_{0I} \cos \gamma_{n} } \right) \\ & \quad + 2g_{3I} - \frac{1}{2}g_{4I} a_{n}^{2} = G_{2} \left( {a_{n} ,\gamma_{n} } \right) \\ \end{aligned} $$
(66)

From Eqs. (65) and (66), we construct the Jacobian matrix at (\(a_{0n} ,\gamma_{0n}\)) as follows

$$ \begin{aligned} & \left[ {\begin{array}{*{20}l} {\partial G_{1} /\partial p_{n} } \hfill & {\partial G_{1} /\partial q_{n} } \hfill \\ {\partial G_{2} /\partial p_{n} } \hfill & {\partial G_{2} /\partial p_{n} } \hfill \\ \end{array} } \right]_{{a_{n} = a_{0n} ,\gamma_{n} = \gamma_{0n} }} \\ & \quad = \left[ {\begin{array}{*{20}l} 0 \hfill & {\frac{1}{4}g_{4I} a_{0n}^{3} - a_{0n} g_{3I} - a_{0n} \delta } \hfill \\ { - 2g_{4I} a_{0n} } \hfill & { - 2g_{3R} } \hfill \\ \end{array} } \right] \\ \end{aligned} $$
(67)

The eigenvalue of the former matrix is calculated by

$$ \begin{aligned} & \lambda^{2} + 2g_{3R} \lambda + g_{4I} a_{0n} \\ & \quad \left( { - \frac{1}{2}g_{4I} a_{0n}^{2} + a_{0n} g_{3I} \mp a_{0n} \sqrt {g_{0R}^{2} + g_{0I}^{2} - g_{3R}^{2} } } \right) = 0 \\ \end{aligned} $$
(68)

Based on the eigenvalues of the characteristic equation (68), it is derived that the first and second nontrivial solutions are, respectively, stable and unstable using the Routh–Hurwitz criterion.

4.3 Numerical Result and Discussion

In this section, numerical examples are presented to disclose the bifurcation phenomena of the steady-state responses for different orders of the principal resonance. The variations of the amplitudes of steady-state vibration responses for the fractional-order viscoelastic pipes conveying fluid as a function of the detuning parameter in the first two principal parametric resonances are shown in Fig. 7. The solid and dot lines, respectively, represent the stable and unstable responses. There only exists the trivial solution, which is always stable for \(\delta < \delta_{1}\). When \(\delta\) increases to \(\delta_{1}\), the steady-state responses undergo the supercritical pitchfork bifurcation, the trivial solution becomes unstable, and there suddenly appears a stable nontrivial solution. For \(\delta = \delta_{2}\), the response amplitude experiences the subcritical pitchfork bifurcation, which is accompanied with the trivial solution with its stability again, and there occurs an unstable nontrivial solution. \(\left[ {\delta_{1} ,\delta_{2} } \right]\) is taken as the instability interval. Furthermore, the response amplitude predicted by the first-order principal resonance is higher than that predicted by the second-order case, which means that the lower-order responses have significant effects on the principal parametric resonances.

Fig. 7
figure 7

The steady-state responses in principle parametric resonances of the first and second modes (\(\eta = 0.001, \kappa = 1,\alpha = 0.5,\gamma_{0} = 1.6,\overline{k}_{1} = \overline{k}_{2} = 0,\quad \gamma_{1} = 0.5\))

Full size image

Figures 8 and 9, respectively, reveal the effects of fractional order and viscoelastic coefficient on the principal resonances of fluid-conveying pipes. It is found that the instability interval diminishes with the increases of fractional order and viscoelastic coefficient, especially for the second-order principal resonance. The reason is that the enhancing of damping makes the piping system more stable. The variations of the amplitudes of principal parametric resonances versus the detuning parameter for different spring and shear stiffnesses are, respectively, depicted in Figs. 10 and 11. It is shown that smaller spring and shear stiffnesses lead to larger instability interval, conspicuously for the first-order principal resonances.

Fig. 8
figure 8

The effect of fractional order on the steady-state responses in the principle parametric resonances (\(\eta = 0.001,\quad \kappa = 1,\quad \beta = 0.5,\quad \gamma_{0} = 1.6,\quad \overline{k}_{1} = \overline{k}_{2} = 0,\quad \gamma_{1} = 0.5\))

Full size image
Fig. 9
figure 9

Variations of steady-state responses in the principle parametric resonances as a function of detuning parameter for various viscoelastic coefficients (\(\beta = 0.5,\quad \kappa = 1,\quad \gamma_{0} = 1.6,\quad \alpha = 0.5,\quad \overline{k}_{1} = \overline{k}_{2} = 0,\quad \gamma_{1} = 0.5\))

Full size image
Fig. 10
figure 10

The steady-state responses in the principle parametric resonances as a function of detuning parameter for different values of spring stiffness (\(\eta = 0.001,\quad \kappa = 1,\quad \alpha = 0.5,\quad \gamma_{0} = 1.6,\quad \beta = 0.5,\quad \gamma_{1} = 0.5,\quad \overline{k}_{2} = 0\))

Full size image
Fig. 11
figure 11

The steady-state responses in the principle parametric resonances as a function of detuning parameter for different values of shear stiffness (\(\eta = 0.001,\quad \kappa = 1,\quad \alpha = 0.5,\quad \gamma_{0} = 1.6,\quad \beta = 0.5,\quad \gamma_{1} = 0.5,\quad \overline{k}_{1} = 0\))

Full size image

5 Conclusions

This paper is devoted to the study of stability and bifurcation in nonlinear parametric vibration of viscoelastic fluid-conveying pipes constituted by fractional-order viscoelastic material resting on the elastic foundation. The nonlinear, fractional-order dynamic equation governing the transverse motion is derived using Hamilton’s principle under the assumption of small but finite stretching. The direct multiple-scale method is employed to derive the solvability conditions of parametric resonances. Numerical solutions are presented to illustrate the influences of physical parameters, such as fractional order on the stability and bifurcation behaviors in different types of parametric resonances. The main conclusions are drawn as follows:

  1. 1.

    The instability regions of the pipes in all types of parametric resonances decrease with the increases of fractional order, viscoelastic coefficient, spring stiffness and shear stiffness.

  2. 2.

    For the steady-state responses of fractional viscoelastic pipes in nonlinear parametric vibration, there exist the trivial and nontrivial solutions. It is always stable (unstable) for the first-order (second-order) nontrivial solution. An instability interval of the detuning parameter is obtained when the trivial response is stable. Furthermore, with the increase of the detuning parameter, the system undergoes supercritical and subcritical pitchfork bifurcations.

  3. 3.

    For the nonlinear principal resonances, the fluid-conveying pipes with smaller fractional order, viscoelastic coefficient, spring stiffness and shear stiffness have a larger instability interval.