1 Introduction

Richard Feynman delivered a forward-looking speech in 1959, in which he made the famous saying [1]. “It would be interesting in surgery if you could swallow the surgeon”. In 1966, the movie “Fantastic Voyage” pictured a small submarine entering a blood vessel to remove blood clots based on Feynman’s envision. The realization of this science fiction has become possible recently owing to the progress in the past few decades in micro-robotics [2]. In 2016, the Nobel Prize Committee in Chemistry awarded Jen Pierre Sauvage, Fraser Stoddart, and Ben Feringa in molecular machine design and synthesis, which exhibited the momentous development of this research field [3]. The past two decades also have witnessed a rapid progress of small robotic equipment, which benefited from the advance of micro–nanotechnology and smart materials [4]. These small-scale devices have shown great potential in medical cargo delivery [5,6,7,8,9] and water remediation [10,11,12].

Soft and small robots have drawn widespread attention owing to their increased flexibility and adaptability as compared to the hard and large ones. The motion of these robots is reminiscent of that of natural micro-swimmers. Thus, a review of the biomechanics of microorganisms is essential to the inspiration of innovative artificial micro-swimmers because the development of the latter usually engages in finding a suitable propulsion and steering strategy [13]. In addition, an overview of the current achievements of artificial micro-swimmers and the corresponding propulsion strategies can foster further innovations. It is noteworthy that an efficient strategy can be a combination of two or even more different propulsion methods, for example, micro-swimmers may be driven by ultrasonic waves, but navigate and perform therapeutic functions in magnetic fields. In the last section of this article, the feasibility of such a mixed strategy will be explored based on a numerical model.

It is noted that there have been numerous review articles in the field of micro-swimmers. While the early articles summarized the studies of microorganisms, most of the recent ones focused on the actuation, manufacturing, and applications of artificial micro-swimmers. In 1976, James Lighthill’s classic lecture [14] gave an overview of biofluid-dynamics of flagellated microorganisms. In 1996, Lighthill reviewed previous studies of swimming dynamics and then developed his classical theories to helical propulsion [15]. In 2009, Lauga concluded the hydrodynamics of microorganisms in his famous review article [16], wherein he discussed an example of applying the theories of natural micro-swimmers to artificial ones. After that, he further reviewed the developments of the theories for microorganisms in 2015 [17] and 2016 [18]. Compared with the reviews on natural micro-swimmers, the review articles on artificial micro-robots are much more abundant due to the rapid growth of this field. For example, the physics of microparticles was reviewed in Refs. [19, 20] because of their potential use for drug-delivery applications, micro-robots driven by chemical, acoustic and magnetic powers were reviewed in Refs. [21,22,23,24,25,26,27,28], and more actuation methods and their potential medical applications were reviewed in Refs. [3, 25, 29,30,31,32]. However, the aforementioned review articles seldom discuss the relation between the theories of microorganisms and the dynamics of micro-robots. In 2009, Abbott et al. [33] analyzed the suitable external powers for artificial micro-swimmers with their review beginning from the theories of microorganisms. After 12 years, as many artificial micro-swimmers have been synthesized and tested, we feel the need to review the studies on both natural and artificial micro-swimmers again and to see how the theories arising from the observation and abstraction of the former can assist the design and optimization of the latter.

In Sect. 2, we summarize some historical achievements and describe theoretical perspectives on natural micro-swimmers. We first introduce some studies on the propulsive mechanisms of microorganisms and review the basic principles and the development of the prehension of them. After that, we focus on micro-swimmers with oscillating flagella, i.e., flagellated micro-swimmers, and elaborate theoretically on the dynamic behaviors of their locomotion. The progress of artificial micro-swimmer is reviewed in Sect. 3, wherein various driving mechanisms, such as catalytic propulsion, magnetic power, and acoustic actuation, are briefly discussed. In Sect. 4, we propose two actuation strategies to realize the steering of a flagellated micro-swimmer by virtue of a bar-joint model method: the first one employs double acoustic sources to break the symmetry of periodic motion to make the micro-swimmer turn; the second combines the acoustic and magnetic power, where magnetic torques generated via magnetic nanoparticles inside the head of a flagellated micro-swimmer are responsible for navigation. The final section concludes the inadequacies of the existing investigations and our perspective on future developments.

2 Overview of Natural Micro-swimmers

2.1 Observations of Microorganisms

We define a “swimmer” as a creature or an object that moves by periodically changing its body configuration. This type of swimmer in nature, if the size is sufficiently small to be invisible to the naked eye, is usually called a microorganism. The biological observation of microorganisms began with the invention of the optical microscope in the seventeenth century when Anthony van Leeuwenhoek first observed swimming bacteria [34]. Since then, people have discovered that our world is full of swimming microorganisms like sperms, bacteria, protozoa, and algae.

Fig. 1
figure 1

Sketches of microscopic swimmers. Reproduced with permission [51]. Copyright 2019, Wiley

Full size image

In accordance with the comprehensive review article [16], many tiny swimmers use one or more appendages to advance. The appendage can be a rather hard spiral that can rotate. For example, the motor organelles of Escherichia coli and Salmonella typhimurium are bacterial flagella, which consist of a rotating motor, a spiral filament, and a hook that connects the motor to the filament [35,36,37]. The diameter of the filament is around 20 nm with a 10-\(\upmu \hbox {m}\) contour length of helix [38]. Each cell usually has several flagella. When the motor rotates counterclockwise, the filaments are wrapped in a bundle and the cell is pushed forward at a speed of 25–35 \(\upmu \hbox {m/s}\) [39]. When the motor runs clockwise, the corresponding filaments get unbundled and undergo a “polymorphic” transformation, in which the helix’s chirality changes; and these transformations can change the direction of cell movement [38]. Aside from the helical filament, the appendage can also be a flexible filament that undergoes whip-like motion driven by molecular motors, such as the sperms of many species [40].

As shown in Fig. 1, swimming bacteria have various flagellar configurations. For example, F. crescentus has a single right-handed spiral filament that is driven by a rotating motor and can be turned in any direction. The motor rotates clockwise preferentially and drives the filament to make the body move forward [41]. When rotating counterclockwise, the filament pulls the body instead of pushing it. The motor of Rhodobacter sphaericus only rotates in one direction, but it stops periodically [42]. The flagellar filaments form a tight coil prior to a stop and then extend into a spiral at a stop. Besides, a fraction of bacteria do not have flagella, they can also move slowly by the sliding [43].

Eukaryotic flagella and cilia are much larger than bacterial flagella, with a diameter of about 200 nm and a complex internal structure [40]. The most common structure has a molecular motor or dynein which slides back and forth causing fluctuations that propagate along the flagella. The beat pattern and length between eukaryotic flagella and cilia are very different. For example, Chlamydomonas reinhardtii have both cilia and flagella. Cilia are short and have random or non-synchronized motions [40], while flagella’s motion has a clear pattern. During the power stroke, each flagellum stretches and bends at the bottom, which is a bit reminiscent of the motion of our arms in breaststroke. During the recovery stroke, the flagella regain their folding, causing less resistance underneath.

Flagellated microorganisms, such as sperms, are more researched in the biomechanics community because of the simplicity—often involving only one flagellum for propulsion—and the large size which simplifies observation. One can notice two kinds of flagellar wave motions, helical and planar, and two corresponding trajectories of organisms. When the flagella move in a helical way, the overall trajectory is, in general, a straight line, though there exists helical rotation around the line [44,45,46,47]. It is interesting that in the case of planar flagellar motion, the trajectory turns out to be circular rather than linear [44, 46,47,48,49]. Further analysis demonstrates that the greater is the asymmetry of the flagellar wave motions, the smaller is the radius of curvature [47]. This indicates the existence of a strong connection between the circular path and the asymmetric tail beating [50]. However, more detailed interpretations of the results of biological observation are requisite, especially for biomimicking. This is the reason why the investigations on the fundamental physics of natural micro-swimmers are emerging in an endless stream.

2.2 Swimming Mechanisms of Natural Micro-swimmers

2.2.1 Principles of Swimming on Microscale

The physics of microscopic swimming is distinct from the macroscopic one. The world of microorganisms is “a world with a very low Reynolds number (LRN)”, where inertia hardly plays a role and viscous damping is predominant. A general definition of the Reynolds number is Re \(= \rho UL/\eta \), where \(\rho \) is the fluid density, \(\eta \) the dynamic viscosity, U and L the characteristic velocity and the length of the fluid, respectively. The specific Reynolds numbers for natural micro-swimmers are worth comparison [52]. In water, with fluid parameters \(\rho \approx 1000\,\hbox {kg/m}^{{3}}\) and \(\eta \approx 10^{{-3}}\,\hbox {Pa}\,\hbox {s}\), a swimming bacterium, such as E. coli, with \(U \approx \) 10 \(\upmu \hbox {m/s}\) and \(L\approx 0.1\,\upmu \hbox {m}\) is of a Reynolds number Re \(\approx 10^{{-5}}{-}10^{{-4}}\). A human sperm with \(U \approx \) 200 \(\upmu \hbox {m/s}\) and \(L\approx 50\,\upmu \hbox {m}\) moves at Re \(\approx 10^{{-2}}\). Some larger ciliates, such as Paramecium, with \(U\approx \) 1 mm/s and \(L\approx \) 100 \(\upmu \hbox {m}\) can have Re \(\approx 0.1\) [53]. Furthermore, for some much larger swimmers, such as fishes, birds, and insects, it is noted that their Reynolds numbers are usually very large, and thus, their swimming strategies will not work on a small scale [52, 54,55,56,57,58].

The study of the mechanisms of locomotion at LRNs has a long history. In 1930, Ludwig [59] remarked that microorganisms that swing their stiff arms like paddles cannot perform net movements. After that, there have been some classical reviews from different perspectives. Some were from the perspective of fluid mechanics at LRNs [53, 60,61,62,63]; some focused on the general animal locomotion [64]; and others found incentive from the investigations of the biophysics and biology of cell mobility [37, 65]. In general, one can simplify the sophisticated hydrodynamic problems at LRNs by studying the limit case \(\hbox {Re} = 0\), in which the Navier–Stokes equations reduce to the Stokes equations [66]:

$$\begin{aligned} -\nabla p+\eta \nabla ^{2} \varvec{u}=0,\qquad \nabla \cdot \varvec{u}=0 \end{aligned}$$
(1)

where \(\varvec{u}\) is the velocity field and p the fluid pressure. Since the Stokes equations, i.e., Eq. (1), are linear, the classical linear superposition method is practicable to solve the fluid field and pressure distribution. The Green’s function to Stokes flow \(\varvec{G}(\varvec{x} -\varvec{x}')\) can be solved analytically [67] and is expressed as:

$$\begin{aligned} \varvec{G}\left( \varvec{x}-\varvec{x}' \right) =\frac{1}{8\pi \eta }\left( {\frac{{\varvec{I}}}{r}+\frac{{\varvec{rr}}}{r^{3}}} \right) ,\qquad {\varvec{r}}={\varvec{x}}-{\varvec{x}}',\qquad r=\left| {{\varvec{r}}} \right| \end{aligned}$$
(2)

where \(\varvec{I}\) is the identity tensor. Physically, \(\varvec{G}(\varvec{x} - \varvec{x}')\) represents the velocity field at position x due to a singularity force \(\varvec{F}\) acting on the fluid domain at position \(\varvec{x}'\), and that is \(\varvec{u}(\varvec{x} - \varvec{x}')= \varvec{G}(\varvec{x} - \varvec{x}')\cdot \varvec{F}\) based on the linear superposition. Evidently, the flow velocity is inversely proportional to the spatial distance, allowing the neglect of the far-field effect.

A significant solution to Eq. (1) in terms of locomotion is denominated as directional anisotropy. For example, for a 2D problem, if there is a force \(\varvec{F}= (F, 0)\) exerted at \(\varvec{x}' = (r, 0)\), one can derive the flow field \(\varvec{u}\) at \(\varvec{x}= (0, 0)\) as (\(F/4\pi \eta r, 0\)) from Eq. (2); if \(\varvec{F}= (0, F)\), \(\varvec{u}\) will then become \((0, F/8\pi \eta r)\). That is, for the same applied force, the flow velocity in the parallel direction is twice that in the perpendicular one (\(u_{{\vert \vert }} = 2u_{{\bot }})\). Alternatively, in order to obtain the same speed, it is imperative to apply a force twice greater in the vertical direction than in the parallel direction \((F_{{\bot }}= 2F_{{\vert \vert }})\). In this case, one may note that the corresponding drag coefficients \(\xi _{{\bot }}\) and \(\xi _{{\vert \vert }}\) (the coefficient of proportionality between the local force and velocity) are different (typically \(\xi _{{\bot }}/\xi _{{\vert \vert }} \approx 2\)), i.e., the drag anisotropy. As we mentioned earlier, most biological swimmers apply elongated appendages, known as flagella, to swim. These slender filaments can intuitively remind us that the fundamental principle of swimming through resistance-based thrust is just the use of drag anisotropy at LRNs. To illustrate these ideas, we can take an undulating filament as an example, as depicted in Fig. 2. An infinitesimal element of the filament can be considered as rigid, and it moves at a speed of u which is at an angle of \(\theta \) with regard to the whole filament. The horizontal component of the drag force \(f_{\mathrm {prop}}\), which propels the swimmer, is expressed as:

$$\begin{aligned} f_{\text{ prop }} =\left( {\xi _{\parallel } -\xi _{\bot } } \right) u\sin \theta \cos \theta \end{aligned}$$
(3)

With Eq. (3), only if the drag is anisotropic, e.g., \(\xi _{{\bot }}/\xi _{{\vert \vert }}\approx 2\), there will be a net force in the propulsive direction. This resistance-based thrust theory is the so-called resistive force theory (RFT).

The feasibility of resistance-based thrust mainly depends on the following two important physical inferences: first, due to the existence of drag anisotropy, a micro-swimmer can generate propulsion in the direction perpendicular to the local movement direction of the filament; second, although the filament deforms reciprocally, it can still produce a nonzero time-averaged propulsive force [68, 69]. It should be accentuated that the periodic deformation of the filament needs to meet certain conditions to produce a nonzero time-averaged force as per Purcell’s scallop theorem [62]. Purcell took scallops as an example for the explanation. Under LRNs, reciprocating motion cannot be used for movement; this is analogous to a scallop whose shell opens and closes alternatively while resulting in zero net displacement. Nevertheless, it is worth emphasizing that Purcell’s scallop theorem is strictly valid only under the limited condition that all relevant Reynolds numbers in the swimming problem are zero. Many recent studies have been devoted to a more general issue by involving the inertia theorem (the Euler regime), and it has been found that the continuity of transition from the Stokerian regime to the Euler regime is normally dependent on the spatial symmetry of the problem [70,71,72,73,74].

Fig. 2
figure 2

Understanding of drag anisotropy for slender filaments. Reproduced with permission [16]. Copyright 2009, IOP Publishing

Full size image
Fig. 3
figure 3

Numerical simulations of natural swimmers: a finite-element mesh around the sperm-like swimmer. Reproduced with permission [88]. It is an open-access article that does not need to seek permission; b onset of vorticity of the fish-like swimmer. Reproduced with permission [99]. Copyright 2016, Elsevier

Full size image

2.3 Swimming Mechanisms

Gray and Hancock [68] and Lighthill [14] developed the aforementioned RFT to describe LRN swimming problems. This theory has been used to model the propulsion generated by spermatozoa [75], small earthworms [76], Chlamydomonas reinhardtii [77], and some swimmers in a granular material [78]. However, the drag coefficients were usually revised to better fit the observations rather than using the theoretical values given in Refs. [14, 68]. This theory has also been employed to interpret the propulsion mechanisms of helical flagella [77, 79,80,81,82,83,84] and nanobots [33, 85,86,87]. However, the RFT ignores the long-range hydrodynamic interaction and only regards the viscous force acting on the immersed body as a function of local velocity [88]. Thus, the slender body theory (SBT) [14, 89] and its advanced version, the regularized Stokeslet theory [90], have been proposed to improve the accuracy of modeling. Intuitively, applying the SBT to a micro-swimmer with an undulating flagellum (whose propulsion is usually estimated by the RFT) can be straightforward. According to the derivation of SBT [91], appropriate geometries of flagella require two properties: a small thickness-to-length ratio and a small displacement amplitude (compared with the wavelength) of the flagellum undulating. Fortunately, within an acceptable range, the predictions of SBT have shown great conformance with experimental observation [92] and the results of more complicated models established by the finite-element method [93], the regularized Stokeslet method [94], the boundary element method [95], etc. The equivalence between the SBT and the RFT has also been explored. For example, for helical flagella, the RFT was employed to express the forces and torques in terms of the translational and rotational velocities [14, 68]. Reference [94] then experimentally examined the reliability of RFT and compared the experimental results with the theoretical predictions of the more involved SBT. All the theoretical predictions were in good accordance with experimental observations.

It is noted that these studies usually assumed idealized modeling conditions; for example, the filament model was assumed to be sufficiently slender, with a prescribed dynamic profile, and far from the boundary to satisfy the SBT. Subsequent research has mainly focused on the dynamics of swimmers close to the non-skid or free-skiing boundary adopting boundary element methods [96,97,98]. Rorai et al. [88] investigated the dynamics of a flagellated micro-swimmer, which is, more specifically, a sperm-like swimmer as demonstrated in Fig. 3a, in an infinite domain by finite element simulation, and an excellent agreement between the regularized Stokeslet method and the finite element simulation was demonstrated. However, their simulation did not consider a fully coupled fluid–structure interaction where the whole structure can have a rigid body motion, thus it became difficult to imitate the holistic locomotion of the swimmer. Curatolo et al. [99] proposed an approach to coupling the overall rigid body motion with the fluid–structure interaction for a fish-like swimmer by employing the automatic remeshing technique, as shown in Fig. 3b. However, they did not test the reliability of the RFT. Obviously, the geometry of the numerical model should be varied for a more comprehensive assessment of the analytical models, but the convergence of simulation can be a problem. Therefore, further research is needed to improve the numerical model of micro-swimmers through the fluid–structure interaction simulation.

According to the aforementioned Purcell’s scallop theorem [62], the breakthrough of the swimming problem for natural swimmers at LRNs is to find a non-reciprocal deformation approach. In 1951, Taylor found that a swimmer who deforms in a wave fashion can advance in an opposite direction to its traveling wave, and such a swimmer was named as Taylor’s swimming sheet thereafter [100]. Generally speaking, all the three-dimensional wave-like deformations can cause net propulsion, especially the spiral wave of flexible flagella [101]. It is noted that the original model of Taylor’s swimming sheet is infinitely long. Nevertheless, the Taylor sheet calculation is of great importance because it can be extended to finite objects. For example, to mimic the movement of ciliates such as opal and paramecium, Lighthill introduced an “envelope model”, where a traveling surface wave was exploited to simulate the swinging cilia embedded in the cell [102,103,104]. It is worth noting that the influence of inertia can be directly considered in the sheet calculation. In addition, it has been shown that if the flow separation is ignored, the swimming speed decreases with the increase of Reynolds number, and the asymptotic value at high Reynolds number is half of Taylor’s result [105, 106]. When the Reynolds number is zero and the waveform is prescribed, the nearby rigid wall, if exists, can increase the swimming speed if the gap between them is reduced [105].

2.3.1 Dynamic Behaviors of Flagellated Micro-swimmers

Inspired by Taylor’s swimming sheet, one may have an intuitive understanding of the general problem of the self-propelled motion of flagellated swimmers at LRNs. For such micro-swimmers, their dynamic behaviors mainly arise from the interplay between the internal and external forces acting on them. Internal forces are normally from elastic properties and can resist the bending of flagella and ensure the inextensibility of their bodies, while external forces are mainly due to the fluid viscosity (which can be estimated from the RFT or the SBT) that can cause deformations of flagella and hence non-trivial dynamic behaviors. In an earlier study, Machin [107] found that the amplitude of the bending wave decayed exponentially with the length of a flagellum due to the fact that the LRN corresponds to overdamping. However, Machin scrutinized the fact that the swing amplitude of the sperm flagella did not attenuate but gradually increased with the distance from the head. Therefore, he concluded that in order to be consistent with experimental observations, there must be an internal actuation torque distribution along flagella, which turns out to be actin filaments afterward. Their study lays the foundation for many subsequent fluid–structure interaction problems at LRN [107,108,109,110,111,112,113,114,115] and has been further extended to the estimation of the actin filament’s persistence length [116, 117]. Wiggins et al. [109] followed Machin’s work and established the so-called hyper-diffusion equation with boundary conditions in terms of the normal drag coefficient \(\xi _{{\bot }}\) and the bending modulus EI for a flagellum with constant cross section, which is given by:

$$\begin{aligned} \left\{ {{\begin{array}{*{20}l} \xi _{\bot } \left( {{\partial y} \big / {\partial t}} \right) =-EI\left( {{\partial ^{4}y} \big / {\partial x^{4}}} \right) , 0\le x\le L\\ {\text{ at }}\,x=0{:}\,y=y_{0} \sin \omega t, y_{x} =\theta _{0} \sin \omega t \\ {\text{ at }}\,x=L{:}\,{\partial ^{2}y} \big / {\partial x^{2}}=0, {\partial ^{3}y} \big / {\partial x^{3}}=0 \\ \end{array} }} \right. \end{aligned}$$
(4)

where y is the transverse displacement of the flagellum with length L and is a function of time t and longitudinal coordinate \(x; y_{{0}}\) and \(\theta _{{0}}\) are the translational and rotational amplitudes of the wiggly head; and \(\omega \) is the angular frequency. Equation (4) can be solved analytically by assuming y as the following:

$$\begin{aligned} y\left( {x,t} \right) =\sum \limits _{n=1}^4 {C_{n} \mathrm{e}^{\lambda _{n} x}} \mathrm{e}^{-\mathrm{i}\omega t} \end{aligned}$$
(5)

where the four characteristic roots \(\lambda _{n}\) are determined by

$$\begin{aligned} \lambda _{n} =\root 4 \of {\frac{i\omega \xi _{\bot } }{EI}}\quad ,n=1,2,3,4 \end{aligned}$$
(6)

It can be seen that Eq. (5) conforms to the above-mentioned Machin’s solution [107], i.e., the fluctuation amplitude decays exponentially along the flagellum. Wiggins et al. [109] calculated the propulsive force by integrating the projected elastic force density along the flagellum because there was no other external force exerted on it. The expression of the normal elastic force \(f_{e}\) is:

$$\begin{aligned} f_{e} =EI\left( {{\partial ^{2}\kappa _{e} } \big / {\partial s^{2}}+\kappa _{e}^{3}/2} \right) \end{aligned}$$
(7)

where \(\kappa _{e}\) denotes the curvature of the flagellum at the arc coordinate s. By integrating \(f_{e}\) over the whole length L, the time-averaged propulsive force \(\bar{{F}}\) for the case of the translational boundary condition (i.e., \(\theta _{0}= 0\)) is expressed as:

$$\begin{aligned} \bar{{F}}=\frac{1}{2}y_{0}^{2}\xi _{\bot } \omega {Y}\left( {S_{p} } \right) \end{aligned}$$
(8)

where Y is a function of the sperm number \(S_{p}\) and they are both dimensionless. The relation between Y and \(S_{p}\) is exhibited in Fig. 4. For the case of pivoting prosthesis (i.e., \(y_{0} = 0\)), \(\bar{{F}}\) has a similar expression in terms of \(Y_{\mathrm {p}}\), which is also a function of the sperm number \(S_{p}\) as shown in Fig. 4. It is noted that based on Eq. (6), the real part of the characteristic root \(\root 4 \of {{\omega \xi _{\bot } } \big / {EI}}\) is of great significance and will influence the dynamic behavior of a sperm-like micro-swimmer. In fact, \(S_{p}\) is defined as \(L\root 4 \of {{\omega \xi _{\bot } } \big / {EI}}\), which is the most popular non-dimensional parameter used in micro-swimmer studies [107, 109, 118, 119] because it indicates the interplay between viscous and elastic forces on the flagellum.

In addition, the computed propulsive force based on the head movement has also been experimentally verified [112]. The propulsive force for a variable cross-section flagellum is proposed by Singh et al. [120]. One can first obtain the elastic force as:

$$\begin{aligned} f_{e} =EI\kappa _{e}^{3}-EI\left( {{\partial ^{2}\kappa _{e} } \big / {\partial s^{2}}} \right) -2E\left( {{\partial I} \big / {\partial s}} \right) \left( {{\partial \kappa _{e} } \big / {\partial s}} \right) -E\left( {{\partial ^{2}I} \big / {\partial s^{2}}} \right) \kappa _{e} \end{aligned}$$
(9)

where EI is a function of the arc length s. The propulsive force can then be estimated by integrating \(f_{e}\). If the diameter of the cross section varies linearly, one can first derive the analytical solution of the equation of motion and then calculate the propulsive force by the RFT [119]. When such a micro-swimmer is placed in a fluid field, the effects of external forces, fluid viscous stresses, and internal bending moments can lead to complex dynamic deformations of the flagellum, which successively influences the swimming properties of the micro-swimmer. There have been many experimental and theoretical studies on the dynamics of such swimmers with regard to different sorts of microscale flows, such as extensional flows [121,122,123], vortex arrays [124,125,126], simple shear flow [127,128,129,130,131,132], pressure-driven channel flows [133], and other micro-fluidic flows [134, 135]. Some investigations have involved more complicated external or internal forces, such as forces at the ends of filaments [109, 136], two-body interactions [137], internal actuation [113, 138, 139], and self-attraction due to capillary interactions [140].

Fig. 4
figure 4

Scaling functions Y and \({Y}_{\mathrm {p}}\) for propulsive force versus the sperm number \(S_{\mathrm{p}}\) (which equals \(L/l_{\bar{{v}}})\). Dotted (Y) and solid lines \(({Y}_{\mathrm {p}})\) indicate functions for the translational and pivoting prosthesis, respectively. Adapted with permission [109]. Copyright 1998, American Physical Society

Full size image

2.4 Summary of Natural Micro-swimmers

In this chapter, we have recapitulated the historical studies and theoretical perspectives on natural micro-swimmers such as sperms, bacteria, protozoa, and algae. They use one or more appendages to advance, which can be relatively hard spirals that can rotate or flexible filaments that undergo whip-like motion. The fundamental principle of swimming through resistance-based thrust is the use of drag anisotropy at LRNs. RFT, SBT, and finite element simulations have also been developed to address more details of hydrodynamics. In short, a periodic non-reciprocal deformation that can generate net propulsion arises from the interplay between the internal and external forces acting on each of the appendages, which has been clearly unveiled in modeling of flagellated micro-swimmers.

3 Overview of Artificial Micro-swimmers

3.1 Experimental Achievements of Artificial Micro-swimmers

3.1.1 Self-propulsion Approach

Artificial micro-swimmers or micro-robots swim in a low-Reynolds-number regime just like microorganisms, requiring swimming strategies that are distinct from those of macroscale swimmers. Researchers have proposed various propulsion mechanisms, many of which are biomimetic, to drive and control artificial micro-swimmers remotely. It is noted that some mechanisms are not biomimicking; however, as they can move microdevices in the fluid, the latter were also called micro-swimmers. In this sense, the concept of swimming has been expanded to describe all artificial microdevices propelled in the fluid. They are generally classified into three categories: self-propulsion, external propulsion, and a combination of them [30].

The foundation of self-propulsion approach is energy conversion. If there are local chemical reactions at the surface of a nano- or micro-swimmer, the chemical energy can be converted into kinetic energy. This energy transformation can eventually bring about the locomotion of artificial swimmers [22, 141,142,143,144,145]. The first fully artificial self-propelled nano-engine was synthesized by Paxton et al. [146] in 2004 and then followed by Fournier-Bidoz et al. [147] Motivated by these achievements, some synthetic self-propelled micro-motors driven by catalytic reactions have been proposed [148,149,150,151]. While these autonomous swimmers can move at the speeds close to 1.5 cm/s, they are not steerable. As a result, the application of these self-propelled micro-swimmers normally limits to imprecise manipulations, which makes them incompetent for medical applications. To accurately control the movement of a swimmer, one may consider using field-propulsion rather than self-propulsion, and the field(s) can be magnetic, acoustic, photonic, thermal, or any combination of them [142, 152, 153].

3.1.2 Magnetic Actuation

The most renowned micro-robots actuated by rotating magnetic fields are helical swimmers [154, 155]. In 2007, Bell et al. [154] first reported a spiral-shaped magnetic swimmer that had a magnetic head and a spiral semiconductor tail made of a thin GaAs bilayer film as shown in Fig. 5a. This swimmer is an artificial duplicate of bacteria in terms of not only the dimensions but also the propulsion mechanism. Then, in 2012, Schuerle et al. reported a process to fabricate these magnetic swimmers by coating self-assembled phospholipidic helices with a magnetic CoNiReP alloy using electroless deposition [156]. After that, Gao et al. [157] developed a biotemplate technique to manufacture magnetic micro-robots through helical plant vessels in 2014. Recently, Nelson’s team has proposed soft micro-swimmers composed of stimuli-responsive hydrogel bilayers [158,159,160], where the layer of poly-N-isopropylacrylamide (PolyNIPAM) is thermally responsive. The blending of magnetic nanoparticles and PolyNIPAM enables the hydrogel to move under magnetic manipulations. Moreover, by exploiting magnetic fields or near-infrared radiation, such magnetic nanoparticles can also generate heat, which is able to deform the temperature-sensitive PolyNIPAM layer. Magnetic nanoparticles can also be exploited to control the rolling direction of the hydrogel and produce magnetic shape anisotropy through appropriate design. These flexible micro-swimmers can adapt the surrounding environment and be actuated by rotating magnetic fields (direction changes with time), revealing the prospects in medical applications [160].

Another widely applied magnetic actuation method is the oscillating magnetic field (intensity changes with time) which is usually perpendicular to the trajectory of the swimmer. Dreyfus et al. [118] fabricated a magnetic micro-swimmer which had a flexible filament composed of DNA-linked chains of paramagnetic colloidal beads and investigated its behaviors under the actuation of oscillating magnetic field. Misra and co-workers demonstrated a sperm-like magnetic micro-swimmer composed of an ellipsoidal CoNi head and a soft flagellum made of SU-8 [161]. The on–off mechanism was also employed to actuate and control artificial micro-swimmers [162]. As shown in Fig. 5b, the repeated switch between “on” and “off” can generate a planar oscillating magnetic field. The basic driving principle of these micro-robots is based on the “dual-mass-spring system” which consists of four main components: a gold head, two nickel segments as the body, and a gold tail. These segments are connected by three springs, which are made of flexible silver, as demonstrated in Fig. 5b. When the magnetic field is “on”, the micro-swimmer is bent due to the magnetic forces on each segment. When “off”, the springs tend to restore the linkage to the original configuration. The transition during the “on/off” switching of the magnetic field brings about various instant configurations. When the magnetic field oscillates near the resonant frequency of the swimmer, the dynamic response of the micro-swimmer can result in a net displacement.

Fig. 5
figure 5

Some examples of magnetic micro-swimmers: a artificial flagellum fabricated from a self-rolled semiconductor. Reproduced with permission [21]. Copyright 2009, AIP Publishing; b magnetic propulsion of an artificial nano-fish using a planar oscillating magnetic field. Reproduced with permission [162]. Copyright 2016, Wiley

Full size image

3.1.3 Acoustic Actuation

Recently, acoustic actuation has been a considerably prevailing driving strategy for generating substantial propulsive force and has attracted much attention in terms of medical applications and lab-on-chip devices. Nevertheless, in consideration of its superb biocompatibility, the mechanisms of acoustic propulsion and precise manipulation deserve deep exploration.

As exhibited in Fig. 6a, some early acoustic artificial nano-swimmers are designed as geometrically asymmetric and composed of rigid metallic nano-rods [163], which can be suspended by an acoustic standing wave. Such an acoustic field, in which a series of nodes and antinodes are established from the bottom to the top, can be generated by the cooperation of a piezo-transducer and a reflector. These nodes are also the extreme points of the acoustic pressure of the standing wave. If the compressibility and the density of a nano-swimmer are greater than those of its surrounding fluid, the swimmer will be propelled toward the nodes. Wang et al. [163, 164] found that the metallic nano-rods could achieve a speed of around 200 \(\upmu \hbox {m/s}\). Moreover, they discovered that these nano-rods exhibited striking interactions between nano-rods. The manipulation of an acoustic nano-swimmer was realized via incorporating Ni into the electrochemically grown nano-rods [165, 166], but it required to import an external magnetic field with a strength of 40–50 mT to steer the nano-rods, which was too strong to be implemented in bioapplications. Although acoustic-based artificial swimmers have been considered feasible in various medical circumstances, further studies are required to determine their driving mechanism and to predict their dynamic behaviors in biological environment.

Another type of acoustically propelled device employs the resonant behavior of microscale trapped bubbles within polyethylene-glycol-based cavities under acoustic actuation [167, 168]. The trapped bubble will oscillate if it is subject to a long-wavelength acoustic wave, especially when the wavelength is much larger than the bubble diameter. Based on this principle, some micro-swimmers embedded with bubbles of different sizes have been designed to navigate under acoustic actuation [167, 168]. However, the controllability is poor due to the limitations of coupling between bubbles and the finite resonant-region [167]. Recently, Ahmed et al. developed a hybrid acousto-magnetic soft micro-robot [169]. As shown in Fig. 6b, their design includes an acoustic bubble at the center of the swimmer, and some superparamagnetic particles are carefully aligned along the length of the swimmer within a polymer matrix. Significant propulsive forces generated by these bubble-based swimmers are demonstrated, and the capability of swimming in viscous fluids makes practical applications more promising. However, the air bubbles can only remain stable for a few hours. Bubble-based swimmers for the next generation require more robust bubbles, and this may be achieved via polymeric coating technique.

We have mentioned in Sect. 2 that many microorganisms swim based on the flagellum, which could be a helix that rotates for propulsion or a flexible filament that wiggles for locomotion [16]. By mimicking the latter, Ahmed et al. [170] demonstrated the first flagellated artificial micro-swimmer propelled by acoustically actuated flagellum motion in 2016. They have shown that the flagellated micro-swimmer can be ten times faster than those propelled by acoustic streaming (i.e., without flagella). In the next year, as shown in Fig. 6c, Kaynak et al. [171] synthesized a larger sperm-like swimmer (\(\sim 180 \times 60\,\upmu \hbox {m}\)) that can achieve the terminal speed of 1.2 mm/s. These two experimental studies suggest that an artificial micro-swimmer with high motility must have one or several flagella; however, the mechanism of acoustic propulsion was not apparently elaborated therein. To comprehend it, Liu et al. [119] later proposed a driving mechanism based on head oscillation, which will be discussed in detail in Sect. 3.2.

3.1.4 Other Actuation Strategies

The possibility of employing optical and thermal energies for micro-swimmer actuation has also been explored. The first optically actuated micro-swimmer was fabricated by Jiang et al. [172], in which micro-swimmers were coated with a thin layer of Au onto silica hemispheres. This layer can absorb light and then generate a local temperature gradient which propels the micro-swimmers by thermophoresis. The further optical micro-swimmers exploit photocatalysis in which the propulsion arises from the light-induced catalytic reaction [173]. When the photocatalytic part of a micro-swimmer in a solution is illuminated, electron–hole pairs are generated by the photons. These holes and electrons are distributed on the surface of the photocatalytic material where they can react with the surrounding solution, and meanwhile, byproducts will be produced. This mechanism is similar to the conventional chemically catalytic propulsion strategy [22]. The collective behavior under light illumination has also been noted. For example, Ibele et al. demonstrated the collective behavior of micro-sized AgCl particles exposed to ultraviolet light in deionized water [174, 175].

Due to the difficulty of heat transfer, the strategy of heat-actuated micro- or nano-motors has seldom been reported. One possible way of employing heat to propel micro-swimmers is to incorporate an array of micro-heaters, which has been used in the study of a micro-swimmer consisting of shape memory alloy (SMA) components. During heating, the deformation of SMA parts can actuate the micro-robot [176,177,178]. The attempt of propelling micro-objects, such as bubbles and droplets, by the temperature gradient technique has also been reported [179, 180].

In general, combinations of some of the aforementioned methods may enhance the propulsion and manipulation of small-scale swimmers. Sitti and Schmidt et al. have recently proposed the application of bioengineered and biohybrid bacteria in drug delivery systems [181, 182]. We can also find several realizations of micro-swimmers that use magnetic power for control purposes (rather than for propulsion), for example, the micromotors that are self-driven in hydrogen peroxide (\(\hbox {H}_{{2}}\hbox {O}_{{2}})\) solutions but magnetically navigated [183, 184]. Our study shown in Sect. 4 can be another example of the combination of acoustic and magnetic strategies.

We have introduced many actuation strategies so far. To have a comparison of them, the representative references are summarized in Table 1. One may notice that the characteristic velocities and dimensions vary remarkably from one actuation mechanism to another. Among them, acoustic bubble oscillation provides the highest speed, but the micro-swimmers propelled through acoustic flagellum whipping probably have the most balanced combination of speed, dimension, and easiness of manufacturing.

Fig. 6
figure 6

Some examples of acoustic micro-swimmers: a schematics of the experimental setup and the acoustic propulsion of the nano-rods. Reproduced with permission [163]. Copyright 2012, American Chemical Society, b schematic illustrates the swimming mechanism of the acousto-magnetic soft micro-robot. Reproduced with permission [169]. Copyright 2017, Wiley, c the experimental observation of flagellated micro-swimmers’ directional movement. Reproduced with permission [171]. Copyright 2017, Royal Society of Chemistry

Full size image
Table 1 Comparison of different actuation mechanisms
Full size table
Fig. 7
figure 7

Three examples of theoretical studies of artificial micro-swimmers: a schematic of motion of a Pt–Au nano-rod driven by the catalytic decomposition of hydrogen peroxide. Reproduced with permission [22]. Copyright 2015, Wiley; b a detailed image of an acoustic bubble actuator with the middle part removed, and the dark cylinder in the tube being the air bubble. Reproduced with permission [190]. Copyright 2006, IOP Publishing; c the idealized model of a sperm-like artificial micro-swimmer. Reproduced with permission [119]. Copyright 2020, IOP Publishing

Full size image

3.2 Theoretical Studies of Artificial Micro-swimmers

Most of the basic mechanisms of propulsion strategies have been reviewed in the above context. In this section, we briefly emphasize the theoretical foundation of different propulsion strategies.

Micro-swimmers with bubbles have been widely studied. It has been unveiled that the driving forces, arising from the bubble oscillation, can be induced by \(\hbox {H}_{\mathrm {2}}\hbox {O}_{\mathrm {2}}\) decomposition. The bubble-based micro-swimmer introduced in [147] has a nickel segment acting as the catalyst for \(\hbox {H}_{{2}}\hbox {O}_{{2}}\) decomposition, i.e.,

$$\begin{aligned} 2\text{ H}_{2} \text{ O}_{2} \left( l \right) =\text{ O}_{2} \left( g \right) +2\text{ H}_{2} \text{ O }\left( l \right) \end{aligned}$$
(10)

Alternatively, if the \(\hbox {O}_{{2}}\) gas generated based on Eq. (10) can be forced to flow along a prescribed direction, a micro-swimmer can also be propelled. This is the case of Pt-Au bimetallic nano-rods [149], as demonstrated in Fig. 7a, which utilizes the difference in the catalytic effects of these two metals. On the Pt surface, oxidation occurs and \(\hbox {O}_{{2}}\) is produced. The electrons flow along the metallic nano-rod toward the Au side where reduction occurs. In this case, \(\hbox {O}_{{2}}\) is pushed toward the Au side because of the proton gradient (\(\hbox {H}^{{+}}\) ions are produced on the Pt side and consumed on the Au side), and the bimetallic nano-rod moves in the opposite direction.

Magnetic power is one of the most often employed propulsive strategies for artificial micro-swimmers due in large part to its prospect of remote and wireless operation, directional movement, and biocompatibility. In a magnetic field \(\varvec{B}\), a permanent magnet or a magnetic dipole with the magnetization of \(\varvec{m}\) is subjected to a magnetic torque \(\tau \), which is the cross product of \(\varvec{m}\) and \(\varvec{B}\), expressed as [187, 188]:

$$\begin{aligned} {\varvec{\tau }}={\varvec{m}}\times {\varvec{B}} \end{aligned}$$
(11a)

If the magnetic gradient \(\nabla \varvec{B}\) is nonzero, the magnet \(\varvec{m}\) is subjected to a magnetic force:

$$\begin{aligned} {\varvec{F}}_{\mathrm{m}} =\left( {{\varvec{m}}\cdot \nabla } \right) { \varvec{B}} \end{aligned}$$
(11b)

This is the reason why either a non-uniform or an oscillating magnetic field is applied to propel a magnetic micro-swimmer.

As for the acoustically actuated artificial micro-swimmers, the understanding of the driving mechanism behind the locomotion is still fragmented to date. A prevailing view regards the acoustic radiation force \(F_{\mathrm {A}}\) as the streaming-induced Stokes drag subject to the micro-swimmer surface \(\partial \Omega _{\mathrm {1}}\), and the integral form is expressed as [170]:

$$\begin{aligned} {F_{\mathrm{A}}} = \oint _{\partial {\Omega _1}} {\left\langle {{{{{\varvec{\sigma }} }}_2}} \right\rangle \cdot {\varvec{n}}{\mathrm{d}}A} - \oint _{\partial {\Omega _1}} {{\rho _1}\left\langle {{{\varvec{v}}_1}{{\varvec{v}}_2}} \right\rangle \cdot {\varvec{n}}{\mathrm{d}}A} \end{aligned}$$
(12)

where \(\varvec{v}_1\) and \(\varvec{v}_2\) are the vibration velocity of a micro-swimmer and the corresponding streaming velocity, respectively. The velocity field can be solved by the perturbation expansion approach [170, 189]. The bracket \(<>\) denotes the time-averaging within an acoustic period. The stress, \({\varvec{\sigma }}_2\), is interlinked to \(\varvec{v}_2\). The first term in Eq. (12) arises from the acoustic microstreaming and the second is due to the structural vibration of the micro-swimmer. However, in most cases, Eq. (12) cannot be straightforwardly used to solve the swimming problem. The finite element simulation is helpful, but it is often ill-conditioned due to the complex fluid–structure interaction circumstance.

Hence, more simplified models for theoretical calculation are in need for acoustic actuation. It is noted that the structure resonance of a micro-swimmer [related to the second term of Eq. (12)] may play an important role in propulsion. For a bubble-based acoustic micro-swimmer, as shown in Fig. 7b [190], the theoretical resonant frequency \(f_{\mathrm {0}}\) of the gas bubble can be estimated by [191]

$$\begin{aligned} f_{0} =\frac{1}{2{\pi }}\sqrt{\frac{\kappa P_{0} }{\rho L_{0} L_{\mathrm{B}} }} \end{aligned}$$
(13)

where \(\kappa \) is a frequency-dependent parameter related to the thermodynamic process, \(P_{{0}}\) the initial pressure inside the bubble, \(\rho \) the fluid density, \(L_{\mathrm {B}}\) the bubble length, and \(L_{{0}}\) the length of a liquid column outside the bubble (Fig. 7b). If the surface tension effect is considered, a correction factor, which is in connection with the geometry of the bubble and the surface tension of the water–air interface, will be introduced into Eq. (13) [192]. The oscillation amplitude and the propulsive force can also be estimated at resonance [193].

For a flagellated micro-robot, e.g., a sperm-like artificial micro-swimmer, the dynamic behavior is more difficult to predict. Nevertheless, the review of natural microorganisms reminds us that we can convert this problem into a simple so-called hyper-diffusion problem [109], in which the drag force and the elastic force on the tail are balanced. Specifically, the simplification can bring about a partial differential equation (PDF) that governs the oscillation of a cantilever beam (the tail) which has oscillatory boundary conditions at its clamped end (the head), as depicted in Fig. 7c. The motion of the head can be determined by finite element simulations as demonstrated in Ref. [119], in which the Galerkin method [194,195,196,197] was employed to solve the PDF with the prescribed head motion (sinusoidal). It is noted that the inertia of the tail was not neglected in Ref. [119], which allows the study of the effect of resonance.

As we have mentioned, many artificial micro-swimmers are bioinspired. However, most studies only focus on the actuation strategies, fabrication processes, or experimental techniques, and the theoretical aspect (i.e., the principles of design and optimization) based on the swimming mechanisms at LRN is insufficiently addressed. Hence, as listed in Table 2, we categorized the references of natural and artificial micro-swimmers based on the types of propulsion mechanisms to remind the close relationship between them. Among them, the success in the theoretical description of microorganisms propelled by oscillating flagella [68, 77, 109, 198, 199] has inspired our preliminary work on artificial flagellated micro-swimmers described in the next chapter.

Table 2 Categorized references of theoretical studies on propulsion mechanisms of microorganisms and their artificial counterparts
Full size table

3.3 Summary of Artificial Micro-swimmers

In this chapter, the actuation methods of artificial micros-swimmers, classified into self-propulsion, external propulsion, and a combination of them, have been reviewed. A self-propulsion approach is generally based on local (electro)chemical reactions, e.g., \(\hbox {H}_{{2}}\hbox {O}_{{2}}\) decomposition at the surface of a nano- or micro-swimmer, by which the chemical energy can be converted into kinetic energy. External propulsions may involve magnetic, acoustic, optical, and thermal fields. Magnetic power is most employed because of its prospect of remote and wireless operation, directional movement, and biocompatibility. A magnetic micro-swimmer can be propelled via either a non-uniform or an oscillating magnetic field. Recently, acoustic actuation has been a considerably popular driving strategy because of its potential for medical applications and lab-on-chip devices. We have introduced acoustic micro-robots driven by acoustic standing waves, resonant trapped bubbles, microstreaming, and head oscillation, which can generate substantial propulsive forces. The employment of optical and thermal energies and combinations of some of the aforementioned methods for micro-swimmer actuation has also been introduced in this chapter.

4 Forward-Looking Strategies of Steering

In this section, let us shed some light on the possible strategies for steering a flagellated micro-swimmer propelled acoustically. We focus on this micro-swimmer because of the anticipation of its great potential in medical applications, considering the facts that ultrasound systems have been the most adopted clinical tools for diagnosis and therapy and a sperm-like structure is effortless to fabricate through 3D printing. It is noted that the governing equations for 2D flagellar dynamics are normally of significant nonlinearity due to the geometric nonlinearity of the flagellum and the influence of the rigid body motion. Besides, decoupling of the equations is generally difficult and may involve improper simplification and render convergence problems. Therefore, a discrete flagellum model is desired to solve the swimming problem. In 1976 [62], Purcell proposed a symmetric linkage with three links articulated at two hinges, known as Purcell’s three-link swimmer, which could be regarded as the “simplest animal” that could achieve locomotion at LRNs. Purcell demonstrated that this swimmer would be propelled along a straight line over one cycle of sequentially moving its front and rear bars. The dynamics of Purcell’s three-link swimmer has been further investigated since then [69, 226,227,228,229]. Inspired by Purcell’s three-link swimmer, using multi-link bars or bar-joint models, the dynamics of flexible flagella has been modeled. Alouges et al. [230] proposed a slender magnetoelastic swimmer, along which the magnetic-induced torques were distributed, and the governing equations of the elastic tail were reduced to a system of ordinary differential equations (ODEs). Their group has also investigated the optimal stroke of a multi-link swimmer based on the criterion of optimal energy efficiency [231]. Moreau et al. [232] compared the classical elasto-hydrodynamic formulation with the bar-joint model via the coarse-graining formalism and demonstrated that the latter could achieve better numerical performance.

Following the above methodology, a bar-joint model of a flagellum, which is equivalent to a linear discretization of a continuous beam, is employed. As shown in Fig. 8, a flexible flagellum can be discretized into multiple segments which are considered as straight and rigid bars. The joint between two bars has the property of a spring hinge and can provide the restoring moment. This kind of bar-joint model can easily be validated by comparing its solutions with those of the beam theory in terms of static deformation or vibration. Note that this model intrinsically fulfills the inextensibility constraints of a flexible tail without the introduction of the Lagrange multiplier [17, 233]. The RFT is employed to estimate the fluid forces on the bars. For a 2D problem, each segment (including the head) has three degrees of freedom, and the trajectory of the micro-swimmer can be manifested by the time history of the location of the head centroid. The balance of momentum of each segment, as well as the head, will lead to the governing equations in the form of ODEs, which can be solved numerically. Since the external excitations will eventually appear in the boundary conditions at the head, it is straightforward to adjust the governing equations according to external excitations.

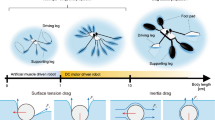
The turning mechanism demonstrated in Fig. 8 involves two piezoelectric acoustic transducers (commercially available) with frequencies \(f_{{1}}= 3.6\) kHz and \(f_{{2}} = 4.6\) kHz [171]. In this strategy, what matters is breaking the harmonicity of the symmetrically lateral motion. A special on–off function is added to the \(f_{\mathrm {2}}\) acoustic transducer to break the harmonicity in each of the common periods of the two-frequency acoustic waves. When the on–off function is inactive, the trajectory is horizontal. The swimmer will start to align with the Y-axis when the special on–off function of the \(f_{\mathrm {2}}\) acoustic transducer is turned on. It has also been noted that the swimmer oscillates symmetrically about the X-axis (the mean trajectory is still straight) if the on–off function is not applied. The role of the on–off function is to break the symmetry at a series of “right” moments to prompt the direction change; therefore, these “right” moments must be different from the common periods of \(f_{\mathrm {1}}\) and \(f_{\mathrm {2}}\). However, this steering strategy could be difficult to implement because the sharp on–off function is practically unachievable. Therefore, the steering strategy shown in Fig. 9 seems more feasible, where the acoustic waves are exploited to propel the swimmer, and a magnetic-induced torque is exerted to the head to turn the swimmer. This magnetic torque can be implemented via embedding magnetic nanoparticles into the head. As exhibited in Fig. 9a, the magnetic torque was on only for a certain time. When the magnetic torque vanished (at around Period 500 as shown in Fig. 9a), the acoustic wave changed its direction by \(90^{\circ }\) to continue propelling the swimmer along the new orientation. The value of magnetization m of the magnetic nanoparticles used in hyperthermia-based therapy is usually between 8 and 16 kA/m [234], and the corresponding magnetic flux density will be around \(4\times 10^{{-15}}\) T, which is sufficiently safe for most applications. Note that the degree of the wavevector is about the X-axis shown in Fig. 9b, and compared with Fig. 9a, b, one may find that the principal advance direction of the micro-swimmer is perpendicular to the acoustic wavevector.

Fig. 8
figure 8

Turning mechanism based on two-frequency acoustic waves. L is the length of the tail, which is 180 \(\upmu \hbox {m}\)

Full size image
Fig. 9
figure 9

The effect of the head magnetic torque on the micro-swimmer turning: a the amplitude of the magnetic torque and the direction of the acoustic wavevector, with \(f_{{1}}\) the actuation frequency, which is 3600 Hz; b the trajectory of the head centroid and the configurations of the swimmer at three instants, with \(L = 180 \upmu \hbox {m}\) the length of the tail

Full size image

5 Outlook

In this article, it has been aimed to elucidate the theoretical framework which is essential to fathom the dynamic behaviors of natural and artificial swimmers at LRNs. We first reviewed the studies on microorganisms, which are reminiscent of the propulsive mechanisms of natural micro-swimmers. After reviewing the basic principles and the development of the understanding of them, we took the flagellated micro-swimmers as an example to elaborate on the theories of their locomotion. We then reviewed the progress in actuation strategies, such as the magnetic and acoustic actuations, of artificial flagellated micro-swimmers, and finally proposed two possible strategies to realize the turning of a flagellated micro-swimmer—the key step toward manipulation through remote fields. Despite these impressive advances made in the field, inadequacies need to be addressed particularly when the applications require high accuracy and controllability.

First, the present experimental validation for theoretical models, such as the RFT and SBT, is inadequate due to the difficulties caused by the small scale. Hence, in view of the advancement of the experimental techniques, prospective work for further revealing the mechanisms of these micro-swimmers with more comprehensive experimental validation is of great significance and worth exploring. Second, the practical world is complex and Reynolds numbers are nonzero; therefore, simple theories based on the Stokes equations are insufficient. Numerical simulations are in principle the most powerful theoretical tool with the development of computational power. Thus, more studies dealing with multi-physical couplings, e.g., the entanglement of fluids, acoustic waves, and flexible swimmers, are imminently essential. There is certainly much room for improving the performance of numerical simulations. The auto-remeshing technique has been reported, which may be a breakthrough for solving fluid–structure interactions coupled with rigid body motion. The geometry optimization based on numerical simulations of an artificial micro-swimmer is more valuable for application, which should be the next research focus. Finally, although some in vivo experiments with externally powered artificial micro-swimmers have been attempted, there exists a huge gap between laboratory studies and practical applications. The study of the strategies for precise and robust manipulation of artificial micro-swimmers is still in its infancy, which should be the pivotal factor for enabling their applications. Our studies in Sect. 4 theoretically realized the steering of a flagellated micro-swimmer under external actuation. Further investigations could focus on the trajectory planning for demanded application. For example, how to make the swimmer stagnate to implement some targeted missions (e.g., drug release or thermotherapy), how to preciously control its trajectory to pass through a complex biological duct, etc. The strategy we proposed, which is to propel by acoustic waves and turn by a magnetic field, may be a feasible scheme to realize steering. The next studies should focus on the experimental implementation of swimmer navigation. The control system of the magnetic field should be designed, and the fabrication technique of micro-swimmer with magnetic nanoparticles must also be explored. The visualization and precious steering of swimmers in vivo may be the further consideration when it comes to a medical application. It is not straightforward to overcome these interdisciplinary challenges. Scientists in various fields should cooperate to achieve the leap from laboratory trials to practical applications.