A Bertrand–Edgeworth oligopoly with a public firm

Abstract

We determine conditions under which a pure-strategy equilibrium of a mixed Bertrand–Edgeworth oligopoly exists. In addition, we determine its pure-strategy equilibrium whenever it exists and compare the equilibrium outcome with that of the standard Bertrand–Edgeworth oligopoly with only private firms.

1 Introduction

In mixed oligopolies a public firm, which maximizes social surplus, competes with at least one private firm. The literature on mixed oligopolies focused on quantity-setting games (e.g. Harris and Wiens 1980; Beato and Mas-Colell 1984; Cremer et al. 1989; De Fraja and Delbono 1989; Matsumura and Okumura 2013) or on heterogeneous goods price-setting games (e.g. Bárcena-Ruiz 2007). A homogeneous good price-setting mixed duopoly was investigated by Balogh and Tasnádi (2012). Concerning the simultaneous-move game, the current paper extends their findings obtained for duopolies to oligopolies. The importance of considering a homogeneous good market in the price-setting mixed oligopoly framework can be underlined by the fact that the oligopolistic extension and the homogeneous good assumption might be useful in modeling energy markets, especially electricity markets since the storage of electricity is difficult and expensive, capacity constraints play a natural role,¹ and in many countries state-owned firms are present on these markets. The main reason why the Bertrand–Edgeworth model has not been applied to these markets is the lack of explicit results (see for example Bompard et al. 2010). Furthermore, homogeneous good markets play a crucial role in the quantity-setting framework, and therefore it seems natural to establish analogous results for the homogeneous good price-setting case before investigating heterogeneous good price-setting oligopolies for which there are results already available.²

Probably the mixed homogeneous good price-setting oligopoly has not been analyzed so far because of the difficulties in characterizing and determining the equilibrium of its standard counterpart with purely private firms. Although the equilibrium of the relatively simple symmetric oligopoly game with identical firms was solved by Vives (1986), the equilibrium of the general triopolistic case was obtained only recently, independently by Hirata (2009) and De Francesco and Salvadori (2008). In case of $n\ge 4$, the full characterization of the equilibrium is still incomplete but some of its important properties were derived by De Francesco and Salvadori (2008), which enable us to compare the purely private oligopoly with the mixed oligopoly in terms of social surplus.

While in the homogeneous good price-setting game with purely private firms a pure-strategy equilibrium fails to exist for a wide range of capacities (see, for instance, Dasgupta and Maskin 1986; Vives 1986) we show that its mixed version has a pure-strategy equilibrium for a much wider range of capacities. In addition, if the price-setting oligopoly game with purely private firms has an equilibrium in non-degenerated mixed strategies, we prove that the presence of a public firm is strictly social surplus increasing whenever in the latter case a pure-strategy equilibrium exists, which holds if the public firm is sufficiently large. The social surplus increasing effect of a social surplus maximizing firm is far from obvious, since in their seminal paper De Fraja and Delbono (1989) established in the framework of a mixed quantity-setting oligopoly that social surplus may be higher when a public firm maximizes its profit rather than social surplus (for similar results in the heterogeneous goods framework, see Footnote 2). Besides extending the results obtained by Balogh and Tasnádi (2012) from duopolies to oligopolies another interesting feature of having more than two firms in the market is that one Pareto inferior type of Nash equilibrium vanishes when we move from two to more firms.

The remainder of the paper is organized as follows. Section 2 introduces the framework, in Sect. 3 we recall the results of De Francesco and Salvadori (2008), which will serve as a benchmark in our analysis. Section 4 contains the characterization of the pure-strategy equilibria, and also a sufficient condition for its existence. Section 5 summarizes the results and mentions some possible directions for further research.

2 The framework

The demand is given by function D on which we impose the following restrictions:

Assumption 1

The demand function D intersects the horizontal axis at quantity a and the vertical axis at price b. D is strictly decreasing, concave and twice continuously differentiable on (0, b); moreover, D is right-continuous at 0, left-continuous at b and $D(p)=0$ for all $p\ge b$.

Let us denote by P the inverse demand function. Thus, $P\left( q\right) =D^{-1}\left( q\right)$ for $0<q\le a$, $P\left( 0\right) =b$, and $P\left( q\right) =0$ for $q>a$.

On the producers’ side we have a public firm and $n\ge 2$ private firms,³ that is, we consider a so-called mixed oligopoly. We label the public firm with 0 and the private firms with $1,2,\ldots ,n$. Our assumptions imposed on the firms’ cost functions are as follows:

Assumption 2

The firms have zero unit costs up to their respective positive capacity constraints $k_0, k_1,\ldots , k_n$.⁴ We can assume without loss of generality that $k_i\ge k_j$ for each $1\le i\le j\le n$.

We shall denote by $p^c$ the market clearing price, i.e. $p^c=P\left( \sum _{i=0}^{n} k_i\right)$ and $p_0, p_1,\ldots , p_n\in \left[ 0,b\right]$ stand for the prices set by the firms. For all $i\in \{0,1,\ldots , n\}$ we shall denote by $p^m_i$ the unique revenue maximizing price on the firm’s residual demand curve $D_i^r(p)=(D(p)-\sum _{j=0,j\not =i}^{n} k_j)^+$ taking the capacity constraint into account i.e.

Let us denote by $p_i^d$ the smallest price for which $p_i\cdot \min \{k_i,D\left( p_i\right) \}=p_i^mD_i^r\left( p_i^m\right)$ for each $i\in \{0,1,\ldots , n\}$. Provided that private firm i has ‘sufficient’ capacity (i.e. $p^c<p_i^m$), then if it is a profit-maximizer, it is indifferent to whether serving residual demand at price level $p_i^m$ or selling $\min \{k_i,D_i\left( p_i^d\right) \}$ at the lower price level $p_i^d$. Clearly, $p^c$, $p_i^m$ and $p_i^d$ are well-defined, and it can be verified that $p^c \le p_i^d \le p_i^m$, $p_i^m\ge p_j^m$ and $p_i^d\ge p_j^d$ hold, whenever Assumptions 1 and 2 are satisfied and $1 \le i\le j\le n$.

Now we define $\widehat{p}^c$, $\widehat{p}_i^m$ and $\widehat{p}_i^d$ in the same way as $p^c$, $p_i^m$ and $p_i^d$, except that we assume that the public firm does not enter the market, i.e. $\widehat{p}^c=P\left( \sum _{i=1}^{n} k_i\right)$, $\widehat{p}^m_i=\arg \max _{p\in \left[ 0,b\right] }p\cdot \min \left\{ \widehat{D}_i^r\left( p\right) ,k_i\right\}$, where $\widehat{D}_i^r(p)=\big (D(p)-\sum _{j=1,j\not =i}^{n} k_j\big )^+$ and $\widehat{p}_i^d$ is the smallest price for which $\widehat{p}_i\cdot \min \{k_i,D\left( \widehat{p}_i\right) \}=\widehat{p}_i^m\widehat{D}_i^r\left( \widehat{p}_i^m\right)$. Note that $p^c \le \widehat{p}^c$ and $p_i^d \le \widehat{p}_i^d$ for any $i\in \{1,2,\ldots , n\}$.

We assume that the firms play the production-to-order type Bertrand–Edgeworth game, and therefore, the game reduces to a price-setting game since the firms can adjust their productions to the demands they face. Regarding the employed rationing rule and tie-breaking rule, we impose the following assumptions.

Assumption 3

We assume efficient rationing on the market.

For instance, in case of a continuum of consumers with unit demand efficient rationing emerges if the consumers with higher reservation prices receive the units produced by the low-price firm, which would arise if there is a kind of secondary market for the good, where consumers with lower reservation prices can resell the obtained low-price product to consumers with higher reservation prices. From a more theoretical point of view efficient rationing is favored over proportional rationing because it leads to a more tractable model. For instance, many results concerning the mixed-strategy equilibrium beyond its sheer existence are only available or are much simpler in case of efficient rationing. For more details we refer the reader to Vives (1999) or Wolfstetter (1999).⁵

Assumption 4

We assume that in case of price ties the firms setting identical prices divide the residual demand in proportion to their capacities. However, each firm has the option of giving up a part or the whole of its portion of residual demand in favor of the other firms setting the same price.

It is clear that no profit-maximizing firm will give up the demand it is entitled to up to its capacity constraint. Nevertheless, under certain circumstances, as we will see later, the public firm can lead the market to a socially better equilibrium by restricting its supply at ‘sufficiently’ low price levels since it may discourage private firms matching its price from raising their prices.⁶

Concerning the tie-breaking rule described in Assumption 4, it should be mentioned that the division of the residual demand in proportion to the firms’ capacities can be replaced with any another division rule, which is strictly increasing in each firm’s capacity. If it were necessary, the public firm could pre-commit itself to give up parts of its demand with appropriate regulation. Moreover, at this point we do not assume that the public firm gives up parts of its demand, but it will turn out that as a surplus maximizer, it is indeed in its interest to do so, and therefore a commitment through regulation will not be necessary. Nevertheless, one might ask how the public firm can implement such a restriction, which directly or indirectly results in turning customers away. Clearly, consumers obtaining natural gasoline through pipelines or electricity through a network will not observe anything from the public firm’s self-restrictive behavior. Another way to implement the self-restrictive behavior would be that, based on a binding contract, the public firm serves the demand it faces, and thereafter it transfers the respective profits to the other firms.

For each firm $i\in \{1,2,\ldots , n\}$, let $E_i$ mean the set of those firms which set the same price as firm i. The definition of $L_i$ for firms setting lower prices is analogous, so $E_i=\{j \mid p_j = p_i, j=0,1,\ldots , n\}$ and $L_i=\{j \mid p_j < p_i, j=0,1,\ldots , n\}$. Thus, if $\mathbf {p}=\left( p_0,p_1,\dots ,p_n\right)$, $\mathbf {k}=\left( k_0,k_1,\dots ,k_n\right)$ and $\Delta _0\left( \mathbf {p}\right)$ stands for the public firm’s served demand, then

$$\begin{aligned} \min \left\{ k_0,(D(p_0)-\sum \limits _{\begin{array}{c} l \in L_0 \end{array}} k_l-\sum \limits _{\begin{array}{c} j \in E_0 j \ne 0 \end{array}} k_j)^+\right\} \le \Delta _0\left( \mathbf {p}\right) \end{aligned}$$

and

$$\begin{aligned} \Delta _0\left( \mathbf {p}\right) \le \min \left\{ k_0,\frac{k_0\Big (D(p_0)-\sum \limits _{\begin{array}{c} l \in L_0 \end{array}} k_l\Big )^+}{\sum \limits _{\begin{array}{c} j \in E_0 \end{array}} k_j}\right\} \end{aligned}$$

must hold.

Essentially, $\Delta _0\left( \mathbf {p}\right)$ describes the public firm’s behavior of letting or not letting other firms serve its market share. According to Assumptions 3 and 4, the demand served by private firm $i\in \{1,2,\ldots , n\}$ is given by

$$\begin{aligned} \Delta _i\left( \mathbf {p}\right) = \left\{ \begin{array}{ll} \min \left\{ k_i,\frac{k_i}{\sum \limits _{\begin{array}{c} j \in E_i \end{array}} k_j}(D(p_i)-\sum \limits _{\begin{array}{c} l \in L_i \end{array}} k_l)^+\right\} &{}\quad \text {if}\; \,0\notin E_i,\\ \\ \min \left\{ k_i,\frac{k_i}{\sum \limits _{\begin{array}{c} j \in E_i \end{array}} k_j-k_0}(D(p_i)-\sum \limits _{\begin{array}{c} l \in L_i \end{array}} k_l-\Delta _0\left( \mathbf {p}\right) )^+\right\}&\quad \text {if}\; \,0\in E_i. \end{array}\right. \end{aligned}$$

We shall denote by $S_\mathbf {p}(p)=\sum _{\{i\mid p_i\le p\}} k_i$ the supply curve and by $p^s_\mathbf {p}=\min \left\{ p\in [0,b] \mid D(p)\le S_{\mathbf {p}}(p)\right\}$ the price determining social surplus for any given price profile $\mathbf {p}$. The public firm aims to maximize total surplus given by

$$\begin{aligned} \pi _0(\mathbf {p})=\int \limits _{0}^{D(p^s_\mathbf {p})} P(q)\mathrm{d}q. \end{aligned}$$

As the private firms are profit-maximizers, their object function is

$$\begin{aligned} \pi _i(\mathbf {p})=p_i\Delta _i\left( \mathbf {p}\right) . \end{aligned}$$

3 The benchmark

The following results concerning the purely private price-setting oligopoly will serve as a benchmark:

Proposition 1

[De Francesco and Salvadori 2008] For the purely private price-setting oligopoly under Assumptions 1–4, the following statements are known.

• 1. A pure-strategy equilibrium exists if and only if $\max \{p_0^d,p_1^d\} = p^c$. Furthermore, all pure-strategy equilibria are payoff equivalent, and in all of them sales are realized only at price $p^c$.

• 2. Even in case of a mixed-strategy equilibrium, each firm will set a price equal to or larger than $\max \{p_0^d,p_1^d\}$. More precisely, $\max \{p_0^d,p_1^d\}$ is the lowest of the supports’ minima of the firms’ strategies.

Note that the second point necessarily means that in any mixed-strategy equilibrium the social surplus must be lower than in a strategy profile where each firm sets price $p_1^d$.

4 Characterization of the pure-strategy equilibria

Firstly, we examine the possible pure-strategy equilibria with arbitrary $\Delta _0(\mathbf {p})$, which enables us to find a $\Delta _0\left( \mathbf {p}\right)$ that leads to the highest achievable social surplus. After that we can simplify the equilibrium, find a sufficient condition for its existence and compare it with the purely private case. Virtually, we analyze a two-stage game: in the first stage the public firm chooses a suitable $\Delta _0\left( \mathbf {p}\right)$ (a strategy to decide when to share its demand with the private firms in the second stage); in the second stage, after the private firms have been informed about $\Delta _0\left( \mathbf {p}\right)$, price competition takes place. In particular, we find that if for the exogenously given capacities $k_0,\ldots ,k_n$ pure-strategy equilibria can be induced by choosing $\Delta _0$ appropriately, the welfare-maximizing public firm prefers the one yielding the lowest price. We would like to emphasize that we are not determining the SPNE of a two-stage game because of the inherent difficulty of determining the mixed-strategy equilibrium of the price-setting stage as explained in detail at the end of Sect. 4 and in Sect. 5.

Concerning the size of the capacities the following three cases can be distinguished:

• 1. If $p_1^d=p_0^d=p^c$, then similarly to the purely private case the market-clearing outcome $\{p^c,p^c,\dots ,p^c\}$ is a pure-strategy equilibrium and any other equilibrium in pure strategies is payoff-equivalent to the market-clearing one. This means that in this case it has no effect on social surplus whether firm 0 acts as a public firm or not.

• 2. If $p_1^d=p^c<p_0^d$ (i.e. only firm 0 has ‘sufficient’ capacity), then the set of possible pure-strategy equilibria is the same as in the previous case, but here the social surplus is strictly higher if firm 0 acts as a public firm. This case corresponds to the less interesting weak-private firm case in Balogh and Tasnádi (2012).

• 3. If $p^c<p_1^d$, the characterization of the equilibria is more difficult, therefore the rest of Sect. 4 is devoted to this task.

Assumption 5

We only consider $\mathbf {k}$ and D(p) for which $p_1^d>p^c$.

Lemma 1

Under Assumptions 1–4 and $p_1^d>0$, price $p^s_\mathbf {p}$ must be positive in any pure-strategy equilibrium if such an equilibrium exists.

Proof

Assume that $p_1^d>0$ and there is a pure-strategy equilibrium in which $p^s_\mathbf {p}=0$. Since $p_1^d>0$ implies $\sum _{j=0,j\not =1}^{n} k_j < a$, and therefore it follows that firm 1 could still sell a positive amount at a positive price; a contradiction. $\square$

Lemma 2

Under Assumptions 1–5 all private firms must set the same price in any pure-strategy equilibrium.

Proof

Assume that the private firms set at least two different prices in a pure-strategy equilibrium $\mathbf {p}$. Consider one of the private firms with the highest price: If its residual demand is zero, its profit is also zero, and it could benefit by setting a positive price below $p^s_\mathbf {p}$. If its residual demand is positive, any of the firms setting a lower price could increase its profits by setting its price anywhere between the current highest and second highest prices, hence $\mathbf {p}$ cannot be an equilibrium. $\square$

Henceforth, we shall denote by $p^*$ the common pure-strategy equilibrium price of the private firms if such an equilibrium exists.

Lemma 3

Under Assumptions 1–5 in any pure-strategy equilibrium $(p_0,p^*,p^*,$ $\ldots ,p^*)$ we must have that all the private firms sell their entire capacities.

Proof

Otherwise the profit could be increased by slightly undercutting $p^*$. $\square$

Lemma 4

Under Assumptions 1–5 in any pure-strategy equilibrium $(p_0,p^*,p^*,$ $\ldots ,p^*)$ we must have that $D(p^*) \le \sum _{\begin{array}{c} p_j\le p^* \end{array}} k_j$.

Proof

Otherwise each private firm could increase its profit by slightly raising its price. $\square$

We are now ready to specify the pure-strategy equilibria with respect to an arbitrary $\Delta _0\left( \mathbf {p}\right)$.

Proposition 2

(Equilibria with arbitrary $\Delta _0\left( \mathbf {p}\right)$) Assume that Assumptions 1–5 hold. Then the simultaneous-move game can only have the following two types of pure-strategy equilibria:

$$\begin{aligned} p_0=p^*=P\left( \sum \limits _{j=1}^{n} k_j+\Delta _0\left( \mathbf {p^*}\right) \right) \;\;(PSE_1), \end{aligned}$$

where $\mathbf {p^*}=\{p^*,p^*,\dots ,p^*\}$ and which is an equilibrium if and only if $p_1^d \le p^* \le P(k_0)$, $p^* \le \widehat{p}^c$ and $\Delta _0\left( \mathbf {p^*}\right) =D(p^*)-\sum _{j=1}^{n} k_j$. In addition, the strategy profile

$$\begin{aligned} p^*=\widehat{p}^c \;\; \;\;and \;\;p_0> p^* \;\;(PSE_2), \end{aligned}$$

is an equilibrium if and only if $\widehat{p}_1^d = \widehat{p}^c \le P(k_0)$.

Proof

Assume firstly that $p_0<p^*$. In this case $p^*=p^c$ is the only possible equilibrium price due to Lemmas 3 and 4. However, since $p^c<p_1^d$ because of Assumption 5, playing $p_1^m$ instead of $p^c$ would be strictly better for firm 1, hence no such pure-strategy equilibrium can exist.

Now consider the case $p_0=p^*$. Clearly only $p^*=P(\sum _{j=1}^{n} k_j+\Delta _0\left( \mathbf {p^*}\right) )$ with the condition $\Delta _0\left( \mathbf {p^*}\right) =D(p^*)-\sum _{j=1}^{n} k_j\ge 0$ (i.e. $p^*\le \widehat{p}^c$) satisfies Lemmas 3 and 4. The public firm cannot increase social surplus, unless it is able to lower the private firms’ residual demand to 0 by undercutting $p^*$, which is possible if and only if $P(k_0)<p^*$. As the private firms sell their entire capacities, they would not profit by lowering their prices. It is easy to see, that due to the concavity of the demand function, any firm $i\in \{1,2,\ldots , n\}$ can increase its profit by raising its price if and only if $p_i^d>p^*$.

When $p_0>p^*$, $p^*=\widehat{p}^c$ is the only price that satisfies Lemmas 3 and 4. Condition $p^* \le P(k_0)$ can be justified as in the previous case. The condition preventing the private firms from switching to a higher price is also similar, but here we have to use $\widehat{p}_i^d$ instead of $p_i^d$ as the public firm does not compete with private firms unless they set a higher price than $p_0$. $\square$

Considering the existence of the equilibria, note that $PSE_1$ exists only if $p_1^d\le \widehat{p}^c$, and observe that since $p^d_i \le \widehat{p}^d_i$, $PSE_1$ always exists with an appropriately chosen $\Delta _0$ at price $p^*$ when $PSE_2$ does. We consider $PSE_2$ as an unlikely equilibrium, since it implies that the public firm does not enter the market despite knowing that another pure-strategy equilibrium with higher social surplus would be possible. Furthermore, any $PSE_1$ equilibrium price below $\widehat{p}^c$, if a pure-strategy equilibrium exists, weakly dominates any $PSE_2$ equilibrium price.

Proposition 2 already establishes that the public firm can increase social surplus as long as it can reduce the equilibrium price by relinquishing part of its market share to the private firms in case of price ties, and thus hindering the large private firm from increasing its price. Our next statement, Proposition 3, which we consider as our main result, is about choosing the social surplus maximizing $\Delta _0$ and we obtain it as a simple corollary of Proposition 2. Therefore, we may look at Proposition 2 as an auxiliary result and additional interpretations will follow after Proposition 3.

In order to maximize social surplus the public firm should choose $\Delta _0$ in a way that the $PSE_1$ type equilibrium price becomes as small as possible. Taking into consideration that $P\left( \sum _{j=1}^{n} k_j+\Delta _0\left( \mathbf {p}\right) \right) \in [p^c,\widehat{p}^c]$ is a function of $\Delta _0$, an optimal $\Delta _0$ for the public firm, resulting in equilibrium price $p_1^d$, is given by

$$\begin{aligned} \Delta ^*_0\left( \mathbf {p}\right) = \left\{ \begin{array}{ll} \min \bigg \{k_0,\Big (D(p_0)-\sum \limits _{\begin{array}{c} l \in L_0 \\ \end{array}} k_l-\sum \limits _{\begin{array}{c} j \in E_0 \\ j \ne 0 \end{array}} k_j\Big )^+\bigg \} &{}\quad \text {if }\; \forall i: p_i \le p_1^d, \; \\ \min \bigg \{k_0, \frac{k_0}{\sum \limits _{\begin{array}{c} j \in E_0 \end{array}} k_j}\Big (D(p_0)-\sum \limits _{\begin{array}{c} l \in L_0 \end{array}} k_l\Big )^+\bigg \}&\quad \text {if }\; \exists i: p_i > p_1^d, \end{array}\right. \end{aligned}$$

where $i\in \{1,2,\dots ,n\}$.

$PSE_1$ simplifies to $p^*=p_1^d$ with only one necessary and sufficient condition in case of $\Delta ^*_0$ since condition $P\big (\sum _{j=1}^{n} k_j+\Delta _0\left( \mathbf {p}\right) \big ) \le P(k_0)$ takes the form $p^d_1\le P(k_0)$, which holds true by $p^d_1 < p^m_1 < P(\sum _{j\ne 1} k_j) \le P(k_0)$. We formalize this result in the following proposition.

Proposition 3

[Equilibria with optimal $\Delta _0^*\left( \mathbf {p}\right)$] Assume that Assumptions 1–5 are satisfied and that the public firm chooses $\Delta ^*_0$. Then the simultaneous move game can only have the following two types of pure-strategy equilibria:

$$\begin{aligned} p_0=p^*=p_1^d \;\;(PSE_1), \end{aligned}$$

which is an equilibrium if and only if $p_1^d \le \widehat{p}^c$. In addition, the strategy profile

$$\begin{aligned} p^*=\widehat{p}^c \;\; \;\;and \;\;p_0> p^* \;\;(PSE_2), \end{aligned}$$

is an equilibrium if and only if $\widehat{p}_1^d = \widehat{p}^c \le P(k_0)$.

Since under Assumptions 1–5 by Proposition 1 the purely private oligopoly has only a mixed-strategy equilibrium in which no firm sets a price below $\max \{p_1^d,p_0^d\}$, it follows for the respective mixed oligopoly that it has a strictly higher social surplus whenever it has a pure-strategy equilibrium and the $PSE_1$-type equilibrium will be played. The existence of a $PSE_2$-type equilibrium, which exists if and only if $\widehat{p}_1^d = \widehat{p}^c \le P(k_0)$, implies the existence of a $PSE_1$-type equilibrium because of $p^d_1 \le \widehat{p}^d_1$. A $PSE_1$-type and a $PSE_2$-type equilibrium can coexist because $p_1^d<\widehat{p}^c=\widehat{p}_1^d$ can be the case. We have already emphasized after Proposition 2 why a $PSE_1$-type equilibrium is more plausible than a $PSE_2$-type one.

The result formulated in Proposition 3 can be interpreted as whenever the largest private firm is not too big in relation to the other private firms (i.e. $p_1^d \le \widehat{p}^c$), then by selling a certain quantity at the appropriate price the public firm can enforce the private firms to set the market clearing price on the residual demand curve, which leads to an equilibrium price lower than or equal to any price that any private firm would play in the equilibrium of the standard Bertrand–Edgeworth oligopoly game. The driving force behind this result is that under the Assumptions of Proposition 3 the public firm can provide the largest private firm with sufficient market share (i.e. letting sell its entire capacity) at a lower price level so that the private firm has no incentive to raise its price unilaterally as it could be the case in the standard setting. This resembles the result obtained by Corneo and Jeanne (1993) on predatory pricing in the framework of a capacity-constrained price-setting duopoly in which the predator can lower its competitor’s profit by setting prices below the Nash equilibrium price.

Comparing our result with the duopolistic case investigated by Balogh and Tasnádi (2012), $PSE_1$ and $PSE_2$ correspond to their $NE_1$ and $NE_3$, respectively. Surprisingly, the Pareto inferior equilibrium $NE_2$ (i.e. where the public firm plays a price below $p_1^d$ and the private firm maximizes profit on the residual demand curve) of the duopoly game ceases to be a pure-strategy equilibrium in case of more than one private firm. In particular, by Assumption 5 no pure-strategy equilibrium is possible for the private firms on residual demand curve $D-k_0$ because each of them would benefit from unilaterally undercutting each other’s price. Since in the duopolistic setting there is just one private firm, there is no other private firm to undercut its price on the residual demand curve. This explains, concerning the pure-strategy equilibrium of the game, the qualitative difference between the duopolistic and the oligopolistic case with at least three firms. However, the $NE_2$ of the duopolistic case does not completely disappear since it can be associated with the mixed-strategy equilibrium of the oligopolistic case with at least three firms briefly outlined at the end of this Section.

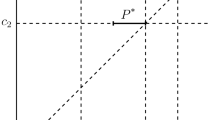
Proposition 3 contains only an implicit necessary and sufficient condition for the existence of a pure-strategy equilibrium. In order to illustrate that replacing a private firm with a public firm substantially increases the capacity region where a pure-strategy equilibrium exists, we consider the following example.

Example 1

$D(p)=1-p$ and $\sum _{i=2}^n k_i=0.2$, that is we keep the aggregate capacity of the smaller private firms fixed.

Example 1 is only meaningful if we have sufficiently many small-capacity private firms such that $k_1\ge k_2$ remains valid. Let $\overline{K}=\sum _{i=2}^n k_i=0.2$.

First, let us start with the region in which the standard Bertrand–Edgeworth oligopoly has a pure-strategy equilibrium, i.e. neither firm 0 nor firm 1 has an incentive to increase its price unilaterally above $p^c$. Let $i,j\in \{0,1\}$ and $i\ne j$. If both of the two firms have capacities of at least $1-\overline{K}=0.8$, then the equilibrium price will be zero since $D_i^r(0)=0$ and a pure-strategy equilibrium exists. This region corresponds to the region labeled with B in Fig. 1. If we have $D_i^r(0)>0$ and $D_j^r(0)=0$, then $p^c=0$ and $p_i^m>0$, and therefore a pure-strategy equilibrium does not exit. If both firms face positive residual demand, then $k_0<0.8$ and $k_1<0.8$, and thus from $p_i^m=(0.4-0.5k_j)^+\le p^c=(0.8-k_0-k_1)^+$ it follows that we also have a pure-strategy equilibrium in the region labeled with A in Fig. 1. Otherwise, the standard Bertrand–Edgeworth oligopoly does not have a pure-strategy equilibrium.

Fig. 1

Regions with different types of equilibrium: $D(p)=1-p$, $k_1\ge k_2$, and $\sum _{i=2}^n k_i=0.2$

Second, let us consider the mixed Bertrand–Edgeworth oligopoly. It can be easily verified for capacities within the regions labeled with A and B that the mixed oligopoly game also has a pure-strategy equilibrium. In addition, region A can be enlarged since $p_i^m=(0.4-0.5k_j)^+\le p^c=(0.8-k_0-k_1)^+$ is only meaningful for $i=1$ and $j=0$. Hence it follows that in regions $k_1\le 0.4-0.5k_0$ and $k_0\ge 0.8$ we have $p^c=p_1^m$ in a pure-strategy equilibrium. By Proposition 3 we know that under Assumptions 1–5 $p_1^d \le \widehat{p}^c$ is a necessary and sufficient condition for the existence of a pure-strategy equilibrium. Assume that $k_1\le 1-p_1^d$. Then $p_1^d=\left( 0.4-0.5k_0\right) ^2/k_1$ and a pure-strategy equilibrium exists if and only if

$$\begin{aligned} k_1\le 0.4+\sqrt{0.4k_0-0.25k_0^2}. \end{aligned}$$

(1)

It can be verified that if $k_1\ge 0.4$ inequality (1) implies $k_1\le 1-p_1^d$. If $k_1< 0.4$, then we immediately have $k_1< 1-k_1<1-p_1^d$ where the second inequality holds since $k_1>0.4-0.5k_0$ follows from Assumption 5. Therefore, we already know that region D in Fig. 1 contains capacity pairs for which a pure-strategy equilibrium exists due to the public firm. Assume that $k_1>1-p_1^d$. Then

$$\begin{aligned} p_1^d=0.5-\sqrt{0.25-(0.4-0.5k_0)^2} \end{aligned}$$

(2)

and a pure-strategy equilibrium exists if and only if $p_1^d \le \widehat{p}^c$, i.e.

$$\begin{aligned} k_1\le 0.3+\sqrt{0.09+0.4k_0-0.25k_0^2}; \end{aligned}$$

(3)

however, inequality (3) does not provide a new region of capacity pairs for which a pure-strategy equilibrium exists because the expression for $p_1^d$ given by (2) is only valid for $k_1>1-p_1^d$, i.e.

$$\begin{aligned} k_1>0.5+\sqrt{0.09+0.4k_0-0.25k_0^2}, \end{aligned}$$

(4)

and it is obvious that inequalities (3) and (4) are incompatible. Hence, region C in Fig. 1 consists of those capacity pairs for which neither the standard game nor the mixed game has a pure-strategy equilibrium.

Finally, we provide an explicit simple sufficient condition for the existence of an equilibrium in pure strategies. In particular, we show that if the public firm is the largest, an equilibrium in pure strategies exists. It is worthwhile mentioning that the region covered by this condition intersects the boundary of the region in which a pure-strategy equilibrium does not exist. In particular, the neighborhood of point (0.8, 0.8) in Fig. 1 contains capacity pairs for which the largest private firm has a slightly larger capacity than the public firm and a pure-strategy equilibrium does not exist.

Proposition 4

(Sufficient condition for the existence of an equilibrium in pure strategies) Under Assumptions 1–5 and $\Delta _0^*$, if $k_0\ge k_1$, then the game has an equilibrium in pure strategies.

Proof

By Assumption 5 $p_1^d>p^c$, and the necessary and sufficient condition for the existence of $PSE_1$ (which always exists whenever $PSE_2$ does) is $p_1^d\le \widehat{p}^c$. Note that $D(p_1^m) > \sum _{j=0,j \ne 1}^{n} k_j \ge \sum _{j=1}^{n} k_j$ holds whenever $k_0 \ge k_1$, which leads to $p_1^d < p_1^m < \widehat{p}^c$. $\square$

Observe that in the above proof there are several overestimations, so in a particular game we can expect that the public firm does not need to be the largest one to be able to drive the market to a socially better equilibrium in pure strategies. Put in another way the previous proposition, when the market is not competitive enough to have an equilibrium in pure-strategies, the state can increase social welfare by acquiring a sufficiently large private firm and operating it as a public firm.

An equilibrium in mixed strategies can be determined quite easily from the mixed-strategy equilibria characterized by De Francesco and Salvadori (2008) for the standard version of the game in the following way: Let the private firms play their mixed equilibrium strategies according to De Francesco and Salvadori (2008) assuming they face demand function $(D-k_0)^+$. Let $\underline{p}$ denote the minimum of the infima of the supports of the strategies and suppose the public firm sets its price below $\underline{p}$. It can be verified that all these strategy profiles constitute equilibria in mixed strategies and $p_1^d\le \underline{p}$. However, in contrast to the case when an equilibrium in pure strategies exists, comparing the social surpluses of the mixed and standard versions of the Bertrand–Edgeworth oligopoly seems to be a very complicated task if one considers the complexity of the possibly multiple mixed-strategy equilibria of the standard Bertrand–Edgeworth oligopoly described by De Francesco and Salvadori (2008).

From our analysis it is clear that the existence of a social surplus increasing pure-strategy equilibrium is a function of the capacities of the public firm and of the largest private firm, as well as the sum of the other private firms’ capacities. Considering a distribution of capacities among firms such that a pure-strategy equilibrium of type $PSE_1$ exists, if the private firms could achieve through mergers that the pure-strategy equilibrium vanishes, then since by the previous paragraph their profits would be higher in a mixed-strategy equilibrium, the private firms could benefit from mergers. In contrast considering a distribution of capacities among firms such that only a mixed-strategy equilibrium exists, if the state could drive the market to a $PSE_1$-type pure-strategy equilibrium by buying some private firms and merging it with the public firm, the state could increase social surplus. These two types of mergers are a function of market regulation and state expenditures. These observations show us which forces the government and antitrust agencies should take into account.

5 Concluding remarks

Investigating a mixed Bertrand–Edgeworth oligopoly, we have determined the necessary and sufficient conditions for the existence of an equilibrium in pure strategies. Though the mixed version of the Bertrand–Edgeworth oligopoly has an equilibrium in pure strategies for a larger range of capacities than its standard version with purely private firms we still may face the problem of non-existence of an equilibrium in pure strategies.

One could try to compare the social surpluses of the standard and mixed Bertrand–Edgeworth triopoly even if the latter does not have a pure-strategy equilibrium. For the standard Bertrand–Edgeworth triopoly (De Francesco and Salvadori 2015) fully characterized the equilibrium payoffs and the supports of the mixed-strategy equilibrium. However, determining or characterizing the social surplus appears to be an even more difficult task since we do not know explicitly the mixed-strategy equilibrium of the triopolistic case.

Determining the endogenous order of moves, would be another interesting question. However, the solution seems out of reach even for the standard Bertrand–Edgeworth oligopoly game. For instance, solving the game in which two price-setting firms move first and two price-setting firms move second seems to be an extremely difficult problem. Tasnádi (2012) provides a solution for the triopolistic case in the standard Bertrand–Edgeworth framework, furthermore contains more details on the difficulties on determining the endogenous order of moves in case of at least four firms. The mixed framework seems to cause even more difficulties.

To keep this paper coherent we restricted ourselves to the pure-strategy equilibrium of the mixed Bertrand–Edgeworth oligopoly, while there is some hope to answer some further question for triopolies, especially determining the endogenous ordering of moves. We plan to address these questions for triopolies in future research.