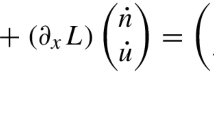1 Introduction

Nonlinear Maxwell–Klein–Gordon equations are written by

$$\begin{aligned} \left\{ \begin{aligned}&D_\alpha D^{\alpha }\phi = (mc)^2\phi -|\phi |^{p-2}\phi , \\&\partial ^{\beta }F_{\alpha \beta } = \frac{q}{c}\text {Im}(\phi \overline{D_\alpha \phi }), \end{aligned}\right. \quad \text {in } \mathbb {R}^{1+3}. \end{aligned}$$
(NMKG)

where \(D_\alpha {:}{=}\partial _\alpha +\frac{q}{c}iA_\alpha , \alpha = 0, 1, 2, 3\) and \(F_{\alpha \beta } {:}{=}\partial _\alpha A_\beta -\partial _{\beta }A_{\alpha }\). Here, \(m>0\) represents the mass of a particle, \(q>0\) is a unit charge and \(c > 0\) is the speed of light. We write \(\partial _0 = \frac{\partial }{c\partial t}\), \(\partial _i = \frac{\partial }{\partial x_{j}}, j = 1, 2, 3\). Indices are raised under the Minkowski metric \(g_{\alpha \beta } = \text {diag}(-1, 1, 1, 1)\), i.e., \(X^{\alpha } {:}{=}g_{\alpha \beta }X_{\beta }\). If we pay attention to the electrostatic situation, that is, \(A_1 = A_2 = A_3 = 0\), then NMKG is reduced to

$$\begin{aligned} \left\{ \begin{aligned}&\left( -\frac{\partial ^2}{c^2\partial t^2}+\Delta \right) \phi -\frac{2q}{c^2}iA_0 \frac{\partial \phi }{\partial t} -\frac{q}{c^2}i\frac{\partial A_0}{\partial t} \phi +\left( \frac{q}{c}\right) ^2A_0^2\phi = (mc)^2\phi -|\phi |^{p-2}\phi , \\&-\Delta A_0= \frac{q}{c^2}\text{ Im }\left( \phi \overline{\frac{\partial \phi }{\partial t}}\right) -\left( \frac{q}{c}\right) ^2A_0|\phi |^2, \end{aligned}\right. \quad \text {in } \mathbb {R}^{1+3}. \end{aligned}$$
(1)

This paper is concerned with the nonrelativistic limit for NMKG in electrostatic case. By modulating the solution as \(\phi (t,x) = e^{imc^2t}\psi (t,x)\), the system of equations (1) transforms into

$$\begin{aligned} \left\{ \begin{aligned}&-\frac{\partial ^2\psi }{c^2\partial t^2} -2mi\frac{\partial \psi }{\partial t} +\Delta \psi +2qmA_0\psi -\frac{2q}{c^2}iA_0\frac{\partial \psi }{\partial t} -\frac{q}{c^2}i\frac{\partial A_0}{\partial t} \psi +\left( \frac{q}{c}\right) ^2A_0^2\psi = -|\psi |^{p-2}\psi , \\&-\Delta A_0 +\left( \frac{q}{c}\right) ^2|\psi |^2A_0 = \frac{q}{c^2}\text{ Im }\left( \psi \overline{\frac{\partial \psi }{\partial t}}\right) -qm|\psi |^2. \end{aligned}\right. \end{aligned}$$
(2)

Then, taking so-called nonrelativistic limit \(c\rightarrow \infty \), the relativistic system (2) formally converges to nonlinear equations of Schrödinger type, called the nonlinear Schrödinger–Poisson equations

$$\begin{aligned} \left\{ \begin{aligned}&-2mi\frac{\partial \psi }{\partial t} +\Delta \psi +2qmA_0\psi = -|\psi |^{p-2}\psi , \\&-\Delta A_0 = -qm|\psi |^2, \end{aligned}\right. \quad \text {in } \mathbb {R}^{1+3}. \end{aligned}$$
(NSP)

When the nonlinear potential term \(|\psi |^{p-2}\psi \) is absent, the rigorous justifications of this limit are carried out by Masmoudi-Nakanishi [17] and Bechouche-Mauser-Selberg [4]. As for the stuides on the nonlinear Klein-Gordon equations without the Maxwell gauge terms (\(A_\mu = 0, \mu =0,1,2,3\)), we refer to a series for works [15, 16, 18].

The main interest of this paper lies in investigating the correspondence between solitary waves of NMKG and NSP under the nonrelativistic limit \(c\rightarrow \infty \). During recent two decades, existence theories for solitary waves of NMKG and NSP have been well developed. Inserting the standing wave ansatz \(\psi (t,x) = e^{-i\mu t}u(x),\, u \in \mathbb {R}\) into (2), we get

$$\begin{aligned} \left\{ \begin{aligned}&-\Delta u+\Big (m^2c^2-\big (\frac{mc^2-\mu }{c}+\frac{q\Phi }{c}\big )^2\Big )u-|u|^{p-2}u=0, \\&-\Delta \Phi +\frac{q^2}{c^2}u^2\Phi =-\frac{q}{c}\left( \frac{mc^2-\mu }{c}\right) u^2, \end{aligned}\right. \qquad \text{ in } \mathbb {R}^3. \end{aligned}$$
(3)

Lax-Milgram theorem implies that for each \(u\in H^1(\mathbb {R}^3)\), there exists a unique solution \(\Phi _u\in D^{1,2}(\mathbb {R}^3)\) of

$$\begin{aligned} -\Delta \Phi +\frac{q^2}{c^2}u^2\Phi =-q(m-\frac{\mu }{c^2}) u^2 \text{ in } \mathbb {R}^3. \end{aligned}$$
(4)

Then, by [6, Proposition 3.5], \((u,\Phi )\in H^1(\mathbb {R}^3)\times D^{1,2}(\mathbb {R}^3)\) is a solution of (3) if and only if \(u\in H^1(\mathbb {R}^3)\) is a critical point of \(I_c\), and \(\Phi =\Phi _u\), where

$$\begin{aligned} I_c(u)&=\frac{1}{2}\int _{\mathbb {R}^3}|\nabla u|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u^2-q\Big (m-\frac{\mu }{c^2}\Big ) u^2 \Phi _u dx-\frac{1}{p}\int _{\mathbb {R}^3}|u|^pdx, \end{aligned}$$

which is a \(C^1\) functional on \(H^1(\mathbb {R}^3)\). We note that the system of equations (3) is equivalent to the single nonlocal equation

$$\begin{aligned} -\Delta u+\Big (m^2c^2-\big (\frac{mc^2-\mu }{c}+\frac{q\Phi _u}{c}\big )^2\Big )u-|u|^{p-2}u=0 \text{ in } \mathbb {R}^3. \end{aligned}$$
(5)

Before stating the existence results for (5), we simplify the parameters by denoting \(\bar{m} = mc\), \(e = q/c\) and \(\omega = (mc^2-\mu )/c\) to rewrite (5) as

$$\begin{aligned} \begin{aligned} -\Delta u+\left( \bar{m}^2-\left( \omega +e\varphi _u\right) ^2\right) u-|u|^{p-2}u=0 \text{ in } \mathbb {R}^3, \end{aligned} \end{aligned}$$
(6)

where \(e > 0\), \(0< \omega <\bar{m} \) and \(\varphi _u\) is a unique solution of \(-\Delta \varphi +e^2u^2\varphi =-e\omega u^2\). The corresponding action functional is given by

$$\begin{aligned} I_{\bar{m},e,\omega }(u)=\frac{1}{2}\int _{\mathbb {R}^3}|\nabla u|^2+(\bar{m}^2-\omega ^2)u^2-e\omega u^2 \varphi _u dx-\frac{1}{p}\int _{\mathbb {R}^3}|u|^pdx. \end{aligned}$$

For fixed \(e > 0\), Benci and Fortunato [6] first proved by applying critical point theory to \(I_{\bar{m},e,\omega }\) that there exist infinitely many solutions of (6) for \(4< p <6\) and \(0< \omega < \bar{m}\). This result is extended by D’Aprile and Mugnai [12] to the cases \(4\le p < 6\) and \(0< \omega < \bar{m}\) or \(2< p < 4\) and \(0< \sqrt{2}\omega < \bar{m}\sqrt{p-2}\). They also proved in [13] that there exist no nontrivial solutions if \(p \le 2\) or \(p \ge 6\) and \(0 < \omega \le \bar{m}\). In [3], Azzollini, Pisani and Pomponio widened the existence range of \(\bar{m}, \omega \) for the case \(2< p < 4\) by showing that (6) admits a nontrivial solution when \(0< \omega < \bar{m}g(p)\), where

$$\begin{aligned} g(p) {:}{=}\left\{ \begin{array}{rl} \sqrt{(p-2)(4-p)} &{} \text {if } 2< p< 3, \\ 1 &{} \text {if } 3 \le p < 4. \end{array}\right. \end{aligned}$$

Azzollini and Pomponio also focused on the existence of a ground state solution of (6). A critical point of \(I_{\bar{m},e,\omega }\) is said to be a ground state solution to (6) if it minimizes the value of \(I_{\bar{m},e,\omega }\) among all nontrivial critical points of \(I_{\bar{m},e,\omega }\). In [2], they showed (6) admits a ground state solution if \(4\le p<6\) and \( 0< \omega < \bar{m}\) or \(2< p < 4\) and \(\bar{m}\sqrt{p-1} > \omega \sqrt{5-p}\). Wang [23] established the same result to the range of parameters that \(2< p < 4\) and \(0< \sqrt{h(p)}\omega < \bar{m}\), where

$$\begin{aligned} h(p) {:}{=}1+\frac{(4-p)^2}{4(p-2)}. \end{aligned}$$

We now turn to the standing wave solutions for NSP. We again insert the same ansatz \(\psi (t,x) = e^{-i\mu t}u(x),\, u \in \mathbb {R}\) into NSP to obtain

$$\begin{aligned} \begin{aligned}&-\Delta u+2m\mu u-2qmu \phi -|u|^{p-2}u=0 \text{ in } \mathbb {R}^3,\\&\quad -\Delta \phi =-qm u^2 \text{ in } \mathbb {R}^3. \end{aligned} \end{aligned}$$
(7)

For any \(u\in H^1(\mathbb {R}^3)\), there exists a unique \(\phi _u\in D^{1,2}(\mathbb {R}^3)\) satisfying

$$\begin{aligned} -\Delta \phi _u=-qm u^2 \text{ in } \mathbb {R}^3, \end{aligned}$$
(8)

by Lax-Milgram theorem (note that actually \(\phi _u=-\frac{qm}{4\pi |x|}*u^2\)). We define the corresponding action integral as

$$\begin{aligned} \begin{aligned} I_\infty (u)&=\frac{1}{2}\int _{\mathbb {R}^3}|\nabla u|^2+2m\mu u^2-qmu^2\phi _u dx-\frac{1}{p}\int _{\mathbb {R}^3}|u|^pdx. \end{aligned} \end{aligned}$$
(9)

Then, by [12, Lemma 3.2], \((u,\phi )\in H^1(\mathbb {R}^3)\times D^{1,2}(\mathbb {R}^3)\) is a solution of (7) if and only if \(u\in H^1(\mathbb {R}^3)\) is a critical point of \(I_\infty \), and \(\phi =\phi _u\). It is also standard to show that \(I_\infty \in C^1(H^1(\mathbb {R}^3),\mathbb {R})\) and a critical point u of \(I_\infty \) satisfies

$$\begin{aligned} \begin{aligned} -\Delta u+&2m\mu u-2qmu \phi _u-|u|^{p-2}u=0 \text{ in } \mathbb {R}^3. \end{aligned} \end{aligned}$$
(10)

We summarize some existence results for problem (10). D’Aprile-Mugnai [12] and Coclite [7] proved the existence of a radial positive solution of (10) for \(4\le p<6\). On the other hand, using a Pohozaev equality, D’Aprile-Mugnai [13] showed that there exists no non-trivial solutions of (10) for \(p\le 2\) or \(p\ge 6\). By a new approach, Ruiz [21] fills a gap for the range \(2<p<4\). More precisely, he proved the following results:

  1. (i)

    (\(3<p<6\) and \(q>0\)) \(\exists \) a nontrivial solution, which is a ground state in radial class;

  2. (ii)

    (\(2<p<3\) and small \(q>0\)) \(\exists \) a nontrivial solution, which is a minimizer of \(I_\infty \);

  3. (iii)

    (\(2<p\le 3\) and small \(q>0\)) \(\exists \) a nontrivial solution emanating from a ground state solution of

    $$\begin{aligned} -\Delta u+2m\mu u-|u|^{p-2}u=0 \text{ in } \mathbb {R}^3; \end{aligned}$$
    (11)
  4. (iv)

    (\(2 < p \le 3\) and large \(q > 0\)) \(\not \exists \) nontrivial solution of (10).

In [1], Azzollini and Pomponio constructed a ground state solution of (10) for \(3<p<6\), which is possibly non-radial. It was shown by Colin and Watanabe [8] that a ground state is unique and radial up to a translation for small \(q > 0\). This result implies that the solution found by Ruiz coincides with the ground state constructed by Azzollini and Pomponio for small \(q > 0\) if \(3< p < 6\). As far as we know, it is unknown whether the ground states is radial when \(q > 0\) is arbitrary.

Concerning the nonrelativistic limit between solitary waves, one can naturally ask is the following:

Question: For any positive solution u of (10), is there a corresponding family of positive solutions \(u_c\) of (5), which converges to u as \(c\rightarrow \infty \)?

In this paper, we not only give a complete answer to this question, but also construct blow up solutions to NMKG for \(2<p<3\). Our first theorem states the convergence of nonrelativistic limit of ground states between (5) and (10) for \(3< p < 6\). The theorem contains the existence of a ground state to (5) for \(3< p < 4\) with arbitrary parameters \(m, q, \mu , c > 0\) and \(c > \sqrt{\mu /m}\), which is not covered by the aforementioned results of Azzollini-Pomponio [2] or Wang [23] (see Proposition 3).

Theorem 1

(Existence and nonrelativistic limit of ground states) Fix arbitrary \(\mu , m, q>0\) and \(3< p < 6\). Then there holds the following:

  1. (i)

    There exists a ground state solution of (5) for any \(c > \sqrt{\mu /m}\).

  2. (ii)

    Any ground state solution \(u_c\) of (5) belongs to \(H^2(\mathbb {R}^3)\), and there exists a sequence \(\{x_c\} \in \mathbb {R}^3\) such that \(\{u_c(\cdot +x_c)\}\) converges to a ground state solution of (10) in \(H^2(\mathbb {R}^3) \) as \(c\rightarrow \infty \), after choosing a subsequence.

Based on the strategies proposed in [10, 11], we shall prove the convergence of nonrelativistic limit in Theorem 1 by establishing the following steps:

  1. 1.

    Uniform upper estimate of ground energy levels for (5) by the ground energy level for (10), i.e.,

    $$\begin{aligned} \limsup _{c\rightarrow \infty }E_c \le E_\infty , \end{aligned}$$
    (12)

    where

    $$\begin{aligned} E_c = \inf \{ I_c(u) ~|~ u \ne 0,\, I_c'(u) = 0 \} \quad \text {and} \quad E_\infty = \inf \{ I_\infty (u) ~|~ u \ne 0,\, I_\infty '(u) = 0 \}; \end{aligned}$$
  2. 2.

    Uniform \(H^1\) bounds for ground states {\(u_c\}\) of (5) and solvability of its weak limit \(u_\infty \) to (10);

  3. 3.

    Energy estimates for establishing \(u_\infty \) to be a ground state;

  4. 4.

    \(H^1\) convergence of \(u_c\) to \(u_\infty \) and its upgrade to \(H^2\).

A new difficulty arises when we prove the step 1 in the case \(3< p < 4\). It is worth to point out that we couldn’t construct a ground state of (5) by using a constrained minimization method for \(3< p < 4\). It seems not possible to find a suitable constraint working for every admissible parameters \(\mu , m, q, c\). As a consequence, we couldn’t compare ground states energy levels between (5) and (10). To bypass the obstacle, we directly construct a ground state that satisfies the upper estimate (12). That is, we first show the existence of a family of nontrivial solutions to (5) satisfying the upper estimate (12) by applying a deformation argument developed in [5]. Then, by the compactness of a sequence of solutions to (5), we prove that aforementioned nontrivial solutions to (5) is ground state solutions to (5) (see Proposition 3).

The next theorem covers the case that \(2< p < 3\) and q is small. We recall the aforementioned results by Ruiz [21], which say the existence of at least two positive radial solutions \(u_\infty \) and \(v_\infty \) of (10); \(u_\infty \) is a perturbation of the ground state to (11) and \(v_\infty \) is a global minimizer of \(I_\infty \). In Theorem 2, we show the existence of two radial positive solutions \(u_{c}\) and \(v_{c}\) to (5) such that \(u_c\) and \(v_c\) converges to \(u_\infty \) and \(v_\infty \), respectively.

Theorem 2

(Correspondence of two positive solutions for \(2< p < 3\)) Assume \(2< p < 3\). Fix arbitrary but sufficiently small \(q > 0\) that guarantees the existence of at least two positive radial solutions \(u_\infty \) and \(v_\infty \) to (10) mentioned above. If \(c > 0\) is sufficiently large, then there exist two distinct radially symmetric positive solutions \(u_c\) and \(v_c\) of (5) such that

$$\begin{aligned} \text{(i) } \lim _{c\rightarrow \infty } \Vert u_c-u_\infty \Vert _{H^1(\mathbb {R}^3)} = 0, \qquad \text{(ii) } \lim _{c\rightarrow \infty }\Vert v_c-v_\infty \Vert _{H^1(\mathbb {R}^3)}= 0. \end{aligned}$$

In [21], Ruiz proved that a global minimizer \(v_\infty \) of \(I_\infty \) blows up in \(H^1\) as \(q\rightarrow 0\), which implies that the solution \(v_c\) constructed in Theorem 2 blows up in \(H^1\) as \(q\rightarrow 0\) and \(c\rightarrow \infty \). We point out that Theorem 2 not only proves the correspondence between solitary waves but also establishes a new existence result to (5) for \(2< p < 3\). As we have seen above, the previous approaches [2, 3, 12, 23] doesn’t cover the case that \(\omega > 0\) is less than but sufficiently close to \(\bar{m}\). In this respect, one family of solutions \(u_c\) is actually not brand new because it is a simple consequence of implicit function theorem, which relies on nondegeneracy of the solution \(u_\infty \). However, the other family of solutions \(v_c\) is brand new because \(v_c\) bifurcates from a global minimizer of \(I_\infty \), which blows up in \(H^1\). As for the construction of \(v_c\), it seems not easy to show whether the global minimum of \(I_c\) is finite, unlike \(I_\infty \). This prevents us from simply adopting the minimization argument. To overcome this difficulty, we develop a new deformation argument, which strongly depends on the fact that the global minimum level of \(I_\infty \) is bounded below. We conjecture that if c is sufficiently large, there exists a global minimizer of \(I_c\), which converges to \(v_\infty \).

We organize the paper as follows: In sect. 2, we give variational settings for NSP and NMKG, and a simple proof for the existence of a ground state to (6) for \(3<p<6\). Section 3 is devoted to construct nontrivial solutions to (5) with the energy bound \(E_\infty \) when \(3< p < 6\). In Sect. 4, we prove Theorem 1 by combining the results in Sect. 3. In Sect. 5, we deal with the case \(2<p<3\). We construct two radial positive solutions of (5) and prove the convergence of their nonrelativistic limit. Finally, in Appendix, we give basic estimates, which are used in the proofs of main theorems.

2 Preliminaries

This preliminary section introduces basic functional and variational settings for NMKG and NSP. In addition, we provide a simple proof for the existence of a ground state to (6) for every \(3< p < 6\) and every \(e,\bar{m}, \omega > 0\) such that \(\bar{m} > \omega \).

2.1 Function spaces

The space \(D^{1,2}(\mathbb {R}^3)\) is defined by the completion of \(C_0^\infty (\mathbb {R}^3)\) with respect to the norm

$$\begin{aligned} \Vert u\Vert _{D^{1,2}(\mathbb {R}^3)}=\Big (\int _\Omega |\nabla u|^2 dx\Big )^{1/2}. \end{aligned}$$

For an open set \(\Omega \subset \mathbb {R}^3\) and \(r\in [1,\infty )\), let us denote the norms

$$\begin{aligned} \Vert u\Vert _{L^r(\Omega )}=\Big (\int _\Omega |u|^r dx\Big )^{1/r}, \quad \Vert u\Vert _{L^\infty (\Omega )}={\mathop {{{\,\mathrm{ess\,sup}\,}}}\limits _{x\in \Omega }} |u(x)|, \quad \Vert u\Vert _{H^1(\Omega )}=\Big (\int _\Omega |\nabla u|^2+u^2 dx\Big )^{1/2}. \end{aligned}$$

We also use the following abbreviations,

$$\begin{aligned} \Vert u\Vert _{L^r}=\Vert u\Vert _{L^r(\mathbb {R}^3)}, \quad \Vert u\Vert _{D^{1,2}}=\Vert u\Vert _{D^{1,2}(\mathbb {R}^3)} \quad \text {and} \quad \Vert u\Vert _{H^1}=\Vert u\Vert _{H^1(\mathbb {R}^3)}. \end{aligned}$$

We denote by \(H^1_r\) the Sobolev space of radial functions u such that u, \(\nabla u\) are in \(L^2(\mathbb {R}^3)\).

2.2 Variaional settings for NSP

Recall the action functional for (10),

$$\begin{aligned} \begin{aligned} I_\infty (u)&=\frac{1}{2}\int _{\mathbb {R}^3}|\nabla u|^2+2m\mu u^2+|\nabla \phi _u|^2 dx-\frac{1}{p}\int _{\mathbb {R}^3}|u|^pdx\\&=\frac{1}{2}\int _{\mathbb {R}^3}|\nabla u|^2+2m\mu u^2-qmu^2\phi _u dx-\frac{1}{p}\int _{\mathbb {R}^3}|u|^pdx. \end{aligned} \end{aligned}$$

The map \(\lambda :u\in H^1\rightarrow \phi _u\in D^{1,2}\) is continuously differentiable, where \(\phi _u\) satisfies (8) (see [12]). Since \(\lambda ^\prime (u) [v]\) satisfies

$$\begin{aligned} -\Delta (\lambda ^\prime (u) [v])=-2qm uv \text{ in } \mathbb {R}^3 \qquad \text {for } v \in H^1, \end{aligned}$$

we have

$$\begin{aligned} \int _{\mathbb {R}^3}\nabla (\lambda ^\prime (u) [v])\cdot \nabla \phi _u dx=-2qm\int _{\mathbb {R}^3}uv\phi _u dx. \end{aligned}$$

Then we see that

$$\begin{aligned} I_\infty ^\prime (u)v&= \int _{\mathbb {R}^3}\nabla u\cdot \nabla v+2m\mu uv+\nabla (\lambda ^\prime (u) [v])\cdot \nabla \phi _u dx- \int _{\mathbb {R}^3}|u|^{p-2}uvdx\\&=\int _{\mathbb {R}^3}\nabla u\cdot \nabla v+2m\mu uv-2qmuv \phi _u dx- \int _{\mathbb {R}^3}|u|^{p-2}uvdx, \end{aligned}$$

which shows that a critical point of \(I_\infty \) is a weak solution to (10). We define the Nehari and Pohozaev functionals for (10) by

$$\begin{aligned} \begin{aligned} J_\infty (u)&\equiv I_\infty ^\prime (u)u=\int _{\mathbb {R}^3}|\nabla u|^2+2m\mu u^2-2qm u^2\phi _u-|u|^{p} dx, \\ P_\infty (u)&\equiv \int _{\mathbb {R}^3}\frac{1}{2}|\nabla u|^2+3m\mu u^2-\frac{5}{2} qm u^2\phi _u-\frac{3}{p}|u|^{p} dx. \end{aligned} \end{aligned}$$

We note that the values of \(J_\infty \) and \(P_\infty \) should be zero at every critical point of \(I_\infty \) (see [21]). By defining \(G_\infty (u)\equiv 2 J_\infty (u)-P_\infty (u)\), we denote

$$\begin{aligned} M_\infty \equiv \Big \{u\in H^1\setminus \{0\}\&\Big |\ G_\infty (u)\equiv \int _{\mathbb {R}^3}\frac{3}{2}|\nabla u|^2+m\mu u^2-\frac{3}{2} qm u^2\phi _u-\frac{2p-3}{p}|u|^{p} dx=0\Big \} \end{aligned}$$

and

$$\begin{aligned} E_\infty \equiv \inf _{u\in M_\infty }I_\infty (u). \end{aligned}$$
(13)

It is proved in [21] that for \(3< p < 6\), \(E_\infty \) equals to the ground energy level for (10), i.e.

$$\begin{aligned} E_\infty = \inf \{ I_\infty (u) ~|~ u \ne 0,\, I_\infty '(u) = 0 \}. \end{aligned}$$

2.3 Variational settings for NMKG

The action functional for (5) is given by

$$\begin{aligned} I_c(u)&=\frac{1}{2}\int _{\mathbb {R}^3}|\nabla u|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u^2+|\nabla \Phi _u|^2+\Big (\frac{q}{c}\Big )^2u^2\Phi _u^2dx-\frac{1}{p}\int _{\mathbb {R}^3}|u|^pdx\\&=\frac{1}{2}\int _{\mathbb {R}^3}|\nabla u|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u^2-q\Big (m-\frac{\mu }{c^2}\Big ) u^2 \Phi _u dx-\frac{1}{p}\int _{\mathbb {R}^3}|u|^pdx. \end{aligned}$$

The map \(\Lambda :u\in H^1\rightarrow \Phi _u\in D^{1,2}\) is continuously differentiable, where \(\Phi _u\) satisfies (4) (see [12]). For \(v\in H^1\), since \(\Lambda ^\prime (u) [v]\) satisfies

$$\begin{aligned} -\Delta (\Lambda ^\prime (u) [v])+\Big (\frac{q}{c}\Big )^2u^2 (\Lambda ^\prime (u) [v])=-2\Big (\frac{q}{c}\Big )^2uv \Phi _u-2q\Big (m-\frac{\mu }{c^2}\Big ) uv, \end{aligned}$$

we have

$$\begin{aligned} \int _{\mathbb {R}^3}\nabla (\Lambda ^\prime (u) [v])\cdot \nabla \Phi _u+\Big (\frac{q}{c}\Big )^2u^2 (\Lambda ^\prime (u) [v])\Phi _u dx=\int _{\mathbb {R}^3}-2\Big (\frac{q}{c}\Big )^2uv \Phi _u^2-2q\Big (m-\frac{\mu }{c^2}\Big ) uv\Phi _u dx. \end{aligned}$$

Then we see that for \(v\in H^1\),

$$\begin{aligned} I_c^\prime (u)v&=\int _{\mathbb {R}^3}\nabla u\cdot \nabla v+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )uv+\nabla \Phi _u\cdot \nabla (\Lambda ^\prime (u) [v])+\Big (\frac{q}{c}\Big )^2 uv \Phi _u^2\\&\quad +\Big (\frac{q}{c}\Big )^2u^2\Phi _u (\Lambda ^\prime (u) [v])-|u|^{p-2}uvdx\\&=\int _{\mathbb {R}^3}\nabla u\cdot \nabla v+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )uv-\Big (\frac{q}{c}\Big )^2 uv \Phi _u^2-2q\Big (m-\frac{\mu }{c^2}\Big ) u v\Phi _u-|u|^{p-2}uvdx. \end{aligned}$$

In particular, we have

$$\begin{aligned} J_c(u)\equiv I_c^\prime (u)u=\int _{\mathbb {R}^3}|\nabla u|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u^2-\Big (\frac{q}{c}\Big )^2 u^2 \Phi _u^2-2q\Big (m-\frac{\mu }{c^2}\Big ) u^2\Phi _u-|u|^{p} dx. \end{aligned}$$

For any critical point \(w_c\) of \(I_c\), it is clear that \(J_c(w_c) = 0\) and it is shown in [13] that the Pohozaev’s identity \(P_c(w_c)=0\) holds true, where

$$\begin{aligned} P_c(u)\equiv \int _{\mathbb {R}^3}\frac{1}{2} |\nabla u|^2+ \frac{3}{2}\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u^2-\frac{q^2}{c^2}\Phi _u^2u^2- \frac{5}{2}q\Big (m-\frac{\mu }{c^2}\Big )u^2\Phi _u -\frac{3}{p}|u|^pdx. \end{aligned}$$

2.4 Existence of a ground state for \(3< p < 6\)

We recall the equation (6)

$$\begin{aligned} -\Delta u+\big (\bar{m}^2-(e\varphi _u+\omega )^2\big )u=|u|^{p-2}u \text{ in } \mathbb {R}^3 \end{aligned}$$

where \(e>0\), \(0<\omega <\bar{m}\) and \(\varphi _u\) is a unique solution of

$$\begin{aligned} -\Delta \varphi +e^2\varphi u^2=-e\omega u^2. \end{aligned}$$

Here we point out that by the maximum principle, we have the uniform bound

$$\begin{aligned} -\frac{\omega }{e}\le \varphi _u\le 0. \end{aligned}$$

Proposition 3

Assume that \(3<p<6\), \(e>0\) and \(0<\omega <\bar{m}\). If there exists a non-trivial solution of (6), then there exists a non-trivial ground state solution of (6).

Proof

Suppose that there exists a non-trivial solution solution of (6). We recall the action functional of (6)

$$\begin{aligned} I(u) =\frac{1}{2}\int _{\mathbb {R}^3}|\nabla u|^2+(\bar{m}^2-\omega ^2)u^2-e\omega \varphi _u u^2dx-\frac{1}{p}\int _{\mathbb {R}^3}|u|^pdx. \end{aligned}$$

and consider the minimization problem

$$\begin{aligned} \mathcal {S}=\inf \{ I(u)\ | \ u \in \mathcal {B}\}, \end{aligned}$$

where

$$\begin{aligned} \mathcal {B}\equiv \{ u \in H^1\ | \ u \text{ is } \text{ a } \text{ non-trivial } \text{ solution } \text{ solution } \text{ of } (6)\}. \end{aligned}$$

By the definition, a ground state solution u of (6) is a nontrivial critical point of I satisfying \(I(u) = \mathcal {S}\). Let us define

$$\begin{aligned} \left\{ \begin{aligned} T(u)&{:}{=}I^\prime (u)u= \int _{\mathbb {R}^3}|\nabla u|^2+(\bar{m}^2-\omega ^2)u^2-2e\omega \varphi _u u^2-e^2\varphi _u^2 u^2-|u|^pdx \\ Q(u)&{:}{=}\int _{\mathbb {R}^3}\frac{1}{2}|\nabla u|^2+\frac{3}{2}(\bar{m}^2-\omega ^2)u^2-\frac{5}{2}e\omega \varphi _u u^2-e^2\varphi _u^2 u^2-\frac{3}{p}|u|^pdx. \end{aligned}\right. \end{aligned}$$

Since \(T(v)=Q(v)=0\) for any \(v \in \mathcal {B}\), (see [13]), one has

$$\begin{aligned} \frac{5p-12}{2}I(v)&=\frac{5p-12}{2}I(v)-T(v)+\frac{4-p}{2}Q(v) \\&=\int _{\mathbb {R}^3}(p-3)|\nabla v|^2+\frac{p-2}{2}(\bar{m}^2-\omega ^2)v^2+\frac{p-2}{2}e^2 v^2\varphi _{v}^2dx \end{aligned}$$

for \(v\in \mathcal {B}\). This implies that \(\mathcal {S}\ge 0\).

Let \(\{u_n\}\) be a minimizing sequence of \(\mathcal {S}\). From the estimates

$$\begin{aligned} \begin{aligned} \frac{5p-12}{2}\mathcal {S}+o(1) =\int _{\mathbb {R}^3}(p-3)|\nabla u_n|^2+\frac{p-2}{2}(\bar{m}^2-\omega ^2)u_n^2+\frac{p-2}{2}e^2 u_n^2\varphi _{u_n}^2dx \end{aligned} \end{aligned}$$
(14)

and

$$\begin{aligned} 0=T(u_n)&= \int _{\mathbb {R}^3}|\nabla u_n|^2+(\bar{m}^2-\omega ^2)u_n^2- \varphi _{u_n}(2e\omega +e^2\varphi _{u_n} )u_n^2-|u_n|^pdx\\&\ge \int _{\mathbb {R}^3}|\nabla u_n|^2+(\bar{m}^2-\omega ^2)u_n^2-|u_n|^pdx\ge C\Vert u_n\Vert _{L^p}^{2/p}-\Vert u_n\Vert _{L^p}^p, \end{aligned}$$

we deduce that \((u_n)\) is bounded in \(H^1\) and \(\Vert u_n\Vert _{L^p}\ge C_1\) for some positive constant \(C_1\). Then we see from Lemma 1.1 in [14],

$$\begin{aligned} \sup _{x\in \mathbb {R}^3}\int _{B_1(x)}|u_n|^2dx=\int _{B_1(x_n)}|u_n|^2dx\ge C_2>0, \end{aligned}$$

where \(x_n\in \mathbb {R}^3\) and \(C_2\) is a positive constant. Then we may assume that \(u_n(\cdot +x_n)\) converges to \(u\not \equiv 0\) weakly in \(H^1\). It is standard to show that u is a non-trivial critical point of I. Moreover, by (14) and the fact that u is a non-trivial critical point of I, we see that

$$\begin{aligned} \frac{5p-12}{2}\mathcal {S}&=\liminf _{n\rightarrow \infty }\int _{\mathbb {R}^3}(p-3)|\nabla u_n|^2+\frac{p-2}{2}(\bar{m}^2-\omega ^2)u_n^2+\frac{p-2}{2}e^2 u_n^2\varphi _{u_n}^2dx\\&\ge \int _{\mathbb {R}^3}(p-3)|\nabla u|^2+\frac{p-2}{2}(\bar{m}^2-\omega ^2)u^2+\frac{p-2}{2}e^2 u^2\varphi _{u}^2dx =\frac{5p-12}{2}I(u), \end{aligned}$$

which implies that u is a non-trivial ground state solution of (6). \(\square \)

Observe that Proposition 3 implies the existence of a ground state to (6) for any \(e, \bar{m}, \omega > 0\) such that \(0< \omega < \bar{m}\) since there exists a nontrivial solution at those ranges of parameters by [3].

3 Construction of nontrivial solutions to NKGM with the energy bound \(E_\infty \)

In this section, based on the idea of [5], we shall construct a family of nontrivial solutions \(w_c\) to (5) satisfying

$$\begin{aligned} \limsup _{c\rightarrow \infty }I_c(w_c) \le E_{\infty }. \end{aligned}$$

Before proceeding further, we first introduce a modified functional \(\tilde{I}_c\) as

$$\begin{aligned} \tilde{I}_c(u)=\frac{1}{2}\int _{\mathbb {R}^3}|\nabla u|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u^2-q\Big (m-\frac{\mu }{c^2}\Big ) u ^2 \Phi _{u} dx-\frac{1}{p}\int _{\mathbb {R}^3}u_+^pdx, \end{aligned}$$

where \(c>0\) and \(u_+=\max \{u,0\}\). A critical point of \(\tilde{I}_c\) corresponds to a solution of

$$\begin{aligned} \begin{aligned}&-\Delta u+\Big (2m\mu -\Big (\frac{\mu }{c}\Big )^2\Big )u-\Big (\frac{q}{c}\Big )^2u \Phi ^2-2q\Big (m-\frac{\mu }{c^2}\Big )u\Phi -u_+^{p-1}=0 \text{ in } \mathbb {R}^3,\\&\quad -\Delta \Phi +\frac{q^2}{c^2}u^2\Phi =-q\Big (m-\frac{\mu }{c^2}\Big ) u^2 \text{ in } \mathbb {R}^3. \end{aligned} \end{aligned}$$
(15)

It is possible to show from the maximum principle that a critical point u of \(\tilde{I}_c\) is positive everywhere in \(\mathbb {R}^3\) for \(c\ge \sqrt{\frac{2m}{\mu }}\). Indeed, since \(-\frac{c^2}{q}\Big (m-\frac{\mu }{c^2}\Big )\le \Phi _u \le 0 \), multiplying \(u_-\) to the equation

$$\begin{aligned} -\Delta u+ \Big (2m\mu -\big (\frac{\mu }{c}\big )^2\Big )u-\Big (\frac{q}{c}\Big )^2u \Phi _u^2-2q\Big (m-\frac{\mu }{c^2}\Big )u\Phi _u -u_+^{p-1}=0 \text{ in } \mathbb {R}^3 \end{aligned}$$

and then integrating over \(\mathbb {R}^3\), we have

$$\begin{aligned}&\int _{\mathbb {R}^3}|\nabla u_-|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u_-^2dx\\&\quad \le \int _{\mathbb {R}^3}|\nabla u_-|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u_-^2-u_-^2 \Phi _u\Big [\Big (\frac{q}{c}\Big )^2 \Phi _u+2q\Big (m-\frac{\mu }{c^2}\Big ) \Big ] dx=0, \end{aligned}$$

where \(u_-=\min \{u,0\}\). Therefore a nontrivial critical point of \(\tilde{I}_c\) gives a positive solution to (5). We also define

$$\begin{aligned} \tilde{I}_\infty (u)&{:}{=}\frac{1}{2}\int _{\mathbb {R}^3}|\nabla u|^2+2m\mu u^2-qmu ^2\phi _{u} dx-\frac{1}{p}\int _{\mathbb {R}^3}u_+^pdx, \\ \tilde{J}_\infty (u)&{:}{=}I_\infty ^\prime (u)u=\int _{\mathbb {R}^3}|\nabla u|^2+2m\mu u^2-2qm u ^2\phi _{u}-u_+^{p} dx, \\ \tilde{P}_\infty (u)&{:}{=}\int _{\mathbb {R}^3}\frac{1}{2}|\nabla u|^2+3m\mu u^2-\frac{5}{2} qm u ^2\phi _{u}-\frac{3}{p}u_+^{p} dx. \end{aligned}$$

Let \(\mathcal {A}\equiv \{u\in H^1\ | \ \tilde{I}_\infty ^\prime (u)=0, \tilde{I}_\infty (u)=E_\infty , \text{ and } \max _{\mathbb {R}^3}u=u(0)\}.\) We note that \(\mathcal {A}\ne \emptyset \). Indeed, if \(u\in \mathcal {M}_\infty \) satisfies \(I_\infty (u)=E_\infty \), we see that |u| satisfies \(\tilde{I}_\infty (|u|)=E_\infty \) and \(\tilde{I}_\infty ^\prime (|u|)=0\).

Proposition 4

For \(3< p<6\), there exist positive constants \(C_1\) and \(C_2\) independent of \(U\in \mathcal {A}\) such that for \(U\in \mathcal {A}\),

$$\begin{aligned} U(x)+|\nabla U(x)|\le C_1 \exp (-C_2|x|). \end{aligned}$$

Moreover, \(\inf _{U\in \mathcal {A}}\Vert U\Vert _{L^\infty }>0\).

Proof

Let \(U\in \mathcal {A}\). It follows from

$$\begin{aligned} \begin{aligned} E_\infty&=\tilde{I}_\infty (U)=\tilde{I}_\infty (U)-\frac{2}{5p-12}\tilde{J}_\infty (U)-\frac{p-4}{5p-12}\tilde{P}_\infty (U)\\&=\int _{\mathbb {R}^3}\frac{2(p-3)}{5p-12}|\nabla U|^2+\frac{2(p-2)}{5p-12}m\mu U^2dx \end{aligned} \end{aligned}$$
(16)

where \(U\in \mathcal {A},\) that \(\mathcal {A}\) is bounded in \(H^1\) if \(3< p<6\). Then, since

$$\begin{aligned} \Vert \phi _{U}+|U|^{p-2}\Vert _{L^\frac{6}{p-2}(\Omega )}&\le \Vert \phi _{U}\Vert _{L^\frac{6}{p-2}(\Omega )}+\Vert U\Vert _{L^6(\Omega )}^{p-2}\le |\Omega |^{\frac{p-2}{6}-\frac{1}{6}}\Vert \phi _{U}\Vert _{L^6(\Omega )}+\Vert U\Vert _{L^6(\Omega )}^{p-2}\\&\le C\big (|\Omega |^{\frac{p-2}{6}-\frac{1}{6}}\Vert U\Vert _{H^1}^2 +\Vert U\Vert _{H^1}^{p-2}\big ), \end{aligned}$$

where \(3<p<6\), \(U\in \mathcal {A}\), \(\Omega \) is a bounded domain in \(\mathbb {R}^3\) and C is a positive constant independent of \(U\in \mathcal {A}\), we see that \(\mathcal {A}\) is bounded in \(L^\infty \) (see [22, Theorem 4.1]).

We claim that \(\lim _{|x|\rightarrow \infty } U(x)=0\) uniformly for \(U\in \mathcal {A}\). Indeed, contrary to our claim, suppose that there exist \(\{U_i\}_{i=1}^\infty \subset \mathcal {A}\) and \(\{x_i\}_{i=1}^\infty \subset \mathbb {R}^N\) satisfying \(\lim _{i\rightarrow \infty }|x_i|=\infty \) and \(\liminf _{i\rightarrow \infty }U_i(x_i)>0\). Denote \(V_i\equiv U_i(\cdot +x_i)\). We note that if \(u_i\rightharpoonup u\) in \(H^1\), \(\phi _{u_i}\rightharpoonup \phi _{u}\) in \(D^{1,2}\). Then if \(u_i\rightharpoonup u\) in \(H^1\), for \(\psi \in C_0^\infty (\mathbb {R}^3)\),

$$\begin{aligned} \int _{\mathbb {R}^3}(u_i \phi _{u_i}-u\phi _{u})\psi dx=\int _{\mathbb {R}^3}(u_i-u) \phi _{u_i}\psi +u(\phi _{u_i}-\phi _{u})\psi dx=o(1) \end{aligned}$$
(17)

as \(i\rightarrow \infty \). By (17) and the fact that \(\{U_i, V_i\}_{i=1}^\infty \) is bounded in \(H^1\), we see that \(U_i\) and \(V_i\) converge to U and V weakly in \(H^1\) as \(i\rightarrow \infty \) , up to a subsequence, respectively, where U and V are non-trivial solutions of (10). It follows from (16) that for \(2R\le |x_i|\),

$$\begin{aligned} \begin{aligned} E_\infty&=\liminf _{i\rightarrow \infty }\tilde{I}_\infty (U_i)=\liminf _{i\rightarrow \infty }\int _{\mathbb {R}^3}\frac{2(p-3)}{5p-12}|\nabla U_i|^2+\frac{2(p-2)}{5p-12}m\mu U_i^2dx\\&\ge \liminf _{i\rightarrow \infty }\int _{B(0,R)}\frac{2(p-3)}{5p-12}|\nabla U_i|^2 +\frac{2(p-2)}{5p-12}m\mu U_i^2dx\\&\quad +\liminf _{i\rightarrow \infty }\int _{B(x_i,R)}\frac{2(p-3)}{5p-12}|\nabla U_i|^2+\frac{2(p-2)}{5p-12}m\mu U_i^2dx\\&\ge \int _{B(0,R)}\frac{2(p-3)}{5p-12}|\nabla U |^2 +\frac{2(p-2)}{5p-12}m\mu U ^2dx\\&\quad +\int _{B(0,R)}\frac{2(p-3)}{5p-12}|\nabla V|^2+\frac{2(p-2)}{5p-12}m\mu V^2dx. \end{aligned} \end{aligned}$$
(18)

Since

$$\begin{aligned} \tilde{I}_\infty (U), \tilde{I}_\infty (V)\ge \tilde{I}_\infty (W) \text{ for } \text{ any } W\in \mathcal {A}, \end{aligned}$$

if we take large \(R>0\) in (18), we deduce a contradiction. This implies that \(\lim _{|x|\rightarrow \infty }U(x)=0\) uniformly for \(U\in \mathcal {A}\).

We note that for large |x|,

$$\begin{aligned} \begin{aligned} \phi _{U}(x)&=-\frac{qm}{4\pi }\int _{\mathbb {R}^3}\frac{U ^2(y)}{|x-y|}dy =-\frac{qm}{4\pi }\int _{B(x,R)}\frac{U^2(y)}{|x-y|}dy-\frac{qm}{4\pi }\int _{\mathbb {R}^3\setminus B(x,R)}\frac{U ^2(y)}{|x-y|}dy\\&=o(1)R^2+O(1)\frac{1}{R}=o(1) \end{aligned} \end{aligned}$$

uniformly in \(U\in \mathcal {A}\). Then, by the comparison principle and the elliptic estimates, we see that for \(U\in \mathcal {A}\),

$$\begin{aligned} U(x)+|\nabla U(x)|\le C_1 \exp (-C_2|x|), \end{aligned}$$

where \(C_1\) and \(C_2\) are positive constants independent of \(U\in \mathcal {A}\).

To show \(\inf _{U\in \mathcal {A}}\Vert U\Vert _{L^\infty }>0\), we assume that there exists \(\{U_i\}_{i=1}^\infty \subset \mathcal {A}\) such that \(\Vert U_i\Vert _{L^\infty }\rightarrow 0\) as \(i\rightarrow \infty \). Then, since \(U_i\) satisfies

$$\begin{aligned} -\Delta U_i+2m\mu U_i-U_i^{p-1}\le -\Delta U_i+ 2m\mu U_i -2qm U_i \phi _{U_i}-U_i^{p-1}=0 \text{ in } \mathbb {R}^3, \end{aligned}$$

we see that \(\Vert U_i\Vert _{H^1}\rightarrow 0\) as \(i\rightarrow \infty \), which is a contradiction to (16). \(\square \)

For a fixed \(U_0\in \mathcal {A}\), we define \(\gamma (t)(x)=t^2U_0(tx)\). It follows from

$$\begin{aligned} \tilde{I}_\infty (\gamma (t))=\frac{1}{2}\int _{\mathbb {R}^3}t^3|\nabla U_0|^2+2m\mu tU_0^2-qmt^3U_0 ^2\phi _{U_0 } dx-\frac{t^{2p-3}}{p}\int _{\mathbb {R}^3}U_0^pdx \end{aligned}$$

that for \(3<p<6\), there exists \(t_0>1\) such that \(\tilde{I}_\infty (\gamma (t))<0\) for \(t\ge t_0\). Moreover, by [21, Lemma 3.3] and the fact that \(U_0\) is a critical point of \(\tilde{I}_\infty \), we see that for \(3<p<6\), \(t=1\) is a unique critical point of \(\tilde{I}_\infty (\gamma (t))\), corresponding to its maximum.

We define

$$\begin{aligned} \hat{e}_c {:}{=}\max _{t\in [0,t_0]}\tilde{I}_c(\gamma (t)), \quad \text {and} \quad e_c{:}{=}\inf _{\Gamma \in \mathcal {W}}\max _{s\in [0,1]}\tilde{I}_c(\Gamma (s)), \end{aligned}$$

where \(\mathcal {W}\equiv \{\Gamma \in C([0,1],H^1)\ | \ \Gamma (0)=0, \Gamma (1)=\gamma (t_0)\}\).

Proposition 5

Let \(3<p<6\). Then we have

$$\begin{aligned} \limsup _{c\rightarrow \infty } \hat{e}_c\le E_\infty . \end{aligned}$$

Proof

We see from Lemma 21 and the scaling \(\phi _{t^2U_0(t\cdot )}=t^2\phi _{U_0}(t\cdot ),\) that for \(t\in [0,t_0]\),

$$\begin{aligned} \begin{aligned} \tilde{I}_c(\gamma (t))&=\frac{1}{2}\int _{\mathbb {R}^3}|t^3(\nabla U_0)(tx)|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )t^4U_0^2(tx)-q\Big (m-\frac{\mu }{c^2}\Big )t^4 U_0^2(t x) \Phi _{t^2U_0(t\cdot )} dx\\&\quad -\frac{t^{2p}}{p}\int _{\mathbb {R}^3}(U_0(tx))^pdx\\&=\frac{1}{2}\int _{\mathbb {R}^3}|t^3(\nabla U_0)(tx)|^2+ 2m\mu t^4U_0^2(tx)-q m t^4 U_0^2(t x) \phi _{t^2U_0(t\cdot )} dx\\&\quad -\frac{t^{2p}}{p}\int _{\mathbb {R}^3}(U_0(tx))^pdx+o(1)\\&= \frac{1}{2} \int _{\mathbb {R}^3}t^3|\nabla U_0|^2+2m\mu tU_0^2-qmt^3U_0^2\phi _{U_0} dx-\frac{t^{2p-3}}{p}\int _{\mathbb {R}^3}(U_0)^pdx+o(1)\\&=\tilde{I}_\infty (\gamma (t))+o(1), \end{aligned} \end{aligned}$$
(19)

where o(1) is uniform in \(t\in [0,t_0]\) as \(c\rightarrow \infty \). Thus, since \(t=1\) is a unique maximum point of \(\tilde{I}_\infty (\gamma (t))\) for \(3<p<6\), we deduce that

$$\begin{aligned} \hat{e}_c = \max _{s\in [0,1]}\tilde{I}_c(\gamma (t_0s))=\tilde{I}_\infty (U_0)+o(1) =E_\infty +o(1) \end{aligned}$$

as \(c\rightarrow \infty \). \(\square \)

Proposition 6

Let \(3<p<6\). Then we have

$$\begin{aligned} \liminf _{c\rightarrow \infty }e_c\ge E_\infty . \end{aligned}$$

Proof

We note that for \(\Gamma \in \mathcal {W}\),

$$\begin{aligned} \tilde{I}_c(\Gamma (t))&=\frac{1}{2}\int _{\mathbb {R}^3}|\nabla \Gamma (t)|^2+ 2m\mu \Gamma ^2(t)-qm \Gamma ^2(t) \phi _{\Gamma (t)} dx-\frac{1}{p}\int _{\mathbb {R}^3}(\Gamma (t))_+^pdx\\&\quad -\frac{1}{c^2}\int _{\mathbb {R}^3}\mu ^2\Gamma ^2(t)- q\mu \Gamma ^2(t) \Phi _{\Gamma (t)} dx-\frac{1}{2} qm\int _{\mathbb {R}^3}\Gamma ^2(t)(\Phi _{\Gamma (t)} -\phi _{\Gamma (t)})dx\\&=\tilde{I}_\infty (\Gamma (t))+G_c(t), \end{aligned}$$

where \(G_c(t)\equiv -\frac{1}{c^2}\int _{\mathbb {R}^3}\mu ^2\Gamma ^2(t)- q\mu \Gamma ^2(t) \Phi _{\Gamma (t)} dx-\frac{1}{2} qm\int _{\mathbb {R}^3}\Gamma ^2(t)(\Phi _{\Gamma (t)} -\phi _{\Gamma (t)})dx\). By Lemma 21, we have

$$\begin{aligned} |G_c(t)|=o(1) \text{ uniformly } \text{ in } t\in [0,1] \text{ as } c\rightarrow \infty . \end{aligned}$$

Then, since

$$\begin{aligned} \max _{t\in [0,1]}\tilde{I}_\infty (\Gamma (t))\ge E_\infty , \end{aligned}$$

where \(\Gamma \in \mathcal {W}\) (see [1, Lemma 2.4]), we have

$$\begin{aligned} e_c&\ge E_\infty +\inf _{\Gamma \in \mathcal {W}}\max _{t\in [0,1]}G_c(t) \ge E_\infty -\inf _{\Gamma \in \mathcal {W}}\max _{t\in [0,1]}|G_c(t)|= E_\infty +o(1) \end{aligned}$$

as \(c\rightarrow \infty \). \(\square \)

We define

$$\begin{aligned} \mathcal {X} \equiv \{U(\cdot -y)\ | \ U\in \mathcal {A}, y\in \mathbb {R}^3\} \end{aligned}$$

and

$$\begin{aligned} N_d(\mathcal {X})\equiv \{u\in H^1\ | \ \inf _{v\in \mathcal {X}}\Vert u-v\Vert _{H^1}\le d\}, \end{aligned}$$

where \(d>0\) is a constant and \(\mathcal {A}\equiv \{u\in H^1\ | \ \tilde{I}_\infty ^\prime (u)=0, \tilde{I}_\infty (u)=E_\infty , \text{ and } \max _{\mathbb {R}^3}u=u(0)\}.\)

Proposition 7

Let \(3<p<6\). For large \(c>0\), for small \(d>0\), and for any \(d^\prime \in (0,d)\), there exists \(\nu \equiv \nu (d,d^\prime )>0\) independent of \(c>0\) such that

$$\begin{aligned} \inf \{\Vert \tilde{I}_c^\prime (u)\Vert _{H^{-1}}\ | \ \tilde{I}_c(u)\le \hat{e}_c, u\in N_d(\mathcal {X})\setminus N_{d^\prime }(\mathcal {X})\}\ge \nu >0. \end{aligned}$$

Proof

Let \(\{c_i\}_{i=1}^\infty \) be such that \(\lim _{i\rightarrow \infty }c_i=\infty \). It suffices to show that for small \(d>0\), if

$$\begin{aligned} u_{c_i}\in N_{d}(\mathcal {X}),\ \ \tilde{I}_{c_i}(u_{c_i})\le \hat{e}_{c_i},\ \text{ and } \ \Vert \tilde{I}^\prime _{c_i}(u_{c_i})\Vert _{H^{-1}}\rightarrow 0 \end{aligned}$$

as \(i\rightarrow \infty \), then

$$\begin{aligned} \inf _{v\in \mathcal {X}}\Vert u_{c_i}-v\Vert _{H^1}\rightarrow 0 \text{ as } i\rightarrow \infty . \end{aligned}$$

For the sake of simplicity of notation, we write c for \(c_i\). Since \(u_c\in N_{d}(\mathcal {X})\), we have

$$\begin{aligned} \Vert u_c(x)-U_c(x-y_c)\Vert _{H^1}\le d, \end{aligned}$$
(20)

where \(U_c\in \mathcal {A}\) and \(y_c\in \mathbb {R}^3\). We define \(\eta \in C_0^\infty (\mathbb {R}^3)\) such that \(0\le \eta \le 1\), \(\eta (x)=1\) for \(|x|\le 1\), \(\eta (x)=0\) for \(|x|\ge 2\), and \(|\nabla \eta |\le 2\). Also, we set \(\tilde{\eta }_c(x)=\eta (\frac{x-y_c}{c})\). We divide the proof into three steps.

Step 1. \( \tilde{I}_c(u_c)\ge \tilde{I}_\infty (v_c)+\tilde{I}_\infty (w_c)+o(1)\) as \(c\rightarrow \infty \), where \(v_c =\tilde{\eta }_cu_c\) and \(w_c=(1-\tilde{\eta }_c)u_c\).

We claim first that for \(\alpha \in (2,6)\),

$$\begin{aligned} \lim _{c\rightarrow \infty }\int _{B(y_c,2c)\setminus B(y_c,c)}|u_c|^{\alpha }dx=0. \end{aligned}$$
(21)

Suppose that there exist \(z_c\in B(y_c,2c)\setminus B(y_c,c)\) and \(R>0\) such that

$$\begin{aligned} \liminf _{c\rightarrow \infty }\int _{B(z_c,R)}|u_c|^2dx>0. \end{aligned}$$
(22)

Denote \(\tilde{u}_c=u_c(\cdot +z_c)\). We note that, by Lemma 21 and the fact that \(\Vert u_c\Vert _{H^1}\) is bounded, for \(\psi \in C_0^\infty (\mathbb {R}^3)\),

$$\begin{aligned} \begin{aligned}&\tilde{I}_c^\prime (\tilde{u}_c)\psi \\&\quad =\int _{\mathbb {R}^3}\nabla \tilde{u}_c\cdot \nabla \psi +\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )\tilde{u}_c \psi -\Big (\frac{q}{c}\Big )^2 \tilde{u}_c \psi \Phi _{\tilde{u}_c}^2-2q\Big (m-\frac{\mu }{c^2}\Big ) \tilde{u}_c \psi \Phi _{\tilde{u}_c}-(\tilde{u}_c)_+^{p-1} \psi dx\\&\quad =\int _{\mathbb {R}^3}\nabla \tilde{u}_c\cdot \nabla \psi +2m\mu \tilde{u}_c \psi -2qm \tilde{u}_c \psi \phi _{\tilde{u}_c}-(\tilde{u}_c)_+^{p-1} \psi dx\\&\quad + \int _{\mathbb {R}^3}-\frac{\mu ^2}{c^2}\tilde{u}_c \psi -\Big (\frac{q}{c}\Big )^2 \tilde{u}_c \psi \Phi _{\tilde{u}_c}^2+2q\frac{\mu }{c^2} \tilde{u}_c \psi \Phi _{\tilde{u}_c}-2qm \tilde{u}_c \psi (\Phi _{\tilde{u}_c}-\phi _{\tilde{u}_c})dx\\&\quad =\tilde{I}_\infty ^\prime (\tilde{u}_c)\psi +o(1) \end{aligned} \end{aligned}$$
(23)

as \(c\rightarrow \infty \). By (17) and the assumption that \(\Vert \tilde{I}^\prime _{c}(u_{c})\Vert _{H^{-1}}\rightarrow 0\) as \(c\rightarrow \infty \) , we have \(u_c(\cdot +z_c)\rightharpoonup \tilde{U}\not \equiv 0\) in \(H^1\), where \(\tilde{U}\) satisfies \(\tilde{I}_\infty ^\prime (\tilde{U})=0\). By (16), we have

$$\begin{aligned} \int _{\mathbb {R}^3}|\nabla \tilde{U}|^2+\tilde{U}dx\ge E_\infty \Big (\max \Big \{\frac{2(p-3)}{5p-12},\frac{2(p-2)}{5p-12}m\mu \Big \}\Big )^{-1}. \end{aligned}$$
(24)

Then, by Proposition 4 and the fact that \(|z_c-y_c|\ge c\), we see that for \(R>0\),

$$\begin{aligned} d^2&\ge \Vert u_c(x)-U_c(x-y_c)\Vert _{H^1}^2 =\Vert \tilde{u}_c(x)-U_c(x+z_c-y_c)\Vert _{H^1}^2\\&\ge \Vert \tilde{u}_c(x)-U_c(x+z_c-y_c)\Vert _{H^1(B(0,R))}^2 =\Vert \tilde{u}_c(x)\Vert _{H^1(B(0,R))}^2+o(1)\ge \Vert \tilde{U}\Vert _{H^1(B(0,R))}^2 \end{aligned}$$

as \(c\rightarrow \infty \). If we take small \(d>0\), by (24), we deduce a contradiction. Since there does not exists such a sequence \(\{z_c\}\) satisfying (22), by [14, Lemma 1.1], we deduce (21). Then, by (21), we have

$$\begin{aligned} \int _{\mathbb {R}^3}(u_c)_+^p-(v_c)_+^p-(w_c)_+^pdx=o(1) \end{aligned}$$
(25)

as \(c\rightarrow \infty \), where \(v_c\) and \(w_c\) are given in (21) above. By (21) and Lemma 17,

$$\begin{aligned} \int _{B(y_c,2c)\setminus B(y_c,c)}u_c^2|\phi _{u_c}|dx&\le \Vert \phi _{u_c}\Vert _{L^6(B(y_c,2c)\setminus B(y_c,c))}\Vert u_c^2\Vert _{L^{6/5}(B(y_c,2c)\setminus B(y_c,c))}\\&\le C_1\Vert u_c\Vert _{H^1}^2\Vert u_c\Vert _{L^{12/5}(B(y_c,2c)\setminus B(y_c,c))}^2\rightarrow 0 \end{aligned}$$

as \(c\rightarrow \infty \), where \(C_1\) is a positive constant. From this and the fact that \(|\nabla \eta _c|\le 2/c\), we see that

$$\begin{aligned} \begin{aligned}&\int _{\mathbb {R}^3}v_c^2 \phi _{v_c}+w_c^2 \phi _{w_c}-u_c^2 \phi _{u_c}dx\\&\quad =\int _{ B(y_c,c)\cup (\mathbb {R}^3\setminus B(y_c,2c) )}v_c^2 \phi _{v_c}+w_c^2 \phi _{w_c}-u_c^2 \phi _{u_c}dx+o(1)\\&\quad =\frac{qm}{4\pi }\int _{B(y_c,c)\cup (\mathbb {R}^3\setminus B(y_c,2c) )}\int _{\mathbb {R}^3}\frac{u_c^2(x)u_c^2(y)-v_c^2(x)v_c^2(y)-w_c^2(x)w_c^2(y)}{|x-y|}dydx+o(1)\\&\quad =\frac{qm}{4\pi }\int _{B(y_c,c)}\int _{\mathbb {R}^3}\frac{u_c^2(x)(u_c^2(y)-v_c^2(y))}{|x-y|}dydx\\&\qquad +\frac{qm}{4\pi }\int _{\mathbb {R}^3\setminus B(y_c,2c)}\int _{\mathbb {R}^3}\frac{u_c^2(x)(u_c^2(y)-w_c^2(y))}{|x-y|}dydx+o(1)\ge o(1) \end{aligned} \end{aligned}$$
(26)

as \(c\rightarrow \infty \). Thus, by (25), (26), Lemma 21 and the fact that \(|\nabla \eta _c|\le 2/c\), we have

$$\begin{aligned} \tilde{I}_c(u_c)&=\frac{1}{2}\int _{\mathbb {R}^3}|\nabla u_c|^2+ 2m\mu u_c^2-qm u_c^2 \phi _{u_c} dx-\frac{1}{p}\int _{\mathbb {R}^3}(u_c)_+^pdx\\&\quad -\frac{1}{2c^2}\int _{\mathbb {R}^3}\mu ^2u_c^2- q\mu u_c^2 \Phi _{u_c} dx-\frac{1}{2} qm\int _{\mathbb {R}^3}u_c^2(\Phi _{u_c} -\phi _{u_c})dx\\&\ge \tilde{I}_\infty (v_c)+\tilde{I}_\infty (w_c)+\int _{\mathbb {R}^3}\nabla v_c\cdot \nabla w_c+2m\mu v_c w_cdx+o(1)\\&=\tilde{I}_\infty (v_c)+\tilde{I}_\infty (w_c)+\int _{\mathbb {R}^3}(1-\tilde{\eta }_c)\tilde{\eta }_c|\nabla u_c|^2+2m\mu (1-\tilde{\eta }_c)\tilde{\eta }_cu_c^2dx+o(1)\\&\ge \tilde{I}_\infty (v_c)+\tilde{I}_\infty (w_c)+o(1) \end{aligned}$$

as \(c\rightarrow \infty \).

Step 2. \(\tilde{I}_\infty (w_c)\ge 0\) for large c, where \(w_c=(1-\tilde{\eta }_c)u_c\).

We note that, by Lemma 17,

$$\begin{aligned} \Big |\int _{\mathbb {R}^3} w_c^2 \phi _{w_c} dx\Big |&\le \Vert \phi _{w_c}\Vert _{L^6}\Vert w_c^2\Vert _{L^{6/5}} \le C_2\Vert w_c\Vert _{H^1}^4, \end{aligned}$$

where \(C_2\) is a positive constant independent of c. Moreover, by (20) and Proposition 4, \(\Vert w_c\Vert _{H^1}\le 2d\) for large \(c>0\). Then we have

$$\begin{aligned} \begin{aligned} \tilde{I}_\infty (w_c)&=\frac{1}{2}\int _{\mathbb {R}^3}|\nabla w_c|^2+ 2m\mu w_c^2-qm w_c^2 \phi _{w_c} dx-\frac{1}{p}\int _{\mathbb {R}^3}(w_c)_+^pdx\\&\ge \Vert w_c\Vert _{H^1}^2\Big (\min \Big \{\frac{1}{2},m\mu \Big \}-qmC_2(\Vert w_c\Vert _{H^1}^2+\Vert w_c\Vert _{H^1}^{p-2})\Big ). \end{aligned} \end{aligned}$$
(27)

Taking \(d>0\) small, we deduce that \(\tilde{I}_\infty (w_c)\ge 0\) for large c.

Step 3. \(v_c\rightarrow \tilde{V}(\cdot -z)\) in \(H^1\), where \(\tilde{V}\in \mathcal {A}\), \(z\in \mathbb {R}^3\) and \(v_c=\tilde{\eta }_cu_c\).

Let \(W_c\equiv v_c(\cdot +y_c)\). We can assume that \(W_c\rightharpoonup W\not \equiv 0\) in \(H^1\), up to a subsequence, as \(c\rightarrow \infty .\) Since \(W_c-u_c(\cdot +y_c)\rightharpoonup 0\) in \(H^1\), \(\phi _{W_c}-\phi _{u_c(\cdot +y_c)}\rightharpoonup 0\) in \(D^{1,2}\). Then for any \(\psi \in C_0^\infty (\mathbb {R}^3)\),

$$\begin{aligned} \int _{\mathbb {R}^3}(W_c\phi _{W_c}-u_c(\cdot +y_c)\phi _{u_c(\cdot +y_c)})\psi dx&= \int _{\mathbb {R}^3} (W_c-W)\big (\phi _{W_c}-\phi _{u_c(\cdot +y_c)}\big )\psi +W\big (\phi _{W_c}-\phi _{u_c(\cdot +y_c)}\big )\psi \\&\quad +(W_c -u_c(\cdot +y_c))\phi _{u_c(\cdot +y_c)} \psi dx\rightarrow 0 \end{aligned}$$

as \(c\rightarrow \infty \). From this, (17), (23) and the assumption that \(\Vert \tilde{I}^\prime _{c}(u_{c})\Vert _{H^{-1}}\rightarrow 0\) as \(c\rightarrow \infty \), we can see that W satisfies \(\tilde{I}^\prime _\infty (W)=0\). By the maximum principle, W is positive. Suppose that there exist \(R>0\) and a sequence \(\tilde{z}_c\in B(y_c,2c)\) satisfying

$$\begin{aligned} \liminf _{c\rightarrow \infty }|\tilde{z}_c-y_c|=\infty \text{ and } \liminf _{c\rightarrow \infty }\int _{B(\tilde{z}_c,R)}|v_c|^2dx>0. \end{aligned}$$

Then \(v_c(\cdot +z_c)\) converges weakly to \(\tilde{W}\) in \(H^1\), where \(I_\infty ^\prime (\tilde{W})=0\). By the same arguments in Step 1, we deduce a contradiction. By [14, Lemma 1.1], we have

$$\begin{aligned} \lim _{c\rightarrow \infty }\int _{\mathbb {R}^3}(W_c)_+^pdx=\int _{\mathbb {R}^3}W^pdx. \end{aligned}$$
(28)

We note that

$$\begin{aligned} \begin{aligned} \liminf _{c\rightarrow \infty }\Big (- \int _{\mathbb {R}^3}W_c^2 \phi _{W_c} dx\Big )&=\liminf _{c\rightarrow \infty }\int _{\mathbb {R}^3}\int _{\mathbb {R}^3}\frac{W_c^2(x)W_c^2(y)}{|x-y|}dydx\\&\ge \int _{\mathbb {R}^3}\int _{\mathbb {R}^3}\frac{W ^2(x)W ^2(y)}{|x-y|}dydx =-\int _{\mathbb {R}^3}W^2 \phi _{W} dx. \end{aligned} \end{aligned}$$
(29)

Then, by (28), (29) and Lemma 21, we have

$$\begin{aligned} \begin{aligned} \liminf _{c\rightarrow \infty }\tilde{I}_\infty (W_c)&=\liminf _{c\rightarrow \infty } \frac{1}{2}\int _{\mathbb {R}^3}|\nabla W_c|^2+ 2m\mu W_c^2-qm W_c^2 \phi _{W_c} dx-\frac{1}{p}\int _{\mathbb {R}^3}(W_c)_+^pdx\\&\ge \tilde{I}_\infty (W). \end{aligned} \end{aligned}$$
(30)

By (30), the results of Step1 and Step 2, and the assumption that \(\tilde{I}_c(u_c)\le \hat{e}_c\), we see that \(\tilde{I}_\infty (W)=E_\infty .\) By (28), (29) and (30), we have

$$\begin{aligned}&\limsup _{c\rightarrow \infty } \int _{\mathbb {R}^3}|\nabla W_c|^2+ 2m\mu W_c^2-qm W_c^2 \phi _{W_c} dx =\int _{\mathbb {R}^3}|\nabla W|^2+ 2m\mu W^2-qm W^2 \phi _{W} dx\\&\quad \le \int _{\mathbb {R}^3}|\nabla W|^2+ 2m\mu W^2+\limsup _{c\rightarrow \infty }\Big (-\int _{\mathbb {R}^3}qm W_c^2 \phi _{W_c} dx\Big ), \end{aligned}$$

which implies that \(W_c\rightarrow W\) in \(H^1\). By (27), the result of Step 1 and the fact that \(\hat{e}_c\rightarrow E_\infty \), we have for small \(d>0\),

$$\begin{aligned} \hat{e}_c\ge \tilde{I}_c(u_c)&\ge \tilde{I}_\infty (v_c)+\frac{1}{2}\min \Big \{\frac{1}{2},m\mu \Big \} \Vert w_c\Vert _{H^1}^2+o(1)\\&\ge E_\infty +\frac{1}{2}\min \Big \{\frac{1}{2},m\mu \Big \} \Vert w_c\Vert _{H^1}^2+o(1) \end{aligned}$$

as \(c\rightarrow \infty \), which implies that \(\Vert w_c\Vert _{H^1}\rightarrow 0\) as \(c\rightarrow \infty \). Thus, letting \(W=\tilde{V}(\cdot -z)\), where \(\tilde{V}\in \mathcal {A}\) and \(z\in \mathbb {R}^3\), we have

$$\begin{aligned} \Vert u_c-\tilde{V}(\cdot -y_c-z)\Vert _{H^1}\le \Vert v_c(\cdot +y_c)-\tilde{V}(\cdot -z)\Vert _{H^1}+\Vert w_c\Vert _{H^1}\rightarrow 0 \end{aligned}$$

as \(c\rightarrow \infty \). \(\square \)

Proposition 8

Let \(3<p<6\). For a fixed \(c\in (\sqrt{\frac{\mu }{m}},\infty )\), suppose that for some \(b\in \mathbb {R}\), there exists a sequence \(\{u_j\}\subset H^1\) satisfying

$$\begin{aligned}&u_j\in N_d(\mathcal {X}),\\&\quad \Vert \tilde{I}_c^\prime (u_j)\Vert _{H^{-1}}\rightarrow 0,\\&\quad \tilde{I}_c(u_j)\rightarrow b \text{ as } j\rightarrow \infty , \end{aligned}$$

where \(d>0\) is a constant. Then for small \(d>0\), b is a critical value of \(\tilde{I}_c\), and the sequence \(\{u_j(\cdot +x_j)\}_{j=1}^\infty \subset H^1\) has a strongly convergent subsequence in \(H^1\), where \(x_j\in \mathbb {R}^3\).

Proof

Since \(u_j\in N_d(\mathcal {X})\), \(\{u_j\}_{j=1}^\infty \) is bounded in \(H^1\). Then we can extract a subsequence such that \(\tilde{u}_{j_k}\equiv u_{j_k}(\cdot +x_{j_k})\) converges to \(u_0\not \equiv 0\) weakly in \(H^1\) as \(k\rightarrow \infty \), where \(x_{j_k}\in \mathbb {R}^3\). It is standard to show that \(u_0\) is a critical point of \(I_c\).

Next, we show \(\tilde{u}_{j_k}\rightarrow u_0\) in \(H^1\) as \(k\rightarrow \infty \). By Proposition 4, there exists \(R_0>0\) such that

$$\begin{aligned} \Vert \tilde{u}_{j_k}\Vert _{H^1(\mathbb {R}^3\setminus B(0,R_0))}\le 2d. \end{aligned}$$
(31)

We choose a function \(\zeta \in C^\infty (\mathbb {R}^3)\) such that

$$\begin{aligned} \zeta (x)={\left\{ \begin{array}{ll} 1 &{} \text{ for } |x|\ge 2R_0, \\ 0 &{} \text{ for } |x|\le R_0.\end{array}\right. } \end{aligned}$$

Since \(\tilde{I}_c^\prime (\tilde{u}_{j_k})(\zeta (\tilde{u}_{j_k}-u_0))-\tilde{I}_c^\prime (u_0)(\zeta (\tilde{u}_{j_k}-u_0))\rightarrow 0\) as \(k\rightarrow \infty \), we deduce that

$$\begin{aligned} \begin{aligned}&\int _{\mathbb {R}^3\setminus B(0,2R)}|\nabla (\tilde{u}_{j_k}-u_0)|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )(\tilde{u}_{j_k}-u_0)^2dx\\&\quad \le \int _{\mathbb {R}^3\setminus B(0,2R)} \Big (\frac{q}{c}\Big )^2(\tilde{u}_{j_k}- u_0) (\tilde{u}_{j_k} \Phi _{\tilde{u}_{j_k}}^2-u_0\Phi _{u_0}^2) +2q\Big (m-\frac{\mu }{c^2}\Big )(\tilde{u}_{j_k}-u_0)(\tilde{u}_{j_k} \Phi _{\tilde{u}_{j_k}}-u_0\Phi _{u_0})\\&\quad +(\tilde{u}_{j_k}-u_0)((\tilde{u}_{j_k})_+^{p-1} -(u_0)_+^{p-1} )dx+o(1) \end{aligned} \end{aligned}$$
(32)

as \(k\rightarrow \infty \). We note that, by Lemma 18,

$$\begin{aligned}&\int _{\mathbb {R}^3\setminus B(0,2R)}(v- w) (v \Phi _{v}^2-w\Phi _{w}^2)dx\nonumber \\&\quad \le \big (\Vert \Phi _v\Vert _{L^6}^2\Vert v\Vert _{L^3(\mathbb {R}^3\setminus B(0,2R))} +\Vert \Phi _w\Vert _{L^6}^2\Vert w\Vert _{L^3(\mathbb {R}^3\setminus B(0,2R))}\big )\Vert v-w\Vert _{L^3(\mathbb {R}^3\setminus B(0,2R))}\nonumber \\&\quad \le C_1\big (\Vert v\Vert _{H^1}^4\Vert v\Vert _{H^1(\mathbb {R}^3\setminus B(0,2R))}+\Vert w\Vert _{H^1}^4\Vert w\Vert _{H^1(\mathbb {R}^3\setminus B(0,2R))}\big )\Vert v-w\Vert _{H^1(\mathbb {R}^3\setminus B(0,2R))}, \nonumber \\\end{aligned}$$
(33)
$$\begin{aligned}&\int _{\mathbb {R}^3\setminus B(0,2R)}(v- w) (v \Phi _{v}-w\Phi _{w} )dx\nonumber \\&\quad \le \big (\Vert \Phi _v\Vert _{L^6} \Vert v\Vert _{L^3(\mathbb {R}^3\setminus B(0,2R))}+\Vert \Phi _w\Vert _{L^6} \Vert w\Vert _{L^3(\mathbb {R}^3\setminus B(0,2R))}\big )\Vert v-w\Vert _{L^2(\mathbb {R}^3\setminus B(0,2R))}\nonumber \\&\quad \le C_2\big (\Vert v\Vert _{H^1}^2\Vert v\Vert _{H^1(\mathbb {R}^3\setminus B(0,2R))}+\Vert w\Vert _{H^1}^2\Vert w\Vert _{H^1(\mathbb {R}^3\setminus B(0,2R))}\big )\Vert v-w\Vert _{H^1(\mathbb {R}^3\setminus B(0,2R))},\nonumber \\ \end{aligned}$$
(34)

and

$$\begin{aligned} \begin{aligned}&\int _{\mathbb {R}^3\setminus B(0,2R)}((v)_+^{p-1} -(w)_+^{p-1})(v-w)dx=(p-1)\int _{\mathbb {R}^3\setminus B(0,2R)}(tv+(1-t)w)_+^{p-2}(v-w)^2dx\\&\quad \le (p-1)\Vert tv+(1-t)w\Vert _{L^p(\mathbb {R}^3\setminus B(0,2R))}^{p-2}\Vert v-w\Vert _{L^p(\mathbb {R}^3\setminus B(0,2R))}^2\\&\quad \le C_3\big (\Vert v\Vert _{H^1(\mathbb {R}^3\setminus B(0,2R))}^{p-2}+\Vert w\Vert _{H^1(\mathbb {R}^3\setminus B(0,2R))}^{p-2}\big )\Vert v-w\Vert _{H^1(\mathbb {R}^3\setminus B(0,2R))}^2, \end{aligned} \end{aligned}$$
(35)

where \(t\in [0,1]\). Then, by (31)–(35), we see that for small \(d>0\),

$$\begin{aligned} \Vert \tilde{u}_{j_k}-u_0\Vert _{H^1(\mathbb {R}^3\setminus B(0,2R))}\rightarrow 0 \end{aligned}$$
(36)

as \(k\rightarrow \infty \). Thus, by (36) and the Rellich-Kondrachov compactness theorem, we see that \(\tilde{u}_{j_k}\rightarrow u_0\) in \(H^1\) as \(k\rightarrow \infty \). \(\square \)

Proposition 9

For \(3< p<6\), there exist \(\bar{c}_0>0\) and \(\bar{d}_0>0\) such that for \(c>\bar{c}_0\) and for \(0<d<\bar{d}_0\), \(\tilde{I}_c\) has a critical point u in \(N_d(\mathcal {X})\) with \(\tilde{I}_c(u)\le \hat{e}_c\).

Proof

Arguing indirectly, suppose \(\tilde{I}_c^\prime (u)\ne 0\) for \(u\in N_d(\mathcal {X})\) with \(\tilde{I}_c(u)\le \hat{e}_c\). By Proposition 7 and Proposition 8, we can take positive constants \(\bar{c}_0\) and \(\bar{d}_0\) such that for \(c>\bar{c}_0\) and for \(0<d<\bar{d}_0\),

$$\begin{aligned} \Vert \tilde{I}_c^\prime (u)\Vert _{H^{-1}}\ge \nu \end{aligned}$$

for \(u\in N_d(\mathcal {X})\setminus N_{d/2}(\mathcal {X})\) with \(\tilde{I}_c(u)\le \hat{e}_c\), and

$$\begin{aligned} \Vert \tilde{I}_c^\prime (u)\Vert _{H^{-1}}\ge \sigma _c \end{aligned}$$

for \(u\in N_d(\mathcal {X})\) with \(\tilde{I}_c(u)\le \hat{e}_c\), where \(\nu >0\) is a constant independent of c, and \(\sigma _c>0\) is a constant depending on c. Then, by a deformation argument using Proposition 5 and Proposition 6 (see Proposition 7 in [5] for a detailed argument), we get a contradiction. \(\square \)

4 Nonrelativistic limit of ground states for \(3< p < 6\)

In this section, we complete the proof of Theorem 1. By Proposition 3, Proposition 5 and Proposition 9, we see that for every \(3< p < 6\), there exists a ground state solutions \(u_c\) to (5) such that

$$\begin{aligned} \ \limsup _{c\rightarrow \infty }I_c(u_c) \le E_\infty . \end{aligned}$$
(37)

Proposition 10

Let \(3< p<6\) and \(u_c\) be a ground state solution of (5). Then we have

$$\begin{aligned} \sup _{c>\sqrt{\frac{ \mu }{ m}}}\Vert u_c\Vert _{H^1}\le C \text{ and } \inf _{c>\sqrt{\frac{ \mu }{ m}}}\Vert u_c\Vert _{L^p}\ge \frac{1}{C}, \end{aligned}$$

where \(C>0\) is a constant independent of c.

Proof

We note by (37) that

$$\begin{aligned} \begin{aligned} C_1&\ge \frac{5p-12}{2}I_c(u_c)-J_c(u_c)+\frac{4-p}{2}P_c(u_c)\\&=\int _{\mathbb {R}^3}(p-3)|\nabla u_c|^2+\frac{p-2}{2}\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u_c^2+\frac{p-2}{2}\Big (\frac{q}{c}\Big )^2 u_c^2\Phi _{u_c}^2dx, \end{aligned} \end{aligned}$$
(38)

where \(C_1>0\) is a constant independent of c. This implies \(\Vert u_c\Vert _{H^1}\) is bounded uniformly in \(c>\sqrt{\frac{ \mu }{ m}}\). Moreover, since \(J_c(u_c)=0\) and \( -\frac{1}{q}(c^2m-\mu )\le \Phi _{u_c}\le 0\), we have for \(c>\sqrt{\frac{ \mu }{ m}}\),

$$\begin{aligned} \begin{aligned} \int _{\mathbb {R}^3}|u_c|^p&=\int _{\mathbb {R}^3}|\nabla u_c|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u_c^2-\Big (\frac{q}{c}\Big )^2\Phi _{u_c} u_c^2\bigg ( \Phi _{u_c}+\Big (\frac{c}{q}\Big )^22q\Big (m-\frac{\mu }{c^2}\Big ) \bigg )dx\\&\ge \int _{\mathbb {R}^3}|\nabla u_c|^2+m\mu u_c^2dx+\Big (\frac{q}{c}\Big )^2|\Phi _{u_c}| u_c^2\Big ( \Phi _{u_c}+2 \frac{1}{q} (c^2m- \mu ) \Big )dx\\&\ge \int _{\mathbb {R}^3}|\nabla u_c|^2+m\mu u_c^2dx \ge {C_2}\Big (\int _{\mathbb {R}^3}|u_c|^pdx\Big )^{2/p}, \end{aligned} \end{aligned}$$
(39)

where \(C_2\) is a positive constant indendent of c. Then we have \(\int _{\mathbb {R}^3}|u_c|^pdx \ge \frac{1}{C}\), where C is a positive constant indendent of c. \(\square \)

Proposition 11

For \(3< p <6\), let \(\{u_c\}_{c>\sqrt{\frac{ \mu }{ m}}}\subset H^1\) be a ground state solution of (5). Then there exists a sequence \(\{x_c\} \in \mathbb {R}^3\) such that \(\bar{u}_c(\cdot )\equiv u_c(\cdot +x_c)\) converges to \(u_\infty \) in \(H^1(\mathbb {R}^3)\) as \(c\rightarrow \infty \), up to a subsequence, where \(u_\infty \) is a ground state solution of (10).

Proof

By Proposition 10 and [14, Lemma 1.1], we have

$$\begin{aligned} \sup _{x\in \mathbb {R}^3}\int _{B_1(x)}|u_c|^2dx=\int _{B_1(x_c)}|u_c|^2dx\ge \bar{C}>0, \end{aligned}$$

where \(\bar{C}\) is a constant indepnedent of c.

It follows from Proposition 10 that \(\{u_c\}_{c>\sqrt{\frac{ \mu }{ m}}}\) is bounded in \(H^1\) uniformly in c. Then we may assume \(\bar{u}_c\equiv u_c(\cdot +x_c)\) converges to \(u_\infty \not \equiv 0\) weakly in \(H^1\) and strongly in \(L_{loc}^q(\mathbb {R}^3)\), where \(0<q<6\). Let \(\Phi _{\bar{u}_c}\) be the solution of

$$\begin{aligned} -\Delta \Phi +\frac{q^2}{c^2}\bar{u}_c^2\Phi =-q(m-\frac{\mu }{c^2}) \bar{u}_c^2 \text{ in } \mathbb {R}^3. \end{aligned}$$

Since \(\Vert \Phi _{\bar{u}_c}\Vert _{D^{1,2}}\le C_1q(m-\frac{\mu }{c^2}) \Vert \bar{u}_c\Vert _{H^1}^2\le C_2,\) where \(C_1, C_2>0\) are constants independent of c, we may assume that

$$\begin{aligned} \Phi _{\bar{u}_c}\rightharpoonup \phi _{u_\infty } \text{ weakly } \text{ in } D^{1,2} \text{ and } \Phi _{\bar{u}_c}\rightarrow \phi _{u_\infty } \text{ in } L^q_{loc}(\mathbb {R}^3), \end{aligned}$$

as \(c\rightarrow \infty \), where \(0<q<6\) and \(\phi _{u_\infty }\) is a weak solution of \( -\Delta \phi +qmu_\infty ^2=0.\) Then it is standard to show that \(u_\infty \) is a non-trivial weak solution of (10).

Next, we claim that \(u_\infty \) is a ground state solution of (10). We note that, since \(u_\infty \) is a non-trivial weak solution of (10), we have

$$\begin{aligned} J_\infty (u_\infty )= P_\infty (u_\infty ) =0 \end{aligned}$$

and

$$\begin{aligned} \frac{5p-12}{2}I_\infty (u_\infty )-J_\infty (u_\infty )+\frac{4-p}{2}P_\infty (u_\infty )=\int _{\mathbb {R}^3}(p-3)|\nabla u_\infty |^2+ (p-2)m\mu u_\infty ^2. \end{aligned}$$
(40)

Then, by (37), (38) and (40), we have

$$\begin{aligned} \frac{5p-12}{2}E_\infty&\ge \frac{5p-12}{2}\liminf _{c\rightarrow \infty }I_c( {u}_c)\\&=\liminf _{c\rightarrow \infty }\Big (\int _{\mathbb {R}^3}(p-3)|\nabla u_c|^2+\frac{p-2}{2}\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u_c^2+\frac{p-2}{2}\Big (\frac{q}{c}\Big )^2 u_c^2\Phi _{u_c}^2dx\Big )\\&\ge \int _{\mathbb {R}^3}(p-3)|\nabla u_\infty |^2+(p-2)m\mu u_\infty ^2 =\frac{5p-12}{2} I_\infty (u_\infty ), \end{aligned}$$

which proves the claim.

Finally, to prove the strong convergence in \(H^1(\mathbb {R}^3)\), we note that, by (37), (38), (40), Proposition 10 and the fact that \(\bar{u}_c\) converges to \(u_\infty \not \equiv 0\) weakly in \(H^1\),

$$\begin{aligned} \frac{5p-12}{2}E_\infty&\ge \frac{5p-12}{2}\lim _{c\rightarrow \infty }I_c(\bar{u}_c)\\&=\lim _{c\rightarrow \infty }\int _{\mathbb {R}^3}(p-3)|\nabla \bar{u}_c|^2+\frac{p-2}{2}\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )\bar{u}_c^2+\frac{p-2}{2}\Big (\frac{q}{c}\Big )^2 \bar{u}_c^2\Phi _{\bar{u}_c}^2dx\\&=\int _{\mathbb {R}^3}(p-3)|\nabla u_\infty |^2+ (p-2)m\mu u_\infty ^2dx\\&\quad +\lim _{c\rightarrow \infty }\int _{\mathbb {R}^3}(p-3)|\nabla (\bar{u}_c-u_\infty )|^2+(p-2)m\mu (\bar{u}_c-u_\infty )^2dx\\&=\frac{5p-12}{2}E_\infty +\lim _{c\rightarrow \infty }\int _{\mathbb {R}^3}(p-3)|\nabla (\bar{u}_c-u_\infty )|^2+(p-2)m\mu (\bar{u}_c-u_\infty )^2dx. \end{aligned}$$

From this, we deduce that \( \bar{u}_c\rightarrow u_\infty \) in \(H^1\) as \(c\rightarrow \infty \), up to a subsequence. This completes the proof. \(\square \)

Proof of Theorem 1

It is sufficient to show \(H^2\) convergence of \(\bar{u}_c\) to \(u_\infty \). We may rewrite \(\bar{u}_c\) as \(u_c\). We note that, by Lemma 18 and [22, Theorem 4.1], for \(u\in H^1\),

$$\begin{aligned} \sup _{x\in \Omega } |\Phi _u(x)|\le C_1\Vert u\Vert _{H^1}^2 \ \ \text{ and } \ \, \Vert |u|^{p-2} \Vert _{L^{\frac{6}{p-2}}(\Omega )} =\Vert u\Vert _{L^{{6}}(\Omega )}^{p-2}\le C_2\Vert u\Vert _{H^1}^{p-2}, \end{aligned}$$

where \(\Omega \) is bounded domain in \(\mathbb {R}^3\), and \(C_1\) and \(C_2\) are positive constants independent of u and \(\Omega \). Then, since \(\{\Vert u_{c}\Vert _{H^1}\}_{c}\) is bounded, we see that \(\{\Vert u_{c}\Vert _{L^\infty }\}_{c}\) is bounded (see [22, Theorem 4.1]).

Since \(u_{\infty }\) and \(u_{c}\) are solutions of (10) and (5) respectively, we have

$$\begin{aligned} \begin{aligned} -\Delta (u_{c}-u_{\infty })&= -2m\mu (u_{c}-u_{\infty })+\Big (\frac{\mu }{c}\Big )^2u_{c}+\Big (\frac{q}{c}\Big )^2u_{c} \Phi _{u_{c}}^2-2q\frac{\mu }{c^2} u_{c}\Phi _{u_{c}}\\&\quad +2qm(u_{c}\Phi _{u_{c}}-u_{\infty }\phi _{u_{\infty }})+|u_{c}|^{p-2}u_{c}-|u_{\infty }|^{p-2}u_{\infty }. \end{aligned} \end{aligned}$$
(41)

We note that, by Lemma 17, Lemma 19, Lemma 21 and Proposition 11,

$$\begin{aligned} \begin{aligned}&\Vert u_{c}\Phi _{u_{c}}-u_{\infty }\phi _{u_{\infty }}\Vert _{L^2}\\&\quad =\Vert u_{c}(\Phi _{u_{c}}-\phi _{u_{c}})+(u_{c}-u_{\infty })\phi _{u_{c}}+(\phi _{u_{c}}-\phi _{u_{\infty }})u_{\infty }\Vert _{L^2}\\&\quad \le \Vert u_{c}\Vert _{L^3}\Vert \Phi _{u_{c}}-\phi _{u_{c}}\Vert _{L^6}+\Vert u_{c}-u_{\infty }\Vert _{L^3}\Vert \phi _{u_{c}}\Vert _{L^6}+\Vert \phi _{u_{c}}-\phi _{u_{\infty }}\Vert _{L^6}\Vert u_{\infty }\Vert _{L^3}\rightarrow 0 \end{aligned} \end{aligned}$$
(42)

as \(c\rightarrow \infty \), and by the fact that \(\{\Vert u_{c}\Vert _{L^\infty }\}_{c}\) is bounded,

$$\begin{aligned} \big \Vert |u_{c}|^{p-2}u_{c}-|u_{\infty }|^{p-2}u_{\infty }\big \Vert _{L^2}=(p-1)\big \Vert |u_{\infty }+t(u_{c}-u_{\infty })|^{p-2} (u_{c}- u_{\infty })\big \Vert _{L^2}\rightarrow 0 \end{aligned}$$
(43)

as \(c\rightarrow \infty \), where \(t\in [0,1]\). Thus, by (41)-(43) and the Calderón–Zygmund inequality, we have

$$\begin{aligned} \Vert u_{c}-u_{\infty }\Vert _{H^2(\mathbb {R}^3)}=\Vert -\Delta (u_{c}-u_{\infty })\Vert _{L^2}+o(1)=o(1) \end{aligned}$$

as \(c\rightarrow \infty \). \(\square \)

5 Nonrelativistic limit of two positive solutions for \(2<p< 3\)

In this section, we will construct two radially symmetric positive solutions of NMKG for \(2<p< 3\). We prove first the existence of a radially symmetric positive solution \(v_{c,q}\) of (5) satisfying

$$\begin{aligned} \lim _{c\rightarrow \infty }\Vert v_{c,q}-v_\infty \Vert _{H^1}= 0, \end{aligned}$$

where \(v_\infty \) is a global minimizer of \(I_\infty \).

We assume \(2<p< 3\) and denote

$$\begin{aligned} e_\infty \equiv \inf _{u\in H_r^1}\tilde{I}_\infty (u),\ \ \mathcal {X} _r\equiv \{u\in H_r^1\ | \ \tilde{I}_\infty (u)=e_\infty \} \end{aligned}$$

and

$$\begin{aligned} N_d(\mathcal {X}_r)\equiv \{u\in H_r^1\ | \ \inf _{v\in \mathcal {X}_r}\Vert u-v\Vert _{H^1}\le d\}, \end{aligned}$$

where \(d>0\) is a constant. We remark that, by [21, Theorem 4.3, Corollary 4.4], \(\mathcal {X}_r\) is bounded in \(H^1\), and for small \(q>0\), \(e_\infty <0\) and \(\mathcal {X}_r\ne \emptyset \). Moreover, since \(e_\infty <0\) for small \(q>0\), and for \(u\in \mathcal {X}_r\),

$$\begin{aligned} e_\infty =\tilde{I}_\infty (u)&=\frac{1}{2}\int _{\mathbb {R}^3}|\nabla u|^2+2m\mu u^2-qmu^2\phi _u dx-\frac{1}{p}\int _{\mathbb {R}^3}(u)_+^pdx\\&\ge \frac{1}{2}\int _{\mathbb {R}^3}|\nabla u|^2+2m\mu u^2 dx-\frac{C_1}{p}\Big (\int _{\mathbb {R}^3}|\nabla u| ^2+u^2dx\Big )^{p/2}, \end{aligned}$$

where \(C_1>0\) is a constant independent of \(u\in \mathcal {X}_r\), we see that there exists \(\hat{q}_0>0\) such that for \(0<q<\hat{q}_0\), \(\mathcal {X}_r\ne \emptyset \) and

$$\begin{aligned} \inf _{u\in \mathcal {X}_r}\Vert u\Vert _{H^1}>\hat{d}_0>0, \end{aligned}$$
(44)

where \(\hat{d}_0\) is a positive constant. Taking \(d\in (0,\frac{\hat{d}_0}{2}),\) we deduce that for \(0<q<\hat{q}_0\), \(0\notin N_d(\mathcal {X}_r)\). For \(d\in (0,\frac{\hat{d}_0}{2})\) and \(0<q<\hat{q}_0\), take \(V_0\in \mathcal {X}_r\) and set

$$\begin{aligned} \alpha _c=\inf _{u\in N_d(\mathcal {X}_r)}\tilde{I}_c(u) \ \text{ and } \ m_c=\tilde{I}_c(V_0). \end{aligned}$$

Clearly, we have \(m_c\ge \alpha _c\). We try to find a critical point of \(\tilde{I}_c\) in \(N_d(\mathcal {X}_r)\).

Proposition 12

For \(2<p< 3\), \(0<q<\hat{q}_0\) and \(d\in (0,\frac{\hat{d}_0}{2})\), we have

$$\begin{aligned} \liminf _{c\rightarrow \infty }\alpha _c\ge e_\infty . \end{aligned}$$

Proof

It is standard to show that there exists \(v_c\in N_d(\mathcal {X}_r)\) such that

$$\begin{aligned} \alpha _c=\tilde{I}_c(v_c), \end{aligned}$$

because \(\mathcal {X}_r\) is bounded in \(H^1\). Since \(v_c\) is bounded in \(H^1_r\) uniformly in c, we assume that \(v_c\) converges to v in \(L^s\) and weakly in \(H^1\) as \(c\rightarrow \infty \), where \(s\in (2,6)\) and \(v\in N_d(\mathcal {X}_r)\). Then, by Lemma 21, we have

$$\begin{aligned} \liminf _{c\rightarrow \infty }\alpha _c&=\liminf _{c\rightarrow \infty }\tilde{I}_c(v_c)\\&= \liminf _{c\rightarrow \infty }\Big [\frac{1}{2}\int _{\mathbb {R}^3}|\nabla v_c|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )v_c^2-q\Big (m-\frac{\mu }{c^2}\Big ) v_c^2 \Phi _{v_c} dx-\frac{1}{p}\int _{\mathbb {R}^3}(v_c)_+^pdx\Big ]\\&\ge \frac{1}{2}\int _{\mathbb {R}^3}|\nabla v|^2+2m\mu v^2-qmv^2\phi _v dx-\frac{1}{p}\int _{\mathbb {R}^3}(v)_+^pdx =\tilde{I}_\infty (v)\ge e_\infty . \end{aligned}$$

Proposition 13

For \(2<p< 3\) and \(0<q<\hat{q}_0\), we have

$$\begin{aligned} m_c\rightarrow e_\infty \end{aligned}$$

uniformly in q as \(c\rightarrow \infty \).

Proof

By Lemma 21,

$$\begin{aligned} \tilde{I}_c(V_0)&=\frac{1}{2}\int _{\mathbb {R}^3}|\nabla V_0|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )V_0^2-q\Big (m-\frac{\mu }{c^2}\Big ) V_0^2 \Phi _{V_0} dx-\frac{1}{p}\int _{\mathbb {R}^3}(V_0)_+^pdx\\&=\frac{1}{2}\int _{\mathbb {R}^3}|\nabla V_0|^2+2m\mu V_0^2-qmV_0^2\phi _{V_0} dx-\frac{1}{p}\int _{\mathbb {R}^3}(V_0)_+^pdx+o(1)\\&=\tilde{I}_\infty (V_0)+o(1)=e_\infty +o(1) \end{aligned}$$

as \(c\rightarrow \infty \). \(\square \)

Proposition 14

Let \(2<p< 3\), \(0<q<\hat{q}_0\) and \(d\in (0,\frac{\hat{d}_0}{2})\). For large \(c>0\) and for any \(d^\prime \in (0,d)\), there exists \(\nu _0\equiv \nu _0(d,d^\prime )>0\) independent of \(c>0\) such that

$$\begin{aligned} \inf \{\Vert \tilde{I}_c^\prime (u)\Vert _{H^{-1}}\ |\ \tilde{I}_c(u)\le m_c, u\in N_d(\mathcal {X}_r)\setminus N_{d^\prime }(\mathcal {X}_r)\}\ge \nu _0>0. \end{aligned}$$

Proof

Let \(\{c_i\}_{i=1}^\infty \) be such that \(\lim _{i\rightarrow \infty }c_i=\infty \). It suffices to show that if

$$\begin{aligned} u_{c_i}\in N_{d}(\mathcal {X}_r),\ \ \tilde{I}_{c_i}(u_{c_i})\le m_{c_i},\ \text{ and } \ \Vert \tilde{I}^\prime _{c_i}(u_{c_i})\Vert _{H^{-1}}\rightarrow 0 \end{aligned}$$

as \(i\rightarrow \infty \), then

$$\begin{aligned} \inf _{v\in \mathcal {X}_r}\Vert u_{c_i}-v\Vert _{H^1}\rightarrow 0 \text{ as } i\rightarrow \infty . \end{aligned}$$

For the sake of simplicity of notation, we write c for \(c_i\). Since \(\{u_c\}\subset H_r^1\) is bounded in \(H^1\), we see that \(u_c\) converges to u in \(L^s\) and weakly in \(H^1\) as \(c\rightarrow \infty \), up to a subsequence, where \(s\in (2,6)\). Then, by Lemma 21 and Proposition 13, we have

$$\begin{aligned} e_\infty&=\liminf _{c\rightarrow \infty } m_c \ge \liminf _{c\rightarrow \infty }\tilde{I}_c(u_c)\\&= \liminf _{c\rightarrow \infty }\Big [\frac{1}{2}\int _{\mathbb {R}^3}|\nabla u_c|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u_c^2-q\Big (m-\frac{\mu }{c^2}\Big ) u_c^2 \Phi _{u_c} dx-\frac{1}{p}\int _{\mathbb {R}^3}(u_c)_+^pdx\Big ]\\&\ge \frac{1}{2}\int _{\mathbb {R}^3}|\nabla u|^2+2m\mu u^2-qmu^2\phi _u dx-\frac{1}{p}\int _{\mathbb {R}^3}(u)_+^pdx =\tilde{I}_\infty (u), \end{aligned}$$

which implies that \(e_\infty =\tilde{I}_\infty (u)\).

We claim that \(u_c\rightarrow u\) in \(H^1\). Indeed, by Lemma 21 and the fact that \(\Vert \tilde{I}^\prime _c(u_c)\Vert _{H^{-1}}\rightarrow 0\) as \(c\rightarrow \infty \), we see that

$$\begin{aligned} \begin{aligned} o(1)&=\tilde{I}_c^\prime (u_c)u\\&=\int _{\mathbb {R}^3}\nabla u_c\cdot \nabla u+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u_c u-\Big (\frac{q}{c}\Big )^2 u_cu \Phi _{u_c}^2-2q\Big (m-\frac{\mu }{c^2}\Big ) u_c u\Phi _{u_c}-(u_c)_+^{p-1} udx\\&=\int _{\mathbb {R}^3} |\nabla u|^2+2m\mu u^2-2qm u^2\phi _u-(u)_+^{p} dx+o(1) \end{aligned} \end{aligned}$$
(45)

as \(c\rightarrow \infty \), and

$$\begin{aligned} \begin{aligned} o(1)&=\tilde{I}_c^\prime (u_c)u_c\\&=\int _{\mathbb {R}^3}|\nabla u_c|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u_c^2-\Big (\frac{q}{c}\Big )^2 u_c^2 \Phi _{u_c}^2-2q\Big (m-\frac{\mu }{c^2}\Big ) u_c^2\Phi _{u_c}-(u_c)_+^{p} dx\\&=\int _{\mathbb {R}^3}|\nabla u_c|^2+2m\mu u_c^2+2qmu^2\phi _u-(u)_+^pdx+o(1) \end{aligned} \end{aligned}$$
(46)

as \(c\rightarrow \infty \). Thus, by (45) and (46), we have \(u_c\rightarrow u\) in \(H^1\). \(\square \)

Proposition 15

Let \(2<p< 3\), \(0<q<\hat{q}_0\) and \(d\in (0,\frac{\hat{d}_0}{2}).\) For a fixed \(c\in (\sqrt{\frac{\mu }{m}},\infty )\), suppose that for some \(b\in \mathbb {R}\), there exists a sequence \(\{u_j\}\subset H_r^1\) satisfying

$$\begin{aligned}&u_j\in N_d(\mathcal {X}_r),\\&\quad \Vert \tilde{I}_c^\prime (u_j)\Vert _{H^{-1}}\rightarrow 0,\\&\quad \tilde{I}_c(u_j)\rightarrow b \text{ as } j\rightarrow \infty . \end{aligned}$$

Then b is a critical value of \(\tilde{I}_c\), and the sequence \(\{u_j\}_{j=1}^\infty \subset H_r^1\) has a strongly convergent subsequence in \(H^1\).

Proof

Since \(\{u_j\}\subset N_d(\mathcal {X}_r)\) is bounded in \(H^1\), we see that \(u_j\) converges to u in \(L^s\) and weakly in \(H^1\) as \(c\rightarrow \infty \), up to a subsequence, where \(s\in (2,6)\). It is standard to show that u is a critical point of \(\tilde{I}_c\).

We claim that \(u_j\rightarrow u\) in \(H^1\). Indeed, by Lemma 20 and the fact that \(\Vert \tilde{I}_c^\prime (u_j)\Vert _{H^{-1}}\rightarrow 0\) as \(j\rightarrow \infty \), we have

$$\begin{aligned} o(1)&=\tilde{I}_c^\prime (u_j)u_j\\&=\int _{\mathbb {R}^3}|\nabla u_j|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u_j^2-\Big (\frac{q}{c}\Big )^2 u_j^2 \Phi _{u_j}^2-2q\Big (m-\frac{\mu }{c^2}\Big ) u_j^2\Phi _{u_j}-|u_j|^{p} dx\\&=\int _{\mathbb {R}^3}|\nabla u_j|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u_j^2-\Big (\frac{q}{c}\Big )^2 u^2 \Phi _{u}^2-2q\Big (m-\frac{\mu }{c^2}\Big ) u^2\Phi _{u}-|u|^{p} dx+o(1) \end{aligned}$$

as \(j\rightarrow \infty \) and

$$\begin{aligned} 0=\tilde{I}_c^\prime (u)u=\int _{\mathbb {R}^3}|\nabla u|^2+\Big (2m\mu -\frac{\mu ^2}{c^2}\Big )u^2-\Big (\frac{q}{c}\Big )^2 u^2 \Phi _u^2-2q\Big (m-\frac{\mu }{c^2}\Big ) u^2\Phi _u-|u|^{p} dx. \end{aligned}$$

Thus, we deduce that \(u_j\rightarrow u\) in \(H^1\) as \(j\rightarrow \infty \). \(\square \)

Proposition 16

Let \(2<p< 3\), \(0<q<\hat{q}_0\) and \(d\in (0,\frac{\hat{d}_0}{2})\). Then there exists \(\hat{c}_0>0\) such that for \(c>\hat{c}_0\), \(\tilde{I}_c\) has a non-trivial critical point u in \(N_d(\mathcal {X}_r)\) with \(\tilde{I}_c(u)\le m_c\).

Proof

Assume that \(2<p< 3\), \(0<q<\hat{q}_0\) and \(d\in (0,\frac{\hat{d}_0}{2})\). Suppose \(\tilde{I}_c^\prime (u)\ne 0\) for \(u\in N_d(\mathcal {X}_r)\) with \(\tilde{I}_c(u)\le m_c\). By Proposition 12–15, we can take a positive constant \(\hat{c}_0\) such that for \(c>\hat{c}_0\) and for \(0<q<\hat{q}_0\),

$$\begin{aligned}&\alpha _c\ge e_\infty -\epsilon _1,\ \ |m_c-e_\infty |\le \epsilon _1, \end{aligned}$$
(47)
$$\begin{aligned}&\Vert \tilde{I}_c^\prime (u)\Vert _{H^{-1}}\ge \nu _0 \end{aligned}$$
(48)

for \(u\in N_{\frac{2}{3} d}(\mathcal {X}_r)\setminus N_{\frac{1}{3} d}(\mathcal {X}_r)\) with \(\tilde{I}_c(u)\le m_c\), and

$$\begin{aligned} \Vert \tilde{I}_c^\prime (u)\Vert _{H^{-1}}\ge \hat{\sigma }_c \end{aligned}$$
(49)

for \(u\in N_d(\mathcal {X}_r)\) with \(\tilde{I}_c(u)\le m_c\), where \(d\in (0,\frac{\hat{d}_0}{2}),\) \(\epsilon _1\in (0,\frac{d\nu _0}{6})\), and \(\hat{\sigma }_c>0\) is a constant depending on c. For \(u\in N_d(\mathcal {X}_r)\) with \(\tilde{I}_c(u)\le m_c\), we consider the following ODE:

$$\begin{aligned} {\left\{ \begin{array}{ll} &{}\frac{d\eta }{d\tau }=-\varphi _1(\tilde{I}_c(\eta ))\varphi _2(dist_{H^1}(\eta , \mathcal {X}_r ))\frac{\tilde{I}_c^\prime (\eta )}{\Vert \tilde{I}_c^\prime (\eta )\Vert _{H^{-1}}},\\ &{}\quad \eta (0,u)=u, \end{array}\right. } \end{aligned}$$

where

$$\begin{aligned} dist_{H^1}(w, \mathcal {X}_r )=\inf \{\Vert w-v\Vert _{H^1}\ |\ v\in \mathcal {X}_r\} \end{aligned}$$

for \(w\in H^1\), and \(\varphi _1, \varphi _2:\mathbb {R}\rightarrow [0,1]\) are Lipschitz continuous functions such that

$$\begin{aligned}&\varphi _1(\xi )={\left\{ \begin{array}{ll} 1 &{} \text{ if } \xi \ge e_\infty - \epsilon _1, \\ 0 &{} \text{ if } \xi \le e_\infty - 2\epsilon _1, \end{array}\right. } \ \ \ \ \ \ \varphi _2(\xi )={\left\{ \begin{array}{ll} 1 &{} \text{ if } \xi \le \frac{2}{3} d, \\ 0 &{} \text{ if } \xi \ge d. \end{array}\right. } \end{aligned}$$

Let \(T= 3\epsilon _1/\hat{\sigma }_c\) and \(V_0\in \mathcal {X}_r\). Since \(\tilde{I}_c(\eta (\tau , V_0))\ge \alpha _c\ge e_\infty -\epsilon _1\) for \(\tau \in [0,T]\), we deduce that there exists \(t_0\in [0,T]\) such that

$$\begin{aligned} dist_{H^1}(\eta (t_0,V_0))=\frac{2}{3} d. \end{aligned}$$
(50)

Indeed, if \(dist_{H^1}(\eta (\tau ,V_0))<\frac{2}{3} d\) for \(\tau \in [0,T]\), by (47) and (49),

$$\begin{aligned} \tilde{I}_c(\eta (T,V_0))&=\tilde{I}_c(V_0)+\int _0^T \frac{d}{d\tau }\tilde{I}_c(\eta (\tau , V_0))d\tau \le e_\infty +\epsilon _1- T\hat{\sigma }_c=e_\infty -2\epsilon _1, \end{aligned}$$

which is a contradiction. Assume that \(t_0\) is the first time that satisfies (50). Since \(\Vert \frac{d}{d\tau }\eta \Vert _{H^1}\le 1\), we see that \(t_0\ge \frac{2}{3} d\) and

$$\begin{aligned} \eta (\tau , V_0)\in N_{\frac{2}{3} d}(\mathcal {X}_r)\setminus N_{\frac{1}{3} d}(\mathcal {X}_r) \text{ for } \tau \in [t_0-\frac{1}{3} d, t_0]. \end{aligned}$$

Then, by (47) and (48), we have

$$\begin{aligned} \tilde{I}_c(\eta (T,V_0))&=\tilde{I}_c(V_0)+\int _0^T \frac{d}{d\tau }\tilde{I}_c(\eta (\tau , V_0))d\tau \le e_\infty +\epsilon _1+\int _{t_0-\frac{1}{3} d}^{t_0} \frac{d}{d\tau }\tilde{I}_c(\eta (\tau , V_0))d\tau \\&=e_\infty +\epsilon _1-\frac{1}{3} d \nu _0<e_\infty -\epsilon _1, \end{aligned}$$

which is a contradiction. \(\square \)

Proof of Theorem 2

Let \(2<p<3\). By Proposition 16 and the proof of Proposition 14, we prove the existence of a radially symmetric positive solution \(v_{c,q}\) of (5) satisfying

$$\begin{aligned} \limsup _{c\rightarrow \infty } \tilde{I}_c(v_{c,q})\le \inf _{u\in H^1_r}\tilde{I}_\infty (u). \end{aligned}$$

By repeating the same procedure in the proof of Proposition 14, we can prove Theorem 2 (ii).

On the other hand, it is known that the ground state solution \(w_0\) of the equation

$$\begin{aligned} -\Delta u+2m\mu -|u|^{p-2}u=0 \text{ in } \mathbb {R}^3 \end{aligned}$$
(51)

is positive, radially symmetric, up to a translation. It is also non-degenerate in the radial class, i.e., \(\text {Ker} L_0 = \{0\}\), where \(L_0:H_r^1\rightarrow H^{-1}_r\) is the linearized operator of (51) at \(w_0\), given by \(L_0(w)\equiv -\Delta w+2m\mu w-(p-1)|u_0|^{p-2}w.\)

Exploiting the non-degeneracy of \(w_0\), we see from the implicit function theorem that there exists of a family of radially symmetric solutions \(w_{\infty ,q}\) of (10) for small \(q > 0\) such that \(w_{\infty ,q}\rightarrow w_0\) as \(q\rightarrow 0\) in \(H^1\) (refer to [20, Proposition 2.1] for detail). As a consequence, one can easily see that \(w_{\infty , q}\) is also non-degenerate in the radial class for any small fixed \(q > 0\) (see [9, Proposition 3.2]). Then one can once more invoke the implicit function theorem to find a family of nontrivial radial solutions \(w_{c,q}\) of (5) for large value \(c > 0\) and small \(q > 0\), which converges in \(H^1\) to \(w_{\infty ,q}\) as \(c\rightarrow \infty \). This proves Theorem 2 (i). \(\square \)