An extended JKR model for adhesion of a rigid sphere on a supported compressible elastic thin layer

Abstract

This study aimed to develop an explicit JKR-type model for adhesive contact of a rigid sphere (of radius R) on a compressible elastic thin layer (of thickness h, Young’s modulus E, and Poisson ratio $\nu$) bonded or sliding on a rigid substrate. Based on a simple Kerr model for a compressible elastic layer, an explicit expression for strain energy of the elastic layer is derived in terms of the two JKR-type variables $\left( {\delta ,\;a} \right)$, where a is the radius of contact zone and $\delta$ is the indentation depth of the rigid sphere. Thus the equilibrium values of $\left( {\delta ,\;a} \right)$ can be determined as the stationary point of the potential energy. The explicit model is justified by detailed comparison of the predicted results (for Poisson ratio $\nu \le 0.45$) with known data reported in recent literature. For example, the validity and accuracy of the present model are demonstrated for moderately soft elastic thin layers under the condition ${2\left( {1-v^{2}} \right) WR^{2}} \big /{\left( {Eh^{3}} \right) }\ge 100$ (where W is adhesion energy per unit contact area, for instance, for typical materials with $R=500\ \upmu \mathrm{m}$ $E=100\ \mathrm{KPa}$ and $W=100\ \mathrm{mJ}/\mathrm{m}^{{2}}$, the condition requests $\;h<20\ \upmu \mathrm{m}$). As compared to existing methods that request more substantial numerical calculations, the present model achieves an explicit expression for strain energy of a compressible elastic layer and could offer a simpler analytical method for adhesion mechanics of a rigid sphere on a compressible thin elastic layer bonded or sliding on a rigid substrate.

1 Introduction

The well-known Johnson–Kendall–Roberts (JKR) model [1, 2] for adhesive contact between two elastic spheres is based on the classical Boussinesq solution of an elastic half-space, which cannot be applied to a thin elastic layer bonded or sliding on a rigid substrate [3,4,5,6,7,8] (such as a MEMS component coated by a soft thin layer [3] or atomic force microscopy (AFM) on a biological shell bonded on a petri dish [4, 6]). In such cases, the contact radius can be much larger than the thickness of the elastic thin layer and the classical JKR model [1, 2] is inapplicable.

Recently, a remarkable research effort has been made to extend the classical JKR theory [1, 2] to adhesive contact of a rigid sphere on an elastic layer bonded or sliding on a rigid substrate [9,10,11,12,13,14,15,16,17,18,19,20,21,22,23]. For example, Choi [9] studied adhesive contact of a rigid sphere on an elastic film bonded to a rigid substrate by using an elasticity solution of axisymmetric indentation with an elastic film, and concluded that the classical JKR model fails to correctly evaluate the adhesion energy between the spherical tip and the elastic film when the contact radius is larger than the thickness of elastic film. Yang [14] used a perturbation technique to analyze the axisymmetric indentation of a rigid sphere on a compressible elastic thin film bonded to a rigid substrate when the contact radius is much larger than the thickness of thin film. Sridhar et al. [11, 12] obtained empirical relations for the indentation load and contact compliance as a function of contact radius for several practical layer–substrate material systems. Chung et al. [16] studied mechanical properties of sufficiently thin polymer films supported by stiff substrates using AFM nanoindentation experiments. Zhu and Xu [18] investigated adhesive contact of a rigid sphere on an elastic film bonded or sliding on a rigid substrate using linear fracture mechanics, and formulated a semi-analytical approach to study the relation between the indentation force, penetration depth and contact radius. However, most of the mentioned works request substantial numerical calculations, and it remains an interesting problem how to achieve a simpler explicit method to analyze adhesive contact of a rigid sphere on an elastic thin layer bonded or sliding on a rigid substrate.

The present work aims to develop an explicit JKR-like model based on an expression for strain energy of an elastic layer on a rigid substrate. This paper is organized as follows. In Sect. 2, the Kerr model is used to derive an explicit expression for strain energy of a compressible elastic layer bonded or sliding on a rigid substrate, based on which the indentation depth and contact radius can be determined as the stationary point of the potential energy. The present model is validated in Sect. 3 by detailed comparison with known data available in the recent literature. Section 4 is devoted to a further study on the role of Young’s modulus and thickness of the elastic layer in adhesion mechanics. Finally, the main conclusions are summarized in Sect. 5.

2 An extended JKR model based on Kerr model for a compressible elastic layer

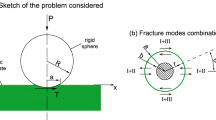
An extended JKR-type model is developed here for adhesion of a rigid sphere (of radius R) on a compressible elastic thin layer (of Young’s modulus E and Poisson ratio $\nu )$ of thickness h which is either perfectly bonded or sliding on a rigid substrate under the condition $R \gg a \gg h$ (see Fig. 1).

Fig. 1

Adhesive contact of a rigid sphere on a compressible elastic thin layer bonded or sliding on a rigid substrate

The adhesion contact is defined by two geometrical variables $(\delta a)$, where $\delta$ is the indentation depth of the rigid sphere, and a is the radius of circular contact zone. The total potential energy of the rigid sphere-elastic layer system is given by

$$\begin{aligned} U=U_{\mathrm{e}} -\pi a^{2}W-F\delta , \end{aligned}$$

(1)

where $U_{\mathrm{e}}$ is the strain energy of elastic layer, F is the external indentation force applied to the rigid sphere, and $W=\gamma _{1} +\gamma _{2} -\gamma _{12}$ is the adhesion energy (per unit area) of contact zone, and $\gamma _{1}$, $\gamma _{2}$ and $\gamma _{12}$ are the surface energy (per unit area) of the rigid sphere, the elastic layer and the interfacial energy of contact zone, respectively. The key problem is how to derive an explicit expression $U_{\mathrm{e}}(\delta , a)$ for the strain energy of a compressible elastic thin layer bonded or sliding on a rigid substrate, in terms of the two variables ($\delta , a)$.

Clearly, strain energy $U_{\mathrm{e}}$ of the elastic layer can be calculated by

$$\begin{aligned} U_{\mathrm{e}} (a,\delta )=\frac{1}{2}\int \limits _0^a {2\pi p(r)} w(r)r\mathrm{d}r, \end{aligned}$$

(2)

where w(r) is the surface deflection of the elastic layer, and p(r) is the surface pressure in the circular contact zone $(0 \le r\le a)$. In what follows, the pressure p(r) inside the contact zone will be determined by Kerr model [24] which offers a simple differential relationship between the surface deflection w(r) and the surface pressure p(r) inside the contact zone.

2.1 Kerr model for a compressible elastic thin layer on a rigid substrate

It is known that the Kerr model [24] gives a simple differential relation between the surface pressure p and the downward surface deflection w of an elastic layer (of thickness h) on a rigid substrate. For example, up to the second-order differential terms, the Kerr model in axisymmetric case gives

$$\begin{aligned} w-Ah^{2}\nabla ^{2}w=C\frac{h}{E}p-D\frac{h^{3}}{E}\nabla ^{2}p, \end{aligned}$$

(3)

where $\nabla ^{2}=\left( {\frac{\partial ^{2}}{\partial r^{2}}+\frac{1}{r}\frac{\partial }{\partial r}} \right)$, E is Young’s modulus of the elastic layer, and A, C, D are some nonnegative constants determined by the Poisson ratio $\nu$ of the elastic layer. For an elastic layer bonded on a rigid substrate [25]

$$\begin{aligned} A=\frac{1}{1-\nu },\quad C=\frac{\left( {1-2\nu } \right) \left( {1+\nu } \right) }{1-\nu },\quad D=\frac{\left( {3-4\nu } \right) \left( {1+\nu } \right) }{3\left( {1-\nu } \right) }, \end{aligned}$$

(4)

and for an elastic layer sliding on a rigid substrate [24]

$$\begin{aligned} A=\frac{1}{3},\quad C=1-\nu ^{2},\quad D=\frac{1-\nu ^{2}}{3}. \end{aligned}$$

(5)

Similar to existing related works [9, 11, 12, 14], let us focus on compressible elastic layers with $\nu <0.5$, and thus $C\ne 0$. Therefore, all results given in this paper are applied to either a bonded compressible layer or a (compressible/incompressible) sliding layer, but should not be applied to a bonded incompressible layer (for which $C=0$ in Eq. (4)).

2.2 Pressure p(r) inside contact zone

Assume that the rigid sphere of radius R penetrates into the elastic layer by depth $\delta$, with a circular contact zone of radius a. Thus, inside the contact zone ($r \le a)$ between the rigid sphere and the elastic layer, the axisymmetric surface (downward) deflection w(r) of the elastic layer is given by (see Fig. 1, with $R \gg a$)

$$\begin{aligned} w(r)=\sqrt{R^{2}-r^{2}} -R+\delta \approx \delta -\frac{r^{2}}{2R}. \end{aligned}$$

(6)

It follows from Kerr model (Eq. 3) that the surface pressure p(r) inside the contact zone is determined by the second-order differential equation

$$\begin{aligned} C\frac{h}{E}p-D\frac{h^{3}}{E}\nabla ^{2}p\approx \left( {\delta +2A\frac{h^{2}}{R}} \right) -\left( {1-4A\frac{h^{2}}{R^{2}}} \right) \frac{r^{2}}{2R}. \end{aligned}$$

(7)

An exact particular solution of the above non-homogeneous equation is of the form

$$\begin{aligned} p(r)=c+dr^{2} \end{aligned}$$

(8)

with two coefficients c and d given by

$$\begin{aligned} c= & {} \frac{E}{C}\left[ {\left( {\frac{\delta }{h}+2A\frac{h}{R}} \right) -\frac{2hD}{CR}\left( {1-4A\frac{h^{2}}{R^{2}}} \right) } \right] , \nonumber \\ d= & {} \frac{-E}{2hCR}\left( {1-4A\frac{h^{2}}{R^{2}}} \right) . \end{aligned}$$

(9)

Under the assumed conditions $R \gg a \gg h$ (i.e., $\left( {\frac{a}{R}} \right) ^{2}\ll 1$ and $\left( {\frac{h}{a}} \right) ^{2}\ll 1)$, we have

$$\begin{aligned} c\approx \frac{E}{C}\left[ {\left( {\frac{\delta }{h}} \right) +2\left( {A-\frac{D}{C}} \right) \frac{h}{R}} \right] ,\quad d\approx \frac{-E}{2hCR}. \end{aligned}$$

(10)

Since the homogeneous equation $C\frac{h}{E}p-D\frac{h^{3}}{E}\nabla ^{2}p=0$ is a modified Bessel equation of order 0 (the solution is given by $eI_{0} \left( {\sqrt{\frac{C}{D}} \frac{r}{h}} \right) )$, the general solution p(r) of Eq. (7) inside the contact zone is given by

$$\begin{aligned} p(r)=eI_{0} \left( \sqrt{\frac{C}{D}} \frac{r}{h}\right) +c+dr^{2},\quad 0\le r\le a, \end{aligned}$$

(11)

where $I_{0} \left( {\sqrt{\frac{C}{D}} \frac{r}{h}} \right)$ is the zeroth-order modified Bessel function of the first kind which becomes infinite at infinity and remains bounded at $\sqrt{\frac{C}{D}} \frac{r}{h}{=}0$, and the coefficient e is to be determined by an extra condition derived by Betti reciprocal theorem as shown below. Here, it should be noted that, unlike elastic contact of a rigid sphere on an elastic layer without adhesion (for which $p(a) = 0$ at $r = a$ inside contact zone, see [20]), adhesive contact of JKR type causes a nonzero value of p(a) at $r = a$ inside contact zone. (Actually, it is known from JKR model [1, 2] that p(r) can be tensile and even infinite as $r = a$ is approached inside contact zone.) In addition, the slope of deflection $\frac{\mathrm{d}w}{\mathrm{d}r}$ is generally discontinuous at the edge $r = a$ of contact zone. Therefore, for the present adhesive contact problem, the only continuity condition imposed at the edge $r =a$ is the continuity of the deflection w(r).

2.3 Deflection w(r) of elastic layer outside contact zone

The coefficient e in Eq. (11) will be determined in Sect. 2.4 by an application of Betti reciprocal theorem to the entire elastic layer. For this end, the deflection w(r) outside the contact zone ($r > a$) needs to be determined. Outside the contact zone ($r > a$), the deflection w(r) is determined by Kerr model (Eq. 3) with the continuity condition of w(r) on the edge $r = a$ of contact zone as follows

$$\begin{aligned} w-Ah^{2}\left( {\frac{\partial ^{2}}{\partial r^{2}}+\frac{1}{r}\frac{\partial }{\partial r}} \right) w=0;\qquad \left. w \right| _{r=\infty } =0,\quad \left. w \right| _{r=a} =w_{a} =\left( {\delta -\frac{a^{2}}{2R}} \right) . \end{aligned}$$

(12)

Equation (12) is written as the modified Bessel equation of order 0 and variable s (defined by $s=\frac{r}{h\sqrt{A} })$

$$\begin{aligned} w(s)-\left( {\frac{\mathrm{d}^{2}w}{\mathrm{d}s^{2}}+\frac{1}{s}\frac{\mathrm{d}w}{\mathrm{d}s}} \right) =0. \end{aligned}$$

(13)

Thus, the solution of Eq. (12) is given by

$$\begin{aligned} w(r)=bK_{0} \left( \frac{r}{h\sqrt{A} }\right) ,\qquad b=\frac{w_{a} }{K_{0} \left( \frac{a}{h\sqrt{A} }\right) }, \end{aligned}$$

(14)

where $K_{0} (s)$ is the zeroth-order modified Bessel function of the second kind which goes to zero at infinity and becomes infinity at $s=\textit{0}$, and b is a constant coefficient determined by the edge condition $\left. w \right| _{r=a} =w_{a}$.

2.4 Determination of the coefficient (Betti theorem)

To use Betti reciprocal theorem, let us consider the uniform deflection $w_{{0}}$ of the elastic layer under a uniform surface pressure $p_{0}$, related by the Kerr model as follows

$$\begin{aligned} w_{0} =C\frac{h}{E}p_{0}. \end{aligned}$$

(15)

Thus, since no mechanical work appears on the bottom interface between the elastic layer and the rigid substrate for a bonded layer or a sliding layer, it follows from Betti reciprocal theorem that

$$\begin{aligned} 2\pi p_{0} \int \limits _0^\infty {w(r)r\mathrm{d}r} =2\pi C\frac{h}{E}p_{0} \int \limits _0^a {p(r)r\mathrm{d}r} \end{aligned}$$

(16)

Inside the contact zone, w(r) is given by Eq. (6) and p(r) is given by Eq. (11) with the constants c and d given by Eq. (10). Outside the contact zone, $p(r) =0$ and w(r) is given by Eq. (14). Thus, substituting the expressions of w(r) (Eq. 6) and p(r) (Eq. 11) and the expressions of c and d (Eq. 10) into Eq. (16) and using the formulas

$$\begin{aligned} \frac{\mathrm{d}}{\mathrm{d}x}\left[ {xI_{1} (x)} \right] =xI_{0} (x);\quad \frac{\mathrm{d}}{\mathrm{d}x}\left[ {xK_{1} (x)} \right] =-xK_{0} (x), \end{aligned}$$

(17)

where $K_{1} (x)$ is the first-order modified Bessel function of the second kind, and $I_{1} (x)$ is the first-order modified Bessel function of the first kind, we get

$$\begin{aligned} \sqrt{A} \left( {\delta -\frac{a^{2}}{2R}} \right) \frac{K_{1} \left( \frac{a}{h\sqrt{A} }\right) }{K_{0} \left( \frac{a}{h\sqrt{A} }\right) }=\frac{Ch}{E}e\sqrt{\frac{D}{C}} I_{1} \left( {\sqrt{\frac{C}{D}} \frac{a}{h}} \right) +\left( {A-\frac{D}{C}} \right) \frac{ah}{R}. \end{aligned}$$

(18)

Finally, the coefficient e is expressed, in terms of ($\delta$, a), by

$$\begin{aligned} e(a,\delta )=\frac{\sqrt{A} \left( {\delta -\frac{a^{2}}{2R}} \right) \frac{K_{1} \left( {\frac{a}{h\sqrt{A} }} \right) }{K_{0} \left( {\frac{a}{h\sqrt{A} }} \right) }-\left( {A-\frac{D}{C}} \right) \frac{ah}{R}}{\frac{h}{E}\sqrt{CD} I_{1} \left( {\sqrt{\frac{C}{D}} \frac{a}{h}} \right) } \end{aligned}$$

(19)

2.5 Explicit expression of $U_{\mathrm{e}}$ for a compressible elastic layer on a rigid substrate

Thus, by introducing Eqs. (6) and (11) into Eq. (2), the strain energy $U_{\mathrm{e}} (\delta ,a)$ of a compressible elastic layer, as a function of ($\delta , a)$, is given by

$$\begin{aligned} U_{\mathrm{e}} (a,\delta )=\pi \left[ {e\delta ah\sqrt{\frac{D}{C}} I_{1} \left( {\sqrt{\frac{C}{D}} \frac{a}{h}} \right) -\frac{e}{2R}Y+\frac{c\delta a^{2}}{2}-\frac{ca^{4}}{8R}+\frac{\mathrm{d}\delta a^{4}}{4}-\frac{\mathrm{d}a^{6}}{12R}} \right] \end{aligned}$$

(20)

where

$$\begin{aligned} Y=\frac{Da^{2}h^{2}}{C}\left[ {\sqrt{\frac{C}{D}} \frac{a}{h}I_{1} \left( {\sqrt{\frac{C}{D}} \frac{a}{h}} \right) -2I_{2} \left( {\sqrt{\frac{C}{D}} \frac{a}{h}} \right) } \right] \end{aligned}$$

(21)

and c and d are determined by Eq. (10) and e is determined by Eq. (19). Substituting the derived expression of $U_{\mathrm{e}} (a,\delta )$ (Eq. 20) into the total potential energy U (Eq. 1) gives

$$\begin{aligned} U=\pi \left[ {e\delta ah\sqrt{\frac{D}{C}} I_{1} \left( {\sqrt{\frac{C}{D}} \frac{a}{h}} \right) -\frac{e}{2R}Y+\frac{c\delta a^{2}}{2}-\frac{ca^{4}}{8R}+\frac{\mathrm{d}\delta a^{4}}{4}-\frac{\mathrm{d}a^{6}}{12R}} \right] -\pi a^{2}W-F\delta . \end{aligned}$$

(22)

Thus, the equilibrium values of $\left( {\delta ,a} \right)$ can be determined by the two equilibrium conditions “$\partial U/\partial \delta =0$” and “$\partial U/\partial a=0$” in terms of all given parameters, with the coefficients A, C and D given by Eq. (4) for a compressible bonded layer, or with the coefficients A, C, and D given by Eq. (5) for a (compressible/incompressible) sliding layer. Compared to existing methods [11, 12, 14, 16], only for a bonded layer that request more substantial numerical calculations, the present model is characterized by an explicit expression (20) for strain energy of the compressible elastic layer and could offer a simpler analytical method for a bonded or a sliding layer on a rigid substrate.

3 Comparison with known data for a bonded compressible layer

Now let us first compare the results given by the present model with some known data available in the recent literature on adhesion of a rigid sphere on a compressible elastic layer (for Poisson ratio $\nu \le$ 0.45) bonded on a rigid substrate.

3.1 Without an external force (F =0) ($\nu \le 0.45$)

First, let us consider the case in the absence of an applied force ($F=0$). Through solving the two equilibrium equations “$\partial U/\partial \delta =0$” and “$\partial U/\partial a=0$” with $F=\textit{0}$, we calculate the normalized contact radius $a_{0} \;\left[ {4E/\left( {9\pi WR^{2}\left( {1-v^{2}} \right) } \right) } \right]$ with respect to a non-dimensional parameter $\alpha$ defined by

$$\begin{aligned} \alpha =\sqrt{\left( {{2\left( {1-v^{2}} \right) WR^{2}} \big /{\left( {Eh^{3}} \right) }} \right) } \end{aligned}$$

(23)

for Poisson ratio $\nu =0.2$ or $\nu =0.3$ or $\nu =0.45$, respectively, as shown in Fig. 2, with a comparison to Sridhar et al.’s numerical results (see Fig. 7(a) in [12]). Figure 2 shows that our results well fit Sridhar et al.’s results for the cases of a bonded compressible layer ($\nu \le 0.45$) under the condition $\alpha \ge 1$, with the relative error less than 10%. Therefore, it is concluded that the results predicted by the present model are in good agreement with known data (with relative errors less than 10%) under the condition that the bonded compressible ($\nu \le 0.45$) elastic thin layer is relatively soft so that ${2\left( {1-v^{2}} \right) WR^{2}} \big /{\left( {Eh^{3}} \right) }\ge 1$ for case $F=0$.

Fig. 2

Comparison for the normalized contact radius $a_{0} \;\left[ {4E/\left( {9\pi WR^{2}\left( {1-v^{2}} \right) } \right) } \right]$ versus the non-dimensional parameter $\alpha$ (defined by Eq. 23) with Sridhar’s model [12] (Poisson ratio $\nu =0.2$, $\nu =0.3$ and $\nu =0.45$)

3.2 With an external force $({\textit{F}} \ne \textit{0})$

Now let us make detailed comparison of the present model with available known data in the case of an applied force $F\ne$0.

3.2.1 Comparison with Sridhar et al. [12] $(\nu \le {0.45)}$:

In the case of an applied force $F\ne \textit{0}$, through solving the two equilibrium equations (“$\partial U/\partial \delta =0$” and “$\partial U/\partial a=0$”) and using the condition ${\mathrm{d}F}/{\mathrm{d}a}=0$, we plot the normalized pull-off force $F_{\mathrm{off}}/\left( {3\pi WR} \right)$ with respect to a non-dimensional parameter $\alpha$ (defined by Eq. (23)) for the cases of a compressible layer (Poisson ratio $\nu =0.2$ or $\nu =0.3$ or $\nu =0.45$), respectively, as shown in Fig. 3, in comparison with the results of Sridhar et al. (see Fig. 6(a) in [12]). Figure 3 shows that our results for the normalized pull-off force $F_{\mathrm{off}} /\left( {3\pi WR} \right)$ agree well to Sridhar et al.’s results (with the relative error less than 10%) under the condition $\alpha \ge 10$. It is concluded that the results for pull-off force predicted by the present model are in good agreement with known data (with relative errors less than 10%) under the condition that the bonded compressible ($\nu \le 0.45$) elastic thin layer is relatively soft so that ${2\left( {1-v^{2}} \right) WR^{2}}/{\left( {Eh^{3}} \right) }\ge 100$ for case $F\ne \textit{0}$.

Fig. 3

Comparison for the non-dimensional parameter $\alpha$ (defined by Eq. 23) versus the normalized pull-off force $F_{\mathrm{off}}/\left( {3\pi WR} \right)$ with Sridhar’s model [12] (Poisson ratio $\nu =0.2$, $\nu =0.3$ and $\nu =0.45$)

3.2.2 Comparison with Choi [9] ($\nu =0.3$):

In addition, the dimensionless pulling force ${-F}\big /{\left( {\mu h^{2}} \right) }$ ($\mu$ is shear modulus defined by $\mu =E \big /{\left( {2\left( {1+v} \right) } \right) })$ is calculated against a/h for two values of $R/h (R/h=10$ and $R/h=100$) with Poisson ratio $v = 0.3$. Then, using the condition ${\mathrm{d}\left( {{-F}\big /{\left( {\mu h^{2}} \right) }} \right) } \big /{\mathrm{d}\left( {a/h} \right) }=0$, the relations between a non-dimensional parameter $\alpha ^{2}/\left( {1-v} \right)$ (i.e., $WR^{2}/\left( {\mu h^{3}} \right)$ used in Choi’s results in [9]), where $\alpha$ is defined by Eq. (23), the normalized contact radius at pull-off $a_{\mathrm{off}}/h$, and the associated normalized pull-off force $-F_{\mathrm{off}}/\left( {\mu h^{2}} \right)$ are obtained and plotted in Figs. 4 and 5, respectively, with a comparison to Choi’s results (see the Figs. 6 and 8 in [9]) and the JKR model (included in Choi’s Figs. 6 and 8 in [9]). Figure 4 shows that our results for the normalized contact radius at pull-off $a_{\mathrm{off}}/h$ well fit to Choi’s results within the range of the non-dimensional parameter $\alpha ^{2}/\left( {1-v} \right) \ge 1.5$ (approximately, $\alpha ^{2}\ge 1)$ with relative errors less than 10%, consistent with our previous conclusion shown for the comparison with Sridhar’s results (see Fig. 2). On the other hand, Fig. 5 shows that our results for the associated normalized pull-off force $-F_{\mathrm{off}}/\left( {\mu h^{2}} \right)$ for both $R/h = 10$ and $R/h = 100$ fit well to Choi’s results with relative errors less than 10% when the normalized contact radius at pull-off $a_{\mathrm{off}} /h\ge 4$ (approximately, $\alpha ^{2}/\left( {1-v} \right) \ge 150$ or $\alpha ^{2}\ge 100$, as shown in Fig. 4), also consistent with our previous conclusion shown for the comparison with Sridhar’s results (see Fig. 3).

Therefore, it is concluded that the present model for adhesion of a rigid sphere on a compressible elastic layer bonded on a rigid substrate is valid with robust accuracy under the condition that the compressible thin layer (defined by $\nu \le 0.45$) is relatively soft so that ${\alpha ^{2}=2\left( {1-v^{2}} \right) WR^{2}} \big /{\left( {Eh^{3}} \right) }\ge 1$ in the absence of an external force ($F=0$) or ${\alpha ^{2}=2\left( {1-v^{2}} \right) WR^{2}} \big / {\left( {Eh^{3}} \right) }\ge 100$ for a pulling force $F< 0$. Since the contact radius a is expected to increase with the indentation force F and reaches its minimum value with the pulling-off force, this conclusion is consistent with the fact that Kerr model (Eq. 3) for a thin elastic layer, on which the present model is based, is increasingly accurate under the assumption $a \gg h$.

Fig. 4

Comparison for the non-dimensional parameter $\alpha ^{2}/\left( {1-v} \right)$ ($\alpha$ is defined by Eq. 23) versus the normalized contact radius at pull-off $a_{\mathrm{off}}/h$ with JKR model and Choi’s model [9] (Poisson ratio $\nu =0.3$)

Fig. 5

Comparison for the normalized pull-off force $-F_{\mathrm{off}}/\left( {\mu h^{2}} \right)$ versus the normalized contact radius at pull-off $a_{\mathrm{off}}/h$ with JKR model and Choi’s model [9] a $R/h = 10$; b $R/h = 100$ (Poisson ratio $\nu =0.3$)

3.2.3 Comparison with Yang [14] ($\nu =0.4):$

Next, with the values of parameters used in Yang’s work [14] ($R=50\ \upmu \mathrm{m}\ v=0.4,E=24.5\ \mathrm{Mpa},\;h=100\ \mathrm{nm}$, $W=28\ \mathrm{mJ}/\mathrm{m}^{\mathrm {2}}$, then ${2\left( {1-v^{2}} \right) WR^{2}} \big /{\left( {Eh^{3}} \right) }=4800$ and $\nu \le 0.45$, which satisfy the above condition identified for the present model) for the indentation of a PDMS (polydimethylsiloxane) thin film of thickness 100 nm by a spherical indenter of radius $50\ \upmu \mathrm{m}$, we calculate the normalized applied force $F/\left( {\pi WR} \right)$ with respect to the normalized contact radius a/h, as shown in Fig. 6, with a comparison to Yang’s results (see Fig. 2 in [14]) and the JKR model (included in Yang’s Fig. 2 in [14]). It is seen from Fig. 6 that the results predicted by the present model are in good agreement with Yang’s results. Also, as shown in Fig. 6, both our model and Yang’s model predict that, for the same contact area, higher indentation force is required for a thin layer than for an elastic half-space (predicted by the JKR theory). In addition, higher pull-off force is required to separate the indenter from a thin elastic layer than that to separate the indenter from an elastic half-space as given by the JKR theory, as shown in Fig. 6.

Fig. 6

Comparison for the normalized contact radius a/h versus normalized applied force $F/\left( {\pi WR} \right)$ with JKR model and Yang’s model [14] (Poisson ratio $\nu =0.4$)

4 Effects of Young’s modulus and thickness of the elastic layer $(\nu =0.4)$

The comparisons with known data shown in Sect. 3 indicate that the present model based on Kerr model for a thin elastic layer [Eq. (3), which is increasingly accurate under the assumption $a \gg h$] is reasonably accurate for adhesion-driven contact of a rigid sphere on a softer thin layer on a rigid substrate. In this section, the role of Young’s modulus and thickness of the elastic layer will be further studied with reference to recent results of Zhu and Xu [18] for Poisson ratio $v=0.4$ and various values of the non-dimensional parameter $\alpha ^{2}$ ($\alpha$ is defined by Eq. (23) and $\alpha ^{2}=8 \big / {\left( {9\pi h^{{*}{3}}} \right) }$, where $h^{*}$ is defined as the normalized thickness of the elastic layer $h^{*}=h\;\left[ {4E/\left( {9\pi \left( {1-v^{2}} \right) WR^{2}} \right) } \right] ^{\frac{1}{3}}$ in Zhu and Xu’s work [18]). In Fig. 7, the relation between the normalized applied force $F^{{*}}{=}F/\left( {3\pi WR} \right)$and the associated normalized contact radius $a^{*}=a\;\left[ {4E/\left( {9\pi \left( {1-v^{2}} \right) WR^{2}} \right) } \right] ^{\frac{1}{3}}$ (see our Fig. 7a), and the relation between the normalized applied force $F^{{*}}{=}F/\left( {3\pi WR} \right)$and the normalized indent depth $\delta ^{*}=\delta \;\left[ {16E^{2}/\left( {3\pi ^{2}\left( {1-v^{2}} \right) ^{2}W^{2}R} \right) } \right] ^{\frac{1}{3}}$ (see our Fig. 7b) are shown for a thin elastic layer bonded or sliding on a rigid substrate, respectively. Here, in addition to Zhu and Xu [18] who focused on the range $h^{*}>0.1$ (approximately, $\alpha ^{2}<100)$, we examine the case $\alpha ^{2}\ge 100$ with four values $\alpha ^{2}{=}100$, $\alpha ^{2}{=}2500$, $\alpha ^{2}{=}2.5\times 10^{5}$ and $\alpha ^{2}{=1.0}\times 10^{8}$ (approximately, $h^{*}{=}\;0.1$, $h^{*}{=}\;0.05$, $h^{*}{=}\;0.01$ and $h^{*}{=}\;0.001$, respectively)

Figure 7a shows that within the range of $\alpha ^{2}\ge 100$, the normalized pull-off forces $F_{\mathrm{off}}$ needed to separate the rigid sphere from the elastic layer bonded or sliding on a rigid substrate keep almost unchanged (around $F_{\mathrm{off}} {=}F_{\mathrm{off}}^{{*}} \left( {3\pi WR} \right) {=}-0.65\left( {3\pi WR} \right) \approx -6WR)$, which is consistent with Sridhar et al.’s results (see Fig. 6(a) in [12] or our Fig. 3). In other words, if the parameters satisfy the condition (${\alpha ^{2}=2\left( {1-v^{2}} \right) WR^{2}} \big /{\left( {Eh^{3}} \right) }\ge 100$ and $\nu \le$ 0.45), the pull-off force predicted by the present model is insensitive to the Young’s modulus and thickness of the elastic layer. Meanwhile, it is seen from Fig. 7a that with the same value of $\alpha ^{2}$, the contact radius at pull-off case and the contact radius with ($F=\textit{0}$) predicted for a sliding elastic layer are larger than those for a bonded elastic layer under otherwise identical conditions, in qualitative agreement with Zhu and Xu’s results (see Fig. 2 in [18]). Here, it should be noted that, similar with Zhu and Xu’s assumption [18], the sliding layer is assumed to slide but yet remain contact to the rigid substrate and thus can support a pulling force. In addition, Fig. 7b shows that in the absence of the applied force ($F=\textit{0}$), with the same value of $\alpha ^{2}$, the indentation depth predicted for a sliding layer is larger than those for a bonded layer under otherwise identical conditions, also qualitatively consistent with Zhu and Xu’s results (see Fig. 5 in [18]).

Thus, it is concluded that for adhesion-driven contact of a rigid sphere on a soft thin compressible ($\nu \le$ 0.45) layer bonded or sliding on a rigid substrate (defined by ${\alpha ^{2}=2\left( {1-v^{2}} \right) WR^{2}} \big / {\left( {Eh^{3}} \right) }\ge 100)$, the pull-off force predicted by our model keeps almost unchanged ($F_{\mathrm{off}} \approx -6WR)$, insensitive to the Young’s modulus and thickness of the elastic layer. In addition, the contact radius at pull-off case, the contact radius and the indentation depth with ($F=\textit{0}$) predicted for a sliding layer are larger than those for a bonded layer under otherwise identical conditions.

Fig. 7

a the normalized contact radius $a^{{*}}$ versus normalized applied force $F^{{*}}$, and b the normalized applied force $F^{{*}}$ versus the normalized indent depth $\delta ^{*}$, with different values of the non-dimensional parameter $\alpha ^{2}$ ($\alpha$ is defined by Eq. 23) for a bonded or a sliding layer (Poisson ratio $\nu =0.4$)

5 Conclusions

An extended JKR model is proposed in this paper to examine adhesion contact of a rigid sphere (of radius R) on a compressible elastic thin layer (of thickness h, Young’s modulus E and Poisson ratio $\nu$) bonded or sliding on a rigid substrate. The present model is based on an explicit expression for strain energy of the elastic layer derived from Kerr’s model in terms of the two JKR-type variables $(a, \delta )$, where a is the radius of contact zone and $\delta$ is the indent depth of the rigid particle. Our main results are summarized as follows

• 1. An explicit expression for strain energy of the elastic layer is derived, based on which the equilibrium values of contact radius and indentation depth ($a, \delta$) can be determined as the stationary point of the potential energy.

• 2. The results given by the present model agree well to known data with relative errors typically less than 10% for adhesion-driven contact of a rigid sphere on a soft thin compressible layer under the conditions $\nu \le 0.45$ and ${2\left( {1-v^{2}} \right) WR^{2}} \big / {\left( {Eh^{3}} \right) }\ge 100$(where W is the adhesion energy per unit contact area, for instance, for typical materials with $R=500\ \upmu \mathrm{m}$ $E=100\ \mathrm{KPa}$ and $W=100\ \mathrm{mJ/m}^{\mathrm {2}}$, the condition requests $h<20\ \upmu \mathrm{m}$). Since the Kerr model for a thin elastic layer, on which the present model is based, is increasingly accurate under the assumption $a \gg h$, this condition “${2\left( {1-v^{2}} \right) WR^{2}} \big / {\left( {Eh^{3}} \right) }\ge 100$”    actually ensures that the contact radius a is large as compared to the thickness of soft elastic thin layer

• 3. When the conditions listed in 2) are met, the present model predicts that the pull-off force needed to separate the rigid sphere from an elastic layer bonded or sliding on a rigid substrate is nearly $F_{\mathrm{off}} \approx -6WR$, in agreement with some known results, which is insensitive to the Young’s modulus and thickness of the elastic layer.

• 4. Based on the assumption that a sliding layer is sliding but yet remain contact to the rigid substrate, the present model predicts that the contact radius at pull-off case, the contact radius and the indentation depth in the absence of the applied force ($F=\textit{0}$) for a sliding layer are larger than those for a bonded layer under otherwise identical conditions, also in agreement with some known results.