Stability of semi-wavefronts for delayed reaction–diffusion equations

Abstract

This paper deals with the asymptotic behavior of solutions to the delayed monostable equation: $(*)$ $u_{t}(t,x) = u_{xx}(t,x) - u(t,x) + g(u(t-h,x)),$ $x \in \mathbb {R},\ t >0;$ here $h>0$ and the reaction term $g: \mathbb {R}_+ \rightarrow \mathbb {R}_+$ is Lipschitz continuous and has exactly two fixed points (zero and $\kappa >0$). Under certain condition on the derivative of g at $\kappa$ (without assuming classic KPP condition for g) the global stability of fast semi-wavefronts is proved. Also, when the Lipschitz constant $L_g$ is equal to $g'(0)$ the stability of all semi-wavefronts (e.g., critical, non-critical and asymptotically periodic semi-wavefronts) on each interval in the form $(-\infty , N], N\in {\mathbb {R}}$, to $(*)$ is established, which includes classic equations such as the Nicholson’s model.

1 Main results and discussion

In this work, the main object of study is the equation:

$$\begin{aligned} u_{t}(t,x)= & {} u_{xx}(t,x) - u(t,x) + g(u(t-h,x)),\quad x \in {\mathbb {R}},\ t >0, \end{aligned}$$

(1)

where $h>0$ and the nonlinear reaction term $g:{\mathbb {R}}_+\rightarrow {\mathbb {R}}_+$ is Lipschitz continuous with Lipschitz constant $L_g$ which satisfies the monostability condition $L_g\ge g'(0)>1$ and has exactly two fixed points: 0 and $\kappa >0.$ The Eq. (1) is frequently considered to model problems of population dynamics. In this case g stands for the birth rate function, h is the age when the individual reaches the sexual maturity and u(t, x) is the adult population at location x and time t. The diffusion and death rates have been normalized. In this framework is relevant the stability properties of the positive equilibrium $\kappa$ and the existence of colonization waves so-called wavefronts (see [2, 12, 21, 28, 34, 35] and references therein). Wavefronts with speed c are non-negative entire bounded solutions $u(t,x)=\psi _c(x+ct)$ such that the profile $\psi _c:{\mathbb {R}}\rightarrow {\mathbb {R}}$ satisfies $\psi _c(-\infty )=0$ and $\psi _c(+\infty )=\kappa .$ It is well known that when g is monotone and $h\ge 0$ then there exists a positive number $c_*=c_*(h)$ so-called critical speed or minimal speed such that (1) has wavefronts if and only if $c\ge c_*$ [18, 40, 43]; wavefronts with speed $c_*$ are called critical wavefronts. Moreover, these wavefronts are monotone and unique modulo translation. The main tool to obtain the stability and existence of wavefronts is to construct sub and super- solutions by using monotony arguments.

However, when g is non-monotone the associated semi-flow is non-monotone in general and wavefronts are replaced by positive bounded solutions $u(t, x)=\psi _c(x+ct)$ such that $\psi _c(-\infty )=0$ and $\liminf _{x\rightarrow +\infty }\psi _c(x)>0$ which are called semi-wavefronts. For g satisfying the subtangency condition $g(u)\le g'(0)u$, for all $u\ge 0$, has been demonstrated the existence of a minimal speed $c_*=c_*(h)$ for the existence of semi-wavefronts to (1) for all $h\ge 0$ (see [37, Theorems 4.5 and 5.4] and [13, 43]). Under the Diekmann- Kaper (D-K, for short) condition $L_g=g'(0)$ (see [11, Theorem 6.4]) Aguerrea, Gomez and Trofimchuk demonstrated the uniqueness modulo translation of all semi-wavefronts of (1). In the general case $L_g\ge g'(0)$ it is necessary to consider the following characteristic equation

$$\begin{aligned} E_c(\lambda ):=\lambda ^2-c\lambda -1+L_g\, e^{-\lambda ch}=0, \end{aligned}$$

(2)

for which it has been showed that there exits a speed ${\mathfrak {c}}_*={\mathfrak {c}}_*(L_g)$ defined as

$$\begin{aligned} {\mathfrak {c}}_{*}={\mathfrak {c}}_*(L_g):=\inf \{c>0: E_c(\lambda ) \, \hbox {has a positive root} \}, \end{aligned}$$

(3)

such that $E_c$ has exactly two positive zeros $\lambda _1(c)\le \lambda _2(c)$, also $\lambda _1(c)=\lambda _2(c)$ if and only if $c={\mathfrak {c}}_{*}$ (for a more detailed study of (2) see [16, Lemma 22]). Thus, the authors in [1, Theorem 4] showed that for $c\ge {\mathfrak {c}}_{*}$ semi-wavefronts have the following representation

$$\begin{aligned} \psi _c(z)= A_{\psi _c}(-z)^{j_c}e^{\lambda _1(c) z} + e^{(\lambda _1(c)+\epsilon )z}\, r(z), \end{aligned}$$

(4)

where $A_{\psi _c}, \epsilon \in {\mathbb {R}}_+$, $r\in L^2({\mathbb {R}})\cap C({\mathbb {R}})$ and $j_c= 0, 1$ with $j_c=1$ if and only if $L_g=g'(0)$, moreover, semi-wavefronts are unique (modulo translation) for all $c> {\mathfrak {c}}_{*}$ and $h\ge 0$ [1, Theorem 8]. We should mention that when $L_g$ in (2) is replaced by $g'(0)$ the speed ${\mathfrak {c}}_{*}$ in (3) coincides with the definition of the so-called linear speed $c_{\#}$ and

$$\begin{aligned} c_{\#}\le c_{*}\le {\mathfrak {c}}_{*}, \end{aligned}$$

(5)

(see [37, Theorem 4.5 and Theorem 5.4]), also when g is subtangential then $c_{\#}$ coincides with the critical speed $c_*$ for the existence of semi-wavefronts (see [43, Theorem 4.4]). In particular, if g satisfies the D-K condition $L_g=g'(0)$ then g is subtangential and ${\mathfrak {c}}_{*}=c_*=c_{\#}$. One of the main results of this work is show the stability of semi-wavefronts (with unbounded exponential weights) for $c\ge {\mathfrak {c}}_{*}(h)$ for all delay $h\ge 0$ (the same conditions for c and h to establish the uniqueness of semi-wavefronts in [1]).

In order to overcome the non-monotony of some reaction–diffusion equations with delay a quasi-monotonicity condition is assumed which usually requires the monotony of the delayed argument. Indeed, in a pioneering work, Schaaf [29] considered the following parabolic functional differential equation

$$\begin{aligned} u_t(t, x)=u_{xx}(t, x)+f(u(t, x), u(t-h, x)), \quad x\in {\mathbb {R}}, \, t>0. \end{aligned}$$

(6)

Schaaf proved that for a concave nonlinearity f with exactly two equilibria (0 and 1) satisfying a certain positivity condition (see [29, Section 2.1]) and

$$\begin{aligned} \partial _2f(u,v)\ge 0\quad \hbox {for all}\, u,v\in {\mathbb {R}}_+\quad \quad \hbox { (quasi-monotonicity condition)} \end{aligned}$$

(7)

wavefronts are linearly stable for small delay h [29, Theorem 4.13].

After the pioneering work by Schaaf, a series of other studies appeared where the KPP condition $|g'(u)|\le g'(0),$ for all $u\ge 0$, or the concave condition $g''(u)\le 0$, for all $u\ge 0$, was instrumental for the stability analysis. Among these studies, we would like to distinguish an important contribution [24] by Mei, Ou and Zhao where the authors proved the global stability of monotone wavefronts (critical as well as non-critical ones, see [24, Theorem 2.2]) of the following non-local equation

$$\begin{aligned} u_t(t,x)= u_{xx}(t,x)-u(t,x)+\int _{{\mathbb {R}}}K(y)g(u(t-h,x-y))dy\quad x\in {\mathbb {R}}, \ t>0,\nonumber \\ \end{aligned}$$

(8)

for monotone and concave g and K a heat kernel; here the perturbations are taken in weighted Sobolev spaces. At the same time, Lv and Wang [20] proved the global stability of non-critical wavefronts of (6) for monostable f (with exactly two equilibria: 0 and $\kappa >0$) satisfying (7) and the concavity condition: $\partial _{ij}f(u, v)\le 0$ ($\hbox {i, j}=1, 2$), for all $u, v\in [0, \kappa ]$. The authors in [20] also study (6) with non-local reaction term (which includes (8), for monotone g) and demonstrated the stability of non-critical monotone wavefronts in Sobolev spaces with exponential weights; this result can also be obtained by our approach to (1) even to non-monotone wavefronts, see Remark 17 (for the non-local equation (8) see our recent work [31]).

With respect to non-monotone wavefronts, we should mention a work of Wu et al. [41, Theorem 2.4] where the authors take some type non-monotone $g\in C^2([0, \kappa ], {\mathbb {R}})$ (‘crossing monostable’ nonlinearity) satisfying $|g'(\kappa )|< 1$ and prove the local stability of wavefronts with speed c for $c>2\sqrt{2(L_g-1)}$ and for all $h\ge 0$. Additionally, by assuming the KPP condition and $|g'(\kappa )|$ sufficiently small they prove the local stability of wavefronts with speed c for all $c> c_*$ and $h\ge 0$ [41, Theorem 2.6]; here the existence of non-monotone wavefronts can be deduced, e.g., from [36] and [15]. Our second result for non-linear stability of wavefronts generalizes these results (see Remark 10). In this regard, for unimodal g (i.e., g has exactly one critical point which is the absolute maximum point) satisfying the KPP condition and $|g'(\kappa )|<1$, Lin et al. [19] proved the local stability of non-critical wavefronts for all $h\ge 0$ (monotone or non-monotone) which includes well-known models such as Nicholson’s model (see [15, 19, 33] and references therein) described by

$$\begin{aligned} u_t(t,x)=u_{xx}(t,x)-\delta u(t,x)+\rho u(t-h,x)e^{-u(t-h,x)},\quad t>0, x\in {\mathbb {R}}, \end{aligned}$$

(9)

where $\rho ,\delta >0$, or the Mackey–Glass model [1, 3, 19, 23, 24] given by

$$\begin{aligned} u_t(t,x)=u_{xx}(t,x)-\tau \,u(t,x)+\frac{ab^n u(t-h,x)}{b^n+u^n(t-h,x)},\quad t>0, x\in {\mathbb {R}}. \end{aligned}$$

(10)

where $\tau ,a,b >0$ and $n\in {\mathbb {Z}}_+$. These stability results were stablished in weighted Sobolev spaces to initial data with a suitable convergence to $\kappa$ at $x=+\infty$.

When $\rho /\delta \in (1, e]$ in (9) wavefronts are monotone and by [24] they (critical as well as non-critical ones) are globally stable. The authors in [19] proved the local stability of (monotone and non-monotone) non-critical wavefronts to (9) when $\rho /\delta \in (e, e^2)$ for all $h\ge 0$ and for small delay h when $\rho /\delta \in (1, +\infty )$. Then, assuming $|g'(\kappa )|<1$, Chern et al. [8, Theorem 2.3] have demonstrated the local stability of critical wavefronts (monotone or non-monotone) in the same Sobolev spaces.

Next, for Lipschitz continuous function g satisfying the D-K condition $L_g= g'(0)$ and $|g'(u)|<1$ in some neighborhood of $\kappa$, Solar and Trofimchuk have established the global stability of (monotone or non-monotone) non-critical wavefronts [33, Corollary 3]. In particular, they obtained the global stability of non-critical wavefronts for (9) when $\rho /\delta \in (1,e^2)$ for all $h\ge 0$. Here initial data are not required to convergence to $\kappa$ at $x=+\infty$ as above mentioned works. Then, in a recent work, for unimodal $g\in C^2[0, +\infty )$ satisfying the KPP condition, Mei et al. [22] have generalized the results in [8, 19] for a global perturbation in the same Sobolev spaces.

On the other hand, non-subtangential models have recently attracted a lot of interest because of their connection to the so-called Allee effect in population dynamics [6, 7, 10, 26]. More precisely, if we only consider as benefit to species a greater availability of resources then the per capita growth rate g(u) / u attains its maximum at $u=0$, however if animal behavior is cooperative then individuals obtain benefits for intermediate densities $u>0$ (individual fitness) which are not generated for low densities ($u=0$), so that the per capita growth rate g(u) / u attains its maximum at some $u_0>0$. In this case model is said to have an Allee effect [9, Chapter 1] (since in our case the per capita growth birth rate g(u) / u is non-decreasing in a neighborhood of $u=0$ model is said to have a weak Allee effect). In contrast to subtangential case, for a model with Allee effect it could occur $c_*>c_{\#}$, critical wavefronts with speed $c_*>c_{\#}$ are called pushed wavefronts. In this direction, for monotone g (necessarily non-subtangential), it has been possible to establish the stability of pushed wavefronts (see [32] and [42]) as well as that of non-critical wavefronts [33, Theorem 1]. These results show that pushed wavefronts are more attractive than critical wavefronts with speed $c_*=c_\#$, for instance pushed wavefronts attract (orbitally) to the solution of (1) generated by the Heaviside step function while a critical wavefront (which is not a pushed wavefront) requires a logarithmic correction to attract this solution (see e.g. [12, 39] for $h=0$ and [4] for $h>0$). It is important to mention that the problem of the existence of semi-wavefronts for non-subtangential models is not completely solved (e.g., see [36, Corollary 4]: of course, in the available literature there are some partial results on the existence of semi-wavefronts for certain subclasses of equations, e.g., see [37, Theorem 2.4]).

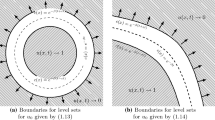
Hence, in the above mentioned works, we can find stability results for Eq. (1) only when g either is monotone or meets the sub-tangency condition. In this work we study the stability of semi-wavefronts without assuming the quasi-monotonicity nor the sub-tangency condition on g. Our approach uses ideas from [33] and a suitable Fourier analysis for partial functional differential equations. In the particular case when g is unimodal and satisfies the KPP condition, i.e. $L_g=g'(0)$ and $g\in C^1[0, +\infty )$, our estimates (in different spaces) are similar than [8, 19, 22, 24] for perturbations of wavefronts, but our Fourier analysis (for wavefronts and proper semi-wavefronts) is different in many aspects, for instance by our approach Fourier transforms are estimated by means of a Halanay inequality on Banach spaces (see Lemma 12) instead of finite-dimensional spaces while the non-critical case $c>c_*$ (for wavefronts and proper semi-wavefronts) does not require Fourier analysis as in [19] (see Corollary 20 and Remark 17 below). However, approach used in [8, 19, 22, 24] allows us to obtain stability results of wavefronts on the real line when $|g'(\kappa )|>1$ for small h whenever the initial datum $u_0(s, x)$ converges to $\kappa$ at $x=+\infty$. In this regard, we obtain a general stability result for semi-wavefronts on each semi-infinite interval $(-\infty ,N]$, $N\in {\mathbb {R}}$, without assume the restriction $|g'(\kappa )|<1$ for all $h\ge 0$ (Theorem 3) which also includes critical semi-wavefronts and asymptotically periodic semi-wavefronts (see, e.g [36, Theorem 3]). This kind of stability seems to be transversal to another models, indeed in a recent work [5] Benguria and Solar have stablished the stability of a class of non-monotone semi-wavefronts for the Hutchinson diffusive equation

$$\begin{aligned} u_t(t, x)= u_{xx}(t, x)+u(t,x)(1-u(t-h, x)), \quad \quad t>0, \, x\in {\mathbb {R}}, \end{aligned}$$

(11)

on each semi-infinite interval $(-\infty , N]$.

More precisely, by a suitable Fourier analysis we can show that for $c\ge c_{*}$, an initial perturbation

$$\begin{aligned} u_0(s, x+cs)-\psi _c(x+cs)= e^{\lambda (x+cs)}\, r(s, x),\quad \hbox {for all}\quad (s, x)\in [-h, 0]\times {\mathbb {R}}, \end{aligned}$$

with $r\in C([-h,0], L^1({\mathbb {R}}))$ and $\lambda$ satisfying $E_c(\lambda )\le 0$ [according to definition (2)], evolves as

$$\begin{aligned} u(t, x+ct)-\psi _c(x+ct)= O(t^{-1/2}e^{\gamma t}),\quad \hbox {for all}\quad t\ge -h, \end{aligned}$$

uniformly for $x+ct\in (-\infty , N]$, $N\in {\mathbb {R}}$ and some $\gamma =\gamma (\lambda )\le 0$. Moreover, $\gamma =0$ if and only if $E(\lambda )=0$, i.e. $\lambda =\lambda _1(c)$ or $\lambda =\lambda _2(c)$.

On the other hand, in our second main result we study the convergence of perturbations of wavefronts on the remaining domain $(N, +\infty )$, $N\in {\mathbb {R}}$. In this case it is necessary to assume the stability condition $|g'(\kappa )|<1$ in order to establish (without assuming monotonicity or sub-tangency condition on g) the local stability of wavefronts with $c> {\mathfrak {c}}_*$ on whole the real line $(-\infty , +\infty )$ (Corollary 20). Additionally, assuming $|g'(u)|<1$ for u in a suitable neighborhood of $\kappa$ we obtain the global stability of wavefronts with $c> {\mathfrak {c}}_*$ on whole real line $(-\infty , +\infty )$ (see Theorem 9 below).

In order to obtain these stability results we study the decay of solutions of the constant coefficient linear equation with delay,

$$\begin{aligned} u_t(t,x)=u_{xx}(t,x)+mu_x(t,x)+pu(t,x)+qu(t-h,x+d), \quad x\in {\mathbb {R}}, t>0,\nonumber \\ \end{aligned}$$

(12)

where the parameters m, p, q y d are real numbers.

For an initial datum $u_0\in C([-h,0], L^{1}({\mathbb {R}}))$, let us denote $C_{u_0}:=\sup _{s\in [-h,0]}||u_0(s,\cdot )||_{L^1}$.

Theorem 1

Suppose that $-p\ge q\ge 0$ and $m, d\in {\mathbb {R}}$. Let $\gamma \le 0$ be the only real solution of the following equation:

$$\begin{aligned} \gamma -p= qe^{-h\gamma }. \end{aligned}$$

(13)

If the initial datum $u_0$ belongs to $C ([-h,0]; L^1({\mathbb {R}}))$ then the solution u(t, x) of (12) satisfies the estimate:

$$\begin{aligned} \sup _{x\in {\mathbb {R}}}|u(t,x)| <A_0 \frac{e^{\gamma t}}{\sqrt{t}}, \quad \quad \hbox {for all} \quad t>h, \end{aligned}$$

(14)

where $A_0=C_{u_0}/2\sqrt{1+h(\gamma -p)}.$

We note that in the special case $-p = q$ (which implies $\gamma = 0$) an exponential estimate is no longer available. In some cases, it can be established that the decay is not faster than that given by (14). For instance, if $d = 0$ for the evolution equation (12), the behavior of the solutions in the $L^1({\mathbb {R}})$ phase space with an appropriate weight can be specified. In fact, we obtain the exact behavior which is embodied in Theorem below

Theorem 2

(Asymptotic behavior) Let us consider (12) with $m, p\in {\mathbb {R}}$, $q\ge 0$ and $d = 0$. Let u(t, x) be the solution generated by the initial data $u(s,\cdot )=e^{\sigma s}u_0$ where $u_0$ is such that $e^{\frac{m}{2}\cdot }\,u_0\in L^1({\mathbb {R}})$ and $\sigma$ is the only real solution of

$$\begin{aligned} qe^{-\sigma h}=\sigma +\frac{m^2}{4}-p, \end{aligned}$$

(15)

then

$$\begin{aligned} \lim _{t\rightarrow \infty }\sqrt{t}e^{-\sigma t}u(t,x+o(\sqrt{t}))=\frac{\sqrt{1+hqe^{-\sigma h}}}{2\sqrt{\pi }}e^{-\frac{m}{2}x}\int _{{\mathbb {R}}}e^{\frac{m}{2}y}u_0(y)dy, \end{aligned}$$

(16)

for all $x\in {\mathbb {R}}$.

Now, for the study of the stability of semi-wavefronts with speed c , the following equation should be considered

$$\begin{aligned} v_t(t,z)=v_{zz}(t,z)-cv_z(t,z)-v(t,z)+g(v(t-h,z-ch)), \quad t>0, z\in {\mathbb {R}}.\nonumber \\ \end{aligned}$$

(17)

For $c\ge {\mathfrak {c}}_*$ let us fix $\lambda _c\in [\lambda _1(c),\lambda _2(c)]$ and let us denote by $\xi _c(z):=e^{-\lambda _c z}$. Now, the first main result of this article can be set out.

Theorem 3

(Stability with weight) Assume that $c\ge {\mathfrak {c}}_*$. Let $v_0(s, z)$ and $\psi _0 (s, z)$ be two initial data to (17) such that $v_0,\psi _0\in C([-h,0]; L^{\infty }({\mathbb {R}})\cap C^{0,\alpha }({\mathbb {R}}))$, some $\alpha \in (0,1]$, and

$$\begin{aligned} u_0(s,z):=\xi _c (z)|v_0(s,z)-\psi _0(s,z)|\in C([-h,0], L^{1}({\mathbb {R}})), \end{aligned}$$

(18)

then there are unique solutions v(t, z) and $\psi (t,z)$ of (17) with initial data $v_0$ and $\psi _0$, respectively, and these solutions satisfies $v(\cdot +kh, \cdot ), \psi (\cdot +kh, \cdot )\in C([-h,0]; L^{\infty }({\mathbb {R}})\cap C^{0, \alpha }({\mathbb {R}}))$ for all $k\in {\mathbb {Z}}_+$. Moreover, if u(t, z) satisfies (12) with initial data $u_0(s, z)$ and with parameters $m=m(\lambda _c)=2\lambda _c -c$, $p=p(\lambda _c)=\lambda _c^{2}-c\lambda _c-1$, $q=q(\lambda _c)=L_ge^{-\lambda _c ch}$ and $d=-ch$, then

$$\begin{aligned} \xi _c(z)|v(t,z)-\psi (t,z)|\le u(t,z),\quad \hbox {for all}\quad t\ge -h, z\in {\mathbb {R}}, \end{aligned}$$

(19)

in particular

$$\begin{aligned} |v(t,z)-\psi (t,z)|\le A_0\, \xi _c(-z)\,\frac{e^{\gamma t}}{\sqrt{t}},\quad \hbox {for all}\quad t> h, z\in {\mathbb {R}}\end{aligned}$$

(20)

where $\gamma =\gamma (\lambda _c)$ is defined by (13) with $p=p(\lambda _c)$ and $q=q(\lambda _c)$.

Corollary 4

(Uniqueness) If $\psi _c(z)$ and $\phi _c(z)$ are two semi-wavefronts with speed $c\ge {\mathfrak {c}}_*$ satisfying (18) then there exists $z_*\in {\mathbb {R}}$ such that $\psi _c(z+z_*)=\phi _c(z)$ for all $z\in {\mathbb {R}}$.

Remark 5

If $h=0$ in (1) then semi-wavefronts are monotone wavefronts and by taking a wavefront $\psi (t, z)=\psi _c(z)$ in Theorem 3 we get the stability of the wavefront on the sets $(-\infty , N ], N \in {\mathbb {R}},$ which is comparable to a result obtained by Uchiyama [39, Theorem 4.1].

It has recently been showed that the estimation $u(t, x)= O(t^{-1/2})$ in (19)—(20) for critical semi-wavefronts, in the D-K case, is actually $u(t, x)= o(t^{-1/2})$ for all $h\ge 0$ (see [4, Corollary 1.2]). Also, since Theorem 3 does not assume some stability condition on $\kappa$ then semi-wavefronts could be asymptotically periodic at $+\infty$ [36, Theorem 3] and oscillations around $\kappa$ can be approximated by the solution v(t, z) on each interval in the form $(-\infty , N]$ with $N\in {\mathbb {R}}$. The Corollary 4 refers essentially to the fact that semi-wavefronts are equal (up to translation) if they have the same one-order asymptotic terms at $z=-\infty$, i.e., the condition (18).

By the change of variable $t':=\delta ^{-1} t$ and $x':= \delta ^{-1/2}x$ Eq.  (9) can be reduced to (1) with delay $h':=h\delta$ so that by Theorem 3 we obtain the stability of semi-wavefronts with speed c for the Nicholson’s model,

Corollary 6

(Nicholson Model) Let $\rho /\delta \in [1,+\infty )$ be in (9). Consider $N\in {\mathbb {R}}$ and the initial datum satisfying the conditions of Theorem 3, if $c\ge c_*$ and $\lambda _c\in [\lambda _1(c), \lambda _2(c)]$ then

$$\begin{aligned} \sup _{z\in (-\infty , N]}|v(t,z)-\psi _c(z)|=O(t^{-1/2}e^{\gamma t}), \end{aligned}$$

where $\gamma =\gamma (\lambda _c)\le 0$ is determined by (13).

It is well known that if $\rho /\delta \in (e, e^{2})$ then there are non-monotone wavefronts (see [15, Theorem 2.3]). Moreover, for some critical value $\nu _0=2.808\ldots$ and some delay $h_0$ if $\rho /\delta \in [\nu _0, +\infty )$ then each minimal wavefront has oscillations around $\kappa$ at $+\infty$ and there exist a critical value $c^*$ (a extended real number) such that each semi-wavefront with speed $c>c_*$ has non-decaying slow oscillations [36, Theorem 3].

Nevertheless, the semi-wavefronts of Theorem 3 could exhibit a type of convective instability due to the positive equilibrium (e.g., see [27]), however by controlling the size of the slope of g at the positive equilibrium, the stability of the semi-wavefront on the remaining domain $[N, +\infty )$, $N\in {\mathbb {R}}$, can be obtained. In this framework, it is necessary to assume some additional hypotheses in order to establish the existence of semi-wavefronts, such as the following condition.

(M) The function $g:{\mathbb {R}}_+\rightarrow {\mathbb {R}}_+$ is such that the equation $g(x)= x$ has exactly two solutions on $[0,+\infty )$ : 0 and $\kappa >0$. Moreover, g is $C^1$-smooth in some $\delta _0$-neighborhood of the equilibria where $g'(0)>1>g'(\kappa ).$ In addition, there are $C >0,\ \theta \in (0,1],$ such that $\left| g'(u)- g'(0)\right| +|g'(\kappa ) - g'(\kappa -u)| \le Cu^\theta$ for $u\in (0,\delta _0].$

We note that for g satisfying (M), there are real numbers $0 < \zeta _1\le \zeta _2$ such that

• (B1) $g([\zeta _1,\zeta _2])\subset [\zeta _1,\zeta _2]$ and $g([0,\zeta _1])\subset [0,\zeta _2]$;

• (B2) $\min _{\zeta \in [\zeta _1,\zeta _2]}g(\zeta )=g(\zeta _1)$;

• (B3) $g(x)>x$ for $x\in [0,\zeta _1]$ and $1<g'(0)\le g^*_+:=\sup _{s\ge 0}g(s)/s<\infty$;

• (B4) In $[0,\zeta _2]$, the equation $g(x)=x$ has exactly two solutions 0 and $\kappa$.

Thus, from [37, Theorem 4.5] we obtain the following result to the existence of semi-wavefronts.

Proposition 7

(Existence of semi-wavefronts) Let g satisfy (M). Then, for each $c > {\mathfrak {c}}_*(g^*_+)$ (according to definition (3)) Eq, (1) has semi-wavefronts with speed c. Moreover, if $\, 0 < \zeta _1\le \zeta _2$ meet (B1)–(B4) then each semi-wavefront $\psi _c$ satisfies:

$$\begin{aligned} \zeta _1\le \liminf _{z\rightarrow -\infty }\psi _c(z)\le \limsup _{z\rightarrow +\infty }\psi _c(z)\le \zeta _2,\quad \hbox {for all} \ z\in {\mathbb {R}}. \end{aligned}$$

Remark 8

(Minimal speed for semi-wavefronts) Due [16, Theorem 18], in the case that $g^*_+=g'(0)$ the number ${\mathfrak {c}}_*(g^*_+)$ is actually the minimal speed for the existence of semi-wavefronts.

Now, let us introduce some notation. If $I\subset {\mathbb {R}}_+ = Dom(g)$, let us denote by

$$\begin{aligned} L_g(I):=\sup _{x\ne y;\, x,y\in I}\frac{|g(x)-g(y)|}{|x-y|}, \end{aligned}$$

and for $b \in {\mathbb {R}}$, let us denote by $\eta _b(z)=\min \{1,e^{\lambda _c(z-b)}\}$, with $\lambda _c\in [\lambda _1(c), \lambda _2(c)]$. With these notations, the second main result of this paper can be established

Theorem 9

(Global stability) Let $c> {\mathfrak {c}}_*$ and ${\bar{g}}$ be a non decreasing function satisfying (M) with equilibrium K such that ${\bar{g}}(u)\ge g(u)$ for all $u\in {\mathbb {R}}_+$ such that $L_{{\bar{g}}}\le L_g$. We denote by $m_K=\min _{u\in [\kappa ,K]}g(u)$ and ${\mathcal {I}}_K:=[m_K,K]$ and we suppose that $L_g({\mathcal {I}}_K)<1$. If for some $q_0>0$ and $z_0\in {\mathbb {R}}$ the initial datum satisfies

$$\begin{aligned} v_0(s,z)\ge q_0\quad \hbox {for all}\quad (s,z)\in [-h,0]\times [z_0,+\infty ) \end{aligned}$$

(21)

and for some wavefront $\psi _c$, $b\in {\mathbb {R}}$ and $q>0$

$$\begin{aligned} |v_0(s,z)-\psi _c(z)|\le q\eta _{b}(z)\quad \hbox {for all}\quad (s,z)\in [-h,0]\times {\mathbb {R}}, \end{aligned}$$

(22)

then there exists $C=C({\bar{g}},m_K,b)>0$ and $\gamma _0\ge 0$ satisfying

$$\begin{aligned} -\lambda _c ^2+c\lambda _c+1\ge \gamma _0+ L_ge^{\gamma _0 h}e^{-\lambda _c ch}\ \hbox {and}\quad L_g({\mathcal {I}}_K)\le e^{-\gamma _0 h}(1-\gamma _0), \end{aligned}$$

(23)

such that

$$\begin{aligned} |v(t,z)-\psi _c(z)|\le Cqe^{-\gamma _0 t},\quad \hbox {for all}\quad (t,z)\in [-h,\infty )\times {\mathbb {R}}. \end{aligned}$$

(24)

Remark 10

(Crossing-monostable case) In [41] Wu et al. established the local stability for sufficiently fast wavefronts of the so-called crossing-monostable case. Theorem 9 generalizes those results by including global perturbations of wavefronts. More precisely, we suppose that for some positive number $K\ge \kappa$, the birth function g is such that

($C_1$):

g satisfies (M)

($C_2$):

$g(u)\le {\bar{g}}(u):=\max \{g'(0)u,K\}$ for all $u\ge 0$ and

($C_3$):

$L_g({\mathcal {I}}_K)<1,$

then the non decreasing function ${\bar{g}}(u)$ clearly satisfies $L_{{\bar{g}}}=g'(0)\le L_g$, therefore g satisfies the conditions of Theorem 9. Hence if g satisfies ($C_1$)-($C_3$) then (22) implies (24). Note that the condition $(A_3)$ in [41, Theorem 2.4] is essentially our condition ($C_3$). Moreover, by (2) and the definition of ${\mathfrak {c}}_*(h)$ we have ${\mathfrak {c}}_*(h)$ is a non-increasing function of h, therefore we have ${\mathfrak {c}}_{*}(h)\le {\mathfrak {c}}_{*}(0)=2\sqrt{L_g-1}$ for all $h\ge 0$, so that we have improved the minimal speed ${\tilde{c}}:=2\sqrt{2(L_g-1)}$ given in [41] for the local stability of wavefronts with speed $c>{\tilde{c}}.$

Now, if we take ${\bar{g}}(u)=\max _{s\in [0,u]}g(s)$ then we have that $K=M_g:=\max _{s\in [0,\kappa ]}g(s)$ and by writing $m_g=\min _{u\in [\kappa ,M_g]}g(u)$ and ${\mathcal {I}}_K=I_g:=[m_g, M_g]$ the following global stability result is obtained

Corollary 11

Let g satisfy (M) such that $L_g (I_g ) < 1$. If $\psi _c$ is a semi-wavefront with speed $c > {\mathfrak {c}}_*$, then $\psi _c$ is globally stable in the sense of Theorem 9.

Corollary 11 generalizes results for wavefronts which assume the D-K condition (see, e.g. [33]). In the Allee case with monotone g, Corollary 11 is an improvement, in terms of the globality of the disturbance, of [33, Theorem 2] for wavefronts with a speed greater than ${\mathfrak {c}}_*$ and it also gives us an exponential convergence rate for these waves. In this regard, exponential (in the time) stability as in (24) for pushed wavefronts was not studied in [32] but a recent work [42] by Wu, Niu and Hsu, has given a positive answer to this problem.

This paper is organized as follows. The linear theorems (Theorems 1 and 2) are proven in Sect. 2. Finally, results on the stability of semi-wavefronts are proven in Sect. 3.

2 Proof of Linear Theorems

In order to demonstrate both Theorems 1 and 2, the following two lemmas will be needed. The first one is an abstract version of the Halanay type inequalities [17]

Lemma 12

(Halanay Type Inequality) Let X be a complex Banach space. Suppose that $\sigma ,k\in {\mathbb {C}}$ and $h>0$. If $r\in C([-h,\infty ),X)$ is a function satisfying:

$$\begin{aligned} r_t(t)= \sigma r(t)+kr(t-h), \quad \hbox {a.e.,} \end{aligned}$$

then

$$\begin{aligned} |r(t)|_{X}\,\le \sup _{s\in [-h,0]} |r(s)|_X \ e^{\max \{0,\lambda \}h }\, e^{\lambda t},\quad \hbox {for all}\quad t>-h, \end{aligned}$$

(25)

where $\lambda$ is the only real root of the equation :

$$\begin{aligned} \lambda =Re(\sigma )+|k|e^{-\lambda h}. \end{aligned}$$

(26)

Moreover

• (i) $\lambda \le 0 \iff -Re(\sigma )\ge |k|.$

• (ii) $\lambda = 0 \iff -Re(\sigma )= |k|.$

Proof

It is clear that:

$$\begin{aligned} \frac{d}{dt}(r(t)e^{-\sigma t})=ke^{-\sigma t}r(t-h)\quad a.e. \end{aligned}$$

and from here, it is obtained that $|r(t)|_X$ meets the following inequality:

$$\begin{aligned} x(t)\le |k|\int _{0}^{t} e^{Re(\sigma )(t-s)}x(s-h)ds+x(0)e^{Re(\sigma )t}\quad \hbox {for all}\quad t>0 \end{aligned}$$

(27)

We note that for $A\in {\mathbb {R}}$ the function $e_A (t)=Ae^{\lambda t}$ meets (27) with equality. Now, for $A:= \sup _{s\in [-h,0]}|r(s)|_X\,e^{\max \{0,\lambda \} h}$ the function $\delta (t)=|r(t)|_X-e_{A}$ satisfies (27) for $t\in [0,h]$ and therefore $\delta (t)\le 0$ for all $t\in [0,h]$. Similarly, it is concluded that $\delta (t)\le 0$ for the intervals $[h,2h],[2h,3h]\ldots$ This proves (25).

Let us prove (i). If $-Re(\sigma )\ge |k|$ then: $\lambda \le |k|(e^{-h\lambda }-1)$ which necessarily implies that $\lambda \le 0$. Otherwise, if $\lambda \le 0$ let us suppose that $-Re(\sigma )<b$, then $\lambda >|k|(e^{-h\lambda }-1)$ which is a contradiction.

In order to prove (ii) let us note that since the derivative of $f(\lambda ):=\lambda -Re(\sigma )-|k|e^{-h\lambda }$ is always positive then $f(\lambda )$ has at most one zero. So, if $Re(\sigma )=|k|$ then $\lambda =0$ is the only solution of (26), this proves (ii). $\square$

Now, let us consider the function $\lambda : {\mathbb {R}}\rightarrow {\mathbb {R}}$ defined by

$$\begin{aligned} \lambda (\zeta )=-\zeta ^2 +p+qe^{-h\lambda (\zeta )}, \end{aligned}$$

(28)

where $q\ge 0$. Next, we proceed to estimate the even function $\lambda (\zeta )$.

For $\epsilon _h=\frac{1}{1+h(\gamma -p)}$ we define the function

$$\begin{aligned} \alpha _{h}(\zeta ):=-\frac{1}{h}\log (1+h\epsilon _h \zeta ^2). \end{aligned}$$

Here $\gamma \in {\mathbb {R}}$ is defined by (13) for any $p\in {\mathbb {R}}$ and $q\ge 0$.

Lemma 13

If $\lambda$ is defined by (28) then

$$\begin{aligned} -\epsilon _h\zeta ^2+\gamma \le \lambda (\zeta )\le \alpha _{h}(\zeta )+\gamma \quad \hbox {for all}\ \zeta \in {\mathbb {R}}. \end{aligned}$$

(29)

Moreover, if $q>0$ then

$$\begin{aligned} \lim _{|\zeta |\rightarrow \infty }q^{-1}\zeta ^2\,e^{h\lambda (\zeta )}=1. \end{aligned}$$

(30)

Remark 14

The function $\alpha _{h}$ is a generalization of the function $\alpha _0 (\zeta ):=-\zeta ^2=\lim _{h\rightarrow 0}\alpha _h(\zeta )$ for each $\zeta \in {\mathbb {R}}$. Also, when $h=0$ then $\gamma =p+q$ [according to definition (13)] therefore $\lambda (\zeta )=-\zeta ^2+\gamma$ in (28). Thus, by passing the limit $h\rightarrow 0$ in (29) we have the equality $-\zeta ^2=\lambda (\zeta )-\gamma =\alpha _0(\zeta ).$ In this regard, the estimates in (29) are sharp.

Proof

Let us denote $\beta (\zeta )=\lambda (\zeta )-\alpha (\zeta )-\gamma$. Then $\beta (\zeta )$ satisfies the following equation

$$\begin{aligned} \beta (\zeta )=-\zeta ^{2}+\frac{1}{h}\log (1+h\epsilon _h\zeta ^2)-\gamma +p+qe^{-h\gamma }(1+h\epsilon _h\zeta ^2)e^{-h\beta (\zeta )}. \end{aligned}$$

From Lemma 12 we have that $\beta (\zeta )\le 0$ if and only if:

$$\begin{aligned} \zeta ^{2}-\frac{1}{h}\log (1+h\epsilon _h\zeta ^2)+\gamma -p\ge qe^{-h\gamma }(1+h\epsilon _h\zeta ^2). \end{aligned}$$

(31)

Now, using $\log (1+x)\le x$, fo rall $x\ge 0$, in order to obtain (31) it is enough to have

$$\begin{aligned}&\zeta ^2-\epsilon _{h}\zeta ^2+\gamma -p\ge qe^{-h\gamma }(1+h\epsilon _h\zeta ^2)\quad \hbox {for all}\quad \zeta \in {\mathbb {R}}\\&\quad \iff (1-\epsilon _{h}-qh\epsilon _h e^{-h\gamma })\zeta ^2+\gamma -p- qe^{-h\gamma }\ge 0\quad \hbox {for all}\quad \zeta \in {\mathbb {R}},\\ \end{aligned}$$

which is a consequence of definition of $\gamma$ and $\epsilon _h$. So that, this proves the upper estimate in (29)

To complete left hand side of (29) we note that due to (28), (13) and upper estimate in (29)

$$\begin{aligned} \lambda (\zeta )\ge & {} -\zeta ^2+\gamma -q^{-\gamma h}+qe^{-h\gamma }[1+h\epsilon _h\zeta ^2]\\= & {} -\epsilon _h\zeta ^2+\gamma . \end{aligned}$$

Next, by multiplying (28) by $e^{h\lambda (\zeta )}$ and by using that $\lambda (\zeta )\rightarrow -\infty$ as $|\zeta |\rightarrow +\infty$ [which is obtained from upper estimation in (29)] we conclude

$$\begin{aligned} \lim _{\zeta \rightarrow \pm \infty }e^{h\lambda (\zeta )}\zeta ^2=p \end{aligned}$$

which implies (30). $\square$

Consider the following equation

$$\begin{aligned} u_t(t,z)=u_{zz}(t,z)+d_1u_z(t,z)+d_2u(t,z)+e^{-\lambda z}g(e^{\lambda (z-ch)}u(t-h,z-ch))\nonumber \\ \end{aligned}$$

(32)

where $d_1,d_2,\lambda \in {\mathbb {R}}$

Proposition 15

If $u_0\in C([-h,0]; L^{\infty }({\mathbb {R}})\cap C^{0,\alpha }({\mathbb {R}}))$, some $\alpha \in (0,1]$, then there is a unique solution u(t, z) of (32) with initial data $u_0$ and this solution satisfies $u(\cdot +kh, \cdot )\in C([-h,0]; L^{\infty }({\mathbb {R}})\cap C^{0, \alpha }({\mathbb {R}}))$ for all $k\in {\mathbb {Z}}_+$. Moreover, if $u_0\in C([-h,0]; L^1({\mathbb {R}}))$ and $\lambda =0$ in (32) then $u(t,\cdot ), u_z(t, \cdot )\in L^{1}({\mathbb {R}})$ for all $t\ge 0$ and $u_{zz}(t, \cdot )\in L^1({\mathbb {R}})$ for all $t>h$.

Proof

By defining

$$\begin{aligned} d_3(t,z):= e^{-\lambda ch}g(e^{\lambda (z-ch)}u(t-h,z-ch))/e^{\lambda (z-ch)}u(t-h, z-ch) \end{aligned}$$

we have $d_3\in L^{\infty }({\mathbb {R}}_+\times {\mathbb {R}})$ and the function u satisfies

$$\begin{aligned} u_t(t,z)=u_{zz}(t,z)+d_1u_z(t,z)+d_2u(t,z)+d_3(t,z)u(t-h,z-ch) \end{aligned}$$

(33)

By making the change of variables ${\bar{u}}(t,z):=u(t,z-d_1t)e^{-d_2 t}$ the equation (33) is reduced to an inhomogeneous heat equation

$$\begin{aligned} {\bar{u}}_t(t,z)={\bar{u}}_{zz}(t,z)+f(t,z), \end{aligned}$$

(34)

where

$$\begin{aligned} f(t,z)=e^{-d_2h}d_3(t,z-d_1t){\bar{u}}(t-h,z-h(c+d_1)), \end{aligned}$$

(35)

Now, note that for $1\le p\le \infty$

$$\begin{aligned} |f(t,\cdot )|_{L^{p}({\mathbb {R}})}\le e^{-d_2h}|d_3|_{L^{\infty }}\max _{s\in [-h,0]}|{\bar{u}}_0(s, \cdot )|_{L^{p}({\mathbb {R}})}\quad \hbox {for all}\ t\in [0,h]. \end{aligned}$$

(36)

Similarly, by using the definition of $d_3$, we get

$$\begin{aligned} |f(t,\cdot )|_{C^{0,\alpha }({\mathbb {R}})}\le L_g\,e^{-d_2h} \max _{s\in [-h,0]}|{\bar{u}}_0(s, \cdot )|_{C^{0,\alpha }({\mathbb {R}})} \quad \hbox {for all} \ t\in [0,h]. \end{aligned}$$

(37)

So that, by [14, Chapter 1, Theorems 12 and 16] there exist a unique solution to (32) and this solution satisfies

$$\begin{aligned} {\bar{u}}(t):= \Gamma _t*{\bar{u}}(0)+\int _0^t\Gamma _{t-s}*f(s)ds, \end{aligned}$$

(38)

where $\Gamma _t$ is the one-dimensional heat kernel.

Now, we take $1\le p\le \infty$. Then, for $t\in [0,h]$ and $t_n\rightarrow t$ we have

$$\begin{aligned} \nonumber |{\bar{u}}(t)-{\bar{u}}(t_n)|_{L^p}\le & {} |\Gamma _t-\Gamma _{t_n}|_{L^1}\,|{\bar{u}}(0)|_{L^p}+\int _{0}^t|\Gamma _{t-s}-\Gamma _{t_n-s}|_{L^1}|f(s)|_{L^p}ds\\&+ \int _{t}^{t_n}|\Gamma _{t_n-s}|_{L^1}|f(s)|_{L^p}ds, \end{aligned}$$

(39)

and by using (36),

$$\begin{aligned}&|{\bar{u}}(t)-{\bar{u}}(t_n)|_{L^p}\le (|\Gamma _t-\Gamma _{t_n}|_{L^1}\nonumber \\&\quad +\int _{0}^t|\Gamma _{t-s}-\Gamma _{t_n-s}|_{L^1}ds+| t-t_n|)R \max _{s\in [-h,0]}|{\bar{u}}_0(s,\cdot )|_{L^p({\mathbb {R}})}, \end{aligned}$$

(40)

where $R=\max \{1, e^{-d_2h}|d_3|_{L^{\infty }}\}$. Since $|\Gamma _{t_n}|_{L^1}=|\Gamma _t |_{L^1}=1$ the last inequality implies $|{\bar{u}}(t)-{\bar{u}}(t_n)|\rightarrow 0$ as $t_n\rightarrow t$, therefore if $u_0(\cdot , \cdot )\in C([-h,0]; L^p({\mathbb {R}}))$ then $u(\cdot +h, \cdot )\in C([-h,0]; L^p({\mathbb {R}}))$. Similarly, we get $u(\cdot +h, \cdot )\in C([-h,0]; C^{0, \alpha }({\mathbb {R}}))$ whenever $u_0(\cdot , \cdot )\in C([-h,0]; C^{0,\alpha }({\mathbb {R}}))$

Analogously, by using the initial data $u(t+h,\cdot ), u(t+2h,\cdot )\ldots$ we obtain $u(\cdot +kh, \cdot )\in C([-h,0]; L^p({\mathbb {R}})\cap C^{0,\alpha }({\mathbb {R}}))$ for $k=2,3\ldots$ Therefore, with $p=\infty$ we obtain the first assertion of the Proposition 15.

Otherwise, if $u_0\in C([-h,0], L^1({\mathbb {R}}))$ then with $p=1$ we get $u(\cdot + kh, \cdot )\in C([-h,0]; L^1({\mathbb {R}}))$ for all $k\in {\mathbb {Z}}_+$. Then, note that by (38) for $t>0$ we get

$$\begin{aligned} {\bar{u}}_z(t,z)= & {} \int _{{\mathbb {R}}}\frac{(y-z)e^{-(z-y)^2/4t}}{4t^{3/2}\sqrt{\pi }}u_0(0, y)dy\nonumber \\&+ \int _{0}^t\int _{{\mathbb {R}}}\frac{(y-z)e^{-(z-y)^2/4(t-s)}}{4(t-s)^{3/2}\sqrt{\pi }}f(s,y)dyds, \end{aligned}$$

(41)

and using (36) with $p=1$ for $t\in (0,h]$ we obtain

$$\begin{aligned} |{\bar{u}}_z(t,\cdot )|_{L^1({\mathbb {R}})}\le & {} \frac{|u_0(0,\cdot )|_{L^1({\mathbb {R}})}}{\sqrt{\pi t}}\int _{{\mathbb {R}}}|y|e^{-y^2}dy\nonumber \\&+2\sqrt{\frac{t}{\pi }}\int _{{\mathbb {R}}}|y|e^{-y^2}dy \max _{s\in [-h,0]}|f(s,\cdot )|_{L^1({\mathbb {R}})}\nonumber \\\le & {} (\frac{1}{\sqrt{t}}+2\sqrt{t}e^{-d_2h}|d_3|_{L^{\infty }({\mathbb {R}}_+\times {\mathbb {R}})})\, \frac{1}{\sqrt{\pi }}\max _{s\in [-h,0]}|{\bar{u}}_0(s, \cdot )|_{L^1({\mathbb {R}})}\nonumber \\ \end{aligned}$$

(42)

and by using the initial data ${\bar{u}}(t+h,\cdot ), {\bar{u}}(t+2h,\cdot )\ldots$, with $t\in (0,h]$, we obtain ${\bar{u}}_z(t+kh, \cdot )\in L^1({\mathbb {R}})$ for $k\in {\mathbb {Z}}_+$ and $t\in (0,h]$. Moreover, if we differentiate in (32) and proceed as in (39) and (40) then we have $|{\bar{u}}_z(t, \cdot )|_{L^1({\mathbb {R}})}$ continuously depends on $t\in {\mathbb {R}}_+$.

Finally, if $T>h$ then ${\bar{u}}(T+\cdot , \cdot )\in C([-h,0]; L^1({\mathbb {R}}))$, by taking $\lambda =0$, we obtain ${\bar{u}}_z(t,z)$ satisfies (33) with $d_3(t,z)= g'({\bar{u}}(t-h, z-ch))$ and taking as initial datum the function ${\bar{u}}(T+s, z)$ and using (42) (replacing ${\bar{u}}_z$ by ${\bar{u}}_{zz}$) we obtain ${\bar{u}}_{zz}(T+t, \cdot )\in L^{1}({\mathbb {R}})$ for all $t\in (0,h]$. Similarly, by using the initial data ${\bar{u}}_z(t+h,\cdot ), {\bar{u}}_z(t+2h,\cdot )\ldots$, with $t\in (0,h]$, we obtain ${\bar{u}}_{zz}(t+T+kh, \cdot )\in L^1({\mathbb {R}})$ for $k\in {\mathbb {Z}}_+$ and $t\in (0,h]$, which completes the proof. $\square$

Remark 16

Since by Proposition 15 $u(\cdot +hk, \cdot )\in C([-h, 0], L^{\infty }({\mathbb {R}}))$ for all $k\in {\mathbb {Z}}$ then for each $t>0$ we have $f(\cdot , \cdot )\in C([0, t], L^{\infty }({\mathbb {R}}))$, therefore

$$\begin{aligned} \left| \int _{{\mathbb {R}}}\frac{(y-z)e^{-(z-y)^2/4(t-s)}}{4(t-s)^{3/2}\sqrt{\pi }}f(s,y)dy\right| \le \frac{C}{\sqrt{t-s}}\quad \hbox {for all}\quad t>s, \end{aligned}$$

for some constant $C>0$ (which does not depend on (z, t, s)) so that from (41) we conclude $u(t, \cdot )\in C^1({\mathbb {R}})$ for all $t>0$.

Proof of Theorem 1

By using Proposition 15 with $d_1=m$, $d_2=p$, $\lambda =0$ and $g(u)=qu$ we get $u(t,\cdot ), u_z(t, \cdot ), u_{zz}(t, \cdot )\in L^1({\mathbb {R}})$ for all $t>h$. Next, by applying the Fourier transform, here

$$\begin{aligned} {\hat{u}}(z):= \frac{1}{2\pi }\int _{{\mathbb {R}}} e^{-izy}u(y)dy, \end{aligned}$$

to Eq. (12) we have

$$\begin{aligned} {\hat{u}}_t(t,\zeta )=\sigma (\zeta ) {\hat{u}}(t,\zeta )+k(\zeta ){\hat{u}}(t-h,\zeta )\quad \hbox {for all}\quad t>h, \end{aligned}$$

where $\sigma (\zeta )=-\zeta ^2+im\zeta +p$ and $k(\zeta )=qe^{-id\zeta }.$

Since $-Re(\sigma (\zeta ))\ge |k(\zeta )|$, by Lemma 12 we obtain $\lambda (\zeta )\le 0$ for all $\zeta \in {\mathbb {R}}$ and :

$$\begin{aligned} |{\hat{u}}(t,\zeta )|\le C_{u_0} e^{\lambda (\zeta )t}\quad \hbox {for all} \ \zeta \in {\mathbb {R}}. \end{aligned}$$

If $t>h$ then by the Fourier’s inversion formula (since by Remark 16 $u(t,\cdot )\in C^1({\mathbb {R}})$ for $t>0$) and Lemma 13, we have

$$\begin{aligned} |u(t,x)|\le \frac{1}{2\pi }\int _{{\mathbb {R}}} |{\hat{u}}(t,\zeta )|d\zeta \le \frac{C_{u_0}}{2\pi }\int _{{\mathbb {R}}} e^{\lambda (\zeta )t}d\zeta \le \frac{C_{u_0}}{2\pi }e^{\gamma t}\int _{{\mathbb {R}}} \frac{d\zeta }{(1+\epsilon \zeta ^2)^{\frac{t}{h}}}. \end{aligned}$$

Moreover, by Bernoulli’s inequality, we conclude that

$$\begin{aligned} \int _{{\mathbb {R}}} \frac{d\zeta }{(1+\epsilon \zeta ^2)^{\frac{t}{h}}}\le \int _{{\mathbb {R}}}\frac{d\zeta }{1+\frac{t\epsilon }{h}\zeta ^2}=\frac{1}{\sqrt{t}}\left[ \sqrt{\frac{h}{\epsilon }}\int _{{\mathbb {R}}}\frac{d\zeta }{1+\zeta ^2}\right] =\frac{1}{\sqrt{t}}\sqrt{\frac{h}{\epsilon }}\pi . \end{aligned}$$

$\square$

Proof of Theorem 2

If we make the change of variable $v(t,x)=e^{\frac{m}{2}x}u(t,x)$, then v(t, x) solves

$$\begin{aligned} v_t(t,x)=v_{xx}(t,x)+\left( p-\frac{m^2}{4}\right) v(t,x) +qv(t-h,x). \end{aligned}$$

(43)

By applying the Fourier transform to (43) we get

$$\begin{aligned} {\hat{v}}_t(t,z)=\left( -z^2+p-\frac{m^2}{4}\right) {\hat{v}}(t,z)+q{\hat{v}}(t-h,z)\quad \hbox {for all}\quad t>0 \end{aligned}$$

(44)

Let us note that due to $q \ge 0$, we have that (44) satisfies the Comparison Principle; that is, if for each $z\in {\mathbb {R}}$ we consider two solutions v(s) and w(s) of (44) defined on $[-h, +\infty )$ then, by denoting $\mathfrak {R}({\hat{u}}(t))=u_1(t), \mathfrak {I}({\hat{u}}(t))=u_2(t)$, the inequality

$$\begin{aligned} v_i(s)\le w_i(s)\quad \hbox {for all} \ s\in [-h,0] \ \hbox {and}\quad i=1,2. \end{aligned}$$

implies

$$\begin{aligned} v_i(s)\le w_i(s)\quad \hbox {for all} \ s\in [-h,+\infty ) \ \hbox {and}\quad i=1,2 \end{aligned}$$

Let us denote by $e_{A}(t,z)=Ae^{\lambda (z)t}$, where $\lambda (z)$ satisfies

$$\begin{aligned} \lambda (z)=-z^2+p-\frac{m^2}{4}+qe^{-\lambda (z)h}. \end{aligned}$$

(45)

Let us note that $e_A(t,z)$ satisfies (44) for all $A\in {\mathbb {C}}$. Also, let us denote that

$$\begin{aligned} m_i(z)= & {} \min _{s\in [-h,0]}(v_i(s,z) e^{-\lambda (z)s})\quad \hbox {and}\quad \\ M_i(z)= & {} \max _{s\in [-h,0]}(v_i(s,z) e^{-\lambda (z)s})\quad i=1, 2 \end{aligned}$$

then we have that

$$\begin{aligned} e_{m_i}(s,z)\le v_i(s,z)\le e_{M_i}(s,z)\quad \hbox {for all} \ (s,z)\in [-h,0]\times {\mathbb {R}}; i=1,2. \end{aligned}$$

By the comparison principle applied to real and imaginary part in (44), we have that

$$\begin{aligned} e_{m_i}(t,z)\le v_i(t,z)\le e_{M_i}(t,z)\quad \hbox {for all}\quad (t,z)\in [-h,\infty )\times {\mathbb {R}}; i=1,2\nonumber \\ \end{aligned}$$

(46)

or

$$\begin{aligned} m_i(z)e^{\lambda (z)t}\le v_i(t,z)\le M_i(z)e^{\lambda (z)t}\quad \hbox {for all}\quad (t,z)\in [-h,\infty )\times {\mathbb {R}}; i=1,2\nonumber \\ \end{aligned}$$

(47)

Now, by the Fourier inversion formula, we have that

$$\begin{aligned} v(t,x)=\frac{1}{\sqrt{t}}\int _{{\mathbb {R}}}e^{\frac{xy}{\sqrt{t}}}{\hat{v}}(t,y/\sqrt{t})dy. \end{aligned}$$

(48)

However, if we apply Lemma 13 to (45) with $\gamma =\sigma$ we have

$$\begin{aligned} \lim _{t\rightarrow \infty }t[\lambda (y/\sqrt{t})-\sigma ]=-\frac{y^2}{1+hqe^{-\sigma h}}. \end{aligned}$$

(49)

and due to $v(s, \cdot ) \in L^1 ({\mathbb {R}})$ by the Lebesgue’s dominated convergence theorem

$$\begin{aligned} \lim _{t\rightarrow \infty }M_1(y/\sqrt{t})=\lim _{t\rightarrow \infty }m_1(y/\sqrt{t})=\int _{{\mathbb {R}}}e^{\frac{m}{2}x}v(s,x)dx \end{aligned}$$

and

$$\begin{aligned} \lim _{t\rightarrow \infty }M_2(y/\sqrt{t})=\lim _{t\rightarrow \infty }m_2(y/\sqrt{t})=0. \end{aligned}$$

Therefore by (47)

$$\begin{aligned} \lim _{t\rightarrow \infty }{\hat{v}}(t, y/\sqrt{t})=e^{-\frac{y^2}{1+hqe^{-\sigma h}}}\int _{{\mathbb {R}}}e^{\frac{m}{2}x}v(s,x)dx \end{aligned}$$

(50)

However, by (25) there exists $C (p, q, m) > 0$ such that

$$\begin{aligned} |{\hat{v}}(t,y/\sqrt{t})|\le C\sup _{s\in [-h,0]}|{\hat{v}}(s,y/\sqrt{t})| e^{\lambda (y/\sqrt{t})t} \end{aligned}$$

but by (29) and Bernoulli’s Inequality

$$\begin{aligned} |{\hat{v}}(t,y/\sqrt{t})|\le \frac{Ce^{|\sigma |h}||e^{\frac{m}{2}\cdot }u_0(\cdot )||_{L^1({\mathbb {R}})}}{1+\epsilon _h y^2}\quad \hbox {for all}\quad t>0. \end{aligned}$$

(51)

Finally, by (48), (51), Lebesgue’s dominated convergence theorem and (50), the result obtained. $\square$

3 Proof of results of stability of semi-wavefronts

Proof of Theorem 3

The first assertion follows from Proposition 15 with $\lambda =0$. Next, for a solution w(t, z) of (17), let us denote the function ${\tilde{w}}(t,z)=\xi _c(z)w(t,z)$ which satisfies

$$\begin{aligned} {\tilde{w}}_t(t,z)= & {} {\tilde{w}}_{zz}(t,z)+m{\tilde{w}}_z(t,z)+p{\tilde{w}}(t,z)\\&+\xi _c(z)g(\xi _c(-z+ch){\tilde{w}}(t-h,z-ch)). \end{aligned}$$

We consider the linear operator

$$\begin{aligned} {\mathcal {L}}\delta (t,z):=\delta _{zz}(t,z)+m\delta _z(t,z)+p\delta (t,z)-\delta _t(t,z). \end{aligned}$$

If $\delta _{\pm }(t,z):=\pm [{\tilde{v}}(t,z)-{\tilde{\psi }}(t,z)]-u(t,z)$, then by (18): $\delta _{\pm }(s,z)\le 0$ for $(s,z)\in [-h,0]\times {\mathbb {R}}$. For $(t,z)\in [0,h]\times {\mathbb {R}}$ by (17) and (18) we have

$$\begin{aligned} {\mathcal {L}}\delta _{\pm }(t,z)= & {} \mp \xi (z)[g(\xi (-z+ch){\tilde{\psi }}(t-h,z-ch))\\&-g(\xi (-z+ch){\tilde{v}}(t-h,z-ch))]-{\mathcal {L}}u(t,z)\\\ge & {} -L_ge^{-\lambda ch}|{\tilde{v}}(t-h,z-ch)-{\tilde{\psi }}(t-h,z-ch)|-{\mathcal {L}}u(t,z)\\\ge & {} - L_ge^{-\lambda ch}u(t-h,z-ch)-{\mathcal {L}}u(t,z)= 0. \end{aligned}$$

Now, by Proposition 15, ${\tilde{w}}(\cdot +kh,\cdot )\in C([-h,0]; L^{\infty }({\mathbb {R}}))$ for all $k\in {\mathbb {Z}}_+$ therefore by using the Phragmèn–Lindelöf principle from [25, Chapter 3, Theorem 1], we have $\delta _{\pm }(t,z)\le 0$ for $(t,z)\in [0,h]\times {\mathbb {R}}$. The argument is repeated for intervals $[h, 2h], [2h, 3h]\ldots$ to conclude (19). Finally, the estimate in (20) is obtained using Theorem 1. $\square$

Remark 17

Note that in Proof of Theorem 3 it was only necessary to have an initial datum $u_0$ exponentially bounded to apply the Phragmèn–Lindelöf principle in order to obtain estimate (20). So, we could use the elementary exponential solutions of (12) of the form $u(t, z)=Be^{\gamma t+r\,z}$, with r and $\gamma$ satisfying

$$\begin{aligned} q(\lambda _c)e^{-rch }\,e^{-\gamma h}=-r^2-(2 \lambda _c-c)r-p(\lambda _c)+\gamma . \end{aligned}$$

(52)

Here, $\gamma \le 0$ if and only if

$$\begin{aligned} -r^2-(2\lambda _c-c)r-p(\lambda _c)\ge q(\lambda _c)\,e^{-r ch }, \end{aligned}$$

(53)

with $\gamma =0$ if and only if (53) holds with equality. Thus, for $c>{\mathfrak {c}}_*$ and $\lambda _c\in (\lambda _1(c), \lambda _2(c))$ we have $-p(\lambda _c)>q(\lambda _c)$ and therefore by taking $r=0$ in (53) we obtain $\gamma <0$ in (52) and therefore the asymptotic stability of non-critical is obtained. However, when $c={\mathfrak {c}}_*$ we have $\lambda _{{\mathfrak {c}}_*}=\lambda _1({\mathfrak {c}}_*)=\lambda _2({\mathfrak {c}}_*)$ and $-p(\lambda _{{\mathfrak {c}}_*})=q(\lambda _{{\mathfrak {c}}_*})$ in (53), also due to the curves $-\lambda ^2+c\lambda +1$ and $L_g\,e^{-\lambda ch}$ in (2) are tangent at $\lambda =\lambda _{{\mathfrak {c}}_*}$ the function $\Theta (r):=q(\lambda _{{\mathfrak {c}}_*})\,e^{-r {\mathfrak {c}}_* h }+r^2+(2\lambda _{{\mathfrak {c}}_*}-{\mathfrak {c}}_*)r+p(\lambda _{{\mathfrak {c}}_*})$ holds $\Theta \, '(0)=0$. Consequently, since $\Theta$ is strictly convex and $\Theta (0)=0$ we conclude $r=0$ is the only solution in (53) and therefore $-p(\lambda _{{\mathfrak {c}}_*})=q(\lambda _{{\mathfrak {c}}_*})$ implies $\gamma =0$ in (52). Thus, this approach does not allow us to obtain the asymptotic stability of critical semi-wavefronts.

Theorem 18

Let v(t, z) and $\psi (t,z)$ be solutions of equation (17) for $c\ge {\mathfrak {c}}_*$. Assume that for some compact interval $I \subset {\mathbb {R}}$, such that $L_g (I ) < 1,$ and $b\in {\mathbb {R}}$ we have

$$\begin{aligned} \psi (t,z),v(t,z)\in I \quad \hbox {for all} \ (t,z)\in [-h,\infty )\times [b-ch,\infty ), \end{aligned}$$

(54)

and for some $q > 0$ and $\lambda _c\in [\lambda _1(c), \lambda _2(c)]$

$$\begin{aligned} |v_0(s,z)-\psi _0(s,z)|\le q\eta _b(z)\quad \hbox {for all}\ (s,z)\in [-h,0]\times {\mathbb {R}}. \end{aligned}$$

(55)

If $\gamma _0 \ge 0$ satisfies

$$\begin{aligned} -\lambda _c ^2+c\lambda _c+1\ge \gamma _0+ L_ge^{\gamma _0 h}e^{-\lambda _c ch}\ \hbox {and}\quad L_g(I)\le e^{-\gamma _0 h}(1-\gamma _0), \end{aligned}$$

(56)

then

$$\begin{aligned} |v(t,z)-\psi (t,z)|\le qe^{-\gamma _0 t}\eta _b(z)\quad \hbox {for all}\ (t,z)\in [-h,\infty )\times {\mathbb {R}}. \end{aligned}$$

(57)

Proof

We define $\eta (t,z)=qe^{-\gamma _0 t}\eta _b(z)$ and write the operator

$$\begin{aligned} {\mathcal {L}}_0\delta (t,z):=\delta _{zz}(t,z)-c\delta _z(t,z)-\delta (t,z)-\delta _t(t,z). \end{aligned}$$

Note that by (55) if $\delta _{\pm }(t,z):=\pm [v(t,z)-\psi (t,z)]-\eta (t,z)$ then $\delta _{\pm }(s,z)\le 0$ for $(s,z)\in [-h,0]\times {\mathbb {R}}$. Now, for $(t,z)\in [0,h]\times (-\infty ,b]$ due to (17), (22) and (56) we have that

$$\begin{aligned} {\mathcal {L}}_0\delta _{\pm }(t,z)= & {} \pm [-g(v(t-h,z-ch))+g(\psi (t-h,z-ch))]-{\mathcal {L}}_0\eta (t,z)\\\ge & {} qe^{-\gamma _0 t+\lambda _c (z-b)}[-L_ge^{\gamma _0 h}e^{-\lambda ch}-(\lambda _c^{2}-c\lambda _c-1+\gamma _0)]\ge 0. \end{aligned}$$

Similarly, if $(t,z)\in [0,h]\times [b,\infty )$ we obtain:

$$\begin{aligned} {\mathcal {L}}_0\delta _{\pm }(t,z)= & {} \pm [-g(v(t-h,z-ch))+g(\psi (t-h,z-ch))]-{\mathcal {L}}_0\eta (t,z)\\\ge & {} qe^{-\gamma _0 t}[-L_g(I)e^{\gamma _0 h}\eta (z-ch)-(-1+\gamma _0)]\\\ge & {} qe^{-\gamma _0 t}[-L_g(I)e^{\gamma _0 h}+1-\gamma _0]\ge 0. \end{aligned}$$

Now, as in the proof of the [33, Lemma 1], due to

$$\begin{aligned} \frac{\partial \delta _{\pm }(t,b+)}{\partial z}- \frac{\partial \delta _{\pm }(t, b-)}{\partial z} > 0, \end{aligned}$$

(58)

we have that $\delta _{\pm }(t,z) \le 0$ for all $t \in [0,h], \ z \in {\mathbb {R}}$. Indeed, otherwise there exists $r_0> 0$ such that $\delta (t,z)$ restricted to any rectangle $\Pi _r= [-r,r]\times [0,h]$ with $r>r_0$, reaches its maximum positive value $M_r >0$ at some point $(t',z') \in \Pi _r$.

We claim that $(t',z')$ belongs to the parabolic boundary $\partial \Pi _r$ of $\Pi _r$. Indeed, suppose on the contrary, that $\delta (t,z)$ reaches its maximum positive value at some point $(t',z')$ of $\Pi _r\setminus \partial \Pi _r$. Then clearly $z' \not =z_*$ because of (58). Suppose, for instance that $z' > z_*$. Then $\delta (t,z)$ considered on the subrectangle $\Pi = [z_*,r]\times [0,h]$ reaches its maximum positive value $M_r$ at the point $(t',z') \in \Pi \setminus \partial \Pi$. Then the classical results [25, Chapter 3, Theorems 5,7] show that $\delta (t,z) \equiv M_r >0$ in $\Pi$, a contradiction.

Hence, the usual maximum principle holds for each $\Pi _r, \ r \ge r_0,$ so that we can appeal to the proof of the Phragmèn–Lindelöf principle from [25] (see Theorem 10 in Chapter 3 of this book), in order to conclude that $\delta (t,z) \le 0$ for all $t \in [0,h], \ z \in {\mathbb {R}}$.

We can again repeat the above argument on the intervals [h, 2h],  $[2h, 3h], \dots$ establishing that the inequality $w_-(t,z) \le w(t,z)\le w_+(t,z),$ $z\in {\mathbb {R}},$ holds for all $t \ge -h$. $\square$

Remark 19

We can generalize the function $\eta _b (z)$ for $b = +\infty$ and, thus, have $\eta _{\infty }(z)=\xi _c(-z)$. In this proof, it was not necessary to use the condition (54) for $z \le b$ so by replacing $\xi _c(-z)$ by $\eta _b(z)$ it can be concluded that (55) implies (57).

Corollary 20

(Local stability) Suppose that there exist $M, b \in {\mathbb {R}}$ and $l_0 > 0$, such that:

$$\begin{aligned} \psi (t,z)\in [M-l_0,M+l_0]\quad \hbox {for all}\quad (t,z)\in [-h,\infty )\times [b-ch,\infty ), \end{aligned}$$

(59)

and that for some $l_1 > l_0$ the initial data satisfy

$$\begin{aligned} |v_0(s,z)-\psi _0(s,z)|<(l_1-l_0)e^{-\gamma _0 s}\eta _b(z)\quad \hbox {for all} \quad (s,z)\in [-h,0]\times {\mathbb {R}}.\nonumber \\ \end{aligned}$$

(60)

where $\gamma _0\ge 0$ is defined by (56). If $L_g({\mathcal {I}}_1) < 1$, where ${\mathcal {I}}_1 := [M - l_1, M + l_1]$, then

$$\begin{aligned} |v(t,z)-\psi (t,z)|\le (l_1-l_0)e^{-\gamma _0 t}\eta _b(z)\quad \hbox {for all} \ (t,z)\in [-h,\infty )\times {\mathbb {R}}. \end{aligned}$$

(61)

Proof

Clearly, $\psi (t,z)\in {\mathcal {I}}_1$ for all $(t,z)\in [-h,\infty )\times [b-ch,\infty ).$ Now if we suppose that the inequality in (60) is satisfied for $v_0(s, z)= v(hk+s, z)$ and $\psi _0(s, z)=\psi (hk+s, z)$, with $k\in {\mathbb {Z}}_+$, then $v(hk+t-h, z)\in {\mathcal {I}}_1$ for all $(t, z)\in [0, h]\times {\mathbb {R}}$ and, arguing as in the proof of Theorem 18, we get

$$\begin{aligned} {\mathcal {L}}_0\,\delta _{\pm }^k(t,z)\le 0\quad \hbox {for all}\quad (t, z)\in [0, h]\times {\mathbb {R}}, \end{aligned}$$

where $\delta _{\pm }^k(t, z)=\pm [v(hk+t,z)-\psi (hk+t,z)]- (l_1-l_0)e^{-\gamma _0 (hk+t)}\eta _b(z)$ and from [33, Lemma 1] we conclude

$$\begin{aligned} \delta _{\pm }^k(t,z)\le 0\quad \hbox {for all}\ (t, z)\in [0, h]\times {\mathbb {R}}. \end{aligned}$$

(62)

But (62) implies $v((k+1)h+t-h, z)\in {\mathcal {I}}_1\, \hbox {for all} \ (t, z)\in [0, h]\times {\mathbb {R}}$ and, arguing as above, by using (62) we obtain $\delta _{\pm }^{k+1}(t,z)\le 0\, \hbox {for all}\ (t, z)\in [0, h]\times {\mathbb {R}}.$ Therefore, it is sufficient to suppose (60) in order to conclude (61) for $(t,z)\in [0,h]\times {\mathbb {R}}$ and then we procede inductively to obtain (61) for all $(t,z)\in [-h, \infty )\times {\mathbb {R}}$. $\square$

To prove Theorem 9, we will use the following lemma

Lemma 21

Suppose that functions $g_1,g_2: D\subset {\mathbb {R}}_+\rightarrow {\mathbb {R}}_+$ satisfy: $g_1(u)\le g_2(u)$ for all $u\in D$. Let $v_1(t, z), v_2(t, z) : [-h, \infty )\times {\mathbb {R}}\rightarrow D$ be solutions to (17), with $g = g_1$ and $g = g_2$, respectively, such that: $v_1(s, z) \le v_2 (s, z)$ for $(s, z) \in [h, 0] \times {\mathbb {R}}$. If $g_1$ or $g_2$ is a non decreasing function, then we have : $v_1(t, z) \le v_2(t, z)$ for all $(t, z) \in {\mathbb {R}}_+ \times {\mathbb {R}}.$

Proof

We take $\delta (t,z)=v_1(t,z)-v_2(t,z)$. Let us note that if $(t,z)\in [0,h]\times {\mathbb {R}}$ then

$$\begin{aligned} {\mathcal {L}}_0\delta (t,z)=g_2(v_2(t-h,z-ch))-g_1(v_1(t-h,z-ch))\ge 0, \end{aligned}$$

because if $g_2$ is a non decreasing function we have that

$$\begin{aligned}&g_2(v_2(t-h,z-ch))-g_1(v_1(t-h,z-ch))\\&\quad \ge g_2(v_1(t-h,z-ch))-g_1(v_1(t-h,z-ch))\ge 0, \end{aligned}$$

or if $g_1$ is a non decreasing function, we have

$$\begin{aligned}&g_2(v_2(t-h,z-ch))-g_1(v_1(t-h,z-ch))\\&\quad \ge g_2(v_2(t-h,z-ch))-g_1(v_2(t-h,z-ch))\ge 0 \end{aligned}$$

Now, as $\delta (t,z)\le 0$ for all $(t,z)\in [-h,0]\times {\mathbb {R}}$ the Phragmèn–Lindelöf principle from [25][Chapter 3, Theorem 10] implies that $\delta (t,z)\le 0$ for $(t,z)\in [0,h]\times {\mathbb {R}}$. The argument is repeated for intervals $[h,2h],[2h,3h]\ldots$ $\square$

Proof Theorem 9

Let us take $\epsilon >0$ such that $L_g({\mathcal {I}}_{\epsilon })<1$, where ${\mathcal {I}}_{\epsilon }:=[m_K-\epsilon ,K+\epsilon ]\subset {\mathbb {R}}_+.$Then, there is an increasing function ${\bar{g}}_{\epsilon }$ satisfying (M) with positive equilibrium $\kappa _+\in (K,K+\epsilon )$, ${\mathfrak {c}}_*(L_{{\bar{g}}_{\epsilon }})\le {\mathfrak {c}}_*( L_g)$ and $g\le {\bar{g}}_{\epsilon }$. Furthermore, there is also an increasing ${\underline{g}}_{\epsilon }$ function meeting (M) with positive equilibrium $\kappa _-\in (m_K-\epsilon ,m_K)$ and ${\mathfrak {c}}_*(L_{{\underline{g}}_{\epsilon }})\le {\mathfrak {c}}_* (L_g)$ such that: ${\underline{g}}_{\epsilon }(x)\le g(x)$ for $x\in [0,K+\epsilon ]$.

Now, if ${\bar{v}}(t)$ is the homogenous solution of (17) replacing g by ${\bar{g}}_\epsilon$ with initial datum $v_0(s)=q, s\in [-h,0],$ and $c>{\mathfrak {c}}_*(L_g)$ then by Lemma 21 and the global stability of $\kappa _+$ there is a number $T>0$ such that

$$\begin{aligned} v(t,z)\le {\bar{v}}(t)\le K+\epsilon \quad \hbox {for all}\quad (t,z)\in [T,+\infty )\times {\mathbb {R}}. \end{aligned}$$

(63)

Next, by (63)

$$\begin{aligned} \sup _{(t,z)\in {\mathbb {R}}_+\times {\mathbb {R}}}v(t,z)=: v_{\infty }<\infty , \end{aligned}$$

and by denoting $\kappa _*:=\min _{u\in [\kappa ,u_{\infty }]}g(u)$ we take an increasing function $g_{\infty }$ satisfying (M) with equilibrium $\kappa _{\infty }\in (\kappa _*-\epsilon ,\kappa _*)$, ${\mathfrak {c}}_*(L_{g_{\infty }})\le {\mathfrak {c}}_*(L_g)$ and $g_{\infty }(u)\le g(u)$ for all $u\in [0,u_{\infty }].$ Next, without loss of generality we take $q_{ \infty }:=\kappa _{\infty }-q_0>0$. Then, by [1, Theorem 3], (21) and (22) there exist a monotone wavefront $\phi ^{\infty }_c$ to (17) (with nonlinearity $g_{\infty }$) such that

$$\begin{aligned} \phi ^{\infty }_c(z)-q\eta _b(z)\le v_0(s,z)\quad (s,z)\in [-h,0]\times {\mathbb {R}}\end{aligned}$$

(64)

thus by [32, Lemma 2.1] there are $C_0>0$ and $\gamma \ge 0$ such that

$$\begin{aligned} \phi _c^{\infty }(z-C_0q)-q^{-\gamma t}\eta _b(z)\le v_{\infty }(t,z)\quad (t,z)\times [-h,\infty )\times {\mathbb {R}}\end{aligned}$$

(65)

where $v_{\infty }(t,z)$ is the solution generated by the initial datum v(s, z) to Eq. (17). Now, by applying Lemma 21 with $D=[0,u_{\infty }]$

$$\begin{aligned} \phi _c^{\infty }(z-C_0q)-q^{-\gamma t}\eta _b(z)\le v(t,z)\quad (t,z)\times [-h,\infty )\times {\mathbb {R}}. \end{aligned}$$

So, there are $z_0'$ and $q'_0>0$ such that

$$\begin{aligned} v(t,z)\ge q'_0>0\quad (t,z)\in [-h,\infty )\times [z'_0,\infty ) \end{aligned}$$

(66)

Otherwise, denoting ${\underline{v}}(t,z)$ the solution of (17) replacing g by ${\underline{g}}_{\epsilon }$ with initial data ${\underline{v}}_0(s,z)=v(s+T+h,z).$ Due to (66) and Remark 19 the initial datum ${\underline{v}}_0$ satisfies (21) and (22). Next, if we denote by ${\underline{v}}(t)$ the homogenous solution of (17) replacing g by ${\underline{g}}_\epsilon$ with initial datum $v_0(s)=K+\epsilon , s\in [-h,0],$ then by [30, Corollary 2.2,p.82] ${\underline{v}}(t)$ converges monotonically to $\kappa _-$, therefore

$$\begin{aligned} {\underline{v}}(t,z)\le {\underline{v}}(t)\le K+\epsilon \quad \hbox {for all} \ (t,z)\in [-h,+\infty )\times {\mathbb {R}}\end{aligned}$$

So, for $c>c(L_g)$ by Lemma 21 (with $D=[0,K+\epsilon ]$), Proposition 7 and [33, Theorem 1] there is a wavefront ${\underline{\phi }}_c$ and $T_0>0$ such that

$$\begin{aligned} m_K-\epsilon \le {\underline{\phi }}_c(z)+\epsilon /2\le {\underline{v}}(t,z)\le v(t,z)\quad \hbox {for all}\quad (t,z)\in [T_0,\infty )^2. \end{aligned}$$

(67)

Thus there is $T_{v}$ such that the function ${\tilde{v}}(t,z):=v(t+T_v+h,z)$ satisfies (54) with $b=t_v+ch$ and $I={\mathcal {I}}_{\epsilon }$. Analogously, for some $T_{\psi _c}$ we have $\psi _c(z)\in {\mathcal {I}}_{\epsilon }$ for all $z\ge T_{\psi _c}$. Finally, by applying Theorem 18 we conclude (24) with $C:=\max _{z\in {\mathbb {R}}}\eta _{b}(z)/\eta _{t_0+ch}(z)$ where $t_0:=\max \{T_v,T_{\psi _c} \}.$ $\square$