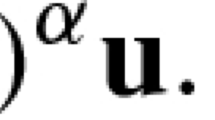Global existence of solutions of the time fractional Cahn–Hilliard equation in ${\mathbb {R}}^3$

Abstract

Cauchy problem for the Caputo-type time fractional Cahn–Hilliard equation in ${\mathbb {R}}^3$ is examined. The local existence and uniqueness of mild solutions and strong solutions are obtained for the initial data $u_0$ satisfying $u_0-{\bar{u}}\in L^\infty ({\mathbb {R}}^3)\cap L^1({\mathbb {R}}^3)$, where ${\bar{u}}$ is an equilibrium constant. The local solutions are extended globally if $u_0-{\bar{u}}$ is small in $L^1({\mathbb {R}}^3)$. These results are consistent with those of the traditional Cahn–Hilliard equation such as the property of mass conservation. However, extra difficulties arise in dealing with the singularity of Mittag-Leffler operators and non-Markovian property in the Caputo-type time fractional problem.

1 Introduction

In recent years, partial differential equations involving time fractional derivatives have attracted much attention since the time fractional derivatives can provide a nice instrument for the description of memory and hereditary properties of various materials and processes. These advantages of fractional models in comparison with classical integer-order models have offered strong motivation on many applications, such as dispersive anomalous diffusion problems [25, 26], control engineering investigations [6, 28], biological and medical systems [11, 13, 16, 17], financial study [31] and image processing researches [14, 20].

In present paper, we are interested in the following Cauchy problem for the time fractional Cahn–Hilliard equation in ${\mathbb {R}}^3$,

$$\begin{aligned} {\left\{ \begin{array}{ll} {_{c}{D}}^{\alpha }_{t} u +\Delta ^2 u + \Delta \varphi (u)=0, \qquad &{} \text{ in } {\mathbb {R}}^3\times (0,\infty ),\\ u(0) = u_0, &{} \text{ in } {\mathbb {R}}^3, \end{array}\right. } \end{aligned}$$

(1.1)

where $0<\alpha <1$, ${_{c}{D}}^{\alpha }_{t}$ denotes the Caputo fractional derivative operator of order $\alpha$, $\Delta ^2$ is the biharmonic operator and $\varphi$ is a smooth nonlinear function satisfying $\varphi (u)=O(1)|u-{\bar{u}}|^\sigma$ as $u\rightarrow {\bar{u}}$ with equilibrium constant ${\bar{u}}>0$ and $\sigma = \frac{5}{3}$.

Recall the classical Cahn–Hilliard equation

$$\begin{aligned} \partial _t u+\Delta ^2 u+ \Delta \varphi (u)=0, \qquad \text{ in } {\mathbb {R}}^3\times (0,\infty ) \end{aligned}$$

(1.2)

arising in the study of phase separation on cooling binary solutions such as glasses, alloys and polymer mixtures [5]. Here, u is the relative concentration between two phases. The nonlinearity of the problem is defined by the function

$$\begin{aligned} \Phi (u)=-\int _0^u\varphi (s)\mathrm {d}s,\end{aligned}$$

a double-well potential with two equal minima at $u^-\le u^+$ corresponding to the two pure phases. A typical example of such potential is $\Phi (u) = (1-u^2)^2$, and $u^-=-1$, $u^+=1$.

For the Cauchy problem of the classical Cahn–Hilliard equation (1.2), Caffarelli and Muler [4] assumed that the function $B(u) = \varphi (u)-u$ is Lipschitz continuous and equals to a constant outside a bounded interval with respect to u. They obtained the $L^\infty$ bound of solutions with initial data $u_0 \in W^{1, \infty }({{\mathbb {R}}}^N)$. Bricmont et al. [3] considered nonlinear stability and long-time asymptotic behaviors of solutions in ${\mathbb {R}}^1$ with the initial data close to the stationary solution ${\tilde{u}} = \tanh (\frac{x}{2})$. Liu et al. [23] obtained global solution existence and its asymptotic behaviors under the assumption that the nonlinear function $\varphi (u)$ satisfies a certain local growth condition at a fixed point $u^-$ and $\Vert u_0 - u^-\Vert _{L^1}$ is small.

On the other hand, the theory of time fractional partial differential equations has been developed by many authors. Eidelman and Kochubei [9] constructed a fundamental solution of an equation with time fractional derivative and a uniformly elliptic operator with variable coefficients acting in the spatial variables. Wang et al. [37] obtained the existence of mild solutions and classical solutions to an equation with almost sectorial operators by constructing a pair of families of operators in terms of the generalized Mittag-Leffler-type functions and the properties of resolvent operators. Andrade et al. [1] studied several questions concerning to abstract fractional Cauchy problems, including the existence of local mild solutions for the problem, and its possible continuation to a maximal interval of existence. Carvalho-Neto and Planas [7] shown the existence and uniqueness of mild solutions to time fractional Navier–Stokes equations. Li et al. [21] investigated nonlinear fractional time-space generalized Keller–Segel equation. They obtain the existence and uniqueness of mild solutions and some other properties, such as the nonnegativity preservation, mass conservation and blowup behaviors. A general review on mild solutions of time fractional partial differential equations is presented in [8].

In the understanding of nonlinear phenomena for the anomalously subdiffusive transport behaviors in heterogeneous porous materials or memory effects of certain materials, numerical simulations for the time fractional Cahn–Hilliard equation have been obtained in [12, 22, 32, 35, 36, 38, 39]. For example, the study of numerical solutions was ever given by Tripathi et al. [36] by using the homotopy analysis method to a time fractional Cahn–Hilliard equation involving an advection and reaction term. Tang et al. [35] proved for the first time that the time-fractional phase-field models indeed admit an energy dissipation law of an integral type. In addition to the numerical study of time fractional Cahn–Hilliard equation, a theoretical study was given by Jan et al. [30] on the well-posedness and long-time behaviour for the non-isothermal Cahn–Hilliard equation with memory in a bounded domain of ${\mathbb {R}}^3$.

To the best of our knowledge, the well-posedness for the solutions of the time fractional Cahn–Hilliard equation in ${\mathbb {R}}^3$ in $L^p$ spaces is not clear at present. The main purpose of this paper is to show some rigorous analytical theory on the problem (1.1), including the existence and uniqueness of mild solutions and strong solutions. Due to the observation that the biharmonic operator in the linear part of problem (1.1) can be regarded as a sectorial operator on some spaces, we follow some ideas in [8], properly adapted to our problem. It’s worth mentioning that the singularity together with strong nonlinearity arising from the time fractional derivative ${_{c}{D}}^{\alpha }_{t} u$ and the nonlinear term $\Delta \varphi (u)$ make its mathematical analysis more difficult in comparison with the classical Cahn–Hilliard equation (1.2). For example, the Mittag-Leffler operators $E_{\alpha }(-t^{\alpha }{\mathcal {A}})$ and $E_{\alpha ,\alpha }(-t^{\alpha }{\mathcal {A}})$ (see (2.7) below) do not satisfy the semigroup properties. So we need to overcome these essential difficulties to get some a priori estimates and extend the local solution to a global one. For more details, one can refer to Sects. 4 and 5.

This paper is organized as follows. In the next section, we introduce some elementary properties of Mittag-Leffler operators, which are essential throughout the whole paper and give the main results of this paper. In Sect. 3, $L^p-L^q$ estimates are given for the associated linear homogeneous problem. In Sect. 4, we first establish the existence and uniqueness of local mild solutions of the Cauchy problem (1.1) by the Banach contraction mapping principle, and then extend the local solution globally. Section 5 is dedicated to show that the global mild solution obtained in Sect. 4 is a strong solution.

2 Preliminaries and main results

Let ${\mathcal {S}}({\mathbb {R}}^3)$ be the Schwartz class of rapidly decreasing functions and $\mathring{L}^p({\mathbb {R}}^3)$ be the subspace of $L^p({\mathbb {R}}^3)$ so that ${\mathcal {S}}({\mathbb {R}}^3)$ is dense in $\mathring{L}^p({\mathbb {R}}^3)$ for $1\le p\le \infty$. It is readily seen that $\mathring{L}^p({\mathbb {R}}^3)=L^p({\mathbb {R}}^3)$ and $\mathring{L}^\infty ({\mathbb {R}}^3)= C_0({\mathbb {R}}^3)$ which denote the space of all continuous functions decaying to zero at infinity. For convenience, we always assume $1\le p\le \infty$ for the symbol $\mathring{L}^p({\mathbb {R}}^3)$. Given $f\in {\mathcal {S}}({\mathbb {R}}^3)$, its Fourier transform ${\mathcal {F}}f={\hat{f}}$ and inverse Fourier transform ${\mathcal {F}}^{-1}f={\check{f}}$ are defined by

$$\begin{aligned} {\hat{f}}(\xi )=(2\pi )^{-\frac{3}{2}}\int _{{\mathbb {R}}^3}f(x)e^{-ix\cdot \xi }\,\mathrm {d}x \quad \text {and}\quad {\check{f}}(\xi )=(2\pi )^{-\frac{3}{2}}\int _{{\mathbb {R}}^3} f(x)e^{ix\cdot \xi }\,\mathrm {d}x. \end{aligned}$$

The following lemma about the continuity for the shift functions is followed by Theorem 4.26 in [2].

Lemma 2.1

For any fixed $f\in \mathring{L}^p({\mathbb {R}}^3)$, $\Vert f(x+h)\Vert _{L^p({\mathbb {R}}^3)}$ is uniformly continuous with respect to $h\in {\mathbb {R}}^3$.

Then we get the uniform continuity of a convolution product.

Lemma 2.2

Let $g\in {\mathcal {S}}({\mathbb {R}}^3)$ and $f\in \mathring{L}^p({\mathbb {R}}^3)$. Then $g*f$ is uniformly continuous. Moreover, $\lim _{|x|\rightarrow \infty }g*f(x)=0$.

Proof

Take $f\in \mathring{L}^p({\mathbb {R}}^3)$. Applying Hölder inequality yields

$$\begin{aligned} |g*f(x+h)-g*f(x)|&\le \int _{{\mathbb {R}}^3}g(y)|f(x-y+h)-f(x-y)|\,\mathrm {d}y\\&\le \Vert g\Vert _{L^q({\mathbb {R}}^3)}\cdot \Vert f(x+h)-f(x)\Vert _{L^p({\mathbb {R}}^3)}, \end{aligned}$$

where $q\in [1,\infty ]$ such that $\frac{1}{p}+\frac{1}{q}=1$. Then Lemma 2.1 implies the uniform continuity of $g*f$. Since $g\in {\mathcal {S}}({\mathbb {R}}^3)$, it is easy to check that $g*f$ vanishes at infinity. $\square$

It is clear that the solution of the following linear problem

$$\begin{aligned} {\left\{ \begin{array}{ll} \partial _tu + \Delta ^2u = 0, \quad (x,t)\in {\mathbb {R}}^3\times (0,\infty ), \\ u(x,0) = u_0(x) \end{array}\right. } \end{aligned}$$

(2.1)

can be written as

$$\begin{aligned} u(x,t)=G_t*u_0, \end{aligned}$$

where $G_t(x)={\mathcal {F}}^{-1}(e^{-|\xi |^4t})$ is the fundamental solution of $\partial _t u + \Delta ^2 u = 0$. For any fixed $t>0$, we define the operator T(t) on $L^p({\mathbb {R}}^3)$ by

$$\begin{aligned} T(t)f:=G_t*f. \end{aligned}$$

By a simple calculation ([23]), we could get $\Vert G_t\Vert _{L^1({\mathbb {R}}^3)}=C$ for $t>0$, where $C>0$ is independent of t. Then the Young’s inequality yields

$$\begin{aligned} \Vert T(t)f\Vert _{L^p({\mathbb {R}}^3)}\le \Vert G_t\Vert _{L^1({\mathbb {R}}^3)}\Vert f\Vert _{L^p({\mathbb {R}}^3)}=C\Vert f\Vert _{L^p({\mathbb {R}}^3)}. \end{aligned}$$

(2.2)

So the operator $T(t): L^p({\mathbb {R}}^3)\rightarrow L^p({\mathbb {R}}^3)$ with $1\le p\le \infty$ are well defined for any fixed $t>0$. Moreover, we have the following result.

Proposition 2.1

([10], Proposition 2.13 ) The operators $\{T(t); t>0\}$ with $T(0)=I$ form a strongly continuous semigroup on $\mathring{L}^p({\mathbb {R}}^3)$, and its generator ${\mathcal {A}}$ coincides with the closure of the biharmonic operator

$$\begin{aligned} (-\Delta )^2f(x)=\sum _{i,j=1}^{3}\frac{\partial ^2}{\partial x_i^2}\frac{\partial ^2}{\partial x_j^2}f(x) \end{aligned}$$

defined for every $f\in {\mathcal {S}}({\mathbb {R}}^3)$.

Let $T>0$ and X be a Banach space. We introduce the definitions of fractional integration and derivation as follows.

Definition 2.1

Let $0<\alpha <1$ and $v\in L^1(0,T; X)$, the Riemann-Liouville fractional integral of order $\alpha >0$ is given by

$$\begin{aligned} J_t^{\alpha }v(t):=(g_{\alpha }*v)(t)=\frac{1}{\Gamma (\alpha )}\int _0^t(t-s)^{\alpha -1}v(s)\,\mathrm {d}s, \end{aligned}$$

where $\Gamma$ is the Gamma function and

$$\begin{aligned} \begin{array}{ll} &{} {g_{\alpha }(t)=\left\{ \begin{array}{lll} &{}\frac{1}{\Gamma (\alpha )}t^{\alpha -1}, &{} t>0, \\ &{}0, &{} t\le 0. \end{array} \right. } \end{array} \end{aligned}$$

Based on the Riemann-Liouville fractional integral operator, we present the Caputo fractional differential operator.

Definition 2.2

Let $0<\alpha <1$ and $v\in C([0,T]; X)$ such that the convolution $g_{1-\alpha }*v\in W^{1,1}(0,T; X)$. The Caputo fractional derivative of order $\alpha$ of v is defined by

$$\begin{aligned} {_{c}{D}}^{\alpha }_{t} v(t):=\frac{\mathrm {d}}{\mathrm {d}t}J_t^{1-\alpha }(v-v(0))(t)=\frac{1}{\Gamma (1-\alpha )}\frac{\mathrm {d}}{\mathrm {d}t}\int _0^t(t-s)^{-\alpha }(v(s)-v(0))\,\mathrm {d}s.\nonumber \\ \end{aligned}$$

(2.3)

Let’s recall some properties of Mittag-Leffler operators. For $\alpha \in (0,1)$, we denote the entire function $M_{\alpha }: {\mathbb {C}}\rightarrow {\mathbb {C}}$ the Mainardi function by

$$\begin{aligned} M_{\alpha }(z):=\sum _{n=0}^{\infty }\frac{(-z)^n}{n!\Gamma (1-\alpha (1+n))}, \end{aligned}$$

which is a particular case of the Wright type function introduced by Mainardi in [24] in order to characterize the fundamental solutions for some standard boundary value problems in physics. The following classical result gives some essential relations used in this paper to obtain the main estimates.

Proposition 2.2

([7], Proposition 2) Let $0<\alpha <1$ and $-1<\gamma <\infty$. If we restrict $M_{\alpha }$ to the positive real line, then it holds that

$$\begin{aligned} M_{\alpha }(t)\ge 0 \ \ \text {for all}\ t\ge 0 \ \ \text {and } \int _0^{\infty }t^{\gamma }M_{\alpha }(t)\,\mathrm {d}t=\frac{\Gamma (\gamma +1)}{\Gamma (\alpha \gamma +1)}. \end{aligned}$$

For each $\alpha \in (0,1)$, the Mittag-Leffler families is given by

$$\begin{aligned} E_{\alpha }(-t^{\alpha }{\mathcal {A}}):=\int _0^{\infty }M_{\alpha }(s)T(st^{\alpha })\,\mathrm {d}s, \end{aligned}$$

and

$$\begin{aligned} E_{\alpha ,\alpha }(-t^{\alpha }{\mathcal {A}}):=\int _0^{\infty }\alpha sM_{\alpha }(s)T(st^{\alpha })\,\mathrm {d}s. \end{aligned}$$

It is interesting to notice that the Mainardi functions act as a bridge between the fractional and the classical abstract theories. Indeed we have

$$\begin{aligned} E_{\alpha }(-t^{\alpha }{\mathcal {A}}) = \frac{1}{2 \pi i} \int _{H_a} e^{\lambda t} \lambda ^{\alpha -1}\left( \lambda ^{\alpha }+{\mathcal {A}}\right) ^{-1} d \lambda , \end{aligned}$$

(2.4)

and

$$\begin{aligned} E_{\alpha ,\alpha }(-t^{\alpha }{\mathcal {A}}) = \frac{t^{1-\alpha }}{2 \pi i} \int _{H_a} e^{\lambda t}\left( \lambda ^{\alpha }+{\mathcal {A}}\right) ^{-1} d \lambda , \end{aligned}$$

(2.5)

where $H_a$ is any Hankel’s path, i.e. a loop which starts and ends at $-\infty$ and encircles the circular disk $|\lambda |\le |z|^{1/\alpha }$. For more details see [8, 37].

Similarly to [37], we have the following continuity property.

Proposition 2.3

$E_{\alpha }(-t^{\alpha }{\mathcal {A}})$ and $E_{\alpha ,\alpha }(-t^{\alpha }{\mathcal {A}})$ are well defined from $\mathring{L}^p({\mathbb {R}}^3)$ to $\mathring{L}^p({\mathbb {R}}^3)$. For $t>0$, $E_{\alpha }(-t^{\alpha }{\mathcal {A}})$ and $E_{\alpha ,\alpha }(-t^{\alpha }{\mathcal {A}})$ are continuous in the uniform operator topology on $\mathring{L}^p({\mathbb {R}}^3)$. Moreover, for every $r>0$, the continuity is uniform on $[r, \infty )$.

Proof

Taking $v\in \mathring{L}^p({\mathbb {R}}^3)$, we have

$$\begin{aligned} \Vert E_{\alpha }(-t_2^{\alpha }{\mathcal {A}})v-E_{\alpha }(-t_1^{\alpha }{\mathcal {A}})v\Vert _{L^{p}({\mathbb {R}}^3)}&\le \int _0^{\infty }M_{\alpha }(s)\Vert T(st_2^{\alpha })v-T(st_1^{\alpha })v\Vert _{L^{p}({\mathbb {R}}^3)}\,\mathrm {d}s, \end{aligned}$$

where $1\le p\le \infty$. Let $\varepsilon >0$ and $r>0$. By the properties of the Mainardi function given in Proposition 2.2, we may choose $0<\delta _1<\delta _2$ such that

$$\begin{aligned} 2\Vert v\Vert _{L^{p}({\mathbb {R}}^3)}\int _0^{\delta _1}M_{\alpha }(s)\,\mathrm {d}s< \frac{\varepsilon }{3} \quad \mathrm{and}\quad 2\Vert v\Vert _{L^{p}({\mathbb {R}}^3)}\int _{\delta _2}^{\infty }M_{\alpha }(s)\,\mathrm {d}s< \frac{\varepsilon }{3}. \end{aligned}$$

In addition, by the strongly continuity and the uniform boundedness of T(t), we deduce that there exists a positive constant $\gamma >0$ such that

$$\begin{aligned} \int _{\delta _1}^{\delta _2}M_{\alpha }(s)\Vert T(st_1^{\alpha })-T(st_2^{\alpha })\Vert \Vert v\Vert _{L^{p}({\mathbb {R}}^3)}\,\mathrm {d}s < \frac{\varepsilon }{3} \end{aligned}$$

for any $t_1, t_2\ge r$ and $|t_2-t_1|<\gamma$. Therefore, combining with Proposition 3.1 below, we obtain that

$$\begin{aligned} \Vert E_{\alpha }(-t_2^{\alpha }{\mathcal {A}})-E_{\alpha }(-t_1^{\alpha }{\mathcal {A}})\Vert <\varepsilon , \end{aligned}$$

which implies that $E_{\alpha }(-t^{\alpha }{\mathcal {A}})$ is uniformly continuous on $[r,\infty )$ in the uniform operator topology on $\mathring{L}^p({\mathbb {R}}^3)$. By the arbitrariness of $r>0$, $E_{\alpha }(-t^{\alpha }{\mathcal {A}})$ is continuous in the uniform operator topology for $t>0$. A similar argument enables us to give the characterization of continuity on $E_{\alpha ,\alpha }(-t^{\alpha }{\mathcal {A}})$. $\square$

We end this section with the concept of the solution of (1.1) and our main results. For a given function f and a smooth function $u: {\mathbb {R}}^3 \times {\mathbb {R}}^+ \rightarrow X$, we formally give a mild solution for the Cauchy problem

$$\begin{aligned} {\left\{ \begin{array}{ll} {_{c}{D}}^{\alpha }_{t} u =-{\mathcal {A}} u+f(x, t), \quad t>0 \\ u(x, 0)=u_{0}(x) \in X, \end{array}\right. } \end{aligned}$$

(2.6)

where the rigorous deduction could be found in [8, 15, 18, 37]. Denote ${\mathcal {L}}$ the operator of Laplace transform. By the convolution property of Laplace transform, we have

$$\begin{aligned} {\mathcal {L}}({{_{c}{D}}^{\alpha }_{t}u})=\lambda ^{\alpha }{\mathcal {L}}(u)-\lambda ^{\alpha -1}u_0, \end{aligned}$$

as in [18, Proposition 3.13]. So taking the Laplace transform to (2.6) gives

$$\begin{aligned} {\mathcal {L}}(u) = \lambda ^{\alpha -1}(\lambda ^\alpha +{\mathcal {A}})^{-1} u_0 + (\lambda ^\alpha + {\mathcal {A}})^{-1} {\mathcal {L}}(f). \end{aligned}$$

Application of Laplace inversion ([8, Proposition 2.43]) implies

$$\begin{aligned} u(t)=E_{\alpha }\left( -t^{\alpha } {\mathcal {A}}\right) u_{0} +\int _{0}^{t}(t-s)^{\alpha -1} E_{\alpha , \alpha }\left( -(t-s)^{\alpha } {\mathcal {A}}\right) f(s) \mathrm {d} s. \end{aligned}$$

This formal computation then motivates the definition of the mild solution of (1.1) as follows:

Definition 2.3

Let $0<\alpha <1$ and $T>0$ .

(i) A function u such that $u-{\bar{u}}\in C([0,T]; L^{1}({\mathbb {R}}^3))$ defined by

$$\begin{aligned} u(x,t)=E_{\alpha }(-t^{\alpha }{\mathcal {A}})u_0-\int _0^t(t-s)^{\alpha -1}\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\,\mathrm {d}s \end{aligned}$$

(2.7)

is called a local mild solution of the Cauchy problem (1.1).

(ii) If $T=\infty$, we say that u is a global mild solution of the Cauchy problem (1.1).

Now we are the position to give the first result in this paper.

Theorem 2.1

(Global existence of mild solution) Assume that $u_0-{\bar{u}}\in L^1({\mathbb {R}}^3)\cap L^{\infty }({\mathbb {R}}^3)$ with sufficiently small $\Vert u_0-{\bar{u}}\Vert _{L^1({\mathbb {R}}^3)}$ and the nonlinear function $\varphi (u)\in C^2({\mathbb {R}})$ satisfies $\varphi (u)=O(1)|u-{\bar{u}}|^\sigma$ as $u\rightarrow {\bar{u}}$ with $\sigma = \frac{5}{3}$. Then the Cauchy problem (1.1) admits a unique global mild solution u satisfying

$$\begin{aligned} \Vert u(t)-{\bar{u}}\Vert _{L^{\infty }({\mathbb {R}}^3)}\le 2\Vert u_0-{\bar{u}}\Vert _{L^{\infty }({\mathbb {R}}^3)},\quad t\ge 0 \end{aligned}$$

and the integral preservation property,

$$\begin{aligned} \int _{{\mathbb {R}}^3}(u(x,t)-{\bar{u}})\,\mathrm {d}x=\int _{{\mathbb {R}}^3}(u_0(x)-{\bar{u}})\,\mathrm {d}x. \end{aligned}$$

The second result of this paper is concerned with the global existence of the strong solutions, of which we give the definition as follows.

Definition 2.4

Let $0<\alpha <1$ and $T>0$. By a strong solution to problem (1.1), we mean that a function u such that $u-{\bar{u}}\in C([0,T]; L^{1}({\mathbb {R}}^3))\cap C((0,T]; W^{4,1}({\mathbb {R}}^3))$ and ${_{c}{D}}^{\alpha }_{t} u \in C((0,T]; L^{1}({\mathbb {R}}^3))$ satisfies (1.1) almost everywhere. If $T=\infty$, we call u a global strong solution.

If the unique mild solution u satisfies $u-{\bar{u}}\in C((0,T]; W^{4,1}({\mathbb {R}}^3))$, it’s easy to verify that $u-{\bar{u}}\in C^{\alpha }((0,T]; L^1({\mathbb {R}}^3))$ by [19, Proposition 3.3]. Naturally, we expect that

$$\begin{aligned} {_{c}{D}}^{\alpha }_{t} u(t)={_{c}{D}}^{\alpha }_{t} (u-{\bar{u}})(t)=\frac{\mathrm {d}}{\mathrm {d}t}J_t^{1-\alpha }(u-u(0))(t) \end{aligned}$$

exists at least almost everywhere with respect to $t\in (0,\infty )$. However, this expectation may not be true (see [33]). That is to say, ${_{c}{D}}^{\alpha }_{t} u$ may be just a distribution. This disadvantage is another difficulty arises from the singularity of the time fractional derivative. In order to prove that the mild solution u is a strong solution, we have to employ other methods to show that

$$\begin{aligned} {_{c}{D}}^{\alpha }_{t} u \in C((0,T]; L^{1}({\mathbb {R}}^3)). \end{aligned}$$

Theorem 2.2

(Global existence of strong solution) Assume that the assumptions in Theorem 2.1 are satisfied and $\varphi (u)\in C^3({\mathbb {R}})$, then the global mild solution u of the Cauchy problem (1.1) is in fact a global strong solution and satisfies $u-{\bar{u}}\in C((0,\infty ); W^{4,p}({\mathbb {R}}^3)\cap C_0({\mathbb {R}}^3))$ for each $1\le p <\infty$.

Remark 2.1

Although the $L^1$-norm of $u(x,t)-{\bar{u}}$ is small in our results, the $L^{\infty }$-norm of $u(x,t)-{\bar{u}}$ is not necessarily small.

Remark 2.2

The existence and uniqueness of the solution obtained this paper can be easily extended to the case of ${\mathbb {R}}^N$ with $N=1,2$. While for larger N, due to the limitation of $L^p-L^q$ estimates of Mittag-Leffler operators, we can’t get the same results now.

Remark 2.3

If we take the usual Cahn–Hilliard potential $\Phi (u) = (1-u^2)^2$ and set ${\bar{u}} = 1$, then we have $\varphi (u) = u-u^3$. As mentioned in [23], the equation (1.1) could be rewritten as

$$\begin{aligned} {_{c}{D}}^{\alpha }_{t} u(x, t) +\Delta ^2 u(x, t) - 2\Delta u + \Delta (1-u)^2(u+2)=0. \end{aligned}$$

By taking ${\mathcal {A}} = \Delta ^2 - 2\Delta$, we could obtain the same results as Theorem 2.1 and Theorem 2.2 since $(1-u)^2(u+2) = O(1)|u-1|^{\frac{5}{3}}$ as $u\rightarrow 1$.

3 $L^p-L^q$ estimates

We first recall the $L^p-L^q$ estimates for the operator T(t) in the following result. For convenience, we will usually use C to denote a generic positive constant which may vary from line to line.

Proposition 3.1

([27], Lemma 3.1) Let $1\le q\le p\le \infty$ and $\nu \ge 0$. For any $u\in L^{q}({\mathbb {R}}^3)$, we have

$$\begin{aligned} \Vert (-\Delta )^{\frac{\nu }{2}}T(t)u\Vert _{L^p({\mathbb {R}}^3)}&\le C\, t^{-\frac{3}{4}(\frac{1}{q}-\frac{1}{p})-\frac{\nu }{4}}\Vert u\Vert _{L^q({\mathbb {R}}^3)}, \quad t>0, \end{aligned}$$

where $(-\Delta )^{\frac{\nu }{2}} = {\mathcal {F}}^{-1} (|\xi |^{\nu })$ denote the differential operator of $\nu$-th order.

Now we show the similar $L^p-L^q$ estimates for both families of Mittag-Leffler operators.

Proposition 3.2

Let $1\le q\le p\le \infty$ and $\frac{1}{q}-\frac{1}{p}<\frac{4-\nu }{3}$ with $\nu \in [0,4)$. For $u\in L^{q}({\mathbb {R}}^3)$, we have

$$\begin{aligned} \Vert (-\Delta )^{\frac{\nu }{2}}E_{\alpha }(-t^{\alpha }{\mathcal {A}})u\Vert _{L^p({\mathbb {R}}^3)}\le C\, t^{-\frac{3\alpha }{4}(\frac{1}{q}-\frac{1}{p})-\frac{\alpha \nu }{4}}\Vert u\Vert _{L^q({\mathbb {R}}^3)},\quad t>0. \end{aligned}$$

Moreover, if $\nu =4$, for $u\in \mathring{L}^{q}({\mathbb {R}}^3)$, we have

$$\begin{aligned} \Vert (-\Delta )^{2}E_{\alpha }(-t^{\alpha }{\mathcal {A}})u\Vert _{L^q({\mathbb {R}}^3)}\le C\, t^{-\alpha }\Vert u\Vert _{L^q({\mathbb {R}}^3)},\quad t>0. \end{aligned}$$

Proof

By Proposition 2.2 and 3.1, we obtain

$$\begin{aligned}&\Vert (-\Delta )^{\frac{\nu }{2}}E_{\alpha }(-t^{\alpha }{\mathcal {A}})u\Vert _{L^p({\mathbb {R}}^3)} \\&\quad \le \ \int _0^{\infty }M_{\alpha }(s)\Vert (-\Delta )^{\frac{\nu }{2}}T(st^{\alpha })u\Vert _{L^p({\mathbb {R}}^3)}\,\mathrm {d}s \\&\quad \le \ C\left( \int _0^{\infty }M_{\alpha }(s)s^{-\frac{3}{4}(\frac{1}{q}-\frac{1}{p})-\frac{\nu }{4}}\,\mathrm {d}s\right) \left( t^{-\frac{3\alpha }{4}(\frac{1}{q}-\frac{1}{p})-\frac{\alpha \nu }{4}}\Vert u\Vert _{L^q({\mathbb {R}}^3)}\right) \\&\quad \le \ \ C\, t^{-\frac{3\alpha }{4}(\frac{1}{q}-\frac{1}{p})-\frac{\alpha \nu }{4}}\Vert u\Vert _{L^q({\mathbb {R}}^3)}, \quad \nu \in [0,4). \end{aligned}$$

If $\nu =4$, taking the $\nu$-th derivative of $E_{\alpha }(-t^{\alpha }{\mathcal {A}})u$, we have

$$\begin{aligned} (-\Delta )^{\frac{\nu }{2}}E_{\alpha }(-t^{\alpha }{\mathcal {A}})u&= (-\Delta )^{\frac{\nu }{2}}\int _0^{\infty }M_{\alpha }(s)T(st^{\alpha })u\,\mathrm {d}s \nonumber \\&= (-\Delta )^{\frac{\nu }{2}}\int _0^{1}\left( M_{\alpha }(s)-M_{\alpha }(0)\right) T(st^{\alpha })u\,\mathrm {d}s \nonumber \\&\quad +M_{\alpha }(0)(-\Delta )^{\frac{\nu }{2}}\int _0^{1}T(st^{\alpha })u\,\mathrm {d}s +(-\Delta )^{\frac{\nu }{2}}\int _1^{\infty }M_{\alpha }(s)T(st^{\alpha })u\,\mathrm {d}s \nonumber \\&= I_1+I_2+I_3. \end{aligned}$$

(3.1)

By Proposition 2.2 and 3.1, it’s easy to see that

$$\begin{aligned} \Vert I_1\Vert _{L^q}&\le \int _0^{1}\left| M_{\alpha }(s)-M_{\alpha }(0)\right| \cdot \Vert (-\Delta )^{\frac{\nu }{2}} T(st^{\alpha })u\Vert _{L^q({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le C\left( \int _0^{1}\left| M_{\alpha }(s)-M_{\alpha }(0)\right| s^{-1}\,\mathrm {d}s\right) \left( t^{-\alpha }\Vert u\Vert _{L^q({\mathbb {R}}^3)}\right) \nonumber \\&\le C\, t^{-\alpha }\Vert u\Vert _{L^q({\mathbb {R}}^3)}, \end{aligned}$$

(3.2)

where we use the fact that

$$\begin{aligned} \sup _{0<s\le 1}|M_{\alpha }(s)-M_{\alpha }(0)|s^{-1}\le C. \end{aligned}$$

Similarly, we have

$$\begin{aligned} \Vert I_3\Vert _{L^q}&\le \int _1^{\infty }M_{\alpha }(s)\Vert (-\Delta )^{\frac{\nu }{2}}T(st^{\alpha })u\Vert _{L^q({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le C\left( \int _1^{\infty }M_{\alpha }(s)s^{-1}\,\mathrm {d}s\right) t^{-\alpha }\Vert u\Vert _{L^q({\mathbb {R}}^3)} \nonumber \\&\le C\, t^{-\alpha }\Vert u\Vert _{L^q({\mathbb {R}}^3)}. \end{aligned}$$

(3.3)

To estimate $I_2$, we see that for any $u\in \mathring{L}^{q}({\mathbb {R}}^3)$,

$$\begin{aligned} \frac{\mathrm d}{{\mathrm d}s}T(s)u=-{\mathcal {A}}\,T(s)u, \quad s>0. \end{aligned}$$

Then

$$\begin{aligned} \frac{\mathrm d}{{\mathrm d}s}T(st^{\alpha })u=-t^{\alpha }{\mathcal {A}}\,T(st^{\alpha })u, \quad s,t>0. \end{aligned}$$

Integrating the above equality with respect to s over [0, 1], we arrive at

$$\begin{aligned} T(t^{\alpha })u-u&= -t^{\alpha }\int _{0}^{1}{\mathcal {A}}\,T(st^{\alpha })u\,\mathrm {d}s. \end{aligned}$$

Then, we obtain

$$\begin{aligned} \Vert I_2\Vert _{L^q({\mathbb {R}}^3)}\le |M_{\alpha }(0)|\left\| \int _{0}^{1}{\mathcal {A}}\,T(st^{\alpha })u\,\mathrm {d}s\right\| _{L^q({\mathbb {R}}^3)}&\le C\, t^{-\alpha }\Vert u\Vert _{L^q({\mathbb {R}}^3)}. \end{aligned}$$

(3.4)

Inserting estimates (3.2)–(3.4) into (3.1), we complete the proof of Proposition 3.2. $\square$

Proposition 3.3

Let $1\le q\le p\le \infty$ and $\frac{1}{q}-\frac{1}{p}<\frac{8-\nu }{3}$ with $\nu \in [0,8)$. Then for $u\in L^{q}({\mathbb {R}}^3)$, we have

$$\begin{aligned} \Vert (-\Delta )^{\frac{\nu }{2}}E_{\alpha ,\alpha }(-t^{\alpha }{\mathcal {A}})u\Vert _{L^p({\mathbb {R}}^3)}\le C\, t^{-\frac{3\alpha }{4}(\frac{1}{q}-\frac{1}{p})-\frac{\alpha \nu }{4}}\Vert u\Vert _{L^q({\mathbb {R}}^3)},\quad t>0. \end{aligned}$$

Moreover, if $\nu =8$, for $u\in \mathring{L}^q({\mathbb {R}}^3)$, we have

$$\begin{aligned} \Vert (-\Delta )^{4}E_{\alpha ,\alpha }(-t^{\alpha }{\mathcal {A}})u\Vert _{L^q({\mathbb {R}}^3)}\le C\, t^{-2\alpha }\Vert u\Vert _{L^q({\mathbb {R}}^3)},\quad t>0. \end{aligned}$$

Proof

The proof is similar with that of Proposition 3.2. By Proposition 2.2 and 3.1, we obtain

$$\begin{aligned}&\Vert (-\Delta )^{\frac{\nu }{2}}E_{\alpha ,\alpha }(-t^{\alpha }{\mathcal {A}})u\Vert _{L^p({\mathbb {R}}^3)}\\&\quad \le \ \int _0^{\infty }\alpha sM_{\alpha }(s)\Vert (-\Delta )^{\frac{\nu }{2}}T(st^{\alpha })u\Vert _{L^p({\mathbb {R}}^3)}\,\mathrm {d}s \\&\quad \le \ C\left( \int _0^{\infty }M_{\alpha }(s)s^{1-\frac{3}{4}(\frac{1}{q}-\frac{1}{p})-\frac{\nu }{4}}\,\mathrm {d}s\right) \left( t^{-\frac{3\alpha }{4}(\frac{1}{q}-\frac{1}{p})-\frac{\alpha \nu }{4}}\Vert u\Vert _{L^q({\mathbb {R}}^3)}\right) \\&\quad \le \ C\, t^{-\frac{3\alpha }{4}(\frac{1}{q}-\frac{1}{p})-\frac{\alpha \nu }{4}}\Vert u\Vert _{L^q({\mathbb {R}}^3)}, \quad \nu \in [0,8). \end{aligned}$$

If $\nu =8$, taking the $\nu$-th derivative of $E_{\alpha ,\alpha }(-t^{\alpha }{\mathcal {A}})u$, we have

$$\begin{aligned} (-\Delta )^{\frac{\nu }{2}}E_{\alpha ,\alpha }(-t^{\alpha }{\mathcal {A}})u&= (-\Delta )^{\frac{\nu }{2}}\int _0^{\infty }\alpha sM_{\alpha }(s)T(st^{\alpha })u\,\mathrm {d}s \nonumber \\&= (-\Delta )^{\frac{\nu }{2}}\int _0^{1}\alpha s\left( M_{\alpha }(s)-M_{\alpha }(0)\right) T(st^{\alpha })u\,\mathrm {d}s \nonumber \\&\quad +M_{\alpha }(0)(-\Delta )^{\frac{\nu }{2}}\int _0^{1}\alpha sT(st^{\alpha })u\,\mathrm {d}s\nonumber \\&\quad +(-\Delta )^{\frac{\nu }{2}}\int _1^{\infty }\alpha sM_{\alpha }(s)T(st^{\alpha })u\,\mathrm {d}s \nonumber \\&= I_1+I_2+I_3. \end{aligned}$$

(3.5)

The estimates for $I_1$ and $I_3$ can be derived similarly as (3.2) and (3.3). To estimate $I_2$, we see that for any $u\in \mathring{L}^{q}({\mathbb {R}}^3)$,

$$\begin{aligned} st^{\alpha }{\mathcal {A}}\frac{\mathrm d}{{\mathrm d}s}T(st^{\alpha })u=-st^{2\alpha }{\mathcal {A}}^2\,T(st^{\alpha })u, \quad s,t>0. \end{aligned}$$

By the theory of analytic semigroups (see [10, 29]), it is not difficult to verify that $\{s{\mathcal {A}}T(s);\, s>0\}$ is strongly continuous on $\mathring{L}^q({\mathbb {R}}^3)$ with respect to $s\in [0,\infty )$. Integrating the above equality with respect to s over [0, 1] and integrating by parts, we arrive at

$$\begin{aligned} -t^{2\alpha }\int _{0}^{1}s{\mathcal {A}}^2\,T(st^{\alpha })u\,\mathrm {d}s&= \int _{0}^{1}st^{\alpha }{\mathcal {A}}\frac{\mathrm d}{{\mathrm d}s}T(st^{\alpha })u\,\mathrm {d}s\\&= t^{\alpha }{\mathcal {A}}T(t^{\alpha })u-u-\int _{0}^{1}t^{\alpha }{\mathcal {A}}T(st^{\alpha })u\,\mathrm {d}s \end{aligned}$$

Then, by the estimate (3.4) and Proposition 3.1, we obtain

$$\begin{aligned} \Vert I_2\Vert _{L^q({\mathbb {R}}^3)}&= \left\| \alpha M_{\alpha }(0)\int _{0}^{1}s{\mathcal {A}}^2\,T(st^{\alpha })u\,\mathrm {d}s\right\| _{L^q({\mathbb {R}}^3)} \nonumber \\&\le C\, t^{-2\alpha }\Vert t^{\alpha }{\mathcal {A}}T(t^{\alpha })u\Vert _{L^q({\mathbb {R}}^3)} +C\, t^{-2\alpha }\Vert u\Vert _{L^q({\mathbb {R}}^3)} \nonumber \\&\quad +C\, t^{-2\alpha }\left\| \int _{0}^{1}t^{\alpha }{\mathcal {A}}T(st^{\alpha })u\,\mathrm {d}s\right\| _{L^q({\mathbb {R}}^3)} \nonumber \\&\le C\, t^{-2\alpha }\Vert u\Vert _{L^q({\mathbb {R}}^3)}. \end{aligned}$$

(3.6)

Inserting the estimates for $I_1, I_2, I_3$ into (3.5), we complete the proof of Proposition 3.3. $\square$

Remark 3.1

It is a known fact that for unbounded generators of resolvent families, the solution becomes smoother at most by one unit of regularity in terms of the generator, see the monograph by Jan Pruss [29], which prevents us from getting more smooth solutions of the problem (1.1). In addition, the non-integrability of Mainardi function $M_{\alpha }$ with $\gamma \le -1$ implies that we can’t obtain the estimate for $(-\Delta )^{\frac{\nu }{2}}E_{\alpha }(-t^{\alpha }{\mathcal {A}})u$ with $\nu >4$, which also implies this disadvantage.

4 Mild solution

In this section, we shall construct a local mild solution u for the Cauchy problem (1.1) by employing Banach contracting mapping principle, and then extend u globally by virtue of the auxiliary estimate (4.6) below.

Proposition 4.1

([10, 29]) For any fixed $\nu >0$, $\{(-\Delta )^{\frac{\nu }{2}}T(t);\, t>0\}$ is strongly continuous on $\mathring{L}^p({\mathbb {R}}^3)$ with respect to $t\in (0,\infty )$.

Based on Proposition 4.1, we can obtain the strong continuity of both families of Mittag-Leffler operators.

Proposition 4.2

$(-\Delta )^{\frac{\nu _1}{2}}E_{\alpha }(-t^{\alpha }{\mathcal {A}})$ and $(-\Delta )^{\frac{\nu _2}{2}}E_{\alpha ,\alpha }(-t^{\alpha }{\mathcal {A}})$ are strongly continuous on $\mathring{L}^p({\mathbb {R}}^3)$ with respect to $t\in (0,\infty )$, where $\nu _1\in (0,4]$ and $\nu _2\in (0,8]$.

Proof

Taking $v\in \mathring{L}^p({\mathbb {R}}^3)$ and $t_0\in (0,\infty )$, we have

$$\begin{aligned}&\Vert (-\Delta )^{\frac{\nu _1}{2}}E_{\alpha }(-t^{\alpha }{\mathcal {A}})v-(-\Delta )^{\frac{\nu _1}{2}} E_{\alpha }(-t_0^{\alpha }{\mathcal {A}})v\Vert _{L^{p}({\mathbb {R}}^3)} \\&\le \int _0^{\infty }M_{\alpha }(s)\Vert (-\Delta )^{\frac{\nu _1}{2}}T(st^{\alpha })v- (-\Delta )^{\frac{\nu _1}{2}}T(st_0^{\alpha })v\Vert _{L^{p}({\mathbb {R}}^3)}\mathrm {d}s, \end{aligned}$$

where $1\le p\le \infty$ and $\nu _1\in (0,4]$. For any $\varepsilon >0$ and $\lambda \in (0,t_0)$, by Proposition 3.1 and the properties of the Mainardi function given in Proposition 2.2, we may choose $0<\delta _1<\delta _2$ such that

$$\begin{aligned} 2C\lambda ^{-\frac{\alpha \nu _1}{4}}\Vert v\Vert _{L^{p}({\mathbb {R}}^3)}\int _0^{\delta _1}M_{\alpha }(s)s^{-\frac{\nu _1}{4}}\,\mathrm {d}s< \frac{\varepsilon }{3} \end{aligned}$$

and

$$\begin{aligned} 2C\lambda ^{-\frac{\alpha \nu _1}{4}}\Vert v\Vert _{L^{p}({\mathbb {R}}^3)}\int _{\delta _2}^{\infty }M_{\alpha }(s)s^{-\frac{\nu _1}{4}}\,\mathrm {d}s< \frac{\varepsilon }{3}, \end{aligned}$$

where the positive constant C comes from Proposition 3.1 which is independent of $\lambda$ and $\varepsilon$. Then by the strong continuity of $(-\Delta )^{\frac{\nu _1}{2}}T(t)$, we deduce that there exists a positive constant $\gamma >0$ such that

$$\begin{aligned} \int _{\delta _1}^{\delta _2}M_{\alpha }(s)\Vert (-\Delta )^{\frac{\nu _1}{2}}T(st^{\alpha })v-(-\Delta )^{\frac{\nu _1}{2}}T(st_0^{\alpha })v\Vert _{L^{p}({\mathbb {R}}^3)}\mathrm {d}s < \frac{\varepsilon }{3} \end{aligned}$$

for $|t-t_0|<\gamma$ and $t, t_0>\lambda$. Therefore, we obtain that

$$\begin{aligned} \lim _{t\rightarrow t_0}\Vert (-\Delta )^{\frac{\nu _1}{2}}E_{\alpha }(-t^{\alpha }{\mathcal {A}})v-(-\Delta )^{\frac{\nu _1}{2}}E_{\alpha }(-t_0^{\alpha }{\mathcal {A}})v\Vert _{L^{p}({\mathbb {R}}^3)}=0, \end{aligned}$$

which implies that $(-\Delta )^{\frac{\nu _1}{2}}E_{\alpha }(-t^{\alpha }{\mathcal {A}})$ is strongly continuous in $(0,\infty )$. A similar argument enables us to give the strong continuity of $(-\Delta )^{\frac{\nu _2}{2}}E_{\alpha ,\alpha }(-t^{\alpha }{\mathcal {A}})$ in $(0,\infty )$. $\square$

Now we are ready to show the local existence of mild solutions for the time fractional Cahn–Hilliard equation.

Theorem 4.1

Assume that $u_0-{\bar{u}}\in L^1({\mathbb {R}}^3)\cap L^{\infty }({\mathbb {R}}^3)$ with $\Vert u_0-{\bar{u}}\Vert _{L^{\infty }({\mathbb {R}}^3)}\le r$ for some $r>0$ and $\varphi (u)\in C^2({\mathbb {R}})$. Then there exists $t_1>0$ such that the Cauchy problem (1.1) admits a unique mild solution u satisfying $u-{\bar{u}}\in C([0,t_1]; L^{1}({\mathbb {R}}^3))$ and

$$\begin{aligned} \sup _{0\le t\le t_1}\Vert u(t)-{\bar{u}}\Vert _{L^1({\mathbb {R}}^3)}\le 2\Vert u_0-{\bar{u}}\Vert _{L^1({\mathbb {R}}^3)}, \quad \Vert u(t)-{\bar{u}}\Vert _{L^{\infty }({\mathbb {R}}^3)}\le 2r,\quad 0\le t\le t_1.\nonumber \\ \end{aligned}$$

(4.1)

Furthermore, the integral is preserved, that is,

$$\begin{aligned} \int _{{\mathbb {R}}^3}(u(x,t)-{\bar{u}})\,\mathrm {d}x=\int _{{\mathbb {R}}^3}(u_0(x)-{\bar{u}})\,\mathrm {d}x. \end{aligned}$$

(4.2)

Proof

Noting that

$$\begin{aligned} T(t)1=G_t*1=\int _{{\mathbb {R}}^3}G_t(x)\,\mathrm {d}x=(e^{|\xi |^4t})|_{\xi =0}=1,\quad t>0, \end{aligned}$$

by Proposition 2.2, we obtain

$$\begin{aligned} E_{\alpha }(-t^{\alpha }{\mathcal {A}}){\bar{u}}=\int _0^{\infty }M_{\alpha }(s)T(st^{\alpha }){\bar{u}}\,\mathrm {d}s={\bar{u}}. \end{aligned}$$

Hence, the integro-differential equation (2.7) could be rewritten as

$$\begin{aligned} u(x,t)-{\bar{u}}=E_{\alpha }(-t^{\alpha }{\mathcal {A}})(u_0-{\bar{u}})-\int _0^t(t-s)^{\alpha -1}\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\,\mathrm {d}s. \end{aligned}$$

To employ the Banach contraction mapping principle, for some $t_1>0$, we define the set

$$\begin{aligned} {\mathcal {E}}_{t_1}= & {} \Big \{v\in C([0,t_1]; L^1({\mathbb {R}}^3))\cap L^{\infty }({\mathbb {R}}^3\times (0,t_1)); \\&\sup _{0\le t\le t_1}\Vert v(t)\Vert _{L^1({\mathbb {R}}^3)}\le 2\Vert u_0-{\bar{u}}\Vert _{L^1({\mathbb {R}}^3)} \ \mathrm{and}\ \Vert v(t)\Vert _{L^{\infty }({\mathbb {R}}^3\times (0,t_1))}\le 2r\Big \} \end{aligned}$$

and a map on this set

$$\begin{aligned} S_{t_1}v(x,t)&= E_{\alpha }(-t^{\alpha }{\mathcal {A}})(u_0-{\bar{u}}) -\int _{0}^{t}(t-s)^{\alpha -1}\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (v+{\bar{u}})\,\mathrm {d}s. \end{aligned}$$

(4.3)

Indeed, we shall prove there exists only one fixed point v(x, t) of the map $S_{t_1}$. Then $v(x,t)+{\bar{u}}$ is the mild solution. For this purpose, we need to show that $S_{t_1}$ is a contraction mapping from ${\mathcal {E}}_{t_1}$ to itself for some small $t_1>0$. The following proof is divided into two steps.

(i). In the first step, we show that $S_{t_1}: {\mathcal {E}}_{t_1}\rightarrow {\mathcal {E}}_{t_1}$. By Propositions 3.2 and 3.3, we have

$$\begin{aligned} \Vert S_{t_1}v(t)\Vert _{L^{\infty }({\mathbb {R}}^3)}&\le \Vert E_{\alpha }(-t^{\alpha }{\mathcal {A}})(u_0-{\bar{u}})\Vert _{L^{\infty }({\mathbb {R}}^3)} \\&\ \ \ \ +\int _0^t(t-s)^{\alpha -1}\Vert \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\left( \varphi (v(s)+{\bar{u}})-\varphi ({\bar{u}})\right) \Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le \Vert u_0-{\bar{u}}\Vert _{L^{\infty }}+Cb\int _0^t(t-s)^{\frac{\alpha }{2}-1}\Vert v(s)\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le r+\frac{4r}{\alpha }Cbt_1^{\frac{\alpha }{2}}, \quad 0<t\le t_1, \end{aligned}$$

where $b=\max _{u\in {\bar{B}}({\bar{u}},2r)}\sum _{k=1}^2|D^k\varphi (u)|$. Obviously, if $t_1\le (\frac{\alpha }{8Cb})^{\frac{2}{\alpha }}$, then

$$\begin{aligned} \Vert S_{t_1}v\Vert _{L^{\infty }({\mathbb {R}}^3\times (0,t_1))}\le 2r. \end{aligned}$$

Similarly, for $0<t\le t_1$, we have

$$\begin{aligned} \Vert S_{t_1}v(t)\Vert _{L^{1}({\mathbb {R}}^3)}&\le \Vert E_{\alpha }(-t^{\alpha }{\mathcal {A}})(u_0-{\bar{u}})\Vert _{L^{1}({\mathbb {R}}^3)} \\&\quad +\int _0^t(t-s)^{\alpha -1}\Vert \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (v+{\bar{u}})\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le \Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)}+Cb\int _0^t(t-s)^{\frac{\alpha }{2}-1}\Vert v(s)\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le \Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)}+\frac{4}{\alpha }Cbt_1^{\frac{\alpha }{2}}\Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)} \\&\le 2\Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)}. \end{aligned}$$

Now we show the continuity of $S_{t_1}v$ for $t\in [0,t_1]$. Fixing $t_0\in (0,t_1)$, we have

$$\begin{aligned} \Vert S_{t_1}v(t)-S_{t_1}v(t_0)\Vert _{L^{1}({\mathbb {R}}^3)}&\le \Vert (E_{\alpha }(-t^{\alpha }{\mathcal {A}})-E_{\alpha }(-t_0^{\alpha }{\mathcal {A}}))(u_0-{\bar{u}})\Vert _{L^{1}({\mathbb {R}}^3)} \nonumber \\&\quad +\int _{t_0}^t(t-s)^{\alpha -1}\left\| \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (v(s)+{\bar{u}})\right\| _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\quad +\int _0^{t_0}\left\| (t-s)^{\alpha -1}\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (v(s)+{\bar{u}})\right. \nonumber \\&\quad \left. -(t_0-s)^{\alpha -1}\Delta E_{\alpha , \alpha }(-(t_0-s)^{\alpha }{\mathcal {A}})\varphi (v(s)+{\bar{u}})\right\| _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&= I_1+I_2+I_3, \end{aligned}$$

(4.4)

where $t_0<t<t_1$. Using the strong continuity of $E_{\alpha }(-t^{\alpha }{\mathcal {A}})$ on $L^1({\mathbb {R}}^3)$ for $t\in [0,\infty )$, we deduce easily that the first term $I_1$ goes to zero as $t\rightarrow t_0^+$. By Proposition 3.3, the estimate of the second term yields

$$\begin{aligned} I_2&\le C\int _{t_0}^t(t-s)^{\frac{\alpha }{2}-1} \left\| \varphi (v(s)+{\bar{u}}) - \varphi ({\bar{u}})\right\| _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le Cb\int _{t_0}^t(t-s)^{\frac{\alpha }{2}-1}\Vert v(s)\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le 2Cb(t-t_0)^{\frac{\alpha }{2}}\Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)}. \end{aligned}$$

Obviously, this term vanishes as $t\rightarrow t_0^+$. For the estimate of the third term, we first denote

$$\begin{aligned} f(t,s)=&\left\| (t-s)^{\alpha -1}\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (v(s)+{\bar{u}})\right. \\&\qquad \qquad \left. -(t_0-s)^{\alpha -1}\Delta E_{\alpha , \alpha }(-(t_0-s)^{\alpha }{\mathcal {A}})\varphi (v(s)+{\bar{u}})\right\| _{L^{1}({\mathbb {R}}^3)}, \end{aligned}$$

where $0<s<t_0<t$. It is easy to see that

$$\begin{aligned} f(t,s)&\le \left| (t-s)^{\alpha -1}-(t_0-s)^{\alpha -1}\right| \cdot \big \Vert \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (v(s)+{\bar{u}}) \big \Vert _{L^{1}({\mathbb {R}}^3)} \\&\ \ \ \ +(t_0-s)^{\alpha -1}\big \Vert \Delta E_{\alpha ,\alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (v(s)+{\bar{u}}) \\&\ \ \ \ \ \ \ \ -\Delta E_{\alpha , \alpha }(-(t_0-s)^{\alpha }{\mathcal {A}})\varphi (v(s)+{\bar{u}})\big \Vert _{L^{1}({\mathbb {R}}^3)} \\&\le Cb\left| (t-s)^{\alpha -1}-(t_0-s)^{\alpha -1}\right| \cdot (t-s)^{-\frac{\alpha }{2}}\Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)} \\&\ \ \ \ +(t_0-s)^{\alpha -1}\big \Vert \Delta E_{\alpha ,\alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (v(s)+{\bar{u}}) \\&\ \ \ \ \ \ \ \ -\Delta E_{\alpha , \alpha }(-(t_0-s)^{\alpha }{\mathcal {A}})\varphi (v(s)+{\bar{u}})\big \Vert _{L^{1}({\mathbb {R}}^3)}. \end{aligned}$$

Then combining with the strong continuity of $\Delta E_{\alpha ,\alpha }(-t^{\alpha }{\mathcal {A}})$ on $L^1({\mathbb {R}}^3)$ for $t\in (0,\infty )$, we have

$$\begin{aligned} \lim _{t\rightarrow t_0^+}f(t,s)=0 \end{aligned}$$

for any fixed $s\in (0,t_0)$. In addition, for $0<s<t_0<t$, we have

$$\begin{aligned} I_3&= \int _0^{t_0} f(t,s)\,\mathrm {d}s \\&\le Cb\int _0^{t_0}(t_0-s)^{\frac{\alpha }{2}-1}\Vert v(s)\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le Cb \int _0^{t_0}(t_0-s)^{\frac{\alpha }{2}-1}\,\mathrm {d}s\Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)}, \\&\le Cbt_0^{\frac{\alpha }{2}}\Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)}. \end{aligned}$$

Applying dominated convergence theorem yields the third term $I_3$ also tends to zero as $t\rightarrow t_0^+$. Similarly, we can obtain the same limit as $t\rightarrow t_0^-$ with $t_0\in (0,t_1]$. Thus,

$$\begin{aligned} \lim _{t\rightarrow t_0}\Vert S_{t_1}v(t)-S_{t_1}v(t_0)\Vert _{L^{1}({\mathbb {R}}^3)} =0,\quad t_0\in (0,t_1]. \end{aligned}$$

Continuity up to $t=0$ of $S_{t_1}v$ follows from the following estimate and the strong continuity of $E_{\alpha }(-t^{\alpha }{\mathcal {A}})$, we have

$$\begin{aligned}&\Vert S_{t_1}v(t)-S_{t_1}v(0)\Vert _{L^{1}({\mathbb {R}}^3)} \nonumber \\&\le \Vert (E_{\alpha }(-t^{\alpha }{\mathcal {A}})-I)(u_0-{\bar{u}})\Vert _{L^{1}({\mathbb {R}}^3)} \nonumber \\&\ \ \ \ +\int _{0}^t(t-s)^{\alpha -1}\left\| \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (v(s)+{\bar{u}})\right\| _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le \Vert (E_{\alpha }(-t^{\alpha }{\mathcal {A}})-I)(u_0-{\bar{u}})\Vert _{L^{1}({\mathbb {R}}^3)} +2Cb\int _{0}^t(t-s)^{\frac{\alpha }{2}-1}\Vert v(s)\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le \Vert (E_{\alpha }(-t^{\alpha }{\mathcal {A}})-I)(u_0-{\bar{u}})\Vert _{L^{1}({\mathbb {R}}^3)} +\frac{4}{\alpha }Cbt^{\frac{\alpha }{2}}\Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)}. \end{aligned}$$

(4.5)

Then $S_{t_1}v\in C([0,t_1]; L^1({\mathbb {R}}^3))$.

(ii) In the second step, we need to show that $S_{t_1}$ is a contraction mapping on ${\mathcal {E}}_{t_1}$. Letting $v_1\in {\mathcal {E}}_{t_1}$ and $v_2\in {\mathcal {E}}_{t_1}$, we have

$$\begin{aligned}&\Vert S_{t_1}v_1(t)-S_{t_1}v_2(t)\Vert _{L^{1}({\mathbb {R}}^3)} \\&\le \int _0^t(t-s)^{\alpha -1}\Vert \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})(\varphi (v_1+{\bar{u}})-\varphi (v_2+{\bar{u}}))\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le Cb\int _0^t(t-s)^{\frac{\alpha }{2}-1}\mathrm {d}s\sup _{0\le t\le t_1}\Vert v_1(t)-v_2(t)\Vert _{L^1({\mathbb {R}}^3)} \\&\le \frac{2}{\alpha }Cb\, t_1^{\frac{\alpha }{2}}\sup _{0\le t\le t_1}\Vert v_1(t)-v_2(t)\Vert _{L^1({\mathbb {R}}^3)} \\&\le \frac{1}{4}\sup _{0\le t\le t_1}\Vert v_1(t)-v_2(t)\Vert _{L^1({\mathbb {R}}^3)}. \end{aligned}$$

That is,

$$\begin{aligned} \sup _{0\le t\le t_1}\Vert S_{t_1}v_1(t)-S_{t_1}v_2(t)\Vert _{L^{1}({\mathbb {R}}^3)}\le \frac{1}{4}\sup _{0\le t\le t_1}\Vert v_1(t)-v_2(t)\Vert _{L^1({\mathbb {R}}^3)}. \end{aligned}$$

Therefore, $S_{t_1}$ has a fixed point in ${\mathcal {E}}_{t_1}$.

In addition, subtracting ${\bar{u}}$ from both sides of (2.7), integrating the result equation with respect to $x\in {\mathbb {R}}^3$ and noting that $E_{\alpha }(-t^{\alpha }{\mathcal {A}})1=1$, we obtain

$$\begin{aligned} \int _{{\mathbb {R}}^3}(u(x,t)-{\bar{u}})\,\mathrm {d}x=\int _{{\mathbb {R}}^3}(u_0(x)-{\bar{u}})\,\mathrm {d}x, \end{aligned}$$

which completes the proof of Theorem 4.1. $\square$

Remark 4.1

It is readily seen that the proof of Theorem 4.1 also implies

$$\begin{aligned} u-{\bar{u}}\in C([0,t_1]; \mathring{L}^p({\mathbb {R}}^3)),\quad 1\le p\le \infty . \end{aligned}$$

The following key lemma plays an essential role to extend the local solutions globally.

Lemma 4.1

Suppose that the mild solution u obtained in Theorem 4.1 has been extended up to some time $T (T\ge t_1>0)$ and (4.1) are kept unchanged. In addition, assume that $\Vert u_0-{\bar{u}}\Vert _{L^1({\mathbb {R}}^3)}$ is sufficiently small and $\varphi (u)=O(1)|u-{\bar{u}}|^\sigma$ as $u\rightarrow {\bar{u}}$, where $\sigma =\frac{5}{3}$. Then there exists a positive constant C depending only on $\Vert u_0-{\bar{u}}\Vert _{L^{\infty }({\mathbb {R}}^3)}$ such that

$$\begin{aligned} \Vert u(t)-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)}+t^{\frac{\alpha }{2\sigma }}\Vert u(t)-{\bar{u}}\Vert _{L^{\sigma }({\mathbb {R}}^3)}\le C\Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)}, \quad 0\le t\le T.\nonumber \\ \end{aligned}$$

(4.6)

Proof

Let

$$\begin{aligned} X_T=\left\{ u(t,x); u-{\bar{u}}\in C([0,T]; L^1({\mathbb {R}}^3)), t^{\frac{\alpha }{2\sigma }}(u-{\bar{u}}) \in C([0,T]; L^\sigma ({\mathbb {R}}^3))\right\} \end{aligned}$$

equipped with the norm

$$\begin{aligned} \Vert v\Vert _{X_T}=\sup _{0\le t\le T}\{\Vert v(t)\Vert _{L^1({\mathbb {R}}^3)}+t^{\frac{\alpha }{2\sigma }}\Vert v(t)\Vert _{L^\sigma ({\mathbb {R}}^3)}\}. \end{aligned}$$

Upon the observation

$$\begin{aligned} u(x,t)-{\bar{u}}=E_{\alpha }(-t^{\alpha }{\mathcal {A}})(u_0-{\bar{u}})-\int _0^t(t-s)^{\alpha -1}\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\,\mathrm {d}s, \end{aligned}$$

it then follows that

$$\begin{aligned} \Vert u-{\bar{u}}\Vert _{X_T}&\le \Vert E_{\alpha }(-t^{\alpha }{\mathcal {A}})(u_0-{\bar{u}})\Vert _{X_T}\\&\quad +\left\| \int _0^t(t-s)^{\alpha -1}\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\,\mathrm {d}s\right\| _{X_T} \\&\le \Vert E_{\alpha }(-t^{\alpha }{\mathcal {A}})(u_0-{\bar{u}})\Vert _{X_T}\\&\quad +\sup _{0\le t\le T}\int _0^t(t-s)^{\alpha -1}\left\| \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\right\| _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \\&\ \ \ \ +\sup _{0\le t\le T}t^{\frac{\alpha }{2\sigma }}\int _0^t(t-s)^{\alpha -1}\left\| \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\right\| _{L^\sigma ({\mathbb {R}}^3)}\,\mathrm {d}s \\&= I_1+I_2+I_3. \end{aligned}$$

For the estimate of the first term, we have

$$\begin{aligned} I_1&= \sup _{0\le t\le T}\left\{ \Vert E_{\alpha }(-t^{\alpha }{\mathcal {A}})(u_0-{\bar{u}})\Vert _{L^1({\mathbb {R}}^3)} +t^{\frac{\alpha }{2\sigma }}\Vert E_{\alpha }(-t^{\alpha }{\mathcal {A}})(u_0-{\bar{u}})\Vert _{L^\sigma ({\mathbb {R}}^3)}\right\} \\&\le \sup _{0\le t\le T}\{\Vert u_0-{\bar{u}}\Vert _{L^1({\mathbb {R}}^3)}+Ct^{\frac{\alpha }{2\sigma }}t^{-\frac{3\alpha }{4}(1-\frac{1}{\sigma })}\Vert u_0-{\bar{u}}\Vert _{L^1({\mathbb {R}}^3)}\} \\&\le (1+C)\Vert u_0-{\bar{u}}\Vert _{L^1({\mathbb {R}}^3)}. \end{aligned}$$

In addition, by employing a similar argument, we can deduce from $\varphi (u)=O(1)|u-{\bar{u}}|^\sigma$ that

$$\begin{aligned} I_2&\le \sup _{0\le t\le T}\int _0^t(t-s)^{\alpha -1}\Vert \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C\sup _{0\le t\le T}\int _0^t(t-s)^{\frac{\alpha }{2}-1}\Vert \varphi (u(s))\Vert _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C\sup _{0\le t\le T}\int _0^t(t-s)^{\frac{\alpha }{2}-1}\Vert u(s)-{\bar{u}}\Vert ^\sigma _{L^\sigma ({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C\sup _{0\le t\le T}t^{\frac{\alpha }{2}}\Vert u(t)-{\bar{u}}\Vert ^\sigma _{L^\sigma ({\mathbb {R}}^3)} \\&\le C\Vert u-{\bar{u}}\Vert ^\sigma _{X_T}, \end{aligned}$$

and

$$\begin{aligned} I_3&\le \sup _{0\le t\le T}t^{\frac{\alpha }{2\sigma }}\int _0^t(t-s)^{\alpha -1}\Vert \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^\sigma ({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C\sup _{0\le t\le T}t^{\frac{\alpha }{2\sigma }}\int _0^t(t-s)^{\frac{\alpha }{5}-1}\Vert \varphi (u(s))\Vert _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C\sup _{0\le t\le T}t^{\frac{\alpha }{2\sigma }}\int _0^t(t-s)^{\frac{\alpha }{5}-1}\Vert u(s)-{\bar{u}}\Vert ^\sigma _{L^\sigma ({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C\sup _{0\le t\le T}t^{\frac{\alpha }{2}}\Vert u(t)-{\bar{u}}\Vert ^\sigma _{L^\sigma ({\mathbb {R}}^3)} \\&\le C\Vert u-{\bar{u}}\Vert ^\sigma _{X_T}. \end{aligned}$$

Summing up, we immediately conclude

$$\begin{aligned} \Vert u-{\bar{u}}\Vert _{X_T}\le (1+C)\Vert u_0-{\bar{u}}\Vert _{L^1({\mathbb {R}}^3)}+C\Vert u-{\bar{u}}\Vert ^\sigma _{X_T}, \end{aligned}$$

where C is independent of T. Thus, if $\Vert u-{\bar{u}}\Vert _{L^1({\mathbb {R}}^3)}$ is sufficiently small, by Strauss’s inequality (cf. [23, 34]), we can obtain (4.6) immediately. The proof of Lemma 4.1 is completed. $\square$

It is worth mentioning that (4.6) holds in [0, T] even if $T=+\infty$, since the constant C in the estimate (4.1) is independent of T.

Now we are going to extend the local mild solution u globally. In comparison with that of classical integer-order Cahn–Hilliard equation in [23, Theorem 1.1], the proof given here is more concise since it doesn’t rely on the estimates of high order derivatives of u.

Proof of Theorem 2.1

Let u defined on $[0,t_1]$ be the local mild solution obtained in the previous sections. We first extend u from $[0,t_1]$ to $[0,t_1+t_2]$ for some $t_2\in (0,t_1)$. Define the complete metric space

$$\begin{aligned} {\mathcal {E}}_{t_1+t_2}= & {} \Big \{v\in C([t_1,t_1+t_2]; L^1({\mathbb {R}}^3))\cap L^{\infty }({\mathbb {R}}^3\times [t_1,t_1+t_2]);\\&v(x,t_1)=u(x,t_1)-{\bar{u}},\\&\sup _{t_1\le t\le t_1+t_2}\Vert v(t)\Vert _{L^1({\mathbb {R}}^3)}\le 2\Vert u_0-{\bar{u}}\Vert _{L^1({\mathbb {R}}^3)} \ \mathrm{and}\ \Vert v(t)\Vert _{L^{\infty }({\mathbb {R}}^3\times [t_1,t_1+t_2])}\\&\le 2\Vert u_0-{\bar{u}}\Vert _{L^\infty ({\mathbb {R}}^3)}\Big \} \end{aligned}$$

and the operator

$$\begin{aligned} S_{t_1+t_2}v(x,t)&= E_{\alpha }(-t^{\alpha }{\mathcal {A}})(u_0-{\bar{u}}) -\int _{0}^{t_1}(t-s)^{\alpha -1}\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha } {\mathcal {A}})\varphi (u)\,\mathrm {d}s\\&\ \ \ \ \ -\int _{t_1}^t(t-s)^{\alpha -1}\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (v+{\bar{u}})\,\mathrm {d}s \quad \text {for}\ \ t\ge t_1. \end{aligned}$$

By Proposition 3.2, 3.3, Lemma 4.1 and recalling that $\varphi (u)=O(1)|u-{\bar{u}}|^\sigma$ as $u\rightarrow {\bar{u}}$, we obtain

$$\begin{aligned}&\Vert S_{t_1+t_2}v(t)\Vert _{L^{\infty }({\mathbb {R}}^3)} \\&\le \Vert u_0-{\bar{u}}\Vert _{L^\infty ({\mathbb {R}}^3)}+\int _{0}^{t_1-\frac{t_2}{2}}(t-s)^{\alpha -1}\Vert \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \\&\ \ \ +\int _{t_1-\frac{t_2}{2}}^{t_1}(t-s)^{\alpha -1}\Vert \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \\&\ \ \ +\int _{t_1}^t(t-s)^{\alpha -1}\Vert \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (v+{\bar{u}})\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le \Vert u_0-{\bar{u}}\Vert _{L^\infty ({\mathbb {R}}^3)}+C\int _{0}^{t_1-\frac{t_2}{2}} (t-s)^{-\frac{\alpha }{4}-1}\Vert \varphi (u)\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\ \ \ +C\int _{t_1-\frac{t_2}{2}}^{t_1}(t-s)^{\frac{\alpha }{2}-1}\Vert \varphi (u)\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s +C\int _{t_1}^t(t-s)^{\frac{\alpha }{2}-1}\Vert \varphi (v+{\bar{u}})\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le \Vert u_0-{\bar{u}}\Vert _{L^\infty ({\mathbb {R}}^3)}+C\Big (t-\big (t_1-\frac{t_2}{2}\big )\Big )^{-\frac{3\alpha }{4}} \int _{0}^{t_1-\frac{t_2}{2}}(t-s)^{\frac{\alpha }{2}-1}s^{-\frac{\alpha }{2}}\left( s^{\frac{\alpha }{2}} \Vert u-{\bar{u}}\Vert ^\sigma _{L^{\sigma }({\mathbb {R}}^3)}\right) \mathrm {d}s \\&\ \ \ +C\int _{t_1-\frac{t_2}{2}}^{t_1}(t-s)^{\frac{\alpha }{2}-1}\Vert u-{\bar{u}}\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s +C\int _{t_1}^t(t-s)^{\frac{\alpha }{2}-1}\Vert v\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le \Vert u_0-{\bar{u}}\Vert _{L^\infty ({\mathbb {R}}^3)}+C\Big (t-\big (t_1-\frac{t_2}{2}\big ) \Big )^{-\frac{3\alpha }{4}}\Vert u_0-{\bar{u}}\Vert ^{\sigma }_{L^1({\mathbb {R}}^3)} +C(t-t_1)^{\frac{\alpha }{2}}\Vert u_0-{\bar{u}}\Vert _{L^\infty ({\mathbb {R}}^3)} \end{aligned}$$

for $v\in {\mathcal {E}}_{t_1+t_2}$ and $t\in [t_1, t_1+t_2]$, where C is independent of t and $t_1$. Choose small $t_2$ such that

$$\begin{aligned} C(t-t_1)^{\frac{\alpha }{2}}\le Ct_2^{\frac{\alpha }{2}}<\frac{1}{2},\quad t\in [t_1, t_1+t_2]. \end{aligned}$$

Then for sufficiently small $\Vert u_0-{\bar{u}}\Vert _{L^1({\mathbb {R}}^3)}$, we can obtain that

$$\begin{aligned} \Vert S_{t_1+t_2}v(t)\Vert _{L^{\infty }({\mathbb {R}}^3)}\le 2\Vert u_0-{\bar{u}}\Vert _{L^\infty ({\mathbb {R}}^3)}, \quad t\in [t_1, t_1+t_2]. \end{aligned}$$

Similarly, for $t\in [t_1, t_1+t_2]$, we have

$$\begin{aligned} \Vert S_{t_1+t_2}v(t)\Vert _{L^{1}({\mathbb {R}}^3)}&\le \Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)}\\&\quad +\int _{0}^{t_1}(t-s)^{\alpha -1}\Vert \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u(s))\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\ \ \ \ +\int _{t_1}^t(t-s)^{\alpha -1}\Vert \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (v+{\bar{u}})(s)\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le \Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)}+C\int _{0}^{t_1}(t-s)^{\frac{\alpha }{2}-1}\Vert \varphi (u(s))\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\ \ \ \ +C\int _{t_1}^t(t-s)^{\frac{\alpha }{2}-1}\Vert \varphi (v+{\bar{u}})\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le \Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)}+C\int _{0}^{t_1}(t_1-s)^{\frac{\alpha }{2}-1}s^{-\frac{\alpha }{2}} \left( s^{\frac{\alpha }{2}}\Vert u(s)-{\bar{u}}\Vert ^\sigma _{L^{\sigma }({\mathbb {R}}^3)}\right) \,\mathrm {d}s \\&\ \ \ \ +C\int _{t_1}^t(t-s)^{\frac{\alpha }{2}-1}\Vert v(s)\Vert ^\sigma _{L^{\sigma }({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le \Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)}+C\Vert u_0-{\bar{u}}\Vert ^\sigma _{L^{1}({\mathbb {R}}^3)} +C(t-t_1)^{\frac{\alpha }{2}}\Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)}, \\&\le 2\Vert u_0-{\bar{u}}\Vert _{L^{1}({\mathbb {R}}^3)} \end{aligned}$$

with sufficiently small $\Vert u_0-{\bar{u}}\Vert _{L^1({\mathbb {R}}^3)}$ and small $t_2$. The continuity of $\Vert S_{t_1+t_2}v(t)\Vert _{L^1({\mathbb {R}}^3)}$ for $t\in [t_1,t_1+t_2]$ follows the proof Theorem 4.1. Therefore, we obtain $S_{t_1+t_2}v\in {\mathcal {E}}_{t_1+t_2}$.

On the other hand, for $v_1, v_2\in {\mathcal {E}}_{t_1+t_2}$ and $t_1\le t\le t_1+t_2$, we have

$$\begin{aligned}&\Vert S_{t_1+t_2}v_1(t)-S_{t_1+t_2}v_2(t)\Vert _{L^{1}({\mathbb {R}}^3)} \\&\le \int _{t_1}^t(t-s)^{\alpha -1}\Vert \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})(\varphi (v_1+{\bar{u}})-\varphi (v_2+{\bar{u}}))\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C\int _{t_1}^t(t-s)^{\frac{\alpha }{2}-1}\mathrm {d}s\sup _{t_1\le t\le t_1+t_2}\Vert v_1(t)-v_2(t)\Vert _{L^1({\mathbb {R}}^3)} \\&\le C(t-t_1)^{\frac{\alpha }{2}}\sup _{t_1\le t\le t_1+t_2}\Vert v_1(t)-v_2(t)\Vert _{L^1({\mathbb {R}}^3)} \\&\le \frac{1}{2}\sup _{t_1\le t\le t_1+t_2}\Vert v_1(t)-v_2(t)\Vert _{L^1({\mathbb {R}}^3)}. \end{aligned}$$

That is,

$$\begin{aligned} \sup _{t_1\le t\le t_1+t_2}\Vert S_{t_1+t_2}v_1(t)-S_{t_1+t_2}v_2(t)\Vert _{L^{1}({\mathbb {R}}^3)}\le \frac{1}{2} \sup _{t_1\le t\le t_1+t_2}\Vert v_1(t)-v_2(t)\Vert _{L^1({\mathbb {R}}^3)}. \end{aligned}$$

According to Banach contracting mapping principle, there exists a unique solution in ${\mathcal {E}}_{t_1+t_2}$, which is also denoted by u(x, t). Therefore, the solution u(x, t) can be extended up to time $t_1+t_2$ and

$$\begin{aligned} \Vert u(t)-{\bar{u}}\Vert _{L^{\infty }({\mathbb {R}}^3)}\le 2\Vert u_0-{\bar{u}}\Vert _{L^\infty ({\mathbb {R}}^3)}, \quad 0\le t\le t_1+t_2. \end{aligned}$$

Repeating above procedure, we can extend the solution u(x, t) to time $t_1+mt_2$ for any $m\in {\mathbb {Z}}_+$ such that

$$\begin{aligned} \Vert u(t)-{\bar{u}}\Vert _{L^{\infty }({\mathbb {R}}^3)}\le 2\Vert u_0-{\bar{u}}\Vert _{L^\infty ({\mathbb {R}}^3)}, \quad 0\le t\le t_1+mt_2. \end{aligned}$$

We thus establish the existence of the solution u in all $t\ge 0$. The integral preservation property of u follows directly from Theorem 4.1. The proof of Theorem 2.1 is completed. $\square$

5 Strong solution

By Remark 3.1, the mild solution u obtained in Theorem 2.1 may not be a smooth solution. However, we will prove that u is in fact a strong solution of the problem (1.1) in this section. For this purpose, we first give some a priori estimates to show $u-{\bar{u}}\in C((0,\infty ); W^{4,1}({\mathbb {R}}^3))$. For simplicity, we assume ${\bar{u}}=0$ and take $r=\Vert u_0\Vert _{L^\infty ({\mathbb {R}}^3)}$ in the rest of this paper.

It is worth mentioning that the equation in (1.1) is non-Markovian and $E_{\alpha }(-t^{\alpha }{\mathcal {A}})$ and $E_{\alpha , \alpha }(-t^{\alpha }{\mathcal {A}})$ with $0<\alpha <1$ are not semigroup operators, which means that for $t>{\bar{t}}>0$,

$$\begin{aligned} u(x,t)&= E_{\alpha }(-t^{\alpha }{\mathcal {A}})u_0-\int _0^t(t-s)^{\alpha -1}\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\,\mathrm {d}s \\&\ne E_{\alpha }(-(t-{\bar{t}})^{\alpha }{\mathcal {A}})u({\bar{t}})-\int _{{\bar{t}}}^t(t-s)^{\alpha -1}\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\,\mathrm {d}s. \end{aligned}$$

Thus the way to obtaining the regularity of solution as given in [23] for the traditional Cahn–Hilliard equation is no longer valid. However, by dividing the integro-differential equation (2.7) into the following three terms,

$$\begin{aligned} u(x,t)&= E_{\alpha }(-t^{\alpha }{\mathcal {A}})u_0 -\int _{{\bar{t}}}^t(t-s)^{\alpha -1}\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\,\mathrm {d}s \nonumber \\&\ \ \ \ -\int _{0}^{{\bar{t}}}(t-s)^{\alpha -1}\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\,\mathrm {d}s, \quad t\ge {\bar{t}}>0. \end{aligned}$$

(5.1)

we see that the first two terms on the right hand side of (5.1) can also be estimated similarly. So it suffices to find how to deal with the third term on the right hand side of (5.1).

Lemma 5.1

Let the assumptions of Lemma 4.1 be satisfied. Then for any $0<s_1<s_2<t$, we have

$$\begin{aligned} \Vert D u(t)\Vert _{L^{\infty }({\mathbb {R}}^3)}\le (t-s_1)^{-\frac{\alpha }{4}}C_1(r,s_2-s_1,t-s_1), \end{aligned}$$

(5.2)

where $C_1$ is a continuous increasing function of $t-s_1$.

Proof

Applying the derivative operator D to (5.1) and taking ${\bar{t}}=s_1$, we get

$$\begin{aligned} \Vert D u(t)\Vert _{L^{\infty }} \le \ \&\Vert DE_{\alpha }(-t^{\alpha }{\mathcal {A}})u_0\Vert _{L^{\infty }({\mathbb {R}}^3)} \\&+\int _{s_1}^t(t-s)^{\alpha -1}\Vert D\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \\&+\int _0^{s_1}(t-s)^{\alpha -1}\Vert D\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \\ = \ \&I+II. \end{aligned}$$

By Propositions 3.2, 3.3 and Theorem 4.1, we have

$$\begin{aligned} I&= \Vert DE_{\alpha }(-t^{\alpha }{\mathcal {A}})u_0\Vert _{L^{\infty }({\mathbb {R}}^3)}+\int _{s_1}^t(t-s)^{\alpha -1}\Vert D\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le C(r)(t-s_1)^{-\frac{\alpha }{4}}+C\int _{s_1}^t(t-s)^{\frac{\alpha }{40}-1} \Vert \varphi (u)\Vert _{L^{2\sigma }({\mathbb {R}}^3)}\mathrm {d}s \nonumber \\&\le C(r)(t-s_1)^{-\frac{\alpha }{4}}+C(r)\int _{s_1}^t(t-s)^{\frac{\alpha }{40}-1} \Vert u\Vert ^{\frac{1}{2}}_{L^{\sigma }({\mathbb {R}}^3)}\mathrm {d}s \nonumber \\&\le C(r)(t-s_1)^{-\frac{\alpha }{4}}+C(r)\int _{s_1}^t(t-s)^{\frac{\alpha }{40}-1} s^{-\frac{\alpha }{4\sigma }}\left( s^{\frac{\alpha }{2\sigma }}\Vert u\Vert _{L^{\sigma }({\mathbb {R}}^3)}\right) ^{\frac{1}{2}}\mathrm {d}s \nonumber \\&\le C(r)(t-s_1)^{-\frac{\alpha }{4}}+C(r)(t-s_1)^{-\frac{\alpha }{8}}. \end{aligned}$$

(5.3)

Similarly, we could deduce that

$$\begin{aligned} II&= \int _{0}^{s_1}(t-s)^{\alpha -1}\Vert D \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le C\int _{0}^{s_1}(t-s)^{-\frac{\alpha }{2}-1}\Vert \varphi (u)\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le C\int _{0}^{s_1}(t-s)^{-\frac{\alpha }{2}-1}s^{-\frac{\alpha }{2}} \left( s^{\frac{\alpha }{2}}\Vert u\Vert ^\sigma _{L^{\sigma }({\mathbb {R}}^3)}\right) \,\mathrm {d}s \nonumber \\&\le C(t-s_1)^{-\alpha }\int _{0}^{s_1}(s_1-s)^{\frac{\alpha }{2}-1}s^{-\frac{\alpha }{2}}\,\mathrm {d}s \nonumber \\&\le C(t-s_1)^{-\frac{\alpha }{4}}(s_2-s_1)^{-\frac{3\alpha }{4}}. \end{aligned}$$

(5.4)

Combining (5.3) with (5.4), we obtain

$$\begin{aligned} \Vert D u(t)\Vert _{L^{\infty }({\mathbb {R}}^3)}\le (t-s_1)^{-\frac{\alpha }{4}}C_1(r, s_2-s_1, t-s_1), \end{aligned}$$

which completes the proof of Lemma 5.1. $\square$

Next, we give the estimates of $\Vert D^k u(t)\Vert _{L^1({\mathbb {R}}^3)}$ with $k=1,2,3$.

Lemma 5.2

Let the assumptions of Lemma 4.1 be satisfied. Then

$$\begin{aligned} \Vert D u(t)\Vert _{L^{1}({\mathbb {R}}^3)}\le M_1(r)t^{-\frac{\alpha }{4}}\Vert u_0\Vert _{L^{1}({\mathbb {R}}^3)}, \quad t>0, \end{aligned}$$

(5.5)

where $M_1(r)$ is a positive constant depending on r.

Proof

According to (2.7) and (4.6), we have

$$\begin{aligned} t^{\frac{\alpha }{4}}\Vert D u(t)\Vert _{L^{1}({\mathbb {R}}^3)} \le \ \&t^{\frac{\alpha }{4}}\Vert DE_{\alpha }(-t^{\alpha }{\mathcal {A}})(u_0)\Vert _{L^1({\mathbb {R}}^3)} \\&+t^{\frac{\alpha }{4}}\int _0^t(t-s)^{\alpha -1}\Vert D\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \\ \le \ \&\Vert u_0\Vert _{L^1({\mathbb {R}}^3)}+Ct^{\frac{\alpha }{4}}\int _0^t(t-s)^{\frac{\alpha }{4}-1}\Vert \varphi (u(s))\Vert _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \\ \le \ \&\Vert u_0\Vert _{L^1({\mathbb {R}}^3)}+Ct^{\frac{\alpha }{4}}\int _0^t(t-s)^{\frac{\alpha }{4}-1}\Vert u(s)\Vert ^\sigma _{L^\sigma ({\mathbb {R}}^3)}\,\mathrm {d}s \\ \le \ \&\Vert u_0\Vert _{L^1({\mathbb {R}}^3)}+Ct^{\frac{\alpha }{4}}\int _0^t(t-s)^{\frac{\alpha }{4}-1}s^{-\frac{\alpha }{2}} (s^{\frac{\alpha }{2\sigma }}\Vert u(s)\Vert _{L^\sigma ({\mathbb {R}}^3)})^\sigma \,\mathrm {d}s \\ \le \ \&\Vert u_0\Vert _{L^1({\mathbb {R}}^3)}+Cc^\sigma _1(r)\Vert u_0\Vert ^\sigma _{L^1({\mathbb {R}}^3)}. \end{aligned}$$

The proof is completed. $\square$

Lemma 5.3

Let the assumptions of Lemma 4.1 be satisfied. Then for any $0<s_1<s_2<{\bar{s}}_2<{\bar{s}}_3<t$, we have

$$\begin{aligned} \Vert D^2 u(t)\Vert _{L^{1}({\mathbb {R}}^3)}\le (t-{\bar{s}}_2)^{-\frac{\alpha }{2}}M_2(r, t-{\bar{s}}_2)\Vert u_0\Vert _{L^{1}({\mathbb {R}}^3)} \end{aligned}$$

(5.6)

and

$$\begin{aligned} \Vert D^3 u(t)\Vert _{L^{1}({\mathbb {R}}^3)}\le (t-{\bar{s}}_3)^{-\frac{3\alpha }{4}}M_3(r, s_2-s_1,{\bar{s}}_3-{\bar{s}}_2, t-{\bar{s}}_3)\Vert u_0\Vert _{L^{1}({\mathbb {R}}^3)}, \end{aligned}$$

(5.7)

where $M_k$ denote continuous increasing functions of $t-{\bar{s}}_k$, $k=2,3$.

Proof

We first prove (5.6). For $t>{\bar{s}}_2$, we have

$$\begin{aligned} \Vert D^2 u(t)\Vert _{L^{1}({\mathbb {R}}^3)} \le \&\Vert D^2E_{\alpha }(-t^{\alpha }{\mathcal {A}})u_0\Vert _{L^{1}({\mathbb {R}}^3)} \\&+\int _{{\bar{s}}_2}^t(t-s)^{\alpha -1}\Vert D\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})D\varphi (u)\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \\&+\int _0^{{\bar{s}}_2}(t-s)^{\alpha -1}\Vert D^2\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \\ = \&I+II. \end{aligned}$$

By Propositions 3.2, 3.3 and Lemma 5.2, we obtain

$$\begin{aligned} I&\le C (t-{\bar{s}}_2)^{-\frac{\alpha }{2}}\Vert u_0\Vert _{L^1({\mathbb {R}}^3)} + C(r) \int _{{\bar{s}}_2}^t (t-s)^{\frac{\alpha }{4}-1}\Vert Du\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le C (t-{\bar{s}}_2)^{-\frac{\alpha }{2}}\Vert u_0\Vert _{L^1({\mathbb {R}}^3)}+C(r) \int _{{\bar{s}}_2}^t(t-s)^{\frac{\alpha }{4}-1} s^{-\frac{\alpha }{4}}\left( s^{\frac{\alpha }{4}}\Vert Du\Vert _{L^{1}({\mathbb {R}}^3)}\right) \,\mathrm {d}s \nonumber \\&\le C (t-{\bar{s}}_2)^{-\frac{\alpha }{2}} \Vert u_0\Vert _{L^1({\mathbb {R}}^3)}+C(r)\Vert u_0\Vert _{L^1({\mathbb {R}}^3)}. \end{aligned}$$

(5.8)

By virtue of $\varphi (u)=O(1)|u|^\sigma$, we have

$$\begin{aligned} II&= \int _{0}^{{\bar{s}}_2}(t-s)^{\alpha -1}\Vert D^2 \Delta E_{\alpha , \alpha } (-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le C\int _{0}^{{\bar{s}}_2}(t-s)^{-1}\Vert \varphi (u)\Vert _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le C\int _{0}^{{\bar{s}}_2}({\bar{s}}_2-s)^{\frac{\alpha }{2}-1} (t-{\bar{s}}_2)^{-\frac{\alpha }{2}}\Vert u\Vert ^\sigma _{L^\sigma ({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le C(t-{\bar{s}}_2)^{-\frac{\alpha }{2}}\int _{0}^{{\bar{s}}_2}({\bar{s}}_2-s)^{\frac{\alpha }{2}-1}s^{-\frac{\alpha }{2}} \left( s^{\frac{\alpha }{2}}\Vert u\Vert ^\sigma _{L^\sigma ({\mathbb {R}}^3)}\right) \mathrm {d}s \nonumber \\&\le (t-{\bar{s}}_2)^{-\frac{\alpha }{2}}C(r)\Vert u_0\Vert _{L^{1}({\mathbb {R}}^3)}. \end{aligned}$$

(5.9)

Combining (5.8) with (5.9), we immediately conclude the estimate (5.6).

Next, we prove (5.7). Similarly, for $t>{\bar{s}}_3$, we have

$$\begin{aligned} \Vert D^3 u(t)\Vert _{L^1}\le \,\, \&\Vert D^3E_{\alpha }(-t^{\alpha }{\mathcal {A}})u_0\Vert _{L^1({\mathbb {R}}^3)} \\&\quad +\int _{{\bar{s}}_3}^t(t-s)^{\alpha -1}\Vert D\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})D^2\varphi (u)\Vert _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \\&\quad +\int _0^{{\bar{s}}_3}(t-s)^{\alpha -1}\Vert D^3\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \\&= I'+II'. \end{aligned}$$

By Propositions 3.2 and 3.3, we have

$$\begin{aligned} I'&= \Vert D^3E_{\alpha }(-t^{\alpha }{\mathcal {A}})u_0\Vert _{L^1({\mathbb {R}}^3)}\nonumber \\ {}&+\int _{{\bar{s}}_3}^t(t-s)^{\alpha -1}\Vert D\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})D^2\varphi (u)\Vert _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\quad \le C(t-{\bar{s}}_3)^{-\frac{3\alpha }{4}} \Vert u_0\Vert _{L^1({\mathbb {R}}^3)}+C\int _{{\bar{s}}_3}^t(t-s)^{\frac{\alpha }{4}-1} \Vert D^2 \varphi (u)\Vert _{L^{1}({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le C (t-{\bar{s}}_3)^{-\frac{3\alpha }{4}}\Vert u_0\Vert _{L^1({\mathbb {R}}^3)}+C\int _{{\bar{s}}_3}^t(t-s)^{\frac{\alpha }{4}-1} \left( \Vert D u\Vert ^2_{L^{2}}+\Vert D^2 u\Vert _{L^{1}({\mathbb {R}}^3)}\right) \mathrm {d}s \nonumber \\&\le C(t-{\bar{s}}_3)^{-\frac{3\alpha }{4}}\Vert u_0\Vert _{L^1({\mathbb {R}}^3)}\nonumber \\&\quad +C\int _{{\bar{s}}_3}^t(t-s)^{\frac{\alpha }{4}-1} \left( \Vert D u\Vert _{L^{\infty }({\mathbb {R}}^3)}\Vert D u\Vert _{L^{1}({\mathbb {R}}^3)}+\Vert D^2 u\Vert _{L^{1}({\mathbb {R}}^3)}\right) \mathrm {d}s. \end{aligned}$$

(5.10)

It should be notice that by virtue of (5.3) and (5.4) in the proof of Lemma 5.1, the function in the right hand side of (5.2) is the continuous decreasing function with respect to $t\in (s_1, \infty )$. So, for $0<s_1<s_2<{\bar{s}}_2<{\bar{s}}_3<s<t$, we have

$$\begin{aligned} \Vert D u(s)\Vert _{L^{\infty }}&\le (s-s_1)^{-\frac{\alpha }{4}}C_1(r,s_2-s_1,s-s_1) \nonumber \\&\le (s_2-s_1)^{-\frac{\alpha }{4}}C_1(r,s_2-s_1,s_2-s_1) \nonumber \\&= C(r,s_2-s_1). \end{aligned}$$

(5.11)

Then combining with Lemma 5.2, we have

$$\begin{aligned}&\int _{{\bar{s}}_3}^t(t-s)^{\frac{\alpha }{4}-1}\Vert D u\Vert _{L^{\infty }({\mathbb {R}}^3)} \Vert D u\Vert _{L^{1}({\mathbb {R}}^3)}\mathrm {d}s \nonumber \\&\quad \le \, \, C(r,s_2-s_1)\Vert u_0\Vert _{L^{1}({\mathbb {R}}^3)} \int _{{\bar{s}}_3}^t(t-s)^{\frac{\alpha }{4}-1}s^{-\frac{\alpha }{4}}\mathrm {d}s \nonumber \\&\quad \le \, \, C(r,s_2-s_1)\Vert u_0\Vert _{L^{1}({\mathbb {R}}^3)}. \end{aligned}$$

(5.12)

Similarly, by virtue of (5.6), for $0<{\bar{s}}_2<{\bar{s}}_3<s<t$, we have

$$\begin{aligned} \Vert D^2 u(s)\Vert _{L^1({\mathbb {R}}^3)}&\le ({\bar{s}}_3-{\bar{s}}_2)^{-\frac{\alpha }{2}}M_2(r, {\bar{s}}_3-{\bar{s}}_2)\Vert u_0\Vert _{L^{1}({\mathbb {R}}^3)}, \end{aligned}$$

and then

$$\begin{aligned}&\int _{{\bar{s}}_3}^t(t-s)^{\frac{\alpha }{4}-1}\Vert D^2 u\Vert _{L^{1}({\mathbb {R}}^3)}\mathrm {d}s \nonumber \\&\quad \le \ \, ({\bar{s}}_3-{\bar{s}}_2)^{-\frac{\alpha }{2}}M_2(r, {\bar{s}}_3-{\bar{s}}_2) \Vert u_0\Vert _{L^{1}({\mathbb {R}}^3)}\int _{{\bar{s}}_3}^t(t-s)^{\frac{\alpha }{4}-1}\mathrm {d}s \nonumber \\&\quad \le \ \, ({\bar{s}}_3-{\bar{s}}_2)^{-\frac{\alpha }{2}}M_2(r, {\bar{s}}_3-{\bar{s}}_2) \Vert u_0\Vert _{L^{1}({\mathbb {R}}^3)}\int _{{\bar{s}}_3}^t(t-s)^{\frac{\alpha }{4}-1}\mathrm {d}s \nonumber \\&\quad \le \, \ (t-{\bar{s}}_3)^{-\frac{3\alpha }{4}}M_3(r, {\bar{s}}_3-{\bar{s}}_2, t-{\bar{s}}_3)\Vert u_0\Vert _{L^{1}({\mathbb {R}}^3)}. \end{aligned}$$

(5.13)

Inserting (5.12) and (5.13) into (5.10), we obtain

$$\begin{aligned} I'\le (t-{\bar{s}}_3)^{-\frac{3\alpha }{4}}M_3(r, s_2-s_1,{\bar{s}}_3-{\bar{s}}_2, t-{\bar{s}}_3)\Vert u_0\Vert _{L^{1}}. \end{aligned}$$

By the similar method, we have

$$\begin{aligned} II'&= \int _{0}^{{\bar{s}}_3}(t-s)^{\alpha -1}\Vert D^3 \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C\int _{0}^{{\bar{s}}_3}(t-s)^{-\frac{\alpha }{4}-1}\Vert \varphi (u)\Vert _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C\int _{0}^{{\bar{s}}_3}(t-s)^{-\frac{3\alpha }{4}}(t-s)^{\frac{\alpha }{2}-1}\Vert u\Vert ^\sigma _{L^\sigma ({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C(t-{\bar{s}}_3)^{-\frac{3\alpha }{4}}\int _{0}^{{\bar{s}}_3}({\bar{s}}_3-s)^{\frac{\alpha }{2}-1}s^{-\frac{\alpha }{2}} \left( s^{\frac{\alpha }{2}}\Vert u\Vert ^\sigma _{L^\sigma ({\mathbb {R}}^3)}\right) \mathrm {d}s \\&\le (t-{\bar{s}}_3)^{-\frac{3\alpha }{4}}C(r)\Vert u_0\Vert _{L^{1}({\mathbb {R}}^3)}. \end{aligned}$$

Summing up, we immediately conclude the estimate (5.7). $\square$

Lemma 5.4

Let the assumptions of Lemma 4.1 be satisfied. Then we have

$$\begin{aligned} \Vert D^k u(t)\Vert _{L^{\infty }({\mathbb {R}}^3)}\le (t-s_k)^{-\frac{\alpha k}{4}}C_k(r, s_2-s_1, \ldots , s_{k+1}-s_k, t-s_k) \end{aligned}$$

(5.14)

for any $0<s_1<s_2<\cdots<s_k<s_{k+1}<t$, where $C_k$ are the continuous increasing functions of $t-s_k$, $k=2,3$.

Proof

We first prove (5.14) for $k=2$. Letting $t>s_3$, we have

$$\begin{aligned} \Vert D^2 u(t)\Vert _{L^{\infty }({\mathbb {R}}^3)} \le \ \&\Vert D^2E_{\alpha }(-t^{\alpha }{\mathcal {A}})u_0\Vert _{L^{\infty }({\mathbb {R}}^3)} \\&+\int _{s_2}^t(t-s)^{\alpha -1}\Vert D\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})D\varphi (u)\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \\&+\int _0^{s_2}(t-s)^{\alpha -1}\Vert D^2\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \\ = \ \&I+II. \end{aligned}$$

By Proposition 3.2 and 3.3, we obtain

$$\begin{aligned} I \le (t-s_2)^{-\frac{\alpha }{2}}Cr+C\int _{s_2}^t (t-s)^{\frac{\alpha }{40}-1}\Vert D\varphi (u)\Vert _{L^{{2\sigma }}({\mathbb {R}}^3)}\,\mathrm {d}s. \end{aligned}$$

(5.15)

Combining (5.5) with (5.11), we have

$$\begin{aligned} \Vert D\varphi (u(s))\Vert _{L^{{2\sigma }}({\mathbb {R}}^3)}&\le \Vert Du(s)\Vert ^{\frac{1}{2\sigma }}_{L^{1}({\mathbb {R}}^3)}\,\Vert Du(s)\Vert ^{1-\frac{1}{2\sigma }}_{L^{{\infty }}({\mathbb {R}}^3)} \\&\le C(r,s_2-s_1) s^{-\frac{3\alpha }{40}}, \quad s_2<s<t. \end{aligned}$$

Inserting this inequality into (5.15), we get

$$\begin{aligned} I&\le (t-s_2)^{-\frac{\alpha }{2}}Cr+C\int _{s_2}^t (t-s)^{\frac{\alpha }{40}-1}s^{-\frac{3\alpha }{40}}C(r,s_2-s_1)\,\mathrm {d}s \nonumber \\&\le (t-s_2)^{-\frac{\alpha }{2}}Cr+(t-s_2)^{-\frac{\alpha }{20}}C(r,s_2-s_1). \end{aligned}$$

(5.16)

In addition, we have

$$\begin{aligned} II&= \int _{0}^{s_2}(t-s)^{\alpha -1}\Vert D^2 \Delta E_{\alpha , \alpha } (-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le C\int _{0}^{s_2}(t-s)^{-\frac{3\alpha }{4}-1}\Vert \varphi (u)\Vert _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le C\int _{0}^{s_2}(t-s)^{-\frac{3\alpha }{4}-1}s^{-\frac{\alpha }{2}}\,\mathrm {d}s \sup _{t}\left( t^{\frac{\alpha }{2}}\Vert u\Vert ^\sigma _{L^\sigma ({\mathbb {R}}^3)}\right) \nonumber \\&\le (t-s_2)^{-\frac{\alpha }{2}-\frac{3\alpha }{4}}\int _{0}^{s_2}(s_2-s)^{\frac{\alpha }{2}-1} s^{-\frac{\alpha }{2}}\,\mathrm {d}s\,C(r)\Vert u_0\Vert _{L^{1}({\mathbb {R}}^3)} \nonumber \\&\le (t-s_2)^{-\frac{\alpha }{2}}(s_3-s_2)^{-\frac{3\alpha }{4}}C(r)\Vert u_0\Vert _{L^{1}({\mathbb {R}}^3)} \nonumber \\&\le (t-s_2)^{-\frac{\alpha }{2}}\,C_2(r, s_3-s_2). \end{aligned}$$

(5.17)

Here we used the fact that $s_2<s_3<t$. Combining (5.16) with (5.17), we immediately conclude the estimate (5.14) for $k=2$. It is also worth mentioning that the term on the right hand side of (5.14) for $k=2$ is a continuous decreasing function of $t-s_2$, even though $C_2(r, s_2-s_1, s_3-s_2, t-s_2)$ is continuous increasing with respect to $t-s_2$.

Next, we prove (5.14) for $k=3$. Similarly, for $t>s_4$, we have

$$\begin{aligned} \Vert D^3 u(t)\Vert _{L^{\infty }({\mathbb {R}}^3)} \le \ \&\Vert D^3E_{\alpha }(-t^{\alpha }A)u_0\Vert _{L^{\infty }({\mathbb {R}}^3)} \\&+\int _{s_3}^t(t-s)^{\alpha -1}\Vert D\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }A)D^2\varphi (u)\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \\&+\int _0^{s_3}(t-s)^{\alpha -1}\Vert D^3\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }A)\varphi (u)\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \\ = \ \&I'+II'. \end{aligned}$$

By Proposition 3.2 and 3.3, we get

$$\begin{aligned} I'&\le (t-s_3)^{-\frac{3\alpha }{4}}Cr+C\int _{s_3}^t(t-s)^{\frac{\alpha }{4}-1} \Vert D^2 \varphi (u)\Vert _{L^{{\infty }}({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le (t-s_3)^{-\frac{3\alpha }{4}}Cr+C\int _{s_3}^t(t-s)^{\frac{\alpha }{4}-1} \left( \Vert D u\Vert ^2_{L^{\infty }({\mathbb {R}}^3)}+\Vert D^2 u\Vert _{L^{\infty }({\mathbb {R}}^3)}\right) \mathrm {d}s. \end{aligned}$$

(5.18)

For $0<s_1<s_2<s_3<s<t$, we have

$$\begin{aligned} \Vert D u(s)\Vert _{L^{\infty }}&\le (s-s_1)^{-\frac{\alpha }{4}}C_1(r,s_2-s_1,s-s_1) \\&\le (s_2-s_1)^{-\frac{\alpha }{4}}C_1(r,s_2-s_1,s_2-s_1) \\&= C(r,s_2-s_1) \end{aligned}$$

and

$$\begin{aligned} \Vert D^2 u(s)\Vert _{L^{\infty }}&\le (s-s_2)^{-\frac{\alpha }{2}}C_2(r, s_2-s_1, s_3-s_2, s-s_2) \\&\le (s_3-s_2)^{-\frac{\alpha }{2}}C_2(r, s_2-s_1, s_3-s_2, s_3-s_2) \\&= C(r, s_2-s_1, s_3-s_2). \end{aligned}$$

Inserting these two estimates into (5.18), we obtain

$$\begin{aligned} I'&\le (t-s_3)^{-\frac{3\alpha }{4}}Cr+C(r, s_2-s_1, s_3-s_2)\int _{s_3}^t(t-s)^{\frac{\alpha }{4}-1}\,\mathrm {d}s \nonumber \\&\le (t-s_3)^{-\frac{3\alpha }{4}}Cr+(t-s_3)^{\frac{\alpha }{4}}C(r, s_2-s_1, s_3-s_2) \nonumber \\&\le (t-s_3)^{-\frac{3\alpha }{4}}C_3(r, s_2-s_1,s_3-s_2, t-s_3). \end{aligned}$$

(5.19)

In addition, we have

$$\begin{aligned} II'= & {} \int _{0}^{s_3}(t-s)^{\alpha -1}\Vert D^3 \Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }A) \varphi (u)\Vert _{L^{\infty }({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\\le & {} C\int _{0}^{s_3}(t-s)^{-\frac{3\alpha }{4}-1}\Vert D\varphi (u)\Vert _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\\le & {} C\int _{0}^{s_3}(t-s)^{-\alpha }(t-s)^{\frac{\alpha }{4}-1}s^{-\frac{\alpha }{4}} \mathrm {d}s\sup _{t}\left( t^{\frac{\alpha }{4}}\Vert Du\Vert _{L^1({\mathbb {R}}^3)}\right) \nonumber \\\le & {} C(t-s_3)^{-\alpha }\int _{0}^{s_3}(s_3-s)^{\frac{\alpha }{2}-1}s^{-\frac{\alpha }{2}} \mathrm {d}s \nonumber \\\le & {} C(t-s_3)^{-\frac{3\alpha }{4}}(s_4-s_3)^{-\frac{\alpha }{4}}. \end{aligned}$$

(5.20)

Here we used the fact that $s_3<s_4<t$. Combining (5.19) and (5.20), we immediately obtain the estimate (5.14) for $k=3$. $\square$

Lemma 5.5

Let the assumptions of Lemma 4.1 be satisfied and $\varphi (u)\in C^3({\mathbb {R}})$. Then we have

$$\begin{aligned} \Vert D^4 u(t)\Vert _{L^{p}({\mathbb {R}}^3)}\le (t-{\bar{s}}_4)^{-\alpha }C_4(r, s_4-s_1, {\bar{s}}_4-s_1, t-{\bar{s}}_4)\Vert u_0\Vert ^{\frac{1}{p}}_{L^1({\mathbb {R}}^3)} \end{aligned}$$

(5.21)

for any $p\in [1,\infty )$ and $0<s_1<{\bar{s}}_1<s_2<{\bar{s}}_2<s_3<{\bar{s}}_3<s_4<{\bar{s}}_4<t$. Here, $C_4$ is a continuous increasing function of $t-{\bar{s}}_4$.

Proof

For $t>{\bar{s}}_4$, we have

$$\begin{aligned} \Vert D^4 u(t)\Vert _{L^p({\mathbb {R}}^3)}\le \ \&\Vert D^4E_{\alpha }(-t^{\alpha }{\mathcal {A}})u_0\Vert _{L^p({\mathbb {R}}^3)} \\&+\int _{{\bar{s}}_4}^t(t-s)^{\alpha -1}\Vert D\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})D^3\varphi (u)\Vert _{L^p({\mathbb {R}}^3)}\,\mathrm {d}s \\&+\int _0^{{\bar{s}}_4}(t-s)^{\alpha -1}\Vert D^4\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u)\Vert _{L^p({\mathbb {R}}^3)}\,\mathrm {d}s \\ = \ \&I+II+III. \end{aligned}$$

Since $p\in [1,\infty )$, by Proposition 3.2, we have

$$\begin{aligned} I \le (t-{\bar{s}}_4)^{-\alpha }C\Vert u_0\Vert _{L^p({\mathbb {R}}^3)} \le (t-{\bar{s}}_4)^{-\alpha }C(r)\Vert u_0\Vert ^{\frac{1}{p}}_{L^1({\mathbb {R}}^3)}. \end{aligned}$$

(5.22)

Combining Proposition 3.3 with Lemma 5.1–5.4, we obtain

$$\begin{aligned} II= & {} \int _{{\bar{s}}_4}^t(t-s)^{\alpha -1}\Vert D\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})D^3\varphi (u)\Vert _{L^p({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\\le & {} C\int _{{\bar{s}}_4}^t(t-s)^{\frac{\alpha }{4}-1} \Vert D^3 \varphi (u)\Vert _{L^{p}({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\\le & {} C\int _{{\bar{s}}_4}^t(t-s)^{\frac{\alpha }{4}-1} \left( \Vert D u\Vert ^3_{L^{3p}({\mathbb {R}}^3)}+\Vert Du\cdot D^2 u\Vert _{L^{p}({\mathbb {R}}^3)}+\Vert D^3 u\Vert _{L^{p}({\mathbb {R}}^3)}\right) \mathrm {d}s \nonumber \\\le & {} C\int _{{\bar{s}}_4}^t(t-s)^{\frac{\alpha }{4}-1} \Big (\Vert D u\Vert ^{3-\frac{1}{p}}_{L^{\infty }({\mathbb {R}}^3)}\Vert D u\Vert ^{\frac{1}{p}}_{L^1({\mathbb {R}}^3)} \nonumber \\&+\Vert D u\Vert ^{1-\frac{1}{p}}_{L^{\infty }({\mathbb {R}}^3)}\Vert D u\Vert ^{\frac{1}{p}}_{L^1({\mathbb {R}}^3)}\Vert D^2 u\Vert _{L^{\infty }({\mathbb {R}}^3)} +\Vert D^3u\Vert ^{1-\frac{1}{p}}_{L^{\infty }({\mathbb {R}}^3)}\Vert D^3u\Vert ^{\frac{1}{p}}_{L^1({\mathbb {R}}^3)}\Big )\mathrm {d}s \nonumber \\\le & {} \int _{{\bar{s}}_4}^t(t-s)^{\frac{\alpha }{4}-1} (s-{\bar{s}}_3)^{-\frac{3\alpha }{4}} \Vert u_0\Vert ^{\frac{1}{p}}_{L^{1}({\mathbb {R}}^3)}\Big (C_1^{3-\frac{1}{p}}(r,s_2-s_1, s-s_1) \nonumber \\&+C_1^{1-\frac{1}{p}}(r,s_2-s_1, s-s_1)C_2(r,s_2-s_1, s_3-s_2, s-s_2)M_1^{\frac{1}{p}}(r) \nonumber \\&+C_3^{1-\frac{1}{p}}(r,s_2-s_1, s_3-s_2, s_4-s_3, s-s_3)M_3^{\frac{1}{p}} (r,s_2-s_1, {\bar{s}}_3-{\bar{s}}_2, s-{\bar{s}}_3)\Big )\mathrm {d}s \nonumber \\\le & {} (t-{\bar{s}}_4)^{-\alpha }C_4(r, s_4-s_1, {\bar{s}}_4-s_1, t-{\bar{s}}_4)\Vert u_0\Vert ^{\frac{1}{p}}_{L^1({\mathbb {R}}^3)}, \end{aligned}$$

(5.23)

where we also used the monotonicity of $C_1$, $C_2$, $C_3$ and $M_3$ with respect to t. By the similar method, we have

$$\begin{aligned} III&= \int _{0}^{{\bar{s}}_4}(t-s)^{\alpha -1}\Vert D^4 \Delta E_{\alpha , \alpha } (-(t-s)^{\alpha }A)\varphi (u)\Vert _{L^p({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le C\int _{0}^{{\bar{s}}_4}(t-s)^{-\frac{\alpha }{2}-1}\Vert \varphi (u)\Vert _{L^p({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le C(r)\int _{0}^{{\bar{s}}_4}(t-s)^{-\frac{\alpha }{2}(1+\frac{1}{p})}(t-s)^{\frac{\alpha }{2p}-1} \Vert u\Vert ^{\frac{\sigma }{p}}_{L^\sigma ({\mathbb {R}}^3)}\,\mathrm {d}s \nonumber \\&\le C(r)(t-{\bar{s}}_4)^{-\frac{\alpha }{2}(1+\frac{1}{p})}\int _{0}^{{\bar{s}}_4} ({\bar{s}}_4-s)^{\frac{\alpha }{2p}-1}s^{-\frac{\alpha }{2p}}\mathrm {d}s \sup _{t}\left( t^{\frac{\alpha }{2}}\Vert u\Vert ^\sigma _{L^\sigma ({\mathbb {R}}^3)}\right) ^{\frac{1}{p}} \nonumber \\&\le (t-{\bar{s}}_4)^{-\alpha }C(r, t-{\bar{s}}_4)\Vert u_0\Vert ^{\frac{1}{p}}_{L^{1}}. \end{aligned}$$

(5.24)

Summing up, we immediately conclude the estimate (5.21). $\square$

Theorem 5.1

Let the assumptions of Theorem 4.1 be satisfied and $\varphi (u)\in C^3({\mathbb {R}})$. If $\Vert u_0\Vert _{L^1({\mathbb {R}}^3)}$ is sufficiently small and $\varphi (u)=O(1)|u|^\sigma$ as $u\rightarrow 0$, where $\sigma =\frac{5}{3}$, then the mild solution of the problem (1.1) $u\in C((0,\infty ); W^{4,p}({\mathbb {R}}^3)\cap C_0({\mathbb {R}}^3))$ for $1\le p <\infty$.

Proof

Let $1\le p<\infty$. By Lemma 5.5, we see that $u(t): (0,\infty )\mapsto W^{4,p}({\mathbb {R}}^3)$. Remark 4.1 shows that $u\in C([0,\infty ); L^p({\mathbb {R}}^3)\cap C_0({\mathbb {R}}^3))$. So it suffices to show the continuity of $\Vert u(t)\Vert _{W^{4,p}({\mathbb {R}}^3)}$ with respect to $t\in (0,\infty )$. For simplicity, we only prove the continuity of $\Vert D^4u(t)\Vert _{L^p({\mathbb {R}}^3)}$, since the proofs of the other cases ($k=1,2,3$) are similar.

Fixing $t_0\in (0,\infty )$, we have the following estimate

$$\begin{aligned} \Vert D^4u(t)-D^4u(t_0)\Vert _{L^{p}({\mathbb {R}}^3)} \le \ \&\Vert (D^4E_{\alpha }(-t^{\alpha }{\mathcal {A}})-D^4E_{\alpha }(-t_0^{\alpha }{\mathcal {A}}))u_0\Vert _{L^{p}({\mathbb {R}}^3)} \\&+ \int _{t_0}^t(t-s)^{\alpha -1}\left\| D\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}}) D^3\varphi (u(s))\right\| _{L^{p}({\mathbb {R}}^3)}\,\mathrm {d}s \\&+ \int _0^{t_0}\left\| (t-s)^{\alpha -1}D^4\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u(s))\right. \\&\quad \quad \left. -(t_0-s)^{\alpha -1}D^4\Delta E_{\alpha , \alpha }(-(t_0-s)^{\alpha }{\mathcal {A}}) \varphi (u(s))\right\| _{L^{p}({\mathbb {R}}^3)}\,\mathrm {d}s \\ \le \ \&I_1+I_2+I_3, \quad t>t_0. \end{aligned}$$

Using the strong continuity of $D^4E_{\alpha }(-t^{\alpha }{\mathcal {A}})$ on $L^p({\mathbb {R}}^3)$ for $t\in (0,\infty )$, we deduce that the first term $I_1$ goes to zero as $t\rightarrow t_0^+$. Take

$$\begin{aligned} 0<s_1<{\bar{s}}_1<s_2<{\bar{s}}_2<s_3<{\bar{s}}_3<s_4<{\bar{s}}_4=\frac{t_0}{2}<t_0. \end{aligned}$$

Similarly with the estimate (5.23), we can obtain that

$$\begin{aligned} I_2&= \int _{t_0}^t\left\| (t-s)^{\alpha -1}D\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}}) D^3\varphi (u(s))\right\| _{L^{p}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C\int _{t_0}^t(t-s)^{\frac{\alpha }{4}-1} \left\| D^3\varphi (u(s))\right\| _{L^{p}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C\int _{t_0}^t(t-s)^{\frac{\alpha }{4}-1} \Big (\Vert D u\Vert ^{3-\frac{1}{p}}_{L^{\infty }({\mathbb {R}}^3)}\Vert D u\Vert ^{\frac{1}{p}}_{L^1({\mathbb {R}}^3)} \\&\ \ \ \ +\Vert D u\Vert ^{1-\frac{1}{p}}_{L^{\infty }({\mathbb {R}}^3)}\Vert D u\Vert ^{\frac{1}{p}}_{L^1({\mathbb {R}}^3)}\Vert D^2 u\Vert _{L^{\infty }} +\Vert D^3u\Vert ^{1-\frac{1}{p}}_{L^{\infty }({\mathbb {R}}^3)}\Vert D^3u\Vert ^{\frac{1}{p}}_{L^1({\mathbb {R}}^3)}\Big )\mathrm {d}s \\&\le \int _{t_0}^t(t-s)^{\frac{\alpha }{4}-1} (s-{\bar{s}}_3)^{-\frac{3\alpha }{4}} \Vert u_0\Vert ^{\frac{1}{p}}_{L^{1}({\mathbb {R}}^3)}\Big (C_1^{3-\frac{1}{p}}(r,s_2-s_1, s-s_1) \\&\ \ \ \ +C_1^{1-\frac{1}{p}}(r,s_2-s_1, s-s_1)C_2(r,s_2-s_1, s_3-s_2, s-s_2) \\&\ \ \ \ +C_3^{1-\frac{1}{p}}(r,s_2-s_1, s_3-s_2, s_4-s_3, s-s_3)M_3^{\frac{1}{p}} (r,s_2-s_1, {\bar{s}}_3-{\bar{s}}_2, s-{\bar{s}}_3)\Big )\mathrm {d}s \\&\le \Vert u_0\Vert ^{\frac{1}{p}}_{L^{1}}C(r,s_2-s_1, s_3-s_2, s_4-s_3, {\bar{s}}_3-{\bar{s}}_2, t-s_1)(t_0-{\bar{s}}_3)^{-\frac{3\alpha }{4}}\int _{t_0}^t(t-s)^{\frac{\alpha }{4}-1}\mathrm {d}s \\&\le \Vert u_0\Vert ^{\frac{1}{p}}_{L^{1}}C(r,s_2-s_1, s_3-s_2, s_4-s_3, {\bar{s}}_3-{\bar{s}}_2, t-s_1)(t_0-{\bar{s}}_3)^{-\frac{3\alpha }{4}}(t-t_0)^{\frac{\alpha }{4}}, \end{aligned}$$

where we used the monotonicity of $C_1$, $C_2$, $C_3$ and $M_3$ with respect to t. Obviously, this term vanishes as $t\rightarrow t_0^+$.

For the third term $I_3$, we denote

$$\begin{aligned} f(t,s)=\big \Vert (t-s)^{\alpha -1}&D^4\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u(s)) \\&-(t_0-s)^{\alpha -1}D^4\Delta E_{\alpha , \alpha }(-(t_0-s)^{\alpha }{\mathcal {A}})\varphi (u(s))\big \Vert _{L^{p}({\mathbb {R}}^3)} \end{aligned}$$

and it is easy to see that

$$\begin{aligned} f(t,s)&\le \left| (t-s)^{\alpha -1}-(t_0-s)^{\alpha -1}\right| \cdot \big \Vert D^4\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u(s)) \big \Vert _{L^{p}({\mathbb {R}}^3)} \\&\ \ \ \ +(t_0-s)^{\alpha -1}\big \Vert D^4\Delta E_{\alpha ,\alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u(s)) \\&\ \ \ \ \ \ \ \ -D^4\Delta E_{\alpha , \alpha }(-(t_0-s)^{\alpha }{\mathcal {A}})\varphi (u(s))\big \Vert _{L^{p}({\mathbb {R}}^3)} \\&\le C\left| (t-s)^{\alpha -1}-(t_0-s)^{\alpha -1}\right| \cdot (t-s)^{-\frac{\alpha }{2}}\Vert u_0-{\bar{u}}\Vert _{L^{p}({\mathbb {R}}^3)} \\&\ \ \ \ +(t_0-s)^{\alpha -1}\big \Vert D^4\Delta E_{\alpha ,\alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u(s)) \\&\ \ \ \ \ \ \ \ -D^4\Delta E_{\alpha , \alpha }(-(t_0-s)^{\alpha }{\mathcal {A}})\varphi (u(s))\big \Vert _{L^{p}({\mathbb {R}}^3)}. \end{aligned}$$

Combining with the strong continuity of $D^4\Delta E_{\alpha ,\alpha }(-t^{\alpha }{\mathcal {A}})$ on $L^p({\mathbb {R}}^3)$, we have

$$\begin{aligned} \lim _{t\rightarrow t_0^+}f(t,s)=0 \end{aligned}$$

for any fixed $s\in (0,t_0)$. In addition, we can control $I_3$ by the following two inequalities,

$$\begin{aligned} I'_3&= \int _{\frac{t_0}{2}}^{t_0}\left\| (t-s)^{\alpha -1}D\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})D^3\varphi (u(s))\right. \\&\ \ \ \ \left. -(t_0-s)^{\alpha -1}D\Delta E_{\alpha , \alpha }(-(t_0-s)^{\alpha }{\mathcal {A}})D^3\varphi (u(s))\right\| _{L^{p}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C\int _{\frac{t_0}{2}}^{t_0}(t_0-s)^{\frac{\alpha }{4}-1} \left\| D^3\varphi (u(s))\right\| _{L^{p}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C\int _{\frac{t_0}{2}}^{t_0}(t_0-s)^{\frac{\alpha }{4}-1} \Big (\Vert D u\Vert ^{3-\frac{1}{p}}_{L^{\infty }}\Vert D u\Vert ^{\frac{1}{p}}_{L^1} \\&\ \ \ \ +\Vert D u\Vert ^{1-\frac{1}{p}}_{L^{\infty }}\Vert D u\Vert ^{\frac{1}{p}}_{L^1}\Vert D^2 u\Vert _{L^{\infty }} +\Vert D^3u\Vert ^{1-\frac{1}{p}}_{L^{\infty }}\Vert D^3u\Vert ^{\frac{1}{p}}_{L^1}\Big )\mathrm {d}s \\&\le \int _{\frac{t_0}{2}}^{t_0}(t_0-s)^{\frac{\alpha }{4}-1} (s-{\bar{s}}_3)^{-\frac{3\alpha }{4}} \Vert u_0\Vert ^{\frac{1}{p}}_{L^{1}}\Big (C_1^{3-\frac{1}{p}}(r,s_2-s_1, s-s_1) \\&\ \ \ \ +C_1^{1-\frac{1}{p}}(r,s_2-s_1, s-s_1)C_2(r,s_2-s_1, s_3-s_2, s-s_2) \\&\ \ \ \ +C_3^{1-\frac{1}{p}}(r,s_2-s_1, s_3-s_2, s_4-s_3, s-s_3)M_3^{\frac{1}{p}} (r,s_2-s_1, {\bar{s}}_3-{\bar{s}}_2, s-{\bar{s}}_3)\Big )\mathrm {d}s \\&\le \Vert u_0\Vert ^{\frac{1}{p}}_{L^{1}}C(r,s_2-s_1, s_3-s_2, s_4-s_3, {\bar{s}}_3-{\bar{s}}_2, t_0-s_1)(t_0-{\bar{s}}_3)^{-\frac{3\alpha }{4}}(\frac{t_0}{2})^{\frac{\alpha }{4}}, \end{aligned}$$

and

$$\begin{aligned} I''_{3}&= \int _0^{\frac{t_0}{2}}\left\| (t-s)^{\alpha -1}D^4\Delta E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\varphi (u(s))\right. \\&\ \ \ \ \left. -(t_0-s)^{\alpha -1}D^4\Delta E_{\alpha , \alpha }(-(t_0-s)^{\alpha }{\mathcal {A}})\varphi (u(s))\right\| _{L^{p}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C\int _0^{\frac{t_0}{2}}(t_0-s)^{-\frac{\alpha }{2}-1} \left\| \varphi (u(s))\right\| _{L^{p}({\mathbb {R}}^3)}\,\mathrm {d}s \\&\le C(r, \Vert u_0\Vert _{L^1})\,(\frac{t_0}{2})^{-\frac{\alpha }{2}}, \end{aligned}$$

which implies

$$\begin{aligned} I_3=I'_3+I''_3 \le \int _0^{t_0} g(s)\,\mathrm {d}s= C(r, t_0, \Vert u_0\Vert _{L^1}), \end{aligned}$$

for some integrable function g and constant C independent of t. Then the application of dominated convergence theorem to $I_3$ shows that $I_3\rightarrow 0$ as $t\rightarrow t_0^+$.

Analogously, we can also obtain the same limit of $\Vert D^4u(t)-D^4u(t_0)\Vert _{L^{p}({\mathbb {R}}^3)}$ as $t\rightarrow t_0^-$ with $t_0\in (0,\infty )$. Therefore,

$$\begin{aligned} \lim _{t\rightarrow t_0}\Vert D^4u(t)-D^4u(t_0)\Vert _{L^{p}({\mathbb {R}}^3)}=0,\quad t_0\in (0,\infty ), \end{aligned}$$

which completes the proof. $\square$

Next, we are going to prove Theorem 2.2. Several additional properties of both families of Mittag-Leffler operators are necessary in our proof which can be found in [8, 37].

Proposition 5.1

Let $0<\alpha <1$ and $v\in \mathring{L}^p({\mathbb {R}}^3)$. Then we have

• (i) ${_{c}{D}}^{\alpha }_{t} E_{\alpha }\left( -t^{\alpha } {\mathcal {A}}\right) v=-{\mathcal {A}}E_{\alpha }\left( -t^{\alpha } {\mathcal {A}}\right) v$, $t>0$;

• (ii) $\frac{\mathrm d}{{\mathrm d}t}E_{\alpha }\left( -t^{\alpha } {\mathcal {A}}\right) v=-t^{\alpha -1}{\mathcal {A}}\,E_{\alpha ,\alpha }\left( -t^{\alpha } {\mathcal {A}}\right) v$, $t>0$;

• (iii) $E_{\alpha }(-t^{\alpha } {\mathcal {A}}) v=J_t^{1-\alpha }(t^{\alpha -1}E_{\alpha ,\alpha }\left( -t^{\alpha } {\mathcal {A}}\right) v)$, $t>0$.

Proof of Theorem 2.2

Let $u\in C([0,\infty ); L^{1}({\mathbb {R}}^3))$ be the global mild solution of the problem (1.1). By Theorem 5.1, we have $u\in C((0,\infty ); W^{4,1}({\mathbb {R}}^3))$. Denote

$$\begin{aligned} u=u_1-u_2, \end{aligned}$$

where

$$\begin{aligned} u_1=E_{\alpha }(-t^{\alpha }{\mathcal {A}})u_0 \quad \mathrm{and}\quad u_2=\int _0^t(t-s)^{\alpha -1}E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})\Delta \varphi (u)\,\mathrm {d}s. \end{aligned}$$

By Proposition 5.1 (i) and Theorem 5.1, it’s to see that

$$\begin{aligned} {_{c}{D}}^{\alpha }_{t} u_1 =-{\mathcal {A}}\,E_{\alpha }\left( -t^{\alpha } {\mathcal {A}}\right) u_0=-{\mathcal {A}}\,u_1 \in C((0,\infty ); L^{1}({\mathbb {R}}^3)). \end{aligned}$$

Next we show ${_{c}{D}}^{\alpha }_{t} u_2 \in C((0,\infty ); L^{1}({\mathbb {R}}^3))$. Noting $u_2(0)=0$ and combining with Proposition 5.1 (iii), we derive that

$$\begin{aligned} {_{c}{D}}^{\alpha }_{t} u_2(t)&= \frac{\mathrm {d}}{\mathrm {d}t}J_t^{1-\alpha }u_2(t) \\&= \frac{\mathrm {d}}{\mathrm {d}t}J_t^{1-\alpha }\left( {\mathcal {Q}}_{\alpha }*\Delta \varphi (u)\right) \\&= \frac{\mathrm {d}}{\mathrm {d}t}\left( J_t^{1-\alpha }{\mathcal {Q}}_{\alpha }\right) *\Delta \varphi (u) \\&= \frac{\mathrm {d}}{\mathrm {d}t}E_{\alpha }(-t^{\alpha } {\mathcal {A}}) *\Delta \varphi (u), \end{aligned}$$

where ${\mathcal {Q}}_{\alpha }(t):=t^{1-\alpha }E_{\alpha , \alpha }(-(t-s)^{\alpha }{\mathcal {A}})$. So it suffices to prove that

$$\begin{aligned} w(t):=(E_{\alpha }(-t^{\alpha } {\mathcal {A}}) *\Delta \varphi (u))(t)\in C^1((0,\infty ); L^{1}({\mathbb {R}}^3)). \end{aligned}$$

Fix $t_0>0$ and take $0<h<1$. Then we can easily to obtain that

$$\begin{aligned} \frac{w(t_0+h)-w(t_0)}{h} =&\ \frac{1}{h}\int _0^{t_0}\Big (E_{\alpha }(-(t_0+h-s)^{\alpha }{\mathcal {A}})-E_{\alpha } (-(t_0-s)^{\alpha }{\mathcal {A}})\Big )\Delta \varphi (u)\,\mathrm {d}s \nonumber \\&\ +\frac{1}{h}\int _{t_0}^{t_0+h}E_{\alpha }(-(t_0+h-s)^{\alpha }{\mathcal {A}})\Delta \varphi (u)\,\mathrm {d}s \nonumber \\ :=&\ I_1+I_2. \end{aligned}$$

(5.25)

Similarly with (5.23) and (5.24), we obtain there exists $s_0\in (0,t_0)$ such that

$$\begin{aligned} \int _{0}^{t_0}(t_0-s)^{\alpha -1}\Vert {\mathcal {A}}\,E_{\alpha ,\alpha }\left( -t_0^{\alpha } {\mathcal {A}}\right) \Delta \varphi (u)\Vert _{L^1({\mathbb {R}}^3)}\,\mathrm {d}s \le C\Vert u_0\Vert _{L^1({\mathbb {R}}^3)}, \end{aligned}$$

where C is dependent of $t_0-s_0$. Therefore, applying dominated convergence theorem and Proposition 5.1 (ii) to the first term $I_1$, we get that

$$\begin{aligned} \lim _{h\rightarrow 0^+}I_{1}&= \lim _{h\rightarrow 0^+}\frac{1}{h}\int _0^{t_0}\Big (E_{\alpha }(-(t_0+h-s)^{\alpha }{\mathcal {A}})-E_{\alpha } (-(t_0-s)^{\alpha }{\mathcal {A}})\Big )\Delta \varphi (u)\,\mathrm {d}s \nonumber \\&= -\int _0^{t_0}(t_0-s)^{\alpha -1}{\mathcal {A}}\,E_{\alpha ,\alpha }\left( -(t_0-s)^{\alpha } {\mathcal {A}}\right) \Delta \varphi (u)\,\mathrm {d}s \nonumber \\&= -{\mathcal {A}}\,u_2(t_0). \end{aligned}$$

(5.26)

Furthermore, note that

$$\begin{aligned} I_2 =&\ \frac{1}{h}\int _{t_0}^{t_0+h}E_{\alpha }(-(t_0+h-s)^{\alpha }{\mathcal {A}})\Delta \varphi (u(s))\,\mathrm {d}s \\ =&\ \frac{1}{h}\int _{t_0}^{t_0+h}\Big (E_{\alpha }(-(t_0+h-s)^{\alpha }{\mathcal {A}})-I\Big )\Delta \varphi (u(s))\,\mathrm {d}s \\&\ +\frac{1}{h}\int _{t_0}^{t_0+h}\Delta \varphi (u(s))\,\mathrm {d}s. \end{aligned}$$

By the strongly continuity property of $E_{\alpha }(-t^{\alpha }{\mathcal {A}})$ over $[0,\infty )$ and the fact that $\Delta \varphi (u)\in C((0,\infty ); L^{1}({\mathbb {R}}^3))$, we can see that

$$\begin{aligned} \lim _{h\rightarrow 0^+}I_{2}=\Delta \varphi (u(t_0)). \end{aligned}$$

(5.27)

Combining (5.26) with (5.27), we deduce that w is differential from the right at $t_0$ and $w'_+(t_0)=-{\mathcal {A}}\,u_2(t_0)+\Delta \varphi (u(t_0))$. Analogously, we can also obtain the same limit of $w'_-(t_0)$ as $h\rightarrow 0^-$.

Hence, we see that ${_{c}{D}}^{\alpha }_{t} u \in C((0,\infty ); L^{1}({\mathbb {R}}^3))$ and

$$\begin{aligned} {_{c}{D}}^{\alpha }_{t} u = -{\mathcal {A}}\,u+\Delta \varphi (u),\quad t>0, \end{aligned}$$

which completes the proof of Theorem 2.2. $\square$