Reference Groups and the Poverty Line: An Axiomatic Approach with an Empirical Illustration

Abstract

A recent trend in the study of poverty is to consider a relative poverty line, one that is responsive to the nature of the income distribution. We develop an axiomatic approach to the determination of an amalgam poverty line. Given a reference income (e.g., the mean or the median), the amalgam poverty line becomes a weighted average of the absolute poverty line and the reference income, where the weights depend on the policy maker’s preferences for aggregating the two components. The paper ends with an empirical illustration comparing urban and rural areas in the People’s Republic of China and India.

Kind permission received from Emerald Publishing for reprinting this article from Research On Economic Inequality, Volume 24, 2017, is acknowledged thankfully.

1 Introduction

Even in the early years of the twenty-first century, removal of poverty remains one of the major goals of economic policy in many countries of the world. A wide variety of poverty indices have been proposed in the literature and the determination of an income or consumption threshold on which the definition of poverty relies has been a debatable issue for quite some time (see among others, Ruggles 1990; Ravallion 1994; Citro and Michael 1995). A distinction is made between an “absolute poverty line”, which has a fixed real value over time and is given exogenously, and a “relative poverty line” which is responsive to the income distribution.¹ The major distinction between the relative and absolute thresholds arises not from specification of their values but from how the values change under changes in the distribution.

In fact virtually all developing countries use absolute poverty lines, whereby any standard measure of poverty decreases if all incomes grow at the same rate (leaving relative inequality unchanged). Following Ravallion et al. (1991), the World Bank thus used a $1 per day poverty line for the developing world and this threshold was updated by Ravallion et al. (2009) to $1.25 a day at 2005 purchasing power parity (PPP). Deaton (2010) argued that many problems are involved in the calculation of a global poverty line and correction for international price differences using PPP exchange rates. More recently the World Bank adopted a new international poverty line equal to $1.90 (see, Ferreira et al. 2015).

Developed countries, especially the OECD countries, on the other hand use a constant proportion of mean or median income as the poverty line so that an equi-proportionate increase in all incomes leaves the poverty level unchanged. This is called a strongly relative poverty measure (SR). Such an approach requires, however, quite implausible assumptions, namely that people are concerned solely with relative deprivation and/or that the costs of social inclusion can fall to nearly zero in the poorest places.

Various attempts have been made to incorporate relativity in poverty measurement. Some studies suggested adjusting poverty lines across demographic subgroups. The idea of equivalence scale has as well been used for determining a relative poverty line.² More recently, Kakwani (2011) employed consumer theory to construct food and nonfood poverty thresholds.

An original approach was taken by Ravallion and Chen (2011) who introduced the concept of weakly relative poverty. They argued that selecting a strongly relative poverty measure (SR) in terms of the costs of social exclusion, where an implicit assumption is made according to which this cost is proportional to mean income, may not be tenable in the case of the developing world. They therefore proposed a weakly relative poverty line (WR) whose elasticity with respect to the mean income is positive with unity as its upper bound. In their model, they made a distinction between an income and a welfare space and assumed that $\overline{V} = V\left( {Z, \left( {\frac{Z}{M}} \right)} \right)$, where $\overline{V}$ is the fixed welfare poverty line, Z is the income poverty line and M is the mean or median income. For a non-welfarist interpretation of relative poverty line, they proposed a generalization of the Atkinson and Bourguignon (AB) approach. The AB approach links the physical survival needs to absolute poverty line and the social inclusion needs to the relative line. Ravallion and Chen (2011) specified that $Z = Z^{*} + \varPsi \left( M \right)$, where $Z^{*}$ and $\varPsi (M)$ are, respectively, the minimum expenditure required to assure the basic consumption needs and the cost of the incremental social needs beyond basic consumption. This ensures domination of absolute lines at low consumption levels (developing world) while the poverty line becomes relative beyond some higher level (developed world) and sets up a framework to make global poverty comparisons.

In another paper, Chen and Ravallion (2013) argued that there may in fact be two quite different reasons why poverty lines might vary systematically with the average consumption or income of a society. One reason is that there may be a common underlying poverty level of welfare, but that the level of consumption needed to attain it varies, stemming from social effects. The other reason does not require such effects, but rather postulates that social norms vary, implying different reference levels of welfare. Furthermore, the choice between these two interpretations has implications concerning the choice of relative versus absolute poverty lines. If one thinks that it is really only social norms that differ, with welfare depending solely on one’s own consumption, then one would probably prefer an absolute measure, imposing a common norm (though one may want to consider more than one possible line). If however one is convinced that there are social effects on welfare, then one would be more inclined to use a relative line in the consumption or income space, anchored to a common welfare standard. The weakly relative poverty measures entail that the poverty line only rises with the mean above some critical value and it then does so with elasticity less than one. A process of distribution-neutral growth will then reduce the incidence of weakly relative poverty. The absolute measure is only obtained as a special case for sufficiently poor countries. Ideas quite similar to those expressed in Ravallion and Chen (2011) and Chen and Ravallion (2013) may be found in Ravallion (2008) and Ravallion (2012).

An obvious place to look for identifying the parameters of a schedule of weakly relative poverty lines is the set of national poverty lines found across developing countries. It then appears that national poverty lines among developing countries show a systematic nonnegative relationship with the average consumption of a country. Given that the determination of the poverty line is still a disputable matter, we wish to propose an axiomatic approach to the calculation of a relative poverty line. It is assumed that the poverty line is relative in the income/consumption space. Our approach follows a long tradition of identifying welfare with utility. Utility depends on the absolute income and the relative income, that is, income relative to some reference standard. There is in fact a vast literature that stresses the importance of incorporating relative position in decision-making analysis (see, Duesenberry 1949; Kahneman and Tversky 1991; Frank 1985, 1999; Clark and Oswald 1996; Easterlin 2001; Falk and Knell 2004; Ferrer-i-Carbonell 2005). The focus on relative economic position in utility analysis has also been recognized in the theory of relative deprivation (Runciman 1966).³

In this paper, we assume that individual utility is increasing, concave in absolute income but decreasing, convex in the reference standard (see, Clark and Oswald 1998). Our analysis relies on a general reference income level, of which some proportions of mean or median income can be special cases. An additive form and a multiplicative form of the utility function are characterized using two different sets of intuitively reasonable axioms. Now, suppose a reference income is given. We then employ a utility-consistency condition to determine the poverty line uniquely in terms of a reference income and a given poverty line. More precisely, given a reference income and a person with income equal to an arbitrarily set poverty line, who is just poor, we determine the level of the corresponding utility. We then consider an alternative setting where the person is again just poor, that is, with income at some alternative poverty line. However, in this situation, his utility is not affected by the reference income. Since the effect of the reference income on utility is captured through the absolute or relative divergence of a person’s income with the reference income, the annulment of the effect is obtained by setting his own income to be his reference income. Utility-consistency requires that the person is equally satisfied in both positions. In other words, we equate the utility in this later state of affairs with the level of utility derived for the arbitrarily set poverty line and reference income situation to determine the arbitrary poverty line uniquely. This assumption of equal satisfaction is quite plausible because in each case the individual is at the existing poverty line income.

It may be worthwhile to note that the idea of utility-consistency goes back a long way in classical welfare measurement. The interpretation of the poverty line as a money metric of utility can be found in Blackorby and Donaldson (1987). A more recent treatment of the issue in the context of poverty analysis can be found in Kakwani (2011).

An innovative feature of our paper is that, for either form of the utility function, the new poverty line becomes an amalgam, a weighted average, of the given poverty line and the reference income. Therefore, our derivation allows the possibility of a change in the question “absolute or relative?” to “how much relative?” A second novelty of our contribution is that Foster’s (1998) suggestion for a “hybrid” poverty threshold, a weighted geometric mean of a relative threshold and an absolute threshold, can be supported by our utility-consistency condition. Thus, our suggestion bears a close similarity with that of Foster (1998) and hence can as well be treated as a hybrid approach.

Another attractive feature of our framework is that some of the suggestions that exist in the literature (e.g., Atkinson-Bourguignon (2001) and EU standard) for basing the poverty line directly on some location parameter, such as the mean or median, become particular cases of our formulation.

The paper is organized as follows. Section 2 develops the theoretical framework. The main contribution of this section is that we characterize the utility functions using both the ratio and difference form comparisons. For the sake of completeness, Sect. 2 also provides a systematic comparison of our framework with the approach of Blackorby–Donaldson (1987). Next, Sect. 3 gives a short empirical illustration based on separate data on rural and urban areas in the People’s Republic of China and India. Section 4 then briefly concludes.

2 Formal Framework

The model relies on two assumptions about an individual’s utility function. The first assumption specifies that utility depends in part on the individual’s absolute income. According to the second assumption, utility also depends on the relative income, income relative to some reference standard. This latter condition is one way of ensuring that utility partly depends on his relative position (or “status”) in the society in terms of some attribute of wellbeing. Such assumptions about utility functions are quite common in the literature (see, for example, Clark and Oswald 1998). As Clark and Oswald (1998) suggested, relativity can be incorporated into the framework by having difference comparisons or ratio comparisons.

Let x and m, respectively, be the absolute income and reference income of an individual in the society. Both x and m are assumed to be drawn from the finite nonnegative nondegenerate interval $\left[ {0,\infty } \right)$, that is, $x,m \in \left[ {0,\infty } \right).$ The reference income m can be treated as a positional good and it is assumed that x does not exceed the reference income.⁴ Examples of m can be the mean and the median incomes in the population or some positive scalar transformations of them.

Let U denote the nonconstant real valued utility function of the individual. Following Clark and Oswald (1998), the function $U\left( {x,m} \right)$ is assumed to be increasing, concave in x and decreasing, convex in m. Increasingness and concavity assumptions in absolute income are quite standard.⁵ Suppose a person with a low income regards the income level m as his targeted income. He may be optimistic about receiving this income by working hard and/or receiving some subsidy. An increase in m might increase his difficulty to fulfil the objective of receiving the higher targeted income. This means that his additional utility from an increase in m will be negative, that is, U is decreasing in m. Convexity means that his dissatisfaction from an increase in m increases at a nondecreasing rate. Assume also that $U(.)$ is differentiable.

The difference form comparison demands that the utility function should be of the form $U\left( {x,x - m} \right).$ The argument $x - m$ can be thought of as capturing dis-utility from comparison. That is, in this case the determinant of relative status depends on difference $x - m$. Since $x,m \in \left[ {0,\infty } \right)$ and $x \le m$, it is clear that $x - m \in \left( { - \infty ,0} \right]$.

We now propose the following axioms for a utility function $U:\left[ {0,\infty } \right) \times \left( { - \infty ,0} \right] \to R$ involving difference form comparison, where R is the set of real numbers.

Linear Translatability (LIT): For any real c such that $x + c \in \left[ {0,\infty } \right)$, $U\left( {x + c,\left( {x + c} \right) - \left( {m + c} \right)} \right) = U\left( {x,x - m} \right) + kc$, where $k > 0$ is some scalar.

Linear Homogeneity (LIH): For any $c \in \left( {0,\infty } \right)$, $U\left( {cx,cx - cm} \right) = cU\left( {x,x - m} \right)$.

Since under equal increase of the absolute and reference incomes the relative status $\left( {x - m} \right)$ remains unchanged but the absolute income increases, individual utility should increase. LIT is a simple way of specifying this increment. It demands that when the absolute and reference incomes are changed by a given amount, then utility changes by a constant time of the given amount. In other words, it shows how utility changes when the absolute and reference incomes are diminished or augmented by the same amount. This axiom can be treated as an absolute counterpart to LIH, which says that an equi-proportionate change in the absolute and the reference incomes changes utility equi-proportionately. This postulate is weaker than the requirement that U is increasing in x.

In the literature, on income inequality measurement, a social welfare function that satisfies linear homogeneity and linear translatability simultaneously is called a compromise welfare function. The Gini welfare function is an example of a welfare function of this type (see Blackorby and Donaldson 1980). Such welfare functions are helpful for measuring economic distance between income distributions, which quantifies well-being of one population relative to that of another (see Chakravarty and Dutta 1987).

Proposition 1

The only utility function that satisfies LIT and LIH is of the form

$$ U\left( {x,x - m} \right) = \left( {k - a} \right)x + am, $$

(1)

where $k > 0$ is same as in LIT and $a < 0$ is a constant.

Proof

By LIT $U\left( {x - x,x - x - m + x} \right) = U\left( {x,x - m} \right) - kx$. We rewrite this equation as $U\left( {x,x - m} \right) = U\left( {0, - m + x} \right) + kx$. By LIH it follows that $U\left( {0, - m + x} \right) = \left( {m - x} \right)U\left( {0, - 1} \right) = a\left( {m - x} \right)$, where $a = U\left( {0, - 1} \right)$. Hence $U\left( {x,x - m} \right) = \left( {k - a} \right)x + am$. Decreasingness of U in m requires that $a < 0$. This establishes the necessity part of the proposition. The sufficiency part can be checked easily. $\square$

The weights $\left( {k - a} \right)$ and a in (1) provide a simple way of capturing the mixture of two effects. For $a = 0$ the preferences are private and self-interested. This becomes ensured under the mild condition that $k > 0$. The individual does not look at his position in terms of the reference income. He does not care about what other individuals are doing. It also follows that U is concave in x and convex in m under the restrictions $\left( {k - a} \right) > 0$ and $a < 0$. The utility function in (1) is a particular form of the “additive comparisons model” suggested by Clark and Oswald (1998). However, no characterization has been developed by them.

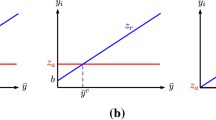
Let us now consider a situation in which an individual does not compare his/her absolute income with the reference income because the reference income itself is identical to the absolute income. If we denote this absolute income by $z_{0}$, then from (1) we have, $U\left( {z_{0} ,0} \right) = kz_{0}$. This absolute income can be taken as the current poverty line. The utility corresponding to some arbitrary poverty line $z_{1}$ and the reference income m will then be given by $U\left( {z_{1} ,z_{1} - m} \right) = \left( {k - a} \right)z_{1} + am$. Let us now find the income $z_{1}$ which would guarantee the individual a level of utility identical to the utility level $U\left( {z_{0} ,0} \right)$. That is, the level of happiness that the person had in the earlier scenario when he was enjoying the poverty line income remains the same in the present case characterized by a new poverty line and a reference income. Equality of the two utility levels can be justified on the ground that in both circumstances the individual’s income coincides with the poverty line income. We refer to this as a utility-consistency condition. (See Blackorby and Donaldson 1987, and Kakwani 2011). To understand this further, suppose for a given time point the absolute poverty line is well-defined at $z_{0}$. Suppose now the distribution changes. Given a reference income m, if we want to determine a poverty line $z_{1}$ that will keep the utility of the person at the old poverty line unchanged, we should readjust the poverty line. The readjustment is done by equating the utility levels.

Equating the two expressions $U\left( {z_{0} ,0} \right)$ and $U\left( {z_{1} ,z_{1} - m} \right)$, we get

$$ z_{1} = qz_{0} + \left( {1 - q} \right)m, $$

(2)

where $q = \frac{k}{{\left( {k - a} \right)}}$. Given that $a < 0$, we can say that the revised poverty line is a convex mixture, a weighted average, of the existing poverty line and the specified reference income. For a 1 unit increase in the living standard (m), $\left( {1 - q} \right)$ represents the increase in the threshold $z_{1}$. Therefore, q may be interpreted as a policy parameter in the sense that it reflects the relative importance of the current poverty line in getting its revised estimate. As the weight q increases from 0 to 1, more and more importance is assigned to the current poverty line in the averaging in (2). For $q = 1$, $z_{1}$ coincides with the existing poverty line $z_{0}$, whereas for $q = 0$, $z_{1}$ becomes the reference income m. A compromise choice for q is $q = 0.5$.

As Clark and Oswald (1998) argued, an alternative specification can be a ‘ratio comparisons model’. In this case the individual’s utility depends directly on the absolute income x and also on the relative factor $\frac{x}{m}$. Thus, in this case the determinant of the status is the ratio $\frac{x}{m}$. We consider a general form of the utility function $U\left( {x,f\left( {\frac{x}{m}} \right)} \right)$, where f is a positive valued and increasing transformation of the ratio $\frac{x}{m}$. This is a fairly general version of a ratio comparisons model. As before, we maintain the assumptions that U is increasing, concave in x and decreasing, convex in m. By our formulation, U is increasing in $f\left( {\frac{x}{m}} \right)$.

In order to characterize a particular form of the utility function which we wish to use for determining a poverty line in the ratio comparisons framework, we consider the following axioms for $U:\left( {0,\infty } \right) \times \left( {0,\infty } \right) \to R_{ + + }$, where $R_{ + + }$ is the strictly positive part of R.

Linear Homogeneity (LIH): For any $\left( {x,f\left( {\frac{x}{m}} \right)} \right) \in \left( {0,\infty } \right) \times \left( {0,\infty } \right)$,

$U\left( {cx,f\left( {\frac{cx}{cm}} \right)} \right) = cU\left( {x,f\left( {\frac{x}{m}} \right)} \right)$, where c > 0 is arbitrary.

Since $f\left( {\frac{x}{m}} \right)$ remains unaltered under positive scale transformation of the absolute income x and the reference income m, LIH shows how utility should be adjusted under such transformation of the variables.

Normalization (NOM): If $x = 1$, then $U\left( {x,f\left( {\frac{x}{m}} \right)} \right) = f\left( {\frac{1}{m}} \right)$.

Constancy of Marginal Utility of Reference Income (CMR): $\frac{{\partial U\left( {x,f\left( {\frac{x}{m}} \right)} \right)}}{\partial m} = - \theta < 0$.

Continuity (CON): U is continuous in its arguments.

NOM is a cardinality principle which says that if the individual’s income is 1, then corresponding utility value is given simply by the transformed value $f\left( {\frac{1}{m}} \right)$ of the ratio $\frac{1}{m}$. Variants of this are certainly possible. But given that the income is fixed at 1, the utility should be dependent on the ratio $\frac{1}{m}$ in a negative monotonic way and NOM ensures this. Continuity assures that minor observational errors in incomes will not change utility abruptly. CMR reflects the view that with an increase in reference income utility decreases at a nonincreasing rate, an assumption we have made at the outset of this section. While alternative possibilities definitely exist, CMR is quite simple and easy to understand.

Axioms LIH, NOM, CMR, and CON uniquely identify a specific functional form of the utility function.

Proposition 2

The only utility function $U:\left( {0,\infty } \right) \times \left( {0,\infty } \right) \to R_{ + + }$ that satisfies LIH, NOM, CMR and CON is of the form

$$ U\left( {x,f\left( {\frac{x}{m}} \right)} \right) = x\left( {\beta - \frac{\theta m}{x}} \right), $$

(3)

where $\beta > \theta > 0$ are constants such that $U\left( {x,f\left( {\frac{x}{m}} \right)} \right) > 0$.

Proof

Let us denote the ratio $\frac{x}{m}$ by A. LIH implies that

$$ U\left( {cx,f\left( A \right)} \right) = cU\left( {x,f\left( A \right)} \right), $$

(4)

where $c > 0$. This equality holds for all $x > 0$ and $c > 0$. Consequently, for any $c > 0$ it holds for $x = 1$ also.

Now, given $x = 1$, using NOM in (4), we get

$$ U\left( {c.1,f\left( A \right)} \right) = cU\left( {1,f\left( A \right)} \right) = cf\left( A \right). $$

(5)

From (5) it follows that

$$ c = \frac{{U\left( {c,f\left( A \right)} \right)}}{f\left( A \right)}. $$

(6)

Plugging the value of c from (6) into (4) we get

$$ U\left( {cx,f\left( A \right)} \right) = cU\left( {x,f\left( A \right)} \right) = \frac{{U\left( {c,f\left( A \right)} \right)}}{f\left( A \right)}U\left( {x,f\left( A \right)} \right). $$

(7)

Let

$$ V\left( {x,f\left( A \right)} \right) = \frac{{U\left( {x,f\left( A \right)} \right)}}{f\left( A \right)}. $$

(8)

From (7) and (8) it now follows that

$$ \begin{aligned} V\left( {cx,f\left( A \right)} \right) & = \frac{{U\left( {cx,f\left( A \right)} \right)}}{f\left( A \right)} \\ & = \frac{1}{f\left( A \right)}\left[ {\frac{{U\left( {c,f\left( A \right)} \right)}}{f\left( A \right)}U\left( {x,f\left( A \right)} \right)} \right] \\ & = \left[ {\frac{{U\left( {c,f\left( A \right)} \right)}}{f\left( A \right)}} \right]\left[ {\frac{{U\left( {x,f\left( A \right)} \right)}}{f\left( A \right)}} \right] \\ & = V\left( {c,f\left( A \right)} \right)V\left( {x,f\left( A \right)} \right). \\ \end{aligned} $$

(9)

Now, define $g_{f\left( A \right)} \left( x \right) = V\left( {x,f\left( A \right)} \right)$ so that we can rewrite (9) as

$$ g_{f\left( A \right)} \left( {cx} \right) = g_{f\left( A \right)} \left( c \right)g_{f\left( A \right)} \left( x \right). $$

(10)

Given A, by non-constancy of U we rule out the trivial solutions $g_{f\left( A \right)} \left( t \right) = 0$ and $g_{f\left( A \right)} \left( t \right) = 1$ of the functional Eq. (10). Since U (hence g) is positive valued, we can take logarithmic transformation on both sides of (10) to get

$$ \log \left( {g_{f\left( A \right)} \left( {cx} \right)} \right) = \log \left( {g_{f\left( A \right)} \left( c \right)} \right) + \log \left( {g_{f\left( A \right)} \left( x \right)} \right). $$

(11)

Substitution of $c = {\text{e}}^{u}$ and $x = {\text{e}}^{v}$ into (11) yields the functional equation

$$ \log \left( {g_{f\left( A \right)} \left( {{\text{e}}^{u + v} } \right)} \right) = \log \left( {g_{f\left( A \right)} \left( {{\text{e}}^{u} } \right)} \right) + \log \left( {g_{f\left( A \right)} \left( {{\text{e}}^{v} } \right)} \right). $$

(12)

Define $h_{f\left( A \right)} \left( t \right) = log\left( {g_{f\left( A \right)} \left( {{\text{e}}^{t} } \right)} \right)$, where $t \in R$. CON implies continuity of $h_{f\left( A \right)}$. Then the functional Eq. (12) reduces to

$$ h_{f\left( A \right)} \left( {u + v} \right) = h_{f\left( A \right)} \left( u \right) + h_{f\left( A \right)} \left( v \right), $$

(13)

of which the only continuous solution is $h_{f\left( A \right)} \left( u \right) = \delta u$, where $\delta$ is a nonzero constant that depends on $f\left( A \right)$ (Aczel 1966, p. 34). Using $h_{f\left( A \right)} \left( u \right) = \delta u$, in the definition of $h_{f\left( A \right)} \left( t \right)$, we get $log\left( {g_{f\left( A \right)} \left( {{\text{e}}^{u} } \right)} \right) = \delta u$ and with $u = \log t$, it follows that $g_{f\left( A \right)} \left( t \right) = t^{\delta }$.

From the definition of $g_{f\left( A \right)}$ it then follows that

$$ V\left( {x,f\left( A \right)} \right) = x^{{\delta \left( {f\left( A \right)} \right)}} . $$

(14)

Using the definition of $V\left( {x,f\left( A \right)} \right)$ in (14) we get

$$ U\left( {x,f\left( A \right)} \right) = x^{{\delta \left( {f\left( A \right)} \right)}} f\left( A \right). $$

(15)

LIH ensures that $\delta\left( {f\left( A \right)} \right) = 1$, which in turn shows that

$$ U\left( {x,f\left( A \right)} \right) = xf\left( A \right) = xf\left( {\frac{x}{m}} \right). $$

(16)

From (16), by CMR, it now follows that $f^{\prime } \left( {\frac{x}{m}} \right)\frac{{x^{2} }}{{m^{2} }} = - \theta$, which gives $f\left( {\frac{x}{m}} \right) = \beta - \theta \frac{m}{x}$, where $\beta$ is the constant of integration and $f^{\prime }$ is the derivative of f. Substituting this form of f in (16) we get $U\left( {x,f\left( {\frac{x}{m}} \right)} \right) = x\left( {\beta - \frac{\theta m}{x}} \right)$.

Now, when $x = m$, we have $U\left( {m,f(1)} \right) = m(\beta - \theta )$ which becomes positive only when $\beta > \theta$ (since $m > 0$). Since the functional form $U\left( {x,f\left( {\frac{x}{m}} \right)} \right) = x\left( {\beta - \frac{\theta m}{x}} \right)$ holds for all $x \le m$, we must choose $\beta > \theta > 0$ such that U becomes positive unambiguously. This establishes the necessity part of the proposition. The sufficiency can be checked easily. □

Clark and Oswald (1998) specified, without characterization, a utility function which is additively separable in the absolute income x and the relative income $\frac{x}{m}$. However, the functional form we have characterized is of product type in its arguments. The essential idea of dependence of the utility function on the relative as well as absolute statuses is well-maintained in our characterized form also. Further, our form becomes additively separable under the logarithmic transformation.

As in the additive case, we now wish to determine the value of $z_{1}$ such that $U\left( {z_{0} ,f\left( {\frac{{z_{0} }}{{z_{0} }}} \right)} \right) = U\left( {z_{1} ,\frac{{z_{1} }}{m}} \right)$. For the characterised form of $f\left( {\frac{x}{m}} \right)$, in view of (3), this equality becomes, $z_{0} \left( {\beta - \theta } \right) = z_{1} \left( {\beta - \theta \frac{m}{{z_{1} }}} \right)$, from which we get

$$ z_{1} = wz_{0} + \left( {1 - w} \right)m, $$

(17)

where $w = \frac{\beta - \theta }{\beta }$. Since $\beta > \theta > 0$, it follows that $0 < w < 1$. Thus, as in (2), here also the revised poverty line becomes a compound of the existing poverty line and the reference income. The parameter w has the same policy interpretation as in (2). Thus, irrespective of the form of the utility function, we have the same procedure of generating a relative poverty line from an existing poverty line and a reference income. For an observed income distribution, $\beta$ and $\theta$ can be taken as $\beta = \frac{u}{l} + 1$ and $\theta = 1$, where $l > 0$ and u are, respectively, the lower and upper bounds on income. The corresponding utility function turns out to be $x\left( {\beta - \frac{m}{x}} \right)$.

Since in general $m > z_{0}$, and $z_{1}$ is a weighted average of $z_{0}$ and m, it follows that $z_{1} > z_{0}$. Therefore, in order to provide illustrations of our characterized poverty line, we have to choose hybrid poverty lines greater than the absolute poverty line. (See also the discussion below on the suggestions put forward by Atkinson and Bourguignon 2001; EU and Foster 1998).⁶

The choice of the weight w is evidently related to that of the parameters. Assuming that the function f may be written as $f\left( {x/m} \right) = \beta - \left( {m/x} \right)$, we can express U as $U = x\beta - m$, from which we derive that ${\text{d}}U = \frac{\partial U}{\partial x}{\text{d}}x + \frac{\partial U}{\partial m}{\text{d}}m = \beta {\text{d}}x - {\text{d}}m$ so that for a given utility level, $\frac{{{\text{d}}m}}{{{\text{d}}x}} = \beta$.

There are very few papers in the literature on subjective welfare that have estimated the simultaneous impact on happiness, ceteris paribus, of an increase in one’s own income and in that of the reference group’s income. One of these papers is a very recent study by Clark et al. (2013). In Table 4 of their paper the authors report the results of a regression where the dependent variable refers to satisfaction with income. It then appears that the coefficient of own income is about three times as high as that of self-reported reference income, and of opposite sign. This would imply that the value of β is around 3 and, as a consequence, the value of the weight w would be equal to (2/3). We now show that some of the existing suggestions for treating the poverty line as some fraction of the mean or median income can be accommodated in our framework. The EU standard set poverty line as 60% of the median is equivalent to choosing a particular weight for the reference income in our formulation. If we take $\left( {1 - w} \right) = \frac{{0.6m - z_{0} }}{{m - z_{0} }}$ in (17), where in m is the median, then we get the poverty line set by the EU. Likewise, for $\left( {1 - w} \right) = \frac{{0.37m - z_{0} }}{{m - z_{0} }}$, where m now stands for the mean, we get the Atkinson-Bourguignon (2001) relative poverty line.

It will now be worthwhile to compare our proposal with Foster’s (1998) recommendation for a hybrid threshold. If m represents the median, then the threshold $\alpha \, m$, where $0 < \alpha < 1$, is a general relative cutoff (Citro and Michael 1995). If we denote $\alpha \, m$ by $z_{m}$, then Foster (1998) suggested the use of a weighted geometric mean of the absolute threshold $z_{0}$ and the relative threshold $z_{m}$, namely, $z_{0}^{\rho } z_{m}^{1 - \rho }$ as a threshold limit, where $0 < \rho < 1$ is a constant. A 1% increase in the living standard m increases the poverty line by $\rho \%$ (see also Fisher 1995). Now, assume that the individual utility function is of the form $U\left( {x,\frac{x}{m}} \right) = x^{\rho } \left( {\frac{x}{m}} \right)^{1 - \rho }$, $0 < \rho < 1$ is a constant. This utility function is increasing, concave in absolute income but decreasing convex in the reference level. Then our utility-consistency condition reveals that $z_{1} = z_{0}^{\rho } z_{m}^{1 - \rho }$, the hybrid cutoff advocated by Foster (1998). Thus, the Foster proposition can be justified by our utility-consistency condition.

Remark 1

The two forms of U given by $U\left( {x,m} \right) = \left( {k - a} \right)x^{\delta } + am^{\delta }$ and $U\left( {x,m} \right) = x^{\delta } \left( {\beta - \left( {\frac{m}{x}} \right)^{\delta } } \right)$, where $a < 0$, $k > 0$, $0 < \delta < 1$ and $\beta - \left( {\frac{m}{x}} \right)^{\delta } > 0$, are increasing and strictly concave in absolute income but decreasing and strictly convex in reference income. For each of these two specifications of U, by the utility-consistency condition, we have $z_{1} = \left( {sz_{0}^{\delta } + \left( {1 - s} \right)m^{\delta } } \right)^{{\frac{1}{\delta }}}$, where $0 < s < 1$. Thus, we have examples of two different utility functions each of which leads to the same hybrid poverty lines. For $\delta = 1$, $z_{1}$ coincides with (2), whereas as $\delta \to 0$, it becomes Foster’s hybrid poverty line. This form of $z_{1}$ is known as a quasilinear mean. Such a form has been characterized by Chakravarty (2011) as a generalized human development index using several dimensions of human well-being. A similar characterization can be developed in the current context.

We now make a systematic comparison between utility-consistency (equating $U\left( {z_{0} ,0} \right)$ with $U\left( {z_{1} ,z_{1} - m} \right)$, and $U\left( {z_{0} ,f\left( {\frac{{z_{0} }}{{z_{0} }}} \right)} \right)$ with $U\left( {z_{1} ,\frac{{z_{1} }}{m}} \right)$) and the Blackorby–Donaldson (1987) formulation. In their framework, preferences are assumed to be represented by a real valued utility function whose image is $u = U\left( {y,\lambda } \right)$, where U is the utility that each member of the family derives with the characteristic $\lambda \in B$ when the household consumption is y, where B is the set of household characteristics. The parameter $\lambda \in B$ enables to take into account economies of consumption due to household consumption. Household preferences remain unaltered if we consider an increasing function $\overline{U}$ of U, that is, $\overline{U} = L\left( {U\left( {y,\lambda } \right),\lambda } \right)$, where L is increasing in its first argument for all $\lambda \in B$. However, interpersonal comparison of utility cannot be achieved only by household preferences. Some external value judgement has to be imposed on a particular U that makes interpersonal comparisons possible. As Blackorby and Donaldson (1987) pointed out, one such judgement is provided by a set poverty consumption bundles $\left\{ {y\left( \lambda \right)\left| {\lambda \in B} \right.} \right\}$. This judgement requires that $u^{r} = U\left( {y\left( \lambda \right),\lambda } \right)$, for all $\lambda \in B$, where $u^{r}$ is the poverty utility level corresponding to U. This equation becomes meaningful if and only if $L\left( {u^{r} ,\lambda^{1} } \right) = L\left( {u^{r} ,\lambda^{2} } \right)$ for all $\lambda^{1} ,\lambda^{2} \in B$. Given that two utility functions satisfy $u^{r} = U\left( {y\left( \lambda \right),\lambda } \right)$, if they also satisfy $L\left( {u^{r} ,\lambda^{1} } \right) = L\left( {u^{r} ,\lambda^{2} } \right)$, then they are said to fulfil informational invariance for interpersonal comparisons with respect to reference utility indexed by $u^{r}$. As Blackorby and Donaldson (1987) argued, L must be independent of $\lambda$ for interpersonal comparisons to be meaningful.⁷

Thus, the essential idea of equating two utility levels is the same in both the cases. While in our case two utility values are equated to determine a hybrid poverty line, in the Blackorby–Donaldson structure this is done for a given poverty consumption bundle in order to determine the necessary and sufficient condition for interpersonal utility comparison.

3 An Empirical Illustration

In this section, we present several measures of the extent of poverty in rural and urban areas of the People’s Republic of China and India, when an “amalgam poverty line”, a weighted average of an absolute poverty line and of the mean or median income, is introduced. As absolute poverty line, we have used a monthly income of $38 (at 2005 PPP) which corresponds to $1.25 per day, as originally suggested by Ravallion et al. (2009). We assumed various possible weights. More precisely, we supposed that the weight w given to the absolute poverty line [the weight of the median or of the mean being then $\left( {1 - w} \right)$], could be 1, 0.9, 0.66, and 0.5.

The database consisted of information on the income shares of ten deciles in the rural and urban areas of the two countries mentioned previously. Two computation methods were used. The first one is based on an algorithm originally proposed by Kakwani and Podder (1973) allowing one to estimate the Lorenz curve for each country and year on the basis of these 10 observations (income shares). On the basis of this Lorenz curve, it was then easy to find out which percentage of the population had an income (or expenditure level) smaller than that corresponding to some poverty line. The second approach used an algorithm proposed by Shorrocks and Wan (2009), which allows to “ungroup” income distributions, that is, to derive, for example, the share of each centile when the only data available originally are the income shares of deciles.

In Table 1, we present the values of the headcount ratio (in percentage) in the rural and urban areas in the People’s Republic of China and India, under several possible scenarios. We give two sets of results: those based on the Shorrocks and Wan (2009) algorithm (part A) and those derived from the Kakwani and Podder approach (part B). In parentheses, we give also bootstrap confidence intervals. As expected, for a given weight, the headcount ratio is higher when the weight $\left( {1 - w} \right)$ refers to the mean rather than the median. Needless to say, the headcount ratio increases with the weight w. Looking at the bootstrap confidence intervals it appears that these differences are always significant, except in the case of a weight of 90% given to the $38 poverty line when the Kakwani and Podder approach is implemented. In this specific case, the adjusted headcount ratio is the same whether a weight of 10% is given to the mean or the median income. Table 1a, b show also that, whatever weights are selected, the headcount ratio is higher in rural than in urban India. The percentage of poor is also higher in rural than in urban China. These differences are clearly significant, as can be checked by looking at the corresponding confidence intervals. Note also that whereas with the regular $38 poverty line, there is almost no urban poverty in China, when some weight is given to the mean or median income when defining the poverty line, the headcount ratio becomes significant, being even higher than 30% when the weight of the mean is equal to 50%. The differences between the urban and rural sectors are much less striking in India, poverty being quite high in both areas.

Table 1 Headcount ratios (in percentages) under various scenarios

We then combined the data on the headcounts given in Table 1 with the data on the total population around 2010, to derive an estimate of the total number of poor in the urban and rural areas of each of the two countries examined. These results are given in Table 2, together with the corresponding confidence intervals. To simplify the presentation, we give only results based on the Shorrocks and Wan algorithm. It is then easy to compare the number of poor under various scenarios with those obtained on the basis of a weight w equal to 1 (so that the “amalgam poverty line” is also equal to $38). Here also we observe a very important increase in the number of poor in urban areas in China, when the poverty line depends on the median or mean income.

Table 2 Number of poor (in million) in rural and urban areas of the People’s Republic of China and India, depending on the weighting scheme

Finally, Table 3 gives the income gap ratios in the rural and urban areas of the People’s Republic of China and India under the various scenarios, the results being again based on the Shorrocks and Wan algorithm. This index is an indicator of poverty depths of different individuals. Here, also the income gap ratio increases with the weight given to the median or mean income, whether in India or in the People’s Republic of China. The income gap ratio is much smaller in urban than in rural areas of the People’s Republic of China but this is not true for India since when the weight given to the mean or median income becomes higher, the income gap ratio, becomes higher in urban than in rural areas.

Table 3 Poverty Gap Ratios in the rural and urban areas of the People’s Republic of China and of India, depending on the weighting scheme

Note finally that when multiplied by the poverty line and the total number of poor, this summary measure has a direct policy interpretation in the sense that the multiplied formula determines the total amount of money required to put all the poor persons at the poverty line. Now, for a given country and area, with a given poverty line and the reference income, we determine the amalgam poverty line using a specific weighting scheme. Given an amalgam poverty line, we can then directly estimate the amount of money necessary to place the poor persons of a given area in a given country at its poverty line, using the country’s area income gap ratio from Table 3 and the number of poor from Table 2.

4 Conclusions

We have followed Clark and Oswald’s (1998) suggestion that an individual cares about his absolute position (his own income) and his relative position (his own income in comparison with a reference income, such as the mean or the median). Two different forms of the utility function that depend on a person’s absolute and relative statuses have been characterized. These two utility functions have been employed to determine a relative poverty line endogenous to the income distribution. It turns out that in either case, the relative poverty line becomes a combination, a weighted mean, of a given poverty line and a reference income, where the weights add up to one. This is similar in spirit to Foster’s (1998) hybrid poverty threshold, a weighted geometric mean of a relative and an absolute cutoff point. This weight enables a policy maker to express his preference for absolute or relative poverty. Interestingly enough, some of the existing suggestions for the choice of the relative poverty line drop out as special cases of our general approach. The empirical illustration has shown that no matter how we define the “amalgam poverty line” the extent of poverty is generally smaller in the People’s Republic of China than in India.

Appendices

Appendix 1: On Shorrocks and Wan’s (2009) “Ungrouping Income Distributions”

Assume a Lorenz curve with $\left( {m + 1} \right)$ coordinates $(p_{k}^{*} , L_{k}^{*} )$ where $p_{k}^{*}$ and $L_{k}^{*} (k = 1, \ldots,m)$ (refer respectively to the cumulative shares in the total population and in total income of income classes 1 to k, while $p_{0}^{*} = L_{0}^{*} = 0$. These Lorenz coordinates can, for example, refer to decile shares published on a given country. Since often the corresponding average income is not available, it will be assumed to be equal to 1 so that the mean income $\mu_{k}^{*}$ of class k will be expressed as

$$ \mu_{k}^{*} = \frac{{L_{k}^{*} - L_{k - 1}^{*} }}{{p_{k}^{*} - p_{k - 1}^{*} }}\quad k = 1\,,2\,,\ldots.\,.,m. $$

(18)

The goal is to obtain a synthetic sample of n equally weighted observations whose mean value is 1 and which are conform to the original data. These n observations are therefore partitioned into m non-overlapping and ordered groups having each $m_{k} = n\left( {p_{k}^{*} - p_{k - 1}^{*} } \right)$ observations. Call $x_{ki}$ the i^th observation in class k, the sample mean of this class being $\mu_{k}$.

The algorithm proposed by Shorrocks and Wan (2009) includes two stages.

The first step consists of building an initial sample with unit mean which is generated from a parametric form fitted to the grouped data [see, for example, Ryu and Slottje (1999), for a survey of various parameterizations of the Lorenz curve].⁸

In the second stage the algorithm adjusts the observations generated in the initial sample to the true values available from the grouped data. More precisely the initial sample value $x_{j}$, assumed to belong to class k, is transformed into an intermediate value $\widehat{{x_{{\jmath }} }}$ via the following rule:

$$ \frac{{\widehat{{x_{{\jmath }} }} - \mu_{k}^{*} }}{{\mu_{k + 1}^{*} - \mu_{k}^{*} }} = \frac{{x_{j} - \mu_{k} }}{{\mu_{k + 1} - \mu_{k} }}. $$

(19)

For the first class we will write that

$$ \frac{{\widehat{{x_{{\jmath }} }}}}{{\mu_{1}^{*} }} = \frac{{x_{j} }}{{\mu_{1} }}\quad {\text{for}}\quad x_{j} \le \mu_{1}, $$

(20)

while for the last class we have

$$ \frac{{\widehat{{x_{{\jmath }} }}}}{{\mu_{m}^{*} }} = \frac{{x_{j} }}{{\mu_{m} }}\quad {\text{for}}\quad x_{j} \ge \mu_{m}. $$

(21)

Obviously in the next iteration the intermediate values $\widehat{{x_{{\jmath }} }}$ are themselves transformed into new values until the algorithm produces an ordered sample which exactly replicates the properties of the original grouped data. Convergence is in fact very quickly obtained.

Appendix 2: The Kakwani and Podder (1973) Approach

Let L refer to the height of the Lorenz curve (cumulative income share) and z to the corresponding abscissa (cumulative population share). Kakwani and Podder (1973) proposed then the following equation for the Lorenz curve (and showed that such a formulation satisfies all the desired properties of a Lorenz curve):

$$ lnL = - h + lnz + hz. $$

(22)

It is hence possible to derive the value of the parameter h by regressing $lnL$ on $lnz$ and z.

From (22) we also derive that

$$ L = {\text{e}}^{{lnz + h\left( {z - 1} \right)}} = {\text{e}}^{lnz} {\text{e}}^{{h\left( {z - 1} \right)}} = z{\text{e}}^{{h\left( {z - 1} \right)}}. $$

(23)

Remembering that the slope along the Lorenz curve is equal to the ratio of the income corresponding to this point of the Lorenz curve to the mean income, we can apply (23) to the poverty line and write that

$$ \begin{aligned} \frac{\partial L}{\partial z} = \left( {\frac{{\text{poverty\,line}}}{\text{mean}}} \right) & = {\text{e}}^{{h\left( {z - 1} \right)}} + zhe^{{h\left( {z - 1} \right)}} \\ & = {\text{e}}^{{h\left( {z - 1} \right)}} \left( {1 + zh} \right). \\ \end{aligned} $$

(24)

We are therefore looking for the population share z for which the equation below holds

$$ \ln \left( {\frac{{\text{poverty\, line}}}{\text{mean}}} \right) = h\left( {z - 1} \right) + { \ln }\left( {1 + zh} \right), $$

(25)

that is,

$$ \ln \left( {\text{poverty\,line}} \right) = \ln \left( {\text{mean}} \right) + h\left( {z - 1} \right) + { \ln }\left( {1 + zh} \right). $$

(26)

Given the poverty line selected, the mean income and the parameter h determined previously, it is easy to derive the value of z for which (26) holds, that is, the headcount ratio corresponding to the chosen poverty line.