Iwasawa Modules Arising from Deformation Spaces of p-Divisible Formal Group Laws

Abstract

Let G be a p-divisible formal group law over an algebraically closed field of characteristic p. We show that certain equivariant vector bundles on the universal deformation space of G give rise to pseudocompact modules over the Iwasawa algebra of the automorphism group of G. Passing to global rigid analytic sections, we obtain representations which are topologically dual to locally analytic representations. In studying these, one is led to the consideration of divided power completions of universal enveloping algebras. The latter seem to constitute a novel tool in p-adic representation theory.

These keywords were added by machine and not by the authors. This process is experimental and the keywords may be updated as the learning algorithm improves.

1 Introduction

Let p be a prime number, and let k be an algebraically closed field of characteristic p. Let W = W(k) denote the ring of Witt vectors with coefficients in k, and let K denote the quotient field of W. We fix a p-divisible commutative formal group law G of height h over k and denote by $R:= R_{G}^{\mathop{\text{def}}\nolimits }$ the universal deformation ring of G representing isomorphism classes of deformations of G to complete noetherian local W-algebras with residue class field k. Denote by $\mathbb{G}$ the universal deformation of G to R and by $\mathop{\text{Lie}}\nolimits (\mathbb{G})$ the Lie algebra of $\mathbb{G}$. For any integer m, the m-th tensor power $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ of $\mathop{\text{Lie}}\nolimits (\mathbb{G})$ can be viewed as the space of global sections of a vector bundle on the universal deformation space $\mathop{\text{Spf}}\nolimits (R)$ which is equivariant for a natural action of the automorphism group $\varGamma:=\mathop{ \text{Aut}}\nolimits (G)$ of G.

If G is of dimension one, then the formal scheme $\mathop{\text{Spf}}\nolimits (R)$ is known as the moduli space of Lubin-Tate. It plays a crucial role in Harris’ and Taylor’s construction of the local Langlands correspondence for $\mathrm{GL}_{h}(\mathbb{Q}_{p})$. Moreover, the Γ-representations $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ and their cohomology figure prominently in stable homotopy theory (cf. the introduction to Devinatz and Hopkins (1995)). Still assuming G to be one dimensional, a detailed study of the Γ-representation R was given in Kohlhaase (2013). For h = 2 it led to the computation of the continuous Γ-cohomology of R, relying on the foundational work of Devinatz, Gross, Hopkins and Yu. The only prior analysis of p-adic representations stemming from equivariant vector bundles on deformation spaces of p-divisible formal groups concern the p-adic symmetric spaces of Drinfeld. These were studied extensively by Morita, Orlik, Schneider and Teitelbaum (cf. Orlik 2008; Schneider and Teitelbaum 2002 and our remarks at the end of Sect. 2).

The aim of the present article is to generalize and strengthen some of the results of Gross and Hopkins (1994) and of the author in Kohlhaase (2013). To this end, Sect. 1 and the first part of Sect. 2 give a survey of the theory of p-divisible commutative formal group laws. This includes the classification results of Dieudonné, Lazard and Manin, as well as the deformation theoretic results of Cartier, Lubin, Tate and Umemura. It follows from the work of Dieudonné and Manin that the group Γ is a compact Lie group over $\mathbb{Q}_{p}$ (cf. Corollary 1).

In the second part of Sect. 2, we prove that the action of Γ on $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ extends to the Iwasawa algebra $\varLambda:= W[\![\varGamma ]\!]$ of Γ over W. This gives $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ the structure of a pseudocompact module over Λ (cf. Corollary 2 and Theorem 5). In Sect. 3, we pass to the global rigid analytic sections $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ of our vector bundles and show that the action of Γ extends to a continuous action of the locally analytic distribution algebra D(Γ) of Γ over K. As a consequence, the action of Γ on the strong continuous K-linear dual of $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ is locally analytic in the sense of Schneider and Teitelbaum (cf. Theorems 6 and 7).

We note that the continuity and the differentiability of the action of Γ on $R^{\mathop{\text{rig}}\nolimits }$ were first proven by Gross and Hopkins if G is of dimension one (cf. Gross and Hopkins 1994, Propositions 19.2 and 24.2). Using the structure theory of the algebra D(Γ), we arrive at a more precise result for arbitrary m and G, avoiding the use of the period morphism. Our approach essentially relies on a basic lifting lemma for endomorphisms of G which is also at the heart of the strategy followed by Gross and Hopkins (cf. Lemma 1 and Proposition 1).

A major question that we have to leave open concerns the coadmissibility of the D(Γ)-modules $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ in the sense of Schneider and Teitelbaum (2003), section 6. Taking sections over suitable affinoid subdomains of $\mathop{\text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits }$, it is related to the finiteness properties of the resulting Banach spaces as modules over certain Banach completions of Λ ⊗ _W K. In Sect. 4, we assume G to be of dimension one and consider the restriction of $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ to an affinoid subdomain of $\mathop{\text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits }$ over which the period morphism of Gross and Hopkins is an open immersion. By spelling out the action of the Lie algebra of Γ, we show that one naturally obtains a continuous module over a complete divided power enveloping algebra $\hat{U}_{K}^{\mathop{\text{dp}}\nolimits }(\mathring{ \mathfrak{g}})$ constructed by Kostant (cf. Theorem 8). Here $\mathring {\mathfrak{g}}$ is a Chevalley order in the split form of the Lie algebra of Γ. If h = 2 and m ≥ −1 then in fact $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ gives rise to a cyclic module over $\hat{U}_{K}^{\mathop{\text{dp}}\nolimits }(\mathring {\mathfrak{g}})$ (cf. Theorem 9). This result might indicate that $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ does not give rise to a coherent sheaf for the Fréchet-Stein structure of D(Γ) considered in Schneider and Teitelbaum (2003), section 5 (cf. Remark 3).

Gross and Hopkins (1994) consider formal modules of dimension one and finite height over the valuation ring $\mathfrak{o}$ of an arbitrary non-Archimedean local field. The case of p-divisible formal groups corresponds to the case $\mathfrak{o} = \mathbb{Z}_{p}$. However, neither the deformation theory nor the theory of the period morphism have been worked out in detail for formal $\mathfrak{o}$-modules of dimension strictly greater than one. This is why we restrict to one dimensional formal groups in Sect. 4 and to p-divisible formal groups throughout.

Conventions and notation. If S is a commutative unital ring, if r is a positive integer, and if X = (X ₁, …, X _r ) is a family of indeterminates, then we denote by $S[\![X]\!] = S[\![X_{1},\ldots,X_{r}]\!]$ the ring of formal power series in the variables X ₁, …, X _r over S. We write $f = f(X) = f(X_{1},\ldots,X_{r})$ for an element $f \in S[\![X]\!]$. If $n = (n_{1},\ldots,n_{r}) \in \mathbb{N}^{r}$ is an r-tuple of non-negative integers then we set $\vert n\vert:= n_{1} +\ldots +n_{r}$ and $X^{n}:= X_{1}^{n_{1}}\cdots X_{r}^{n_{r}}$. If i and j are elements of a set then we denote by δ _ij the Kronecker symbol with value 1 ∈ S if i = j and 0 ∈ S if i ≠ j. If $\mathfrak{h}$ is a Lie algebra over S then we denote by $U(\mathfrak{h})$ the universal enveloping algebra of $\mathfrak{h}$ over S. Throughout the article, p will denote a fixed prime number.

2 Formal Group Laws

Let R be a commutative unital ring, and let d be a positive integer. A d-dimensional commutative formal group law (subsequently abbreviated to formal group) is a d-tuple G = (G ₁, …, G _d ) of formal power series in 2d variables $G_{i} \in R[\![X,Y ]\!] = R[\![X_{1},\ldots,X_{d},Y _{1},\ldots,Y _{d}]\!]$, satisfying

• (F1) G _i (X, 0) = X _i ,

• (F2) G _i (X, Y ) = G _i (Y, X), and

• (F3) G _i (G(X, Y ), Z) = G _i (X, G(Y, Z))

for all 1 ≤ i ≤ d. It follows from the formal implicit function theorem (cf. Hazewinkel 1978, A.4.7) that for a given d-dimensional commutative formal group G there exists a unique d-tuple $\iota _{G} \in R[\![X]\!]^{d}$ of formal power series with trivial constant terms such that

$$\displaystyle{G_{i}(X,\iota _{G}(X)) = 0\quad \mbox{ for all}\quad 1 \leq i \leq d}$$

(cf. also Zink 1984, Korollar 1.5). Thus, if S is a commutative R-algebra, and if I is an ideal of S such that S is I-adically complete, then the set I ^d becomes a commutative group with unit element (0, …, 0) via

$$\displaystyle{x + _{G}y:= G(x,y)\quad \mbox{ and}\quad - x:=\iota _{G}(x).}$$

Example 1.

Let $R = \mathbb{Z}$ and d = 1. The formal group $\hat{\mathbb{G}}_{a}(X,Y ) = X + Y$ is called the one dimensional additive formal group. We have $\iota _{\hat{\mathbb{G}}_{a}}(X) = -X$. The formal group $\hat{\mathbb{G}}_{m}(X,Y ) = (1 + X)(1 + Y ) - 1$ is called the one dimensional multiplicative formal group. We have $\iota _{\hat{\mathbb{G}}_{m}}(X) =\sum _{ n=1}^{\infty }(-X)^{n}$.

Let G and H be formal groups over R of dimensions d and e, respectively. A homomorphism from G to H is an e-tuple $\varphi = (\varphi _{1},\ldots,\varphi _{e})$ of power series $\varphi _{i} \in R[\![X]\!] = R[\![X_{1},\ldots,X_{d}]\!]$ in d-variables over R with trivial constant terms, satisfying

$$\displaystyle{\varphi (G(X,Y )) = H(\varphi (X),\varphi (Y )).}$$

If $\varphi: G \rightarrow G'$ and ψ: G′ → G″ are homomorphisms of formal groups then we define $\psi \circ \varphi$ through $(\psi \circ \varphi )(X):=\psi (\varphi (X))$. This is a homomorphism from G to G″. We let $\mathop{\text{End}}\nolimits (G)$ denote the set of endomorphisms of a d-dimensional commutative formal group G over R, i.e. of homomorphisms from G to G. It is a ring with unit $1_{G} = X = (X_{1},\ldots,X_{d})$, in which addition and multiplication are defined by $(\varphi +_{G}\psi )(X):= G(\varphi (X),\psi (X))$, $(-\varphi )(X):=\iota _{G}(\varphi (X))$ and $\psi \cdot \varphi:=\psi \circ \varphi$. In particular, $\mathop{\text{End}}\nolimits (G)$ is a $\mathbb{Z}$-module. Given $m \in \mathbb{Z}$, we denote by $[m]_{G} \in R[\![X]\!]^{d}$ the corresponding endomorphism of G. We denote by $\mathop{\text{Aut}}\nolimits (G)$ the automorphism group of G, i.e. the group of units of the ring $\mathop{\text{End}}\nolimits (G)$.

Denoting by (X) the ideal of $R[\![X]\!]$ generated by X ₁, …, X _d , the free R-module

$$\displaystyle{\mathop{\text{Lie}}\nolimits (G):=\mathop{ \text{Hom}}\nolimits _{R}((X)/(X)^{2},R)}$$

of rank $d =\dim (G)$ is called the Lie algebra of G (or its tangent space at 1 _G). It is an R-Lie algebra for the trivial Lie bracket. Non-commutative Lie algebras occur only for non-commutative formal groups (cf. Zink 1984, Kapitel I.7). An R-basis of $\mathop{\text{Lie}}\nolimits (G)$ is given by the linear forms $( \frac{\partial } {\partial X_{i}})_{1\leq i\leq d}$ sending f + (X)² to $\frac{\partial f} {\partial X_{i}}(0)$. Here $\frac{\partial f} {\partial X_{i}}$ denotes the formal derivative of the power series f with respect to the variable X _i .

Any homomorphism $\varphi: G \rightarrow H$ of formal groups as above gives rise to an R-linear ring homomorphism $\varphi ^{{\ast}}: R[\![Y _{1},\ldots,Y _{e}]\!] \rightarrow R[\![X_{1},\ldots,X_{d}]\!]$, determined by $\varphi ^{{\ast}}(Y _{i}) =\varphi _{i}$ for all 1 ≤ i ≤ e. It is called the comorphism of $\varphi$. It maps (Y ) to (X), hence (Y )² to (X)², and therefore induces an R-linear map

$$\displaystyle{\mathop{\text{Lie}}\nolimits (\varphi ):\mathop{ \text{Lie}}\nolimits (G)\longrightarrow \mathop{\text{Lie}}\nolimits (H)}$$

via $\mathop{\text{Lie}}\nolimits (\varphi )(\delta )(h + (Y )^{2}):=\delta (\varphi ^{{\ast}}(h) + (X)^{2})$. In the R-bases $( \frac{\partial } {\partial X_{i}})_{i}$ (resp. $( \frac{\partial } {\partial Y _{j}})_{j}$) of $\mathop{\text{Lie}}\nolimits (G)$ (resp. $\mathop{\text{Lie}}\nolimits (H)$), the map $\mathop{\text{Lie}}\nolimits (\varphi )$ is given by the Jacobian matrix $( \frac{\partial \varphi _{i}} {\partial X_{j}}(0))_{i,j} \in R^{e\times d}$ of $\varphi$. If $\varphi: G \rightarrow G'$ and $\psi: G' \rightarrow G''$ are homomorphisms of formal groups, then $(\psi \circ \varphi )^{{\ast}} =\varphi ^{{\ast}}\circ \psi ^{{\ast}}$ and $\mathop{\text{Lie}}\nolimits (\psi \circ \varphi ) =\mathop{ \text{Lie}}\nolimits (\psi ) \circ \mathop{\text{Lie}}\nolimits (\varphi )$. If H = G then one can use (F1) to show that the map $(\varphi \mapsto \mathop{\text{Lie}}\nolimits (\varphi )):\mathop{ \text{End}}\nolimits (G) \rightarrow \mathop{\text{End}}\nolimits _{R}(\mathop{\text{Lie}}\nolimits (G))$ is a homomorphism of rings. In particular, $\mathop{\text{Lie}}\nolimits (G)$ becomes a module over $\mathop{\text{End}}\nolimits (G)$ and we have $\mathop{\text{Lie}}\nolimits ([m]_{G}) = m \cdot \mathrm{id}_{\mathop{\text{Lie}}\nolimits (G)}$ for any integer m.

If p is a prime number and if R is a complete noetherian local ring of residue characteristic p, then a homomorphism $\varphi: G \rightarrow H$ of formal groups is called an isogeny if the comorphism $\varphi ^{{\ast}}$ makes $R[\![X]\!]$ a finite free module over $R[\![Y ]\!]$ (cf. Tate 1967, section 2.2). Of course, this can only happen if d = e. A formal group G over a complete noetherian local ring R with residue characteristic p is called p-divisible, if the homomorphism [p] _G : G → G is an isogeny. In this case the rank of $R[\![X]\!]$ over itself via [p] _G ^∗ is a power of p, say p ^h (cf. Tate 1967, section 2.2; this result can also be deduced from Zink 1984, Satz 5.3). The integer $h =:\mathop{ \text{ht}}\nolimits (G)$ is called the height of the p-divisible formal group G.

If R = k is a perfect field of characteristic p, the necessary tools to effectively study the category of p-divisible commutative formal groups over k were first developed by Demazure (1986, Chapter III). His methods were later generalized by Cartier in order to describe commutative formal groups over arbitrary rings (cf. Lazard 1975, Chapters III & IV, or Zink 1984, Chapters III & IV).

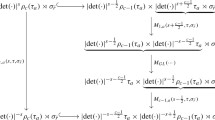
Sticking to the case of a perfect field k of characteristic p, we denote by W: = W(k) the ring of Witt vectors over k. Let σ = (x ↦ x ^p) denote the Frobenius automorphism of k, as well as its unique lift to a ring automorphism of W. Recall that a σ ⁻¹ -crystal over k is a pair (M, V ), consisting of a finitely generated free W-module M and a map V: M → M which is σ ⁻¹-linear, i.e. which is additive and satisfies

$$\displaystyle{V (am) =\sigma ^{-1}(a)V (m)\quad \mbox{ for all}\quad a \in W,\;m \in M.}$$

We shall be interested in those σ ⁻¹-crystals (M, V ) which satisfy the following two extra conditions (here D stands for Dieudonné):

• (D1) pM ⊆ V (M)

• (D2) V mod p is a nilpotent endomorphism of M∕pM.

For the following fundamental result cf. Zink (1984), page 109.

Theorem 1 (Dieudonné).

If k is a perfect field of characteristic p then the category of p-divisible commutative formal groups over k is equivalent to the category of σ ⁻¹ -crystals over k, satisfying (D1) and (D2).□

Let W[F, V ] be the non-commutative ring generated by two elements F and V over W subject to the relations

$$\displaystyle{V F = FV = p,\quad V a =\sigma ^{-1}(a)V \quad \mbox{ and}\quad Fa =\sigma (a)F\quad \mbox{ for all}\quad a \in W.}$$

The equivalence of Theorem 1 associates with a p-divisible commutative formal group G its (covariant) Cartier-Dieudonné module M _G. This is a V -adically separated and complete module over W[V, F] such that the action of V is injective. Since G is p-divisible, also the action of F is injective, and the underlying W-module of M _G is finitely generated and free. In particular, the pair (M _G , V ) is a σ ⁻¹-crystal over k, satisfying pM _G = VFM _G ⊆ VM _G , i.e. condition (D1). Condition (D2) follows from the V -adic completeness of M _G . We also note that V and F give rise to a short exact sequence

of k-vector spaces in which $\dim _{k}(M_{G}/pM_{G}) =\mathop{ \text{ht}}\nolimits (G)$ and $\dim _{k}(M_{G}/V M_{G}) =\dim (G)$.

Conversely, if (M, V ) is a σ ⁻¹-crystal over k satisfying (D1), then V is injective. In fact, (D1) implies that V becomes surjective (and hence bijective) over the quotient field K of W. Setting $F:= V ^{-1}p$, the W-module M becomes a module over W[F, V ] which is V -adically separated and complete if condition (D2) is satisfied.

Recall that a σ ⁻¹ -isocrystal over k is a pair (N, f) consisting of a finite dimensional K-vector space N and a σ ⁻¹-linear bijection f: N → N. If (M, V ) is a σ ⁻¹-crystal over k which satisfies (D1) then $(M \otimes _{W}K,V \otimes \mathrm{id}_{K})$ is a σ ⁻¹-isocrystal over k. The σ ⁻¹-isocrystal which in this way is associated with the Cartier-Dieudonné module of a p-disivible commutative formal group G over k, classifies G up to isogeny (cf. Zink 1984, Satz 5.26 and the remarks on page 110; alternatively, consult Demazure 1986, Chapter IV.1).

Given integers r and s with r > 0, consider the σ ⁻¹-isocrystal over k given by $(K[t]/(t^{r} - p^{s}),t\circ \sigma )$. Here K[t] denotes the usual commutative polynomial ring in the variable t over K on which σ acts coefficientwise. If k is algebraically closed, we have the following fundamental classification result of Dieudonné and Manin (cf. Zink 1984, Satz 6.29; Demazure 1986, Chapter IV.4; Lazard 1975, Proposition VI.7.42).

Theorem 2 (Dieudonné-Manin).

If k is an algebraically closed field of characteristic p then the category of σ ⁻¹ -isocrystals over k is semisimple. The simple objects are given by the σ ⁻¹ -isocrystals $(K[t]/(t^{r} - p^{s}),t\circ \sigma )$ , where r and s are relatively prime integers with r > 0. □

To a pair (r, s) of integers as in Theorem 2 corresponds a particular p-divisible commutative formal group G _rs over k inside the isogeny class determined by the σ ⁻¹-isocrystal $(K[t]/(t^{r} - p^{s}),t\circ \sigma )$. According to Lazard (1975), Proposition VI.7.42, the endomorphism ring of G _rs is isomorphic to the maximal order of the central division algebra of invariant $\frac{s} {r} + \mathbb{Z} \in \mathbb{Q}/\mathbb{Z}$ and dimension r ² over $\mathbb{Q}_{p}$.

Corollary 1.

If G is a p-divisible commutative formal group over an algebraically closed field k of characteristic p then the endomorphism ring $\mathop{\text{End}}\nolimits (G)$ of G is an order in a finite dimensional semisimple $\mathbb{Q}_{p}$ -algebra. Endowing $\mathop{\text{End}}\nolimits (G)$ with the p-adic topology and the automorphism group $\mathop{\text{Aut}}\nolimits (G)$ of G with the induced topology, $\mathop{\text{Aut}}\nolimits (G)$ is a compact Lie group over $\mathbb{Q}_{p}$ .

Proof.

That $\mathop{\text{End}}\nolimits (G)$ is a p-adically separated and torsion free $\mathbb{Z}_{p}$-module can easily be proved directly, using that G is p-divisible. It also follows from the fact that the Cartier-Dieudonné module of G is free over W. According to Theorem 2 and the subsequent remarks there are central division algebras D ₁, …, D _n over $\mathbb{Q}_{p}$ and natural numbers m ₁, …, m _n such that

$$\displaystyle{\mathop{\text{End}}\nolimits (G) \otimes _{\mathbb{Z}_{p}}\mathbb{Q}_{p} \simeq \mathrm{ Mat}(m_{1} \times m_{1},D_{1}) \times \ldots \times \mathrm{Mat}(m_{n} \times m_{n},D_{n})}$$

as $\mathbb{Q}_{p}$-algebras. Since $\mathop{\text{End}}\nolimits (G)$ is p-adically separated, it is bounded in $\mathop{\text{End}}\nolimits (G) \otimes _{\mathbb{Z}_{p}}\mathbb{Q}_{p}$. Thus, it is a lattice in a finite dimensional $\mathbb{Q}_{p}$-vector space and must be finitely generated over $\mathbb{Z}_{p}$. This proves the first assertion. Endowing $\mathop{\text{End}}\nolimits (G)$ with the p-adic topology, it becomes a topological $\mathbb{Z}_{p}$-algebra and $\mathop{\text{Aut}}\nolimits (G)$ becomes a compact topological group for the subspace topology. By the above arguments, it is isomorphic to an open subgroup of $\prod _{i=1}^{n}\mathrm{GL}_{m_{i}}(D_{i})$, hence naturally carries the structure of a Lie group over $\mathbb{Q}_{p}$.

3 Deformation Problems and Iwasawa Modules

We continue to denote by k a fixed algebraically closed field of characteristic p. We also fix a p-divisible commutative formal group G of dimension d over k. Denote by W = W(k) the ring of Witt vectors of k and by $\mathcal{C}_{k}$ the category of complete noetherian commutative local W-algebras with residue class field k. Let R be an object of $\mathcal{C}_{k}$ and let $\mathfrak{m}$ be the maximal ideal of R. A deformation of G to R is a pair (G′, ρ _G′), where G′ is commutative formal group over R and $\rho _{G'}: G \rightarrow G'\,\mathrm{mod}\,\mathfrak{m}$ is an isomorphism of formal groups over k. Two deformations (G′, ρ _G′) and (G″, ρ _G″) of G to R are said to be isomorphic if there is an isomorphism f: G′ → G″ of formal groups over R such that the diagram

is commutative. Let $\mathop{\text{Def}}\nolimits _{G}$ denote the functor from $\mathcal{C}_{k}$ to the category $\mathit{Sets}$ of sets which associates with an object R of $\mathcal{C}_{k}$ the set of isomorphism classes of deformations of G to R. If $\dim (G) = 1$, then the following theorem was first proved by Lubin and Tate (1966, Theorem 3.1), building on the work of Lazard. It was later generalized by Cartier und Umemura, independently (cf. Cartier 1968,1969; Umemura 1977).

Theorem 3.

The functor $\mathop{\text{Def}}\nolimits _{G}: \mathcal{C}_{k} \rightarrow \mathit{Sets}$ is representable, i.e. there is an object $R_{G}^{\mathop{\text{def}}\nolimits }$ of $\mathcal{C}_{k}$ and a deformation $\mathbb{G}$ of G to $R_{G}^{\mathop{\text{def}}\nolimits }$ with the following universal property. For any object R of $\mathcal{C}_{k}$ and any deformation (G′,ρ _G′ ) of G to R there is a unique W-linear local ring homomorphism $\varphi: R_{G}^{\mathop{\text{def}}\nolimits } \rightarrow R$ and a unique isomorphism $[\varphi ]:\varphi _{{\ast}}(\mathbb{G},\rho _{\mathbb{G}}) \simeq (G',\rho _{\mathbb{G}'})$ of deformations of G to R. ¹ If $h =\mathop{ \text{ht}}\nolimits (G)$ and $d =\dim (G)$ denote the height and the dimension of G, respectively, then the W-algebra $R_{G}^{\mathop{\text{def}}\nolimits }$ is non-canonically isomorphic to the power series ring $W[\![u_{1},\ldots,u_{(h-d)d}]\!]$ in (h − d)d variables over W. □

It follows from the universal property of the deformation $(\mathbb{G},\rho _{\mathbb{G}})$ that the automorphism group $\mathop{\text{Aut}}\nolimits (G)$ of G acts on the universal deformation ring $R_{G}^{\mathop{\text{def}}\nolimits }$ by W-linear local ring automorphisms. Indeed, given $\gamma \in \mathop{\text{Aut}}\nolimits (G)$, there is a unique W-linear local ring endomorphism γ of $R_{G}^{\mathop{\text{def}}\nolimits }$ and a unique isomorphism $[\gamma ]:\gamma _{{\ast}}(\mathbb{G},\rho _{\mathbb{G}}) \simeq (\mathbb{G},\rho _{\mathbb{G}}\circ \gamma )$ of deformations of G to $R_{G}^{\mathop{\text{def}}\nolimits }$. It follows from the uniqueness that the resulting map $\mathop{\text{Aut}}\nolimits (G) \rightarrow \mathop{\text{End}}\nolimits (R_{G}^{\mathop{\text{def}}\nolimits })$ factors through a homomorphism

$$\displaystyle{\mathop{\text{Aut}}\nolimits (G)\longrightarrow \mathop{\text{Aut}}\nolimits (R_{G}^{\mathop{\text{def}}\nolimits })}$$

of groups. It is this type of representation that we are concerned with in this article. To ease notation we shall denote by

$$\displaystyle{R:= R_{G}^{\mathop{\text{def}}\nolimits }}$$

the universal deformation ring of our fixed p-divisible commutative formal group G over k. Let $\mathfrak{m}$ denote the maximal ideal of R. For any non-negative integer n we denote by $\mathbb{G}_{n}:= \mathbb{G}\,\mathrm{mod}\,\mathfrak{m}^{n+1}$ the reduction of the universal deformation $\mathbb{G}$ modulo the ideal $\mathfrak{m}^{n+1}$ of R. We have $G \simeq \mathbb{G}_{0}$ via $\rho _{\mathbb{G}}$.

Lemma 1.

If n is a non-negative integer then the ring homomorphism $\mathop{\text{End}}\nolimits (\mathbb{G}_{n+1}) \rightarrow \mathop{\text{End}}\nolimits (\mathbb{G}_{n})$ , induced by reduction modulo $\mathfrak{m}^{n+1}$ , is injective.

Proof.

The formal group $\mathbb{G}_{n+1}$ is p-divisible because the comorphism $[p]_{\mathbb{G}_{n+1}}^{{\ast}}$ is finite and free. Indeed, it is so after reduction modulo $\mathfrak{m}$, and one can use Bourbaki (2006), III.2.1 Proposition 14 and III.5.3 Théorème 1, to conclude. Since the ideal $\mathfrak{m}^{n+1}(R/\mathfrak{m}^{n+2})$ of $R/\mathfrak{m}^{n+2}$ is nilpotent, the claim follows from the rigidity theorem in Zink (1984), Satz 5.30.

The preceding lemma allows us to regard all endomorphism rings $\mathop{\text{End}}\nolimits (\mathbb{G}_{n})$ as subrings of $\mathop{\text{End}}\nolimits (\mathbb{G}_{0})$. The main technical result of this section is the following assertion.

Proposition 1.

For any non-negative integer n the subring $\mathop{\text{End}}\nolimits (\mathbb{G}_{n})$ of $\mathop{\text{End}}\nolimits (\mathbb{G}_{0})$ contains $p^{n}\mathop{ \text{End}}\nolimits (\mathbb{G}_{0})$ .

Proof.

We proceed by induction on n, the case n = 0 being trivial. Let n ≥ 1 and assume the assertion to be true for n − 1. Set $R_{n}:= R/\mathfrak{m}^{n+1}$. Let $\varphi \in p^{n-1}\mathop{ \text{End}}\nolimits (\mathbb{G}_{0}) \subseteq \mathop{\text{End}}\nolimits (\mathbb{G}_{n-1})$ and choose a family $\tilde{\varphi }\in R_{n}[\![X]\!]^{d}$ of power series with trivial constant terms such that $\tilde{\varphi }\,\mathrm{mod}\,\mathfrak{m}^{n}R_{n} =\varphi$. The d-tuple of power series $[p]_{\mathbb{G}_{n}}\circ \tilde{\varphi }$ is then a lift of $p\varphi$. We claim that it is an endomorphism of $\mathbb{G}_{n}$.

Note first that $[p]_{\mathbb{G}_{n}}\circ \tilde{\varphi }$ depends only on $\varphi$ and not on the choice of a lift $\tilde{\varphi }$. Indeed, if $\tilde{\varphi }'$ is a second lift of $\varphi$ with trivial constant terms, set $\psi:=\tilde{\varphi } '-\tilde{\varphi }$. Setting $\chi:= (\tilde{\varphi }+\psi ) -_{\mathbb{G}_{n}}\tilde{\varphi }$, we have $\tilde{\varphi }' =\tilde{\varphi } +_{\mathbb{G}_{n}}\chi$. Further, the power series χ satisfies $\chi \,\mbox{ mod}\,\mathfrak{m}^{n} =\varphi -_{\mathbb{G}_{n-1}}\varphi = 0$, hence has coefficients in $\mathfrak{m}^{n}R_{n}$. Since $p\mathfrak{m}^{n} \subseteq \mathfrak{m}^{n+1}$ and $(\mathfrak{m}^{n})^{m} \subseteq \mathfrak{m}^{n+1}$ for any integer m ≥ 2, we have $[p]_{\mathbb{G}_{n}}\circ \chi = 0$ and hence

$$\displaystyle{[p]_{\mathbb{G}_{n}} \circ \tilde{\varphi }' = [p]_{\mathbb{G}_{n}}(\tilde{\varphi }+_{\mathbb{G}_{n}}\chi ) = ([p]_{\mathbb{G}_{n}}\circ \tilde{\varphi }) + _{\mathbb{G}_{n}}([p]_{\mathbb{G}_{n}}\circ \chi ) = [p]_{\mathbb{G}_{n}}\circ \tilde{\varphi },}$$

as desired.

If $\eta \in R_{n}[\![X]\!]^{d}$ is a family of power series with trivial constant terms, set $\delta _{\eta }:=\delta _{\eta }(X,Y ):=\eta (X + _{\mathbb{G}_{n}}Y ) -_{\mathbb{G}_{n}}\eta (X) -_{\mathbb{G}_{n}}\eta (Y )$. Since $\tilde{\varphi }$ reduces to an endomorphism of $\mathbb{G}_{n-1}$, the power series $\delta _{\tilde{\varphi }}$ has coefficients in $\mathfrak{m}^{n}$. As above, this implies $[p]_{\mathbb{G}_{n}} \circ \delta _{\tilde{\varphi }} = 0$ and thus

$$\displaystyle\begin{array}{rcl} \delta _{[p]_{\mathbb{G}_{ n}}\circ \tilde{\varphi }}& =& ([p]_{\mathbb{G}_{n}}\circ \tilde{\varphi })(X + _{\mathbb{G}_{n}}Y ) -_{\mathbb{G}_{n}}([p]_{\mathbb{G}_{n}}\circ \tilde{\varphi })(X) -_{\mathbb{G}_{n}}([p]_{\mathbb{G}_{n}}\circ \tilde{\varphi })(Y ) {}\\ & =& [p]_{\mathbb{G}_{n}}(\delta _{\tilde{\varphi }}) = 0. {}\\ \end{array}$$

As a consequence, $[p]_{\mathbb{G}_{n}}\circ \tilde{\varphi }\in \mathop{\text{End}}\nolimits (\mathbb{G}_{n})$, and thus $p\varphi \in \mathop{\text{End}}\nolimits (\mathbb{G}_{n})$. Since $\varphi$ was arbitrary, we obtain the desired inclusion $p^{n}\mathop{ \text{End}}\nolimits (\mathbb{G}_{0}) \subseteq \mathop{\text{End}}\nolimits (\mathbb{G}_{n})$.

According to Corollary 1, the group $\mathop{\text{Aut}}\nolimits (G)$ is a profinite topological group. A basis of open neighborhoods of its identity is given by the subgroups $1 + p^{n}\mathop{ \text{End}}\nolimits (G)$ with n ≥ 1. If $\mathfrak{m}$ denotes the maximal ideal of the local ring R, the W-algebra R is a topological ring for the $\mathfrak{m}$-adic topology. We are now ready to prove the following result, a particular case of which was treated in Kohlhaase (2013), Proposition 3.1. The argument is borrowed from the proof of Gross and Hopkins (1994), Lemma 19.3. Let us put

$$\displaystyle{\varGamma:=\varGamma _{0}:=\mathop{ \text{Aut}}\nolimits (G)\quad \mbox{ and}\quad \varGamma _{n}:= 1 + p^{n}\mathop{ \text{End}}\nolimits (G)\quad \mbox{ for}\quad n \geq 1.}$$

Theorem 4.

The action of Γ on $R = R_{G}^{\mathop{\text{def}}\nolimits }$ is continuous in the sense that the map $((\gamma,f)\mapsto \gamma (f)):\varGamma \times R \rightarrow R$ is a continuous map of topological spaces. Here Γ × R carries the product topology. If n is a non-negative integer then the induced action of Γ _n on $R/\mathfrak{m}^{n+1}$ is trivial.

Proof.

As in the proof of Kohlhaase (2013), Proposition 3.1, it suffices to prove the second statement. Let γ ∈ Γ _n and consider the deformation $(\mathbb{G}_{n},\rho _{\mathbb{G}}\circ \gamma )$ of G to $R_{n} = R/\mathfrak{m}^{n+1}$. Denote by $\mathrm{pr}_{n}: R \rightarrow R_{n}$ the natural projection and let γ _n denote the unique ring homomorphism γ _n : R → R _n for which there exists an isomorphism of deformations $[\gamma _{n}]: (\gamma _{n})_{{\ast}}(\mathbb{G},\rho _{\mathbb{G}}) \simeq (\mathbb{G}_{n},\rho _{\mathbb{G}}\circ \gamma )$ (cf. Theorem 3). Note that also the ring homomorphism pr _n ∘γ: R → R _n admits an isomorphism of deformations $(\mathrm{pr}_{n}\circ \gamma )_{{\ast}}(\mathbb{G},\rho _{\mathbb{G}}) \simeq (\mathbb{G}_{n},\rho _{\mathbb{G}}\circ \gamma )$, namely the reduction of [γ] modulo $\mathfrak{m}^{n+1}$. By uniqueness, we must have γ _n = pr _n ∘γ and $[\gamma _{n}] = [\gamma ]\mod \mathfrak{m}^{n+1}$.

Since the map $(\sigma \mapsto \rho _{\mathbb{G}} \circ \sigma \circ \rho _{\mathbb{G}}^{-1})$ is a ring isomorphism $\mathop{\text{End}}\nolimits (G) \rightarrow \mathop{\text{End}}\nolimits (\mathbb{G}_{0})$, Proposition 1 shows that $\rho _{\mathbb{G}} \circ \gamma \circ \rho _{\mathbb{G}}^{-1} \in \mathop{\text{Aut}}\nolimits (\mathbb{G}_{n})$ and therefore defines an isomorphism of deformations $(\mathrm{pr}_{n})_{{\ast}}(\mathbb{G},\rho _{\mathbb{G}}) = (\mathbb{G}_{n},\rho _{\mathbb{G}}) \simeq (\mathbb{G}_{n},\rho _{\mathbb{G}}\circ \gamma )$. By uniqueness again, we must have $\gamma _{n} =\mathrm{ pr}_{n}\circ \gamma =\mathrm{ pr}_{n}$. This implies that γ acts trivially on R _n and that $[\gamma ]\mod \mathfrak{m}^{n+1} =\rho _{\mathbb{G}} \circ \gamma \circ \rho _{\mathbb{G}}^{-1}$.

If H is a profinite topological group then we denote by

$$\displaystyle{\varLambda (H):= W[\![H]\!]:= \mathop{\lim }\limits_\longleftarrow _{n\geq 1,\,N\unlhd _{o}H}(W/p^{n}W)[H/N]}$$

the Iwasawa algebra (or completed group ring) of H over W. The above projective limit runs over all positive integers n and over all open normal subgroups N of H. If n and n′ are positive integers with n′ ≤ n, and if N and N′ are two open normal subgroups of H with N ⊆ N′, then the transition map $(W/p^{n}W)[H/N] \rightarrow (W/p^{n'}W)[H/N']$ is the natural homomorphism of group rings induced by the surjective homomorphism $H/N \rightarrow H/N'$ of groups and the surjective ring homomorphism $W/p^{n}W \rightarrow W/p^{n'}W$. Endowing each ring $(W/p^{n}W)[H/N]$ with the discrete topology, Λ(H) is a topological ring for the projective limit topology. It is a pseudocompact ring in the terminology of Brumer (1966), page 442, because each of the rings $(W/p^{n}W)[H/N]$ is Artinian. Recall that a complete Hausdorff topological Λ(H)-module M is called pseudocompact, if it admits a basis $(M_{i})_{i\in I}$ of open neighborhoods of zero such that each M _i is a Λ(H)- submodule of M for which the Λ(H)-module M∕M _i has finite length. For brevity, we will set

$$\displaystyle{\varLambda:=\varLambda (\mathop{\text{Aut}}\nolimits (G)).}$$

Corollary 2.

The action of $\mathop{\text{Aut}}\nolimits (G)$ on $R = R_{G}^{\mathop{\text{def}}\nolimits }$ extends to an action of Λ and gives R the structure of a pseudocompact Λ-module.

Proof.

Since R is $\mathfrak{m}$-adically separated and complete, we may consider the natural isomorphism

$$\displaystyle{R \simeq \mathop{\lim }\limits_\longleftarrow _{n\geq 0}R/\mathfrak{m}^{n+1}.}$$

According to Theorem 4, the action of the group ring $W[\mathop{\text{Aut}}\nolimits (G)]$ on $R/\mathfrak{m}^{n+1}$ factors through $(W/p^{n+1}W)[\mathop{\text{Aut}}\nolimits (G)/(1 + p^{n}\mathop{ \text{End}}\nolimits (G))]$ where $1 + p^{n}\mathop{ \text{End}}\nolimits (G)$ is an open normal subgroup of $\mathop{\text{Aut}}\nolimits (G)$. Thus, $R/\mathfrak{m}^{n+1}$ can be viewed as a Λ-module via the natural ring homomorphism $\varLambda \rightarrow (W/p^{n+1}W)[\mathop{\text{Aut}}\nolimits (G)/(1 + p^{n}\mathop{ \text{End}}\nolimits (G))]$. The transition maps in the above projective limit are then Λ-equivariant. This proves the first assertion.

As for the second assertion, the ideals $\mathfrak{m}^{n+1}$ of R are open and Λ-stable, being the kernels of the Λ-equivariant projections $R \rightarrow R/\mathfrak{m}^{n+1}$. They form a basis of open neighborhoods of zero of R, and the quotients $R/\mathfrak{m}^{n+1}$ are even of finite length over W ⊆ Λ.

Let $\mathop{\text{Lie}}\nolimits (\mathbb{G})$ denote the Lie algebra of the universal deformation $\mathbb{G}$ of G. This is a free module of rank $d =\dim (G)$ over R. Given $\gamma \in \mathop{\text{Aut}}\nolimits (G)$, we extend the ring automorphism γ: R → R to an automorphism $\gamma: R[\![X]\!] \rightarrow R[\![X]\!]$ by setting γ(X _i ) = X _i for all 1 ≤ i ≤ d. It induces a homomorphism $\gamma:\mathop{ \text{Lie}}\nolimits (\mathbb{G}) \rightarrow \mathop{\text{Lie}}\nolimits (\gamma _{{\ast}}\mathbb{G})$ of additive groups. We define $\tilde{\gamma }:\mathop{ \text{Lie}}\nolimits (\mathbb{G}) \rightarrow \mathop{\text{Lie}}\nolimits (\mathbb{G})$ as the composite of the two additive maps

with $[\gamma ]:\gamma _{{\ast}}\mathbb{G} \rightarrow \mathbb{G}$ as above. Given a second element $\gamma ' \in \mathop{\text{Aut}}\nolimits (G)$, we define $\gamma ':\mathop{ \text{Lie}}\nolimits (\gamma _{{\ast}}\mathbb{G}) \rightarrow \mathop{\text{Lie}}\nolimits (\gamma '_{{\ast}}(\gamma _{{\ast}}\mathbb{G}))$ as before. Further, $\gamma '_{{\ast}}[\gamma ]:\gamma '_{{\ast}}(\gamma _{{\ast}}\mathbb{G}) \rightarrow \gamma '_{{\ast}}\mathbb{G}$ denotes the homomorphism obtained by applying $\gamma ' \in \mathop{\text{Aut}}\nolimits (R)$ to the coefficients of $[\gamma ] \in R[\![X]\!]^{d}$. One readily checks that the diagram

is commutative. Further, the uniqueness assertion in Theorem 3 implies that $[\gamma '\gamma ] = [\gamma '] \circ \gamma '_{{\ast}}[\gamma ]$. Therefore,

$$\displaystyle\begin{array}{rcl} (\gamma '\gamma )^{\sim }& =& \mathop{\text{Lie}}\nolimits ([\gamma '\gamma ]) \circ (\gamma '\gamma ) =\mathop{ \text{Lie}}\nolimits ([\gamma ']) \circ \mathop{\text{Lie}}\nolimits (\gamma '_{ {\ast}}[\gamma ]) \circ \gamma '\circ \gamma {}\\ & =& \mathop{\text{Lie}}\nolimits ([\gamma ']) \circ (\gamma ' \circ \mathop{\text{Lie}}\nolimits ([\gamma ]) \circ (\gamma ')^{-1}) \circ \gamma '\circ \gamma =\tilde{\gamma } ' \circ \tilde{\gamma }. {}\\ \end{array}$$

As a consequence, we obtain an action of $\mathop{\text{Aut}}\nolimits (G)$ on the additive group $\mathop{\text{Lie}}\nolimits (\mathbb{G})$ which is semilinear for the action on R in the sense that

$$\displaystyle{\tilde{\gamma }(f\cdot \delta ) =\gamma (f) \cdot \tilde{\gamma } (\delta )\quad \mbox{ for all}\quad f \in R,\delta \in \mathop{\text{Lie}}\nolimits (\mathbb{G}).}$$

To ease notation, we will again write γ(δ) for $\tilde{\gamma }(\delta )$.

Given a positive integer m we denote by $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ the m-fold tensor product of $\mathop{\text{Lie}}\nolimits (\mathbb{G})$ over R with itself. This is a free R-module of rank d ^m with a semilinear action of $\mathop{\text{Aut}}\nolimits (G)$ defined by

$$\displaystyle{\gamma (\delta _{1} \otimes \cdots \otimes \delta _{m}):=\gamma (\delta _{1}) \otimes \cdots \otimes \gamma (\delta _{m}).}$$

We also set $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes 0}:= R$ and $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}:=\mathop{ \text{Hom}}\nolimits _{R}(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes (-m)},R)$ if m is a negative integer. In the latter case $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ is a free R-module of rank d ^−m with a semilinear action of $\mathop{\text{Aut}}\nolimits (G)$ defined through

$$\displaystyle{\gamma (\varphi )(\delta _{1} \otimes \cdots \otimes \delta _{-m}):=\gamma (\varphi (\gamma ^{-1}(\delta _{ 1}) \otimes \cdots \otimes \gamma ^{-1}(\delta _{ -m}))).}$$

For any integer m we endow the R-module $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ with the $\mathfrak{m}$-adic topology for which it is Hausdorff and complete. By the semilinearity of the $\mathop{\text{Aut}}\nolimits (G)$-action, the R-submodules $\mathfrak{m}^{n}\mathop{ \text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ are $\mathop{\text{Aut}}\nolimits (G)$-stable for any non-negative integer n.

As an easy consequence of Proposition 1 and Theorem 4, we obtain the following result.

Theorem 5.

Let m and n be integers with n ≥ 0. The action of $\mathop{\text{Aut}}\nolimits (G)$ on $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ is continuous in the sense that the structure map $\mathop{\text{Aut}}\nolimits (G) \times \mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m} \rightarrow \mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ of the action is continuous. Here the left hand side carries the product topology. The induced action of $1 + p^{2n+1}\mathop{ \text{End}}\nolimits (G)$ on the quotient $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}/\mathfrak{m}^{n+1}\mathop{ \text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ is trivial. In particular, the action of $\mathop{\text{Aut}}\nolimits (G)$ on $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ extends to an action of Λ and gives $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ the structure of a pseudocompact Λ-module.

Proof.

As in the proof of Theorem 4 and Corollary 2, it suffices to show that the action of $1 + p^{2n+1}\mathop{ \text{End}}\nolimits (G)$ on $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}/\mathfrak{m}^{n+1}\mathop{ \text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ is trivial. By definition of the action and Theorem 4 we may assume m = 1. Setting $\mathbb{G}_{n} = \mathbb{G}\mod \mathfrak{m}^{n+1}$, as before, we have $\mathop{\text{Lie}}\nolimits (\mathbb{G})/\mathfrak{m}^{n+1}\mathop{ \text{Lie}}\nolimits (\mathbb{G}) =\mathop{ \text{Lie}}\nolimits (\mathbb{G}_{n})$. Since 2n + 1 ≥ n, Theorem 4 and its proof show that the map $\gamma \mod \mathfrak{m}^{n+1}:\mathop{ \text{Lie}}\nolimits (\mathbb{G}_{n}) \rightarrow \mathop{\text{Lie}}\nolimits (\mathbb{G}_{n})$ is given by $\mathop{\text{Lie}}\nolimits (\rho _{\mathbb{G}} \circ \gamma \circ \rho _{\mathbb{G}}^{-1})$ where $\rho _{\mathbb{G}} \circ \gamma \circ \rho _{\mathbb{G}}^{-1}$ is contained in $1 + p^{2n+1}\mathop{ \text{End}}\nolimits (\mathbb{G}_{0}) \subseteq 1 + p^{n+1}\mathop{ \text{End}}\nolimits (\mathbb{G}_{n})$ (cf. Proposition 1). Therefore, it suffices to show that the natural action of $1 + p^{n+1}\mathop{ \text{End}}\nolimits (\mathbb{G}_{n}) \subset \mathop{\text{End}}\nolimits (\mathbb{G}_{n})$ on $\mathop{\text{Lie}}\nolimits (\mathbb{G}_{n})$ is trivial. However, if $\varphi \in \mathop{\text{End}}\nolimits (\mathbb{G}_{n})$ and if $\delta \in \mathop{\text{Lie}}\nolimits (\mathbb{G}_{n})$, then

$$\displaystyle{\mathop{\text{Lie}}\nolimits (1 + p^{n+1}\varphi )(\delta ) =\delta +p^{n+1}\mathop{ \text{Lie}}\nolimits (\varphi )(\delta ) =\delta,}$$

because $p^{n+1} \in \mathfrak{m}^{n+1}$.

Before we continue, let us point out an important variant of the deformation problem considered above. It concerns the moduli problems considered by Rapoport and Zink (1996).

Let G be a fixed p-divisible group over the algebraically closed field k of characteristic p, i.e. an fppf -group scheme over Spec(k) for which multiplication by p is an epimorphism. Denoting by Nil _p the category of W-schemes on which p is locally nilpotent, let $\mathcal{M}_{G}: \mathit{Nil}_{p} \rightarrow \mathit{Sets}$ denote the set valued functor which associates to an object S of Nil _p the set of isomorphism classes of pairs (G′, ρ _G′), where G′ is a p-divisible group over S and $\rho _{G'}: G_{\overline{S}} \rightarrow G'_{\overline{S}}$ is a quasi-isogeny (cf. Rapoport and Zink 1996, Definition 2.8). Here $\overline{S}$ denotes the closed subscheme of S defined by the sheaf of ideals $p\mathcal{O}_{S}$. According to Rapoport and Zink (1996), Theorem 2.16, the functor $\mathcal{M}_{G}$ is represented by a formal scheme which is locally formally of finite type over $\mathrm{Spf}(W)$. If G is a p-divisible one dimensional commutative formal group law as in Sect. 1, then $\mathcal{M}_{G}$ is the disjoint union of open subschemes $\mathcal{M}_{G}^{n}$, $n \in \mathbb{Z}$, which are non-canonically isomorphic to $\mathrm{Spf}(R_{G}^{\mathop{\text{def}}\nolimits })$ (cf. Rapoport and Zink 1996, Proposition 3.79). The reason is that any quasi-isogeny of height zero between one dimensional p-divisible formal group laws over k is an isomorphism.

One can generalize the moduli problem even further by considering deformations of p-divisible groups with additional structures such as polarizations or actions by maximal orders in finite dimensional semisimple $\mathbb{Q}_{p}$-algebras (cf. Rapoport and Zink 1996, Definition 3.21). The corresponding deformation functors are again representable, as was proven by Rapoport and Zink (1996, Theorem 3.25). An important example was studied by Drinfeld (cf. Rapoport and Zink 1996, 3.58). The generic fiber of the representing formal scheme is known as Drinfeld’s upper half space over K. Instead of continuous representations of $\mathop{\text{Aut}}\nolimits (G)$ as in Theorem 4, it gives rise to an important class of p-adic locally analytic representations in the sense of Schneider and Teitelbaum. This particular class of representations was studied extensively by Morita, Orlik, Schneider and Teitelbaum (cf. Orlik 2008; Schneider and Teitelbaum 2002). It found aritheoremetic applications to the de Rham cohomology of varieties which are p-adically uniformized by Drinfeld’s upper half space (cf. Kohlhaase and Schraen 2012). In the next section we shall see that the deformation spaces we consider here give rise to locally analytic representations, as well.

4 Rigidification and Local Analyticity

We keep the notation of the previous section and denote by k an algebraically closed field of characteristic p and by G a fixed commutative p-divisible formal group over k. Let h and d denote the height and the dimension of G, respectively. We denote by W the ring of Witt vectors of k and by K the quotient field of W. We let $R = R_{G}^{\mathop{\text{def}}\nolimits }$ denote the universal deformation ring of G (cf. Theorem 3).

According to Theorem 3, the rigidification $\mathop{\text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits }$ of the formal scheme $\mathop{\text{Spf}}\nolimits (R)$ in the sense of Berthelot (cf. de Jong 1995, section 7) is isomorphic to the (h − d)d-dimensional rigid analytic open unit polydisc $\mathring {\mathbb{B}}_{K}^{(h-d)d}$ over K. We let

$$\displaystyle{R^{\mathop{\text{rig}}\nolimits }:= \mathcal{O}(\mathop{\text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits })}$$

denote the ring of global rigid analytic functions on $\mathop{\text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits }$. Any isomorphism $R \simeq W[\![u]\!]$ of local W-algebras extends to an isomorphism

$$\displaystyle{R^{\mathop{\text{rig}}\nolimits } \simeq \{\sum _{\alpha \in \mathbb{N}^{(h-d)d}}c_{\alpha }u^{\alpha }\;\vert \;c_{\alpha } \in K\mbox{ and }\lim _{\vert \alpha \vert \rightarrow \infty }\vert c_{\alpha }\vert r^{\vert \alpha \vert } = 0\mbox{ for all }0 < r < 1\}}$$

of K-algebras, where | ⋅ | denotes the p-adic absolute value on K. This allows us to view $R^{\mathop{\text{rig}}\nolimits }$ as a topological K-Fréchet algebra whose topology is defined by the family of norms | | ⋅ | | _ℓ , given by

$$\displaystyle{\vert \vert \sum _{\alpha }c_{\alpha }u^{\alpha }\vert \vert _{\ell}:=\sup _{\alpha }\{\vert c_{\alpha }\vert p^{-\vert \alpha \vert /\ell}\}}$$

for any positive integer ℓ. Letting $R_{\ell}^{\mathop{\text{rig}}\nolimits }$ denote the completion of $R^{\mathop{\text{rig}}\nolimits }$ with respect to the norm | | ⋅ | | _ℓ , the K-algebra $R_{\ell}^{\mathop{\text{rig}}\nolimits }$ can be identified with the ring of rigid analytic functions on the affinoid subdomain

$$\displaystyle{\mathbb{B}_{\ell}^{(h-d)d}:=\{ x \in \mathop{\text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits }\;\vert \;\vert u_{ i}(x)\vert \leq p^{-1/\ell}\mbox{ for all }1 \leq i \leq (h - d)d\}}$$

of $\mathop{\text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits }$. Further, $R^{\mathop{\text{rig}}\nolimits } \simeq \mathop{\lim }\limits_\longleftarrow _{\ell}R_{\ell}^{\mathop{\text{rig}}\nolimits }$ is the topological projective limit of the K-Banach algebras $R_{\ell}^{\mathop{\text{rig}}\nolimits }$. In fact, by a cofinality argument and Bosch et al. (1984), 6.1.3 Theorem 1, $R^{\mathop{\text{rig}}\nolimits }$ is the topological projective limit of the system of affinoid K-algebras corresponding to any nested admissible open affinoid covering of $\mathop{\text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits }$.

By functoriality, the automorphism group $\varGamma =\mathop{ \text{Aut}}\nolimits (G)$ of G acts on $\mathop{\text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits }$ by automorphisms of rigid analytic K-varieties. This gives rise to an action of Γ on $R^{\mathop{\text{rig}}\nolimits }$ by K-linear ring automorphisms. By the above cofinality argument, any of these automorphisms is continuous. The goal of this section is to show that the induced action on the strong topological K-linear dual of $R^{\mathop{\text{rig}}\nolimits }$ is locally analytic in the sense of Schneider and Teitelbaum (2002, page 451).

Fix an algebraic closure $K^{\mathop{\text{alg}}\nolimits }$ of K. According to de Jong (1995), Lemma 7.19, the maximal ideals of the ring $R_{K}:= R \otimes _{W}K$ are in bijection with the points of $\mathop{\text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits }$. It follows from Bosch et al. (1984), 7.1.1 Proposition 1, that the latter are in bijection with the $\mathrm{Gal}(K^{\mathop{\text{alg}}\nolimits }\vert K)$-orbits of

$$\displaystyle{\mathring {\mathbb{B}}_{K}^{(h-d)d}(K^{\mathop{\text{alg}}\nolimits }):=\{ x \in (K^{\mathop{\text{alg}}\nolimits })^{(h-d)d}\;\vert \;\vert x_{ i}\vert < 1\mbox{ for all }1 \leq i \leq (h-d)d\}.}$$

A point x representing one of these orbits corresponds to the kernel of the surjective K-linear ring homomorphism $R_{K} \rightarrow K(x):= K(x_{1},\ldots,x_{(h-d)d}) \subseteq K^{\mathop{\text{alg}}\nolimits }$, sending f(u) to f(x).

The following result constitutes the technical heart of this section. It is a straightforward generalization of Gross and Hopkins (1994), Lemma 19.3.

Proposition 2.

Let n and ℓ be integers with n ≥ 0 and ℓ ≥ 1. If γ ∈Γ _n and if $f \in R^{\mathop{\text{rig}}\nolimits }$ then $\vert \vert \gamma (f) - f\vert \vert _{\ell}\leq p^{-n/\ell}\vert \vert f\vert \vert _{\ell}$ .

Proof.

First assume f = u _i for some 1 ≤ i ≤ (h − d)d. If

$$\displaystyle{\mathbb{B}_{\ell}^{(h-d)d}(K^{\mathop{\text{alg}}\nolimits }):=\{ x \in (K^{\mathop{\text{alg}}\nolimits })^{(h-d)d}\;\vert \;\,\vert x_{ i}\vert \leq p^{-1/\ell}\mbox{ for all }1 \leq i \leq (h - d)d\},}$$

then $\vert \vert g\vert \vert _{\ell} =\sup \{ \vert g(x)\vert \;\vert \;x \in \mathbb{B}_{\ell}^{(h-d)d}(K^{\mathop{\text{alg}}\nolimits })\}$ for any $g \in R^{\mathop{\text{rig}}\nolimits }$. Thus, we need to see that if $x \in \mathbb{B}_{\ell}^{(h-d)d}(K^{\mathop{\text{alg}}\nolimits })$ and if $y:= x\cdot \gamma =\gamma (u)(x)$, then $\vert x_{i} - y_{i}\vert \leq p^{-(n+1)/\ell}$.

Denoting by $W^{\mathop{\text{alg}}\nolimits }$ the valuation ring of $K^{\mathop{\text{alg}}\nolimits }$, consider the commutative diagram

of homomorphisms of W-algebras, in which the left and right oblique arrow is given by evaluation at y and x, respectively. Choosing $z \in W^{\mathop{\text{alg}}\nolimits }$ with $\vert z\vert = p^{-1/\ell}$, we have $x_{j} \in zW^{\mathop{\text{alg}}\nolimits }$ for any j. Further, $p \in zW^{\mathop{\text{alg}}\nolimits }$ because ℓ ≥ 1. As a consequence, the right oblique arrow maps $\mathfrak{m}_{R}$ to $zW^{\mathop{\text{alg}}\nolimits }$. Note that $\gamma (u_{j}) \in \mathfrak{m}_{R}$, so that we obtain $y_{j} = u_{j}(x\cdot \gamma ) =\gamma (u_{j})(x) \in zW^{\mathop{\text{alg}}\nolimits }$, as well. Therefore, also the left oblique arrow maps $\mathfrak{m}_{R}$ to $zW^{\mathop{\text{alg}}\nolimits }$. Now consider the induced diagram

According to Theorem 4, the upper horizontal arrow is the identity. It follows that $x_{i} - y_{i} \in z^{n+1}W^{\mathop{\text{alg}}\nolimits }$, i.e. $\vert x_{i} - y_{i}\vert \leq p^{-(n+1)/\ell}$, as required. In particular, γ stabilizes $\mathbb{B}_{\ell}^{(h-d)d}(K^{\mathop{\text{alg}}\nolimits })$ and therefore is an isometry for the norm | | ⋅ | | _ℓ on $R^{\mathop{\text{rig}}\nolimits }$.

To prove the proposition, the continuity of γ allows us to assume f = u ^α for some $\alpha \in \mathbb{N}^{(h-d)d}$. The assertion is trivial for | α | = 0. If | α | > 0 choose an index i with α _i > 0. Define β through β _j : = α _j if j ≠ i and $\beta _{i}:=\alpha _{i} - 1$. If $x \in \mathbb{B}_{\ell}^{(h-d)d}(K^{\mathop{\text{alg}}\nolimits })$ and if y = x ⋅ γ, then

$$\displaystyle\begin{array}{rcl} \vert \gamma (u^{\alpha })(x) - u^{\alpha }(x)\vert & =& \vert y^{\alpha } - x^{\alpha }\vert = \vert y_{i}y^{\beta } - x_{i}x^{\beta }\vert {}\\ &\leq & \max \{\vert y_{i}\vert \vert y^{\beta } - x^{\beta }\vert,\vert y_{i} - x_{i}\vert \vert x^{\beta }\vert \}. {}\\ \end{array}$$

Here $\vert y_{i}\vert \vert y^{\beta } - x^{\beta }\vert \leq p^{-1/\ell}\vert \vert \gamma (u^{\beta }) - u^{\beta }\vert \vert _{\ell}\leq p^{-(n+1)/\ell}\vert \vert u^{\beta }\vert \vert _{\ell} = p^{-n/\ell}\vert \vert u^{\alpha }\vert \vert _{\ell}$ by the induction hypothesis. Further, $\vert y_{i} - x_{i}\vert \vert x^{\beta }\vert \leq p^{-(n+1)/\ell}p^{-\vert \beta \vert /\ell} = p^{-n/\ell}\vert \vert u^{\alpha }\vert \vert _{\ell}$, as seen above. Thus, we obtain $\vert \gamma (u^{\alpha })(x) - u^{\alpha }(x)\vert \leq p^{-n/\ell}\vert \vert u^{\alpha }\vert \vert _{\ell}$ for all $x \in \mathbb{B}_{\ell}^{(h-d)d}(K^{\mathop{\text{alg}}\nolimits })$. This proves the proposition.

A topological group is a Lie group over $\mathbb{Q}_{p}$ if and only if it contains an open subgroup which is a uniform pro-p group (cf. Dixon et al. 2003, Definition 4.1 and Theorem 8.32). For the compact p-adic Lie group $\varGamma =\mathop{ \text{Aut}}\nolimits (G)$ we have the following more precise result. We let

$$\displaystyle{\varepsilon:= 1\quad \mbox{ if}\quad p > 2\quad \mbox{ and}\quad \varepsilon:= 2\quad \mbox{ if}\quad p = 2.}$$

Lemma 2.

For any non-negative integer n we have $\varGamma _{\varepsilon }^{p^{n} } =\varGamma _{\varepsilon +n}$ . The open subgroup $\varGamma _{\varepsilon +n}$ of Γ is a uniform pro-p group.

Proof.

As for the first assertion, the proofs of Dixon et al. (2003), Lemma 5.1 and Theorem 5.2, can be copied word by word on replacing $\mathrm{M}_{d}(\mathbb{Z}_{p})$ by $\mathop{\text{End}}\nolimits (G)$ and $\mathrm{GL}_{d}(\mathbb{Z}_{p})$ by $\mathop{\text{Aut}}\nolimits (G)$. Further, $\varGamma _{\varepsilon +n}$ is a powerful pro-p group by Dixon et al. (2003), Theorem 3.6 (i) and the remark after Definition 3.1. That it is uniform follows from Dixon et al. (2003), Theorem 3.6 (ii), and the first assertion.

Fix an integer $n \geq \varepsilon$. By Lemma 2 and Dixon et al. (2003), Theorem 3.6, the group $\varGamma _{n}/\varGamma _{n+1}$ is a finite dimensional $\mathbb{F}_{p}$-vector space. Choosing elements γ ₁, …, γ _r ∈ Γ _n whose images modulo Γ _n+1 form an $\mathbb{F}_{p}$-basis of $\varGamma _{n}/\varGamma _{n+1}$, (Dixon et al. 2003), Theorem 4.9, shows that (γ ₁, …, γ _r ) is an ordered basis of Γ _n in the sense that the map $\mathbb{Z}_{p}^{r} \rightarrow \varGamma _{n}$, sending λ to $\gamma _{1}^{\lambda _{1}}\cdots \gamma _{r}^{\lambda _{r}}$, is a homeomorphism.

Set $b_{i}:=\gamma _{i} - 1 \in \varLambda (\varGamma _{n})$ and $b^{\alpha }:= b_{1}^{\alpha _{1}}\cdots b_{r}^{\alpha _{r}}$ for any $\alpha \in \mathbb{N}^{r}$. By Dixon et al. (2003), Theorem 7.20, any element $\delta \in \varLambda (\varGamma _{n})$ admits a unique expansion of the form

$$\displaystyle{\lambda =\sum _{\alpha \in \mathbb{N}^{r}}d_{\alpha }b^{\alpha }\mbox{ with }d_{\alpha } \in W\mbox{ for all }\alpha \in \mathbb{N}^{r}.}$$

For any ℓ ≥ 1 this allows us to define the K-norm | | ⋅ | | _ℓ on the algebra $\varLambda (\varGamma _{n})_{K}:=\varLambda (\varGamma _{n}) \otimes _{W}K$ through

$$\displaystyle{\vert \vert \sum _{\alpha }d_{\alpha }b^{\alpha }\vert \vert _{\ell}:=\sup _{\alpha }\{\vert d_{\alpha }\vert p^{-\varepsilon \vert \alpha \vert /\ell}\}.}$$

Remark 1.

A more accurate notation would be the symbol $\vert \vert \cdot \vert \vert _{\ell}^{(n)}$ for the above norm on Λ(Γ _n ) _K . It does generally not coincide with the restriction of | | ⋅ | | _ℓ ^(m) to $\varLambda (\varGamma _{n})_{K} \subseteq \varLambda (\varGamma _{m})_{K}$ if n ≥ m. However, there is an explicit rescaling relation between the families of norms $(\vert \vert \cdot \vert \vert _{\ell}^{(n)})_{\ell}$ and $(\vert \vert \cdot \vert \vert _{\ell}^{(m)})_{\ell}$ on Λ(Γ _n ) _K (cf. Schmidt 2008, Proposition 6.2). Since we will never work with two different groups Γ _n and Γ _m at once, we decided to ease notation and use the somewhat ambiguous symbol | | ⋅ | | _ℓ .

By Schmidt (2008), Proposition 2.1 and Schneider and Teitelbaum (2003), Proposition 4.2, the norm | | ⋅ | | _ℓ on Λ(Γ _n ) _K is submultiplicative whenever ℓ ≥ 1. As a consequence, the completion

$$\displaystyle{\varLambda (\varGamma _{n})_{K,\ell} =\{\sum _{\alpha }d_{\alpha }b^{\alpha }\;\vert \;d_{\alpha } \in K,\;\lim _{\vert \alpha \vert \rightarrow \infty }\vert d_{\alpha }\vert p^{-\varepsilon \vert \alpha \vert /\ell} = 0\}}$$

of Λ(Γ _n ) _K with respect to | | ⋅ | | _ℓ is a K-Banach algebra. The natural inclusions $\varLambda (\varGamma _{n})_{K,\ell+1} \rightarrow \varLambda (\varGamma _{n})_{K,\ell}$ endow the projective limit

$$\displaystyle{D(\varGamma _{n}):= \mathop{\lim }\limits_\longleftarrow _{\ell}\varLambda (\varGamma _{n})_{K,\ell}}$$

with the structure of a K-Fréchet algebra. As is explained in Schneider and Teitelbaum (2003), section 4, a theorem of Amice allows us to identify it with the algebra of K-valued locally analytic distributions on Γ _n. Similarly, we denote by D(Γ) the algebra of K-valued locally analytic distributions on Γ (cf. Schneider and Teitelbaum 2002, section 2).

Theorem 6.

For any integer ℓ ≥ 1 the action of $\varGamma _{\varepsilon }$ on $R^{\mathop{\text{rig}}\nolimits }$ extends to $R_{\ell}^{\mathop{\text{rig}}\nolimits }$ and makes $R_{\ell}^{\mathop{\text{rig}}\nolimits }$ a topological Banach module over the K-Banach algebra $\varLambda (\varGamma _{\varepsilon })_{K,\ell}$ . The action of Γ on $R^{\mathop{\text{rig}}\nolimits }$ extends to a jointly continuous action of the K-Fréchet algebra D(Γ). The action of Γ on the strong continuous K-linear dual $(R^{\mathop{\text{rig}}\nolimits })'_{b}$ of $R^{\mathop{\text{rig}}\nolimits }$ is locally analytic in the sense of Schneider and Teitelbaum (2002), page 451.

Proof.

First, we prove by induction on | α | that $\vert \vert b^{\alpha }f\vert \vert _{\ell}\leq \vert \vert b^{\alpha }\vert \vert _{\ell}\vert \vert f\vert \vert _{\ell}$ for any $f \in R^{\mathop{\text{rig}}\nolimits }$. This is clear if | α | = 0. Otherwise, let i be the minimal index with α _i > 0 and define β through β _j = α _j if j ≠ i and $\beta _{i}:=\alpha _{i} - 1$. In this case, Proposition 2 and the induction hypothesis imply

$$\displaystyle\begin{array}{rcl} \vert \vert b^{\alpha }f\vert \vert _{\ell}& =& \vert \vert (\gamma _{i} - 1)b^{\beta }f\vert \vert _{\ell}\leq p^{-\varepsilon /\ell}\vert \vert b^{\beta }f\vert \vert _{\ell} {}\\ &\leq & p^{-\varepsilon /\ell}p^{-\varepsilon \vert \beta \vert /\ell}\vert \vert f\vert \vert _{\ell} = \vert \vert b^{\alpha }\vert \vert _{\ell}\vert \vert f\vert \vert _{\ell}, {}\\ \end{array}$$

as required. This immediately gives $\vert \vert \lambda \cdot f\vert \vert _{\ell}\leq \vert \vert \lambda \vert \vert _{\ell}\vert \vert f\vert \vert _{\ell}$ for all $\lambda \in \varLambda (\varGamma _{\varepsilon })_{K}$ and f ∈ R _K . Thus, the multiplication map $\varLambda (\varGamma _{\varepsilon })_{K} \times R_{K} \rightarrow R_{K}$ is continuous, if $\varLambda (\varGamma _{\varepsilon })_{K}$ and R _K are endowed with the respective | | ⋅ | | _ℓ -topologies, and if the left hand side carries the product topology. Since R _K is dense in $R_{\ell}^{\mathop{\text{rig}}\nolimits }$, we obtain a map $\varLambda (\varGamma _{\varepsilon })_{K,\ell} \times R_{\ell}^{\mathop{\text{rig}}\nolimits } \rightarrow R_{\ell}^{\mathop{\text{rig}}\nolimits }$ by passing to completions. By continuity, it gives $R_{\ell}^{\mathop{\text{rig}}\nolimits }$ the structure of a topological Banach module over $\varLambda (\varGamma _{\varepsilon })_{K,\ell}$.

Passing to the projective limit, we obtain a continuous map D(Γ _ɛ ) × R ^rig → R ^rig, giving $R^{\mathop{\text{rig}}\nolimits }$ the structure of a jointly continuous module over $D(\varGamma _{\varepsilon })$. Since D(Γ) is topologically isomorphic to the locally convex direct sum $\oplus _{\gamma \varGamma _{\varepsilon }\in \varGamma /\varGamma _{\varepsilon }}\gamma D(\varGamma _{\varepsilon })$ (cf. Schneider and Teitelbaum 2002, page 447 bottom), $R^{\mathop{\text{rig}}\nolimits }$ is a jointly continuous module over D(Γ).

It follows from Schneider (2002), Proposition 19.9 and the arguments proving the claim on page 98, that the K-Fréchet space $R^{\mathop{\text{rig}}\nolimits }$ is nuclear. Therefore, Schneider and Teitelbaum (2002), Corollary 3.4, implies that the locally convex K-vector space $(R^{\mathop{\text{rig}}\nolimits })'_{b}$ is of compact type and that the action of Γ obtained by dualizing is locally analytic.

Using Theorem 5, the preceding result can be generalized as follows. Fixing an integer m, the free R-module $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ gives rise to a locally free coherent sheaf on $\mathop{\text{Spf}}\nolimits (R)$. For any positive integer ℓ we denote by $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{\ell}^{\mathop{\text{rig}}\nolimits }$ the sections of its rigidification over the affinoid subdomain $\mathbb{B}_{\ell}^{(h-d)d}$ of $\mathop{\text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits }$. This is a free $R_{\ell}^{\mathop{\text{rig}}\nolimits }$-module for which the natural $R_{\ell}^{\mathop{\text{rig}}\nolimits }$-linear map

$$\displaystyle{R_{\ell}^{\mathop{\text{rig}}\nolimits } \otimes _{R}\mathop{ \text{Lie}}\nolimits (\mathbb{G})^{\otimes m}\longrightarrow (\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{\ell}^{\mathop{\text{rig}}\nolimits }}$$

is bijective (cf. de Jong 1995, 7.1.11). We denote by $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ the space of global sections of the rigidification of $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ over $\mathop{\text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits }$. This is a free $R^{\mathop{\text{rig}}\nolimits }$-module for which the natural $R^{\mathop{\text{rig}}\nolimits }$-linear maps

$$\displaystyle{ R^{\mathop{\text{rig}}\nolimits } \otimes _{R}\mathop{ \text{Lie}}\nolimits (\mathbb{G})^{\otimes m}\longrightarrow (\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }\longrightarrow \mathop{\lim }\limits_\longleftarrow _{\ell}(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{\ell}^{\mathop{\text{rig}}\nolimits } }$$

(1)

are bijective. Further, $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits } \simeq (\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\mathop{\text{rig}}\nolimits })^{\otimes m}$, where the latter tensor products and dualities are with respect to $R^{\mathop{\text{rig}}\nolimits }$.

By functoriality, the group $\varGamma =\mathop{ \text{Aut}}\nolimits (G)$ acts on $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ in such a way that the left map in (1) becomes Γ-equivariant for the diagonal action on the left. In particular, it is semilinear for the action of Γ on $R^{\mathop{\text{rig}}\nolimits }$. We endow $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ and $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{\ell}^{\mathop{\text{rig}}\nolimits }$ with the natural topologies of finitely generated modules over $R^{\mathop{\text{rig}}\nolimits }$ and $R_{\ell}^{\mathop{\text{rig}}\nolimits }$, respectively. This makes them a nuclear K-Fréchet space and a K-Banach space, respectively. The right map in (1) is then a topological isomorphism for the projective limit topology on the right. With the same cofinality argument as for $R^{\mathop{\text{rig}}\nolimits }$ one can show that any element of Γ acts on $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ through a continuous K-linear automorphism.

Theorem 7.

Let m be an integer. For any integer ℓ ≥ 1 the action of $\varGamma _{2\varepsilon -1}$ on $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ extends to $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{\ell}^{\mathop{\text{rig}}\nolimits }$ and makes $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{\ell}^{\mathop{\text{rig}}\nolimits }$ a topological Banach module over the K-Banach algebra $\varLambda (\varGamma _{2\varepsilon -1})_{K,\ell}$ . The action of Γ on $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ extends to a jointly continuous action of the K-Fréchet algebra D(Γ). The action of Γ on the strong continuous K-linear dual $[(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }]'_{b}$ of $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ is locally analytic.

Proof.

Set $M_{\ell}^{m}:= (\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{\ell}^{\mathop{\text{rig}}\nolimits }$. Any R-basis $(\delta _{1},\ldots,\delta _{s})$ of $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$ can be viewed as an $R_{\ell}^{\mathop{\text{rig}}\nolimits }$-basis of M _ℓ ^m. Writing $M_{\ell}^{m} = \oplus _{i=1}^{s}R_{\ell}^{\mathop{\text{rig}}\nolimits }\delta _{i}$, the topology of M _ℓ ^m is defined by the norm

$$\displaystyle{\vert \vert \sum _{i=1}^{s}f_{ i}\delta _{i}\vert \vert _{\ell} =\sup _{i}\{\vert \vert f_{i}\vert \vert _{\ell}\}\quad \mbox{ if}\quad f_{1},\ldots,f_{s} \in R_{\ell}^{\mathop{\text{rig}}\nolimits }.}$$

We choose an ordered basis $(\gamma _{1},\ldots,\gamma _{r})$ of $\varGamma _{2\varepsilon -1}$ and let $b_{i}:=\gamma _{i} - 1$ be as before. By induction on | α | we will first prove the fundamental estimate $\vert \vert b^{\alpha }\delta \vert \vert _{\ell}\leq \vert \vert b^{\alpha }\vert \vert _{\ell}\vert \vert \delta \vert \vert _{\ell}$ for all $\alpha \in \mathbb{N}^{r}$ and $\delta \in (\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$. As in the proof of Theorem 6 this is reduced to the case | α | = 1, i.e. $b^{\alpha } =\gamma _{i} - 1$ for some 1 ≤ i ≤ r. Further, we may assume δ = f δ _j for some $f \in R^{\mathop{\text{rig}}\nolimits }$ and $1 \leq j \leq s$.

There are elements r ₁, …, r _s ∈ R such that $\gamma _{i}(\delta _{j}) =\sum _{ \nu =1}^{s}r_{\nu }\delta _{\nu }$. According to Theorem 5 we have $(\gamma _{i} - 1)(\delta _{j}) \in \mathfrak{m}^{\varepsilon }\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m}$, i.e. $r_{j} - 1 \in \mathfrak{m}^{\varepsilon }$ and $r_{\nu } \in \mathfrak{m}^{\varepsilon }$ for $\nu \not =j$. We claim that $\vert \vert r\vert \vert _{\ell}\leq p^{-c/\ell}$ for any integer c ≥ 0 and any element $r \in \mathfrak{m}^{c}$. Indeed, this is clear for c = 0. For general c, the ideal $\mathfrak{m}^{c}$ of R is generated by all elements of the form p ^a u ^β with $a \in \mathbb{N}$, $\beta \in \mathbb{N}^{(h-d)d}$ and $a + \vert \beta \vert = c$. Since ℓ ≥ 1 we have $\vert p^{a}\vert = p^{-a} \leq p^{-a/\ell}$, and the claim follows from the multiplicativity of the norm | | ⋅ | | _ℓ on R. Now

$$\displaystyle\begin{array}{rcl} \vert \vert (\gamma _{i} - 1)(f\delta _{j})\vert \vert _{\ell}& \leq & \max \{\vert \vert (\gamma _{i} - 1)(f) \cdot \gamma _{i}(\delta _{j})\vert \vert _{\ell},\vert \vert f \cdot (\gamma _{i} - 1)(\delta _{j})\vert \vert _{\ell}\} {}\\ & =& \max \{\vert \vert \sum _{\nu }(\gamma _{i} - 1)(f)r_{\nu }\delta _{\nu }\vert \vert _{\ell},\vert \vert f\vert \vert _{\ell}\vert \vert \delta _{j} -\sum _{\nu }r_{\nu }\delta _{\nu }\vert \vert _{\ell}\}, {}\\ \end{array}$$

where $\vert \vert (\gamma _{i} - 1)(f) \cdot r_{\nu }\vert \vert _{\ell}\leq \vert \vert (\gamma _{i} - 1)(f)\vert \vert _{\ell}\leq p^{(2\varepsilon -1)/\ell}\vert \vert f\vert \vert _{\ell}$ by Proposition 2. Here $p^{(2\varepsilon -1)/\ell} \leq p^{-\varepsilon /\ell} = \vert \vert \gamma _{i} - 1\vert \vert _{\ell}$. Moreover, $\vert \vert r_{j} - 1\vert \vert _{\ell}\leq p^{-\varepsilon /\ell}$ and $\vert \vert r_{\nu }\vert \vert _{\ell}\leq p^{-\varepsilon /\ell}$ if ν ≠ j by the above claim. This finishes the proof of the fundamental estimate.

As an immediate consequence, we obtain that $\vert \vert \lambda \cdot \delta \vert \vert _{\ell}\leq \vert \vert \lambda \vert \vert _{\ell}\vert \vert \delta \vert \vert _{\ell}$ for any $\lambda \in \varLambda (\varGamma _{2\varepsilon -1})_{K}$ and any $\delta \in \mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m} \otimes _{W}K$. The proof proceeds now as in Theorem 6.

According to Schneider and Teitelbaum (2003), Theorem 4.10, the projective system $(\varLambda (\varGamma _{2\varepsilon -1})_{K,\ell})_{\ell}$ of K-Banach algebras endow their projective limit $D(\varGamma _{2\varepsilon -1})$ with the structure of a K-Fréchet-Stein algebra. In the terminology of Schneider and Teitelbaum (2003), section 8, the family $((\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{\ell}^{\mathop{\text{rig}}\nolimits })_{\ell}$ is a sheaf over $(D(\varGamma _{2\varepsilon -1}),(\vert \vert \cdot \vert \vert _{\ell})_{\ell})$ with global sections $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ for any integer m. One of the main open questions in this setting is whether this sheaf is coherent, i.e. whether the $\varLambda (\varGamma _{2\varepsilon -1})_{K,\ell}$-modules $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{\ell}^{\mathop{\text{rig}}\nolimits }$ are finitely generated and whether the natural maps

$$\displaystyle{\varLambda (\varGamma _{2\varepsilon -1})_{K,\ell} \otimes _{\varLambda (\varGamma _{2\varepsilon -1})_{K,\ell+1}}(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{\ell +1}^{\mathop{\text{rig}}\nolimits }\longrightarrow (\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{\ell}^{\mathop{\text{rig}}\nolimits }}$$

are always bijective. This would amount to the admissibility of the locally analytic Γ-representation $[(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }]'_{b}$ in the sense of Schneider and Teitelbaum (2003), section 6. Nothing in this direction is known. In the next section, however, we will have a closer look at the case $\dim (G) = 1$ and ℓ = 1. We will see that in order to obtain finitely generated objects, one might be forced to introduce yet another type of Banach algebras.

5 Non-commutative Divided Power Envelopes

In this final section we assume that our fixed p-divisible formal group G over the algebraically closed field k of characteristic p is of dimension one. If h denotes the height of G then the endomorphism ring of G is isomorphic to the maximal order $\mathfrak{o}_{D}$ of the central $\mathbb{Q}_{p}$-division algebra D of invariant $\frac{1} {h} + \mathbb{Z}$ (cf. Gross and Hopkins 1994, Proposition 13.10). In the following we will identify $\mathop{\text{End}}\nolimits (G)$ and $\mathfrak{o}_{D}$ (resp. $\mathop{\text{Aut}}\nolimits (G)$ and $\mathfrak{o}_{D}^{{\ast}}$). We will also exclude the trivial case h = 1. We continue to denote by $R = R_{G}^{\mathop{\text{def}}\nolimits }$ the universal deformation ring of G (cf. Theorem 3).

Consider the period morphism $\varPhi:\mathop{ \text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits } \rightarrow \mathbb{P}_{K}^{h-1}$ of Gross and Hopkins, where $\mathbb{P}_{K}^{h-1}$ denotes the rigid analytic projective space of dimension h − 1 over K (cf. Gross and Hopkins 1994, section 23). In projective coordinates $\varPhi$ can be defined by $\varPhi (x) = [\varphi _{0}(x):\ldots:\varphi _{h-1}(x)]$ where $\varphi _{0},\ldots,\varphi _{h-1} \in R^{\mathop{\text{rig}}\nolimits }$ are certain global rigid analytic functions on $\mathop{\text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits }$ without any common zero. The power series expansions of the functions $\varphi _{i}$ in suitable coordinates u ₁, …, u _h−1 can be written down explicitly by means of a closed formula of Yu (cf. Kohlhaase 2013, Proposition 1.5 and Remark 1.6). According to Gross and Hopkins (1994), Lemma 23.14, the function $\varphi _{0}$ does not have any zeroes on $\mathbb{B}_{1}^{h-1} \subset \mathop{\text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits }$, hence is a unit in $R_{1}^{\mathop{\text{rig}}\nolimits }$. We set

$$\displaystyle{w_{i}:= \frac{\varphi _{i}} {\varphi _{0}} \in R_{1}^{\mathop{\text{rig}}\nolimits }\quad \mbox{ for}\quad 1 \leq i \leq h - 1.}$$

By Gross and Hopkins (1994), Lemma 23.14, any element $f \in R_{1}^{\mathop{\text{rig}}\nolimits }$ admits a unique expansion of the form $f =\sum _{\alpha \in \mathbb{N}^{h-1}}d_{\alpha }w^{\alpha }$ with d _α ∈ K and $\lim _{\vert \alpha \vert \rightarrow \infty }\vert d_{\alpha }\vert p^{-\vert \alpha \vert } = 0$. Further, Φ restricts to an isomorphism $\varPhi: \mathbb{B}_{1}^{h-1} \rightarrow \varPhi (\mathbb{B}_{1}^{h-1})$ (cf. Gross and Hopkins 1994, Corollary 23.15).

Denote by $\mathbb{Q}_{p^{h}}$ the unramified extension of degree h of $\mathbb{Q}_{p}$ and by $\mathbb{Z}_{p^{h}}$ its valuation ring. It was shown by Devinatz, Gross and Hopkins, that there exists an explicit closed embedding $\mathfrak{o}_{D}^{{\ast}}\hookrightarrow \mathrm{GL}_{h}(\mathbb{Q}_{p^{h}})$ of Lie groups over $\mathbb{Q}_{p}$ such that Φ is $\mathfrak{o}_{D}^{{\ast}}$-equivariant (cf. Kohlhaase 2013, Proposition 1.3 and Remark 1.4). Here $\mathfrak{o}_{D}^{{\ast}}$ acts on $\mathop{\text{Spf}}\nolimits (R)^{\mathop{\text{rig}}\nolimits }$ through the identification $\mathfrak{o}_{D}^{{\ast}}\simeq \mathop{\text{Aut}}\nolimits (G)$, and it acts by fractional linear transformations on $\mathbb{P}_{K}^{h-1}$ via the embedding $\mathfrak{o}_{D}^{{\ast}}\hookrightarrow \mathrm{GL}_{h}(\mathbb{Q}_{p^{h}})$.

The morphism Φ is constructed in such a way that $\varPhi ^{{\ast}}\mathcal{O}_{\mathbb{P}_{K}^{h-1}}(1) =\mathop{ \text{Lie}}\nolimits (\mathbb{G})^{\mathop{\text{rig}}\nolimits }$. It follows from general properties of the inverse image functor that $\varPhi ^{{\ast}}\mathcal{O}_{\mathbb{P}_{K}^{h-1}}(m) = (\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ for any integer m. Restricting to $\mathbb{B}_{1}^{h-1}$, we obtain an $\mathfrak{o}_{D}^{{\ast}}$-equivariant and $R_{1}^{\mathop{\text{rig}}\nolimits }$-linear isomorphism $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{1}^{\mathop{\text{rig}}\nolimits } \simeq R_{1}^{\mathop{\text{rig}}\nolimits } \cdot \varphi _{0}^{m}$ of free R ₁ ^rig-modules of rank one.

We denote by $\mathfrak{d}$ the Lie algebra of the Lie group $\mathfrak{o}_{D}^{{\ast}}$ over $\mathbb{Q}_{p}$. It is isomorphic to the Lie algebra associated with the associative $\mathbb{Q}_{p}$-algebra D. According to Schneider and Teitelbaum (2002), page 450, the universal enveloping algebra $U_{K}(\mathfrak{d}):= U(\mathfrak{d} \otimes _{\mathbb{Q}_{p}}K)$ of $\mathfrak{d}$ over K embeds into the locally analytic distribution algebra $D(\varGamma _{2\varepsilon -1})$. Together with the natural map $D(\varGamma _{2\varepsilon -1}) \rightarrow \varLambda (\varGamma _{2\varepsilon -1})_{K,1}$, Theorem 7 allows us to view

$$\displaystyle{M_{1}^{m}:= (\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{ 1}^{\mathop{\text{rig}}\nolimits }}$$

as a module over $U_{K}(\mathfrak{d}) \simeq U(\mathfrak{g} \otimes _{\mathbb{Q}_{ p^{h}}}K) =: U_{K}(\mathfrak{g})$, where $\mathfrak{g}:= \mathfrak{d} \otimes _{\mathbb{Q}_{p}}\mathbb{Q}_{p^{h}} \simeq \mathfrak{g}\mathfrak{l}_{h}$ as Lie algebras over $\mathbb{Q}_{p^{h}}$. Explicitly, the action of an element $\mathfrak{x} \in \mathfrak{g}$ on M ₁ ^m is given by

$$\displaystyle{\mathfrak{x}(\delta ) = \frac{d} {\mathit{dt}}(\exp (t\mathfrak{x})(\delta ))\vert _{t=0}.}$$

Here

is the usual exponential map which is defined locally around zero in $\mathfrak{g}$. Further, a sufficiently small open subgroup of $\mathrm{GL}_{h}(\mathbb{Q}_{p^{h}})$ acts on M ₁ ^m through the isomorphism $M_{1}^{m} \simeq \mathcal{O}_{\mathbb{P}_{K}^{h-1}}(m)(\varPhi (\mathbb{B}_{1}^{h-1}))$. Writing an element $\mathfrak{x} \in \mathfrak{g}$ as a matrix $\mathfrak{x} = (a_{rs})_{0\leq r,s\leq h-1}$ with coefficients $a_{rs} \in \mathbb{Q}_{p^{h}}$, fix indices $0 \leq i,j \leq h - 1$ and denote by $\mathfrak{x}_{ij}$ the matrix with entry 1 at the place (i, j) and zero everywhere else. In the following we will formally put w ₀: = 1.

Lemma 3.

Let i,j and m be integers with 0 ≤ i,j ≤ h − 1. If $f \in R_{1}^{\mathop{\text{rig}}\nolimits }$ then

$$\displaystyle{\mathfrak{x}_{ij}(f\varphi _{0}^{m}) = \left \{\begin{array}{l@{\quad }l} w_{i} \frac{\partial f} {\partial w_{j}}\varphi _{0}^{m}, \quad &\mbox{ if }j\not =0, \\ (mf -\sum _{\ell=1}^{h-1}w_{\ell} \frac{\partial f} {\partial w_{\ell}})\varphi _{0}^{m}, \quad &\mbox{ if }i = j = 0, \\ w_{i}(mf -\sum _{\ell=1}^{h-1}w_{\ell} \frac{\partial f} {\partial w_{\ell}})\varphi _{0}^{m},\quad &\mbox{ if }i > j = 0. \end{array} \right.}$$

Proof.

If i = j and if t is sufficiently close to zero in $\mathbb{Q}_{p^{h}}$ then $\exp (t\mathfrak{x}_{ii})$ is the diagonal matrix with entry exp(t) at the place (i, i) and 1 everywhere else on the diagonal. Recall that $\mathrm{GL}_{h}(\mathbb{Q}_{p^{h}})$ acts by fractional linear transformations on the projective coordinates $\varphi _{0},\ldots,\varphi _{h-1}$ of $\mathbb{P}_{K}^{h-1}$. Thus, $\exp (t\mathfrak{x}_{ii})(w_{\ell}) = w_{\ell}$ if $\ell\not =i\not =0$, $\exp (t\mathfrak{x}_{ii})(w_{i}) =\exp (t)w_{i}$ if i ≠ 0, and $\exp (t\mathfrak{x}_{00})(w_{\ell}) = \frac{1} {\exp (t)}w_{\ell}$ for all 1 ≤ ℓ ≤ h − 1.

If i ≠ j then $\exp (t\mathfrak{x}_{ij}) = 1 + t\mathfrak{x}_{ij}$ in $\mathrm{GL}_{h}(\mathbb{Q}_{p^{h}})$. Thus, $\exp (t\mathfrak{x}_{ij})(w_{\ell}) = w_{\ell}$ if $\ell\not =j\not =0$, $\exp (t\mathfrak{x}_{ij})(w_{j}) = w_{j} + tw_{i}$ if j ≠ 0, and $\exp (t\mathfrak{x}_{i0})(w_{\ell}) = w_{\ell}/(1 + tw_{i})$ for all 1 ≤ ℓ ≤ h − 1. Writing $f = f(w_{1},\ldots,w_{h-1})$ we have

$$\displaystyle{\exp (t\mathfrak{x}_{ij})(f\varphi _{0}^{m}) = f(\exp (t\mathfrak{x}_{ ij})(w_{1}),\ldots,\exp (t\mathfrak{x}_{ij})(w_{h-1})) \cdot \exp (t\mathfrak{x}_{ij})(\varphi _{0})^{m}.}$$

Here $\exp (t\mathfrak{x}_{ij})(\varphi _{0}) =\varphi _{0}$ if j ≠ 0, $\exp (t\mathfrak{x}_{00})(\varphi _{0}) =\exp (t)\varphi _{0}$ and $\exp (t\mathfrak{x}_{i0})(\varphi _{0}) =\varphi _{0} + t\varphi _{i}$ if 1 ≤ i ≤ h − 1. It is now an exercise in elementary calculus to derive the desired formulae.

Note that $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})^{\mathop{\text{rig}}\nolimits }$ is a $D(\varGamma _{2\varepsilon -1})$-stable K-subspace of M ₁ ^m and hence is $\mathfrak{g}$-stable. If m = 0 then Lemma 3 shows that in order to describe the $\mathfrak{g}$-action in the coordinates u ₁, …, u _h−1, one essentially has to compute the functional matrix

$$\displaystyle{F:= ( \frac{\partial u_{i}} {\partial w_{j}})_{1\leq i,j\leq h-1}.}$$

Proposition 3.

The matrix $A:= ( \frac{\partial \varphi _{i}} {\partial u_{j}}\varphi _{0} - \frac{\partial \varphi _{0}} {\partial u_{j}}\varphi _{i})_{1\leq i,j\leq h-1}$ over $R^{\mathop{\text{rig}}\nolimits }$ is invertible over the localization $R_{\varphi _{0}}^{\mathop{\text{rig}}\nolimits }$ . We have $F =\varphi _{ 0}^{2}A^{-1}$ , which is a matrix with entries in $\varphi _{0}R^{\mathop{\text{rig}}\nolimits }$ . Moreover, we have $\sum _{j=1}^{h-1}\varphi _{j} \frac{\partial u_{i}} {\partial w_{j}} \in \varphi _{0}^{2}R^{rig}$ for any index 1 ≤ i ≤ h − 1.

Proof.

Let $B:= ( \frac{\partial \varphi _{i}} {\partial u_{j}})_{0\leq i,j\leq h-1}$ with $\frac{\partial \varphi _{i}} {\partial u_{0}}:=\varphi _{i}$. We have $B \in \mathrm{GL}_{h}(R^{\mathop{\text{rig}}\nolimits })$ by a result of Gross and Hopkins (1994, Corollary 21.17). Setting

$$\displaystyle{N:= \left (\begin{array}{cccc} 1 &0&\cdots &0\\ -\varphi _{ 1} & \varphi _{0} & & 0\\ \vdots & & \ddots & \\ -\varphi _{ h-1} & 0& & \varphi _{0} \end{array} \right ),\mbox{ we have }NB = \left (\begin{array}{cccc} \varphi _{0} & \frac{\partial \varphi _{0}} {\partial u_{1}} & \cdots & \frac{\partial \varphi _{0}} {\partial u_{h-1}} \\ 0& & & \\ \vdots & &A \\ 0& & & \end{array} \right ).}$$

This already shows that A is invertible over $R_{\varphi _{0}}^{\mathop{\text{rig}}\nolimits }$. Denoting by c ₀, …, c _h−1 the columns of $B^{-1} = (c_{ij})_{i,j} \in \mathrm{GL}_{h}(R^{\mathop{\text{rig}}\nolimits })$, we obtain

$$\displaystyle{(\varphi _{0}^{-1}\sum _{ j=0}^{h-1}\varphi _{ j}c_{j},\varphi _{0}^{-1}c_{ 1},\ldots,\varphi _{0}^{-1}c_{ h-1}) = B^{-1}N^{-1} = \left (\begin{array}{cccc} \varphi _{0}^{-1} & {\ast}& \cdots &{\ast} \\ 0 & & & \\ \vdots & &A^{-1} & \\ 0 & & & \end{array} \right ).}$$

By the chain rain rule we have

$$\displaystyle{\delta _{ij} = \frac{\partial w_{i}} {\partial w_{j}} =\sum _{ \ell=1}^{h-1}\frac{\partial w_{i}} {\partial u_{\ell}} \cdot \frac{\partial u_{\ell}} {\partial w_{j}} =\sum _{ \ell=1}^{h-1}\varphi _{ 0}^{-2}( \frac{\partial \varphi _{i}} {\partial u_{\ell}}\varphi _{0} - \frac{\partial \varphi _{0}} {\partial u_{\ell}}\varphi _{i}) \frac{\partial u_{\ell}} {\partial w_{j}},}$$

so that $F =\varphi _{ 0}^{2}A^{-1}$. As seen above, the right hand side has entries in $\varphi _{0}R^{\mathop{\text{rig}}\nolimits }$. Further, we have $\sum _{j=1}^{h-1}\varphi _{j} \frac{\partial u_{i}} {\partial w_{j}} =\sum _{ j=1}^{h-1}\varphi _{j}\varphi _{0}c_{ij} = -\varphi _{0}^{2}c_{i0} \in \varphi _{0}^{2}R^{\mathop{\text{rig}}\nolimits }$ for any index 1 ≤ i ≤ h − 1.

Together with Lemma 3, Proposition 3 shows that $\mathfrak{x}(u_{i}) \in R^{\mathop{\text{rig}}\nolimits }$ for any $\mathfrak{x} \in \mathfrak{g}$ and any 1 ≤ i ≤ h − 1, as was clear a priori. For h = 2, Lemma 3 and Proposition 3 reprove Gross and Hopkins (1994), formula (25.14).

Coming back to the $\mathfrak{g}$-module M ₁ ^m for general m, consider the subalgebra $\mathfrak{s}\mathfrak{l}_{h}$ of $\mathfrak{g}$ over $\mathbb{Q}_{p^{h}}$. Let $\mathfrak{t}$ denote the Cartan subalgebra of diagonal matrices in $\mathfrak{s}\mathfrak{l}_{h}$, and let $\{\varepsilon _{1},\ldots,\varepsilon _{h-1}\} \subset \mathfrak{t}^{{\ast}}$ denote the basis of the root system of $(\mathfrak{s}\mathfrak{l}_{h},\mathfrak{t})$ given by $\varepsilon _{i}(\mathrm{diag}(t_{0},\ldots,t_{h-1})):= t_{i-1} - t_{i}$. We let $\lambda _{1} \in \mathfrak{t}^{{\ast}}$ denote the fundamental dominant weight defined by $\lambda _{1}:= \frac{1} {h}\sum _{i=1}^{h-1}(h - i)\varepsilon _{ i}$. We have

$$\displaystyle{\lambda _{1}(\mathrm{diag}(t_{0},\ldots,t_{h-1})) = \frac{1} {h}\sum _{i=1}^{h-1}(h-i)(t_{ i-1} -t_{i}) = \frac{1} {h}((h- 1)t_{0} -\sum _{i=1}^{h-1}t_{ i}) = t_{0}}$$

for any element $\mathrm{diag}(t_{0},\ldots,t_{h-1}) \in \mathfrak{t} \subset \mathfrak{s}\mathfrak{l}_{h}$.

Proposition 4.

For any integer m ≥ 0, the subspace $W:=\sum _{\vert \alpha \vert \leq m}K \cdot w^{\alpha }\varphi _{0}^{m}$ of M ₁ ^m is $\mathfrak{g}$ -stable. The action of $\mathfrak{s}\mathfrak{l}_{h}$ on W is irreducible. More precisely, W is the irreducible $\mathfrak{s}\mathfrak{l}_{h}$ -representation of highest weight $m \cdot \lambda _{1}$ .

Proof.

It follows from Lemma 3 that W is stable under any element $\mathfrak{x}_{ij}$ with j ≠ 0 or $i = j = 0$. If 1 ≤ i ≤ h − 1 and if n is a non-negative integer then

$$\displaystyle{\mathfrak{x}_{i0}^{n}(w^{\alpha }\varphi _{ 0}^{m}) = [\prod _{\ell =0}^{n-1}(m -\vert \alpha \vert -\ell)] \cdot w^{\alpha }w_{ i}^{n}\varphi _{ 0}^{m},}$$

as follows from Lemma 3 by induction. Therefore, $\mathfrak{x}_{i0}(w^{\alpha }\varphi _{0}^{m}) = 0$ if | α | = m. If | α | < m then $\mathfrak{x}_{i0}(w^{\alpha })$ has degree | α | + 1 ≤ m. This proves that W is $\mathfrak{g}$-stable.

The above formula also shows that W is generated by $\varphi _{0}^{m}$ as an $\mathfrak{s}\mathfrak{l}_{h}$-representation. If $f\varphi _{0}^{m} \in W$ is non-zero, then Lemma 3 shows that $(\mathfrak{x}_{01}^{\alpha _{1}}\cdots \mathfrak{x}_{0(h-1)}^{\alpha _{h-1}})(f\varphi _{0}^{m})$ is a non-zero scalar multiple of $\varphi _{0}^{m}$ for a suitable multi-index α. Therefore, the $\mathfrak{s}\mathfrak{l}_{h}$-representation W is irreducible.

Finally, if $\mathfrak{x} =\mathrm{ diag}(t_{0},\ldots,t_{h-1}) \in \mathfrak{t}$ then $\mathfrak{x}(w^{\alpha }\varphi _{0}^{m}) = (t_{0}(m -\vert \alpha \vert ) +\sum _{ i=1}^{h-1}\alpha _{i}t_{i}) \cdot w^{\alpha }\varphi _{0}^{m}$ by Lemma 3. Here,

$$\displaystyle{t_{0}(m -\vert \alpha \vert ) +\sum _{ i=1}^{h-1}\alpha _{ i}t_{i} = t_{0}m +\sum _{ i=1}^{h-1}\alpha _{ i}(t_{i} - t_{0}) = (m \cdot \lambda _{1} -\sum _{i=1}^{h-1}\alpha _{ i}\sum _{\ell=1}^{i}\varepsilon _{ \ell})(\mathfrak{x}).}$$

This shows that m ⋅ λ ₁ is the highest weight of the $\mathfrak{s}\mathfrak{l}_{h}$-representation W.

Remark 2.

The statement of Proposition 4 can be deduced from a stronger result of Gross and Hopkins. Namely, if m = 1 then $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\mathop{\text{rig}}\nolimits }$ contains an h-dimensional algebraic representation of $\mathfrak{o}_{D}^{{\ast}}$ (cf. Gross and Hopkins 1994, Proposition 23.2). Under the restriction map $\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\mathop{\text{rig}}\nolimits } \rightarrow \mathop{\text{Lie}}\nolimits (\mathbb{G})_{1}^{\mathop{\text{rig}}\nolimits }$, the derived representation of $\mathfrak{g} = \mathfrak{d} \otimes _{\mathbb{Q}_{p}}\mathbb{Q}_{p^{h}}$ maps isomorphically to the $\mathfrak{g}$-representation W above.

We will now see that the action of $\mathfrak{g}$ on M ₁ ^m naturally extends to a certain divided power completion of the universal enveloping algebra $U_{K}(\mathfrak{g})$. Note that if i, j, r and s are indices between 0 and h − 1, then $\mathfrak{x}_{ij} \cdot \mathfrak{x}_{rs} =\delta _{jr}\mathfrak{x}_{is}$ in $\mathfrak{g} \simeq \mathfrak{g}\mathfrak{l}_{h}$. Therefore,

$$\displaystyle{[\mathfrak{x}_{ij},\mathfrak{x}_{rs}] =\delta _{jr}\mathfrak{x}_{is}-\delta _{is}\mathfrak{x}_{rj} = \left \{\begin{array}{l@{\quad }l} 0, \quad &\mbox{ if }j\not =r\mbox{ and }i\not =s, \\ \mathfrak{x}_{is}, \quad &\mbox{ if }j = r\mbox{ and }i\not =s, \\ - \mathfrak{x}_{rj}, \quad &\mbox{ if }j\not =r\mbox{ and }i = s, \\ \mathfrak{x}_{ii} - \mathfrak{x}_{jj},\quad &\mbox{ if }j = r\mbox{ and }i = s. \end{array} \right.}$$

Setting $\mathfrak{x}_{ij}':= p^{\delta _{0i}-\delta _{0j}}\mathfrak{x}_{ij}$, one readily checks that the same relations hold on replacing $\mathfrak{x}_{ij}$ by $\mathfrak{x}_{ij}'$ and $\mathfrak{x}_{rs}$ by $\mathfrak{x}_{rs}'$ everywhere. It follows that the elements $\mathfrak{x}_{ij}'$ span a free $\mathbb{Z}_{p^{h}}$-Lie subalgebra of $\mathfrak{g}$ that we denote by $\mathring {\mathfrak{g}}$. Since $\mathrm{ad}(\mathfrak{x}_{ij}')^{2} = 0$ if i ≠ j, and since $(\varepsilon _{i+1} -\varepsilon _{j})([\mathfrak{x}_{ij}',\mathfrak{x}_{ij}']) = 2$ if i < j, it follows from Bourbaki (2006), VIII.12.7 Théorème 2 (iii), that the W-lattice $\mathring{ \mathfrak{g}}$ of $\mathfrak{g}$ is the base extension from $\mathbb{Z}$ to W of a Chevalley order of $\mathfrak{g}$ in the sense of Bourbaki (2006), VIII.12.7 Définition 2.

For 0 ≤ i ≤ h − 1 and n ≥ 0 we set

$$\displaystyle{{\mathfrak{x}_{ii}'\choose n}:= \frac{\mathfrak{x}_{ii}'(\mathfrak{x}_{ii}' - 1)\cdots (\mathfrak{x}_{ii}' - n + 1)} {n!} \in U_{K}(\mathfrak{g}).}$$

We let $\mathcal{U}$ denote the W-subalgebra of $U_{K}(\mathfrak{g})$ generated by the elements $(\mathfrak{x}_{ij}')^{n}/n!$ for i ≠ j and n ≥ 0, as well as by the elements ${\mathfrak{x}_{ii}'\choose n}$ for 0 ≤ i ≤ h − 1 and n ≥ 0. It follows from Bourbaki (2006), VIII.7.12 Théorème 3, that $\mathcal{U}$ is a free W-module and that a W-basis of $\mathcal{U}$ is given by the elements

$$\displaystyle{b_{\ell mn}:= (\prod _{i<j}\frac{(\mathfrak{x}_{ij}')^{\ell_{ij}}} {\ell_{ij}!} ) \cdot (\prod _{i=0}^{h-1}{\mathfrak{x}_{ ii}'\choose m_{i}}) \cdot (\prod _{i>j}\frac{(\mathfrak{x}_{ij}')^{n_{ij}}} {n_{ij}!} )}$$

with $\ell= (\ell_{ij}),n = (n_{ij}) \in \mathbb{N}^{h(h-1)/2}$ and $m = (m_{i}) \in \mathbb{N}^{h}$. Here the products of the $\mathfrak{x}_{ij}'$ for i < j and i > j have to be taken in a fixed but arbitrary ordering of the factors. For split semisimple Lie algebras these constructions and statements are due to Kostant (cf. Kostant 1966, Theorem 1, where $\mathcal{U}$ is denoted by B).

We denote by $\hat{\mathcal{U}}$ the p-adic completion of the ring $\mathcal{U}$ and set

$$\displaystyle{\hat{U}_{K}^{\mathop{\text{dp}}\nolimits }(\mathring {\mathfrak{g}}):=\hat{ \mathcal{U}}\otimes _{ W}K.}$$

According to the above freeness result, any element of $\hat{U}_{K}^{\mathop{\text{dp}}\nolimits }(\mathring {\mathfrak{g}})$ can be written uniquely in the form $\sum _{\ell,m,n}d_{\ell mn}b_{\ell mn}$ with coefficients d _{ℓ m n} ∈ K satisfying d _{ℓ m n} → 0 as $\vert \ell\vert + \vert m\vert + \vert n\vert \rightarrow \infty$. Therefore, $\hat{U}_{K}^{\mathop{\text{dp}}\nolimits }(\mathring {\mathfrak{g}})$ is a K-algebra containing $U_{K}(\mathfrak{g})$. We view it as a K-Banach algebra with unit ball $\hat{\mathcal{U}}$ and call it the complete divided power enveloping algebra of $\mathring {\mathfrak{g}}$.

Theorem 8.

For any integer m the action of $\mathfrak{g}$ on $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{1}^{\mathop{\text{rig}}\nolimits }$ extends to a continuous action of $\hat{U}_{K}^{\mathop{\text{dp}}\nolimits }(\mathring {\mathfrak{g}})$ .

Proof.

The ring of continuous K-linear endomorphisms of $M_{1}^{m} = (\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{1}^{\mathop{\text{rig}}\nolimits }$ is a K-Banach algebra for the operator norm. Since the latter is submultiplicative, the set of endomorphisms with operator norm less than or equal to one is a p-adically separated and complete W-algebra. Therefore, it suffices to prove that any element of the form $(\mathfrak{x}_{ij}')^{n}/n!$, i ≠ j, or ${\mathfrak{x}_{ii}'\choose n}$, 0 ≤ i ≤ h − 1, has operator norm less than or equal to one on M ₁ ^m whenever n ≥ 0. If $\alpha \in \mathbb{N}^{h-1}$ and 0 ≤ i, j ≤ h − 1 then

$$\displaystyle{ \mathfrak{x}_{ij}^{n}(w^{\alpha }\varphi _{ 0}^{m}) = \left \{\begin{array}{l@{\quad }l} \alpha _{j}^{n}w^{\alpha }\varphi _{0}^{m}, \quad &\mbox{ if }i = j\not =0, \\ (m -\vert \alpha \vert )^{n}w^{\alpha }\varphi _{0}^{m}, \quad &\mbox{ if }i = j = 0, \\ n!{\alpha _{j}\choose n}w^{\alpha }w_{j}^{-n}w_{i}^{n}\varphi _{0}^{m},\quad &\mbox{ if }i\not =j\not =0, \\ n!{m -\vert \alpha \vert \choose n}w^{\alpha }w_{i}^{n}\varphi _{0}^{m},\quad &\mbox{ if }i\not =j = 0,\\ \quad \end{array} \right. }$$

(2)

as follows from Lemma 3 by induction. Here the generalized binomial coefficients are defined by

$$\displaystyle{{x\choose n}:= \frac{x(x - 1)\cdots (x - n + 1)} {n!} \in \mathbb{Z}}$$

for any integer x. Now $\vert \vert (\sum _{\alpha }d_{\alpha }w^{\alpha })\varphi _{0}^{m}\vert \vert _{1} =\sup _{\alpha }\{\vert d_{\alpha }\vert p^{-\vert \alpha \vert }\}$. Bearing in mind our convention w ₀ = 1, we obtain the claim for $(\mathfrak{x}_{ij}')^{n}/n!$ if i ≠ j. If 0 ≤ i ≤ h − 1 then we obtain

$$\displaystyle{{\mathfrak{x}_{ii}'\choose n}(w^{\alpha }\varphi _{0}^{m}) = \left \{\begin{array}{l@{\quad }l} {\alpha _{i}\choose n}w^{\alpha }\varphi _{0}^{m}, \quad &\mbox{ if }i\not =0, \\ {m -\vert \alpha \vert \choose n}w^{\alpha }\varphi _{0}^{m},\quad &\mbox{ if }i = 0. \end{array} \right.}$$

This completes the proof.

Theorem 9.

Let m be an integer and set $c:= w_{1}^{\max \{-1,m\}+1}\varphi _{0}^{m}$ . The $U(\mathfrak{g})$ -submodule $U(\mathfrak{g}) \cdot c$ of $(\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{1}^{\mathop{\text{rig}}\nolimits }$ is dense. If h = 2 and m ≥−1 then $\hat{U}_{K}^{\mathop{\text{dp}}\nolimits }(\mathring {\mathfrak{g}}) \cdot c = (\mathop{\text{Lie}}\nolimits (\mathbb{G})^{\otimes m})_{1}^{\mathop{\text{rig}}\nolimits }$ .

Proof.

Equation (2) shows that $\mathfrak{x}_{01}^{\max \{-1,m\}+1}\mathfrak{x}_{10}^{\alpha _{1}}\cdots \mathfrak{x}_{(h-1)0}^{\alpha _{h-1}} \cdot c$ is a non-zero scalar multiple of $w^{\alpha }\varphi _{0}^{m}$. Thus, $K[w] \cdot \varphi _{0}^{m} \subset U_{K}(\mathfrak{g}) \cdot c$, proving the first assertion.

If h = 2 and m ≥ −1 let us be more precise. Setting $m':=\max \{ -1,m\} + 1$, w: = w ₁ and $\mathfrak{x}:= \mathfrak{x}_{10}'$, we have $\mathfrak{x}^{n} \cdot c = (-1)^{n}n!p^{-n}w^{n+m'}\varphi _{0}^{m}$ for any n ≥ 0 because ${-1\choose n} = (-1)^{n}$. If $f =\sum _{n\geq 0}d_{n}w^{n} \in R_{1}^{\mathop{\text{rig}}\nolimits }$ then d _n p ⁿ → 0 in K. Therefore, $\lambda:=\sum _{n\geq 0}d_{n+m'}(-p)^{n}\frac{\mathfrak{x}^{n}} {n!}$ converges in $\hat{U}_{K}^{\mathop{\text{dp}}\nolimits }(\mathring {\mathfrak{g}})$ and we have $f\varphi _{0}^{m} -\lambda \cdot c =\sum _{ n=0}^{m'-1}d_{n}w^{n}\varphi _{0}^{m}$. The latter is contained in $K[w] \cdot \varphi _{0}^{m} \subset U_{K}(\mathfrak{g}) \cdot c$, as seen above.

Remark 3.

By a result of Lazard, the image of $U_{K}(\mathfrak{g}) \simeq U_{K}(\mathfrak{d})$ in $\varLambda (\varGamma _{2\varepsilon -1})_{K,1}$ is dense (cf. Lazard 1965, Chapitre IV, Théorème 3.2.5). We state without proof that the completion of $U_{K}(\mathfrak{g})$ for the norm | | ⋅ | | ₁ embeds continuously into $\hat{U}_{K}^{\mathop{\text{dp}}\nolimits }(\mathring {\mathfrak{g}})$. However, a formal series like $\sum _{n\geq 0}p^{n}\frac{(\mathfrak{x}_{10}')^{n}} {n!} =\sum _{n\geq 0}\frac{\mathfrak{x}_{10}^{n}} {n!}$ does not converge in $\varLambda (\varGamma _{2\varepsilon -1})_{K,1}$. Therefore, one might have doubts whether M ₁ ^m is still finitely generated over $\varLambda (\varGamma _{2\varepsilon -1})_{K,1}$.