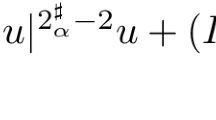Bifurcation Transitions and Expansions

Abstract

It is believed in matrix model theory that when the eigenvalue density on one interval is split to a new density on two disjoint intervals, a phase transition occurs. The complexity for the mathematical details of this physical phenomenon comes not only from the elliptic integral calculations, but also from the organization of the parameters in the model. Generally, the elliptic integrals do not have simple analytic formulations for discussing the transition. The string equations can be applied to find the critical point for the transition from the parameter bifurcation, and the bifurcation clearly separates the different phases for analyzing the free energy. Based on the expansion method for elliptic integrals, the third-order bifurcation transition for the Hermitian matrix model with a general quartic potential is discussed in this chapter by applying the nonlinear relations obtained from the string equations. The density on multiple disjoint intervals for higher degree potential and the corresponding free energy are discussed in association with the Seiberg-Witten differential. In the symmetric cases for the quartic potential, the third-order phase transitions are explained with explicit formulations of the free energy function.

It is believed in matrix model theory that when the eigenvalue density on one interval is split to a new density on two disjoint intervals, a phase transition occurs. The complexity for the mathematical details of this physical phenomenon comes not only from the elliptic integral calculations, but also from the organization of the parameters in the model. Generally, the elliptic integrals do not have simple analytic formulations for discussing the transition. The string equations can be applied to find the critical point for the transition from the parameter bifurcation, and the bifurcation clearly separates the different phases for analyzing the free energy. Based on the expansion method for elliptic integrals, the third-order bifurcation transition for the Hermitian matrix model with a general quartic potential is discussed in this chapter by applying the nonlinear relations obtained from the string equations. The density on multiple disjoint intervals for higher degree potential and the corresponding free energy are discussed in association with the Seiberg-Witten differential. In the symmetric cases for the quartic potential, the third-order phase transitions are explained with explicit formulations of the free energy function.

3.1 Free Energy for the One-Interval Case

In this section, we discuss the free energy [16]

$$ E = \int_{\varOmega} W(\eta) \rho(\eta) d \eta - \int_{\varOmega} \int_{\varOmega} \ln|\lambda- \eta| \rho(\lambda) \rho(\eta) d \lambda d \eta, $$

(3.1)

for the density

$$ \rho(\eta) = { 1\over\pi} k_{2m-2}(\eta) \sqrt{( \eta_{+} - \eta) (\eta- \eta_{-})},\quad \eta\in\varOmega= [ \eta_{-}, \eta_{+}], $$

(3.2)

obtained in Sect. 2.4 with the parameter conditions

$$\begin{aligned} &\sum_{j=2}^{2m} j g_j \sum _{p=0}^{ [ {j \over2} ]-1} \left ( \begin{array}{c} j-1 \\ 2 p+1 \end{array} \right ) \left ( \begin{array}{c} 2p+1 \\ p \end{array} \right ) a^{j - 2p - 2} b^{2p +2} = 1, \end{aligned}$$

(3.3)

$$\begin{aligned} &\sum_{j=1}^{2m} j g_j \sum _{p=0}^{ [ {j-1 \over2} ]} \left ( \begin{array}{c} j-1 \\ 2 p \end{array} \right ) \left ( \begin{array}{c} 2p \\ p \end{array} \right ) a^{j - 2p - 1} b^{2p } = 0. \end{aligned}$$

(3.4)

The following discussions are based on the results in [15]. As always, we assume ρ(η) is non-negative in the discussions.

Lemma 3.1

For ρ(η) defined by (3.2) on [η ₋,η ₊] with the parameters a, b and g _j (j=1,…,2m) satisfying the conditions (3.3) and (3.4), there is

$$\begin{aligned} \int_{\eta_{-}}^{\eta_{+}} \eta^{k} \rho(\eta) d \eta =& \sum_{j=2}^{2m} j g_{j} \sum_{q=1}^{j-1} \left ( \begin{array}{c} j-1 \\ q \end{array} \right ) a^{j-q-1} b^{q+1} \\ &{}\times \sum _{r=0}^{[q/2]-\mu_q} \left ( \begin{array}{c} q \\ {[q/2]+r+1} \end{array} \right ) R_{2r + \mu_{q}+1, k} \end{aligned}$$

(3.5)

where

$$ R_{l, k} ={i \over\pi} \int _{-\pi}^{\pi} (a + 2 b \cos\theta)^{k} e^{-i l \theta} \sin\theta d \theta, $$

(3.6)

with l=2r+μ _q +1 and μ _q =(1+(−1)^q)/2.

Proof

Let Ω ^∗ be the closed counterclockwise contour around lower and upper edges of [η ₋,η ₊], and Γ be a large counterclockwise circle. Since Ω ^∗ is counterclockwise, by the relation $\omega(\eta)|_{[\eta_{-}, \eta_{+}]^{\pm}} = \pm\pi i \rho (\eta)|_{[\eta_{-}, \eta_{+}]}$ and the Cauchy theorem we have

$$\int_{\eta_{-}}^{\eta_{+}} \eta^{k} \rho(\eta) d \eta = - {1 \over2 \pi i}\int_{\varOmega^{*}} \eta^{k} \omega(\eta) d \eta = - {1 \over2 \pi i}\int_{\varGamma} \eta^{k} \omega(\eta) d \eta. $$

So the problem becomes the calculation of the integral ∫ _Γ η ^k ω(η)dη.

By using the binomial formula and ∫ _Γ η ^k(α+b ² α ⁻¹)^q dη=∫ _Γ η ^k(η−a)^q dη=0, we can obtain

$$\begin{aligned} \int_{\eta_{-}}^{\eta_{+}} \eta^{k} \rho(\eta) d \eta =& {1 \over2 \pi i} \sum_{j=2}^{2m} j g_{j} \sum_{q=1}^{j-1} \left ( \begin{array}{c} j-1 \\ q \end{array} \right ) a^{j-q-1} \\ &{}\times\sum _{s=[q/2]+1}^{q} \left ( \begin{array}{c} q \\ s \end{array} \right ) b^{2s} \int_{\varGamma} \eta^{k} \alpha^{-(2s-q)} d \eta. \end{aligned}$$

(3.7)

Notice that the index q is changed to start from 1, and j is changed to start from 2.

On Ω ^∗, there is η=a+2bcosθ,−π≤θ≤π, where a=(η ₊+η ₋)/2 and 2b=(η ₊−η ₋)/2>0. Then α ⁻¹=b ⁻¹ e ^−iθ, where the square root takes positive and negative imaginary value on upper and lower edge of [η ₋,η ₊] respectively. By Cauchy theorem, the integral along Γ can be changed to along Ω ^∗, that implies

$$\int_{\varGamma} \eta^{k} \alpha^{-(2s-q)} d \eta = -2 b^{q-2s+1} \int_{-\pi}^{\pi} (a + 2 b \cos \theta)^{k} e^{-i (2s-q) \theta} \sin\theta d \theta. $$

Let r=s−[q/2]−1 in (3.7). Because the range of s is from [q/2]+1 to q, and q=2[q/2]−μ _q +1, the range of r is from 0 to [q/2]−μ _q . Since 2s−q=2r+μ _q +1, this lemma is proved. □

Lemma 3.2

For ρ(η) defined by (3.2) on [η ₋,η ₊] with the parameters a, b and g _j (j=1,…,2m) satisfying the conditions (3.3) and (3.4), there is

$$\begin{aligned} &\int_{\eta_{-}}^{\eta_{+}} \ln|\eta- a| \rho(\eta) d \eta \\ &\quad= \ln(2 b)- \sum_{j=2}^{2m} j g_{j} \sum_{q=1}^{j-1} \left ( \begin{array}{c} j-1 \\ q \end{array} \right ) a^{j-q-1} b^{q+1} \\ &\qquad{}\times\sum_{r=0}^{[q/2]-\mu_q} \left ( \begin{array}{c} q \\ {[q/2]+r+1} \end{array} \right ) \varTheta_{2r + \mu_{q}+1} \end{aligned}$$

(3.8)

where

$$ \varTheta_{l} = \operatorname{Re} {i \over\pi} \int_{0}^{\pi} \theta e^{i \theta} \bigl[ \bigl( e^{i \theta} + \sqrt{e^{2 i \theta} - 1} \bigr)^{l} - \bigl( e^{i \theta} - \sqrt{e^{2 i \theta} - 1} \bigr)^{l} \bigr] d \theta, $$

(3.9)

with l=2r+μ _q +1 and μ _q =(1+(−1)^q)/2.

Proof

Let γ=γ ₁∪γ ₂∪γ ₃ be a closed counterclockwise contour, where γ ₁ is the upper edges of [η ₋,a], γ ₂ is the upper edges of [a,η ₊], and γ ₃ is the semi-circle of radius 2b with center a. Applying Cauchy theorem for ln(η−a)ω(η), we have

$$\int_{\gamma_1} (\ln|\eta- a| + \pi i ) \omega(\eta) d \eta+ \int _{\gamma_2} \ln|\eta- a| \omega(\eta) d \eta+ \int _{\gamma_3} \ln\bigl(2 b e^{i \theta}\bigr) \omega(\eta) d \eta=0. $$

When η∈γ ₁∪γ ₂, ω(η)=πiρ(η). Then taking imaginary part for the above equation, we get

$$ \int_{\eta_{-}}^{\eta_{+}} \ln|\eta- a| \rho(\eta) d \eta - \ln(2 b) + {1 \over\pi} \operatorname{Re} \int _{\gamma_3} \theta \omega(\eta) d \eta= 0, $$

(3.10)

where we have used $\int_{\gamma_{3}} \omega(\eta) d \eta= - \int_{\gamma_{1} \cup\gamma_{2}} \omega(\eta) d \eta= - \pi i \int_{\gamma_{1} \cup\gamma_{2}} \rho(\eta) d \eta= - \pi i$. So the problem becomes the calculation of the integral $\int_{\gamma_{3}} \theta \omega(\eta) d \eta$.

Rewrite the formula of ω(η) given in Sect. 2.4 for the one-interval case as

$$\omega(\eta) = {1 \over2} \sum_{j=2}^{2m} j g_j \sum_{q=1}^{j-1} \left ( \begin{array}{c} j -1 \\ q \end{array} \right ) a^{j-q-1} \sum _{s=0}^{ [q/2] - \mu_q} \left ( \begin{array}{c} q \\ s \end{array} \right ) b^{2s} \bigl( \alpha^{q-2s} - \bigl(b^2 \alpha^{-1}\bigr)^{q-2s} \bigr). $$

Let r=[q/2]−μ _q −s. The range of r is from 0 to [q/2]−μ _q . Since q=2[q/2]−μ _q +1, and q−2s=2([q/2]−μ _q −s)+μ _q +1, we have the following,

$$\begin{aligned} \omega(\eta) =& {1 \over2} \sum_{j=2}^{2m} j g_{j} \sum_{q=1}^{j-1} \left ( \begin{array}{c} j-1 \\ q \end{array} \right ) a^{j-q-1} \\ &{}\times\sum_{r=0}^{[q/2]-\mu_q} \left ( \begin{array}{c} q \\ { [q/2] + r+1} \end{array} \right ) b^{2 ([q/2]- \mu_{q} -r)} \bigl( \alpha^{2r + \mu_{q}+1} - \bigl(b^2 \alpha^{-1} \bigr)^{2r + \mu_{q}+1} \bigr). \end{aligned}$$

On γ ₃, we have η−a=2be ^iθ, which implies $\alpha= b ( e^{i \theta} + \sqrt{e^{2 i \theta} - 1} ), b^{2} \alpha^{-1} = b ( e^{i \theta} - \sqrt{e^{2 i \theta} - 1} )$. It follows that

$$\begin{aligned} &\int_{\gamma_3} \theta \bigl( \alpha^{2r + \mu_{q}+1} - \bigl(b^2 \alpha ^{-1}\bigr)^{2r + \mu_{q}+1} \bigr) d \eta \\ &\quad= 2 i b^{2r + \mu_{q}+2} \int_{0}^{\pi} \theta e^{i \theta} \bigl[ \bigl( e^{i \theta} + \sqrt{e^{2 i \theta} - 1} \bigr)^{2r + \mu_{q}+1} \\ &\qquad{}- \bigl( e^{i \theta} - \sqrt{e^{2 i \theta} - 1} \bigr)^{2r + \mu_{q}+1} \bigr] d \theta. \end{aligned}$$

We finally have

$$\begin{aligned} &{1 \over\pi} \operatorname{Re} \int_{\gamma_3} \theta \omega(\eta) d \eta \\ &\quad=\sum_{j=2}^{2m} j g_{j} \sum_{q=1}^{j-1} \left ( \begin{array}{c} j-1 \\ q \end{array} \right ) a^{j-q-1} b^{q+1} \sum _{r=0}^{[q/2]-\mu_q} \left ( \begin{array}{c} q \\ {[q/2]+r+1} \end{array} \right ) \varTheta_{2r + \mu_{q}+1}. \end{aligned}$$

Then by (3.10), the lemma is proved. □

The Θ _l in the above lemma can be further simplified by the recursion for some elementary integrals as described in the following.

Lemma 3.3

For k=0,1,2,…, there are

$$\begin{aligned} & \int_{0}^{\pi} \theta e^{2 i \theta} \bigl(1 - e^{2 i \theta}\bigr)^{k + {1 \over2}} d \theta= { \pi\over(2 k + 3) i}, \end{aligned}$$

(3.11)

$$\begin{aligned} & \int_{0}^{\pi} \theta e^{ i \theta} \bigl(1 - e^{2 i \theta}\bigr)^{k + {1 \over2}} d \theta= - 2 \int_{0}^{1} \int_{0}^{1} \bigl( 1 - x^2 y^2 \bigr)^{k + {1 \over2}} dx dy + {\pi i \over2} B \biggl( {1 \over2}, k + {3 \over2} \biggr), \end{aligned}$$

(3.12)

where B(⋅,⋅) is the Euler beta function.

Proof

The first equation in this lemma can be easily verified by using integration by parts,

$$\int_{0}^{\pi} \theta e^{2 i \theta} \bigl(1 - e^{2 i \theta}\bigr)^{k + {1 \over2}} d \theta = {1 \over(2 k + 3) i} \int _{0}^{\pi} \bigl(1 - e^{2 i \theta} \bigr)^{k + {3 \over2}} d \theta = { \pi\over(2 k + 3) i}. $$

To prove the second equation, consider the initial value problems for J(γ) and I(γ) defined by

$$J(\gamma) = \int_{0}^{\pi} e^{i \theta} \bigl(1 - \gamma e^{2 i \theta}\bigr)^{k + {1 \over2}} d \theta,\qquad I(\gamma) = \int _{0}^{\pi} \theta e^{i \theta} \bigl(1 - \gamma e^{2 i \theta}\bigr)^{k + {1 \over2}} d \theta, $$

for 0≤γ≤1. It can be calculated that $( \gamma^{1 \over2} J(\gamma) )^{\prime} = i \gamma^{- {1 \over2}} (1 - \gamma)^{k + {1 \over2}}$, where ′=d/dγ. Then $\gamma^{1 \over2} J(\gamma) = i \int_{0}^{\gamma} t^{- {1 \over2}} (1 - t)^{k + {1 \over2}} d t$, which implies

$$ J(\gamma) = 2 i \int_{0}^{1} \bigl(1 - \gamma x^2\bigr)^{k + {1 \over2}} d x, $$

(3.13)

by taking t=γx ².

It can be calculated that $( \gamma^{1 \over2} I(\gamma) )^{\prime} = {\pi i \over2} \gamma^{- {1 \over2}} (1 - \gamma)^{k + {1 \over2}} - {1 \over2 i} \gamma^{- {1 \over2}} J(\gamma)$. Then by (3.13) and taking integral from 0 to 1, we have

$$I(1) = {\pi i \over2} \int_{0}^{1} \gamma^{- {1 \over2}} (1 - \gamma )^{k + {1 \over2}} d \gamma - \int _{0}^{1} \gamma^{-{1 \over2}} \biggl( \int _{0}^{1} \bigl( 1 - \gamma x^2 \bigr)^{k + {1 \over2}} dx \biggr) d\gamma, $$

which gives the second equation in this lemma by taking γ=y ². □

To further calculate the real part of the right hand side of (3.12), consider the following line and double integrals for k=0,1,2,…,

$$l_k = \int_{0}^{1} \bigl( 1 - x^2 \bigr)^{k + {1 \over2}} dx,\qquad d_k = \int _{0}^{1} \int_{0}^{1} \bigl( 1 - x^2 y^2 \bigr)^{k + {1 \over2}} dx dy. $$

First, $l_{0} = {\pi\over4}$, and

$$ d_0 = {1 \over2} \int_{0}^{1} \biggl( \sqrt{1 - y^2} + {1 \over y} \sin ^{-1} y \biggr) dy ={ \pi\over8} + {\pi\over4} \ln2. $$

(3.14)

When k≥1, by integration by parts, we can verify that l _k satisfy a recursive relation $l_{k} = l_{k-1} - {1 \over2 k+1} l_{k}$, which gives $l_{k} = {(2k+1)!! \over(2k+2)!!} {\pi\over2}$. Also by integration by parts, we have $d_{k} = d_{k-1} + {1 \over2 k + 1} (l_{k} - d_{k})$, which implies

$$ d_{k} = {2 k+ 1 \over2 k + 2} d_{k-1} + {(2k+1)!! \over(2k+2)!!} {\pi \over4(k+1)}. $$

(3.15)

Specially

$$ d_1 = {9 \pi\over64} + {3 \pi\over16} \ln2, $$

(3.16)

which will be used in the non-symmetric density discussed later. By combining the above discussions, we have the following result for the free energy.

Theorem 3.1

For ρ(η) defined by (3.2) on [η ₋,η ₊] with the parameters a, b and g _j (j=1,…,2m) satisfying the conditions (3.3) and (3.4), there is the following formula for the free energy:

$$\begin{aligned} E =& {1 \over2} W(a) - \ln(2b) + \sum _{j=2}^{2m} j g_{j} \sum _{q=1}^{j-1} \left ( \begin{array}{c} j-1 \\ q \end{array} \right ) a^{j-1-q} b^{q+1} \\ &{}\times\sum _{r=0}^{[q/2]-\mu_q} \left ( \begin{array}{c} q \\ {[q/2]+r+1} \end{array} \right ) Y_{2r + \mu_{q}+1}, \end{aligned}$$

(3.17)

where

$$ Y_{l} = {1 \over2} \sum_{k=0}^{2m} g_{k} R_{l, k} + \varTheta_{l}, $$

(3.18)

with l=2r+μ _q +1 and μ _q =(1+(−1)^q)/2.

Proof

By taking the integral on the variational equation $\mbox{(P)} \int_{\varOmega} {\rho(\lambda) \over\eta- \lambda} d\lambda= {1 \over2} W^{\prime}(\eta)$ from a to η for the variable η, we have $\int_{\eta_{-}}^{\eta_{+}} \ln|\lambda- \eta| \rho(\lambda) d \lambda= {1 \over2} W(\eta) - {1 \over2} W(a) + \int_{\eta_{-}}^{\eta_{+}} \ln |\lambda-a| \rho(\lambda) d \lambda$. Multiplying ρ(η) and taking $\int_{\eta_{-}}^{\eta_{+}} d \eta$ on both sides of this equation, we get the following by using $\int_{\eta_{-}}^{\eta_{+}} \rho(\eta) d \eta= 1$,

$$\begin{aligned} &\int_{\eta_{-}}^{\eta_{+}} \int_{\eta_{-}}^{\eta_{+}} \ln|\lambda- \eta| \rho(\lambda) \rho(\eta) d \lambda d \eta \\ &\quad= {1 \over2} \int_{\eta_{-}}^{\eta_{+}} W(\eta) \rho(\eta) d \eta - {1 \over2} W(a) + \int _{\eta_{-}}^{\eta_{+}} \ln|\lambda-a| \rho(\lambda) d \lambda. \end{aligned}$$

According to the definition of the free energy, there is

$$E = {1 \over2} W(a) + {1 \over2} \sum _{k=0}^{2m} g_k \int_{\eta_{-}}^{\eta_{+}} \eta^{k} \rho(\eta) d \eta - \int_{\eta_{-}}^{\eta_{+}} \ln|\eta-a| \rho(\eta) d \eta. $$

By Lemma 3.1 and Lemma 3.2, the integrals above can be expressed in terms of R _l,k and Θ _l . After simplifications, the result is proved. □

For the potential W(η)=g ₀+g ₁ η+g ₂ η ²+g ₃ η ³+g ₄ η ⁴, based on the above results and the restriction conditions

$$\begin{aligned} & 2 g_2 + 6 g_3 a + 12 g_4 \bigl(a^2 + b^2\bigr) - b^{-2} = 0, \end{aligned}$$

(3.19)

$$\begin{aligned} & g_1 + 2 g_2 a + 3 g_3 \bigl(a^2 + 2 b^2\bigr) + 4 g_4 a \bigl(a^2 + 6 b^2\bigr) = 0. \end{aligned}$$

(3.20)

simplified from (3.3) and (3.4), there are

$$\begin{aligned} &Y_1 = {1 \over2} \biggl( W(a) + {1 \over2} - 4 g_4 b^4\biggr) + {1 \over2} + \ln2, \\ &Y_2 = -2 (g_3 + 4 g_4 a) b^3, \\ &Y_3 = {1 \over2} \biggl( {1 \over2} - 3 g_4 b^4 \biggr) - {3 \over4}. \end{aligned}$$

The integrals in the free energy function are discussed in Appendix A. Therefore, by (3.17) and the parameter conditions (3.19) and (3.20), the free energy function in this case becomes

$$E = {1 \over2} W(a) - \ln(2 b) + Y_1 + 3 (g_3 + 4 g_4 a) b^3 Y_2 + 4 g_4 b^4 Y_3, $$

which can be further simplified to

$$ E = W(a) + {3 \over4} - \ln b - 4 g_4 b^4 - 6 (g_3 + 4 g_4 a)^2 b^6 - 6 g_4^2 b^8. $$

(3.21)

If we choose g ₂ as a variable, g ₀,g ₁,g ₃ and g ₄ as constants, and a and b as functions of g ₂, then we have

$$ {\partial^2 \over\partial g_2^2} E = -2 b^2 \bigl(2 a^2 + b^2\bigr), $$

(3.22)

where the derivatives of a and b have fractional formulas, but there are some common factors in the calculations that can be canceled, and finally we get the above result. It will be seen that this formula is consistent with the formula of $\partial^{2} / \partial t_{2}^{2} \ln Z_{n}$ to be discussed in next section. The domain of the above free energy function is determined by the condition k ₂(η)≥0 when η∈[η ₋,η ₊], which is generally not trivial. Let us consider a simple case in the following.

When W(η)=g ₀+g ₃ η ³+g ₄ η ⁴, the free energy function is

$$ E = g_0 + {3 \over8} - \ln b - { 8 \over3 \tau\tau_1} - {15 \tau+ 32 \over3 \tau_1} - {140 \tau - 40 \over3 \tau_{1}^2}, $$

(3.23)

where

$$\tau= {4 b^2 \over a^2},\quad \tau_1 = 5 + 3 (1 - \tau)^2. $$

As discussed in Sect. 2.5, the parameter τ is restricted in the intervals (0,τ ₋] and [τ ₊,∞), where τ ₋≈0.28 and τ ₊≈3.24.

When W(η)=g ₂ η ²+g ₄ η ⁴, the free energy becomes

$$ E = g_{0} + {3 \over4} - \ln b + {1 \over24} \bigl(2 g_2 b^2 - 1\bigr) \bigl(9 - 2 g_2 b^2\bigr), $$

(3.24)

which agrees with the result

$$ E(g) = E(0) + {1 \over24} \bigl(b^2 - 1\bigr) \bigl(9 - b^2\bigr) - {1 \over2} \ln b^2 . $$

(3.25)

obtained in [3] for $W(\eta) = {1 \over2} \eta^{2} + g \eta^{4}$. If we think E as a function of 2g ₂ b ², it can be seen that E has an extreme minimum point at 2g ₂ b ²=2, or at $g_{4} = g_{4}^{c}$, where $g_{4}^{c} = - {g_{2}^{2} \over12}$, which is always not positive. For the non-symmetric density discussed above, g ₄ is positive at such point.

If W(η)=g ₃ η ³+g ₄ η ⁴ is degenerated to W(η)=g ₄ η ⁴ by taking a→0, the free energy becomes E=3/8−lnb. It is the same result as W(η)=g ₂ η ²+g ₄ η ⁴ is degenerated to W(η)=g ₄ η ⁴. So the results obtained above are consistent. In this chapter, we talk about the third-order phase transitions for the potential

$$ W(\eta) = g_1 \eta+ g_2 \eta^2 + g_3 \eta^3 + g_4 \eta^4, $$

(3.26)

caused by the bifurcation of the parameter(s) in the density models, or when the density on one-interval is split to a two-interval case, that will be discussed in the following sections.

3.2 Partition Function and Toda Lattice

We have seen in last section that the free energy in the one-interval case can be explicitly derived. For the multiple-interval cases, the free energy is expressed in terms of elliptic integrals that are generally hard to be simplified. However, the derivatives of lnZ _n , which is how the free energy is defined, have some general properties that are useful to discuss the multiple-interval cases. In this section, we are going to discuss the derivatives of lnZ _n by using the equations obtained from the orthogonal polynomials for the potential

$$ V(z) = \sum_{j=0}^{4} t_j z^{j},\quad t_4 >0. $$

(3.27)

Let us first consider the derivatives ∂u _k /∂t ₁ for k=0,1,…,n−1, where $u_{0} h_{0} = \int_{-\infty}^{\infty} e^{-V(z)} dz$, and ∂v _k /∂t ₁ for k=1,2,…,n−1. Since $h_{0} = \int_{-\infty}^{\infty} e^{-V(z)} d z$, there is ${\partial h_{0} \over\partial t_{1}} = -\int_{-\infty}^{\infty} z e^{-V(z)} d z = - u_{0} h_{0}$, which implies ${\partial\over\partial t_{1}} \ln h_{0} = -u_{0}$. When k≥1, $h_{k} = \int_{-\infty}^{\infty} p_{k}^{2} e^{-V(z)} d z$, and

$${\partial h_k \over\partial t_1} = -\int_{-\infty}^{\infty} z p_k^2 e^{-V(z)} d z = - u_k h_k. $$

Therefore, we have

$$ {\partial\over\partial t_1} \ln h_k = -u_k, $$

(3.28)

for k≥0. Since p ₀=1, zp ₀=p ₁+u ₀ p ₀, p ₁=z−u ₀, and $p_{1, t_{1}} = v_{1} p_{0}$ obtained from the orthogonality $\int_{-\infty}^{\infty} p_{1, t_{1}} p_{0} e^{-V(z)} d z = \int_{-\infty}^{\infty} p_{1, t_{1}} p_{0} V_{t_{1}} (z) e^{-V(z)} d z = v_{1} h_{0}$, we have

$$ {\partial u_{0} \over\partial t_1} = - v_1. $$

(3.29)

When k≥1, taking ∂/∂t ₁ on both sides of the recursion formula zp _k =p _k+1+u _k p _k +v _k p _k−1 and applying the relation $p_{k, t_{1}} = v_{k} p_{k-1}$, there is

$$ {\partial u_{k} \over\partial t_1} = v_k - v_{k+1}. $$

(3.30)

In addition, since v _k =h _k /h _k−1 for k≥1, there is

$$ {\partial v_{k} \over\partial t_1} = v_k \biggl({\partial\ln h_k \over\partial t_1} - {\partial\ln h_{k-1} \over\partial t_1} \biggr) = v_k (u_{k-1} - u_k). $$

(3.31)

The differential equations above and in the following for the u _k and v _k are generally called Toda lattice in the Hermitian matrix models. In the t ₁ direction, these equations can be changed to the original Toda lattice for a chain of particles with nearest neighbor interaction by using the Flaschka’s variables. Since the partition function

$$Z_n = \int_{-\infty}^{\infty} \cdots\int _{-\infty}^{\infty} e^{- \varSigma_{i=1}^{n} V(z_i)} {\prod _{j<k}} (z_j - z_k)^2 dz_1 \cdots dz_n $$

can be expressed as

$$ Z_n = n! h_0 h_1 \cdots h_{n-1}, $$

(3.32)

we then get

$$ {\partial\over\partial t_1} \ln Z_n = - (u_0 + \cdots+ u_{n-1}), $$

(3.33)

and

$$ {\partial^2 \over\partial t_1^2} \ln Z_n = v_n. $$

(3.34)

Next, let us consider the derivatives with respect to t ₂. According to (3.32), we first need ∂h _k /∂t ₂ for k=0,1,…,n−1. Since $h_{0} = -\int_{-\infty}^{\infty} e^{-V(z)} d z$, there is

$${\partial h_0 \over\partial t_2} = -\int z^2 e^{-V(z)} d z = - \int(p_1 - u_0)^2 e^{-V(z)} d z = - \bigl(u_0^2 + v_1\bigr) h_0, $$

which implies

$$ {\partial\over\partial t_2} \ln h_0 = -\bigl(u_0^2 + v_1\bigr). $$

(3.35)

When k≥1, there is

$$ {\partial\over\partial t_2} \ln h_k = -\bigl(u_k^2 + v_k + v_{k+1}\bigr), $$

(3.36)

by applying the recursion formula twice to $\partial h_{k} / \partial t_{2} = -\int z^{2} p_{k}^{2} e^{-V(z)} d z$. Hence, we get

$$ {\partial\over\partial t_2} \ln Z_n = - \bigl(u_0^2 + v_1\bigr) - \sum_{k=1}^{n-1} \bigl(u_k^2 + v_k + v_{k+1}\bigr). $$

(3.37)

To get the second-order derivative of lnZ _n , we need to consider the derivatives of u _k and v _k . Since u ₀ h ₀=∫ze ^−V(z) dz, we can get ∂u ₀/∂t ₂=−(u ₀+u ₁)v ₁, and similarly

$${\partial v_1 \over\partial t_2} = v_1 \biggl({\partial\ln h_1 \over\partial t_2} - {\partial\ln h_0 \over\partial t_2} \biggr) = - v_1 \bigl(u_1^2 - u_0^2 + v_2\bigr). $$

Then, there is

$$ {\partial \over\partial t_2}\bigl(u_0^2 + v_1 \bigr) = - v_1 \bigl((u_0 + u_1)^2 + v_2\bigr). $$

(3.38)

When k≥1, by $u_{k} h_{k} = \int z p_{k}^{2} e^{-V(z)} dz$ we can get

$$ {\partial u_k \over\partial t_2} = - (u_{k+1} + u_k) v_{k+1} + (u_{k} + u_{k-1}) v_{k}. $$

(3.39)

Similar to ∂v ₁/∂t ₂, there is

$$ {\partial v_k \over\partial t_2} = v_k \biggl({\partial\ln h_k \over\partial t_2} - {\partial\ln h_{k-1} \over\partial t_2} \biggr) = - v_k \bigl(u_k^2 - u_{k-1}^2 + v_{k+1} - v_{k-1}\bigr). $$

(3.40)

When all the u _k ’s vanish in the even potential case, this equation becomes the equation discussed by Fokas, Its and Kitaev in 1991. The following result is true for k≥1,

$$ {\partial\over\partial t_2} \bigl(u_k^2 + v_k + v_{k+1}\bigr) = - v_{k+2} v_{k+1} + v_k v_{k-1} - v_{k+1} (u_{k+1} + u_k)^2 + v_k (u_k + u_{k-1})^2. $$

(3.41)

By taking derivative with respect to t ₂ on both sides of (3.37), and applying the formulas (3.38) and (3.41) above, we get the following identity after canceling the like terms,

$$ {\partial^2 \over\partial t_2^2} \ln Z_n = v_n\bigl( (u_n + u_{n-1})^2 + v_{n-1} + v_{n+1}\bigr). $$

(3.42)

We can also study the second-order derivatives in the t ₃ or t ₄ direction. It can be found that the second-order derivatives have simple formulations in terms of u _n ’s and v _n ’s. As we have experienced and to be discussed later that the u _n and v _n formulation for the second-order derivatives of lnZ _n always leads to the continuity of the second-order derivatives of the free energy. If the second-order derivative of the free energy has a singularity, then the center or radius parameter reduced from u _n or v _n also has singularity, and vice versa. These observations partially explain the cause of the third-order transitions we will discuss next by using the string equation and Toda lattice.

3.3 Merged and Split Densities

In this section, we discuss the properties of the densities on one or two intervals to get ready for the analysis for the phase transition problems. In some literatures, the densities are classified as weak or strong densities. For a convenience of the geometrical imagination, the densities discussed here are just called merged or split density depending the parameters in the density is in the merged or split state. Also, we will explain that it is generally hard to have a explicit or simple formula for the free energy function or the derivative the free energy to analyze the discontinuity at the critical point since for the split density the free energy is generally expressed in terms of the elliptic integrals, even in some special cases, like the symmetric case to be discussed in Sect. 3.5, the free energy has a simple formula as the one-interval case given in Sect. 3.1. Therefore, the ε-expansion method discussed in Sect. 3.4 would be generally applicable.

Let us consider the potential $W(\eta) = \sum_{j=1}^{4} g_{j} \eta^{j}$ and the densities discussed in Chap. 1 on Ω ₁=[η ₋,η ₊] and $\varOmega_{2}=[\eta_{-}^{(1)}, \eta_{+}^{(1)}] \cup[\eta_{-}^{(2)}, \eta _{+}^{(2)}]$ on the real line. For the purpose in this section, let us write the density on Ω ₁ in the following form,

$$ \rho_{1}(\eta) = {1 \over2 \pi} \bigl(2 g_2 + 3 g_3 (\eta+a) + 4 g_4 \bigl(\eta^2 + a \eta+ a^2 + 2 b^2\bigr)\bigr) \sqrt{4 b^2 - ( \eta-a)^2}, $$

(3.43)

where η∈Ω ₁=[a−2b,a+2b] and the parameters are restricted to satisfy the following relations

$$\begin{aligned} & 2 g_2 + 6 g_3 w_1 + 12 g_4 \bigl(w_1^2 + w_2\bigr) - w_2^{-1} = 0, \end{aligned}$$

(3.44)

$$\begin{aligned} & g_1 + 2 g_2 w_1 + 3 g_3 \bigl(w_1^2 + 2 w_2\bigr) + 4 g_4 w_1 \bigl(w_1^2 + 6 w_2\bigr) = 0, \end{aligned}$$

(3.45)

and

$$ w_1 = a, \qquad w_2 = b^2. $$

(3.46)

For the density on Ω ₂, let us first write it in the following form,

$$\begin{aligned} \rho_2(\eta) =& {1 \over2 \pi} \bigl(3 g_{3} + 4 g_{4} (\eta+ a_1+a_2 ) \bigr) \\ &{}\times\operatorname{Re} \sqrt{e^{-\pi i} \bigl[ \bigl( ( \eta-a_1) (\eta-a_2) - b_1^2 -b_2^2 \bigr)^2 - 4 b_1^2 b_2^2\bigr]}, \end{aligned}$$

(3.47)

subject to the following conditions

$$\begin{aligned} & 4 g_4 w_2^{2} - 1 = 0, \end{aligned}$$

(3.48)

$$\begin{aligned} & 2 g_2 + (3g_3 + 4g_4 w_1) w_1 - 4 g_4 w_3 = 0, \end{aligned}$$

(3.49)

$$\begin{aligned} & g_1 - (3 g_3 + 4 g_4 w_1) w_3 = 0, \end{aligned}$$

(3.50)

where

$$ w_1=(a_1+a_2)/2, \qquad w_2=b_1 b_2,\qquad w_3=a_1 a_2 - b_1^2 - b_2^2. $$

(3.51)

The w _j parameters are introduced in an attempt to have a unified density formula for the merged and split phases. The parameters of the polynomial in the square root in (3.47) can be written in terms of the w _j ’s according to the following factorization,

$$\begin{aligned} & \bigl( (\eta-a_1) (\eta-a_2) - b_1^2 -b_2^2 \bigr)^2 - 4 b_1^2 b_2^2 \\ &\quad= \biggl[ \biggl( \eta- {a_1+a_2 \over2} \biggr)^2 - {1 \over4} (a_1-a_2)^2 - (b_1 - b_2)^2 \biggr] \\ &\qquad{}\times \biggl[ \biggl( \eta- {a_1+a_2 \over2} \biggr)^2 - {1 \over4} (a_1-a_2)^2 - (b_1 + b_2)^2 \biggr] \\ &\quad= \bigl[ ( \eta- w_1 )^2 - w_1^2 + 2 w_2 + w_3 \bigr] \bigl[ ( \eta- w_1 )^2 - w_1^2 - 2 w_2 + w_3 \bigr] \\ &\quad = \bigl(\eta- \eta_{-}^{(1)} \bigr) \bigl(\eta- \eta_{+}^{(1)}\bigr) \bigl(\eta- \eta _{-}^{(2)}\bigr) \bigl(\eta- \eta_{+}^{(2)} \bigr),\quad \eta_{-}^{(1)} < \eta_{+}^{(1)} < \eta_{-}^{(2)} < \eta_{+}^{(2)}. \end{aligned}$$

(3.52)

The real part of the square root in (3.47) takes a negative sign when $\eta\in[\eta_{-}^{(1)}, \eta_{+}^{(1)}]$, and it takes a positive sign when $\eta\in[\eta_{-}^{(2)}, \eta_{+}^{(2)}]$. In fact, if we denote $Q(\eta) = (\eta- \eta_{-}^{(1)}) (\eta- \eta_{+}^{(1)}) (\eta- \eta _{-}^{(2)}) (\eta- \eta_{-}^{(2)})$, then

$$ \sqrt{ e^{-\pi i} Q(\eta) } = \begin{cases} {|\sqrt{ Q(\eta) }|} e^{ \pi i}, & \eta_{-}^{(1)} < \eta< \eta_{+}^{(1)}, \\ {|\sqrt{ Q(\eta) }|} , & \eta_{-}^{(2)} < \eta< \eta_{+}^{(2)}, \end{cases} $$

(3.53)

obtained according to the following argument values that if $\eta\in(\eta_{-}^{(1)}, \eta_{+}^{(1)})$, then $\operatorname{arg}(\eta- \eta_{-}^{(1)})=0$, and $\operatorname{arg}(\eta- \eta^{\prime})=\pi$ for $\eta^{\prime} = \eta_{+}^{(1)}, \eta_{-}^{(2)}, \eta_{+}^{(2)}$; and if $\eta\in(\eta_{-}^{(2)}, \eta_{+}^{(2)})$, there are $\operatorname{arg}(\eta- \eta^{\prime})=0$ for $\eta^{\prime} = \eta_{-}^{(1)}, \eta_{+}^{(1)}, \eta_{-}^{(2)}$, and $\operatorname{arg}(\eta- \eta_{+}^{(2)})=\pi$. Therefore, we also need 3g ₃+4g ₄(η+a ₁+a ₂)≤0 when $\eta\in[\eta_{-}^{(1)}, \eta_{+}^{(1)}]$, and 3g ₃+4g ₄(η+a ₁+a ₂)≥0 when $\eta\in[\eta_{-}^{(2)}, \eta_{+}^{(2)}]$ such that ρ ₂ keeps non-negative on Ω ₂.

When a ₁=a ₂=a=a _c and b ₁=b ₂=b=b _c for the fixed constants a _c and b _c >0, both of the density functions above are degenerated to

$$ \rho_c(\eta) = {1 \over\pi} 2 g_4^{c} (\eta- a_c)^2 \sqrt{ 4 b_c^2 - (\eta- a_c)^2 },\quad \eta\in[a_c - 2 b_c, a_c + 2 b_c], $$

(3.54)

at the critical point, and the parameters should take the values satisfying the following relations

$$\begin{aligned} & 4 g_4^{c} b_c^{4} = 1, \end{aligned}$$

(3.55)

$$\begin{aligned} & g_3^{c} + 4 g_4^{c} a_c = 0, \end{aligned}$$

(3.56)

$$\begin{aligned} & g_2^{c} + 3 g_3^{c} a_c + 2 g_4^{c} \bigl(3 a_c^2 + 2 b_c^2\bigr) = 0, \end{aligned}$$

(3.57)

$$\begin{aligned} & g_1^{c} - \bigl(3 g_3^{c} + 8 g_4^{c} a_c\bigr) \bigl(a_c^2 - 2 b_c^2\bigr) = 0, \end{aligned}$$

(3.58)

or equivalently

$$ \begin{aligned} &g_1^{c} = a_c \bigl(2 b_c^2 -a_c^2\bigr) b_c^{-4},\qquad 2 g_2^{c} = \bigl(3 a_c^2 - 2 b_c^2\bigr) b^{-4},\\ &3 g_3^{c} = - 3 a_c b_c^{-4}, \qquad 4 g_4^{c} = b_c^{-4}. \end{aligned} $$

(3.59)

Here, the relation (3.56) is obtained based on the non-negative requirement for ρ ₁ and ρ ₂ as discussed above.

One can image that it is hard to get the explicit formulas of the free energy function for the density ρ ₂. If we consider the difference of the derivatives of the free energy for ρ ₁ and ρ ₂ at the critical point, the computations may be easier since the densities have a unified structure that many common terms could be canceled to simplify the calculations. In the following, let us discuss whether this strategy works.

Recall that the density ρ ₁ on Ω ₁=[η ₋,η ₊]=[a−2b,a+2b] can be written as $\rho_{1} = {1 \over\pi} \sqrt{\det A^{(1)} }$ as shown in Chap. 1, where

$$ A^{(1)} = c_1 J^{(1)} + c_2 \bigl(J^{(1)}\bigr)^2 + c_3 \bigl(J^{(1)} \bigr)^3 - {1 \over2} W'(\eta) I, $$

(3.60)

with c ₁=2g ₂+6g ₃ a+12g ₄(a ²+b ²), c ₂=3g ₃+12g ₄ a, c ₃=4g ₄, I is the identity matrix, and

$$ J^{(1)} = \left ( \begin{array}{c@{\quad}c} 0 & 1 \\ -b^2 & \eta- a \end{array} \right ). $$

(3.61)

The density ρ ₂ can be changed to $\rho_{2} = {1 \over\pi} \sqrt{\det A^{(2)} }$, where

$$ A^{(2)} = \bigl(3 g_3 + 4 g_4 (\eta+ a_1 + a_2)\bigr) J^{(2)} - {1 \over2} W'(\eta) I, $$

(3.62)

with

$$ J^{(2)} = \left ( \begin{array}{c@{\quad}c} 0 & 1 \\ -b_1^2 & \eta- a_1 \end{array} \right ) \left ( \begin{array}{c@{\quad}c} 0 & 1 \\ -b_2^2 & \eta- a_2 \end{array} \right ). $$

(3.63)

Further, we have

$$ A^{(1)} = B^{(1)} \bigl(J^{(1)}\bigr)^2 - {1 \over2} W'(\eta) I, \quad\mbox{and}\quad A^{(2)} = B^{(2)} J^{(2)} - {1 \over2} W'(\eta) I, $$

(3.64)

where

$$B^{(1)} = \bigl(3 g_3 + 4 g_4 (\eta+ 2a)\bigr) I + \bigl(b^{-4} - 4 g_4\bigr) \left ( \begin{array}{c@{\quad}c} \eta- a & -1 \\ b^2 & 0 \end{array} \right ), $$

and

$$B^{(2)} = \bigl(3 g_3 + 4 g_4 (\eta+ a_1 + a_2)\bigr) I. $$

If we define

$$\begin{aligned} Q(\eta) &= {1 \over4} \bigl(W'\bigr)^2 - w_2^2 \bigl(3 g_3 + 4 g_4 ( \eta+ 2 w_1)\bigr)^2 \\ &\quad{}- \bigl(3 g_3 + 4 g_4 (\eta+ 2 w_1) \bigr) \bigl(1 - 4 g_4 w_2^2\bigr) (\eta- w_1) - w_2^{-1} \bigl(1 - 4 g_4 w_2^2\bigr)^2, \end{aligned}$$

(3.65)

for $\eta\in\mathbb{C}$, then

$$ - \det A^{(1)} = Q \vert_{\rho_1} \quad\mbox{and}\quad - \det A^{(2)} = Q \vert_{\rho_2}, $$

(3.66)

since $1 - 4 g_{4} w_{2}^{2}=0$ for ρ ₂, where $Q \vert_{\rho_{j}}$ means the parameters in Q are restricted by the parameter conditions for ρ _j , j=1,2.

Let us consider the continuity or discontinuity of the derivatives of the free energy function as the density is changed from ρ ₁ to ρ ₂. First, there is

$${\partial\sqrt{ Q } \over\partial g} { \bigg\vert}_{\rho_j} = {\langle Q_\mathbf{w}, \mathbf{w}^{\prime}\rangle + \langle Q_\mathbf{g}, \mathbf{g}^{\prime}\rangle \over2 \sqrt{ Q} } { \bigg\vert}_{\rho_j}, \quad j=1,2, $$

where w=(w ₁,w ₂,w ₃), g=(g ₁,g ₂,g ₃,g ₄), Q _w and Q _g are the gradients, ′=∂/∂g and g is one of the g _j ’s. It follows that

$${\partial\sqrt{ Q } \over\partial g} { \bigg\vert}_{\rho_{1}, g=g^c} - {\partial\sqrt{ Q } \over\partial g} { \bigg\vert}_{\rho_{2}, g=g^c} = {\langle Q_\mathbf{w}, \mathbf{w}^{\prime}\rangle \over2 \sqrt{Q} }{ \bigg\vert}_{\rho_{1}, g=g^c} - {\langle Q_\mathbf{w}, \mathbf{w}^{\prime}\rangle \over2 \sqrt{Q} }{ \bigg\vert}_{\rho_{2}, g=g^c}, $$

that implies the first-order derivative of the free energy is continuous at g=g ^c by the direct calculations from the free energy formula. For the second-order derivatives, we can get

$${\partial^2 \over\partial g^2} E(\rho_1) {\bigg \vert}_{g=g^c} - {\partial^2 \over\partial g^2} E(\rho_2) {\bigg \vert}_{g=g^c} = {-1 \over2 \pi i} \oint{\partial W(\eta) \over\partial g} {\langle Q_\mathbf{w}^{c}, \mathbf{w}^{(1)} - \mathbf{w}^{(2)}\rangle \over2 \sqrt{Q^c} } d \eta, $$

where $Q^{c} = Q \vert_{g = g^{c}}$, and w ⁽¹⁾ and w ⁽²⁾ are the corresponding vectors following the discussion above. It can be obtained that the second-order derivative is also continuous at the critical point.

However, for the higher order derivatives this method will lead to complicated calculations. So the analysis for the phase transition will not be easy if one hopes to use the explicit formulas in such a way to get the discontinuity. Similar complexities have been experienced in other researches, for example, see [1, 12, 20]. In the next section, we discuss a different method to simplify the calculations for analyzing the nonlinear properties in the transitions.

3.4 Third-Order Phase Transition by the ε-Expansion

After we have experienced the different strategies or properties in the previous sections, let us talk about the ε-expansion method. We will see that the ε-expansions for the parameters based on the algebraic equations reduced from the string equation can quickly give the phase transition properties including the critical phenomena to be discussed in the later chapters.

Consider the Hermitian matrix model with the potential $W(\eta) = \sum_{j=1}^{4} g_{j} \eta^{j}$. The phase transition problem is to discuss whether a derivative of the free energy function

$$ E = \int_{\varOmega} W(\eta) \rho(\eta) d \eta - \int _{\varOmega} \int_{\varOmega} \ln|\lambda- \eta| \rho( \lambda) \rho(\eta)d \lambda d \eta $$

(3.67)

becomes discontinuous as the density ρ is changed from one to another, for example, from ρ ₁ to ρ ₂. In the following, we will discuss that the discontinuity is mainly caused by the change of the parameter conditions.

Rewrite (3.47) as

$$ \rho_2(\eta) = {1 \over2 \pi} \bigl(3 g_{3} + 4 g_{4} (\eta+ 2 u ) \bigr) \operatorname{Re} \sqrt {e^{-\pi i} \bigl[ (\eta-u)^2 -x_1^2 \bigr] \bigl[ (\eta -u)^2 -x_2^2\bigr] }, $$

(3.68)

for η∈Ω ₂=[u−x ₂,u−x ₁]∪[u+x ₁,u+x ₂], where

$$\begin{aligned} &x_1^2 = u^2 - w -2 v = {1 \over4} (a_1 - a_2)^2 + (b_1 - b_2)^2, \end{aligned}$$

(3.69)

$$\begin{aligned} & x_2^2 = u^2 - w +2 v = {1 \over4} (a_1 - a_2)^2 + (b_1 + b_2)^2 \end{aligned}$$

(3.70)

and

$$ u=(a_1+a_2)/2,\qquad v=b_1 b_2,\qquad w=a_1 a_2 - b_1^2 - b_2^2. $$

(3.71)

Denote

$$ \omega_2(\eta) = {1 \over2} \bigl(3 g_{3} + 4 g_{4} (\eta+ 2 u ) \bigr) \sqrt{ \bigl[ ( \eta-u)^2 -x_1^2\bigr] \bigl[ ( \eta-u)^2 -x_2^2\bigr] }, $$

(3.72)

for η in the complex plane outside Ω ₂.

For the models considered in this book, we can discuss the first-order derivative as follows. Consider the following formulas for the density ρ ₂,

$$ \begin{cases} \mbox{(P)} \int_{\varOmega_2} {\rho_2(\lambda) \over\eta- \lambda} d\lambda={1 \over2} W^{\prime}(\eta),& \eta\in(\eta_{-}^{(1)}, \eta_{+}^{(1)}) \cup(\eta_{-}^{(2)}, \eta_{+}^{(2)}) , \\ \int_{\varOmega_2} {\rho_2(\lambda) \over\eta- \lambda} d\lambda={1 \over2} W^{\prime}(\eta) - \omega_2(\eta), & \eta\in(\eta_{+}^{(1)}, \eta_{-}^{(2)}). \end{cases} $$

(3.73)

The first formula above is the variational equation for the eigenvalue density. The second formula is, in fact, true for any η outside the cuts. For the discussion of the free energy, we specially pay attention to $\eta\in(\eta_{+}^{(1)}, \eta_{-}^{(2)})$. To prove the second formula, consider the counterclockwise contours $\varOmega_{2}^{*}$ around the cuts Ω ₂ and the counterclockwise circle $\gamma_{\eta}^{*}$ around a point η in the complex plane, see Fig. 3.1.

Fig. 3.1

Contours for the second formula in (3.73)

By Cauchy theorem, there is

$${1 \over2 \pi i} \int_{\varOmega_2^* \cup\gamma_{\eta}^*} {\omega_2(\lambda) \over\lambda- \eta} d \lambda= {1 \over2 \pi i} \int_{|\lambda|=R} {\omega_2(\lambda) \over\lambda- \eta} d \lambda, $$

where R is a large number, and the right hand side is equal to ${1 \over2} W'(\eta)$ as we have discussed before. For the left hand side, there is

$${1 \over2 \pi i} \int_{\varOmega_2^* } {\omega_2(\lambda) \over\lambda- \eta} d \lambda + {1 \over2 \pi i} \int_{ \gamma_{\eta}^*} {\omega_2(\lambda) \over \lambda- \eta} d \lambda = \int_{\varOmega_2} {\rho_2(\lambda) \over\eta- \lambda} d \lambda + \omega_2(\eta), $$

which shows the second formula above. Note that the point η can be large in the complex plane. The formula $\omega_{2}(\eta) = {1 \over2} W^{\prime}(\eta) + \int_{\varOmega_{2}} {\rho_{2}(\lambda) \over \lambda- \eta} d\lambda$ can be connected to the asymptotics $\omega_{2}(\eta) = {1 \over2} W'(\eta) - \eta^{-1} + O(\eta^{-2})$ as η→∞. In fact, when η→∞, $\int_{\varOmega_{2}} {\rho_{2}(\lambda) \over \lambda- \eta} d\lambda$ collects all the rest terms in the expansion of ω ₂ after the leading term ${1 \over2} W'(\eta)$.

Now, by the free energy formula (3.67) there is

$$\begin{aligned} {\partial \over\partial g} E(\rho_2) =& \int_{\varOmega_2} {\partial W(\eta) \over\partial g} \rho_2(\eta) d \eta \\ &{}- 2 \biggl( \int_{\eta_{-}^{(1)}}^{\eta_{+}^{(1)}} + \int _{\eta _{-}^{(2)}}^{\eta_{+}^{(2)}} \biggr) \biggl( \int _{\varOmega_2} \ln|\eta- \lambda| \rho_2(\lambda) d \lambda - {1 \over2} W(\eta) \biggr) {\partial\rho_2(\eta) \over\partial g} d \eta, \end{aligned}$$

(3.74)

where g represents any one of the g _j ’s. We can get that for $\eta\in(\eta_{-}^{(1)}, \eta_{+}^{(1)} )$, there is

$$ \int_{\varOmega_2} \ln|\eta- \lambda| \rho_2(\lambda) d \lambda- {1 \over2} W(\eta) = \int_{\varOmega_2} \ln| \eta_{-}^{(1)} - \lambda| \rho_2(\lambda) d \lambda- {1 \over2} W\bigl(\eta_{-}^{(1)}\bigr), $$

(3.75)

and for $\eta\in(\eta_{-}^{(2)}, \eta_{+}^{(2)} )$, there is

$$\begin{aligned} &\int_{\varOmega_2} \ln|\eta- \lambda| \rho_2(\lambda) d \lambda- {1 \over2} W(\eta) \\ &\quad= \int_{\varOmega_2} \ln|\eta_{-}^{(1)} - \lambda| \rho_2(\lambda) d \lambda- {1 \over2} W\bigl( \eta_{-}^{(1)}\bigr) - \int_{\eta_{+}^{(1)}}^{\eta_{-}^{(2)}} \omega_2(\eta) d \eta. \end{aligned}$$

(3.76)

Consequently, by using $\int_{\varOmega_{2}} {\partial\over\partial g} \rho _{2}(\eta) d \eta = {\partial\over\partial g} \int_{\varOmega_{2}} \rho_{2}(\eta) d \eta= 0$ there is the following result since ρ ₂ is equal to 0 at the end points of Ω ₂.

Theorem 3.2

For the free energy function (3.67), we have

$$ {\partial \over\partial g} E(\rho) = \begin{cases} \int_{\varOmega_1} {\partial W(\eta) \over\partial g} \rho_1(\eta) d \eta, &\rho=\rho_1, \\ \int_{\varOmega_2} {\partial W(\eta) \over\partial g} \rho_2(\eta) d \eta + 2 \int_{\eta_{+}^{(1)}}^{\eta_{-}^{(2)}} \omega_2(\eta) d \eta {\partial\over\partial g} \int_{\eta_{-}^{(2)}}^{\eta_{+}^{(2)}} \rho_2(\eta) d \eta,& \rho=\rho_2, \end{cases} $$

(3.77)

where g is one of the g _j ’s.

However, the higher order derivatives of the free energy will involve the elliptic integral calculations. It is experienced [1, 9, 12, 20] that the elliptic integral calculations are complicated. We have also discussed in last section that the algebraic method does not help too much to get a simple result for the free energy on the entire domain of the parameters. For the transition problems, it would be easier to just work on the behaviors around the critical point. The algebraic equations for the parameters obtained from the string equation can give the behaviors on both sides of the critical point by the ε-expansions. Also, when the degree of the potential is higher, the elliptic integrals will become more complicated, but the expansion method still works for the models of higher degree potentials to get at least the behaviors at the critical point(s) for analyzing the continuity or discontinuity.

Let us first consider the parameters in ρ ₂. Applying the notations u=(a ₁+a ₂)/2, v=b ₁ b ₂ and $w=a_{1} a_{2} - b_{1}^{2} - b_{2}^{2}$ into (3.48), (3.49) and (3.50). we see that (3.49) and (3.50) become

$$\begin{aligned} & 2 g_2 + 6 g_3 u + 12 g_4 \bigl(4 u^2 - w\bigr) =0, \end{aligned}$$

(3.78)

$$\begin{aligned} & g_1 - (3 g_3 + 8 g_4 u) w =0. \end{aligned}$$

(3.79)

Since the above equations do not involve v, let us expand u and w in terms of $\varepsilon_{1} = g_{2} - g_{2}^{c}$,

$$\begin{aligned} &u = u_c\bigl( 1 + \alpha_1 \varepsilon_1 + \alpha_2 \varepsilon_1^2 + \alpha_3 \varepsilon_1^3 + \cdots\bigr), \end{aligned}$$

(3.80)

$$\begin{aligned} &w = w_c \bigl(1 + \gamma_1 \varepsilon_1 + \gamma_2 \varepsilon_1^2 + \gamma_3 \varepsilon_1^3 + \cdots\bigr), \end{aligned}$$

(3.81)

where u _c =a _c , $w_{c}=a_{c}^{2} - 2 b_{c}^{2}$, $g_{j} = g_{j}^{c}$ for j=1,3,4, and

$$ \begin{aligned} &g_1^c = a_c\bigl(2 b_c^2 - a_c^2\bigr) b_c^{-4},\qquad g_2^c = {1 \over2} \bigl(3 a_c^2 - 2 b_c^2 \bigr) b_c^{-4},\\ & g_3^c = - a_c b_c^{-4},\qquad g_4^c = {1 \over4} b_c^{-4}, \end{aligned} $$

(3.82)

for fixed constants a _c and b _c >0. The ε is denoted as ε ₁ when it is negative, and we will explain next why it is negative in this case. The coefficients α _j and γ _j can be determined by using the equations above,

$$ \alpha_1 = - {b_c^2 \over2},\qquad \gamma_1 = - b_c^2,\qquad \alpha_2 = - {b_c^4 \over2} ,\qquad \gamma_2 = 0. $$

(3.83)

Note that u ²−w−2v, denoted as $x_{1}^{2}$ in the later discussion, is never negative because it is equal to (a ₁−a ₂)²/4+(b ₁−b ₂)². The expansions must be consistent with this property. The expansion results obtained above imply $u^{2} - w - 2 v= -2 b_{c}^{4} \varepsilon_{1} + O(\varepsilon_{1}^{2})$ that further imply ε ₁<0 and consequently $g_{2} < g_{2}^{c}$.

If we consider the g ₁ direction by choosing $g_{1} = g_{1}^{c} + \varepsilon _{1}$, then we get $\alpha_{1} = - {b_{c}^{2} \over4 a_{c}}$, $\gamma_{1} = - {a_{c} b_{c}^{2} \over2 (a_{c}^{2} - 2 b_{c}^{2}) }$, α ₂=0 and $\gamma_{2} = { b_{c}^{3} \over4 (a_{c}^{2} - 2 b_{c}^{2}) }$. In this case, it can be verified that $u^{2} - w - 2 v= -{3 \over16} b_{c}^{4} \varepsilon_{1}^{2} + O(\varepsilon_{1}^{3})$ which implies u ²−w−2v can be negative when ε ₁ is small. This is a contradiction since u ²−w−2v≥0. So there is no transition in the g ₁ direction in this case. In the following, we keep working on the g ₂ direction and consider the derivatives of the free energy in this direction, and g ₂ is related to the mass quantity in physics [9].

Now, let us consider the asymptotic expansion of the important term

$$ I_0 \equiv2 \int_{\eta_{+}^{(1)}}^{\eta_{-}^{(2)}} \omega_2 (\eta) d \eta {\partial \over\partial g} \int _{\eta_{-}^{(2)}}^{\eta_{+}^{(2)}} \rho_2 (\eta) d \eta, $$

(3.84)

in the formula of the first-order derivative of free energy (3.77). Basically, as g ₂ approaches to the critical value $g_{2}^{c}$, a ₁ and a ₂ approach to a _c , and b ₁ and b ₂ approach to b _c , that imply $\int_{\eta_{+}^{(1)}}^{\eta_{-}^{(2)}} \omega_{2} (\eta) d \eta$ is small, and ${\partial \over\partial g} \int_{\eta_{-}^{(2)}}^{\eta_{+}^{(2)}} \rho_{2} (\eta) d \eta$ is O(1). To get the higher order terms in the expansions, we use the contour integral technique in [15] (Sect. 6). In Sect. B.1, there is the following result,

$$ \int_{x_1}^{x_2} \sqrt{ \bigl(x_2^2 - x^2\bigr) \bigl(x^2 - x_1^2\bigr)} d x = {x_2^3 \over3} + O \bigl(x_1^2 \ln x_1\bigr), $$

(3.85)

as x ₁→0 with 0<x ₁<x ₂, based on which we can show the following lemma.

Lemma 3.4

For ρ ₂ defined by (3.47), there is

$$ \int_{\eta_{-}^{(2)}}^{\eta_{+}^{(2)}} \rho_2(\eta) d \eta = {1 \over2} - {2 \over\pi} a_c b_c \varepsilon_1 + O\bigl(\varepsilon_1^2 \ln| \varepsilon_1|\bigr), $$

(3.86)

as ε ₁→0, where $g_{2} = g_{2}^{c} + \varepsilon_{1}$ and ε ₁<0.

Proof

First, we have

$$\int_{\eta_{-}^{(2)}}^{\eta_{+}^{(2)}} \rho_2(\eta) d \eta = {1 \over2 \pi} \int_{\eta_{-}^{(2)}}^{\eta_{+}^{(2)}} \bigl(3 g_{3} + 4 g_{4} (\eta+ a_1+a_2 ) \bigr) \sqrt{ ( 2 v - \varLambda) ( \varLambda+2 v) } d \eta, $$

where v=b ₁ b ₂ and $\varLambda= (\eta-a_{1})(\eta-a_{2}) - b_{1}^{2} -b_{2}^{2}$. Then, $\int_{\eta_{-}^{(2)}}^{\eta_{+}^{(2)}} \rho_{2}(\eta) d \eta$ is equal to

$${ g_4 \over\pi} \int_{x_1^2}^{x_2^2} \sqrt { \bigl( x_2^2 - \zeta\bigr) \bigl( \zeta- x_1^2\bigr) } d \zeta + {1 \over2 \pi} (3 g_{3} + 12 g_{4} u ) \int_{x_{1}}^{x_{2}} \sqrt{ \bigl( x_2^2 - x^2\bigr) \bigl( x^2 - x_1^2\bigr) } d x, $$

where ζ=(η−u)², x=η−u and u=(a ₁+a ₂)/2 given above. We then obtain the following since $\int_{x_{1}^{2}}^{x_{2}^{2}} \sqrt{ ( x_{2}^{2} - \zeta) ( \zeta- x_{1}^{2}) } d \zeta = 2 \pi v^{2}$,

$$\int_{\eta_{-}^{(2)}}^{\eta_{+}^{(2)}} \rho_2(\eta) d \eta = 2 g_4 v^2 + {1 \over2 \pi} (3 g_{3} + 12 g_{4} u ) \int_{x_{1}}^{x_{2}} \sqrt { \bigl( x_2^2 - x^2\bigr) \bigl( x^2 - x_1^2\bigr) } d x. $$

By the formula (3.85), we further have

$$\int_{\eta_{-}^{(2)}}^{\eta_{+}^{(2)}} \rho_2(\eta) d \eta = 2 g_4 b_c^4 + {1 \over2 \pi} (3 g_{3} + 12 g_{4} u ) {x_2^3 \over3} + O\bigl( (3 g_{3} + 12 g_{4} u ) x_1^2 \ln x_1\bigr). $$

Since $4 g_{4} b_{c}^{4} = 1$, $3 g_{3} + 12 g_{4} u = 12 g_{4} a_{c} \alpha_{1} \varepsilon_{1} + O(\varepsilon_{1}^{2})$, $x_{1}^{2} = O(\varepsilon_{1})$ and x ₂=2b _c +O(ε ₁), the above expansion becomes

$$\int_{\eta_{-}^{(2)}}^{\eta_{+}^{(2)}} \rho_2(\eta) d \eta = {1 \over2} - {2 \over\pi} a_c b_c \varepsilon_1 + O\bigl(\varepsilon_1^2 \ln| \varepsilon_1|\bigr). $$

Then the lemma is proved. □

Lemma 3.5

$$ \int_{\eta_{+}^{(1)}}^{\eta_{-}^{(2)}} \omega_2(\eta) d \eta = {3 \pi \over2 } a_c b_c^{3} \varepsilon_1^2 + O\bigl(\varepsilon_1^3 \bigr), $$

(3.87)

as ε ₁→0, where $\varepsilon_{1} = g_{2} - g_{2}^{c} < 0$.

Proof

The formula

$$\int_{\eta_{+}^{(1)}}^{\eta_{-}^{(2)}} \omega_2(\eta) d \eta = {1 \over2 } \int_{\eta_{+}^{(1)}}^{\eta_{-}^{(2)}} \bigl(3 g_{3} + 4 g_{4} (\eta+ a_1+a_2 ) \bigr) \sqrt{ ( \varLambda- 2 v) ( \varLambda+2 v) } d \eta, $$

can be changed to

$$\begin{aligned} \int_{\eta_{+}^{(1)}}^{\eta_{-}^{(2)}} \omega_2(\eta) d \eta =& 2 g_4 \int_{u - x_1}^{u + x_1} (\eta- u) \sqrt{ \bigl( x_2^2 - (\eta-u)^2 \bigr) \bigl( x_1^2 - (\eta- u)^2\bigr) } d \eta \\ &{} + {1 \over2 } (3 g_{3} + 12 g_{4} u ) \int_{-x_{1}}^{x_{1}} \sqrt{ \bigl( x_2^2 - x^2\bigr) \bigl( x^2 - x_1^2\bigr) } d x, \end{aligned}$$

where $x_{1}^{2} = u^{2} - w -2 v$, $x_{2}^{2} = u^{2} - w +2 v$, and x=η−u. It is easy to see that $\int_{-x_{1}}^{x_{1}} t \sqrt{ ( x_{2}^{2} - t^{2} ) ( x_{1}^{2} - t^{2}) } d t = 0$ by the symmetry, that implies the first integral on the right hand side is equal to 0. Note that the integral here is from −x ₁ to x ₁, that is different from the integral in the normalization of the density. For the second integral, we first have the expansion $3 g_{3} + 12 g_{4} u = 12 g_{4} a_{c} \alpha_{2} \varepsilon_{1} + O(\varepsilon_{1}^{2}) = - {3 \over2} a_{c} b_{c}^{-2} \varepsilon_{1} + O(\varepsilon_{1}^{2})$. Then it follows that

$$\int_{\eta_{+}^{(1)}}^{\eta_{-}^{(2)}} \omega_2(\eta) d \eta = -{3 \over4 } a_c b_c^{-2} \varepsilon_1 \int_{-x_{1}}^{x_{1}} \sqrt { \bigl( x_2^2 - x^2\bigr) \bigl( x^2 - x_1^2\bigr) } d x + O\bigl( \varepsilon_1^3\bigr). $$

Since x ₂=2b _c +O(ε ₁) and $x_{1}^{2} = -2 b_{c}^{4} \varepsilon_{1} + O(\varepsilon_{1}^{2})$, where ε ₁<0, we have

$$\int_{-x_{1}}^{x_{1}} \sqrt{ \bigl( x_2^2 - x^2\bigr) \bigl( x^2 - x_1^2\bigr) } d x = {\pi\over2} x_2 x_1^2 + O\bigl(\varepsilon_1^2 \bigr) = -2 b_c^5 \varepsilon_1 + O\bigl( \varepsilon_1^2\bigr) . $$

Therefore, we finally get

$$\int_{\eta_{+}^{(1)}}^{\eta_{-}^{(2)}} \omega_2(\eta) d \eta = {3 \pi \over2 } a_c b_c^{3} \varepsilon_1^2 + O\bigl(\varepsilon_1^3 \bigr), $$

and the lemma is proved. □

By the lemmas above and (3.84), we have the following.

Lemma 3.6

$$ I_0 = -6 a_c^2 b_c^4 \varepsilon_1^2 + O\bigl(\varepsilon_1^3 \ln|\varepsilon_1|\bigr), $$

(3.88)

as ε ₁→0, where $\varepsilon_{1} = g_{2} - g_{2}^{c} < 0$.

The remaining work to find the expansion for

$${\partial E(\rho_2) \over\partial g_2} = \int_{\varOmega_2} \eta^2 \rho_2 d \eta+ I_0 $$

is the ε-expansion for $\int_{\varOmega_{2}} \eta^{2} \rho_{2} d \eta$. By the asymptotic expansion of $\omega_{2} = {1 \over2} (3 g_{3} + 4 g_{4} (\eta+ 2 u)) (\varLambda^{2} - 4 v^{2})^{1/2}$ as η→∞, we can get that the coefficient of the η ⁻³ is −[6g ₃ u+4g ₄(8u ²−w)]v ², which implies

$$\int_{\varOmega_2} \eta^2 \rho_2 d \eta = {-1 \over2 \pi i} \int_{|\eta| = R} \eta^2 \omega_2 d \eta = \bigl[6 g_3 u + 4 g_4\bigl(8 u^2 - w\bigr) \bigr] v^2. $$

Since we discuss the changes in the g ₂ direction by keeping other g _j ’s to their critical values and 4g ₄ v ²=1, we have that v is constant when $g_{2} < g_{2}^{c}$ for the ρ ₂ phase. Then, the ε ₁ expansions for u and w obtained above imply

$$ \int_{\varOmega_2} \eta^2 \rho_2 d \eta = a_c^2 + 2 b_c^2 - \bigl(4 a_c^2 + 2 b_c^2 \bigr) b_c^2 \varepsilon_1 - 3 a_c^2 b_c^4 \varepsilon_1^2 + O\bigl( \varepsilon_1^3 \ln|\varepsilon_1|\bigr). $$

(3.89)

Combining the discussions above, we finally get the following result for the free energy for the ρ ₂ as g ₂ approaches to $g_{2}^{c}$ from the left.

Theorem 3.3

For the ρ ₂ defined by (3.47), there is

$$ {\partial E(\rho_2) \over\partial g_2} = a_c^2 + 2 b_c^2 - \bigl(4 a_c^2 + 2 b_c^2 \bigr) b_c^2 \varepsilon_1 - 9 a_c^2 b_c^4 \varepsilon_1^2 + O\bigl(\varepsilon_1^3 \ln|\varepsilon_1|\bigr), $$

(3.90)

as ε ₁→0, where $\varepsilon_{1} = g_{2} - g_{2}^{c} <0$.

Now, let us consider the free energy for the density ρ ₁. By the asymptotic expansion of ω ₁(η) defined by

$$ \omega_{1}(\eta) = {1 \over2 } \bigl(2 g_2 + 3 g_3 (\eta+a) + 4 g_4 \bigl(\eta^2 + a \eta+ a^2 + 2 b^2\bigr)\bigr) \bigl((\eta-a)^2 - 4 b^2 \bigr)^{1/2}, $$

(3.91)

as η→∞ for η∉Ω ₁=[a−2b,a+2b], we can obtain that

$$\begin{aligned} {\partial E(\rho_1) \over\partial g_2} =& \int_{\varOmega_1} \eta^2 \rho_1 d \eta = {-1 \over2 \pi i} \int_{|\eta| = R} \eta^2 \omega_1 d \eta \\ =& u^2 + v + 6 g_3 u v^2 + 4 g_4 \bigl(6 u^2 v^2 + v^3\bigr), \end{aligned}$$

where u=a and v=b ². According to (3.19) and (3.20), the parameters satisfy

$$\begin{aligned} & 2 g_2 + 6 g_3 u + 12 g_4 \bigl(u^2 + v\bigr) - v^{-1} = 0, \\ & g_1 + 2 g_2 u + 3 g_3 \bigl(u^2 + 2 v\bigr) + 4 g_4 u \bigl(u^2 + 6 v\bigr) = 0. \end{aligned}$$

To get the concrete value of the derivative at the critical point, consider the expansions

$$\begin{aligned} &u = a_c\bigl( 1 + \alpha_1 \varepsilon+ \alpha_2 \varepsilon^2 + \cdots\bigr), \end{aligned}$$

(3.92)

$$\begin{aligned} &v = b_c^2 \bigl(1 + \beta_1 \varepsilon+ \beta_2 \varepsilon^2 + \cdots\bigr), \end{aligned}$$

(3.93)

where $\varepsilon= g_{2} - g_{2}^{c}$, and other g _j ’s take their critical values. Substituting the ε expansions above to the restriction equations above, we can derive the following results,

$$ \alpha_1 = - {b_c^2 \over2},\qquad \beta_1 = - {b_c^2 \over2},\qquad \alpha_2 = - {b_c^4 \over8}, \qquad \beta_2 = {b_c^2 \over16} \bigl( b_c^2 - 3 a_c^2 \bigr). $$

(3.94)

Then, the following result holds.

Theorem 3.4

For the ρ ₁ defined by (3.43), there is

$$ {\partial E(\rho_1) \over\partial g_2} = a_c^2 + 2 b_c^2 - \bigl(4 a_c^2 + 2 b_c^2 \bigr) b_c^2 \varepsilon + \bigl(3 a_c^2 + b_c^2\bigr) b_c^4 \varepsilon^2 + O\bigl( \varepsilon^3\bigr), $$

(3.95)

as ε→0, where $\varepsilon= g_{2} - g_{2}^{c} >0$.

By the results obtained above, we have that ${\partial^{j} E(\rho_{1}) /\partial g_{2}^{j}}$ and ${\partial^{j} E(\rho_{2}) / \partial g_{2}^{j}}$ are the same at the critical point $g_{2}^{c}$ for each j=1 or 2, but

$$ {\partial^3 E(\rho_2) \over\partial g_2^3} { \bigg\vert}_{g_2 \to g_2^c -0} = -18 a_c^2 b_c^4 < 2 \bigl(3 a_c^2 + b_c^2\bigr) b_c^4 = {\partial^3 E(\rho_1) \over\partial g_2^3} { \bigg\vert}_{g_2 \to g_2^c + 0}, $$

(3.96)

since b _c >0 in any case even a _c can be 0. It can be checked that the discontinuity mainly comes from the different coefficients (3.83) and (3.94) in the expansions. Because of the bifurcation of the center and radius parameters, a becomes a ₁ and a ₂, and b becomes b ₁ and b ₂, the combination or organization of the coefficients in the expansions is changed, and the transition is then called bifurcation transition, which is a third-order phase transition in the g ₂ direction. The I ₀ above enhances the discontinuity when a _c ≠0. In the symmetric case to be discussed in next section, there will be I ₀=0, but the discontinuity still exists. The importance of I ₀ appears in the Seiberg-Witten theory when the density is extended to the general density on multiple disjoint intervals as explained in the following.

The first-order derivatives of the free energy discussed above is related to the derivatives of the prepotential studied in the Seiberg-Witten theory [19], which is proportional to the logarithm of the partition function [4]. Let us consider the discussions in Sect. 2.4 in association with the multi-cut large-N limit of matrix models [4, 7]. For the eigenvalue density on multiple disjoint intervals $\varOmega=\bigcup_{j=1}^{l} \varOmega_{j} \equiv \bigcup_{j=1}^{l} [\eta_{-}^{(j)}, \eta_{+}^{(j)}]$, we have

$$ \begin{cases} \mbox{(P)} \int_{\varOmega} {\rho(\lambda) \over\eta- \lambda} d\lambda= {1 \over2} W^{\prime}(\eta), &\eta\in\varOmega, \\ \int_{\varOmega} {\rho(\lambda) \over\eta- \lambda} d\lambda= {1 \over2} W^{\prime}(\eta) - \omega(\eta),& \eta\in\mathbb{C} \diagdown\varOmega, \end{cases} $$

(3.97)

where $W(\eta) = \sum_{j=0}^{2m} g_{j} \eta^{j}$. Denote

$$ S_0(\eta) = {1 \over2} W(\eta) - \int _{\varOmega} \ln|\eta- \lambda| \rho(\lambda) d \lambda, $$

(3.98)

for η in the complex plane. By (3.97), for a point η in $(\eta_{-}^{(j)}, \eta _{+}^{(j)})$, there is $S_{0}(\eta) = S_{0}(\eta_{-}^{(1)}) + \sum_{k=1}^{j-1} \int_{\hat{\varOmega}_{k}} \omega (\eta) d \eta$, where $\hat{\varOmega}=\bigcup_{k=1}^{l-1} \hat{\varOmega}_{k} \equiv \bigcup_{k=1}^{l-1} (\eta_{+}^{(k)}, \eta_{-}^{(k+1)})$. And if $\eta\in(\eta_{+}^{(j)}, \eta_{-}^{(j+1)})$, then there is $S_{0}(\eta) = S_{0}(\eta_{-}^{(1)}) + \operatorname{Re} \int_{\eta_{-}^{(1)}}^{\eta} \omega(\lambda) d \lambda$. Since ω(η) is real on $\hat{\varOmega}$ and imaginary on Ω, there is the following general formula

$$ S_0(\eta) = S_0\bigl(\eta_{-}^{(1)} \bigr) + \operatorname{Re} \int_{\eta_{-}^{(1)}}^{\eta} \omega( \lambda) d \lambda, $$

(3.99)

or $d S_{0}(\eta) = \operatorname{Re} \omega(\eta) d \eta$ in the differential form. It can be seen that if η∈Ω, then

$$ {\partial S_0(\eta) \over\partial\eta} = 0, $$

(3.100)

since $\operatorname{Re} \omega(\eta) = 0$ on the cuts Ω. The function S ₀(η) is then a step function on $\varOmega\cup\hat{\varOmega}$, as discussed in the Seiberg-Witten theory, for example, see [4, 10]. The differential dS(η)=ω(η)dη for $\eta\in\mathbb{C} \diagdown\varOmega$ is corresponding to the Seiberg-Witten differential or the extended differentials defined on the Riemann surface, and free energy E is proportional to the prepotential.

Since the derivative of the free energy with respect to a potential direction g can be represented as

$$ {\partial \over\partial g} E = \int_{\varOmega} {\partial W(\eta) \over\partial g} \rho(\eta) d \eta + 2\int_{\varOmega} S_0(\eta) {\partial\rho(\eta) \over\partial g} d \eta, $$

(3.101)

as shown before, it is then equal to

$$ \int_{\varOmega} {\partial W(\eta) \over\partial g} \rho(\eta) d \eta + 2 S_0\bigl(\eta_{-}^{(1)}\bigr) \int _{\varOmega} {\partial\rho(\eta) \over\partial g} d \eta + 2 \operatorname{Re} \int_{\varOmega} \biggl( \int_{\eta_{-}^{(1)}}^{\eta} \omega(\lambda) {\partial\rho(\eta) \over\partial g} d \lambda \biggr) d\eta. $$

(3.102)

After simplifications, there is

$$ {\partial \over\partial g} E = \int_{\varOmega} {\partial W(\eta) \over\partial g} \rho(\eta) d \eta + 2 \sum_{j=2}^{l} \sum_{k=1}^{j-1} \int_{\hat{\varOmega}_{k}} \omega(\lambda) d \lambda {\partial\over\partial g} \int_{\varOmega_{j}} \rho(\eta) d \eta, $$

(3.103)

which is consistent to the result (3.77) for the two-cut case (l=2).

The Seiberg-Witten theory is developed to study the mass gap problem in the quantum Yang-Mills theory [11]. The linear combination of a and a _D [4, 10, 19], which are the integrals of the Seiberg-Witten differential over different intervals, is used to define the mass quantity according to the physical literatures. The connection between the Seiberg-Witten differential and the eigenvalue density in matrix models is important, that involves complicated mathematical and physical problems. A simple linear combination example of the integrals of the ρ and ω over different intervals is discussed in Sect. B.2 in order to experience the mathematical properties, and it is seen that the integrals are related to the Legendre’s relation in the elliptic integral theory.

The relevant researches, such as instanton, integrable systems, string theory, supersymmetric Yang-Mills theory and Whitham equations can be found, for example, in [2, 5–7, 14, 17, 18] and the references therein. There are also other methods to investigate the mass gap problem, including, for example, Euler-Lagrange equation, energy-momentum operator, renormalization, propagator and much more that can be found in the publications and the archive journals. There are many challenging problems in this field, and one important problem related to our discussion is to balance the finite freedoms and the integrability. In the position models such as the soliton integrable systems with infinitely many constants, it is usually complicated to reduce the infinite dimensional space by using the periodic conditions as studied in the quantum inverse scattering method [13]. In the momentum aspect, the integrable system characterized by the infinitely many functions such as the u _n and v _n can be reduced to the physical model with finite number of parameters such as the a _j and b _j satisfying certain conditions, and the degree of the potential polynomial determines the degree of the freedoms.

The string equations are related to the density problems in the Seiberg-Witten theory as explained above. But the entire story would be complicated. As Michael R. Douglas mentioned in 2004 [8], it remains a fertile ground for mathematical discovery. There is a large field behind this fundamental problem that will connect many different researches and motivate the developments of sciences. Uncertainty and Fourier transform are fundamental in this research area, that have been typically emphasized in [11], and are associated with diverse problems.

3.5 Symmetric Cases

On the real line, denote Ω ₁=[−η ₁,η ₁] and Ω ₂=[−η ₂,−η ₀]∪[η ₀,η ₂], and consider the potential W(η)=g ₂ η ²+g ₄ η ⁴, where g ₄>0. The densities on Ω ₁ and Ω ₂ are all symmetric now.

According to the discussion in last section, the density on Ω ₁ now becomes

$$ \rho_1 (\eta) = {1 \over \pi} \bigl( g_2 + g_4 \bigl(2 \eta^2 + \eta_1^2\bigr) \bigr) \sqrt { \eta_1^2 - \eta^2}, \quad \eta\in \varOmega_1, $$

(3.104)

where η ₁=2b, and the parameters satisfy the following conditions

$$\begin{aligned} & g_2 + 4 g_4 b^2 \ge0, \end{aligned}$$

(3.105)

$$\begin{aligned} & 2 g_2 b^2 + 12 g_4 b^4 = 1. \end{aligned}$$

(3.106)

It is easy to see that (3.105) implies ρ ₁(η)≥0 for η∈Ω ₁. The conditions (3.105) and (3.106) imply g ₂ b ²+1= 3(g ₂+4g ₄ b ²)b ²≥0, or −g ₂≤1/b ². When g ₂ is negative, there is (−g ₂)²≤(1/b ²)(4g ₄ b ²)=4g ₄, or

$$ g_2 \ge-2 \sqrt{g_4}. $$

(3.107)

When g ₂ is positive, (3.107) is also true. So (3.107) defines the region of the parameters for ρ ₁, and the parameters for ρ ₂ will stay in the region $g_{2} \le-2 \sqrt{g_{4}}$. As a remark, the ρ ₁ is an extension of the planar diagram density [3] $\rho(\eta) = {1 \over\pi} ({1 \over2} + 4 g b^{2} + 2 g \eta^{2}) \sqrt{4 b^{2} - \eta^{2}}$ for potential $W(\eta) = {1\over2} \eta^{2} + g_{4} \eta^{4}$ since the g ₂ considered here is negative around the critical point. The parameter g ₄ in [3] is negative as they discuss the singular point for the free energy. Here, we consider positive g ₄. The critical point $g_{2}/\sqrt{g_{4}} = -2$ was found early in 1982 by Shimamune [20], and the relevant discussions about the phase transition can also be found in [1, 9, 12], for instance.

The densities given above satisfy the normalization and the variational equation as shown before. It has been discussed in Sect. 3.1 that the free energy can be calculated by using the analytic function

$$ \omega_1(\eta) = \bigl( g_2 + g_4 \bigl(2 \eta^2 + \eta_1^2 \bigr) \bigr) \sqrt{\eta^2 - \eta _1^2}, \quad \eta\in\mathbb{C} \diagdown\varOmega_1, $$

(3.108)

which has the following asymptotics

$$ \omega_1(\eta) = {1 \over2} \bigl( 2 g_2 \eta^2 + 4 g_4 \eta^3 \bigr) - \bigl(g_2 + 6 g_4 b^2\bigr) {2 b^2 \over\eta} - \bigl(g_2 + 8 g_4 b^2\bigr) {2 b^4 \over\eta^3} + O\biggl({1 \over\eta^{5}} \biggr), $$

(3.109)

as η→∞ in the complex plane.

Now, let us consider

$$ \rho_2(\eta) = {2 g_4 \over\pi} \eta \operatorname{Re} \sqrt{e^{-\pi i} \bigl(\eta^2 - \eta_0^2\bigr) \bigl(\eta^2 - \eta_2^2\bigr) },\quad \eta\in\varOmega_2=[- \eta_2, -\eta_0] \cup[\eta_0, \eta_2], $$

(3.110)

where η ₀=b ₂−b ₁, η ₂=b ₁+b ₂, and the parameters satisfy

$$\begin{aligned} & 4 g_4 b_1^2 b_2^2 = 1, \end{aligned}$$

(3.111)

$$\begin{aligned} & g_2 + 2 g_4 \bigl(b_1^2 + b_2^2\bigr) = 0, \end{aligned}$$

(3.112)

which imply

$$ g_2 = -2 g_4 \bigl(b_1^2 + b_2^2\bigr) \le-4 g_4 b_1 b_2 = -2 \sqrt{g_4}. $$

(3.113)

Therefore, the regions of the parameters in densities (3.104) and (3.110) are separated by the curve

$$ g_2 = - 2 \sqrt{g_4}, $$

(3.114)

in the (g ₂,g ₄) plane. The ρ ₁ is defined when $g_{2} \ge- 2 \sqrt{g_{4}}$, and ρ ₂ is defined when $g_{2} \le- 2 \sqrt{g_{4}}$. In addition, since

$$ \sqrt{ \bigl(\eta^2 - \eta_0^2 \bigr) \bigl(\eta^2 - \eta_2^2\bigr) } = \begin{cases} |\sqrt{ (\eta^2 - \eta_0^2) (\eta^2 - \eta_2^2) }| e^{3 \pi i/2}, & -\eta_2 < \eta< -\eta_0, \\ |\sqrt{ (\eta^2 - \eta_0^2) (\eta^2 - \eta_2^2) }| e^{\pi i/2}, & \eta _0 < \eta< \eta_2, \end{cases} $$

(3.115)

there is ρ ₂(η)>0 when η∈(−η ₂,−η ₀)∪(η ₀,η ₂). In fact, if η∈(−η ₂,−η ₀). there are $\operatorname{arg}(\eta- (-\eta_{2}))=0$, and $\operatorname{arg}(\eta- \eta')=\pi$ for η′=−η ₀,η ₀,η ₂; and if η∈(η ₀,η ₂), there are $\operatorname{arg}(\eta- \eta')=0$ for η′=−η ₂,−η ₀,η ₀, and $\operatorname{arg}(\eta- \eta_{2})=\pi$.

Define another analytic function

$$ \omega_2(\eta) = 2 g_4 \eta\sqrt {\bigl(\eta^2 - \eta_0^2\bigr) \bigl(\eta^2 - \eta _2^2\bigr)}, \quad \eta\in \mathbb{C} \diagdown\varOmega_2, $$

(3.116)

which has the asymptotics

$$ \omega_2(\eta) = {1 \over2} \bigl( 2 \eta+ 4 g_4 \eta^3 \bigr) - {4 g_4 b_1^2 b_2^2 \over\eta} + O\bigl(\eta^{-3}\bigr), $$

(3.117)

as η→∞ in the complex plane. It can be checked that if b ₁=b ₂=b, then η ₀=0 and η ₁=η ₂, and the conditions (3.111) and (3.112) become 4g ₄ b ⁴=1 and g ₂+4g ₄ b ²=0 respectively, which imply (3.106). In other words, (3.111) and (3.112) can be thought as (3.106) is split to two equations as b is bifurcated to the two parameters b ₁ and b ₂. In the symmetric cases, we can experience the third-order phase transitions with the explicit formulations of the free energy function.

To calculate the free energy function, let us make a change for ρ ₂ by the following

$$ \rho_2(\eta) d \eta= {1 \over2} \hat{ \rho}_2 (\zeta) d \zeta, $$

(3.118)

where

$$ \hat{\rho}_2 (\zeta) = {2 g_4 \over\pi} \sqrt{( \zeta_{+} - \zeta) (\zeta- \zeta_{-}) }, $$

(3.119)

$\zeta_{-} = \eta_{0}^{2}$, $\zeta_{+} = \eta_{2}^{2}$, ζ=η ², and $W(\eta) = {1 \over2} \hat{W}(\zeta)$ with $\hat{W}(\zeta) = 2 g_{2} \zeta+ 2 g_{4} \zeta^{2}$ such that $\eta^{-1} \partial W(\eta) /\partial\eta= \partial\hat {W}(\zeta) /\partial\zeta$. The coefficients 1/2 and 2 above will make the following calculations easy. Since ρ ₂(η) satisfies $\int_{-\eta_{2}}^{-\eta_{0}} \rho_{2} (\eta) d \eta+ \int_{\eta_{0}}^{\eta_{2}} \rho_{2} (\eta) d \eta= 1$, and

$$\mbox{(P)} \int_{-\eta_2}^{-\eta_0} {\rho_2 (\lambda') \over\eta - \lambda'} d \lambda' + \mbox{(P)} \int_{\eta_0}^{\eta_2} {\rho_2 (\lambda) \over\eta- \lambda} d \lambda= {1 \over2} W'( \eta), $$

where ′ for W(η) means ∂/∂η, we can get $\int_{\zeta_{-}}^{\zeta_{+}} \hat{\rho}_{2} (\zeta) d \zeta=1$, and

$$ \mbox{(P)} \int_{\zeta_{-}}^{\zeta_{+}} {\hat{\rho}_2 (\xi) \over \zeta- \xi} d \xi= {1 \over2} \hat{W}'(\zeta), $$

(3.120)

by taking λ′=−λ, ξ=λ ² and ζ=η ², where ′ for $\hat{W}(\zeta)$ means ∂/∂ζ. Then, the free energy becomes

$$ E = {1 \over2} \biggl( \hat{W}(\hat{a}) + {3 \over4} - \ln\hat{b} \biggr), $$

(3.121)

by using the free energy for the one-interval case discussed in Sect. 3.1 for m=1, where $\hat{a} = {1 \over2} (\zeta_{-} + \zeta_{+}) = - {g_{2} \over2 g_{4}}$ and $\hat{b} = {1 \over4} (\zeta_{+} - \zeta_{-}) = {1 \over2 \sqrt{g_{4}}}$. One can get the same result by using the method in Sect. 3.1 and the asymptotics (3.117). The details are left to interested readers as an exercise.

The general formulas of the free energy for the potential W(η)=g ₂ η ²+g ₄ η ⁴ can be summarized in the following with the parameters restricted in the different regions,

$$ E = \begin{cases} {1 \over24} (2 g_2 v - 1) (9 - 2 g_2 v) - {1 \over2} \ln v + {3 \over4},& g_2 \ge-2 \sqrt{g_4}, \\ {3 \over8} - {g_2^2 \over4 g_4} + {1 \over4} \ln(4 g_4),& g_2 \le-2 \sqrt{g_4}, \end{cases} $$

(3.122)

where

$$ 2 g_2 v + 12 g_4 v^2 = 1. $$

(3.123)

The free energy function and its first- and second-order derivatives are always continuous. but its third-order derivatives are discontinuous as the parameters pass through the Shimamune’s critical curve $g_{2} = - 2 \sqrt{g_{4}}$ [20], which is different from the critical point $g_{2}^{2} + 12 g_{4} = 0$ in the planar diagram model [3]. In the following, we discuss the third-order discontinuities by choosing different forms of the potential W(η).

When $W(\eta) = - \hat{g} \eta^{2} + \eta^{4}$, we have from (3.122) that

$$ E = \begin{cases} - {1 \over24} (1 + 2 \hat{g} v) (9 + 2 \hat{g} v) - {1 \over2} \ln v + {3 \over4}, & \hat{g} \le2, \\ {3 \over8} - {\hat{g}^2 \over4} + {1 \over2} \ln2, & \hat{g} \ge2, \end{cases} $$

(3.124)

where $-2 \hat{g} v + 12 v^{2} =1$. At the critical point $\hat {g} = 2$, there are v=1/2 and v′=−1/8 where $'=d/d\hat{g}$. The free energy E is a continuous function of $\hat{g}$, and at the critical point $\hat{g}=2$ there is $E|_{\hat{g} \to2^{-}} = -5/8 + \ln\sqrt{2} = E|_{\hat{g} \to2^{+}}$. The derivative of E with respect to $\hat{g}$ can be obtained by direct calculations

$$ {d E \over d \hat{g}} = \begin{cases} -v - 4 v^3, & \hat{g} \le2; \\ - {\hat{g} \over2}, & \hat{g} \ge2. \end{cases} $$

(3.125)

Therefore, we have ${d E \over d \hat{g}} |_{\hat{g} \to2^{-}} = -1 = {d E \over d \hat{g}} |_{\hat{g} \to2^{+}}$, which implies the first-order derivative is continuous. The second-order derivative can be obtained as

$$ {d^2 E \over d \hat{g}^2} = \begin{cases} -2 v^2, & \hat{g} \le2, \\ - {1 \over2}, & \hat{g} \ge2. \end{cases} $$

(3.126)

And then

$$ {d^3 E \over d \hat{g}^3} = \begin{cases} - {4 v^3 \over1 + \hat{g} v}, & \hat{g} \le2, \\ 0, & \hat{g} \ge2. \end{cases} $$

(3.127)

Obviously, the third-order derivative is not continuous at the critical point $\hat{g} = 2$.

When W(η)=−2η ²+gη ⁴, we have

$$ E = \begin{cases} -{1 \over24} (1 + v ) (9 +v) - {1 \over2} \ln v + {3 \over4},& g \ge1, \\ {3 \over8} - {1 \over g} + {1 \over4} \ln(4 g), & 0 < g \le1, \end{cases} $$

(3.128)

where −4v+12gv ²=1. At the critical point g=1, there are v=1/2 and v′=−3/8 where ′=d/dg. We have

$$ {d E \over d g} = \begin{cases} (3 + 2 v) v^2, & g \ge1, \\ {1 \over g^2} + {1 \over4g}, & 0 < g \le1, \end{cases} $$

(3.129)

and

$$ {d^2 E \over d g^2} = \begin{cases} - 36 v^4, & g \ge1, \\ -{2 \over g^3} - {1 \over4 g^2}, & 0 < g \le1. \end{cases} $$

(3.130)

Then it can be seen that the first- and second-order derivatives are all continuous, and the third-order derivative is discontinuous at g=1.

When W(η)=T ⁻¹(−2η ²+η ⁴), we have

$$ E = \begin{cases} -{v \over3 T^2} (2 v + 5 T) - {1 \over2} \ln v + {3 \over8},& T \ge1, \\ {3 \over8} - {1 \over T} - {1 \over4} \ln{T \over4}, & T \le1, \end{cases} $$

(3.131)

for a positive parameter T (temperature) with −4v+12v ²=T such that v=1/2 when T=1. It can be get that $E|_{T \to1^{+}} = -5/8 + \ln\sqrt{2} = E|_{T \to1^{-}}$. Then, as a function of T, the derivative of the free energy takes the following form

$$ {d E \over d T} = \begin{cases} {v^2 (4 v + 5 T) \over T^3 (6 v -1)} - {1 \over8 v (6 v -1)},& T \ge1, \\ {1 \over T^2} - {1 \over4T}, & T \le1. \end{cases} $$

(3.132)

At the critical point T=1, dE/dT is continuous, and explicitly ${d E \over d T} |_{T \to1^{+}} = {3 \over4} = {d E \over d T} |_{T \to1^{-}}$. It is just direct calculations to check that the second-order derivative is continuous and the third-order derivative is discontinuous at T=1. As a remark, there is no difference if we consider a more general potential W(η)=T ⁻¹(−c ² η ²+η ⁴) for analyzing the discontinuous property. For simplicity, here we just show the results for c=1.

The criticality discussed above has negative $g_{2}^{c}$ and positive $g_{4}^{c}$. For potential $W(\eta) = \sum_{j=1}^{4} g_{j} \eta^{j}$, the critical point $g_{2}= g_{2}^{c}$ can be positive if $3 a_{c}^{2} > 2 b_{c}^{2}$ according to (3.59). One can experience that for the potential g ₂ η ²+g ₄ η ⁴+g ₆ η ⁶, the critical point $g_{2}^{c}$ will be positive. The parameter g ₂ is important because in physics it is related to the mass quantity as discussed in some literatures, for example, see [9]. In the planar diagram model [3], g ₄ is negative to let the parabola cover the semicircle forming a Gaussian kind distribution. By the relation (3.123), if we take g ₄ as a constant and g ₂ as a function of lnv, then

$$ {d g_2 \over d \ln v} = g_2 - v^{-1}. $$

(3.133)

So dg ₂/dlnv=0 implies g ₂=v ⁻¹ and $g_{4} =- {1 \over 12} v^{-2}$ (negative) satisfying $g_{2}^{2}/g_{4} = -12$. This is the critical case for the planar diagram model different from the critical point $g_{2}/\sqrt{g_{4}} = -2$ above. The transition problem for the planar diagram model will be discussed in Sect. 4.2.2.

The parameter bifurcation introduces a preliminary knowledge about the cause of the transition. The double zero point at the critical point, which is formed in two ways as explained in the following, is a main factor for the transition. For the potential W(η)=g ₂ η ²+g ₄ η ⁴ with the density $\rho_{c} = {2 g_{4} \over\pi} \eta^{2} \sqrt{4 b^{2} - \eta ^{2}}$ at the critical point, we have experienced that the double zero η=0 is from the parabola pushing the semicircle to give a gap in the density when we consider the density on one interval. For the density on two intervals, one zero is from the outside of the square root in the density model, and another zero is from the inside of the square root meeting with the outside zero to form a double zero. The density functions have behaviors O(|η−η ₀|²) at the bifurcation point η ₀=0 at the critical point, while normally at the end points the behaviors are of square root order. It is the same behaviors for the higher degree potentials except more double zero points. This would indicate that a matter is transferred during the transitions with the splitting or merging deformations at the middle points of the densities based on the bifurcations. The transition can be also caused by the deformation of the density at the largest (or smallest) end point of the eigenvalues in the distribution, in which case there will be a small system released or added at the largest eigenvalue point, that will be discussed in Sect. 4.3.3 by using double scaling method.