Keywords

1 Introduction

Engineering systems are exposed to dynamic effects because of the change in the location of the loads in the system over time. In such a structure, there is a dynamic response according to changing conditions that depends both on the loads acting on the system, and on the changes in the amplitude of loads. One of the moving load systems is a crane system on top of which there are moving carriages. Currently, there is a rich corpus of theoretical and experimental work on moving-load problems and a lot of related works and studies that can be outlined below.

K.H. Low presented a vibration analysis by using eigenfunctions for the various beams carrying multiple masses. Polynomial approximation mode analysis was given in comparison with analytical and experimental results in order to compare and validate the results for the proposed models [1].

Another study was outlined by K.H. Low—a comparative study of the eigenfrequency analysis for an Euler-Bernoulli beam carrying a concentrated mass to an arbitrary location [2].

Cha briefly summarized researches on the approximate and exact analyses and classified the analyzing approaches commonly used on the free vibration of a linear elastic structure carrying lumped mass, spring and damping at different points (such as Lagrange approach, Dynamic Green function approach, Laplace transform, and analytical and numerical solution methods) [3–5].

In a study conducted by Gürgöze, there were added several spring and mass systems along Euler-Bernoulli beam equations and it was developed an alternative formulation for the frequency of the beam [6]. Then, in another study by Gürgöze and Erol, was conducted a vibration analysis of the beam in which the system made of a spring and of a damper was investigated [7].

A simple supported uniformly Euler-Bernoulli beam carrying a crane which consisted of a carriage and a payload was modelled by D.C.D. Oguamanamand, J.S. Hansen, and G.R. Heppler. The crane carriage was modelled as a particle as the payload was assumed to be suspended from the carriage on a massless rigid rod and restricted to motion in the plane defined by the beam axis and the gravity vector. The two coupled integro-differential equations of motion were derived using Hamilton’s principle and the operational calculus was used to determine the vibration of the beam. Beam-natural frequencies of the vibrations for a crane system were detected, and the precise frequency was derived from the equations for these cases. Were presented numerical examples which covered the range of carriage speeds, carriage masses, pendulum lengths, and payload masses. The maximum deflection of the beam occurred at the end of the beam at high speeds because of inertia effects and in the middle of the beam at low speed because of the fact that the system was reduced to a quasi-static situation [8, 9].

In this study, was investigated a crane systems bridge element with both a single beam and two beams. Also, we considered a different number of carriages moving on top of the beams on the dynamic and the subsequent effects that occur under the moving load were analyzed by using FEM.

2 Dynamics of Bridge Crane System

Three dimensional bridge cranes are systems in which the load is lifted, moved, and dropped to the desired location by means of carriages. They are used in all factories and ports in different industries that handle heavy loads. The main requirement related to the crane is that the operations are performed to the desired destination as quickly as possible. Overhead cranes, built to support high constructions, consist of a bridge placed between the two crane paths. The movement to be performed by an overhead crane can be defined as follows:

  • Vertical movement along the OY axis: lifting and lowering movement;

  • Horizontal movement along the OZ axis: translational motion of the bridge;

  • Horizontal movement along the OX axis: the translational motion of the carriage on the bridge.

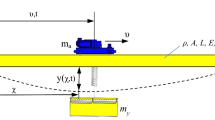
The front of a crane system while lifting and lowering and in the moving state of the carriage is shown in Fig. 1. Each state of the crane is specified in the system with a different available dynamic response. In this study, the carriage is moving on the crane system and all of the carried loads are applied. The total force in this case is shown in Fig. 2.

Fig. 1
figure 1

Bridge crane system and load carrying stages

Full size image
Fig. 2
figure 2

Analysis of loads moving on a beam

Full size image

A dynamic analysis is also performed for the case in which these forces move at a constant velocity on the system. Here, the bridge is considered both as a single beam and as double beam, and the beam is divided into 20 equal parts. The 20 separate loads are assumed to be moving to another area every one second and the time for moving a load is identified as shown in Fig. 3.

Fig. 3
figure 3

Moving load modelling

Full size image

The general motion equation with multiple degrees of freedom of the systems is given by [10]:

$$ \left[m \right]\left\{\ddot{\textit{q}} \right\} + \left[c \right]\left\{{\dot{q}} \right\} + \left[k \right]\left\{q \right\} = \left\{{P(t)} \right\} $$
(1)

where; \( \left[ m \right] \) is the matrix of mass of the classified structure, \( \left[ c \right] \) is the damping matrix, \( \left[ k \right] \) is the rigidity matrix,\( \left\{\ddot {\textit{q}} \right\} \) is the vector of acceleration of all structures, \( \left\{ {\dot{q}} \right\} \) is the vector of velocity of all structures, \( \left\{ q \right\} \) is the vector of displacement of all structures, and \( \left\{ {P(t)} \right\} \) is the vector or all external forces to the system.

Moving load analysis was performed on the basis of this simulation as external load time-dependent finite elements are applied to the model. As can be seen in Fig. 3, the maximum load specified when applying to the first region and other regions is considered to be zero. According to the time, other field force definition is considered zero. Clough and Penzien have expressed the specified outer force vector in the form defined according to [11]:

$$ \left\{ {P(t)} \right\} = \left\{ {0\;\;0\;\;0\;\; \ldots \;\;f_{1}^{(s)} f_{2}^{(s)} f_{3}^{(s)} f_{4}^{(s)} \;\; \ldots \;\;0\;\;0\;\;0} \right\} $$
(2)

where \( f_{i}^{(s)} (t),(i = 1,2,3,4) \) is defined as equivalent node forces:

$$ \left\{ {f^{(s)} (t)} \right\} = P\left\{ N \right\} $$
(3)

where P is the value of the vertical force applied from the top of the beam and \( \left\{ N \right\} \) is a function of shape and is expressed as follows:

$$ \left\{ N \right\} = \left[ {N_{1} N_{2} N_{3} N_{4} } \right]^{T} $$
(4)

Shape functions can be described in the following equations as in [11, 12]:

$$ N_{1} = 1 - 3\xi^{2} + 2\xi^{3} $$
(5)
$$ N_{2} = l\left( {\xi - 2\xi^{2} + \xi^{3} } \right) $$
(6)
$$ N_{3} = 3\xi^{2} - 2\xi^{3} $$
(7)
$$ N_{4} = l\left( { - \xi^{2} + \xi^{3} } \right) $$
(8)
$$ \xi = \frac{x}{l} $$
(9)

where l is the length of the element (s), implemented on the vertical direction along the element x. P is the distance from the point where the force was applied, as shown in Fig. 4.

Fig. 4
figure 4

Equivalent nodal forces of the element s subjected to a moving load

Full size image

3 Simulation Results and Discussions

In this study, the length of the bridge is L = 20 m both on one beam and on two beams. 600 mm width 1000 mm height and 8 mm thickness rectangular box profiles are taken as sections of the beam (-s). The selected parameters for the bridge material are the following. The material is steel St37; the density of material is ρ = 7850 kg/m3, the Poisson ratio used is v = 0.3, and the modulus of elasticity is E = 210 GPa.

As shown in Fig. 1, a one beam and a two beam bridge solid models were created after a transient structural analysis, the (Finite Element Software) ANSYS Workbench 15.0 was used. The results obtained were presented at the mid-point of the bridge span compared to the results of the mid-point of the bridge due to displacement of the main design parameters in crane design.

The design taken into account uses cranes on top of which there is one or several cars for investigating the dynamic effects occurred. Therefore we performed an analysis with one carriage and two carriages using FEM, considering a carriage moving on a girder bridge with the total moving load of 12,000 N applied and assuming a payload of 10,000 N and a carriage weight of 2000 N. When transporting two carriages with the same load on a beam two separate moving loads in the system a 7000 N load was applied. Displacement, velocity and acceleration results at the mid-point of the beam are given in Figs. 5, 6, and 7. As shown in Fig. 5 when using a two-carriage beam the displacement at midpoint is greater than when using a single carriage beam.

Fig. 5
figure 5

Displacement variation at the mid-point of a single beam, with one carriage and two carriages

Full size image
Fig. 6
figure 6

Velocity variation at the mid-point of a single beam, with one carriage and two carriages

Full size image
Fig. 7
figure 7

Acceleration variation at the mid-point of a single beam, with one carriage and two carriages

Full size image

In Figs. 6 and 7 are depicted the speed and acceleration changes examined for both the cases of one and of two carriages and the dynamic response of a crane system with a carriage system is very much the same as in the case of two carriages and both speed and acceleration increase are depicted below. This slight increase in values in the second scenario can be considered as dynamic effects that are brought into the system by the weight of the second carriage.

In the event of a single carriage on a double beam, a 6000 N force was applied to both one and two beams as the payload and the weight of the carriage was split in two. The load moving model defined in the single beam scenario was also defined for a double beam. The double beam payload and weight of the two cars were divided into 4 moving loads and 4 separate forces of 3500 N were identified. According to the circumstances mentioned above, the first displacement, velocity and acceleration changes occurring on the double beam are given in Figs. 8, 9, and 10. Also, when the second beam substitution occurred, the changes in velocity and acceleration were given in Figs. 11, 12, and 13.

Fig. 8
figure 8

Displacement variation at the mid-point of a double beam, with one carriage and two carriages on the first beam

Full size image
Fig. 9
figure 9

Velocity variation at the mid-point of a double beam, with one and two carriages on the first beam

Full size image
Fig. 10
figure 10

Acceleration variation at the mid-point of a double beam, with one and two carriages on the first beam

Full size image
Fig. 11
figure 11

Displacement variation at the mid-point of a double beam, with one and two cars on the second beam

Full size image
Fig. 12
figure 12

Velocity variation at the mid-point of a double beam, with one and two cars on the second beam

Full size image
Fig. 13
figure 13

Acceleration variation at the mid-point of a double beam, with one and two cars on the second beam

Full size image

In case of a double beam with two carriages the larger displacement is shown in Fig. 8. As can be seen from, Figs. 9 and 10, in the case of using two carriages, the velocity and acceleration produces the same effects. Figure 9 describes the two carriage case velocity change on the second beam, and the effect on the beam is less visible. Also, Fig. 11 shows the changes in acceleration.

Figure 11 shows the changes in displacement caused by the second beam and Fig. 12 shows speed variation caused by the second. The change in acceleration induced by the second beam is shown in Fig. 13.

4 Conclusions

Cranes systems are designed to move the carriages on double beam bridges. However, the design of the bridge was analyzed taking into account dynamic effects and based on a FEM. As can be seen from the results, both single carriage and two carriages move in these systems. Furthermore, the total force for moving the second carriage has increased in each of the two beam system, thus the increases in dynamic effects. At the same time, using a double beam system reduces the dynamic effects that strongly impact on the results. As a result, based on a double beam bridge system, although the system domain consists of both beams, the symmetric displacement, velocity and acceleration values can help to determine the different behaviour of the system.

As can be outlined from the results, the carriage has high vibrations caused by the effects of inertia in the system during the first move. They decreased after a while under the operating conditions and, at the same time, when the moving load approaches the point of the measurement, there is a decrease in velocity and acceleration amplitude and after passing this point, both values increase again.