Keywords

1 Introduction

Two basic questions underpin the consideration of the mechanical testing of materials: how to define “materials”, and how to explain the testing and evaluating procedures. It is difficult to find an exact definition for “materials”. Entering the expression “definition of materials” into the Google search engine returns over 600 million results. Reading the definitions provided by the first 20 web addresses listed is not enlightening, bringing to mind Enrico Fermi’s comment: “Before listening to your presentation I was confused about this topic; after finishing it I am still confused, but on a higher level!” Unfortunately, there appears to be no universal definition of materials that can be accepted as a model. Yet the natural sciences are based on models, i.e. ways of thinking that provide a description of natural processes. Understanding the working of these natural processes depends on having a defined model and then applying mathematical procedures to this. It therefore follows that the first step for understanding the mechanical testing of materials should be a “generalised model”, or the definition of materials; however, as yet this is missing, or it is not exact.

2 How Can a Generalised Model of Materials Be Defined?

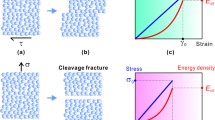
Defining materials in a general way is difficult and complex. The definition needs to summarise concisely all the substantial features of materials used for their characterisation, including behaviours, properties, structures, etc. A possible preliminary suggestion (for further discussion) is shown in Fig. 1.

Fig. 1
figure 1

A possible elementary model of a “material”

Full size image

As a general case, a “material”, represented by its mass (or energy), can be defined as a region of space with an elementary volume Ve and an elementary surface Se, with at least one parameter differentiating the region within the material from the space outside it. This model can be regarded as the “elementary cell of materials” (analogous to an elementary lattice cell). Bulk materials, representing a set of bulk properties in volume V, can be built up from these elementary cells, with internal and external borders. Regarding to the internal surfaces (borders) they could be the grain- or sub grain borders the twin-interfaces or the borders of different phases. The surface S of the bulk material, which represents the material’s surface properties, is formed from the sum of the external sections of the material cell surfaces. In this model, the bulk material behaviour can be influenced by the elementary bulk properties, the elementary internal surface behaviours, the properties of the phases and their volume ratios, etc. In this approach, SIZE and GEOMETRIC effects are included directly because both of these can be considered to be factors that influence the LOCAL modification of the GLOBAL EXTERNAL conditions (loading or loading rate, temperature, environmental conditions, etc.).

3 The Role of the Ratio of Surface and Bulk Behaviours in Size Effects

The relative weights of the bulk and surface behaviours of an elementary cell are constants. But for a real structural element the relative weights of these behaviours depend on the size of the structures. This can easily be illustrated with a sphere of material that has a radius R, a volume V with bulk behaviour properties Bp and a surface S with surface properties Sp. The ratio of surface properties to bulk behaviour is then as follows:

$$ S_{p} /B_{p} = Surface/Volume = 4R^{2} \pi /(4R^{3} \pi /3) = C/R $$
(1)

where C is a constant. From this, it directly follows that when a material’s geometry or volume is

  • larger, then bulk behaviour dominates,

  • smaller, then the surface properties dominate.

This last conclusion underpins the importance of nanotechnology or the “operating circumstances” of nature, because of many of the processes take place through the surfaces.

4 Mechanical Testing of Materials

Engineering structures are designed on the basis of loading, external operating conditions, and certain material properties. These material properties can change during a long period of operation; this is an ageing process which needs to be monitored. Mechanical testing, such as for monitoring an ageing process, is performed in a material during specific operating conditions (e.g., for a pipeline, Fig. 2). In principle there are two possibilities: monitoring the ageing process directly on the structures, or testing the properties of sample specimens.

Fig. 2
figure 2

Ageing monitoring is necessary for engineering structures and components, such as this pipeline

Full size image

If using specimens for testing, these need to be taken from the operating structure, prepared, and used to measure the properties that are most important for the safety of the structure. A basic problem with this approach is TRANSFERABILITY: how well do the properties measured in the specimen represent the actual properties of the real engineering component? This question requires consideration of the SIZE EFFECT and/or the GEOMETRICAL PROBLEM.

A pure, straightforward size effect could be the dominant problem in, for example, a fracture process of a statistical nature (such as high cycle fatigue or creep) or during procedures for testing small specimens. The GEOMETRICAL PROBLEM may be dominant in the determination of crack growth resistance properties. This problem follows directly from the stress and strain distributions in the vicinity of cracks in different types and sizes of specimens, whereas there is always a state of plane strain in the middle plane of the specimens and a state of plane stress on the free surface of the specimen. This problem is considered in depth in the theories of various kinds of fracture mechanics [1–10].

Whether or not there is a size effect or geometrical problem, use of a sample specimen allows completely free determination of the external testing conditions. These are the following:

  • the loading condition with all its parameters, i.e. uniaxial, biaxial, complex, load history, loading rate of each components,

  • temperature (or time dependence of temperature field),

  • environmental conditions (or time dependence of environmental conditions).

During the mechanical testing, the MATERIAL (specimen) is subjected to these freely selected external conditions (in Fig. 1 indicated with a simple expression of “LOADING” and the RESPONSE(s) measured). These responses are the material’s behaviours and properties. The RESPONSES are always answers (reactions) of the given (tested) material. Because non-living materials are the simplest self-organised systems, their responses to a given set of external conditions have only a statistical nature.

The RESPONSE(s) can be evaluated at different levels. The simplest way is to consider the response of the system to be proportional (linear or non-linear) to the external condition. In this case the simplest relationship is a power law with two parameters, i.e.

$$ {\mathbf{y = Cx}}^{{\mathbf{n}}} {\mathbf{ = B(x/x}}_{{\mathbf{0}}} {\mathbf{)}}^{{\mathbf{n}}} $$
(2)

where y is the parameter describing the material’s RESPONSE, x is the LOADING (EXTERNAL) parameter, and C and n—or, in the x0 normalised form, B and n—are the independent material characteristics. In such an evaluation procedure, the physical nature and parameters of any DAMAGE process or processes are not taken into consideration.

The response of the MATERIAL to a given external condition can also be evaluated at the microscopic level. In this case, the description of the damage process includes not only the MATERIAL parameters but also the external LOADING parameters (Fig. 3).

Fig. 3
figure 3

Mechanical testing as the RESPONSE of a self-organised system to the freely selected external conditions

Full size image

An obvious example of this is the process of thermoactivated damage, which can be described by the following relationship:

$$ {\text{t}}_{\text{t}} = {\text{t}}_{ 0} \cdot { \exp } \cdot \left[ {U\left( \sigma \right)/{\text{kT}}} \right] $$
(3)

where t t is the time the given damage process takes effect (residual time), U(σ) is the activation energy, which deepens on the external loading conditions, T is temperature, k is the Boltzman constant, and t 0 is a constant that is independent of the temperature, the material and other circumstances (t0 = 10−12–10−13 s). Equation (3) contains the parameters of the selected external LOADING conditions, i.e. U(σ) and T. From this relationship it directly follows that the thermoactivated damage processes in different materials differ only in the function U(σ). This means that a given thermoactivated damage process (or residual life time) in different materials differs only in a single U(σ) parameter. From this, the following question and consequences arise:

  • If the damage process is the same in different materials for the same external LOADING conditions, why does Eq. (2) need to be characterised by two parameters (C and n)?

  • If the microstructure or damage process changes (for instance, for different type of cast iron, or for aluminium alloys with different microstructures), then the possible relationship between C and n in the equation has to change.

  • If the external LOADING conditions are the same and only the testing temperature changes, the lifetime is then proportional to ln (1/T).

Regarding Eq. (2), much discussion can be found in the literature about the obvious correlation between the material parameters C and n in the form of ln C = B + n ln x, where C and B are the independent material constants. This argument is based on the following. Each power law relationship based on Eq. (2) can be expressed in the following form: ln y = ln C + n ln x, or in the normalised form, ln y = ln B + n(ln x − ln x0) = ln B + n ln x − n ln x 0 .

From this it follows that ln C = ln B − n ln x 0 , and thus that C and B are independent constants if x0 ≡ 1.

5 Correlation of Fatigue Crack Growth Resistance Parameters in the Paris Region

Around 40 years ago, in the mid-1970s, correlations between the two parameters of the Paris–Erdogan law [da/dN = C(ΔK)n] were published for the first time. The authors of the first papers were Gurney, Kobayashi, Kanazawa and their co-workers. They found correlations between the two parameters in the following forms:

$$ \begin{array}{*{20}l} {\text{GURNEY}} \hfill & {{\text{C}} = 1. 3 1 5 { 1}0^{ - 4} / 8 9 5^{\text{n}} } \hfill & 1. 8\,<\, {\text{n}}\, <\, 4.0 \hfill \\ {\text{KOBAYASHI}} \hfill & {{\text{C}} = 2. 7 20{ 1}0^{ - 4} / 1 1 2 9^{\text{n}} } \hfill & 3.0\, <\, {\text{n}}\, <\, 6. 5 \hfill \\ {\text{KANAZAWA}} \hfill & {{\text{C}} = 2. 4 8 { 1}0^{ - 5} / 5 7 7. 8^{\text{n}} } \hfill & 2.0\,<\,{\text{n}}\,<\,5. 5 \hfill \\ \end{array} $$

where ΔK is in units of N/mm3/2 and da/dN in units of mm/cycle (Fig. 4).

Fig. 4
figure 4

Correlation between the parameters n and lg C in the Paris region of STEELS. There were 352 data points; ΔK is in units of MPam0.5 and da/dN in units of mm/cycle

Full size image

These correlations initiated a systematic data collection for different materials. The results are summarized in Table 1.

Table 1 Correlations between the parameters n and lg C in the Paris regions of different materials
Full size table

Many other expressions for describing the connections between the two parameters of the Paris region can be found in the literature, but these do not differ significantly from each other [14, 17–21].

From the above, it can be concluded that the crack growth resistance of material in the Paris region depends only a single factor, i.e. the damage process in the second, in the stationary fatigue crack growth range consists of only one parameter, but this depends on the type of material. This is manifested exactly in the case of aluminium alloys with different microstructures, i.e. tempered and non-tempered.

6 Correlation of the Manson–Coffin Parameters in the Low Cycle Fatigue Range

The relationship between plastic strain amplitude and lifetime is commonly described by the power law proposed by Manson in 1954. One of the most generally used versions is the following:

$$ N_{f} = C\varepsilon_{ap}^{n} $$
(4)

where ε ap is a measure of the plastic strain amplitude (i.e. the component of the external effect that is directly connected with the damage process taking place in the material), N f is the lifetime (i.e. the “response” of the material, the number of cycles to failure), and C and n are material constants.

It is obvious that the damage processes would be different in completely different types of materials, but they are quite similar within a given material at different temperatures. The parameters of Eq. (4) for different materials have been collected from the handbooks and evaluated [22–25]. The results are summarised in Table 2. From the evaluation of almost 300 data items in the table, it can be seen that

  • unambiguous correlations exist between ln C and n from Eq. (4)

  • the correlations always depend on the type of steel (unalloyed, low alloyed or high alloyed) and also the temperature

  • the coefficients of the correlations are over 90 % (except for a single case out of the 10)

  • as the temperature increases, the correlation coefficient for the n values decreases.

Table 2 Correlations between the parameters n and ln C [from Eq. (4)] for different materials and temperatures
Full size table

From the evaluated data series it can also be concluded that the low cycle fatigue (LCF) resistances of materials characterised by Manson–Coffin laws depend only on a single parameter, but that these correlations depend on the types of material and the temperature. Considering that the Manson–Coffin relationship provides an empirically good description of the stationary range of low cycle fatigue, it can be concluded that the damage process that takes place in this region is determined by a single material characteristic. This is the same as for fatigue crack propagation, as described earlier. In both of these cases the damage process (d) took place over its whole range, i.e. 0 < d < 1.

7 Correlation of the Wöhler Line (Basquin Relationship) Parameters in the Lifetime Fatigue Rang

For high cycle loading conditions in the lifetime range, the following relationship, proposed by Basquin in 1910, is widely used:

$$ {\text{N}}_{\text{f}} = {\text{C}}({\Delta \upsigma })^{\text{n}} $$
(5)

In this relationship, used for more than a century, the external effect is characterised by the value of the stress amplitude (if the asymmetry factor is given and is constant) and the response of the material by parameters C and n. Using data from handbooks and catalogues [20, 26], C and n values have been calculated for Wöhler lines for more than 100 types of steel and approximately 50 titanium alloys. These results are summarised in Figs. 5 and 6.

Fig. 5
figure 5

Correlation between the parameters n and lg C in the Basquin relationship for STEELS lg C = 2.61–5.71 n

Full size image
Fig. 6
figure 6

Correlation between the parameters n and lg C in the Basquin relationship for titanium alloys lg C = 2.70–4.4 n

Full size image

Comparing these two graphs, the following conclusions can be drawn:

  • clear correlations can be seen for both types of metals but the expressions are slightly different, even though the values of the constant are almost the same (2.61 for steels and 2.70 for titanium alloys)

  • the scatter band of the parameters calculated from the Wöhler lines for steels is much greater for the steels in the lowest part of the slope, i.e. for 0.05 < n < 0.20.

As with fatigue crack growth and LCF data evolution described earlier, here again the damage process (d) in each analysed case took place over its whole range, i.e. 0 < d ≤ 1.

8 Correlation of Material Parameters in Power Law Relationships Used to Describe Creep Processes

The following empirical relationships are widely used to characterise material behaviour at a given temperature in conditions where there is creep:

$$ \dot{\varepsilon } = {\text{C}}_{1}\upsigma^{\text{n}} $$
(6)
$$ {\text{t}}_{\text{f}} = {\text{C}}_{2}\upsigma^{\text{m}} $$
(7)
$$ {\dot{\upvarepsilon }} = {\text{C}}_{3} \left({{\text{t}}_{\text{f}}} \right)^{\text{i}} $$
(8)

where σ is the loading stress, i.e. the external condition, and the response of the material can be characterised by the time to fracture (lifetime) t f , the stationary creep rate \( {\dot{\varvec{\upvarepsilon}}}, \) and two parameters of the material, i.e. C 1 and n, or C 2 and m, or C 3 and i, from Eqs. (6), (7) or (8), respectively. All the material parameters are functions of the external conditions, i.e. the temperature and environment (Fig. 7, 8, 9, 10).

Fig. 7
figure 7

Correlation between the material parameters lg C 1 and n [from Eq. (6)] for tungsten and tungsten alloys at 1500 and 2000 °C (Tmelting = 3422 °C)

Full size image
Fig. 8
figure 8

Correlation between material parameters lg C 1 and n [from Eq. (6)] for molybdenum alloys at 1500 and 2000 °C (Tmelting = 2623 °C)

Full size image
Fig. 9
figure 9

Correlation between the material parameters lg C 2 and m [from Eq. (7)] for tungsten and tungsten alloys at 1500 and 2000 °C (Tmelting = 3422 °C)

Full size image
Fig. 10
figure 10

Correlation between the material parameters lg C 2 and m [from Eq. (7)] for molybdenum alloys at 1500 and 2000 °C (Tmelting = 2623 °C)

Full size image

The situation is the same if the correlation of the material properties is evaluated in relationship (7). The results for tungsten and tungsten alloys at 1500 and 2000 °C can be seen in Fig. 11; the damage processes in the given material are the same at both temperatures. For molybdenum alloys at the same temperatures, there is a correlation between the two material characteristics from Eq. (8).

Fig. 11
figure 11

Correlation between the material parameters lg C 3 and i [from Eq. (8)] for tungsten and tungsten alloys at 1500 and 2000 °C (Tmelting = 3422 °C)

Full size image

For creep in materials where the damage process (d) extends over the entire range, i.e. to 0 < d ≤ 1 (as was the case for fatigue crack propagation, LCF and the lifetime range of the Wöhler line), there exist clear correlations between the two parameters in the widely used power law relationships.

Unfortunately, similar data has not been collected for steels and steel groups used in and around power stations. This could be carried out using the material data sheets that have been collected in many countries and by many organisations, such as the European Creep Collaborative Committee formed in 1991.

The possible thermomechanical background of the correlations has also been analysed for creep [27–31] and for low cycle fatigue behaviours [34].

9 Power Law Relationships in the Evaluation of Other Mechanical Testing Results

Power law relationships are widely used in the evaluation of other mechanical testing procedures. One well-known relationship used in hardness testing is Meyer’s law, which expresses the relationship between indentation depths (as the RESPONSE of materials) and load values (as the EXTERNAL conditions). The most important difference from the cases considered earlier is that during hardness testing some kind of damage process takes place (as the RESPONSE of materials) but this does not extend to the unit, to d = 1. The following two series of hardness testing have been performed for more than 20 selected materials:

  • Brinell testing with 2.5 mm diameter ball and different load levels

  • Vickers harness testing with various loads.

The material characteristics have been determined in the following relationships:

$$ {\text{F}} = {\text{ad}}^{\text{n}} $$
(9)
$$ {\text{F}} = {\text{a}}_{1} {\text{d}}_{1}^{\text{m}} $$
(10)

where the RESPONSE of materials is characterised by the diameters of the indentations d in the Brinell testing, and by the diagonals of the indentations d 1 in the Vickers testing. The material parameters in these two power law relationships are a and n or a 1 and m, respectively (Figs. 12, 13).

Fig. 12
figure 12

Correlation between the material parameters a and n [from Eq. (9)] when measuring the Brinell hardness of more than 20 types of steel. (Ball diameter D = 2.5 mm)

Full size image
Fig. 13
figure 13

Correlation between the material parameters a 1 and m [from Eq. (10)] when measuring the Vickers hardness of more than 20 types of steel

Full size image

The results of tensile testing performed on smooth and notched specimens can be used to characterise the “notch sensitivity” sensitivity of materials in quasistatic loading conditions. The absorbed energy until fracture can be measured for both smooth (W0) and notched specimens (Wm) as the area under true stress (σ′) true strain (φ) diagrams. True stress is defined as the ratio of the actual load to the actual cross-sectional area, and the true strain for cylindrical specimens is φ = 2 ln (d 0 /d), where d 0 is the initial diameter and d is the actual measured diameter at the given load. The notch of the specimen is characterised by the stress concentration factor Kt, i.e. by the ratio of the notch tip stress to the nominal stress. The value of Kt characterises the external geometrical effect (i.e. localisation of the damage process) on material behaviour. In this case, the notch sensitivity of materials can be characterised by measuring the reduction in the absorbed energy until fracture caused by a notch for any value of Kt. This can be expressed in the following form:

$$ {\text{W}}_{\text{m}} = {\text{W}}_{0} {\text{K}}_{\text{t}}^{{ - {\text{b}}}} $$
(11)

where W 0 is the absorbed specific energy measured for a smooth specimen, W m is measured on a notched specimen with stress concentration factor K t , and b is the notch sensitivity factor expressed as the tendency of the fracture energy to decrease with the stress concentration factor. The material RESPONSE has been determined experimentally for 11 different materials, as summarised in Fig. 14.

Fig. 14
figure 14

Correlation between the material parameters W 0 and b [from Eq. (11)] when measuring the Vickers hardness of more than 20 different types of steel

Full size image

It can clearly be seen from this that there is no relationship between the parameters for the steels investigated.

As no correlation was found either in the material parameters of Meyer’s law used in hardness testing, nor in the characterisation of the notch sensitivity of materials, the following conclusion can be drawn. In the absence of correlations between the two material parameters are expressed not the bulk behaviour (as the response of a material—the result of a damage process), but the relocalisation of the damage process, as the geometrical effect on the bulk behaviour.

10 Summary

The following points summarise the topics and detailed results discussed in this paper:

  1. 1.

    A generalised definition for materials with bulk and surface properties has been proposed.

  2. 2.

    The size and geometry effects in specimens used for the determination of mechanical (bulk) properties were distinguished.

  3. 3.

    It was suggested that the bulk properties of materials can be explained as the response of a self-organised system.

  4. 4.

    In practice, power law relationships with two material parameters are widely used to describe a self-organised system, i.e. the relationship between the external effect and the response.

  5. 5.

    Close correlations have been found between such material parameters for groups of materials if the external conditions are the same and the types of damage processes (taking place at the given external loading conditions from zero-to-unit) are similar. These have been demonstrated for fatigue crack growth, for Wöhler lines and for creep conditions.

  6. 6.

    No correlation was found between the two material characteristics for other power law relationships used to describe the relocalisation of the damage process (such as Meyer’s law in hardness testing, or the notch sensitivity of the materials).

  7. 7.

    The possible physical backgrounds for the observed correlations between the empirical material constants for LCF and creep circumstances have been analysed in the publications cited in this paper.