Keywords

1 Introduction

Nonlinear micropolar continuum model allows to describe complex micro-structured media, for example, polycrystals, foams, cellular solids, lattices, masonries, particle assemblies, magnetic rheological fluids, liquid crystals, etc., for which the rotational degrees of freedom of material particles are important. In the case of inelastic behavior the constitutive equations of the micropolar continuum have more complicated structure, the stress and couple stress tensors as well as other quantities depend on the history of strain measures. For the basics of micropolar mechanics we refer to Nowacki (1986), Eringen (1999), Eringen and Kafadar (1976), Eringen (2001), Eremeyev et al. (2013), Łukaszewicz (1999).

Below we discuss the constitutive equations of the nonlinear micropolar continuum considering strain rates. The discussion of strain measures for polar elastic materials presented by Pietraszkiewicz and Eremeyev (2009a, b), where the natural Lagrangian and Eulerian strain measures are introduced. Strain–stress pairs within the framework of micropolar mechanics are discussed in Ramezani and Naghdabadi (2007). Using strain rate tensors incremental equations of the micropolar hypo-elasticity were presented in Ramezani and Naghdabadi (2010), Ramezani et al. (2008). The interrelations between strain rates in discrete and continual models are discussed in Trovalusci and Masiani (1997), Pau and Trovalusci (2012). Here we discuss the Rivlin–Ericksen analogues of strain rate tensors for micropolar mechanics and several types of the constitutive equations of inelastic micropolar solids are summarized.

2 Basic Relations of the Micropolar Mechanics

Following Eringen (1999), Pietraszkiewicz and Eremeyev (2009a), Eremeyev et al. (2013) let us recall the basic equations of micropolar mechanics under finite deformations. In what follows we use the standard direct tensor notations (Lebedev et al. 2010; Truesdell 1966; Truesdell and Noll 2004). For example, the gradient and divergence operators in the actual and reference configurations are defined as follows

$$ \begin{array}{lll} \displaystyle \text{ grad }\,(\bullet ) = \frac{\partial (\bullet )}{\partial x_k} \otimes \mathbf r^k, \qquad &{}\displaystyle \text{ div }\,(\bullet ) = \frac{\partial (\bullet )}{\partial x_k} \cdot \mathbf r^k, \quad &{}\displaystyle \mathbf r_i=\frac{\partial \mathbf r}{\partial x_i},\quad \mathbf r_i\cdot \mathbf r^k=\delta _i^k, \\[1em] \displaystyle \text{ Grad }\,(\bullet ) = \frac{\partial (\bullet )}{\partial X_k} \otimes \mathbf R^k, \qquad &{}\displaystyle \text{ Div }\,(\bullet ) = \frac{\partial (\bullet )}{\partial X_k} \cdot \mathbf R^k, \quad &{}\displaystyle \mathbf R_i=\frac{\partial \mathbf R}{\partial x_i},\quad \mathbf R_i\cdot \mathbf R^k=\delta _i^k, \end{array} $$

where \(x_i\) and \(X_i\) are the Eulerian and Lagrangian coordinates, respectively, and \(\delta _i^j\) is the Kronecker symbol.

2.1 Kinematics

We describe the micropolar continuum deformation by the following relations:

$$\begin{aligned} \mathbf r=\mathbf r(\mathbf R, t),\quad \mathbf H\equiv \mathbf d_k\otimes \mathbf D_k= \mathbf H(\mathbf R, t). \end{aligned}$$
(1)

Vector \(\mathbf r(t)\) describes the position of the particle of the continuum at time t, whereas \(\mathbf H(t)\) defines its rotation. The linear velocity is given by the relation

$$\begin{aligned} \mathbf v=\frac{{\, \mathrm {d}}\mathbf r}{{\, \mathrm {d}}t}, \end{aligned}$$
(2)

the angular velocity vector \(\pmb \upomega \) can be presented by

$$ \frac{{\, \mathrm {d}}\mathbf d_k}{{\, \mathrm {d}}t}=\pmb \upomega \times \mathbf d_k,\quad k=1,2,3, $$

where \(\times \) is the vector (cross) product. \(\pmb \upomega \) can be also expressed using the derivative of \(\mathbf H\) as follows

$$\begin{aligned} \pmb \upomega =-\frac{1}{2}\left( \frac{{\, \mathrm {d}}\mathbf H}{{\, \mathrm {d}}t} \cdot \mathbf H^T\right) _\times , \end{aligned}$$
(3)

where subindex \(\times \) stands for the vectorial invariant of second-order tensor (Lebedev et al. 2010).

2.2 Motion Equations

The Eulerian equations of motion of micropolar media are

$$\begin{aligned} \rho \frac{{\, \mathrm {d}}\mathbf v}{{\, \mathrm {d}}t}= \text{ div }\,\mathbf T+\rho \mathbf f,\quad j\frac{{\, \mathrm {d}}\pmb \upomega }{{\, \mathrm {d}}t}=\text{ div }\,\mathbf M-\mathbf T_\times +\rho \mathbf m, \end{aligned}$$
(4)

where \(\mathbf T\) and \(\mathbf M\) are the stress and couple stress tensors of Cauchy type which are non-symmetric, in general, \(\rho \) is the density in the actual configuration, j is the measure of rotatory inertia of particles of micropolar medium, \(\mathbf f\) and \(\mathbf m\) are the external forces and couples, respectively.

2.3 Constitutive Equations

In the pure mechanical theory of the micropolar continuum with memory the constitutive equations consist of dependence of the stress and the couple stress tensors of the history of deformations. As a result, \(\mathbf T\) and \(\mathbf M\) take the following form:

$$\begin{aligned} \mathbf T(t)=\mathscr {A}_1 [\mathbf F^t(s),\mathbf H^t(s),\text{ Grad }\,\mathbf H^t(s)], \quad \mathbf M(t)=\mathscr {A}_2 [\mathbf F^t(s),\mathbf H^t(s),\text{ Grad }\,\mathbf H^t(s)], \end{aligned}$$
(5)

where we introduced the histories of the deformation gradient

$$ \mathbf F^t(s)=\mathbf F(t-s), \quad \mathbf F(t)=\text{ Grad }\,\mathbf r(t), \quad s\ge 0, $$

and of the microrotation tensor

$$ \mathbf H^t(s)=\mathbf H(t-s),\quad s\ge 0. $$

Here \(\mathscr {A}_1 \) and \(\mathscr {A}_2 \) are operators describing the micropolar material behavior.

The further reduction of (5) is possible using the principle of material frame-indifference. The stress measures \(\mathbf T\) and \(\mathbf M\) should be indifferent (objective) quantities. In classical mechanics, two motions \(\mathbf r\) and \(\mathbf r^*\) are called equivalent if they relate as follows

$$\begin{aligned} \mathbf r^*=\mathbf a(t)+ \mathbf O(t)\cdot (\mathbf r-\mathbf r_0) , \end{aligned}$$
(6)

where \(\mathbf O(t)\) is an arbitrary orthogonal tensor, \(\mathbf a(t)\) is an arbitrary vector function and the constant vector \(\mathbf r_0\) represents a fixed point position (a pole). We assume that in the equivalent motion the directors \(\mathbf d_k\) transform similarly to \(\mathbf r\):

$$\begin{aligned} \mathbf d_k^*=\mathbf O(t)\cdot \mathbf d_k \qquad \text{ or } \qquad \mathbf H^*= \mathbf O(t)\cdot \mathbf H. \end{aligned}$$
(7)

Denoting by superscript “*” the stress tensors in the equivalent motions we formulate the property of objectivity for \(\mathbf T\) and \(\mathbf M\) as follows

$$\begin{aligned} \mathbf T^*= \mathbf O(t)\cdot \mathbf T\cdot \mathbf O(t)^T,\quad \mathbf M^*=\det \mathbf O(t) \, \mathbf O(t)\cdot \mathbf M\cdot \mathbf O(t)^T \end{aligned}$$
(8)

for any orthogonal tensor \(\mathbf O (t)\). Let us note that \(\mathbf M\) is an pseudotensor, that is a reason of difference in transformation rules (8).

Thus, operators \(\mathscr {A}_1 \) and \(\mathscr {A}_2 \) satisfy the relations

$$\begin{aligned} \begin{array}{ll} \mathscr {A}_1&{}[\mathbf O^t(s)\cdot \mathbf F^t(s),\mathbf O^t(s)\cdot \mathbf H^t(s),\mathbf O^t(s)\cdot \text{ Grad }\,\mathbf H^t(s)] \\ &{}= \mathbf O (t)\cdot \mathscr {A}_1 [\mathbf F^t(s), \mathbf H^t(s), \text{ Grad }\,\mathbf H^t(s)]\cdot \mathbf O^T (t), \end{array}\end{aligned}$$
(9)
$$\begin{aligned} \begin{array}{ll} \mathscr {A}_2&{}[\mathbf O^t(s)\cdot \mathbf F^t(s),\mathbf O^t(s)\cdot \mathbf H^t(s),\mathbf O^t(s)\cdot \text{ Grad }\,\mathbf H^t(s)] \\ &{}=\det \mathbf O(t) \, \mathbf O (t)\cdot \mathscr {A}_2 [\mathbf F^t(s), \mathbf H^t(s), \text{ Grad }\,\mathbf H^t(s)]\cdot \mathbf O^T (t) \end{array} \end{aligned}$$
(10)

Finally, we can prove that (5) can be represented as follows

$$\begin{aligned} \mathbf T(t)=\mathbf H(t)\cdot \mathscr {B}_1 [\mathbf E^t(s),\mathbf K^t(s)]\cdot \mathbf H^T (t), \quad \mathbf M(t)=\mathbf H(t)\cdot \mathscr {B}_2 [\mathbf E^t(s),\mathbf K^t(s)]\cdot \mathbf H^T (t), \end{aligned}$$
(11)

where \(\mathscr {B}_1 \) and \(\mathscr {B}_2 \) are operators depending on histories of two Lagrangian strain measures \(\mathbf E \) and \(\mathbf K \) defined by formulas (Pietraszkiewicz and Eremeyev 2009a)

$$\begin{aligned} \mathbf E =\mathbf H^T\cdot \mathbf F-\mathbf I, \quad \mathbf K=-\frac{1}{2}\pmb \epsilon :(\mathbf H^T\cdot \text{ Grad }\,\mathbf H). \end{aligned}$$
(12)

2.4 Elastic Materials

In the case of elastic behaviour Eq. (11) reduce to

$$\begin{aligned} \mathbf T(t)=\mathbf H(t)\cdot {f}_1 [\mathbf E(t),\mathbf K (t)]\cdot \mathbf H^T (t), \quad \mathbf M(t)=\mathbf H(t)\cdot {f}_2 [\mathbf E (t),\mathbf K (t)]\cdot \mathbf H^T (t), \end{aligned}$$
(13)

where vector functions \(f_1\) and \(f_2\) can be expressed with use of the strain energy function \(W=W(\mathbf E,\mathbf K)\). For isotropic micropolar elastic solids W is considered as a function of two strain measures which can be represented as a scalar function depending on 15 joint invariants of \(\mathbf E\) and \(\mathbf K\), see Eringen and Kafadar (1976), Eremeyev and Pietraszkiewicz (2012)

$$ W=W(I_1,\ldots , I_{15}). $$

In particular, assuming W in the form \(W=W_1(\mathbf E)+W_2(\mathbf K)\) one obtains that functions \(W_1\) and \(W_2\) depend on six invariants of \(\mathbf E\) and \(\mathbf K\). An isotropic scalar-valued function of one non-symmetric tensor \(\mathbf E\) can be constructed as a function of six invariants \(I_n\), \(n=1,\ldots , 6\), where

$$\begin{aligned} I_1=\text{ tr }\,\mathbf E, I_2=\text{ tr }\,\mathbf E ^2, I_3=\text{ tr }\,\mathbf E^3, I_4=\text {tr}\,(\mathbf E\cdot \mathbf E^T), I_5=\text{ tr }\,(\mathbf E^2\cdot \mathbf E^T), I_6= \text {tr}\,(\mathbf E^2\cdot {\mathbf E^{T2}}). \end{aligned}$$
(14)

An isotropic scalar-valued function of two non-symmetric tensors \(\mathbf E\) and \(\mathbf K\) depends on the following 15 invariants:

$$\begin{aligned} \begin{array}{llllllllc} I_1&{} = &{} \text {tr}\,\mathbf E, &{} I_2 &{} = &{} \text {tr}\,\mathbf E^2, &{} I_3 &{} = &{} \text{ tr }\,\mathbf E^3, \\ I_4 &{} = &{} \text {tr}\,(\mathbf E\cdot \mathbf E^T), &{} I_5 &{} = &{} \text {tr}\,(\mathbf E^2 \cdot \mathbf E^T), &{} I_6 &{} = &{} \text{ tr }\,(\mathbf E^2\cdot {\mathbf E^{T 2}}), \\ I_7 &{} = &{} \text {tr}\,(\mathbf E\cdot \mathbf K), &{} I_8 &{} = &{} \text {tr}\,(\mathbf E^2\cdot \mathbf K), &{} I_9 &{} = &{} \text{ tr }\,(\mathbf E\cdot \mathbf K^2), \\ I_{10} &{} = &{} \text {tr}\,\mathbf K, &{} I_{11} &{} = &{} \text {tr}\,\mathbf K^2, &{} I_{12} &{} = &{} \text{ tr }\,\mathbf K^3,\\ I_{13} &{} = &{} \text {tr}\,(\mathbf K\cdot \mathbf K^T), &{} I_{14}&{} = &{} \text {tr}\,(\mathbf K^2\cdot \mathbf K^T), &{} I_{15} &{} = &{} \text{ tr }\,(\mathbf K^2\cdot {\mathbf K^{T 2}}) \end{array} \end{aligned}$$
(15)

3 Relative Strain Measures

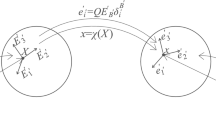
For using the fading memory concept let us introduce relative strain measures. Within the framework of so-called relative description of deformation of continuum we consider the actual configuration \(\chi \) at instant t as the reference one while the actual configuration \(\chi \) at instant \(\tau \) is considered as actual one. The relative deformation gradient and the relative microrotation tensor are introduced by formulas

$$\begin{aligned} \mathbf F_t(\tau )=\mathbf F(\tau )\cdot \mathbf F^{-1}(t), \quad \mathbf H_t(\tau )=\mathbf d_k(\tau )\times \mathbf d_k(t)=\mathbf H(\tau )\cdot \mathbf H^{-1}(t). \end{aligned}$$
(16)

Obviously, \(\mathbf F_t(t)=\mathbf I\), \(\mathbf H_t(t)=\mathbf I\). Using \(\mathbf F_t(\tau )\) and \(\mathbf H_t(\tau )\) we introduce the relative strain measures \(\mathbf E_t(\tau )\), \(\mathbf K_t(\tau )\) by the relations

$$\begin{aligned} \mathbf E_t(\tau ) =\mathbf H_t(\tau )^T\cdot \mathbf F_t(\tau )-\mathbf I, \quad \mathbf K_t(\tau )=-\frac{1}{2}\pmb \epsilon :\left[ \mathbf H_t(\tau )^T\cdot \text{ Grad }\,\mathbf H_t(\tau )\right] . \end{aligned}$$
(17)

In what follows we denote the histories of relative tensors \(\mathbf F_t(\tau )\), \(\mathbf H_t(\tau )\), etc., as follows

$$ \mathbf F_t^t(s)=\mathbf F_t(t-s), \quad \mathbf H_t^t(s)=\mathbf H_t(t-s). $$

From (16) and (17) it follows the following relations:

$$\begin{aligned} \mathbf U^t(s)=\mathbf H^T(t)\cdot&\mathbf U_t^t(s)\cdot \mathbf H(t)\cdot \mathbf U(t),\quad \mathbf Y^t(s)=\mathbf H^T(t)\cdot \mathbf Y_t^t(s)\cdot \mathbf H(t)\cdot \mathbf U(t),\end{aligned}$$
(18)
$$\begin{aligned}&\mathbf E_t^t(s)=\mathbf U_t^t(s) -\mathbf I, \quad \mathbf K_t^t(s)=\mathbf Y_t^t(s)-\mathbf B(t) \end{aligned}$$
(19)

and

$$ \mathbf U_t^t(0)=\mathbf I, \quad \mathbf Y_t^t(0)=\mathbf B(t),\quad \mathbf E_t^t(0)=\mathbf 0, \quad \mathbf K_t^t(0)=\mathbf 0, $$

where we introduced the histories

$$ \mathbf U_t^t(s)=\mathbf U_t(t-s), \mathbf Y_t^t(s)=\mathbf Y_t(t-s), \mathbf E_t^t(s)= \mathbf E_t(t-s), \mathbf K_t^t(s)= \mathbf K_t(t-s). $$

4 Relations of Isotropic Materials with Relative Strain Measures

Substituting Eqs. (18) and (19) into (11) we obtain

$$\begin{aligned} \begin{array}{lll} \mathbf T(t) &{}=&{} \mathbf H(t)\cdot \mathscr {B}_1 \left[ \mathbf H^T(t)\cdot \left( \mathbf U_t^t(s)-\mathbf I\right) \cdot \mathbf H(t)\cdot \mathbf U(t),\right. \\ &{}&{}\qquad \qquad \qquad \left. \mathbf H^T(t)\cdot \left( \mathbf Y_t^t(s)-\mathbf B(t)\right) \cdot \mathbf H(t)\cdot \mathbf U(t)\right] \cdot \mathbf H^T (t), \end{array} \\ \begin{array}{lll} \mathbf M(t)&{}=&{}\mathbf H(t)\cdot \mathscr {B}_2 \left[ \mathbf H^T(t)\cdot \left( \mathbf U_t^t(s)-\mathbf I\right) \cdot \mathbf H(t)\cdot \mathbf U(t),\right. \\ &{}&{}\qquad \qquad \qquad \left. \mathbf H^T(t)\cdot \left( \mathbf Y_t^t(s)-\mathbf B(t)\right) \cdot \mathbf H(t)\cdot \mathbf U(t)\right] \cdot \mathbf H^T (t). \end{array} \end{aligned}$$

The latter relations transform to

$$ \begin{array}{lll} \mathbf T(t)&{}=&{}\mathbf H(t)\cdot \mathscr {C}_1 \left[ \mathbf U(t),\mathbf Y(t),\mathbf H^T(t)\cdot \mathbf U_t^t(s)\cdot \mathbf H(t),\mathbf H^T(t)\cdot \mathbf Y_t^t(s) \cdot \mathbf H(t) \right] \cdot \mathbf H^T (t),\\ \mathbf M(t)&{}=&{}\mathbf H(t)\cdot \mathscr {C}_2 \left[ \mathbf U(t),\mathbf Y(t),\mathbf H^T(t)\cdot \mathbf U_t^t(s)\cdot \mathbf H(t),\mathbf H^T(t)\cdot \mathbf Y_t^t(s) \cdot \mathbf H(t)\right] \cdot \mathbf H^T (t) \end{array} $$

with new operators \(\mathscr {C}_1 \) and \(\mathscr {C}_2\).

Further reduction of constitutive equation is possible assuming some type of anisotropy as was done by Eremeyev and Pietraszkiewicz (2012). In what follows we restrict ourselves by isotropic behavior. In this case \(\mathscr {C}_1 \) and \(\mathscr {C}_2\) should satisfy the restrictions

$$\begin{aligned} \begin{array}{rl} \mathbf O^T \cdot \mathscr {C}_1&{}\left[ \mathbf U(t),\mathbf Y(t),\mathbf H^T(t)\cdot \mathbf U_t^t(s)\cdot \mathbf H(t),\mathbf H^T(t)\cdot \mathbf Y_t^t(s) \cdot \mathbf H(t) \right] \cdot \mathbf O\\ &{}=\mathscr {C}_1 \left[ \mathbf O^T \cdot \mathbf U(t)\cdot \mathbf O,(\det \mathbf O)\,\mathbf O^T \cdot \mathbf Y(t)\cdot \mathbf O,\right. \\ &{}\left. \mathbf O^T \cdot \mathbf H^T(t)\cdot \mathbf U_t^t(s)\cdot \mathbf H(t)\cdot \mathbf O,(\det \mathbf O)\,\mathbf O^T \cdot \mathbf H^T(t)\cdot \mathbf Y_t^t(s) \cdot \mathbf H(t)\cdot \mathbf O \right] ,\\ (\det \mathbf O)\mathbf O^T \cdot \mathscr {C}_2&{} \left[ \mathbf U(t),\mathbf Y(t),\mathbf H^T(t)\cdot \mathbf U_t^t(s)\cdot \mathbf H(t),\mathbf H^T(t)\cdot \mathbf Y_t^t(s) \cdot \mathbf H(t) \right] \cdot \mathbf O\\ &{} =\mathscr {C}_2 \left[ \mathbf O^T \cdot \mathbf U(t)\cdot \mathbf O,(\det \mathbf O)\,\mathbf O^T \cdot \mathbf Y(t)\cdot \mathbf O,\mathbf O^T \cdot \mathbf H^T(t)\cdot \mathbf U_t^t(s)\cdot \mathbf H(t)\cdot \mathbf O,\right. \\ &{}\left. (\det \mathbf O)\,\mathbf O^T \cdot \mathbf H^T(t)\cdot \mathbf Y_t^t(s) \cdot \mathbf H(t)\cdot \mathbf O \right] \end{array} \end{aligned}$$
(20)

for all orthogonal tensors \(\mathbf O\), \(\mathbf O^{-1}=\mathbf O^T\). As the result we obtain

$$\begin{aligned} \mathbf T(t)= \mathscr {C}_1 \left[ \mathbf H \cdot \mathbf U(t)\cdot \mathbf H^T(t),\mathbf H \cdot \mathbf Y(t)\cdot \mathbf H^T(t),\mathbf U_t^t(s),\mathbf Y_t^t(s) \right] , \\ \mathbf M(t)= \mathscr {C}_2 \left[ \mathbf H \cdot \mathbf U(t)\cdot \mathbf H^T(t),\mathbf H \cdot \mathbf Y(t)\cdot \mathbf H^T(t),\mathbf U_t^t(s),\mathbf Y_t^t(s)\right] . \end{aligned}$$

Thus, the constitutive equations of any isotropic micropolar medium with memory take the following form

$$\begin{aligned} \mathbf T(t)= \mathscr {D}_1 [\mathbf e(t), \mathbf k(t),\mathbf U_t^t(s),\mathbf Y_t^t(s)], \quad \mathbf M(t)= \mathscr {D}_2 [\mathbf e(t), \mathbf k(t), \mathbf U_t^t(s),\mathbf Y_t^t(s)], \end{aligned}$$
(21)

where \(\mathscr {D}_1\) and \(\mathscr {D}_2\) are isotropic operators and the Eulerian strain measures defined by

$$\begin{aligned} \mathbf e =\mathbf I-\mathbf H\cdot \mathbf F^{-1},\quad \mathbf k = \mathbf H\cdot \mathbf K\cdot \mathbf F^{-1}, \quad \mathbf u= \mathbf H\cdot \mathbf F^{-1}. \end{aligned}$$
(22)

5 Rivlin–Ericksen Tensors

The history of \(\mathbf U_t(\tau )\) and \(\mathbf Y_t(\tau )\) can be represented as series with respect of two families of tensors \(\mathbf {A}_k\) and \(\mathbf {B}_k\) as follows

$$\begin{aligned} \mathbf {U}_t^t(s)=\sum \limits _{k=0}^\infty \frac{(-1)^k}{k\!}s^k\mathbf {A}_k(t),\quad \mathbf {Y}_t^t(s)=\sum \limits _{k=0}^\infty \frac{(-1)^k}{k\!}s^k\mathbf {B}_k(t). \end{aligned}$$
(23)

In the micropolar continuum tensors \(\mathbf {A}_k\) and \(\mathbf {B}_k\) play a role of the Rivlin–Ericksen tensors used in the nonlinear viscoelasticity of simple materials. They are given by the recurrent relations

$$ \begin{array}{lll} \mathbf {A}_{k+1}&{}=&{}\displaystyle \frac{\mathrm {d}}{\mathrm {d}t}\mathbf {A}_k +\mathbf {A}_k\cdot \mathrm {grad} \mathbf {v}-\varvec{\upomega }\times \mathbf {A}_k,\quad \mathbf {A}_0=\mathbf {I},\\[1em] \mathbf {B}_{k+1}&{}=&{}\displaystyle \frac{\mathrm {d}}{\mathrm {d}t}\mathbf {B}_k +\mathbf {B}_k\cdot \mathrm {grad} \mathbf {v}-\varvec{\upomega }\times \mathbf {B}_k,\quad \mathbf {B}_0=\mathbf {B}. \end{array} $$

Tensors \(\mathbf {A}_k\) and \(\mathbf {B}_k\) can be also represented using the derivative of \(\mathbf {U}\) and \(\mathbf {Y}\) as follows

$$\begin{aligned} \mathbf A_k=\mathbf H\cdot \frac{{\, \mathrm {d}}^k\mathbf U}{{\, \mathrm {d}}t^k}\cdot \mathbf F^{-1},\quad \mathbf B_k=\mathbf H\cdot \frac{{\, \mathrm {d}}^k\mathbf Y}{{\, \mathrm {d}}t^k}\cdot \mathbf F^{-1}, \end{aligned}$$
(24)

or by formulae

$$\begin{aligned} \mathbf A_{k+1}= \mathbf A_k^\circ ,\quad \mathbf B_{k+1}= \mathbf B_k^\circ , \end{aligned}$$
(25)

where the corotational derivative is defined by the relations

$$\begin{aligned} (\ldots )^\circ =\mathbf H\cdot \frac{\mathrm {d}}{\mathrm {d}t}\left[ \mathbf H^T\cdot (\ldots )\cdot \mathbf F\right] \cdot \mathbf F^{-1}\equiv \frac{\mathrm {d}}{\mathrm {d}t}(\ldots )+(\ldots )\cdot \mathrm {grad} \mathbf {v}-\varvec{\upomega }\times (\ldots ). \end{aligned}$$
(26)

Let us note that \(\mathbf {A}_1\) and \(\mathbf {B}_1\) coincide with the strain rates used in the theory of micropolar continuum

$$\begin{aligned} \mathbf A_1=\pmb \varepsilon \equiv \text{ grad }\,\mathbf v-\mathbf I\times \pmb \upomega ,\quad \mathbf B_1=\pmb \upkappa \equiv \text{ grad }\,\pmb \upomega . \end{aligned}$$
(27)

For example, stress power in the micropolar continuum is given by \(w = \mathbf T: \pmb \varepsilon + \mathbf M:\pmb \upkappa .\)

6 Examples of Constitutive Equations

6.1 Linear Viscous Micropolar Fluid

The simplest example of an inelastic micropolar material is the micropolar viscous fluid with the constitutive equations (Aero et al. 1965; Eringen 1966)

$$\begin{aligned} \mathbf T=-p(\rho ) \mathbf I +\alpha _1\pmb \varepsilon +\alpha _2\pmb \varepsilon ^T+\alpha _3\mathbf I\,\text {tr}\,\pmb \varepsilon ,\quad \mathbf M= \beta _1\pmb \upkappa +\beta _2\pmb \upkappa ^T+\beta _3\mathbf I\,\text {tr}\,\pmb \upkappa , \end{aligned}$$
(28)

where p is the pressure, \(\rho \) is the density, \(\alpha _1\), \(\alpha _2\), \(\alpha _3\) and \(\beta _1\), \(\beta _2\), \(\beta _2\) are viscosities.

6.2 Non-linear Viscous Micropolar Fluid

The further generalization of (28) is non-linear viscous micropolar fluid with the following constitutive equations:

$$\begin{aligned} \mathbf T=-p(\rho ) \mathbf I +\mathbf T_v(\pmb \varepsilon , \pmb \upkappa ),\quad \mathbf M= \mathbf M_v(\pmb \varepsilon , \pmb \upkappa ), \end{aligned}$$
(29)

where \(\mathbf T_v(\pmb \varepsilon , \pmb \upkappa )\) and \(\mathbf M_v(\pmb \varepsilon , \pmb \upkappa )\) are non-linear isotropic functions of two non-symmetric 2nd order tensors. Such model may be applied for highly viscous suspensions or ferrofluids. Assuming the existence of a dissipative potential that is a scalar isotropic function \(\varPhi (\pmb \varepsilon , \pmb \upkappa )\ge 0 \) such that

$$ \mathbf T_v =\frac{\partial \varPhi }{\partial \pmb \varepsilon }, \quad \mathbf M_v =\frac{\partial \varPhi }{\partial \pmb \upkappa }, $$

we can apply the theory of invariants for representation of \(\varPhi \). As a result \(\varPhi \) depends on 15 invariants of \(I_j(\pmb \varepsilon ,\pmb \upkappa )\), \(j=1,\ldots ,15\) and should satisfy the requirement

$$\begin{aligned} \varPhi&(I_1,I_2,I_3,I_4,I_5,I_6,I_7,I_8,I_9,I_{10},I_{11},I_{12},I_{13},I_{14},I_{15})\\\nonumber&=\varPhi (I_1,I_2,I_3,I_4,I_5,I_6,-I_7,-I_8,I_9,-I_{10},I_{11},-I_{12},I_{13},-I_{14},I_{15}), \end{aligned}$$
(30)

since \(I_7\), \(I_8\), \(I_{10}\), \(I_{12}\), \(I_{14}\) are the relative invariants and change sign during the non-proper transformations. For linear viscous fluid \(\varPhi \) is a quadratic potential

$$ \varPhi = \alpha _1 I_1^2+\alpha _2 I_2+\alpha _3 I_4+ \beta _1 I_{10}^2+\beta _2 I_{11}+\beta _3 I_{13}$$

and Eq. (29) reduce to the linear case (28).

6.3 Viscoelastic Micropolar Fluids

The model of viscous micropolar fluid can be generalized to the case of viscoelastic behaviour. The viscoelastic micropolar fluid has the following constitutive relations (Yeremeyev and Zubov 1999):

$$\begin{aligned} \mathbf T =\mathscr {H}_1[\rho (t),\mathbf B(t), \mathbf E_t^t(s),\mathbf K_t^t(s)],\quad \mathbf M = \mathscr {H}_2[\rho (t),\mathbf B(t), \mathbf E_t^t(s),\mathbf K_t^t(s)], \end{aligned}$$
(31)

where \(\mathscr {H}_1\) and \(\mathscr {H}_2\) are isotropic operators. In particular, we define the viscoelastic micropolar fluid of differential type of order (m, n) as a micropolar fluid with following constitutive dependencies:

$$\begin{aligned} \mathbf T =\mathbf h_1(\rho ,\mathbf B , \mathbf A_1\ldots \mathbf A_m,\mathbf B_1\ldots \mathbf B_n),\quad \mathbf M = \mathbf h_2(\rho ,\mathbf B , \mathbf A_1\ldots \mathbf A_m,\mathbf B_1\ldots \mathbf B_n), \end{aligned}$$
(32)

where \(\mathbf h_1\) and \(\mathbf h_2\) are tensor-valued isotropic functions of \(m+n+1\) tensorial arguments. Since \(\mathbf M\), \(\mathbf B_k \), \(k=0,1,\ldots n\) are pseudo-tensors we prove that \(\mathbf h_1\) and \(\mathbf h_2\) satisfy the relations

$$\begin{aligned} \begin{array}{rll} \mathbf h_1(\rho ,\mathbf B , \mathbf A_1\ldots \mathbf A_m,\mathbf B_1\ldots \mathbf B_n)&{}=&{}\mathbf h_1(\rho ,-\mathbf B , \mathbf A_1\ldots \mathbf A_m,-\mathbf B_1\ldots -\mathbf B_n),\\ \nonumber -\mathbf h_2(\rho ,\mathbf B , \mathbf A_1\ldots \mathbf A_m,\mathbf B_1\ldots \mathbf B_n)&{}=&{}\mathbf h_2(\rho ,-\mathbf B , \mathbf A_1\ldots \mathbf A_m,-\mathbf B_1\ldots -\mathbf B_n). \end{array} \end{aligned}$$

6.4 Micropolar Hypo-elasticity

Original model of hypoelastic material was introduced in Truesdell (1963). For micropolar solids it was generalized in Tejchman and Bauer (2005), Ramezani et al. (2008), Ramezani and Naghdabadi (2010), Surana and Reddy (2015). Within the framework of the hypo-elastic micropolar solids the constitutive equations for \(\mathbf T\) and \(\mathbf M\) are formulated as follows

$$\begin{aligned} \mathbf T^\circ =\pmb \eta _1(\mathbf T,\pmb \varepsilon ,\pmb \upkappa ),\quad \mathbf M^\circ =\pmb \eta _2(\mathbf M,\pmb \varepsilon ,\pmb \upkappa ), \end{aligned}$$
(33)

where \(^\circ \) denotes an objective time derivative, and \(\pmb \eta _1\) and \(\pmb \eta _2\) are isotropic functions of their arguments and linear with respect to strain rates \(\pmb \varepsilon \) and \(\pmb \upkappa \). As a result, Eq. (33) take the form

$$\begin{aligned} \mathbf T^\circ =\mathbf C_1(\mathbf T):\pmb \varepsilon +\mathbf C_2(\mathbf T):\pmb \upkappa ,\quad \mathbf M^\circ =\mathbf C_3(\mathbf M):\pmb \varepsilon +\mathbf C_4(\mathbf M):\pmb \upkappa , \end{aligned}$$
(34)

where \(\mathbf C_1\), \(\mathbf C_2\), \(\mathbf C_3\), \(\mathbf C_4\) are 4th-order tensors, which depend on stress and couple stress tensors, in general. The following restrictions for \(\pmb \eta _1\) and \(\pmb \eta _2\) and \(\mathbf C_k\):

$$\begin{aligned} \pmb \eta _1(\mathbf T,\pmb \varepsilon ,\pmb \upkappa )=\pmb \eta _1(\mathbf T,\pmb \varepsilon ,-\pmb \upkappa ),\quad -\pmb \eta _2(\mathbf M,\pmb \varepsilon ,\pmb \upkappa )=\pmb \eta _2(\mathbf M,\pmb \varepsilon ,-\pmb \upkappa ), \end{aligned}$$
(35)

which lead to constraints \(\mathbf C_2=\mathbf 0\), \(\mathbf C_3(\mathbf M) =-\mathbf C_3(-\mathbf M)\), \(\mathbf C_4(\mathbf M)=\mathbf C_4(-\mathbf M)\).

Constitutive equations (33) or (34) can be extended as follows

$$\begin{aligned} \begin{array}{lllllll} \mathbf T^\circ &{}=&{}\pmb \eta _1(\mathbf T,\mathbf M,\pmb \varepsilon ,\pmb \upkappa ),&{}\qquad &{} \mathbf M^\circ &{}=&{}\pmb \eta _2(\mathbf T,\mathbf M,\pmb \varepsilon ,\pmb \upkappa ), \\ \mathbf T^\circ &{}=&{}\mathbf C_1(\mathbf T,\mathbf M):\pmb \varepsilon +\mathbf C_2(\mathbf T,\mathbf M):\pmb \upkappa , &{}\qquad &{} \mathbf M^\circ &{}=&{}\mathbf C_3(\mathbf T,\mathbf M):\pmb \varepsilon +\mathbf C_4(\mathbf T,\mathbf M):\pmb \upkappa \end{array} \end{aligned}$$
(36)

with the following constraints

$$\begin{aligned} \pmb \eta _1(\mathbf T,\mathbf M,\pmb \varepsilon ,\pmb \upkappa )=\pmb \eta _1(\mathbf T,-\mathbf M,\pmb \varepsilon ,-\pmb \upkappa ),\,\, -\pmb \eta _2(\mathbf T,\mathbf M,\pmb \varepsilon ,\pmb \upkappa )=\pmb \eta _2(\mathbf T,-\mathbf M, \pmb \varepsilon ,-\pmb \upkappa ), \end{aligned}$$
(37)
$$ \begin{array}{rlllrll} \mathbf C_1(\mathbf T,\mathbf M)&{}=&{}\mathbf C_1(\mathbf T,-\mathbf M),&{}\qquad &{} -\mathbf C_2(\mathbf T,\mathbf M)&{}=&{}\mathbf C_2(\mathbf T,-\mathbf M),\\ -\mathbf C_3(\mathbf T,\mathbf M)&{}=&{}\mathbf C_3(\mathbf T,-\mathbf M),&{}&{}\mathbf C_4(\mathbf T,\mathbf M)&{}=&{}\mathbf C_4(\mathbf T,-\mathbf M). \end{array} $$

6.5 Viscoelastic Materials

Considered finite approximation of series (23) we obtain the model of micropolar material of order (m, n)

$$\begin{aligned} \begin{array}{lll} \mathbf T(t) &{}=&{} \mathscr {F}_1 [\mathbf e(t), \mathbf k(t),\mathbf B(t), \mathbf A_1(t)\ldots \mathbf A_m(t),\mathbf B_1(t)\ldots \mathbf B_n(t)],\\ \mathbf M(t) &{}=&{} \mathscr {F}_2 [\mathbf e(t), \mathbf k(t), \mathbf B(t), \mathbf A_1(t)\ldots \mathbf A_m(t),\mathbf B_1(t)\ldots \mathbf B_n(t)], \end{array} \end{aligned}$$
(38)

where \(\mathscr {F}_1\) and \(\mathscr {F}_2\) are isotropic operators.

Finally, in order to consider various form of rate-type constitutive equations of micropolar materials we introduce implicit constitutive equations of differential type in the following form

$$\begin{aligned} \begin{array}{lll} \mathbf g_1 [\mathbf T^{\circ \{M\} }\ldots \mathbf T^{\circ },\mathbf M^{\circ \{N\} }\ldots \mathbf M^{\circ }, \mathbf M(t), \mathbf e(t), \mathbf k(t),\mathbf B , \mathbf A_1\ldots \mathbf A_m,\mathbf B_1\ldots \mathbf B_n]&{}=&{}\mathbf 0, \\ \mathbf g_2 [\mathbf T^{\circ \{M\} }\ldots \mathbf T^{\circ },\mathbf M^{\circ \{N\} }\ldots \mathbf M^{\circ }, \mathbf M(t),\mathbf e(t), \mathbf k(t), \mathbf B , \mathbf A_1\ldots \mathbf A_m,\mathbf B_1\ldots \mathbf B_n]&{}=&{}\mathbf 0, \end{array} \end{aligned}$$
(39)

where \(\mathbf g_1\) and \(\mathbf g_2\) are isotropic tensor-valued functions of \(M+N+m+n+3 \) tensorial arguments, and \(\circ \{k\} \) stands for kth objective derivative.

The constitutive equation (21) include various forms of micropolar viscoelastic behaviour under finite deformations. Here we present few examples of constitutive equations of differential type. The constitutive equations of the form

$$\begin{aligned} \mathbf T= \pmb \varPhi _1(\mathbf e,\mathbf k,\pmb \varepsilon ,\pmb \upkappa ),\quad \mathbf M= \pmb \varPhi _2(\mathbf e,\mathbf k,\pmb \varepsilon ,\pmb \upkappa ),\end{aligned}$$
(40)
$$\begin{aligned} \tau _1\mathbf T^\circ + \mathbf T= \pmb \varPsi _1( \pmb \varepsilon ,\pmb \upkappa ),\quad \tau _2\mathbf M^\circ + \mathbf M= \pmb \varPsi _2(\pmb \varepsilon ,\pmb \upkappa ),\end{aligned}$$
(41)
$$\begin{aligned} \tau _1\mathbf T^\circ + \mathbf T= \pmb \varOmega _1(\mathbf e,\mathbf k,\pmb \varepsilon ,\pmb \upkappa ),\quad \tau _2\mathbf M^\circ + \mathbf M= \pmb \varOmega _2(\mathbf e,\mathbf k,\pmb \varepsilon ,\pmb \upkappa ) \end{aligned}$$
(42)

play a role of Kelvin–Voigt, Maxwell and standard models in micropolar viscoelasticity, respectively. Here \(\circ \) denotes an objective time derivative, \(\tau _1\) and \(\tau _2\) are relaxation time parameters and \(\pmb \varPhi _1\), \(\pmb \varPhi _2\), \(\pmb \varPsi _1\), \(\pmb \varPsi _2\), \(\pmb \varOmega _1\) and \(\pmb \varOmega _2\) are constitutive tensor-valued functions. Using higher order objective time derivatives and tensors \(\mathbf A_k\), \(\mathbf B_k\) one can present constitutive equations of differential type of any order.

7 Conclusions and Discussion

Following Altenbach and Eremeyev (2014) we presented a family of non-symmetric strain rate tensors for micropolar materials and discussed constitutive equations of inelastic micropolar materials. Using basics principles of continuum mechanics that is the principle of equipresence and the material frame indifference we discussed the constraints for the constitutive equations. In particular, considering the fact that part of strain rates are not true tensors but pseudotensors we obtain some constitutive restrictions following from the material frame indifference principle.

Considering difference between models of classic continua and Cosserat continua let us note that some classic methods of constitutive modelling used in the Cauchy mechanics can be straightforward extended for the micropolar continuum. Among them are the theory of local material symmetry group, invariance properties applied for the micropolar elasticity (Eringen 1999; Ramezani et al. 2009; Pietraszkiewicz and Eremeyev 2009a; Eremeyev and Pietraszkiewicz 2012, 2016), micropolar hypo-elasticity (Ramezani and Naghdabadi 2010), and mechanics of viscous micropolar fluids (Aero et al. 1965; Eringen 1966, 2001). Several generalizations of yield criterium for elasto-plastic materials and other models for micropolar elastoplasticity are given by (Lippmann 1969; de Borst 1993; Steinmann 1994; Grammenoudis and Tsakmakis 2007, 2009). But in some case such straightforward extensions are impossible, let us mention the logarithmic Hencky’s strain measure and related logarithmic strain rate (Xiao et al. 1997a, b; Bruhns 2014). Introduction of similar strain tensors based on logarithmic objective derivative in micropolar mechanics is more difficult or impossible, in general.

Similar to introduced above non-symmetric strain measures and strain rates are also used for description of two-level deformations of inelastic materials considering independent spin (Trusov et al. 2015) for derivation of generalized models of elasticity (Lurie et al. 2005).