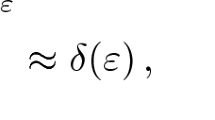Lebesgue Sequence Spaces

Abstract

In this chapter, we will introduce the so-called Lebesgue sequence spaces, in the finite and also in the infinite dimensional case. We study some properties of the spaces, e.g., completeness, separability, duality, and embedding. We also examine the validity of Hölder, Minkowski, Hardy, and Hilbert inequality which are related to the aforementioned spaces. Although Lebesgue sequence spaces can be obtained from Lebesgue spaces using a discrete measure, we will not follow that approach and will prove the results in a direct manner. This will highlight some techniques that will be used in the subsequent chapters.

1 Hölder and Minkowski Inequalities

In this section we study the Hölder and Minkowski inequality for sums. Due to their importance in all its forms, they are sometimes called the workhorses of analysis.

Definition 2.1.

The space ℓ_p ⁿ, with $1 \leq p < \infty$, denotes the n-dimensional vector space $\mathbb{R}^{n}$ for which the functional

$$\displaystyle{ \mathinner{\!\left \Vert \mathbf{x}\right \Vert }_{\ell_{p}^{n}} = \left (\sum _{i=1}^{n}\vert x_{ i}\vert ^{p}\right )^{\frac{1} {p} } }$$

(2.1)

is finite, where x = (x ₁, …, x _n ). In the case of $p = \infty$, we define ℓ_∞ ⁿ as

$$\displaystyle{\mbox{ $\mathinner{\!\left \Vert \mathbf{x}\right \Vert }_{\ell_{\infty }^{n}}$} =\sup _{i\in \left \{1,\ldots,n\right \}}\vert x_{i}\vert.}$$

⊘

From Lemma 2.4 we obtain in fact that $\mathinner{\!\left \Vert \cdot \right \Vert }_{\ell_{ p}^{n}}$ defines a norm in $\mathbb{R}^{n}$.

Example 2.2.

Let us draw the unit ball for particular values of p for n = 2, as in Figs. 2.1, 2.2, and 2.3.

Fig. 2.1

Unit ball for ℓ ₁ ²

Fig. 2.2

Unit ball for $\ell_{\infty }^{2}$

Fig. 2.3

Unit ball for ℓ ₂ ²

Lemma 2.3 (Hölder’s inequality).

Let p and q be real numbers with $1 < p < \infty$ such that $\frac{1} {p} + \frac{1} {q} = 1$ . Then

$$\displaystyle{ \sum _{k=1}^{n}\vert x_{ k}y_{k}\vert \leq \left (\sum _{k=1}^{n}\vert x_{ k}\vert ^{p}\right )^{1/p}\left (\sum _{ k=1}^{n}\vert y_{ k}\vert ^{q}\right )^{1/q}. }$$

(2.2)

for x _k , $y_{k} \in \mathbb{R}$ .

Proof.

Let us take

$$\displaystyle{\alpha = \dfrac{\vert x_{k}\vert } {\left (\sum _{k=1}^{n}\vert x_{k}\vert ^{p}\right )^{1/p}},\quad \beta = \dfrac{\vert y_{k}\vert } {\left (\sum _{k=1}^{n}\vert y_{k}\vert ^{q}\right )^{1/q}}.}$$

By Young’s inequality (1.15) we get

$$\displaystyle{ \dfrac{\vert x_{k}\vert \vert y_{k}\vert } {\left (\sum _{k=1}^{n}\vert x_{k}\vert ^{p}\right )^{1/p}\left (\sum _{k=1}^{n}\vert y_{k}\vert ^{q}\right )^{1/q}} \leq \dfrac{1} {p} \dfrac{\vert x_{k}\vert ^{p}} {\sum _{k=1}^{n}\vert x_{k}\vert ^{p}} + \dfrac{1} {q} \dfrac{\vert y_{k}\vert ^{q}} {\sum _{k=1}^{n}\vert y_{k}\vert ^{q}}.}$$

Termwise summation gives

$$\displaystyle{ \dfrac{\sum _{k=1}^{n}\vert x_{k}\vert \vert y_{k}\vert } {\left (\sum _{k=1}^{n}\vert x_{k}\vert ^{p}\right )^{1/p}\left (\sum _{k=1}^{n}\vert y_{k}\vert ^{q}\right )^{1/q}} \leq \dfrac{1} {p} + \dfrac{1} {q}}$$

and from this we get

$$\displaystyle{\sum _{k=1}^{n}\vert x_{ k}y_{k}\vert \leq \left (\sum _{k=1}^{n}\vert x_{ k}\vert ^{p}\right )^{1/p}\left (\sum _{ k=1}^{n}\vert y_{ k}\vert ^{q}\right )^{1/q}.}$$

 □ 

We can interpret the inequality (2.2) in the following way: If x ∈ ℓ _p ⁿ and y ∈ ℓ _q ⁿ then x ⊙y ∈ ℓ ₁ ⁿ where ⊙ stands for component-wise multiplication and moreover

$$\displaystyle{\mathinner{\!\left \Vert \mathbf{x} \odot \mathbf{y}\right \Vert }_{\ell_{1}^{n}} \leq \mathinner{\!\left \Vert \mathbf{x}\right \Vert }_{\ell_{p}^{n}}\mathinner{\!\left \Vert \mathbf{y}\right \Vert }_{\ell_{q}^{n}}.}$$

Lemma 2.4 (Minkowski’s inequality).

Let p ≥ 1, then

$$\displaystyle{ \left (\sum _{k=1}^{n}\vert x_{ k} + y_{k}\vert ^{p}\right )^{1/p} \leq \left (\sum _{ k=1}^{n}\vert x_{ k}\vert ^{p}\right )^{1/p} + \left (\sum _{ k=1}^{n}\vert y_{ k}\vert ^{p}\right )^{1/p} }$$

(2.3)

for $x_{k},\,y_{k} \in \mathbb{R}$ .

Proof.

We have

$$\displaystyle\begin{array}{rcl} \sum _{k=1}^{n}\vert x_{ k} + y_{k}\vert ^{p}& =& \sum _{ k=1}^{n}\vert x_{ k} + y_{k}\vert ^{p-1}\vert x_{ k} + y_{k}\vert {}\\ &\leq & \sum _{k=1}^{n}\vert x_{ k}\vert \vert x_{k} + y_{k}\vert ^{p-1} +\sum _{ k=1}^{n}\vert y_{ k}\vert \vert x_{k} + y_{k}\vert ^{p-1} {}\\ \end{array}$$

By Lemma 2.3 we get

$$\displaystyle{\sum _{k=1}^{n}\vert x_{ k} + y_{k}\vert ^{p} \leq \left [\left (\sum _{ k=1}^{n}\vert x_{ k}\vert ^{p}\right )^{1/p} + \left (\sum _{ k=1}^{n}\vert y_{ k}\vert ^{p}\right )^{1/p}\right ]\left (\sum _{ k=1}^{n}\vert x_{ k} + y_{k}\vert ^{(p-1)q}\right )^{1/q}.}$$

Since $\dfrac{1} {p} + \dfrac{1} {q} = 1$, then $p = (p - 1)q$, from which

$$\displaystyle{\sum _{k=1}^{n}\vert x_{ k} + y_{k}\vert ^{p} \leq \left [\left (\sum _{ k=1}^{n}\vert x_{ k}\vert ^{p}\right )^{1/p} + \left (\sum _{ k=1}^{n}\vert y_{ k}\vert ^{p}\right )^{1/p}\right ]\left (\sum _{ k=1}^{n}\vert x_{ k} + y_{k}\vert ^{p}\right )^{1/q},}$$

then

$$\displaystyle{\left (\sum _{k=1}^{n}\vert x_{ k} + y_{k}\vert ^{p}\right )^{1-\frac{1} {q} } \leq \left (\sum _{k=1}^{n}\vert x_{k}\vert ^{p}\right )^{1/p} + \left (\sum _{k=1}^{n}\vert y_{k}\vert ^{p}\right )^{1/p},}$$

which entails (2.3). □ 

2 Lebesgue Sequence Spaces

We now want to extend the n-dimensional ℓ _p ⁿ space into an infinite dimensional sequence space in a natural way.

Definition 2.5.

The Lebesgue sequence space (also known as discrete Lebesgue space) with $1 \leq p < \infty$, denoted by ℓ _p or sometimes also by $\ell_{p}(\mathbb{N})$, stands for the set of all sequences of real numbers $\mathbf{x} = \left \{x_{n}\right \}_{n\in \mathbb{N}}$ such that $\sum _{k=1}^{\infty }\vert x_{k}\vert ^{p} < \infty.$ We endow the Lebesgue sequence space with the norm,

$$\displaystyle{ \|\mathbf{x}\|_{\ell_{p}} =\|\{ x_{n}\}_{n\in \mathbb{N}}\|_{\ell_{p}} = \left (\sum _{k=1}^{\infty }\vert x_{ k}\vert ^{p}\right )^{1/p}, }$$

(2.4)

where x ∈ ℓ _p . ⊘

We leave as Problem 2.24 to show that this is indeed a norm in ℓ _p , therefore $(\ell_{p},\mathinner{\!\left \Vert \cdot \right \Vert }_{\ell_{p}})$ is a normed space.

We will denote by $s\mathbb{R}^{\infty }$ the set of all sequences of real numbers $\mathbf{x} = \left \{x_{n}\right \}_{n\in \mathbb{N}}$.

Example 2.6.

The Hilbert cube $\mathfrak{H}$ is defined as the set of all real sequences $\{x_{n}\}_{n\in \mathbb{N}}$ such that 0 ≤ x _n  ≤ 1∕n, i.e.

$$\displaystyle{\mathfrak{H} \mathop{:}=\{ \mathbf{x} \in \mathbb{R}^{\infty }\mathop{:} 0 \leq x_{ n} \leq 1/n\}.}$$

By the hyper-harmonic series we have that the Hilbert cube is not contained in ℓ ₁ but is contained in all ℓ _p with p > 1. ⊘

Let us show that ℓ _p is a subspace of the space $\mathbb{R}^{\infty }$. Let x and y be elements of ℓ _p and α, β be real numbers. By Lemma 2.4 we have that

$$\displaystyle{ \left (\sum _{k=1}^{n}\vert \alpha x_{ k} +\beta y_{k}\vert ^{p}\right )^{1/p} \leq \vert \alpha \vert \left (\sum _{ k=1}^{n}\vert x_{ k}\vert ^{p}\right )^{1/p} + \vert \beta \vert \left (\sum _{ k=1}^{n}\vert y_{ k}\vert ^{p}\right )^{1/p}. }$$

(2.5)

Taking limits in (2.5), first to the right-hand side and after to the left-hand side, we arrive at

$$\displaystyle{ \left (\sum _{k=1}^{\infty }\vert \alpha x_{ k} +\beta y_{k}\vert ^{p}\right )^{1/p} \leq \vert \alpha \vert \left (\sum _{ k=1}^{\infty }\vert x_{ k}\vert ^{p}\right )^{1/p} + \vert \beta \vert \left (\sum _{ k=1}^{\infty }\vert y_{ k}\vert ^{p}\right )^{1/p}, }$$

(2.6)

and this shows that α x +β y is an element of ℓ _p and therefore ℓ _p is a subspace of $\mathbb{R}^{\infty }$.

The Lebesgue sequence space ℓ _p is a complete normed space for all $1 \leq p \leq \infty$. We first prove for the case of finite exponent and for the case of $p = \infty$ it will be shown in Theorem 2.11.

Theorem 2.7.

The space $\ell_{p}(\mathbb{N})$ is a Banach space when $1 \leq p < \infty$ .

Proof.

Let $\{\mathbf{x}_{n}\}_{n\in \mathbb{N}}$ be a Cauchy sequence in $\ell_{p}(\mathbb{N})$, where we take the sequence x _n as x _n  = (x ₁ ⁽ⁿ⁾, x ₂ ⁽ⁿ⁾, …). Then for any $\varepsilon > 0$ there exists an $n_{0} \in \mathbb{N}$ such that if n, m ≥ n ₀, then $\|\mathbf{x}_{n} -\mathbf{x}_{m}\|_{\ell_{p}} <\varepsilon$, i.e.

$$\displaystyle{ \left (\sum _{j=1}^{\infty }\vert x_{ j}^{(n)} - x_{ j}^{(m)}\vert ^{p}\right )^{1/p} <\varepsilon, }$$

(2.7)

whenever n, m ≥ n ₀. From (2.7) it is immediate that for all j = 1, 2, 3, …

$$\displaystyle{ \vert x_{j}^{(n)} - x_{ j}^{(m)}\vert <\varepsilon, }$$

(2.8)

whenever n, m ≥ n ₀. Taking a fixed j from (2.8) we see that (x _j ⁽¹⁾, x _j ⁽²⁾, …) is a Cauchy sequence in $\mathbb{R}$, therefore there exists $x_{j} \in \mathbb{R}$ such that $\lim _{m\rightarrow \infty }x_{j}^{(m)} = x_{j}.$

Let us define x = (x ₁, x ₂, …) and show that x is in ℓ _p and $\lim _{n\rightarrow \infty }\mathbf{x}_{n} = \mathbf{x}$.

From (2.7) we have that for all n, m ≥ n ₀

$$\displaystyle{\sum _{j=1}^{k}\vert x_{ j}^{(m)} - x_{ j}^{(n)}\vert ^{p} <\varepsilon ^{p},\quad k = 1,2,3,\ldots }$$

from which

$$\displaystyle{\sum _{j=1}^{k}\vert x_{ j} - x_{j}^{(n)}\vert ^{p} =\sum _{ j=1}^{k}\vert \lim \limits _{ m\rightarrow \infty }x_{j}^{(m)} - x_{ j}^{(n)}\vert ^{p} \leq \varepsilon ^{p},}$$

whenever n ≥ n ₀, This shows that x −x _n  ∈ ℓ _p and we also deduce that $\lim _{n\rightarrow \infty }\mathbf{x}_{n} = \mathbf{x}$. Finally in virtue of the Minkowski inequality we have

$$\displaystyle\begin{array}{rcl} \left (\sum _{j=1}^{\infty }\vert x_{ j}\vert ^{p}\right )^{1/p}& =& \left (\sum _{ j=1}^{\infty }\vert x_{ j}^{(n)} + x_{ j} - x_{j}^{(n)}\vert ^{p}\right )^{1/p} {}\\ & \leq & \left (\sum _{j=1}^{\infty }\vert x_{ j}^{(n)}\vert ^{p}\right )^{1/p} + \left (\sum _{ j=1}^{\infty }\vert x_{ j} - x_{j}^{(n)}\vert ^{p}\right )^{1/p}, {}\\ \end{array}$$

which shows that x is in $\ell_{p}(\mathbb{N})$ and this completes the proof. □ 

The next result shows that the Lebesgue sequence spaces are separable when the exponent p is finite, i.e., the space ℓ _p admits an enumerable dense subset.

Theorem 2.8.

The space $\ell_{p}(\mathbb{N})$ is separable whenever $1 \leq p < \infty$ .

Proof.

Let M be the set of all sequences of the form q = (q ₁, q ₂, …, q _n , 0, 0, …) where $n \in \mathbb{N}$ and $q_{k} \in \mathbb{Q}$. We will show that M is dense in ℓ _p . Let $\mathbf{x} =\{ x_{k}\}_{k\in \mathbb{N}}$ be an arbitrary element of ℓ _p , then for $\varepsilon > 0$ there exists n which depends on $\varepsilon$ such that

$$\displaystyle{\sum _{k=n+1}^{\infty }\vert x_{ k}\vert ^{p} <\varepsilon ^{p}/2.}$$

Now, since $\overline{\mathbb{Q}} = \mathbb{R}$, we have that for each x _k there exists a rational q _k such that

$$\displaystyle{\vert x_{k} - q_{k}\vert < \dfrac{\varepsilon } {\root{p}\of{2^{n}}},}$$

then

$$\displaystyle{\sum _{k=1}^{n}\vert x_{ k} - q_{k}\vert ^{p} <\varepsilon ^{p}/2,}$$

which entails

$$\displaystyle{\|\mathbf{x} -\mathbf{q}\|_{\ell_{p}}^{p} =\sum _{ k=1}^{n}\vert x_{ k} - q_{k}\vert ^{p} +\sum _{ k=n+1}^{\infty }\vert x_{ k}\vert ^{p} <\varepsilon ^{p},}$$

and we arrive at $\|\mathbf{x} -\mathbf{q}\|_{\ell_{p}} <\varepsilon.$ This shows that M is dense in ℓ _p , implying that ℓ _p is separable since M is enumerable. □ 

With the notion of Schauder basis (recall the definition of Schauder basis in Definition B.3), we now study the problem of duality for the Lebesgue sequence space.

Theorem 2.9.

Let $1 < p < \infty$ . The dual space of $\ell_{p}(\mathbb{N})$ is $\ell_{q}(\mathbb{N})$ where $\frac{1} {p} + \frac{1} {q} = 1$ .

Proof.

A Schauder basis of ℓ _p is $e_{k} =\{\delta _{kj}\}_{j\in \mathbb{N}}$ where $k \in \mathbb{N}$ and δ _kj stands for the Kronecker delta, i.e., δ _kj  = 1 if k = j and 0 otherwise. If f ∈ (ℓ _p )^∗, then $f(\mathbf{x}) =\sum _{k\in \mathbb{N}}\alpha _{k}f(e_{k})$, $\mathbf{x} =\{\alpha _{k}\}_{k\in \mathbb{N}}$. We define $T(f) =\{ f(e_{k})\}_{k\in \mathbb{N}}$. We want to show that the image of T is in ℓ _q , for that we define for each n, the sequence $\mathbf{x}^{n} = (\xi _{k}^{(n)})_{k=1}^{\infty }$ with

$$\displaystyle{\xi _{k}^{(n)} = \left \{\begin{array}{ll} \frac{\vert f(e_{k})\vert ^{q}} {f(e_{k})} & \text{if}\quad k \leq n\text{ and }f(e_{k})\neq 0, \\ 0 &\text{if}\quad k > n\text{ or }f(e_{k}) = 0. \end{array} \right.}$$

Then

$$\displaystyle{f(\mathbf{x}^{n}) =\sum _{ k\in \mathbb{N}}\xi _{k}^{(n)}f(e_{ k}) =\sum _{ k=1}^{n}\vert f(e_{ k})\vert ^{q}.}$$

Moreover

$$\displaystyle\begin{array}{rcl} f(\mathbf{x}^{n})& \leq & \|f\|\|\mathbf{x}^{n}\|_{ p} {}\\ & =& \|f\|\left (\sum _{k=1}^{n}\vert \xi _{ k}^{(n)}\vert ^{p}\right )^{\frac{1} {p} } {}\\ & =& \|f\|\left (\sum _{k=1}^{n}\vert f(e_{ k})\vert ^{qp-p}\right )^{\frac{1} {p} } {}\\ & =& \|f\|\left (\sum _{k=1}^{n}\vert f(e_{ k})\vert ^{q}\right )^{\frac{1} {p} }, {}\\ \end{array}$$

from which

$$\displaystyle\begin{array}{rcl} \left (\sum _{k=1}^{n}\vert f(e_{ k})\vert ^{q}\right )^{1-\frac{1} {p} }& =& \left (\sum _{k=1}^{n}\vert f(e_{k})\vert ^{q}\right )^{\frac{1} {q} } {}\\ & \leq & \|f\|. {}\\ \end{array}$$

Taking $n \rightarrow \infty$, we obtain

$$\displaystyle{\left (\sum _{k=1}^{\infty }\vert f(e_{ k})\vert ^{q}\right )^{\frac{1} {q} } \leq \| f\|}$$

where $\{f(e_{k})\}_{k\in \mathbb{N}} \in \ell_{q}$.

Now, we affirm that:

• (i) T is onto. In effect given $b = (\beta _{k})_{k\in \mathbb{N}} \in \ell_{q}$, we can associate a bounded linear functional g ∈ (ℓ _p )^∗, given by $g(\mathbf{x}) =\sum _{ k=1}^{\infty }\alpha _{k}\beta _{k}$ with $\mathbf{x} = (\alpha _{k})_{k\in \mathbb{N}} \in \ell_{p}$ (the boundedness is deduced by Hölder’s inequality). Then g ∈ (ℓ _p )^∗.

• (ii) T is 1-1. This is almost straightforward to check.

• (iii) T is an isometry. We see that the norm of f is the ℓ _q norm of Tf

  $$\displaystyle\begin{array}{rcl} \vert f(\mathbf{x})\vert & =& \left \vert \sum _{k\in \mathbb{N}}\alpha _{k}f(e_{k})\right \vert {}\\ &\leq &\left (\sum _{k\in \mathbb{N}}\vert \alpha _{k}\vert ^{p}\right )^{\frac{1} {p} }\left (\sum _{k\in \mathbb{N}}\vert f(e_{k})\vert ^{q}\right )^{\frac{1} {q} } {}\\ & =& \|x\|\left (\sum _{k\in \mathbb{N}}\vert f(e_{k})\vert ^{q}\right )^{\frac{1} {q} }. {}\\ \end{array}$$

  Taking the supremum over all x of norm 1, we have that

  $$\displaystyle{\|f\| \leq \left (\sum _{k\in \mathbb{N}}\vert f(e_{k})\vert ^{q}\right )^{\frac{1} {q} }.}$$

  Since the other inequality is also true, we can deduce the equality

  $$\displaystyle{\|f\| = \left (\sum _{k\in \mathbb{N}}\vert f(e_{k})\vert ^{q}\right )^{\frac{1} {q} },}$$

  with which we establish the desired isomorphism $f \rightarrow \{ f(e_{k})\}_{k\in \mathbb{N}}$.

 □ 

The ℓ _p spaces satisfy an embedding property, forming a nested sequence of Lebesgue sequences spaces.

Theorem 2.10.

If $0 < p < q < \infty$ , then $\ell_{p}(\mathbb{N}) \subsetneq \ell _{q}(\mathbb{N})$ .

Proof.

Let x ∈ ℓ _p , then $\sum _{n=1}^{\infty }\vert x_{n}\vert ^{p} < \infty$. Therefore there exists $n_{0} \in \mathbb{N}$ such that if n ≥ n ₀, then | x _n  |  < 1. Now, since 0 < p < q, then 0 < q − p and | x _n  | ^q−p < 1 if n > n ₀, by which | x _n  | ^q <  | x _n  | ^p if n > n ₀. Let $M =\max \{ \vert x_{1}\vert ^{q-p},\vert x_{2}\vert ^{q-p},\ldots,\vert x_{n_{0}}\vert ^{q-p},1\}$, then

$$\displaystyle{\sum _{n=1}^{\infty }\vert x_{ n}\vert ^{q} =\sum _{ n=1}^{\infty }\vert x_{ n}\vert ^{p}\vert x_{ n}\vert ^{q-p} < M\sum _{ n=1}^{\infty }\vert x_{ n}\vert ^{p} < +\infty,}$$

implying that x ∈ ℓ _q .

To show that $\ell_{p}(\mathbb{N})\neq \ell_{q}(\mathbb{N})$, we take the following sequence $x_{n} = n^{-1/p}$ for all $n \in \mathbb{N}$ with $1 \leq p < q \leq \infty$, and since p < q, then $\dfrac{q} {p} > 1$. Now we have

$$\displaystyle{\sum _{n=1}^{\infty }\vert x_{ n}\vert ^{q} =\sum _{ n=1}^{\infty } \dfrac{1} {n^{q/p}} < \infty.}$$

The last series is convergent since it is a hyper-harmonic series with exponent bigger than 1, therefore $\mathbf{x} \in \ell_{q}(\mathbb{N})$. On the other hand

$$\displaystyle{\sum _{n=1}^{\infty }\vert x_{ n}\vert ^{p} =\sum _{ n=1}^{\infty }\dfrac{1} {n}}$$

and we get the harmonic series, which entails that $\mathbf{x}\notin \ell_{p}(\mathbb{N})$. □ 

3 Space of Bounded Sequences

The space of bounded sequences, denoted by $\ell_{\infty }$ or sometimes $\ell_{\infty }(\mathbb{N})$, is the set of all real bounded sequences $\{x_{n}\}_{n\in \mathbb{N}}$ (it is clear that $\ell_{\infty }$ is a vector space). We will take the norm in this space as

$$\displaystyle{ \mathinner{\!\left \Vert \mathbf{x}\right \Vert }_{\infty } =\| \mathbf{x}\|_{\ell_{\infty }} =\sup _{n\in \mathbb{N}}\vert x_{n}\vert, }$$

(2.9)

where x = (x ₁, x ₂, …, x _n , …). The verification that (2.9) is indeed a norm is left to the reader.

An almost immediate property of the $\ell_{\infty }$-space is its completeness, inheriting this property from the completeness of the real line.

Theorem 2.11.

The space $\ell_{\infty }$ is a Banach space.

Proof.

Let $\{\mathbf{x}_{n}\}_{n\in \mathbb{N}}$ be a Cauchy sequence in $\ell_{\infty }$, where x _n  = (x ₁ ⁽ⁿ⁾, x ₂ ⁽ⁿ⁾, …). Then for any $\varepsilon > 0$ there exists n ₀ > 0 such that if m, n ≥ n ₀ then

$$\displaystyle{\|\mathbf{x}_{m} -\mathbf{x}_{n}\|_{\infty } <\varepsilon.}$$

Therefore for fixed j we have that if m, n ≥ n ₀, then

$$\displaystyle{ \vert x_{j}^{(m)} - x_{ j}^{(n)}\vert <\varepsilon }$$

(2.10)

resulting that for all fixed j the sequence (x _j ⁽¹⁾, x _j ⁽²⁾, …) is a Cauchy sequence in $\mathbb{R}$, and this implies that there exists $x_{j} \in \mathbb{R}$ such that $\lim _{m\rightarrow \infty }x_{j}^{(m)} = x_{j}$.

Let us define x = (x ₁, x ₂, …). Now we want to show that $\mathbf{x} \in \ell_{\infty }$ and $\lim _{n\rightarrow \infty }\mathbf{x}_{n} = \mathbf{x}$.

From (2.10) we have that for n ≥ n ₀, then

$$\displaystyle{ \left \vert x_{j} - x_{j}^{(n)}\right \vert = \left \vert \lim _{ n\rightarrow \infty }x_{j}^{(m)} - x_{ j}^{(n)}\right \vert \leq \varepsilon, }$$

(2.11)

since $\mathbf{x}_{n} =\{ x_{j}^{(n)}\}_{j\in \mathbb{N}} \in \ell_{\infty }$, there exists a real number M _n such that $\left \vert x_{j}^{(n)}\right \vert \leq M_{n}$ for all j.

By the triangle inequality, we have

$$\displaystyle{\left \vert x_{j}\right \vert \leq \left \vert x_{j} - x_{j}^{(n)}\right \vert + \left \vert x_{ j}^{(n)}\right \vert \leq \varepsilon +M_{ n}}$$

whenever n ≥ n ₀, this inequality being true for any j. Moreover, since the right-hand side does not depend on j, therefore $\{x_{j}\}_{j\in \mathbb{N}}$ is a sequence of bounded real numbers, this implies that $\mathbf{x} =\{ x_{j}\}_{j\in \mathbb{N}} \in \ell_{\infty }$.

From (2.11) we also obtain

$$\displaystyle{\|\mathbf{x}_{n} -\mathbf{x}\|_{\ell_{\infty }} =\sup _{j\in \mathbb{N}}\left \vert x_{j}^{(n)} - x_{ j}\right \vert <\varepsilon.}$$

whenever n ≥ n ₀. From this we conclude that $\lim _{n\rightarrow \infty }\mathbf{x}_{n} = \mathbf{x}$ and therefore $\ell_{\infty }$ is complete. □ 

The following result shows a “natural” way to introduce the norm in the $\ell_{\infty }$ space via a limiting process.

Theorem 2.12.

Taking the norm of Lebesgue sequence space as in (2.4)we have that $\lim _{p\rightarrow \infty }\|\mathbf{x}\|_{\ell_{p}} =\| \mathbf{x}\|_{\ell_{\infty }}$ .

Proof.

Observe that $\vert x_{k}\vert \leq \left (\sum _{k=1}^{n}\vert x_{k}\vert ^{p}\right )^{\frac{1} {p} }$, therefore $\vert x_{k}\vert \leq \|\mathbf{x}\|_{\ell_{p}}$ for k = 1, 2, 3, …, n, from which

$$\displaystyle{\sup _{1\leq k\leq n}\vert x_{k}\vert \leq \|\mathbf{x}\|_{\ell_{p}},}$$

whence

$$\displaystyle{ \|\mathbf{x}\|_{\ell_{\infty }} \leq \liminf _{p\rightarrow \infty }\|\mathbf{x}\|_{\ell_{p}}. }$$

(2.12)

On the other hand, note that

$$\displaystyle{\left (\sum _{k=1}^{n}\vert x_{ k}\vert ^{p}\right )^{\frac{1} {p} } \leq \left (\sum _{k=1}^{n}\left (\sup _{1\leq k\leq n}\vert x_{k}\vert \right )^{p}\right )^{\frac{1} {p} } \leq n^{\frac{1} {p} }\|\mathbf{x}\|_{\ell_{ \infty }},}$$

then for all ε > 0, there exists N such that

$$\displaystyle{\|\mathbf{x}\|_{\ell_{p}} \leq \left (\sum _{k=1}^{N}\vert x_{ k}\vert ^{p}+\epsilon \right )^{\frac{1} {p} } \leq \left (\|\mathbf{x}\|_{\ell_{ \infty }}^{p}N+\epsilon \right )^{\frac{1} {p} } \leq \|\mathbf{x}\|_{\ell_{ \infty }}\left (N + \frac{\epsilon } {\|\mathbf{x}\|_{\ell_{\infty }}^{p}}\right )^{\frac{1} {p} },}$$

therefore

$$\displaystyle{ \limsup _{p\rightarrow \infty }\|\mathbf{x}\|_{\ell_{p}} \leq \|\mathbf{x}\|_{\ell_{\infty }}. }$$

(2.13)

Combining (2.12) and (2.13) results

$$\displaystyle{\|\mathbf{x}\|_{\ell_{\infty }} \leq \liminf _{p\rightarrow \infty }\|\mathbf{x}\|_{\ell_{p}} \leq \limsup _{p\rightarrow \infty }\|\mathbf{x}\|_{\ell_{p}} \leq \|\mathbf{x}\|_{\ell_{\infty }},}$$

and from this we conclude that $\lim _{p\rightarrow \infty }\|\mathbf{x}\|_{\ell_{p}} =\| \mathbf{x}\|_{\ell_{\infty }}.$ □ 

Now we study the dual space of ℓ ₁ which is $\ell_{\infty }$.

Theorem 2.13.

The dual space of ℓ ₁ is $\ell_{\infty }$ .

Proof.

For all x ∈ ℓ ₁, we can write $\mathbf{x} =\sum _{ k=1}^{\infty }\alpha _{k}e_{k}$, where $e_{k} = (\delta _{kj})_{j=1}^{\infty }$ forms a Schauder basis in ℓ ₁, since

$$\displaystyle{\mathbf{x} -\sum _{k=1}^{n}\alpha _{ k}e_{k} = (\mathop{\underbrace{0,\ldots,0}}\limits _{n},\alpha _{n+1},\ldots )}$$

and

$$\displaystyle{\left \|\mathbf{x} -\sum _{k=1}^{n}\alpha _{ k}e_{k}\right \|_{\ell_{1}} = \left \|\sum _{k=n+1}^{\infty }\alpha _{ k}e_{k}\right \|_{\ell_{1}} \rightarrow 0}$$

since the series $\sum _{k=1}^{\infty }\alpha _{k}e_{k}$ is convergent.

Let us define $T(f) =\{ f(e_{k})\}_{k\in \mathbb{N}}$, for all f ∈ (ℓ ₁)^∗. Since $f(\mathbf{x}) =\sum _{k\in \mathbb{N}}\alpha _{k}f(e_{k})$, then $\vert f(e_{k}) \leq \| f\|$, since $\|e_{k}\|_{\ell_{1}} = 1$. In consequence, $\sup _{k\in \mathbb{N}}\vert f(e_{k})\vert \leq \| f\|$, therefore $\{f(e_{k})\}_{k\in \mathbb{N}} \in \ell_{\infty }$.

We affirm that:

• (i) T is onto. In fact, for all $b =\{\beta _{k}\}_{k\in \mathbb{N}} \in \ell_{\infty }$, let us define $q \mathop{:}\ell _{1} \rightarrow \mathbb{R}$ as $g(\mathbf{x}) =\sum _{k\in \mathbb{N}}\alpha _{k}\beta _{k}$ if $\mathbf{x} =\{\alpha _{k}\}_{k\in \mathbb{N}} \in \ell_{\infty }$. The functional g is bounded and linear since

  $$\displaystyle{\vert g(\mathbf{x})\vert \leq \sum _{k\in \mathbb{N}}\vert \alpha _{k}\beta _{k}\vert \leq \sup _{k\in \mathbb{N}}\vert \beta _{k}\vert \sum _{k\in \mathbb{N}}\vert \alpha _{k}\vert =\| \mathbf{x}\|_{\ell_{1}} \cdot \sup _{k\in \mathbb{N}}\vert \beta _{k}\vert,}$$

  then g ∈ (ℓ ₁)^∗. Moreover, since $g(e_{k}) =\sum _{j\in \mathbb{N}}\delta _{kj}\beta _{j}$,

  $$\displaystyle{T(g) =\{ g(e_{k})\}_{k\in \mathbb{N}} =\{\beta _{k}\}_{k\in \mathbb{N}} = b.}$$

• (ii) T is 1-1. If Tf ₁ = Tf ₂, then f ₁(e _k ) = f ₂(e _k ), for all k. Since we have $f_{1}(\mathbf{x}) =\sum _{k\in \mathbb{N}}\alpha _{k}f_{1}(e_{k})$ and $f_{2}(\mathbf{x}) =\sum _{k\in \mathbb{N}}\alpha _{k}f_{2}(e_{k})$, then f ₁ = f ₂.

• (iii) T is an isometry. In fact,

  $$\displaystyle{ \|Tf\|_{\infty } =\sup _{k\in \mathbb{N}}\vert f(e_{k})\vert \leq \| f\| }$$

  (2.14)

  and

  $$\displaystyle{\vert f(\mathbf{x})\vert = \left \vert \sum _{k\in \mathbb{N}}\alpha _{k}f(e_{k})\right \vert \leq \sup _{j\in \mathbb{N}}\vert f(e_{k})\vert \sum _{k\in \mathbb{N}}\vert \alpha _{k}\vert =\| \mathbf{x}\|_{\ell_{1}}\sup _{k\in \mathbb{N}}\vert f(e_{k})\vert.}$$

  Then

  $$\displaystyle{ \|f\| \leq \sup _{k\in \mathbb{N}}\vert f(e_{k})\vert =\| Tf\|_{\infty }. }$$

  (2.15)

  Combining (2.14) and (2.15) we get that $\|Tf\|_{\infty } =\| f\|$. We thus showed that the spaces (ℓ ₁)^∗ and $\ell_{\infty }$ are isometric.

 □ 

One of the main difference between ℓ _p and $\ell_{\infty }$ spaces is the separability issue. The space of bounded sequence $\ell_{\infty }$ is not separable, contrasting with the separability of the ℓ _p spaces whenever $1 \leq p < \infty$, see Theorem 2.8.

Theorem 2.14.

The space $\ell_{\infty }$ is not separable.

Proof.

Let us take any enumerable sequence of elements of $\ell_{\infty }$, namely $\left \{\mathbf{x}_{n}\right \}_{n\in \mathbb{N}}$, where we take the sequences in the form

$$\displaystyle\begin{array}{rcl} \mathbf{x}_{1}& =& \left (x_{1}^{(1)},x_{ 2}^{(1)},x_{ 3}^{(1)},\ldots,x_{ k}^{(1)},\ldots \right ) {}\\ \mathbf{x}_{2}& =& \left (x_{1}^{(2)},x_{ 2}^{(2)},x_{ 3}^{(2)},\ldots,x_{ k}^{(2)},\ldots \right ) {}\\ \mathbf{x}_{3}& =& \left (x_{1}^{(3)},x_{ 2}^{(3)},x_{ 3}^{(3)},\ldots,x_{ k}^{(3)},\ldots \right ) {}\\ \cdots & \cdots & \cdots \cdots \cdots \cdots \cdots \cdots \cdots \cdots \cdots {}\\ \mathbf{x}_{k}& =& \left (x_{1}^{(k)},x_{ 2}^{(k)},x_{ 3}^{(k)},\ldots,x_{ k}^{(k)},\ldots \right ) {}\\ \cdots & \cdots & \cdots \cdots \cdots \cdots \cdots \cdots \cdots \cdots \cdots {}\\ \end{array}$$

We now show that there exists an element in $\ell_{\infty }$ which is at a distance bigger than 1 for all elements of $\left \{\mathbf{x}_{n}\right \}_{n\in \mathbb{N}}$, showing the non-separability nature of the $\ell_{\infty }$ space.

Let us take $\mathbf{x} =\{ x_{n}\}_{n\in \mathbb{N}}$ as

$$\displaystyle{x_{n} = \left \{\begin{array}{@{}l@{\quad }l@{}} 0, \quad &\text{if }\vert x_{n}^{(n)}\vert \geq 1; \\ x_{n} = x_{n}^{(n)} + 1,\quad &\text{if }\vert x_{n}^{(n)}\vert < 1. \end{array} \right.}$$

It is clear that $\mathbf{x} \in \ell_{\infty }$ and $\mathinner{\!\left \Vert \mathbf{x} -\mathbf{x}_{n}\right \Vert }_{\ell_{\infty }} > 1$ for all $n \in \mathbb{N}$, which entails that $\ell_{\infty }$ is not separable. □ 

We now define some subspaces of $\ell_{\infty }$, which are widely used in functional analysis, for example, to construct counter-examples.

Definition 2.15.

Let x = (x ₁, x ₁, …).

By c we denote the subspace of $\ell_{\infty }$ such that $\lim _{n\rightarrow \infty }x_{n}$ exists and is finite.

By c ₀ we denote the subspace of $\ell_{\infty }$ such that $\lim _{n\rightarrow \infty }x_{n} = 0$.

By c ₀₀ we denote the subspace of $\ell_{\infty }$ such that $\mathop{\mathrm{supp}}\limits (\mathbf{x})$ is finite. ⊘

These newly introduced spaces enjoy some interesting properties, e.g., c ₀ is the closure of c ₀₀ in $\ell_{\infty }$. For more properties, see Problem 2.20.

4 Hardy and Hilbert Inequalities

We now deal with the discrete version of the well-known Hardy inequality.

Theorem 2.16 (Hardy’s inequality).

Let $\{a_{n}\}_{n\in \mathbb{N}}$ be a sequence of real positive numbers such that $\sum _{n=1}^{\infty }a_{n}^{p} < \infty$ . Then

$$\displaystyle{\sum _{n=1}^{\infty }\left ( \frac{1} {n}\sum _{k=1}^{n}a_{ k}\right )^{p} \leq \left ( \frac{p} {p - 1}\right )^{p}\sum _{ n=1}^{\infty }a_{ n}^{p}.}$$

Proof.

Let $\alpha _{n} = \frac{A_{n}} {n}$ where $A_{n} = a_{1} + a_{2} + \cdots + a_{n}$, i.e., A _n  = n α _n , then

$$\displaystyle{ a_{1} + a_{2} + \cdots + a_{n} = n\alpha _{n}, }$$

(2.16)

from which we get that $a_{n} = n\alpha _{n} - (n - 1)\alpha _{n-1}.$ Let us consider now

$$\displaystyle\begin{array}{rcl} \alpha _{n}^{p} - \frac{p} {p - 1}\alpha _{n}^{p-1}a_{ n}& =& \alpha _{n}^{p} - \frac{p} {p - 1}\left [n\alpha _{n} - (n - 1)\alpha _{n-1}\right ]\alpha _{n}^{p-1} {}\\ & =& \alpha _{n}^{p} - \frac{pn} {p - 1}\alpha _{n}\alpha _{n}^{p-1} + \frac{p(n - 1)} {p - 1} \alpha _{n-1}\alpha _{n}^{p-1}. {}\\ \end{array}$$

In virtue of Corollary 1.10 we have

$$\displaystyle\begin{array}{rcl} \frac{p(n - 1)} {p - 1} \alpha _{n-1}\alpha _{n}^{p-1}& \leq & \frac{p(n - 1)} {p - 1} \frac{\alpha _{n-1}^{p}} {p} + \frac{p(n - 1)} {p - 1} \frac{\alpha _{n}^{q(p-1)}} {q} {}\\ & =& \frac{n - 1} {p - 1} \alpha _{n-1}^{p} + \frac{p(n - 1)} {p - 1} \left (1 -\frac{1} {p}\right )\alpha _{n}^{p} {}\\ & =& \frac{n - 1} {p - 1} \alpha _{n-1}^{p} + (n - 1)\alpha _{ n}^{p}, {}\\ \end{array}$$

therefore

$$\displaystyle\begin{array}{rcl} \alpha _{n}^{p} - \frac{p} {p - 1}\alpha _{n}^{p-1}a_{ n}& \leq & \alpha _{n}^{p} - \frac{pn} {p - 1}\alpha _{n}^{p} + \frac{n - 1} {p - 1} \alpha _{n-1}^{p} + (n - 1)\alpha _{ n}^{p} {}\\ & =& \frac{p\alpha _{n}^{p} -\alpha _{n}^{p} - pn\alpha _{n}^{p}} {p - 1} + \frac{(n - 1)\alpha _{n-1}^{p} + (p - 1)(n - 1)\alpha _{n}^{p}} {p - 1} {}\\ & =& \frac{p\alpha _{n}^{p} -\alpha _{n}^{p} - pn\alpha _{n}^{p} + (n - 1)\alpha _{n-1}^{p} + (pn - p - n + 1)\alpha _{n}^{p}} {p - 1} {}\\ & =& \frac{1} {p - 1}\left [(n - 1)\alpha _{n-1}^{p} - n\alpha _{ n}^{p}\right ], {}\\ \end{array}$$

from which

$$\displaystyle\begin{array}{rcl} \sum _{n=1}^{N}\alpha _{ n}^{p} - \frac{p} {p - 1}\sum _{n=1}^{N}\alpha _{ n}^{p-1}a_{ n}& \leq & \frac{1} {p - 1}\sum _{n=1}^{N}\left [(n - 1)\alpha _{ n-1}^{p} - n\alpha _{ n}^{p}\right ] {}\\ & =& \frac{1} {p - 1}\left [-\alpha _{1}^{p} +\alpha _{ 1}^{p} - 2\alpha _{ 2}^{p} + \cdots - N\alpha _{ N}^{p}\right ] {}\\ & =& -\frac{N\alpha _{N}^{p}} {p - 1} \leq 0. {}\\ \end{array}$$

Then

$$\displaystyle{\sum _{n=1}^{N}\alpha _{ n}^{p} \leq \frac{p} {p - 1}\sum _{n=1}^{N}\alpha _{ n}^{p-1}a_{ n}.}$$

By Hölder’s inequality we have that

$$\displaystyle\begin{array}{rcl} \sum _{n=1}^{\infty }\alpha _{ n}^{p}& \leq & \frac{p} {p - 1}\left (\sum _{n=1}^{\infty }a_{ n}^{p}\right )^{\frac{1} {p} }\left (\sum _{n=1}^{\infty }\alpha _{n}^{q(p-1)}\right )^{\frac{1} {q} } {}\\ & =& \frac{p} {p - 1}\left (\sum _{n=1}^{\infty }a_{ n}^{p}\right )^{\frac{1} {p} }\left (\sum _{n=1}^{\infty }\alpha _{n}^{p}\right )^{\frac{1} {q} }, {}\\ & & {}\\ \end{array}$$

then

$$\displaystyle{ \left (\sum _{n=1}^{\infty }\alpha _{ n}^{p}\right )^{1-\frac{1} {q} } \leq \frac{p} {p - 1}\left (\sum _{n=1}^{\infty }a_{ n}^{p}\right )^{\frac{1} {p} } }$$

and this implies

$$\displaystyle{\sum _{n=1}^{\infty }\left ( \frac{1} {n}\sum _{k=1}^{\infty }a_{ k}\right )^{p} \leq \left ( \frac{p} {p - 1}\right )^{p}\sum _{ n=1}^{\infty }a_{ n}^{p}.}$$

 □ 

We now want to study the so-called Hilbert inequality. We need to remember some basic facts about complex analysis, namely

$$\displaystyle{ {\frac{\uppi } {\sin (\uppi z)}} = {\frac{1} {z}} +\sum _{ n=1}^{\infty }(-1)^{n}\left ( {\frac{1} {z + n}} + {\frac{1} {z - n}}\right ). }$$

(2.17)

Let us consider the function

$$\displaystyle{f(z) = \frac{1} {\root{p}\of{z}(z + 1)}\qquad (p > 1)}$$

defined in the region $D_{1} =\{ z \in \mathbb{C} \mathop{:} 0 < \vert z\vert < 1\}$. We want to obtain the Laurent expansion. In fact, if | z |  < 1, then

$$\displaystyle{ \frac{1} {1 + z} = \frac{1} {1 - (-z)} =\sum _{ n=0}^{\infty }(-z)^{n} =\sum _{ n=0}^{\infty }(-1)^{n}z^{n}, }$$

therefore

$$\displaystyle{ f(z) =\sum _{ n=0}^{\infty }(-1)^{n}z^{n-\frac{1} {p} }. }$$

(2.18)

By the same reasoning, let us consider

$$\displaystyle{g(z) = \frac{1} {z^{1+ \frac{1} {p} }\left (1 + \frac{1} {z}\right )}}$$

defined in the region $D_{2} =\{ z \in \mathbb{C} \mathop{:} \vert z\vert > 1\}$. Since $\left \vert \frac{1} {z}\right \vert < 1$, then

$$\displaystyle{ \frac{1} {1 + \frac{1} {z}} = \frac{1} {1 - (-\frac{1} {z})} =\sum _{ n=0}^{\infty }\left (-\frac{1} {z}\right )^{n} =\sum _{ n=0}^{\infty }(-1)^{n}z^{-n}. }$$

Therefore

$$\displaystyle{ g(z) =\sum _{ n=0}^{\infty }(-1)^{n}z^{-n-1-\frac{1} {p} }. }$$

(2.19)

We now obtain some auxiliary inequality before showing the validity of the Hilbert inequality (2.20).

Theorem 2.17

For each positive number m and for all real p > 1 we have

$$\displaystyle{\sum _{n=1}^{\infty } {\frac{m^{\frac{1} {p} }} {n^{\frac{1} {p} }(m + n)}} \leq {\frac{\uppi } {\sin \left ( {\frac{\uppi }{p}}\right )}}.}$$

Proof.

Note that

$$\displaystyle\begin{array}{rcl} \sum _{n=1}^{\infty } \frac{m^{\frac{1} {p} }} {n^{\frac{1} {p} }(m + n)}& \leq & \int \limits _{0}^{\infty } \frac{m^{\frac{1} {p} }} {x^{\frac{1} {p} }(m + x)}\,\mathop{d\!}\nolimits x {}\\ & =& \int \limits _{0}^{\infty } \frac{\mathop{d\!}\nolimits z} {z^{\frac{1} {p} }(1 + z)} {}\\ & =& \int \limits _{0}^{1} \frac{\mathop{d\!}\nolimits z} {z^{\frac{1} {p} }(1 + z)} +\int \limits _{ 1}^{\infty } \frac{\mathop{d\!}\nolimits z} {z^{1+ \frac{1} {p} }\left (1 + \frac{1} {z}\right )}. {}\\ \end{array}$$

By (2.18) and (2.19) we deduce that

$$\displaystyle\begin{array}{rcl} \sum _{n=1}^{\infty } \frac{m^{\frac{1} {p} }} {n^{\frac{1} {p} }(m + n)}& \leq & \int \limits _{0}^{1}\left (\sum _{ n=0}^{\infty }(-1)^{n}z^{n-\frac{1} {p} }\right )\,\mathop{d\!}\nolimits z +\int \limits _{ 1}^{\infty }\left (\sum _{n=0}^{\infty }(-1)^{n}z^{-n-1-\frac{1} {p} }\right )\,\mathop{d\!}\nolimits z {}\\ & =& \sum _{n=0}^{\infty }(-1)^{n}\int \limits _{ 0}^{1}z^{n-\frac{1} {p} }\,\mathop{ d\!}\nolimits z +\sum _{ n=0}^{\infty }(-1)^{n}\int \limits _{1}^{\infty }z^{-n-1-\frac{1} {p} }\,\mathop{ d\!}\nolimits z {}\\ & =& \sum _{n=0}^{\infty } \frac{(-1)^{n}} {n -\frac{1} {p} + 1} +\sum _{ n=0}^{\infty }\frac{(-1)^{n}} {\frac{1} {p} + n} {}\\ & =& \sum _{n=1}^{\infty }\frac{(-1)^{n}} {\frac{1} {p} - n} + p +\sum _{ n=1}^{\infty }\frac{(-1)^{n}} {\frac{1} {p} + n} {}\\ & =& p +\sum _{ n=1}^{\infty }(-1)^{n}\left ( \frac{1} {\frac{1} {p} - n} + \frac{1} {\frac{1} {p} + n}\right ) {}\\ & =& \frac{\uppi } {\sin \left ( \frac{\uppi }{p}\right )}. {}\\ \end{array}$$

This last one is obtained by (2.17) with $z = \frac{1} {p}$. □ 

Remark 2.18.

In fact the proof of Theorem 2.17 is a two line proof if we remember that

$$\displaystyle{\int \limits _{0}^{\infty } \frac{x^{\alpha -1}} {(1 + x)^{\alpha +\beta }}\mathop{ d\!}\nolimits x = B(\alpha,\beta )}$$

and the fact that $B(1-\alpha,\alpha ) = {\frac{\uppi }{\sin \uppi \alpha }}$, 0 < α < 1, see Appendix C. ⊘

Before stating and proving the Hilbert inequality we need to digress into the concept of double series. Let $\left \{x_{k,j}\right \}_{j,k\in \mathbb{N}}$ be a double sequence, viz. a real-valued function $x \mathop{:} \mathbb{N} \times \mathbb{N} \rightarrow \mathbb{R}$. We say that a number L is the limit of the double sequence, denoted by

$$\displaystyle{\lim _{k,j\rightarrow \infty }x_{k,j} = L,}$$

if, for all $\varepsilon > 0$ there exists $n = n(\varepsilon )$ such that

$$\displaystyle{\vert x_{k,j} - L\vert <\varepsilon }$$

whenever k > n and j > n. We can now introduce the notion of double series using the known construction for the series, namely

$$\displaystyle{\sum _{k,j=1}^{\infty }x_{ k,j} =\varSigma }$$

if there exists the double limit

$$\displaystyle{\lim _{k,j\rightarrow \infty }\varSigma _{k,j} =\varSigma }$$

where $\varSigma _{k,j}$ is the rectangular partial sum given by

$$\displaystyle{\varSigma _{k,j} =\sum _{ m=1}^{k}\sum _{ n=1}^{j}x_{ m,n}.}$$

A notion related to the double series is the notion of iterated series, given by

$$\displaystyle{\sum _{k=1}^{\infty }\left (\sum _{ j=1}^{\infty }x_{ k,j}\right )\quad \mathrm{and}\quad \sum _{j=1}^{\infty }\left (\sum _{ k=1}^{\infty }x_{ k,j}\right ).}$$

We can visualize the iterated series in the following way. We first represent the double sequence as numbers in an infinite rectangular array and then sum by lines and by columns in the following way:

$$\displaystyle{\begin{array}{cccccc} x_{1,1} & x_{1,2} & x_{1,3} & \cdots & \rightarrow &\sum _{j=1}^{\infty }x_{1,j} =\mathop{:} L_{1} \\ x_{2,1} & x_{2,2} & x_{2,3} & \cdots & \rightarrow &\sum _{j=1}^{\infty }x_{2,j} =\mathop{:} L_{2} \\ x_{3,1} & x_{3,2} & x_{3,3} & \cdots & \rightarrow &\sum _{j=1}^{\infty }x_{2,j} =\mathop{:} L_{3}\\ \vdots&\vdots&\vdots&&& \\ \downarrow & \downarrow & \downarrow & & & \\ C_{1} \mathop{:}=\sum _{ k=1}^{\infty }x_{k,1} & C_{2} \mathop{:}=\sum _{ k=1}^{\infty }x_{k,2} & C_{3} \mathop{:}=\sum _{ k=1}^{\infty }x_{k,3} & & & \\ \end{array} }$$

and now the iterated series are given by $\sum _{j=1}^{\infty }C_{j}$ and $\sum _{k=1}^{\infty }L_{k}$.

It is necessary some caution when dealing with iterated series since the equality $\sum _{j=1}^{\infty }C_{j} =\sum _{ k=1}^{\infty }L_{k}$ is in general not true even if the series converges, as the following example shows

$$\displaystyle{\begin{array}{cccccccc} \frac{1} {2} & -\frac{1} {2} & 0 & 0 & 0 &\cdots & \rightarrow &0 \\ 0 & \frac{3} {4} & -\frac{3} {4} & 0 & 0 &\cdots & \rightarrow &0 \\ 0 & 0 & \frac{7} {8} & -\frac{7} {8} & 0 &\cdots & \rightarrow &0 \\ 0 & 0 & 0 & \frac{15} {16} & -\frac{15} {16} & \cdots & \rightarrow &0\\ \vdots&\vdots&\vdots&\vdots&\vdots&&&\\ \downarrow &\downarrow &\downarrow &\downarrow &\downarrow &&& \\ \frac{1} {2} & \frac{1} {4} & \frac{1} {8} & \frac{1} {16} & \frac{1} {32} & & & \\ \end{array} }$$

and clearly the obtained series are different. Fortunately we have a Fubini type theorem for series which states that when a double series is absolutely convergent then the double series and the iterated series are the same, i.e.

$$\displaystyle{\sum _{k,j=1}^{\infty }x_{ k,j} =\sum _{ k=1}^{\infty }\left (\sum _{ j=1}^{\infty }x_{ k,j}\right ) =\sum _{ j=1}^{\infty }\left (\sum _{ k=1}^{\infty }x_{ k,j}\right ).}$$

Not only that, it is also possible to show a stronger result, that if the terms of an absolutely convergent double series are permuted in any order as a simple series, their sum tends to the same limit.

Theorem 2.19 (Hilbert’s inequality).

Let p,q > 1 be such that $\frac{1} {p} + \frac{1} {q} = 1$ and $\{a_{n}\}_{n\in \mathbb{N}}$ , $\{b_{n}\}_{n\in \mathbb{N}}$ be sequences of nonnegative numbers such that $\sum _{m=1}^{\infty }a_{m}^{p}$ and $\sum _{n=1}^{\infty }b_{n}^{q}$ are convergent. Then

$$\displaystyle{ \sum _{m,n=1}^{\infty } \frac{a_{m}b_{n}} {m + n} \leq \frac{\uppi } {\sin \left ( \frac{\uppi }{p}\right )}\left (\sum _{m=1}^{\infty }a_{ m}^{p}\right )^{\frac{1} {p} }\left (\sum _{n=1}^{\infty }b_{n}^{q}\right )^{\frac{1} {q} }. }$$

(2.20)

Proof.

Using Hölder’s inequality and Proposition 2.17 we get

$$\displaystyle\begin{array}{rcl} & & \sum _{m,n=1}^{\infty } \frac{a_{m}b_{n}} {m + n} {}\\ & =& \sum _{m,n=1}^{\infty }\frac{m^{ \frac{1} {pq} }} {n^{ \frac{1} {pq} }} \frac{a_{m}} {(m + n)^{\frac{1} {p} }} \frac{n^{ \frac{1} {pq} }} {m^{ \frac{1} {pq} }} \frac{b_{n}} {(m + n)^{\frac{1} {q} }} {}\\ & \leq & \left (\sum _{m,n=1}^{\infty }\left ( \frac{m^{\frac{1} {q} }} {n^{\frac{1} {q} }(m + n)}\right )a_{m}^{p}\right )^{\frac{1} {p} }\left (\sum _{m,n=1}^{\infty }\left ( \frac{n^{\frac{1} {p} }} {m^{\frac{1} {p} }(m + n)}\right )b_{n}^{q}\right )^{\frac{1} {q} } {}\\ & =& \left (\sum _{m=1}^{\infty }\left (\sum _{ n=1}^{\infty } \frac{m^{\frac{1} {q} }} {n^{\frac{1} {q} }(m + n)}\right )a_{m}^{p}\right )^{\frac{1} {p} }\left (\sum _{n=1}^{\infty }\left (\sum _{m=1}^{\infty } \frac{n^{\frac{1} {p} }} {m^{\frac{1} {p} }(m + n)}\right )b_{n}^{q}\right )^{\frac{1} {q} } {}\\ & \leq & \left (\sum _{m=1}^{\infty } \frac{\uppi } {\sin \frac{\uppi } {q}}a_{m}^{p}\right )^{\frac{1} {p} }\left (\sum _{n=1}^{\infty } \frac{\uppi } {\sin \frac{\uppi } {p}}b_{n}^{q}\right )^{\frac{1} {q} } {}\\ & \leq & \left (\sum _{m=1}^{\infty } \frac{\uppi } {\sin \frac{\uppi } {p}}a_{m}^{p}\right )^{\frac{1} {p} }\left (\sum _{n=1}^{\infty } \frac{\uppi } {\sin \frac{\uppi } {p}}b_{n}^{q}\right )^{\frac{1} {q} } {}\\ & =& \left ( \frac{\uppi } {\sin \frac{\uppi }{p}}\right )^{\frac{1} {p} }\left ( \frac{\uppi } {\sin \frac{\uppi }{p}}\right )^{\frac{1} {q} }\left (\sum _{m=1}^{\infty }a_{m}^{p}\right )^{\frac{1} {p} }\left (\sum _{n=1}^{\infty }b_{n}^{q}\right )^{\frac{1} {q} } {}\\ & =& \frac{\uppi } {\sin \frac{\uppi }{p}}\left (\sum _{m=1}^{\infty }a_{ m}^{p}\right )^{\frac{1} {p} }\left (\sum _{n=1}^{\infty }b_{n}^{q}\right )^{\frac{1} {q} }, {}\\ \end{array}$$

which shows the result. □ 

5 Problems

2.20. Prove the following properties of the subspaces of $\ell_{\infty }$ introduced in Definition 2.15

• (a) The space c ₀ is the closure of c ₀₀ in $\ell_{\infty }$.

• (b) The space c and c ₀ are Banach spaces.

• (c) The space c ₀₀ is not complete.

2.21. Show that (s, ϱ) is a complete metric space, where s is the set of all sequences x = (x ₁, x ₂, …) and ϱ is given by

$$\displaystyle{\varrho (x,y) =\sum _{ k=1}^{\infty } \frac{1} {2^{k}} \frac{\vert x_{k} - y_{k}\vert } {1 + \vert x_{k} - yk\vert }.}$$

2.22. Let ℓ_p(w), p ≥ 1 be the set of all real sequences x = (x ₁, x ₂, …) such that

$$\displaystyle{\sum _{k=1}^{\infty }\vert x_{ k}\vert ^{p}w_{ k} < \infty }$$

where w = (w ₁, w ₂, …) and w _k  > 0. Does $\mathcal{N}\mathop{:}\ell _{p}(\mathbf{w})\longrightarrow \mathbb{R}$ given by

$$\displaystyle{\mathcal{N}(\mathbf{x}) \mathop{:}= \left (\sum _{k=1}^{\infty }\vert x_{ k}\vert ^{p}w_{ k}\right )^{\frac{1} {p} }}$$

defines a norm in ℓ _p (w)?

2.23. As in the case of Example 2.2, draw the unit ball for ℓ ₁ ³, $\ell_{\infty }^{3}$, and ℓ ₂ ³.

2.24. Prove that (2.4) defines a norm in the space $\ell_{p}(\mathbb{N})$.

2.25. Prove the Cauchy-Bunyakovsky-Schwarz inequality

$$\displaystyle{ \left (\sum _{i=1}^{n}x_{ i}y_{i}\right )^{2}\leqslant \left (\sum _{ i=1}^{n}x_{ i}^{2}\right )\left (\sum _{ i=1}^{n}y_{ i}^{2}\right ) }$$

without using Jensen’s inequality. This inequality is sometimes called Cauchy, Cauchy-Schwarz or Cauchy-Bunyakovsky.

Hint: Analyze the quadratic form $\sum _{i=1}^{n}\left (x_{i}u + y_{i}v\right )^{2} = u^{2}\sum _{i=1}^{n}x_{i}^{2} + 2uv\sum _{i=1}^{n}x_{i}y_{i} + v^{2}\sum _{i=1}^{n}y_{i}^{2}.$

2.26. Let {a _n } _{n ∈ ℤ} and {b _n } _{n ∈ ℤ} be sequences of real numbers such that

$$\displaystyle{k =\sum _{ n=-\infty }^{\infty }\vert a_{ n}\vert < \infty \quad \text{and}\quad \sum _{m=-\infty }^{\infty }\vert b_{ m}\vert ^{p} < \infty }$$

where p > 1. Let $C_{n} =\sum _{ m=-\infty }^{\infty }a_{n-m}b_{m}$. Prove that

• (a) $\vert C_{n}\vert \leq k^{1/q}\left (\sum _{m=-\infty }^{\infty }\vert a_{n-m}\vert \vert b_{m}\vert ^{p}\right )^{1/p}$ where $\frac{1} {p} + \frac{1} {q} = 1$.

• (b) $\left (\sum _{n=-\infty }^{\infty }\vert C_{n}\vert ^{p}\right )^{1/p} \leq k\left (\sum _{n=-\infty }^{\infty }\vert b_{n}\vert ^{p}\right )^{1/p}$.

2.27. If a _n  > 0 for n = 1, 2, 3, … show that

$$\displaystyle{\sum _{n=1}^{\infty }\root{n}\of{a_{ 1}a_{2}\cdots a_{n}} \leq \mathrm{ e}\sum _{n=1}^{\infty }a_{ n}.}$$

If a ₁ ≥ a ₂ ≥ ⋯ ≥ a _k  ≥ ⋯ ≥ a _n  ≥ 0 and α ≥ β > 0. Demonstrate that

$$\displaystyle{\left (\sum _{k=1}^{n}a_{ k}^{\alpha }\right )^{1/\alpha } \leq \left (\sum _{ k=1}^{n}a_{ k}^{\beta }\right )^{1/\beta }.}$$

2.28. Use Theorem 10.5 to show the Theorem 2.16.

Hint: Choose a sequence $\ \{a_{n}\}_{n\in \mathbb{N}}$ of positive numbers such that a _n+1 ≥ a _n $\forall n \in \mathbb{N}$. Consider $A_{N} =\sum _{ n=1}^{N}a_{n}$ and define $f =\sum _{ n=1}^{\infty }a_{n}\chi _{(n-1,n)}$.

2.29. Demonstrate that ℓ ₁ is not the dual space of $\ell_{\infty }$.

2.30. Show that

$$\displaystyle{ \mathinner{\!\left \Vert \mathbf{x}\right \Vert }_{\ell_{q}} \leq \mathinner{\!\left \Vert \mathbf{x}\right \Vert }_{\ell_{p}} }$$

(2.21)

whenever $1 \leq p < q < \infty$.

Hint: First, show the inequality (2.21) when $\mathinner{\!\left \Vert \mathbf{x}\right \Vert }_{\ell_{ p}} \leq 1$. Use that result and the homogeneity of the norm to get the general case.

6 Notes and Bibliographic References

The history of Hölder’s inequality can be traced back to Hölder [32] but the paper of Rogers [61] preceded the one from Hölder just by one year, for the complete history see Maligranda [48].

The Minkowski inequality is due to Minkowski [51] but it seems that the classical approach to the Minkowski inequality via Hölder’s inequality is due to Riesz [58].

The Hardy inequality (Theorem 2.16) appeared in Hardy [26] as a generalization of a tool to prove a certain theorem of Hilbert.

According to Hardy, Littlewood, and Pólya [30], the Hilbert inequality (Theorem 2.19) was included by Hilbert for p = 2 in his lectures, and it was published by Weyl [82], the general case p > 1 appeared in Hardy [27].

The Cauchy-Bunyakovsky-Schwarz inequality, which appears in Problem 2.25, was first proved by Cauchy [6].