Keywords

These keywords were added by machine and not by the authors. This process is experimental and the keywords may be updated as the learning algorithm improves.

1 Introduction

It is ‘a-priori’ unclear whether there are coherent structures in turbulence, or how they should be defined. Their most compelling support derives from free-shear flows, where visualisations reveal a wave-like organisation of advected scalars [1], which can be traced for relatively long times, and that can be linked to the Kelvin–Helmholtz instability of the mean velocity profile. Besides that visual impression, which did a lot to crystallise a structural view of turbulent flows with which to complement earlier stochastic descriptions, the identification of those waves in terms of a known dynamical process was important. It opened the way to the prediction of their properties, and later to effective control strategies [2, 3].

The situation is not as satisfactory for wall-bounded turbulence, in which the mean velocity profile does not sustain a linear modal instability. Long-lived structures were identified in wall turbulence even earlier than in free-shear flows [4], mainly in the form of long streaky structures of the streamwise velocity, but it was soon realised that the streaks could not survive by themselves, and that other structures were needed to complete a self-sustaining regeneration cycle [5–7]. In the past 20 years, numerous models for the dynamics of such flows have been proposed, such as linearised approximations, both modal and non-modal [8–10]; specific structures such as hairpin vortices [11–13]; instabilities of the velocity streaks [14, 15] and equilibrium and time-periodic exact solutions of the Navier–Stokes equations [16, 17].

In most cases, structures that approximately answer to the description in those models can be identified in turbulent flows, and there are physical grounds to believe that they really exist and may even be common. Moreover, all of them share some degree of intellectual appeal that is at the root of the original proposal. However, the question of how often the structures and the evolution that they predict actually occur in the flow, and how relevant are they to its dynamics, has generally been addressed at most qualitatively or partially (e.g. hairpin ‘heads’, or wall-normal velocity ‘bursts’).

There is little doubt that exact structures of any kind are unlikely to be found in turbulence. All of the examples given above either exist only at Reynolds numbers well below those of fully developed turbulence, or are unstable or transient. On the other hand, it has been persuasively argued that equilibrium solutions and other simple trajectories in phase space, even if unstable or transient, are approached by the flow more often than other random non-equilibrium states, and are therefore more relevant to the statistics than other kinematically possible flow fields [17, 18].

In recent years, temporally and spatially resolved numerical databases have begun to allow the quantitative analysis of how closely a particular solution is approached by the flow, and how often that happens, although the analysis often involves isolating a particular subset of the flow, a restricted range of wavenumbers, or both. It is usually, but not always, also necessary to inhibit or discount the effect of interactions between different structures. We will discuss some examples in which it has recently become possible to test particular simplified turbulence ‘cartoons’ in essentially natural flows, examine their shortcomings, and point to possible avenues for future tests of other popular (or less popular) ones.

The organisation of the paper is as follows. Section 2 describes in some detail what has been found about two particular kinds of long-lived structures in wall-bounded flows, about how they evolve in time, and about how they are related to similar structures in shear flows far from the wall. It will be seen that a lot is known, but that optimistic impression is tempered in Sect. 3, which discussed how little we really know about the dynamical origin of what is observed, and how far are we from conclusively identifying the observations with any of the conceptual models mentioned above. Section 4 summarises and concludes.

2 We Know Everything About Turbulent Structures

Channels are often used as archetypes for the theoretical characterisation of wall-bounded turbulence because they are relatively simple to simulate (although apparently hard to realise experimentally), and because numerical simulations offer the best chance of studying the flow in detail. There are channel simulations available at relatively high Reynolds numbers [19–23], and time-resolved databases of their evolution have recently become available for analysis [24].

Two kinds of structures have been studied in detail for this flow: Vortex ‘clusters’ are connected objects of particularly strong discriminant of the velocity gradient [25], and ‘Qs’ [26] are connected regions of strong Reynolds stresses that are the three-dimensional analogues of the classical ‘quadrant’ events studied by experimentalists from single-point signals [27]. The most important Qs are those in the second ‘quadrant’ (Q2 or ejections) and in the fourth quadrant (Q4 or sweeps), i.e. those for which the wall-normal velocity fluctuations, v, have opposite sign to the streamwise velocity fluctuations, u, so that they carry a Reynolds stress consistent with the mean shear (\(uv<0\) for \(\partial _y U>0\)). Both the clusters and the Qs can be classified into wall-attached and wall-detached families, depending on whether or not their roots reach the neighbourhood of the wall. The wall-attached structures have been studied in most detail. They are larger than the local Corrsin scale [28], interact directly with the ambient mean shear, and presumably draw their energy from it [29]. The wall-attached sweeps and ejections carry over 60 % of the total tangential Reynolds stress.

Above the viscous layer near the wall, Qs and clusters are complicated objects with fractal dimensions of the order of \(D_F=2-2.5\). Two examples are given in Fig. 1, both of which are wall-attached and large enough to extend into the logarithmic layer \((L_y/h=0.15-0.20)\), where \(L_y\) is the wall-normal dimension, and h is the channel half-width. It is known that the large attached eddies of both kinds \((L_y^+\gtrsim 100)\) have self-similar aspect ratios, \(L_z\approx L_y\) and \(L_x\approx 3 L_y\) [25, 26], and lifetimes that also scale linearly with their height, \(t^+\approx L_y^+\) [30].

Fig. 1
figure 1

a Wall-attached vortex cluster. b Ejection (‘second quadrant’ Reynolds stress event). Turbulent channel at \(Re_\tau =4200\) [22]. Flow is from left to right, and axes are in wall units

Full size image

Attached sweeps and ejections are almost always found in side-by-side pairs, with a vortex cluster near their base. The mean flow conditioned to one of these pairs is a quasi-streamwise roller sitting at the boundary between a high- and a low-velocity streak of the streamwise velocity, in an arrangement reminiscent of the better-known associations of streaks and vortices in the viscous layer [15, 31]. Above the buffer region, they are typically much larger than those closer to the wall, and involve disorganised turbulent objects similar to those in Fig. 1. In those cases, the association of Qs with rollers can only be recognised in a conditional statistical sense. For an example of an individual pair, the reader is referred to Fig. 12 in [26], or to the three-dimensional version of that figure in the supplementary material to that paper.

It is interesting that, even if attached eddies play a dominant role in wall-bounded flows, the proximity of the wall does not appear to be required for their formation. A recent simulation of homogeneous shear flow [32] was found to contain vortex clusters, sweeps and ejections with statistical properties very similar to those of the large detached eddies in channels (see Fig. 2a for an example), and these large Qs are also responsible for most of the tangential Reynolds stress. It is also known that channels with rough-like walls [33] or even with no wall at all [34] have vortex clusters indistinguishable from those of normal channels.

Fig. 2
figure 2

a Large ejection in a homogeneous shear flow with microscale Reynolds number \(Re_\lambda =100\) [32]. Flow is from left to right. Compare with Fig. 1b. b Probability density function of the logarithm of the wall-normal dimension of sweeps and ejections (Q2+Q4). , Attached or detached eddies with a given maximum height, \(y_{max}/h=0.3, 0.5, 0.7\), increasing in the direction of the arrow. Turbulent channel at \(Re_\tau =2000\) [22]. , Homogeneous shear, \(Re_\lambda =100\) [32]. Axes in both panels are in Kolmogorov units

Full size image

In fact, attached eddies appear to be particular cases of detached ones that have become large enough to collide with the wall. Figure 2b shows the probability distribution of the vertical dimensions of sweeps and ejections in a channel and in homogeneous shear. Those in the channel have been separated in bands of the position of their farthest point from the wall. The probability of their vertical dimension decreases for larger eddies, roughly as \(\mathrm {pdf}(L_y)\sim L_y^{-5/2}\), until it reaches \(L_y=y_{max}\). The eddies then hit the wall, and the p.d.f. accumulates into the distribution of attached eddies. Choosing a higher band simply extends the probability tail to taller eddies before they reach the wall. The figure also contains data from a homogeneous shear, where the eddies follow the same probability distribution, but never accumulate at the (non-existent) wall.

Even more revealing is the temporal evolution of these structures, which requires tracking them in time and unravelling the numerous interactions in which they split or merge with one another along their lifetimes [24, 30]. It was in this way that the previously mentioned lifetimes were computed, although they refer to ‘primary’ eddies that are not born from a split or disappear into a merger. When interactions are taken into account, it turns out that most eddies larger than a few Kolmogorov scales \((\eta )\) lose or receive some fragments of comparable size at some point in their lives [30], and that such direct or inverse ‘cascade’ events are responsible for the largest part of their growth and decay. Numerically, however, most of the splits and mergers take place between a larger eddy and a smaller fragment of the order of the Kolmogorov scale.

Fig. 3
figure 3

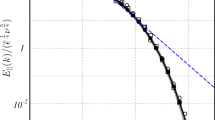
a Sketch of the splitting tree of a large eddy into smaller fragments. The index of each branch is the number of splits that separates it from its farthest descendent. b Probability density function of the (cube root of) the volume of Q-structures, separated by their splitting index, ranges from 0 to 6 in the direction of the arrow [35]. Turbulent channel at \(Re_\tau =950\) [22]

Full size image

The connection of these events with a cascade can be made more quantitative. Centring on the direct cascade, the interactions between eddies can be best studied by ordering the different branches, each of which represents the evolution of a particular eddy, into a graph in which the nodes are the splits. In each split, one ‘main’ branch survives and one is created anew. Each branch can be assigned a ‘split index’ as the number of splits that separate it from its farthest descendent. A sketch is Fig. 3a. It can be expected that eddies with a larger index are also bigger, and that their size decreases as they approach the extremal branches in which they are dissipated by viscosity. This is demonstrated in Fig. 3b which shows the p.d.f. of the eddy size (the cubic root of its volume) for eddies with a given index [35]. The characteristics size of the smallest eddies (\(\mathrm{index}=0\)) is \(L\approx 0.05h\approx 10\eta \), comparable to the diameter of the small-scale vortices [36], and those with the highest index are of the order of the integral scale, \(L\approx 0.2h\). It is interesting that the ratio between the mean volume of consecutive indices changes from about 0.2 in the viscous range \((L<30\eta )\) to about 0.5 later on. There is a complicated relation between this ratio and the average volume fraction typically lost in a split, because a given eddy can break into fragments with very different future histories, and therefore with very different indices. But, if we take the volume ratio between consecutive indices as an estimate of the ratio between the volume of successive eddy ‘generations’, the first value can be interpreted as being dominated by the loss of Kolmogorov scale fragments, and the second one as a more classical equilateral inertial cascade. A similar analysis can be done for the merging of structures, giving information on the inverse component of the cascade.

3 We Know Nothing About Turbulent Structures

We have seen in the previous section that what amounts to a complete characterization of the behaviour of two kinds of coherent structures in channel flows. This has been an important advancement of the last few years that would have been difficult to predict a decade ago. Although most of it has been based on information from simulations, some experimental time-resolved datasets, generally limited to two-dimensional sections, have contributed substantially [37–40]. All these information have given us a fresh outlook on what is going on inside the turbulent wall-bounded flows, but it can be argued that our understanding of these flows is still well below that of the free-shear turbulence.

We lack a theoretical model for the behaviour that we observe. As mentioned in the introduction, the problem is not as much a lack of models, but a superabundance. It is probably true that several of those models are equivalent, although that remains to be proved. For example, it is not inconceivable that the structures described in the previous section can be described as packets of approximate hairpin vortices. There have been numerous attempts to show that particular theories are ‘compatible’ with statistical data, e.g. [41]. They are often successful, but that should not be considered as a sufficient proof. We know from RANS modelling that suitably tuned semiempirical models with little or no structural information can predict turbulence statistics very accurately.

That is true even when fairly detailed statistics are used. For example, it was shown in [42] that the temporal evolution of minimal channels was consistent with the linearised Orr’s mechanism of inviscid transient growth. The similarities include such high-order quantities as temporal correlation functions and the detailed exchange of energy among velocity components. Similarly, it was shown in [9] that optimally amplified linearised perturbations agree well with the highest POD modes of full channels. However, it has proved difficult in both cases to identify in the flow the three-dimensional structures implied by the linear models, or their temporal evolution.

There may be several reasons for these failures. In the first place, as mentioned in the introduction, it is highly unlikely that any structure predicted by an essentially laminar model would be found in the chaotic environment of real turbulence at even moderate Reynolds numbers. The most that can probably be expected is some approximation, such as is evident, for example in the steady retreat from the identification of hairpin packets into ‘heads’, ‘legs’ or ‘incomplete’ hairpins. This is not necessarily bad, but it may easily become meaningless without a clear definition of the metric used to define the ‘presence’ of a structure.

The second problem is that many of these models actually predict transient phenomena. This is probably the best that can be expected from a system without modal instabilities, but it means that we have to identify something that only lives for a fraction of the system evolution. For example, the Orr model in [42] describes a relatively short burst in the wall-normal velocity that quickly decays after creating a streamwise velocity streak that lasts much longer. This is a fairly accurate description of the evolution of the flow in minimal channels [5–7, 43], but it means that we are trying to identify something that is not there most of the time. Again, we need to define a metric to describe not only how close is the system to a given solution, but also how often it happens. Note that this may be more complicated than just tracking time intervals or the contribution to the statistics. In the previous example, the bursts are probably not large contributors to the statistics of u or v [44], but they are necessary ‘catalysts’ for the injection of energy into the turbulent fluctuations.

A newer development has been the attempt to identify turbulent structures with exact solutions of the Navier–Stokes equations, whether permanent waves or more complex invariant sets. These efforts have generally centred on transitional flows, but some of them extend into the incipient turbulent regime. Early attempts to represent the statistics of pipes in terms of permanent waves were only moderately successful [45], and the focus quickly moved to recurrent solutions [46, 47], and to homoclinic and heteroclinic orbits as models for bursting [48–51]. All these studies share the usual ambiguity about the norm used as a measure of proximity. A promising technique that partially bypasses this limitation is to use an arbitrary norm to identify approximately a recurrent flow state, which is then used as an initial condition for the computation of exact recurrent solutions [46, 47]. However, most of these techniques have only been used on flows at low Reynolds numbers, or even on two-dimensional ones, and it is unclear whether they can be extrapolated to more complex situations.

4 Discussion and Conclusions

We have tried to show how we are both very near and very far from understanding the dynamics of shear-dominated wall-bounded turbulent flows. Very near in the sense that we have collected in the past decades what is probably a reasonably complete catalogue of coherent structures in these flows, of their properties, and of their temporal evolution. But very far in the sense that we lack a consensus theoretical model for their behaviour. Two questions suggest themselves. The first one is whether coherent structures are more than an observational construct, and whether we should really expect a theory for them. The second one is whether such a theory would be of any use.

Tackling the second question, first, our best guide could be the experience with free-shear flows mentioned at the beginning of this paper. In that case, a linearised theory for the nature of the structures naturally led to control strategies. The same has been true of our partial theoretical understanding of wall-bounded flows, even if the applications are as yet limited to simulations at moderate Reynolds numbers. For example, the early realisation of the role of sublayer streaks in determining friction drag, and of the buffer-layer vortices in sustaining the streaks, led to successful drag reduction strategies based on damping the vortices [52]. Further removed from intuition, [53] was able to laminarise turbulent Couette flow by acting on the system as it spontaneously approaches a fixed point in the ‘edge’ of the basin of attraction of turbulence. Although it is impossible to predict whether a better theoretical understanding would lead to better control strategies at realistic Reynolds numbers, the success rate of past ‘intuitive’ approaches has been at most moderate, and the previous examples offer some hope that better methods lay hidden within deeper theories.

The question about the relation between theory and structure is more complex, and cannot probably be answered conclusively at this point. Many of the theoretical models mentioned above were motivated by attempts to explain (‘postdict’) structures observed in highly constrained simulations. They were usually successful, but we have seen that the inverse question of identifying theoretical solutions in real flows has been more problematic. It is also clear that the idea of a coherent blob of strong vorticity is a very different concept from an invariant set of the Navier–Stokes equations. On the other hand, structures stay coherent for some reason, and turbulent flows are nothing but solutions of the Navier–Stokes equations. Consider one of the problems of describing any statistically stationary solution of a dissipative system such as turbulence, which is to understand how the energy is injected into the system. This is a basic question as much in fully turbulent flows, in permanent waves, or in any invariant set. In shear-dominated flows, it is known that energy injection is mediated by the production term, \(-\langle uv\rangle \partial _y U\), and it is reasonable to expect that the Q-structures described in Sect. 2, which are the dominant carriers of \(\langle uv\rangle \), play a role in that process. Similarly, vortex clusters, which label regions of high energy dissipation can be expected to be controlled by, and to modulate, the energy balance.

The basic message of this paper should be that, in spite of all the new observational information on the behaviour of turbulent wall-bounded flows, a lot remains to be understood about the reasons for that behaviour. It is the opinion of the present authors that, in the same way as a dominant thread of turbulence research during the past 50 years has been the reconciliation of the structural and statistical views of the flow, an important task for the next years will be to relate theoretical models with structural observations.