Abstract
The purpose of this work is to offer a brief survey of some of the mathematical methods useful to bridge different levels of description, i.e. from the set-up of classical kinetic theory of gases to hydrodynamics. Most of the standard mathematical techniques, in this field, stem from the seminal Chapman–Enskog expansion, which constitutes an important success in kinetic theory, as it made possible to formally derive the Navier-Stokes hydrodynamics from the Boltzmann equation. Yet, almost a century of effort to extend the hydrodynamic description beyond the Navier-Stokes-Fourier approximation failed even in the case of small deviations around the equilibrium, due to the onset of instabilities which also cause the violation of the H-Theorem. A different route, in kinetic theory, is represented by the recent Invariant Manifold method. The latter technique allows one to restore stability in the hydrodynamic equations, which remain thus applicable even at short length scales, under the hypothesis of validity of the Local Equilibrium condition.
Access provided by Autonomous University of Puebla. Download chapter PDF
Similar content being viewed by others
Keywords
These keywords were added by machine and not by the authors. This process is experimental and the keywords may be updated as the learning algorithm improves.
1 Introduction
A certain number of techniques have been designed, in kinetic theory of gases, to derive macroscopic time evolution equations from the Boltzmann equation. Most of these methods require the single-particle distribution function to be parameterized by a set of distinguished fields, such as the standard hydrodynamic ones: the number (or mass) density, momentum vector, and temperature. This stands as a plausible assumption as long as the microscopic dynamics enjoys a vast separation of time and length scales, and provided that the condition of Local Equilibrium is granted. Furthermore, the derivation of hydrodynamics from kinetic theory is often understood in terms of the so-called hydrodynamic limit of the Boltzmann equation. Loosely speaking, one is interested, typically, in the scaling of the Boltzmann equation with respect to some reference macroscopic length and time scales, which are expected to largely dominate the intrinsic kinetic scales. Nonetheless, it makes sense to consider the extension of the hydrodynamic description even beyond the purely hydrodynamic limit, so as to take into account reference scales which may happen to be comparable with the kinetic ones. This is the subject dealt with by the theory of generalized hydrodynamics [1–4]. There are several delicate aspects hindering this line of investigation. A first, natural, objection points to the fact that, below a certain length scale, the notion itself of “Local Equilibrium”, which is brought about by a sufficiently large number of particle collisions, is questionable. Moreover, from the technical side, one typically deals, in this context, with perturbative methods, such as Hilbert’s procedure or the Chapman–Enskog (CE) technique, which, at a certain order of truncation, may give rise to artificial instabilities [5, 6]. In particular, the CE method introduces an expansion of the distribution function in terms of a parameter, the Knudsen number, defined as the ratio of the mean free path to a representative macroscopic length. For small values of the Knudsen number, the CE method recovers the standard Navier-Stokes-Fourier (NSF) equations of hydrodynamics. In more refined approximations, referred to as the Burnett and super-Burnett hydrodynamics, the hydrodynamic modes become polynomials of higher order in the wavevector. In such an extension, the resulting hydrodynamic equations may become unstable and violate the H-Theorem, as first shown by Bobylev [5] for a particular case of Maxwell molecules. This indicates that the CE theory can not be immediately trusted, away from the hydrodynamic limit. Thus, while the mathematical framework concerning the hydrodynamic limit of the Boltzmann equation is well-established [7, 8], there is no consolidated counterpart addressing the short scales domain. Thus, developing a theory of Microfluidics, is a novel challenge for theorists, and has also been fostered by recent technological trends [9, 10], which call for an extension of the hydrodynamic description to the regime of finite Knudsen numbers. In this work we describe the application of the Invariant Manifold theory, which makes it possible to derive the equations of exact linear hydrodynamics from the Boltzmann equation. The adjective “exact” refers to the fact that the method allows one to perform an exact summation of all the contributions of the CE expansion. We will show, through the analysis of some solvable model, that the divergences of the hydrodynamic modes can be actually removed by taking into account also the very remote terms of the expansion. This is made possible by solving a closed integral equation, called invariance equation, which connects the microscopic evolution of the distribution function with its “projected” (in a sense to be specified below) dynamics, triggered by the set of hydrodynamic fields.
This work is structured as follows. In Sect. 3.2 we will outline some standard model reduction techniques, which allow to derive hydrodynamics from the Boltzmann equation. In Sect. 3.3 we will derive the equations of linear hydrodynamics from certain kinetic models of the Boltzmann equation, thus providing the desired bridge between the kinetic and the hydrodynamic descriptions, valid even at short length scales. Conclusions will be drawn in Sect. 3.4.
2 Bridging Time and Length Scales: From Kinetic Theory to Hydrodynamics
In this section we will offer a bird’s eye view on some of the analytical methods which yield approximate solutions of the Boltzmann equation. In particular, we will outline the structure of the Hilbert and Chapman–Enskog perturbation techniques and will also comment on the essential features of the Invariant Manifold method [1, 2], which is based on the assumption of time scale separation and applies also beyond the strict hydrodynamic limit.
Before introducing the wealth of different techniques, we shortly review the notion of hydrodynamic limit of the Boltzmann equation, and shed also light on the role of the (often invoked) time scale separation, which is one of the main ingredients underpinning the onset of a collective behavior in a many-particle system.
2.1 Hydrodynamic Limit of the Boltzmann Equation
Let \(f(\mathbf{r},\mathbf{v},t)\) denote the single-particle distribution function, depending on the position r, on the velocity v and on time t, which describes the statistics of a dilute gas made of N particles of mass m, inside a box \(\varLambda _{\varepsilon }\) with volume V. In absence of external forces, we assume that the distribution f obeys the Boltzmannequation [8]
where Q(f, f) denotes a nonlinear integral collision operator whose detailed analytical structure is not relevant here. We just need to recall that Q(f, f) vanishes when the distribution function attains the so-called local Maxwellianstructure:
which is parameterized by the fields n (number density, i.e. number of particles per unit volume), u (bulk velocity of the fluid), and T (temperature). A point of remarkable interest, in kinetic theory, concerns the study of the scaling properties of the Boltzmann equation (3.1). In particular, one may investigate the structure of the solutions of the Boltzmann equation in the so-called hydrodynamic limit, which is obtained by taking the limits N → ∞, V → ∞, with N∕V finite. Following [7], we introduce a small parameter \(\varepsilon\), and let the side of the box \(\varLambda _{\varepsilon }\) be proportional to \(\varepsilon ^{-1}\). We assume, then, that the total number of particles contained in the box, obtained by integrating f over \(\varLambda _{\varepsilon }\) and over the whole velocity space, is proportional to the volume of the box itself:
and introduce the following scaling:
with \(\hat{\mathbf{r}} \in \varLambda = [0,1]\), and
It is readily seen that the rescaled distribution \(\hat{f}(\hat{\mathbf{r}},\mathbf{v},\tau )\) describes the statistics of particle system on the scale of the box, and is normalized to unity:
While the description afforded in terms of the distribution f is called mesoscopic (or kinetic), the description provided by the rescaled distribution \(\hat{f}\) can be thought of as a macroscopic one, because it describes the particle system in the limit of large length and time scales. Using (3.4) and (3.5), the Boltzmann equation, in the absence of external forces, can be shown to attain the following rescaled structure [8]
which will be the starting point of the methods of reduced description to be described below.
2.2 The Bogoliubov Hypothesis and Macroscopic Equations
Let us denote by \(\mathbf{M}_{f} = [M_{1},\mathbf{M}_{2},M_{3}]\) the lower-order moments of the distribution function f, defined as:
where \(\boldsymbol{\psi }(\mathbf{v})\) are known as the collisional invariants:
with
and \(v \equiv \vert \mathbf{v}\vert \). Thus, by integrating, one finds:
where \(\rho (\mathbf{r},t) = \mathit{mn}(\mathbf{r},t)\) is the mass density, and
denotes the internal energy per unit mass, which is related, via the equipartition theorem, to the temperature T. The hydrodynamic fields [ρ, u, T] are thus related to the moments M f via:
We consider, from here onwards, a special class of distribution functions called normal solutions of the Boltzmann equation [8]. These are distribution functions whose dependence on the variables (r, t) is parameterized by a set of fields \(\mathbf{x}(\mathbf{r},t)\), which may correspond to the hydrodynamic fields themselves, but in certain models may also include higher-order moments of the distribution function [11–13]. Thus, we start writing the single-particle distribution function in the form:
A physical rationale behind Eq. (3.13) can be traced back to the Bogoliubov hypothesis [14–16]. In his seminal work, Bogoliubov introduced three different time scales, labeled \(\tau _{\mathit{int}},\tau _{\mathit{mf }}\) and τ macro : the time interval τ int is the time during which two molecules are in each other’s interaction domain, τ mf denotes the mean time between collisions, and τ macro corresponds to the average time needed for a molecule to traverse the container in which the gas is confined. The Bogoliubov hypothesis thus states that the existence of a vast time scale separation
is a prerequisite underpinning the relaxation of a gas towards equilibrium and the onset of a hydrodynamic behavior. In a similar fashion, it is possible to introduce three corresponding displacements, denoted as \(\lambda _{\mathit{int}},\lambda _{\mathit{mf }},\lambda _{\mathit{macro}}\). The displacement λ int corresponds the range of particle interaction, λ mf is the mean free path and λ macro denotes a macroscopic reference length, such as the edge length of the confining container. Typical values of these parameters are listed in Table 3.1.
The Bogoliubov hypothesis addresses the structural form of the phase space density \(F(\boldsymbol{z}_{1},\ldots,\mathbf{z}_{N},t)\) (we used the shorthand notation z = (r, v)) pertaining to three different stages during the relaxation of the gas to equilibrium, cf. also Table 3.2.
In the initial interval there is no collisional exchange between the particles, and the gas experiences no equilibrating force. Therefore, Bogoliubov conjectured that, in such initial stage, the full N-particle density is required to properly describe the state of the gas. In the kinetic stage, the molecules experience a sequence of collisions, which give rise to the onset of Local Equilibrium in the gas. According to Bogoliubov’s hypothesis, during this stage all s-particle marginals may be expressed as functionals of the single-particle density, i.e.
where the time dependence of F s is entirely contained in \(F_{1}(\boldsymbol{z}_{1},t)\). For instance, if the particles are statistically independent from each other, F s factorizes as follows:
Finally, in the hydrodynamic stage, the relevant time scale is τ macro , which characterizes the time evolution of the macroscopic variables x(r, t). It is worth noticing that, in the course of the relaxation, a crucial loss of information occurs [15]: while, in the initial stage, the microscopic state of the particle system is described by the full phase space density, close to equilibrium the statistical description is suitably afforded only in terms of the single-particle distribution function, parameterized by the variables x.
We also remark that the condition \(\tau _{\mathit{int}} \ll \tau _{\mathit{mf }}\) is essential in order to write the Boltzmann equation in the form (3.1). The distribution function f(r, v, t) must be regarded as an average of the single-particle distribution in a time interval dt, with
This condition, in fact, allows one to disregard the variation experienced by the distribution function of the hitting particle, f(r, v 1, t), during the time τ int of interaction with the target particle. If the condition (3.17) does not hold, one should use, in Eq. (3.1), the distribution function \(f(\mathbf{r},\mathbf{v}_{1},t -\tau _{\mathit{int}})\) evaluated at an earlier time and the rate of change of f at time t would depend not only on the instantaneous value of f, but also on its previous history [17]. This would make the Boltzmann equation a non-markovian process. We see, hence, that the separation between \(\tau _{\mathit{int}}\) and τ mf makes it possible to identify an intermediate scale dt, which guarantees the markovian character of the Boltzmann equation. We can also conceive one further time scale, which we denote by Δ t, which corresponds to the characteristic “mesoscopic” time scale characterizing the onset of Local Equilibrium. A macroscopic description of a particle system, based on the hydrodynamic fields x(r, t), can be obtained by confining the description to time scales not shorter than Δ t, which is typically intermediate between \(\tau _{\mathit{mf }}\) and τ macro . The role of the time scale Δ t can be better evinced by introducing a partition of the volume of the gas into mesoscopic cells of linear size ℓ meso , as portrayed in Fig. 3.1. Local equilibrium is reached, within each cell, after the time interval Δ t. Therefore, although the hydrodynamic variables may vary over macroscopic length and time scales, within each cell they obey, after the time interval Δ t, the usual relations of equilibrium thermodynamics [18].
The onset of Local Equilibrium in mesoscopic cells of size ℓ meso , taking place after a time interval Δ t. Local Equilibrium results from a large number of collisions, occurring on time scales of the order of τ mf . The hydrodynamic fields attain a local value within each of the cells, and evolve on a time scale τ macro (not shown in the picture) much larger than Δ t, according to the time scale separation hypothesis
The dimensionless parameter \(\varepsilon\) is the Knudsen number [8, 19] and is defined as the ratio of λ mf to λ macro . The aim of a generalized hydrodynamic theory is, hence, to extend the macroscopic description to finite Knudsen numbers, well beyond the standard hydrodynamic limit (corresponding to \(\varepsilon \ll 1\)). This, in turn, requires a proper estimate, for the model under consideration, of the magnitude of the length scale ℓ meso , below which Local Equilibrium no longer holds. Thus, if we consider macroscopic length and time scales compatible, respectively, with ℓ meso and Δ t, it makes sense to discuss the derivation of macroscopic equations from the Boltzmann equation and to investigate their properties. To this aim, we integrate Eq. (3.1), multiplied by the collision invariants (3.9), over the velocity space, and obtain:
where the non-hydrodynamic fields P and q, called pressure tensor and heat flux, are defined as:
The pressure tensor can be written as \(\mathbf{P} = p\mathbf{I} + \boldsymbol{\sigma }\), where I is the identity matrix, the scalar p, defined as:
corresponds to the hydrostatic pressure and \(\boldsymbol{\sigma }\) is a symmetric tensor (it is also, typically, a traceless one, depending on the magnitude of the bulk viscosity, defined below). A visible feature of Eq. (3.18) is that the equations are not closed, because of the presence of the non-hydrodynamic fields \(\boldsymbol{\sigma }\) and q. As discussed by C. Cercignani in [8], [Eq. (3.18)] “constitute an empty scheme, since there are 5 equation for 13 quantities. In order to have useful equations, one must have some expressions for \(\boldsymbol{\sigma }\) and q in terms of ρ, u and e. Otherwise, one has to go back to the Boltzmann equation (3.1) and solve it; and once it has been done, everything is done, and Eq. (3.18) are useless!”. This corresponds to the well known problem of seeking a suitable closure to the macroscopic equations. This problem can be tackled either from the kinetic theory standpoint, i.e. by employing some model reduction or coarse-graining techniques [11], or from a purely macroscopic perspective, i.e. by employing macroscopic balance or phenomenological relations which disregard the underlying particle-like picture. In particular, the following set of constitutive equations, written in component notation,
with i, j = 1, 2, 3, yields the so-called Euler equations of inviscid hydrodynamics, which can be also derived from the Boltzmann equation by retaining only the Maxwellian contribution to the distribution function. The Navier-Stokes-Fourier (NSF) equations, instead, are obtained from the following constitutive equations:
where we used the repeated index summation convention and η, ζ, λ correspond to the transport coefficients called, respectively, shear viscosity, bulk viscosity (usually negligible) and thermal conductivity. The NSF equations deserve a special mention in fluid dynamics, because Eqs. (3.24) and (3.25) may be derived not only from the macroscopic principles of conservation of mass, momentum, and energy, but also, rigorously, from kinetic theory [8, 19, 20]. The latter derivation can be performed by using some perturbative schemes, such as those discussed in the next section, which refer to the hydrodynamic limit of the Boltzmann equation.
2.3 The Hilbert and the Chapman–Enskog Methods
We provide, here, an overview about the Hilbert and the Chapman–Enskog (CE) methods of solution of the Boltzmann equation in the hydrodynamic limit. The reader is referred to [2, 8] for an exhaustive treatment of the subject. To simplify the notation, we omit, hereafter in this section, the hat over the single-particle distribution referring to a rescaled Boltzmann equation of the form (3.7), introduced in Sect. 3.2.1.
In the Hilbert method, the normal solutions are expanded in powers of the Knudsen number \(\varepsilon\), i.e.:
which, substituted in Eq. (3.7), yields a sequence of integral equations
to be solved order by order. Here L denotes the linearization of collision integral Q in (3.1). From Eq. (3.27) it follows that f (0) corresponds to the local Maxwellian distribution [19]. The Fredholm alternative, applied to (3.28), results in [2]:
-
Solvability condition,
$$\displaystyle{ \int \left (\partial _{t} + \mathbf{v} \cdot \nabla _{\mathbf{r}}\right )f^{(0)}\boldsymbol{\psi }(\mathbf{v})d\mathbf{v} = 0\quad, }$$(3.30)which corresponds to the Euler equations described by Eqs. (3.22) and (3.23);
-
General solution \(f^{(1)} = f^{(1),1} + f^{(1),2}\), where f (1), 1 denotes the special solution to the linear integral equation (3.28) and f (1), 2 a yet undetermined linear combination of the summational invariants;
-
Solvability condition, when applied to Eq. (3.29), yields f (1), 2 which is obtained from solving the linear hyperbolic differential equations
$$\displaystyle{ \int \left (\partial _{t} + \mathbf{v} \cdot \nabla _{\mathbf{r}}\right )\left (f^{(1),1} + f^{(1),2}\right )\boldsymbol{\psi }(\mathbf{v})d\mathbf{v} = 0\quad. }$$(3.31)
Hilbert showed that this procedure can be applied up to an arbitrary order n, so that the function f (n) is determined from the solvability condition applied at the (n + 1)-th order [2]. Loosely speaking, the description provided by the Hilbert method is essentially in terms of the Euler equations, but it is supplemented by corrections which can by computed by solving linearized equations [8]. It is also worth remarking that the Hilbert method can not provide uniformly valid solutions, as it can be evinced by noticing the singular manner in which the Knudsen number enters the rescaled Boltzmann equation (3.7). Nevertheless, a truncated Hilbert expansion can reproduce, with arbitrary accuracy, the solution of the Boltzmann equation in a properly chosen region of time-space, provided that \(\varepsilon\) is sufficiently small.
The CE approach, developed by D. Enskog and S. Chapman, is based, instead, on an expansion of the time derivatives of the hydrodynamic variables, rather than seeking the time-space dependence of these functions, as in the Hilbert method. Also the CE method starts with the singularly perturbed Boltzmann equation (3.7), and with the expansion (3.26). Nevertheless, the procedure of evaluation of the functions f (i) is different, and reads as follows:
Equation (3.32) implies, as in the Hilbert method, that the function f (0) is the local Maxwellian. The operator \(\partial _{t}^{(0)}\) is defined from the expansion of the right hand side of the hydrodynamic equations,
Equation (3.34) correspond to the inviscid Euler equations, and \(\partial _{t}^{(0)}\) acts on various functions g(ρ, ρ u, e) according to the chain rule
whereas the time derivatives \(\partial _{t}^{(0)}\) of the hydrodynamic fields are expressed using the right hand side of (3.34). Finally, the method requires that the hydrodynamic variables, obtained by integrating over the velocity space the function \(f^{(0)} +\varepsilon f^{(1)}\), coincide with the parameters of the local Maxwellian f (0):
Thus, one finds that the first correction, f (1), adds the terms
to the time derivatives of the hydrodynamic fields. These novel terms yield the dissipative NSF hydrodynamics. However, higher-order corrections of the CE method, which result in hydrodynamic equations with higher derivatives (the so-called Burnett and super-Burnett hydrodynamics) are affected by severe difficulties, mainly related to the onset of instabilities of the solutions [5, 21, 22].
2.4 The Grad’s Moment Method
An alternative technique to solve the Boltzmann equation was proposed by H. Grad, and is known as the Grad’s moment method [12]. The essence of the method relies on the time scale separation hypothesis, introduced in Sect. 3.2.2:
-
During the fast evolution, which occurs on a time scale of the order of the mesoscopic time scale Δ t, a set of distinguished moments x, cf. Sect. 3.2.2, does not change significantly in comparison to the rest of higher-order “fast” moments of f, denoted as y.
-
Towards the end of the fast evolution, the values of the moments y become determined by the values of the distinguished moments x.
-
During a time interval of the order of τ macro , the dynamics of the distribution function is governed by the dynamics of the distinguished moments, while the rest of moments remain to be determined by the distinguished moments [2].
In the Grad’s moment method, the distribution function is expanded as:
where H k (v −u) are Hermite tensor polynomials, orthogonal with respect to a weight given by the Maxwellian distribution f LM, whereas the coefficients a k are known functions of the distinguished moments x. The fast moments y are assumed to be functions of x, i.e. y = y(x). By inserting Eq. (3.38) into the Boltzmann equation (3.1) and by using the orthogonality of the Hermite polynomials with respect to the Maxwellian distribution f LM, one can determine the time evolution of the set of distinguished moments x. According to Grad’s argument, this approximation can be refined by extending the set of distinguished moments x, as done, for instance in the Grad’s 13 moment approximation [12], which set the stage to the development of the theory of Extended Irreversible Thermodynamics [2, 11, 13].
2.5 The Invariant Manifold Theory
The Invariant Manifold method can be considered as a generalization of the theory of normal solutions, which is inherent in the Hilbert and CE expansions [2]. The method is based on a projector operator formalism [23–25], which confines the dynamics onto a manifold of slow motion and disregards the fluctuations of the fast variables. The same approach is also at the basis of Haken’s slaving principle and of the procedure of adiabatic elimination of fast variables in stochastic processes [26, 27]. The description of the time evolution of the system resembles the picture given in Sect. 3.2.4: from the initial condition, the system quickly approaches a small neighbourhood of the invariant manifold, and, from then onwards, it proceeds slowly along such manifold with a characteristic time scale of the order of τ macro . The main geometrical structures which characterize the Invariant Manifold theory are illustrated in Fig. 3.2.
The geometrical structures of the Invariant Manifold method: U is the space of distribution functions, J(w) is the vector field of the system under consideration, Ω is an ansatz manifold, X is the space of macroscopic variables (coordinates on the manifold), the map F maps points x ∈ X into the corresponding points w = F(x), T w is the tangent space to the manifold Ω at the point w, PJ(w) is the projection of the vector J(w) onto tangent space T w , d x∕dt describes the induced dynamics on X, Δ is the defect of invariance, and the affine subspace w +ker P is the plain of fast motions [2]
We summarize, below, the essential mathematical framework of the method. Let U be the phase space, and Ω ⊂ U an ansatz manifold, which corresponds to the current approximation to the invariant manifold to be sought. We denote by J(w) the vector field
which generates the dynamics in U. Let X be the linear space of the macroscopic variables x, which act as coordinates on the manifold Ω, described as the image of the map F: X → U. We remark that the choice of the space X of macroscopic variables is a crucial step of the method: the corrections of the current ansatz manifold correspond to the images of various maps F for a given X. Let us also denote by
the derivative of the map F with respect to the set of distinguished variables. We indicate by Π: U → X a regular map which fulfills the condition
with 1 the identity operator, and by D w Π the functional derivative of the map x = Π(w) computed at the point w. Thus, the time evolution of the distinguished variables x reads:
where \(d\mathbf{x}/\mathit{dt}\) is an element of the tangent space to X. Therefore, joining Eqs. (3.40) and (3.42), one obtains:
where the operator
projects J(f) onto T w , which denotes the tangent space to the manifold Ω at the point w. In particular, the projector P determines a decomposition of the motion near Ω: w +ker[P] is the plane of fast motion and T w the plane of slow motion. We call slow invariant manifolds those maps F which fulfill the condition (3.41) and solve the invariance equation:
which is a differential equation for the unknown map F. The solutions of Eq. (3.44) are “invariant” in the sense that the vector field J(F) is tangent to the manifold Ω = F(X) for each point w ∈ Ω. A crucial aspect of the method concerns the definition of the projector P. Gorban et al. introduced, in [2], the Thermodynamic Projector, which characterizes, in a thermodynamic sense, the plane of fast motion w +kerP: the physical entropy grows during the fast motion and the point w is the point of entropy maximum along the plane w +kerP.
The geometrical setting described above can be readily adapted to the Boltzmann equation theory. To this aim, one identifies w with the single-particle distribution function f, x = Π(f) denotes a set of distinguished fields which parameterize f and F becomes a “closure”, i.e. a distribution function parameterized by the variables x. Moreover, the vector field J(f) attains the form:
whereas D f Π reads:
with \(\boldsymbol{\psi }(\mathbf{v})\) defined in (3.10). Therefore, the Thermodynamic Projector P, which depends on f, attains, in this case, the structure:
The invariance equation (3.44) constrains the kinetic evolution of the distribution function to coincide with its “macroscopic” evolution, ruled by the projector P (3.45) and driven by the dynamics of the distinguished variables x. It should be noticed that the method does not require the smallness of the parameter \(\varepsilon\), hence it is not restricted to the strict hydrodynamic limit. A straightforward application of the formalism described above is obtained by considering an ansatz manifold, Ω LM , given by the locally five-dimensional manifold of local Maxwellians (3.2). We take, hence, the set M f of moments (3.11) as the coordinates x on this manifold. The manifold Ω LM is commonly referred to as the quasi-equilibrium manifold for the set of moments x, because f LM corresponds to the unique solution of the variational problem:
with \(H(f) =\int f\log fd\mathbf{v}\) denoting the celebrated H-function. We thus define the projector \(P_{f^{\mathit{LM}}}\) onto the tangent space \(T_{f^{\mathit{LM}}}\) as:
Returning to the hydrodynamic variables [ρ, u, T] via the transformations (3.12), one obtains [2]:
Equation (3.47) reveals that the quasi-equilibrium manifold is not an invariant manifold of the Boltzmann equation, because temperature and bulk velocity It is worth remarking that, strictly speaking, the statistics of the many-particle system is actually not driven towards the local Maxwellian distribution, or, if it accidentally starts in that state, it moves away from it, due to the flow term \(\left (\mathbf{v} \cdot \frac{\partial f} {\partial \mathbf{r}} f\right )\) in the Boltzmann equation. The effect of the latter term is, in fact, to smooth out the spatial inhomogeneities [17]. Nevertheless, for small Knudsen numbers, such term acts over time scales much larger than the collisions. Consequently, at all times, the instantaneous single-particle distribution function is very close to the local Maxwellian one, described by Eq. (3.2). The latter may, hence, be regarded as the standard reference distribution when relying on perturbation theories which are concerned with the hydrodynamic limit of the Boltzmann equation.
3 Linear Hydrodynamics from the Boltzmann Equation
Several solution techniques have been introduced in the literature to obtain approximate solutions of the Boltzmann equation. In particular, the CE method extends the hydrodynamics beyond the NSF approximation in such a way that the decay rate of the next order approximations (Burnett and super-Burnett) are polynomials of higher order in k [2, 21, 22]. In such an extension, relaxation rates may become completely unphysical (amplification instead of attenuation), as first shown by Bobylev [5] for a particular case of Maxwell molecules. Therefore, several regularization techniques have been proposed to restore the thermodynamic admissibility of the generalized hydrodynamic equations [28–30]. A promising route, in particular, is based on the notion of Invariant Manifold [2], introduced in Sect. 3.2.5. The method requires a neat separation between hydrodynamic and kinetic (time and length) scales, and postulates the existence of a stable Invariant Manifold in the space of distribution functions, parameterized by the values of the hydrodynamic fields. Following the approach traced in [31], we will employ, here, the Invariant Manifold technique to determine the hydrodynamic modes and the transport coefficients beyond the standard hydrodynamic regime. In particular, we expect to recover the asymptotic form of the dynamic structure factor in the free-particle regime and to shed light on the properties of the hydrodynamic equations in the intermediate regime of finite Knudsen numbers.
This section is structured as follows.
We will review, in Sect. 3.3.1, the eigenvalue problem associated with the linearized Boltzmann equation. In Sect. 3.3.2, we will derive the invariance equation for the Boltzmann equation equipped with an arbitrary linearized collision operator. In Sect. 3.3.3 we will introduce a suitable coordinate system which allows one to highlight the symmetries of the solutions of the invariance equation. In Sect. 3.3.4 we will, then, solve the invariance equation for the linearized BGK model [32]. In Sect. 3.3.5 we will investigate the properties of the solution of the invariance equation for a gas of Maxwell molecules. We will, hence, comment on the structure of the obtained hydrodynamic modes and cast the generalized transport coefficients in the Green-Kubo formalism [33]. We will, finally, determine the spectrum of the density fluctuations and discuss some relevant features of the resulting short wavelength hydrodynamics.
3.1 Eigenfrequencies of the Boltzmann Equation
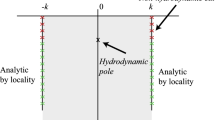
In this section we consider the eigenvalue problem for the linearized Boltzmann equation. Namely, for a inhomogeneous gas, the eigenvalues correspond to k-dependent frequencies (i.e. inverse of characteristic collision rates). The connection between the fluctuations of the macroscopic variables and the underlying characteristic kinetic rates is a central problem in statistical mechanics [34, 35] which still demands a conclusive understanding. In order to appreciate the problem, we recall that in the NSF approximations, the hydrodynamic modes are quadratic in the wave vector [36] and are unbounded. On the other hand, Boltzmann’s collision term features equilibration with finite characteristic rates. Hence, such “finite collision frequency” is at variance with the arbitrary decay rates in the NSF approximation: intuitively, the hydrodynamic modes at large k cannot relax faster than the collision frequencies. In his seminal work [37] on the eigenfrequencies of the Boltzmann equation, Resibois provided an explicit connection between the generalized frequencies of the linearized Boltzmann equation and the decay rate of the hydrodynamic fluctuations. He tackled the problem by solving, perturbatively, the eigenvalue problem associated to the Boltzmann equation and to the NSF equations of hydrodynamics.
Let us rewrite the Boltzmann equation (3.1):
We introduce the dimensionless peculiar velocity \(\mathbf{c} = (\mathbf{v} -\mathbf{u}_{0})/v_{T}\) and the equilibrium values of hydrodynamic fields: equilibrium particle number n 0, equilibrium mean velocity u 0 = 0, and equilibrium temperature T 0. The global Maxwellian reads: \(f^{\text{GM}} = (n_{0}/v_{T}^{3})f_{0}(c)\) where \(f_{0}(c) =\pi ^{-3/2}e^{-c^{2} }\) is a Gaussian in the velocity space (c = | c | ). We linearize (3.48) by considering only small disturbances from the global equilibrium. Moreover, we write the nonequilibrium distribution function (cf. also Table 3.3) as:
where f LM denotes the local Maxwellian to be made precise in Sect. 3.3.2, and δ f the deviation from local equilibrium. An alternative notation is introduced via \(\delta f = f^{\text{GM}}\delta \varphi\). We also consider a reference frame moving with the flow velocity and linearize the collision operator around global equilibrium. When passing over to Fourier space, we seek solutions of the form:
where ω is a complex-valued frequency and k is a real-valued wavevector. Thus, the Boltzmann equation (3.48) reduces to:
where we made use of the fact that \(\mathit{Lf }^{\text{LM}} = 0\). In the sequel of this section, we will investigate the spectrum of the operator \(\boldsymbol{\Lambda } \equiv \hat{ L} - i\mathbf{k} \cdot \mathbf{c}\), which determines the time evolution of the single-particle distribution function [38]. This is readily seen by inspection of the inverse Laplace transform:
where the closed path encircles the poles of the function inside the integral. According to the Spectral Theorem, these poles correspond to the spectrum of \(\boldsymbol{\Lambda }\). The flow term \(-i\mathbf{k} \cdot \mathbf{c}f\) is treated, here, as a small perturbation [17] (this amounts to considering small gradients in the real space). Equation (3.50) can be, hence, written in the form:
The investigation of the spectrum of the operator Λ, in Eq. (3.52), is a formidable mathematical problem, which can be tackled by considering, first, the hydrodynamic limit k → 0. In this limit, Eq. (3.52) reads:
The linear operator \(\hat{L}\) can be shown to be self-adjoint with respect to a suitably defined scalar product [1], hence the corresponding eigenfunctions are (or can be made) orthogonal and constitute a complete set. In particular, a subset of them is related to the fivefold degenerate zero eigenvalue. These functions correspond to the collision invariants \(f^{\text{GM}}\mathbf{X}^{(0)}\), with X (0) denoting a set of lower-order Sonine (or associated Laguerre) polynomials:
One, also, typically assumes that the eigenvalues of \(\hat{L}\) other than 0 have no accumulation point at the origin. This assumption is always implicit in any calculation of transport coefficients based on kinetic theory. Physically, it corresponds to invoking a separation between the kinetic time scale \(\lambda _{i}^{-1}\), and the hydrodynamic time scale \((v_{t}k)^{-1}\), as discussed in Sect. 3.2.2. For finite values of k, there will be a set of eigenvalues of Λ, denoted by ω α , with α = 1, …, 5, which, in the k → 0 limit, reduce to the aforementioned degenerate zero eigenvalue. Hence, we shortly review, here, the results of a perturbative method, outlined in [37], which allows one to determine the dependence of the set ω α from k. Denoting by Ψ α (k) the eigenfunctions corresponding to ω α (k), the yet unknown eigenfunctions and eigenvalues can be expanded in powers of the wavevector k:
where \(\varPsi _{\alpha }^{(0)}\) are linear combinations of the collision invariants (3.54), whose detailed expression is not relevant here (cf. [39] for details). The use of a Rayleigh-Schödinger perturbation theory leads to the following polynomial expression for the set {ω α }:
where c 0 denotes the speed of sound.
On the other hand, in Fourier space, the linearized NSF equations read:
The condition of a non-trivial solvability of the linear system (3.57) with respect to the variables \([n_{k}(\omega ),\mathbf{u}_{k}(\omega ),T_{k}(\omega )]\), yields the dispersion relation ω(k), i.e. the normal mode frequencies of the system. It can be shown, in particular, that the real part of these modes is quadratic in the wavevector [2, 37, 39]:
which can be regarded, in fact, as the hallmark of the NSF approximation. By requiring the equivalence of the hydrodynamic frequencies with the set of kinetic frequencies ω α , given in Eq. (3.56), leads to approximate expressions for the transport coefficients, which can be shown to be equivalent to the reduced expressions determined by many-body autocorrelation functions [37]. As also pointed out in [17], the results obtained by Resibois, based on the correspondence between hydrodynamic modes and kinetic frequencies, reveals that, in the limit of long wavelengths, the possible modes of motion of the gas correspond to rather ordered motions, such as a sound wave propagation. These modes are referred to, in the literature, as collective modes, because they involve the coordinate action of a huge number of particles. The onset of such an ordered motion as a result of the underlying chaoticity of the individual motion of the particles is a striking feature of statistical mechanics. The reason of this can be traced back to the effect of the collisions, which drive very quickly the system towards the local equilibrium state, that is a highly organized one. From then onwards, the flow term produces slow variations in space and time of this basic state, which reduce the local gradients of the hydrodynamic fields. In the sequel of this section, we will employ the Invariant Manifold theory to obtain a generalization of the pioneering approach developed by Resibois.
3.2 The Invariant Manifold Technique
Following the notation of Sect. 3.2, we denote by U and x(r, t), respectively, the space of single-particle distribution functions and a set of distinguished moments of the latter. We then introduce the locally finite-dimensional manifold \(\varOmega \subset \textit{ U}\) as the set of functions \(f(\mathbf{x}(\mathbf{r},t),\mathbf{c})\) whose dependence on the space-time variables (r, t) is parameterized through x(r, t). In this section, we will identify the distinguished moments \(\mathbf{x}(\mathbf{r},t)\) with the hydrodynamic fields. Moreover, P is the Thermodynamic Projection operator which, as discussed in Sect. 3.2.5, allows to decompose the dynamics into a fast motion on the affine subspace f + ker[P] and a slow motion, which occurs along the tangent space T f . The use of the Thermodynamic Projector guarantees the persistence of dissipation: it can be shown [2] that the entropy production rate is unaltered when the dynamic is projected along the manifold of slow motion.
In order to derive exact hydrodynamic equations from the general eigenvalue problem (3.50), we proceed as follows:
-
1.
We determine the invariant manifold, i.e. the distribution function solving the invariance equation
$$\displaystyle{ (\mathbf{1} - P)\varLambda \varDelta f = 0\quad, }$$(3.58)where \(\bigtriangleup f \equiv f - f^{\text{GM}}\) (cf. also Table 3.3).
-
2.
We derive the equations of linear hydrodynamics by integrating the kinetic equation (3.50), with f given by the solution of Eq. (3.58). By construction, the hydrodynamic modes coincide, then, with the set ω α of eigenfrequencies of the Boltzmann equation, which vanish in the limit k → 0.
We also denote by x k the Fourier components of the dimensionless hydrodynamic fluctuations \([\tilde{n},\tilde{\mathbf{u}},\tilde{T}]\): \(\tilde{n} \equiv (n - n_{0})/n_{0} =\) (particle number perturbation), \(\tilde{\mathbf{u}} \equiv \mathbf{u}/v_{T} =\) (velocity perturbation) and \(\tilde{T} \equiv (T - T_{0})/T_{0}\) (temperature perturbation). Further, we split the mean velocity \(\tilde{\mathbf{u}}\) uniquely as \(\tilde{\mathbf{u}} = u^{\|}\mathbf{e}_{\|} + u^{\perp }\mathbf{e}_{\perp }\), where the unit vector \(\mathbf{e}_{\|}\) is parallel to k, and \(\mathbf{e}_{\perp }\) orthonormal to \(\mathbf{e}_{\|}\), i.e., \(\mathbf{e}_{\perp }\) lies in the plane perpendicular to k. Due to isotropy, \(u^{\perp }\) alone fully represents the twice degenerated (shear) dynamics. By linearizing around the global equilibrium, we write the local Maxwellian contribution to f in (3.49) as \(f^{\text{LM}} = f^{\text{GM}}(1 +\varphi _{0})\) where \(\varphi _{0}\) takes a simple form, \(\varphi _{0} = \mathbf{X}^{(0)} \cdot \mathbf{x}\) (linear quasi equilibrium manifold), where \(\mathbf{X}^{(0)}(\mathbf{c})\) was defined in Eq. (3.54). It is conveniently considered as four-dimensional vector using the four-dimensional version \(\mathbf{x}_{k} = [\tilde{n}_{k},u^{\|},\tilde{T}_{k},u^{\perp }]\), and is then given by (3.68). It proves convenient to introduce a vector of velocity polynomials, ξ(c), which is similar to X 0 and defined below in Eq. (3.69), such that:
Hence, the fields x k are obtained as \(\langle \boldsymbol{\xi }(\mathbf{c})\rangle _{f^{\mathit{LM}}} = \mathbf{x}_{k}\), where averages are defined, here, as:
We introduce yet unknown fields \(\delta \mathbf{X}(\mathbf{c},\mathbf{k})\) which characterize the part δ f of the distribution function. As long as deviations from the local Maxwellian are small, we seek a nonequilibrium manifold which is also linear in the hydrodynamic fields x themselves. Therefore, we set:
The “eigen”-closure (3.60), which formally and very generally addresses the fact that we wish to not include other than hydrodynamic variables, implies a closure between moments of the distribution function, to be worked out in detail below. By using the above form (3.60) for \(\delta f = f^{\text{GM}}\delta \varphi\), with \(\hat{L}\delta f = f^{\text{GM}}L[\delta \mathbf{X}] \cdot \mathbf{x}_{k}\), and the canonical abbreviations \(\bigtriangleup \mathbf{X} \equiv \mathbf{X}^{0}(\mathbf{c}) +\delta \mathbf{X}(\mathbf{c},\mathbf{k})\), Eq. (3.52)reads:
The microscopic projected dynamics is obtained through the projector P, which, when acting upon the vector field \(J(f) =\varLambda \varDelta f\), gives:
where \(D_{\mathbf{x}_{k}}\varDelta f \equiv \partial \varDelta f/\partial \mathbf{x}_{k}\) and the quantity inside the integral in (3.62) represents the time evolution equations for the moments x k . These are readily obtained by integration of the weighted (3.52) as
Due to the eigen-closure (3.60), cf. also Table 3.3, one finds \(D_{\mathbf{x}_{k}}\varDelta f = f^{\text{GM}}\bigtriangleup \mathbf{X}\), whereas (3.63) is linear in x k and can be written as:
Equation (3.64) defines the matrix M of hydrodynamic coefficients, whose explicit structure will be made clear in Eq. (3.74). Using (3.64), Eq. (3.62) can be cast in the form:
In the derivation of (3.65), one needs to take into account the constraints \(\langle \boldsymbol{\xi }(\mathbf{c})\rangle _{\delta f}\,=\,\mathbf{0}\) (because the fields \(\mathbf{x}_{k}\) are defined through the local Maxwellian part of the distribution function only) and \(\langle \boldsymbol{\xi }(\mathbf{c})\rangle _{\hat{L}\delta f} = \mathbf{0}\) (conservation laws). The dependence of the matrix elements of M upon moments of δ f is explicitly given in Table 3.4. Combining (3.61) and (3.65), and requiring that the result holds for any x k (invariance condition), we obtain a closed, singular integral equation (the invariance equation) for complex-valued \(\delta \mathbf{X}\),
Notice that \(\delta \mathbf{X} =\varDelta \mathbf{X} -\mathbf{X}^{(0)}\) vanishes for k = 0, which implies that, in the limit k → 0, the invariant manifold is given by the set of local Maxwellians f LM. The implicit equation (3.66) for δ X (or Δ X, as X (0) is known) is identical with the eigen-closure (3.60), and is our main and practically useful result.
3.3 Coordinate Representation and Symmetries
In order to calculate the averages occurring in Sect. 3.3.2, we switch to spherical coordinates. For each (at present arbitrary) wave vector \(\mathbf{k} = k\mathbf{e}_{\|}\), we choose the coordinate system in such a way that its (vertical) z-direction aligns with \(\mathbf{e}_{\|}\) and that its x–direction aligns with \(\mathbf{e}_{\perp }\). The velocity vector we had been decomposed earlier as \(\tilde{\mathbf{u}} = u^{\|}\mathbf{e}_{\|} + u^{\perp }\mathbf{e}_{\perp }\). We can then express c, over which we are going to perform all integrals, in terms of its norm c, a vertical variable z and plane vector e ϕ (azimuthal angle \(\mathbf{e}_{\phi } \cdot \mathbf{e}_{\perp } =\cos \phi\); the plane contains e ⊥ ) for the present purpose as:
as shown in Fig. 3.3.
Schematic drawing introducing a orthonormal frame \(\mathbf{e}_{\|}\), e ⊥ , and \(\mathbf{e}_{\perp }\times \mathbf{e}_{\|}\) which is defined by the wavevector \(\mathbf{k}\,\|\,\mathbf{e}_{\|}\) and the heat flux q (not shown), which lies in the \(\mathbf{e}_{\|}\)–\(\mathbf{e}_{\perp }\)–plane. Shown is the velocity vector c (3.67) relative to this frame (characterized by length c, coordinate z, and angle ϕ) and its various components. The integration over \(d\mathbf{c} = c^{2}\mathit{dcdzd}\phi\) is done in spherical coordinates with respect to the local orthonormal basis
The local Maxwellian, linearized around global equilibrium, takes the form: \(f^{\text{LM}}/f^{\text{GM}} = 1 +\varphi _{0} = 1 + \mathbf{X}^{(0)} \cdot \mathbf{x}_{k}\), where the four-dimensional \(\mathbf{X}^{(0)}\), and the related vector \(\boldsymbol{\xi }\), employing four-dimensional \(\mathbf{x}_{k} = [\tilde{n}_{k},u^{\|},\tilde{T}_{k},u^{\perp }]\), are given by the expressions
Here, we introduced, for later use, the abbreviations
such that \(i\mathbf{k} \cdot \mathbf{c} = \mathit{ik}c_{\|}\). We can then rewrite (3.67) as \(\mathbf{c} = c_{\phi }\mathbf{e}_{\phi } + c_{\|}\mathbf{e}_{\|}\) with \(c_{\|} = \mathit{cz}\) and \(c_{\phi } = c\sqrt{1 - z^{2}}\). The latter two components, contrasted by \(c_{\perp }\) (and e ϕ ), do not depend on the azimuthal angle. We further introduced yet unknown fields \(\delta \mathbf{X}(\mathbf{c},\mathbf{k})\) which characterize the nonequilibrium part of the distribution function, \(\delta \varphi =\delta f/f^{\text{GM}}\).
By analogy with the structure of the local Maxwellian, we postulate that, close to equilibrium, \(\delta \varphi\) depends linearly on the hydrodynamic fields x k themselves. Equation (3.60) can, hence, be cast in the form:
The functions δ X 1, 2, 3, which are associated to the longitudinal fields, inherit the full rotational symmetry of the corresponding Maxwellian components, i.e. \(\delta X_{1,2,3} =\delta X_{1,2,3}(c,z)\), whereas δ X 4 factorizes as \(\delta X_{4}(c,z,\phi ) = 2\delta Y _{4}(c,z)\sum _{m=1}^{\infty }y_{m}\cos m\phi\). In this context it is an important technical aspect of our derivation to work with a suitable orthogonal set of basis functions to represent δ f uniquely. The matrix M in (3.65) contains the non-hydrodynamic fields: the heat flux \(\mathbf{q}_{k} \equiv \langle \mathbf{c}(c^{2} -\frac{5} {2})\rangle _{f}\) and the stress tensor

, where

denotes the symmetric traceless part of a tensor s [40],

, where I is the identity matrix. Using (3.60) and the above mentioned angular dependence of the δ X functions (the only term in δ X 4 playing a role in our calculations is the first order term cosϕ, with y 1 = 1), constraints, such as the required decoupling between longitudinal and transversal dynamics of the hydrodynamic fields, are automatically dealt with correctly when performing integrals over ϕ.
More explicitly, the stress tensor and heat flux uniquely decompose as follows:

with the moments \(\sigma ^{\|} = (\sigma _{1}^{\|},\sigma _{2}^{\|},\sigma _{3}^{\|}) \cdot (\tilde{n}_{k},u^{\|},\tilde{T}_{k})\) and \(\sigma ^{\perp } =\sigma _{4}u^{\perp }\), and similarly for \(\mathbf{q}_{k}\) (see Row 2 of Table 3.4).
The prefactors arise from the identities

and

.
We note in passing that, while the stress tensor has, in general, three different eigenvalues, in the present symmetry adapted coordinate system it exhibits a vanishing first normal stress difference. Since the integral kernels of all moments in (3.72) do not depend on the azimuthal angle, these are actually two-dimensional integrals over c ∈ [0, ∞] and z ∈ [−1, 1], each weighted by a component of \(2\pi c^{2}f^{\text{GM}}\delta \mathbf{X}\).
Stress tensor and heat flux can yet be written in an alternative form, defined by Row 3 of Table 3.4, in terms of the functions A–Z, which correspond to moments of the nonequilibrium distribution function and are related to the generalized transport coefficients, see [4, 31, 36] and below.
Due to fundamental symmetry considerations, the hereby introduced generalized transport coefficients A–Z are real-valued. To show this, we use the functions A–Z to split M into parts as \(\mathbf{M} = \mathrm{Re}(\mathbf{M}) - i\,\mathrm{Im}(\mathbf{M})\),
with abbreviations \(\tilde{B} \equiv \frac{1} {2} - k^{2}B\), \(\tilde{C} \equiv \frac{1} {2} - k^{2}C\), and \(\tilde{Z} \equiv \frac{2} {3}(1 - k^{2}Z)\). The checkerboard structure of the matrix M (3.74) is particularly useful for studying properties of the hydrodynamic equations (3.65), such as hyperbolicity and stability [21, 22], once the functions A–Z are explicitly evaluated. Moreover, we remind the reader that we use orthogonal basis functions (irreducible moments, cf. Table 3.4) to solve (3.66). In order to show how the above functions enter the definition of the M matrix, we first notice that its elements are—a priori—complex valued. We wish, then, to make use of the fact that all integrals over z vanish for odd integrands. To this end we introduce abbreviations ⊕ (⊖) for a real-valued quantity which is even (odd) with respect to the transformation z → −z. One notices \(\mathbf{X}^{(0)} = (\oplus,\ominus,\oplus,\oplus )\), and we recall that A–Z are integrals over either even or odd functions in z, times a component of δ X (see Table 3.4). Let us prove the consistency of the specified symmetry of M and the invariance condition: start by assuming A–Z to be real-valued functions. Then M μ ν = ⊕ if μ +ν is even, and M μ ν = i⊕ otherwise. This implies δ X 1 = ⊕ + i⊖, \(\delta X_{2} = \ominus + i\oplus \), \(\delta X_{3} = \oplus + i\ominus \), and δ X 4 = ⊕ + i⊖, i.e., different symmetry properties for real and imaginary parts. With these “symmetry” expressions for X (0), δ X, and M at hand, and by noticing that symmetry properties for δ X take over to \(\hat{L}(\delta \mathbf{X})\) because the ψ r, l are (i) symmetric (antisymmetric) in z for even (odd) l and (ii) eigenfunctions of \(\hat{L}\), we can insert into the right hand side of the equation, \(\hat{L}(\delta \mathbf{X}) = (\mathbf{X}^{(0)} +\delta \mathbf{X}) \cdot (\mathbf{M} + i \ominus \mathbf{I})\), which is identical with the invariance equation (3.66). There are only two cases to consider, because M has a checkerboard structure, i.e., only two types of columns: Columns μ = 1 and μ = 3: δ X μ = ⊕ + i⊖ because M 1−3, 4 = 0; Columns μ ∈ { 2, 4}: δ X μ = ⊕ + i⊖ if M μ, 1−3 = 0 (which is the case for column 4) and ⊖ +i⊕ if M μ, 4 = 0 (which is the case for column 2). These observations complete the proof.
3.4 The BGK Kinetic Model
The solution of the invariance equation (3.66) can be obtained in some simple cases amenable to an analytic or numerical treatment. In this section we focus on the linearized version of the BGK kinetic model, which remains popular in applications [8] and is characterized by a single collision frequency. The invariance equation for this model is readily obtained from Eq. (3.66) by using \(\hat{L}(\delta \mathbf{X}) = -\delta \mathbf{X}\), which, hence, yields:
Notice that δ X vanishes for k = 0, and that (3.75) is supplemented with the basic constraint \(\langle \boldsymbol{\xi }\rangle _{\delta f} = 0\), which, however, is automatically dealt with if we only evaluate anisotropic (irreducible) moments with δ f, such as those listed in Table 3.4.
The non-perturbative derivation is made possible with an optimal combination of analytical and numerical approaches to solve the invariance equation. The result for the hydrodynamic modes is demonstrated in Fig. 3.4. It is clear from Fig. 3.4 that the relaxation of none of the hydrodynamic modes is faster than ω = −1 which is the collision frequency in the units adopted in this section. Thus, the result for the exact hydrodynamics indeed corresponds to the following intuitive picture: the hydrodynamic modes, at large k, cannot relax faster than the (single) collision frequency itself.
Exact hydrodynamic modes ω of the Boltzmann-BGK kinetic equation as a function of wave number k (two complex-conjugated acoustic modes ω ac, twice degenerated shear mode ω shear and thermal diffusion mode ω diff). The non-positive decay rates Re(ω) attain the limit of collision frequency ( − 1) in the k ≫ 1 regime
Sample distribution function \(f(\mathbf{c},\mathbf{k})\) at k = 1, fully characterized by the four quantities δ X 1, 2, 3(c, z) and δ Y 4(c, z). Shown here are both their real (left) and imaginary parts (right column). In order to improve contrast, we actually plot \(\ln \vert 1 + f^{\mathrm{GM}}\delta X_{\mu }\vert \) multiplied by the sign of δ X μ . Same color code for all plots, ranging from − 0. 2 (red) to + 0. 2 (blue)
We iteratively calculated (i) δ X directly from (3.75) for each k in terms of M, (ii) subsequently calculate moments from δ X by either symbolical or numerical integration (both approaches produce same results within machine precision, we found simple numerical integration on a regular 500 × 100 grid in c, z-space with grid spacing 0. 01 on both axes sufficient to reproduce analytical results). Importantly, the fix point of the iteration (i)–(ii)–(i)-.. is unique for each k, i.e., does not depend on the initial values for moments A–Z.
In addition, two other computational strategies were implemented: First, we used continuation of functions A–Z from their values at k = 0 to solve (3.75) with an incremental increase of k, where the solution at k was used as the initial guess for k +dk. Second, we used also a continuation “backwards” in which the solution at some k (obtained by convergent iterations with a random initial condition) was used as the initial guess for a solution at k −dk. Both these strategies returned the same values of functions A–Z as computed by iterations from arbitrary initial condition.
Moments A–Z vs. wave number k obtained with the solution of (3.75)
The solution δ X allows one to calculate the whole distribution function f via (3.60) as illustrated by Fig. 3.5. For the resulting moments A–Z, for a wide range of k-values, see Fig. 3.6. With the result for the functions A–Z at hand, the extended hydrodynamic equations are closed.
Let us briefly discuss the pertinent properties of this system. First, the generalized transport coefficients are given by the nontrivial eigenvalues of \(-k^{-2}\mathrm{Re}(\mathbf{M})\): λ 2 = −A (elongation viscosity), \(\lambda _{3} = -\frac{2} {3}Y\) (thermal diffusivity), and λ 4 = −D (shear viscosity). All these generalized transport coefficients are non-negative (see Fig. 3.6). Second, computing the eigenvalues of matrix M, we obtain the dispersion relation ω(k) of the corresponding hydrodynamic modes already presented in Fig. 3.4. Third, a suitable transform of the hydrodynamic fields, \(\mathbf{z}_{\mathbf{k}} = \mathbf{T} \cdot \mathbf{x}_{\mathbf{k}}\), where T is a real-valued matrix, can be established such that the transformed hydrodynamic equations read \(\partial _{t}\mathbf{z}_{\mathbf{k}} = \mathbf{M}^{{\prime}}\cdot \mathbf{z}_{\mathbf{k}}\), where \(\mathbf{M}^{{\prime}} = \mathbf{T} \cdot \mathbf{M} \cdot \mathbf{T}^{-1}\) is manifestly hyperbolic and stable; \(\mathrm{Im}(\mathbf{M}^{{\prime}})\) is symmetric, \(\mathrm{Re}(\mathbf{M}^{{\prime}})\) is symmetric and non-positive semidefinite. The corresponding transformation matrix T can be easily read off the results obtained in [21] for Grad’s systems since the structure of the matrix M (3.74) is identical to the one studied in [21, 22]. It can be explicitly verified that matrix T with the functions A–Z derived herein is real-valued and thus render the transformed hydrodynamic equations manifestly hyperbolic and stable.
We note that this result—hyperbolicity of exact hydrodynamic equations—strongly supports a recent suggestion by Bobylev to consider a hyperbolic regularization of the Burnett approximation [30]. Similarly, using the hyperbolicity, an H-theorem is elementary proven as in [22, 30]. Finally, using the accurate data for functions A–Z, we can write analytic approximations for the corresponding hydrodynamic equations in such a way that hyperbolicity and stability is not destroyed in such an approximation [21].
In conclusion, we derived exact hydrodynamic equations from the linearized Boltzmann-BGK equation [36]. The main novelty is the numerical non-perturbative procedure to solve the invariance equation. In turn, the highly efficient approach is made possible by choosing a convenient coordinate system and establishing symmetries of the invariance equation. The invariant manifold in the space of distribution functions is thereby completely characterized, that is, not only equations of hydrodynamics are obtained but also the corresponding distribution function is made available.
The predicted smoothness and extendibility of the spectrum to all k is expected to have some implications for micro-resonators where the quality of the resonator becomes better at very high frequency: that is compatible with our prediction. The damping of all the modes saturates while the imaginary part of the acoustic modes frequency grows. The pertinent data can be used, in particular, as a much needed benchmark for computation-oriented kinetic theories such as lattice Boltzmann models, as well as for constructing novel models [41].
It is worth remarking that the above derivation of hydrodynamics is done under the standard assumption of local equilibrium, however the assumption itself is open to further study [42].
3.5 The Maxwell Molecules Gas
In this section we investigate another kinetic model for which an exact solution of the invariance equation (3.66) can be obtained: the Maxwell molecules gas, i.e. a gas constituted by particles repelling each other with a force proportional to the inverse fifth power of the distance. Wang Chang and Uhlenbeck [43] provided an analytical solution to the eigenvalue problem for the linearized collision operator L pertaining to this case. For the Maxwell molecules gas, the collision probability per unit time, g σ(g, θ), is independent of the magnitude of the relative velocity g. Since the collision operator is spherically symmetric in the velocity space, the dependence of the eigenfunctions upon the direction of c is expected to be spherically harmonic. Indeed, the eigenvalue problem admits the following solutions:
where \(S_{l+1/2}^{(r)}(x)\) are Sonine polynomials, and P l (z) are Legendre polynomials which act on the azimuthal component of the peculiar velocity c. The Legendre and Sonine polynomials are each orthogonal sets, i.e.:
Accordingly, the ψ r, l are normalized to unity with the weight factor f 0(c):
The corresponding eigenvalues for Maxwell molecules are given by:
The collision operator \(\hat{L}\) is negative semidefinite, i.e. all eigenvalues are negative except λ 0, 0, λ 0, 1, and λ 1, 0 which are zero and correspond to the elementary collision invariants. As shown by Wang Chang and Uhlenbeck [43], the spectrum of \(\hat{L}\) for Maxwell molecules is discrete and, for r → ∞, the eigenvalues λ r, l tend to −∞. Chang and Uhlenbeck’s investigation on the dispersion of sound in a Maxwell molecules gas was based upon writing the deviation from the global equilibrium as:
so that (3.52) reduces to an algebraic equation for the coefficients a (r, l):
with:
The hydrodynamic modes for the Maxwell-molecules gas are determined, as seen in Sect. 3.3.1, from the condition of non-trivial solvability of the linear system (3.79). This approach allows to solve the eigenvalue problem (3.50) for an arbitrary number of modes, which is made possible just by tuning the number of eigenfunctions taken into account in (3.78).
Another approach [31] is based, instead, on the expansion of the functions \([\mathbf{X}^{(0)},\delta \mathbf{X}]\) in terms of the orthonormal basis \(\psi _{r,l} =\psi _{r,l}(c,z)\):
The equilibrium coefficients \(a_{\mu }^{(0)}\) are known, and can be determined, by taking advantage of the orthogonality of the eigenfunctions, as follows:
Inserting (3.80) and (3.81) into the invariance equation (3.66), we obtain the following nonlinear set of algebraic equations for the unknown coefficients\(a_{\mu }^{(r,l)}(k)\):
with, \(\forall _{\mu,r,l}\), \(b_{\mu }^{(r,l)} = \left (a_{\mu }^{(0)(r,l)} + a_{\mu }^{(r,l)}\right )\), and
For any order of expansion, the solutions of (3.83) characterize an invariant manifold in the phase space. The matrix elements \(\mathfrak{L}_{(r,l,r^{{\prime}},l^{{\prime}})}\) can be easily evaluated in few kinetic models, such as the linearized BGK model [36] and Maxwell molecules [31]. In particular, the Maxwell molecules case is recovered by setting:
whereas the linearized BGK model is recovered by setting all nonvanishing eigenvalues equal to λ = −1. The calculation of the coefficients a (r, l), via the reformulated invariance equation (3.83), is easily achieved. Through these coefficients, the invariant manifold is fully characterized: the distribution function is determined and the corresponding matrix M of linear hydrodynamics as well as moments A–Z, are made accessible.
Solving the invariance equation (3.66) and thus obtaining the distribution function via the coefficients a (r, l), cf. Fig. 3.7, required minor computational effort [31]. The components A–Z of M are related to the coefficients a (r, l) by theexpressions:
In the regime of large Knudsen numbers the coefficients a (r, l) may be used to, e.g., directly calculate phoretic accelerations onto moving and rotating convex particles [44], while in the opposite limit of small k we recover the classical hydrodynamic equations.
All contributions \(\delta X_{1-4}(\mathbf{c},\mathbf{k})\) vs. c (horizontal, c = | c | ) and z ∈ [−1, 1] (vertical axis, z is the cosine of the angle between k and peculiar velocity c) to the nonequilibrium distribution function \(\delta f = f^{\text{GM}}\delta \mathbf{X} \cdot \mathbf{x}_{k}\) (3.60) at k = 1, obtained with the fourth order expansion, N = 4. Shown here are both their real (top) and imaginary parts (bottom row)
3.6 Hydrodynamic Modes and Transport Coefficients
With M at hand, the hydrodynamic modes are obtained from the condition of non-trivial solvability of the linear system (3.64). Figure 3.8 illustrates the damping rates of the fluctuations given by the real part of the hydrodynamic modes, obtained by truncating the series (3.80) and (3.81) at the fourth order. The picture does not qualitatively change upon further increase of the order N. For any given order of expansion, the modes extend smoothly over all the wavevector domain and, for large k, they attain an asymptotic value, which is clearly in agreement with the asymptotic behavior of the hydrodynamic modes obtained for the linearized BGK model discussed in Sect. 3.3.4.
The generalized transport coefficients are obtained by the nontrivial eigenvalues of − k 2Re(M): λ 2 = −A (elongation viscosity), \(\lambda _{3} = -\frac{2} {3}Y\) (thermal diffusivity) and λ 4 = −D (shear viscosity). In the limit k → 0, one recovers the hydrodynamic limit. This limit had been worked out in detail in [21, 22]. In that limit, the generalized transport coefficients A–Z become the classical transport coefficients. As can be seen from Fig. 3.9, and by also inspecting the invariance equation (3.66), in the limit of small k, all moments A–Z approach constant values in the limit of small k. These constants are compatible with those obtained in [21, 22] for the case of Navier-Stokes equations and the Burnett correction [45].
Moments A–Z of the distribution function (see Table 3.4 and Eq. (3.84)) vs. wave number k obtained with the solution of (3.66). Non-triangles (black symbols): Moments entering only the longitudinal component of hydrodynamic equations. Triangles (blue symbols): Moments entering the transverse component of hydrodynamic equations
The stress tensor and heat flux are given in terms of these moments in Table 3.4. For example, the parallel component of the stress tensor related to density fluctuations, \(\sigma _{1}^{\|}\), cf. Eq. (3.72), is given by − k 2 B, so that it approaches − k 2 for small k, as it results from the Burnett approximation [2].
Moreover, under suitable assumptions, one may also cast the matrix of hydrodynamic coefficients M in the structure of a Green-Kubo formula [33]. We summarize below the main steps of the proof given in [46], to which we refer the reader for an exhaustive derivation. From Eqs. (3.64) and (3.66), the time evolution of the hydrodynamic fields can be formally written as:
where we skipped, for brevity, the spatial dependence of the fields. In Eq. (3.85) the time τ is of the order of τ macro , which denotes a characteristic time scale related to the evolution of the hydrodynamic fields. As discussed in Sect. 3.2.2, the presence of a definite time scale separation, in a particle system, entails that \(\tau _{\mathit{macro}} \gg \tau _{\mathit{mf }}\), where τ mf denotes a kinetic time scale (i.e. the mean time between collisions). Hence, by invoking the Bogoliubov hypothesis of time scale separation, the time τ becomes large with respect to the characteristic time scale of the dynamics of the distribution function (r.h.s. of the second equality in (3.85)). Thus, from Eq. (3.85), we can write the matrix of hydrodynamic coefficients in the form:
Next, we use the operator identity:
and make the following assumptions:
-
(i)
The underlying kinetic evolution is such that the term between square brackets, in Eq. (3.87), can be approximated, for large τ, by \(\int _{0}^{\infty }e^{\varLambda t}\mathit{dt}\).
-
(ii)
The expansion of the logarithm to first order in τ is a valid approximation before the limit τ → ∞ is taken.
Thus, using the symmetry of the operator Λ, and neglecting, for simplicity, kinematic contributions [46] of the form \(\langle \boldsymbol{\xi }(0)\vert \varLambda \delta \boldsymbol{X}(0)\rangle\), Eq. (3.86) can be finally written in the form:
Equation (3.88) allows one to extend the Green-Kubo formalism, which relates the response function to a suitable time correlation function, to the short wavelength domain. Moreover, it can be evinced from an inspection of Fig. 3.9 that in the free-particle regime, k → ∞, the transport coefficients vanish and dissipative effects fade off. This observation finds a sound confirmation in [18]: “Operationally, of course, transport coefficients cannot even be defined for a gas of non-interacting particles. A measurement of the thermal conductivity, for example, is only possible if we can apply, quasi-statically, a temperature gradient and maintain it while we measure the heat current. However, only for a system with a finite mean free path can a temperature gradient be maintained quasi-statically. A free gas would “run away”, and the standard measurements of transport coefficients cannot be performed. Still, it may be satisfying for some that, in this case, the Kubo expressions give the most sensible result: zero.”
3.7 Short Wavelengths Hydrodynamics
The existence of collective modes at short wavelengths, in real fluids, is a long-standing issue in fluid dynamics [48, 49]. In their seminal work [50], Ford et al. illustrated, on the basis of a model kinetic equation approximating the linearized Boltzmann equation, that the sound modes extended to length scales comparable with the mean free path in the gas. Similarly, our analysis showed that hydrodynamic modes and the generalized transport coefficients extend smoothly over the whole k domain. Therefore, the Invariant Manifold technique allows to refine the hydrodynamic description beyond the strictly hydrodynamic regime. Our results also strengthen those previously reported in [3, 51, 52] on dense fluids, which revealed that the hydrodynamic laws provide a sensible description of fluids even at length scales comparable with λ mf .
It would be interesting, hence, to investigate the features of the equations of generalized hydrodynamics we obtained in the regime of finite frequencies and wavevectors. In Fig. 3.10a comparison is shown about inverse phase velocity and damping for acoustic waves between our results, former approaches [12, 29] and experimental data performed by Meyer and Sessler [47]. As it is seen, our results are very close to the predictions of the regularized 13 (Reg13) moments method [29] and closer to experimental data than (Reg13) concerning the phase spectrum. Our theory also predicts a phase speed which remains finite also at high frequencies, a property which is not enjoyed by any hydrodynamics derived from the CE expansion [28].
(a) Damping spectrum, i.e., the negative imaginary part of k divided by frequency ω vs. the negative logarithm of ω. Results obtained in this work (by solving Eq. (3.66), and subsequently Eq. (3.65) for w(k) with complex-valued k and real-valued ω) are compared with previous approaches including Navier Stokes (NS), regularized 13 moment (Reg13) [29], Grad’s 13 moment (Grad13), and experimental data presented in [47]. (b) Phase spectrum, i.e, real part of k times velocity of sound c 0 and divided by ω vs. the negative logarithm of ω. Again, we compare with reference results
A further clue about the features of our model at finite frequencies and wavevectors can be achieved by investigating the spectrum of density fluctuations, \(S_{\tilde{n},\tilde{n}}\) [4] From the knowledge of the functions A–Z, it is possible to compute the coefficients D T and Γ, related to the damping, respectively, of thermal and pressure fluctuations in fluids. In the limit of small k, and following standard textbooks [39], theyread:
It is worth pointing out that, unlike standard treatments of hydrodynamic fluctuations, the generalized transport coefficient X enters the expression of the coefficients D T and Γ, even though its contribution, as it is evident from Fig. 3.9 is fairly small. The calculation of \(S_{\tilde{n},\tilde{n}}\) is a standard textbook exercise [39, 53]. We give, hence, the final result:
(a) Dynamic structure factor \(S_{\tilde{n},\tilde{n}}(k,\omega )\) vs. ω for a small k = 0. 4 and (b) large k = 100. \(\omega _{s} = c_{0}k\) denotes the hydrodynamic predicted sound mode of the spectrum, and the widths are related to the moments A–Z (see Fig. 3.9). For small k, these are given by \(D_{T} = \frac{2} {5}(X - Y )\) and \(\varGamma = -(\frac{1} {2}A + \frac{1} {5}X + \frac{2} {15}Y )\), where A is the generalized longitudinal kinetic viscosity, Y the generalized thermal diffusion coefficient and X is a cross-coupling transport coefficient, relating heat flux to density gradients. (c) Width \(D_{\mathit{Tk}}^{2}\) of the Rayleigh peak vs. k (double-logarithmic). At small k, \(D_{\mathit{Tk}}^{2} \propto k^{2}\) as all moments A–Z, except X, reach a finite value in this limit. The inflection point at k = k ∗(N) ≫ 1 (shown to be increasing with the order of expansion N) denotes the onset of departure from the ideal Maxwellian behavior, where the width of the peak starts to behave sublinearly in k, and is used to quantify the range of validity for results obtained at finite order
Representative plots of S(k, ω) are shown in Fig. 3.11a, b. For small k (hydrodynamic limit), the obtained spectrum recovers the usual results of neutron (or light) scattering experiments and consists of the three Lorentzian peaks. The one centered in ω = 0 is the Rayleigh peak, which corresponds to the diffusive thermal mode. The two side peaks centered in ω ± c 0 k are the Brillouin peaks, and represent the two propagating sound waves.
By increasing the wave-vector, one enters an “intermediate” regime, in which the structure of (3.89) is unchanged, except that the generalized coefficients D T and Γ must then be replaced by more complicate expressions [31]. The net effect observed is that sound waves get damped and disappear, whereas the central Rayleigh peak decreases and broadens. Density fluctuations are, therefore, driven only by a diffusive thermal mode for large enough k. A deeper look about the behavior of the width at half maximum of the central Rayleigh peak with increasing wavevectors allows us to bridge, hence, the gap between the hydrodynamic and the free-particle regimes. For k ≪ 1 the width of the central peak increases with the square wavevector, ∝ k 2, whereas, in the opposite regime, k ≫ 1 the width of the central peak is expected to grow up linearly in k.
Our results, see Fig. 3.11, predict a width which is truly quadratic for small enough k, reaches the regime of linear behavior for large k and terminates, for some large k, with a sub-linear dependence on k. The onset of the terminal regime at k = k ∗(N) marks the range of validity which can be accessed at a given finite order of expansion N. Increasing N does not alter the overall picture obtained at a moderate order of expansion, and, more generally, results obtained with N + 1 will not change those obtained with N below k ∗(N), cf. Fig. 3.11c.
By varying the parameter N in the expansions (3.80) and (3.81) one is able to tune the number of nonequilibrium contributions to be included into the distribution function, thus refining the hydrodynamic description to an arbitrary level of accuracy.
The onset of a critical length scale marking the limit of the hydrodynamic description is reminiscent of the discussion made in Sect. 3.2. Namely, we know that hydrodynamics is founded on the notion of Local Equilibrium. Thus, for a given N, one may be tempted to link the length scale at which hydrodynamics breaks down, \([k^{{\ast}}(N)]^{-1}\), with the mesoscopic scale \(\ell_{\mathit{meso}}\). Further investigation is called for to shed light on this proposal: should such connection be true, this would then endow the generalized hydrodynamic equations obtained via the Invariant Manifold theory with a deeper thermodynamic content.
4 Conclusions
In this work, we described the use of the Invariant Manifold technique to derive closed hydrodynamic equations from some kinetic models. The main novelty of our approach stems from the use of a non-perturbative technique, which makes it possible to sum up exactly the classical Chapman–Enskog expansion. The method postulates a separation between slow and fast moments, and allows one to extract the slow invariant manifold in the space of distribution functions.
The obtained equations of exact hydrodynamics, derived by solving the invariance equation, are hyperbolic and admit a H-Theorem. Our solution of the invariance equation was considerably simplified by considering linear deviations from global equilibrium.
The generalized transport coefficients have been numerically determined and settled into expressions which recover the Green-Kubo formulae. Finally, by also comparing with available experimental data and previous approaches, we discussed the range of validity of our approach, which turned out to be amenable of extending the hydrodynamic scenario to length scales comparable with the mean free path. Our approach may help shedding new light on the investigation about the transition between the kinetic, particle-like, description of matter and the macroscopic, “continuum”, one.
References
Colangeli, M.: From Kinetic Models to Hydrodynamics. Some Novel Results. Springer, New York (2013)
Gorban, A.N., Karlin, I.V.: Invariant Manifolds for Physical and Chemical Kinetics. Lecture Notes in Physics, vol. 660. Springer, Berlin (2005)
Alder, B.J., Alley, W.E.: Generalized hydrodynamics. Phys. Today 37, 56 (1984)
Boon, J.P., Yip, S.: Molecular Hydrodynamics. Dover, New York (1991)
Bobylev, A.V.: Sov. Phys. Dokl. 27, 29 (1982)
Uribe, F.J., Velasco, R.M., Garcìa-Colìn, L.S.: Bobylev’s instability. Phys. Rev. E 62, 5835 (2000)
Cercignani, C., Illner, R., Pulvirenti, M.: The Mathematical Theory of Dilute Gases. Springer, Berlin (1994)
Cercignani, C.: Theory and Application of the Boltzmann Equation. Scottish Academic Press, Edinburgh (1975)
Beskok, A., Karniadakis, G.E.: Microflows: Fundamentals and Simulation. Springer, Berlin (2001)
Karabacak, D.M., Yakhot, V., Ekinci, K.L.: High-frequency nanofluidics: an experimental study using nanomechanical resonators. Phys. Rev. Lett. 98, 254505 (2007)
Öttinger, H.C.: Beyond Equilibrium Thermodynamics. Wiley, New York (2005)
Grad, H.: On the kinetic theory of rarefied gases. Commun. Pure Appl. Math. 2, 331 (1949)
Jou, D., Casas-Vázquez, J., Lebon, G.: Extended Irreversible Thermodynamics. Springer, Berlin (2010)
Bogoliubov, N.N.: In: de Boer, J., Uhlenbeck, G.E. (eds.) Studies in Statistical Mechanics, vol. 1. Wiley, New York (1962)
Liboff, R.: Kinetic Theory Classical, Quantum and Relativistic Descriptions. Springer, New York (2003)
Liboff, R.: Generalized Bogoliubov hypothesis for dense fluids. Phys. Rev. A 31, 1883 (1985)
Balescu, R.: Equilibrium and Nonequilibrium Statistical Mechanics. Wiley, New York (1975)
Forster, D.: Hydrodynamic Fluctuations, Broken Symmetry, and Correlation Functions. W. A. Benjamin, New York (1975)
Chapman, S., Cowling, T.G.: The Mathematical Theory of Nonuniform Gases. Cambridge University Press, New York (1970)
Lachowicz, M.: From kinetic to Navier-Stokes-type equations. Appl. Math. Lett. 10, 19 (1997)
Colangeli, M., Karlin, I.V., Kröger, M.: From hyperbolic regularization to exact hydrodynamics for linearized Grad’s equations. Phys. Rev. E 75, 051204 (2007)
Colangeli, M., Karlin, I.V., Kröger, M.: Hyperbolicity of exact hydrodynamics for three-dimensional linearized Grad’s equations. Phys. Rev. E 76, 022201 (2007)
Zwanzig, R.: Memory effects in irreversible thermodynamics. Phys. Rev. 124, 983 (1961)
Zubarev, D.N., Kalashnikov, V.P.: Extremal properties of the nonequilibrium statistical operator. Theor. Math. Phys. 1, 108 (1969)
Grabert, H.: Projection Operator Techniques in Nonequilibrium Statistical Mechanics. Springer, Berlin (1982)
Haken, H.: Synergetics: An Introduction: Nonequilibrium Phase Transitions and Self-organization in Physics, Chemistry and Biology. Springer, New York (1978)
Gardiner, C.W.: Handbook of Stochastic Methods. Springer, Berlin (2004)
Struchtrup, H.: Macroscopic Transport Equations for Rarefied Gas Flows. Approximation Methods in Kinetic Theory. Springer, Berlin/Heidelberg (2005)
Struchtrup, H., Torrilhon, M.: H-theorem, regularization, and boundary conditions for linearized 13 moment equations. Phys. Rev. Lett. 99, 014502 (2007)
Bobylev, A.V.: Instabilities in the Chapman-Enskog expansion and hyperbolic Burnett equations. J. Stat. Phys. 124, 371 (2006)
Colangeli, M., Kröger, M., Öttinger, H.C.: Boltzmann equation and hydrodynamic fluctuations. Phys. Rev. E 80, 051202 (2009)
Bhatnagar, P.L., Gross, E.P., Krook, M.: A model for collision processes in gases. I. Small amplitude processes in charged and neutral one-component systems. Phys. Rev. 94, 511 (1954)
Kubo, R.: The fluctuation-dissipation theorem. Rep. Prog. Phys. 29, 255 (1966)
Onsager, L., Machlup, S.: Fluctuations and irreversible processes. Phys. Rev. 91, 1505 (1953)
Lebowitz, J.L., Presutti, E., Spohn, H.: Microscopic models of hydrodynamic behavior. J. Stat. Phys. 51, 841 (1988)
Karlin, I.V., Colangeli, M., Kröger, M.: Exact linear hydrodynamics from the Boltzmann equation. Phys. Rev. Lett. 100, 214503 (2008)
Resibois, P.: On linearized hydrodynamic modes in statistical physics. J. Stat. Phys. 2, 1 (1970)
Blatt, J.M.: Model equations in the kinetic theory of gases. J. Phys. A: Math. Theor. 8, 980 (1975)
Reichl, L.E.: A Modern Course in Statistical Physics. University of Texas Press, Austin (1980)
Kröger, M.: Models for Polymeric and Anisotropic Liquids. Springer, Berlin (2005)
Chikatamarla, S.S., Ansumali, S., Karlin, I.V.: Entropic lattice Boltzmann models for hydrodynamics in three dimensions. Phys. Rev. Lett. 97, 010201 (2006)
Öttinger, H.C., Struchtrup, H.: The mathematical procedure of coarse graining: from Grad’s ten-moment equations to hydrodynamics. Multiscale Model. Simul. 6, 53 (2007)
Wang Chang, C.S., Uhlenbeck, G.E.: The Kinetic Theory of Gases. North-Holland Pub. Co., Amsterdam (1970)
Kröger, M., Hütter, M.: Unifying kinetic approach to phoretic forces and torques for moving and rotating convex particles. J. Chem. Phys. 125, 044105 (2006)
Burnett, D.: The distribution of velocities and mean motion in a slight nonuniform gas. Proc. Lond. Math. Soc. 39, 385 (1935)
Gorban, A.N., Karlin, I.V.: Reconstruction lemma and fluctuation-dissipation theorem. Revista Mexicana de Fisica 48(Suppl. 1), 238 (2002)
Meyer, E., Sessler, G.: Schallausbreitung in gasen bei hohen frequenzen and sehr niedrigen drucken. Z. Phys. 149, 15 (1947)
de Schepper, I.M., Cohen, E.G.D.: Very-short-wavelength collective modes in fluids. J. Stat. Phys. 27, 2 (1982)
Gorban, A.N., Karlin, I.V.: Short-wave limit of hydrodynamics: a soluble example. Phys. Rev. Lett. 77, 282 (1996)
Foch, J.D., Ford, G.W. (eds.): Studies in Statistical Mechanics. North-Holland, Amsterdam (1970)
Alder, B.J., Alley, W.E.: Generalized transport coefficients for hard spheres. Phys. Rev. A 27, 3158 (1983)
Kirkpatrick, T.R.: Short-wavelength collective modes and generalized hydrodynamic equations for hard-sphere particles. Phys. Rev. A 32, 3130 (1985)
Hansen, J.-P., McDonald, I.R.: Theory of Simple Liquids. Academic, New York (2006)
Author information
Authors and Affiliations
Corresponding author
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2015 Springer International Publishing Switzerland
About this chapter
Cite this chapter
Colangeli, M. (2015). Small Scale Hydrodynamics. In: Banerjee, S., Rondoni, L. (eds) Applications of Chaos and Nonlinear Dynamics in Science and Engineering - Vol. 4. Understanding Complex Systems. Springer, Cham. https://doi.org/10.1007/978-3-319-17037-4_3
Download citation
DOI: https://doi.org/10.1007/978-3-319-17037-4_3
Publisher Name: Springer, Cham
Print ISBN: 978-3-319-17036-7
Online ISBN: 978-3-319-17037-4
eBook Packages: Physics and AstronomyPhysics and Astronomy (R0)