Abstract
We present a kinetic framework describing mixtures of polyatomic species undergoing chemical reactions. Using a generalized Chapman-Enskog expansion, we derive the corresponding macroscopic fluid model. The hyperbolic-parabolic structure of the resulting system of partial differential equations is investigated and closely related to the underlying kinetic framework. We also discuss the Cauchy problem for smooth solutions as well as numerical algorithms for the evaluation of multicomponent transport coefficients using structural properties derived from the kinetic theory.
Access provided by Autonomous University of Puebla. Download conference paper PDF
Similar content being viewed by others
Keywords
1 Introduction
Multicomponent reactive flows undergoing chemical reactions arise in many engineering applications such as chemical reactors [1–3], Earth reentry of space vehicles [4, 5], or flames [6–8]. This is an important motivation for investigating the derivation of the corresponding fluid equations from the kinetic theory of gases as well as analyzing the mathematical structure of the resulting system of partial differential equations [9].
We first present a kinetic framework describing mixtures of polyatomic species undergoing chemical reactions. The Boltzmann equations governing the species distribution functions are presented in a semi-quantum framework [10–19] with reactive sources [20–34]. We only consider mixtures at thermodynamic equilibrium with a single temperature and thermodynamic nonequilibrium lay out of the scope of the present notes [35–46]. The kinetic entropy production is shown to be nonnegative, which yields the Boltzmann H-theorem. We present the Enskog expansion and focus on regimes where the chemical characteristic times are larger than the collision times and than the times for relaxation of internal energy.
We derive the zeroth order as well as the first order corresponding macroscopic equations including conservation equations, thermodynamic properties, transport fluxes, transport coefficients and chemical production rates. The transport coefficients are defined in terms of bracket products involving solutions of integral linearized Boltzmann equations and their mathematical structure is extracted from the underlying kinetic framework. The macroscopic entropy conservation equation is obtained and we discuss the link between the kinetic entropy and the macroscopic fluid entropy. This kinetic study of reactive mixtures is performed in a physical framework, mathematical aspects of kinetic theory laying out of the scope of these notes [47–56]. The conservation equations, the transport fluxes as well as the source terms may also be obtained from different theories like the thermodynamic of irreversible processes or statistical thermodynamics but such theories do not yields the transport coefficients [57–64]. We further establish that upon neglecting the chemical pressure and the perturbations of the zeroth order source terms, both the Maxwellian reaction regime and the tempered reaction regime yield similar fluid models.
The transport fluxes of the resulting fluid conservation equations are written in terms of transport coefficients. The evaluation of accurate transport coefficients is therefore an important modeling and computational task [65–69]. However, evaluating the transport coefficients for gas mixtures require solving transport linear systems arising from Galerkin solution of systems of linearized Boltzmann equations. The mathematical structure of the transport linear systems is derived from the kinetic theory and the fast evaluation of accurate transport coefficients using either generalized conjugate gradient methods or stationary iterative algorithms is discussed [70–75]. The importance and/or influence of multicomponent transport for computing laminar flows is also addressed [76–85].
The fluid model derived from the kinetic theory is next embedded in a mathematical framework and recast in quasilinear form. The structural properties extracted from the kinetic underlying framework are transformed into relevant mathematical assumptions, thereby soundly founding the mathematical model. We then investigate the mathematical structure of the resulting system of partial differential equations. We discuss symmetrizability properties first using entropic variables and next using normal forms [86–104]. We explicitly evaluate the natural entropic form and next the natural normal form of the system of partial differential equations. We investigate the hyperbolic-parabolic structure using a definition of hyperbolicity from Denis Serre and further establish that for symmetric second order systems strong parabolicity is equivalent to Petrovsky parabolicity.
We then study the Cauchy problem for symmetrized systems of partial differential equations [105–118]. We present global existence theorems around constant equilibrium states as well as asymptotic stability and decay estimates [95]. The method of proof relies on the normal form of the governing equations, on entropic estimates, and on the local strict dissipativity properties of the linearized equations [89, 95, 106, 107].
The kinetic theory of reactive polyatomic gas mixtures is presented in Sects. 2 and 3. The evaluation of transport coefficients is addressed in Sect. 4. The resulting system of partial differential equations is imbedded in a mathematical framework in Sect. 5. Its hyperbolic-parabolic structure in investigated in Sect. 6 and the Cauchy problem is addressed in Sect. 7.
2 Kinetic Framework
We investigate the kinetic theory of mixtures of polyatomic gases [9–17, 19] with chemical reactions [20–34]. We present the Boltzmann equations governing the species distribution functions in a semi-quantum—or semi-classical—framework. We perform the Enskog expansion and obtain the zeroth order fluid governing equations. We only consider the situation of one temperature fluids, thermodynamic desequilibrium lying out of the scope of the present work [35–46]. Both Sects. 2 and 3 describing the physical derivation of the fluid equations are not formalized mathematically and only the macroscopic fluid equations will be investigated in a mathematical framework. Mathematical aspects concerning Boltzmann-type equations lay out of the scope of these notes and we refer the reader to [47–56].
2.1 Boltzmann Equations
We consider a reactive mixture composed of \(n\) species with internal degrees of freedom. The species equations are governed by Boltzmann equations written in a semi-quantum framework that may be obtained from Waldmann [11], Ludwig and Heil [24], Ferziger and Kaper [16], Alexeev et al. [31], Ern and Giovangigli [33], and Grunfeld [18].
The state of the mixture is described by the species distribution functions denoted by \(f_i(t,\varvec{x},\varvec{c}_{i},{\textsc {i}})\), \(i\in S\), where \(t\) denotes the time, \(\varvec{x}\) the three-dimensional spatial coordinate, \(\varvec{c}_{i}\) the velocity of the \(i\)th species, \({\textsc {i}}\) the index for the quantum state of the \(i\)th species, and \(S=\{1,\ldots ,n\}\) the set of species indices. We denote by \(\mathrm{{Q}}_i\) the indexing set of the quantum states of the \(i\)th species. The quantity \(f_i(t,\varvec{x},\varvec{c}_{i},{\textsc {i}}) \delta \varvec{x}\delta \varvec{c}_{i}\) represents the expected number of molecules of type \(i\) in quantum state \({\textsc {i}}\) in the volume element \(\delta \varvec{x}\) located at \(\varvec{x}\), whose velocities lie in \(\delta \varvec{c}_{i}\) about velocity \(\varvec{c}_{i}\) at time \(t\).
We denote by \({\mathfrak m}_i\) the mass of the molecule of the \(i\)th species, \(E_{i{\textsc {i}}}\) the internal energy of the molecules of species \(i\) in quantum state \({\textsc {i}}\) and \(a_{i{\textsc {i}}}\) the degeneracy of the \({\textsc {i}}\)th quantum state. The fluid macroscopic properties are directly obtained from the distribution functions. More specifically, the number of molecules of the \(i\)th species per unit volume \(\mathfrak {n}_i\) is given by
and the mass dentity of the \(i\)th species is then \(\rho _i={\mathfrak m}_i \mathfrak {n}_i\). The mass averaged velocity \(\varvec{v}\) is obtained from
where \(\rho = \sum _{i\in S} \rho _i\) is the total mass density. The total energy per unit volume is also defined by
where \(\mathcal {E}\) is the internal energy per unit volume. The family of distribution functions will be written for convenience in the form \((f_i)_{i\in S}\). More generally, for a family of functions \(\xi _i\), \(i\in S\), where \(\xi _i\) depends on \(\varvec{c}_{i}\) and \({\textsc {i}}\), we will use the compact notation \(\xi =(\xi _i)_{i\in S}\), the dependence on \((t,\varvec{x})\) being left implicit.
The species distribution functions are solutions of generalized Boltzmann equations in the form
where \(\mathcal {D}_{i}(f_i)\) is the streaming differential operator, \(\mathcal {S}_{i}(f)\) the scattering source term and \(\mathcal {C}_{i}(f)\) the reactive source term. These Boltzmann equations express the conservation of particles in the phase space and may also be derived from the BBGKY-hierarchy [10–13, 15–19]. The streaming differential operator \(\mathcal {D}_{i}(f_i)\) may be written
where \(\varvec{b}_i\) is the force per unit mass acting on the \(i\)th species.
The scattering term may be written
where, in a direct collision, \({\textsc {i}}\) and \({\textsc {j}}\) are the quantum states before collision, \({\textsc {i}}'\) and \({\textsc {j}}'\) the states after collision, \(a_{i{\textsc {i}}}\) the degeneracy of the \({\textsc {i}}\)th quantum state, and \(\mathtt {W}_{ij}^{{\textsc {i}}{\textsc {j}}{\textsc {i}}'{\textsc {j}}'}\) the transition probability for nonreactive collisions. We have denoted by \(f'_i\) the distribution \(f'_i=f_i(t,\varvec{x},\varvec{c}_{i}',{\textsc {i}}')\) where \(\varvec{c}_{i}'\) is the velocity and \({\textsc {i}}'\) the quantum state after collision. The following reciprocity relations are satisfied by the transition probabilities [11]
These reciprocity relations are of fundamental importance in the theory since they are related to the symmetry properties of the collision operator and are also requisites for Boltzmann H-theorem. We are using transition probabilities rather than collision cross sections for convenience since the reactive collision terms are then much simpler to write [9, 24, 31]. For binary nonreactive collisions, denoting by \(\sigma _{ij}^{{\textsc {i}}{\textsc {j}}{\textsc {i}}'{\textsc {j}}'}\) the collision cross section, we have
where \(g_{ij}\) denotes the norm of the relative velocity \(\varvec{c}_{i}-\varvec{c}_{j}\) of the collision partners before collision and \({\varvec{e}}_{ij}'\) the unit vector in the direction of the relative velocity \(\varvec{c}_{i}' - \varvec{c}_{j}'\) after collision [18, 24, 31]. The conservation of mass, momentum and energy during collision is taken into account by using Dirac delta functions in the transition probabilities [18, 24, 31].
The reactive source term \(\mathcal {C}_{i}(f)\) is due to chemical reaction between the species of the mixture. We consider an arbitrary chemical reaction mechanism including binary as well as ternary mixtures. Even though ternary collisions may be neglected in the nonreactive collision term \(\mathcal {S}_{i}(f)\), ternary collisions play an important role in chemistry since it is often the unique chemical path in order to form some type of molecules [24, 31]. Ternary collision may also be seen as a succession of two rapid binary collisions [31]. The chemical reactions are indexed by \(r\in {\mathfrak {R}}=\{1,\ldots ,n^{\mathrm{r}}\}\) where \(n^{\mathrm{r}}\) is the number of reactions and may be written [33]
where \(\mathcal {F}^r\) and \(\mathcal {B}^r\) are the indices of reactants and products with their multiplicity. We denote by \(\nu _{i r}^{\text {f}}\) and \(\nu _{i r}^{\text {b}}\) the stoichiometric coefficients of the \(i\)th species in the \(r\)th reaction, that is, the multiplicity of species \(i\) in \(\mathcal {F}^r\) and \(\mathcal {B}^r\), respectively, and by \({\textsc {f}}^r\) and \({\textsc {b}}^r\) the indices of the quantum energy states of the reactants and products, respectively. We also denote by \(\mathcal {F}_{i}^r\) the subset of \(\mathcal {F}^r\) where the index \(i\) has been removed once with similar notation for \(\mathcal {B}_{k}^r\), \(\textsc {f}_{{\textsc {i}}}^r\) and \(\textsc {b}_{{\textsc {k}}}^r\). The reactive collision term for the \(i\)th species may then be written [9, 33]
where \(\mathcal {C}_{i}^r(f)\) represents the contribution of the \(r\)th reaction. This term \(\mathcal {C}_{i}^r(f)\) is given by

where \(\beta _{i {\textsc {i}}} = \text {h}_{\text {P}}^3/(a_{i{\textsc {i}}}{\mathfrak m}_i^3)\), \(\text {h}_{\text {P}}\) is the Planck constant, and \(\mathcal {W}_{\mathcal {F}^r\mathcal {B}^r}^{{\textsc {f}}^r{\textsc {b}}^r}\) denotes the transition probability that a collision between the reactants \(\mathcal {F}^r\) with energies \({\textsc {f}}^r\) lead to the products \(\mathcal {B}^r\) with energies \({\textsc {b}}^r\). The sommation over \({\textsc {f}}^r\) in (11) represents the sum over all quantum indices \({\textsc {j}}\) for all \(j\in \mathcal {F}^r\) with similar conventions for \(\textsc {f}_{{\textsc {i}}}^r\), \({\textsc {b}}^r\), and \(\textsc {b}_{{\textsc {i}}}^r\). Finally, the following reciprocity relations hold between transition probabilities [9, 24, 31, 33]
and generalize the relations (7) between the nonreactive transition probabilities. The reciprocity relations (12) between reactive transition probabilities are also important and imply in particular the symmetry properties of linearized chemical source at equilibrium that may be seen as Onsager relations for chemistry.
2.2 Collisional Invariants
A collisional invariant \(\psi \) of the rapid collision operator \(\mathcal {S}_{}=(\mathcal {S}_{i}(f))_{i\in S}\) is by definition a family \(\psi =(\psi _i)_{i\in S}\) such that \(\psi _i + \psi _j = \psi _i' + \psi _j'\) for any nonreactive collision between species \(i\) and \(j\). The scalar collisional invariants of the rapid collision operator form a vector space spanned by the invariants \(\psi ^l\), \(l\in \{1,\ldots ,n+4\}\), defined by
where \({\mathfrak m}_i\) is the mass of the molecule of the \(i\)th species and \(c_{i\nu }\) the component of \(\varvec{c}_{i}\) in the \(\nu \) spatial direction [10–13, 15, 16]. The \(n\) first invariants \(\psi ^l\), \(l\in \{1,\ldots ,n\}\), are associated with species conservation in nonreactive collisions, the invariants \(\psi ^{n+1}\), \(\psi ^{n+2}\), and \(\psi ^{n+3}\), with momentum conservation in the three spatial directions, and \(\psi ^{n+4}\) with total energy conservation [9, 11, 15, 16]. The collisional invariants of the complete collision operator \(\mathcal {S}_{}+\mathcal {C}_{}=\bigl (\mathcal {S}_{i}(f)+\mathcal {C}_{i}(f)\bigr )_{i\in S}\) are associated with the conservation of momentum, total energy and chemical elements or atoms [33]. Denoting by \({\mathfrak {a}}_{il}\) the number of \(l\)th atom in the \(i\)th species, \({\mathfrak {A}}=\{1,\ldots ,n^{\mathrm{a}}\}\) the set of atom indices, and \(n^{\mathrm{a}}\geqslant 1\) the number of atoms—or elements—in the mixture, the atomic collisional invariants of the complete collision operator may be written \({\mathfrak {a}}_l=({\mathfrak {a}}_{il})_{i\in S}\) for \(l\in {\mathfrak {A}}\).
Remark 2.1
When molecules are not spherically symmetric, there could be another summational invariant, namely angular momentum. However, we are only interested in these notes with isotropic distributions without micro-polarizations associated with strong magnetic fields. For such isotropic distributions the angular momentum summational invariant plays no role [16].
For two tensor families \(\xi ={(\xi _i)}_{i\in S}\) and \(\zeta ={(\zeta _i)}_{i\in S}\), we define the scalar product
where \(\xi _i \odot \zeta _i\) is the full contracted product between tensor \(\xi _i\) and tensor \(\zeta _i\). We introduce families of tensors since they naturally arise in the definition of transport coefficients. The macroscopic properties naturally associated with the fluid may then be written in the compact form
where \(v_\nu \) denotes the component in direction \(\nu \) of the mass average velocity \(\varvec{v}\).
2.3 Kinetic Entropy
The kinetic entropy per unit volume is defined by
where \({k_{\!{\scriptscriptstyle \mathrm{{B}}}}}\) is the Boltzmann constant and \(\beta _{i {\textsc {i}}} = \text {h}_{\text {P}}^3/(a_{i{\textsc {i}}}{\mathfrak m}_i^3)\). Multiplying the Boltzmann equation (4) by \(\log (\beta _{i {\textsc {i}}}f_i)\), integrating with respect to \(\mathrm {d}\varvec{c}_{i}\), summing over the species \(i\in S\) and over the quantum states \({\textsc {i}}\in \mathrm{{Q}}_i\), we obtain a balance equation for \(\mathcal {S}^{\text {kin}}\) in the form
where \(\varvec{\mathcal {J}}^{\text {kin}}\) is the entropy diffusive flux,
and \(\mathfrak {v}^{\text {kin}}\) the entropy source term. The entropy source term \(\mathfrak {v}^{\text {kin}}\) may be written \(\mathfrak {v}^{\text {kin}}= \mathfrak {v}^{\mathcal {S}}+ \mathfrak {v}^{\mathcal {C}}\) with contributions from the nonreactive collision
and reactive collisions
After some algebra, it is obtained that
where \(\varUpsilon \) denotes the function \(\varUpsilon (x,y)=(x-y)(\log x - \log y)\). Since this function only takes nonnegative values, we conclude that both quantities \(\mathfrak {v}^{\mathcal {S}}\) and \(\mathfrak {v}^{\mathcal {C}}\) are sum of nonnegative terms. All collisions, nonreactive or reactive, thus yield nonnegative entropy productions [9, 33]. The generalized Boltzmann equations are thus compatible with the Boltzmann H-theorem and will lead to a dissipative structure at the molecular level. This important property must be recovered at the macroscopic level, that is, both type of collisions should yield nonnegative macroscopic entropy production.
2.4 Enskog Expansion
An approximate solution of Boltzmann equations (4) is obtained with Enskog expansion and we assume to this aim that the chemical time scales are larger than the collision times as well as the times for relaxation of internal energy. We thus write the Boltzmann equations (4) in the form
where \(\epsilon \) is the formal parameter associated with Enskog expansion and \(a\) is a integer which depends upon the regime under consideration. We only consider in these notes the situation of Maxwellian reactions \(a=1\) or the situation of tempered reactions \(a=0\), whereas the kinetic equilibrium regime \(a=-1\) investigated in [33] lay out of the scope of the present notes.
The species distribution functions are expanded in the form
and the Chapman-Enskog method requires that \(f\) and \(f^0\) have the same macroscopic observables
We establish in next section that \(f^0_i\) is the local Maxwellian and introduce the following convenient notation \(f^0 = {(f^0_i)}_{i\in S}\), \(\mathcal {D}_{}(\xi )={\bigl (\mathcal {D}_{i}(\xi _i)\bigr )}_{i\in S}\), \(\mathcal {S}_{}(\xi )={\bigl (\mathcal {S}_{i}(\xi )\bigr )}_{i\in S}\), \(\mathcal {C}_{}(\xi )= {\bigl (\mathcal {C}_{i}(\xi )\bigr )}_{i\in S}\) and \(\mathcal {C}_{}^r(\xi )= {\bigl (\mathcal {C}_{i}^r(\xi )\bigr )}_{i\in S}\), \(r\in {\mathfrak {R}}\), where \(\xi = {(\xi )}_{i\in S}\) is a family of functions \(\xi _i\) depending on \((\varvec{c}_{i},{\textsc {i}})\).
2.5 Maxwellian Distributions
The zeroth order distributions \(f^0={(f_i^0)}_{i\in S}\) satisfy the zeroth order equations
Multiplying (23) by \(\log (\beta _{i {\textsc {i}}}f_i^0)\), integrating with respect to \(\mathrm {d}\varvec{c}_{i}\), summing over \(i\in S\) and over \({\textsc {i}}\in \mathrm{{Q}}_i\), it is obtained that
Using the expression (18) of the source term \(\mathfrak {v}^{\mathcal {S}}\) we obtain that \({\bigl (\log (\beta _{i {\textsc {i}}}f_i^0)\bigr )}_{i\in S}\) is a collision invariant. It is then a linear combination of the invariants \(\psi ^l\), \(1\le l \le n+ 4\), in such a way that
where \(\alpha _i\in {\mathbb {R}}\), \({\varvec{\beta }}\in {\mathbb {R}}^3\) and \(\gamma \in {\mathbb {R}}\), are parameters determined by the macroscopic constraints (22). After some algebra, it is obtained that
where \(\varvec{C}_{i}=\varvec{c}_{i}-\varvec{v}\) is the relative velocity of the \(i\)th species, \(T\) the temperature and \(\textsc {z}_{i}\) the partition function per unit volume of the \(i\)th species. This partition function \(\textsc {z}_{i}\) of the \(i\)th species may be written
where \(\textsc {z}_{i}^{\text {int}}\) and \(\textsc {z}_{i}^{\text {tr}}\) denote respectively the internal partition function and the translational partition function per unit volume
and another convenient expression of the local Maxwellian \(f_i^0\) reads
2.6 Zeroth Order Equations
Macroscopic equations are generally obtained by taking scalar products of the Boltzmann equations by collisional invariants. At zeroth order, only the terms that are \(O(\epsilon ^0)\) are taken into account and it is obtained that
for \(l\in \{1,\ldots ,n+4\}\). After a few algebra, we deduce for \(l=1,\ldots ,n\), the species mass conservation equations
where \(\rho _i= {\mathfrak m}_i \mathfrak {n}_i\) is the mass density of the \(i\)th species and \({\mathfrak w}_{i}^0\) the zeroth order chemical production rate
The momentum conservation equations are obtained for \(l=n+1,n+2,n+3\), and may be written in vector form
where \(\varvec{I}\) is the unit tensor, \(p\) the pressure, and \(\varvec{b}\) the average force
Finally, the equation obtained with \(l=n+4\) express the conservation of total energy
The macroscopic zeroth order equations are thus the compressible Euler equations for a reactive mixture of polyatomic gases.
2.7 Thermal Properties
The internal energy per unit volume \(\mathcal {E}\) may be evaluated by using \(\mathcal {E}= {\langle \! \langle f, \psi ^\mathrm{int} \rangle \! \rangle } = {\langle \! \langle f^0, \psi ^\mathrm{int} \rangle \! \rangle }\) and the expression of the Maxwellian distributions, where the collisional invariant \(\psi ^\mathrm{int}=(\psi ^\mathrm{int}_i)_{i\in S}\) is defined by \(\psi ^\mathrm{int}_i = E_{i{\textsc {i}}} + \tfrac{1}{2}{\mathfrak m}_i \vert \varvec{C}_{i}\vert ^2\). After some algebra, it is obtained that
where \(\overline{E}_{i}\) denotes the average internal energy per molecule of the \(i\)th species
Defining the energy per unit mass of the \(i\)th species by \(e_i = (\tfrac{3}{2}{k_{\!{\scriptscriptstyle \mathrm{{B}}}}}T+\overline{E}_{i})/{\mathfrak m}_i\) we also have \(\mathcal {E}= \rho e = \sum _{i\in S} \rho _i e_i\). Similarly, the enthalpy per unit volume \(\mathcal {H}\) is given by
or equivalently by \(\mathcal {H}= \rho h = \sum _{i\in S} \rho _i h_i\) where \(h_i = (\tfrac{5}{2}{k_{\!{\scriptscriptstyle \mathrm{{B}}}}}T+\overline{E}_{i})/{\mathfrak m}_i\) is the enthalpy per unit mass of the \(i\)th species.
We define the internal specific heat \(\mathfrak {c}_i^{\text {int}}\) per molecule of the \(i\)th species by \(\mathfrak {c}_i^{\text {int}} = \frac{\mathrm {d}\overline{E}_{i}}{\mathrm {d}T}\) and from the expression of \(\overline{E}_{i}\) we obtain that
The translational specific heat at constant volume \(\mathfrak {c}_\mathrm{{v}}^{\text {tr}}\) and the specific heat at constant volume \(\mathfrak {c}_{i \mathrm{{v}}}\) of the \(i\)th species are then defined by
The mixture internal specific heat \(\mathfrak {c}^{\text {int}}\) and heat at constant volume are also defined by
where \(\mathfrak {n}= \sum _{i\in S} \mathfrak {n}_i\) denotes the total number density. On the other hand, the pressure is given by the state law
and we recover the perfect gas law.
It will be necessary in the following to use a zeroth equation for the temperature \(T\). After some algebra, the following zeroth order conservation equation is obtained for the temperature \(T\)
2.8 Maxwellian Production Rates
The zeroth order source term may be written in the form [9]
where \(\overline{\tau }_{r}\) denotes the rate of progress of the \(r\)th reaction. The rate of progress \(\overline{\tau }_{r}\) is obtained from \((\nu _{i r}^{\text {b}}-\nu _{i r}^{\text {f}}) \overline{\tau }_{r}= {\langle \! \langle \mathcal {C}_{}^r(f^0),\psi ^i \rangle \! \rangle }\) and may be written
where \(\mathcal {K}_{\text {r}}\) is the rate constant of the \(r\)th reaction [9, 33]
These zeroth order chemical production rates are compatible with the law of mass action and with traditional thermochemistry [9].
Denoting by \({\mathfrak {a}}_{il}\) the number of \(l\)th atom in the \(i\)th species, \({\mathfrak {A}}=\{1,\ldots ,n^{\mathrm{a}}\}\) the set of atom indices, and \(n^{\mathrm{a}}\geqslant 1\) the number of atoms—or elements—in the mixture, the stoichiometric coefficients satisfy the atom conservation relations
Using (34), these constraints imply that
which states that atoms are conserved by chemical reactions. On the other hand, the species being constituted by atoms, denoting by \({\widetilde{{\mathfrak m}}}_l\) the mass of the \(l\)th atom, we have the relations
From these relations between the atom and species mass, and from the conservation of atoms, mass is also conserved during chemical reactions so that
as well as
so that there is no mass production due to chemical reactions.
3 Dissipative Regime
We obtain in this section the first order macroscopic equations governing polyatomic gas mixtures [10–19] with chemical reactions [20–34]. We derive in particular the transport fluxes—which yield dissipative effects like viscosity, diffusion or thermal conduction, and we also obtain the transport coefficients. For a single gas, the corresponding equations are the Navier-Stokes-Fourier system.
3.1 Linearized Boltzmann Equations
The linearized Boltzmann collision operator \(\mathcal {I}= \bigl (\mathcal {I}_i\bigr )_{i\in S}\) is defined by
An important property of this linearized collision operator is that it is isotropic so that it transforms a tensor built with \({(\varvec{c}_{i})}_{i\in S}\) into an analog tensor as in the monatomic case [11, 15, 16].
We introduce the corresponding bracket operator
between two families of tensors \(\xi =\bigl (\xi _i\bigr )_{i\in S}\), \(\zeta =\bigl (\zeta _i\bigr )_{i\in S}\), where \(\xi _i\) and \(\zeta _i\) depend on \(\varvec{c}_{i}\) and \({\textsc {i}}\). This bilinear operator is symmetric \([ \! [ \xi ,\zeta ] \! ] = [ \! [ \zeta ,\xi ] \! ]\), positive semi-definite \([ \! [ \xi ,\xi ] \! ] \ge 0\), and its nullspace is constituted by the collisional invariants, that is, \([ \! [ \xi ,\xi ] \! ] = 0\) if and only if \(\xi \) is a tensorial collisional invariant so that all its components are scalar collisional invariants [15, 16]. These symmetry properties are notably consequences of reciprocity relations between the transition probabilities or equivalently the collision cross sections.
The first order equations that govern the perturbed species distribution functions \(\phi = {(\phi _i)}_{i\in S} \) are directly obtained from (20) to (21). These linearized equations are in the form
where
In addition, the relations (22) yield the scalar constraints
The term \(\mathcal {D}_{i}(\log f_i^0)\) appearing in the right hand side is evaluated from the zeroth order macroscopic equations as required by the Chapman-Enskog method. After lengthy calculations, it is obtained that
with
where \(p_i=\mathfrak {n}_i{k_{\!{\scriptscriptstyle \mathrm{{B}}}}}T\) is the partial pressure of the \(i\)th species and \(\varvec{C}_{i}= \varvec{c}_{i}-\varvec{v}\) the relative velocity of the \(i\)th species. In these expressions, we have denoted
so that \({\varvec{\varPsi }}_i^{\eta }\) is a symmetric traceless tensor, \(\varPsi _i^{\kappa }\) a scalar and \({\varvec{\varPsi }}_i^{D_j}\), \(j\in S\), and \({\varvec{\varPsi }}_i^{\widehat{\lambda }}\) are vectors. In order to expand \(\varPsi _i^{\mathcal {C}_{}}\) we also write
where \( \overline{\tau }_{r}\) is the zeroth order rate of the \(r\)th reaction (35) and where \(\varPsi _i^r \) is given by
By linearity of the operator \(\mathcal {I}\), the solution \(\phi = {(\phi _i)}_{i\in S}\) of (43) and (44) may be expanded in a similar form
where
The functions \(\phi ^\mu \), for \(\mu \in \{ \eta , \kappa , D_1, \ldots ,D_n, \widehat{\lambda }\} \cup {\mathfrak {R}}\), are now of tensor type and satisfy the integral equations
with the constraints
These integral equations are generally shown to be well posed by using Fredholm alternative [17, 54, 56].
3.2 First Order Equations
The conservation equations at first order are obtained by taking the scalar product of Boltzmann equations by the collisional invariants and by keeping all terms that are \(O(\epsilon ^0)\) or \(O(\epsilon ^1)\)
where \(l\in \{1,\ldots ,n+4\}\) and \(\partial _f \mathcal {C}_{}(f^0) f^0 \phi ={(\partial _f \mathcal {C}_{i}(f^0) f^0 \phi )}_{i\in S}\).
The equations for the conservation of species mass are obtained for \(l=1,\ldots ,n\), and are in the form
where \(\mathbf {v}_i\) is the diffusion velocity and \({\mathfrak {w}}_{i}\) the production term for the \(i\)th species. The diffusion velocities are defined by
and the source term by
The mass flux \(\varvec{\mathcal {F}}_i\) of the \(i\)th species is further defined as \(\varvec{\mathcal {F}}_i = \rho _i \mathbf {v}_i = {\mathfrak m}_i \mathfrak {n}_i \mathbf {v}_i\) and satisfy the constraint
since \(f^0\phi \) is orthogonal to the vector collision invariant \(({\mathfrak m}_i \varvec{C}_{i})_{i\in S}\).
The momentum equations are obtained for \(l=n+1,n+2,n+3\), and may be written in vector form
where the viscous tensor \(\varvec{\Pi }_{}\) is defined by
The energy conservation equation obtained for \(l=n+4\), is finally in the form
where \(\varvec{\mathcal {Q}}\) is the heat flux
Once the transport fluxes \(\mathbf {v}_i\), \(i\in S\), \(\varvec{\Pi }_{}\), and \(\varvec{\mathcal {Q}}\) are expressed in terms of macroscopic quantities and their gradients, these are the conservation equation governing multicomponent reactive flows. Many simplifications are then possible depending on the particular application under concern but we are only interested here in the general equations.
3.3 Transport Fluxes and Coefficients
Using the definition (60) of the species diffusion velocities \(\mathbf {v}_i\) and the expression (50) of \({\varvec{\varPsi }}_i^{D_j}\) one may establish after some algebra that [9, 19]
Substituting the expansion (53)–(55) in Eq. (66) and using the isotropy of the linearized collision operator, only the terms \({\varvec{\phi }}^{D_j}\), \(j\in S\) and \({\varvec{\phi }}^{\widehat{\lambda }}\) yield nonzero contributions—in agreement with the Curie principle—and we obtain that
where
is the unconstrained diffusion driving force of the \(j\)th species and where the transport coefficients are defined by
The coefficients \(D_{ij}\), \(i,j\in S\), are termed the multicomponent diffusion coefficients, the coefficients \(\theta _i\), \(i\in S\), the thermal diffusion or Soret coefficients, and mass diffusion due to temperature gradients is termed the Soret effect. A fundamental property of the multicomponent diffusion coefficients is that they are symmetric since
from the symmetry of the bracket operator. We only consider in these notes such symmetric diffusion coefficients—more interesting both theoretically and numerically—that have been obtained by many authors [9, 11–13, 15, 16, 19]. The symmetric diffusion coefficients have been introduced by Waldmann [11] and used in particular by Chapman and Cowling [15] and Ferziger and Kaper [16]. Following Hirschfelder, Curtiss, and Bird, various authors have considered nonsymmetric coefficients [10] hereby destroying the natural symmetries associated with kinetic processes [13]. But after the remarks of Van de Ree [13], symmetric coefficients have also been used by Curtiss [14]. We also introduce the flux diffusion coefficients
that are such that
We denote by \({\mathsf {y}}\) the mass fraction vector \({\mathsf {y}}= ({\mathsf {y}}_1,\ldots ,{\mathsf {y}}_n)^t\) and by \(\langle \xi ,\zeta \rangle = \sum _{i\in S} \xi _i\zeta _i\) the Euclidean product between two vectors \(\xi = (\xi _1,\ldots ,\xi _n)^t\) and \(\zeta = (\zeta _1,\ldots ,\zeta _n)^t\). Regrouping the diffusion coefficient in a matrix \(D=(D_{ij})_{i,j\in S}\) and the thermal diffusion coefficients in a vector \(\theta = (\theta _1,\ldots ,\theta _n)^t\), we have established that \(D=D^t\) and it is shown in the next section that \(D\) is positive semi-definite with nullspace \({\mathbb {R}}{\mathsf {y}}\) and that \(\langle {\mathsf {y}},\theta \rangle =0\). Taking into account these mass conservation constraints \(D{\mathsf {y}}=0\) and \(\langle {\mathsf {y}},\theta \rangle =0\), instead of using \(\widehat{\varvec{d}}_{i}\), \(i\in S\), it is possible to equivalently use the constrained diffusion driving forces
which sum up to zero [11, 15, 16]. From the constraints \(D{\mathsf {y}}=0\) and \(\langle {\mathsf {y}},\theta \rangle =0\) it is also directly obtained that \(\sum _{i\in S} \varvec{\mathcal {F}}_i = \sum _{i\in S} \rho _i \mathbf {v}_i =0\) independently of the diffusion driving forces and the temperature gradients.
With the definition (65) of the heat flux \(\varvec{\mathcal {Q}}\) and the expression (51) of \({\varvec{\varPsi }}_i^{\widehat{\lambda }}\) one may establish that [9, 19]
Substituting the expansion (53)–(55) in (74) we obtain that
where the transport coefficients are defined by
The coefficient \(\widehat{\lambda }\) is termed the partial thermal conductivity and since
the coefficient \(\theta _i\) is the same in the diffusion velocities and in the heat flux. The diffusion of heat due to concentration gradients in termed the Dufour effect and is reciprocal of the Soret effect.
There are many alternative expressions for the diffusion velocities and the heat flux in a multicomponent mixture [10, 11, 15, 16]. We present here the relations involving the thermal diffusion ratios \(\chi = {(\chi _i)}_{i\in S}\) and the thermal conductivity \(\lambda \) which are interesting both from a mathematical and a computational point of view. The thermal diffusion ratios \(\chi = {(\chi _i)}_{i\in S}\) have been introduced by Waldmann [11] and are defined by
where \({}{1\!\!\!\mathrm {I}}\) is the vector with \(n\) components unity \({}{1\!\!\!\mathrm {I}}=(1)_{i\in S}\), whereas the thermal conductivity is defined by
The coefficients \(\lambda \) and \(\chi \) may also be defined through solutions of integral equations as for the other coefficients [19]. More specifically, letting
we have \(\mathcal {I}_i({\varvec{\phi }}^\lambda ) = {\varvec{\varPsi }}^\lambda \) with the constraints \({\langle \! \langle f^0\phi ^\lambda ,\psi ^l \rangle \! \rangle } =0\) for \(l\in \{1,\ldots , n+4\}\), and
where \(\mathfrak {V}_k = ({\mathfrak m}_i \varvec{C}_{i}\delta _{ki})_{i\in S}\). From these definitions, and after a little algebra, the following alternative expressions for the diffusion velocities and the heat flux are obtained
These alternative formulations are interesting from a computational point of view since it is faster to directly evaluate \(\lambda \) and \(\chi \) rather that to evaluate \(\widehat{\lambda }\) and \(\theta \) [19]. When the Soret and Dufour effects are neglected, that is when \(\chi =0\) and \(\theta =0\), the partial thermal conductivity \(\widehat{\lambda }\) and the traditional thermal conductivity \(\lambda \) then coincide.
Finally, with the relations (48) and (49) and the expression (63) one may establish the following expression for the viscous tensor \(\varvec{\Pi }_{}\)
Keeping in mind the isotropy of the linearized collision operator, the term \({\langle \! \langle \varPsi ^\kappa ,f^0\phi \rangle \! \rangle }\) is evaluated in the form
Defining the volume viscosity by \(\kappa \) by
the reactive pressure by
and the shear viscosity by
it is obtained that
where \(\varvec{\nabla }\varvec{v}+ (\varvec{\nabla }\varvec{v})^t - \frac{2}{3}(\varvec{\nabla }\varvec{\cdot }\varvec{v})\,\varvec{I}\) is the deviatoric part of the rate of strain tensor.
3.4 Properties of Transport Coefficients
The mathematical properties of the transport coefficients may be extracted from the linearized Boltzmann equations [19, 72, 75]. These coefficients satisfy symmetry properties, mass conservation constraints, as well as positivity properties associated with the bracket operator or equivalently with entropy production.
From the definition (69) of the multicomponent transport coefficients \(D_{ij}\) and the symmetry of the bracket operator it has already been obtained that \(D_{ij} = D_{ji}\) and that the thermal diffusion coefficients \(\theta _i\), \(i\in S\), in the diffusion velocities are identical with the coefficients relating the heat flux to the diffusion driving forces. These properties, which may be interpreted as Onsager type relations, are direct consequences of the symmetry of the bracket operator \([ \! [ \ ,\ ] \! ]\), and hence of the reciprocity relations for transition probabilities or collision cross sections.
Concerning the diffusion matrix \(D\), we also have for any \(x\in {\mathbb {R}}^n\)
The matrix \(D\) is thus symmetric positive semi-definite since the bracket operator is positive semi-definite. Moreover, the family of right hand sides \({\varvec{\varPsi }}^{D_1},\ldots ,{\varvec{\varPsi }}^{D_n}\) of the integral equations defining \({\varvec{\phi }}^{D_1},\ldots ,{\varvec{\phi }}^{D_n}\) is easily shown to be of rank \(n-1\) with the constraint \(\sum _{i\in S}{{\mathsf {y}}_{i}}{\varvec{\varPsi }}^{D_{i}}=0\) [19]. By linearity, keeping in mind that  are orthogonal to the nullspace of the linearized collision operator, the family
are orthogonal to the nullspace of the linearized collision operator, the family  is also of rank
is also of rank  and satisfy
and satisfy
Then \(Dx=0\) if and only if \(\langle Dx,x\rangle =0\) which is equivalent to the property that \(\sum _{i\in S} x_i {\varvec{\phi }}^{D_i} =0\) (since by construction all \( {\varvec{\phi }}^{D_i}\) are orthogonal to the collisional invariants), and we obtain that \(Dx=0\) if and only if \(x\) is proportional to the mass fraction vector \({\mathsf {y}}\) and \(N(D) = {\mathbb {R}}{\mathsf {y}}\). We also obtain from the definition of thermal diffusion coefficients \(\theta _i\), \(i\in S\), that \(\sum _{i\in S} {{\mathsf {y}}_{i}} \theta _i = -\tfrac{1}{3} [ \! [ \sum _{i\in S}{{\mathsf {y}}_{i}}{\varvec{\phi }}^{D_i}, {\varvec{\phi }}^{\widehat{\lambda }} ] \! ]=0\) so that \(\langle \theta ,{\mathsf {y}}\rangle =0\).
Similarly, defining the matrix
we observe that for any \(x'=(x,x_0) \in {\mathbb {R}}^{n+1}\) with \(x\in {\mathbb {R}}^n\), \(x_0\in {\mathbb {R}}\), we have
so that \(A\) is positive semi-definite with nullspace spanned by \(({\mathsf {y}},0)^t\). Evaluating then \(\langle Ax', x' \rangle \) for \(x'=(0,\ldots ,0,1)^t\) and \(x'=(-\chi ,1)^t\) it is obtained that \(\widehat{\lambda }>0\) and \(\lambda >0\). The positivity of \(\widehat{\lambda }\) and \(\lambda \) may also directly be deduced from \(\widehat{\lambda }= [ \! [ {\varvec{\phi }}^{\widehat{\lambda }},{\varvec{\phi }}^{\widehat{\lambda }} ] \! ] /3 {k_{\!{\scriptscriptstyle \mathrm{{B}}}}}T^2\) and \(\lambda = \bigl [ \bigl [ {\varvec{\phi }}^\lambda , {\varvec{\phi }}^\lambda \bigr ] \bigr ]/3 {k_{\!{\scriptscriptstyle \mathrm{{B}}}}}T^2\) since neither \({\varvec{\phi }}^\lambda \) nor \({\varvec{\phi }}^{\widehat{\lambda }}\) are collision invariants because neither \({\varvec{\varPsi }}^\lambda \) nor \({\varvec{\varPsi }}^{\widehat{\lambda }}\) are zero.
Finally, we deduce from \(\eta = [ \! [ {\varvec{\phi }}^\eta ,{\varvec{\phi }}^\eta ] \! ]/10 {k_{\!{\scriptscriptstyle \mathrm{{B}}}}}T\) that \(\eta \) is positive and from \(\kappa = [ \! [ \phi ^\kappa ,\phi ^\kappa ] \! ] /9 {k_{\!{\scriptscriptstyle \mathrm{{B}}}}}T\) that \(\kappa \) is nonnegative, and that \(\kappa \) is positive unless \(\varPsi ^\kappa =0\) when there are only monatomic species.
Remark 3.1
The evaluation of the transport coefficients will be discussed in Sect. 4 and the structural properties of the transport coefficients may also be obtained from the transport linear systems [19].
3.5 Perturbed Production Terms
The first order chemical production rates for the \(i\)th species (61) may be written in the form
where \({\mathfrak w}_{i}^0\) is the zeroth order rate \({\mathfrak w}_{i}^0= \sum _{r\in {\mathfrak {R}}} (\nu _{i r}^{\text {b}}-\nu _{i r}^{\text {f}}) \overline{\tau }_{r}\) already discussed in Sect. 2.8 and \({\mathfrak {w}}_i^1\) is the perturbed rate in the dissipative or Navier-Stokes regime
The structure of the perturbed chemical source terms \({\mathfrak {w}}_i^1\) has been investigated in [9]. The perturbed source term is a quadratic expression of the zeroth order rates \(\overline{\tau }_{r}\), \(r\in {\mathfrak {R}}\), plus a linear combination of the same quantities multiplied by the divergence of the velocity field \(\varvec{\nabla }\varvec{\cdot }\varvec{v}\). A few estimates have been made of these perturbed terms in the monoatomic case by Prigogine and Mathieu [20], Prigogine and Xhrouet [21], Present [23], Takanayaki [22], Shizghal and Karplus [26–28] and they are generally believed to be small. In the polyatomic case, however, these perturbed terms may be significant behind shocks, especially with strong thermodynamic nonequilibrium [84]. Nevertheless, we will assume in the following that they are negligible so that
and furthermore that
Note that this is automatic when \(a=1\) in the Boltzmann equations. In this situation, neglecting both the perturbed source terms \({\mathfrak {w}}_i^1\) as well as the chemical pressure \(p^\mathrm{reac}\), we have
and the equations obtained in both regime \(a=0\) and \(a=1\) coincide. These equations will be investigated mathematically in Sects. 5, 6, and 7.
3.6 Thermodynamics
In the framework of Enskog expansion, one may expand the kinetic entropy (13) up to second order in order to obtain that \(\mathcal {S}^{\text {kin}}= \mathcal {S}+ {\mathcal O}(\epsilon ^2)\) and the zeroth order term \(\mathcal {S}\) is the fluid entropy of the mixture
The second order terms \({\mathcal O}(\epsilon ^2)\) have been investigated in [123, 124] but lay out of the scope of the present work. Note that such a thermodynamics obtained in the framework of the kinetic theory of gases is valid out of static equilibrium and has, therefore, a wider range of validity than classical thermodynamics introduced for stationary homogeneous equilibrium states.
Defining the entropy per unit mass of the \(i\)th species by
we may also express the fluid entropy in the form \(\mathcal {S}= \sum _{i\in S} \rho _i s_i\). We may similarly define the Gibbs function per unit mass of the \(i\)th species
and the Gibbs function per unit volume of the mixture \(\mathcal {G}\) is then given by \(\mathcal {G}= \sum _{i\in S} \rho _i g_i\). We also introduce the reduced chemical potential of the \(i\)th species
and the reaction rates of progress may then be rewritten in the convenient form
where \(\nu _i^\mathrm{{f}}= (\nu _{1i}^\mathrm{{f}}, \ldots , \nu _{ni}^\mathrm{{f}})^t\), \(\nu _i^\mathrm{{b}}= ( \nu _{1i}^\mathrm{{b}}, \ldots , \nu _{ni}^\mathrm{{b}})^t\), and \(\mu = (\mu _1, \ldots , \mu _n)^t\).
The balance equation for the macroscopic fluid entropy \(\mathcal {S}\) is obtained in the form
where \(\varvec{\mathcal {F}}_i = \rho _i \mathbf {v}_i\), and is \(\mathfrak {v}\) the entropy production term given by
Using (34) and (98) the entropy production due to chemical reactions reads
so that
Similarly, we have
and
The structure of the chemical source term and of the transport coefficients then guarantee that the two first terms as well as the sum of the two last terms in the expression (100) of entropy production \(\mathfrak {v}\) are nonnegative [9]. The entropy production due to macroscopic gradients may also be written in the form \({k_{\!{\scriptscriptstyle \mathrm{{B}}}}}[ \! [ \phi ^{\mathcal {S}_{}},\phi ^{\mathcal {S}_{}} ] \! ]\) [9]. We recover here the important property that macroscopic gradients as well as chemical production independently lead to nonnegative entropy production at the fluid level as at the molecular level.
3.7 From Molecules to Moles
It is traditional to write macroscopic fluid equations in terms of mass or mole densities rather that number densities and we summarize here the corresponding new notation. Denoting by \({\mathcal {N}}\) the Avogadro number, we define the molar production rate of the \(k\)th species by
and the molar mass by
The number of mole per unit volume is then defined by
and we have the traditional relations \(\rho _k = m_k n_k\). We also define the molar rate of progress \(\tau _r = \overline{\tau }_r/{\mathcal {N}}\) in such a way that \(\omega _k = \sum _{r\in {\mathfrak {R}}} \nu _{kr} \tau _r\) and we also define
The specific heats per unit mole are also given by
as well as
and we also have \(R= {k_{\!{\scriptscriptstyle \mathrm{{B}}}}}{\mathcal {N}}\).
4 Evaluation of Transport Coefficients
The transport fluxes appearing in the conservation equations governing multicomponent flows are expressed in terms of transport coefficients. The fast and accurate evaluation of these coefficients is therefore an important modeling and computational task [65–69].
The transport coefficients are expressed in terms bracket bilinear products involving solutions of systems of integral equations under constraints. These systems of integral equations are generally solved with Galerkin variational approximation procedure and the structure of the resulting transport linear systems may be deduced from the kinetic theory. These linear systems are typically semi-definite systems under constraints. Generalized conjugate gradient algorithms as well as stationary methods are then shown to be convergent using the structural properties obtained from the kinetic theory [70–75].
4.1 Transport Linear Systems
The Chapman-Enskog method requires solving the systems of integral linearized Boltzmann equations with constraints governing the perturbed distribution functions \(\phi ^\mu =(\phi ^\mu _i)_{i\in S}\). These integral equations have been shown to be in the generic form
where \(\mu \in \{\kappa ,\eta ,\widehat{\lambda }\}\cup \{D_1,\ldots ,D_{n}\}\) and the various right hand sides have been evaluated in Sect. 3.3. These systems of integral equations are of matrix type for \(\mu =\eta \), of vector type for \(\mu =\widehat{\lambda }\) or \(\mu \in \{D_1,\ldots ,D_{n}\}\), and of scalar type for \(\mu =\kappa \). The corresponding transport coefficients are then typically obtained through bracket products in the form \(\mu = [ \! [ \phi ^\mu ,\phi ^\mu ] \! ] = {\langle \! \langle f^{(0)}\varPsi ^\mu ,\phi ^\mu \rangle \! \rangle }\) as detailed in Sect. 3.3.
A Galerkin variational approximation procedure is generally used to solve the system of integral Eq. (101). A variational approximation space is first selected
where \(\xi ^{rk}\), \(rk\in \mathcal{B}^\mu \), are basis functions of the same tensorial type than \(\phi ^\mu \) and \(\varPsi ^\mu \). The set \(\mathcal{B}^\mu \) is the basis indexing set and \(\mathcal{B}^\mu \subset \mathcal {F}\times S\) where \(\mathcal {F}\) is the indexing set of function type and \(S\) the species indexing set, that is, when \(rk\in \mathcal{B}^\mu \) then \(r\in \mathcal {F}\) and \(k\in S\). We denote by  the dimension of \(\varXi ^\mu \), that is
the dimension of \(\varXi ^\mu \), that is  . The unknown \(\phi ^\mu \) is then expanded in the form
. The unknown \(\phi ^\mu \) is then expanded in the form
and the variational equations read \({\langle \! \langle f^{(0)} \xi ^{rk}, \mathcal {I}(\phi ^\mu ) \rangle \! \rangle } = {\langle \! \langle f^{(0)} \xi ^{rk}, \varPsi ^\mu \rangle \! \rangle }\) for \(rk\in \mathcal{B}^\mu \). Letting \(G^{rs}_{kl} = {\langle \! \langle f^{(0)} \xi ^{rk}, \mathcal {I}(\xi ^{sl}) \rangle \! \rangle } = [ \! [ \xi ^{rk}, \xi ^{sl} ] \! ]\), and \(\beta ^{r\mu }_k = {\langle \! \langle f^{(0)} \xi ^{rk}, \varPsi ^\mu \rangle \! \rangle }\) we have obtained the linear system
The linear constraints \({\langle \! \langle f^{(0)} \phi ^\mu , \psi ^l \rangle \! \rangle }=0\) are also rewritten as
where \(\mathcal {G}^{rl\nu }_k = {\langle \! \langle f^{(0)} \xi ^{rk}, \mathcal {T}_\nu \psi ^l \rangle \! \rangle }\) and \(\mathcal {T}_\nu \) denotes the canonical basis for tensor of type \(\varPsi ^\mu \) and \(\phi ^\mu \).
Defining now the constrained space by
where \(\mathcal {G}^{l\nu }=(\mathcal {G}^{rl\nu }_k )_{rk\in \mathcal{B}^\mu }\), the vectors \(\alpha ^\mu =(\alpha ^{r\mu }_k)_{rk\in \mathcal{B}^\mu }\) and \(\beta ^\mu =(\beta ^{r\mu }_k)_{rk\in \mathcal{B}^\mu }\), and the matrix \(G\) by \(G = \bigl ( G^{rs}_{kl} \bigr )_{rk,sl\in \mathcal{B}^\mu }\), the transport linear system is in the form
and the bracket \(\mu = [ \! [ \phi ^\mu ,\phi ^\mu ] \! ] = {\langle \! \langle f^{(0)}\varPsi ^\mu ,\phi ^\mu \rangle \! \rangle }\) is typically obtained with a scalar product
We note then that the matrix \(G\) of the transport linear system is symmetric since
and positive semi-definite from \(\langle Gx,x\rangle = [ \! [ \xi ,\xi ] \! ]\) where \(\xi = \sum _{rk\in \mathcal{B}^\mu } x^r_k \xi ^{rk}\). Moreover, the nullspace of \(G\) is directly associated with the collisional invariants of the same tensorial type that \(\varPsi ^\mu \) and \(\phi ^\mu \) that are in the variational space \(\varXi ^\mu \). One may further establish that \(\beta \in R(G)\) is in the range of \(G\) using that \(\varPsi ^\mu \) is orthogonal to the collisional invariants [19]. Throughout these notes, for any matrix \(A\), we denote by \(N(A)\) its nullspace and \(R(A)\) its range. Symmetric transport linear systems have been considered by many authors [11, 12, 15, 16]. Nevertheless, following Hirschfelder, Curtiss, and Bird, various authors have considered nonsymmetric transport linear systems [10] hereby again destroying the natural symmetries associated with kinetic processes. The explicit calculation of symmetric transport linear systems for mixtures of polyatomic species has first been performed in [19].
Denoting by \(\mathfrak {I}^\mu \) these collisional invariants of the same tensorial type than \(\varPsi ^\mu \) and \(\phi ^\mu \), we assume that the following perpendicularity property holds
where the orthogonal \((\varXi ^\mu )^\perp \) of the variational approximation space \(\varXi ^\mu \) is taken with respect to the scalar product \({\langle \! \langle f^0\xi ,\zeta \rangle \! \rangle }\). In this situation, one may establish [19] that that the well posedness condition holds

We further introduce the sparse transport matrix [19]
and one may establish that when
that is, when the basis functions are orthogonal to the constant collisional invariants \(\psi ^i\), \(i\in S\), then for \(x=(x^r_k)_{rk\in \mathcal{B}^\mu }\) we have
where \(\xi = \sum _{rk\in \mathcal{B}^\mu } x^r_k \xi ^{rk}\) and the superscript \(\tilde{}\) is used to distinguish the collision partners when \(i=j\). In comparison, it is interesting to note that
in such a way that
where \(\xi = \sum _{rk\in \mathcal{B}^\mu } x^r_k \xi ^{rk}\) and \(\mathfrak {I}_{ij}\) denotes the collision invariants for the species pair \((i,j)\), whereas
Using these properties,and since the only linear subspaces of collisional invariants that may lay in the variational approximation spaces \(\varXi ^\mu \) are at most one-dimensional, being either proportional to \(({\mathfrak m}_i \varvec{C}_{i})_{i\in S}\) in the vector case or \((\tfrac{1}{2}{\mathfrak m}_i \vert \varvec{C}_{i}\vert ^2 + E_{i{\textsc {i}}})_{i\in S}\) in the scalar case, the invariant \(\psi ^i\), \(i\in S\) being excluded from (112), it is established that when there are at least \(n\ge 3\) species, then \(2db(G)-G\) is positive definite [19]. In this situation, the matrix \(db(G)\), which is easily invertible, is also positive definite [19].
We have thus established that when the perpendicularity property (109) holds, and when the variational space \(\varXi ^\mu \) is orthogonal to constants (112), then \(G\) is symmetric positive semi-definite, the well posedness condition  holds, \(\beta \in R(G)\), and \(2db(G)-G\) and \(db(G)\) are positive definite when \(n\ge 3\). In the special cases \(n=1\) or \(n=2\) the corresponding nullspaces are explicitely evaluated. Various variational approximation spaces may also be used as reduced spaces [19] or spaces for a direct evaluation of the thermal conductivity and the thermal diffusion ratios [71].
holds, \(\beta \in R(G)\), and \(2db(G)-G\) and \(db(G)\) are positive definite when \(n\ge 3\). In the special cases \(n=1\) or \(n=2\) the corresponding nullspaces are explicitely evaluated. Various variational approximation spaces may also be used as reduced spaces [19] or spaces for a direct evaluation of the thermal conductivity and the thermal diffusion ratios [71].
4.2 Transport Algorithms
The transport linear systems have been obtained in their natural symmetric form for most useful transport coefficients [11, 12, 15, 16, 19]. These linear system associated with any coefficient \(\mu \) then take on either a regular form or a singular form [9, 19]. Only the later singular form is discussed here since the regular case is easier to treat. The singular form can be written in the form
where  ,
,  ,
,  is the dimension of the variational space and the coefficient is obtained with a scalar product \(\mu = \langle \alpha ,\beta '\rangle \) [16, 19]. In other words, in practice, the constrained subspace is found to be one dimensional so that \(\mathcal {C} = {\mathcal G}^\perp \). The matrix \(G\) is symmetric positive semi-definite, its nullspace is one dimensional \(N(G) = {\mathbb {R}}{\mathcal N}\), \(\beta \in R(G)\), and the well posedness condition
is the dimension of the variational space and the coefficient is obtained with a scalar product \(\mu = \langle \alpha ,\beta '\rangle \) [16, 19]. In other words, in practice, the constrained subspace is found to be one dimensional so that \(\mathcal {C} = {\mathcal G}^\perp \). The matrix \(G\) is symmetric positive semi-definite, its nullspace is one dimensional \(N(G) = {\mathbb {R}}{\mathcal N}\), \(\beta \in R(G)\), and the well posedness condition  holds [19]. The sparse transport matrix \(db(G)\) is a submatrix [19] composed of diagonals of blocks of \(G\), and \(2db(G) - G\) and \(db(G)\) are symmetric positive definite for \(n\ge 3\). All these properties have been extracted from the properties of the Boltzmann linearized collision operator and that of the variational approximation spaces [19].
holds [19]. The sparse transport matrix \(db(G)\) is a submatrix [19] composed of diagonals of blocks of \(G\), and \(2db(G) - G\) and \(db(G)\) are symmetric positive definite for \(n\ge 3\). All these properties have been extracted from the properties of the Boltzmann linearized collision operator and that of the variational approximation spaces [19].
The solution of the transport linear system can then be obtained either from the symmetric positive definite system \((G + {\mathcal G} \otimes {\mathcal G})\alpha =\beta \) or from iterative techniques. Among iterative techniques, we may use generalized conjugate gradients algorithms that are possible because the natural symmetries of transport processes have been taken into account. A very good preconditioner is then the sparse transport matrix \(db(G)\).
Stationary techniques are also feasible and are associated with a splitting \(G = {\mathcal {M}}- {\mathcal {Z}}\) where \({\mathcal {M}}=db(G)\). These methods yields that
where \( {\mathcal {T}}= {\mathcal {M}}^{-1}{\mathcal {Z}}\) and \({\mathcal {P}}= I - {\mathcal N}{\otimes }{\mathcal G} /\langle {\mathcal N},{\mathcal G}\rangle \). It can then be shown that the spectral radius of the product \({\mathcal {P}}{\mathcal {T}}\) is strictly lower than unity since the matrix \({\mathcal {M}}+{\mathcal {Z}}= 2db(G) - G\) is positive definite but this is a consequence of Boltzmann linearized equations. These stationary and generalized conjugate gradients methods have been found to be efficient for mixture of neutral gases [7, 70–74].
The situation of ionized mixtures is more complex since the convergence rate of stationary iterative techniques deteriorate as the ionization level increases. On the contrary, the convergence properties of generalized conjugate gradient algorithms do not depend on the ionization level. New algorithms have thus been introduced with more singular versions of the transport linear systems. These algorithms have led to fast convergence rates for all ionization levels and magnetic field intensities [75].
The assumptions for transport coefficients when some mass fractions are vanishing are more complex and lay out of the scope of these notes [9, 19]. Zero mass fractions lead to artificial singularities in the transport linear systems which are eliminated by considering rescaled systems [19]. Provided the diffusion matrix is replaced by the flux diffusion matrix \(C_{kl} = \rho {\mathsf {y}}_k D_{kl}\), \(k,l\in S\), all transport coefficients are smooth functions of the mass fractions and admit finite limits when some mass fractions become arbitrarily small. The iterative algorithms obtained for positive mass fractions can also be rewritten in terms of rescaled systems that are still defined for nonnegative mass fractions [19].
4.3 Stefan-Maxwell Equations
As a typical illustration of transport linear systems, we discuss in this section the multicomponent diffusion matrix \(D=(D_{ij})_{i,j\in S}\). We assume that a state of the mixture is given with \(T>0\), \(p>0\), and \({\mathsf {y}}>0\), that is, \({\mathsf {y}}_k>0\) for \(k\in S\). We assume that the mass fraction sum up to unity \(\langle {\mathsf {y}},{}{1\!\!\!\mathrm {I}}\rangle =1\) and the mole fractions are denoted by \(\mathsf {x}_1,\ldots ,\mathsf {x}_n\). The mole fractions may be evaluated from \(\mathsf {x}_i = m {{\mathsf {y}}_{i}}/m_i\) where \(m\) is the mean molar weight given by \(\langle {\mathsf {y}},{}{1\!\!\!\mathrm {I}}\rangle /m = \sum _{i\in S} {{\mathsf {y}}_{i}}/m_i\).
The usual diffusion matrix \(D\), obtained with linear systems of size  , satisfy \(R(D)\subset {\mathsf {y}}^\perp \) and
, satisfy \(R(D)\subset {\mathsf {y}}^\perp \) and
where \(I_n\) is the identity of size \(n\) and \(\varDelta \) the Stefan-Maxwell matrix [16, 19]. Diffusion coefficients associated with larger transport linear systems—required for plasmas—lay out of the scope of these notes [19, 75]. The matrix \(\varDelta \) reads
where \(\mathcal{D}^\mathrm{{bin}}_{kl}(T,p)\) is the binary diffusion coefficient of the species pair \((k,l)\) depending on pressure and temperature. The structure of the matrix \(\varDelta \) is investigated in the following Lemma [9, 70]
Lemma 4.1
Assume that the molar masses \(m_k\), \(k\in S\), are positive constants, that the coefficients \(\mathcal{D}^\mathrm{{bin}}_{kl}\), \(k,l\in S\), \(k\ne l\), are positive and symmetric, and that \({\mathsf {y}}>0\). Then \(\varDelta \) is symmetric positive semidefinite, \(N(\varDelta )={\mathbb {R}}\, {}{1\!\!\!\mathrm {I}}\), \(\varDelta \) is irreducible and is a singular M-matrix.
We will need the following lemma about generalized inverses with prescribed range and nullspace that may be found in [9, 70].
Proposition 4.2
Let  be a matrix, and let \(\mathfrak {p}\) and \(\mathfrak {q}\) be two subspaces of
be a matrix, and let \(\mathfrak {p}\) and \(\mathfrak {q}\) be two subspaces of  such that
such that  and
and  . Then, there exists a unique matrix \(Z\) such that \(GZG= G\), \(ZGZ= Z\), \(N(Z) = \mathfrak {q}\), and \(R(Z) = \mathfrak {p}\). The matrix \(Z\)—termed the generalized inverse of \(G\) with prescribed range \(\mathfrak {p}\) and nullspace \(\mathfrak {q}\)—satisfies \(GZ= P_{R(G), \mathfrak {q}}\) and \(ZG= P_{\mathfrak {p}, N(G)}\), where \(P_{\mathfrak {a},\mathfrak {b}}\) is defined for linear spaces \(\mathfrak {a}\) and \(\mathfrak {b}\), such that
. Then, there exists a unique matrix \(Z\) such that \(GZG= G\), \(ZGZ= Z\), \(N(Z) = \mathfrak {q}\), and \(R(Z) = \mathfrak {p}\). The matrix \(Z\)—termed the generalized inverse of \(G\) with prescribed range \(\mathfrak {p}\) and nullspace \(\mathfrak {q}\)—satisfies \(GZ= P_{R(G), \mathfrak {q}}\) and \(ZG= P_{\mathfrak {p}, N(G)}\), where \(P_{\mathfrak {a},\mathfrak {b}}\) is defined for linear spaces \(\mathfrak {a}\) and \(\mathfrak {b}\), such that  , and denotes the projector onto \(\mathfrak {a}\) along \(\mathfrak {b}\).
, and denotes the projector onto \(\mathfrak {a}\) along \(\mathfrak {b}\).
If in addition \(G\) is symmetric positive semi-definite and \(\mathfrak {p}=\mathfrak {q}^\perp \) then \(Z\) is symmetric positive semi-definite.
The diffusion matrix may then be defined as a generalized inverse of \(\varDelta \) with prescribed range and nullspace [9, 19, 70].
Proposition 4.3
Keeping the assumptions of Lemma 4.1, there exists a unique matrix \(D\) such that \(\varDelta D = I_n- {\mathsf {y}}{\otimes }{}{1\!\!\!\mathrm {I}}\) and \(R(D)\subset {\mathsf {y}}^\perp \). This matrix \(D\) is the generalized inverse of \(\varDelta \) with prescribed range \({\mathsf {y}}^\perp \) and nullspace \({\mathbb {R}}{\mathsf {y}}\). The matrix \(D\) is symmetric positive semidefinite, \(N(D)={\mathbb {R}}{\mathsf {y}}\), \(D\) is irreductible, and for any \(a>0\) we have \(D=(\varDelta +a{\mathsf {y}}{\otimes }{\mathsf {y}})^{-1} \,-\, (1/a) {}{1\!\!\!\mathrm {I}}{\otimes }{}{1\!\!\!\mathrm {I}}\).
The transport linear systems associated with the flux matrix \(C\) are of a similar nature [19, 70, 74] and are well posed for \({\mathsf {y}}\ge 0\), \({\mathsf {y}}\ne 0\). With the mathematical properties of the matrix \(C\) it is then possible to establish the following diffusion inequality involving the entropy production quadratic form \(\langle Dx,x \rangle \) on the hyperplane of zero sum gradients [9, 119].
Proposition 4.4
Let \(T\) be a fixed temperature and \(\mathcal{Y}=\mathrm {diag}({\mathsf {y}}_1,\ldots ,{\mathsf {y}}_n)\). There exists a positive constant \(\delta \) such that
In other words, the natural entropic production norm associated with diffusion processes involve expressions in the form \(\sum _{i\in S} \vert \varvec{\nabla }{\mathsf {y}}_i\vert ^2/{\mathsf {y}}_i\). Such norms have first been used for an existence theorem of traveling waves with complex chemistry and detailed transport [119]. This lemma also implies that the nonzero eigenvalues of \(\mathcal{Y}^{1/2}\rho D \mathcal{Y}^{1/2}\) are bounded away form zero since \(\mathcal{Y}^{1/2} \rho D \mathcal{Y}^{1/2} x = \lambda x\) with \(\lambda \ne 0\) implies that \(x\in R(\mathcal{Y}^{1/2} D) = ({\mathsf {y}}^{1/2})^\perp \) and \(\sum _{i\in S} {\mathsf {y}}^{1/2} x_i=0\) and from (118) that \(\delta \le \lambda \).
One can also derive from (115) to (117) after some algebra that for any \(k \in S\)
These equations are usually termed Stefan–Maxwell equations and must be completed by the constraint \(\sum _{k \in S} {{\mathsf {y}}_{k}} \mathbf {v}_k = 0\) associated with mass conservation to define uniquely the diffusion velocities. An elementary derivation of these equations has been given by Williams [66].
During a multicomponent flow computation, when an explicit time algorithm is used, it is sufficient to solely evaluate the diffusion velocities \(\mathbf {v}_k\), \(k\in S\), by solving the Stefan-Maxwell equations for each spatial direction, say by using a projected conjugate gradient method [74]. However, when an implicit time marching technique is used, evaluating the diffusion matrix \(D\) is generally required. Accurate approximation of the diffusion matrix \(D\) may be obtained by considering \({\mathcal {M}}= \mathrm {diag}({\mathsf {x}_{1}}/D^*_1,\ldots ,{\mathsf {x}_{n}}/D^*_n)\) where
and \(\varDelta ={\mathcal {M}}- {\mathcal {Z}}\), \({\mathcal {T}}= {\mathcal {M}}^{-1}{\mathcal {Z}}\), and \({\mathcal {P}}= I_n- {}{1\!\!\!\mathrm {I}}{\otimes }{\mathsf {y}}\). The spectral radius of \({\mathcal {P}}{\mathcal {T}}\) is then strictly lower than unity and we have the convergent series expansion [70]
One may then introduce the approximate diffusion matrices
that are symmetric, satisfy the mass constraint \(D^{[i]} {\mathsf {y}}= 0\) and yields a positive entropy production. The first approximation \(D^{[0]} = {\mathcal {P}}{\mathcal {M}}^{-1} {\mathcal {P}}^t\) corresponds to the Curtiss–Hirschfelder approximation \(\mathbf {v}_k = - D^*_k\varvec{d}_k/\mathsf {x}_k + \mathbf {v}_\mathrm{cor}\) with a mass corrector \(\mathbf {v}_\mathrm{cor}\) ensuring the constraint \(\sum _{k\in S} {{\mathsf {y}}_{k}} \mathbf {v}_k =0\) and arising here from the projector \({\mathcal {P}}\) [19, 70].
4.4 Impact of Multicomponent Transport
Recent numerical investigations have brought further support for the importance of accurate transport property in various multicomponent reactive flows [76–85]. Thermal diffusion effects have been shown to be important in the study of vortex-flame interaction, catalytic effects near walls, interfacial phenomena, gaseous or spray diffusion flames [83], chemical vapor deposition reactors [3] and reentry [85]. The impact of multicomponent diffusion has also been shown to be important in multidimensional hydrogen/air and methane/air Bunsen flames [7], in freely propagating flames—especially with oxygen as pure oxydizer—as well as in direct numerical simulation of turbulent flames.
Theoretical calculations and experimental measurements have also shown that the ratio \(\kappa /\eta \) is not small for polyatomic gases [9, 80–82, 84]. Volume viscosity also arises in dense gases and in liquids, and its absence in dilute monatomic gases is an exception rather than a rule. Despite its potential importance, volume or bulk viscosity has seldom been included in computational models of multidimensional reactive flows. For small Mach number flows, however, the whole term \(\varvec{\nabla }{\cdot }\bigl (\kappa (\varvec{\nabla }{\cdot }\varvec{v}) \varvec{I}\bigr )\) has a weak influence because of its structure, even though both the ratio \(\kappa /\eta \) and the dilatation \(\varvec{\nabla }{\cdot }\varvec{v}\) may not be small [9]. However, it has been shown that volume viscosity has an important impact during a shock/hydrogen bubble interaction [82] and its influence on shock heated and expanding flows in investigated in [81, 84].
5 Mathematical Framework
We first summarize in this section the system of partial differential equations modeling reactive fluids derived from the kinetic theory of gases in the previous sections. We also introduce a mathematical framework, notably the assumptions on the transport coefficients, and recast the system in quasilinear form. The precise form of the thermodynamic functions obtained in the previous sections is not specifically required and they are simply defined here in terms of internal specific heats and formation constants. Furthermore the mathematical properties of the transport coefficients are extracted from the kinetic theory. Note that we only investigate here the equations governing ideal mixture of perfect gases derived from the kinetic theory and we refer to [109, 111, 112, 114, 115] for other models. We have often rewritten some of the relations deduced from the kinetic theory in the previous sections in order to facilitate an independent lecture of the following sections.
5.1 Conservation Equations
The equations for conservation of species mass, momentum and energy may be recast in the form [9]
where \(\partial _t\) denotes the time derivative, \(\varvec{\nabla }\) the space derivative operator, \(\rho _k\) the mass density of the \(k\)th species, \(\varvec{v}\) the mass average flow velocity, \(\varvec{\mathcal {F}}_k\) the diffusion flux of the \(k\)th species, \(m_k\) the molar mass of the \(k\)th species, \(\omega _k\) the molar production rate of the \(k\)th species, \(S=\{1,\ldots ,n\}\) the set of species indices, \(n\geqslant 1\) the number of species, \(\rho =\sum _{k\in S} \rho _k\) the total mass density, \(p\) the pressure, \(\varvec{\Pi }\) the viscous tensor, \(\varvec{b}_i\) the force per unit mass acting on the \(i\)th species, \({\mathcal E}\) the internal energy per unit volume and \({\varvec{Q}}\) the heat flux. These equations have to be completed by the relations expressing the thermodynamic properties like \(p\) and \({\mathcal E}\), the chemical production rates \(\omega _k\), \(k\in S\), and the transport fluxes \(\varvec{\Pi }\), \(\varvec{\mathcal {F}}_k\), \(k\in S\), and \({\varvec{Q}}\).
Assuming that the force acting on the chemical species are species independent \(\varvec{b}_i=\varvec{b}\), \(i\in S\), as gravity for instance, then the energy production term \(\sum _{i\in S} \rho _i \varvec{b}_i\varvec{\cdot }(\varvec{v}+\mathbf {v}_i)\) simplifies into \(\rho \varvec{b}\varvec{\cdot }\varvec{v}\). In the following, we will assume that there is no force acting on the chemical species so that
Such zeroth order force terms \(\rho \varvec{b}\) and \(\rho \varvec{b}\varvec{\cdot }\varvec{v}\) do not significantly influence the mathematical structure of the resulting set of partial differential equations. The spatial dimension is denoted by \(d\) and the components of \(\varvec{v}\) and \(\varvec{\nabla }\) are written \(\varvec{v}= (v_1,\ldots ,v_d)^t\) and \(\varvec{\nabla }= (\partial _1,\ldots ,\partial _d)^t\) where \(v_i\) denotes the velocity in the \(i\)th spatial direction, \(\partial _i^{}\) the derivation in the \(i\)th spatial direction and bold symbols are used for vector or tensor quantities in the physical space \({\mathbb {R}}^d\).
5.2 Thermodynamics
We will use for convenience the state variable \(T,\rho _1,\ldots ,\rho _n\) where \(T\) is the absolute temperature and also denote \(\varrho =(\rho _1,\ldots ,\rho _n)^t\). Other state variables could be used as well and may lead to slightly different mathematical formalisms [9]. The internal energy per unit volume \({\mathcal E}\) and the pressure \(p\) can be written in terms of the state variables \(T,\rho _1,\ldots ,\rho _n\) as
where \(e_k\) is the internal energy per unit mass of the \(k\)th species and \(R\) the gas constant. The internal energy \(e_k\) of the \(k\)th species is given by
where \(e_k^\mathrm{st}\) is the standard formation energy of the \(k\)th species at the standard temperature \(T^\mathrm{st}\) and \(c_{\mathrm{{v}}k}\) the constant volume specific heat of the \(k\)th species. We also define the formation energy at zero temperature by letting \(e_k^0 = e_k(0) = e_k^\mathrm{st}- \int _0^{T^\mathrm{st}} \!\!\! c_{\mathrm{{v}}k}(\tau )\, d\tau \). The (physical) entropy per unit volume \({\mathcal S}\) can be written in the form
where \(s_k\) is the entropy per unit mass of the \(k\)th species. This quantity is in the form
where \(s_k^\mathrm{st}\) is the formation entropy of the \(k\)th species at the standard temperature \(T^\mathrm{st}\) and standard pressure \(p^\mathrm{st}= p^\mathrm{atm}\) and \(\gamma ^\mathrm{st}= p^\mathrm{st}/ RT^\mathrm{st}\) is the standard concentration. Similarly, one can introduce the mixture enthalpy \({\mathcal H} = \sum _{k\in S} \rho _k h_k(T)\) with
the mixture Gibbs function per unit volume \({\mathcal G} = \sum _{k\in S} \rho _k g_k(T,\rho _k)\), with
as well as the reduced chemical potential
Finally, the species mass fractions \({\mathsf {y}}_k\), \(k\in S\), partial pressures \(p_k\), \(k\in S\), and mole fractions \(\mathsf {x}_k \), \(k\in S\), are defined by
The mole fractions may also be evaluated from \({\mathsf {x}_{i}} = m {{\mathsf {y}}_{i}}/m_i\) where \(m\) is the mean molar weight given by \(\langle {\mathsf {y}},{}{1\!\!\!\mathrm {I}}\rangle /m = \sum _{i\in S} {{\mathsf {y}}_{i}}/m_i\).
5.3 Chemical Sources
We consider a system of \(n^{\mathrm{r}}\geqslant 1\) elementary reactions for \(n\geqslant 1\) species which can be written formally
where \({\mathfrak M}_k\) is the chemical symbol of the \(k\)th species, \(\nu _{ki}^\mathrm{{f}}\) and \(\nu _{ki}^\mathrm{{b}}\) the forward and backward stoichiometric coefficients of the \(k\)th species in the \(i\)th reaction, \({\mathfrak {R}}=\{ 1,\ldots ,n^{\mathrm{r}}\}\) the set of reaction indices, and \(\nu _{ki} = \nu _{ki}^\mathrm{{b}}-\nu _{ki}^\mathrm{{f}}\) the overall stoichiometric coefficients. The species of the mixture are assumed to be constituted by atoms, and we denote by \({\mathfrak {a}}_{il}\) the number of \(l\)th atom in the \(i\)th species, \({\mathfrak {A}}=\{1,\ldots ,n^{\mathrm{a}}\}\) the set of atom indices, and \(n^{\mathrm{a}}\geqslant 1\) the number of atoms—or elements—in the mixture. It is convenient to introduce at this point some vector notation by letting

We will denote by \(\mathcal {R}\) the vector space spanned by the reaction vectors \({\mathcal R}= \mathrm {Span}\{\, \nu _i, \ i\in {\mathfrak {R}}\, \}\) and by \({\mathcal A}\) the vector space spanned by the atom vectors \({\mathcal A}= \mathrm {Span}\{\, {\mathfrak {a}}_l, \ l\in {\mathfrak {A}}\, \}\). The molar production rates that we consider are the Maxwellian production rates obtained from the kinetic theory [9, 19] when the chemical characteristic times are larger than the mean free times of the molecules and the characteristic times of internal energy relaxation. These rates \(\omega _k\), \(k\in S\), are compatible with the law of mass action and are in the form
where \(\tau _i\) is the rate of progress of the \(i\)th reaction given by
as written by Marcelin [57], Gorban [63] and Keizer [64] and also deduced from the kinetic theory (98). These rates of progress are compatible with the law of mass action and may be rewritten
where \({\mathcal K}_i^\mathrm{{f}}\) and \({\mathcal K}_i^\mathrm{{b}}\) are the forward and backward rate constants of the \(i\)th reaction, respectively. The reaction constants \({\mathcal K}_i^\mathrm{{f}}\), \({\mathcal K}_i^\mathrm{{b}}\) and \(\mathcal{K}_i^\mathrm{{s}}\) are functions of temperature and are Maxwellian averaged values of molecular chemical transition probabilities [19]. In particular, forward and backward chemical transition probabilities are always proportional—as in any Boltzmann equation—and this implies the reciprocity relations [9, 19]
where \({\mathcal K}_i^\mathrm{e}(T) \) is the equilibrium constant of the \(i\)th reaction, \(\mu ^\mathrm{u} = (\mu ^\mathrm{u}_1,\ldots ,\mu ^\mathrm{u}_n)^t\) and \(\mu _k^\mathrm{u}(T) = \mu _k(T,m_k)\), \(k\in S\). These reciprocity relations are closely associated with the reciprocity relations between reactive transition probabilities as well as with symmetric representation of the rate of progress and may be seen as Onsager relations for chemistry. These reactions constants are also related by \(\log {\mathcal K}_i^\mathrm{{s}}= \log {\mathcal K}_i^\mathrm{{f}}- \langle M \nu _i^\mathrm{{f}}, \mu ^\mathrm{u} \rangle = \log {\mathcal K}_i^\mathrm{{b}}- \langle M \nu _i^\mathrm{{b}}, \mu ^\mathrm{u} \rangle \). On the other hand, in practice, the forward reaction constants \({\mathcal K}_i^\mathrm{{f}}\), \(i\in {\mathfrak {R}}\), are often approximated with Arrhenius law
where \(\mathfrak {A}_i\) is the preexponential factor, \(\mathfrak {b}_i\) the temperature exponent and \(\mathfrak {E}_i\) the activation energy of the \(i\)th reaction. Note incidentally that the thermodynamics of irreversible processes only yields rates of progress that are linear in terms of affinities \(\langle \nu _i, \mu \rangle \) instead of exponential as in (126).
5.4 Transport Fluxes
The transport fluxes \(\varvec{\Pi }\), \(\varvec{\mathcal {F}}_k\), \(k\in S\), and \({\varvec{Q}}\) due to macroscopic variable gradients can be written in the form [9, 11, 12, 15, 16, 19]
where \(\kappa \) denotes the volume viscosity, \(\eta \) the shear viscosity, \(\varvec{I}\) the three dimensional identity tensor, \(C_{kl}\), \(k,l\in S\), the multicomponent flux diffusion coefficients, \(\widehat{\varvec{d}}_{k}\), \(k\in S\), the unconstrained species diffusion driving forces, \({}^t\) the transposition operator, \({\widetilde{\chi }}_k\), \(k\in S\), the rescaled thermal diffusion ratios and \(\lambda \) the thermal conductivity. The diffusion driving forces are defined by
keeping in mind that the force term acting on the species are assumed to be zero \(\varvec{b}_k=0\), \(k\in S\). When the mass fractions are nonzero, it is also possible to define the species diffusion velocities \(\mathbf {v}_k\), \(k\in S\), by
where \(D_{kl} = C_{kl}/\rho _k\), \(k,l\in S\).
The transport coefficients have important properties inherited from the underlying kinetic framework [9, 12, 19]. They satisfy symmetry properties, mass conservation constraints, as well as positivity properties as derived in Sect. 3.4 and detailed in the next section. The multicomponent transport coefficients \(\kappa \), \(\eta \), \(\lambda \), \(C=(C_{kl})_{k,l\in S}\), \(D=(D_{kl})_{k,l\in S}\), or \({\widetilde{\chi }}=({\widetilde{\chi }}_k)_{k\in S}\), are also smooth functions of the state variables. Note that the matrices \(C\) and \(D\) are generally irreducible and the governing equations have thus a complex structure [9].
5.5 Mathematical Assumptions
The assumptions on the thermodynamic properties and the transport coefficients have been extracted from the kinetic theory of gases. There are recast in the following form where \(\varkappa \) denotes a regularity class of transport coefficients and thermodynamic functions [9]. Throughout these notes, for any matrix \(A\), we denote by \(N(A)\) its nullspace and \(R(A)\) its range.
- \(\mathsf{(H }_\mathsf{1 }\mathsf ) \) :
-
The molar masses \(m_k\), \(k\in S\), and the perfect gas constant \(R\) are positive constants. The formation energies \(e_k^\mathrm{st}\), \(k\in S\), and entropies \(s_k^\mathrm{st}\), \(k\in S\), are real constants. The specific heats \(c_{\mathrm{{v}}k}\), \(k\in S\), are \(C^{\varkappa -1}\) functions of \(T\in [0,\infty )\). There exist positive constants \({\underline{c}}_\mathrm{{v}}\) and \({\overline{c}}_\mathrm{{v}}\) such that \(0 < {\underline{c}}_\mathrm{{v}}\, \leqslant \, c_{\mathrm{{v}}k}(T) \, \leqslant \, {\overline{c}}_\mathrm{{v}}\) for \(T\ge 0\) and \(k\in S\).
- \(\mathsf{(H }_\mathsf{2 }\mathsf ) \) :
-
The stoichiometric coefficients \(\nu _{ki}^\mathrm{{f}}\) and \(\nu _{ki}^\mathrm{{b}}\), \(k\in S\), \(i\in {\mathfrak {R}}\), the atomic coefficients \({\mathfrak {a}}_{kl}\), \(k\in S\), \(l\in {\mathfrak {A}}\), are nonnegative integers. The atom vectors \({\mathfrak {a}}_l\), \(l\in {\mathfrak {A}}\), and the reaction vectors \(\nu _i=\nu _i^\mathrm{{b}}- \nu _i^\mathrm{{f}}\), \(i\in {\mathfrak {R}}\), satisfy the atom conservation constraints \(\langle \nu _i, {\mathfrak {a}}_l \rangle =0\), \(i\in {\mathfrak {R}}\), \(l\in {\mathfrak {A}}\). The atom masses \({\widetilde{m}}_l\), \(l\in {\mathfrak {A}}\), are positive constants and the vector of species molar masses
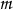 is given by
is given by
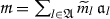 .
. - \(\mathsf{(H }_\mathsf{3 }\mathsf ) \) :
-
The symmetric reaction constants \({\mathcal {K}}_i^{\mathrm{{s}}}\) are \(C^\varkappa \) positive functions of \(T>0\) for \(i\in {\mathfrak {R}}\).
- \(\mathsf{(H }_{\mathsf{4 }}\mathsf ) \) :
-
The flux diffusion matrix \(C=(C_{kl})_{k,l\in S}\), the rescaled thermal diffusion ratios vector \({\widetilde{\chi }} = ({\widetilde{\chi }}_1,\ldots ,{\widetilde{\chi }}_n)^t\), the volume viscosity \(\kappa \), the shear viscosity \(\eta \), and the thermal conductivity \(\lambda \) are \(C^\varkappa \) functions of \((T,\rho _1,\ldots ,\rho _n)\) for \(T >0\) and \(\rho _i>0\), \(i\in S\). These coefficients satisfy the mass conservation constraints \(N(C) = {\mathbb {R}}{\mathsf {y}}\), \(R(C) = {}{1\!\!\!\mathrm {I}}^\perp \), and \({\widetilde{\chi }}\in \mathsf {x}^\perp \).
- \(\mathsf (H _\mathsf{5 }\mathsf ) \) :
-
The thermal conductivity \(\lambda \) and the shear viscosity \(\eta \) are positive. The volume viscosity \(\kappa \) is nonnegative. The diffusion matrix \(D = (1/\rho ) {\mathcal {Y}}^{-1} C\) is symmetric positive semi-definite and its nullspace is \(N(D)= {\mathbb {R}}{\mathsf {y}}\) where \(\mathcal {Y} = \mathrm {diag}({\mathsf {y}}_1,\ldots ,{\mathsf {y}}_n)\).
Remark 5.1
All coefficients \(C\), \(\lambda \), \(\eta \), \({\widetilde{\chi }}\) and \(\kappa \) have smooth extensions to the domain \(\rho _i\ge 0\), \(i\in S\), and \(\rho >0\). This is also the case for the non diagonal coefficients \(D_{ij}\) for \(i\ne j\) whereas the coefficient \(\rho _i D_{ii}\) has a finite positive limit when \(\rho _i\rightarrow 0\) [19, 70].
Remark 5.2
We generally have \({\mathcal R}\subset {\mathcal A}^\perp \) but chemical reaction mechanisms are usually sufficiently rich so that reaction vectors \(\nu _i\), \(i\in {\mathfrak {R}}\), are spanning the maximum space and \({\mathcal R}= {\mathcal A}^\perp \).
5.6 Entropy Production
From Gibbs’ relation \(T \, \,{\mathbb D}{\mathcal S} = \,{\mathbb D}{\mathcal E} - \sum _{k\in S} g_k \,{\mathbb D}\rho _k\), where \(\,{\mathbb D}\) denotes the total derivative, the conservation equations, and the properties of transport coefficients and chemical production rate, one may derive the following balance equation for \(\rho s = {\mathcal S}\)
The viscous tensor has been rewritten for convenience in the form
keeping in mind that \(1 \le d \le 3\). Entropy production (132) therefore appears as a sum of nonnegative terms and the last term represents the entropy production due to chemical reactions \(-R \langle \mu ,\omega \rangle \). From this expression of \(\langle \mu ,\omega \rangle \) we also deduce the following result concerning chemical equilibrium.
Proposition 5.3
Assume that the Properties (\(\mathsf H _\mathsf{1 }\)–\(\mathsf H _\mathsf{3 }\)) hold. Then for any \((T,\rho _1,\ldots ,\rho _n)\in (0,\infty )^{1+n}\) the following statements are equivalent :
-
(i)
The entropy production due to chemistry vanishes \(-R\langle \mu ,\omega \rangle = 0\).
-
(ii)
The reaction rates of progress vanish \(\tau _j=0\), \(j\in {\mathfrak {R}}\).
-
(iii)
The species production rates vanish \(\omega _k=0\), \(k\in S\).
-
(iv)
The vector \(\mu =(\mu _1,\ldots ,\mu _n)^t\) belongs to \(\mathcal {R}^\perp \) where
$$\mathcal {R}= \mathrm{span}\{ \, \nu _i, \ i\in {\mathfrak {R}}\,\}. $$
Proof
From the expression of entropy production due to chemical reactions
and (\(\mathsf H _\mathsf{3 }\)) we obtain that \(\langle \mu ,\omega \rangle =0\) implies \(\langle \mu , \nu _j \rangle =0\), \(j\in {\mathfrak {R}}\), and so \(\tau _j=0\), \(j\in {\mathfrak {R}}\), and we have established that \((i)\) implies \((ii)\). The fact that \((ii)\) implies \((iii)\) is a consequence of \(\omega = \sum _{j\in {\mathfrak {R}}} \tau _j \nu _j\). We also deduce from the expression of entropy production \(- R\langle \mu , \omega \rangle \) that \((iii)\) implies \((i)\) so that the three statements \((i)\), \((ii)\), and \((iii)\) are equivalent. Finally, it is easily established that \((iv)\) is equivalent to \(\langle \mu , \nu _j \rangle =0\), \(j\in {\mathfrak {R}}\), so that \((ii)\) and \((iv)\) are also equivalent. \(\square \)
Definition 5.4
A point \(T^\star >0\), \(\varrho ^\star \in (0,\infty )^n\), which satisfies the equivalent properties of Proposition 5.3 is termed an equilibrium point.
We are only interested here in positive equilibrium states with \(\rho _i>0\), \(i\in S\), which are in the interior of the composition space. Spurious points with zero mass fractions where the source terms \(\omega _k\), \(k\in n\), also vanish—termed ‘boundary equilibrium points’—are of a different nature [9]. Properly structured chemical kinetic mechanisms automatically exclude such spurious points unless some element is missing in the mixture [9].
5.7 Vector Notation
The equations governing multicomponent flows (121)–(123) can be rewritten in the compact vector form
where \(\mathsf {u}\) is the conservative variable, \(\partial _i^{}\) the spatial derivative operator in the \(i\)th spatial direction, \(C=\{1,\ldots ,d\}\) the indexing set of spatial directions, \(d\in \{1,2,3\}\) the spatial dimension, \({\mathsf F}_{i}\) the convective flux in the \(i\)th direction, \({\mathsf F}_{i}^{diss }\) the dissipative flux in the \(i\)th direction, and \(\mathsf {\Omega }\) the source term. Letting \(\mathsf {n}=n+ d+ 1\), the conservative variable \(\mathsf {u}\in {\mathbb {R}}^\mathsf {n}\) is found to be
and the natural variable \(\mathsf {z}\in {\mathbb {R}}^\mathsf {n}\) is defined by
For convenience, the velocity components of vectors in \({\mathbb {R}}^\mathsf {n}_{} = {\mathbb {R}}\times {\mathbb {R}}^d\times {\mathbb {R}}^2\) are generally written as vectors of \({\mathbb {R}}^d\) and bold symbols are used for vector or tensor quantities in the physical space \({\mathbb {R}}^d\). The map \(\mathsf {z}\rightarrow \mathsf {u}\) is a \(C^\varkappa \) diffeomorphism from the open set
onto a convex open set \({\mathcal O}_\mathsf {u}^{}\) of \({\mathbb {R}}^\mathsf {n}\) [9, 95].
Proposition 5.5
The map \(\mathsf {z}\longmapsto \mathsf {u}\) is a \(C^\varkappa \) diffeomorphism from the open set \({\mathcal O}_\mathsf {z}\) onto an open set \({\mathcal O}_\mathsf {u}\). The open set \({\mathcal O}_\mathsf {u}\) is convex and given by
where
and \(e_i^0\) is the energy of formation of the \(i\)th species at zero temperature.
Proof
The map \(\mathsf {z}\rightarrow \mathsf {u}\) is easily shown to be one to one from \({\mathcal O}_\mathsf {z}\) onto \({\mathcal O}_\mathsf {u}\) using the positivity of the constant volume specific heats. The jacobian matrix \(\partial _\mathsf {z}\mathsf {u}\), has a triangular structure and is invertible so that we may use the inverse function theorem. \(\square \)
The convective and diffusive fluxes \({\mathsf F}_{i}\) and \({\mathsf F}_{i}^{diss }\) in the \(i\)th direction are defined by
where \(\varvec{e}_i\) denotes the \(i\)th basis vector in the physical space \({\mathbb {R}}^d\), \(v_i\) the velocity in the \(i\)th direction, \(\mathcal{F}_{ki}\) the diffusion flux of the \(k\)th species in the \(i\)th direction, \(\mathcal{Q}_i\) the heat flux in the \(i\)th direction, \(\varvec{\Pi }=(\Pi _{ij})_{i,j\in C}\) the viscous tensor, and \(\varvec{\Pi }_{i}^{}\) the vector \(\varvec{\Pi }_{i}^{} = (\Pi _{1i}, \ldots , \Pi _{di})^t\), so that \(\varvec{v}= (v_1,\ldots ,v_d)^t\), \(\varvec{\mathcal {F}}_k = (\mathcal{F}_{k1},\ldots ,\mathcal{F}_{kd})^t\), and \({\varvec{Q}}= (\mathcal{Q}_1,\ldots ,\mathcal{Q}_d)^t\).
The dissipative fluxes may further be expressed in the form \({\mathsf F}_{i}^{diss }= - \sum _{j\in C} \widehat{\mathsf B}_{ij}(\mathsf {u}) \partial _j^{}\mathsf {z}\) since all transport fluxes are linear expressions in terms of the gradients of the natural variable \(\mathsf {z}\). Since \(\mathsf {z}\rightarrow \mathsf {u}\) is a smooth diffeomorphism, defining \({\mathsf B}_{ij}(\mathsf {u}) = \widehat{\mathsf B}_{ij}(\mathsf {u}) \partial _\mathsf {u}\mathsf {z}\), for \(i,j\in C\), we obtain that
where the dissipation matrix \({\mathsf B}_{ij}\) relates the dissipative flux in the \(i\)th direction \({\mathsf F}_{i}^{diss }\) to the gradient of the conservative variable in the \(j\)th direction \(\partial _j^{}\mathsf {u}\). Further denoting by \({\mathsf A}_{i}= \partial _\mathsf {u}F_i\), \(i\in C\), the convective flux Jacobian matrices, and \(\mathsf {\Omega }\) the source term
we obtain the quasilinear system
and all the system coefficients \({\mathsf A}_{i}(\mathsf {u})\), \(i\in C\), \({\mathsf B}_{ij}(\mathsf {u})\), \(i,j\in C\), and \(\mathsf {\Omega }(\mathsf {u})\), are smooth functions of \(\mathsf {u}\) on the open convex set \({\mathcal O}_\mathsf {u}^{}\). The mathematical structure of such quasilinear systems is discussed in the next section in an abstract framework.
6 Hyperbolic-Parabolic Structure
We discuss symmetrization with entropic variables and normal variables for abstract systems in quasilinear form. We next investigate the hyperbolic-parabolic structure of the resulting systems of partial differential equations. We further explicitly evaluate the natural entropic symmetrized form as well as the natural normal form for the system of partial differential equations modeling multicomponent reactive fluids.
6.1 Entropic Variables
We consider an abstract second order quasilinear system of conservation laws in the general form
where \(\mathsf {u}\in {\mathcal O}_\mathsf {u}^{}\), \({\mathcal O}_\mathsf {u}^{}\) is an open convex set of \({\mathbb {R}}^\mathsf {n}\), and \(\mathsf {n}\ge 1\). The system coefficients are assumed such that \({\mathsf A}_{i}= \partial _\mathsf {u}{\mathsf F}_{i}\) and the fluxes \({\mathsf F}_{i}\), \(i\in C\), the dissipation matrices \({\mathsf B}_{ij}\), \(i,j\in C\), and the source term \(\mathsf {\Omega }\), are assumed to be \(C^\varkappa \) over \({\mathcal O}_\mathsf {u}^{}\) where \(\varkappa \ge 3\).
A mathematical entropy for the system of partial differential Eq. (137) must be compatible with the convective terms, the dissipative terms as well as the source term and we use the definition presented in [101, 103] simplified to the situation where the set \({\mathcal O}_\mathsf {u}\) is convex. In the following definition, properties (\(\mathsf E _\mathsf{1 }\)), (\(\mathsf E _\mathsf{2 }\)) concerning the convective terms have been adapted from [86, 88], properties (\(\mathsf E _\mathsf{3 }\)), (\(\mathsf E _\mathsf{4 }\)) associated with the dissipative terms have been adapted from [89, 92, 106, 107] and properties (\(\mathsf E _\mathsf{5 }\))–(\(\mathsf E _\mathsf{7 }\)) concerning the source terms have been adapted from [93, 98] and we denote by \(\Sigma ^{d-1}\) the sphere in \(d\) dimension.
Definition 6.1
Consider a \(C^\varkappa \) function \(\mathsf {u}\rightarrow \sigma (\mathsf {u})\) defined over the open convex domain \({\mathcal O}_\mathsf {u}^{}\). The function \(\sigma \) is said to be an entropy function for the system (137) if the following properties hold.
- \(\mathsf (E _\mathsf{1 }\mathsf ) \) :
-
The Hessian matrix \(\partial ^2_\mathsf {u}\sigma (\mathsf {u}) = \partial _\mathsf {u}(\partial _\mathsf {u}\sigma )^t (\mathsf {u})\) is positive definite over \({\mathcal O}_\mathsf {u}\).
- \(\mathsf (E _\mathsf{2 }\mathsf ) \) :
-
There exist \(C^\varkappa \) functions \(\mathsf {u}\rightarrow \mathsf {q}_i(\mathsf {u})\) such that \(\partial _\mathsf {u}^{} \sigma (\mathsf {u}) \, {\mathsf A}_{i}(\mathsf {u}) = \partial _\mathsf {u}^{} \mathsf {q}_i(\mathsf {u})\) for \(\mathsf {u}\in {\mathcal O}_\mathsf {u}^{}\) and \(i\in C\).
- \(\mathsf (E _\mathsf{3 }\mathsf ) \) :
-
We have \(\bigl ( {\mathsf B}_{ij}(\mathsf {u}) \, \bigl (\partial ^2_\mathsf {u}\sigma (\mathsf {u}) \bigr )^{-1} \bigr )^t = {\mathsf B}_{ji}(\mathsf {u}) \bigl (\partial ^2_\mathsf {u}\sigma (\mathsf {u})\bigr )^{-1}\) for \(\mathsf {u}\in {\mathcal O}_\mathsf {u}^{}\) and \(i,j\in C\).
- \(\mathsf (E _\mathsf{4 }\mathsf ) \) :
-
The matrix \(\sum _{i,j\in C} {\mathsf B}_{ij}(\mathsf {u}) \bigl (\partial ^2_\mathsf {u}\sigma (\mathsf {u})\bigr )^{-1}\,\xi _i \xi _j\) is positive semi-definite for \(\mathsf {u}\in {\mathcal O}_\mathsf {u}^{}\) and \(\varvec{\xi }\in {\Sigma }^{d-1}\).
- \(\mathsf (E _\mathsf{5 }\mathsf ) \) :
-
There exists a fixed vector space
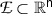 such that
such that  for \(\mathsf {u}\in {\mathcal O}_\mathsf {u}^{}\) and \(\mathsf {\Omega }(\mathsf {u})=0\) if and only if
for \(\mathsf {u}\in {\mathcal O}_\mathsf {u}^{}\) and \(\mathsf {\Omega }(\mathsf {u})=0\) if and only if  and if and only if \(\partial _\mathsf {u}\sigma (\mathsf {u}) \,\, \mathsf {\Omega }(\mathsf {u}) = 0\).
and if and only if \(\partial _\mathsf {u}\sigma (\mathsf {u}) \,\, \mathsf {\Omega }(\mathsf {u}) = 0\). - \(\mathsf (E _\mathsf{6 }\mathsf ) \) :
-
If \(\mathsf {\Omega }(\mathsf {u})=0\), then the matrix \(\partial _\mathsf {u}\mathsf {\Omega }(\mathsf {u}) \, \bigl ( \partial ^2_\mathsf {u}\sigma (\mathsf {u}) \bigr )^{-1}\) is symmetric and its nullspace is given by
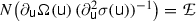 .
. - \(\mathsf (E _\mathsf{7 }\mathsf ) \) :
-
We have \(\partial _\mathsf {u}\sigma (\mathsf {u}) \,\,\mathsf {\Omega }(\mathsf {u}) \le 0\) for \(\mathsf {u}\in {\mathcal O}_\mathsf {u}^{}\).
Existence of an entropy is closely associated with symmetrization properties [86, 88, 89, 92, 93, 98, 101–104, 106, 107]. We do not encounter here the difficulty associated with nonideal fluids where only local symmetrization are feasible and where \({\mathcal O}_\mathsf {u}\) may not be convex [101]. Note also that more general source terms with no symmetry properties at equilibrium have been considered by Chen et al. [93] and Yong [110].
Definition 6.2
Consider a \(C^{\varkappa -1}\) diffeomorphism \(\mathsf {u}\rightarrow \mathsf {v}\) from \({\mathcal O}_\mathsf {u}^{}\) onto an open domain \({\mathcal O}_\mathsf {v}^{}\) and the system in the \(\mathsf {v}\) variable
where \(\widetilde{\mathsf A}_{0} = \partial _\mathsf {v}^{} \mathsf {u}\), \(\widetilde{\mathsf A}_{i}= {\mathsf A}_{i}\partial _\mathsf {v}^{} \mathsf {u}= \partial _\mathsf {v}^{} {\mathsf F}_{i}\), \(\widetilde{\mathsf B}_{ij}= {\mathsf B}_{ij}\partial _\mathsf {v}^{} \mathsf {u}\), and \(\widetilde{\mathsf {\Omega }}= \mathsf {\Omega }\), have at least regularity \(\varkappa -2\). The system is said of the symmetric form if properties (\(\mathsf S _\mathsf{1 }\)–\(\mathsf S _\mathsf{7 }\)) hold.
- \(\mathsf (S _\mathsf{1 }\mathsf ) \) :
-
The matrix \(\widetilde{\mathsf A}_{0}(\mathsf {v})\) is symmetric positive definite for \(\mathsf {v}\in {\mathcal O}_\mathsf {v}^{}\).
- \(\mathsf (S _\mathsf{2 }\mathsf ) \) :
-
The matrices \(\widetilde{\mathsf A}_{i}(\mathsf {v})\), \(i\in C\), are symmetric for \(\mathsf {v}\in {\mathcal O}_\mathsf {v}^{}\).
- \(\mathsf (S _\mathsf{3 }\mathsf ) \) :
-
We have \(\widetilde{\mathsf B}_{ij}^t(\mathsf {v}) = \widetilde{\mathsf B}_{ji}(\mathsf {v})\) for \(i,j\in C\) and \(\mathsf {v}\in {\mathcal O}_\mathsf {v}^{}\).
- \(\mathsf (S _\mathsf{4 }\mathsf ) \) :
-
The matrix \(\widetilde{\mathsf B}_{}(\mathsf {v},\varvec{\xi }) = \sum _{i,j\in C} \widetilde{\mathsf B}_{ij}(\mathsf {v}) \xi _i \xi _j\) is positive semi-definite for \(\mathsf {v}\in {\mathcal O}_\mathsf {v}^{}\) and \(\varvec{\xi }\in {\Sigma }^{d-1}\).
- \(\mathsf (S _\mathsf{5 }\mathsf ) \) :
-
There exists a fixed vector space
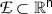 such that
such that 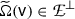 for \(\mathsf {v}\in {\mathcal O}_\mathsf {v}^{}\) and \(\widetilde{\mathsf {\Omega }}(\mathsf {v})=0\) if and only if
for \(\mathsf {v}\in {\mathcal O}_\mathsf {v}^{}\) and \(\widetilde{\mathsf {\Omega }}(\mathsf {v})=0\) if and only if 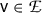 and if and only if \(\bigl \langle \mathsf {v},\widetilde{\mathsf {\Omega }}(\mathsf {v})\bigr \rangle =0\).
and if and only if \(\bigl \langle \mathsf {v},\widetilde{\mathsf {\Omega }}(\mathsf {v})\bigr \rangle =0\). - \(\mathsf (S _\mathsf{6 }\mathsf ) \) :
-
If \(\widetilde{\mathsf {\Omega }}(\mathsf {v})=0\), then \(\partial _\mathsf {v}\widetilde{\mathsf {\Omega }}(\mathsf {v})\) is symmetric and
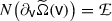 .
. - \(\mathsf (S _\mathsf{7 }\mathsf ) \) :
-
We have \(\bigl \langle \mathsf {v}, \widetilde{\mathsf {\Omega }}(\mathsf {v}) \bigr \rangle \le 0\) for \(\mathsf {v}\in {\mathcal O}_\mathsf {v}^{}\).
The manifold  is naturally termed the equilibrium manifold since \(\widetilde{\mathsf {\Omega }}(\mathsf {v})=0\) when
is naturally termed the equilibrium manifold since \(\widetilde{\mathsf {\Omega }}(\mathsf {v})=0\) when  . The equivalence between symmetrization (\(\mathsf S _\mathsf{1 }\)–\(\mathsf S _\mathsf{7 }\)) and entropy (\(\mathsf E _\mathsf{1 }\)–\(\mathsf E _\mathsf{7 }\)) for hyperbolic-parabolic systems of conservation laws is obtained with \(\mathsf {v}= (\partial _\mathsf {u}^{} \sigma )^t\) [101].
. The equivalence between symmetrization (\(\mathsf S _\mathsf{1 }\)–\(\mathsf S _\mathsf{7 }\)) and entropy (\(\mathsf E _\mathsf{1 }\)–\(\mathsf E _\mathsf{7 }\)) for hyperbolic-parabolic systems of conservation laws is obtained with \(\mathsf {v}= (\partial _\mathsf {u}^{} \sigma )^t\) [101].
Theorem 6.3
Assume that the system (137) admits a \(C^\varkappa \) entropy function \(\sigma \) defined over an open convex domain \({\mathcal O}_\mathsf {u}^{}\). Then the system can be symmetrized with the entropic variable \(\mathsf {v}= (\partial _\mathsf {u}^{} \sigma )^t\). Conversely, assume that the system can be symmetrized with the \(C^{\varkappa -1}\) diffeomorphism \(\mathsf {u}\rightarrow \mathsf {v}\). Then there exists a \(C^\varkappa \) entropy over the open convex set \({\mathcal O}_\mathsf {u}^{}\) such that \(\mathsf {v}= (\partial _\mathsf {u}^{} \sigma )^t\).
Sketch of the proof. The equivalence of (\(\mathsf S _\mathsf{1 }\)–\(\mathsf S _\mathsf{2 }\)) and (\(\mathsf E _\mathsf{1 }\)–\(\mathsf E _\mathsf{2 }\)) is classical and is essentially obtained with Poincaré lemma. Then (\(\mathsf S _\mathsf{3 }\)–\(\mathsf S _\mathsf{7 }\)) and (\(\mathsf E _\mathsf{3 }\)–\(\mathsf E _\mathsf{7 }\)) become identical statements with \(\mathsf {v}= (\partial _\mathsf {u}^{} \sigma )^t\). \(\square \)
6.2 Normal Variables
In order to split the variables between hyperbolic and parabolic variables, we further have to put the system into a normal form, that is,in the form of a symmetric hyperbolic–parabolic composite system [89, 92, 95].
Definition 6.4
Consider a symmetrized system as in Definition 6.2 and let \(\mathsf {v}\rightarrow \mathsf {w}\) be a \(C^{\varkappa -1}\) diffeomorphism from the open set \({\mathcal O}_\mathsf {v}^{}\) onto an open set \({\mathcal O}_\mathsf {w}^{}\). Letting \(\mathsf {v}=\mathsf {v}(\mathsf {w})\) in the symmetrized system (138) and multiplying on the left side by \((\partial _\mathsf {w}^{} \mathsf {v})^t\) we obtain a new system in the variable \(\mathsf {w}\)
where \(\overline{\mathsf A}_{0} = (\partial _\mathsf {w}^{} \mathsf {v})^t \,\, \widetilde{\mathsf A}_{0} \,\,(\partial _\mathsf {w}^{} \mathsf {v})\), \(\overline{\mathsf B}_{ij}= (\partial _\mathsf {w}^{} \mathsf {v})^t \,\, \widetilde{\mathsf B}_{ij}\,\, (\partial _\mathsf {w}^{} \mathsf {v})\), \(\overline{\mathsf A}_{i}= (\partial _\mathsf {w}^{} \mathsf {v})^t \,\, \widetilde{\mathsf A}_{i}\,\, (\partial _\mathsf {w}^{} \mathsf {v})\), \(\overline{\mathsf {\Omega }}= (\partial _\mathsf {w}^{} \mathsf {v})^t {\widetilde{\mathsf {\Omega }}}\), have at least regularity \(\varkappa -2\) and where \(\overline{\mathsf {b}}\) is quadratic in the gradients \(\overline{\mathsf {b}}= - \sum _{i,j\in C}\partial _i^{}(\partial _\mathsf {w}^{} \mathsf {v})^t \, \widetilde{\mathsf B}_{ij}\, (\partial _\mathsf {w}^{} \mathsf {v}) \partial _j^{}\mathsf {w}\). This system satisfies in particular properties (\(\overline{\mathsf{S }}_\mathsf{1 })\)–\((\overline{\mathsf{S }}_\mathsf{4 }\)), that is, properties (\(\mathsf S _\mathsf{1 }\)–\(\mathsf S _\mathsf{4 }\)) rewritten in terms of overbar matrices. This system (139) is said to be of the normal form if there exists a partition of \(\{1,\ldots ,\mathsf {n}\}\) into \(\textsc {i}= \{1,\ldots ,\mathsf {n}_\textsc {i}\}\) and \(\textsc {ii}= \{\mathsf {n}_\textsc {i}+1,\ldots ,\mathsf {n}_\textsc {i}+ \mathsf {n}_\textsc {ii}\}\) with \(\mathsf {n}= \mathsf {n}_\textsc {i}+ \mathsf {n}_\textsc {ii}\) such that the following properties hold.
- \(\mathsf (N _\mathsf{1 }\mathsf ) \) :
-
The matrices \(\overline{\mathsf A}_{0}\) and \(\overline{\mathsf B}_{ij}\) have the block structure
$$ \overline{\mathsf A}_{0} = \left[\begin{array}{cc} \overline{\mathsf A}_{0}^{\textsc {i},\textsc {i}}&{}0_{\mathsf {n}_\textsc {i},\mathsf {n}_\textsc {ii}} \\ {} 0_{\mathsf {n}_\textsc {ii},\mathsf {n}_\textsc {i}}&{}\overline{\mathsf A}_{0}^{\textsc {ii},\textsc {ii}} \end{array}\right], \qquad \overline{\mathsf B}_{ij}= \left[\begin{array}{cc} 0_{\mathsf {n}_\textsc {i},\mathsf {n}_\textsc {i}}&{}0_{\mathsf {n}_\textsc {i},\mathsf {n}_\textsc {ii}}\\ {} 0_{\mathsf {n}_\textsc {ii},\mathsf {n}_\textsc {i}}&{}\overline{\mathsf B}_{ij}^{\textsc {ii},\textsc {ii}} \end{array}\right]. $$ - \(\mathsf (N _\mathsf{2 }\mathsf ) \) :
-
The matrix \(\overline{\mathsf B}_{}^{\textsc {ii},\textsc {ii}}(\mathsf {w},\varvec{\xi }) = \sum _{i,j\in C} \overline{\mathsf B}_{ij}^{\textsc {ii},\textsc {ii}}(\mathsf {w}) \xi _i \xi _j\) is positive definite for \(\mathsf {w}\in {\mathcal O}_\mathsf {w}^{}\) and \(\varvec{\xi }\in {\Sigma }^{d-1}\).
- \(\mathsf (N _\mathsf{3 }\mathsf ) \) :
-
We have \(\overline{\mathsf {b}}(\mathsf {w}, \partial _{\mathsf {x}}^{}\mathsf {w}) = \bigl (\overline{\mathsf {b}}_\textsc {i}(\mathsf {w},\partial _{\mathsf {x}}^{}\mathsf {w}_\textsc {ii}), \overline{\mathsf {b}}_\textsc {ii}(\mathsf {w},\partial _{\mathsf {x}}^{}\mathsf {w})\bigr )^t\).
We have used here the vector and matrix block structure induced by the partitioning of \({\mathbb {R}}^\mathsf {n}\) into \({\mathbb {R}}^\mathsf {n}= {\mathbb {R}}^{\mathsf {n}_\textsc {i}}\times {\mathbb {R}}^{\mathsf {n}_\textsc {ii}}\) so that we have \(\mathsf {w}= (\mathsf {w}_\textsc {i}, \mathsf {w}_\textsc {ii})^t\) for instance and denoted by \(0_{i,j}\) the zero matrix with \(i\) lines and \(j\) columns.
The quadratic residual may also be written in the more elegant form
where \(\overline{\textsc {m}}_{ij}(\mathsf {w})\) are third order tensors that are functions of \(\mathsf {w}\in {\mathcal O}_\mathsf {w}\). From the regularity assumptions of the original system (137), the coefficients of both symmetrized systems (138) and (139) have at least regularity \(\varkappa -2\) and the coefficients \(\overline{\textsc {m}}_{ij}\), \(i,j\in C\), of \(\overline{\mathsf {b}}\) have at least regularity \(\varkappa -3\). A sufficient condition for system (138) to be recast into a normal form is that the nullspace naturally associated with dissipation matrices \(\widetilde{\mathsf B}_{}\) is a fixed subspace of \({\mathbb {R}}^\mathsf {n}\). This is Condition (\(\mathsf N _\mathsf{ }\)) introduced by Kawashima and Shizuta [92] which has been strengthened in [95].
- \(\mathsf (N) \) :
-
The nullspace \(N(\widetilde{\mathsf B}_{})\) of the matrix \(\widetilde{\mathsf B}_{}(\mathsf {v},\varvec{\xi }) = \sum _{i,j\in C} \widetilde{\mathsf B}_{ij}(\mathsf {v}) \xi _i \xi _j\) does not depend on \(\mathsf {v}\in {\mathcal O}_\mathsf {v}^{}\) and \(\varvec{\xi }\in {\Sigma }^{d-1}\) and \(\widetilde{\mathsf B}_{ij}(\mathsf {v}) N(\widetilde{\mathsf B}_{}) =0\), for \(i,j\in C\).
Letting \(\mathsf {n}_\textsc {i}=\dim \bigl (N ( \widetilde{\mathsf B}_{} )\bigr )\) and \(\mathsf {n}_\textsc {ii}= \mathsf {n}- \mathsf {n}_\textsc {i}\) we denote by \(\mathsf {P}\) an arbitrary constant nonsingular matrix of dimension \(\mathsf {n}\) such that its first \(\mathsf {n}_\textsc {i}\) columns span the nullspace \(N(\widetilde{\mathsf B}_{})\). In order to characterize more easily normal forms for symmetric systems of conservation laws satisfying (\(\mathsf N _\mathsf{ }\)) we may introduce the auxiliary variables [9, 95] \(\mathsf {u}^\prime = \mathsf {P}^t \mathsf {u}\) and \(\mathsf {v}^\prime = \mathsf {P}^{-1} \mathsf {v}\). The dissipation matrices corresponding to these auxiliary variables have nonzero coefficients only in the lower right block of size \(\mathsf {n}_\textsc {ii}= \mathsf {n}- \mathsf {n}_\textsc {i}\). Normal symmetric forms are then equivalently—and more easily—obtained from the \(\mathsf {v}^\prime \) symmetric equation [9, 95].
Theorem 6.5
Consider a system of conservation laws (138) that is symmetric in the sense of Definition 6.2 and assume that the nullspace invariance property (\(\mathsf N _\mathsf{ }\)) is satisfied. Denoting by \(\mathsf {u}^\prime = \mathsf {P}^t\mathsf {u}\) and \(\mathsf {v}^\prime = \mathsf {P}^{-1} \mathsf {v}\), the auxiliary variable, any normal form of the system (138) is given by a change of variable in the form \(\mathsf {w}= \bigl ( \mathcal {F}_\textsc {i}(\mathsf {u}_\textsc {i}^\prime ), \mathcal {F}_\textsc {ii}(\mathsf {v}_\textsc {ii}^\prime ) \bigr )^t\) where \(\mathcal {F}_\textsc {i}\) and \( \mathcal {F}_\textsc {ii}\) are two diffeomorphisms of \({\mathbb {R}}^{\mathsf {n}_\textsc {i}}\) and \({\mathbb {R}}^{\mathsf {n}_\textsc {ii}}\), respectively, and we have
where \(\overline{\textsc {m}}_{ij}^{\textsc {ii},\textsc {ii},\textsc {ii}}(\mathsf {w})\) are third order tensors depending on \(\mathsf {w}\) with regularity at least \(\varkappa -3\). Finally, when \(\mathcal {F}_\textsc {ii}\) is linear, the quadratic residual \(\overline{\mathsf {b}}\) is zero.
The main interest of normal forms is that the resulting subsystem of partial differential equations governing the variable \(\mathsf {w}_\textsc {i}\) is symmetric hyperbolic [89, 94] whereas the subsystem governing \(\mathsf {w}_\textsc {ii}\) is symmetric strongly parabolic [89, 92] as discussed in the next section.
Remark 6.6
It is also possible to investigate situations where the general structure of the symmetrized source term \(\widetilde{\mathsf {\Omega }}\) is transfered to the source term \(\overline{\mathsf {\Omega }}\) of the normal variable [104].
6.3 Hyperbolicity and Parabolicity
Consider a first-order abstract system of partial differential equations written in the form
where \(\overline{\mathsf A}_{0}\), \(\overline{\mathsf A}_{i}\), \(i\in C\), and \(\overline{\mathsf {\Omega }}\) are smooth functions of \(\mathsf {w}\) over an open set \({\mathcal O}_{\mathsf {w}}\), \(C= \{1,\ldots ,d\}\) the set of direction indices of \({\mathbb {R}}^d\), and where \(\overline{\mathsf A}_{0}\) is assumed to be invertible. The following definition of hyperbolicity can be found in the book of Denis Serre [94].
Definition 6.7
The system (142) is said to be hyperbolic at a given point \(\mathsf {w}\) if
where for \(\varvec{\xi }\in {\mathbb {R}}^d\) we have defined \(\overline{\mathsf A}_{}(\mathsf {w},\varvec{\xi }) = \sum _{i\in C} \overline{\mathsf A}_{i}(\mathsf {w}) \xi _i\).
When the system (142) is hyperbolic, it is easily established that the matrix \(\bigl (\overline{\mathsf A}_{0}(\mathsf {w})\bigr )^{-1}\overline{\mathsf A}_{}(\mathsf {w},\xi )\) is diagonalizable with real eigenvalues so that it is hyperbolic in the classical sense [94, 96]. We also have the following sufficient condition in terms of eigenvalues and eigenvector matrices established by Serre [94].
Proposition 6.8
Assume that \(\bigl (\overline{\mathsf A}_{0}(\mathsf {w})\bigr )^{-1}\overline{\mathsf A}_{}(\mathsf {w},\varvec{\xi })\) is diagonalizable at \(\mathsf {w}\) for any \(\varvec{\xi }\in {\mathbb {R}}^d\) with real eigenvalues. Let \(P(\varvec{\xi })\) denote a matrix of eigenvectors and assume that
Then the system (142) is also hyperbolic at \(\mathsf {w}\).
A fundamental property of Definition 6.7 is its invariance under a change of variable [94]. Moreover, when a first-order system is symmetrizable, it is hyperbolic [94, 96].
Definition 6.9
The system (142) is said to be symmetric at a given point \(\mathsf {w}\) when \(\overline{\mathsf A}_{0}(\mathsf {w})\) is positive definite and the matrices \(\overline{\mathsf A}_{i}(\mathsf {w})\), \(i\in C\), are symmetric.
Proposition 6.10
A symmetric system of partial differential Eq. (142) is hyperbolic.
Since the existence of an entropy function also implies symmetrizability, it automatically implies hyperbolicity in the sense of Definition 6.7 as well as in the classical sense [94, 96].
We now discuss parabolicity and consider a second-order system in the form
where \(\overline{\mathsf A}_{0}\), \(\overline{\mathsf B}_{ij}\), \(i,j\in C\), are smooth functions of \(\mathsf {w}\) over an open set \({\mathcal O}_\mathsf {w}\), \(\overline{\mathsf {\Omega }}\) is a smooth function over \({\mathcal O}_\mathsf {w}\times {\mathbb {R}}^{d\mathsf {n}}\), and where \(\overline{\mathsf A}_{0}\) is invertible. We will generally consider second-order symmetric systems in the following sense.
Definition 6.11
The system (145) is said to be symmetric at a given point \(\mathsf {w}\) when \(\overline{\mathsf A}_{0}(\mathsf {w})\) is symmetric positive definite and \(\bigl (\overline{\mathsf B}_{ij}(\mathsf {w})\bigr )^t= \overline{\mathsf B}_{ji}(\mathsf {w})\), for \(i,j\in C\).
We then have the following definition for strongly parabolic systems of second-order partial differential equations involving the Legendre-Hadamard condition. Note that this definition should only concern the parabolic subsystem in the variable \(\mathsf {w}_\textsc {ii}\) but we have suppressed all \(\textsc {ii}\) indices to simplify the presentation.
Definition 6.12
Assume that the system (145) is symmetric at a given point \(\mathsf {w}\). This system is said to be strongly parabolic at \(\mathsf {w}\) if there exists a positive constant \(\delta >0\) such that for any \(\varvec{\xi }=(\xi _1,\dots ,\xi _d)^t\) and \(w=(w_1,\dots ,w_\mathsf {n})^t\) we have
Remark 6.13
It is often the case that systems of partial differential equations of physical origin satisfy a stronger property—the Legendre condition—than (146) at a given point \(\mathsf {w}\) and are indeed such that
for any \(\zeta \in {\mathbb {R}}^{d\mathsf {n}}\). The condition (146) then simply corresponds to the situation where \(\zeta \) is constrained to be a tensor product \(\zeta =\xi {\otimes }w\), so that \(\zeta _{ik} = \xi _i w_k\), for \(i\in \{1,\ldots ,d\}\) and \(k\in \{1,\ldots ,\mathsf {n}\}\).
The definition of strong parabolicity is only given here for symmetric systems and will be applied to the symmetrized forms like (138) or (139), thereby naturally involving entropy Hessians. Indeed, the definition of strong parabolicity in the usual sense, which neither require symmetry properties nor entropy hessians, only has a meaning for particular forms of systems of partial differential equation under consideration which need to be specified as shown by the following counter example. Consider the system  where \(\mathsf {w}= (\mathsf {w}_1,\mathsf {w}_2)^t\), \(\varDelta \mathsf {w}= (\varDelta \mathsf {w}_1,\varDelta \mathsf {w}_2)^t\), and
where \(\mathsf {w}= (\mathsf {w}_1,\mathsf {w}_2)^t\), \(\varDelta \mathsf {w}= (\varDelta \mathsf {w}_1,\varDelta \mathsf {w}_2)^t\), and  , with
, with  ,
,  , and
, and  . In other words, consider two uncoupled heat equations which of course form a symmetric strongly parabolic system. Introduce next the modified variable \(\mathsf {w}^{\prime *} = (\mathsf {w}_1+\mathsf {w}_2,\delta \mathsf {w}_2)^t\) where \(\delta >0\) is a positive parameter. We then have
. In other words, consider two uncoupled heat equations which of course form a symmetric strongly parabolic system. Introduce next the modified variable \(\mathsf {w}^{\prime *} = (\mathsf {w}_1+\mathsf {w}_2,\delta \mathsf {w}_2)^t\) where \(\delta >0\) is a positive parameter. We then have  with
with

so that if  the quadratic form associated with
the quadratic form associated with  is not positive definite and the system in the \(\mathsf {w}^{\prime *}\) variable is not strongly parabolic in the usual sense even though it is obtained from a trivially strongly parabolic system.
is not positive definite and the system in the \(\mathsf {w}^{\prime *}\) variable is not strongly parabolic in the usual sense even though it is obtained from a trivially strongly parabolic system.
Definition 6.14
Denoting \(\overline{\mathsf B}_{}(\mathsf {w},\varvec{\xi }) = \sum _{i,j\in C} \xi _i \xi _j \overline{\mathsf B}_{ij}(\mathsf {w})\), a system (145) is said to be parabolic in the sense of Petrovsky at a given point \(\mathsf {w}\) if there exists a positive constant \(\delta \) such that for any \(\varvec{\xi }\in {\mathbb {R}}^d\), the eigenvalues \(\lambda \) of \(\bigl (\overline{\mathsf A}_{0}(\mathsf {w})\bigr )^{-1} \overline{\mathsf B}_{}(\mathsf {w},\varvec{\xi })\), are such that
Various other generalized definitions of parabolicity are discussed in the book of Lady z̃ enskaja et al. [87], in particular that of Douglis and Nirenberg, Shirota, and Eĭdel’man, but these definitions coincide with that of Petrovsky for second-order systems [87] and also coincide with the notion of normal ellipticity. For symmetric systems, we now have the following equivalence result [101].
Proposition 6.15
Consider a second-order system in the form
and assume that the system is symmetric. Then the system is strongly parabolic at \(\mathsf {w}\) if and only if it is Petrovsky parabolic at \(\mathsf {w}\).
Sketch of the proof. The eigenvalues of \(\bigl (\overline{\mathsf A}_{0}(\mathsf {w})\bigr )^{-1} \overline{\mathsf B}_{}(\mathsf {w},\varvec{\xi })\) are essentially Rayleigh quotients with respect to the scalar product \(\langle \!\langle x,y\rangle \!\rangle = \langle \overline{\mathsf A}_{0}x,y\rangle \). \(\square \)
From a practical point of view, for systems of partial differential equations derived from physics, thanks to the existence of a mathematical entropy, we can use symmetrized systems of partial differential equations and then rely of the proper definition of strongly parabolic systems 6.12. In addition, strongly parabolicity is then invariant by a change of variable for symmetric systems, after multiplication of the left by the transpose of the jacobian matrix. Considering for instance the previous system  where \(\mathsf {w}= (\mathsf {w}_1,\mathsf {w}_2)^t\), \(\varDelta \mathsf {w}= (\varDelta \mathsf {w}_1,\varDelta \mathsf {w}_2)^t\), and
where \(\mathsf {w}= (\mathsf {w}_1,\mathsf {w}_2)^t\), \(\varDelta \mathsf {w}= (\varDelta \mathsf {w}_1,\varDelta \mathsf {w}_2)^t\), and  , with
, with  ,
,  , and letting \(\mathsf {w}^{\prime *} = (\mathsf {w}_1+\mathsf {w}_2,\delta \mathsf {w}_2)^t\) where \(\delta >0\) is a positive parameter and
, and letting \(\mathsf {w}^{\prime *} = (\mathsf {w}_1+\mathsf {w}_2,\delta \mathsf {w}_2)^t\) where \(\delta >0\) is a positive parameter and
we then have

which remains symmetric strongly parabolic.
6.4 Natural Entropic form for Multicomponent Flows
We evaluate in this section the natural entropic symmetrized form for the system of partial differential equations modeling multicomponent reative fluids. We use the mathematical entropy \(\sigma = - \mathcal {S}/R\) where the \(1/R\) factor is introduced for convenience. For this particular system of partial differential equations we have \(\mathsf {n}=n+ d+ 1\), the velocity components of all quantities in \({\mathbb {R}}^{n+ d+1}\) are denoted as vectors of \({\mathbb {R}}^d\) and the corresponding partitioning is also used for matrices.
Theorem 6.16
Assume that (\(\mathsf H _\mathsf{1 }\)–\(\mathsf H _\mathsf{5 }\)) hold. Then the function \(\sigma =-\mathcal {S}/R\) is a mathematical entropy for the system (136) governing multicomponent fluids and the corresponding entropic variable is
The map \(\mathsf {u}\rightarrow \mathsf {v}\) is a \(C^{\varkappa -1}\) diffeomorphism from \({\mathcal O}_\mathsf {u}^{}\) onto \({\mathcal O}_\mathsf {v}\). The system written in term of the entropic variable \(\mathsf {v}\) is
with \(\widetilde{\mathsf A}_{0}=\partial _\mathsf {v}\mathsf {u}\), \(\widetilde{\mathsf A}_{i}={\mathsf A}_{i}\partial _\mathsf {v}\mathsf {u}\), \(\widetilde{\mathsf B}_{ij}={\mathsf B}_{ij}\partial _\mathsf {v}\mathsf {u}\), and \(\widetilde{\mathsf {\Omega }}=\mathsf {\Omega }\), and is of the symmetric form. The matrix \(\widetilde{\mathsf A}_{0}\) is given by

where  is the diagonal matrix of size
is the diagonal matrix of size  given by
given by

 is the vector of size
is the vector of size  given by
given by

and  . Since \(\widetilde{\mathsf A}_{0}\) is symmetric, we only give its left lower triangular part and write “Sym” in the upper triangular part. Denoting by \(\varvec{\xi }= (\xi _1,\ldots ,\xi _d)^t\) an arbitrary vector of \({\mathbb {R}}^d\) and letting \(\widetilde{\mathsf A}_{} = \sum _{i\in C} \xi _i \widetilde{\mathsf A}_{i}\), we have
. Since \(\widetilde{\mathsf A}_{0}\) is symmetric, we only give its left lower triangular part and write “Sym” in the upper triangular part. Denoting by \(\varvec{\xi }= (\xi _1,\ldots ,\xi _d)^t\) an arbitrary vector of \({\mathbb {R}}^d\) and letting \(\widetilde{\mathsf A}_{} = \sum _{i\in C} \xi _i \widetilde{\mathsf A}_{i}\), we have

Moreover, we have the decomposition
with

where  is the matrix of size \(n\) with components \(\rho _k \rho _l D_{kl}\) and
is the matrix of size \(n\) with components \(\rho _k \rho _l D_{kl}\) and  is the vector of size \(n\) with components
is the vector of size \(n\) with components  . Moreover, denoting by \(\varvec{\xi }= (\xi _1,\ldots ,\xi _d)^t\) and \(\varvec{\zeta }= (\zeta _1,\ldots ,\zeta _d)^t\) arbitrary vectors of \({\mathbb {R}}^d\), the matrices \(\widetilde{\mathsf B}_{ij}^\kappa \) and \(\widetilde{\mathsf B}_{ij}^\eta \), \(i,j\in C\), are given by
. Moreover, denoting by \(\varvec{\xi }= (\xi _1,\ldots ,\xi _d)^t\) and \(\varvec{\zeta }= (\zeta _1,\ldots ,\zeta _d)^t\) arbitrary vectors of \({\mathbb {R}}^d\), the matrices \(\widetilde{\mathsf B}_{ij}^\kappa \) and \(\widetilde{\mathsf B}_{ij}^\eta \), \(i,j\in C\), are given by
Finally, the equilibrium manifold is given by

where \(\mathcal {R}= \hbox {span}\{\, \nu _i,\ i\in {\mathfrak {R}}\, \} \subset {\mathbb {R}}^n\) is spanned by the reaction vectors and \(M= \mathrm {diag}(m_1,\ldots ,m_n)\). Moreover, at an equilibrium point \(\mathsf {v}^\star \) according to Definition 5.3 where \(\widetilde{\mathsf {\Omega }}(\mathsf {v}^\star )=0\), the linearized source term \(\widetilde{\mathsf {L}}(\mathsf {v}^\star ) = -\partial _\mathsf {v}\widetilde{\mathsf {\Omega }}(\mathsf {v}^\star )\) is in the form
where \({\mathfrak {p}}_i = (m_1\nu _{1i},\ldots ,m_n\nu _{ni},\varvec{0},0)^t\) and \(\varLambda _i = \mathcal{K}_i^\mathrm{{f}}(T^\star ) \, \prod _{k\in S} (\rho _k^\star /m_k)^{\nu _{ki}^\mathrm{{f}}}\).
Sketch of the proof. The proof is lengthy but present no serious difficulty and we refer the reader to [9, 95, 101]. \(\square \)
Remark 6.17
For ideal fluids, the symmetrizing change of variable \(\mathsf {u}\rightarrow \mathsf {v}\) is one to one and is thus a global change of variable [9, 95]. On the contrary, for nonideal fluid, even though the entropy \(\sigma \) is globally defined, a typical situation is that of distinct points \(\mathsf {u}^\sharp \) and \(\mathsf {u}^\flat \) such that \(\mathsf {v}^\sharp = \mathsf {v}^\flat \). Indeed, we see from (149) that the equality \(\mathsf {v}^\sharp = \mathsf {v}^\flat \) corresponds to the chemical equilibrium between the two stable phases \(\mathsf {u}^\sharp \) and \(\mathsf {u}^\flat \) with identical pressure, temperature and Gibbs functions, that may notably be observed for nonideal fluids.
For mixtures of ideal gases, there is also a uniqueness theorem for mathematical entropies that are independent of transport coefficients [103]. This result strengthen the representation theorem of normal variable as well as the importance of the natural entropic symmetrized form.
Theorem 6.18
Let \(\widetilde{\sigma }\) be a \(C^\varkappa \) function defined on the open set \({\mathcal O}_\mathsf {u}\) satisfying (\(\mathsf E _\mathsf{2 }\)), (\(\mathsf E _\mathsf{3 }\)) and such that \(\partial ^2_\mathsf {u}\widetilde{\sigma }\) is invertible. Assuming that \(\widetilde{\sigma }\) is independent of the mass and heat diffusion parameters, then \(\widetilde{\sigma }\) is in the form
where \(\alpha _\mathcal {S}^{}\) \(\alpha _i^{}\), \(i\in S\), \(\alpha _{\varvec{v}}^{}\) and \(\alpha _\mathcal {E}^{}\) are constants.
This shows in particular that mathematical entropies independent of transport parameters—a somewhat natural condition—are indeed unique up to an affine transform, once the trivial factors proportional to conserved quantities have been eliminated and the corresponding entropic variables are then proportional.
6.5 Natural Normal form for Multicomponent Flows
The symmetric system (150) may be rewritten into a normal form, that is, in the form of a symmetric hyperbolic-parabolic composite system, where hyperbolic and parabolic variables are split [89, 92, 95, 100, 105–108, 113]. We first establish that the nullspace invariance property holds for multicomponent flows.
Lemma 6.19
The nullspace of the matrix
is independent of \(\mathsf {v}\in {\mathcal O}_{\mathsf {v}}\) and \(\varvec{\xi }\in \Sigma ^{d-1}\) and given by
and we have \(\widetilde{\mathsf B}_{ij}(\mathsf {v})N(\widetilde{\mathsf B}_{})=0\), \(i,j\in C\), for \(\mathsf {v}\in {\mathcal O}_{\mathsf {v}}^{}\).
Proof
Letting \(\mathsf {x}= \bigl ( \mathsf {x}_1,\ldots ,\mathsf {x}_n, \varvec{\mathsf {x}}_{\varvec{v}}, \mathsf {x}_T)^t\), with \(\mathsf {x}_\varrho = \bigl ( \mathsf {x}_1,\ldots ,\mathsf {x}_n)^t\), \(\varvec{\mathsf {x}}_{\varvec{v}} = \bigl ( \mathsf {x}_{n+1}, \ldots , \mathsf {x}_{n+d})^t\), it is obtained after some algebra with \(\xi \in \Sigma ^{d-1}\) that

Assuming that \(\bigl \langle \widetilde{\mathsf B}_{} \mathsf {x},\mathsf {x}\bigr \rangle =0\) we thus obtain that successively that \(\mathsf {x}_T=0\) and \(\varvec{\mathsf {x}}_{\varvec{v}}=\varvec{0}\) and next since  , we deduce that \((\mathsf {x}_1,\ldots ,\mathsf {x}_n)^t \in {\mathbb {R}}{}{1\!\!\!\mathrm {I}}\). We have thus established that \(N(\widetilde{\mathsf B}_{})\) is spanned by \((1,\ldots ,1,\varvec{0},0)^t\) and it is easily checked that \(\widetilde{\mathsf B}_{ij}(\mathsf {v}) N(\widetilde{\mathsf B}_{})=0\), \(i,j\in C\), for \(\mathsf {v}\in {\mathcal O}_{\mathsf {v}}^{}\). \(\square \)
, we deduce that \((\mathsf {x}_1,\ldots ,\mathsf {x}_n)^t \in {\mathbb {R}}{}{1\!\!\!\mathrm {I}}\). We have thus established that \(N(\widetilde{\mathsf B}_{})\) is spanned by \((1,\ldots ,1,\varvec{0},0)^t\) and it is easily checked that \(\widetilde{\mathsf B}_{ij}(\mathsf {v}) N(\widetilde{\mathsf B}_{})=0\), \(i,j\in C\), for \(\mathsf {v}\in {\mathcal O}_{\mathsf {v}}^{}\). \(\square \)
Since \(N(\widetilde{\mathsf B}_{})\) is spanned by \(({}{1\!\!\!\mathrm {I}},\varvec{0},0)^t\), we define the matrix \(\mathsf {P}\) by
and we may introduce the auxiliary variable \(\mathsf {u}' = \mathsf {P}^t\mathsf {u}\) and the corresponding entropic variable \(\mathsf {v}' = \mathsf {P}^{-1}\mathsf {v}\) given by
and
From the representation theorem 6.5, we deduce that all normal forms of the system (138) are obtained with variables \(\mathsf {w}\) in the form
where \(\mathcal {F}_\textsc {i}\) and \(\mathcal {F}_\textsc {ii}\) are diffeomorphism of \({\mathbb {R}}\) and \({\mathbb {R}}^{n+d}\). In the following theorem, we evaluate the normal form corresponding to the natural normal variable.
Theorem 6.20
Assume that (\(\mathsf H _\mathsf{1 }\)–\(\mathsf H _\mathsf{5 }\)) hold and consider the normal variable
and the diffeomorphism \(\mathsf {v}\longmapsto \mathsf {w}\) from \({\mathcal O}_\mathsf {v}\) onto the open set \({\mathcal O}_\mathsf {w}= (0,\infty )\times {\mathbb {R}}^{n-1}\times {\mathbb {R}}^d\times (-\infty ,0)\). Then the system of partial differential equations in normal form may be written
where the matrix \(\overline{\mathsf A}_{0}\) is given by
with

The matrix  is the square matrix of dimension \(n-1\) with coefficients
is the square matrix of dimension \(n-1\) with coefficients

\({\mathsf a}\) is the vector of dimension \(n-1\) with coefficients

and \(\overline{\varUpsilon }\) is given by

keeping in mind that  ,
,  , and that \(\varLambda = \mathrm {diag}(m_1\rho _1,\ldots ,m_n\rho _n)\). Denoting by \(\varvec{\xi }= (\xi _1,\ldots ,\xi _d)^t\) an arbitrary vector of \({\mathbb {R}}^d\), the matrices \(\overline{\mathsf A}_{i}\), \(i\in C\), are given by
, and that \(\varLambda = \mathrm {diag}(m_1\rho _1,\ldots ,m_n\rho _n)\). Denoting by \(\varvec{\xi }= (\xi _1,\ldots ,\xi _d)^t\) an arbitrary vector of \({\mathbb {R}}^d\), the matrices \(\overline{\mathsf A}_{i}\), \(i\in C\), are given by

where  is the vector of dimension \(n-1\) with components
is the vector of dimension \(n-1\) with components

and

The matrices \(\overline{\mathsf B}_{ij}\) have the structure
where
whereas \(\overline{\mathsf B}^{D\lambda }\) has its first line and first column composed of zeros and its lower right block  equal to
equal to  so that
so that

where  is the matrix of size \(n-1\) with coefficients \((\rho _k \rho _l D_{kl})_{2\le k,l\le n}\) and
is the matrix of size \(n-1\) with coefficients \((\rho _k \rho _l D_{kl})_{2\le k,l\le n}\) and  are the \(n-1\) last components of
are the \(n-1\) last components of  . Finally, the source term for the normal form is given by
. Finally, the source term for the normal form is given by

Proof
The proof is lengthy and tedious but presents no serious difficulties. \(\square \)
7 The Cauchy Problem
The equations governing multicomponent reactive flows have been derived from the kinetic theory of gases and rewritten in normal form. These equations have local smooth solutions [108], global smooth solutions around constant equilibrium states [95] and also traveling wave solutions [119]. We discuss in this section existence theorem around equilibrium states and asymptotic stability. The smooth dependence on a parameter has also been investigated in [97]. We first discuss local strict dissipativity in an abstract setting and then discuss the situation of multicomponent flows and the Cauchy problem [89, 92, 94, 95, 97–102].
7.1 Local Dissipativity
We present in this section the dissipativity properties around equilibrium states that are needed in order to establish global existence and asymptotic stability [89, 95, 106]. We consider a symmetrizable quasilinear system, assume that the nullspace invariance property (\(\mathsf N _\mathsf{ }\)) holds, and that the system has been rewritten in normal form.
We assume that there exists an equilibrium state \(\mathsf {u}^\star \in {\mathcal O}_\mathsf {u}\) with \(\mathsf {\Omega }(\mathsf {u}^\star )=0\) and we denote by \(\mathsf {v}^\star \) and \(\mathsf {w}^\star \) the corresponding constant state in the \(\mathsf {v}\) and \(\mathsf {w}\) variables respectively. If we linearize system (139) around the constant stationary state \(\mathsf {w}^\star \), we obtain a linear system in the variable \(\delta {\mathsf w}=\mathsf {w}-\mathsf {w}^\star \) in the form
where \( \overline{\mathsf L}(\mathsf {w}^\star )\) is defined by \(\overline{\mathsf L}(\mathsf {w}^\star ) = -\partial _\mathsf {w}^{}\overline{\mathsf {\Omega }}(\mathsf {w}^\star )\). Investigating smooth global solutions around constant equilibrium states require such linearized normal forms to be strictly dissipative [89]. When the source term of the normal form is in quasilinear form, the matrix \(\overline{\mathsf L}\) is positive semi-definite. However, since \(\mathsf {w}^\star \) is an equilibrium point, this is a general property deduced form (\(\mathsf S _\mathsf{7 }\)) [101].
Lemma 7.1
Consider a system of conservation law with an entropy and assume that the system is written in normal form (139). Assuming that \(\mathsf {w}^\star \) is such that \(\overline{\mathsf {\Omega }}(\mathsf {w}^\star ) =0\), then, letting \(\overline{\mathsf L}=-\partial _\mathsf {w}^{}\overline{\mathsf {\Omega }}\), the matrix \(\overline{\mathsf L}(\mathsf {w}^\star )\) is symmetric positive semi-definite.
By Fourier transform, the spectral problem associated with the linear system of partial differential equations (164) reads
where \(\zeta \in {\mathbb {R}}\), \(\mathrm {i}^2=-1\), \(\varvec{\xi }\in {\Sigma }^{d-1}\), \(\phi \in {\mathbb C}^\mathsf {n}\), \(\overline{\mathsf A}_{}(\mathsf {w}^\star ,\varvec{\xi }) = \sum _{i\in C}\overline{\mathsf A}_{i}(\mathsf {w}^\star ) \xi _i\) and
We denote by  the set of complex numbers \(\mathsf {\gamma }\) such that there exists \(\phi \in {\mathbb C}^\mathsf {n}\), \(\phi \ne 0\), satisfying (165). The following equivalent forms of the ‘Kawashima condition’ have been established by Shizuta and Kawashima [106] for (\(\mathsf K _\mathsf{1 }\)–\(\mathsf K _\mathsf{4 }\)) and Beauchard and Zuazua [117] for (\(\mathsf K _\mathsf{5 }\)).
the set of complex numbers \(\mathsf {\gamma }\) such that there exists \(\phi \in {\mathbb C}^\mathsf {n}\), \(\phi \ne 0\), satisfying (165). The following equivalent forms of the ‘Kawashima condition’ have been established by Shizuta and Kawashima [106] for (\(\mathsf K _\mathsf{1 }\)–\(\mathsf K _\mathsf{4 }\)) and Beauchard and Zuazua [117] for (\(\mathsf K _\mathsf{5 }\)).
Theorem 7.2
Assume that the matrix \(\overline{\mathsf A}_{0}(\mathsf {w}^\star )\) is symmetric positive definite, that the matrices \(\overline{\mathsf A}_{i}(\mathsf {w}^\star )\), \(i\in C\), are symmetric, that the reciprocity relations \(\overline{\mathsf B}_{ij}(\mathsf {w}^\star )^t = \overline{\mathsf B}_{ji}(\mathsf {w}^\star )\), \(i,j\in C\) hold, that the matrix \(\overline{\mathsf B}_{}(\mathsf {w}^\star ,\varvec{\xi }) = \sum _{i,j\in C} \overline{\mathsf B}_{ij}(\mathsf {w}^\star ) \xi _i \xi _j\) is positive semi-definite for \(\varvec{\xi }\in \Sigma ^{d-1}\), that \(\overline{\mathsf L}(\mathsf {w}^\star )\) is symmetric positive semi-definite, and denote \(\overline{\mathsf A}_{}(\mathsf {w}^\star , \varvec{\xi }) = \sum _{i\in C} \overline{\mathsf A}_{i}(\mathsf {w}^\star ) \xi _i\). The system of partial differential equations is said to be strictly dissipative at \(\mathsf {w}^\star \) when any of the following equivalent properties holds.
- \(\mathsf (K _\mathsf{1 }\mathsf ) \) :
-
There exists a \(C^\infty \) map \(K : \Sigma ^{d-1} \rightarrow {\mathbb {R}}^{d,d}\) such that for any \(\varvec{\xi }\in {\Sigma }^{d-1}\) the product \(K(\varvec{\xi }) \overline{\mathsf A}_{0}(\mathsf {w}^\star ) \) is skew-symmetric, \(K(-\varvec{\xi }) = - K(\varvec{\xi })\), and \(K(\varvec{\xi }) \, \overline{\mathsf A}_{}(\mathsf {w}^\star , \varvec{\xi }) + \overline{\mathsf B}_{}(\mathsf {w}^\star ,\varvec{\xi }) + \overline{\mathsf L}(\mathsf {w}^\star )\) is positive definite.
- \(\mathsf (K _\mathsf{2 }\mathsf ) \) :
-
For any \(\zeta \in \, {\mathbb {R}}\), \(\zeta \ne 0\), and any \(\varvec{\xi }\in {\Sigma }^{d-1}\), the eigenvalues
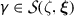 have a negative real part \(\mathfrak {R}(\mathsf {\gamma }) <0\).
have a negative real part \(\mathfrak {R}(\mathsf {\gamma }) <0\). - \(\mathsf (K _\mathsf{3 }\mathsf ) \) :
-
Let \(\phi \in {\mathbb {R}}^\mathsf {n}\backslash \{0\}\) such that \(\overline{\mathsf B}_{}(\mathsf {w}^\star , \varvec{\xi }) \phi =0\) and \(\overline{\mathsf L}(\mathsf {w}^\star )\phi =0\) for some \(\varvec{\xi }\in {\Sigma }^{d-1}\). Then we have \(\zeta \overline{\mathsf A}_{0}(\mathsf {w}^\star )\phi + \overline{\mathsf A}_{}(\mathsf {w}^\star , \varvec{\xi }) \phi \ne 0\) for any \(\zeta \in {\mathbb {R}}\).
- \(\mathsf (K _\mathsf{4 }\mathsf ) \) :
-
There exists \(\delta >0\) such that for any \(\zeta \in {\mathbb {R}}\), \(\varvec{\xi }\in {\Sigma }^{d-1}\), the eigenvalue
 have their real part majorized by \(\mathfrak {R}(\mathsf {\gamma }) \le - \delta \frac{\vert \zeta \vert ^2}{1+\vert \zeta \vert ^2}\).
have their real part majorized by \(\mathfrak {R}(\mathsf {\gamma }) \le - \delta \frac{\vert \zeta \vert ^2}{1+\vert \zeta \vert ^2}\). - \(\mathsf (K _\mathsf{5 }\mathsf ) \) :
-
Letting \(\widehat{\mathsf A}^\star = \bigl (\overline{\mathsf A}_{0}(\mathsf {w}^\star )\bigr )^{-1} \overline{\mathsf A}_{}(\mathsf {w}^\star ,\varvec{\xi })\) and
$$\widehat{\mathsf B}^\star = \bigl (\overline{\mathsf A}_{0}(\mathsf {w}^\star )\bigr )^{-1} \bigl ( \overline{\mathsf B}_{}(\mathsf {w}^\star ,\varvec{\xi }) + \overline{\mathsf L}(\mathsf {w}^\star ) \bigr ), $$the Kalman condition \(\mathrm {rank}\bigl [\, \widehat{\mathsf B}^\star , \widehat{\mathsf A}^\star \,\widehat{\mathsf B}^\star , \ldots , (\widehat{\mathsf A}^\star )^{\mathsf {n}-1}\, \widehat{\mathsf B}^\star \, \bigr ] = \mathsf {n}\) holds.
A physical interpretation of the ‘Kawashima condition’ (\(\mathsf K _\mathsf{1 }\)–\(\mathsf K _\mathsf{5 }\)) is that all waves associated with the hyperbolic operator \(\overline{\mathsf A}_{0}(\mathsf {w}^\star )\partial _t^{}\ + \sum _{i\in C}\overline{\mathsf A}_{i}(\mathsf {w}^\star ) \partial _i^{}\ \) lead to dissipation, i.e., entropy production, since there are not in the nullspace of \(\overline{\mathsf B}_{}\), as shown by (\(\mathsf K _\mathsf{3 }\)). Only the symmetric part of the product \(K(\varvec{\xi }) \, \overline{\mathsf A}_{}(\mathsf {w}^\star , \varvec{\xi })\) plays a role in (\(\mathsf K _\mathsf{1 }\)). The traditional Kalman condition involving the \(\mathsf {n}^2\times \mathsf {n}\) matrix with first block \(\widehat{\mathsf B}^\star \), second block \(\widehat{\mathsf B}^\star \,\widehat{\mathsf A}^\star \), and \(k\)th block \(\widehat{\mathsf B}^\star \, (\widehat{\mathsf A}^\star )^{k-1}\) has been rewritten in the form (\(\mathsf K _\mathsf{5 }\)) with a \(\mathsf {n}\times \mathsf {n}^2\) matrix thanks to the symmetry of \(\overline{\mathsf A}_{0}(\mathsf {w}^\star )\), \(\overline{\mathsf B}_{}(\mathsf {w}^\star ,\varvec{\xi })\) and \(\overline{\mathsf L}(\mathsf {w}^\star )\).
Remark 7.3
It is not known in general if the matrix \(K(\varvec{\xi })\) may be written \(\sum _{j\in C}K_j \xi _j\) although it is generally possible to obtain compensating matrices in this form in practical applications.
7.2 Existence of Solutions
Local in time solutions [108] may first be obtained by using a normal from as well as a general existence theorem from Volpert and Hujaev [105]. The corresponding existence result in [108] has been presented for a more general fluid with vibrational desequilibrium but also directly applies to the system of partial differential equations presented in the previous sections.
On the other hand, global solutions around equilibrium states may also be obtained using the local strict dissipative properties for multicomponent flows. The existence of chemical equilibrium points is first a consequence of the structural properties of thermochemistry and is traditionally obtained by minimizing a thermodynamic function [9].
Proposition 7.4
For \(T^\star >0\) and \(\varrho ^c \in (0,\infty )^n\) there exists a unique equilibrium point \(\mathsf {u}^\star \) associated with \(\mathsf {z}^\star = \bigl ( \rho _1^\star ,\ldots ,\rho _n^\star , \varvec{0}, T^\star \bigr )^t\) such that \(\varrho ^\star - \varrho ^c \in M \mathcal {R}\).
The system of partial differential equations governing multicomponent reactive flows written in normal form is then strictly dissipative [89, 92, 95, 97, 106].
Proposition 7.5
Consider an equilibrium state \(\mathsf {w}^\star \) as obtained in Proposition 7.4. Then the linearized normal form at \(\mathsf {w}^\star \) is strictly dissipative.
Proof
Using for convenience the characterization (\(\mathsf K _\mathsf{3 }\)), we consider \(\varvec{\xi }\in {\Sigma }^{d-1}\) and assume that \(\phi \ne 0\) is such that \(\overline{\mathsf B}_{}(\mathsf {w}^\star , \varvec{\xi })\phi =\overline{\mathsf L}(\mathsf {w}^\star )\phi =0\). We first establish that \(\phi =\alpha (1,0,\ldots ,0)^t\) for some \(\alpha \ne 0\).
From the expression of \(\overline{\mathsf B}_{}\) in Theorem 6.20, letting
we obtain

This is a sum of nonnegative terms and if it is zero, we successively deduce that \(\phi _T=0\) and then that \(\varvec{\phi }_{\varvec{v}}=0\) and finally that  . Since \(N(D)={\mathbb {R}}{\mathsf {y}}\) we have
. Since \(N(D)={\mathbb {R}}{\mathsf {y}}\) we have  so that
so that  is positive definite for vectors in the form \((0,\phi _2,\ldots ,\phi _n)^t\) and \(\phi _2=\ldots =\phi _n=0\). We have established that \(\phi \) is proportional to \((1,0,\ldots ,0)^t\) and such vectors are also in the nullspace of \(\overline{\mathsf L}\). Indeed, using (158) and \(\overline{\mathsf L}(\mathsf {w}^\star ) = \bigl (\partial _\mathsf {w}\mathsf {v}(\mathsf {w}^\star )\bigr )^t \, \widetilde{\mathsf {L}}(\mathsf {v}^\star ) \, \partial _\mathsf {w}\mathsf {v}(\mathsf {w}^\star )\), a direct calculation yields
is positive definite for vectors in the form \((0,\phi _2,\ldots ,\phi _n)^t\) and \(\phi _2=\ldots =\phi _n=0\). We have established that \(\phi \) is proportional to \((1,0,\ldots ,0)^t\) and such vectors are also in the nullspace of \(\overline{\mathsf L}\). Indeed, using (158) and \(\overline{\mathsf L}(\mathsf {w}^\star ) = \bigl (\partial _\mathsf {w}\mathsf {v}(\mathsf {w}^\star )\bigr )^t \, \widetilde{\mathsf {L}}(\mathsf {v}^\star ) \, \partial _\mathsf {w}\mathsf {v}(\mathsf {w}^\star )\), a direct calculation yields
where \({\mathfrak {p}}_i' = (0,m_2\nu _{2i},\ldots ,m_n\nu _{ni},\varvec{0},0)^t\) so that \((1,0,\ldots ,0)^t\) is in the nullspace of \(\overline{\mathsf L}(\mathsf {w}^\star )\).
We must now establish that for any \(\zeta \in {\mathbb {R}}\) we have \(\zeta \overline{\mathsf A}_{0}(\mathsf {w}^\star )\phi + \overline{\mathsf A}_{}(\mathsf {w}^\star , \varvec{\xi }) \phi \ne 0\) where \(\overline{\mathsf A}_{}(\mathsf {w}^\star , \varvec{\xi }) = \sum _{i\in C} \overline{\mathsf A}_{i}(\mathsf {w}^\star ) \xi _i\). However, a direct calculation keeping the notation of Theorem 6.16 yields that
where \(\mathsf {e}_1\in {\mathbb {R}}^n\) is the first basis vector \(\mathsf {e}_1 = (1,0,\ldots ,0)^t\) of \({\mathbb {R}}^n\). As a consequence, the vector \(\zeta \overline{\mathsf A}_{0}(\mathsf {w}^\star )\phi + \overline{\mathsf A}_{}(\mathsf {w}^\star , \varvec{\xi }) \phi \) may have its first component zero with \(\zeta = - \varvec{v}\varvec{\cdot }\varvec{\xi }\) but its velocity components never vanish since \(\varvec{\xi }\in \Sigma ^{d-1}\) and (\(\mathsf K _\mathsf{3 }\)) holds. \(\square \)
The local strict dissipativity properties now imply global existence and asymptotic stability of equilibrium states [89, 95, 97]. The existence proof mainly consists in establishing a priori estimates for linearized equations, then a local existence theorem, next a priori estimates independent of the time interval using strict dissipativity and finally using the local existence repeatedly [89, 95, 97, 106, 107, 118]. We present such a theorem for the equations governing multicomponent reactive flows in their natural normal form.
Theorem 7.6
Let \( d\geqslant 1\), \(l\geqslant [d/2]+2\), and consider the equations governing multicomponent reactive flows in normal form \(\mathsf {w}= (\mathsf {w}_\textsc {i},\mathsf {w}_\textsc {ii})^t\) with
Let \(\mathsf {w}^\star \) be a chemical equilibrium state as in Proposition 7.4. If the initial condition \(\mathsf {w}^0(x)\) is such that \(\Vert \mathsf {w}^0-\mathsf {w}^\star \Vert _{H^l_{}}\) is small enough, then the Cauchy problem with \(\mathsf {w}(0,x) = \mathsf {w}^0(x)\) has a global solution with
Moreover we have the estimates

and  goes to zero as \(t \rightarrow \infty \).
goes to zero as \(t \rightarrow \infty \).
From Theorem 7.6 the equilibrium point \(\mathsf {w}^\star \) is asymptotically stable and with stronger assumptions it is also possible to obtain decay estimates [95].
Theorem 7.7
Let \( d\geqslant 1\), \(l\geqslant [d/2]+3\) and assume that the initial condition \(\mathsf {w}^0(x)\) is such that \(\mathsf {w}^0-\mathsf {w}^\star \in H^l({\mathbb {R}}^d)\cap L^p({\mathbb {R}}^d)\), where \(p=1\), if \(d=1\), and \(p\in [1,2)\), if \(d\geqslant 2\). Then if \(\Vert \mathsf {w}^0-\mathsf {w}^\star \Vert _{H^l_{}}+ \Vert \mathsf {w}^0-\mathsf {w}^\star \Vert _{L^{p}_{}}\) is small enough, the global solution satisfy the dacay estimate
for \(t\in [0,\infty )\), where \(\beta \) is a positive constant and \(\gamma =d\times (1/2p-1/4)\).
Such theorems for hyperbolic-parabolic systems may be used for various other fluid models. They have been notably used for ambipolar plasmas where Poisson Equation is replaced by the zero current limit [97], partial equilibrium flows where some group of chemical reactions are assumed infinitely fast [116], as well as for Saint-Venant equations modeling thin viscous layers over fluid substrates [120].
8 Conclusion and Future Directions
We have presented in these notes the kinetic theory of reactive gas mixtures. We have extracted from this molecular setting the corresponding fluid system of partial differential equations as well as the natural assumptions for the system coefficients. We have established that these fluid equations have a hyperbolic-parabolic structure in such a way that they have local in time solutions [108] as well as global solutions around equilibrium states [9, 95, 101]. These equations also have traveling wave solutions in the low Mach number limit [119].
The models developed in the previous sections may further be generalized to describe gas mixtures in full vibrational nonequilibrium when each vibrational quantum level is treated as a separate “chemical species” allowing detailed state-to-state relaxation models [108]. When the vibrational quantum levels are partially at equilibrium between them but not at equilibrium with the translational/rotational states—allowing the definition of a vibrational temperature—a different structure is obtained. Models at thermodynamic nonequilibrium with two temperatures have also been investigated and the apparition of a volume viscosity term has been justified mathematically [104, 118].
The mathematical analysis of chemical equilibrium flows has been extended to the situation of partial chemical equilibrium [116]. However, the mathematical structure of numerous simplified chemistry methods is still obscure from a mathematical point of view at variance with partial equilibrium.
Various extensions could also consider initial-boundary value problems [121] with the possibility of inflow or outflow conditions, heat losses, surface reactions with complex heterogeneous chemistry, species surface diffusion or heat surface conduction. Various numerical analysis theoretical results could also be extended to the case of mixtures like convergence results of Petrov-Galerkin ‘Streamline–Diffusion’ finite element techniques [90, 122].
The notion of higher order entropy may also be generalized to the situation of multicomponent flows [123–126] as well as the singular limit of small Mach number flow [127, 128]. Multiphase flows with sprays governed by Boltzmann type equations [6], or derived multifluid sectional models for droplets [129], may also be investigated mathematically.
References
Applications
Rosner, D.E.: Transport Processes in Chemically Reacting Flow Systems. Butterworths, Boston (1986)
Roenigk, K.F., Jensen, K.F.: Low pressure CVD of silicon nitride. J. Electrochem. Soc. 134, 1777–1785 (1987)
Ern, A., Giovangigli, V., Smooke, M.: Numerical study of a three-dimensional chemical vapor deposition reactor with detailed chemistry. J. Comp. Phys. 126, 21–39 (1996)
Anderson Jr, J.D.: Hypersonics and High Temperature Gas Dynamics. McGraw-Hill Book Company, New York (1989)
Laboudigue, B., Giovangigli, V., Candel, S.: Numerical solution of a free-boundary problem in hypersonic flow theory: nonequilibrium viscous shock layers. J. Comp. Phys. 102, 297–309 (1992)
Williams, F.A.: Combustion Theory, 2nd edn. The Benjamin/Cummings Pub. Co., Inc., Menlo Park (1985)
Ern, A., Giovangigli, V.: Thermal diffusion effects in hydrogen/air and methane/air flames. Comb. Theor. Mod. 2, 349–372 (1998)
Poinsot, T., Veynante, D.: Theoretical and Numerical Combustion. Edwards, Philadelphia (2005)
Giovangigli, V.: Multicomponent Flow Modeling. Birkhäuser, Boston (1999)
Kinetic theory
Hirschfelder, J.O., Curtiss, C.F., Bird, R.B.: Molecular Theory of Gases and Liquids. Wiley, New York (1954)
Waldmann, L.: Transporterscheinungen in Gasen von mittlerem Druck. Handbuch der Physik 12, 295–514 (1958)
Waldmann, L., Trübenbacher, E.: Formale Kinetische Theorie von Gasgemischen aus Anregbaren Molekülen. Zeitschr. Naturforschg. 17(a), 363–376 (1962)
Van de Ree, J.: On the definition of the diffusion coefficients in reacting gases. Physica 36, 118–126 (1967)
Curtiss, C.F.: Symmetric gaseous diffusion coefficients. J. Chem. Phys. 49, 2917–2919 (1968)
Chapman, S., Cowling, T.G.: The Mathematical Theory of Non-Uniform Gases. Cambridge University Press, Cambridge (1970)
Ferziger, J.H., Kaper, H.G.: Mathematical Theory of Transport Processes in Gases. North Holland Pub. Co., Amsterdam (1972)
Cercignani, C.: The Boltzmann Equation and Its Applications, Applied Mathematical Sciences, vol. 67. Springer, Berlin (1988)
Grunfeld, C.: On a class of Kinetic equations for reacting gas mixtures with multiple collisions. C. R. Acad. Sci. Paris 316(Série I), 953–958 (1993)
Ern, A., Giovangigli, V.: Multicomponent Transport Algorithms, Lecture Notes in Physics, New Series “Monographs”, m 24 (1994)
Reactive Kinetic theory
Prigogine, I., Xhrouet, E.: On the perturbation of Maxwell distribution function by chemical reactions in gases. Physica 15, 913–932 (1949)
Prigogine, I., Mathieu, M.: Sur la Perturbation de la Distribution de Maxwell par des Réactions Chimiques en Phase Gazeuse. Physica 16, 51–64 (1950)
Takayanagi, K.: On the theory of chemically reacting gas. Prog. Theor. Phys. VI, 486–497 (1951)
Present, R.D.: On the velocity distribution in a chemically reacting gas. J. Chem. Phys. 31, 747–750 (1959)
Ludwig, G., Heil, M.: Boundary Layer Theory with Dissociation and Lonization, vol. VI, pp. 39–118. Academic Press, New York (1960) (In Advances in Applied Mechanics )
Ross, J., Mazur, P.: Some deductions from a formal statistical mechanical theory of chemical kinetics. J. Chem. Phys. 35, 19–28 (1961)
Shizgal, B., Karplus, M.: Nonequilibrium contributions to the rate of reaction. I. Perturbation of the velocity distribution function. J. Chem. Phys. 52, 4262–4278 (1970)
Shizgal, B., Karplus, M.: Nonequilibrium contributions to the rate of reaction. II. Isolated multicomponent systems. J. Chem. Phys. 54, 4345–4356 (1971)
Shizgal, B., Karplus, M.: Nonequilibrium contributions to the rate of reaction. III. Isothermal multicomponent systems. J. Chem. Phys. 54, 4357–4362 (1971)
Vallander, S., Nagnibeda, E., Rydalevskaya, M.: Some Questions of the Theory of the Chemically Reacting Gas Mixture. Leningrad University Press, Leningrad (1977) (English translation U.S. Air Force FASTC-ID (RS) TO-0608-93)
Alexeev, B.V.: Mathematical Kinetics of Reacting Gases. Nauka, Moskow (1982)
Alexeev, B.V., Chikhaoui, A., Grushin, I.T.: Application of the generalized Chapman-Enskog method to the transport-coefficient calculation in a reacting gas mixture. Phys. Rev. E 49, 2809–2825 (1994)
Chikhaoui, A., Dudon, J.P., Kustova, E.V., Nagnibeda, E.A.: Transport properties in reacting mixture of polyatomic gases. Physica A 247, 526–552 (1997)
Ern, A., Giovangigli, V.: The Kinetic equilibrium regime. Physica-A 260, 49–72 (1998)
Monaco, R., Pandolfi Bianchi, M., Soares, A.J.: BGK-type models in strong reaction and kinetic chemical equilibrium regimes. J. Phys. A 38, 10413 (2005)
Thermodynamic nonequilibrium
Kogan, M.: Rarefied Gas Dynamics. Nauka, Moskow (1967) (English translation Plenum Press, New York (1969))
Brun, R.: Transport et Relaxation dans les Ecoulements Gazeux. Masson, Paris (1986)
Zdhanov, V., Alievskiy, M.: Relaxation and Transport i Molecular Gases. Nauka, Moskow (1989)
Bruno, D., Capitelli, M., Longo, S.: Direct simulation of non-equilibrium kinetics under shock conditions in nitrogen. Chem. Phys. Lett. 360, 31–37 (2002)
Zhdanov, V.M.: Transport Processes in Multicomponent Plasmas. Taylor and Francis, London (2002)
Nagnibeda, E.A., Kustova, E.A.: Kinetic Theory of Transport and Relaxation Processes in Non-Equilibrium Reacting Flows. Saint-Petersburg University Press, Russian (2003)
Colonna, G., Armenise, I., Bruno, D., Capitelli, M.: Reduction of state-to-state kinetics to macroscopic models in hypersonic flows. J. Thermophys. Heat Transf. 20, 477–486 (2006)
Capitelli, M., Armenise, I., Bruno, D., Cacciatore, M., Celiberto, R., Colonna, G., de Pascale, O., Diomede, P., Esposito, F., Gorse, C., Hassouni, K., Laricchiuta, A., Longo, S., Pagano, D., Pietanza, D., Rutigliano, M.: Non-equilibrium plasma kinetics: a state-to-state approach. Plasma Sourc. Sci. Tech. 16, S30–S44 (2007)
Nagnibeda, E., Kustova, E.: Non-equilibrium Reacting Gas Flows. Springer, Berlin (2009)
Bruno, D., Giovangigli, V.: Relaxation of internal temperature and volume viscosity. Phys. Fluids 23, 093104 (2011)
Bruno, D., Giovangigli, V.: Erratum: Relaxation of internal temperature and volume viscosity. Phys. Fluids 25, 039902 (2013)
Bruno, D., Esposito, F., Giovangigli, V.: Relaxation of rotational-vibrational energy and volume viscosity in H-H\(_2\) mixtures. J. Chem. Phys. 138, 084302 (2013)
Mathematical kinetic theory
DiPerna, R.J., Lions, P.L.: On the global existence for boltzmann equations: global existence and weak stability. Ann. Math. 130, 321–366 (1989)
Cercignani, C., Illner, R., Pulvirenti, M.: The Mathematical Theory of Dilute Gases, Applied Mathematical Sciences, vol. 106. Springer, Berlin (1994)
Grunfeld, C., Georgescu, E.: On a class of Kinetic equations for reacting gas mixtures. Mat. Fiz. Analiz. Geom. 2, 408–435 (1995)
Lions, P.-L., Masmoudi, N.: From Boltzmann equation to the Navier-Stokes and Euler equations I. Arch. Ration. Mech. Anal. 158, 173–193 (2001)
Golse, F., Levermore, C.D.: The Stokes-Fourier and acoustic limits for the Boltzmann equation. Commun. Pure Appl. Math. 55, 336–393 (2002)
Saint-Raymond, L.: Convergence of solutions to the Boltzmann equation in the incompressible Euler limit. Arch. Ration. Mech. Anal. 166, 47–80 (2003)
Golse, F., Saint-Raymond, L.: The Navier-Stokes limit of the Boltzmann equation for bounded collision kernels. Invent. Math. 155, 81–161 (2004)
Golse, F., Saint-Raymond, L.: Hydrodynamic limits for the Boltzmann equation. Riv. Mat. Univ. Parma 7, 1–144 (2005)
Saint-Raymond, L.: Hydrodynamic limits of the Boltzmann equation. In: Lecture Notes in Mathematics 1971. Springer, Berlin-Heidelberg (2009)
Brull, S., Pavan, V., Schneider, J.: Derivation of a BGK model for mixtures. Eur. J. Mech. B/Fluids 33, 74–86 (2012)
Macroscopic theories
Marcelin, M.R.: Sur la Mécanique des Phénomènes Irréversibles. C. R. Acad. Sci. Paris 1052–1055 (1910)
Meixner, J.: Zur Thermodynamik der irreversiblen Prozesse in Gasen mit chemisch reagierenden, dissoziierenden und anregbaren Komponenten. Ann. Phys. 43, 244–270 (1943)
Prigogine, I.: Etude thermodynamique des phénomènes irréversibles. Dunod, Paris (1947)
Irving, J.H., Kirkwood, J.G.: The statistical mechanics of transport processes. IV. The equations of hydrodynamics. J. Chem. Phys. 18, 817–829 (1950)
Bearman, R.J., Kirkwood, J.G.: The statistical mechanics of transport processes. XI. Equations of transport in multicomponent systems. J. Chem. Phys. 28, 136–145 (1958)
de Groot, S.R., Mazur, P.: Non-equilibrium thermodynamics. Dover Publications.Inc, New York (1984)
Gorban, A.N., Bykov, V.I., Yablonski, G.S.: Essays on Chemical Relaxation (in Russian). Nauka, Novosibirsk (1986)
Keizer, J.: Statistical Thermodynamics of Nonequilibrium Processes. Springer, New York (1987)
Multicomponent transport algorithms
Hirschfelder, J.O., Curtiss, C.F.: Theory of propagation of flames. Part I: general equations. Proc. Comb. Inst. 3,121–127 (1949)
Williams, F.A.: Elementary derivation of the multicomponent diffusion equation. Am. J. Phys. 26, 467–469 (1958)
Dixon-Lewis, G.: Flame structure and flame reaction Kinetics, II. Transport phenomena in multicomponent systems. Proc. Roy. Soc. A 307, 111–135 (1968)
Oran, E.S., Boris, J.P.: Detailed modeling of combustion systems. Prog. Energy Combust. Sci. 7, 1–72 (1981)
Giovangigli, V.: Mass conservation and singular multicomponent diffusion algorithms. IMPACT Comput. Sci. Eng. 2, 73–97 (1990)
Giovangigli, V.: Convergent Iterative methods for multicomponent diffusion. IMPACT Comput. Sci. Eng. 3, 244–276 (1991)
Ern, A., Giovangigli, V.: Thermal conduction and thermal diffusion in dilute polyatomic gas mixtures. Physica-A 214, 526–546 (1995)
Ern, A., Giovangigli, V.: The structure of transport linear systems in dilute isotropic gas mixtures. Phys. Rev. E 53, 485–492 (1996)
Ern, A., Giovangigli, V.: Optimized transport algorithms for flame codes. Comb. Sci. Tech. 118, 387–395 (1996)
Ern, A., Giovangigli, V.: Projected iterative algorithms with application to multicomponent transport. Linear Algebra Appl. 250, 289–315 (1997)
Giovangigli, V.: Multicomponent transport algorithms for partially ionized plasmas. J. Comp. Phys. 229, 4117–4142 (2010)
Impact of multicomponent transport
Hancock, R.D., Schauer, F.R., Lucht, R.P., Katta, V.R., Hsu, K.Y.: Thermal diffusion effects and vortex-flame interactions in hydrogen jet diffusion flames. Proc. Combust. Inst. 26, 1087–1093 (1996)
Ern, A., Giovangigli, V.: Impact of multicomponent transport on planar and counterflow hydrogen/air and methane/air flames. Comb. Sci. Tech. 149, 157–181 (1999)
Bendahklia, R., Giovangigli, V., Rosner, D.: Soret effects in laminar counterflow spray diffusion flames. Comb. Theory Mod. 6, 1–17 (2002)
Hilbert, R., Tap, F., El-Rabii, H., Thevenin, D.: Impact of detailed chemistry and transport models on turbulent combustion simulations. Prog. Ener. Comb. Sci. 30, 61–117 (2004)
Galkin, V.S., Rusakov, S.V.: On the theory of bulk viscosity and relaxation pressure. Appl. Math. Mech. 69, 943–954 (2005)
Elizarova, T., Kholkov, A., Montero, S.: Numerical simulaition of shock waves structure in nitrogen. Phys. Fluids 19, 068102 (2007)
Billet, G., Giovangigli, V., de Gassowski, G.: Impact of volume viscosity on a shock/hydrogen bubble interaction. Comb. Theor. Mod. 12, 221–248 (2008)
Dworkin, S., Smooke, M.D., Giovangigli, V.: The impact of detailed multicoponent transport and thermal diffusion effects on soot formation in ethylene/air flames. Proc. Comb. Inst. 32, 1165–1172 (2009)
Kustova, E.: On the role of bulk viscosity and relaxation pressure in nonequilibrium flows. In: Abe (ed.) 26th International Symposiuù on Rarefied Gas Dynamic, AIP Conference Proceedings 1024, pp. 807–812 (2009)
Armenise, I., Kustova, E.: On different contributions to the heat flux and diffusion in nonequilibrium flows. Chem. Phys. 428, 90–104 (2014)
Hyperbolic-parabolic systems
Godunov, S.: An interesting class of quasilinear systems. Sov. Math. Dokl 2, 947–949 (1961)
Lady\(\tilde{\text{ z }}\)enskaja, O.A., Solonikov, V.A., Ural’ceva, N.N.: Linear and Quasilinear Equations of Parabolic Type. Translations of Mathematical Monographs, 23, American Mathematical Society, Providence, Rhode Island (1968)
Friedrichs, K.O., Lax, P.D.: Systems of conservation laws with a convex extension. Proc. Nat. Acad. Sci. USA 68, 1686–1688 (1971)
Kawashima, S.: Systems of Hyperbolic-Parabolic Composite type, with Application to the Equations of Magnetohydrodynamics. In: Doctoral Thesis, Kyoto University (1984)
Hughes, T.J.R., Franca, L.P., Mallet, M.: A new finite element formulation for computational fluid dynamics: I. Symmetric forms of the compressible Euler and Navier-Stokes equations and the second law of thermodynamics. Comp. Meth. Appl. Mech. Eng. 54, 223–234 (1986)
Ruggeri, T.: Thermodynamics and Symmetric Hyperbolic Systems, pp. 167–183. Rend. Sem. Mat. Univ. Torino, Hyperbolic Equations (1987)
Kawashima, S., Shizuta, Y.: On the normal form of the symmetric hyperbolic-parabolic systems associated with the conservation laws. Tôhoku Math. J. 40, 449–464 (1988)
Chen, G.Q., Levermore, C.D., Liu, T.P.: Hyperbolic conservation laws with stiff relaxation terms and entropy. Commun. Pure Appl. Math. 47, 787–830 (1994)
Serre, D.: Systèmes de Lois de Conservation I and II. Diderot Editeur. Art et Science, Paris (1996)
Giovangigli, V., Massot, M.: Asymptotic stability of equilibrium states for multicomponent reactive flows. Math. Mod. Meth. Appl. Sci. 8, 251–297 (1998)
Dafermos, C.: Hyperbolic Conservation Laws in Continuum Physics. Springer, Heidelberg (2000)
Giovangigli, V., Graille, B.: Asymptotic stability of equilibrium states for ambipolar plasmas. Math. Mod. Meth. Appl. Sci. 14, 1361–1399 (2004)
Kawashima, S., Yong, W.-A.: Dissipative structure and entropy for hyperbolic systems of conservation laws. Arch. Ration. Mech. Anal. 174, 345–364 (2004)
Kawashima, S., Yong, W.A.: Decay estimates for hyperbolic balance laws. J. Anal. Appl. 28, 1–33 (2009)
Serre, D.: The structure of dissipative viscous system of conservation laws. Physica D 239, 1381–1386 (2010)
Giovangigli, V., Matuszewski, L.: Mathematical modeling of supercritical multicomponent reactive fluids. Math. Mod. Meth. Appl. Sci. 23, 2193–2251 (2013)
Dafermos, C.M.: Hyperbolic systems of balance laws with weak dissipation II. J. Hyp. Diff. Equat. 10, 173–179 (2013)
Giovangigli, V., Matuszewski, L.: Structure of entropies in dissipative multicomponent fluids. Kin. Relat. Mod. 6, 373–406 (2013)
Giovangigli, V., Yong, W.A.: Volume viscosity and fast internal energy relaxation: symmetrization and Chapman-Enskog expansion. Kin. Relat. Mod. (in press)
Cauchy problem
Vol’Pert, A.I., Hudjaev, S.I.: On the Cauchy problem for composite systems of nonlinear differential equations. Math. USSR Sbornik. 16, 517–544 (1972)
Shizuta, Y., Kawashima, S.: Systems of equations of hyperbolic-parabolic type with applications to the discrete Boltzmann equation. Hokkaido Math. J. 14, 249–275 (1985)
Kawashima, S.: Large-time behavior of solutions to hyperbolic-parabolic systems of conservations laws and applications. Proc. Roy. Soc. Edinb. 106A, 169–1944 (1987)
Giovangigli, V., Massot, M.: The local Cauchy problem for multicomponent reactive flows in full vibrational nonequilibrium. Math. Meth. Appl. Sci. 21, 1415–1439 (1998)
Feireisl, E.: Dynamics of Viscous Compressible Fluids. Oxford University Press, Oxford (2004)
Yong, W.-A.: Entropy and global existence for hyperbolic balance laws. Arch. Ration. Mech. Anal. 172, 247–266 (2004)
Feireisl, E., Novotný, A.: The low mach number limit for the full Navier-Stokes-Fourier system. Arch. Ration. Mech. Anal. 186, 77–107 (2007)
Feireisl, E., Petzeltoá, H., Trivisa, K.: Multicomponent reactive flows: global-in-time existence for large data. Commun. Pure Appl. Anal. 7, 1017–1047 (2008)
Yong, W.A.: An interesting class of partial differential equations. J. Math. Phys. 49, 033503 (2008)
Kwong, Y.S., Trivisa, K.: Stability and large-time behavior for multicomponent reactive flows. Nonlinearity 22, 2443–2471 (2009)
Hopf, D.: Asymptotic behavior of solutions to a model for the flow of a reacting fluid. Arch. Ration. Mech. Anal. 196, 951–979 (2010)
Giovangigli, V., Massot, M.: Entropic structure of multicomponent reactive flows with partial equilibrium reduced chemistry. Math. Meth. Appl. Sci. 27, 739–768 (2004)
Beauchard, K., Zuazua, E.: Large time asymptotics for partially dissipative hyperbolic systems. Arch. Ration. Mech. Anal. 199, 177–227 (2011)
Giovangigli, V., Yong, W.A.: Volume Viscosity and Fast Internal Energy Relaxation: Error Estimates (submitted for publication)
Miscelaneous
Giovangigli, V.: Plane flames with multicomponent transport and complex chemistry. Math. Mod. Meth. Appl. Sci. 9, 337–378 (1999)
Giovangigli, V., Tran, B.: Mathematical analysis of a Saint-Venant model with variable temperature. Math. Mod. Meth. Appl. Sci. 20, 1–47 (2010)
Matsumura, A., Nishida, T.: The initial value problem for the equations of motion of a viscous and heat-conductive fluids. J. Math. Kyoto Univ. 200, 67–104 (1980)
Johnson, C., Szepessy, A., Hansbo, P.: On the convergence of shock-capturing streamline diffusion finite element methods for hyperbolic conservation laws. Math. Comput. 54, 107–129 (1990)
Giovangigli, V.: Higher order entropies. Arch. Ration. Mech. Anal. 187, 221–285 (2008)
Giovangigli, V.: Higher order entropies for compressible fluid models. Math. Mod. Meth. Appl. Sci. 19, 67–125 (2009)
Evans, L.C.: A survey of entropy methods for partial differential equations. Bull. AMS 41, 409–438 (2004)
Bresch, D., Desjardins, B.: On the existence of global weak solutions to the Navier-Stokes equations for viscous compressible and heat conducting fluids. J. Math. Pure Appl. 87, 57–90 (2007)
Alazard, T.: Low mach number limit of the full Navier-Stokes equations. Arch. Ration. Mech. Anal. 180, 1–73 (2006)
Feireisl, E., Novotnỳ, A.: Singular Limits in Thermodynamics of Viscous Fluids. Birkhäuser, Basel (2009)
Laurent, F., Massot, M.: Multi-fluid modeling of laminar poly-dispersed spray flames: origin, assumptions and comparison of the sectional and sampling methods. Comb. Theor. Mod. 5, 537–572 (2001)
Author information
Authors and Affiliations
Corresponding author
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2015 Springer International Publishing Switzerland
About this paper
Cite this paper
Giovangigli, V. (2015). Dissipative Reactive Fluid Models from the Kinetic Theory. In: Gonçalves, P., Soares, A. (eds) From Particle Systems to Partial Differential Equations II. Springer Proceedings in Mathematics & Statistics, vol 129. Springer, Cham. https://doi.org/10.1007/978-3-319-16637-7_2
Download citation
DOI: https://doi.org/10.1007/978-3-319-16637-7_2
Published:
Publisher Name: Springer, Cham
Print ISBN: 978-3-319-16636-0
Online ISBN: 978-3-319-16637-7
eBook Packages: Mathematics and StatisticsMathematics and Statistics (R0)





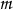 is given by
is given by
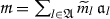 .
.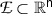 such that
such that  for
for  and if and only if
and if and only if 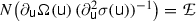 .
.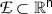 such that
such that 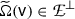 for
for 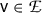 and if and only if
and if and only if 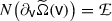 .
.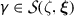 have a negative real part
have a negative real part  have their real part majorized by
have their real part majorized by