On the Stability Margins of Parametrically Excited Rotating Shafts on Gas Foil Bearings: Linear and Nonlinear Approach

Abstract

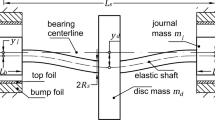
Parametric excitation is applied in a realistic multi-segmented rotating shaft of a turbopump mounted on two active gas foil bearings. The active configuration of the two gas foil bearings is defined by a periodic external load of specific amplitude and frequency which alternates the top foil configuration and in this manner the gas film impedance forces experience periodic variation.

The analytical model of the rotor is obtained using a reduced finite element model, and the Reynolds equation for the compressible flow of the gas is solved applying a reduced finite difference scheme.

Fully balanced rotors are investigated on their potential to shift the threshold speed of instability defined by the rotating speed at which the first bifurcation of limit cycles occurs. The limit cycles are evaluated through pseudo arc length continuation with an embedded collocation method. The respective amplitude and frequency of applied external excitation (parametric excitation) is investigated in order to define those characteristics (amplitude and frequency) which render parametric antiresonance in the rotating system. Two approaches are included. At the first, the parametric excitation is implemented in the system, through periodically varying stiffness and damping coefficients of the gas foil bearings, which are evaluated solving the perturbed Reynolds equation; this is the linear version of the dynamic system. At the second, direct implementation of gas bearing impedance forces is considered; this is the nonlinear version of the dynamic system. Comparing the two approaches, it is found that antiresonance occurs in specific excitation frequencies, and the rotating system operates without bifurcation at speeds two times higher than the respective speed of the reference system without excitation. Several design scenarios for rotor slenderness and bearing configuration are included in the results.

Appendix: Implementation of Parametric Excitation

The following analysis considers the physical and geometrical properties (inner /outer radius $R_{i,r} ,R_{o,r}$, young modulus of elasticity, Poisson’s ratio $v_{r}$) of the bearing ring as known and denotes:

$$ \begin{array}{*{20}c} {\kappa_{1} = 1 - \frac{{\left( {R_{o,r}^{4} - R_{i,r}^{4} } \right)}}{{2R_{i,r}^{2} \left( {R_{o,r}^{2} - R_{i,r}^{2} } \right)}} + \frac{{1.33\left( {1 + 2v_{r} } \right)R_{o,r} }}{{\pi \left( {R_{o,r}^{2} - R_{i,r}^{2} } \right)}},} & {\kappa_{2} = 1 - \frac{{\left( {R_{o,r}^{4} - R_{i,r}^{4} } \right)}}{{2R_{i,r}^{2} \left( {R_{o,r}^{2} - R_{i,r}^{2} } \right)}}} \\ \end{array} $$

(26)

Inspired by the analytically computed deformation of a ring under the effect of a periodic vertical load $F_{0} \left( {1 + \sin \left( {\overline{\Omega }_{ex} \tau } \right)} \right)$, the author defines the horizontal and vertical deformation of the bearing ring as in Eq. (27) where $F_{0}$ denotes the amplitude of the periodic vertical load and $I_{r}$ denotes the polar moment of inertia of the deformable ring.

$$ \begin{gathered} q_{r} \left( {\theta = 0,\pi ,\tau } \right) = dh = \frac{{F_{0} }}{2}\frac{{R_{o,r}^{3} }}{{4.2 \cdot 10^{11} I_{r} }}\left( {\frac{2}{\pi }\kappa_{2}^{2} - \kappa_{2} + \frac{{\kappa_{1} }}{2}} \right)\left( {1 + \sin (\overline{\Omega }_{ex} \tau )} \right) \\ q_{r} \left( {\theta = \pi /2,3\pi /2,\tau } \right) = dv = \frac{{ - F_{0} }}{2}\frac{{R_{o,r}^{3} }}{{4.2 \cdot 10^{11} I_{r} }}\left( {\frac{\pi }{4}\kappa_{1} - \frac{{2\kappa_{2}^{2} }}{\pi }} \right)\left( {1 + \sin (\overline{\Omega }_{ex} \tau )} \right) \\ \end{gathered} $$

(27)

The corresponding derivatives with respect to the dimensionless time are given in Eq. (28).

$$ \begin{gathered} d\dot{h} = \frac{{F_{0} }}{2}\frac{{R_{o,r}^{3} }}{{4.2 \cdot 10^{11} I_{r} }}\left( {\frac{2}{\pi }\kappa_{2}^{2} - \kappa_{2} + \frac{{\kappa_{1} }}{2}} \right)\left( {\overline{\Omega }_{ex} \cos (\overline{\Omega }_{ex} \tau )} \right) \hfill \\ d\dot{v} = \frac{{ - F_{0} }}{2}\frac{{R_{o,r}^{3} }}{{4.2 \cdot 10^{11} I_{r} }}\left( {\frac{\pi }{4}\kappa_{1} - \frac{{2\kappa_{2}^{2} }}{\pi }} \right)\left( {\overline{\Omega }_{ex} \cos (\overline{\Omega }_{ex} \tau )} \right) \hfill \\ \end{gathered} $$

(28)

The deformation of the outer ring and its rate of change are then evaluated in circumferential direction in Eq. (29), and in dimensionless form in Eq. (30).

$$ \begin{gathered} q_{r} \left( {\theta ,\tau } \right) = q_{r} = \sqrt {\left[ {\left( {R_{i,r} + dh} \right)\cos \theta } \right]^{2} + \left[ {\left( {R_{i,r} + dv} \right)\sin \theta } \right]^{2} } - R_{i,r} \\ \dot{q}_{r} \left( {\theta ,\tau } \right) = \dot{q}_{r} = \frac{{\left[ {\left( {R_{i,r} + dh} \right)\cos \theta } \right]\left[ {d\dot{h}\cos \theta } \right] + \left[ {\left( {R_{i,r} + dv} \right)\sin \theta } \right]\left[ {d\dot{v}\sin \theta } \right]}}{{q_{r} + R_{i,r} }} \\ \end{gathered} $$

(29)

$$ \begin{array}{*{20}c} {\overline{q}_{r} = \frac{{q_{r} }}{{c_{r} }},} & {\dot{\overline{q}}_{r} = \frac{{\dot{q}_{r} }}{{c_{r} }}} \\ \end{array} $$

(30)