Keywords

1 Introduction

Evaluation of the breach flood of earthen dams and levees due to overtopping is a subject of common concern that attracted a large volume of research works [1,2,3,4,5,6]. However, their physical models and numerical approaches are still unmatured to-date. Chen et al. [7] discussed the difficulties involved in establishing the physical model that couples the disciplines of soil erosion in hydraulics and slope stability in soil mechanics. Further, shortage of field monitoring hydrographs of dam breaches makes it difficult to validate the analytical approaches developed by various researchers.

China has recently experienced a large number of barrier lake break cases with several well-documented field measured breach hydrographs [8,9,10,11]. This has allowed an in-depth study and improvements to the existing dam breach analytical methods. The research outcomes were incorporated into an Excel/VBA spreadsheet that provides an easy and quick access for practicing engineers to work during an impending dam failure emergency. A comprehensive review of this work has been presented in a latest paper [7]. This paper summaries the key novel technical approaches in its work.

2 Soil Erosion Models

2.1 Relationship Between the Erosion Rate and Shear Stress

The relationship between the rate of soil erosion and the shear stress is a fundamental problem that has great impact on the dam breach analytical results. The relevant studies include the effort in developing better soil erosion models and apparatuses for performing the erosion tests [12].

The decrease in the channel bed elevation due to erosion per unit time, \( \dot{z} \), can be related to the shear stress τ on the eroded bed surface, as follows:

$$ \dot{z} = \frac{\Delta z}{\Delta t} = \Upphi (\tau ) $$
(1)

where z is the channel bed elevation and t is time. The function Φ(τ) proposed by researchers can be divided into the following categories.

Linear Relationship.

This model is expressed as

$$ \dot{z} = \frac{\Delta z}{\Delta t} = a_{L} (\tau - \tau_{c} ) $$
(2)

where τc is the shear stress associated with the incipient velocity Vc, and aL is the detachment rate coefficient [6, 13,14,15].

Power Law Relationship.

There are a number of computer programs using the relationships based on the sedimentation dynamics, such as BREACH [5], BEED [16], and MIKE11 [17]. In general, the power relationship is [18]

$$ \dot{z} = \frac{\Delta z}{\Delta t} =\Phi (\tau ) = a_{1} (\tau - \tau_{c} )^{{b_{1} }} $$
(3)

where \( \dot{z} \) is the erosion rate in 10−3 mm/s, τ is in Pa, t is in second, and a1 and b1 are coefficients either regressed from the test results or based on experience. Zhou et al. [19] compares the predicted soil erosion rates from 16 models with the measured rates in Tangjiashan Barrier Lake, as shown in Fig. 1.

Fig. 1.
figure 1

Comparison of measured soil erosion rates with predicted rates by 16 models in Tangjiashan Barrier Lake. NOTE: According to the data of Tangjiashan barrier lake, the input parameters of this Figure are: water density, ρ = 1000 kg/m3; ρs = 2650 kg/m3; the medium size, d50 = 5 mm; roughness coefficient, n = 0.025; void ratio, e = 0.36; flow velocity, V = (2.36, 2.50, 3.99, 4.61, 5.90) m/s and the corresponding the depth of water in channel, h = (6.94, 7.49, 8.06, 8.19, 11.86) m.

Full size image

Hyperbolic Relationship.

The preceding mentioned linear and power models have been developed via extensive testing works based on the theory of sedimentation dynamics and validated mostly in laboratory at low flow velocity for fine materials. It is difficult to verify these models in a real dam breach, in which the flow velocity can reach 5 m/s or more and the eroded soils contain large rock fragments. As shown in Fig. 1, it can be seen that at low shear stress, the predictions from these models more or less agree with the measured data. However, for the maximum flow velocity when the corresponding shear stress is near the peak, the power law relationship continues to predict high erosion rate. Further, the soil erosion rate is very sensitive to even a small change in the exponent b1 in Eq. (3).

In performing the back analysis for the Tangjiashan Barrier Lake, the authors proposed a hyperbolic relationship (Fig. 2) that takes the following form [1]:

Fig. 2.
figure 2

Relationship between soil erosion rate and shear stress in the hyperbolic model.

Full size image
$$ \dot{z} =\Phi (\tau ) = \frac{v}{a + bv} $$
(4)

where v is the shear stress with reference to its critical component τc

$$ v = k(\tau - \tau_{c} ) $$
(5)

with a unit of Pa for τ and 10−3 mm/s for \( \dot{z} \). k is a unit conversion factor, normally takes a value of 100. The hyperbolic curve has an asymptote represented by 1/b as v approaches infinity, and 1/a represents the tangent of this curve at v = 0.

The proposed model can be interpreted as a combination of the linear relationship with a ‘truncation’ at a certain high flow velocity. Approximately, 1/a equals aL in Eq. (2). The asymptote 1/b represents the ‘strength’, which has been referred to as the ‘maximum possible erosion rate’ in this study. This is based on the understanding that for most structural materials, when the shear strength is sufficiently large, the erosion rate ‘yields’. Use of this model can prevent the calculated erosion rate becoming too large at high flow velocity. Hence, the computation results are less sensitive to the input parameters in the model. Further, since both 1/a and 1/b have sound physical meanings, use of these parameters can be based on common sense, which can be improved with increased knowledge. The authors collected some laboratory testing results to support this model [20].

2.2 Soil Erodibility Test

A variety of testing facilities have been developed as reported by Foster et al. [21], Temple [22], Shaikh et al. [23], Hanson and Simon [24], Wan and Fell [25], Zhu et al. [26], Chang and Zhang [27], and Wu [6] conducted field jet index tests to measure the erodibility of broadly graded landslide deposits and found the soil erosion resistance coefficient of Tangjiashan materials is in a range between 0.2 × 10−3 and 1.2 × 10−3 mm/s/Pa.

A new apparatus, called Cylindrical Erosion Test Apparatus (CETA) has been developed by the authors’ research group, as shown in Fig. 3 (Refer to Ma [28]). It includes a cylindrical, stainless steel container with an internal diameter of 1,040 mm. There are three glass windows to allow for visual inspection during the test. The propeller is driven by a speed adjustable motor with a maximum rotating speed of 1400 r/min. Similar to EFA, the soils under test is contained in a 160 mm long, 80 mm wide box, which can be lifted up to 150 mm manually as the erosion proceeds. Using a pitot tube and the FP111 direct-reading velocity meter, the reel rotating rate has been calibrated to relate to the flow velocity on the soil/water surface (refer to Fig. 4). Figure 5 shows the test results of a medium size sand with d50 = 8 mm. The dry density is 1.8 g/cm3.

Fig. 3.
figure 3

The Cylindrical Erosion Test Apparatus (CETA).

Full size image
Fig. 4.
figure 4

Parts of CETA: (a) Speed adjustable motor (b) propeller (c) manually operated slot (d) box containing soils fixed in the slot.

Full size image
Fig. 5.
figure 5

Erodibility test results of sandy soil. NOTE: The solid line represents a regression curve based on the hyperbolic model. The asymptote 1/b was postulated due to the limitation of insufficiently large flow velocity.

Full size image

3 Modeling Lateral Enlargement

3.1 Slope Stability of the Channel Banks

During the breach, the banks of the discharge channel collapse due to soil erosion that cut the toes. The wedge slide method has been commonly used in various dam breach models to model the processes in destabilizing the bank and widening the discharge channel (Singh and Scarlatos [29]). From the geotechnical point of view, the straight-line slip surface employed in this method is not representative of the actual failures observed in the field. It is better to replace the straight lines with a more generalized shape, such as a circular arc. Apart from this limitation, Wang et al. [30] also discussed the other drawbacks in the existing dam breach models, such as:

  1. (1)

    A majority of the existing models fails to consider the existence of a vertical cut at the slope toe which is the key factor that destabilizes the bank.

  2. (2)

    The method of determining the critical slip surface has not been clearly formulated. Wang et al. [30] discussed the proposed method by Osman and Thorne [31] who did consider the locations of the toe cut and the critical slip surface. However, they tried to find the critical toe-cutting depth by the derivatives with respect to the cohesion value of the material, which is physically unacceptable from geotechnical point of view. It is necessary to adopt the well accepted knowledge in soil mechanics that the critical slip surface is obtained by finding the minimum factor of safety associated with the geometrical coordinates that define the shape and location of the failure surface [32, 33].

  3. (3)

    The pore water pressure has been invariably ignored in the analysis. The dam body prior to a breaching failure is normally saturated if the material is impervious, such as the earth core or tailings. Hence, in the analysis, the pore pressure development that causes sudden changes in the stress field need proper treatment, both in effective stress and in total stress [34,35,36].

3.2 Improved Approaches

In view of the drawbacks, Wang et al. [30] have made the effort to improve the model based on modern geotechnical expertise which includes: (1) stability analysis with circular slip surfaces using Bishop’s simplified method, (2) assignment of a vertical cut at the toe, (3) a search technique to find the minimum factor of safety and the critical depth of toe cutting, and (4) an option for determining total stress and effective stress. Figure 6(a) shows an example that is circular slip surface of modeling lateral enlargement.

Fig. 6.
figure 6

Modeling lateral enlargement: (a) circular slip surfaces (b) straight line simplifications.

Full size image

Although the lateral enlargement simulation offers better geotechnical background (Fig. 6(a)), the details of the 10 steps of circular arcs as input to a computer program are too tedious for a dam breach analysis. Wang et al. [30] found that without too much loss in accuracy, the circular arc can be simplified as a straight line as shown in Fig. 6(b).

Based on an extensive regression work, Chen et al. [37] suggested an empirical hyperbolic relationship to determine the channel width associated with the channel bed elevation z, refer to Fig. 6(b).

$$ B = B_{0} + 2\Delta z + 2h\,\tan \,(\beta - \frac{\pi }{2}) $$
(6)

where

$$ \Delta z = z - z_{0} $$
(7)

and

$$ \beta = \beta_{0} + \Delta \beta = \beta_{0} + \frac{\Delta z}{{m_{ 1} \Delta z{ + }m_{ 2} }} $$
(8)

β is the slope of the channel bed, z0 and β0 are initial values of bed elevation and bank inclination respectively. m1 and m2 are parameters of the hyperbolic relationship which are functions of cohesion c, coefficient of friction tanφ and the soil bulk density γ. Empirical charts for determining m1 and m2 have been provided by the authors.

4 Numerical Modeling of Sam Breach Flood

4.1 Governing Equations

The numerical model considers the balance of water quantity, the continuous vertical toe cutting and lateral enlargement, which can be formulated as follows.

Conservation of Energy and Mass.

This condition necessitates the balance between the volumes of inflow through the breach, which is normally calculated by the broad-crested weir flow equation, and the reduction of the reservoir storage per unit time, as follows:

$$ Q = CB(H - z)^{3/2} = \frac{\Delta W}{\Delta H}\frac{\Delta H}{\Delta t} + q $$
(9)

where C is the discharge coefficient whose theoretical value is 1.7 m1/2/s [29]. Earlier studies have used values of C ranging from 1.3 to 1.7 [38]. H is the reservoir water elevation (Fig. 7, Chen et al. [1]), and q is the natural inflow into the reservoir.

Fig. 7.
figure 7

Flow over a broad-crested weir.

Full size image

Constitutive Model of Soil Erodibility.

The formula describing the rate of channel bed erosion has been given in Eq. (4) in which the shear stress can be calculated using Manning equation, as follows:

$$ \tau = \gamma R^{\prime}J = \frac{{\gamma n^{2} V^{2} }}{{R^{{\prime}^{{\frac{1}{3}}}} }} \approx \frac{{\gamma n^{2} V^{2} }}{{h^{{\frac{1}{3}}} }} $$
(10)

where γ is the density of water, n is the roughness coefficient (= 0.025 m−1/3·s in this study), J is the slope of the channel, and R′ is the hydraulic radius that can be approximated by h if the channel width B is sufficiently larger than the average flow depth h.

Determination of Water Depth Behind the Weir.

The water level normally drops at the entrance of the weir if the flow is not submerged by the tailwater. Therefore, it is necessary to determine the flow depth h as shown in Fig. 7. Assuming the steady, uniform flow through the discharge channel, using Manning equation, Singh and Scarlatos [29] developed the following formula to determine h:

$$ h = \left( {\frac{nQ}{{BJ^{0.5} }}} \right)^{0.6} $$
(11)

Use of this equation requires an input of J which is difficult to be properly defined in a dam breach problem. Experience has shown that this parameter is very sensitive to the calculated peak flow. Chen et al. [1] found that Eq. (11) can be further elaborated as

$$ h = \left( {\frac{nQ}{{BJ^{0.5} }}} \right)^{0.6} = \left( {\frac{{nC(H - z)^{1.5} }}{{J^{0.5} }}} \right)^{0.6} = \frac{{n^{0.6} C^{0.6} (H - z)^{0.9} }}{{J^{0.3} }} $$
(12)

The exponent of (H − z) in Eq. (12), which is 0.9, is very close to unity. Therefore, the depth can be approximately estimated by the following simplified relationship:

$$ h = m(H - z) $$
(13)
$$ m = \frac{h}{H - z} \approx \frac{{n^{0.6} C^{0.6} }}{{J^{0.3} }} $$
(14)

m is called the water drop ratio which has sound physical meaning. The value of m can therefore be assigned based on experience. Chen et al. [1] found that the calculated peak flow is not sensitive to the value of m, if it is between 0.5 and 0.8. The velocity can then be determined by

$$ V = \frac{{C(H - z)^{3/2} }}{h} $$
(15)

4.2 Algorithm

The breach hydrograph can be obtained by solving the equations in Sect. 4.1. However, they are highly nonlinear and coupled; the authors’ following treatments have greatly facilitated the numerical algorithm.

Integration Based on Velocity Increments.

Equations (1), (9), and (15) allow H, z, and V to be solved at a particular time step Δt. However, these equations are highly nonlinear with concerns of numerical tractability. The new method performs the integration based on a velocity increment that completely linearizes of the governing equations and leads to a straight forwards calculation for the flow hydrograph.

At the velocity step from V0 to V0 + ΔV, the average velocity is

$$ \bar{V} = V_{0} + \Delta{V/2} $$
(20)

A variable s is defined as (Δz – ΔH) which can be calculated by

$$ s = \Delta z - \Delta H = 2(\frac{{m\overline{V} }}{C})^{2} - 2(H_{o} - z_{o} ) $$
(21)

from which the average velocity can be determined by

$$ \bar{V} = Cm^{ - 1} \sqrt {(H_{o} - z_{o} + \frac{s}{2}} ) $$
(22)

The increment in channel elevation Δz is first calculated by

$$ \Delta z = \frac{s}{1 - L} $$
(23)

where

$$ L = \frac{{m\bar{V}B_{o} (H_{o} - z_{o} + 0.5\,s) - q}}{{\Phi (\bar{\tau })\dot{W}}} $$
(24)

The other variables can be readily calculated once Δz is determined.

Transition Through the Threshold at Peak Velocity.

The key technology of this new method includes a criterion that detects the threshold at which the velocity attains its maximum Vm. It has been found that both (1 − L) and s in Eq. (23) approach zero at this particular point. Equation (23) is not applicable due to the limited calculation precision provided by the computer. Instead, \( \Delta z \) is calculated based on the Taylor series expansion for a value of V0 less than Vm as follows:

$$ V_{o} = V_{m} - \frac{{{\text{d}}V}}{{{\text{d}}z}}\Delta z + \frac{1}{2}\frac{{{\text{d}}^{2} V}}{{{\text{d}}z^{2} }}\Delta z^{2} = V_{m} + \frac{1}{2}\frac{{{\text{d}}^{2} V}}{{{\text{d}}z^{2} }}\Delta z^{2} $$
(25)

from which we obtain

$$ \Delta z = \sqrt {\frac{{2(V_{o} - V_{m} )}}{{{\text{d}}^{2} V/{\text{d}}z^{2} }} = } \sqrt { - \frac{2\Delta V}{{{\text{d}}^{2} V/{\text{d}}z^{2} }}} $$
(26)

In DB-IWHR, Eq. (26) will be used when the spread sheet calculation finds the value L is sufficiently close to unity, in a range between 0.985 and 1.015. Figure 8 gives the flow chart of the algorithm [37].

4.3 Computer Software

The numerical algorithm has been realized by an Excel spreadsheet supported by VBA. To assist in inputting the required parameters, the spreadsheet ‘Input’ will guide a user to fill up the cells as described in Fig. 9. They are self-explanatory, requiring minimal tutorials. By pushing the bottom “Back to ‘Calculation’ sheet”, the software turns to the main sheet ‘Calculation’.

In sheet ‘Calculation’, the user will check the input and press ‘Re Calculation’ to start the computation (Fig. 10).

DB-IWHR and its illustrating examples are available for downloading at: https://github.com/ChenZuyuIWHR/DB-IWHR.

4.4 Suggested Values of Input for Preliminary Studies

Dam breaches often happen without early warning. This has made it difficult to perform field and laboratory tests to define the material properties. The following empirical ranges of various input parameters based on the authors’ experience have been suggested for field engineers in their quick response to an impending dam failure:

Fig. 8.
figure 8

Flow chart of the algorithm.

Full size image
Fig. 9.
figure 9

Sheet ‘Input’ of DB-IWHR.

Full size image
  1. (1)

    The discharge coefficient C of a broad-crested weir is between 1.3 and 1.7 m0.5/s [38]. For dams made of rock debris and earthen material, it may be taken to be around 1.45 and 1.50, respectively.

  2. (2)

    It has been suggested that the water head drop ratio, m, should be 0.5 [39, 40]. In view of the decreasing pool water level and the rising downstream riverbed elevation Chen et al. suggested a more reasonable range for m ranged between 0.8 and 0.6.

  3. (3)

    It is the responsibility of experienced geologists to suggest the dam material property parameters (γ, c, and ϕ), but conducting quick and simple field or laboratory tests would be useful, if time permits.

  4. (4)

    Determining soil erosion parameters is the most difficult task. It is suggested to refer case records of similar nature and past experience. Table 1 gives some empirical suggestions for preliminary studies.

    Fig. 10.
    figure 10

    Sheet ‘Calculation’ of DB-IWHR.

    Full size image
    Table 1. Suggested values of a and b for preliminary studies [20].
    Full size table

5 Case Study

5.1 Breach of Tangjiashan Barrier Lake

To illustrate the technical details described in this paper, this section uses the Tangjiashan landslide dam as a case study. The authors have in fact used this case previously [1] by investigating the breach hydrograph with the 13 m deep emergency diversion channel. This study, however, investigates the breach hydrograph without the emergency diversion channel. These comparative studies are useful when a decision has to be made.

The right part of the Tangjiashan dam is 105 m high with a crest elevation of 753 m above the sea level. The storage capacity of the reservoir is 3.2 × 109 m3. Construction of a diversion channel enables the water in the reservoir to be drained at the lower elevation of 740 m, thereby reducing the volume of released reservoir water to 2.8 × 109 m3. On one occasion after the reservoir water overflowed into the channel, the measured peak flow breaching the dam was 6,500 m3/s, as compared to the peak flow of 7,160 m3/s from the back analysis [1]. Various advanced instruments were used to measure the hydrograph of the breach, followed by a field survey and laboratory tests of geotechnical properties of the dam material [8]. This study continues the evaluation of the peak flow for the dam with no diversion channel, i.e., the reservoir water starts to drain at the elevation of 753 m.

Target Case.

The inputs for the current case study are almost the same to the previous study and are summarized in Table 2.

Table 2. Input parameters for the current case study.
Full size table

Figure 6 shows the calculated results for the current case study with the inputs γsat = 24 kg/m3, c′ = 22°, and ϕ′ = 25 kPa. The model carried out the calculations for the 10 steps of circular failure to the elevation 727 m. After that, the width of the channel was not widened further because the rock of the right abutment was exposed as indicated by Chen et al. (2015). Figure 6(a) shows the processes of a typical failure.

For the current case study, zend = 727 m has been used to obtain Bend = 69 m, β0 = 143°, and βend = 169.5°. These results have been applied to Eq. (8) to determine the width of the water surface at a particular channel bed elevation.

Illustration of the Computation.

Table 3 shows the condensed contents of DB-IWHR calculations.

Line 61 starts the calculation with the conditions: H0 = 753 m, Vc = 2.7 m/s, ΔV = 0.08 m/s, z0 = 750.42 m and B0 = 16.23 m. The calculated Δz as shown in Column 15 is 0.155 m, with z = 750.273 m, H = 752.999 m, Δt = 384 s for the next step, as shown in Columns 17, 18, and 19, respectively.

Line 62 performs the second round of integration based on the new values of H, z, and V. The calculation proceeds until Line 133 at which the velocity reaches its peak value.

Line 133 represents the transition point after which a negative ΔV has been assigned. The value of L is 1.003, which is close to unity (Column 14), and s is 0.024. Equation (23) results in an abnormal value of Δz = −7.659 m (Column 15), which is due to the insufficient precision in performing the division between two very small values by Eq. (23). Hence, the Taylors formulation (Eq. 26) was used, giving Δz = 0.788 m (Column 16).

Line 134 continues the integration with the negative input of ΔV.

Line 309 indicates the termination of the calculation at V = 2.701 m/s which is equal to the incipient Vc of 2.7 m/s, as specified by Eq. (26).

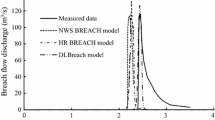
The Solid line in Fig. 11(b) shows the DB-IWHR calculation results of the target case.

Fig. 11.
figure 11

Case study Calculations: (a) regressions for the erosion parameters (b) flow discharge.

Full size image
Table 3. The condensed contents of DB-IWHR calculations.
Full size table

5.2 Sensitivity Analyses

Due to the large number of uncertainties in a dam breach flood analysis, investigation on the impact of different input values is considered as part of the analytical work. In the target case with the channeled dam, Chen et al. [1] performed the sensitivity analyses on six parameters C, m, a, b and c, ϕ. This study has added one more parameter, the initial breach width Bo. Four sets of ‘a and b’ based on the measured data have been selected as shown in Fig. 11(a). Curve 1 (the target case), 2, 3 represent the mean, upper and lower bound of the regressions. Curve 4 is a postulated one whose value of 1/b, the maximum possible erosion rate, is doubled to the target case. The results shown in Table 4 indicate that 1/b is the most sensitive parameter, compared to the others.

A comparative study has been given to a linear model as described by Eq. (2). Following the similar process, three sets of aL have been selected representing the mean, upper and lower bound of the regressions as shown in Fig. 12(a). Figure 12(b) shows the calculated hydrographs that display quite large deviations both in terms of the shape and the magnitude of peak flow. The previous report [1] discussed the concerns on sensitivities related to the power relation model. This gives a reason of adopting the hyperbolic model (Fig. 12).

Table 4. Sensitivity analyses based on various characteristic parameters.
Full size table
Fig. 12.
figure 12

Sensitivity study calculations with the linear model: (a) regressions for the erosion parameters. (b) flow discharge.

Full size image

6 Conclusions

The improvements to the existing dam breach analysis methods described in this paper include a new soil erosion model, an empirical hyperbolic model for lateral enlargement modeling, and a novel numerical algorithm that allows straight forward calculation for the breach flood hydrograph. The new method has been incorporated into the Excel spreadsheets DB-IWHR, which perform the dam breach flood calculation and the lateral enlargement calculation respectively. These spreadsheets are transparent, self-explanatory and ready for group sourcing development on the web. The main highlights are:

  1. (1)

    The hyperbolic soil erosion model developed in this study includes the parameter 1/a which is close to the relevant coefficient in the traditional linear model, and the parameter 1/b which is a ‘truncation’ at large shear stress. The new model makes the calculated peak flow less sensitive to the input parameters. In addition, these two parameters have sound physical meaning. Therefore, with increased knowledge, their applicable values for various soils can be improved in practice. As a new model with limited experience of practical application, the authors would suggest users be cautious when using this model. It is advantageous to adopt different soil erosion models and make comparative studies. DB-IWHR allows another 5 representative models for users to select.

  2. (2)

    The procedures of modeling lateral enlargement has been updated by modern geotechnical expertise on slope stability analysis However, the solutions have been simplified to a series of trapezoidal cross sections in order to facilitate the calculation without substantial loss in accuracy.

  3. (3)

    The new method developed in this study uses velocity increment during the integration of the governing equations, thus allowing a straightforward solution. The calculation procedure has been incorporated into an Excel spreadsheet, providing easy, transparent access to practitioners.

  4. (4)

    The results of this study show that the erosion rate parameter 1/b is the most sensitive input that affects the calculated peak flow. Other parameters are less sensitive, which only cause 5 to10% variations in the peak flow.