A Class of Exact Solutions for Unsteady MHD Natural Convection Flow of a Viscous Fluid over a Moving Inclined Plate with Exponential Heating, Constant Concentration and Chemical Reaction

Abstract

The purpose of this article is to study analytically the hydromagnetic natural convection flow of an electrically conducting, incompressible viscous fluid over a moving infinite inclined plate. Moreover, the dynamic of fluid is studied under the influence of exponential heating and constant concentration. Porous effects are taken into consideration and in order to investigate the influence of the transverse magnetic field, two cases when the transverse magnetic field is held fixed to the fluid or to the plate are considered. The Laplace transform technique is used to obtain exact solutions for such motions. The dimensionless Latin symbols velocity, and also the corresponding skin friction, is presented as sum of mechanical, thermal and concentration components. Finally, for illustration, as well as for a check of results, some special cases with applications in engineering are considered and influence of the system parameters is graphically brought to light.

1 Introduction

From the past few years, the study of magnetohydrodynamic (MHD) natural convection flow of electrically conducting fluids with heat and mass transfer has gained a special attention due to their multiple applications in meteorology, electrical power generation, solar physics, geophysics and chemical engineering. The study of MHD natural convection flow over a moving inclined plate has many useful consequences so attracted the attention of many researchers. For example, the flow characteristics for the natural convection boundary layer flow over a flat plate with arbitrary inclination also depend on the angle of inclination and on the distance from the leading edge [1]. Uddin and Kumar [2] noticed that as the angle of the plate from vertical direction increases the value of friction factor and heat transfer coefficient decreases while studying the unsteady free convection in a fluid flow past an infinite inclined plate immersed in a porous medium has been considered for viscous dissipative heat. Moreover, Palani [3] studied the convection effects on flow past an inclined plate with variable surface temperatures in water at 4$^{\circ }$, Singh and Makinde [4] investigated the MHD free convection flow with Newtonian heating in the presence of exponentially decaying volumetric heat source along the inclined plane. The thermal radiation effect on an unsteady MHD flow past an inclined, porous, heated plate in the presence of chemical reaction and viscous dissipation is investigated by Barik et al. [5]. Chen [6] found that increasing the angle of inclination decreases the effect of buoyancy force while investigating the natural flow over a permeable inclined surface with variable wall temperature and concentration. MHD natural convection flow with Newtonian heating and mass diffusion was analytical solved by Vieru et al. [7], when the plate applies an arbitrary time-dependent shear stress to the fluid. Fetecau et al. [8] investigated the slip effects on the radiative MHD free convection flow over a moving plate with mass diffusion and heat source. Recently, a general study of such flow with radiative effects, heat source and shear stress on the boundary has been developed by Fetecau et al. [9].

However, in all these studies as well as in many other which have been previously published, the magnetic lines of force are fixed to the fluid. There are many interesting papers [10,11,12,13,14,15,16] in which exact solutions are obtained for hydromagnetic free convection flows through porous media with heat and mass transfer, but they also correspond to the case when the magnetic field lines of force are fixed to the fluid.

The first exact solutions for free convection flows when the magnetic lines of force are fixed to the fluid or to the plate seem to be those obtained by Tokis [17] corresponding to motions induced by uniform, constantly accelerating or decaying oscillatory translations of the plate. More recently, Narahari and Debnath [18] developed an interesting study of unsteady MHD free convection flow with constant heat flux and heat source when the magnetic lines of force are fixed to the fluid or to the plate.

In this article, we present a general study of MHD natural convection flow over a moving inclined plate that is embedded in a porous medium with exponential heating, constant concentration and chemical reaction. However, our purpose is not only to extend some previous results by including porous effects and mass transfer, but we also want to provide new results both for general and oscillating motions of the inclined plate. It is worth pointing out the fact that “the fluid velocity does not remain zero at infinity if the magnetic field is fixed to the plate” [19, 20]. Moreover, to investigate the contribution of mechanical, thermal and concentration influence on the fluid velocity as well as on skin friction, we will present these as a sum of mechanical, thermal and concentration components.

2 Statement of the Problem

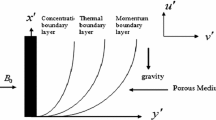
By choosing a suitable cartesian coordinate system $Oxy^{\prime }z$, let us consider the unsteady free convection flow of an electrically conducting incompressible viscous fluid over a non-conducting infinite inclined plate making an angle $\gamma$ with the verticle and in the presence of a uniform magnetic field of strength B. The magnetic field is applied perpendicular to the plate and its magnetic lines of force are fixed to the fluid or to the plate. Initially, the plate and the fluid are at rest at the constant temperature $T_{\infty }^{\prime }$ and species concentration $C_{\infty }^{\prime }$. After the time $t^{\prime }=0^{+}$, the plate begins to slide in its plane against the gravitational field with the velocity $u_{0}g^{\prime }\left( t^{\prime }\right)$ and its temperature is maintained at the value $T_{\infty }^{\prime }+T_{w}^{\prime }\left( 1-ae^{-b^{\prime }t^{\prime }}\right)$ where a, $b^{\prime }$ and $T_{w}^{\prime }$ are constants. Moreover, is a constant velocity, a piecewise continuous function with the condition that $g^{\prime }\left( 0\right) =0$. The plate is also maintained at a constant concentration $C_{w}^{^{\prime }}$.

We assume that all physical properties are constant except the density variation with temperature in the body force and the induced magnetic field is negligible in comparison with the applied magnetic field B. Furthermore, neglecting viscous dissipation and Joule heating and taken into consideration porous and radiative effects, the chemical reaction between the fluid and species concentration. Under the usual Boussinesq’s approximation, our problem reduces to the following set of partial differential equations [18, 19].

$$\begin{aligned} \left\{ \begin{array}{ll} \displaystyle \frac{\partial u^{\prime }}{\partial t^{\prime }}=\nu \frac{\partial ^{2}u}{ \partial y^{\prime 2}}+g\beta _{T}\left( T^{\prime }-T_{\infty }^{\prime }\right) \cos \gamma +g\beta _{c}\left( C^{\prime }-C_{\infty }^{\prime }\right) \cos \gamma -\frac{\nu }{K^{\prime }}u^{\prime }-\frac{\sigma B_{0}^{2}}{\rho }\left( u^{\prime }-lu_{0}g^{\prime }\left( t^{\prime }\right) \right) , \\ \\ \displaystyle \rho c_{p}\frac{\partial T^{\prime }}{\partial t^{\prime }}=k\frac{\partial ^{2}T^{\prime }}{\partial y^{\prime 2}}-\frac{\partial q_{r}}{\partial y^{\prime }},\\ \\ \displaystyle \frac{\partial C^{\prime }}{\partial t^{\prime }}=D \frac{\partial ^{2}C^{\prime }}{\partial y^{\prime 2}}-R^{\prime }\left( C^{\prime }-C_{\infty }^{\prime }\right) ,\\ \\ \displaystyle y^{\prime },t^{\prime }>0, \end{array}\right. \end{aligned}$$

(1)

with the initial and boundary conditions

$$\begin{aligned} \left\{ \begin{array}{ll} \displaystyle u^{\prime }\left( y^{\prime },0\right) =0, T^{\prime }(y^{\prime },0)=T_{\infty }^{\prime }, \text { }C^{\prime }(y^{\prime },0)=C_{\infty }^{\prime }, y^{\prime }\ge 0, \\ \\ \displaystyle u^{\prime }\left( 0,t^{\prime }\right) =u_{0}g^{\prime }\left( t^{\prime }\right) ,T^{\prime }(0,t^{\prime })=T_{\infty }^{\prime }+T_{w}^{\prime }\left( 1-ae^{-b^{\prime }t^{\prime }}\right) ,\text { } C^{\prime }(0,t^{\prime })=C_{w}^{\prime },t^{\prime }\ge 0, \\ \\ \displaystyle u^{\prime }\left( y^{\prime },t^{\prime }\right) \rightarrow 0, T^{\prime }(y^{\prime },t^{\prime })\rightarrow T_{\infty }^{\prime },\text { }C^{\prime }(y^{\prime },t^{\prime })\rightarrow C_{\infty }^{\prime },\text { }y^{\prime }\rightarrow \infty , \end{array}\right. \end{aligned}$$

(2)

Into above equations, the unknown functions $\displaystyle u^{\prime }\left( y^{\prime },t^{\prime }\right)$, $T^{\prime }(y^{\prime },t^{\prime })$ and $\displaystyle C^{\prime }(y^{\prime },t^{\prime })$ are the velocity, the temperature and the species concentration while $\displaystyle \nu ,$ g, $\beta _{T},$ $\beta _{C},$ $K^{\prime }$, $\sigma ,$ $\rho ,$ $c_{p},$ k, D, $R^{\prime }$ and $q_{r}$ are defined in the nomenclature. The parameter l is 0 when the magnetic field is fixed relative to the fluid (MFFRF) and 1 (one) when the magnetic field is fixed relative to the plate (MFFRP).

By adopting the Rosseland diffusion approximation for an optically thick fluid (see Seth et al. [21] or Narahari and Dutta [22])

$$\begin{aligned} \displaystyle q_{r}=-\frac{4}{3}\frac{\sigma }{k_{R}}\frac{\partial T^{\prime 4}}{\partial y^{\prime }}, \end{aligned}$$

(3)

assuming the temperature difference between the fluid temperature and the free stream temperature to be small enough, the energy equation (2.2)$_{1}$ can be written in the form [23, 24].

$$\begin{aligned} \displaystyle {\Pr }_{eff}\frac{\partial T^{\prime }(y^{\prime },t^{\prime })}{\partial t^{\prime }}=\frac{\partial ^{2}T^{\prime }(y^{\prime },t^{\prime })}{ \partial y^{\prime 2}};\text { }y^{\prime },t^{\prime }>0, \end{aligned}$$

(4)

where $\displaystyle {\Pr }_{eff}=\frac{\Pr }{1+N_{r}},$ $\displaystyle \Pr =\frac{\mu c_{p}}{k}$ and $\displaystyle N_{r}=\frac{16}{3}\frac{\sigma }{kk_{R}}T_{\infty }^{3}.$

Introducing the following dimensionless variables, functions and parameters

$$\begin{aligned} \left\{ \begin{array}{ll} \displaystyle y =\frac{u_{0}}{\nu }y^{\prime },\text { }t=\frac{u_{0}^{2}}{\nu }t^{\prime },\text { }u=\frac{u^{\prime }}{u_{0}},\\ \\ \displaystyle T=\frac{T^{\prime }-T_{\infty }^{\prime }}{T_{w}^{\prime }},\text { }C=\frac{C^{\prime }-C_{\infty }^{\prime }}{C_{w}^{\prime }-C_{\infty }^{\prime }},\text { }b= \frac{\nu }{u_{0}^{2}}b^{\prime },\text { }K=\left( \frac{\nu }{u_{0}} \right) ^{2}\frac{1}{K^{\prime }}, \\ \\ \displaystyle R =\frac{\nu }{u_{0}^{2}}R^{\prime },\text { }g\left( t\right) =g^{\prime }\left( \frac{\nu }{u_{0}^{2}}t^{\prime }\right) , \end{array}\right. \end{aligned}$$

(5)

and choosing the characteristic velocity $u_{0}$ to be equal with $\displaystyle \root 3 \of { \nu g\beta _{T}T_{w}}$, our problem reduce to the following dimensionless partial differential equations

$$\begin{aligned} \left\{ \begin{array}{ll} \displaystyle \frac{\partial u\left( y,t\right) }{\partial t}=\frac{\partial ^{2}u\left( y,t\right) }{\partial y^{\prime 2}}+T\left( y,t\right) \cos \gamma +NC\left( y,t\right) \cos \gamma -Ku\left( y,t\right) -M\left( u\left( y,t\right) -lg\left( t\right) \right) , \\ \\ \displaystyle {\Pr }_{eff}\frac{\partial T\left( y,t\right) }{\partial t}=\frac{\partial ^{2}T\left( y,t\right) }{\partial y^{2}},\\ \\ \displaystyle \frac{\partial C\left( y,t\right) }{\partial t}=\frac{1}{Sc}\frac{\partial ^{2}C\left( y,t\right) }{ \partial y^{2}}-RC\left( y,t\right) ,\\ \\ \displaystyle y,t>0, \end{array}\right. \end{aligned}$$

(6)

with the initial and boundary conditions

$$\begin{aligned} \left\{ \begin{array}{ll} \displaystyle u\left( y,0\right) =0,T(y,0)=0,\text { }C(y,0)=0,y\ge 0, \\ \\ \displaystyle u\left( 0,t\right) =g\left( t\right) ,T(0,t)=1-ae^{-b^{\prime }t^{\prime }},\text { }C(0,t)=1,t\ge 0, \\ \\ \displaystyle u\left( y,t\right) \rightarrow 0,T(y,t)\rightarrow 0,\text { } C(y,t)\rightarrow 0,y^{\prime }\rightarrow \infty , \end{array}\right. \end{aligned}$$

(7)

Into above relations, K is the inverse permeability parameter of the porous medium, R is the dimensionless chemical reaction parameter while the ratio of the buoyancy forces N, the magnetic parameter M and Schmidt number Sc are defined by

$$\begin{aligned} \displaystyle N=\frac{\beta _{C}\left( C_{w}-C_{\infty }\right) }{\beta _{T}T_{w}},\text { }M=\frac{\sigma B^{2}}{\rho }\frac{\nu }{V^{2}},\text { }Sc=\frac{\nu }{D}. \end{aligned}$$

where ${\Pr }_{eff}$ and Sc are transport parameters regarding the thermal and mass diffusivity and N represents the relative contribution of the mass transport rate on the free convection flow. Moreover, depending upon $\beta _{C}$, N can be also positive or negative because $\beta _{T}$ is always positive [20] and $N=0$ for the case when the buoyancy force effect from mass diffusion is absent.

3 Solution of the Problem

As the temperature and concentration fields corresponding to this problem can be easily obtained from previous works (see [[20], Eq. (20)], respectively [[25], Eq. (15)]). Our prime interest is to find the fluid velocity, however in order to determine it using the Laplace transform technique, we need the Laplace transforms of T(y, t) and C(y, t), namely

$$\begin{aligned} \displaystyle \bar{T}\left( y,q\right) =\left( \frac{1}{q}-\frac{a}{q+b}\right) e^{-y\sqrt{ \Pr _{eff}q}},\text { }\bar{C}\left( y,q\right) =\frac{1}{q}e^{-y\sqrt{ Sc\left( q+R\right) }}, \end{aligned}$$

(8)

obtained from [20] and [25].

Applying the Laplace transform to Eq. (2.9) and using the corresponding initial and boundary conditions, we find the differential equation

$$\begin{aligned} \displaystyle q\bar{u}\left( y,q\right)&=\frac{\partial ^{2}\bar{u}\left( y,q\right) }{ \partial y^{2}}+\bar{T}\left( y,q\right) \cos \gamma \,+\,N\bar{C}\left( y,q\right) \cos \gamma \,-\,K\bar{u}\left( y,q\right) \nonumber \\ {}&\quad \,-\,M\left( \bar{u}\left( y,q\right) \,-\,lG\left( q\right) \right) ,\text { }y,t>0, \end{aligned}$$

(9)

with the boundary conditions

$$\begin{aligned} \displaystyle \bar{u}\left( 0,q\right) =G\left( q\right) ,\bar{u}\left( y,q\right) \rightarrow 0,\text { as }y\rightarrow \infty , \end{aligned}$$

(10)

where $\displaystyle \bar{u}\left( y,q\right)$ and $\displaystyle G\left( q\right)$ denote the Laplace transforms of $\displaystyle u\left( y,t\right)$, respectively $\displaystyle g\left( t\right)$. Introducing Eqs. (3.1) into (3.2), we get

$$\begin{aligned} \displaystyle \frac{\partial ^{2}\bar{u}\left( y,q\right) }{\partial y^{2}}-\left( q+H\right) \bar{u}\left( y,q\right)= & {} \,-\,lMG\left( q\right) -\left( \frac{1}{ q}-\frac{a}{q+b}\right) e^{-y\sqrt{\Pr _{eff}q}}\cos \gamma \nonumber \\ -\,N\frac{1}{q}e^{-y\sqrt{Sc\left( q+R\right) }}\cos \gamma , \end{aligned}$$

(11)

where $H=M+K.$

The solution of the ordinary differential equation (3.4) with the boundary conditions (3.3) is

$$\begin{aligned} \displaystyle \bar{u}\left( y,q\right)&= G\left( q\right) e^{-y\sqrt{q+H}}+\varepsilon M \frac{G\left( q\right) }{q+H}\left( 1-e^{-y\sqrt{q+H}}\right) \nonumber \\&\quad \displaystyle +\frac{\left( 1-a\right) q+b}{q\left( q+b\right) \left[ \left( 1-\Pr _{eff}\right) q+H\right] }\left( e^{-y\sqrt{\Pr _{eff}q}}-e^{-y\sqrt{q+H} }\right) \cos \gamma \nonumber \\&\quad \displaystyle +\frac{N}{q\left[ \left( 1-Sc\right) q-\left( ScR-H\right) \right] }\left( e^{-y\sqrt{Sc\left( q+R\right) }}-e^{-y\sqrt{q+H}}\right) \cos \gamma . \end{aligned}$$

(12)

Next, introducing the relations

$$\begin{aligned} \displaystyle \frac{\left( 1-a\right) q+b}{q\left( q+b\right) \left[ \left( 1-{\Pr }_{eff}\right) q+H\right] }= & {} \frac{1}{1-{\Pr }_{eff}}\left[ \frac{1}{E} \frac{1}{q}-\frac{a}{E-b}\frac{1}{q+b}\right. \\&+\left. \frac{\left( 1-a\right) E-b}{E\left( b-E\right) }\frac{1}{q+E} \right] , \end{aligned}$$

$$\begin{aligned} \displaystyle&\left. \frac{1}{q\left[ \left( 1-Sc\right) q-\left( ScR-H\right) \right] }= \frac{1}{H-ScR}\left( \frac{1}{q}-\frac{1}{q+F}\right) ,\right. \\&\displaystyle \left. E=\frac{H}{1-{\Pr }_{eff}},\text { }F=\frac{ScR-H}{Sc-1}\right. , \end{aligned}$$

into Eq. (3.5), applying the inverse Laplace transform and using the convolution theorem and Eqs. (A1) and (A2) from Appendix, we can present the velocity field under the form

$$\begin{aligned} \displaystyle \displaystyle u\left( y,t\right) =u_{m}\left( y,t\right) +u_{T}\left( y,t\right) + u_{C}\left( y,t\right) , \end{aligned}$$

(13)

where

$$\begin{aligned} \displaystyle u_{m}\left( y,t\right)= & {} \frac{y}{2\sqrt{\pi }}\int \limits _{0}^{t}\frac{ g\left( t-s\right) }{s\sqrt{s}}\exp \left( \frac{-y^{2}}{4s}-Hs\right) ds \nonumber \\&+\,lM\int \limits _{0}^{t}g\left( t-s\right) e^{-Hs}\,\mathrm{erf}\left( \frac{y}{2 \sqrt{s}}\right) ds, \end{aligned}$$

(14)

$$\begin{aligned} \displaystyle u_{T}\left( y,t\right)&=\frac{1}{1-{\Pr }_{eff}}\left[ \frac{1}{E}\left[ \Psi \left( y\sqrt{{\Pr }_{eff}},t,0,0\right) -\Psi \left( y,t,H,0\right) \right] \right. \nonumber \\&\quad +\frac{a}{b-E}\left[ \Psi \left( y\sqrt{{\Pr }_{eff}},t,0,-b\right) -\Psi \left( y,t,H,-b\right) \right] \nonumber \\&\quad \left. +\frac{\left( 1-a\right) E-b}{E\left( b-E\right) }\left[ \Psi \left( y\sqrt{{\Pr }_{eff}},t,0,-E\right) -\Psi \left( y,t,H,-E\right) \right] \right] \cos \gamma , \end{aligned}$$

(15)

$$\begin{aligned} \displaystyle u_{C}\left( y,t\right)&=\frac{N}{H-ScR}\left[ \Psi \left( y\sqrt{Sc} ,t,R,0\right) -\Psi \left( y,t,H,0\right) \right. \nonumber \\&\quad \left. -\,\Psi \left( y\sqrt{Sc},t,R,-F\right) +\Psi \left( y,t,H,-F\right) \right] \cos \gamma , \end{aligned}$$

(16)

are its mechanical, thermal and concentration components and the function $\displaystyle \Psi \left( y,t,a,b\right)$ is defined in Appendix.

It is not difficult to show that $\displaystyle u\left( y,t\right)$, given by Eqs. (3.6)–(3.9), satisfies the imposed initial and boundary conditions. In order to verify the boundary condition (2.12)$_{1}$, for instance, we rewrite $u_{m}\left( y,t\right)$ in the equivalent form

$$\begin{aligned} \displaystyle u_{m}\left( y,t\right)= & {} \frac{2}{\sqrt{\pi }}\int \limits _{\frac{y}{2\sqrt{ t}}}^{\infty }g\left( t-\frac{y^{2}}{4s^{2}}\right) \exp \left( -s^{2}-\frac{ Hy^{2}}{4s^{2}}\right) ds \nonumber \\&\displaystyle \,+\,lM\int \limits _{0}^{t}g\left( t-s\right) e^{-Hs}\,\mathrm{erf}\left( \frac{y}{2 \sqrt{s}}\right) ds, \end{aligned}$$

(17)

As regards the limit of velocity as $y\rightarrow \infty$, it results that

$$\begin{aligned} \displaystyle \lim _{y\rightarrow \infty }u_{m}\left( y,t\right) =\left\{ \begin{array}{c} \displaystyle 0 \qquad \quad if \quad l=0 \\ \displaystyle M\int \limits _{0}^{t}g\left( t-s\right) e^{-Hs}ds \qquad if \quad l=1 \end{array} \right. \end{aligned}$$

(18)

Consequently, in the case when the MFFRP, the fluid does not remain at rest far away from the plate.

From physical point of view, it is also important to determine the skin friction or shear on the plate. Introducing Eq. (3.5)

$$\begin{aligned} \displaystyle \tau =\left. -\frac{\partial u\left( y,t\right) }{\partial y}\right| _{y=0}=-L^{-1}\left\{ \left. \frac{\partial \bar{u}\left( y,q\right) }{ \partial y}\right| _{y=0}\right\} , \end{aligned}$$

(19)

we find that (see also Eqs. (A3)–(A5) from Appendix)

$$\begin{aligned} \displaystyle \tau =\tau _{m}+\tau _{T}+\tau _{C}, \end{aligned}$$

(20)

where

$$\begin{aligned} \displaystyle \tau _{m}= & {} \int _{0}^{t}g^{\prime }\left( t-s\right) \left[ \sqrt{H}\,\mathrm{ erf}\left( \sqrt{Hs}\right) +\frac{e^{-Hs}}{\sqrt{\pi s}}\right] ds \nonumber \\&\displaystyle -\,l\frac{M}{\sqrt{H}}\int _{0}^{t}g^{\prime }\left( t-s\right) \mathrm{erf} \left( \sqrt{Hs}\right) ds, \end{aligned}$$

(21)

$$\begin{aligned} \displaystyle \tau _{T}&=\frac{1}{1-\Pr _{eff}}\left\{ \left[ \frac{1}{E\sqrt{\pi t}}+ \frac{a}{b-E}\phi \left( t;0,b\right) +\frac{\left( 1-a\right) E-b}{E\left( b-E\right) }\phi \left( t;0,E\right) \right] \right. \nonumber \\&\quad \displaystyle \left. -\frac{1}{E}\phi \left( t;H,0\right) -\frac{a}{b-E}\phi \left( t;H,b\right) -\frac{\left( 1-a\right) E-b}{E\left( b-E\right) }\phi \left( t;H,E\right) \right\} \cos \gamma , \end{aligned}$$

(22)

$$\begin{aligned} \displaystyle \tau _{C}&=\frac{N}{ScR-H}\left\{ \phi \left( t;H,F\right) -\phi \left( t;H,0\right) \right. \nonumber \\&\quad \left. +\,\sqrt{Sc}\left[ \phi \left( t;R,0\right) -\phi \left( t;R,F\right) \right] \right\} \cos \gamma , \end{aligned}$$

(23)

are the mechanical, thermal and concentration components of the skin friction and the function $\phi \left( t;a,b\right)$ is defined in the Appendix.

By taking $K=0$ and $\gamma =0$ into Eqs. (3.6) and (3.13), we recover the corresponding results of [[19], Eqs. (20) and (27)].

As the concentration and thermal parts of velocity and skin friction are independent of $g\left( t\right)$, so in the following section we will discuss the special cases regarding to the mechanical part of velocity and skin friction.

4 Special Cases

In the following, in order to get some physical insight of present results and for validation of the obtained results with possible engineering applications, we consider the following cases.

4.1 Case $g\left( t\right) =H\left( t\right)$ (Uniform Motion of the Plate)

let us take $K=0$, $\gamma =0$, $g\left( t\right) =H\left( t\right)$ (the Heaviside unit step function) in our relations (3.7) and (3.14) and use Eqs. (A6) and (A7) from Appendix, we get

$$\begin{aligned} \displaystyle u_{m}\left( y,t\right) =\left( 1-l\right) \Psi \left( y,t;0,M\right) +l\left[ 1-\exp \left( -Mt\right) \mathrm{erf}\left( \frac{y}{2\sqrt{t}}\right) \right] , \end{aligned}$$

(24)

and

$$\begin{aligned} \displaystyle \tau _{m}\left( t\right) =\left[ \sqrt{H}\,\mathrm{erf}\left( Ht\right) +\frac{ e^{-Ht}}{\sqrt{\pi t}}\right] H\left( t\right) -l\frac{M}{\sqrt{H}}H\left( t\right) \mathrm{erf}\left( \sqrt{Ht}\right) . \end{aligned}$$

(25)

As it was to be expected, the corresponding results are identical to those obtained by Tokis [[17], Eqs. (12) and (13a)] and Narahari and Debnath [[18], Eqs. (11a), (13)] with $a_{0}=0$ and in the absence of thermal and concentration effects and $\gamma =0$.

4.2 Case $\displaystyle g\left( t\right) =H\left( t\right) t^{\alpha }$ (Variably Accelerating Plate)

Thermal and concentration components of velocity do not depend on the plate motion. However, the heat and mass transfer can influence the fluid motion and we have to know if their influence is significant or it can be neglected in some motions with possible engineering applications. Taking, $\displaystyle g\left( t\right) =H\left( t\right) t^{\alpha }$ with $\alpha >0$, the Eqs. (3.7) and (3.14) take the forms

$$\begin{aligned} \displaystyle u_{m}\left( y,t\right)&=\frac{y}{2\sqrt{\pi }}\int _{0}^{t}\frac{\left( t-s\right) ^{\alpha }}{s\sqrt{s}}\exp \left( -\frac{y^{2}}{4s}-Hs\right) ds \nonumber \\&\quad \displaystyle +\,lM\int _{0}^{t}\left( t-s\right) ^{\alpha }e^{-Hs}\,\mathrm{erf}\left( \frac{y }{2\sqrt{s}}\right) ds, \end{aligned}$$

(26)

$$\begin{aligned}&\displaystyle \left. \tau _{m}\left( t\right) =\alpha \int _{0}^{t}\left( t-s\right) ^{\alpha -1}\left[ \sqrt{H}\,\mathrm{erf}\left( \sqrt{H}s\right) +\frac{e^{-Hs}}{ \sqrt{\pi s}}\right] ds\right. \nonumber \\&\qquad \qquad \displaystyle \left. -\,l\alpha \frac{M}{\sqrt{H}}\int _{0}^{t}\left( t-s\right) ^{\alpha -1}\mathrm{erf}\left( \sqrt{H}s\right) ds;\text { }\alpha >0,\right. \end{aligned}$$

(27)

which corresponds to motions induced by a slowly, constantly or highly accelerating plate.

4.3 Case $\displaystyle g\left( t\right) =H\left( t\right) \cos \left( \omega t\right)$ or $H\left( t\right) \sin \left( \omega t\right)$ (Oscillating Plate)

Introducing into Eqs. (3.7) and (3.14) and using the fact that $\displaystyle H^{\prime }\left( t\right) =\delta \left( t\right)$ and

$$\begin{aligned} \displaystyle \int _{0}^{t}\delta \left( t-s\right) g\left( s\right) ds=\int _{0}^{t}\delta \left( s\right) g\left( t-s\right) ds=g\left( t\right) , \end{aligned}$$

(28)

where $\displaystyle \delta \left( .\right)$ is the Dirac delta function, we find that

$$\begin{aligned} \displaystyle u_{cm}\left( y,t\right)= & {} \frac{y}{2\sqrt{\pi }}\int _{0}^{t}\frac{\cos \left[ \omega \left( t-s\right) \right] }{s\sqrt{s}}\exp \left( -\frac{y^{2} }{4s}-Hs\right) ds \nonumber \\&\displaystyle +\,lM\int _{0}^{t}\cos \left[ \omega \left( t-s\right) \right] e^{-Hs}\mathrm{ erf}\left( \frac{y}{2\sqrt{s}}\right) ds, \end{aligned}$$

(29)

$$\begin{aligned} \displaystyle u_{sm}\left( y,t\right)= & {} \frac{y}{2\sqrt{\pi }}\int _{0}^{t}\frac{\sin \left[ \omega \left( t-s\right) \right] }{s\sqrt{s}}\exp \left( -\frac{y^{2} }{4s}-Hs\right) ds \nonumber \\&\displaystyle +\,lM\int _{0}^{t}\sin \left[ \omega \left( t-s\right) \right] e^{-Hs}\mathrm{ erf}\left( \frac{y}{2\sqrt{s}}\right) ds, \end{aligned}$$

(30)

$$\begin{aligned} \displaystyle \tau _{cm}= & {} H\left( t\right) \left\{ \sqrt{H}\,\mathrm{erf}\left( \sqrt{Ht} \right) +\,\frac{e^{-Ht}}{\sqrt{\pi t}}-l\frac{M}{\sqrt{H}}\,\mathrm{erf}\left( \sqrt{Ht}\right) \right\} \nonumber \\&\displaystyle -\,\omega \int _{0}^{t}\sin \left[ \omega \left( t-s\right) \right] \left[ \sqrt{H}\,\mathrm{erf}\left( \sqrt{Hs}\right) +\frac{e^{-Hs}}{\sqrt{\pi s}} \right] ds \nonumber \\&\displaystyle +\,l\omega \frac{M}{\sqrt{H}}\int _{0}^{t}\sin \left[ \omega \left( t-s\right) \right] \mathrm{erf}\left( \sqrt{Hs}\right) ds, \end{aligned}$$

(31)

$$\begin{aligned} \displaystyle \tau _{sm}= & {} \omega \int _{0}^{t}\cos \left[ \omega \left( t-s\right) \right] \left[ \sqrt{H}\,\mathrm{erf}\left( \sqrt{Hs}\right) +\frac{e^{-Hs}}{\sqrt{\pi s} }\right] ds \nonumber \\&\displaystyle -\,l\omega \frac{M}{\sqrt{H}}\int _{0}^{t}\cos \left[ \omega \left( t-s\right) \right] \mathrm{erf}\left( \sqrt{Hs}\right) ds. \end{aligned}$$

(32)

As expected, for $\omega =0,$ the solutions (4.3.2) and (4.3.4) reduce to those given by Eqs. (4.1.1) and (4.1.2) corresponding to the motion with uniform velocity on the boundary.

Indeed, assigning to $g\left( .\right)$ suitable forms, we can determine exact solutions for any motion with technical relevance of this type. Consequently, the problem under debate is completely solved.

5 Numerical Results and Discussion

In this paper, exact general solutions are determined for dimensionless velocity and skin friction corresponding to the MHD natural convection flow over a moving inclined plate with exponential heating, constant concentration and chemical reaction. Radiative and porous effects are taken into consideration and the magnetic field is fixed to the fluid or to the plate. In order to get some physical insight of obtained results and to avoid repetition, three special cases are considered. Figures 1 and 2 present the profiles of the dimensionless velocity $\displaystyle u\left( y,t\right) ,$ respectively mechanical component of velocity $\displaystyle u_{m}\left( y,t\right)$ against y at different times for a slowly accelerating motion of the plate. As expected, both velocities are increasing functions of time. Furthermore, the velocities corresponding to (MFFRP) are appreciably large as compared with (MFFRF). In all cases, the velocities smoothly decrease from maximum values on the boundary to asymptotical values for increasing y. However, as it is clearly seen from these figures, the asymptotic values of both velocities are not zero at infinity if the magnetic field is fixed to the plate.

Fig. 1.

(a) Profiles of $u_{m}\left( y,t\right)$ against y for $\gamma =\frac{\pi }{6}$ and different values of t, (b) 3D plot of $u_{m}\left( y,t\right)$.

Fig. 2.

Profiles of $u\left( y,t\right)$ against y for $\gamma =\frac{\pi }{6}$ and different values of t.

Fig. 3.

Profiles of $u_{\alpha m}\left( y,t\right) ,$ $u_{\alpha m}\left( y,t\right) +u_{T}\left( y,t\right)$ and $u_{\alpha m}\left( y,t\right) +u_{T}\left( y,t\right) +u_{C}\left( y,t\right)$ against y at $\gamma = \frac{\pi }{6},$ $t=1.5.$ and $l=0$ when $g(t)=H(t).t^{\alpha }$.

In Fig. 3 we have plotted velocities $\displaystyle u_{m}\left( y,t\right)$, $u_{m}\left( y,t\right) +u_{C}\left( y,t\right)$ and $u_{m}\left( y,t\right) +u_{C}\left( y,t\right) +u_{T}\left( y,t\right)$ versus y to investigate the contributions of mechanical, thermal and concentration components of velocity on the fluid motion. It is observed that contributions of mechanical, thermal and concentration components of velocity on the fluid motion are significant and they cannot be neglected. In all diagrams $a=0.75,$ $b=0.15,$ $\alpha =0.5,$ $\Pr =0.7,$ $M=0.5,$ $\Pr _{eff}=0.5,$ $Sc=0.6,$ $R=0.7,$ $N=0.5,$ $K=0.3.$

6 Conclusions

Hydromagnetic natural convection flow of an electrically conducting, incompressible viscous fluid over a moving infinite inclined plate with exponentially heating, constant concentration and chemical reaction is analytically and graphically studied. Viscous dissipation and Joule heating are neglected but the porous and radiative effects are taken into consideration. The plate is moving with arbitrary time-dependent velocity in its plane while the transverse magnetic field is fixed to the fluid or to the plate and our interest is focused on the fluid motion. Consequently, exact general expressions for the dimensionless velocity and the corresponding skin friction are established in simple forms in terms of error and complementary error functions of Gauss and the problem under consideration is completely solved. Both the velocity and skin friction are presented as sum of their mechanical, thermal and concentration components.

However, in order to obtain some physical insight of results that have been obtained as well as to avoid repetition, three special cases are considered. Finally, the contributions of mechanical, thermal and concentration components of velocity and skin friction on the fluid motion are brought to light for a slowly accelerating motion of the plate. The main conclusions are:

• Contrary to our expectations, the fluid velocity does not remain zero at infinity if the magnetic lines of force are fixed relative to the plate.

• The dimensionless velocity of the fluid significantly increases in the case (MFFRP) in comparison to the case (MFFRF).

• Contributions of mechanical, thermal and concentration components of velocity and skin friction on the fluid motion are significant and they cannot be neglected.

Appendix

$$\begin{aligned}&\displaystyle \left. L^{-1}\left\{ e^{-y\sqrt{q}}\right\} =\frac{y}{2t\sqrt{\pi t}}\exp \left( \frac{-y^{2}}{4t}\right) ,\text { }L^{-1}\left\{ \frac{e^{-y\sqrt{q}}}{ q}\right\} =\mathrm{erf}\,c\left\{ \frac{y}{2\sqrt{t}}\right\} ,\right. \nonumber \\&\displaystyle \left. L^{-1}\left\{ \frac{e^{-y\sqrt{q+a}}}{q-b}\right\} =\Psi \left( y,t;a,b\right) ,\right. \end{aligned}$$

(33)

$$\begin{aligned} \displaystyle \Psi \left( y,t;a,b\right) =\frac{e^{bt}}{2}\left[ \begin{array}{c} e^{-y\sqrt{a+b}}\mathrm{erf}\,c\left( \frac{y}{2\sqrt{t}}-\sqrt{\left( a+b\right) t}\right) \\ +e^{y\sqrt{a+b}}\mathrm{erf}\,c\left( \frac{y}{2\sqrt{t}}+\sqrt{\left( a+b\right) t}\right) \end{array} \right] . \end{aligned}$$

(34)

$$\begin{aligned} \displaystyle L^{-1}\left\{ qF\left( q\right) \right\} =f^{\prime }\left( t\right) +\delta \left( t\right) f\left( 0\right) \text { }if\text { }L^{-1}\left\{ F\left( q\right) \right\} =f\left( t\right) \left( \delta \left( .\right) \text { is the Dirac delta function}\right) . \end{aligned}$$

(35)

$$\begin{aligned} \displaystyle L^{-1}\left\{ \frac{1}{\left( q+b\right) \sqrt{q+a}}\right\} =\frac{e^{-bt}}{ \sqrt{a-b}}\,\mathrm{erf}\left( \sqrt{\left( a-b\right) t}\right) ,L^{-1}\left\{ \frac{1}{\sqrt{q}}\right\} =\frac{1}{\sqrt{\pi t}}. \end{aligned}$$

(36)

$$\begin{aligned} \displaystyle L^{-1}\left\{ \frac{\sqrt{q+a}}{q+b}\right\} =\frac{e^{-at}}{\sqrt{\pi t}}+ \frac{1}{\sqrt{a-b}}e^{-bt}\mathrm{erf}\left( \sqrt{a-b}t\right) =\phi \left( t;a,b\right) , \end{aligned}$$

(37)

$$\begin{aligned} \displaystyle \int _{0}^{t}\frac{1}{\sqrt{s}}\exp \left( -\frac{y^{2}}{4s}-as\right) ds= & {} \frac{\sqrt{\pi }}{2\sqrt{a}}\left\{ e^{-y\sqrt{a}}\mathrm{erf}\,c\left( \frac{y }{2\sqrt{t}}-\sqrt{at}\right) \right. \nonumber \\&\displaystyle \left. -e^{y\sqrt{a}}\mathrm{erf}\,c\left( \frac{y}{2\sqrt{t}}+\sqrt{at} \right) \right\} , \end{aligned}$$

(38)

$$\begin{aligned} \displaystyle \int _{0}^{t}\frac{1}{s\sqrt{s}}\exp \left( -\frac{y^{2}}{4s}-as\right) ds= & {} \frac{\sqrt{\pi }}{y}\left\{ e^{-y\sqrt{a}}\mathrm{erf}\,c\left( \frac{y}{2\sqrt{ t}}-\sqrt{at}\right) \right. \nonumber \\&\displaystyle \left. -e^{y\sqrt{a}}\mathrm{erf}\,c\left( \frac{y}{2\sqrt{t}}+\sqrt{at} \right) \right\} , \end{aligned}$$

(39)

$$\begin{aligned} \displaystyle \int _{0}^{\infty }e^{-p^{2}s^{2}-\frac{q^{2}}{s^{2}}}\cos \left( a^{2}s^{2}+ \frac{b^{2}}{s^{2}}\right) ds= & {} \frac{\sqrt{\pi }}{2^{4}\sqrt{p^{4}+a^{4}}} e^{-2c\cos \left( \alpha +\beta \right) } \nonumber \\&\displaystyle \cos \left[ \alpha +2c\sin \left( \alpha +\beta \right) \right] , \end{aligned}$$

(40)

$$\begin{aligned} \displaystyle \int _{0}^{\infty }e^{-p^{2}s^{2}-\frac{q^{2}}{s^{2}}}\sin \left( a^{2}s^{2}+ \frac{b^{2}}{s^{2}}\right) ds= & {} \frac{\sqrt{\pi }}{2^{4}\sqrt{p^{4}+a^{4}}} e^{-2c\cos \left( \alpha +\beta \right) } \nonumber \\&\displaystyle \sin \left[ \alpha +2c\sin \left( \alpha +\beta \right) \right] , \end{aligned}$$

(41)

where $\displaystyle \alpha =\frac{1}{2}arctg\left( \frac{a^{2}}{p^{2}}\right) ,$ $\displaystyle \beta = \frac{1}{2}arctg\left( \frac{b^{2}}{q^{2}}\right)$ and $\displaystyle c=\root 4 \of {\left( p^{4}+a^{4}\right) \left( q^{4}+b^{4}\right) }$.