Keywords

1 Introduction

Innovative and increasingly powerful technological tools are introducing new kinds of problem-solving situations where mathematics is useful, thus changing the mathematical abilities needed outside school. So 21st century youths need to have access to and develop the skills to use these tools for mathematical learning and, particularly, in problem solving activities (Forgasz, Vale, & Ursini, 2010). While little is still known about the problem solving that occurs beyond school (English & Sriraman, 2010), further research is recommended to understand the role of digital tools in such activity (Santos-Trigo & Barrera-Mora, 2007).

This study brings new knowledge about the spontaneous use of digital tools in solving non-routine mathematical problems by youngsters engaged in an online problem solving competition. The context in which the research was conducted is that of the mathematical problem solving competition SUB14®, which is aimed at middle graders (12–14 years-old) of the southern regions of Portugal. The Qualifying stage of the competition consists of answering a problem every two weeks, either through e-mail or an online text editor available on the competition website. Participants may solve the problems using their preferred methods and tools but are explicitly required to report on their solving process and must offer a complete explanation of their reasoning. The inclusive character of this competition makes it accessible to average-ability students and its rules permit and encourage help seeking from relevant others at this stage. This context offers the opportunity to study how youngsters are using their mathematical knowledge in problem solving beyond the classroom, where they are also allowed to choose any technological tool at their disposal to solve the problems and express their solutions.

Our research aim is to understand the ways in which the processes of mathematical problem solving are reshaped when these youngsters spontaneously resort to digital technologies. In addressing this purpose, we intend to develop our understanding of how the use of digital technologies, including everyday and general purpose tools, is embedded in the process of solving and expressing a solution to a non-routine mathematical problem. Here, we will limit ourselves to one of the cases selected in the course of the beyond-school competition SUB14 that served as the basis for the construction and application of an analytical model of mathematical problem solving with technology. We assume that the case of Marco, when solving a geometry problem, offers a valuable report on this model and on its strength in providing new insights into young students’ use of digital tools in mathematical problem solving.

2 Theoretical Background

The prevailing theoretical models on solving mathematical problems, which conceive paper and pencil as the predominant tools, do not account for the role of digital technology. Thus, they do not provide the tools to explain the interaction between individuals’ technological and mathematical knowledge in their problem solving activity (Santos-Trigo & Camacho-Machín, 2013).

Our theoretical framework, aiming to address mathematical problem solving with technology, is built upon the notion of humans-with-media, acknowledging the inseparability between the solver and the technological tool whilst solving the problems and expressing their solutions. The youngsters’ interaction with digital media is seen from the point of view of placing affordances in the tools. Furthermore, we address mathematical problem solving with technology by combining two analytical tools: one accounting for the processes involved in mathematical problem solving, and the other for the processes taking place with the use of digital tools in digitally-framed tasks (Jacinto & Carreira, 2017; Jacinto, Carreira, & Mariotti, 2016).

2.1 Solving-and-Expressing: An Overall Concept

In addressing the students’ ways of tackling mathematical problems with digital tools, we consider several theoretical notions and perspectives that offer a theoretical frame for such activity. Problem solving is here understood as the development of a productive way of thinking about a challenging situation (Lesh & Zawojewski, 2007) where the solver must adopt a mathematical point of view in order to carry out mathematization processes. Moreover, it is regarded as a synchronous process of mathematization and expression of mathematical thinking (Carreira, Jones, Amado, Jacinto, & Nobre, 2016), which means that obtaining a solution to a problem is to find the required answer and to create an explanation for it. Hence the solution phase and the reporting stage are closely linked aspects of this problem solving-and-expressing activity. This means that all the material incorporated in the final product, and not merely the result, is actually part of the solution process (Lesh & Doerr, 2003), such as the use of color, diagrams, tables, images, along with textual explanations or descriptions. These descriptive elements carry new knowledge about the situation, which is fundamental in solving-and-expressing each problem.

In this study, we also adopt the notion of humans-with-media (Borba & Villarreal, 2005) as a core conceptual unit that postulates the inseparability between the subject and the tool, thus leading to interlace mathematical thinking and expressing with the representational power of digital technologies. In fact, the introduction of a specific tool in the system of humans-with-media impels relevant changes in the activity, according to the type of media that it encloses, thereby resulting that different collectives originate different ways of thinking and knowing (Jacinto & Carreira, 2013, 2017; Villarreal & Borba, 2010).

The interactions taking place within this conceptual unit, i.e., between the individual and the digital media whilst solving-and-expressing mathematical problems, is seen from the point of view of placing affordances in the tools (Chemero, 2003) in the sense that affordances are both relative to the object and to the subject who realizes its advantages. The affordances emerge from the interaction between the agent and the object, insofar as the perception of the possibility for action and the ability of the agent are not “specifiable in the absence of specifying the other” (Greeno, 1994, p. 338). Hence, the recognition of particular features in the tools that are potentially useful support the individual in solving-and-expressing the problem, thus leading us to consider the impossibility of separating the solver’s mathematical and technological skills (Jacinto et al., 2016).

2.2 Developing a Model of Mathematical Problem Solving with Technology

The development of a new conceptual framework that aims to account for both components of the problem solving process encompasses the redesign and expansion of well-known theoretical models in order to suggest more efficient ways to describe the connection between mathematical knowledge and the affordances of digital tools that solvers bring to their problem solving-and-expressing activity. This lead us in bringing together two frameworks: one addressing the activity of an individual while dealing with a technological task or problem (Martin & Grudziecki, 2006), and another one particularly focused on describing the processes involved in mathematical problem solving (Schoenfeld, 1985).

The DigEuLit Project (Martin, 2006) proposed a framework on Digital Literacy in which a set of processes performed in the context of solving a task or problem that requires the use of a digital resource were stated. These processes comprise: statement—clearly state the problem and the actions likely to be required; identification—identify the digital resources required to achieve the solution; accession—locate and obtain those digital resources; evaluation—assess the accuracy and reliability, and relevance of the digital resources; interpretation—understand the meaning they convey; organization—organize them in ways that may enable the solution; integration—bring these resources together in relevant combinations; analysis—examine them using concepts and models that will enable the solution; synthesis—recombine them in new ways to achieve the solution; creation—create new knowledge objects, units of information or digital outputs that contribute to achieve the solution; communication—interact with others while solving the problem; dissemination—present the solution to others; reflection—consider the success of the task performed (Martin & Grudziecki, 2006, p. 257).

Although this set of processes resembles well-known models in mathematics education, it is necessary to account for the mathematical thinking developed in this activity. Being successful in mathematical problem solving, as Schoenfeld (1985) suggests, requires considering essential facts and procedures, effective use of resources, strategies, and actively engaging in mathematical thinking.

Aiming to describe students’ mathematical problem solving performance, Schoenfeld (1985, pp. 297–298) proposed a model that comprises five stages: read—time spent “ingesting the problems conditions”; analysis—attempt to fully understand the problem “sticking rather closely to the conditions or goals” that may include a selection of ways of approaching the solution; exploration—a “search for relevant information” that moves away from the context of the problem; planning and implementation—defining a sequence of actions and carrying them out orderly; verification—the solver reviews and assesses the solution.

By comparing and relating the processes proposed by Martin and Grudziecki and the stages identified by Schoenfeld, and by selecting the most prominent actions in the two frameworks, we reached the following model by merging some of the processes of digital problem solving and also decomposing some of the stages of mathematical problem solving (see Table 3.1). Even though these processes are clearly defined and have distinct boundaries, as acknowledged by the original models, in this combined model they are flexible enough to be considered in different phases.

Table 3.1 Processes underlying mathematical problem solving with technology
Full size table

3 Research Method

As stated above, the overall goal of our research is to understand the processes of mathematical problem solving by acknowledging the role of digital tools, considering the problem solving activity carried out by young students within the context of the competition SUB14.

Following an interpretative stance that involved qualitative techniques for data collection and analysis (Merriam, 2009), we developed several cases of participants who usually resort to a variety of technological tools to solve the problems of the competition and who present detailed explanations of their solutions (Jacinto, 2017). In this chapter, we confine ourselves to the case of one participant, under the pseudonym of Marco, who has a preference for geometrical problems in which he is able to use his digital skills in implementing visual methods (Jacinto & Carreira, 2015) and resorts to conventional and unconventional tools in developing his approaches to those geometry problems posed by SUB14 (Jacinto et al., 2016). The case serves the purpose of illustrating and substantiating some main results from the broader work that has spanned over several years of data analysis.

The collection of data initially consisted of gathering all the digital solutions produced by Marco along two yearly editions of the competition. This chapter deals initially with the analysis of Marco’s solution to the problem “United and Cropped” (see Sect. 3.4.1), which he developed using GeoGebra. The GeoGebra file allows disclosing the sequence in which the constructions were performed by means of its Construction Protocol.

We proceeded to a second stage of our research by observing and video recording Marco’s work while solving a problem in his home environment, with the consent of his parents. He was asked to choose one out of three problems posted for this purpose on the SUB14 website, then solve it by performing as closely as possible to his usual problem solving activity in the competition, and to explain out loud his actions and thinking. Marco chose to solve the problem “Decorative Drawing” (see Sect. 3.4.2) and resorted to several technological tools during the process.

The NVivo software was used in the organization process, for transcribing the interviews, segmenting and coding data. As for the data analysis we followed an interpretative perspective considering that providing a holistic description of the case would encompass the results in light of the proposed MPST model and the theoretical notions discussed. The following section illustrates the case of Marco-with-media solving-and-expressing problems within the competition SUB14.

4 Data Analysis and Results

Marco is a 13 year-old student enrolled in SUB14 for the second year, who is quite familiar with a diversity of digital tools. While studying geometric transformations at school, he learned to use GeoGebra. Marco enjoyed these lessons so much that, at home, he continued to explore GeoGebra on his own. However, he often uses a spreadsheet or editing tools in solving-and-expressing the problems of the competition. Below, we firstly analyze Marco’s processes while solving a mathematical problem with GeoGebra based on a solution submitted during the qualifying stage of SUB14. We then report on the processes he engages in while solving another problem, based on the in-depth interview and observation.

4.1 Marco’s Processes in Solving a Mathematical Problem with Technology Based on the Digital Solution

Replicating the Complete Sequence of Squares

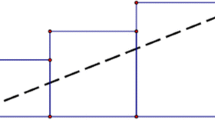
The problem ‘United and Cropped’ is one of the problems that Marco solved when participating in SUB14 and in which he resorted to GeoGebra (Fig. 3.1). The problem refers to a sequence of squares and presents a figure where only a few elements of the sequence are shown. It has to do with finding a way of extending the sequence and find a specific requested area.

Fig. 3.1
figure 1

Statement of the problem ‘United and Cropped’ from the SUB14 competition

Full size image

Marco submitted a file containing his solution to the problem. He decided to use GeoGebra to obtain a figure like to the one presented in the problem (grasp) possibly realizing that he could obtain the sequence of 8 squares by marking their vertices, and later constructing their sides and, from there, find a way to obtain the requested area (notice). He seems to have recognized the advantages of combining two affordances of the GeoGebra graphical view—the axes and the grid. Those provided and supported a visual and orderly way for the construction of the sequence of squares, based on the pattern of increment of the sides (interpret).

Marco then plotted each vertex on the rectangular grid, considering its coordinates according to the dimensions of the sides of each square (Fig. 3.2). Some of the coordinates that are visible in the Construction Protocol (for example, E and F) (Fig. 3.3) suggest that Marco was just using the visual location of the point, based on the grid, to insert each point in an approximate position. Apparently he was convinced that he just needed a sketch of the figure rather than its exact geometrical construction in leading him to a path for the solution.

Fig. 3.2
figure 2

Construction of the sequence of eight squares with GeoGebra

Full size image
Fig. 3.3
figure 3

GeoGebra’s spreadsheet view and excerpt of the Construction Protocol

Full size image

His next step is the construction of the sides of the squares, where he uses segments drawing. Next, he constructs a ray from the origin of the axis to the upper vertex of the sequence and, using the ‘properties of objects’, he changes the color of that ray to orange. While developing the construction of this element Marco is already combining technological and mathematical resources, which sets the beginning of an exploratory approach to the problem (integrate).

The Mathematization: Solving-and-Expressing the Solution

The conceptual model that is apparently starting to be developed will guide Marco to the solution. He realizes the relevance of the GeoGebra spreadsheet (Fig. 3.3) as he chooses to use this tool to deal with the measurements involved in the figure. He creates a sequential list of the lengths of the sides and inserts them in column A, and another list containing the area of each corresponding square, which he organizes in column B (explore). Then he inserts the label “area of 8 Q” (abbreviation of 8 Squares) in cell A14, and turns to the figure to construct the upper side of the surrounding rectangle that contains the sequence of squares. He directly enters the total area of the 8 squares in the table and also the area of the surrounding rectangle. Although such rectangle is not mentioned in the problem, its construction reveals how Marco is developing his approach to the solution (plan) which is based on the realization that he can get the requested area by means of the difference between the area of the 8 squares and the area of a triangle (shown below the cut line), as the cut line is a diagonal of the rectangle. Thus the rectangle is a new object of knowledge and a key element in the mathematization of the situation that Marco uses to solve and express his solution, both drawing on his knowledge about GeoGebra and his knowledge about areas of polygons (create).

He proceeds by inserting the label “area above the line” in cell A16 and “area of half-rectangle” in cell A17. He then calculates the area of the half-rectangle and inserts it directly in cell B17. Below, he uses other cells to compute the difference between the area of the rectangle and the area of the 8 squares; he then subtracts this result to the area of the half-rectangle (verify). Only then he enters in cell B16 the value 60, which was the answer to the problem.

The file he submitted with the solution to the problem contains the construction of the sequence of squares and presents several calculations that are intended to explain and justify his answer, using the GeoGebra spreadsheet view (disseminate).

Although in the digital solution there is no evidence that Marco has sought other sources of information or help during the solving-and-expressing process, he mentioned in his electronic message that he counted on the help of family members during his activity (communicate). However, with the data available it is not possible to specify either the type of help that was provided or the stage at which such aid was relevant to the problem solving-and-expressing process.

The analysis of the Construction Protocol that supports this resolution shows that despite not having made a geometrically rigorous construction, Marco found the solution to the problem and presented it clearly. In addition, he identified a diversity of possibilities of action with GeoGebra although he has freely chosen to just make use of the indispensable tools to develop a feasible approach to the problem. This intentional choice of GeoGebra is based on an explicit knowledge of its affordances, its characteristic mode of multiple views, and embedded tools, but also on the students’ own aptitude, i.e. on the things he knows, and can actually do with GeoGebra to solve the problem and express the solution.

The effective use of the tool appears to be related to the fact that the construction of the sequence of squares infused a visual approach that enabled to bring out an underlying conceptual model of the problem, which sustained the process of obtaining and presenting the solution. We could also say that the student mainly drew on the GeoGebra’s affordances to create an acceptable sketch of the figure needed to represent the givens and goals of the problem. That sketch was then combined with new elements he inserted in the figure and with the visualization of the required area as a difference between specific areas that could be computed by means of the knowledge on polygons. In fact, there were other options that Marco’s construction would have allowed to follow and explore, namely the GeoGebra capacity of constructing general and particular polygons and measuring their areas. This would enable, for example, making use of the points given by the intersection of the ray with each side of the squares (which he actually created in his construction). They would permit to obtain directly in GeoGebra the areas of the pieces of the squares above the cut line. Therefore, what seems to be more significant is that Marco develops his visual thinking through the use of the technology and combines it effectively with his knowledge related to finding areas.

Summary of the Processes of Solving-and-Expressing with GeoGebra

The processes developed by Marco in solving-and-expressing this problem are summarized in the diagram presented in Fig. 3.4. For each of the processes considered in the MPST model, the key aspects that characterize them are identified. Those are then recorded in the diagram, although very succinctly. Since this solution was not subject to observation, the synthesis concerns the analysis of the file submitted by Marco, complemented by the analysis of the various stages of his work recorded in the construction protocol. Although Marco mentioned that he had the support of family members, it is not possible to specify when this exchange took place, so the communication process was not included in the diagram.

Fig. 3.4
figure 4

Processes of solving-and-expressing the problem ‘United and Cropped’

Full size image

Another aspect depicted in the diagram above has to do with the flow along the various processes that took place. As it is apparent in the scheme, this flow is relatively straightforward and shows a linear progression from the initial appropriation of the conditions of the problem to the dissemination of its entire solution and attached products. In the following, we want to reconsider this apparent linearity as we will be addressing data obtained from face-to-face activity and observation of the problem solving activity performed by Marco.

4.2 Marco’s Processes in Solving a Mathematical Problem with Technology Based on the Observed Activity

In a face-to-face interview, Marco solved one of three problems that he was asked to choose from, with the request to recall and reproduce what he usually did during the participation in the competition, Marco began by carefully analyzing the three ‘experimental problems’ posted on the webpage of the competition for this purpose. He seemed hesitant but ended up choosing the one that he considered to be his favorite: the problem ‘Decorative drawing’ (Fig. 3.5). When asked about the reasons for his preference, Marco explained:

Marco::

[This one] has more to do with triangles and stuff and it was in the seventh grade that I had 100 [%] in both tests.

Researcher::

In geometry?

Marco::

Yes, I studied congruence of triangles and such…

Fig. 3.5
figure 5

Statement of the problem ‘Decorative Drawing’ chosen by Marco

Full size image

His choice is based on an initial identification of the mathematical topic and the notions that are apparently needed to solve the problem (geometry, triangles, congruence of triangles) and, at the same time, reflects his familiarity with those ideas and even a certain self-confidence to deal with those concepts since he had obtained excellent grades in this subject in the previous school year (grasp). Although Marco interacted with the researcher throughout his activity, following the request to verbalize his thoughts and procedures, at this initial stage he explicitly requested support for clarifying the meaning of the notion of tangency (communicate).

M::

There’s something that I don’t understand. Tangents, the circles are tangent…

R::

Tangent. Don’t you know what tangent means? [Marco nods affirmatively] It means that they just touch in a single point. In this case, they just touch in this point [pointing to the screen].

Working on Attempts to Develop a Solution

Focusing on the reading of the problem and interacting with the figure presented on the competition website, Marco begins to develop a series of attempts that lead him to conjecture about the solution. His first ideas were drawn on the fact that the triangle is equilateral (notice) and made him believe that he would be able to obtain the desired solution if he would focus on the central circle and from there obtaining the radius of the smaller circles (interpret).

M::

I’m trying. I’m still trying … to see … how to do this. Hmm … since the triangle is equilateral … if you get to the middle circle maybe you can get to the others … [1st attempt].

Then, he silently stares at the screen for a while. The understanding of the situation begins to develop in close relation with his careful observation of the image. He rapidly sketches various visual decompositions of the equilateral triangle: sliding his finger across the screen, he ‘draws’ a bisector of the lower right angle of the triangle (Fig. 3.6) but continues to think aloud while ‘drawing’ also the bisector of the top angle (notice/interpret).

M::

How shall I say this? It’s like they are divided in halves. From each vertex to the midpoint of the opposite side, and then I could try to find out… If I could do it … But I’m still seeing how am I going to do it … [2nd attempt]

Fig. 3.6
figure 6

Formulates and tests 2nd attempt

Full size image

His attempts to find a visual method of approaching the problem continue, and after some time he proposes another analysis of the situation:

M::

This measures 12 cm. The middle of the triangle is less than 12, for sure. It could be 4. If we divide in these parts … [with the forefinger and thumb sets a distance and slides it 3 times covering the height of the triangle]. Yeah, maybe. Because they are tangent… [Silence]… I can say they have the same length. [3rd attempt]

In spite of some imprecision in the language he uses, the student recognizes that the centroid of the triangle does not coincide with the midpoint of its height. In fact, he conjectures that the radius of the larger circle could be 4 cm, which is obtained from a visual intuition supported by a rudimentary measurement based on a fixed distance that he defines with the fingers (Fig. 3.7). Although he concludes that the radius of the larger circle corresponds to 1/3 of the height of the equilateral triangle, he realizes that this statement lacks clear justification, but he seems to find no information in the problem for that (notice/ interpret).

Fig. 3.7
figure 7

Formulates and tests 3rd attempt

Full size image

He knows that he has already attempted different approaches, which he feels that might work to solve the problem but is not totally confident with them. The various approaches consisted of manipulations and mental transformations in the sense they have not actually been operationalized by Marco beyond the ‘drawing’ with the index finger on the screen. He finally decides to follow a strategy involving the decomposition of the equilateral triangle in two figures: a smaller triangle at the top and a trapezoid below (Fig. 3.8). He goes on explaining:

M::

If we draw a triangle here… It’s as if this one is an enlargement of that one. If this is 12, then 12 divided by 3, [equals] 4… It means that the radius is 2. Maybe the radius of the small circle is 2. [4th attempt]

Fig. 3.8
figure 8

Formulates and tests 4th attempt

Full size image

Up to this point, Marco was trying to understand the main ideas involved in the problem (notice) and, in each hypothesis raised, he was considering the plausibility of a mathematical way of approaching the solution (interpret). Therefore, in the first minutes of his activity, there are cycles of notice-interpret, which are successively refined, and pave the way for the development of a conceptual model that will lead to the solution. While Marco is thinking aloud and developing a sequence of ideas, he ‘interacts’ with the figure on the screen by pointing, estimating distances, or by hiding areas with his hands. The development of a visual method to approach the solution starts to take shape, in analyzing the possibilities of decomposition of the figure while simulating transformations such as cut, reorganize or change colors. In this way, editing the figure looked as an indispensable action to get the solution.

A Visual Approach to Get the Solution

Marco then decides to pursue with his fourth attempt. Using the software Snipping Tool, he defines a rectangular area on the screen and crops the top of the large triangle given in the statement, thus obtaining a smaller triangle with a single red circle in its center. Using a similar process, he creates another file containing the original triangle, and then inserts the two images on a new window of the MS Paint (integrate). Once in the same window (Fig. 3.9a), Marco tries to overlap the two images but, as they had a solid white background, it was not possible to visually show that one was an enlargement of the other (explore).

Fig. 3.9
figure 9

Four steps in the editing process with MS Paint

Full size image

This difficulty leads Marco into a slightly different approach: he decides to transform the large triangle so that it looks similar to the smaller triangle. He goes on, expanding the work area so that he can accurately draw a line that would make the bottom side of the smaller figure. In fact, since that figure resulted from a section of the original triangle, one of the sides was not visible, so he needed to complete it by drawing one missing segment. So, rather than just a matter of graphics, the need to draw new elements had a mathematical intentionality (Fig. 3.9b). Then he starts editing the original triangle by using the ‘eyedropper tool’ in MS Paint to identify the exact shade of yellow covering the background of the large triangle; he uses it to change the color of the smaller red circles into the background color so that they vanish from the figure (Fig. 3.9c). Again using the ‘eyedropper tool’ he captures the red shade and then paints the large central circle in that red color (Fig. 3.9d).

The editing of the images described above (integrate) is intended to show that the smaller triangle is, clearly, a reduction of the original triangle (same shape but different size). So Marco is developing and exploring a conceptual model to explain the similarity between those two triangles (explore), and this will guide him in producing the solution. As in the loop of processes identify-interpret, it was observed that integration and exploration also occurred in an iterative way, albeit in a short period of time. Marco studies the best way to demonstrate the similarity of the two triangles in close relation to the recognition of affordances of the image editing tools available and uses them to achieve a transformation that conveys the mathematical relationship in a visually convincing way. When asked about the reason to such a careful work on the graphic elements, he replies: “it’s to better show how you could see that one was an enlargement of the other”. Therefore the graphic treatment is of central importance in his approach to the problem. In addition to illustrating his way of thinking, in the most reliable way he finds, the images also become a visual mathematical argument that must convince those who will evaluate his solution.

Creating and Expressing the Solution

Later, Marco saves the file and opens the OpenOffice spreadsheet. Without resorting to a notebook or pencil, Marco continues to move between the competition website, where he has the problem statement, the image editing tools and the spreadsheet where he starts expressing his solution path (plan). He uses the original image and the two figures produced in MS Paint to compose his answer in the spreadsheet window (Fig. 3.10). The figures support his understanding of the problem and show how Marco visualized the similarity between the triangles. By incorporating mathematical ideas, such as similarity and triangle decomposition, Marco achieves a conceptual model of the problem situation (create).

Fig. 3.10
figure 10

Solution sent by Marco (print screen) with a translation of his written explanation

Full size image

As he usually does in the competition, he identifies the number of the problem on the upper left corner of the worksheet, and inserts or pastes the images he has created and explains in detail his resolution process on the right. Although he reports only a few of the attempts he actually made, he explains that he found “a similarity between the central circle and the smaller ones”, hence considering that the small triangle is a reduction of the larger triangle by a ratio of 12:3 although he does not prove that similarity. Thus, assuming that the radius of the larger circle is 1/3 of the height of the original triangle, Marco explains that the smaller circle has a radius that corresponds to 1/3 the height of the smaller triangle, that is, 1/3 of 4. It is, therefore, while producing a written explanation of the resolution process and making an analysis of the images he edited that Marco finds, effectively, the solution to the problem (verify). Contrary to his last hypothesis (“maybe the radius of the small circle is 2”), Marco now concludes that the radius of the small circle is actually 4/3.

When he considers his work to be finished, Marco saves the file. Then, he accesses the competition website to submit his answer using the online form available, where he uploads the file as an attachment; he fills in his personal data and adds the following sentence: “Here is the answer to the experimental problem 2” (disseminate). Marco also points out that ‘nobody’ helped him with the solution, that he enjoyed ‘very much’ the problem, and that he found it ‘easy’.

Initially, the technological tools assumed a hidden role in the problem solving activity, since Marco only interacted with the screen by visually inspecting the figure given in the statement. However, this visual approach is later developed through processes of transformation of the figure with the technological tools that he chose and with which he shows great familiarity with: he knows how to save the image from the website and knows how to edit it in a way that becomes relevant to find the solution to the problem—a new object of knowledge. His success seems to be anchored in his ability to recognize and make efficient use of various affordances of such tools to broaden his mathematical thinking and to develop a conceptual model for the similarity between the two triangles he seeks to compare.

Moreover, this initial activity appears to have a cyclic nature, in which each argument is formulated as Marco attempts, on the one hand, to assign meaning to the mathematics that may be useful or relevant to him (notice) and, on the other hand, to consider mathematical ways to approach the solution (interpret) while interacting with the figure on the screen. This cyclic activity leads Marco to a final conjecture—”the radius of the small circle is 2”—which is his first guess for the solution and will trigger subsequent exploration activity. Marco’s ability in finding the solution to the problem seems to be related to his aptitude in recognizing the affordances of the selected tools, which broadened his thinking process and ultimately influenced the expression of that thinking. As he starts to explore his guess, the elaboration of images in the graphic environment leads Marco to discover the correct similarity ratio. The use of the spreadsheet supports the combination of objects because it allows him an easy organization of visual and textual inscriptions, that is, he can move images freely and can easily format as well as merge cells.

Summary of the Processes of Solving-and-Expressing on the Screen

The processes of solving-and-expressing the problem ‘Decorative drawing’ are summarized and schematically presented in Fig. 3.11. Marco’s activity was entirely performed in the digital environment, moving only between the various programs that he used. In this second diagram, the flow has some salient differences from the previous one. Here, several loops or micro-cycles involving some specific processes are observed. Therefore, the apparent linearity that the first diagram seemed to indicate is now challenged by a result that is much more complex and less straightforward. In fact, the MPST model proves able to reveal and capture the processes carried out and also their linked and combined occurrence throughout the resolution, when the data available make known the particulars of the in situ and real-time solving-and-expressing of a mathematical problem with digital technologies.

Fig. 3.11
figure 11

Processes of solving-and-expressing the problem ‘Decorative Drawing’

Full size image

4.3 A Summary of the Processes Involved in Solving-and-Expressing Mathematical Problems with Technology

Our MPST model allowed a thorough and detailed description of Marco’s processes while addressing two SUB14 geometry problems. As both the digital solution and the observed activity were analyzed, it is now possible to summarize the aspects that best characterize each of the processes involved in solving-and-expressing those problems with digital tools, also refining the descriptors presented previously in Table 3.1.

The student begins his approach to a new problem by reading the statement several times, in trying to get a sense of the mathematical notions and contents that may be involved, as well as by assessing his confidence on his ability to reach a solution based on the easiness he has with the subject or with possible ways of dealing with the problem (grasp). Sometimes he seeks support, at some point of his activity, by getting help from family members, from searching the Internet, from his teacher or, as it happens in the case of the activity observed, from the researcher (communicating). Then, there is a process of deepening the understanding of the conditions stated in the problems, either realizing that it is necessary and possible to construct the sequence of the 8 squares (in the 1st problem), or realizing some elements in the figures, such as the fact that the triangle is equilateral (in the 2nd problem) (notice).

While in the first solution the available data suggest that Marco proceeds to the recognition of certain affordances of the GeoGebra graphical view (interpret), the second solution offers evidence that this move can be much more complex. As it turned out, the production of a sequence of arguments and the several attempts initiated, that eventually led to the formulation of a conjecture about the unknown value, took place in a back-and-forth between two processes—notice and interpret. This means that the student realizes that the triangle is equilateral (identify) and analyzes the central circle so as to reach the smaller ones (interpret); then finds several possibilities of decomposition of the triangle (identify) and with the fingers draws imaginary bisectors and estimates distances (interpret); finally, he visualizes another way of decomposing the triangle into two that are similar (identify) and simulates this decomposition with the finger, formulating a conjecture about a possible solution to the problem (interpret).

The following processes are aimed at developing the formulated conjectures, which involves the use of digital tools with a mathematical sense: in the first solution, Marco uses the grid in GeoGebra’s graphic view to build the extended sequence of squares, based on the coordinates of its vertices, constructs a ray, and also changes properties of some objects to highlight them; in the second solution, he uses the Snipping Tool to create files with the images of the original triangle and a top triangle resulting from decomposing the large one, then he draws the bottom side of this new one. In this problem, the integrate process is developed in association with the explore, i.e. an attempt is made to analyze the possibility of overlapping the two figures, but as it turned out to be unsuccessful, Marco graphically edits the images in order to transform the original triangle and recolor components of that figure (integrate), therefore visually showing that they are similar (explore). Otherwise, in the first problem, the analysis of a conceptual model occurs when Marco resorts to the spreadsheet in GeoGebra and inserts lists with the lengths of the sides of the squares and their areas (explore).

Then it follows the outline of an approach that leads to the solution from the conceptual models that were previously developed. In one case, completing the construction of a surrounding rectangle around the complete sequence of squares and recording its area in the spreadsheet indicates that Marco has found a way to examine his conjecture. In the other case, it is the abandonment of the editing tools and the move to the spreadsheet, where Marco normally composes the solutions, which indicates that the constructed figures already have a purpose (plan).

The next process concerns the development of the planned approach—in a case getting the difference between the calculated areas, and in the other through the insertion and arrangement of the edited images—during which Marco uses mathematical and technological knowledge to obtain the solution (create). In this process, certain elements intentionally created by Marco reveal a techno-mathematical understanding of the solutions, like the case of the surrounding rectangle or of the transformed triangle to exhibit its similarity to the smaller one. Those are new objects of knowledge created by Marco to solve-and-express the problem.

The following actions are directly related to the explanation of the solution or the justification of the reasoning through mathematical arguments supported by the technological resources (verify). In particular, Marco uses the GeoGebra spreadsheet to record the sequence of steps taken, so the combination of construction and organized calculations generates a techno-mathematical solution that ‘self-explains’ the problem solved. In his other solution, Marco describes in the spreadsheet some of the attempts he performed and explains how he got the solution, which occurred precisely when he articulated his mathematical thinking with the edited images.

Finally, the submission of the solutions is done through the online form of the SUB14 webpage and consists of sending the prepared files, which may contain some indications to the receivers on how to manage the information that he provides in his digital materials (disseminate). In the problems that he solved at distance and also in the case of the problem that Marco solved under the observation of the researcher, the young man made his report on the help he might had or not, about the degree of difficulty of the problem, and about whether he had enjoyed to solve it.

5 Discussion and Conclusions

The problem solving activity reported in this case illustrates how digital tools stimulate altogether the development of mathematical understanding that becomes crucial for finding and expressing the solution to the problems. It also shows that Marco’s ability to perceive affordances in the tools is of significant relevance for achieving success in such activity.

In the first solution, this student-with-media uses GeoGebra in unconventional ways (Jacinto et al., 2016): although he recognizes a number of affordances, the construction is not built to be robust and the spreadsheet is not brought up to compute. Instead, the grid promotes an almost immediate ‘materialization’ of the squares’ vertices and the construction prompts the development and exploration of a visual perception, while the spreadsheet allows recording every step of his strategy, which includes the reasoning and the procedures taken.

The second solution brings forth the relevant role of home-technologies which are often regarded as deprived of mathematical affordances, but were fundamental in the development of a mathematical way of approaching the problem.

At some point, in both solutions, Marco-with-media creates new objects not mentioned in the problems. The new mathematical meanings that he derives from them assist him in solving and in expressing the solutions: the enveloping rectangle, in the first solution, and the transformed triangles, in the second solution. Furthermore, the constructions, transformations, and the explanations Marco provides are not mere postscripts added after the solution is found. Those inscriptions are crucial elements within his work that assume a double role: they simultaneously support the finding and the reporting of the answer.

The MPST model provides analytical means to inspect and to account for the processes involved in Marco’s activity, either based on digital documental data or on the observation of the activity itself. Solving-and-expressing accounts for the synchronous process of mathematization and expression of mathematical thinking (Carreira et al., 2016). Marco’s activity reveals his purpose in producing a solution that is self-explainable, thus, solving-and-expressing-with-technology summarizes the whole process, from the beginning of his approach to the submission of his solutions.

Moreover, the MPST model reveals its potential as it accounts for the analysis of data stemming from multiple sources and characters. This is particularly relevant since the model allows identifying critical moments in the activity characterized by multiple sequences of processes, moving forth and back in an iterative way. For instance, the process of using editing tools to create similar triangles (integrate) lead to an attempt to overlap the figures (explore), while the analysis of this experience and the realization of its impossibility leads to using mathematical and technological resources (integrate) to look for a different way of demonstrating the similarity (explore).

While the integration of mathematical and technological resources aims to develop an exploratory approach, the analysis of such exploration (e.g., manipulation, conjecture, computation) may trigger the integration of new resources and, again engage in an exploration process. Thus, the integration is a key process in the simultaneous activity of mathematizing and expressing mathematical thinking by means of digital technologies.

This research may open new avenues on the kinds of mathematical thinking and problem solving skills that young students are capable of putting forth in challenging situations beyond school, entangling academic and informal knowledge. On the one hand, the results obtained demonstrate that technological resources and mathematical resources are equally indispensable to the problem-solving activity with technologies. On the other hand, they show that the nature of mathematical thinking developed with technology changes: technology opens up more ways of exploration, manipulation, observation, conjecture, and explanation.