A characterization of maximin

Abstract

We characterize maximin on the basis of anonymity, continuity, weak Pareto and weak Hammond equity. In contrast to the standard Hammond equity axiom, weak Hammond equity supports only utility transfers that unambiguously diminish overall inequality.

1 Introduction

Maximin and leximin epitomize the extremely egalitarian position in comparisons of social welfare. Both criteria support complete equality, except if this makes everyone worse off.¹ Maximin focuses solely on the lowest utility. Leximin extends maximin lexicographically by focusing on the second lowest utility if the lowest utilities tie, on the third lowest utility if the second lowest utilities also tie, and so on. The two criteria have been put forward and studied as formalizations of (a utility-based version of) Rawls ’ (1971) well-known difference principle.²

The axiomatic social choice literature has traditionally given less attention to maximin than to leximin.³ In this note, we provide a characterization of maximin in the spirit of Hammond ’s (1976) classic characterization of leximin. Hammond shows that leximin is the only criterion satisfying anonymity, strong Pareto and Hammond equity.

Hammond equity requires that a utility transfer from a better off individual to a worse off individual results in a social improvement, irrespective of how leaky the transfer is. The axiom is usually regarded as a demanding ethical requirement. However, (Hammond (1991), pp. 209–210), stresses that Hammond equity is demanding only if utility differences (as opposed to only utility levels) are interpersonally comparable. Indeed, otherwise utility leaks have no meaning. Our setting allows both the case of comparable and non-comparable utility differences. We, moreover, consider a new variation on Hammond equity. Weak Hammond equity is concerned only with alternatives in which there is a single worst off individual, while the remaining individuals are all equally well off. The axiom recommends transfers that diminish the gap between the worst off individual and the group of best off individuals. Such transfers, contrary to those recommended by Hammond equity, unambiguously diminish inequality in the overall population.

We show that maximin is the only criterion satisfying anonymity, continuity, weak Pareto and weak Hammond equity. As a corollary, we obtain a natural counterpart of Hammond ’s (1976) result, viz., a characterization of maximin on the basis of anonymity, continuity, weak Pareto and the standard Hammond equity axiom.

2 Preliminaries

The set of individuals is $N = \{1, 2, \ldots , n\}$. We denote a utility vector by $u=(u_{1},u_{2},\ldots ,u_{n})$ with $u_{i} \in \mathbb {R}$ the utility level of individual $i\in N$.⁴ We write $(u_{(1)},u_{(2)},\ldots ,u_{(n)})$ for a permutation of $u \in \mathbb {R}^{n}$ such that $u_{(1)}\le u_{(2)}\le \cdots \le u_{(n)}$. A quasi-ordering is a reflexive and transitive binary relation. A social ranking is a quasi-ordering $R$ (“is at least as good as”) on $\mathbb {R}^{n}$. The asymmetric and symmetric parts of $R$ are denoted by $P$ (“is better than”) and $I$ (“is equally good as”).

The maximin quasi-ordering compares utility vectors using only the lowest utility in each. A quasi-ordering $R$ is maximin if, for all $u,v\in \mathbb {R}^{n}$, we have

$$\begin{aligned} uRv \quad \hbox {if and only if} \quad u_{(1)}\ge v_{(1)}. \end{aligned}$$

The leximin quasi-ordering extends maximin lexicographically by moving on to the second lowest utilities if the lowest utilities tie, to the third lowest if the second lowest also tie, and so on. A quasi-ordering $R$ is leximin if, for all $u,v\in \mathbb {R}^{n}$, we have

$$\begin{aligned}&uRv\quad \mathrm{{if\ and\ only\,\,if}}\quad u\ \mathrm{{is\,\,a\ permutation\,\,of\ }} v,\ \mathrm{{or\,\,there\,\,is\,\,a\ }} j\,\,\le \,\,n\,\,\mathrm{{such\ that }}\\&\qquad \qquad \qquad \qquad \quad \qquad \,\, u_{(j)} > v_{(j)}\ \mathrm{{and}}\,\, u_{(i)} = v_{(i)}\,\,\mathrm{{for\ all}}\ i < j. \end{aligned}$$

Note that maximin and leximin are complete quasi-orderings.

We define five axioms for a social ranking. Anonymity requires that the identities of the individuals do not matter.

Anonymity For all $u,v\in {\mathbb {R}}^{n}$, if $u$ is a permutation of $v$, then $uIv$.

Continuity ensures that small changes in a utility vector only cause small changes in its social ranking with respect to other utility vectors.

Continuity For all $u\in \mathbb {R}^{n}$, if a sequence of vectors $\{v^{k}\}_{k\in \mathbb {N}}$ converges to $v$ and $uRv^{k}$ (respectively, $v^{k}Ru$) for all $k\in \mathbb {N}$, then $uRv$ (respectively, $vRu$).

Weak Pareto demands that an increase in the utility of every individual is a social improvement.

Weak Pareto For all $u,v\in {\mathbb {R}}^{n}$, if $u_{i}>v_{i}$ for all $i\in N$, then $uPv$.

Hammond equity considers a change affecting only two individuals. The axiom requires that if the utility of the worse off among the two increases, while the utility of the better off decreases, and the order of their utilities is preserved, then a weak social improvement results.

Hammond equity For all $u,v\in {\mathbb {R}}^{n}$, if $v_{i}<u_{i}<u_{j}<v_{j}$ for some $i,j\in N$, and $u_{k}=v_{k}$ for all $k\in N \setminus \{i,j\}$, then $uRv$.

We introduce a new variation on Hammond equity. Weak Hammond equity says that if there is exactly one worst off individual $i$ and all those better off have equal utilities, then society must weakly approve of a change that increases the utility of $i$ (such that $i$ remains worst off) and decreases the utilities of the best off individuals (such that they remain best off).

Weak Hammond equity For all $u,v\in {\mathbb {R}}^{n}$, if $v_{i} = v_{(1)} < u_{i} = u_{(1)} < u_{(2)} = u_{(3)} = \cdots = u_{(n)} < v_{(2)} = v_{(3)} = \cdots = v_{(n)}$ for some $i\in N$, then $uRv$.

By transitivity, a quasi-ordering that satisfies Hammond equity also satisfies weak Hammond equity.

The transfers recommended by weak Hammond equity unambiguously diminish inequality in the overall population, whereas those recommended by Hammond equity do not. By consequence, Hammond equity may be controversial as an egalitarian principle. To see this, consider the Lorenz criterion, which captures the unanimous inequality judgments of a standard class of inequality measures.⁵ For all $u,v\in \mathbb {R}_{++}^{n}$, we have that $v$ is at least as unequal as $u$ according to the Lorenz criterion if and only if

$$\begin{aligned} \frac{u_{(1)} + u_{(2)} + \cdots + u_{(k)}}{u_{(1)} + u_{(2)} + \cdots + u_{(n)}} \ \ge \ \frac{v_{(1)} + v_{(2)} + \cdots + v_{(k)}}{v_{(1)} + v_{(2)} + \cdots + v_{(n)}} \quad \hbox {for all}\ k = 1, 2, \ldots , n. \end{aligned}$$

⁶Let $n = 4$ and consider the utility vectors $u = (11, 11, 1{,}000, 1{,}000)$ and $v = (10, 100, 1{,}000, 1{,}000)$. Hammond equity implies $uRv$, but the Lorenz quasi-ordering does not compare $u$ and $v$. In fact, according to many well-known Lorenz-consistent inequality measures, including the Gini measure, the Theil measure, the mean logarithmic deviation and the coefficient of variation, $u$ is strictly more unequal than $v$.⁷ Hence, egalitarians committed to such inequality measures may legitimately oppose the preference expressed by Hammond equity. Weak Hammond equity, on the other hand, is always in line with the Lorenz criterion: if a pair of utility vectors $u$ and $v$ is such that weak Hammond equity implies $uRv$, then $u$ is strictly less unequal than $v$ according to the Lorenz criterion.⁸

3 Result and discussion

The following result says that maximin is the only quasi-ordering satisfying anonymity, continuity, weak Pareto and weak Hammond equity.

Theorem

A quasi-ordering $R$ on $\mathbb {R}^{n}$ satisfies anonymity, continuity, weak Pareto and weak Hammond equity if and only if it is maximin.

Proof

It is easy to verify that maximin satisfies the four axioms mentioned in the theorem. Therefore, we concern ourselves with the reverse implication. Let $R$ be a quasi-ordering on $\mathbb {R}^{n}$ that satisfies the four axioms. Consider two utility vectors $u,v\in \mathbb {R}^{n}$.

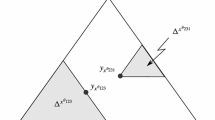
(i) Suppose that $u_{(1)}>v_{(1)}$. We have to show that $uPv$. Construct vectors $w,z\in \mathbb {R}^{n}$ such that

$$\begin{aligned}&v_{(1)}<w_{(1)}<z_{(1)}< z_{(2)}= z_{(3)} = \cdots =z_{(n)} \\&\quad <u_{(1)}\le \max (u_{(n)},v_{(n)}) < w_{(2)} = w_{(3)} = \cdots = w_{(n)}. \end{aligned}$$

By anonymity, weak Pareto and transitivity, we have $uPz$ and $wPv$. By anonymity and weak Hammond equity, we also have $zRw$. Hence, we get $uPv$ using transitivity.

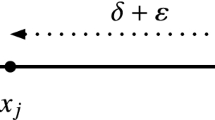
(ii) Suppose that $u_{(1)}=v_{(1)}$. We have to show that $uIv$. Construct two sequences of vectors $\{x^{k}\}_{k\in \mathbb {N}}$ and $\{y^{k}\}_{k\in \mathbb {N}}$ with

$$\begin{aligned} x^{k}=\left( u_{1}+\frac{1}{k},u_{2}+\frac{1}{k},\ldots ,u_{n}+\frac{1}{k}\right) \end{aligned}$$

and

$$\begin{aligned} y^{k}= \left( u_{1}-\frac{1}{k},u_{2}-\frac{1}{k},\ldots ,u_{n}-\frac{1}{k}\right) . \end{aligned}$$

By construction, both sequences converge to $u$. Furthermore, $x_{(1) }^{k}=u_{(1)}+\frac{1}{k}>u_{(1)}=v_{(1)}$ and $y_{(1)}^{k}=u_{(1)}-\frac{1}{k}<u_{(1)}=v_{(1)}$ for all $k\in \mathbb {N}$. Thus, by (i) above, we also have $x^{k}Rv$ and $vRy^{k}$ for all $k\in \mathbb {N}$. Using continuity, we get $uRv$ and $vRu$, and thus $uIv$. $\square$

We show that the axioms in the theorem are independent. For each of the four axioms, we give an example of a quasi-ordering that violates the axiom, but satisfies the other three. Leximin satisfies anonymity, weak Pareto and weak Hammond equity, but not continuity. Let $R$ be a quasi-ordering such that, for all $u,v \in \mathbb {R}^{n}$, we have $uRv$ if and only if $u_{(n)} - u_{(1)} \le v_{(n)} - v_{(1)}$. This quasi-ordering satisfies anonymity, continuity and weak Hammond equity, but not weak Pareto. Let $R$ be a quasi-ordering such that, for all $u,v\in \mathbb {R}^{n}$, we have $uRv$ if and only if $u_{(n)}\ge v_{(n)}$. This ‘maximax’ quasi-ordering satisfies anonymity, continuity, weak Pareto, but not weak Hammond equity. Finally, let $R$ be a quasi-ordering such that, for all $u,v \in \mathbb {R}^{n}$, we have $uRv$ if and only if $u_{i} = u_{(1)}$ and $v_{i} = v_{(1)}$ for some $i\in N$ and $u_{(1)} \ge v_{(1)}$. This quasi-ordering satisfies continuity, weak Pareto and weak Hammond equity, but not anonymity.⁹

The following corollary of the theorem is a natural counterpart of Hammond ’s (1976) characterization of leximin on the basis of anonymity, strong Pareto¹⁰ and Hammond equity.

Corollary

A quasi-ordering $R$ on $\mathbb {R}^{n}$ satisfies anonymity, continuity, weak Pareto and Hammond equity if and only if it is maximin.

To conclude, we note that an alternative characterization of leximin is obtained using anonymity, weak Pareto, weak Hammond equity and separability. Separability demands that the social ranking is independent of the utilities of indifferent individuals.¹¹ We omit the formal statement and proof of this result,¹² as a similar result has already been presented by (Tungodden (2000), Theorem 3). Tungodden’s result uses (i) an equity axiom intermediate in strength between weak Hammond equity and Hammond equity, and (ii) strong Pareto instead of weak Pareto.