1 Introduction

Inflation is defined as a rise in the general level of prices of goods and services in an economy over a period of time. When the general price level rises, each unit of currency buys fewer goods and services. Consequently, inflation reflects a reduction in the purchasing power per unit of money. The problem of inventory systems under inflationary conditions has received attention in recent years. Due to high inflation and consequent sharp decline in the purchasing power of money, especially in the developing countries, the financial situation has been changed and so it is impossible to ignore the effect of inflation and time value of money.

In the production system, the output (i.e., product) of a firm depends upon the combination of production factors. These factors may be raw material, number of labours, production procedure, firm size, quality of the product etc. Because of these changes, production rate and cost are changed too. Moreover, due to increased equipment wear, overtime labor, greater defect rates, etc, the production rate should be a decision variable in determining the lot size or run length of a manufactured item. Also, in practice, the unit production cost varies with production rate, raw material cost, labour charge and advertisement cost of the production process. In this investigation, unit production cost is dependent on the cost of raw materials, labour charges, advertisement cost, produced units, etc.

Generally, high selling price makes a negative impact on a major part of the customers to buy the products. That is, the market demand is inversely related to selling price. Thus, change in price affects the demand, which in turn affects the decisions on production and inventory policies. The advertising by the sales team is one of the most important factors to increase the retailer’s profit in modern marketing system. The purpose of the advertisement is to enhance potential customer’s responses to a business organization. In general, this strategy is only to sell more items in a short time. More demand implies more selling of the items. The advertising intensity increases not only the probability of successful marketing targets but also the demand of the customers. Therefore, the more investment in advertising gives more profits for the company. In this direction, our model encourage the retailers to consider the demand as increasing function of advertising parameter with decreasing value of selling price.

In traditional inventory models, holding cost is known and constant. But holding cost may not always be constant. This is particularly true in the storage of deteriorating and perishable items such as foodstuffs, milk, fruit, vegetables and meat, whose quality drops with each passing day and, as a result, increasing holding costs are necessary to maintain the freshness of the items and to prevent spoilage. Moreover, due to inflation, bank interest, hiring charge, etc., increase with time. Thus some factors contributing to the holding cost changes with time and other remain constant. Hence the holding cost per unit time changes with time and can be assumed to be time dependent.

Deterioration plays a significant role in many inventory systems. Deterioration is defined as decay, damage, dryness and spoilage. It is the process in which an item loses its utility and become useless. Inventory in deteriorating items is a general phenomenon in daily life. Most physical goods undergo decay or deterioration over time, examples being medicines, volatile liquids, blood banks, and so on. So decay or deterioration of physical goods in stock is a very realistic factor and there is a big need to consider this in inventory modeling. The next section reviews the relevant literature on inventory management issues from the perspective of the production industries.

2 Literature review

Most of the inventory models did not take into account the effects of inflation and time value of money. During the last few years, the economic situation of most countries have been changed such that it has not been possible to ignore the effects of inflation and time value of money further. Buzacott [1] first developed the economic order quantity (EOQ) model taking inflation into account. Sarker and Pan [2] considered a finite replenishment model when shortage is allowed. Chung [3] developed an algorithm with finite replenishment and infinite planning horizon. Datta and Pal [4] considered the effects of inflation and time value of money on an inventory model with a linear time-dependent demand rate and shortages. Hariga [5] extended Datta and Pal’s [4] model to consider flexible replenishment time and decreasing demand rate function. Bierman and Thomas [6] investigated an inventory decision under inflationary conditions. Ray and Chaudhuri [7] provided an EOQ model with inflation time discounting. Uthayakumar and Geetha [8] developed a replenishment policy for single item inventory model with money inflation. Thangam and Uthayakumar [9] studied an inventory model for deteriorating items with inflation induced demand and exponential partial backorders–a discounted cash flow approach. Valliathal and Uthayakumar [10] provided the Production—inventory problem for ameliorating/deteriorating items with non-linear shortage cost under inflation and time discounting. Sarkar [11] developed an EMQ model with imperfect production process for time varying demand with inflation and time value of money. Tolgari [12] studied an inventory model for imperfect items under inflationary conditions by considering inspection errors. Guria [13] formulated an inventory policy for an item with inflation induced purchasing price, selling price and demand with immediate part payment. Yang [14] developed a two-warehouse partial backlogging inventory model for deteriorating items with permissible delay in payment under inflation.

In the production lot size models, both production rate and production cost are assumed to be constant and independent of each other. Several researchers developed inventory models for a single item or multiple items taking constant or variable production rate (as a function of demand and/or on-hand inventory). In this connection, one may refer the works of Misra [15], Mandal [16], Mandal [17] and Maiti [18]. In their models, the production cost is taken as constant. Khouja [19] provided an economic production lot size model under volume flexibility where unit production cost depends upon the raw material, labour force and tool wear out cost incurred. Here, unit production cost is a function of production rate. Bhandari [20] extended the work of Khouja [19] including the marketing cost and taking a generalized unit cost function.

In the present competitive market, the marketing policies and promotion of a product in the form of advertisement, display, etc. change the demand pattern of that item amongst the customers and give a motivational effect on the people to buy more. Also, the selling price of an item is one of the important factors in selecting an item for use. It is commonly seen that higher selling price causes decrease in demand whereas lesser selling price has the reverse effect. Hence, it can be concluded that the demand of an item is a function of marketing cost and selling price of an item. Kotler [21] incorporated marketing policies into inventory decisions and studied the relationship between economic order quantity and decision. Ladany [22] discussed the effect of price variation on demand and consequently on EOQ. Subramanyan [23], Urban [24], Goyal and Gunasekaran [25], Luo [26] developed inventory models incorporating the effect of price variations and advertisement on demand. Mondal [27] developed an inventory models for defective items incorporating marketing decisions with variable production cost. Chang [28] studied an economic manufacturing quantity model for a two-stage assembly system with imperfect processes and variable production rate. Soni [29] investigated an optimal strategy for an integrated inventory system involving variable production and defective items under retailer partial trade credit policy. Deane [30] considered a model for scheduling online advertisements to maximize revenue under variable display frequency.

In most of the inventory model, mentioned above, holding cost has been considered as a constant function. But, in real-life situations, when the deteriorating and perishable items such as food products are kept in storage, the more complicated the storage facilities and services needed, and therefore, the higher the holding cost. Therefore, the holding cost is always not a constant function. It’s varying according to time. In generalization of EOQ models, various functions describing holding cost were considered by several researchers like Naddor [31], Veen [32], Muhlemann [33], Weiss [34], and Goh [35]. Alfares [36] proposed an inventory model with stock-level dependent demand rate and variable holding cost. Roy [37] developed an inventory model for deteriorating items with time varying holding cost and demand is price dependent. Mishra [38] developed a deteriorating inventory model for time dependent demand and holding cost with partial backlogging. Shah [39] studied an optimizing inventory and marketing policy for non-instantaneous deteriorating items with generalized type deterioration and holding cost rates. Pando [40] developed the maximizing profits in an inventory model with both demand rate and holding cost per unit time dependent on the stock level. Pando [41] gave an economic lot-size model with non-linear holding cost hinging on time and quantity.

Deterioration is defined as decay, damage, spoilage, evaporation, obsolescence, pilferage, loss of utility or loss of marginal value of a commodity that results in decreased usefulness. For the case of perishable product, the manufacturer may need to backlog demand to avoid costs due to deterioration. So perishability and backlogging are complementary conditions. Many inventory modelers presented their inventory models considering backlogging rate to be linearly dependent on the total number of customers in the waiting line. However the backlogging rate should depend on the time spend in waiting for the arrival of the next lot. Montgomery [42], Park [43], Mak [44], Chang [45], Abad [46,47], Ghosh [48], etc. developed inventory models considering backorders and lost sales. Raafat [49] provided a model on continuously deteriorating items in an excellent way. A joint pricing ordering policy for deteriorating inventory was studied by Mukhopadhyay [50]. Optimal selling price and lot size with time varying deterioration with partial backlogging was developed by Sana [51]. Ghosh [52] discussed an optimal price and lot size determination for a perishable product under conditions of finite production, partial backordering and lost sale. Maihami [53] considered an inventory control model for non-instantaneous deteriorating items with partial backlogging and time and price dependent demand. Krishnamoorthi [54] developed an economic production lot size model for product life cycle (maturity stage) with defective items with shortages. Dye [55] investigated the effect of preservation technology investment on a non-instantaneous deteriorating inventory model.

In the present work, a deterministic EPQ model for perishable items with stock and advertisement dependent demand, variable production rate, unit production cost is a function of raw materials, labour charges, advertisement cost, produced units, etc. and selling price is a mark-up over the unit production cost are proposed. Also inflation and time value of money, the holding cost is expressed as linearly increasing functions of time considered and shortages are allowed and partially backlogged in this model. We have shown the suitable numerical examples to illustrate the model.

3 Assumptions and notations

To develop the mathematical model, the following assumptions are being made:

3.1 Assumptions

  1. 1.

    A single item is considered over an infinite planning horizon.

  2. 2.

    The demand rate D is a deterministic function of selling price, s and advertisement cost, A C per unit item i.e. D(A C , s) = A γ C (x − ys), x .  y, γ ≥ 0.

  3. 3.

    The production rate P is variable, which is more than the demand rate.

  4. 4.

    The unit production cost v(P) = C rw  + A C  + L/P c + KP d where C rw , L and K are non-negative real numbers to be provide the best fit for the estimated unit cost function. C rw , L and K represent the raw material cost, labour charges and a positive constant. Also c, d are chosen to provide the feasible solution to the model. We shall use v and v(P) interchangeable in the rest of the paper.

  5. 5.

    The selling price is determined by a mark-up over the unit production cost, v i.e. s = n v, n > 1, where n is a mark-up .

  6. 6.

    The items deteriorate continuously at a rate σ.

  7. 7.

    There is no replenishment or repair of deteriorated items takes place in a given cycle.

  8. 8.

    The inflation rate is constant.

  9. 9.

    The lead time is zero.

  10. 10.

    C 1(t) = a + bt, a ≥ 0, b ≥ 0 is the holding cost, which is linear function of time.

  11. 11.

    Shortages are allowed and during stock out period, only a fraction B(η) of the demand is backlogged where η is the amount of time for which the customer waits before receiving goods and remaining fraction 1 − B(η) is lost. B(η) is given by \( B\left(\eta \right)={k}_0{e}^{-{k}_1\;\eta },{k}_0<1,{k}_1\ge 0. \)

3.2 Notations

In addition, the following notations are used throughout this paper:

q(t):

The inventory level at any time t.

β, ψ :

The interim time spans.

T :

The duration of an inventory cycle when stockout condition exists.

λ :

The duration of an inventory cycle when there is positive inventory.

A :

The setup cost per cycle.

C 3 :

The shortage cost for backlogged items per unit.

C 4 :

The unit cost of lost sales per unit.

Q :

The order size per cycle.

S :

The maximum inventory level.

r :

The discount rate represents the time value of money.

f :

The inflation rate.

R :

The net discount rate of inflation i.e. R = r − f.

TC :

The total cost of the system with perishable product.

TC N :

The total cost of the system with non-perishable product.

4 Formulation and solution of the model

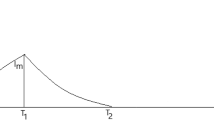
The inventory system is developed as follows: The inventory starts with zero stock at zero time. So shortage begins to build up at the early stage in inventory cycle. The production starts at time β to meet the current and backlogged demand. T is the time when the shortage level reaches to zero; afterwards a positive level of inventory begins to accumulate. At time T + ψ, the production process stops and the inventory level then starts declining. Finally the cycle ends with zero stock at time T + λ. The behaviour of the inventory model is demonstrated in Fig. 1.

Fig. 1
figure 1

Graphical representation of the inventory system

Full size image

Based on the above description, during the time interval [0,β], the differential equation representing the inventory status is given by

$$ \begin{array}{ll}\frac{ dq(t)}{ dt}=-D\;B\left(\beta -t\right),\hfill & 0\le t\le \beta \hfill \end{array} $$
(1)

With the condition q(0) = 0, the solution of Eq. (1) is

$$ \begin{array}{ll}q(t)=\frac{D{k}_0}{k_1}{e}^{-{k}_1\;\beta}\left[1-{e}^{k_1\;t}\right]\hfill & 0\le t\le \beta \hfill \end{array} $$
(2)

In the second interval [β,T], the differential equation below represents the inventory status:

$$ \begin{array}{ll}\frac{ dq(t)}{ dt}=P-D,\hfill & \beta \le t\le T\hfill \end{array} $$
(3)

With the condition q(T) = 0, we get the solution of Eq. (3) is

$$ \begin{array}{ll}q(t)=\left(P-D\right)\left(t-T\right)\hfill & \beta \le t\le T\hfill \end{array} $$
(4)

Put t = β in Eqs. (2) and (4) we find the value of T as

$$ T=\beta -\frac{D{k}_0}{k_1\left(P-D\right)}\left[{e}^{-{k}_1\;\beta }-1\right] $$
(5)

During the third interval [T, T + ψ], the inventory level at time t is governed by the following differential equation:

$$ \begin{array}{ll}\frac{ dq(t)}{ dt}+\sigma\;q(t)=P-D,\hfill & T\le t\le T+\psi \hfill \end{array} $$
(6)

With the condition q(T) = 0, the solution of Eq. (6) is

$$ \begin{array}{ll}q(t)=\frac{P-D}{\sigma}\left[1-{e}^{\sigma \left(T-t\right)}\right]\hfill & T\le t\le T+\psi \hfill \end{array} $$
(7)

During the time interval [T + ψ, T + λ], the inventory status is given by

$$ \begin{array}{ll}\frac{ dq(t)}{ dt}+\sigma\;q(t)=-D,\hfill & T+\psi \le t\le T+\lambda \hfill \end{array} $$
(8)

With the condition q(T + λ) = 0, the solution of Eq. (8) is

$$ \begin{array}{ll}q(t)=\frac{D}{\sigma}\left[{e}^{\sigma \left(T+\lambda -t\right)}-1\right]\hfill & T+\psi \le t\le T+\lambda \hfill \end{array} $$
(9)

Equating the expressions (7) and (9) at t = T + ψ, we have the value of ψ as

$$ \psi =\psi \left(\lambda \right)=\frac{1}{\sigma } \log \left[1+\frac{D}{P}\left[{e}^{\sigma\;\lambda }-1\right]\right]. $$
(10)

We shall use ψ and ψ(λ) interchangeable in the rest of the paper.

Since the production occurs in the continuous time-span [β,T] and [T, T + ψ]. Thus the order size in the problem during total time interval [β,T] and [T, T + ψ] is,

$$ Q=P\left(T-\beta \right)+ P\psi . $$
(11)

The maximum inventory level S is given by

$$ S=q\left(T+\psi \right)=\frac{P-D}{\sigma}\left[1-{e}^{-\sigma\;\psi}\right] $$
(12)

Now we want to find the different inventory costs with the effect of inflation as:

$$ \mathrm{Setup}\ \mathrm{cost}=A. $$
(13)

The production cost \( PC={\displaystyle \underset{\beta }{\overset{T}{\int }} vP{e}^{-R\;t}} dt+{\displaystyle \underset{T}{\overset{T+\psi }{\int }} vP{e}^{-R\;t} dt} \)

$$ PC=\frac{ vP}{R}{e}^{-R\;T}\left[{e}^{R\left(T-\beta \right)}-{e}^{-R\;\psi}\right] $$
(14)

The deteriorating cost DC during the period [T, T + ψ] and [T + ψ, T + λ] is

$$ \begin{array}{l}\begin{array}{c}\hfill DC={\displaystyle \underset{T}{\overset{T+\psi }{\int }} vP{e}^{-R\;t} dt-}{\displaystyle \underset{T}{\overset{T+\lambda }{\int }} vD{e}^{-R\;t}} dt\hfill \\ {}\hfill =\frac{v}{R}{e}^{-R\;T}\left[D\left({e}^{-R\;\lambda }-1\right)-P\left({e}^{-R\;\psi }-1\right)\right]\hfill \end{array}\\ {}\kern1.2em \end{array} $$
(15)

The holding cost HC is given by

$$ \begin{array}{c}\hfill HC={\displaystyle \underset{T}{\overset{T+\psi }{\int }}{C}_1(t)\;}{e}^{-R\;t}q(t) dt+{\displaystyle \underset{T+\psi }{\overset{T+\lambda }{\int }}{C}_1(t)\;}{e}^{-R\;t}q(t) dt\hfill \\ {}\hfill HC=\frac{P}{\sigma }{e}^{- RT}\left\{\left(\frac{1-{e}^{-R\;\psi }}{R}\right)\left(a+ b T+\frac{b}{R}\right)+\left(\frac{e^{-\psi \left(R+\sigma \right)}-1}{R+\sigma}\right)\left(a+ b T+\frac{b}{R+\sigma}\right)+\right.\left. b\psi\;{e}^{-R\;\psi}\left(\frac{e^{-\sigma\;\psi }}{R+\sigma }-\frac{1}{R}\right)\right\}\hfill \\ {}\hfill +\frac{D}{\sigma }{e}^{- RT}\left\{\left(\frac{e^{-R\;\lambda }-1}{R}\right)\left(a+ b T+\frac{b}{R}\right)+\left(\frac{1-{e}^{-R\;\lambda }}{R+\sigma}\right)\left(a+ b T+\frac{b}{R+\sigma}\right)+\left(\frac{e^{\sigma\;\lambda }-1}{R+\sigma}\right)\right.{e}^{-\psi \left(R+\sigma \right)}\hfill \\ {}\hfill \left.\left(a+ b T+ b\psi +\frac{b}{R+\sigma}\right)+ b\lambda\;{e}^{-R\;\lambda}\left(\frac{2R+\sigma }{R\left(R+\sigma \right)}\right)\right\}\hfill \end{array} $$
(16)

Total shortage cost SC during the period [0,T] is given by

$$ \begin{array}{c}\hfill SC={C}_3\left[{\displaystyle \underset{0}{\overset{\beta }{\int }}-q(t)}{e}^{-R\;t} dt+{\displaystyle \underset{\beta }{\overset{T}{\int }}-q(t){e}^{-R\;t}} dt\right]\hfill \\ {}\hfill =-{C}_3\left\{\frac{D{k}_0}{k{}_1}{e}^{-{k}_1\;\beta}\left[\frac{1}{R}\left(1-{e}^{-R\;\beta}\right)+\frac{1}{k_1-R}\left(1-{e}^{\left({k}_1-R\right)\beta}\right)\right]+\left(P-D\right)\left.\left[\frac{e^{-R\;\beta }}{R}\left(\beta -T\right)+\frac{1}{R^2}\left({e}^{-R\;\beta }-{e}^{-R\;T}\right)\right]\right\}\right.\hfill \end{array} $$
(17)

The lost sales cost LC during the period [0,T] is

$$ LC={C}_4{\displaystyle \underset{0}{\overset{\beta }{\int }}\left(1-{k}_0{e}^{-{k}_1\;\left(\beta -t\right)}\right)D\;{e}^{-R\;T} dt}={C}_4D\left[\frac{k_0}{k_1-R}\left({e}^{-{k}_1\;\beta }-{e}^{-R\;\beta}\right)+\frac{1}{R}\left(1-{e}^{-R\;\beta}\right)\right] $$
(18)

Total average cost per cycle = setup cost + production cost + deterioration cost + inventory holding cost + shortage cost + lost sales cost.

So, the total variable cost per unit time is

$$ \begin{array}{l} TC=\frac{1}{T+\lambda}\left[A+ PC+ DC+ HC+ SC+ LC\right]\hfill \\ {}=\left\{A+\frac{ vP}{R}{e}^{-R\;T}\left[{e}^{R\;\left(T-\beta \right)}-{e}^{-R\;\psi}\right]+\frac{v}{R}{e}^{-R\;T}\left[D\left({e}^{-R\;\lambda }-1\right)-P\left({e}^{-R\;\psi }-1\right)\right]+\frac{P}{\sigma }{e}^{- RT}\left\{\left(\frac{1-{e}^{-R\;\psi }}{R}\right)\left(a+ b T+\frac{b}{R}\right)\right.\right.\hfill \\ {}+\left(\frac{e^{-\psi \left(R+\sigma \right)}-1}{R+\sigma}\right)\left(a+ b T+\frac{b}{R+\sigma}\right)+\left. b\psi\;{e}^{-R\;\psi}\left(\frac{e^{-\sigma\;\psi }}{R+\sigma }-\frac{1}{R}\right)\right\}+\frac{D}{\sigma }{e}^{- RT}\left\{\left(\frac{e^{-R\;\lambda }-1}{R}\right)\left(a+ b T+\frac{b}{R}\right)\right.\hfill \\ {}+\left(\frac{1-{e}^{-R\;\lambda }}{R+\sigma}\right)\left(a+ b T+\frac{b}{R+\sigma}\right)+\left(\frac{e^{\sigma\;\lambda }-1}{R+\sigma}\right){e}^{-\psi \left(R+\sigma \right)}\left.\left(a+ b T+ b\psi +\frac{b}{R+\sigma}\right)+ b\lambda\;{e}^{-R\;\lambda}\left(\frac{2R+\sigma }{R\left(R+\sigma \right)}\right)\right\}\hfill \\ {}-{C}_3\left\{\frac{D{k}_0}{k{}_1}{e}^{-{k}_1\;\beta}\left[\frac{1}{R}\left(1-{e}^{-R\;\beta}\right)+\frac{1}{k_1-R}\left(1-{e}^{\left({k}_1-R\right)\beta}\right)\right]+\left(P-D\right)\left.\left[\frac{e^{-R\;\beta }}{R}\left(\beta -T\right)+\frac{1}{R^2}\left({e}^{-R\;\beta }-{e}^{-R\;T}\right)\right]\right\}\right.\hfill \\ {}\left.+{C}_4D\left[\frac{k_0}{k_1-R}\left({e}^{-{k}_1\;\beta }-{e}^{-R\;\beta}\right)+\frac{1}{R}\left(1-{e}^{-R\;\beta}\right)\right]\right\}/\left(T+\lambda \right)\hfill \end{array} $$
(19)

5 Solution procedure

Now the production rate that minimizes the unit production cost is given by v ′ (P) = 0.

Therefore v ′ (P) = 0 implies

$$ \begin{array}{l}-\frac{c\;L}{P^{c+1}}+K\;d{P}^{d-1}=0\hfill \\ {}\Rightarrow P={\left(\frac{L\;c}{K\;d}\right)}^{\frac{1}{c+d}}\hfill \end{array} $$
(20)

The necessary and sufficient conditions to minimize TC(λ) are respectively \( \frac{ d TC\left(\lambda \right)}{ d\lambda}=0 \) and \( \frac{d^2 TC\left(\lambda \right)}{d{\lambda}^2}>0 \).

Now \( \frac{ d TC\left(\lambda \right)}{ d\lambda}=0 \) gives the following equation in λ.

$$ \begin{array}{l}\left\{ vP+v\left\{P+\left[{e}^{-\left(R+\sigma \right)\lambda}\left(D-P\right)-D{e}^{-R\;\lambda}\right]\;{\left[\frac{P+D{e}^{\sigma\;\lambda }-D}{P}\right]}^{\left(R/\sigma \right)}\right\}+\frac{ P D}{\sigma}\left(\frac{e^{\sigma\;\lambda }-1}{P+D{e}^{\sigma\;\lambda }-D}\right)\left\{a+b\left(T+\frac{1}{\sigma}\right.\right.\right.\hfill \\ {}\left.\left. \log \left[\frac{P+D{e}^{\sigma\;\lambda }-D}{P}\right]\right)\right\}+\frac{P{e}^{-\left(R+\sigma \right)\lambda }}{\sigma }{\left[\frac{P+D{e}^{\sigma\;\lambda }-D}{P}\right]}^{\frac{R}{\sigma }+1}\left\{-\left(a+ b T+b/R\right)+\frac{\left(a+ b T+b/\left(R+\sigma \right)\right)}{R+\sigma}\right.\hfill \\ {}\left.-\frac{b\left(2R+\sigma \right)\left( R\lambda -1\right)}{R\left(R+\sigma \right)}\right\}+\left[\frac{P+D{e}^{\sigma\;\lambda }-D}{P{\left(R+\sigma \right)}^2}\right]\;\left\{\frac{R}{\sigma } \log \left(\frac{P+D{e}^{\sigma\;\lambda }-D}{P}\right)\left[b\left(\frac{ P\sigma}{R}+D\right)-\frac{ bDR}{\sigma}\left({e}^{\sigma\;\lambda }+1\right)\right]\right.\hfill \\ {}\left.\left.- RD\left({e}^{\sigma\;\lambda }-1\right)\;\left[a\left(1+\frac{R}{\sigma}\right)+ b T\left(1+\frac{R}{\sigma}\right)-\frac{b}{R}\right]+P\left(a+ b T\right)\left(1-R\right)+ b P\right\}\right\}/\left(T+\lambda \right)\hfill \\ {}-\left\{A+\frac{ vP}{R}{e}^{-R\;T}\left[{e}^{R\;\left(T-\beta \right)}-{e}^{-R\;\psi}\right]+\frac{v}{R}{e}^{-R\;T}\left[D\left({e}^{-R\;\lambda }-1\right)-P\left({e}^{-R\;\psi }-1\right)\right]+\frac{P}{\sigma }{e}^{- RT}\left\{\left(\frac{1-{e}^{-R\;\psi }}{R}\right)\left(a+ b T+\frac{b}{R}\right)\right.\right.\hfill \\ {}+\left(\frac{e^{-\psi \left(R+\sigma \right)}-1}{R+\sigma}\right)\left(a+ b T+\frac{b}{R+\sigma}\right)+\left. b\psi\;{e}^{-R\;\psi}\left(\frac{e^{-\sigma\;\psi }}{R+\sigma }-\frac{1}{R}\right)\right\}+\frac{D}{\sigma }{e}^{- RT}\left\{\left(\frac{e^{-R\;\lambda }-1}{R}\right)\left(a+ b T+\frac{b}{R}\right)\right.\hfill \\ {}+\left(\frac{1-{e}^{-R\;\lambda }}{R+\sigma}\right)\left(a+ b T+\frac{b}{R+\sigma}\right)+\left(\frac{e^{\sigma\;\lambda }-1}{R+\sigma}\right){e}^{-\psi \left(R+\sigma \right)}\left.\left(a+ b T+ b\psi +\frac{b}{R+\sigma}\right)+ b\lambda\;{e}^{-R\;\lambda}\left(\frac{2R+\sigma }{R\left(R+\sigma \right)}\right)\right\}\hfill \\ {}-{C}_3\left\{\frac{D{k}_0}{k{}_1}{e}^{-{k}_1\;\beta}\left[\frac{1}{R}\left(1-{e}^{-R\;\beta}\right)+\frac{1}{k_1-R}\left(1-{e}^{\left({k}_1-R\right)\beta}\right)\right]+\left(P-D\right)\left.\left[\frac{e^{-R\;\beta }}{R}\left(\beta -T\right)+\frac{1}{R^2}\left({e}^{-R\;\beta }-{e}^{-R\;T}\right)\right]\right\}\right.\hfill \\ {}\left.+{C}_4D\left[\frac{k_0}{k_1-R}\left({e}^{-{k}_1\;\beta }-{e}^{-R\;\beta}\right)+\frac{1}{R}\left(1-{e}^{-R\;\beta}\right)\right]\right\}/{\left(T+\lambda \right)}^2=0\hfill \end{array} $$
(21)

By examining the second order sufficient condition, it can be verified that the total cost TC is a convex function of λ, because the second order differential equation of TC with respect to λ is

$$ \begin{array}{l}\frac{d^2 TC\left(\lambda \right)}{d{\lambda}^2}=\left\{ vRD\;{e}^{- R\lambda}{\left[\frac{P+D{e}^{\sigma\;\lambda }-D}{P}\right]}^{\left(R/\sigma \right)}\right.\left\{\left(\frac{\sigma\;{e}^{\sigma\;\lambda }}{P+D{e}^{\sigma\;\lambda }-D}\right)\left({e}^{-\sigma\;\lambda}\left(D-P\right)-D\right)+1-\left(1+\frac{\sigma }{R}\right)\right\}\hfill \\ {}+\left[\frac{ P D\;{e}^{\sigma\;\lambda }}{{\left(P+D{e}^{\sigma\;\lambda }-D\right)}^2}\right]\left\{ bD\left({e}^{\sigma\;\lambda }-1\right)+P\left[a+b\left(T+\frac{1}{\sigma}\right.\left.\;\left.\left. \log \left(\frac{P+D{e}^{\sigma\;\lambda }-D}{P}\right)\right)\right]\;\right\}\right.\right.\hfill \\ {}+{\left[\frac{P+D{e}^{\sigma\;\lambda }-D}{P}\right]}^{\left(R/\sigma \right)}\left[\frac{\left(a+ b T+b/\left(R+\sigma \right)\right)}{R+\sigma }-\left(a+ b T+b/R\right)-\frac{b\left(2R+\sigma \right)\left( R\lambda -1\right)}{R\left(R+\sigma \right)}\right]\hfill \\ {}\left[P\left(\frac{1}{\sigma }-1\right)+D\left(1-{e}^{\sigma\;\lambda}\right)\right]\left[\frac{\left(R+\sigma \right){e}^{-\left(R+\sigma \right)\lambda }}{\sigma}\right]+\left[\frac{ D\sigma\;{e}^{\sigma\;\lambda }}{P{\left(R+\sigma \right)}^2}\right]\left\{\frac{R}{\sigma } \log \left(\frac{P+D{e}^{\sigma\;\lambda }-D}{P}\right)\right.\hfill \\ {}\left[b\left(\frac{ P\sigma}{R}+D\right)-\frac{ bD R}{\sigma}\left({e}^{\sigma\;\lambda }+1\right)\right]+ RD\left(1-{e}^{\sigma\;\lambda}\right)\;\left[a\left(1+\frac{R}{\sigma}\right)+ b T\left(1+\frac{R}{\sigma}\right)-\frac{b}{R}\right]\hfill \\ {}\left.+P\left(a+ b T\right)\left(1-R\right)+ b P\right\}+\left[\frac{P+D{e}^{\sigma\;\lambda }-D}{P{\left(R+\sigma \right)}^2}\right]\left\{\left[b\left(\frac{ P\sigma}{R}+D\right)-\frac{ bD R}{\sigma}\left({e}^{\sigma\;\lambda }+1\right)\right]\right.\hfill \\ {}\left[\frac{ RD\;{e}^{\sigma\;\lambda }}{\left(P+D{e}^{\sigma\;\lambda }-D\right)}\right]- \log \left(\frac{P+D{e}^{\sigma\;\lambda }-D}{P}\right)\left[\frac{ P D{R}^2{e}^{\sigma\;\lambda }}{\sigma}\right]\left.- R D\sigma\;{e}^{\sigma\;\lambda}\left[a\left(1+\frac{R}{\sigma}\right)+ b T\left(1+\frac{R}{\sigma}\right)-\frac{b}{R}\right]\right\}\hfill \\ {}-{\left[\frac{P+D{e}^{\sigma\;\lambda }-D}{P}\right]}^{\left(R/\sigma \right)+1}\left.\left[\frac{ bP\left(2R+\sigma \right){e}^{-\left(R+\sigma \right)\lambda }}{\sigma \left(R+\sigma \right)}\right]\right\}/\left(T+\lambda \right)\hfill \\ {}-2\left\{ vP+v\left\{P+\left[{e}^{-\left(R+\sigma \right)\lambda}\left(D-P\right)-D{e}^{-R\;\lambda}\right]\;{\left[\frac{P+D{e}^{\sigma\;\lambda }-D}{P}\right]}^{\left(R/\sigma \right)}\right\}+\frac{ P D}{\sigma}\left(\frac{e^{\sigma\;\lambda }-1}{P+D{e}^{\sigma\;\lambda }-D}\right)\left\{a+b\left(T+\frac{1}{\sigma}\right.\right.\right.\hfill \\ {}\left.\left. \log \left[\frac{P+D{e}^{\sigma\;\lambda }-D}{P}\right]\right)\right\}+\frac{P{e}^{-\left(R+\sigma \right)\lambda }}{\sigma }{\left[\frac{P+D{e}^{\sigma\;\lambda }-D}{P}\right]}^{\frac{R}{\sigma }+1}\left\{\frac{\left(a+ b T+b/\left(R+\sigma \right)\right)}{R+\sigma}\right.-\left(a+ b T+b/R\right)\hfill \\ {}\left.-\frac{b\left(2R+\sigma \right)\left( R\lambda -1\right)}{R\left(R+\sigma \right)}\right\}+\left[\frac{P+D{e}^{\sigma\;\lambda }-D}{P{\left(R+\sigma \right)}^2}\right]\;\left\{\frac{R}{\sigma } \log \left(\frac{P+D{e}^{\sigma\;\lambda }-D}{P}\right)\left[b\left(\frac{ P\sigma}{R}+D\right)-\frac{ bD R}{\sigma}\left({e}^{\sigma\;\lambda }+1\right)\right]\right.\hfill \\ {}\left.\left.+ RD\left(1-{e}^{\sigma\;\lambda}\right)\;\left[a\left(1+\frac{R}{\sigma}\right)+ b T\left(1+\frac{R}{\sigma}\right)-\frac{b}{R}\right]+P\left(a+ b T\right)\left(1-R\right)+ b P\right\}\right\}/{\left(T+\lambda \right)}^2\hfill \\ {}+2\left\{A+\frac{ vP}{R}{e}^{-R\;T}\left[{e}^{R\left(T-\beta \right)}-{e}^{-R\;\psi}\right]+\frac{v}{R}{e}^{-R\;T}\left[D\left({e}^{-R\;\lambda }-1\right)-P\left({e}^{-R\;\psi }-1\right)\right]+\frac{P}{\sigma }{e}^{- R T}\left\{\left(\frac{1-{e}^{-R\;\psi }}{R}\right)\left(a+ b T+\frac{b}{R}\right)\right.\right.\hfill \\ {}+\left(\frac{e^{-\psi \left(R+\sigma \right)}-1}{R+\sigma}\right)\left(a+ b T+\frac{b}{R+\sigma}\right)+\left. b\psi\;{e}^{-R\;\psi}\left(\frac{e^{-\sigma\;\psi }}{R+\sigma }-\frac{1}{R}\right)\right\}+\frac{D}{\sigma }{e}^{- R T}\left\{\left(\frac{e^{-R\;\lambda }-1}{R}\right)\left(a+ b T+\frac{b}{R}\right)\right.\hfill \\ {}+\left(\frac{1-{e}^{-R\;\lambda }}{R+\sigma}\right)\left(a+ b T+\frac{b}{R+\sigma}\right)+\left(\frac{e^{\sigma\;\lambda }-1}{R+\sigma}\right){e}^{-\psi \left(R+\sigma \right)}\left.\left(a+ b T+ b\psi +\frac{b}{R+\sigma}\right)+ b\lambda\;{e}^{-R\;\lambda}\left(\frac{2R+\sigma }{R\left(R+\sigma \right)}\right)\right\}\hfill \\ {}-{C}_3\left\{\frac{D{k}_0}{k{}_1}{e}^{-{k}_1\;\beta}\left[\frac{1}{R}\left(1-{e}^{-R\;\beta}\right)+\frac{1}{k_1-R}\left(1-{e}^{\left({k}_1-R\right)\beta}\right)\right]+\left(P-D\right)\left.\left[\frac{e^{-R\;\beta }}{R}\left(\beta -T\right)+\frac{1}{R^2}\left({e}^{-R\;\beta }-{e}^{-R\;T}\right)\right]\right\}\right.\hfill \\ {}\left.+{C}_4D\left[\frac{k_0}{k_1-R}\left({e}^{-{k}_1\;\beta }-{e}^{-R\;\beta}\right)+\frac{1}{R}\left(1-{e}^{-R\;\beta}\right)\right]\right\}/{\left(T+\lambda \right)}^3>0\hfill \end{array} $$

since P > D, k 0 < 1, a, b, k 1 ≥ 0 and 0 < σ < 1.

By solving Eq. (21) the optimal value of λ = λ ∗ can be obtained and then from Eqs. (5), (10), (11), (12), (20) and (19), the optimal value of T = T ∗, ψ = ψ ∗, Q = Q ∗, S = S ∗, P = P ∗ and TC = TC ∗ can be found out respectively.

6 Non-perishable product

Suppose the produced items are non-perishable, then we have σ = 0. Therefore taking limit as σ → 0 or by substituting σ = 0 in the Eqs. (6) and (8), we get

$$ \begin{array}{ll}q(t)=\left(P-D\right)\left(t-T\right),\hfill & T\le t\le T+\psi \hfill \end{array} $$
(22)
$$ \begin{array}{ll}q(t)=-D\left(t-T-\lambda \right),\hfill & T+\psi \le t\le T+\lambda \hfill \end{array} $$
(23)
$$ \psi =\psi \left(\lambda \right)=\frac{ D\lambda}{P} $$
(24)

Therefore the holding cost HC N with the effect of inflation is given by

$$ \begin{array}{c}\hfill H{C}_N={\displaystyle \underset{T}{\overset{T+\psi }{\int }}\left(a+ bt\right)}{e}^{-R\;t}\left(P-D\right)\left(t-T\right) dt+{\displaystyle \underset{T+\psi }{\overset{T+\lambda }{\int }}-\left(a+ bt\right)\;}{e}^{-R\;t}D\left(t-T-\lambda \right) dt\hfill \\ {}\hfill \kern1.2em =P\left\{\frac{-{e}^{-R\left(T+\psi \right)}}{R^2}\left[b\left(T+2\psi +\frac{2}{R}+ R\psi \left(\psi +T\right)\right)+a\left(1+ R\psi \right)\right]+\frac{e^{- RT}}{R^2}\left[a+b\left(T+\frac{2}{R}\right)\right]\right\}\hfill \\ {}\hfill -D\left\{\frac{e^{- RT}}{R^2}\left[a+b\left(T+\frac{2}{R}\right)\right]-\frac{e^{-R\left(T+\lambda \right)}}{R^2}\left[a+b\left(T+\lambda +\frac{2}{R}\right)\right]-\frac{\lambda {e}^{-R\left(T+\psi \right)}}{R}\left[a+b\left(T+\psi +\frac{1}{R}\right)\right]\right\}\hfill \end{array} $$
(25)

So, the total variable cost for non-perishable product per unit time is

$$ \begin{array}{l}T{C}_N=\frac{1}{T+\lambda}\left[A+ PC+H{C}_N+ SC+ LC\right]=\left\{A+\frac{ vP}{R}{e}^{-R\;T}\left[{e}^{R\;\left(T-\beta \right)}-{e}^{-R\;\psi}\right]\right.\hfill \\ {}+P\left\{\frac{-{e}^{-R\left(T+\psi \right)}}{R^2}\left[b\left(T+2\psi +\frac{2}{R}+ R\psi \left(\psi +T\right)\right)+a\left(1+ R\psi \right)\right]+\frac{e^{- RT}}{R^2}\left[a+b\left(T+\frac{2}{R}\right)\right]\right\}\hfill \\ {}-D\left\{\frac{e^{- RT}}{R^2}\left[a+b\left(T+\frac{2}{R}\right)\right]-\frac{e^{-R\left(T+\lambda \right)}}{R^2}\left[a+b\left(T+\lambda +\frac{2}{R}\right)\right]-\frac{\lambda {e}^{-R\left(T+\psi \right)}}{R}\left[a+b\left(T+\psi +\frac{1}{R}\right)\right]\right\}\hfill \\ {}-{C}_3\left\{\frac{D{k}_0}{k{}_1}{e}^{-{k}_1\;\beta}\left[\frac{1}{R}\left(1-{e}^{-R\;\beta}\right)+\frac{1}{k_1-R}\left(1-{e}^{\left({k}_1-R\right)\beta}\right)\right]+\left(P-D\right)\left.\left[\frac{e^{-R\;\beta }}{R}\left(\beta -T\right)+\frac{1}{R^2}\left({e}^{-R\;\beta }-{e}^{-R\;T}\right)\right]\right\}\right.\hfill \\ {}\left.+{C}_4D\left[\frac{k_0}{k_1-R}\left({e}^{-{k}_1\;\beta }-{e}^{-R\;\beta}\right)+\frac{1}{R}\left(1-{e}^{-R\;\beta}\right)\right]\right\}/\left(T+\lambda \right)\hfill \end{array} $$
(26)

7 Numerical example

Consider an inventory system with the following data: β = 10; A C  = 50; γ = 0.01; x = 200; y = 0.6; L = 1500; c = 0.76; d = 1.5; C rw  = 45; K = 0.01; n = 1.18; A = 100; a = 3; b = 0.8; σ = 0.2; k 0 = 0.9; k 1 = 0.2; R = 0.1; C 3 = 4; C 4 = 10 in appropriate units.

Then we get the optimal values as T ∗ = 18.7678, ψ ∗ = 1.4946, λ ∗ = 2.0374, P ∗ = 144.4282, Q ∗ = 1482.1822, S ∗ = 57.3526 and TC ∗ = 2887.2717 in appropriate units.

For non-perishable product, the optimal values of Q ∗ and TC ∗ are Q ∗ = 337.9287 and TC ∗ = 3147.3193.

The result shows that the total cost of the system with perishable product is less than the total cost of the system without perishable product.

Figure 2 shows that the total cost TC decreases with λ and it attains the minimum value 2887.2717 at λ = 2.0374. If λ crosses 2.0374, the total cost then increases. The graph (Fig. 2) shows that the function TC is convex with respect λ.

Fig. 2
figure 2

The convexity of total cost function with respect to λ

Full size image

From Fig. 3, it is observed that the total cost TC decreases with respect to λ and ψ and TC attains the minimum value 2887.2717 at λ = 2.0374 and ψ = 1.4946 respectively. After that the total cost will be increased. The graph (Fig. 3) shows that the convexity of the total cost function with respect to λ and ψ.

Fig. 3
figure 3

The total cost with respect to λ and ψ

Full size image

8 Sensitivity analysis

We now study the effects of changes in the values of the system parameters β, A, σ, a, b, k 0, k 1, C 3, C 4, L, C rw and n on the optimal replenishment policy of the Example 1. We change one parameter at a time keeping the other parameters unchanged. The results are summarized in Table 1.

Table 1 Sensitivity analysis for various inventory parameters
Full size table

Based on our numerical results, we obtain the following managerial phenomena:

  1. 1.

    When the labour charge L is increasing, the production rate (P), unit production cost (v), unit selling price (s), duration of an inventory cycle when there is positive inventory (λ), optimal order quantity (Q) and the total optimal cost (TC) are also increasing. That is, the increasing of labour charge will increase the total cost of the retailer. In order to minimize the cost, the retailers should decrease the labour charge.

  2. 2.

    When the raw material cost C rw is increasing, there is no change in the production rate (P). But the increasing of raw material cost C rw will increase the unit production cost (v), unit selling price (s), duration of an inventory cycle when there is positive inventory (λ), optimal order quantity (Q) and the total optimal cost (TC). That is, if the retailer minimize the raw material cost, then the total cost will be reduced.

  3. 3.

    When the mark-up value n is increasing, there are no changes in the production rate (P) and the unit production cost (v). But the unit selling price (s), duration of an inventory cycle when there is positive inventory (λ), optimal order quantity (Q) and the total optimal cost (TC) will increase as n increase. That is, the increasing of selling price will increase the order quantity. So the total cost of the retailer will increase.

  4. 4.

    If the interim time span β increases, then the duration of an inventory cycle when there is positive inventory (λ) and optimal order quantity (Q) are increases. But the total optimal cost (TC) will decrease. That is, to minimize the cost, the retailer should increase the shortage period.

  5. 5.

    When the setup cost A is increasing, the order quantity (Q) and total optimal cost (TC) are also increasing. That is, minimum setup cost will minimize the total cost of the retailer.

  6. 6.

    When the parameters a and b are increasing, the total optimal cost (TC) is also increasing. That is, the minimum cost of holding the items will minimize the total cost of the retailer.

  7. 7.

    When the deterioration rate σ is increasing, there are no changes in the production rate (P), unit production cost (v) and the unit selling price (s). But the duration of an inventory cycle when there is positive inventory (λ), optimal order quantity (Q) and the total optimal cost (TC) will decrease. That is, the increase in σ will decrease the order quantity, therefore the total cost of the retailer will be minimized.

  8. 8.

    When the parameters k 0 and k 1 are increasing, the duration of an inventory cycle when there is positive inventory (λ), optimal order quantity (Q) and the total optimal cost (TC) are also increasing. That is, the minimum backlogging rate of the items will minimize the total cost of the retailer.

  9. 9.

    If the shortage cost (C3) and the lost sales cost (C4) are increasing, then the optimal order quantity (Q) and the total optimal cost (TC) of the retailer also increasing. But there are no changes in the production rate (P), unit production cost (v) and the unit selling price (s).

9 Conclusion

In this paper, the economic production lot size model for determining the optimal production length and the optimal order quantity for perishable items are developed. Holding cost is expressed as linearly increasing functions of time in this model. This model is very practical for the industries in which the holding cost is depending upon the time. In the present situation, Inflation and time value of money are also main factors. In keeping with this reality, these factors are incorporated in the present model. We also developed the model incorporating both marketing decision and variable unit price depending on the rate of production. When a product is perishable, the manufacturer may need to backlog demand in order to avoid high cost due to deterioration. So, shortage is allowed and can be partially backlogged, where the backlogging rate is dependent on the waiting time of the customer. Moreover, we considered SFI (shortage followed by inventory) policy. That is shortage occurs before the starting of inventory. The model is extended to the case of non-perishable product also. The result shows that the total cost of the model will minimum when the items are defective. Furthermore, numerical examples are provided to illustrate the model and the solution procedure. Finally, sensitivity analysis is carried out with respect to the key parameters and useful managerial insights are obtained.

The proposed model can be adopted in inventory control of production system such as automobile, semiconductor, cell phone, food industries, shoe manufacturing company, seasonable clothes, domestic goods etc..