Introduction

The strength of brittle solids such as rocks is dominantly reduced due to the presence of pre-existing cracks which may reduce the mixed mode (mode I and mode II) stress intensity factors near the crack ends where the stress and strain fields may tend to be infinite (becomes singular from a mathematical point of view) (Kato and Nishioka 2005). The mechanical behavior of brittle materials may be affected by the micromechanical behaviors of the cracks. The extension of cracks usually depends on the properties of cracks such as size, location, orientation, and loading conditions. Therefore, the initiation, propagation, and coalescence of cracks may play a vital role in predicting the cyclic breaking process of rock specimens (Wong and Li 2013).

Two types of cracks may usually be observed in the crack propagation process of the brittle materials such as pre-cracked rock specimens. These cracks include the wing cracks and secondary cracks which originate from tips of the pre-existing cracks. Wing cracks are the primarily initiated cracks which are usually produced and extended due to tension, while the secondary cracks (including both coplanar and oblique secondary cracks) are the laterally produced cracks which may initiate due to shear and propagation by a combination of shear and tension for the case of compressive loading. Therefore, initiation and propagation of wing cracks in rocks are favored relative to secondary cracks because of the lower fracture toughness of these materials in tension than in shear (Mohtarami et al. 2014, Haeri et al. 2014a, b, c, d, e). Practically, the pre-existing cracks in rocks are normally under compressive loading rather than under tension, shear, or mixed mode loading (Funatsu et al. 2014). Therefore, it is mainly expected that the crack initiation will approximately follow parallel to the direction of applied compressive loading (Hoek and Bieniawski 1965).

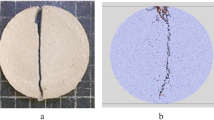
Recently, Haeri et al. (2014d) have experimentally observed that the wing cracks are produced at the first stage of loading and start their propagation toward the direction of compressive line loading in the pre-cracked Brazilian discs. They showed that the development and coalescence of wing cracks in the bridge area (i.e., the area in between the two pre-existing cracks) may be the main cause of the breaking process of rock-like disc specimens (Fig. 1).

Fig. 1
figure 1

Development and coalescence of wing cracks in rock-like disc specimen under compressive line loading (Haeri et al. 2014d)

Full size image

Initiation, propagation, and coalescence of the pre-existing cracks in specimens made of various materials, including natural rocks or rock-like materials under tensile and compressive loadings, are studied in fracture mechanics literature (Ingraffea 1985; Horii and Nemat-Nasser 1985; Huang et al. 1990; Shen et al. 1995; Chen and Hong 1996; Chen and Wong. 1997; Shou 1999; Wong and Chau 1998; Hong and Chen 1988a, b; Bobet and Einstein 1998a; Chen and Hong 1999; Wong et al. 2001; Sahouryeh et al. 2002; Li, et al. 2005; Park and Bobet 2006; Shou 2006; Park 2008; Yang et al. 2009; Park and Bobet 2009; Park and Bobet 2010; Janeiro and Einstein 2010; Yang 2011; Lee and Jeon 2011; Cheng-zhi and Ping 2012; Haeri et al. 2013a, 2014a, d). One of the most suitable tests for studying the fracture mechanics of brittle materials is the Brazilian disc test (Ayatollahi and Aliha 2008; Wang 2010; Dai et al. 2010; Haeri et al. 2014b, c, d). These tests are mostly carried out to evaluate the static and dynamic fracture toughness and stress intensity factors of rocks and rock-like specimens containing central pre-existing crack or cracks. These tests may also be used to study the crack initiation, propagation, and crack coalescence of brittle rocks (Dai et al. 2011; Ayatollahi and Sistaninia 2011; Wang et al. 2011, 2012; Ghazvinian et al. 2013). This testing procedure can be used to measure the tensile strength, fracture toughness, and mixed mode stress intensity factor of the un-cracked and pre-cracked disc specimens of various brittle materials under compressive line loadings (Awaji and Sato 1978; Sanchez 1979; Atkinson 1982; Shetty et al. 1986; Krishnan et al. 1998; Khan and Al-Shayea 2000; Al-Shayea et al. 2000; Al-Shayea 2005). In the Brazilian disc specimens, crack initiation and breakage process of the rock-like specimens may happen very soon due to the low tensile strength of rock-like materials.

Fig. 2
figure 2

A typical rock-like Brazilian disc specimen

Full size image

Ghazvinian et al. (2013) carried out some analytical, experimental, and numerical studies for a better understanding of crack propagation process in the CSCBD specimens. The effects of crack inclination angle and crack length on the fracturing processes of brittle materials have also been confirmed in the existing experimental and numerical analyses. Haeri et al. (2014d) studied the crack propagation and crack coalescence in the bridge area (the area in between the two cracks in the specimens containing two random cracks) of pre-cracked rock-like disc specimens.

Finite element method (FEM), boundary element method (BEM), and discrete element method (DEM) are usually used for the simulation of crack propagation in brittle solids. The most important fracture initiation criteria are as follows: (i) the maximum tangential stress (σ θ criterion) (Erdogan and Sih 1963), (ii) the maximum energy release rate (G criterion) (Hussian et al. 1974), and (iii) the minimum energy density criterion (S criterion) (Sih 1974). Some modified form of these criteria such as F criterion which is a modified form of energy release rate criterion proposed by Shen and Stephansson (1994) may also be used to study the breakage behavior of brittle substances (Marji et al. 2006; Marji and Dehghani 2010; Marji 2013; Haeri et al. 2014e). Based on these criteria, some computer codes were used to model the breakage mechanism of brittle materials such as rocks, for example, FROCK code (Park 2008), Rock Failure Process Analysis (RFPA2D) code (Wong 2002), and 2D Particle Flow Code (PFC2D) (Lee and Jeon 2011; Ghazvinian et al. 2013; Manouchehrian et al. 2014). In the previous researches, a few center cracks have been considered in the Brazilian disc specimens because it is usually difficult to produce specimens with multiple cracks in the laboratory.

In this investigation, multiple cracks in the central part of the Brazilian discs prepared from rock-like materials (prepared from Portland Pozzolana cement (PPC), fine sands, and water) are being analyzed both experimentally and numerically. The multiple center cracks in the disc specimens are developed to be parallel with each other and tested in a Brazilian Testing Apparatus. The breakage loads, the crack propagation, and crack coalescence through the specimens in the bridge area (the areas in between the parallel multiple cracks) have been studied.

It is tried to simulate the experiments by a modified higher order displacement discontinuity method specially developed to study the crack propagation and crack coalescence in the bridge area based on mode I and mode II stress intensity factors (SIFs). This method is basically a special version of the dual boundary element method (DBEM) originally proposed by Hong and Chen (1998a, b) and Chen and Hong (1999).

These results are compared and it has been shown that there is a good agreement between the experimental and numerical results which demonstrates the accuracy and validity of the present work’s analyses. The necessary flexibility in the analysis can be achieved by using the numerical method so that it is readily possible to investigate the effects of bridge area and orientation of cracks on the breakage process of pre-cracked disc specimens with multiple parallel cracks.

Method of specimen’s preparing and testing

A proper mixture of Portland Pozzolana cement (PPC), fine sands, and water are used to produce the pre-cracked rock-like disc specimens having 100 mm in diameter and 27 mm in thickness. Table 1 gives the mechanical properties of the prepared rock-like specimens tested in the rock mechanics laboratory before inserting the cracks.

Table 1 Ingredient ratios (%) and mechanical properties of the rock-like specimens
Full size table

The tensile strength (σ t) for un-cracked rock-like disc specimens is as follows:

$$ {\sigma}_{\mathrm{t}}=\frac{2 F}{\pi BR} $$
(1)

where F is the applied compressive load in KN, B is thickness of the disc specimen, and R is radius of the disc specimen.

Various Brazilian tests were conducted on rock-like disc specimens containing either a single center crack or two to five parallel cracks. The parallel center cracks are specially provided in a center line where the compressive line loading is going to be applied during the test. These cracks are created by inserting thin metal shims with a 20-mm width and 1-mm thickness into the specimens (during the specimens casting in the mold) as shown in Fig. 2.

Fig. 3
figure 3

Geometry of five rock-like disc specimens containing a single center crack, b two parallel center cracks, c three parallel cracks, d four parallel cracks, and e five parallel cracks

Full size image

The reproducibility of the test results has been checked by preparing several Brazilian disc specimens of rock-like materials (with the same crack geometry) and testing them in the laboratory. The Brazilian disc specimens may have a single, two, three, four, or five parallel cracks with inclination angles of 45° (β = 45°). Figure 3 illustrates the Brazilian disc specimens with multiple cracks which are prepared in such a manner that the direction of cracks is kept parallel (in a counterclockwise direction). In the compressive line loading, F was applied and the loading rate was kept at 0.5 MPa/s during the tests. All these cracks have equal lengths, 2b = 20 mm, and the ratio of half crack length, b, to the specimen radius, R, is taken as 0.1 (b/R = 0.1). The inclination angle of all cracks is 45°.

Fig. 4
figure 4

the normalized breaking load versus different multiple cracks in the cracked disc specimens

Full size image

In this research, three specimens were prepared for each experimental work, and as a whole, 15 cracked specimens (Brazilian discs with parallel center cracks) were prepared. The cracked disc specimens (containing one, two, three, four, and five parallel cracks) were also prepared at the center line of each specimen with the spacing S = 20 mm as shown in Fig. 3 (the spacing (S) is taken as the vertical distance between the centers of two cracks expressed in mm).

Fig. 5
figure 5

Experimental results illustrating the fracturing path of rock-like disc specimens containing a single crack, b two parallel cracks, c three parallel cracks, d four parallel cracks, and e five parallel cracks with a constant spacing, S = 20 mm

Full size image

Experimental tests and results

The rock-like Brazilian disc specimens were tested experimentally and the results were used to analyze the breakage loads and the crack propagation process of the pre-cracked disc specimens. The crack propagation process of the disc specimens are discussed considering the five cases of disc specimens with the following: (i) single crack, (ii) two parallel cracks, (iii) three parallel cracks, (iv) four parallel cracks, and (v) five parallel cracks, respectively.

Failure analysis of the pre-cracked disc specimens

The pre-cracked rock-like disc specimens have a lower strength compared to the un-cracked specimens (specimens having no cracks). Analyzing the breaking load of the pre-cracked disc specimens containing either one crack and two to five parallel cracks with the same orientations (β = 45°) is of paramount important to study the behavior of the brittle materials. The final breaking load of the pre-cracked disc specimens is normalized by the average breaking load of the un-cracked specimens. The average breaking load (strength) of un-cracked specimens is about 18,000 N. The normalized breaking load for the five cases shown in Fig. 4 (disc specimens containing one crack or two to five parallel cracks) is usually less than one (1) because the pre-existing crack decreases the final strength of specimen (Fig. 4). The final breaking loads of the pre-cracked specimens at different stages of crack propagation process are decreasing for disc specimens containing one crack to five cracks (i.e., with increasing cracks, the final breaking load is decreased) given in Fig. 4. The variation of normalized breaking load for these five cases is explained in this figure.

Crack propagation process of pre-cracked disc specimens with multiple cracks

The experimental investigation of pre-cracked rock-like specimens is accomplished considering the five cases: (i) specimen containing a single crack, (ii) specimens containing two parallel cracks, (iii) specimens containing three parallel cracks, (iv) specimens containing four parallel cracks, and (v) specimens containing five parallel cracks. These experiments have been established to study the mechanism of crack initiation and crack propagation emanating from pre-cracked specimens containing multiple cracks with the same inclination angle (β = 45°). In the case of single-cracked disc specimen, the wing cracks propagated in a curved path and continue their growth in a direction (approximately) parallel to the direction of maximum compressive load, as shown in Fig. 5a. These wing cracks are initiated at the tips of the pre-existing cracks. Crack coalescence phenomenon may occur when the two pre-existing cracks combine due to propagation of wing and/or secondary cracks in brittle materials under compressive loadings. As shown in Fig. 5, the crack coalescence in the bridge area may also occur during the crack propagation process. In the current experiments, the wing cracks are instantaneously initiated quasi-statically (Fig. 5). The development and coalescence of wing cracks in the bridge area (i.e., the area in between the two pre-existing cracks) may be the main reason for the extensions fracturing paths in rock-like disc specimens. In double cracked specimen, the bridge area may be considered as the area starting from the right tip of the crack 2 to that of the middle of an inclined crack (crack 1) as shown in Fig. 5b (specimen containing two parallel cracks). For the case shown in Fig. 5c (specimen containing three parallel cracks), the crack may or may not propagate from the tips of crack 2 that is the specimen may break away due to crack propagation process starting from the tips of crack 1 and crack 3 (i.e., no coalescence might occur at the tips of cracks). In the four cracked specimen (shown in Fig. 5d), the cracks initiated at the tips of the inclined cracks (crack 1, crack 4) and right tip of crack 3 and then the propagating cracks from the left tip of crack 1, right tip of crack 3, and right tip of crack 4 coalesced to that of the middle of inclined cracks 2 and 3, and no coalescences occurred at the tips of the cracks. Finally, for the case shown in Fig. 5e (for disc specimen containing five parallel cracks), the cracks may start to initiate at all right tips of inclined cracks (cracks 1, 2, 3, 4, and 5) and may not propagate from the left tips of cracks 2, 3, 4, and 5. In all of these fracturing cases, the propagated cracks (wing cracks) from pre-existing cracks may not coalesce at the wing crack tips of other cracks and the specimen may only fail due to the propagation of some inclined cracks to that of the middle of inclined cracks.

Indirect boundary element methods

The broad boundary element method (BEM) in solid mechanics is divided into two main categories: indirect and direct boundary element methods. The indirect boundary element method is divided into two main groups: (i) fictitious stress method (FSM) which is based on the fictitious stresses along a straight line crack element and (ii) displacement discontinuity method (DDM) which is based on the displacement differences on the negative and positive sides of a straight crack element (Crouch and Starfield 1983). A displacement discontinuity-based version of the indirect boundary element method known as higher order displacement discontinuity method (HDDM) that is a special version of dual boundary element method (DBEM) originally proposed by Hong and Chen (1998a) is modified for the crack analysis of brittle solids (Crouch 1967; Marji 1997, Haeri et al. 2013a). In order to obtain more accurate numerical results, cubic collocation displacement discontinuity method is modified for the solution of elasto-static problems in this study to simulate the pre-cracked Brazilian disc specimens (Guo et al. 1990; Scavia 1990; Aliabadi and Rooke 1991; Shou 1999, 2000a, b, 2006; Marji et al. 2006; Haeri et al. 2013b). Finally, a two-dimensional higher order displacement discontinuity computer program using three special crack tip elements is proposed for the analysis of rock fracture mechanics problems.

Higher order displacement discontinuity method (HDDM)

Let consider the cubic variation of the displacement discontinuity function, D k (ε), as shown in Fig. 6. The function, D k (ε), gives the variation of displacement discontinuities along a line crack and can be used to calculate two fundamental variables of each element (the opening displacement discontinuity D y and sliding displacement discontinuity D x ) (Fig. 6).

Fig. 6
figure 6

Cubic shape function showing the variation of higher order displacement discontinuities along an ordinary boundary element

Full size image
Fig. 7
figure 7

Special crack tip element with threeequal sub-elements

Full size image
$$ {D}_k\left(\varepsilon \kern0.24em \right)={\displaystyle \sum_{i=1}^4{\varPi}_i\left(\varepsilon \right){D}_k^i},\kern1.25em k= x, y $$
(2)

where D 1 k (i. e., D 1 x and D 1 y ), D 2 k (i. e., D 2 x and D 2 y ), D 3 k (i. e., D 3 x and D 3 y ), and D 4 k (i. e., D 4 x and D 4 y ) are the cubic nodal displacement discontinuities and

$$ \begin{array}{l}{\prod}_1\left(\varepsilon \right)=-\left(3{a}_1^3-{a}_1^2\varepsilon -3{a}_1{\varepsilon}^2+{\varepsilon}^3\right)/\left(48{a}_1^3\right),\\ {}{\varPi}_2\left(\varepsilon \right)=\left(9{a}_1^3-9{a}_1^2\varepsilon -{a}_1{\varepsilon}^2-{\varepsilon}^3\right)/\left(16{a}_1^3\right),\\ {}{\varPi}_3\left(\varepsilon \right)=\left(9{a}_1^3+9{a}_1^2\varepsilon -{a}_1{\varepsilon}^2-{\varepsilon}^3\right)/\left(16{a}_1^3\right),\mathrm{and}\\ {}{\varPi}_4\left(\varepsilon \right)=-\left(3{a}_1^3+{a}_1^2\varepsilon -3{a}_1{\varepsilon}^2-{\varepsilon}^3\right)/\left(48{a}_1^3\right)\end{array} $$
(3)

are the cubic collocation shape functions using a 1 = a 2 = a 3 = a 4. As shown in Fig. 6, a cubic displacement discontinuity (DD) element is divided into fourequal sub-elements (each sub-element contains a central node for which the nodal displacement discontinuities are evaluated numerically).

The potential functions f (x, y) and g (x, y) for the cubic case can be found from the following:

$$ \begin{array}{l} f\left( x, y\right)=\frac{-1}{4\pi \kern0.24em \left(1-\nu \kern0.24em \right)}{\displaystyle \sum_{i=1}^4{D}_x^i{F}_i\left({I}_0,{I}_1,{I}_2\right)\kern0.24em }\\ {} g\left( x, y\right)=\frac{-1}{4\pi \kern0.24em \left(1-\nu \kern0.24em \right)}{\displaystyle \sum_{i=1}^4{D}_y^i{F}_i\left({I}_0,{I}_1,{I}_2\right)\kern0.24em }\end{array} $$
(4)

in which the common function F i is defined as follows:

$$ {F}_i\left({I}_0,{I}_1,{I}_2,{I}_3\right)={{\displaystyle \int {\varPi}_i\left(\varepsilon \right) \ln \left[\left( x-\varepsilon \right)+{y}^2\right]}}^{\frac{1}{2}} d\varepsilon, \kern1.92em I = 1\ \mathrm{to}\ 4 $$
(5)

where the integrals I 0, I 1, I 2, and I 3 are expressed as follows:

$$ \begin{array}{l}{I}_0\left( x, y\right)={{\displaystyle \int \ln \left[{\left( x-\varepsilon \right)}^2+{y}^2\right]}}^{\frac{1}{2}} d\varepsilon, \\ {}{I}_1\left( x, y\right)={{\displaystyle \underset{- a}{\overset{a}{\int }}\varepsilon \kern0.36em \ln \left[{\left( x-\varepsilon \kern0.24em \right)}^2+{y}^2\right]}}^{\frac{1}{2}} d\varepsilon, \\ {}{I}_2\left( x, y\right)={{\displaystyle \underset{- a}{\overset{a}{\int }}{\varepsilon}^2 \ln \left[{\left( x-\varepsilon \right)}^2+{y}^2\right]}}^{\frac{1}{2}} d\varepsilon, \mathrm{and}\\ {}{I}_3\left( x, y\right)={{\displaystyle \underset{- a}{\overset{a}{\int }}{\varepsilon}^3 \ln \left[{\left( x-\varepsilon \right)}^2+{y}^2\right]}}^{\frac{1}{2}} d\varepsilon \end{array} $$
(6)

The singularities of the stresses and displacements near the crack ends may reduce their accuracies; special crack tip elements can be effectively used to increase the accuracy of the DDs near the crack tips (Marji et al. 2006). As shown in Fig. 7, the DD variations for three nodes can be formulated using a special crack tip element containing three nodes (or having three special crack tip sub-elements).

$$ {D}_k\left(\varepsilon \right)=\left[{\varGamma}_{C1}\left(\varepsilon \right)\right]{D}_k^1(a)+\left[{\varGamma}_{C2}\left(\varepsilon \right)\right]{D}_k^2(a)+\left[{\varGamma}_{C3}\left(\varepsilon \right)\right]{D}_k^3(a),\kern0.84em k= x, y $$
(7)

where each crack tip element has a length a 1 = a 2 = a 3 = a 4. Considering a crack tip element with the three equal sub-elements (a 1 = a 2 = a 3), the shape functions Γ C1(ε), Γ C2(ε), and Γ C3(ε) can be obtained as follows:

$$ \begin{array}{l}{\varGamma}_{C1}\left(\varepsilon \right)=\frac{15{\varepsilon}^{\frac{1}{2}}}{8{a}_1^{\frac{1}{2}}}-\frac{\varepsilon^{\frac{3}{2}}}{a_1^{\frac{3}{2}}}+\frac{\varepsilon^{\frac{5}{2}}}{8{a}_1^{\frac{5}{2}}},\\ {}{\varGamma}_{C2}\left(\varepsilon \right)=\frac{-5{\varepsilon}^{\frac{1}{2}}}{8{a}_1^{\frac{1}{2}}}+\frac{3{\varepsilon}^{\frac{3}{2}}}{2\sqrt{3}{a}_1^{\frac{3}{2}}}-\frac{\varepsilon^{\frac{5}{2}}}{4\sqrt{3}{a}_1^{\frac{5}{2}}},\mathrm{and}\\ {}{\varGamma}_{C3}\left(\varepsilon \right)=\frac{3{\varepsilon}^{\frac{1}{2}}}{8\sqrt{5}{a}_1^{\frac{1}{2}}}-\frac{\varepsilon^{\frac{3}{2}}}{2\sqrt{5}{a}_1^{\frac{3}{2}}}+\frac{\delta^{\frac{5}{2}}}{8\sqrt{5}{a}_1^{\frac{5}{2}}}\end{array} $$
(8)
$$ {F}_C\left( x, y\right)=\frac{-1}{4\pi \kern0.24em \left(1-\nu\;\right)}{\displaystyle \underset{- a}{\overset{a}{\int }}{D}_k\left(\varepsilon \right) \ln {\left[{\left( x-\varepsilon \right)}^2+{y}^2\right]}^{\frac{1}{2}} d\varepsilon, \kern0.5em k= x, y} $$
(9)

Inserting the common displacement discontinuity function D k (ε) (Eq. (7)) in Eq. (9) gives the following:

$$ \begin{array}{l}{F}_C\left( x, y\right)=\frac{-1}{4\pi \kern0.24em \left(1-\nu \right)}\;\left\{\right[{\displaystyle \underset{- a}{\overset{a}{\int }}{\varGamma}_{C1}\left(\varepsilon \right) \ln {\left[{\left( x-\varepsilon \right)}^2+{y}^2\right]}^{\frac{1}{2}} d\varepsilon \Big]{D}_k^1+}\\ {}\left[{\displaystyle \underset{- a}{\overset{a}{\int }}{\varGamma}_{C2}\left(\varepsilon \right) \ln }{\left[{\left( x-\varepsilon \right)}^2+{y}^2\right]}^{\frac{1}{2}} d\varepsilon \right]{D}_k^2\kern0.36em +\left[{\displaystyle \underset{- a}{\overset{a}{\int }}{\varGamma}_{C3}\left(\varepsilon \right) \ln }{\left[{\left( x-\varepsilon \right)}^2+{y}^2\right]}^{\frac{1}{2}} d\varepsilon \right]{D}_k^3, k= x, y\;\end{array} $$
(10)

Inserting the shape functions Γ C1(δ)ε, Γ C2(ε), and Γ C3(ε) in Eq. (10) after some manipulations and rearrangements the following three special integrals are deduced:

$$ \begin{array}{l}{I}_{C1}\left( x, y\right)={\displaystyle \underset{- a}{\overset{a}{\int }}{\varepsilon}^{\frac{1}{2}} \ln {\left[{\left( x-\varepsilon \right)}^2+{y}^2\right]}^{\frac{1}{2}} d\varepsilon},\kern0.5em \\ {}{I}_{C2}\left( x, y\right)={\displaystyle \underset{- a}{\overset{a}{\int }}{\varepsilon}^{\frac{3}{2}} \ln {\left[{\left( x-\varepsilon \right)}^2+{y}^2\right]}^{\frac{1}{2}} d\varepsilon, \mathrm{and}}\\ {}{I}_{C3}\left( x, y\right)={\displaystyle \underset{- a}{\overset{a}{\int }}{\varepsilon}^{\frac{5}{2}} \ln {\left[{\left( x-\varepsilon \right)}^2+{y}^2\right]}^{\frac{1}{2}} d\varepsilon}\end{array} $$
(11)

Based on the linear elastic fracture mechanics (LEFM) principles, the mode I and mode II stress intensity factors K I and K II (expressed in MPa m1/2) can be written in terms of the normal and shear displacement discontinuities (Shou and Crouch 1995; Shou 1997a, b) obtained for the last special crack tip element as follows:

$$ {K}_I=\frac{\mu}{4\left(1-\nu \kern0.24em \right)}{\left(\frac{2\pi}{a_1}\right)}^{\frac{1}{2}}{D}_y\left({a}_1\right)\kern1em \mathrm{and}\kern1.25em {K}_{I I}=\frac{\mu}{4\left(1-\nu \kern0.24em \right)}{\left(\frac{2\pi}{a_1}\right)}^{\frac{1}{2}}{D}_x\left({a}_1\right) $$
(12)

Numerical simulation of the pre-cracked specimens by HDDM

The pre-cracked Brazilian disc specimens (prepared from rock-like materials) under compressive line loading can also be simulated numerically by the higher order displacement discontinuity method (HDDM). Therefore, HDDM is used for the simulation of the experimental works already shown in Fig. 5. The numerically simulated discs are graphically shown in Fig. 8 for comparison. The linear elastic fracture mechanics (LEFM) approach (based on the concept of mode I and mode II stress intensity factors (SIFs) proposed by Irwin (1957)) is implemented in the HDDM code and the maximum tangential stress criterion given by Erdogan and Sih (1963) is used in a stepwise procedure to estimate the propagation paths of the propagating wing cracks. The simulated propagation paths are in good agreement with the corresponding experimental results as can be observed by comparing Fig. 5 with Fig. 8.

Fig. 8
figure 8

Numerical simulation of the crack propagation path for pre-cracked Brazilian disc specimens containing a single crack, b two parallel cracks, c three parallel cracks, d four parallel cracks, and e five parallel cracks with a constant spacing, S = 20 mm

Full size image

The effect of bridge area on breakage paths

Since the experimental analysis of the crack propagation process of disc specimens is somewhat time-consuming, expensive, difficult, and complex, in this study, the numerical simulations of crack propagation process are also accomplished by using the boundary element code, HDDM.

As experimentally shown in the previous section, the number of parallel cracks in rock-like specimens has a significant effect on their final fracturing process. Assessment of breaking process in pre-cracked disc specimens with different spacing is considered here. The numerical simulations are accomplished on pre-cracked disc specimens with constant diameters, D = 100, equal parallel cracks of lengths, 2b = 10 mm.

It should be noted that in the experimental work, the inclination angle of cracks, β, and the spacing, S, in the last molding were 45° and 20 mm, respectively. Figures 9, 10, 11, and 12 present the results of the numerical simulation for disc specimens containing two and three parallel cracks (i.e., at spacing, S, equal to 20, 30, and 40 mm) considering the inclination angle of cracks (β = 30° and 45°) and the equal crack lengths 2b = 10 mm (the ratio, b/D = 0.05).

Fig. 9
figure 9

Numerical simulation of the crack propagation path for Brazilian disc specimens containing two parallel cracks (the inclination angle of cracks, β = 30°) for different spacing: a S = 20 mm, b S = 30 mm, and c S = 40 mm

Full size image
Fig. 10
figure 10

Numerical simulation of the crack propagation path for Brazilian disc specimens containing two parallel cracks (the inclination angle of crack, β = 45°) for different spacing: a S = 20 mm, b S = 30 mm, and c S = 40 mm

Full size image
Fig. 11
figure 11

Numerical simulation of the crack propagation path for Brazilian disc specimens containing three parallel cracks (the inclination angle of crack, β = 30°) for different spacing: a S = 20 mm, b S = 30 mm, and c S = 40 mm

Full size image
Fig. 12
figure 12

Numerical simulation of the crack propagation path for Brazilian disc specimens containing three parallel cracks (the inclination angle of crack, β = 45°) for different spacing: a S = 20 mm, b S = 30 mm, and c S = 40 mm

Full size image

It may be concluded that the final breakage paths of the pre-cracked specimens may be affected by changing the spacing, S, and this can be easily seen by comparing Figs. 9, 10, 11, and 12, respectively.

Table 2 compares the numerical and experimental results considering the crack initiation loads. As shown in this table, the proposed numerical method gives very accurate results and can be effectively used for the crack analysis of pre-cracked Brazilian disc specimens.

Table 2 Comparison of wing crack initiation loads (using the proposed numerical method and the experiments works)
Full size table

Table 2 demonstrates that the proposed numerical method gives very accurate results for pre-cracked Brazilian disc specimens. Thus, this method may be considered as a suitable tool for the analysis of crack propagation and failure process in brittle materials.

In the specimens containing two parallel cracks with the spacings S = 30 and 40 mm, the cracks initiated at the tips of the inclined cracks (cracks 1 and 2) and then the cracks coalesced with each other at the propagating crack tips in the bridge area as shown in Figs. 9b, c and 10c, but for the cases shown in Fig. 9a and 10a, the cracks may start to initiate at the tips of inclined crack (crack 2) first and then the specimen may fail in the direction of the crack propagation paths originating from the tips of crack 2. For the case shown in Fig. 10b, the cracks may start to initiate at the tips of both cracks 1 and 2 and may fail due to the propagation of inclined cracks (crack 1 and crack 2) to that of the middle of inclined cracks.

In the specimens containing three parallel cracks with spacings S = 30 and 40 mm, the cracks initiated at the tips of the inclined cracks (crack 1, crack 2, and crack 3) and then the cracks may propagate toward each other and eventually the crack coalescence may occur in the bridge area (Fig. 11b, c and Fig. 12c), but for the cases shown in Fig. 11a and 12a, the cracks may start to initiate at the tips of inclined cracks (crack 1 and crack 3) first and then the specimen may fail in the direction of the crack propagation paths originating from the tips of crack 1 and crack 3. For the case shown in Fig. 12b, the cracks may start to initiate at the tips of both cracks 1 and 3 and may fail due to the propagation of inclined cracks (crack 1 and crack 3) to that of the middle of inclined cracks.

Conclusions

The mechanism of crack propagation in brittle solids has been studied by comprehensive experimental and numerical studies in the recent years. This mechanism is a complicated process and further research may be devoted to investigate the crack propagation, crack coalescence in the bridge area, and final breakage paths of the rocks and rock-like materials under compressive line loading. Brazilian disc-type specimens of rock-like material can be effectively used to accomplish these investigations.

In this research, multiple parallel cracks in the central part of the Brazilian discs are especially prepared from rock-like materials (prepared from PPC, fine sands, and water) and are being analyzed both experimentally and numerically. These multiple center cracks are produced so that they would be parallel with each other and tested in a Brazilian testing apparatus I a rock mechanics laboratory. The breaking loads, crack propagation, and crack coalescence through the specimens and in the bridge area (the areas in between the parallel multiple cracks) have been investigated. A modified higher order displacement discontinuity method, HDDM (which is a category of the broad boundary element method), is especially developed to simulate the mechanism of crack propagation and crack coalescence in the specimens and in the bridge areas of the parallel cracks. The linear elastic fracture mechanics (LEFM) theory based on mode I and mode II stress intensity factors (SIFs) is used in the numerical simulation. The experimental and numerical models well illustrate the production of the wing cracks and the crack propagation paths produced by the coalescence phenomenon of the multiple pre-existing parallel cracks in the bridge area. These experimental and numerical results are compared with each other and it has been shown that there is a good agreement between them which demonstrates the accuracy and validity of the present analyses. More flexibility in the analysis can be achieved by using the proposed numerical method so that it may be possible to investigate the effects of bridge area and orientation of cracks on the breakage process of pre-cracked disc specimens with multiple parallel cracks.