Thermodynamic Description of the Cu-Ni-Si System

Abstract

A thermodynamic description of the Cu-Ni-Si ternary system has been carried out by using the calculation of phase diagrams method. Among the three binary sub-systems, Cu-Ni, Cu-Si and Ni-Si, are taken from earlier assessments and the Cu-Ni-Si ternary system are optimized in this study by using the reported experimental data. Six isothermal sections and four vertical sections with the experimental data are presented, along with the liquidus projection and the reaction scheme. Comprehensive comparisons between the calculated and measured phase diagrams show that the latest experimental information can be satisfactorily accounted for by the present thermodynamic description.

Introduction

It is well known that copper-based alloys exhibit excellent electrical conductivity and improved mechanical properties. The Cu-Ni-Si alloys play an important role in the industry field for the application in lead frames and connectors.[1] Up to now, researchers have been concentrating on understanding processing and compositional optimization of these Corson-type alloys due to their excellent combination of high strength, conductivity and thermal stability.[2] Because of its broad potential application prospect, comprehensive information about the thermodynamic description of the Cu-Ni-Si ternary system is of great value.

Sun et al. investigated the isothermal section at 700 °C recently.[3] Afterwards, Liu et al.[4] established comprehensive isothermal sections in the system at 800, 900 and 1000 °C. The binary phase diagrams of the Cu-Ni,[5] Cu-Si[6] and Ni-Si[7] were calculated earlier. A thermodynamic description in the copper-rich corner of the Cu-Ni-Si ternary system has been published by Miettinen.[8] However, the set of parameters does not apply in the whole system considering the latest experimental data.[3,4] Thus a thermodynamic assessment of the Cu-Ni-Si ternary system is essential.

The present work aims at a critical evaluation of the Cu-Ni-Si ternary system using the CALPHAD method, as well as developing a set of self-consistent thermodynamic parameters for this ternary system.

Experimental Information

The Cu-Ni-Si ternary system was comprehensively studied by Okamoto,[9–11] several isothermal and vertical sections as well as the liquidus projection were reported. Review of the experimental determination of the ternary phase equilibria was given by Kumar et al.[12] However, according to his review, the data are questionable due to the differences of the homogeneity ranges of some phases.

Recently, Liu et al.[4] have investigated the system at 800, 900, 1000 °C, which provides sufficient data combined with Sun et al.’s[5] work at 700 °C for the present assessment. In their work, the γ (Cu₅₆Si₁₁), θ (Ni₂Si), γ′ (Ni₅Si₂) and δ (Ni₂Si) phases show a noticeable solubility of a third element in the ternary system. The γ (Cu₅₆Si₁₁) and θ (Ni₂Si) phases can be stabilized by substituting Ni and Cu respectively. The ternary compound τ₁ has previously observed by Okamoto[10] below 850 °C around Cu_60.9-66.3Ni_9.9-11.2Si_23.4-28.3, which has also been reported to be formed via the peritectic reaction, liquid + γ (Cu₅₆Si₁₁) + θ (Ni₂Si) ↔ τ₁, at 859 °C.[3,10] It was corroborated by the experimental data of electron probe microanalysis (EPMA) and x-ray diffraction (XRD): the γ (Cu₅₆Si₁₁) can dissolve a large amount of Ni up to 21.9 at.% mainly by substituting Ni for Cu at 700 °C. The solubility of Ni in the γ (Cu₅₆Si₁₁) phase varies from 5.67 to 21.37 at.% at 800 °C, and there still exists a homogeneous region with the solubility of Ni ranging from 16.09 to 22.71 at.% at 900 °C, however, not observed at 1000 °C. The τ₂ phase is only observed at 700 °C and the τ₁ disappears at 1000 °C. The present work takes Liu et al.[4] and Sun et al.’s experimental data for the assessment.

Thermodynamic Models

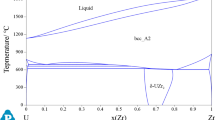
Figure 1 shows the calculated phase diagrams for binaries Cu-Ni,[5] Cu-Si[6] and Ni-Si.[7] They agree well with the experimental phase equilibrium data as presented in these studies.

Fig. 1

Binary phase diagrams constituting the Cu-Ni-Si ternary system

As there are many studies concerning about the optimization of the Ni-Si system, the optimization of the ternary Cu-Ni-Si system was begun with checking the three binary Ni-Si descriptions.[7,13,14] In both Lindholm[14] and Du[13]’s works, the fcc solubility was too high in regard to the experimental observations made by Okamoto.[9–11] In Tokunaga’s work,[7] the agreement was better through integrated into account. In this work, we treat the ordered β₁ (Ni₃Si) phase as a simple stoichiometric phase.

The phase equilibria of the Cu-Ni-Si system in the copper-rich corner above 700 °C have been reviewed by Miettinen.[8] Due to the numerous silicides of binaries Cu-Si and Ni-Si, the ternary system is quite complicated. The following phases and their phase equilibria are present: liquid, fcc, bcc, Cu₁₉Si₆ (η), Cu₅₆Si₁₁ (γ), Ni₅Si₂ (γ′), Ni₂Si (δ), Ni₂Si (θ), Ni₃Si_β₁, Ni₃Si_β₂, Ni₃Si_β₃, NiSi, NiSi₂ (α), τ₁ and τ₂. The disordered solution phases, liquid, fcc, and bcc are described with the substitutional solution model. The ternary γ (Cu₅₆Si₁₁) phase is a nonstoichiometric compound expanding from Cu₅₆Si₁₁ towards Ni₅₆Si₁₁ but it has a large homogeneity range. The γ phase was treated as a simple stoichiometric phase in the earlier Cu-Mn-Si[15] and Cu-Fe-Si[16] descriptions, which allows an easy extension of the phase in a multicomponent system. However, Novikov and Dautova[17] treated the γ phase as a ternary compound. In the present work, we also treat it as a ternary compound with the sublattice model (Cu,Ni)_0.62(Cu,Ni)_0.18(Si)_0.2, which gives a better agreement with the experimental data. The τ₁ and τ₂ phases are modeled as (Cu,Ni)_0.63(Ni)_0.1(Si)_0.27 and (Cu,Ni)_0.46(Ni)_0.25(Si)_0.29, respectively.

Liquid and Solid Solution Phases

By applying the substitutional solution model to the solution phases of the Cu-Ni-Si system, the molar Gibbs energy of the phases becomes

$$ G_{\text{m}}^{\upphi } = \sum\limits_{{i = \text{Cu,Ni,Si}}} {x_{i}^{0} } G_{i}^{\upphi } + RT\sum\limits_{{i = \text{Cu,Ni,Si}}} {x_{i} \ln x_{i}^{\upphi } } + {}^{\text{ex}}G^{\upphi } + {}^{\text{mag}}G^{\upphi } $$

(1)

where G ^ϕ _i is the molar Gibbs free energy of pure element i in the structure ϕ phase, and the term ^ex G ^ϕ is the excess free energy, which is expressed by the Redlich-Kister polynomials[18] as:

$$ {}^{\text{ex}}G^{\upphi } = x_{{\text{Cu}}} x_{{\text{Ni}}} L_{{\text{Cu,Ni}}}^{\upphi } + x_{{\text{Cu}}} x_{{\text{Si}}} L_{{\text{Cu},\text{Si}}}^{\upphi } + x_{{\text{Ni}}} x_{{\text{Si}}} L_{{\text{Ni},\text{Si}}}^{\upphi } + x_{{\text{Cu}}} x_{{\text{Ni}}} x_{{\text{Si}}} L_{{\text{Cu},\text{Ni},\text{Si}}}^{\upphi } $$

(2)

$$L{_{i,j}^{\upphi } = \sum\limits_{{n = 0}}^{m} {{}^{n}} L{_{{i,j}}^{\upphi}}} \left( {x_{i} - x_{j} } \right)^{n} $$

(3)

$$ L_{{\text{Cu,Ni,Si}}}^{\phi } = x_{{\text{Cu}}} {}^{0}L_{{\text{Cu,Ni,Si}}}^{\phi } + x_{{\text{Ni}}} {}^{1}L_{{\text{Cu,Ni,Si}}}^{\phi } + x_{{\text{Si}}} {}^{2}L_{{\text{Cu,Ni,Si}}}^{\phi } $$

(4)

The binary and the ternary interaction parameters ⁿ L ^ϕ _i,j and ⁿ L ^ϕ _i,j,k take the following form:

$$ {}^{n}L_{\text{m}}^{\upphi } = a + bT + cT^{2} , $$

(5)

where a, b and c are the coefficients to be optimized.

In the Cu-Ni-Si ternary system, there is a magnetic contribution to Gibbs free energy for the (Cu, Ni, Si) phase due to the present of Ni. The term ^mag G ^ϕ is expressed as follows.[19]

$$ {}^{\text{mag}}G^{\upphi } = RT\,{ \ln }\left( {\upbeta^{\upphi } + 1} \right)f\left( {\uptau^{\upphi } } \right),\quad \uptau^{\upphi } = T/T_{c}^{\upphi } , $$

(6)

where β^ϕ is a quantity related to the total magnetic entropy, which in most cases is set to Bohr magnetic moment per mole of atoms; f(τ^ϕ) is a polynomial function of τ, and T ^ϕ_c is the critical temperature for magnetic ordering, and f(τ^ϕ) represents the polynomials obtained by Hillert and Jarl[20] as follows:

$$ f\left( {\uptau^{\upphi } } \right) = 1 - \frac{1}{D}\left[ {\frac{{79\uptau^{ - 1} }}{140p} + \frac{474}{497}\left( {\frac{1}{p} - 1} \right)\left( {\frac{{\uptau^{3} }}{6} + \frac{{\uptau^{9} }}{135} + \frac{{\uptau^{15} }}{600}} \right)} \right]\quad {\text{for}}\quad \, \uptau \, \le { 1}, $$

(7)

$$ f\left( {\uptau^{\upphi } } \right) = - \frac{1}{D}\left( {\frac{{\uptau^{ - 5} }}{10} + \frac{{\uptau^{ - 15} }}{315} + \frac{{\uptau^{ - 25} }}{1500}} \right)\quad {\text{for }}\uptau \, > { 1}, $$

(8)

where D = (518/1125) + (11692 + 15975)((1/p) − 1) and p depends on the structure, 0.4 for the bcc structure and 0.28 for others.

The Binary Phases with Ternary Solubilities

Some phases in the Cu-Ni-Si system are formed from the terminal binary phases extending into the ternary system. The phases NiSi, NiSi₂ are extended from the binary phases NiSi, NiSi₂ respectively with partial Si substituted by Cu.

The Gibbs free energy of the phase ϕ $(\upphi = {\text{NiSi or }}\upalpha )$ with the sublattices (Ni)_a(Cu,Si)_c is expressed as follows:[21]

$$ \begin{aligned} G_{\text{m}}^{\upphi } & = y_{\text{Cu}}^{\text{II}} G_{\text{Ni:Cu}} + y_{\text{Si}}^{\text{II}} G_{\text{Ni:Si}} + cRT\left( {y_{\text{Cu}}^{\text{II}} \ln y_{\text{Cu}}^{\text{II}} + y_{\text{Si}}^{\text{II}} \ln y_{\text{Si}}^{\text{II}} } \right) \\ \quad + y_{\text{Cu}}^{\text{II}} y_{\text{Si}}^{\text{II}} \left[ {\sum\limits_{n} {{}^{n}L_{\text{Ni:Cu,Si}} \left( {y_{\text{Cu}}^{\text{II}} - y_{\text{Si}}^{\text{II}} } \right)^{n} } } \right] \\ \end{aligned} $$

(9)

The phases δ, γ′, β₁, β₂, are extended from the binary phases δ (Ni₂Si), γ′ (Ni₅Si₂), β₁ (Ni₃Si), β₂ (Ni₃Si) respectively with partial Ni substituted by Cu. The phase η is extended from the binary phase η (Cu₁₉Si₆) with partial Cu substituted by Ni.

The Gibbs free energy of the phase ϕ $(\upphi = \delta , \, \upgamma^{'} , \, \upbeta_{1} { , }\upbeta_{ 2} {\text{ or }}\upeta )$ with the sublattices (Ni,Cu)_a(Si)_c is expressed as follows:

$$ \begin{aligned} G_{\text{m}}^{\upphi } & = y_{\text{Ni}}^{\text{I}} G_{\text{Ni:Si}} + y_{\text{Cu}}^{\text{I}} G_{\text{Cu:Si}} + aRT\left( {y_{\text{Ni}}^{\text{I}} \ln y_{\text{Ni}}^{\text{I}} + y_{\text{Cu}}^{\text{I}} \ln y_{\text{Cu}}^{\text{I}} } \right) \\ \quad + y_{\text{Ni}}^{\text{I}} y_{\text{Cu}}^{\text{I}} \left[ {\sum\limits_{n} {{}^{n}L_{\text{Ni,Cu:Si}} \left( {y_{\text{Ni}}^{\text{I}} - y_{\text{Cu}}^{\text{I}} } \right)^{n} } } \right] \\ \end{aligned} $$

(10)

where $y_{ * }^{\text{I}}$ and $y_{ * }^{\text{II}}$ are the site fractions of a certain component located on sublattice I and II, respectively, and the parameters ⁰ G _m:n represents the Gibbs free energy of the compound phase when the two sublattices are occupied by element m or n. L _Ni:Cu,Si and L _Ni,Cu:Si are the interaction parameter between Cu and Si as well as Ni and Cu in the second or first sublattice, when the other sublattice is occupied by element Ni or Si. ⁰ G _m:n, L _Ni:Cu,Si and L _Ni,Cu:Si are evaluated in the present work.

Ternary Compounds

As mentioned above, the γ (Cu₅₆Si₁₁) phase was treated as a ternary compound and the θ phase was modeled as (Ni)(Cu,Ni,Va)(Si), the τ₁ and τ₂ phases were modeled as (Cu,Ni)_0.63(Ni)_0.1(Si)_0.27 and (Cu,Ni)_0.46(Ni)_0.25(Si)_0.29 respectively. The Gibbs free energy of the phase $\upphi \,(\upphi = \upgamma , \, \theta , \, \uptau_{1} {\text{ or }}\uptau_{2} )$ with the sublattices (A ₁, A ₂) _a (B ₁, B ₂) _b C is expressed as follows:

$$ \begin{aligned} G_{m}^{\upphi } & = y_{{A_{1} }}^{I} y_{{B_{1} }}^{II} G_{{A_{1} :B_{1} :C}} + y_{{A_{1} }}^{I} y_{{B_{2} }}^{II} G_{{A_{1} :B_{2} :C}} \\ \quad + y_{{A_{2} }}^{I} y_{{B_{1} }}^{II} G_{{A_{2} :B_{1} :C}} + y_{{A_{2} }}^{I} y_{{B_{2} }}^{II} G_{{A_{2} :B_{2} :C}} \\ \quad + aRT\left( {y_{{A_{1} }}^{I} \ln y_{{A_{1} }}^{I} + y_{{A_{2} }}^{I} \ln y_{{A_{2} }}^{I} } \right) + bRT\left( {y_{{B_{1} }}^{II} \ln y_{{B_{1} }}^{II} + y_{{B_{2} }}^{II} \ln y_{{B_{2} }}^{II} } \right) \\ \quad + y_{{A_{1} }}^{I} y_{{A_{2} }}^{I} \left[ {y_{{B_{1} }}^{II} \sum\limits_{n} {{}^{n}L_{{A_{1} ,A_{2} :B_{1} :C}} } \left( {y_{{A_{1} }}^{I} - y_{{A_{2} }}^{I} } \right) + y_{{B_{2} }}^{II} \sum\limits_{n} {{}^{n}L_{{A_{1} ,A_{2} :B_{2} :C}} } \left( {y_{{A_{1} }}^{I} - y_{{A_{2} }}^{I} } \right)} \right] \\ \quad + y_{{B_{1} }}^{II} y_{{B_{2} }}^{II} \left[ {y_{{A_{1} }}^{I} \sum\limits_{n} {{}^{n}L_{{A_{1} :B_{1} ,B_{2} :C}} } \left( {y_{{B_{1} }}^{I} - y_{{B_{2} }}^{I} } \right) + y_{{A_{2} }}^{I} \sum\limits_{n} {{}^{n}L_{{A_{2} :B_{1} ,B_{2} :C}} } \left( {y_{{B_{1} }}^{I} - y_{{B_{2} }}^{I} } \right)} \right] \\ \end{aligned} $$

(11)

where $y_{ * }^{\text{I}}$ and $y_{ * }^{\text{II}}$ are the site fractions of a certain component located on sublattice I and II, respectively, and the parameters ⁰ G _A:B:C represents the Gibbs free energy of the compound phase when the two sublattices are occupied by element A, B or C. $L_{{A_{1} ,A_{2} :B_{1} :C}}$and $L_{{A_{1} ,A_{2} :B_{2} :C}}$ are the interaction parameter between Cu and Ni in the first sublattice, when the second sublattice is occupied by element Cu or Ni, the third sublattice is occupied by element Si. $L_{{A_{1} :B_{1} ,B_{2} :C}}$ and $L_{{A_{2} :B_{1} ,B_{2} :C}}$ are the interaction parameter between Cu and Ni in the second sublattice, when the first sublattice is occupied by element Cu or Ni, the third sublattice is occupied by element Si. ⁰ G _A:B:C , $L_{{A_{1} ,A_{2} :B_{1} :C}} ,\,L_{{A_{1} ,A_{2} :B_{2} :C}} ,\,L_{{A_{1} :B_{1} ,B_{2} :C}}$ and $L_{{A_{2} :B_{1} ,B_{2} :C}}$ are evaluated in the present work.

All the thermodynamic models adopted in this work are listed in Table 1.

Table 1 Thermodynamic models

Results and Discussions

Based on the recent experimental data, the thermodynamic parameters of each phase in the Cu-Ni-Si system are evaluated using the CALPHAD method. Four calculated isothermal sections of this system at 700, 800, 900 and 1000 °C are presented in Fig. 2-5. At 900 °C, the Liquid + NiSi + α NiSi₂ region shows a little discrepancy with the experimental data. We attribute it to the fact that the composition of the liquid phase is not that precise with the poor measuring techniques. Apart from that, the consistency between the experimental data and the calculated results is a good indicator of the accuracy of the proposed assessment. It was found that the γ (Cu₅₆Si₁₁) and θ (Ni₂Si) phases can be stabilized by the increasing temperature, which is also confirmed by the present calculation.[4] Then a prediction of the isothermal sections in the Cu-Ni-Si system at 600 and 1200 °C using the present parameters are presented in Fig. 6 and 7, respectively. It can be seen from Fig. 6 that both the τ₁ and τ₂ phases are stable, there exists an area of phase separation FCC₁ + FCC₂ at 600 °C and the area becomes larger with the temperature decreasing, the δ (Ni₂Si) phase which has been identified responsible for the strengthening effect[22] has a solubility of Cu up to 11.2 at.%. At 1200 °C, the solubility of Cu is predicted to be about 5.1 at.% in the δ (Ni₂Si) phase. Meanwhile, the Ni₃Si_β₂ phase will be stable instead of the Ni₃Si_β₁ phase. Figure 8 shows the calculated liquidus projection for the entire ternary system and also invariant reactions are presented in Table 2. The reaction scheme is presented in Table 3. Among all the seventeen invariant reactions, there are five eutectic transitions: liquid ↔ γ′ (Ni₅Si₂) + δ (Ni₂Si) + β₁ (Ni₃Si) (1303.31 °C), liquid ↔ γ′ (Ni₅Si₂) + FCC + β₂ (Ni₃Si) (1143.95 °C), liquid ↔ γ (Ni₅₆Si₁₁) + η (Cu₁₉Si₆) + τ₁ (862.19 °C), liquid ↔ γ (Ni₅₆Si₁₁) + η (Cu₁₉Si₆) + FCC (856.51 °C), liquid ↔ α (NiSi₂) + η (Cu₁₉Si₆) + (Si) (771.20 °C). The calculated temperature of the peritectic reaction (liquid + γ (Cu₅₆Si₁₁) + θ (Ni₂Si) ↔ τ₁) is 878 °C, which agrees reasonablely well with the experimental data. We have also calculated the enthalpy of mixing in liquid Cu-Ni-Si alloys at 1575 K, at different composition ratios. As can be seen in Fig. 9, expect for the calculated Ni:Si = 4:1 curve shows more negative enthalpy values at high copper contents than the experimental data, the agreement with the experimental data is good on the whole.[23] The enthalpy of mixing in liquid Cu-Ni-Si alloys at 1576 K with Ni:Si = 1:1, at 1577 K with Ni:Si = 4:1, at 1579 K with Ni:Si = 1:4 are shown in Fig. 10. The Ni:Si = 4:1 curve shows a little more negative enthalpy values at high copper contents than the experimental data, the results are in good consistent with the experimental data.[23]

Fig. 2

Calculated isothermal section of the Cu-Ni-Si system at 700 °C

Fig. 3

Calculated isothermal section of the Cu-Ni-Si system at 800 °C

Fig. 4

Calculated isothermal section of the Cu-Ni-Si system at 900 °C

Fig. 5

Calculated isothermal section of the Cu-Ni-Si system at 1000 °C

Fig. 6

Calculated isothermal section of the Cu-Ni-Si system at 600 °C

Fig. 7

Calculated isothermal section of the Cu-Ni-Si system at 1200 °C

Fig. 8

Calculated liquidus projection of the Cu-Ni-Si system

Table 2 Invariant reactions

Table 3 Reaction scheme

Fig. 9

Calculated enthalpy of mixing in liquid Cu-Ni-Si alloys at 1575 K

Fig. 10

Calculated enthalpy of mixing in liquid Cu-Ni-Si alloys at 1576, 1577 and 1579 K

The thermodynamic description of the Cu-Ni-Si system is presented in Table 4. Figure 11-14 show four vertical sections with constant nickel contents of 1, 2, 3 wt.% and silicon content of 0.5 wt.%, respectively. In Fig. 13, the FCC + γ′ area shows a little discrepancy with the experimental data taken from Okamoto’s work.[10] As that experimental work was done long before, the only discrepancy is acceptable. On the whole, the calculated results agree reasonably well with the experimental data.[9–11]

Table 4 Thermodynamic parameters of the Cu-Ni-Si system optimized in the present work

Fig. 11

Calculated vertical section at 1 wt.% Ni of the Cu-Ni-Si system

Fig. 12

Calculated vertical section at 2 wt.% Ni of the Cu-Ni-Si system

Fig. 13

Calculated vertical section at 3 wt.% Ni of the Cu-Ni-Si system

Fig. 14

Calculated vertical section at 0.5 wt.% Si of the Cu-Ni-Si system

Conclusion

On the basis of the latest literature data, a set of self-consistent parameters is obtained in the frame work of CALPHAD method. This set of parameters takes into account the newly found ternary compound τ₂ and reproduces it very satisfactorily; comparisons between the isothermal sections, vertical sections, liquidus projection and invariant reactions show a good agreement with the present description; the liquidus projection and the invariant reaction scheme for the whole system can be used as predictive tool for future experimental studies and materials design.