1 Introduction

In crystals, the atomic vibrations are described by phonons, and the statistical mechanics can be formulated with phonons as the basis. In liquids and glasses, however, phonons are strongly scattered and have a very short lifetime and cannot form the basis for statistical mechanics. An approach more frequently taken today is computer simulation. However, the time scale of computer simulation is vastly different from the experimental time scale, which leaves serious doubts about how realistic the results of simulation are. Over many years, various theoretical approaches to calculate thermodynamic quantities, such as the “free-volume” theory,[1–3] potential energy-landscape theory,[4,5] and the mode-coupling theory,[6,7] have been proposed. However, they are mostly phenomenological and lack atomistic basis. We propose a new approach, the topological fluctuation theory (TFT), based upon the concept of atomic level stresses.[8] In this article, we discuss problems associated with computer simulation and with the free-volume theory and review recent progress with the TFT, including the calculation of the glass transition temperature and the kinetics of structural relaxation.

Because the progress in theory has been so painfully slow, many researchers are now attracted to circumventing the difficulty of the many-body problem inherent in the theory of liquids and glasses through numerical solution by computer simulation. Today calculations of electronic states with the density functional theory (DFT) are so advanced that structures and many properties of solids can be predicted and understood. Software that couples the DFT and molecular dynamics (MD) simulation is now readily available, and the first-principles simulations are beginning to be applied to elucidate the structure and dynamics of metallic glasses. However, apparent successes of such approaches hide inherent danger in the simulation of liquids and glasses that the simulations are done at unrealistic time scales, and consequently some of the results could be totally incorrect. Thermodynamic properties, such as the glass transition temperature, are particularly difficult to simulate realistically, since the simulations tend to leave the systems in a seriously under-relaxed state with much less short-range order than in reality.

The time-step for the MD simulation is usually chosen to be of the order of a femto-second (10−15 seconds), since the time scale for phonons is about 10−13 seconds and the MD step has to be sufficiently small to depict phonon dynamics. For the first-principles MD simulation, the MD steps that can be readily calculated are of the order of 105 steps. Then, the time length of simulation is only 10−10 seconds, or 100 pico-seconds. The time scale can be related to viscosity using the Maxwell relaxation time, τ = η/G ∞ , where η is viscosity and G ∞ is instantaneous shear modulus. Since G ∞  ~ 102 GPa, η is only 10 poise for τ = 10−10 s. Even with long MD simulation with model potentials such as pairwise potentials, it is difficult to reach the equivalent viscosity greater than 104 poise, whereas the glass transition is defined by viscosity reaching 1013 poise. This huge gap in time scale of nine orders of magnitude is difficult to fill, even with the advance in computing techniques. The temperature at which viscosity is 104 poise is much above the glass transition temperature, T g , by 40 pct or more.[9] Thus, the models obtained by simulation have the fictive temperature significantly above T g , and consequently are seriously less relaxed than the real glass. We can still learn from such simulations about the properties which do not critically depend on the fictive temperature, such as the rough outline of the structure. However, details of the structure as well as thermodynamic quantities will be seriously compromised by the lack of relaxation in the model structure.

2 Free-Volume Theory

In order to bridge the gap in time scale and predict the dynamic properties of liquids and glasses, we need to understand the statistical mechanics of liquids and glasses. However, the lack of periodicity does not allow the use of a statistical mechanical theory developed for crystals. To gain physical insight into this very complex problem, various theories, very different from the theories of crystal in outlook and flavor, have been advanced. Most of them are phenomenological in that they require input from the experiment. The one closest to the first-principles theory is the mode-coupling theory (MCT).[4,5] Indeed, the theoreticians who promote MCT claim that it is a first-principles theory without the need of experimental input, but the fact of the matter is that the structure factor, S(Q), needs to be supplied by experiment or simulation. MCT catches certain aspects of the glass transition phenomenon quite well, in terms of the coupling between fast and slow modes of liquid dynamics. Another theory that became popular in recent times is the potential energy landscape theory.[6,7] This theory gives a big and intuitive picture of thermal process in complex systems and helps us to see general, common features of various liquids and glasses. This generality is achieved by glossing over the microscopic atomistic details, but the cost to pay is that it cannot provide help in addressing the atomistic mechanism.

The theory most frequently used by experimentalists in the field of metallic glasses is the free-volume theory. The root of this theory goes back to the observation by Batchinski[10] and Doolittle[11] that the excess volume expansion above T g is related to viscosity. A quantitative theory was formulated by Cohen and Turnbull,[1–3] and applied to account for mechanical properties by Spaepen.[12] This theory is based upon the hard-sphere model and has been successful in explaining various properties of colloids, molecular glasses, and other hard-sphere-like systems. In applying this theory to metals, however, some considerations are needed, because metals are not hard-sphere-like. Cohen and Turnbull noted this point in their original article[1] and have shown that the critical size of the free volume, v*, is only about 10 pct of the atomic volume for metals, whereas it is about 80 pct for hard-sphere systems. They argued that in metals the atomic core plays the role of the hard sphere.[1] It is known that, in a model of metallic glass with a pairwise potential, a large atomic size void is unstable and breaks into smaller voids.[13] For this reason, Argon proposed the concept of distributed free volume.[14]

However, if free volume is distributed into small pieces, what keeps the total? In other words, is there any reason to start the argument with the atomic size void? It may not be necessary to consider the atomic size void at all, but it may suffice just to assume that the free volume in metal is small and amounts only to 10 pct of the atomic volume. Indeed the pressure dependence[15,16] and the isotope effect[17] of diffusivity are much smaller than expected for an atomic size free volume. More recently, it was found by computer simulation that atomic diffusion takes place through collective motions (chain reaction) of small local displacements rather than a big step involving free volume.[18,19] Thus, it is clear that the free-volume theory needs to be modified seriously when applied to metallic glasses.

3 Topological Fluctuation Theory

As mentioned previously, the free-volume theory was developed with a hard-sphere system in mind. Therefore, it has been successful for systems such as colloid and molecular glasses. However, metallic glasses are not hard-sphere-like and have a more harmonic interatomic potential. Even though atomic cores are hard-sphere-like, as noted by Cohen and Turnbull, the energy levels of cores are larger, from few electron Volts (eVs) to tens of thousands of eVs. Unless extremely large forces are applied, atomic cores hardly affect the properties of a metal. The properties of a metal are to a large extent determined by the behavior of nearly free electrons, fluctuations in electron density in particular, such as plasmons and Friedel oscillations.

Therefore, to account for the properties of metals, it is more realistic to start with a model with a harmonic interatomic interaction than the hard-sphere model. Indeed some thermal properties of a liquid, such as compressibility, can be expressed in terms of the local atomic density fluctuations, n(r, t).[20] The MCT is based upon the nonlinear coupling of fluctuations in n(r, t).[4,5] However, the description by n(r, t) reflects the atomic level local structure only through local volume. It is adequate at the long-wave limit and at high temperatures, but in supercooled liquids and glasses, other details of local topology of atomic connectivity, such as the shape of the Voronoi polyhedra, start to play a role. For instance, one of the most puzzling behaviors of a supercooled liquid is that its viscosity changes by as much as 15 orders of magnitude over a temperature range of a few hundreds of degrees above T g , even though the average structure does not seem to change so drastically. Now viscosity is a response of the system to shear deformation, whereas shear stress does not directly couple to density. In MCT, indirect coupling is devised to describe the coupling between n(r, t) and viscosity.

An alternative approach is to describe the local structure of a liquid by a tensor variable, rather than a scalar variable such as n(r, t). Viscosity, η, is given by the Green–Kubo fluctuation-dissipation theorem:

$$ \eta = {\frac{kT}{V}}\int {\left\langle {\sigma^{xy} \left( 0 \right)\sigma^{xy} \left( t \right)} \right\rangle dt} $$
(1)

where σ xy is the shear stress.[21] Microscopically, the shear stress would fluctuate in space and time. In order to describe such fluctuation, we may introduce a local shear stress field, σ xy(r, t), and rewrite Eq. [1] as

$$ \eta = {\frac{kT}{V}}\iiint {\left\langle {\sigma^{xy} \left( {{\user2{r}},0} \right)\sigma^{xy} \left( {{\user2{r^{\prime}}},t} \right)} \right\rangle d{\user2{r}}d{\user2{r^{\prime}}}dt} $$
(2)

The local shear stress field is an off-diagonal element of the local stress field tensor, σ(r, t). The trace of this tensor corresponds to the local pressure, p(r, t). Thus, the local volume, v(r, t), can be given by v(r, t) = 〈v〉[1 + ε v (r, t)], where ε v  = p(r, t)/B(r, t) is the local volume strain, B(r, t) is the local bulk modulus, and the local density, n(r, t), is n(r, t) = 〈n〉[1 − ε v (r, t)] to the first order in strain. If we do not allow local rotation defects, such as vortex, the tensor is symmetric and has six independent elements. In the spherical representation, they become one l = 0 element (pressure) and five l = 2 elements (shear stresses). By reducing the lengthscale of σ(r, t) to an atomic level, we can define the atomic level stress,[8]

$$ \sigma^{\alpha \beta } \left( i \right) = {\frac{1}{{V_{i} }}}\sum\limits_{j} {f_{ij}^{\alpha } r_{ij}^{\beta } } $$
(3)

where V i is the local atomic volume, f ij is the two-body force, and r ij is the separation between atom i and atom j, respectively. The atomic level stresses reflect the topology and geometry of the nearest neighbor atoms. For instance, the pressure is linearly related to the coordination number.[22] For this reason, we call the present theory the TFT. Now the kernel of Eq. [2] in spherical average,

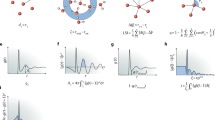
$$ \Upsigma \left( {r,t} \right) = \iint {\left\langle {\sigma^{xy} \left( {{\user2{r^{\prime}}},0} \right)\sigma^{xy} \left( {{\user2{r^{\prime\prime}}},t} \right)} \right\rangle \delta \left( {r - \left| {{\user2{r^{\prime} - r^{\prime\prime}}}} \right|} \right)d{\user2{r^{\prime}}}d{\user2{r^{\prime\prime}}}} $$
(4)

can be calculated by MD simulation using the atomic level stresses defined by Eq. [3]. Figure 1 shows an example of this correlation function in two-dimensional representation. The details of the MD simulation are given in Reference 23. Through this expression, we see that the drastic increase in η with cooling in supercooled liquid occurs by the combination in increases in temporal and spatial correlations.

Fig. 1
figure 1

Shear stress correlation function in time (t) and space (r), Eq. [4] in the text, calculated for a model of liquid iron of 5488 atoms interacting with the modified Johnson potential[23] at T = 2000 K. A periodic boundary condition was used. Propagation of sound waves, both longitudinal and transverse, are visible in the graph. The stripelike oscillations along r reflect the oscillations in the PDF

Full size image

The most important reason why this approach is attractive is that we found that at high temperatures the self-energy of the atomic level stress fluctuation obeys the equipartition theorem of statistical mechanics,

$$ E_{el} = {\left\langle {V} \right\rangle} {\frac{{\left\langle {p^{2} } \right\rangle }}{2\left\langle B \right\rangle }} = {\frac{{{\text{k}}T}}{4}} $$
(5)

and the same for the five components of the shear stress.[23,24] Thus, the total potential energy, U = (3/2)kT, is divided into six, for each stress component. This result suggests that the TFT can form the basis for the statistical mechanical theory of liquid.

Another important component of the theory is the concept of the minimum local strain, defined by ε αβ = σ γδ /C αβγδ, where C αβγδ is the corresponding elastic modulus. In crystals, we tend to assume that the thermal average of the stress field, 〈σ(r, t)〉, is zero. At an atomic level, however, this is true only for an elemental solid with a Bravais lattice structure. In an alloy, if a large atom A and a small atom B are occupying crystallographically equivalent sites, A would be under compression while B would be under tension. If we start with a pure crystal of B and keep replacing B atoms with A atoms, the tension in the B lattice will increase until the lattice becomes unstable. The critical volume strain for such topological instability can be calculated as 5.5 pct,[25] and this was the basis for estimating the composition limit for the stability of solid solution, and thus the formability of binary metallic glasses.[26] The same concept can be applied to the structure of the glass itself. In glasses, every site is different from others. Thus, every site is under some stress. A part of the stress is thermal, and at high temperatures, it is given by Eq. [5]. For local volume fluctuations, the critical volume strain is 11 pct.[27] The site with the strain greater than 11 pct is topologically unstable and will eventually collapse. Thus, we can define these sites as “liquidlike” sites. Now there are two kinds of liquidlike sites, those with the volume strain smaller than −0.11 and those with the volume strain greater than +0.11. The site, with volume strain greater than 0.11, resembles free-volume. Indeed this value of the critical strain is close to the original estimate by Cohen and Turnbull for metals discussed previously.[1] This provides a new interpretation of the free-volume in metallic glasses. When the local density has negative deviation from the average, these sites can be called “n-type defects.”[28]

However, the sites with the volume strain smaller than −0.11 are also unstable and liquid-like, and since they have positive deviation in density from the average, we call them “p-type defects.” We could call them “anti-free-volume.”[28] Thus, we have two kinds of liquidlike sites, as shown in Figure 2. The density of these sites depends on temperature through the temperature dependence of the stresses (Eq. [5]), except that, at low temperatures, the stresses are renormalized by the long-range stress fields they generate. The effect of the long-range stress field can be incorporated[22] using the continuum approximation of Eshelby:[29]

$$ E_{el,LT} = {\left\langle {V} \right\rangle} {\frac{{K_{\alpha } \left\langle {p^{2} } \right\rangle }}{2\left\langle B \right\rangle }} = {\frac{{{\text{k}}T}}{4}},\quad K_{\alpha } = {\frac{{3\left( {1 - \nu } \right)}}{{2\left( {1 - 2\nu } \right)}}} $$
(6)

The glass transition occurs when the density of these liquidlike sites exceeds the percolation concentration.[30] Thus, we obtain[31]

$$ {\text{k}}T_{g} = {\frac{2\left\langle B \right\rangle \left\langle V \right\rangle }{{K_{\alpha } }}}\left( {\varepsilon_{v}^{T} } \right)^{2} $$
(7)

This simple expression agrees excellently with the experimental data,[31] with ε T v  = 0.095, which corresponds to the total density of liquidlike sites being 0.22, approximately the percolation concentration for dense-random-packed structure.

Fig. 2
figure 2

Schematic distribution in the local atomic volume. The sites with the volume strain greater than 0.11 define the n-type defects (free-volume), while those less than −0.11 define the p-type defects (anti-free-volume). Together they represent the liquidlike sites

Full size image

4 Structural Relaxation In Bulk Metallic Glasses

As discussed previously in relation to the MD simulation, when a liquid is cooled rapidly, the structure stays at a state that is in equilibrium at a temperature (fictive temperature) much above T g . With subsequent annealing at a temperature below T g , the structure relaxes into a more stable state. This phenomenon of structural relaxation is commonly seen for glasses. Since volume decreases as a result of structural relaxation, it is often interpreted in terms of decrease in free-volume.[32] However, volume decrease is only a part of the entire array of properties that change as a result of structural relaxation.[33] Whereas the change in volume is quite small, usually of the order of a few tenths of a percent, the heat released by relaxation is a substantial portion of the heat of crystallization.

A recent study of volume and enthalpy relaxation in Pt60Ni15P25[34] illustrates these points clearly. If elimination of free-volume is the mechanism of structural relaxation, the changes in volume, ΔV, and in enthalpy, ΔH, should be proportional to each other. Figure 3 shows that they are not. Furthermore the kinetics of volume relaxation shown in Figure 4 suggests that the kinetics cannot be explained by a single relaxation process. However, if we assume two competing processes, one reducing the volume and the other increasing the volume, it can be satisfactorily explained, as shown by solid curves in Figure 4. This idea fits very nicely to the n-p defects scenario discussed previously. The result of analysis shows

$$ \begin{aligned} \Updelta V_{n} /V & = - 1. 6 9\times 10^{ - 2} \\ \Updelta V_{p} /V & = + 1. 2 2\times 10^{ - 2} \\ \Updelta V_{t} /V & = - 0. 4 8\times 10^{ - 2} \\ \end{aligned} $$

where ΔV n is the change in volume due to annihilation of n-type defects, which is negative; ΔV p is that due to p-type defects, which is positive; and ΔV t  = ΔV n  + ΔV p is the total change in volume. Since ΔV n and ΔV p almost cancel out each other, the total change in volume is much smaller than either volume change. Thus, the total volume of the defects annihilated, \( \Updelta V_{d} = \left| {\Updelta V_{n} } \right| + \left| {\Updelta V_{p} } \right|, \) is much larger than the total change, with ΔV d /V = 2.91 × 10−2. The actual defect volume, ΔV d , is larger by 6 times than the apparent volume change observed.

Fig. 3
figure 3

Direct plot of specific volume change, Δv(t), against the enthalpy change, ΔH g(t), due to structural relaxation in Pt60Ni15P20 metallic glass. The specific volume was measured at room temperature after annealing at 461 K (15 K above T g) for time t, and the enthalpy was measured by the differential scanning calorimeter[34]

Full size image
Fig. 4
figure 4

Changes in specific volume of Pt60Ni15P25 BMG, d(t), measured at room temperature after the sample was annealed at 461 K (15 K above T g) for time t, in the as-quenched and preannealed samples. Solid lines illustrate the calculations with two relaxation components. Reference 34 provides details

Full size image

Now the argument in Reference 34 can be reinforced further by quantitatively examining the amount of heat released by relaxation. The initial slope in Figure 3 suggests ΔH/ΔV = 1.7 × 105 J/cm3 = 1.1 eV/Å3. Since the average atomic volume is V a  = 14.4 Å3 for this glass, the heat to create a free-volume of the atomic size V a ΔH/ΔV is 15.8 eV, which is a ridiculously large number, since the energy to create a vacancy in a metal is about 2 eV. Similar values of ΔH/ΔV were obtained for other metallic glasses. For instance, ΔH/ΔV = 0.42 eV/Å3 for Zr55Cu30Ni5Al10[35] and ΔH/ΔV = 0.46 eV/Å3 for Zr44Ti11Cu10Ni10Be25[36] have been reported. These values correspond to values of V a ΔH/ΔV, which are still 3 to 4 times larger than the enthalpy of vacancy creation.

These results clearly suggest that it is a mistake to relate the enthalpy released by relaxation directly to the total change in volume, ΔV t . As discussed previously, the total change reflects the difference between the volume decrease due to annihilation of the n-type defects and the volume increase due to annihilation of the p-type defects, which almost cancel each other. A more reasonable approach is to consider the total change in enthalpy and then to compare it to the total defect volume, ΔV d . For Pt60Ni15P25, ΔH = 3 J/g, and we obtain ΔH/ΔV d  = 1.4 × 104 J/cm3 = 0.09 eV/Å3. This value gives the energy to create a defect of an atomic size, V a ΔH/ΔV d  = 1.3 eV/Å3, which is indeed comparable to the known enthalpy for vacancy creation in crystals. At the same time, the energy to create a free-volume of V f  = 0.11V a can be evaluated by

$$ E_{FV} = {\frac{{BV_{a} K_{\alpha } }}{2}}\left( {\varepsilon_{FV} } \right)^{2} $$
(8)

With BV a  = 9 eV, ν = 0.25,[30] and ε FV  = V f /V a  = 0.11, we obtain E FV  = 0.12 eV. From the value of ΔH/ΔV d estimated previously, we obtain the defect energy, V f ΔH/ΔV d  = 0.14 eV/Å3, which is very close to E FV . To summarize, the interpretation of the enthalpy released by relaxation by the simple free-volume theory leads to the defect energy as large as 10 eV, a huge value compared to the thermal energy of kT. However, the two-component model and the free-volume threshold of V f /V a  = 0.11 result in the defect energy of ~0.1 eV, which is totally comparable to thermal energy. Thus, the simple free-volume theory fails to explain the enthalpy and volume relaxation, whereas the two-component theory gives a more satisfying elucidation.

These results strongly support the idea that structural relaxation occurs by reducing the density fluctuation by annealing out positive and negative density defects, often through their recombination. Both of them contribute positively to ΔH, but their contributions to the total volume almost cancel each other. Thus, the apparent ΔH/ΔV ratio is unreasonably large, but the more physically meaningful ratio of heat to volume, ΔH/ΔV d , is reasonable. This analysis is also consistent with our contention that the free-volume in metals is much smaller than the atomic volume, and the free-volume theory must be modified when applied to metallic glasses.

This idea of reduction in density fluctuation during structural relaxation was used in successfully explaining the changes in the pair-density function (PDF) by relaxation[37] determined by X-ray diffraction.[38] It is also worth noting that experimental results suggest that the kinetics of volume change during structural relaxation is second order.[32] Therefore, the free-volume theory of relaxation requires the presence of a sink for the free-volume of which density is proportional to free-volume.[32] Thus, it may be fair to say that the current free-volume theory already implicitly assumes the presence of anti-free-volume, such as p-type defects.

5 Conclusions

It is much more difficult to carry out first-principles calculations for metallic glasses compared to crystalline solids, not only because of the large model size required but because of the serious limitation in simulation time scale the computation poses. It is practically impossible to do realistic simulations of thermodynamic behavior of deeply supercooled liquids and glasses, since the shortness of time scale computer simulations can cover leaves the system seriously under-relaxed. For this reason, phenomenological theories are often invoked to explain the experimental observations. The most popular theory is the free-volume theory, in spite of the fact that the creators of the theory warned against its use for metallic liquids.[1] The free-volume theory, indeed, has met with difficulties when it was applied to metallic glasses. We proposed an alternative theory, the TFT, and discussed successes of this theory in explaining the glass transition temperature and structural relaxation. In particular, a recent study of volume and enthalpy relaxation in bilk metallic glasses shows that the results can be satisfactorily explained only when we assume both negative (free-volume) and positive (anti-free-volume) local density fluctuations that become relaxed by structural relaxation. This theory could form the basis for a general theory to explain various physical properties of metallic glasses and liquids.